Microstructural Changes Caused by the Creep Test in ZK60 Alloy Reinforced by SiCp at Intermediate Temperature after KOBO Extrusion and Aging
Abstract
:1. Introduction
2. Materials and Methods
2.1. Composite Preparation
2.2. Creep Test
2.3. Characterizations
3. Results and Discussion
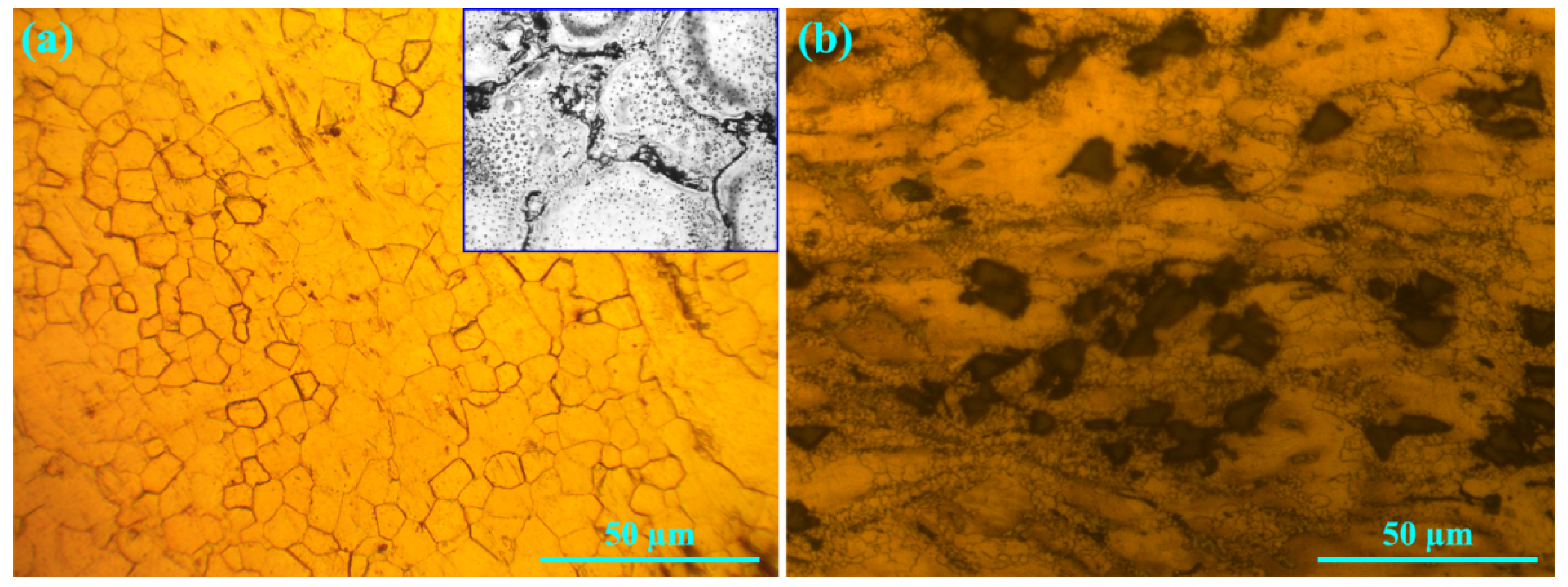
3.1. Microstructure
3.2. Load Transfer Factor
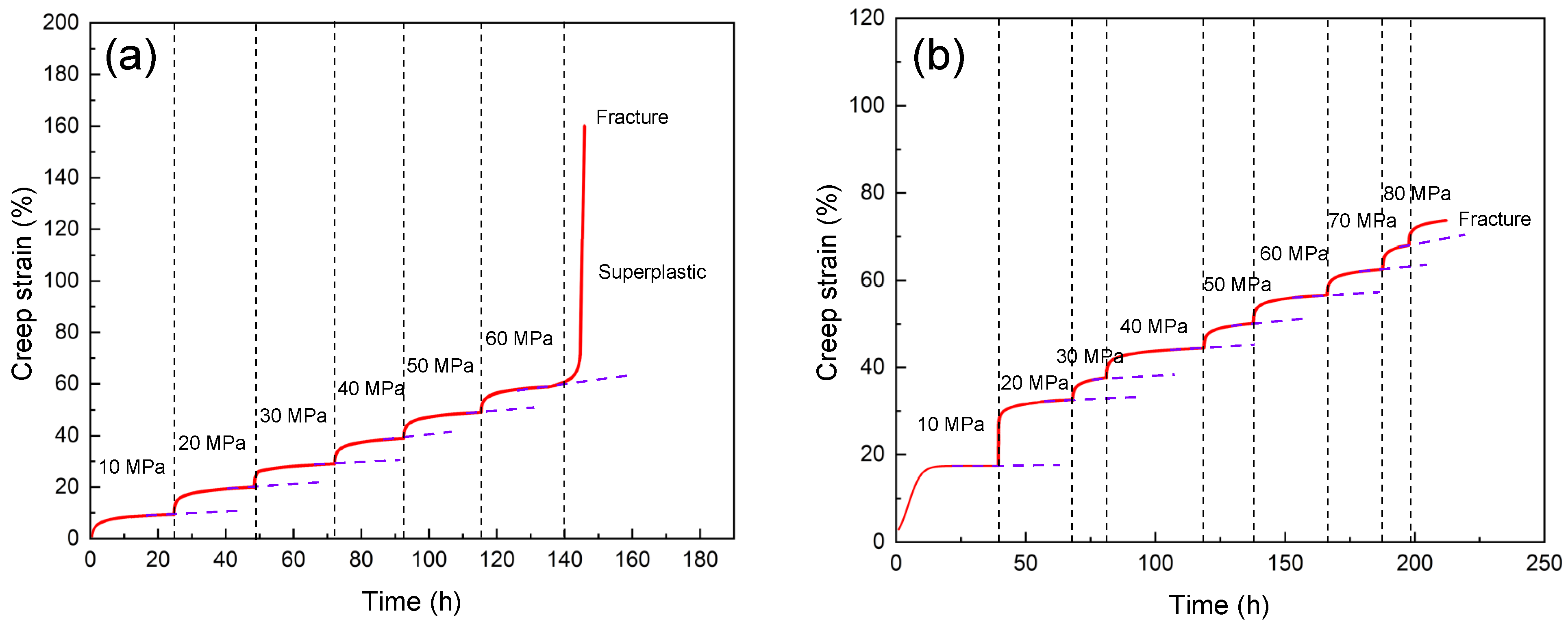
3.3. Creep Curve
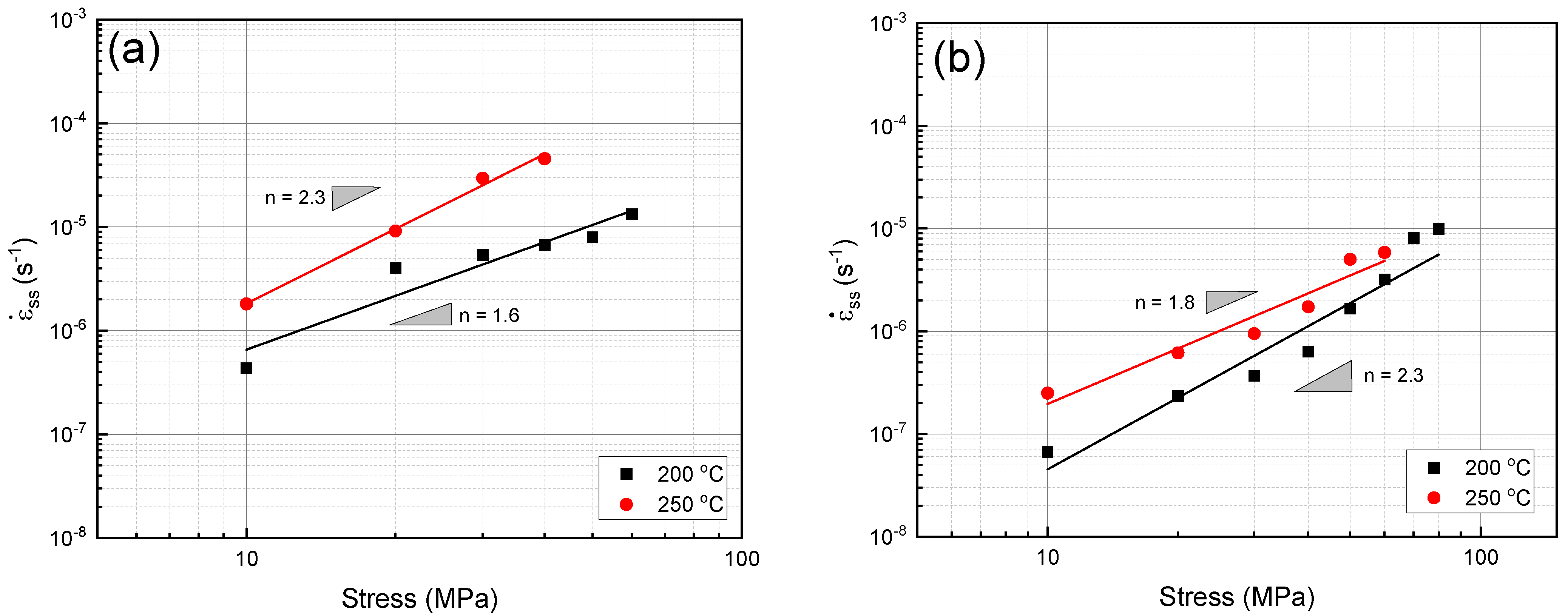
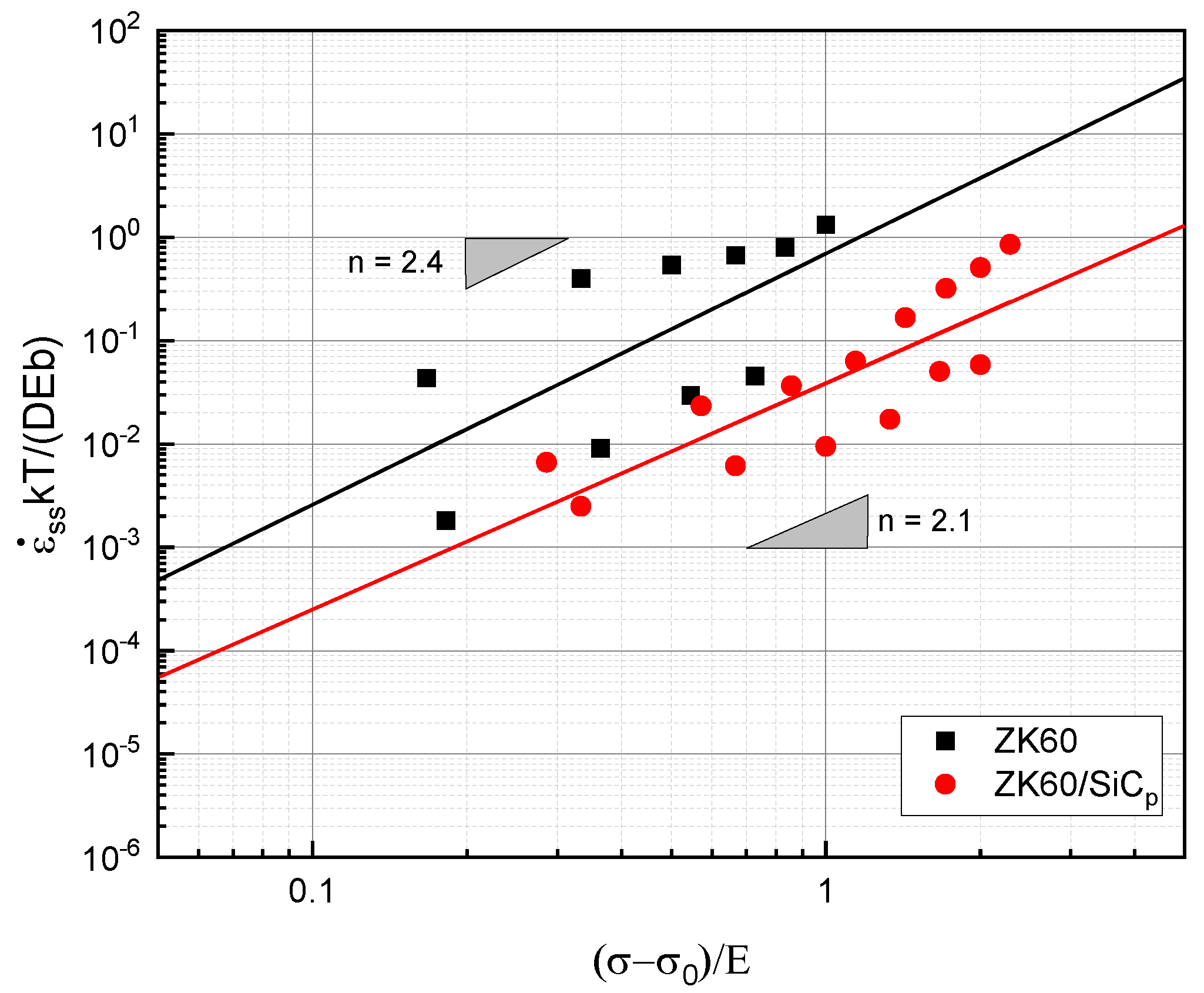
3.4. Stress Exponent
3.5. Activation Energy
3.6. Phenomenological Creep Equation
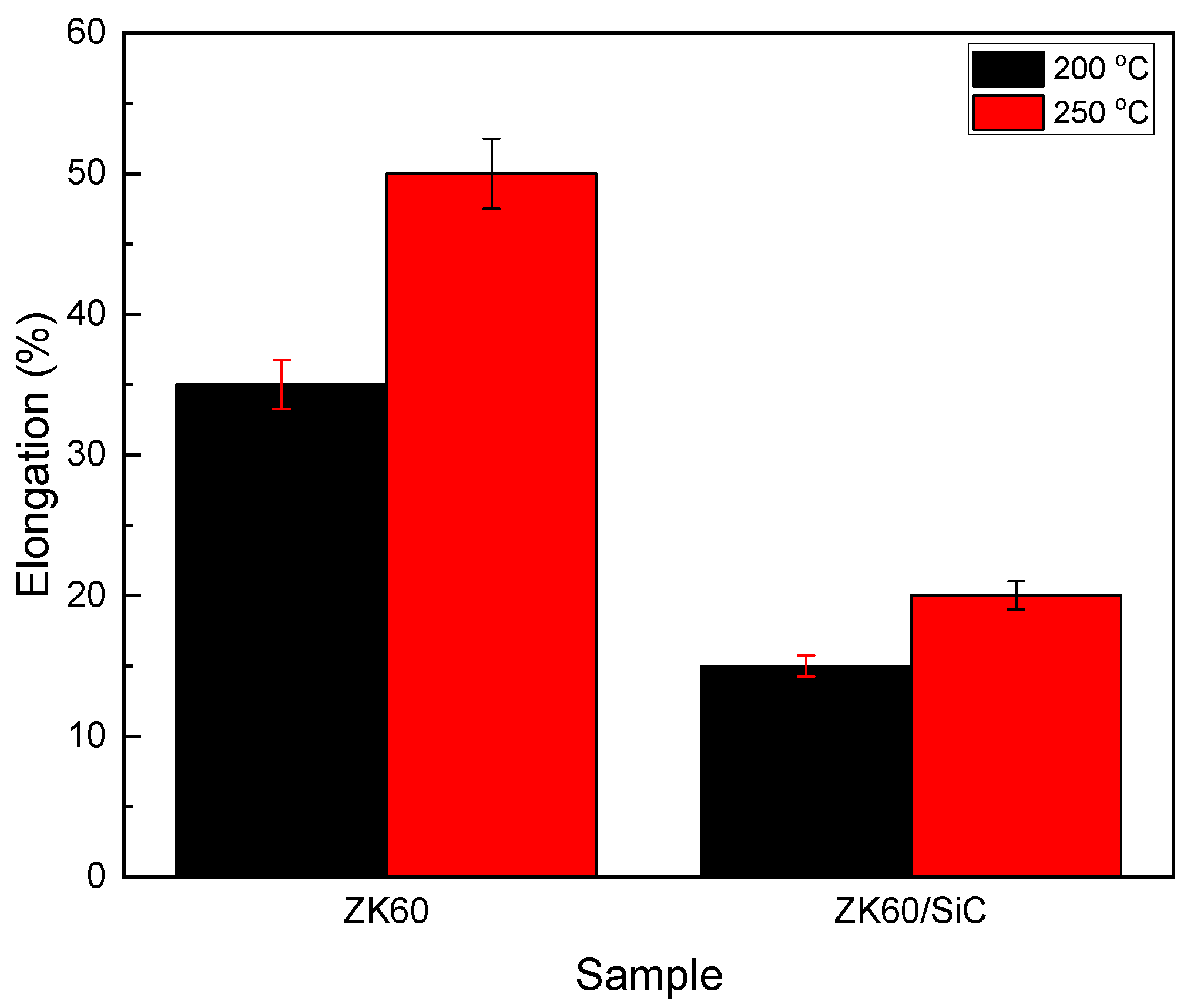
3.7. Elongation
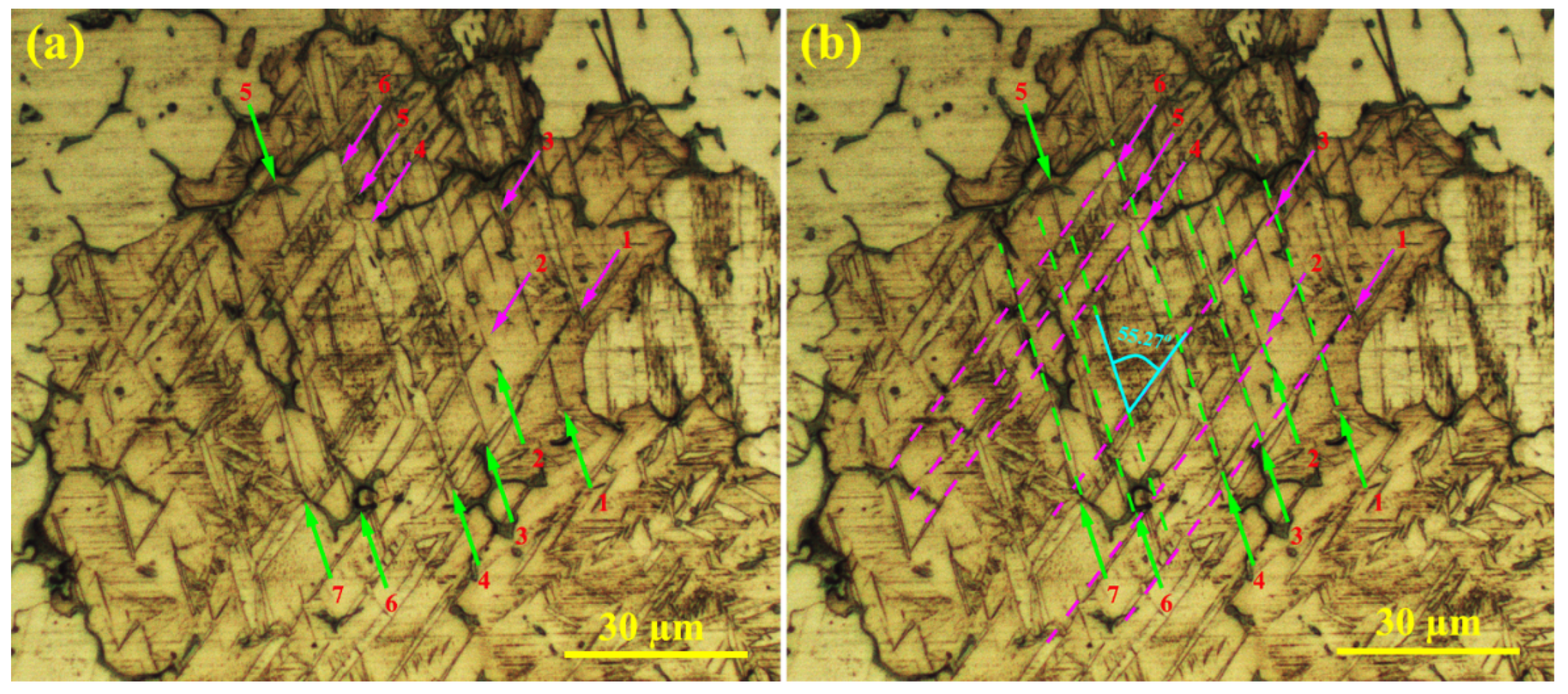
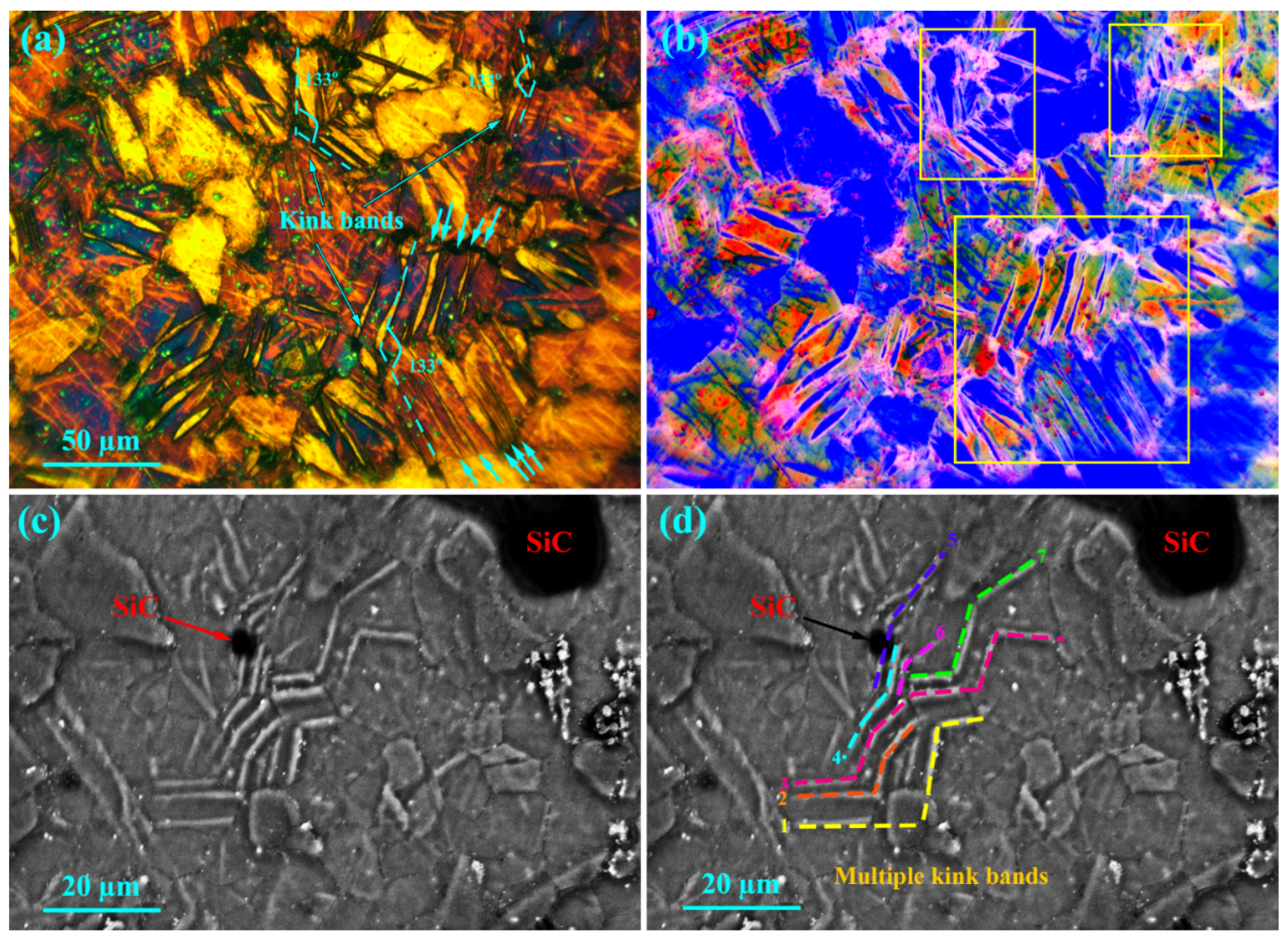
3.8. Microstructure
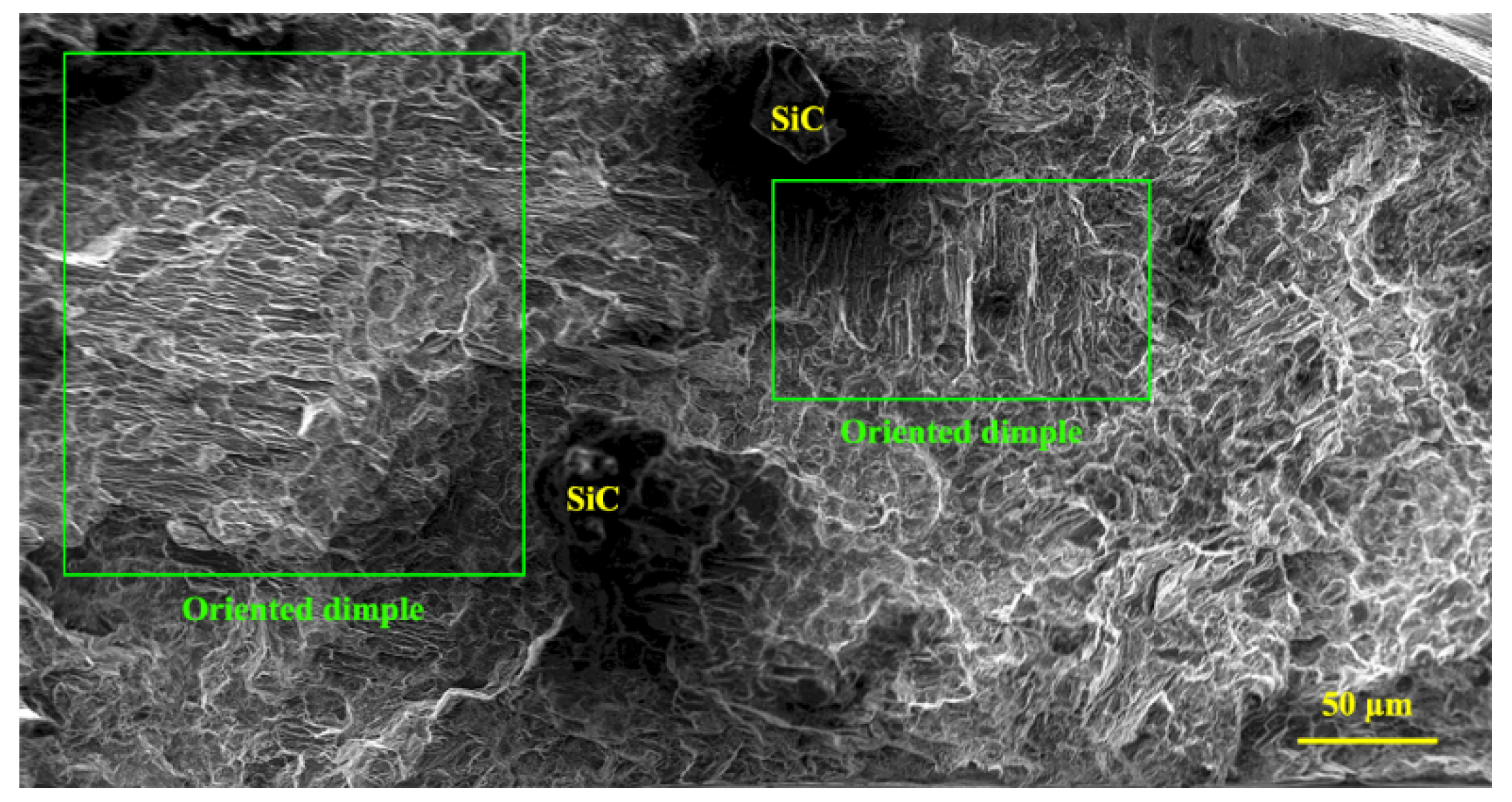
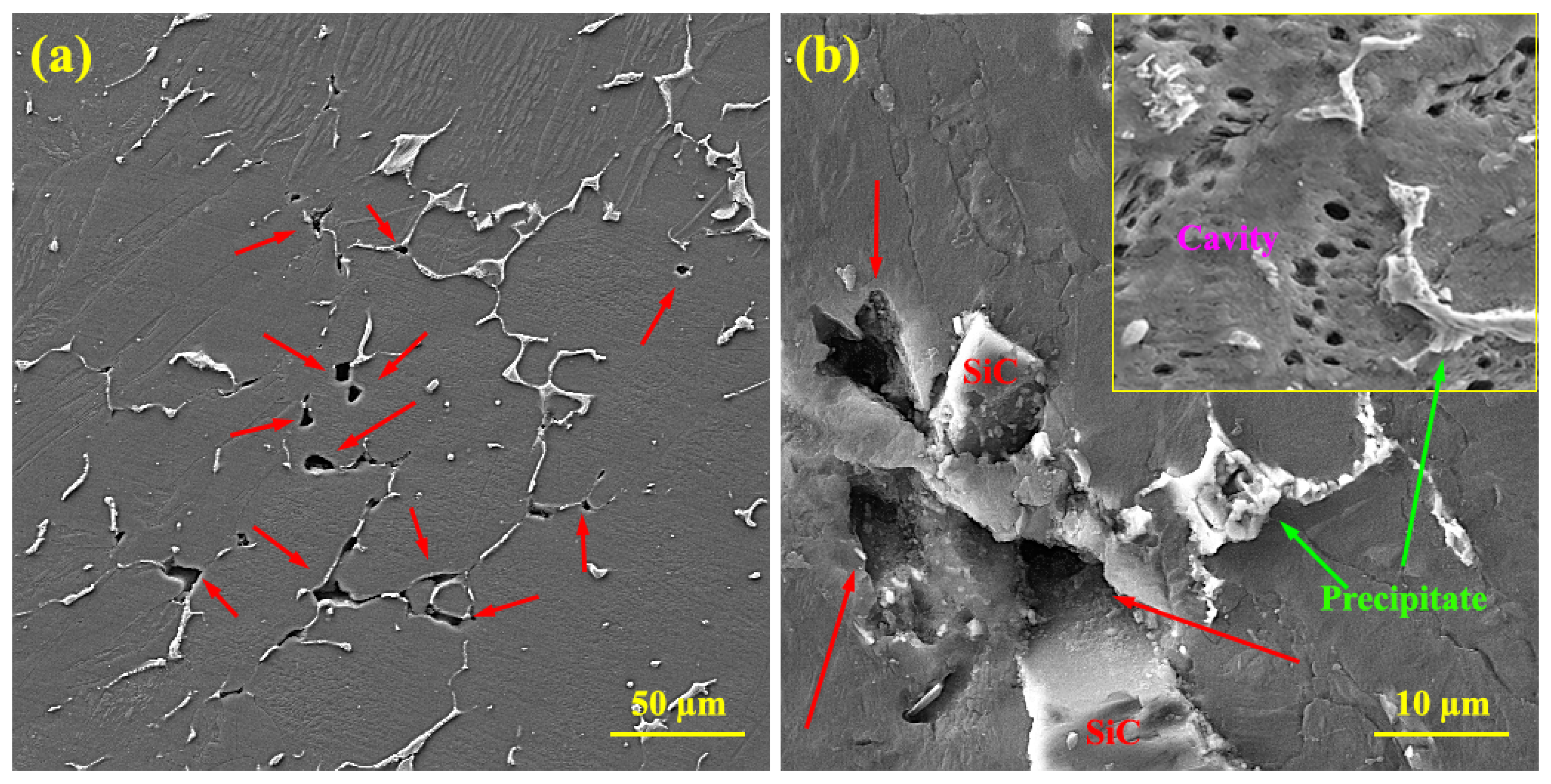
3.9. Failure Mechanism
4. Conclusions
- The load transfer factor was approximately 0.97 at 200 °C and 0.93 at 250 °C under low stresses, and this decreased linearly as the stress increased. This decrease was caused by a decrease in the load ability of the reinforcement and an increase in the load imposed on the matrix with the increase in the applied load.
- The true stress exponent for both the unreinforced alloy and the composite at 200 °C and 250 °C was in the range of 2.1–2.4, and the activation energy was in the range of 80.91–88.09 kJ/mol for the unreinforced alloy and 47.15–81.60 kJ/mol for the composite, indicating the GBS mechanism.
- At 200 °C, the strengthening mechanisms under low stresses were the twinning, double twinning, and shear bands, and as the stress increased, mechanisms such as slip bands and kink bands became active. At 250 °C, it was found that the slip band created during the creep deformation effectively delayed grain boundary sliding.
- A study of the fracture surfaces and microstructures of areas near the fracture surfaces at different temperatures showed that cavitation was the main failure mechanism. The cause of cavitation in the unreinforced alloy differed from that of the composite, and this can be attributed to the presence of particles in the composite. Major cavities were detected at the precipitate intersection and the matrix/particle interface due to the localized stress concentration created in these areas.
- The elongation of the samples after a 100 h creep test showed that at 200 °C the composite elongated more than 15% and the unreinforced alloy elongated more than 35%, and at 250 °C the composite elongated more than 20% and the unreinforced alloy elongated more than 50%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Guan, D.; Liu, X.; Gao, J.; Ma, L.; Wynne, B.P.; Rainforth, W.M. Exploring the mechanism of “Rare Earth” texture evolution in a lean Mg–Zn–Ca alloy. Sci. Rep. 2019, 9, 7152. [Google Scholar] [CrossRef]
- Wang, Y.-Y.; Jia, C.; Tayebi, M.; Hamawandi, B. Microstructural Evolution during Accelerated Tensile Creep Test of ZK60/SiCp Composite after KoBo Extrusion. Materials 2022, 15, 6428. [Google Scholar] [CrossRef] [PubMed]
- Mukai, T.; Nieh, T.G.; Iwasaki, H.; Higashi, K. Superplasticity in doubly extruded magnesium composite ZK60/SiC/17p. Mater. Sci. Technol. 1998, 14, 32–35. [Google Scholar] [CrossRef]
- Zhao, D.; Jiang, C.; Zhao, K. Ultrasonic welding of AZ31B magnesium alloy and pure copper: Microstructure, mechanical properties and finite element analysis. J. Mater. Res. Technol. 2023, 23, 1273–1284. [Google Scholar] [CrossRef]
- Liu, S.; Wang, Y.; Yarigarravesh, M.; Tayyebi, M.; Tayebi, M. Evaluation of whisker alignment and anisotropic mechanical properties of ZK60 alloy reinforced with SiCw during KOBO extrusion method. J. Manuf. Process. 2022, 84, 344–356. [Google Scholar] [CrossRef]
- Wang, Y.; Tayyebi, M.; Tayebi, M.; Yarigarravesh, M.; Liu, S.; Zhang, H. Effect of whisker alignment on microstructure, mechanical and thermal properties of Mg-SiCw/Cu composite fabricated by a combination of casting and severe plastic deformation (SPD). J. Magnes. Alloy. 2023, 11, 966–980. [Google Scholar] [CrossRef]
- Xu, Y.; Liang, Y.; Peng, G. Effect of a compound modification process on the microstructure and mechanical properties of ZK60 magnesium alloys. Mater. Sci. Eng. A 2020, 778, 139117. [Google Scholar] [CrossRef]
- Tekumalla, S.; Gupta, N.; Gupta, M. Influence of turning speed on the microstructure and properties of magnesium ZK60 alloy pre-processed via turning-induced-deformation. J. Alloys Compd. 2020, 831, 154840. [Google Scholar] [CrossRef]
- Tayebi, M.; Najafi, H.; Nategh, S.; Khodabandeh, A. Creep Behavior of ZK60 Alloy and ZK60/SiCw Composite After Extrusion and Precipitation Hardening. Met. Mater. Int. 2021, 27, 3905–3917. [Google Scholar] [CrossRef]
- Koike, J.; Ohyama, R.; Kobayashi, T.; Suzuki, M.; Maruyama, K. Grain-Boundary Sliding in AZ31 Magnesium Alloys at Room Temperature to 523 K. Mater. Trans. 2003, 44, 445–451. [Google Scholar] [CrossRef]
- Bussiba, A.; Ben Artzy, A.; Shtechman, A.; Ifergan, S.; Kupiec, M. Grain refinement of AZ31 and ZK60 Mg alloys—towards superplasticity studies. Mater. Sci. Eng. A 2001, 302, 56–62. [Google Scholar] [CrossRef]
- Chino, Y.; Iwasaki, H.; Mabuchi, M. Cavity growth rate in superplastic 5083 Al and AZ31 Mg alloys. J. Mater. Res. 2004, 19, 3382–3388. [Google Scholar] [CrossRef]
- Srinivasan, A.; Swaminathan, J.; Pillai, U.T.S.; Guguloth, K.; Pai, B.C. Effect of combined addition of Si and Sb on the microstructure and creep properties of AZ91 magnesium alloy. Mater. Sci. Eng. A 2008, 485, 86–91. [Google Scholar] [CrossRef]
- Watanabe, H.; Moriwaki, K.; Mukai, T.; Ohsuna, T.; Hiraga, K.; Higashi, K. Materials Processing for Structural Stability in a ZK60 Magnesium Alloy. Mater. Trans. 2003, 44, 775–781. [Google Scholar] [CrossRef]
- Liu, X.; Wang, J. Low-energy, Mobile Grain Boundaries in Magnesium. Sci. Rep. 2016, 6, 21393. [Google Scholar] [CrossRef] [PubMed]
- Torbati-Sarraf, H.; Torbati-Sarraf, S.A.; Poursaee, A.; Langdon, T.G. Electrochemical behavior of a magnesium ZK60 alloy processed by high-pressure torsion. Corros. Sci. 2019, 154, 90–100. [Google Scholar] [CrossRef]
- Tayebi, M.; Nategh, S.; Najafi, H.; Khodabandeh, A. Tensile properties and microstructure of ZK60/SiCw composite after extrusion and aging. J. Alloys Compd. 2020, 830, 154709. [Google Scholar] [CrossRef]
- Chmelík, F.; Lukáč, P.; Janeček, M.; Moll, F.; Mordike, B.L.; Kainer, K.-U.; Langdon, T.G. An evaluation of the creep characteristics of an AZ91 magnesium alloy composite using acoustic emission. Mater. Sci. Eng. A 2002, 338, 1–7. [Google Scholar] [CrossRef]
- Shamekh, M.; Pugh, M.; Medraj, M. Processing and Characterization of In Situ (TiC–TiB2)p/AZ91D Magnesium Matrix Composites. Adv. Eng. Mater. 2013, 15, 708–717. [Google Scholar] [CrossRef]
- Dieringa, H.; Huang, Y.; Maier, P.; Hort, N.; Kainer, K.U. Tensile and compressive creep behaviour of Al2O3 (Saffil®) short fiber reinforced magnesium alloy AE42. Mater. Sci. Eng. A 2005, 410–411, 85–88. [Google Scholar] [CrossRef]
- Sato, T. Power-Law Creep Behaviour in Magnesium and Its Alloys. Ph.D. Thesis, University of Canterbury, Mechanical Engineering, Christchurch, New Zealand, 2008. [Google Scholar]
- Kim, J.; Kaneko, J.; Sugamata, M. High Temperature Deformation of SiC Whisker/ AZ91 Magnesium Alloy and SiC Whisker/2324 Aluminum Alloy Composites. J. Jpn. Inst. Met. 1992, 56, 819–827. [Google Scholar] [CrossRef]
- Nieh, T.G.; Schwartz, A.J.; Wadsworth, J. Superplasticity in a 17 vol.% SiC particulate-reinforced ZK60A magnesium composite (ZK60/SiC/17p). Mater. Sci. Eng. A 1996, 208, 30–36. [Google Scholar] [CrossRef]
- Moheimani, S.K.; Azadeh, K.; Khademzadeh, S.; Tayebi, M.; Rajaee, A.; Saboori, A. Tribological behaviour of AZ31 magnesium alloy reinforced by bimodal size B4C after precipitation hardening. J. Magnes. Alloy. 2022, 10, 3267–3280. [Google Scholar] [CrossRef]
- Korbel, A.; Bochniak, W.; Ostachowski, P.; Błaż, L. Visco-Plastic Flow of Metal in Dynamic Conditions of Complex Strain Scheme. Met. Mater. Trans. A 2011, 42, 2881–2897. [Google Scholar] [CrossRef]
- Wang, X.; Yang, J.; Chi, P.; Bahonar, E.; Tayebi, M. Effects of the microstructure and precipitation hardening on the thermal expansion behavior of ZK60 magnesium alloy. J. Alloys Compd. 2021, 901, 163422. [Google Scholar] [CrossRef]
- TIAN, J.; SHI, Z. Creep mechanism and creep constitutive model of aluminum silicate short-fiber-reinforced magnesium matrix composite. Trans. Nonferrous Met. Soc. China 2014, 24, 632–640. [Google Scholar] [CrossRef]
- Al-Samman, T.; Molodov, K.D.; Molodov, D.A.; Gottstein, G.; Suwas, S. Softening and dynamic recrystallization in magnesium single crystals during c-axis compression. Acta Mater. 2012, 60, 537–545. [Google Scholar] [CrossRef]
- Chawla, N.; Chawla, K.K. (Eds.) Metal Matrix Composites; Springer US: Boston, MA, USA, 2006; pp. 305–335. [Google Scholar] [CrossRef]
- Kaibyshev, R.; Sitdikov, O. Dynamic Recrystallization of Magnesium at Ambient Temperature. Z. Met. 1994, 85, 738–743. [Google Scholar] [CrossRef]
- Athul, K.R.; Pillai, U.T.S.; Srinivasan, A.; Pai, B.C. A Review of Different Creep Mechanisms in Mg Alloys Based on Stress Exponent and Activation Energy. Adv. Eng. Mater. 2016, 18, 770–794. [Google Scholar] [CrossRef]
- Mukherjee, A.K. Superplasticity in Metals, Ceramics and Intermetallics; VCH Verlagsges: Berlin, Germany, 1993. [Google Scholar]
- Masuda, H.; Tobe, H.; Sato, E.; Sugino, Y.; Ukai, S. Diffusional mass flux accommodating two-dimensional grain boundary sliding in ODS ferritic steel. Acta Mater. 2019, 176, 63–72. [Google Scholar] [CrossRef]
- Khorshidi, R.; Mahmudi, R.; Honarbakhsh-Raouf, A. Compressive creep behavior of a cast Al–15Mg2Si in situ composite. Mater. Sci. Eng. A 2016, 668, 112–119. [Google Scholar] [CrossRef]
- Barnett, M.R. A taylor model based description of the proof stress of magnesium AZ31 during hot working. Met. Mater. Trans. A 2003, 34, 1799–1806. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, S.; Zhang, T.; Zhou, X.; Li, S. Effects of post-weld heat treatment on the microstructure and mechanical properties of laser-welded NiTi/304SS joint with Ni filler. Mater. Sci. Eng. A 2020, 771, 138545. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Jonas, J.J. Formability and Workability of Metals: Plastic Instability and Flow Localization; American Society for Metals: Detroit, MI, USA, 1984; Available online: https://books.google.com/books?id=A5lxAAAACAAJ (accessed on 30 November 2007).
- Sandlöbes, S.; Zaefferer, S.; Schestakow, I.; Yi, S.; Gonzalez-Martinez, R. On the role of non-basal deformation mechanisms for the ductility of Mg and Mg–Y alloys. Acta Mater. 2011, 59, 429–439. [Google Scholar] [CrossRef]
- Su, J.; Sanjari, M.; Kabir, A.S.H.; Jung, I.-H.; Yue, S. Dynamic recrystallization mechanisms during high speed rolling of Mg–3Al–1Zn alloy sheets. Scr. Mater. 2016, 113, 198–201. [Google Scholar] [CrossRef]
- Yu, H.; Xin, Y.; Wang, M.; Liu, Q. Hall-Petch relationship in Mg alloys: A review. J. Mater. Sci. Technol. 2018, 34, 248–256. [Google Scholar] [CrossRef]
- Shi, B.Q.; Cheng, Y.Q.; Shang, X.L.; Yan, H.; Chen, R.S.; Ke, W. Hall-Petch relationship, twinning responses and their dependences on grain size in the rolled Mg-Zn and Mg-Y alloys. Mater. Sci. Eng. A 2019, 743, 558–566. [Google Scholar] [CrossRef]
- Takahashi, T.; Ueda, M.; Iizuka, K.; Yoshimura, A.; Yokozeki, T. Simulation on kink-band formation during axial compression of a unidirectional carbon fiber-reinforced plastic constructed by X-ray computed tomography images. Adv. Compos. Mater. 2019, 28, 347–363. [Google Scholar] [CrossRef]
- Ueta, R.; Shizawa, K. Investigation on Position of Kink Band Formation in Single Crystal of Mg-Based LPSO Phase Using Dislocation-Based Crystal Plasticity Simulation. J. Soc. Mater. Sci. Jpn. 2016, 65, 220–227. [Google Scholar] [CrossRef]
- Wu, S.; Zhang, Z.; Zhang, J.; Xu, C.; Niu, X.; Liu, W. Effects of Phase Content and Evolution on the Mechanical Properties of Mg 95 Y 2.5 Zn 2.5 and Mg 93.1 Y 2.5 Zn 2.5 Ti 1.6 Zr 0.3 Alloys Containing LPSO and W Phases: Effects of Phase Content and Evolution. Adv. Eng. Mater. 2017, 19, e201700185. [Google Scholar] [CrossRef]
- Hémery, S.; Tromas, C.; Villechaise, P. Slip-stimulated grain boundary sliding in Ti-6Al-4V at room temperature. Materialia 2018, 5, 100189. [Google Scholar] [CrossRef]
- He, T.; Feng, M. Combined effects of cooperative grain boundary sliding and migration and reinforced particles on crack growth in fine-grained Mg alloys. J. Alloys Compd. 2018, 749, 705–714. [Google Scholar] [CrossRef]
- Zhang, B.; Wang, Z.; Yu, H.; Ning, Y. Microstructural origin and control mechanism of the mixed grain structure in Ni-based superalloys. J. Alloys Compd. 2022, 900, 163515. [Google Scholar] [CrossRef]
- Barnett, M.R. 3-Twinning and its role in wrought magnesium alloys. In Woodhead Publishing Series in Metals and Surface Engineering; Bettles, C., Barnett, M.B.T.-A., Eds.; Woodhead Publishing: Shaxton, UK, 2012; pp. 105–143. [Google Scholar] [CrossRef]
- Wu, X.J.; Koul, A.K. Grain boundary sliding in the presence of grain boundary precipitates during transient creep. Met. Mater. Trans. A 1995, 26, 905–914. [Google Scholar] [CrossRef]
- Castany, P.; Pettinari-Sturmel, F.; Douin, J.; Coujou, A. TEM quantitative characterization of short-range order and its effects on the deformation micromechanims in a Ti-6Al-4V alloy. Mater. Sci. Eng. A 2017, 680, 85–91. [Google Scholar] [CrossRef]
- Echlin, M.P.; Stinville, J.C.; Miller, V.M.; Lenthe, W.C.; Pollock, T.M. Incipient slip and long range plastic strain localization in microtextured Ti-6Al-4V titanium. Acta Mater. 2016, 114, 164–175. [Google Scholar] [CrossRef]
- Hémery, S.; Dang, V.T.; Signor, L.; Villechaise, P. Influence of Microtexture on Early Plastic Slip Activity in Ti-6Al-4V Polycrystals. Met. Mater. Trans. A 2018, 49, 2048–2056. [Google Scholar] [CrossRef]
- Li, H.; Boehlert, C.J.; Bieler, T.R.; Crimp, M.A. Examination of the distribution of the tensile deformation systems in tension and tension-creep of Ti-6Al-4V (wt.%) at 296 K and 728 K. Philos. Mag. 2015, 95, 691–729. [Google Scholar] [CrossRef]
- Püschl, W.; Schoeck, G.; Kirchner, H.O.K. The line tension of dislocations in anisotropic media. Philos. Mag. A 1987, 56, 553–563. [Google Scholar] [CrossRef]
- Wang, J.; Guo, X.; Qin, J.; Zhang, D.; Lu, W. Microstructure and mechanical properties of investment casted titanium matrix composites with B4C additions. Mater. Sci. Eng. A 2015, 628, 366–373. [Google Scholar] [CrossRef]
- Liang, L.; Xu, M.; Chen, Y.; Zhang, T.; Tong, W.; Liu, H.; Wang, H.; Li, H. Effect of welding thermal treatment on the microstructure and mechanical properties of nickel-based superalloy fabricated by selective laser melting. Mater. Sci. Eng. A 2021, 819, 141507. [Google Scholar] [CrossRef]
- Das, A.; Kumar Chakravartty, J. Fractographic correlations with mechanical properties in ferritic martensitic steels. Surf. Topogr. Metrol. Prop. 2017, 5, 45006. [Google Scholar] [CrossRef]
- Koyama, M.; Akiyama, E.; Tsuzaki, K. Effects of Static and Dynamic Strain Aging on Hydrogen Embrittlement in TWIP Steels Containing Al; J-STAGE, Tetsu-to-Hagane 2014; Elsevier: Amsterdam, The Netherlands, 2014; Volume 100, pp. 1132–1139. [Google Scholar] [CrossRef]




| Sample | Temperature (°C) | Stress (MPa) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| ZK60 alloy | 200 | 10 | 20 | 30 | 40 | 50 | 60 | – | – |
| 250 | 10 | 20 | 30 | 40 | – | – | – | – | |
| ZK60/SiCp composite | 200 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 |
| 250 | 10 | 20 | 30 | 40 | 50 | 60 | – | – | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.-Y.; Jia, C.; Xu, M.; Kaseem, M.; Tayebi, M. Microstructural Changes Caused by the Creep Test in ZK60 Alloy Reinforced by SiCp at Intermediate Temperature after KOBO Extrusion and Aging. Materials 2023, 16, 3885. https://doi.org/10.3390/ma16103885
Wang Y-Y, Jia C, Xu M, Kaseem M, Tayebi M. Microstructural Changes Caused by the Creep Test in ZK60 Alloy Reinforced by SiCp at Intermediate Temperature after KOBO Extrusion and Aging. Materials. 2023; 16(10):3885. https://doi.org/10.3390/ma16103885
Chicago/Turabian StyleWang, Yang-Yang, Chen Jia, Min Xu, Mosab Kaseem, and Morteza Tayebi. 2023. "Microstructural Changes Caused by the Creep Test in ZK60 Alloy Reinforced by SiCp at Intermediate Temperature after KOBO Extrusion and Aging" Materials 16, no. 10: 3885. https://doi.org/10.3390/ma16103885
APA StyleWang, Y.-Y., Jia, C., Xu, M., Kaseem, M., & Tayebi, M. (2023). Microstructural Changes Caused by the Creep Test in ZK60 Alloy Reinforced by SiCp at Intermediate Temperature after KOBO Extrusion and Aging. Materials, 16(10), 3885. https://doi.org/10.3390/ma16103885







