Harmonic and DC Bias Hysteresis Characteristics Simulation Based on an Improved Preisach Model
Abstract
:1. Introduction
2. Dynamic Preisach Hysteresis Model
3. Methodology
4. Results
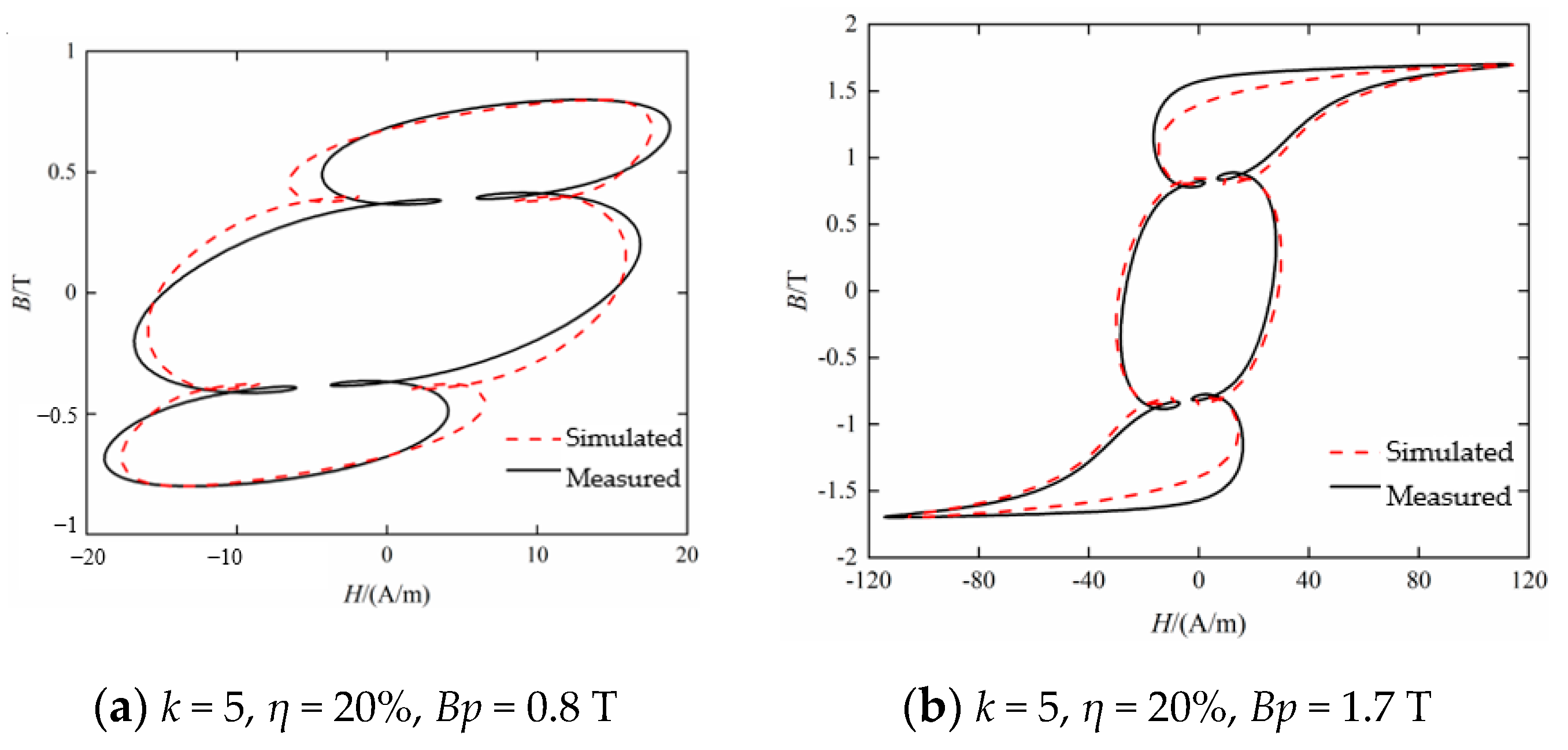
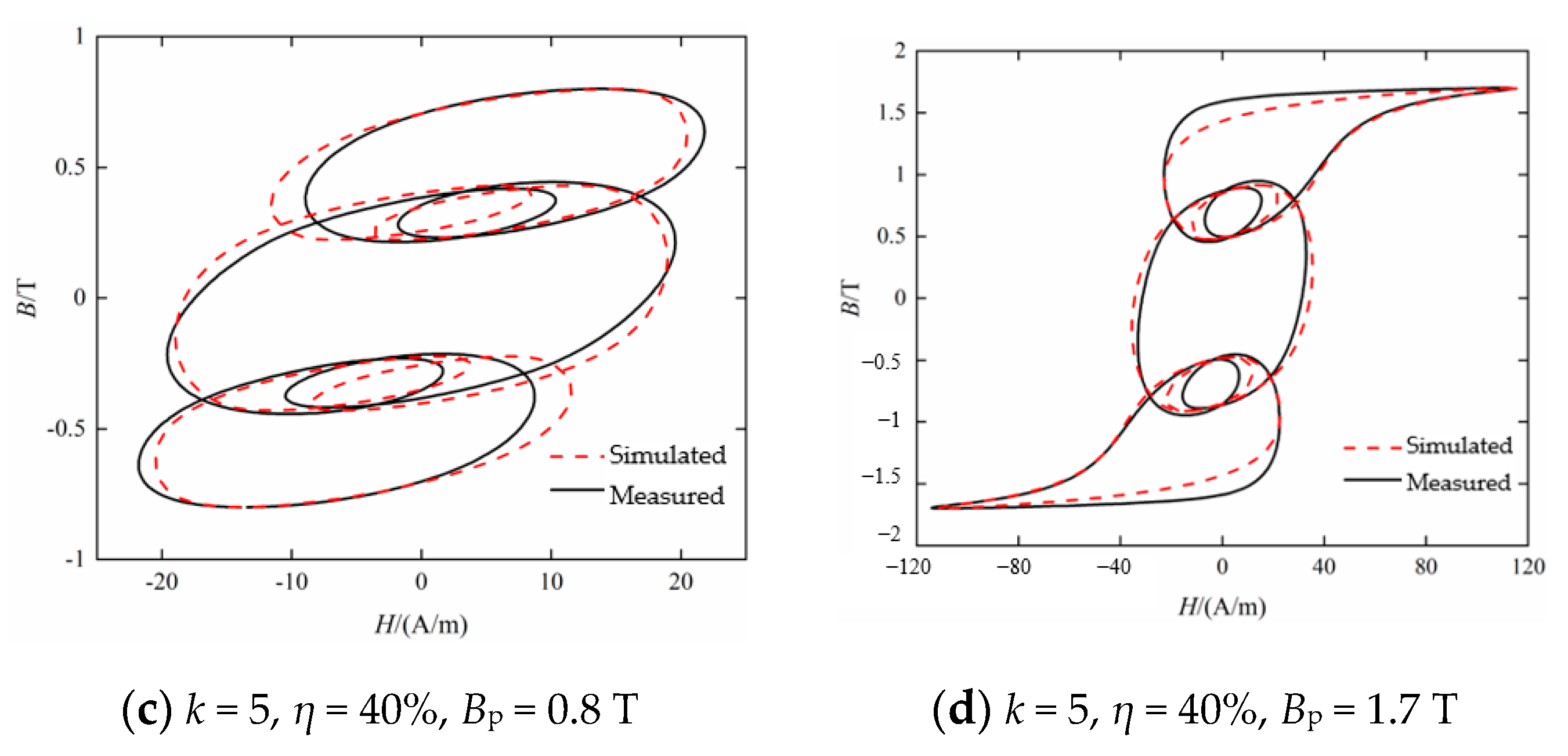
4.1. Fitting Results under Single Harmonic Excitation
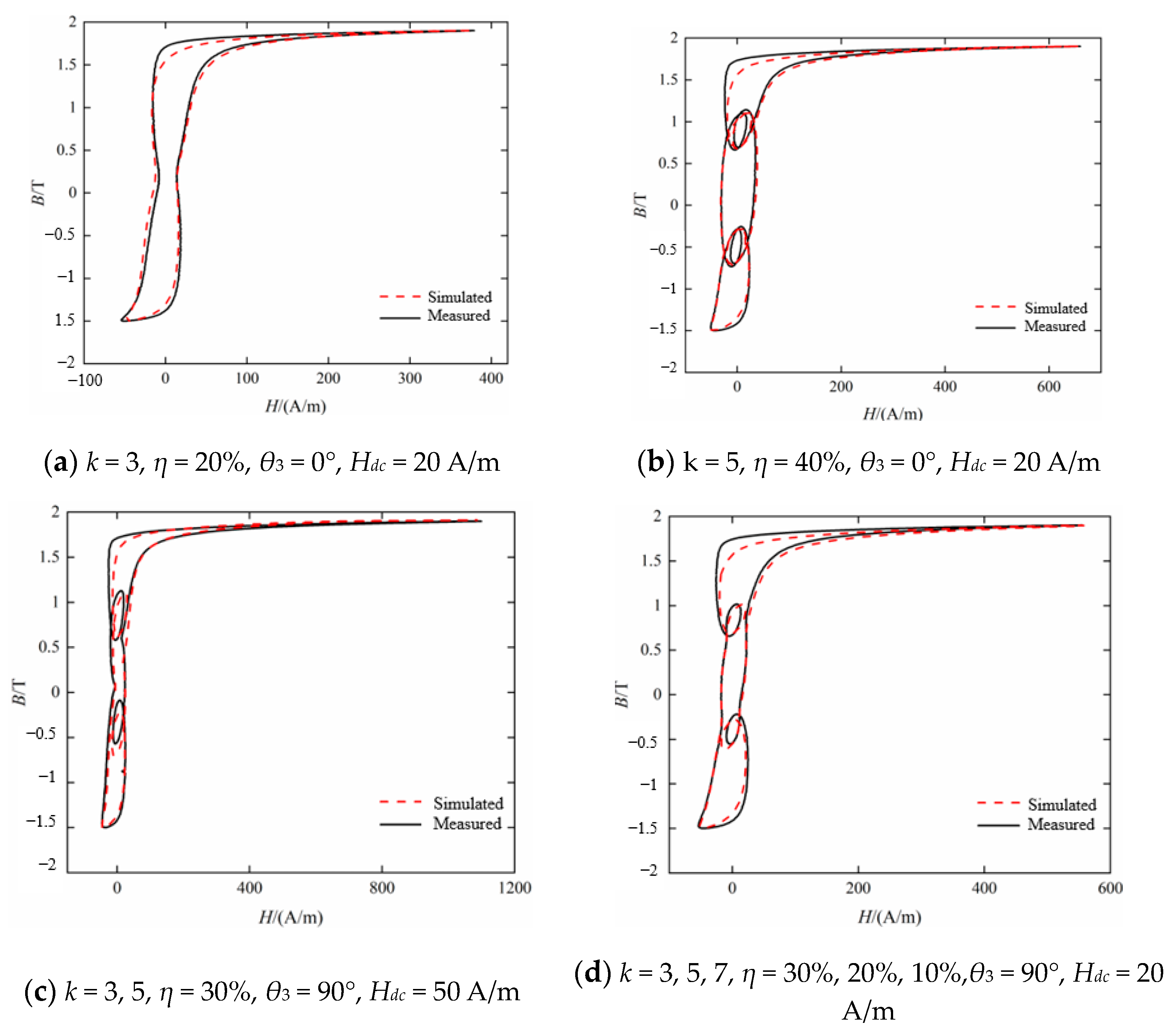
4.2. Simulation of Hysteresis Characteristics under AC-DC Hybrid Excitation
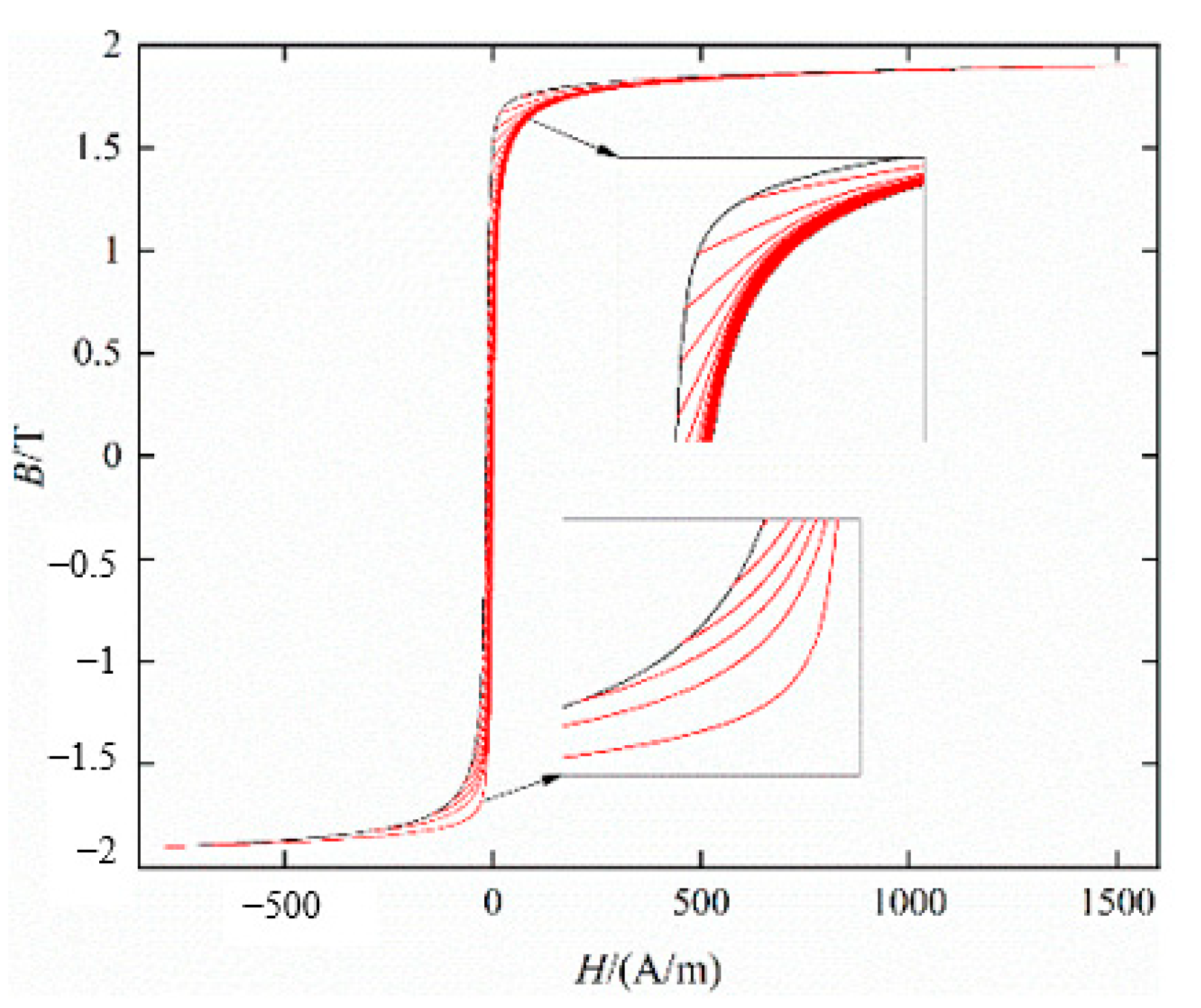
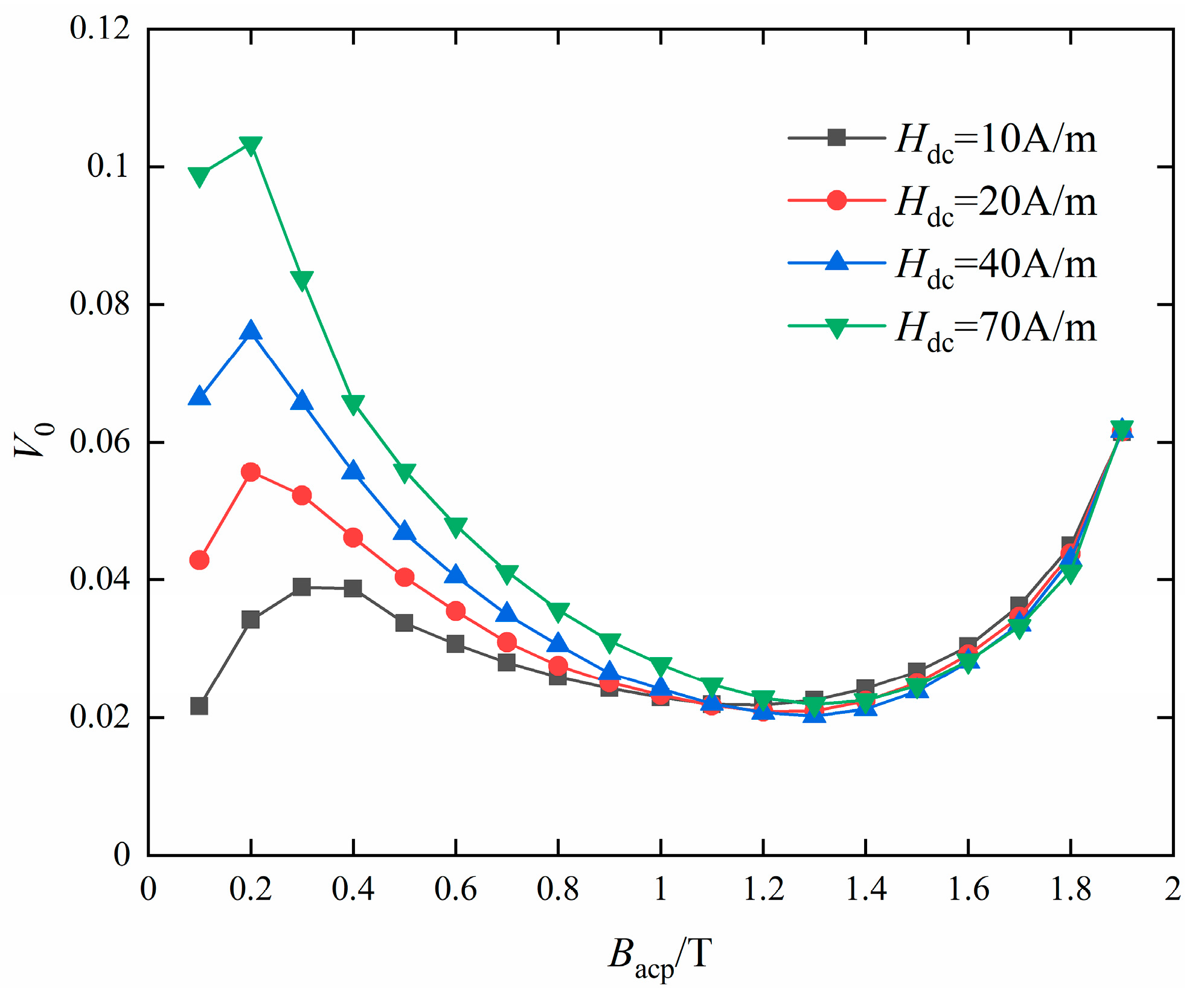
Hysteresis Model under DC Bias
5. Conclusions
- Based on the asymmetric limiting hysteresis loop, the first-order reversal curve under bias magnetic field is generated, and the parameter identification of the Preisach hysteresis model is realized, which can simulate the harmonic bias hysteresis characteristics more accurately.
- Considering the influence of DC bias magnetic field and AC flux density peak on excess loss, the function formula of relevant parameters in excess loss is constructed, and the parameters are extracted based on the experimental results, so as to realize the accurate simulation of hysteresis loop under DC bias conditions.
- When the DC magnetic field strength increases from 0 A/m to 100 A/m, the loss also increases. However, the speed of loss change gradually decreases as the magnetic field strength increases.
- The improved numerical simulation method in this paper is suitable for the simulation of asymmetric hysteresis characteristics. However, due to the different types of silicon steel sheets, the influence of DC bias on total loss and different types of loss will also be different. This paper considers the hysteresis characteristics of DC bias, but not other working conditions, such as the influence of temperature and stress on the hysteresis loop that need to be studied and improved in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mörée, G.; Leijon, M. Review of Play and Preisach models for hysteresis in magnetic materials. Materials 2023, 16, 2422. [Google Scholar] [CrossRef] [PubMed]
- Farrokh, M.; Dizaji, F.S.; Dizaji, M.S. Hysteresis identification using extended preisach neural network. Neural Process. Lett. 2022, 54, 1523–1547. [Google Scholar] [CrossRef]
- Li, Y.; Chen, R.; Cheng, Z.; Liu, L. Dynamic hysteresis loops modeling of electrical steel with harmonic components. IEEE Transactions on Industry Applications 2020, 56, 4804–4811. [Google Scholar] [CrossRef]
- Daniels, B.; Curti, M.; Lomonova, E.; Overboom, T. Interpolation of Measured Hysteresis Loops for Proper Construction of the Everett Map. In Proceedings of the 2022 IEEE 20th Biennial Conference on Electromagnetic Field Computation (CEFC), Denver, CO, USA, 24–26 October 2022. [Google Scholar]
- Arzuza, L.C.C.; Béron, F.; Pirota, K.R. High-frequency GMI hysteresis effect analysis by first-order reversal curve (FORC) method. J. Magn. Magn. Mater. 2021, 534, 168008. [Google Scholar] [CrossRef]
- Wang, H.; Yang, Q.; Li, Y.; Li, Y.; Wang, J. Simulation and Analysis on Harmonic Losses in Structural Parts of Converter Transformer under DC Bias. In Proceedings of the 2020 IEEE International Conference on Applied Superconductivity and Electromagnetic Devices (ASEMD), Tianjin, China, 16–18 October 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1–2. [Google Scholar]
- Krings, A.; Cossale, M.; Tenconi, A.; Soulard, J.; Cavagnino, A.; Boglietti, A. Magnetic materials used in electrical machines: A comparison and selection guide for early machine design. IEEE Ind. Appl. Mag. 2017, 23, 21–28. [Google Scholar] [CrossRef] [Green Version]
- You, J.; Yu, H.; Liang, H.; Xie, Y.; Ding, D. A multi-parameter model of heat treatment process for soft magnetic materials on performance of HSERs. Chin. J. Aeronaut. 2022, 35, 379–388. [Google Scholar] [CrossRef]
- Valdez-Grijalva, M.A.; Nagy, L.; Muxworthy, A.R.; Williams, W.; Roberts, A.P.; Heslop, D. Micromagnetic simulations of first-order reversal curve (FORC) diagrams of framboidal greigite. Geophys. J. Int. 2020, 222, 1126–1134. [Google Scholar] [CrossRef]
- Zhao, X.; Xu, H.; Li, Y.; Zhou, L.; Liu, X.; Zhao, H.; Liu, Y.; Yuan, D. Improved Preisach model for the vector hysteresis property of soft magnetic composite materials based on the hybrid technique of SA-NMS. IEEE Trans. Ind. Appl. 2021, 57, 5517–5526. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, R.; Liu, X.; Li, L. A dynamic hysteresis model for loss estimation of GO silicon steel under DC-biased magnetization. IEEE Trans. Ind. Appl. 2020, 57, 409–416. [Google Scholar] [CrossRef]
- Liu, R.; Li, L. Accurate symmetrical minor loops calculation with a modified energetic hysteresis model. IEEE Trans. Magn. 2020, 56, 7510204. [Google Scholar] [CrossRef]
- Hauser, H. Energetic model of ferromagnetic hysteresis 2: Magnetization calculations of (110)[001] FeSi sheetss by statistic domain behavior. J. Appl. Phys. 1995, 77, 2625–2633. [Google Scholar] [CrossRef]
- Hammouche, A.; Hamimid, M.; Kansab, A. A single phase transformer modeling based on rat dependent classical energetic hysteresis model. Mater. Today Proc. 2022, 51, 2139–2143. [Google Scholar] [CrossRef]
- Enokizono, M.; Fujita, Y. Improvement of E&S modeling for eddy-current magnetic field analysis. IEEE Trans. Magn. 2002, 38, 881–884. [Google Scholar]
- Willerich, S.; Herzog, H.G. A continuous vector preisach model based on vectorial relay operators. IEEE Trans. Magn. 2020, 56, 7511204. [Google Scholar] [CrossRef]
- Chen, J.; Wang, S.; Shang, H.; Hu, H.; Peng, T. Finite Element Analysis of Axial Flux Permanent Magnetic Hysteresis Dampers Based on Vector Jiles-Atherton Model. IEEE Trans. Energy Convers. 2022, 37, 2472–2481. [Google Scholar] [CrossRef]

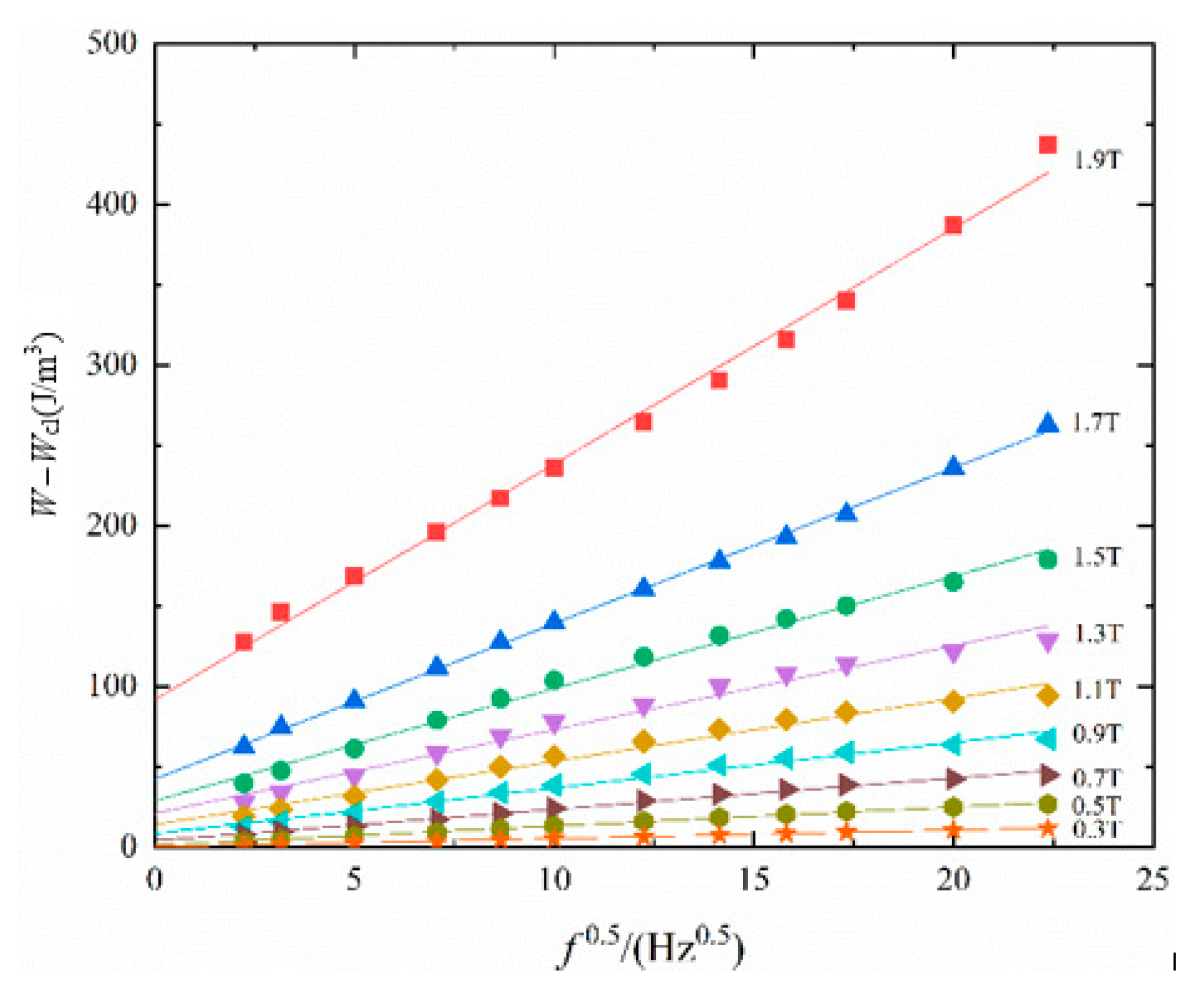
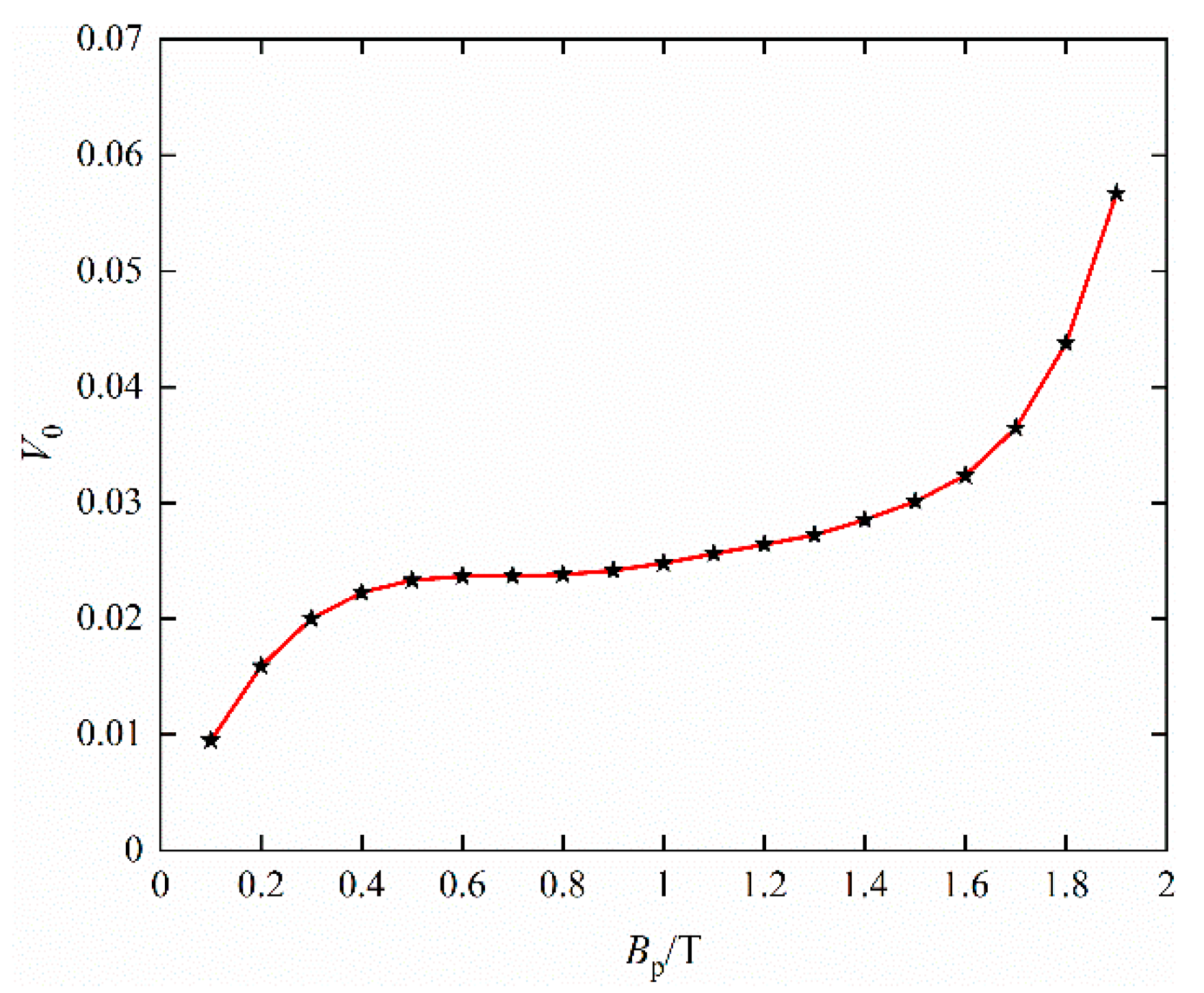

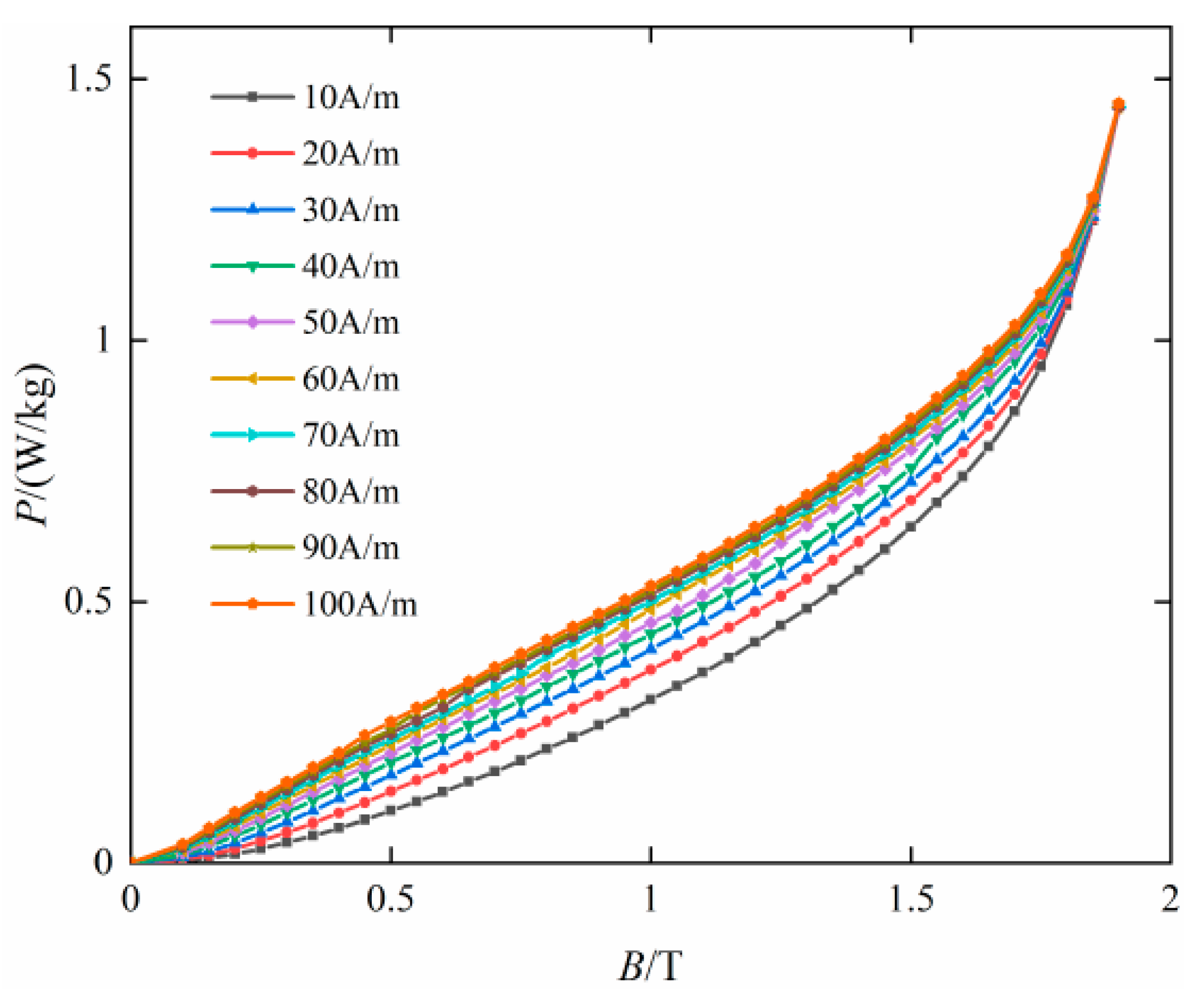
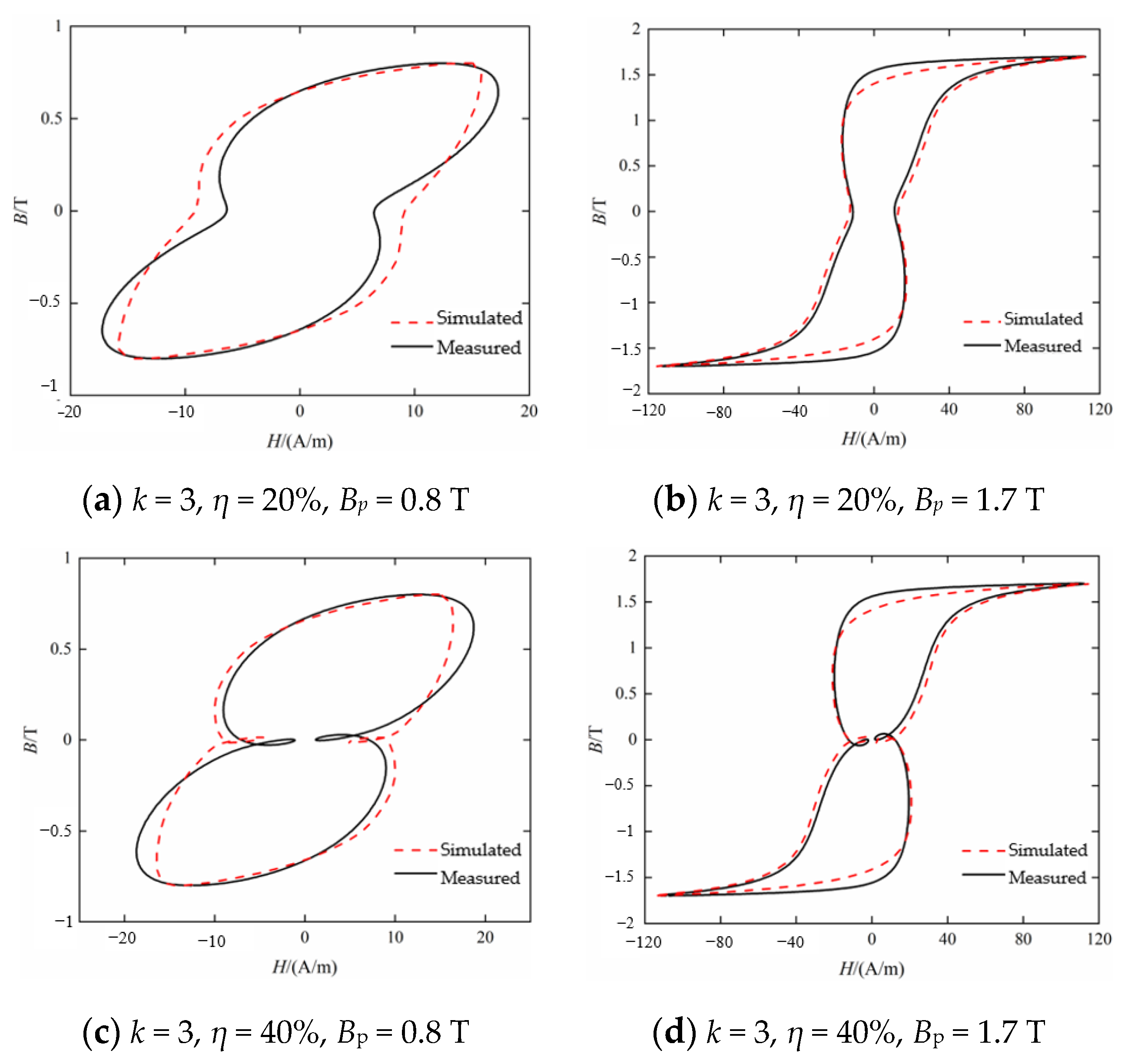




| Parameter | Numeric Value |
|---|---|
| B/T | 0.001~2 |
| H/(A/m) | 1~10,000 |
| N1:N2 | 197:199 |
| Equivalent magnetic circuit length/m | 0.45 |
| Excitation | η (%) | Bp (T) | Pcal (W/kg) | Pmea (W/kg) | Relative Error |
|---|---|---|---|---|---|
| k = 3 θ = 0° | 20 | 0.8 | 0.1856 | 0.1795 | 3.40% |
| 20 | 1.7 | 0.8841 | 0.9129 | 3.15% | |
| 40 | 0.8 | 0.2087 | 0.2101 | 0.67% | |
| 40 | 1.7 | 1.014 | 1.0410 | 2.59% | |
| k = 5 θ = 0° | 20 | 0.8 | 0.2305 | 0.2229 | 3.41% |
| 20 | 1.7 | 1.0658 | 1.0956 | 2.72% | |
| 40 | 0.8 | 0.3541 | 0.3495 | 1.32% | |
| 40 | 1.7 | 1.5893 | 1.6328 | 2.66% |
| Excitation | θ (°) | Hdc(A/m) | Pcal (W/kg) | Pmea (W/kg) | PHdc=0 (W/kg) | Relative Error |
|---|---|---|---|---|---|---|
| k = 3 (20%) | 0 | 20 | 0.9385 | 0.9511 | 0.9129 | 1.32% |
| k = 5 (40%) | 0 | 20 | 1.6549 | 1.7165 | 1.6328 | 3.59% |
| k = 3, 5 (30%) | 90 | 50 | 1.4510 | 1.5152 | 1.3703 | 4.23% |
| k = 3, 5, 7 (30%, 20%, 10%) | 90 | 20 | 1.2157 | 1.2688 | 1.2117 | 4.19% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Li, H.; Tian, Y.; Li, Y.; Yang, Q. Harmonic and DC Bias Hysteresis Characteristics Simulation Based on an Improved Preisach Model. Materials 2023, 16, 4385. https://doi.org/10.3390/ma16124385
Zhang C, Li H, Tian Y, Li Y, Yang Q. Harmonic and DC Bias Hysteresis Characteristics Simulation Based on an Improved Preisach Model. Materials. 2023; 16(12):4385. https://doi.org/10.3390/ma16124385
Chicago/Turabian StyleZhang, Changgeng, Haoran Li, Yakun Tian, Yongjian Li, and Qingxin Yang. 2023. "Harmonic and DC Bias Hysteresis Characteristics Simulation Based on an Improved Preisach Model" Materials 16, no. 12: 4385. https://doi.org/10.3390/ma16124385







