Size- and Chirality-Dependent Structural and Mechanical Properties of Single-Walled Phenine Nanotubes
Abstract
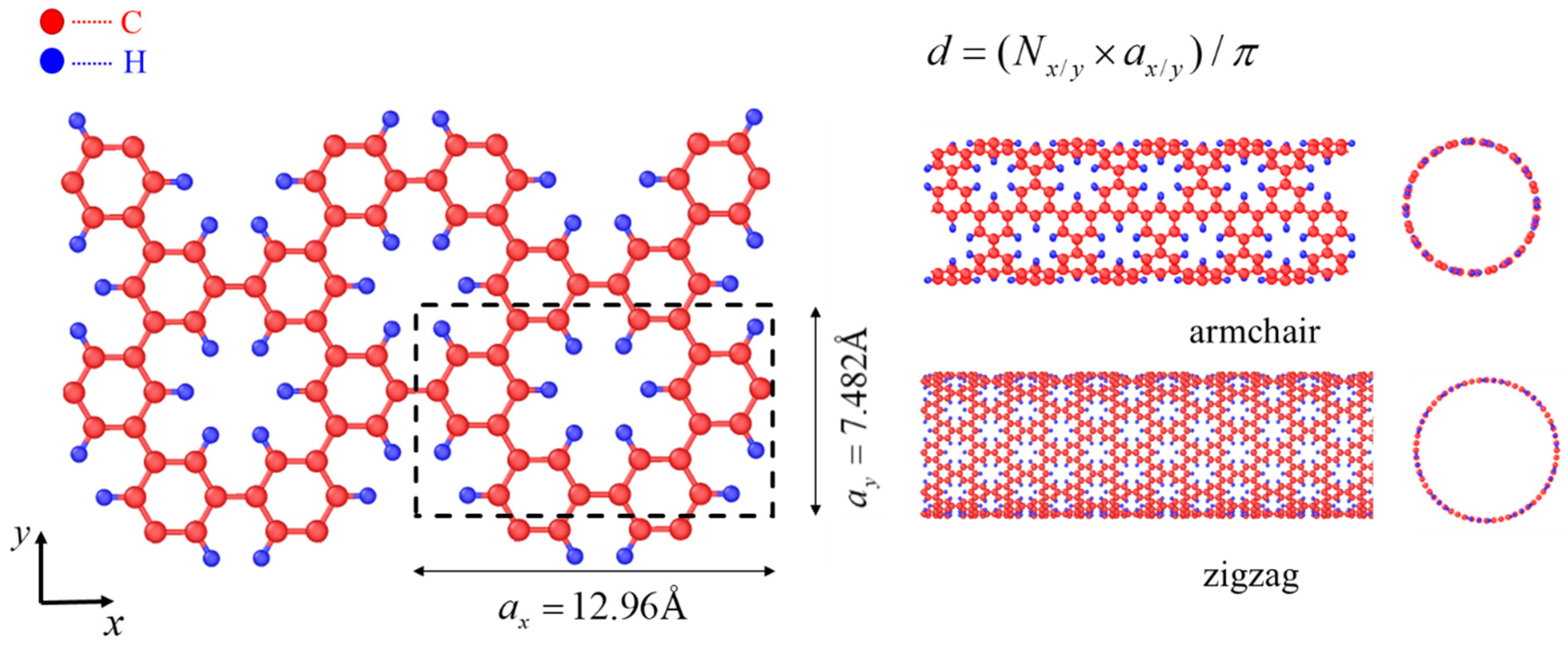
:1. Introduction
2. Simulation Details
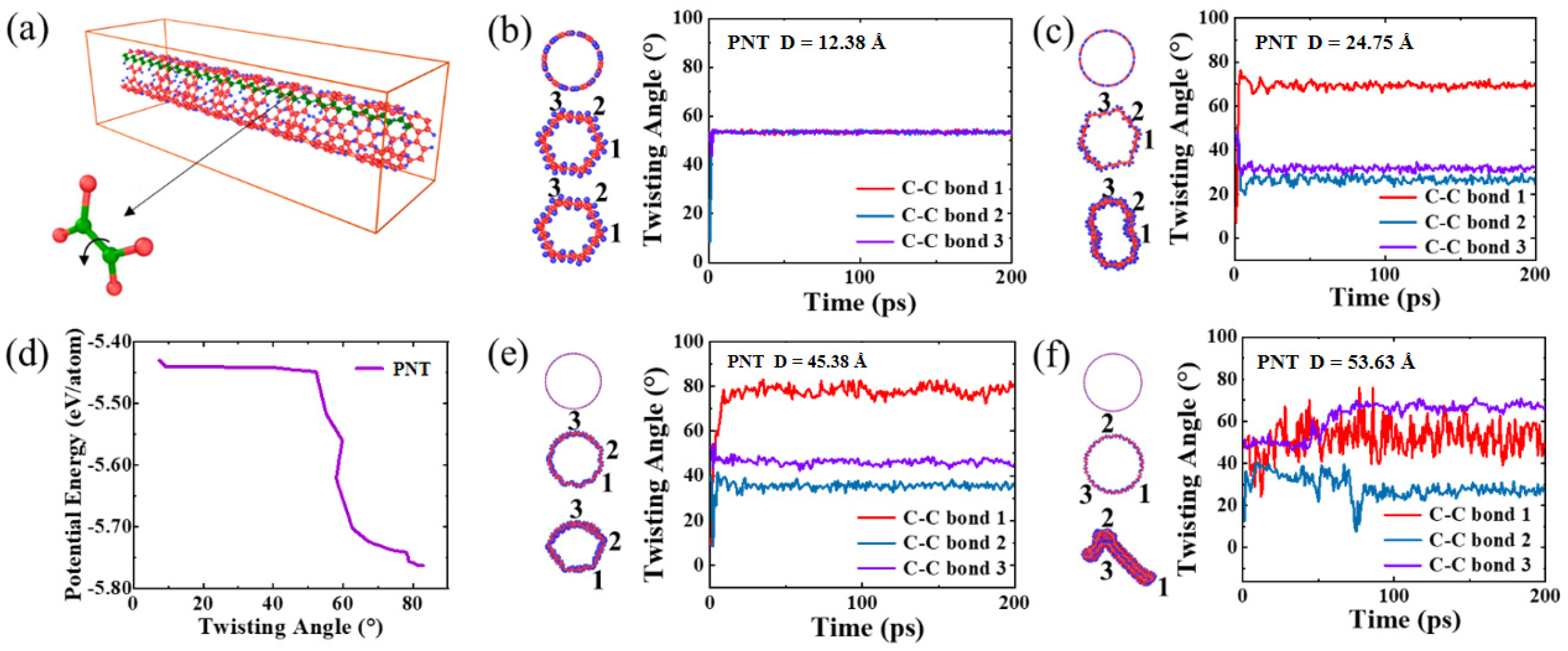
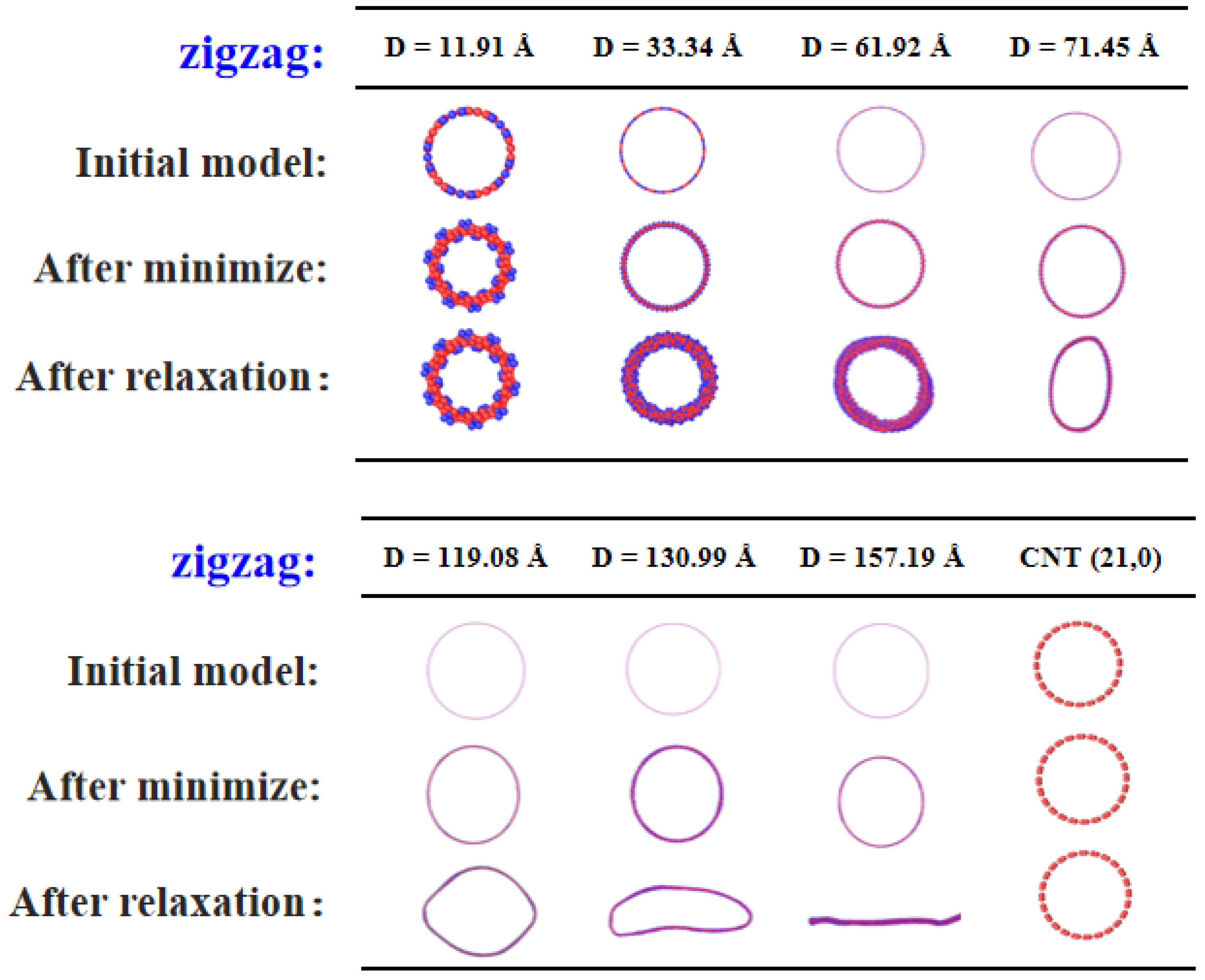
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, J.; Itkis, M.E.; Yu, A.; Bekyarova, E.; Zhao, B.; Haddon, R.C. Continuous spinning of a single-walled carbon nanotube−nylon composite fiber. J. Am. Chem. Soc. 2005, 127, 3847–3854. [Google Scholar] [CrossRef]
- Yang, X.; Tang, H.; Cao, K.; Song, H.; Sheng, W.; Wu, Q. Templated-assisted one-dimensional silica nanotubes: Synthesis and applications. J. Mater. Chem. 2011, 21, 6122–6135. [Google Scholar] [CrossRef]
- Thostenson, E.T.; Ren, Z.; Chou, T.-W. Advances in the science and technology of carbon nanotubes and their composites: A review. Compos. Sci. Technol. 2001, 61, 1899–1912. [Google Scholar] [CrossRef] [Green Version]
- Qian, D.; Wagner, G.J.; Liu, W.K.; Yu, M.-F.; Ruoff, R.S. Mechanics of carbon nanotubes. Appl. Mech. Rev. 2002, 55, 495–533. [Google Scholar] [CrossRef]
- Xiang, J.; Yu, X.-Y.; Paik, U. General synthesis of vanadium-based mixed metal oxides hollow nanofibers for high performance lithium-ion batteries. J. Power Sources 2016, 329, 190–196. [Google Scholar] [CrossRef]
- Wang, C.; Takei, K.; Takahashi, T.; Javey, A. Carbon nanotube electronics—Moving forward. Chem. Soc. Rev. 2013, 42, 2592–2609. [Google Scholar] [CrossRef]
- Peng, L.-M.; Zhang, Z.; Qiu, C. Carbon nanotube digital electronics. Nat. Electron. 2019, 2, 499–505. [Google Scholar] [CrossRef]
- Raychowdhury, A.; Roy, K. Carbon nanotube electronics: Design of high-performance and low-power digital circuits. IEEE Trans. Circuits Syst. I Regul. Pap. 2007, 54, 2391–2401. [Google Scholar] [CrossRef]
- Madani, S.Y.; Naderi, N.; Dissanayake, O.; Tan, A.; Seifalian, A.M. A new era of cancer treatment: Carbon nanotubes as drug delivery tools. Int. J. Nanomed. 2011, 6, 2963–2979. [Google Scholar]
- Choudhary, M.; Sharma, A.; Aravind Raj, S.; Sultan, M.T.H.; Hui, D.; Shah, A.U.M. Contemporary review on carbon nanotube (CNT) composites and their impact on multifarious applications. J. Am. Chem. Soc. 2022, 11, 2632–2660. [Google Scholar] [CrossRef]
- Vigolo, B.; Pénicaud, A.; Coulon, C.; Sauder, C.; Pailler, R.; Journet, C.; Bernier, P.; Poulin, P. Macroscopic fibers and ribbons of oriented carbon nanotubes. Science 2000, 290, 1331–1334. [Google Scholar] [CrossRef]
- Iijima, T.; Oshima, H.; Hayashi, Y.; Suryavanshi, U.B.; Hayashi, A.; Tanemura, M.J.D.; Materials, R. In-situ observation of carbon nanotube fiber spinning from vertically aligned carbon nanotube forest. Diam. Relat. Mater. 2012, 24, 158–160. [Google Scholar] [CrossRef]
- Jee, M.H.; Choi, J.U.; Park, S.H.; Jeong, Y.G.; Baik, D.H. Influences of tensile drawing on structures, mechanical, and electrical properties of wet-spun multi-walled carbon nanotube composite fiber. Macromol. Res. 2012, 20, 650–657. [Google Scholar] [CrossRef]
- Liu, C.; Fan, Y.Y.; Liu, M.; Cong, H.T.; Cheng, H.M.; Dresselhaus, M.S. Hydrogen Storage in Single-Walled Carbon Nanotubes at Room Temperature. Science 1999, 286, 1127–1129. [Google Scholar] [CrossRef] [Green Version]
- Ajayan, P.M. Nanotubes from Carbon. Chem. Rev. 1999, 99, 1787–1800. [Google Scholar] [CrossRef] [PubMed]
- García-Hernández, E.; Chigo-Anota, E. Structural defects on (5,5) single-walled carbon nanotubes: Impact on their electronic properties and chemical reactivity from a DFT perspective. Physica E Low Dimens. Syst. 2021, 134, 114874. [Google Scholar] [CrossRef]
- Collins, P.G.; Hersam, M.; Arnold, M.; Martel, R.; Avouris, P. Current Saturation and Electrical Breakdown in Multiwalled Carbon Nanotubes. Phys. Rev. Lett. 2001, 86, 3128–3131. [Google Scholar] [CrossRef] [PubMed]
- Collins, P.G.; Arnold, M.S.; Avouris, P. Engineering Carbon Nanotubes and Nanotube Circuits Using Electrical Breakdown. Science 2001, 292, 706–709. [Google Scholar] [CrossRef]
- Liao, A.; Alizadegan, R.; Ong, Z.-Y.; Dutta, S.; Xiong, F.; Hsia, K.J.; Pop, E. Thermal dissipation and variability in electrical breakdown of carbon nanotube devices. Phys. Rev. B 2010, 82, 205406. [Google Scholar] [CrossRef] [Green Version]
- Jaehyun, C.; Kyong-Hoon, L.; Junghoon, L.; Diego, T.; George, C.S. Multi-walled carbon nanotubes experiencing electrical breakdown as gas sensors. Nanotechnology 2004, 15, 1596. [Google Scholar]
- Buh, G.H.; Hwang, J.H.; Jeon, E.K.; So, H.M.; Lee, J.O.; Kong, K.J.; Chang, H. On-Chip Electrical Breakdown of Metallic Nanotubes for Mass Fabrication of Carbon-Nanotube-Based Electronic Devices. IEEE Trans. Nanotechnol. 2008, 7, 624–627. [Google Scholar]
- Liu, J.; Wang, C.; Tu, X.; Liu, B.; Chen, L.; Zheng, M.; Zhou, C. Chirality-controlled synthesis of single-wall carbon nanotubes using vapour-phase epitaxy. Nat. Commun. 2012, 3, 1199. [Google Scholar] [CrossRef] [Green Version]
- Sanchez-Valencia, J.R.; Dienel, T.; Gröning, O.; Shorubalko, I.; Mueller, A.; Jansen, M.; Amsharov, K.; Ruffieux, P.; Fasel, R. Controlled synthesis of single-chirality carbon nanotubes. Nature 2014, 512, 61–64. [Google Scholar] [CrossRef] [Green Version]
- Liu, B.; Wu, F.; Gui, H.; Zheng, M.; Zhou, C. Chirality-Controlled Synthesis and Applications of Single-Wall Carbon Nanotubes. ACS Nano 2017, 11, 31–53. [Google Scholar] [CrossRef]
- Tomada, J.; Dienel, T.; Hampel, F.; Fasel, R.; Amsharov, K. Combinatorial design of molecular seeds for chirality-controlled synthesis of single-walled carbon nanotubes. Nat. Commun. 2019, 10, 3278. [Google Scholar] [CrossRef] [Green Version]
- Liu, B.; Wang, C.; Liu, J.; Che, Y.; Zhou, C. Aligned carbon nanotubes: From controlled synthesis to electronic applications. Nanoscale 2013, 5, 9483–9502. [Google Scholar] [CrossRef]
- Sun, Z.; Ikemoto, K.; Fukunaga, T.M.; Koretsune, T. Finite phenine nanotubes with periodic vacancy defects. Science 2019, 363, 151–155. [Google Scholar] [CrossRef]
- Treacy, M.M.J.; Ebbesen, T.W.; Gibson, J.M. Exceptionally high Young’s modulus observed for individual carbon nanotubes. Nature 1996, 381, 678–680. [Google Scholar] [CrossRef]
- Yu, M.-F.; Files, B.S.; Arepalli, S.; Ruoff, R.S. Tensile Loading of Ropes of Single Wall Carbon Nanotubes and their Mechanical Properties. Phys. Rev. Lett. 2000, 84, 5552–5555. [Google Scholar] [CrossRef] [Green Version]
- Tang, C.; Guo, W.; Chen, C. Mechanism for Superelongation of Carbon Nanotubes at High Temperatures. Phys. Rev. Lett. 2008, 100, 175501. [Google Scholar] [CrossRef]
- Hao, X.; Qiang, H.; Xiaohu, Y. Buckling of defective single-walled and double-walled carbon nanotubes under axial compression by molecular dynamics simulation. Compos. Sci. Technol. 2008, 68, 1809–1814. [Google Scholar] [CrossRef]
- Xin, H.; Han, Q.; Yao, X.-H. Buckling and axially compressive properties of perfect and defective single-walled carbon nanotubes. Carbon 2007, 45, 2486–2495. [Google Scholar] [CrossRef]
- Xin, H.; Han, Q. The Strain Rate Effect of Perfect and Defective Single-Walled Carbon Nanotubes Under Axial Compression. J. Comput. Theor. Nanosci. 2012, 9, 371–378. [Google Scholar] [CrossRef]
- Faria, B.; Silvestre, N. Mechanical properties of phenine nanotubes. Extreme Mech. Lett. 2022, 56, 101893. [Google Scholar] [CrossRef]
- Yu, H.T.; Yang, M.; Zhu, W.; Chang, T.; Jiang, J.W. Diameter-dependent polygonal cross section for holey phenine nanotubes. Nanotechnology 2019, 31, 085702. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mat. Sci. Eng. 2009, 18, 015012. [Google Scholar] [CrossRef]
- Stuart, S.J.; Tutein, A.B.; Harrison, J.A. A reactive potential for hydrocarbons with intermolecular interactions. J. Chem. Phys. 2000, 112, 6472–6486. [Google Scholar] [CrossRef] [Green Version]
- Zhan, H.; Zhang, G.; Tan, V.B.C.; Cheng, Y.; Bell, J.M.; Zhang, Y.-W.; Gu, Y. From brittle to ductile: A structure dependent ductility of diamond nanothread. Nanoscale 2016, 8, 11177–11184. [Google Scholar] [CrossRef] [Green Version]
- Zhao, H.; Min, K.; Aluru, N.R. Size and chirality dependent elastic properties of graphene nanoribbons under uniaxial tension. Nano Lett. 2009, 9, 3012–3015. [Google Scholar] [CrossRef]
- Carpenter, C.; Maroudas, D.; Ramasubramaniam, A. Mechanical properties of irradiated single-layer graphene. Appl. Phys. Lett. 2013, 103, 013102. [Google Scholar] [CrossRef]
- Zhou, K.; Wang, L.; Wang, R.; Wang, C.; Tang, C. One Dimensional Twisted Van der Waals Structures Constructed by Self-Assembling Graphene Nanoribbons on Carbon Nanotubes. Materials 2022, 15, 8220. [Google Scholar] [CrossRef]
- Jensen, B.D.; Wise, K.E.; Odegard, G.M. The effect of time step, thermostat, and strain rate on ReaxFF simulations of mechanical failure in diamond, graphene, and carbon nanotube. J. Comput. Chem. 2015, 36, 1587–1596. [Google Scholar] [CrossRef]
- Liu, X.; Pan, D.; Hong, Y.; Guo, W. Bending Poisson Effect in Two-Dimensional Crystals. Phys. Rev. Lett. 2014, 112, 205502. [Google Scholar] [CrossRef] [Green Version]
- Feliciano, J.; Tang, C.; Zhang, Y.; Chen, C. Aspect ratio dependent buckling mode transition in single-walled carbon nanotubes under compression. J. Appl. Phys. 2011, 109, 084323. [Google Scholar] [CrossRef] [Green Version]
- Zhan, H.; Zhang, G.; Bell, J.M.; Tan, V.B.C.; Gu, Y. High density mechanical energy storage with carbon nanothread bundle. Nat. Commun. 2020, 11, 1905. [Google Scholar] [CrossRef] [Green Version]

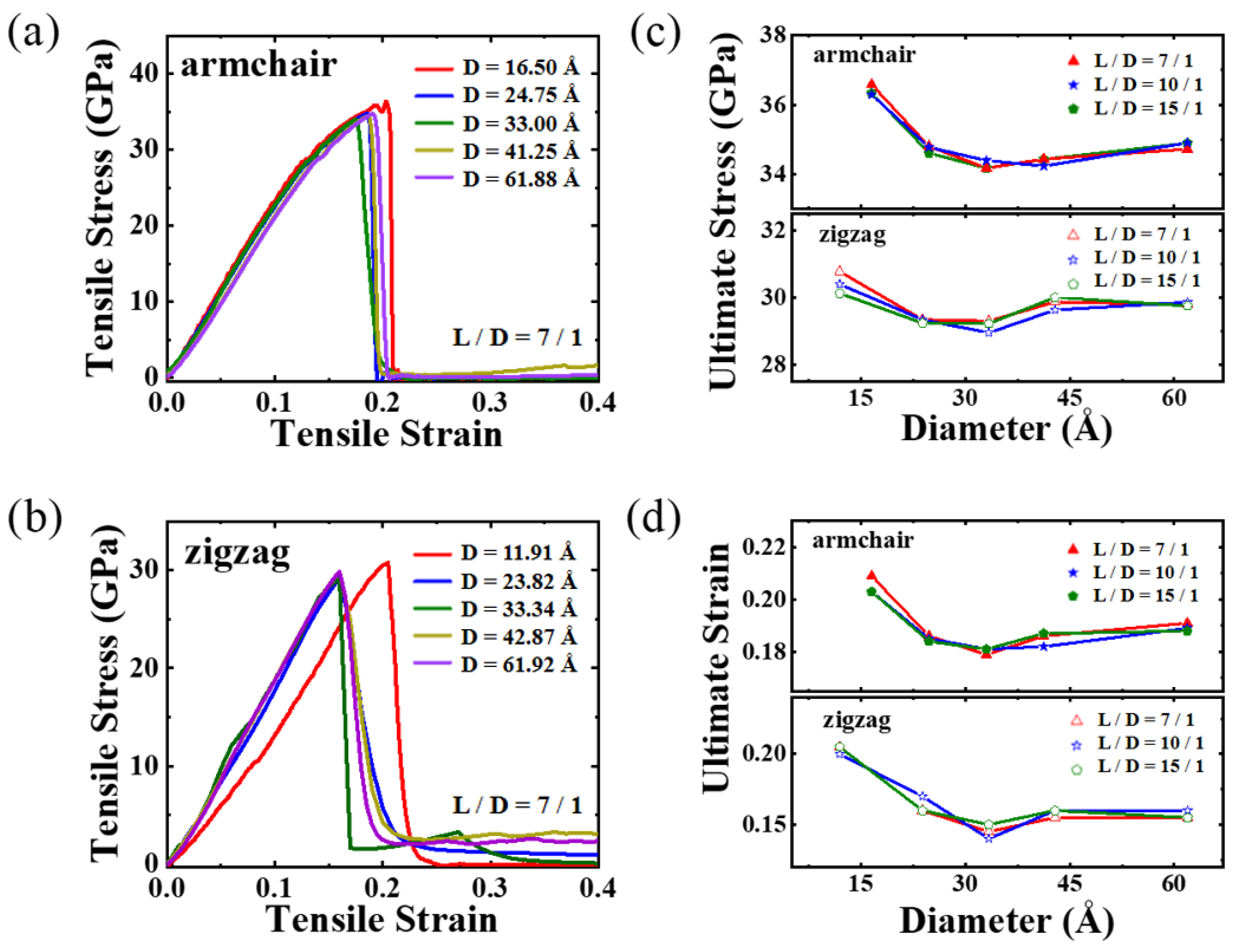

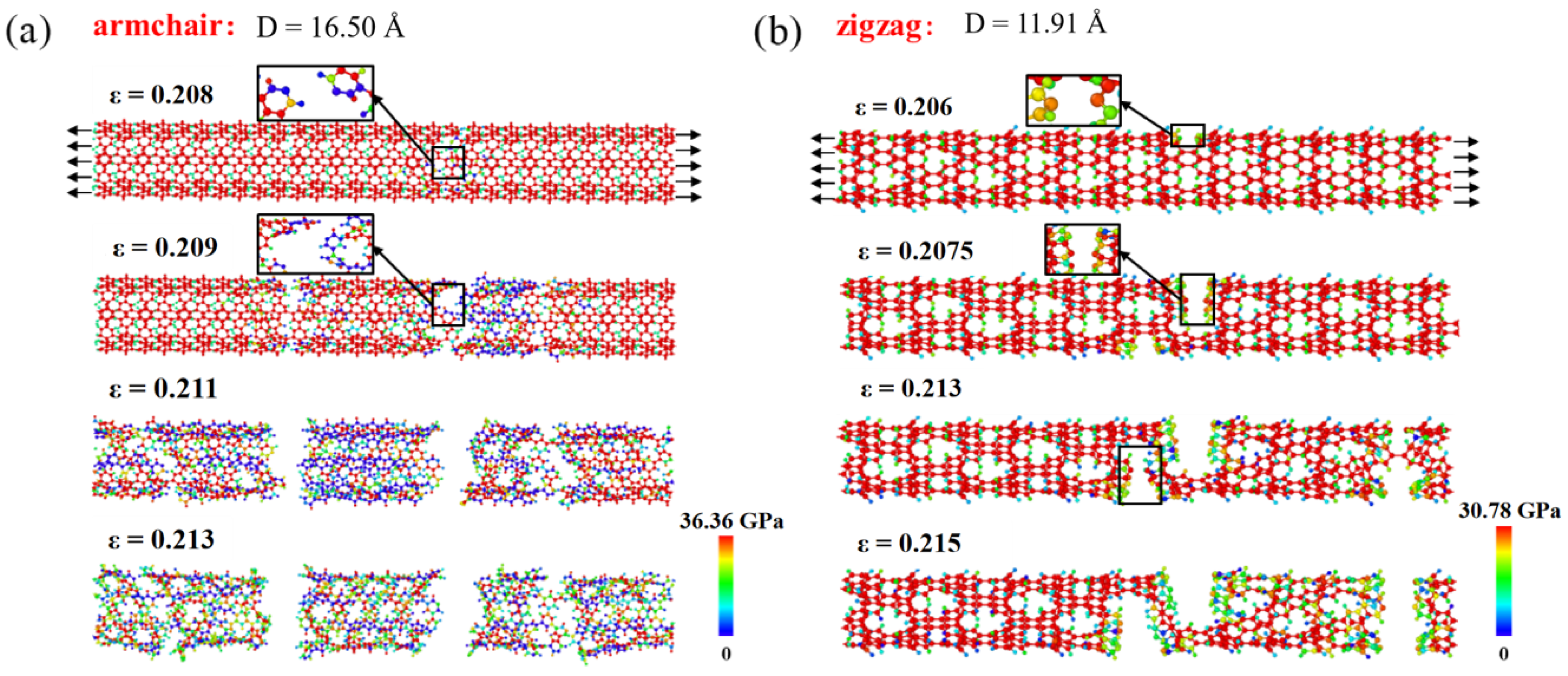
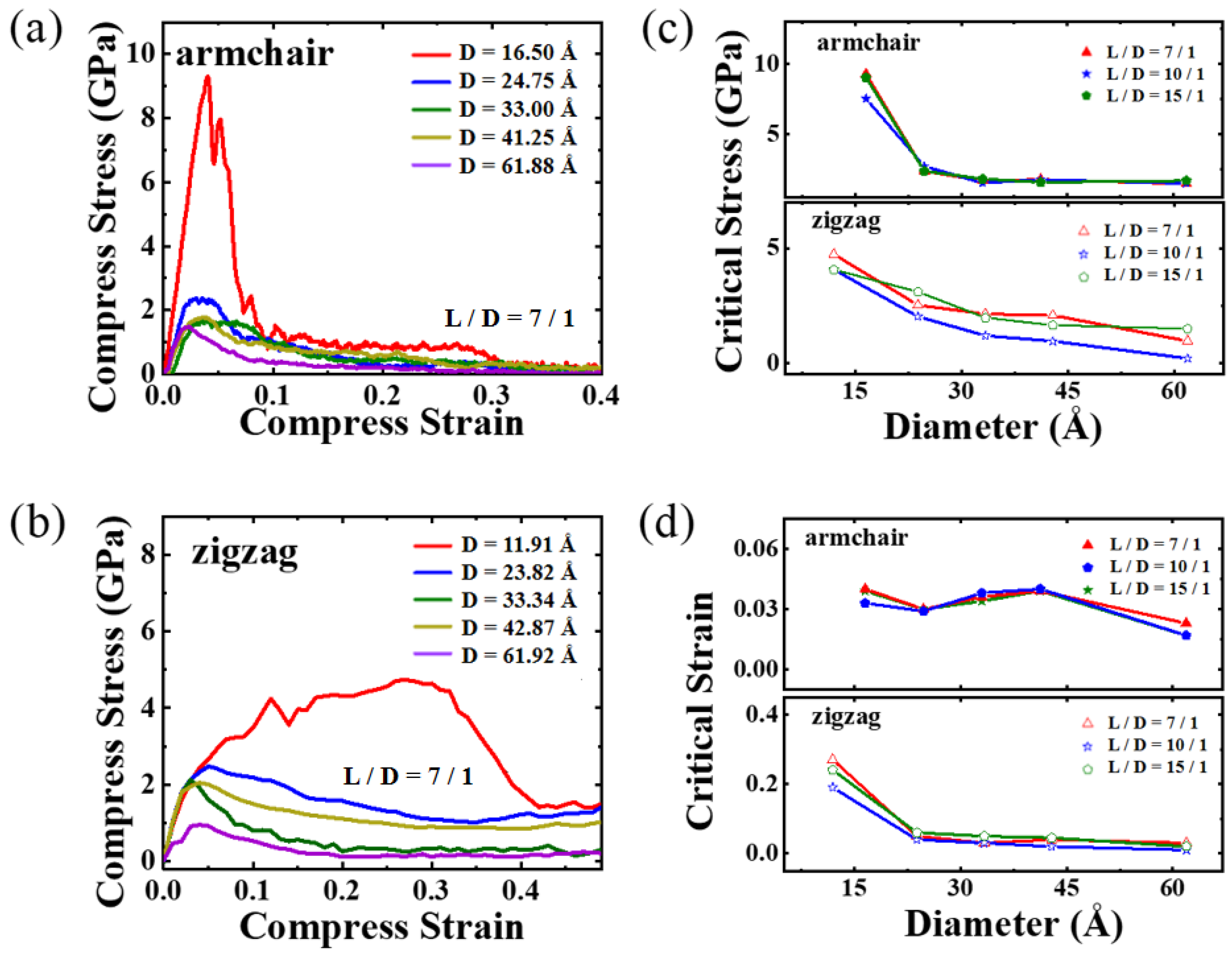

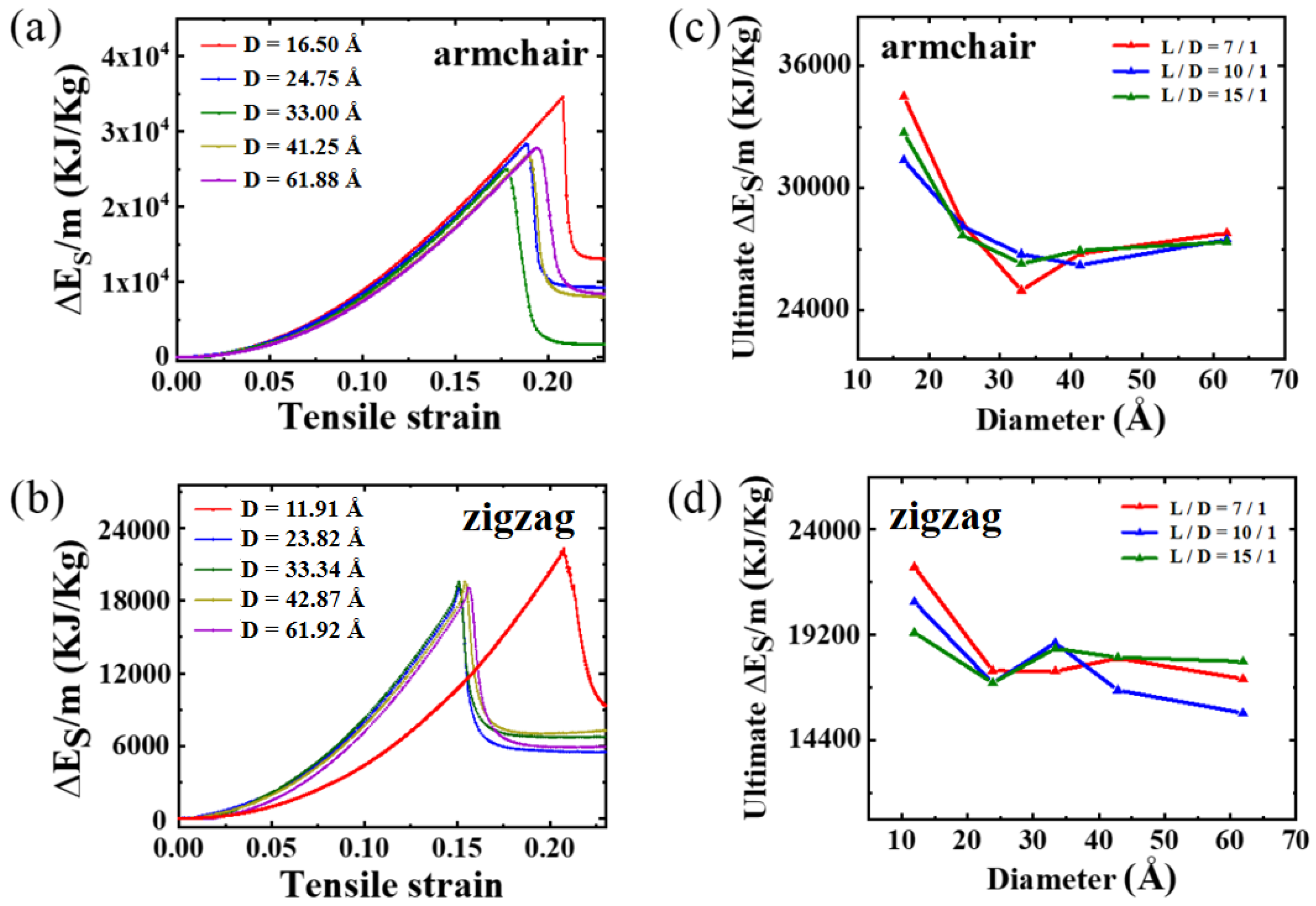
| Diameter | 16.50 Å | 24.75 Å | 33.00 Å | 41.25 Å | 61.88 Å | Unit |
|---|---|---|---|---|---|---|
| Ultimate stress under tension | 36.36 | 34.80 | 34.17 | 34.43 | 34.72 | GPa |
| Ultimate strain under tension | 0.209 | 0.186 | 0.179 | 0.186 | 0.191 | - |
| Young’s modulus | 239 | 219 | 210 | 199 | 197 | GPa |
| Energy storage density | 34,488 | 28,248 | 24,984 | 26,784 | 27,792 | KJ/Kg |
| Critical stress under compression | 9.30 | 2.38 | 1.69 | 1.78 | 1.48 | GPa |
| Critical strain under compression | 0.04 | 0.03 | 0.036 | 0.039 | 0.023 | - |
| Diameter | 11.91 Å | 23.82 Å | 33.34 Å | 42.87 Å | 61.92 Å | Unit |
|---|---|---|---|---|---|---|
| Ultimate stress under tension | 30.78 | 29.34 | 29.30 | 29.87 | 29.79 | GPa |
| Ultimate strain under tension | 0.205 | 0.16 | 0.145 | 0.155 | 0.155 | - |
| Young’s modulus | 114 | 160 | 173 | 166 | 159 | GPa |
| Energy storage density | 22,296 | 17,568 | 17,544 | 18,132 | 17,196 | KJ/Kg |
| Critical stress under compression | 4.74 | 2.52 | 2.13 | 2.07 | 0.95 | GPa |
| Critical strain under compression | 0.27 | 0.05 | 0.03 | 0.04 | 0.03 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Wang, R.; Wang, L.; Xia, J.; Wang, C.; Tang, C. Size- and Chirality-Dependent Structural and Mechanical Properties of Single-Walled Phenine Nanotubes. Materials 2023, 16, 4706. https://doi.org/10.3390/ma16134706
Liu Y, Wang R, Wang L, Xia J, Wang C, Tang C. Size- and Chirality-Dependent Structural and Mechanical Properties of Single-Walled Phenine Nanotubes. Materials. 2023; 16(13):4706. https://doi.org/10.3390/ma16134706
Chicago/Turabian StyleLiu, Yanjun, Ruijie Wang, Liya Wang, Jun Xia, Chengyuan Wang, and Chun Tang. 2023. "Size- and Chirality-Dependent Structural and Mechanical Properties of Single-Walled Phenine Nanotubes" Materials 16, no. 13: 4706. https://doi.org/10.3390/ma16134706






