Alloying Effect on Transformation Strain and Martensitic Transformation Temperature of Ti-Based Alloys from Ab Initio Calculations
Abstract
:1. Introduction
2. Methodology
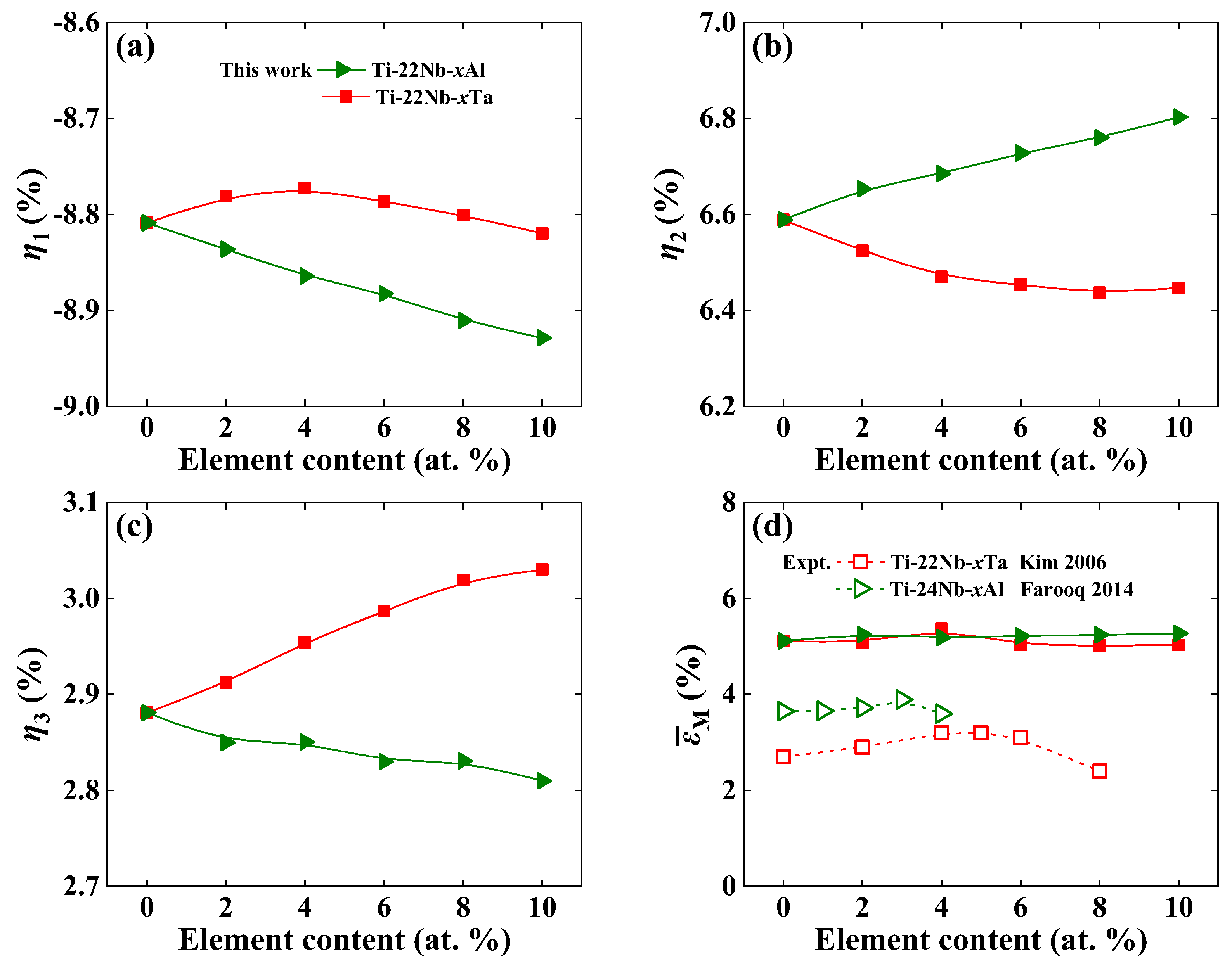
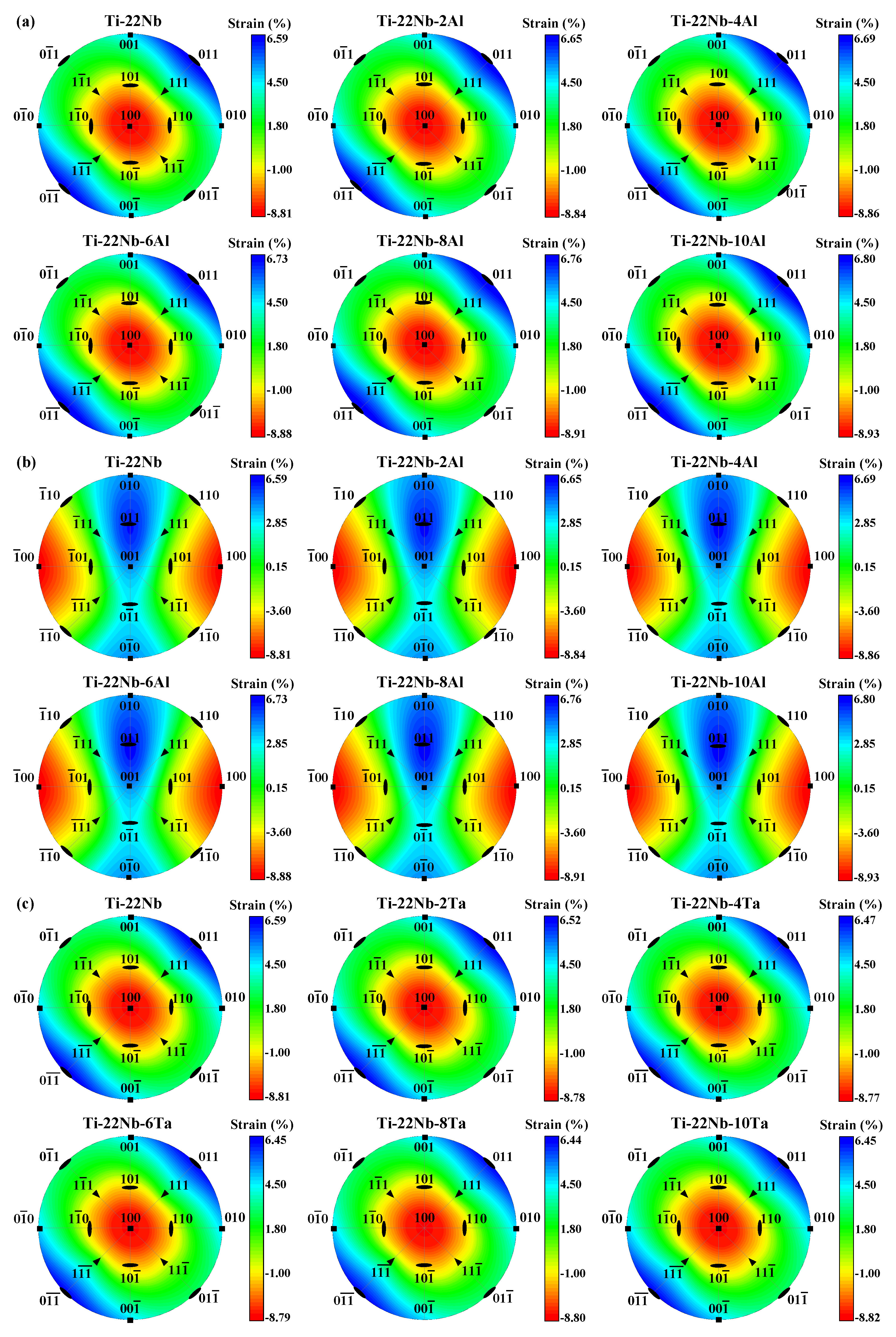
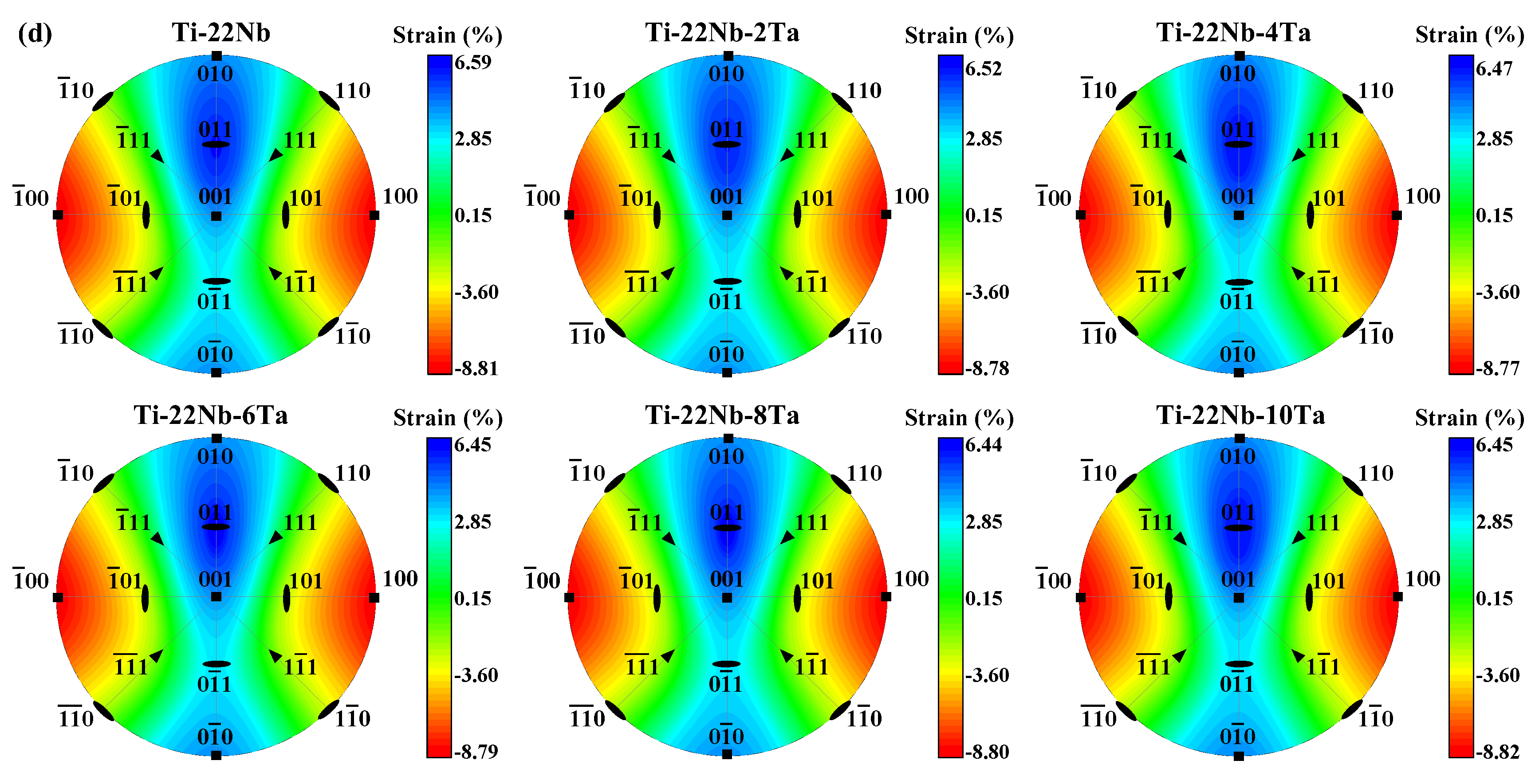
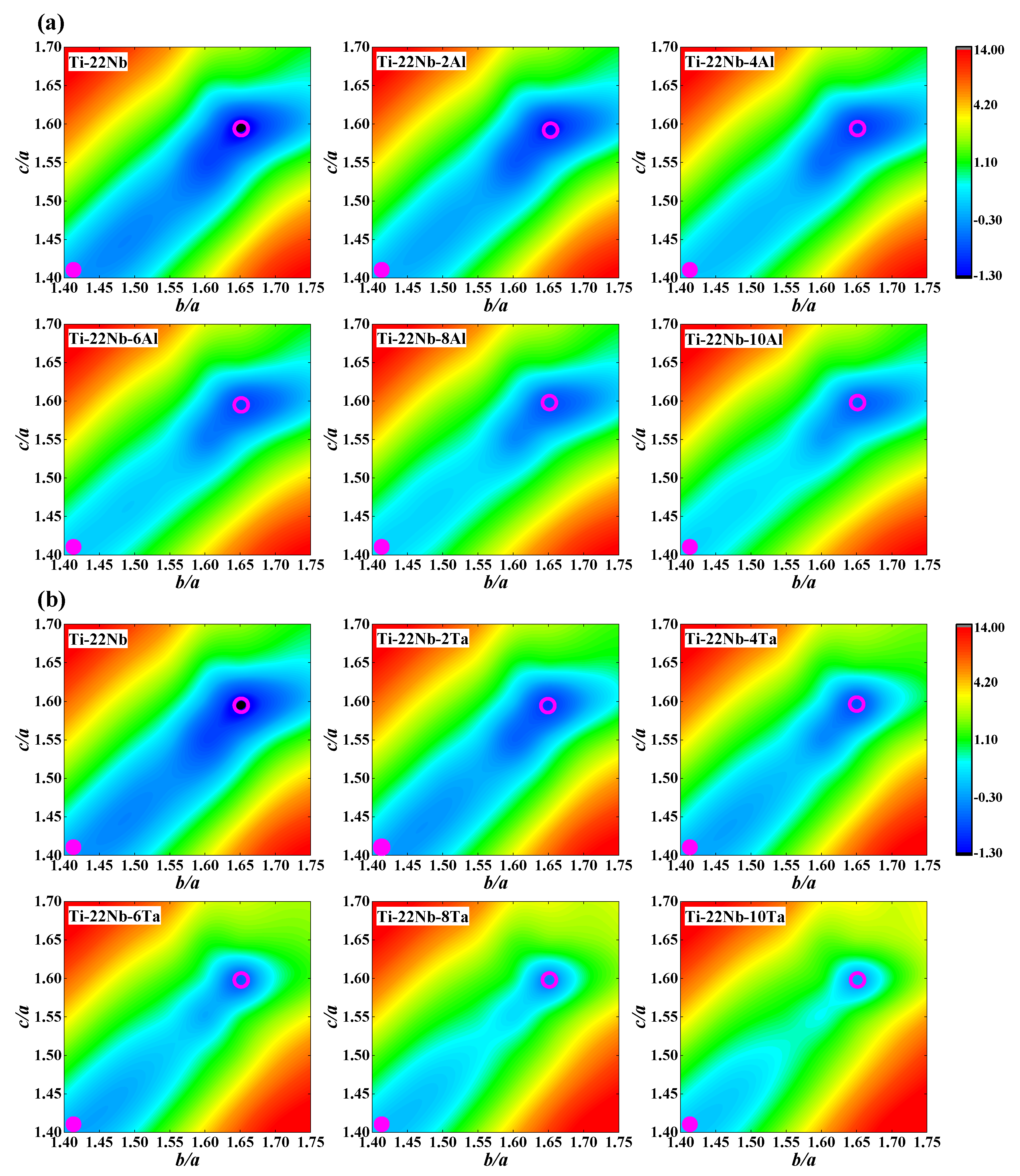
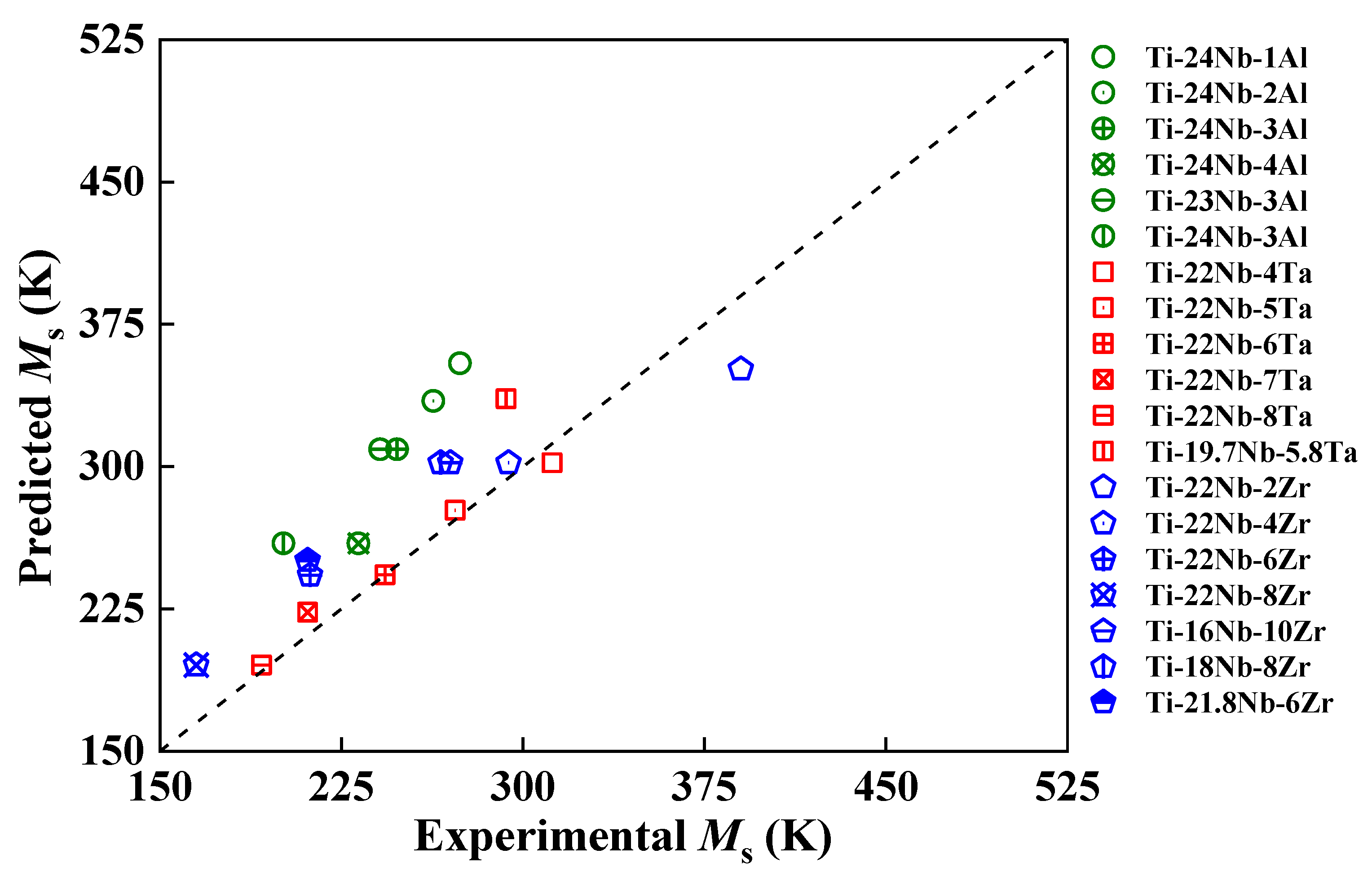
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jafari, J.; Zebarjad, S.; Sajjadi, S. Effect of pre-strain on microstructure of Ni–Ti orthodontic archwires. Mater. Sci. Eng. A 2008, 473, 42–48. [Google Scholar] [CrossRef]
- Dong, W.; Padilla, C.; Epstein, J.; Varadan, V.; Jiang, Y.; Cogbill, A.; Tian, Z.R. New bone implants and stents from nanowire scaffolds corroded on Ti or Ti/Ni alloys. Nanomed. Nanotechnol. Biol. Med. 2007, 3, 355. [Google Scholar] [CrossRef]
- Baker, C. The Shape-Memory Effect in a Titanium-35 wt.-% Niobium Alloy. Met. Sci. J. 1971, 5, 92–100. [Google Scholar] [CrossRef]
- Farooq, M.U.; Khalid, F.A.; Zaigham, H.; Abidi, I.H. Superelastic behaviour of Ti–Nb–Al ternary shape memory alloys for biomedical applications. Mater. Lett. 2014, 121, 58–61. [Google Scholar] [CrossRef]
- Fukui, Y.; Inamura, T.; Hosoda, H.; Wakashima, K.; Miyazaki, S. Mechanical Properties of a Ti-Nb-Al Shape Memory Alloy. Mater. Trans. 2004, 45, 1077–1082. [Google Scholar] [CrossRef]
- Inamura, T.; Kim, J.I.; Kim, H.Y.; Hosoda, H.; Wakashima, K.; Miyazaki, S. Composition dependent crystallography of α″-martensite in Ti–Nb-based β-titanium alloy. Philos. Mag. 2007, 87, 3325. [Google Scholar] [CrossRef]
- Kim, H.; Hashimoto, S.; Kim, J.; Inamura, T.; Hosoda, H.; Miyazaki, S. Effect of Ta addition on shape memory behavior of Ti–22Nb alloy. Mater. Sci. Eng. A 2006, 417, 120–128. [Google Scholar] [CrossRef]
- Brailovski, V.; Prokoshkin, S.; Gauthier, M.; Inaekyan, K.; Dubinskiy, S.; Petrzhik, M.; Filonov, M. Bulk and porous metastable beta Ti–Nb–Zr(Ta) alloys for biomedical applications. Mater. Sci. Eng. C 2011, 31, 643. [Google Scholar] [CrossRef]
- Kim, H.Y.; Fu, J.; Tobe, H.; Kim, J.I.; Miyazaki, S. Crystal Structure, Transformation Strain, and Superelastic Property of Ti-Nb-Zr and Ti-Nb-Ta Alloys. Shape Mem. Superelasticity 2015, 1, 107. [Google Scholar] [CrossRef]
- Hussein, A.H.; Gepreel, M.A.; Gouda, M.K.; Hefnawy, A.M.; Kandil, S.H. Biocompatibility of new Ti-Nb-Ta base alloys. Mater. Sci. Eng. C Mater. Biol. Appl. 2016, 61, 574. [Google Scholar] [CrossRef]
- Kim, H.Y.; Sasaki, T.; Okutsu, K.; Kim, J.I.; Inamura, T.; Hosoda, H.; Miyazaki, S. Texture and shape memory behavior of Ti-22Nb-6Ta alloy. Acta Mater. 2006, 54, 423. [Google Scholar] [CrossRef]
- Zhang, J.; Sun, F.; Hao, Y.; Gozdecki, N.; Lebrun, E.; Vermaut, P.; Portier, R.; Gloriant, T.; Laheurte, P.; Prima, F. Influence of equiatomic Zr/Nb substitution on superelastic behavior of Ti–Nb–Zr alloy. Mater. Sci. Eng. A 2013, 563, 78–85. [Google Scholar] [CrossRef]
- Inaekyan, K.; Brailovski, V.; Prokoshkin, S.; Pushin, V.; Dubinskiy, S.; Sheremetyev, V. Comparative study of structure formation and mechanical behavior of age-hardened Ti-Nb-Zr and Ti-Nb-Ta shape memory alloys. Mater. Charact. 2015, 103, 65. [Google Scholar] [CrossRef]
- Dubinskiy, S.; Markova, G.; Baranova, A.; Vvedenskiy, V.; Minkova, I.; Prokoshkin, S.; Brailovski, V. A non-typical Elinvar effect on cooling of a beta Ti-Nb-Zr alloy. Mater. Lett. 2022, 314, 131870. [Google Scholar] [CrossRef]
- Takahashi, E.; Sakurai, T.; Watanabe, S.; Masahashi, N.; Hanada, S. Effect of Heat Treatment and Sn Content on Superelasticity in Biocompatible TiNbSn Alloys. Mater. Trans. 2002, 43, 2978–2983. [Google Scholar] [CrossRef]
- Matsumoto, H.; Watanabe, S.; Hanada, S. Beta TiNbSn Alloys with Low Young’s Modulus and High Strength. Mater. Trans. 2005, 46, 1070–1078. [Google Scholar] [CrossRef]
- Ibrahim, M.K.; Hamzah, E.; Saud, S.N. Microstructure, Phase Transformation, Mechanical Behavior, Bio-corrosion and Antibacterial Properties of Ti-Nb-xSn (x = 0, 0.25, 0.5 and 1.5) SMAs. J. Mater. Eng. Perform. 2018, 28, 382. [Google Scholar] [CrossRef]
- Cai, S.; Wang, L.; Schaffer, J.; Gao, J.; Ren, Y. Influence of Sn on martensitic beta Ti alloys. Mater. Sci. Eng. A 2019, 743, 764–772. [Google Scholar] [CrossRef]
- Wang, J.; Li, Q.; Xiong, C.; Li, Y.; Sun, B. Effect of Zr on the martensitic transformation and the shape memory effect in Ti-Zr-Nb-Ta high-temperature shape memory alloys. J. Alloys Compd. 2018, 737, 672–677. [Google Scholar] [CrossRef]
- Li, Q.; Ma, X.; Xiong, C.; Qu, W.; Li, Y. Effects of annealing temperature on microstructures and shape memory effect of Ti-19Zr-11Nb-2Ta alloy sheets. J. Alloys Compd. 2022, 897, 162728. [Google Scholar] [CrossRef]
- Li, S.; Rehman, I.U.; Lim, J.-H.; Lee, W.-T.; Seol, J.B.; Kim, J.G.; Nam, T.-H. Effect of Sn content on microstructure, texture evolution, transformation behavior and superelastic properties of Ti−20Zr−9Nb−(2–5)Sn (at. %) shape memory alloys. Mater. Sci. Eng. A 2021, 827, 141994. [Google Scholar] [CrossRef]
- Li, S.; Lim, J.-H.; Rehman, I.U.; Lee, W.-T.; Kim, J.G.; Oh, J.S.; Lee, T.; Nam, T.-H. Tuning the texture characteristics and superelastic behaviors of Ti–Zr–Nb–Sn shape memory alloys by varying Nb content. Mater. Sci. Eng. A 2022, 845, 143243. [Google Scholar] [CrossRef]
- Hao, Y.L.; Li, S.J.; Sun, S.Y.; Yang, R. Effect of Zr and Sn on Young’s modulus and superelasticity of Ti-Nb-based alloys. Mater. Sci. Eng. A 2006, 441, 112. [Google Scholar] [CrossRef]
- Marupalli, B.C.; Adhikary, T.; Sahu, B.; Mitra, R.; Aich, S. Effect of annealing temperature on microstructure and mechanical response of sputter deposited Ti-Zr-Mo high temperature shape memory alloy thin films. Appl. Surf. Sci. Adv. 2021, 6, 100137. [Google Scholar] [CrossRef]
- Kim, Y.-W. Specific mechanical properties of highly porous Ti-Zr-Mo-Sn shape memory alloy for biomedical applications. Scr. Mater. 2023, 231, 115433. [Google Scholar] [CrossRef]
- Buenconsejo, P.J.S.; Kim, H.Y.; Miyazaki, S. Novel β-TiTaAl alloys with excellent cold workability and a stable high-temperature shape memory effect. Scr. Mater. 2011, 64, 1114. [Google Scholar] [CrossRef]
- Ferrari, A.; Paulsen, A.; Frenzel, J.; Rogal, J.; Eggeler, G.; Drautz, R. Unusual composition dependence of transformation temperatures in Ti-Ta-X shape memory alloys. Phys. Rev. Mater. 2018, 2, 073609. [Google Scholar] [CrossRef]
- Kim, H.Y.; Fukushima, T.; Buenconsejo, P.J.S.; Nam, T.-H.; Miyazaki, S. Martensitic transformation and shape memory properties of Ti–Ta–Sn high temperature shape memory alloys. Mater. Sci. Eng. A 2011, 528, 7238–7246. [Google Scholar] [CrossRef]
- Li, J.; Yi, X.; Wang, H.; Sun, K.; Liu, J.; Meng, X.; Gao, Z. Effect of thermo-mechanical treatment on Ti–Ta-Hf high temperature shape memory alloy. Prog. Nat. Sci. 2021, 31, 779–782. [Google Scholar] [CrossRef]
- Yi, X.; Sun, K.; Liu, J.; Zheng, X.; Meng, X.; Gao, Z.; Cai, W. Tailoring the microstructure, martensitic transformation and strain recovery characteristics of Ti-Ta shape memory alloys by changing Hf content. J. Mater. Sci. Technol. 2021, 83, 123–130. [Google Scholar] [CrossRef]
- Chiu, W.; Wakabayashi, K.; Umise, A.; Tahara, M.; Inamura, T.; Hosoda, H. Enhancement of the shape memory effect by the introductions of Cr and Sn into the β–Ti alloy towards the biomedical applications. J. Alloys Compd. 2021, 875, 160088. [Google Scholar] [CrossRef]
- Park, M.S.; Chiu, W.-T.; Nohira, N.; Tahara, M.; Hosoda, H. Effects of Cr and Sn additives on the martensitic transformation and deformation behavior of Ti-Cr-Sn biomedical shape memory alloys. Mater. Sci. Eng. A 2021, 822, 141688. [Google Scholar] [CrossRef]
- Kilmametov, A.; Ivanisenko, Y.; Straumal, B.; Mazilkin, A.A.; Gornakova, A.S.; Kriegel, M.J.; Fabrichnaya, O.B.; Rafaja, D.; Hahn, H. Transformations of α’ martensite in Ti–Fe alloys under high pressure torsion. Scr. Mater. 2017, 136, 46. [Google Scholar] [CrossRef]
- Kriegel, M.J.; Kilmametov, A.; Klemm, V.; Schimpf, C.; Straumal, B.B.; Gornakova, A.S.; Ivanisenko, Y.; Fabrichnaya, O.; Hahn, H.; Rafaja, D. Thermal Stability of Athermal ω-Ti(Fe) Produced upon Quenching of β-Ti(Fe). Adv. Eng. Mater. 2019, 21, 1800158. [Google Scholar] [CrossRef]
- Kilmametov, A.R.; Ivanisenko, Y.; Mazilkin, A.A.; Straumal, B.B.; Gornakova, A.S.; Fabrichnaya, O.B.; Kriegel, M.J.; Rafaja, D.; Hahn, H. The α→ω and β→ω phase transformations in Ti–Fe alloys under high-pressure torsion. Acta Mater. 2018, 144, 337. [Google Scholar] [CrossRef]
- Li, M.; Min, X.; Yao, K.; Ye, F. Novel insight into the formation of α″-martensite and ω-phase with cluster structure in metastable Ti-Mo alloys. Acta Mater. 2019, 164, 322. [Google Scholar] [CrossRef]
- Kilmametov, A.; Gornakova, A.; Karpov, M.; Afonikova, N.; Korneva, A.; Zięba, P.; Baretzky, B.; Straumal, B. Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys. Processes 2020, 8, 1135. [Google Scholar] [CrossRef]
- Kilmametov, A.R.; Ivanisenko, Y.; Straumal, B.B.; Gornakova, A.S.; Mazilkin, A.A.; Hahn, H. The α → ω Transformation in Titanium-Cobalt Alloys under High-Pressure Torsion. Metals 2018, 8, 1. [Google Scholar] [CrossRef]
- Korneva, A.; Straumal, B.; Kilmametov, A.; Gondek, L.; Wierzbicka-Miernik, A.; Litynska-Dobrzynska, L.; Cios, G.; Chulist, R.; Zieba, P. Thermal stability and microhardness of metastable omega-phase in the Ti-3.3 at. % Co alloy subjected to high pressure torsion. J. Alloys Compd. 2020, 834, 155132. [Google Scholar] [CrossRef]
- Kriegel, M.J.; Rudolph, M.; Kilmametov, A.; Straumal, B.B.; Ivanisenko, J.; Fabrichnaya, O.; Hahn, H.; Rafaja, D. Formation and Thermal Stability of ω-Ti(Fe) in α-Phase-Based Ti(Fe) Alloys. Metals 2020, 10, 402. [Google Scholar] [CrossRef]
- Kim, H.Y.; Ikehara, Y.; Kim, J.I.; Hosoda, H.; Miyazaki, S. Martensitic transformation, shape memory effect and superelasticity of Ti-Nb binary alloys. Acta Mater. 2006, 54, 2419. [Google Scholar] [CrossRef]
- Brown, A.R.G.; Clark, D.; Eastabbook, J.; Jepson, K.S.; System, T.T.-N. The Titanium-Niobium System. Nature 1964, 201, 914. [Google Scholar] [CrossRef]
- Kim, H.Y.; Hashimoto, S.; Kim, J.I.; Hosoda, H.; Miyazaki, S. Mechanical Properties and Shape Memory Behavior of Ti-Nb Alloys. Mater. Trans. 2004, 45, 2443–2448. [Google Scholar] [CrossRef]
- Kim, J.I.; Kim, H.Y.; Inamura, T.; Hosoda, H.; Miyazaki, S. Shape memory characteristics of Ti-22Nb-(2-8)Zr(at.%) biomedical alloys. Mater. Sci. Eng. A 2005, 403, 334. [Google Scholar] [CrossRef]
- Neelakantan, S.; Rivera-Díaz-Del-Castillo, P.; van der Zwaag, S. Prediction of the martensite start temperature for β titanium alloys as a function of composition. Scr. Mater. 2009, 60, 611–614. [Google Scholar] [CrossRef]
- Prabha, A.J.; Raju, S.; Jeyaganesh, B.; Rai, A.K.; Behera, M.; Vijayalakshmi, M.; Paneerselvam, G.; Johnson, I. Thermodynamics of α’’→β phase transformation and heat capacity measurements in Ti-15at% Nb alloy. Phys. B Condens. Matter 2011, 406, 4200. [Google Scholar] [CrossRef]
- Minami, D.; Uesugi, T.; Takigawa, Y.; Higashi, K. First-principles study of transformation strains and phase stabilities in α″ and β Ti-Nb-X alloys. J. Alloys Compd. 2017, 716, 37. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, H.L.; Wang, D.; Sun, Q.Y.; Zhao, S.S.; Lu, S.; Li, W.; Vitos, L.; Ding, X.D. Large recoverable strain with suitable transition temperature in TiNb-based multicomponent shape memory alloys: First-principles calculations. Acta Mater. 2021, 221, 117366. [Google Scholar] [CrossRef]
- Hu, Q.; Luo, H.; Li, C.; Vitos, L.; Yang, R. Composition dependent elastic modulus and phase stability of Ni2MnGa based ferromagnetic shape memory alloys. Sci. China Technol. Sci. 2011, 55, 295–305. [Google Scholar] [CrossRef]
- Lu, J.-M.; Hu, Q.-M.; Yang, R. Composition-dependent elastic properties and electronic structures of off-stoichiometric TiNi from first-principles calculations. Acta Mater. 2008, 56, 4913–4920. [Google Scholar] [CrossRef]
- Miyazaki, S.; Imai, T.; Igo, Y.; Otsuka, K. Effect of cyclic deformation on the pseudoelasticity characteristics of Ti-Ni alloys. Met. Trans. A 1986, 17, 115–120. [Google Scholar] [CrossRef]
- Kim, K.M.; Kim, H.Y.; Miyazaki, S. Effect of Zr Content on Phase Stability, Deformation Behavior, and Young’s Modulus in Ti–Nb–Zr Alloys. Materials 2020, 13, 476. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.Y.; Miyazaki, S. Several Issues in the Development of Ti–Nb-Based Shape Memory Alloys. Shape Mem. Superelasticity 2016, 2, 380–390. [Google Scholar] [CrossRef]
- Tada, H.; Yamamoto, T.; Wang, X.; Kato, H. Effect of Al Addition on Superelastic Properties of Aged Ti-Nb-Zr-Al Quaternary Alloys. Mater. Trans. 2012, 53, 1981. [Google Scholar] [CrossRef]
- Vitos, L. Computational Quantum Mechanics for Materials Engineers: The EMTO Method and Applications; Springer Science & Business Media: London, UK, 2007. [Google Scholar]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Korzhavyi, P.A.; Ruban, A.V.; Abrikosov, I.A.; Skriver, H.L. Madelung energy for random metallic alloys in the coherent potential approximation. Phys. Rev. B 1995, 51, 5773–5780. [Google Scholar] [CrossRef] [PubMed]
- Li, C.X.; Luo, H.B.; Hu, Q.M.; Yang, R.; Yin, F.X.; Umezawa, O.; Vitos, L. Lattice parameters and relative stability of α″ phase in binary titanium alloys from first-principles calculations. Solid State Commun. 2013, 159, 70–75. [Google Scholar] [CrossRef]
- Born, M.; Huang, K. Dynamical Theory of Crystal Lattices; Oxford University Press: Oxford, UK, 1956. [Google Scholar]
- Hill, T.R. The elastic behavior of crystalline aggregate. Proc. Phys. Soc. Sect. A 1952, 65, 337. [Google Scholar] [CrossRef]
- Zhang, H.L.; Johansson, B.; Vitos, L. Ab initio calculations of elastic properties of bcc Fe-Mg and Fe-Cr random alloys. Phys. Rev. B 2009, 79, 224201. [Google Scholar] [CrossRef]
- Zhang, H.; Punkkinen, M.P.J.; Johansson, B.; Hertzman, S.; Vitos, L. Single-crystal elastic constants of ferromagnetic bcc Fe-based random alloys from first-principles theory. Phys. Rev. B 2010, 81, 184105. [Google Scholar] [CrossRef]
- Zhang, H.L.; Sun, X.; Lu, S.; Dong, Z.H.; Ding, X.D.; Wang, Y.Z.; Vitos, L. Elastic properties of AlxCrMnFeCoNi (0 ≤ x ≤ 5) high-entropy alloys from ab initio theory. Acta Mater. 2018, 155, 12. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, H.; Lu, S.; Ding, X.; Wang, Y.; Vitos, L. Phase selection rule for Al-doped CrMnFeCoNi high-entropy alloys from first-principles. Acta Mater. 2017, 140, 366–374. [Google Scholar] [CrossRef]
- Hu, Q.-M.; Li, S.-J.; Hao, Y.-L.; Yang, R.; Johansson, B.; Vitos, L. Phase stability and elastic modulus of Ti alloys containing Nb, Zr, and/or Sn from first-principles calculations. Appl. Phys. Lett. 2008, 93, 121902. [Google Scholar] [CrossRef]
- Miyazaki, S.; Kim, H.; Hosoda, H. Development and characterization of Ni-free Ti-base shape memory and superelastic alloys. Mater. Sci. Eng. A 2006, 438–440, 18–24. [Google Scholar] [CrossRef]
- Ojha, A.; Sehitoglu, H. Slip Resistance of Ti-Based High-Temperature Shape Memory Alloys. Shape Mem. Superelasticity 2016, 2, 50–61. [Google Scholar] [CrossRef]
- Bönisch, M.; Waitz, T.; Calin, M.; Skrotzki, W.; Eckert, J. Tailoring the Bain strain of martensitic transformations in Ti Nb alloys by controlling the Nb content. Int. J. Plast. 2016, 85, 190–202. [Google Scholar] [CrossRef]
- Cui, Y.; Li, Y.; Luo, K.; Xu, H.B. Microstructure and shape memory effect of Ti-20Zr-10Nb alloy. Mater. Sci. Eng. A 2010, 527, 652. [Google Scholar] [CrossRef]
- Zhang, F.; Yu, Z.; Xiong, C.; Qu, W.; Yuan, B.; Wang, Z.; Li, Y. Martensitic transformations and the shape memory effect in Ti-Zr-Nb-Al high-temperature shape memory alloys. Mater. Sci. Eng. A 2017, 679, 14–19. [Google Scholar] [CrossRef]
- Yao, T.T.; Zhang, Y.G.; Yang, L.; Bu, Z.Q.; Li, J.F. A metastable Ti-Zr-Nb-Al multi-principal-element alloy with high tensile strength and ductility. Mater. Sci. Eng. A 2022, 851, 143646. [Google Scholar] [CrossRef]
- Bönisch, M.; Calin, M.; Waitz, T.; Panigrahi, A.; Zehetbauer, M.; Gebert, A.; Skrotzki, W.; Eckert, J. Thermal stability and phase transformations of martensitic Ti–Nb alloys. Sci. Technol. Adv. Mater. 2013, 14, 055004. [Google Scholar] [CrossRef] [PubMed]
- Dobromyslov, A.; Elkin, V. Martensitic transformation and metastable β-phase in binary titanium alloys with d-metals of 4–6 periods. Scr. Mater. 2001, 44, 905–910. [Google Scholar] [CrossRef]
- Moffat, D.L.; Larbalestier, D.C. The competition between martensite and omega in quenched Ti-Nb alloys. Metall. Trans. A 1987, 19A, 1677. [Google Scholar]
- Buenconsejo, P.J.S.; Kim, H.Y.; Hosoda, H.; Miyazaki, S. Shape memory behavior of Ti–Ta and its potential as a high-temperature shape memory alloy. Acta Mater. 2009, 57, 1068–1077. [Google Scholar] [CrossRef]
- Ren, X.; Otsuka, K. Why Does the Martensitic Transformation Temperature Strongly Depend on Composition? Mater. Sci. Forum 2000, 327–328, 429. [Google Scholar] [CrossRef]
- Hu, Q.M.; Yang, R.; Lu, J.M.; Wang, L.; Johansson, B.; Vitos, L. Effect of Zr on the properties of (TiZr)Ni alloys from first-principles calculations. Phys. Rev. B 2007, 76, 224201. [Google Scholar] [CrossRef]
- Ren, X.; Miura, N.; Zhang, J.; Otsuka, K.; Tanaka, K.; Koiwa, M.; Suzuki, T.; Chumlyakov, Y.I.; Asai, M. A comparative study of elastic constants of Ti–Ni-based alloys prior to martensitic transformation. Mater. Sci. Eng. A 2001, 312, 196–206. [Google Scholar] [CrossRef]
- Xiong, B.; Zhao, X.; Hu, Y.; Huang, H.; Liu, Y.; Su, Y. Machine learning assisted empirical formula augmentation. Mater. Des. 2021, 210, 110037. [Google Scholar] [CrossRef]
- Luo, H.; Li, C.; Hu, Q.; Yang, R.; Johansson, B.; Vitos, L. Theoretical investigation of the effects of composition and atomic disordering on the properties of Ni2Mn(Al1−xGax) alloy. Acta Mater. 2011, 59, 971–980. [Google Scholar] [CrossRef]
- Cao, P.; Tian, F.; Li, W.; Vitos, L.; Wang, Y. Ideal superelasticity in Ni-based Heusler alloys. Acta Mater. 2021, 210, 116816. [Google Scholar] [CrossRef]
- Ghosh, S.; Ghosh, S. Understanding the origin of the magnetocaloric effects in substitutional Ni-Mn-Sb-Z (Z=Fe, Co, Cu) compounds: Insights from first-principles calculations. Phys. Rev. B 2020, 101, 024109. [Google Scholar] [CrossRef]
- Liang, Q.; Wang, D.; Zheng, Y.; Zhao, S.; Gao, Y.; Hao, Y.; Yang, R.; Banerjee, D.; Fraser, H.L.; Wang, Y. Shuffle-nanodomain regulated strain glass transition in Ti−24Nb−4Zr−8Sn alloy. Acta Mater. 2020, 186, 415. [Google Scholar] [CrossRef]
- Abdel-Hady, M.; Fuwa, H.; Hinoshita, K.; Kimura, H.; Shinzato, Y.; Morinaga, M. Phase stability change with Zr content in β-type Ti–Nb alloys. Scr. Mater. 2007, 57, 1000. [Google Scholar] [CrossRef]

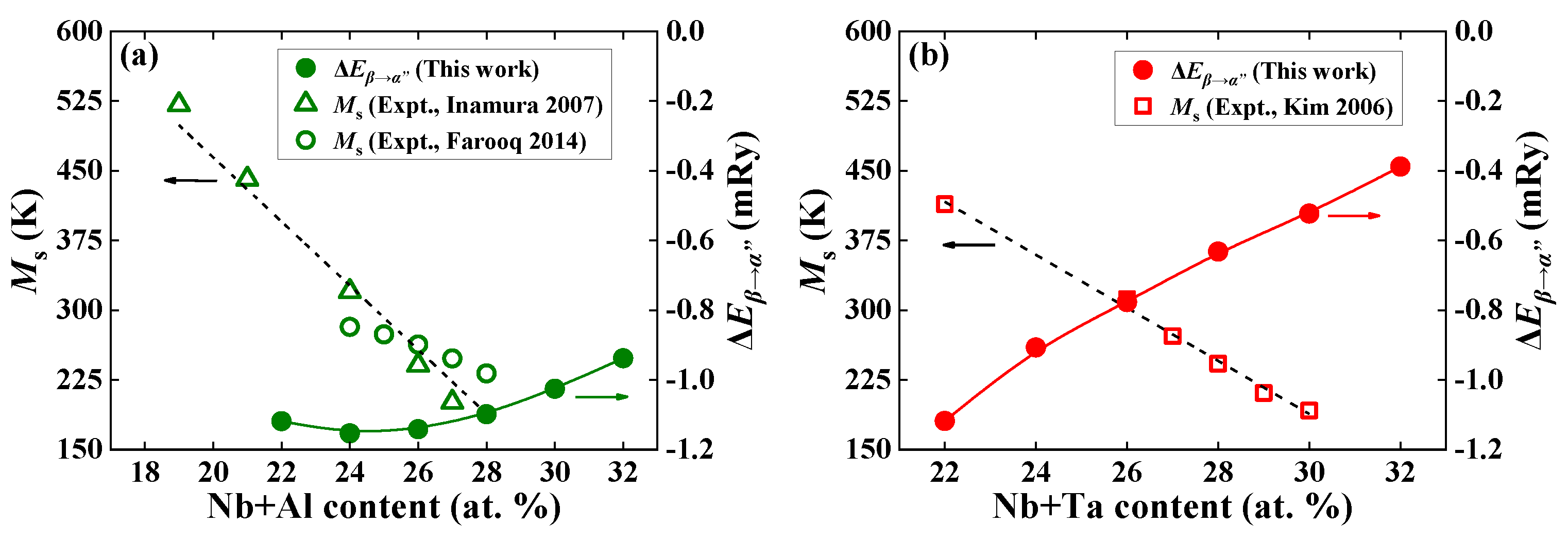

| (a) Ti−Nb−Al | ΔEβ→α″ | [48] | ||
| Ti−22Nb−2Al | −1.153 | 354.8 | 625.4 | - |
| Ti−22Nb−4Al | −1.141 | 335.2 | 623.2 | - |
| Ti−22Nb−6Al | −1.098 | 260.3 | 615.2 | - |
| Ti−22Nb−8Al | −1.025 | 116.9 | 601.1 | - |
| Ti−22Nb−10Al | −0.938 | −85.5 | 583.5 | - |
| Ti−23Nb−3Al | −1.141 | 335.2 | 623.2 | 241 [6] |
| Ti−24Nb−3Al | −1.126 | 309.1 | 620.4 | 201 [6] |
| Ti−24Nb-1Al | −1.147 | 344.5 | 624.3 | 274 [4] |
| Ti−24Nb−2Al | −1.141 | 335.2 | 623.2 | 263 [4] |
| Ti−24Nb−3Al | −1.126 | 309.1 | 620.4 | 248 [4] |
| Ti−24Nb−4Al | −1.098 | 260.3 | 615.1 | 232 [4] |
| (b) Ti−Nb−Ta | ΔEβ→α″ | [48] | ||
| Ti−22Nb−2Ta | −0.907 | 351.4 | 577.1 | - |
| Ti−22Nb−4Ta | −0.777 | 302.0 | 548.9 | 312 [7] |
| Ti−22Nb−5Ta | −0.714 | 276.9 | 534.5 | 272 [7] |
| Ti−22Nb−6Ta | −0.632 | 242.9 | 514.6 | 243 [7] |
| Ti−22Nb−7Ta | −0.586 | 223.2 | 503.2 | 211 [7] |
| Ti−22Nb−8Ta | −0.523 | 195.5 | 486.7 | 192 [7] |
| Ti−22Nb−10Ta | −0.388 | 132.6 | 448.1 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, H.; Xu, X.; Zhang, H.; Sun, Q.; Sun, J. Alloying Effect on Transformation Strain and Martensitic Transformation Temperature of Ti-Based Alloys from Ab Initio Calculations. Materials 2023, 16, 6069. https://doi.org/10.3390/ma16176069
Fang H, Xu X, Zhang H, Sun Q, Sun J. Alloying Effect on Transformation Strain and Martensitic Transformation Temperature of Ti-Based Alloys from Ab Initio Calculations. Materials. 2023; 16(17):6069. https://doi.org/10.3390/ma16176069
Chicago/Turabian StyleFang, Honglin, Xingge Xu, Hualei Zhang, Qiaoyan Sun, and Jun Sun. 2023. "Alloying Effect on Transformation Strain and Martensitic Transformation Temperature of Ti-Based Alloys from Ab Initio Calculations" Materials 16, no. 17: 6069. https://doi.org/10.3390/ma16176069
APA StyleFang, H., Xu, X., Zhang, H., Sun, Q., & Sun, J. (2023). Alloying Effect on Transformation Strain and Martensitic Transformation Temperature of Ti-Based Alloys from Ab Initio Calculations. Materials, 16(17), 6069. https://doi.org/10.3390/ma16176069






