Experimental Analysis of Channel Steel Member under Tension Load with Damage in the Unconnected Legs
Abstract
:1. Introduction
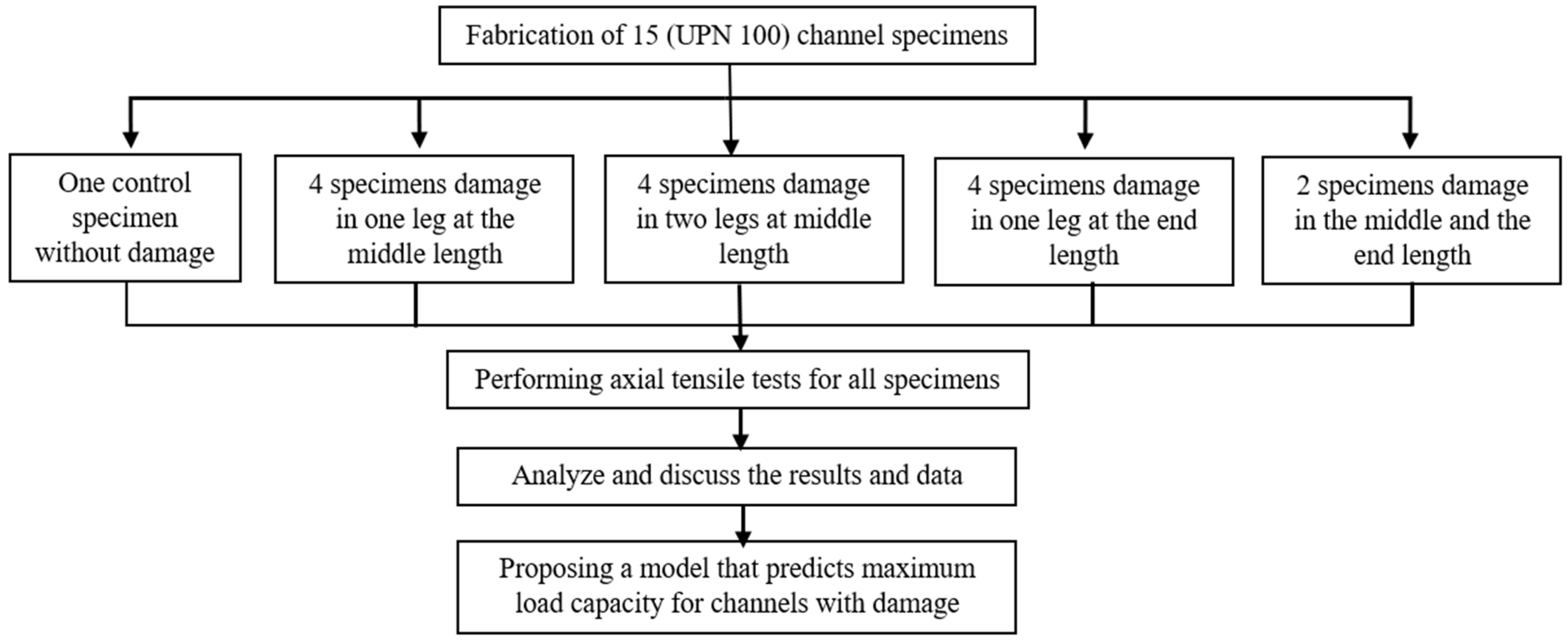
2. Experimental Tests
2.1. Specimen Details
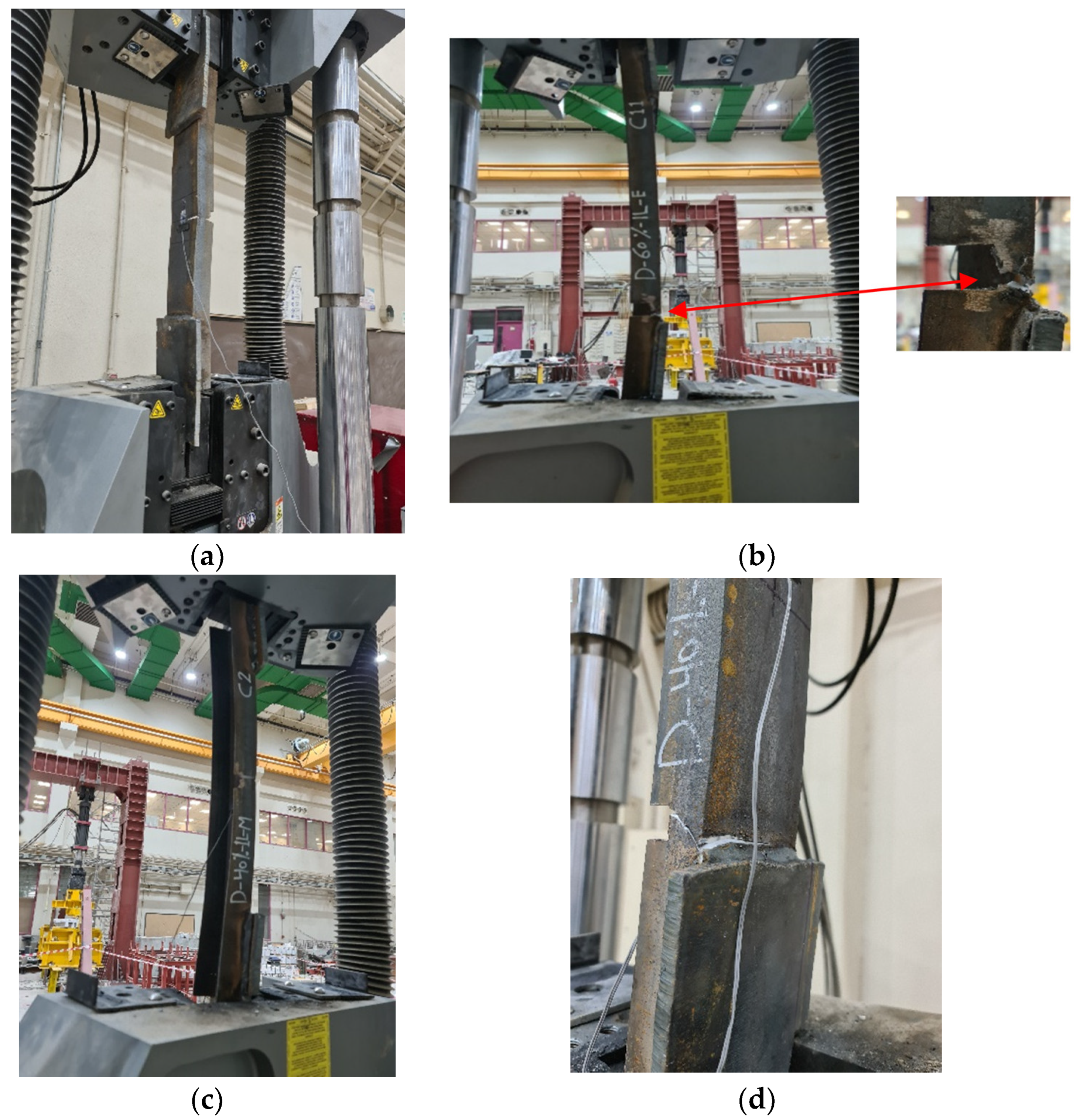
2.2. Instrumentation and Test Setup
2.3. Determining the Properties of the Steel Used
3. Experimental Results and Discussion
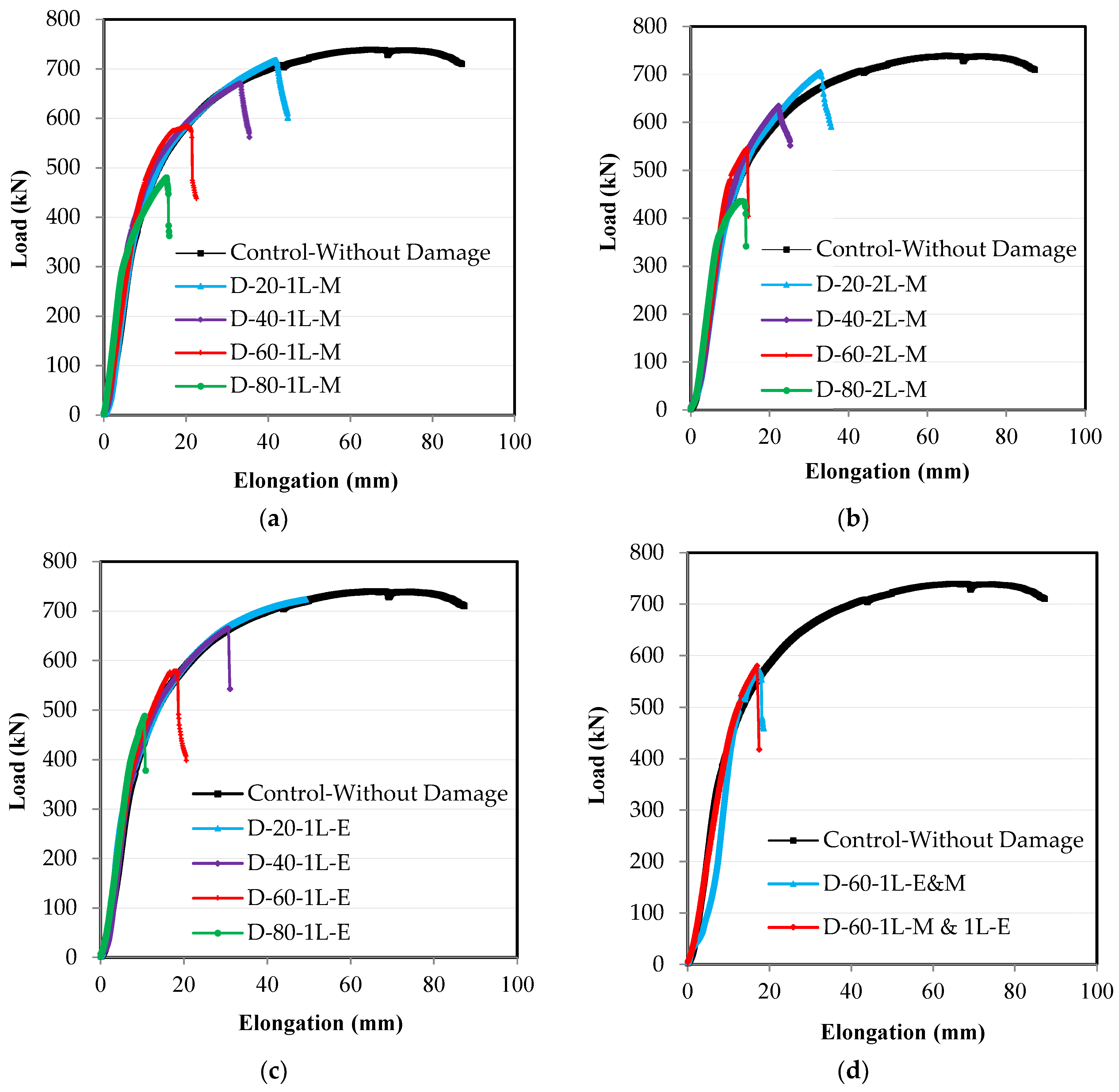
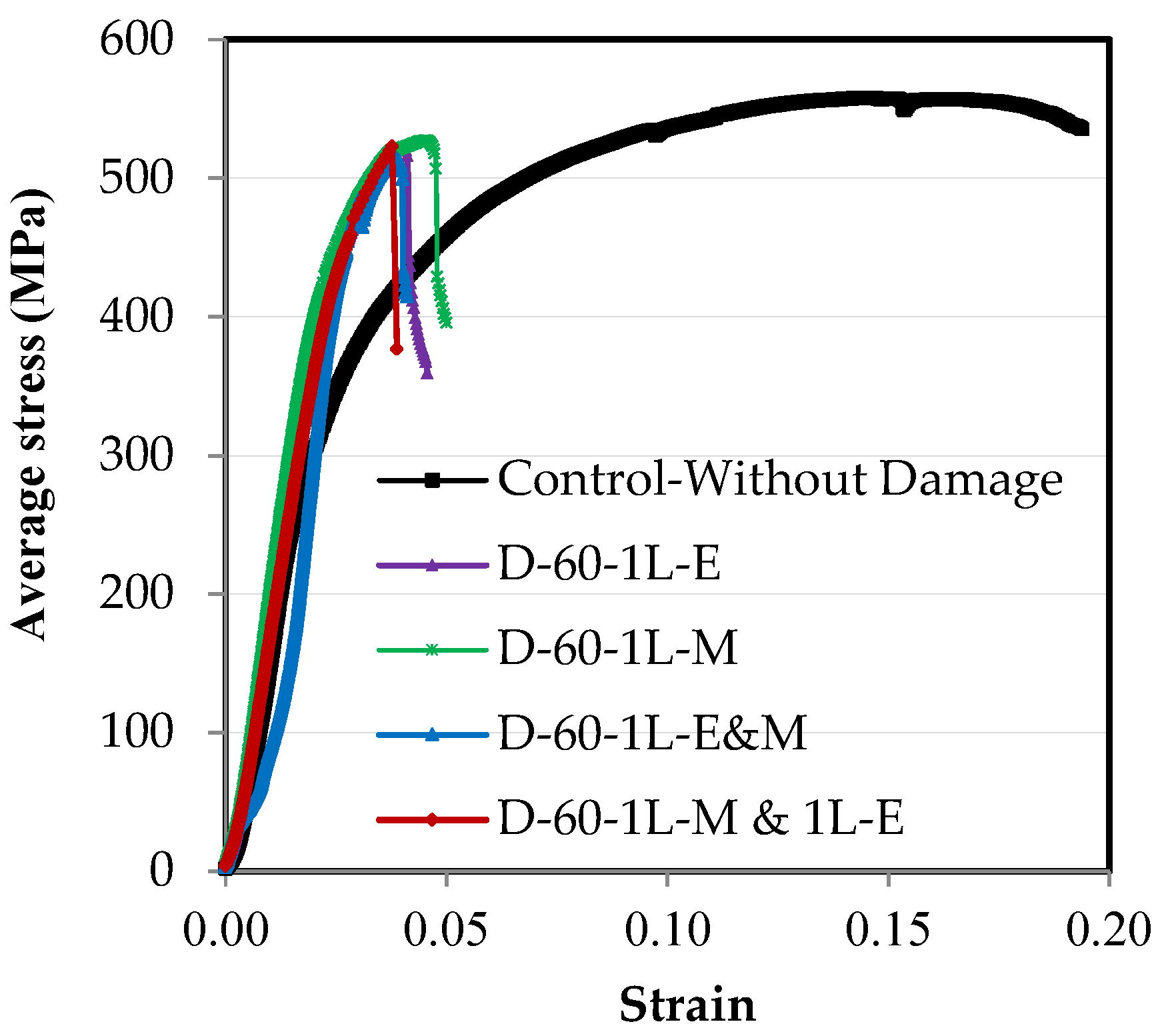
3.1. Damage Impact on Load–Elongation Relationships
3.2. Damage Impact on Cross-Section Stresses
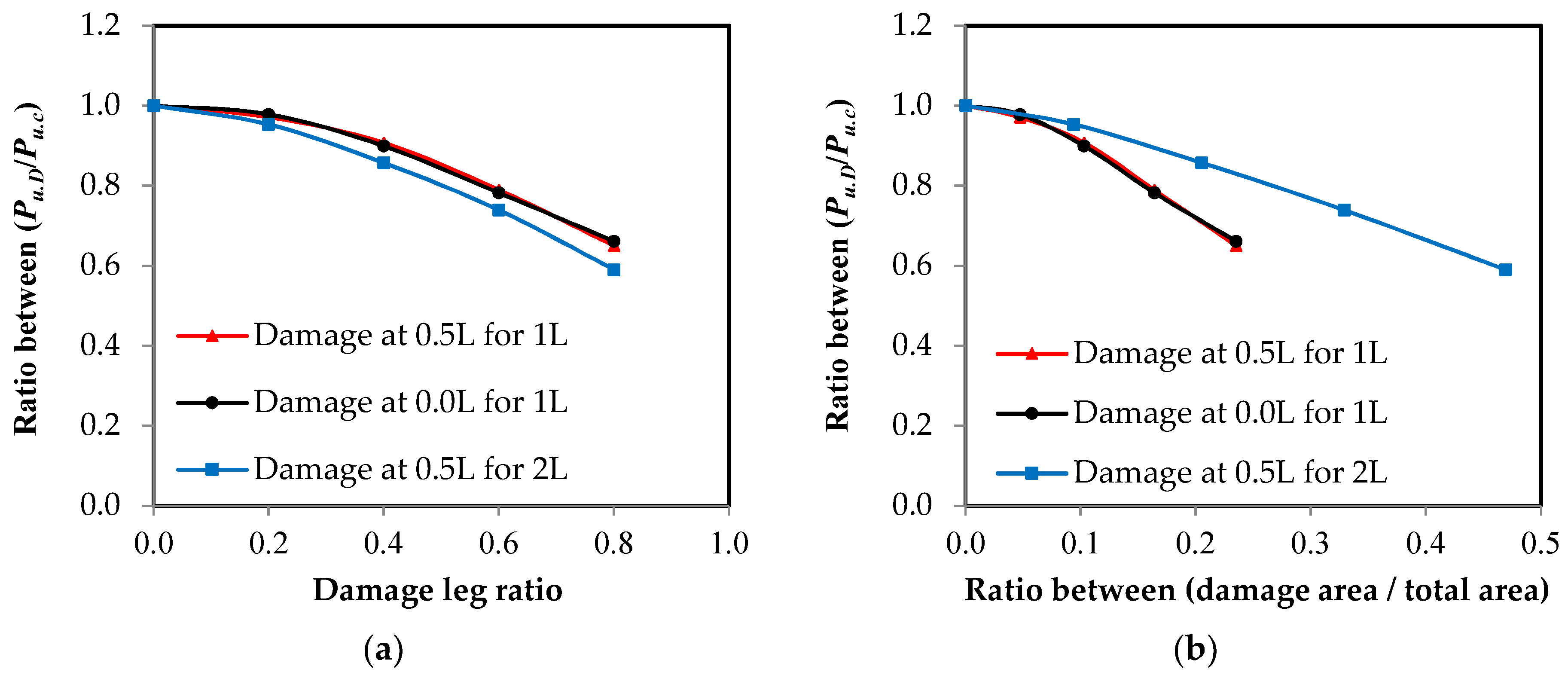
3.3. Damage Impact on Member Axial Load Capacity
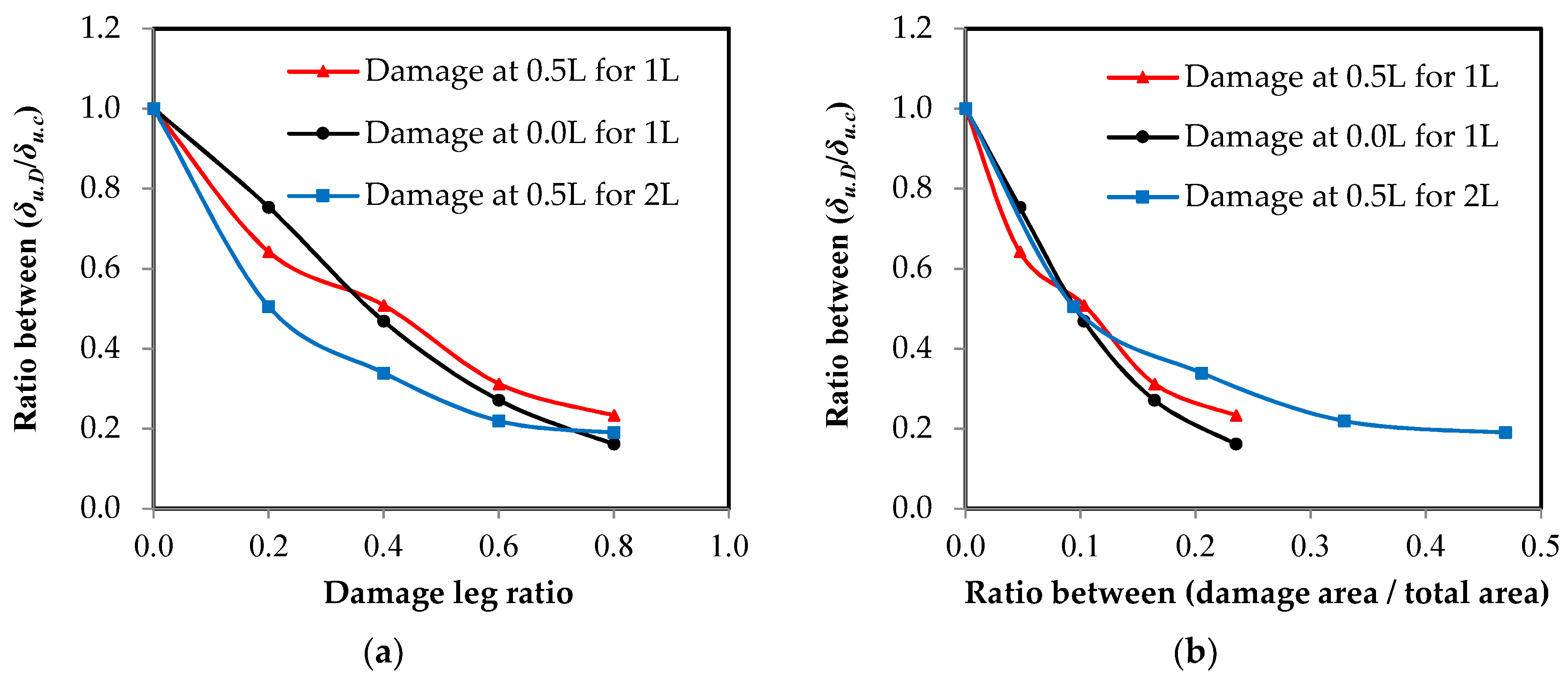
3.4. Damage Impact on Member Elongation
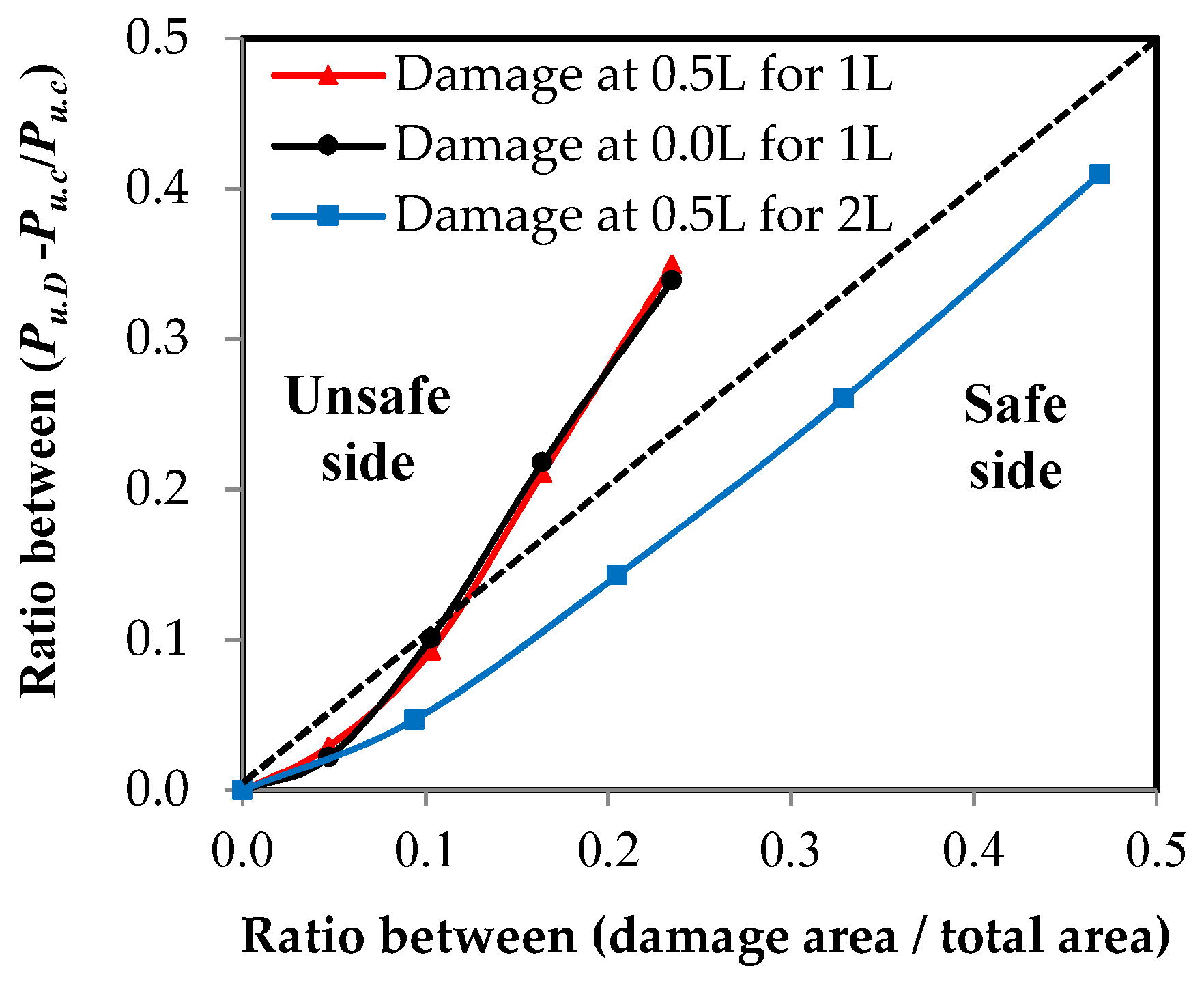
3.5. Impact of Damage Location in Relation to Element’s Length
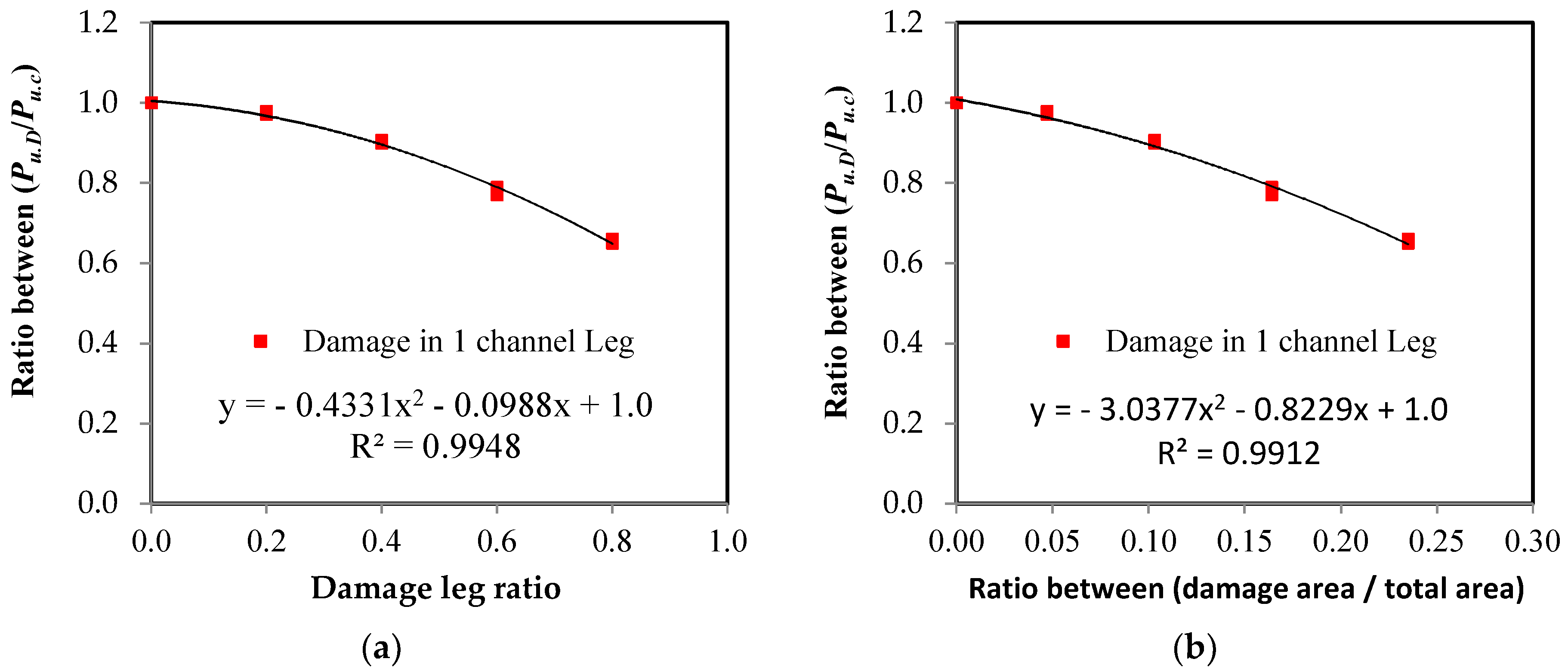
4. Predicting the Load Capacity of Steel Channel Element with Damage in the Unconnected Leg
5. Conclusions
- The impact of the damage in the two unconnected legs at any ratio, or the damage at a ratio of less than or equal to 40% in only one leg, causes a reduction of the load capacity that is less than that of the reduced area of the cross-section (i.e., a safe design).
- A damage effect that is greater than 40% on one leg only causes a reduction of the load capacity that is greater than that of the reduced area of the cross-section (i.e., unsafe design).
- Damage in only one leg causes an additional decentralization of the cross-section in the transverse direction, resulting in a concentration of stresses at the corner of the connection between the damaged leg and the web of the channel section, which accelerates the failure with a carrying capacity that is less than the design load of the cross-section.
- As the damage ratio increases in the two legs together, the eccentricity of the cross-section reduces, improving the shape of the stress distributions through the cross-section.
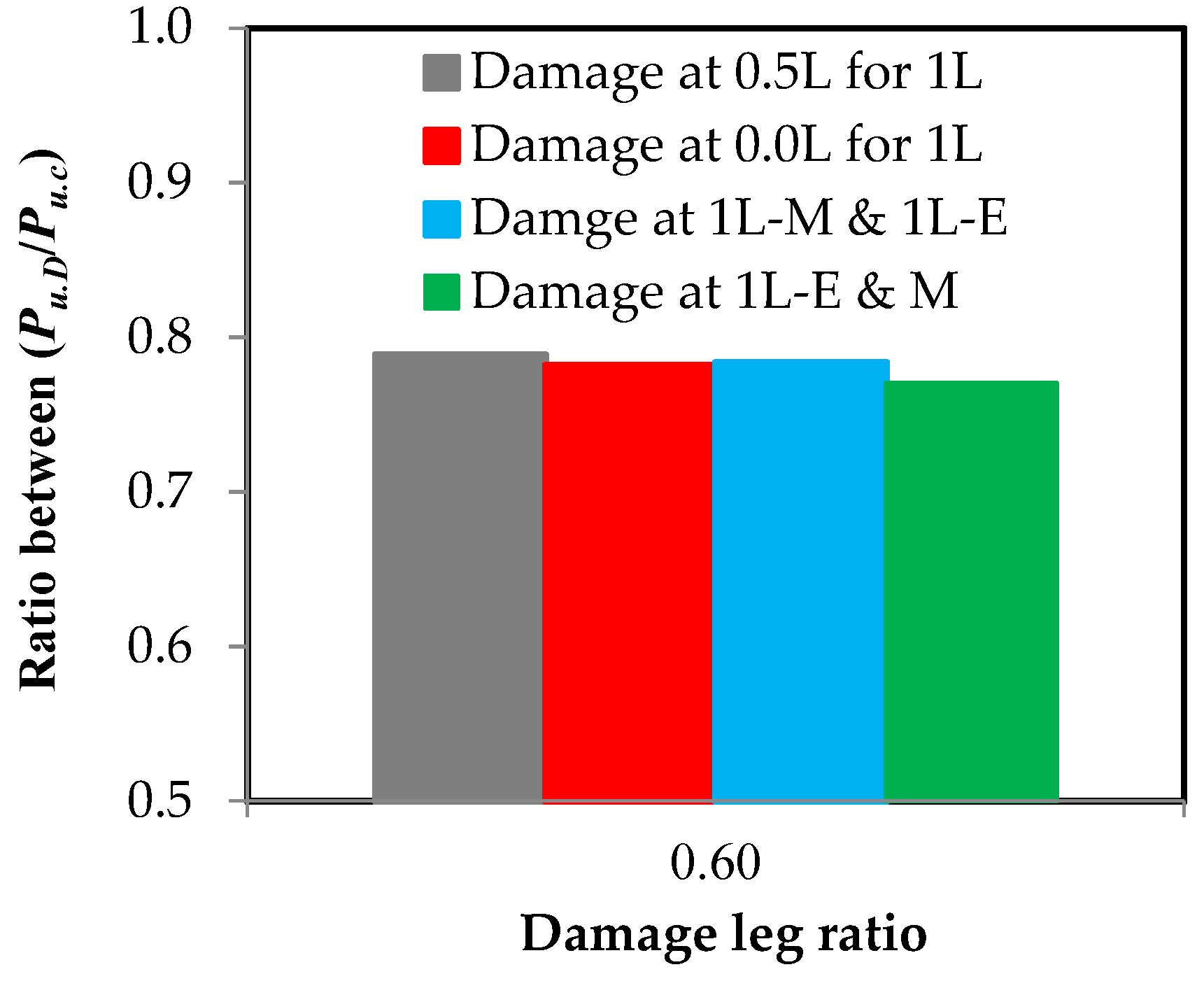
- The damage location along the element’s length does not significantly impact the measured axial load capacity, with the maximum difference being 1.9%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fogarty, J.; Yossef, N.M.; El-Tawil, S. Collapse resistance of locally damaged steel columns. J. Constr. Steel Res. 2013, 82, 195–202. [Google Scholar] [CrossRef]
- Priya, C.B.; Keerthana, M.; Verma, M.; Banjara, N.K. Design of steel channel tension members-proposal to IS 800:2007. J. Struct. Eng. 2013, 38, 122–130. [Google Scholar]
- Teh, L.H.; Gilbert, B.P. Net section tension capacity of cold-reduced sheet steel channel braces bolted at the web. J. Struct. Eng. 2013, 139, 740–747. [Google Scholar] [CrossRef] [Green Version]
- Charalampakis, A.E. Full plastic capacity of equal angle sections under biaxial bending and normal force. Eng. Struct. 2011, 33, 2085–2090. [Google Scholar] [CrossRef]
- Kirkham, W.J.; Miller, T.H. Examination of AISC LRFD shear lag design provisions. AISC Eng. J. 2000, 37, 83–98. [Google Scholar]
- Mohan, G.; Gupta, L.M. Evaluation of stress distribution in bolted steel angles under tension. Elec. J. Struct. Eng. 2004, 4, 17–25. [Google Scholar] [CrossRef]
- Može, P.; Cajot, L.G.; Sinur, F.; Rejec, K.; Beg, D. Residual stress distribution of large steel equal leg angles. Eng. Struct. 2014, 71, 35–47. [Google Scholar] [CrossRef]
- Zhu, H.T.; Yam, M.C.H.; Lam, A.C.C.; Iu, V.P. The shear lag effects on welded steel single angle tension members. J. Constr. Steel Res. 2009, 65, 1171–1186. [Google Scholar] [CrossRef]
- Kim, T.S.; Kim, M.S.; Cho, T.J. Strength of channel bolted connection with austenitic stainless steel (304 type). Int. J. Steel Struct. 2015, 15, 719–731. [Google Scholar] [CrossRef]
- Shi, G.; Zhou, W.J.; Bai, Y.; Liu, Z. Local buckling of steel equal angle members with normal and high strengths. Int. J. Steel Struct. 2014, 14, 447–455. [Google Scholar] [CrossRef]
- Barth, K.E.; Orbison, J.G.; Nukala, R. Behaviour of steel tension members subjected to uniaxial loading. J. Constr. Steel Res. 2002, 58, 1103–1120. [Google Scholar] [CrossRef]
- Hussain, A.; Liu, Y.P.; Chan, S.L. Finite element modeling and design of single angle member under bi-axial bending. Structures 2018, 16, 373–389. [Google Scholar] [CrossRef]
- Epstein, H.I.; Chamarajanagar, R. Finite element studies for correlation with block shear tests. Comput. Struct. 1996, 61, 967–974. [Google Scholar] [CrossRef]
- Sayed, A.M. Numerical analysis of single-angle steel member under tension load with damage in the unconnected leg. Structures 2020, 25, 920–929. [Google Scholar] [CrossRef]
- Kulak, G.L.; Wu, E.Y. Shear lag in bolted angle tension members. J. Struct. Eng. ASCE 1997, 123, 1144–1152. [Google Scholar] [CrossRef]
- Epstein, H.I.; Aiuto, C.L.D. Using moment and axial interaction equations to account for moment and shear lag effects in tension members. AISC Eng. J. 2002, 39, 91–99. [Google Scholar]
- Kitipornchai, S.; Lee, H. Inelastic buckling of single-angle, tee and double-angle struts. J. Constr. Steel Res. 1986, 6, 3–20. [Google Scholar] [CrossRef]
- Teh, L.H.; Gilbert, B.P. Net section tension capacity of cold-reduced sheet steel angle braces bolted at one leg. J. Struct. Eng. 2013, 139, 328–337. [Google Scholar] [CrossRef] [Green Version]
- Makesh, A.P.; Arivalagan, S. Experimental and analytical study on behaviour of cold formed steel using angle section under tension members. Int. J. Eng. Technol. Manag. Res. 2018, 5, 20–28. [Google Scholar] [CrossRef]
- Liu, Y.; Chantel, S. Experimental study of steel single unequal-leg angles under eccentric compression. J. Constr. Steel Res. 2011, 67, 919–928. [Google Scholar] [CrossRef]
- Kuralkar, S.D.; Oswal, S.S. Biaxial bending of steel angle section. Int. J. Eng. Res. 2016, 5, 232–234. [Google Scholar] [CrossRef]
- Trahair, N. Moment capacities of steel angle sections. J. Struct. Eng. 2002, 128, 1387–1393. [Google Scholar] [CrossRef] [Green Version]
- Trahair, N. Biaxial bending of steel angle section beams. J. Struct. Eng. 2004, 130, 554–561. [Google Scholar] [CrossRef]
- Ye, J.; Hajirasouliha, I.; Becque, J.; Pilakoutas, K. Development of more efficient cold-formed steel channel sections in bending. Thin-Walled Struct. 2016, 101, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Chen, B.; Roy, K.; Fang, Z.; Uzzaman, A. Shear capacity of cold-formed steel channels with edge-stiffened web holes, unstiffened web holes, and plain webs. J. Struct. Eng. 2022, 148, 04021268. [Google Scholar] [CrossRef]
- Uzzaman, A.; Lim, J.B.P.; Nash, D.; Rhodes, J.; Young, B. Web crippling behaviour of cold-formed steel channel sections with offset web holes subjected to interior-two-flange loading. Thin-Walled Struct. 2012, 50, 76–86. [Google Scholar] [CrossRef] [Green Version]
- Uzzaman, A.; Lim, J.B.P.; Nash, D.; Roy, K. Web crippling behaviour of cold-formed steel channel sections with edge-stiffened and unstiffened circular holes under interior-two-flange loading condition. Thin-Walled Struct. 2020, 154, 106813. [Google Scholar] [CrossRef]
- Uzzaman, A.; Lim, J.B.P.; Nash, D.; Roy, K. Cold-formed steel channel sections under end-two-flange loading condition: Design for edge-stiffened holes, unstiffened holes and plain webs. Thin-Walled Struct. 2020, 147, 106532. [Google Scholar] [CrossRef]
- Uzzaman, A.; Lim, J.B.P.; Nash, D.; Rhodes, J.; Young, B. Cold-formed steel sections with web openings subjected to web crippling under two-flange loading conditions—Part I: Tests and finite element analysis. Thin-Walled Struct. 2012, 56, 38–48. [Google Scholar] [CrossRef] [Green Version]
- Park, Y.S.; Kang, D.H. Method for evaluating damage caused to steel columns by local buckling. KSCE J. Civil. Eng. 2018, 22, 3592–3599. [Google Scholar] [CrossRef]
- Huang, C.; Chen, T.; Wang, X. Compressive characteristics of damaged circular hollow section (CHS) steel columns repaired by CFRP or grout jacketing. Thin-Walled Struct. 2017, 119, 635–645. [Google Scholar] [CrossRef]
- Wang, W.Y.; Li, G.Q. Behavior of steel columns in a fire with partial damage to fire protection. J. Constr. Steel Res. 2009, 65, 1392–1400. [Google Scholar] [CrossRef]
- El-taly, B.A.; El-Hameed, M.F.A. The parameters effect on the structural performance of damaged steel box beam using Taguchi method. Int. J. Adv. Struct. Eng. 2018, 10, 1–15. [Google Scholar] [CrossRef]
- Zeinoddini, M.; Parke, G.A.R.; Harding, J.E. Axially pre-loaded steel tubes subjected to lateral impacts: An experimental study. J. Impact Eng. 2002, 27, 669–690. [Google Scholar] [CrossRef]
- Zeinoddini, M.; Harding, J.E.; Parke, G.A.R. Axially pre-loaded steel tubes subjected to lateral impacts (a numerical simulation). J. Impact Eng. 2008, 35, 1267–1279. [Google Scholar] [CrossRef]
- Harding, J.E.; Onoufriou, T. Behaviour of ring-stiffened cylindrical members damaged by local denting. J. Constr. Steel Res. 1995, 33, 237–257. [Google Scholar] [CrossRef]
- Zeinoddini, M.; Harding, J.E.; Parke, G.A.R. Effect of impact damage on the capacity of tubular steel members of offshore structures. J. Mar. Struct. 1998, 11, 141–158. [Google Scholar] [CrossRef]
- Zeinoddini, M.; Harding, J.E.; Parke, G.A.R. Dynamic behaviour of axially pre-loaded tubular steel members of offshore structures subjected to impact damage. J. Ocean Eng. 1999, 26, 963–978. [Google Scholar] [CrossRef]
- Kaya, A.; Dawood, M.; Gencturk, B. Repair of corroded and buckled short steel columns using concrete-filled GFRP jackets. Constr. Build. Mater. 2015, 94, 20–27. [Google Scholar] [CrossRef]
- Karagah, H.; Shi, C.; Dawood, M.; Belarbi, A. Experimental investigation of short steel columns with localized corrosion. Thin Walled Struct. 2015, 87, 191–199. [Google Scholar] [CrossRef]
- Beaulieu, L.; Legeron, F.; Langlois, S. Compression strength of corroded steel angle members. J. Constr. Steel Res. 2010, 66, 1366–1373. [Google Scholar] [CrossRef]
- Saad-Eldeen, S.; Garbatov, Y.; Soares, C.G. Experimental assessment of the ultimate strength of a box girder subjected to severe corrosion. Mar. Struct. 2011, 24, 338–357. [Google Scholar] [CrossRef]
- Saad-Eldeen, S.; Garbatov, Y.; Soares, C.G. Strength assessment of a severely corroded box girder subjected to bending moment. J. Constr. Steel Res. 2014, 92, 90–102. [Google Scholar] [CrossRef]
- ASTM Standards A370–08a; Standard Test Methods and Definitions for Mechanical Testing of Steel Products. American Society for Testing and Materials: West Conshohocken, PA, USA, 2008.
- ASTM A370/ASME SA-370; Standard Test Methods and Definitions for Mechanical Testing of Steel Products. American Society for Testing and Materials: West Conshohocken, PA, USA, 2016.









| Steel Plate Specimens | Net Width (mm) | Yielding Stress σy (MPa) | Ultimate Stress σu (MPa) | Strain at Failure εf | Modulus of Elasticity E (GPa) |
|---|---|---|---|---|---|
| S-1 | 40.1 | 389.27 | 626.94 | 0.18752 | 204.74 |
| S-2 | 40.3 | 399.84 | 619.22 | 0.20113 | 209.23 |
| S-3 | 39.8 | 406.72 | 631.51 | 0.21001 | 200.19 |
| Average | --- | 398.61 | 625.89 | 0.19955 | 204.72 |
| Channel Specimen | Damage at Unconnected Leg (%) | Damage Location from Length | Ultimate Load P (kN) | PU.D/PU.C | Elongation at Ultimate δ (mm) | δF.D/δF.C | Average Stresses at the Damage Cross-Section | ||
|---|---|---|---|---|---|---|---|---|---|
| Area (mm2) | AD/AC | Stress (MPa) | |||||||
| Control-without-D | without | — | 739.61 | 1.000 | 65.35 | 1.000 | 1326 | 1.000 | 557.78 |
| D-20%-1L-M | 20 | 0.50L | 718.17 | 0.971 | 41.98 | 0.642 | 1264 | 0.953 | 568.17 |
| D-40%-1L-M | 40 | 0.50L | 670.56 | 0.907 | 33.23 | 0.508 | 1190 | 0.897 | 563.50 |
| D-60%-1L-M | 60 | 0.50L | 583.68 | 0.789 | 20.36 | 0.312 | 1108 | 0.836 | 526.79 |
| D-80%-1L-M | 80 | 0.50L | 480.40 | 0.650 | 15.21 | 0.233 | 1015 | 0.765 | 473.30 |
| D-20%-2L-M | 20 | 0.50L | 705.20 | 0.953 | 32.97 | 0.505 | 1202 | 0.906 | 586.69 |
| D-40%-2L-M | 40 | 0.50L | 634.00 | 0.857 | 22.18 | 0.339 | 1054 | 0.795 | 601.52 |
| D-60%-2L-M | 60 | 0.50L | 546.65 | 0.739 | 14.32 | 0.219 | 890 | 0.671 | 614.21 |
| D-80%-2L-M | 80 | 0.50L | 436.08 | 0.590 | 12.42 | 0.190 | 704 | 0.531 | 619.43 |
| D-20%-1L-E | 20 | L | 723.39 | 0.978 | 49.22 | 0.753 | 1264 | 0.953 | 572.30 |
| D-40%-1L-E | 40 | L | 664.54 | 0.899 | 30.61 | 0.468 | 1190 | 0.897 | 558.44 |
| D-60%-1L-E | 60 | L | 578.51 | 0.782 | 17.74 | 0.271 | 1108 | 0.836 | 522.12 |
| D-80%-1L-E | 80 | L | 488.64 | 0.661 | 10.50 | 0.161 | 1015 | 0.765 | 481.42 |
| D-60%-1L-E and M | 60 | 0.5L + L | 569.36 | 0.770 | 17.43 | 0.267 | 1108 | 0.836 | 513.86 |
| D-60%-1L m and 1L-E | 60 | 0.5L + L | 579.80 | 0.784 | 16.94 | 0.259 | 1108 | 0.836 | 523.29 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sayed, A.M.; Alanazi, H.; Abadel, A.A.; Alharbi, Y.R.; Shamsudin, M.F. Experimental Analysis of Channel Steel Member under Tension Load with Damage in the Unconnected Legs. Materials 2023, 16, 527. https://doi.org/10.3390/ma16020527
Sayed AM, Alanazi H, Abadel AA, Alharbi YR, Shamsudin MF. Experimental Analysis of Channel Steel Member under Tension Load with Damage in the Unconnected Legs. Materials. 2023; 16(2):527. https://doi.org/10.3390/ma16020527
Chicago/Turabian StyleSayed, Ahmed M., Hani Alanazi, Aref A. Abadel, Yousef R. Alharbi, and Mohd F. Shamsudin. 2023. "Experimental Analysis of Channel Steel Member under Tension Load with Damage in the Unconnected Legs" Materials 16, no. 2: 527. https://doi.org/10.3390/ma16020527
APA StyleSayed, A. M., Alanazi, H., Abadel, A. A., Alharbi, Y. R., & Shamsudin, M. F. (2023). Experimental Analysis of Channel Steel Member under Tension Load with Damage in the Unconnected Legs. Materials, 16(2), 527. https://doi.org/10.3390/ma16020527






