A Design Process for Preventing Brittle Failure in Strengthening RC Slabs with Hybrid FRP-HPC Retrofit Systems
Abstract
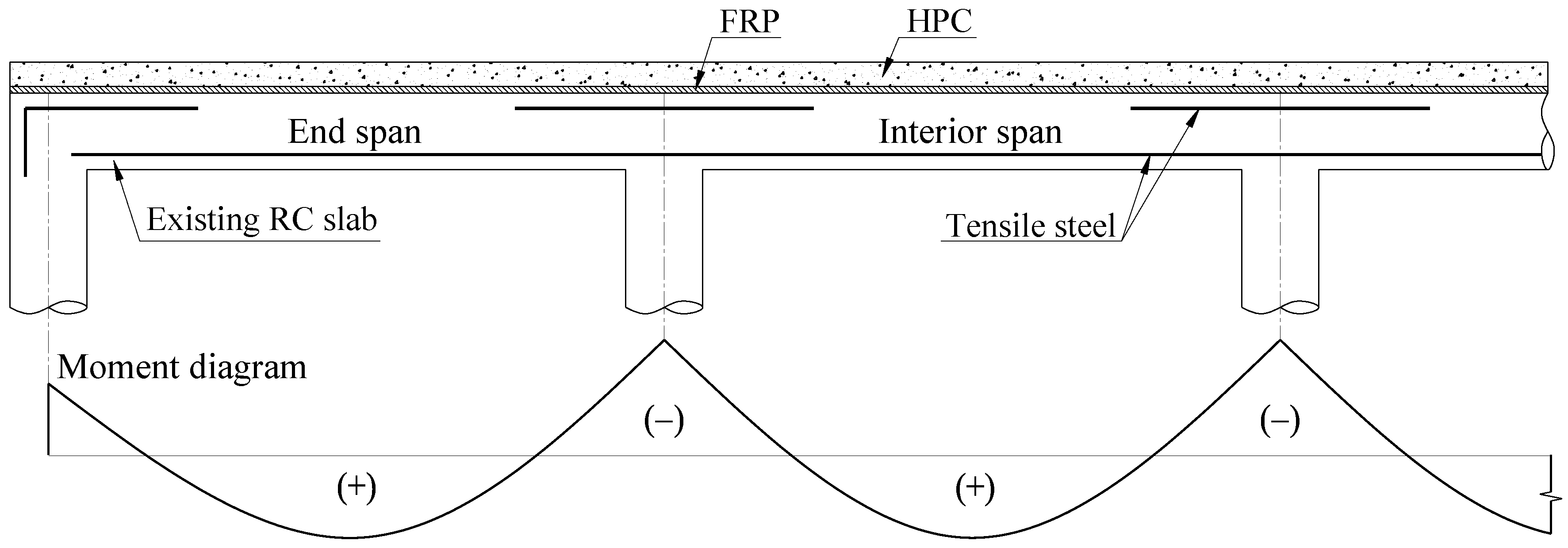
:1. Introduction
2. Overview Theory and Proposed Process
2.1. Failure Limits Overview
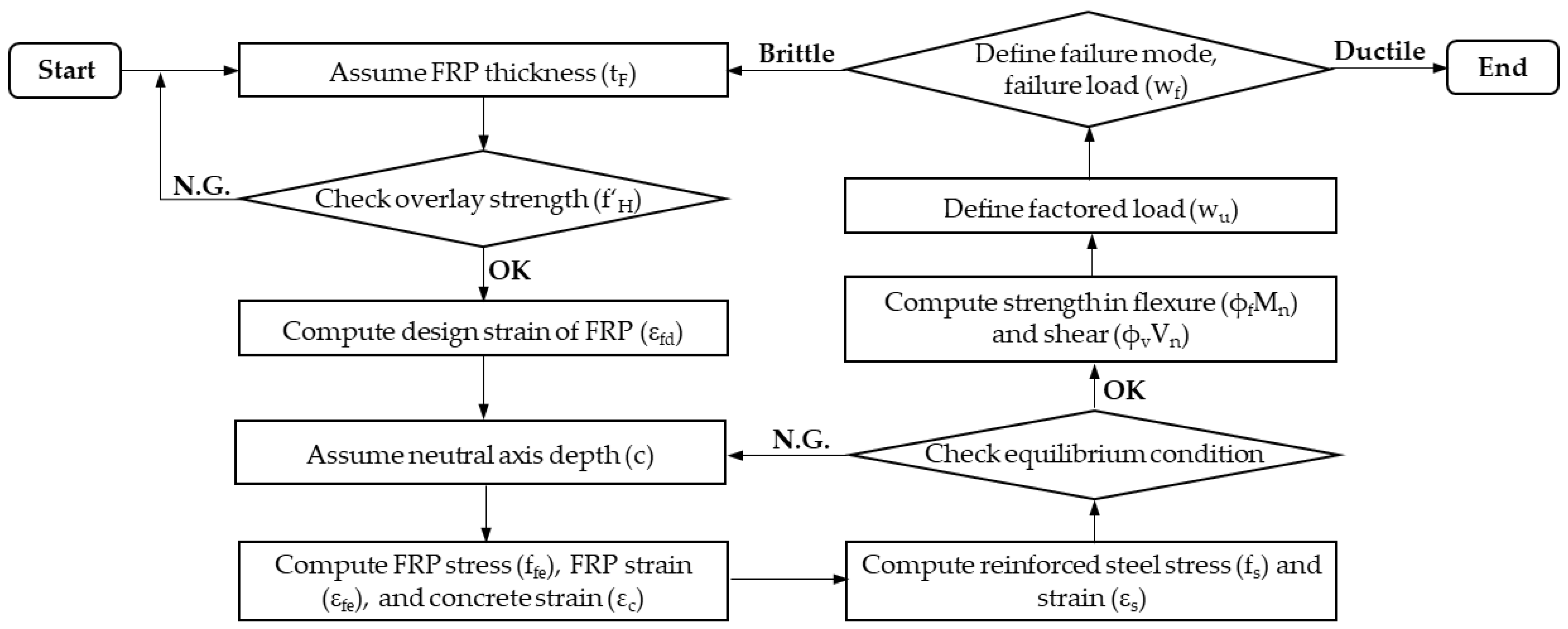
2.2. Retrofitting Mechanism and Design Process Preventing Brittle Failure
- (1)
- Assume FRP thickness (tF).
- (2)
- The overlay strength () should be greater than the limits in the following equations to ensure the neutral axis within the overlay and FRP in the tensile zone [15]; otherwise, re-assume FRP thickness.
- (3)
- Compute the design strain of FRP (εfd) at support.
- (4)
- Assume the neutral axis depth (c).
- (5)
- Compute FRP stress (ffe) corresponding to FRP strain (εfe) and concrete strain at failure (εc) by applying similar triangles based on strain compatibility.For the support section:For the mid-span section:
- (6)
- Compute reinforced steel stress (fs) and strain (εs).For the support section (εs,N):For the mid-span section (εs,P):
- (7)
- Check the equilibrium condition by comparing c defined in Equation (33) with the value in step 4. If it is satisfied, go to step 9; otherwise, return to step 4.
- (8)
- Compute strength in flexure (ϕfMn) and shear (ϕvVn)For the support section, the contribution of steel (Mns,N) and FRP (Mnf,N), asFor the mid-span section, the contribution of steel (Mns,P) and FRP (Mnf,P), as
- (9)
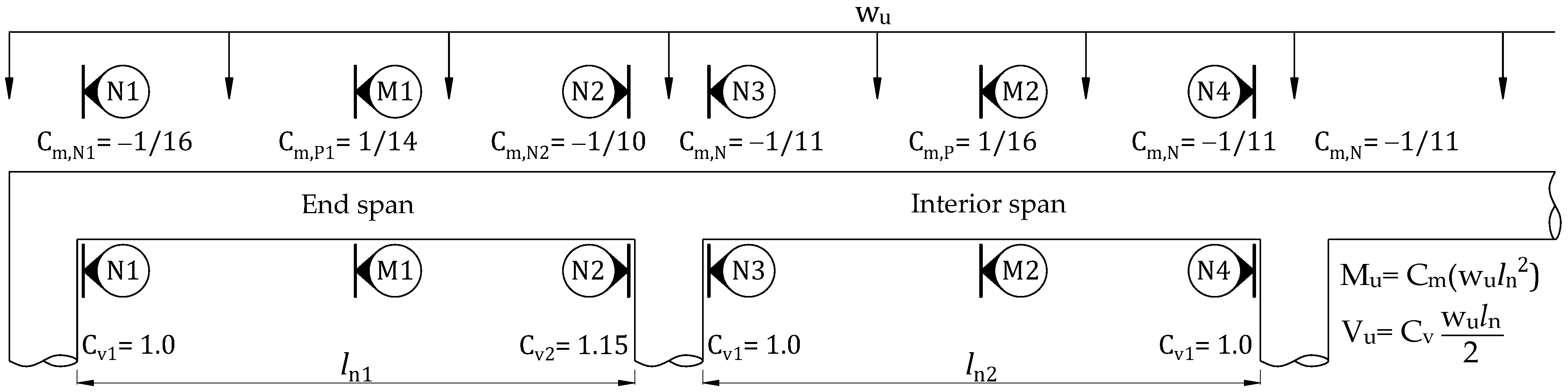
- Define the design factored load as specified in Figure 2.
- (10)
- Define the failure mode and failure load (wf) in accordance with the failure limits. If the failure mode is ductile, the design process preventing brittle failure can be achieved; otherwise, re-assume FRP thickness.
3. Design Example
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| AF, As | Area of CFRP and tensile steel |
| b | Width of an existing slab |
| c | The distance between the extreme compression fiber and the neutral axis |
| CE | Environmental reduction coefficient |
| Cm,Ni | Moment coefficients at support section ith |
| Cm,Pi | Moment coefficients at mid-span section ith |
| Cvi | Shear coefficients at section ith |
| d | The distance between the extreme compression fiber and the center of the steel |
| Ec, Es, Efe | Elastic modulus of concrete, steel, and CFRP |
| , | Compressive strength concrete of existing slab and overlay |
| ffe | CFRP effective stress |
| ffu | Design ultimate strength of CFRP |
| FRP’s ultimate tensile strength, according to the manufacturer | |
| fs | Tension steel’s stress |
| fy | Yield stress of tension steel |
| h | Height of an existing RC slab |
| Icr | Cracked moment |
| k | The ratio of the neutral axis depth to tensile steel depth measured from extreme compression fiber |
| lni | Length of clear span ith |
| n | The number of CFRP layers |
| Mn, Vn | Moment and shear carrying capacity |
| Mn,P, Mn,N | Mid-span and support sections’ moment-carrying capacities |
| Mns, Mnf | Moments contributed by tensile steel and CFRP |
| MN1 | Moment-carrying capacities of the N1 section |
| MD,N2 | Moment-carrying of the N-2 section |
| Mu, Vu | Factored moment and shear at sections |
| tF, tH | The thickness of CFRP and HPC overlay |
| wf | Ultimate failure load |
| wu | Design factored load |
| wuM, wuV | Design factored load follow moment and shear carrying capacities |
| ϕf, ϕv | Flexural and shear strength reduction factors |
| ψf | CFRP strength reduction factor |
| α1, β1 | Stress block factors |
| εbi | Existing state strain of CFRP installation |
| εcu, εfu | Ultimate strains of concrete and CFRP |
| εfd | Debonding strain of CFRP |
| εfe, εs | Strains of CFRP and tensile steel |
| γc | Concrete unit weight |
References
- Maxineasa, S.G.; Taranu, N. 24-Life cycle analysis of strengthening concrete beams with FRP. In Eco-Efficient Repair and Rehabilitation of Concrete Infrastructures; Pacheco-Torgal, F., Melchers, R.E., Shi, X., De Belie, N., van Tittelboom, K., Sáez, A., Eds.; Woodhead Publishing: Cambridge, UK, 2018; pp. 673–721. [Google Scholar]
- Ma, Z.; Shen, J.; Wang, C.; Wu, H. Characterization of sustainable mortar containing high-quality recycled manufactured sand crushed from recycled coarse aggregate. Cem. Concr. Compos. 2022, 132, 104629. [Google Scholar] [CrossRef]
- Martínez, S.; de Diego, A.; Castro, V.J.; Echevarría, L.; Barroso, F.J.; Rentero, G.; Soldado, R.P.; Gutiérrez, J.P. Strengthening of Low-Strength Concrete Columns with Fibre Reinforced Polymers. Full-Scale Tests. Infrastructures 2020, 5, 91. [Google Scholar] [CrossRef]
- Hariri-Ardebili, M.A.; Sanchez, L.; Rezakhani, R. Aging of Concrete Structures and Infrastructures: Causes, Consequences, and Cures. Adv. Mater. Sci. Eng. 2020, 2020, 9370591. [Google Scholar] [CrossRef]
- Nguyen, X.T.; Park, J.S. Flexural Behavior of Steel Beams Strengthened with CFRP Under Fire. Int. J. Steel Struct. 2022, 22, 1769–1785. [Google Scholar] [CrossRef]
- Vu, H.D.; Phan, D.N. A framework for predicting the debonding failure modes of RC beams strengthened flexurally with FRP sheets. Innov. Infrastruct. Solut. 2022, 7, 292. [Google Scholar] [CrossRef]
- Haji, M.; Naderpour, H.; Kheyroddin, A. Experimental study on influence of proposed FRP-strengthening techniques on RC circular short columns considering different types of damage index. Compos. Struct. 2019, 209, 112–128. [Google Scholar] [CrossRef]
- Barris, C.; Sala, P.; Gómez, J.; Torres, L. Flexural behaviour of FRP reinforced concrete beams strengthened with NSM CFRP strips. Compos. Struct. 2020, 241, 112059. [Google Scholar] [CrossRef]
- Basaran, B.; Kalkan, I. Development length and bond strength equations for FRP bars embedded in concrete. Compos. Struct. 2020, 251, 112662. [Google Scholar] [CrossRef]
- Mukhtar, F.M.; Arowojolu, O. Recent developments in experimental and computational studies of hygrothermal effects on the bond between FRP and concrete. J. Reinf. Plast. Compos. 2020, 39, 422–442. [Google Scholar] [CrossRef]
- Wang, X.; Yang, Y.; Yang, R.; Liu, P. Experimental Analysis of Bearing Capacity of Basalt Fiber Reinforced Concrete Short Columns under Axial Compression. Coatings 2022, 12, 654. [Google Scholar] [CrossRef]
- Xian, G.; Guo, R.; Li, C.; Wang, Y. Mechanical performance evolution and life prediction of prestressed CFRP plate exposed to hygrothermal and freeze-thaw environments. Compos. Struct. 2022, 293, 115719. [Google Scholar] [CrossRef]
- Li, C.; Guo, R.; Xian, G.; Li, H. Effects of elevated temperature, hydraulic pressure and fatigue loading on the property evolution of a carbon/glass fiber hybrid rod. Polym. Test. 2020, 90, 106761. [Google Scholar] [CrossRef]
- Lu, Z.; Li, W.; Zeng, X.; Pan, Y. Durability of BFRP bars and BFRP reinforced seawater sea-sand concrete beams immersed in water and simulated seawater. Constr. Build. Mater. 2023, 363, 129845. [Google Scholar] [CrossRef]
- Mosallam, A.; Taha, M.R.; Kim, J.; Nasr, A. Strength and ductility of RC slabs strengthened with hybrid high-performance composite retrofit system. Eng. Struct. 2012, 36, 70–80. [Google Scholar] [CrossRef]
- Ho, H.V.; Choi, E.; Park, S.J. Investigating stress distribution of crimped SMA fibers during pullout behavior using experimental testing and a finite element model. Compos. Struct. 2021, 272, 114254. [Google Scholar] [CrossRef]
- ACI PRC-440.2R-17; Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Concrete Structures. ACI: Farmington Hills, MI, USA, 2017.
- Nguyen, H.Q.; Nguyen, T.N.M.; Lee, D.H.; Kim, J.J. The Effects of Bond-Slip Laws on the Debonding Failure and Behavior of Flexural Strengthened RC Slabs in Hybrid FRP Retrofit Systems. Materials 2022, 15, 7453. [Google Scholar] [CrossRef] [PubMed]
- Kim, N.; Shin, Y.S.; Choi, E.; Kim, H.S. Relationships between interfacial shear stresses and moment capacities of RC beams strengthened with various types of FRP sheets. Constr. Build. Mater. 2015, 93, 1170–1179. [Google Scholar] [CrossRef]
- Lezgy-Nazargah, M.; Dezhangah, M.; Sepehrinia, M. The Effects of Different FRP/Concrete Bond–Slip Laws on the 3D Nonlinear FE Modeling of Retrofitted RC Beams—A Sensitivity Analysis. Steel Compos. Struct. 2018, 26, 347–360. [Google Scholar]
- Moon, J.; Taha, M.R.; Kim, J. Flexural Strengthening of RC Slabs Using a Hybrid FRP-UHPC System Including Shear Connector. Adv. Mater. Sci. Eng. 2017, 2017, 4387545. [Google Scholar] [CrossRef] [Green Version]
- Gunes, O.; Lau, D.; Tuakta, C.; Büyüköztürk, O. Ductility of FRP–concrete systems: Investigations at different length scales. Constr. Build. Mater. 2013, 49, 915–925. [Google Scholar] [CrossRef]
- Abdallah, M.; Al Mahmoud, F.; Khelil, A.; Mercier, J.; Almassri, B. Assessment of the flexural behavior of continuous RC beams strengthened with NSM-FRP bars, experimental and analytical study. Compos. Struct. 2020, 242, 112127. [Google Scholar] [CrossRef]
- Dai, M.N.; Toong, K.C.; Hee, K.C. Brittle Failure and Bond Development Length of CFRP-Concrete Beams. J. Compos. Constr. 2001, 5, 12–17. [Google Scholar]
- Rabinovitch, O.; Frostig, Y. Experiments and analytical comparison of RC beams strengthened with CFRP composites. Compos. Part B Eng. 2003, 34, 663–677. [Google Scholar] [CrossRef]
- Breveglieri, M.; Barros, J.; Dalfre, G.; Aprile, A. Assessment of the Effectiveness of a NSM-CFRP Flexural Strengthening Technique for Continuous RC Slabs. In Proceedings of the Czech Concrete Society (CBS) 2011, Prague, Czech Republic, 8–10 June 2011. [Google Scholar]
- Yasmeen, T.O.; Heyden, S.; Dahlblom, O. Evaluation of Parameters of Bond Action between FRP and Concrete. J. Compos. Constr. 2013, 17, 626–635. [Google Scholar]
- Hawileh, R.A.; Rasheed, H.A.; Abdalla, J.A.; Al-Tamimi, A.K. Behavior of reinforced concrete beams strengthened with externallybonded hybrid fiber reinforced polymer systems. Mater. Des. 2014, 53, 972–982. [Google Scholar] [CrossRef] [Green Version]
- Bocciarelli, M.; Pisani, M.A. Modified force method for the nonlinear analysis of FRP reinforced concrete beams. Compos. Struct. 2015, 131, 645–653. [Google Scholar] [CrossRef]
- Haciyev, V.; Sofiyev, A.; Kuruoglu, N. Free bending vibration analysis of thin bidirectionally exponentially graded orthotropic rectangular plates resting on two-parameter elastic foundations. Compos. Struct. 2018, 184, 372–377. [Google Scholar] [CrossRef]
- Al-Rousan, R.; Issa, H.S.M. Performance of reinforced concrete slabs strengthened with different types and configurations of CFRP. Compos. Part B Eng. 2012, 43, 510–521. [Google Scholar] [CrossRef]
- Duy, N.P.; Dang, V. Limiting Reinforcement Ratios for Hybrid GFRP/Steel Reinforced Concrete Beams. Int. J. Eng. Technol. Innov. 2021, 11, 1–11. [Google Scholar]
- Wei, L.; Ueda, T.; Matsumoto, K.; Zhu, J.-H. Experimental and analytical study on the behavior of RC beams with externally bonded carbon-FRCM composites. Compos. Struct. 2021, 273, 114291. [Google Scholar] [CrossRef]
- Nguyen, H.Q.; Nguyen, T.N.M.; Lee, D.H.; Kim, J.J. A Design Method to Induce Ductile Failure of Flexural Strengthened One-Way RC Slabs. Materials 2021, 14, 7647. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Noh, H.-C.; Taha, M.R.; Mosallam, A. Design limits for RC slabs strengthened with hybrid FRP–HPC retrofit system. Compos. Part B Eng. 2013, 51, 19–27. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, H.Q.; Yang, K.; Kim, J.J. An Efficient Method for Optimizing HPC-FRP Retrofit Systems of Flexural Strengthened One-Way Continuous Slabs Based on ACI 440.2R. Materials 2022, 15, 8430. [Google Scholar] [CrossRef] [PubMed]
- ACI 318M-19; Building Code Requirements for Structural Concrete and Commentary, Metric. ACI: Farmington Hills, MI, USA, 2019.


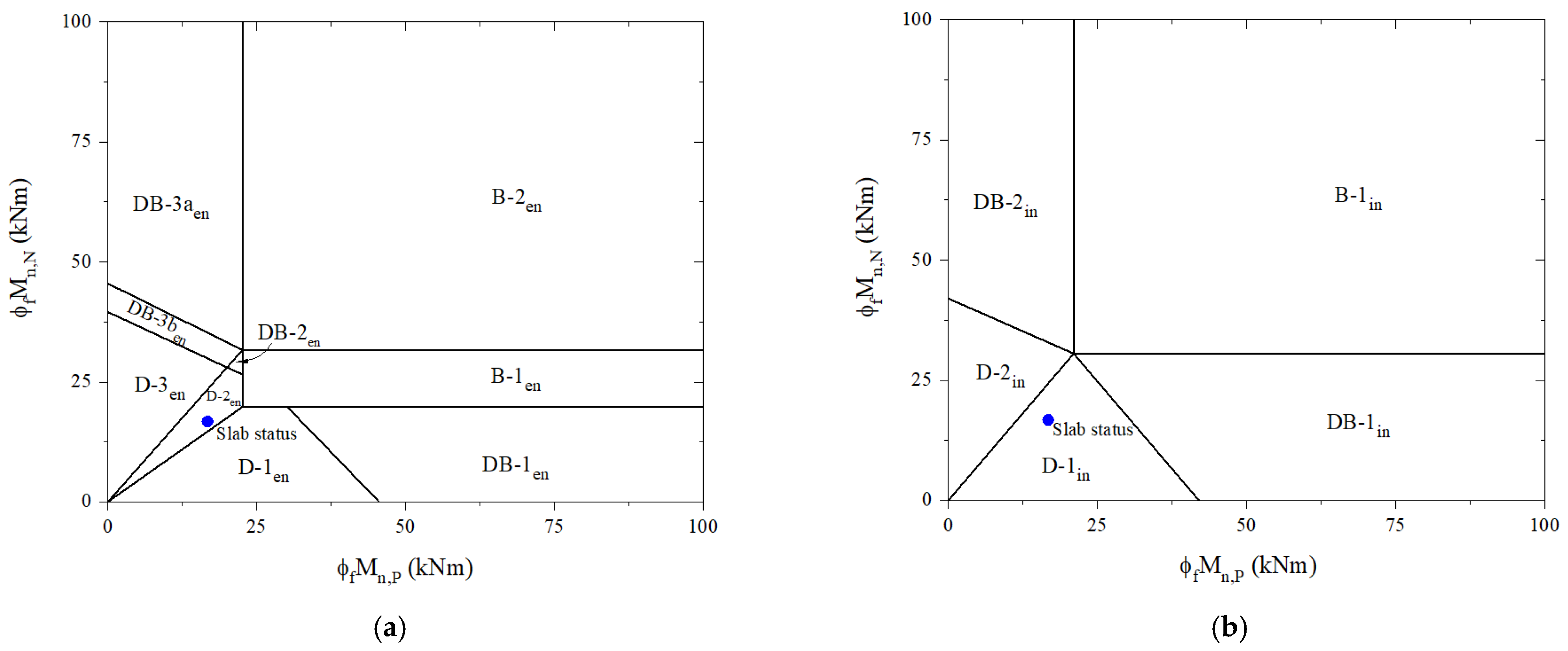
| Span Type | Failure Modes | First Plastic Hinge | Second Plastic Hinge | Third Plastic Hinge | Shear Failure | Failure Type |
|---|---|---|---|---|---|---|
| End span | D-1en | N2 | N1 | M1 | - | Ductile failure |
| D-2en | N2 | M1 | N1 | - | Ductile failure | |
| D-3en | M1 | N2 | N1 | - | Ductile failure | |
| DB-1en | N2 | N1 | - | N2 | Brittle failure | |
| DB-2en | N2 | M1 | - | N2 | Brittle failure | |
| DB-3aen | M1 | - | - | N2 | Brittle failure | |
| DB-3ben | M1 | N2 | - | N2 | Brittle failure | |
| B-1en | N2 | - | - | N2 | Brittle failure | |
| B-2en | - | - | - | N2 | Brittle failure | |
| Interior span | D-1in | N3, N4 | M2 | - | Ductile failure | |
| D-2in | M2 | N3, N4 | - | Ductile failure | ||
| DB-1in | N3, N4 | - | N3, N4 | Brittle failure | ||
| DB-2in | M2 | - | N3, N4 | Brittle failure | ||
| B-1in | - | - | N3, N4 | Brittle failure |
| Failure Modes | Failure Load | |
|---|---|---|
| D-1en | (15) | |
| D-2en | (16) | |
| D-3en | (17) | |
| DB-1en, DB-2en, DB-3aen, DB-3ben, B-1en, B-2en | (18) | |
| D-1in | (19) | |
| D-2in | (20) | |
| DB-1in, DB-2in, B-1in | (21) |
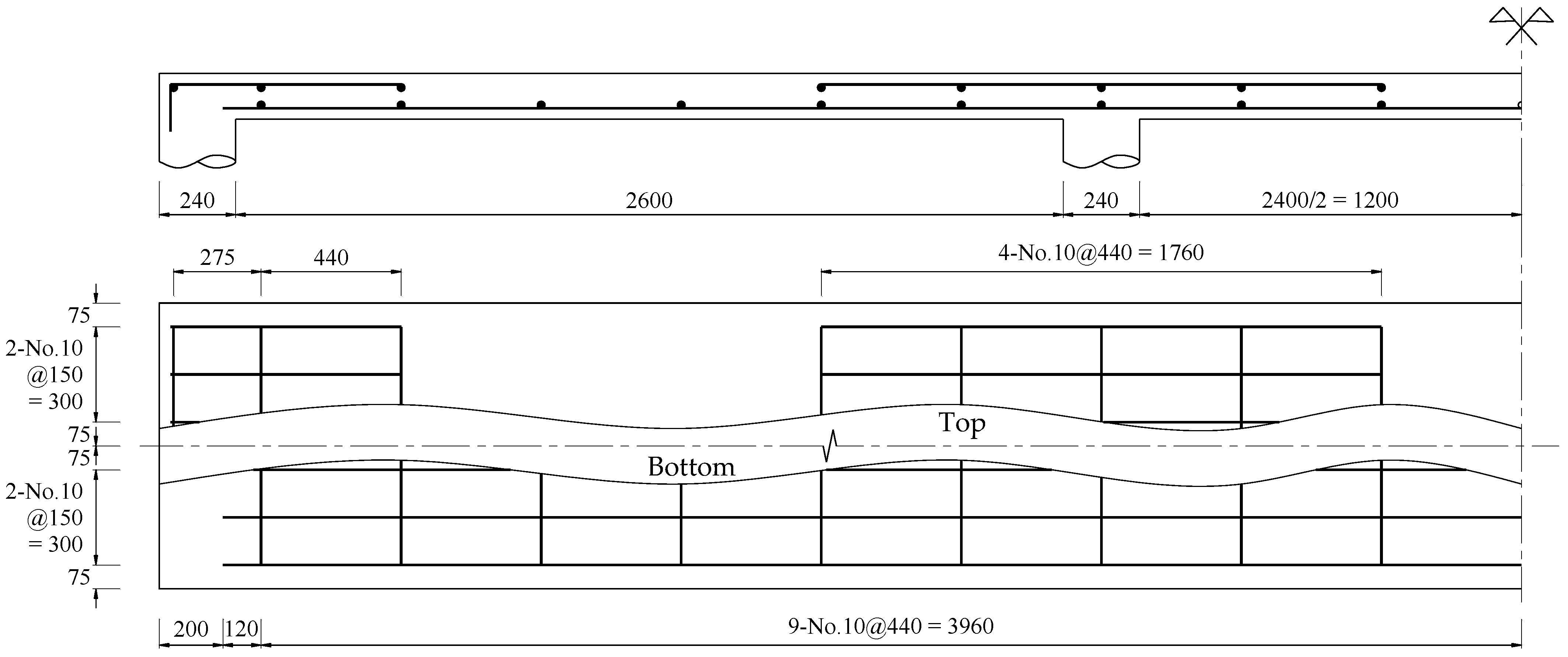
| Type | l (mm) | h (mm) | b (mm) | As (mm2) | d (mm) | fy (MPa) | Es (GPa) | |
|---|---|---|---|---|---|---|---|---|
| End span | 2600 | 145 | 900 | 32 | 426 | 110 | 410 | 200 |
| Interior span | 2400 | - | - | - | - | - | - | - |
| HPC Overlay | CFRP | |||
|---|---|---|---|---|
| tH (mm) | (MPa) | tF (mm) | (MPa) | Efe (GPa) |
| 30 | 75 | 1 | 600 | 40 |
| Analysis | Reference Slab |
|---|---|
| Sectional capacity | = 16.73 kNm; = 16.73 kNm; = 70 kN |
| Design factored load | For end span: = min(39.6; 24.7; 34.6; 53.8; 46.8) = 24.7 kN/m For interior span: = min(32; 46.5; 58.3) = 32 kN/m |
| Define failure mode and failure load | For end span: D-2en according to Figure 7a; Equation (16), = 31.3 kN/m For interior span: D-1in according to Figure 7b; Equation (19), = 39.2 kN/m |
| Self-weight | = 24(0.9)(0.145) = 3.13 kN/m |
| Elastic modulus | = = 26,600 MPa |
| At support, kd | kd = 24.65 mm |
| The crack moment at support | = |
| The ultimate strength and strain of CFRP | = 570 MPa; = 0.0143 |
| Moment due to dead load | At N2 section: = = 2.12 kNm At N3 and N4 sections: = = = 1.64 kNm |
| The existing state of strain | At N2 section: = = 0.00034 At N3 and N4 sections: = = 0.00027 |
| Process | End Span | Interior Span |
|---|---|---|
| 1. Assume CFRP thicknesses | tF = 1 mm | tF = 1 mm |
| 2. Check overlay strength | For both spans = 9.05 MPa (OK) = 12.49 MPa (OK) | |
| 3. Compute the design strain of FRP at support | = = 0.0116 = 0.0128 | |
| 4. Assume neutral axis depth | At the N2 section: 27.61 mm At mid-span section: 10.26 mm | At support sections (N3 and N4): 27.64 mm At mid-span section: 10.26 mm |
| 5. Compute FRP stress (ffe), FRP strain (εfe), and concrete strain (εc) | At the N2 section = = 0.0124 = 0.0116 = = 0.0028 = 0.0116(40,000) = 463.86 MPa At mid-span section = = 0.0058 = = 0.003 = 0.0058(40,000) = 230.97 MPa | At support sections = = 0.0125 = 0.0116 = = 0.0028 = 0.0116(40,000) = 463.86 MPa At mid-span section (same as the end span case) |
| 6. Compute reinforced steel stress (fs) and strain (εs) | At the N2 section = = 0.0084 = = 1680 MPa At mid-span section = = 0.0382 = 0.0382(200,000) = 7640 MPa > | At the support sections = = 0.0083 = 0.0083(200,000) = 1660 MPa > = 410 MPa At mid-span section (same as the end span case) |
| 7. Check the equilibrium condition | At the N2 section, due to = = 0.002; = = 0.807 = = 0.922 = = 27.61 mm (OK) At mid-span section, due to = 0.65; = 0.85 = = 10.26 mm (OK) | At support sections, due to = = 0.002; = = 0.806 = = 0.923 = = 27.64 mm (OK) At mid-span section (same as the end span case) |
| 8. Compute strength in flexure and shear 8.1 Compute strength in flexure at the support section | = = 17.27 kNm = = 55.89 kNm = = 58.29 kNm | = = 17.27 kNm = = 55.89 kNm = = 58.29 kNm |
| 8.2 Compute strength in flexure at the mid-span section | = = 24.04 kNm = = 5.54 kNm = = 25.88 kNm | same as end span case |
| 8.3 Compute strength in shear | = = 99.23 kN | same as end span case |
| 9. Define design factored load | = = 53.6 kN/m = = 53.6 kN/m = = 66.38 kN/m | = = 71.46 kN/m = = 71.46 kN/m = 82.68 kN/m |
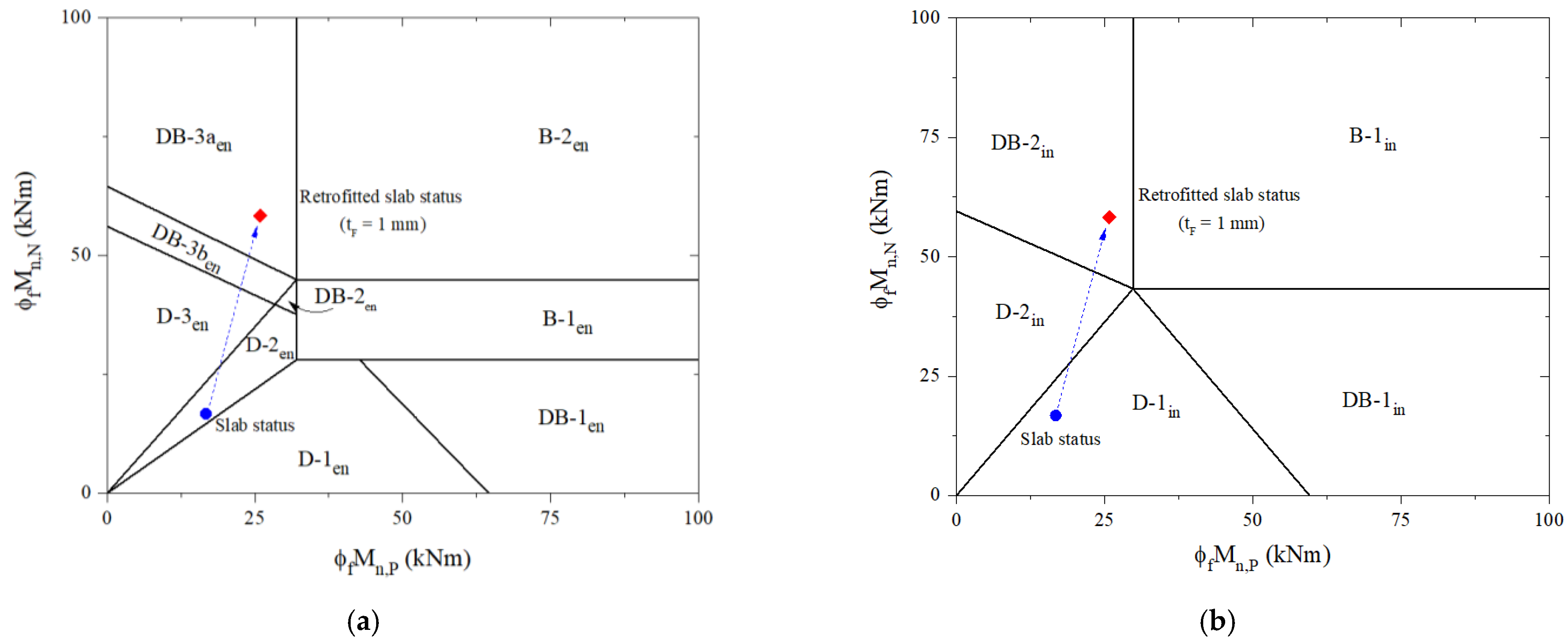
| 10. Define failure mode and failure load | DB-3aen according to Figure 6a Equation (18), = = 66.38 kN/m | DB-2in according to Figure 6b Equation (21), = = 82.68 kN/m |
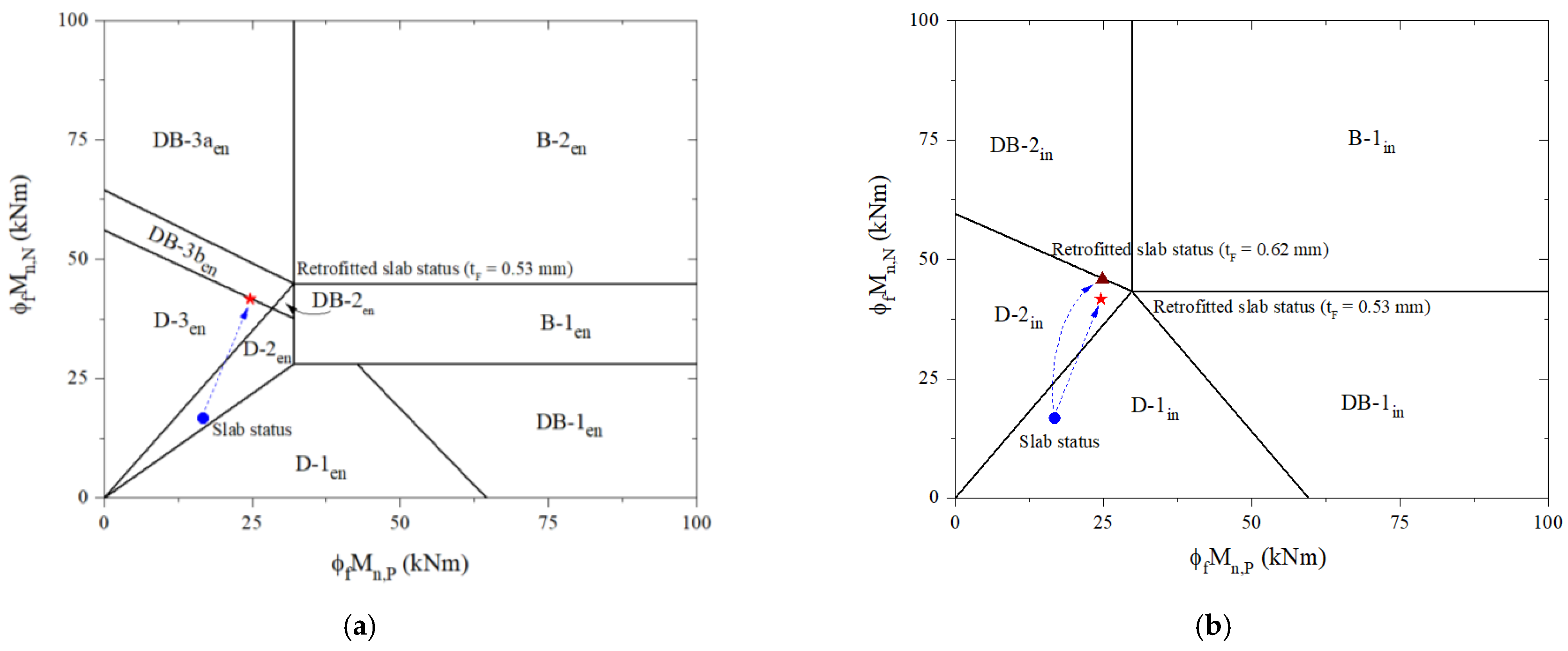
| Adjust iteratively CFRP thicknesses to achieve ductile failure mode | It can be achieved with tF = 0.53 mm; wu = 51.05 kN/m; failure mode D-3en; = 62.95 kN/m, as shown in Figure 9a. | It can be achieved with tF = 0.62 mm; wu = 68.92 kN/m; failure mode D-2in; = 82.38 kN/m. However, to be consistent with the end span, tF = 0.53 mm; wu = 68.17 kN/m; failure mode D-2in; = 76.48 kN/m, as shown in Figure 9b. |
| Span | Failure Mode | wu (kN/m) | wf (kN/m) | tF (mm) | ||
|---|---|---|---|---|---|---|
| Existing end span | D-2en | 24.70 | [100%] | 31.30 | [100%] | - |
| Retrofitted end span | D-3en | 51.05 | [207%] | 62.95 | [201%] | 0.53 |
| Existing interior span | D-1in | 32.00 | [100%] | 39.20 | [100%] | - |
| Retrofitted interior span | D-2in | 68.92 | [215%] | 82.83 | [211%] | 0.62 |
| Retrofitted interior span (for consistency) | D-2in | 68.17 | [213%] | 76.48 | [195%] | 0.53 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, H.Q.; Han, T.H.; Park, J.K.; Kim, J.J. A Design Process for Preventing Brittle Failure in Strengthening RC Slabs with Hybrid FRP-HPC Retrofit Systems. Materials 2023, 16, 755. https://doi.org/10.3390/ma16020755
Nguyen HQ, Han TH, Park JK, Kim JJ. A Design Process for Preventing Brittle Failure in Strengthening RC Slabs with Hybrid FRP-HPC Retrofit Systems. Materials. 2023; 16(2):755. https://doi.org/10.3390/ma16020755
Chicago/Turabian StyleNguyen, Huy Q., Taek Hee Han, Jun Kil Park, and Jung J. Kim. 2023. "A Design Process for Preventing Brittle Failure in Strengthening RC Slabs with Hybrid FRP-HPC Retrofit Systems" Materials 16, no. 2: 755. https://doi.org/10.3390/ma16020755
APA StyleNguyen, H. Q., Han, T. H., Park, J. K., & Kim, J. J. (2023). A Design Process for Preventing Brittle Failure in Strengthening RC Slabs with Hybrid FRP-HPC Retrofit Systems. Materials, 16(2), 755. https://doi.org/10.3390/ma16020755








