Molecular Dynamics Study on Crack Propagation in Al Containing Mg–Si Clusters Formed during Natural Aging
Abstract
:1. Introduction
2. Experimental Details
3. Molecular Dynamics (MD) Simulation
3.1. Interatomic Potential
3.2. Atomistic Modeling
3.3. Numerical Algorithm for Solving MD Equations
4. Results
5. Discussion
6. Conclusions
- -
- The cracked Al containing Mg–Si clusters exhibited earlier crack opening than that without Mg–Si clusters. The Al crack tip with the Mg–Si cluster emitted Shockley partial dislocations. The strains at which the crack opened and at which dislocations began to be emitted decreased as the natural aging proceeds.
- -
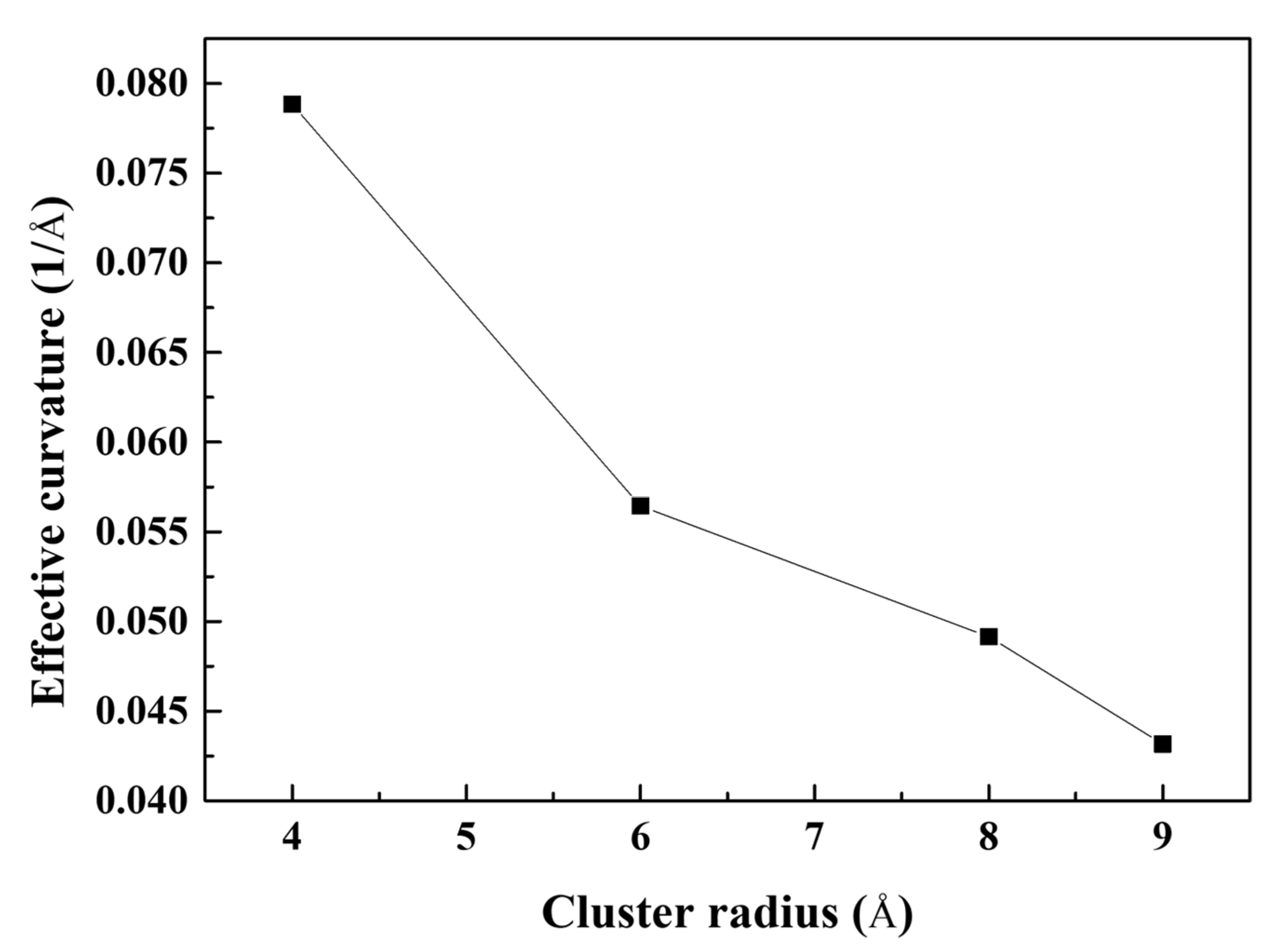
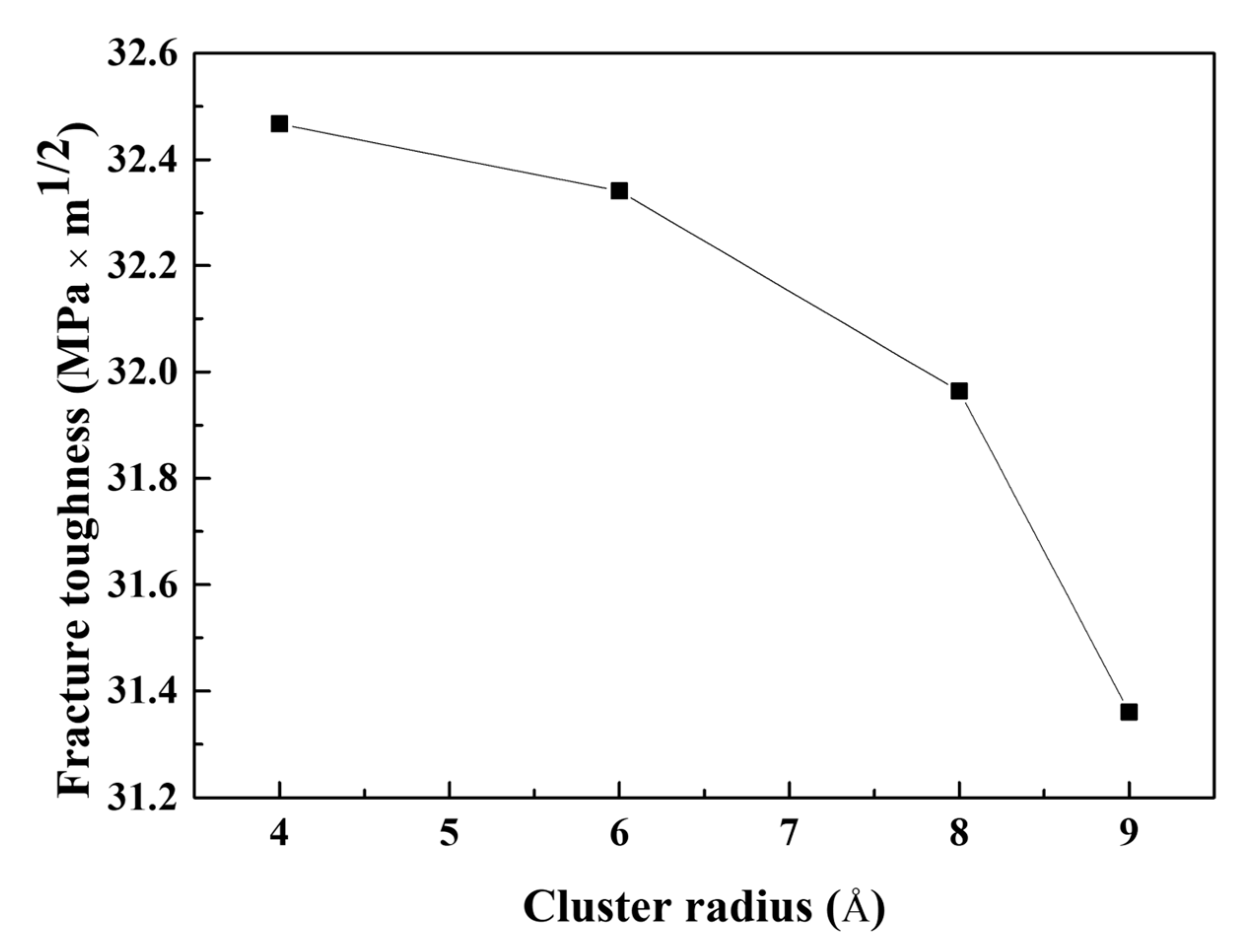
- The stress of the atoms near the crack tip for Al with larger Mg–Si clusters was more concentrated. This indicated a reduction in the effective curvature of the crack tip, which was comparable to the crack tip sharpening effect. This results from the competition between cack thinning and thickening forces applied on the solute atoms. Therefore, the crack spread more rapidly when the Mg–Si clusters were larger.
- -
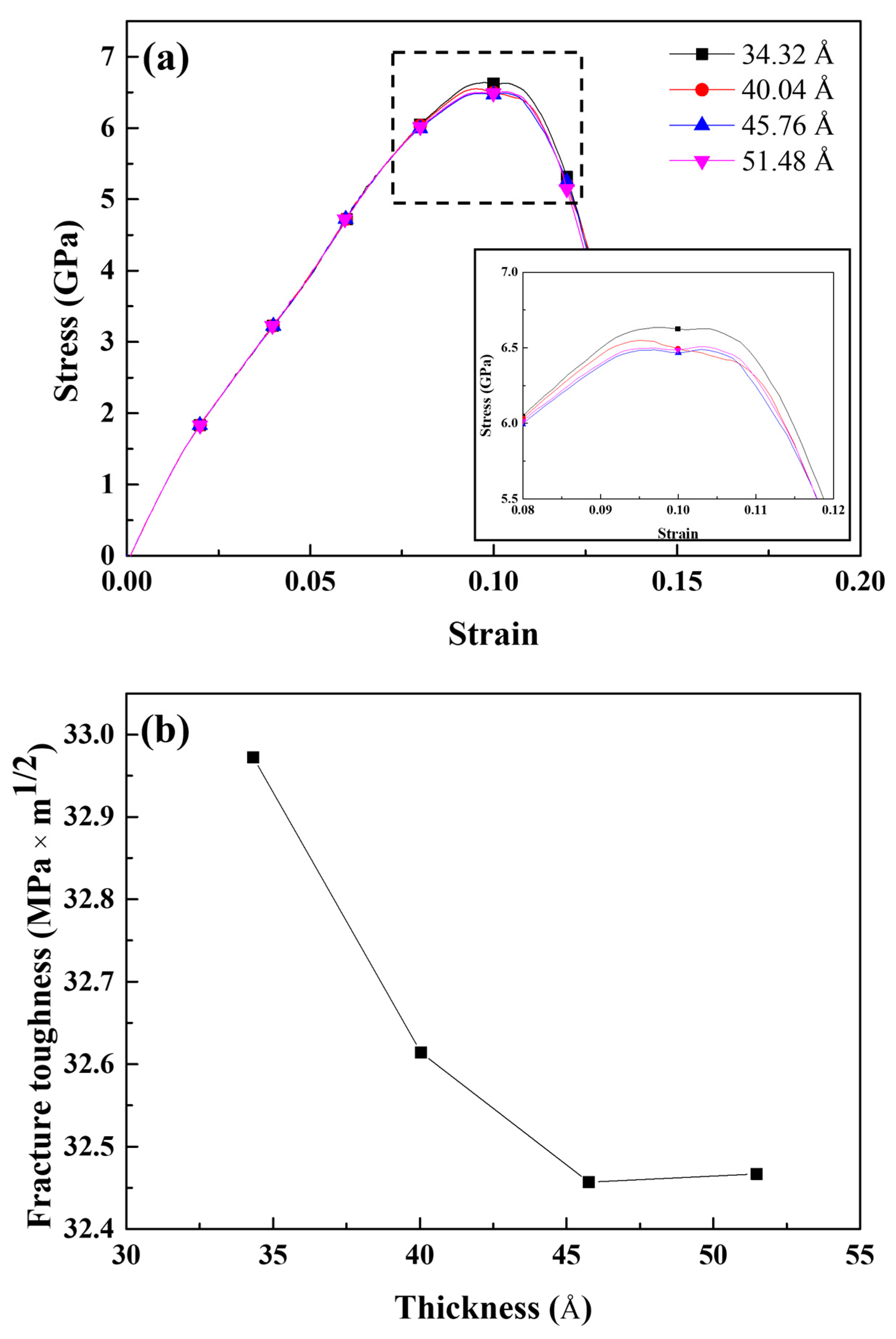
- The influence of geometrical factors was also analyzed. Owing to the crack tip sharpening effect, crack lengthening significantly contributed to decreasing the fracture toughness and tensile stress. The fracture toughness decreased as the simulation area thickness decreased; however, the magnitude of fracture toughness variation was less than that of crack lengthening.
- -
- Considering that Mg–Si clusters grow during natural aging, it is reasonable to conclude that long-term natural aging promotes rapid crack propagation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, J.; Tan, M.-J.; Jarfors, A.-E.W.; Aue-u-lan, Y.; Castagne, S. Formability in AA5083 and AA6016 alloys for light weight applications. Mater. Des. 2010, 31, S66–S70. [Google Scholar] [CrossRef]
- Zheng, K.; Zhu, L.; Lin, J.; Dean, T.A.; Li, N. An experimental investigation of the drawability of AA6082 sheet under different elevated temperature forming processes. J. Mater. Process. Technol. 2019, 273, 116225. [Google Scholar] [CrossRef]
- Ashby, M.F. Materials Selection in Mechanical Design, 4th ed.; Butterworth–Heinemann: Oxford, UK, 2010. [Google Scholar]
- Zhu, S.; Shih, H.-C.; Cui, X.; Yu, C.-Y.; Ringer, S.P. Design of solute clustering during thermomechanical processing of AA6016 Al–Mg–Si alloy. Acta Mater. 2021, 203, 116455. [Google Scholar] [CrossRef]
- Guo, M.X.; Zhang, Y.D.; Li, G.J.; Jin, S.B.; Sha, G.; Zhang, J.S.; Zhuang, L.Z.; Lavernia, E.J. Solute clustering in Al-Mg-Si-Cu-(Zn) alloys during aging. J. Alloys Compd. 2010, 774, 347–363. [Google Scholar] [CrossRef]
- Seyedrezai, H.; Grebennikov, D.; Mascher, P.; Zurob, H.S. Study of the early stages of clustering in Al–Mg–Si alloys using the electrical resistivity measurements. Mater. Sci. Eng. A 2009, 525, 186–191. [Google Scholar] [CrossRef]
- Werinos, M.; Antrekowitsch, H.; Ebner, T.; Prillhofer, R.; Uggowitzer, P.J.; Pogatscher, S. Hardening of Al–Mg–Si alloys: Effect of trace elements and prolonged natural aging. Mater. Des. 2016, 107, 257–268. [Google Scholar] [CrossRef]
- Aruga, Y.; Kim, S.; Kozuka, M.; Kobayashi, E.; Sato, T. Effects of cluster characteristics on two-step aging behavior in Al–Mg–Si alloys with different Mg/Si ratios and natural aging periods. Mater. Sci. Eng. A 2018, 718, 371–376. [Google Scholar] [CrossRef]
- Aruga, Y.; Kozuka, M.; Sato, T. Formulation of initial artificial age-hardening response in an Al–Mg–Si alloy based on the cluster classification using a high-detection-efficiency atom probe. J. Alloys Compd. 2018, 739, 1115–1123. [Google Scholar] [CrossRef]
- Fallah, V.; Langelier, B.; Ofori-Opoku, N.; Raeisinia, B.; Provatas, N.; Esmaeili, S. Cluster evolution mechanisms during aging in Al–Mg–Si alloys. Acta Mater. 2016, 103, 290–300. [Google Scholar] [CrossRef]
- Tao, G.H.; Liu, C.H.; Chen, J.H.; Lai, Y.X.; Ma, P.P.; Liu, L.M. The influence of Mg/Si ratio on the negative natural aging effect in Al–Mg–Si–Cu alloys. Mater. Sci. Eng. A 2015, 642, 241–248. [Google Scholar] [CrossRef]
- Gupta, A.K.; Lloyd, D.J.; Court, S.A. Precipitation hardening in Al–Mg–Si alloys with and without excess Si. Mater. Sci. Eng., A 2001, 316, 11–17. [Google Scholar] [CrossRef]
- Zhen, L.; Kang, S.B. The effect of pre-aging on microstructure and tensile properties of Al–Mg–Si alloys. Scr. Mater. 1997, 36, 1089–1094. [Google Scholar] [CrossRef]
- Zhu, S.; Li, Z.; Yan, L.; Li, X.; Huang, S.; Yan, H.; Zhang, Y.; Xiong, B. Natural aging behavior in pre-aged Al–Mg–Si–Cu alloys with and without Zn addition. J. Alloys Compd. 2019, 773, 496–502. [Google Scholar] [CrossRef]
- Fischer, F.D.; Svoboda, J.; Appel, A.; Kozeschnik, E. Modeling of excess vacancy annihilation at different types of sinks. Acta Mater. 2011, 59, 3463–3472. [Google Scholar] [CrossRef]
- Im, J.; Jeon, J.; Song, M.; Hong, S.; Kim, J. Influence of natural aging time and Mg/Si ratio (Mg + Si = 1.3 mass%) on the two-step aging behavior in Al–Mg–Si alloys. Met. Mater. Int. 2019, 25, 860–868. [Google Scholar] [CrossRef]
- Lados, D.A.; Apelian, D. Fatigue crack growth characteristics in cast Al–Si–Mg alloys, Part I. Effect of processing conditions and microstructure. Mater. Sci. Eng. A 2004, 385, 200–211. [Google Scholar]
- Wu, M.-Z.; Zhang, J.-W.; Zhang, Y.-B.; Wang, H.-Q. Effects of Mg content on the fatigue strength and fracture behavior of Al-Si-Mg casting alloys. J. Mater. Eng. Perform. 2018, 27, 5992–6003. [Google Scholar] [CrossRef]
- Bogdanoff, T.; Lattanzi, L.; Merlin, M.; Ghassemali, E.; Seifeddine, S. The influence of copper addition on crack initiation and propagation in an Al–Si–Mg alloy during cyclic testing. Materialia 2020, 12, 100787. [Google Scholar] [CrossRef]
- Lee, J.G. Computational Materials Science: An Introduction, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Cui, C.B.; Beom, H.G. Molecular dynamics simulations of edge cracks in copper and aluminum single crystals. Mater. Sci. Eng. A 2014, 609, 102–109. [Google Scholar] [CrossRef]
- Fang, W.; Xie, H.; Yin, F.; Li, J.; Khan, D.F.; Fang, Q. Molecular dynamics simulation of grain boundary geometry on crack propagation of bi-crystal aluminum. Mater. Sci. Eng. A 2016, 666, 314–319. [Google Scholar] [CrossRef]
- Ding, J.; Wang, L.-S.; Song, K.; Liu, B.; Huang, X. Molecular dynamics simulation of crack propagation in single-crystal aluminum plate with central cracks. J. Nanomater. 2017, 2017, 5181206. [Google Scholar] [CrossRef]
- Kedharnath, A.; Panwar, A.S.; Kapoor, R. Molecular dynamics simulation of the interaction of a nano-scale crack with grain boundaries in α-Fe. Comput. Mater. Sci. 2017, 137, 85–99. [Google Scholar] [CrossRef]
- Tang, T.; Kim, S.; Horstemeyer, M.F.; Wang, P. Atomistic modeling of crack growth in magnesium single crystal. Eng. Fract. Mech. 2011, 78, 191–201. [Google Scholar] [CrossRef]
- Sung, P.-H.; Chen, T.-C. Studies of crack growth and propagation of single-crystal nickel by molecular dynamics. Comput. Mater. Sci. 2015, 102, 151–158. [Google Scholar] [CrossRef]
- Qiu, R.-Z.; Li, C.-C.; Fang, T.-H. Mechanical properties and crack growth behavior of polycrystalline copper using molecular dynamics simulation. Phys. Scr. 2017, 92, 085702. [Google Scholar] [CrossRef]
- Chang, L.; Liu, X.; Zhao, J.; Zhou, C. Effect of interatomic potential on modelling fracture behavior in hcp titanium: A molecular dynamics study. J. Mater. Res. Technol. 2022, 17, 2118–2133. [Google Scholar] [CrossRef]
- Rajput, A.; Paul, S.K. Effect of soft and hard inclusions in tensile deformation and damage mechanism of Aluminum: A molecular dynamics study. J. Alloys Compd. 2021, 869, 159213. [Google Scholar] [CrossRef]
- Rafii-Tabar, H.; Shodja, H.M.; Darabi, M.; Dahi, A. Molecular dynamics simulation of crack propagation in fcc materials containing clusters of impurities. Mech. Mater. 2006, 38, 243–252. [Google Scholar] [CrossRef]
- Chandra, S.; Kumar, N.N.; Samal, M.K.; Chavan, V.M.; Patel, R.J. Molecular dynamics simulations of crack growth behavior in Al in the presence of vacancies. Comput. Mater. Sci. 2016, 117, 518–526. [Google Scholar] [CrossRef]
- Banhart, J.; Chang, C.S.T.; Liang, Z.; Wanderka, N.; Lay, M.D.H.; Hill, A.J. Natural aging in Al–Mg–Si alloys—A process of unexpected complexity. Adv. Eng. Mater. 2010, 12, 559–571. [Google Scholar] [CrossRef]
- Hirth, S.M.; Marshall, G.J.; Court, S.A.; Lloyd, D.J. Effects of Si on the aging behaviour and formability of aluminium alloys based on AA6016. Mater. Sci. Eng. A 2001, 319–321, 452–456. [Google Scholar] [CrossRef]
- Nandy, J.; Yedla, N.; Gupta, P.; Sarangi, H.; Sahoo, S. Sintering of AlSi10Mg particles in direct metal laser sintering process: A molecular dynamics simulation study. Mater. Chem. Phys. 2019, 236, 121803. [Google Scholar] [CrossRef]
- Mishin, Y.; Farkas, D.; Mehl, M.J.; Papaconstantopoulos, D.A. Interatomic potentials for monoatomic metals from experimental data and ab initio calculations. Phys. Rev. B 1999, 59, 3393–3407. [Google Scholar] [CrossRef] [Green Version]
- Sun, D.Y.; Mendelev, M.I.; Becker, C.A.; Kudin, K.; Haxhimali, T.; Asta, M.; Hoyt, J.J.; Karma, A.; Srolovitz, D.J. Crystal-melt interfacial free energies in hcp metals: A molecular dynamics study of Mg. Phys. Rev. B 2006, 73, 024116. [Google Scholar] [CrossRef]
- Daw, M.S.; Baskes, M.I. Semiempirical, quantum mechanical calculation of hydrogen embrittlement in metals. Phys. Rev. Lett. 1983, 50, 1285–1288. [Google Scholar] [CrossRef]
- Tersoff, J. New empirical model for the structural properties of silicon. Phys. Rev. Lett. 1986, 56, 632–635. [Google Scholar] [CrossRef]
- Galashev, A.; Ivanichkina, K.A. Computational study of the properties of silicon thin films on graphite. Russ. J. Phys. Chem. 2017, 91, 2448–2452. [Google Scholar] [CrossRef]
- Sanga, L.V.; Hoang, V.V.; Hang, N.T.T. Molecular dynamics simulation of melting of fcc Lennard-Jones nanoparticles. Eur. Phys. J. D 2013, 67, 64. [Google Scholar] [CrossRef]
- Plimptom, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Marioara, C.D.; Andersen, S.J.; Jansen, J.; Zandbergen, H.W. Atomic Model for GP-zones in a 6082 Al–Mg–Si system. Acta Mater. 2001, 49, 321–328. [Google Scholar] [CrossRef]
- Lee, S.; Kang, H.; Jeon, J.; Bae, D. Influence of the composition and vacancy concentration on cluster decomposition behavior in Al–Si–Mg alloy: A kinetic Monte Carlo study. Materials 2022, 15, 6552. [Google Scholar] [CrossRef] [PubMed]
- Swenson, R.J. Comments on virial theorems for bounded systems. Am. J. Phys. 1983, 51, 940. [Google Scholar] [CrossRef]
- Kelchner, C.L.; Plimpton, S.J.; Hamilton, J.C. Dislocation nucleation and defect structure during surface indentation. Phys. Rev. B 1998, 58, 11085–11088. [Google Scholar] [CrossRef]
- Ahmadi, M.R.; Povoden-Karadeniz, E.; Öksüz, K.I.; Falahati, A.; Kozeschnik, E. A model for precipitation strengthening in multi-particle systems. Comput. Mater. Sci. 2014, 91, 173–186. [Google Scholar] [CrossRef]
- Lianga, Z.; Chang, C.S.T.; Abromeit, C.; Banhart, J.; Hirsch, J. The kinetics of clustering in Al–Mg–Si alloys studied by Monte Carlo simulation. Int. J. Mater. Res. 2012, 103, 980–986. [Google Scholar] [CrossRef]
- De Vaucorbeil, A.; Sinclair, C.W.; Poole, W.J. Atomistic insights into cluster strengthening in aluminum alloys. Materialia 2018, 4, 566–574. [Google Scholar] [CrossRef] [Green Version]
- Dowling, N.E. Mechanical Behavior of Materials, 4th ed.; Pearson: New York, NY, USA, 2012. [Google Scholar]
- Courtney, T.H. Mechanical Behavior of Materials, 2nd ed.; Waveland Pr. Inc.: Long Grove, IL, USA, 2005. [Google Scholar]
- Moustabchir, H.; Arbaoui, J.; El Moussaid, M.; Azari, Z.; Pruncu, C.I. Characterization of fracture toughness properties of aluminium alloy for pipelines. Exp. Tech. 2018, 42, 593–604. [Google Scholar] [CrossRef]
- Kumar, M.; Londhe, N.V. Fracture toughness measurement of aluminium and steel inserted aluminium using numerical method. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1013, 012010. [Google Scholar] [CrossRef]
- Read, D.T.; Reed, R.P. Effects of specimen thickness on fracture toughness of an aluminum alloy. Int. J. Fract. 1977, 13, 201–213. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, L.K.; Zhang, B.; Chen, Y.Z.; Duan, S.Y.; Liu, G.; Yang, C.L.; Liu, F. Enhanced strength and ductility of A356 alloy due to composite effect of near-rapid solidification and thermo-mechanical treatment. Mater. Sci. Eng. A 2019, 753, 168–178. [Google Scholar] [CrossRef]
- Xu, W.F.; Liu, J.H.; Chen, D.L.; Luan, G.H.; Yao, J.S. Improvements of strength and ductility in aluminum alloy joints via rapid cooling during friction stir welding. Mater. Sci. Eng. A 2012, 548, 89–98. [Google Scholar] [CrossRef]
- Tsuji, N.; Ito, Y.; Saito, Y.; Minamino, Y. Strength and ductility of ultrafine grained aluminum and iron produced by ARB and annealing. Scr. Mater. 2002, 47, 893–899. [Google Scholar] [CrossRef]
- Samaee, M.; Najafi, S.; Eivani, A.R.; Jafarian, H.R.; Zhou, J. Simultaneous improvements of the strength and ductility of fine-grained AA6063 alloy with increasing number of ECAP passes. Mater. Sci. Eng. A 2016, 669, 350–357. [Google Scholar] [CrossRef] [Green Version]
- Pedersen, K.O.; Lademo, O.-G.; Berstad, T.; Furu, T.; Hopperstad, O.S. Influence of texture and grain structure on strain localisation and formability for AlMgSi alloys. J. Mater. Process. Technol. 2008, 200, 77–93. [Google Scholar] [CrossRef]
- Barnwal, V.K.; Raghavan, R.; Tewari, A.; Narasimhan, K.; Mishra, S.K. Effect of microstructure and texture on forming behaviour of AA-6061 aluminium alloy sheet. Mater. Sci. Eng. A 2017, 679, 56–65. [Google Scholar] [CrossRef]
- Jeon, J.G.; Shin, J.H.; Shin, S.E.; Bae, D.H. Improvement in the anisotropic mechanical properties and formability of Al–Si–Mg–Cu-based alloy sheets. Mater. Sci. Eng. A 2021, 799, 140199. [Google Scholar] [CrossRef]
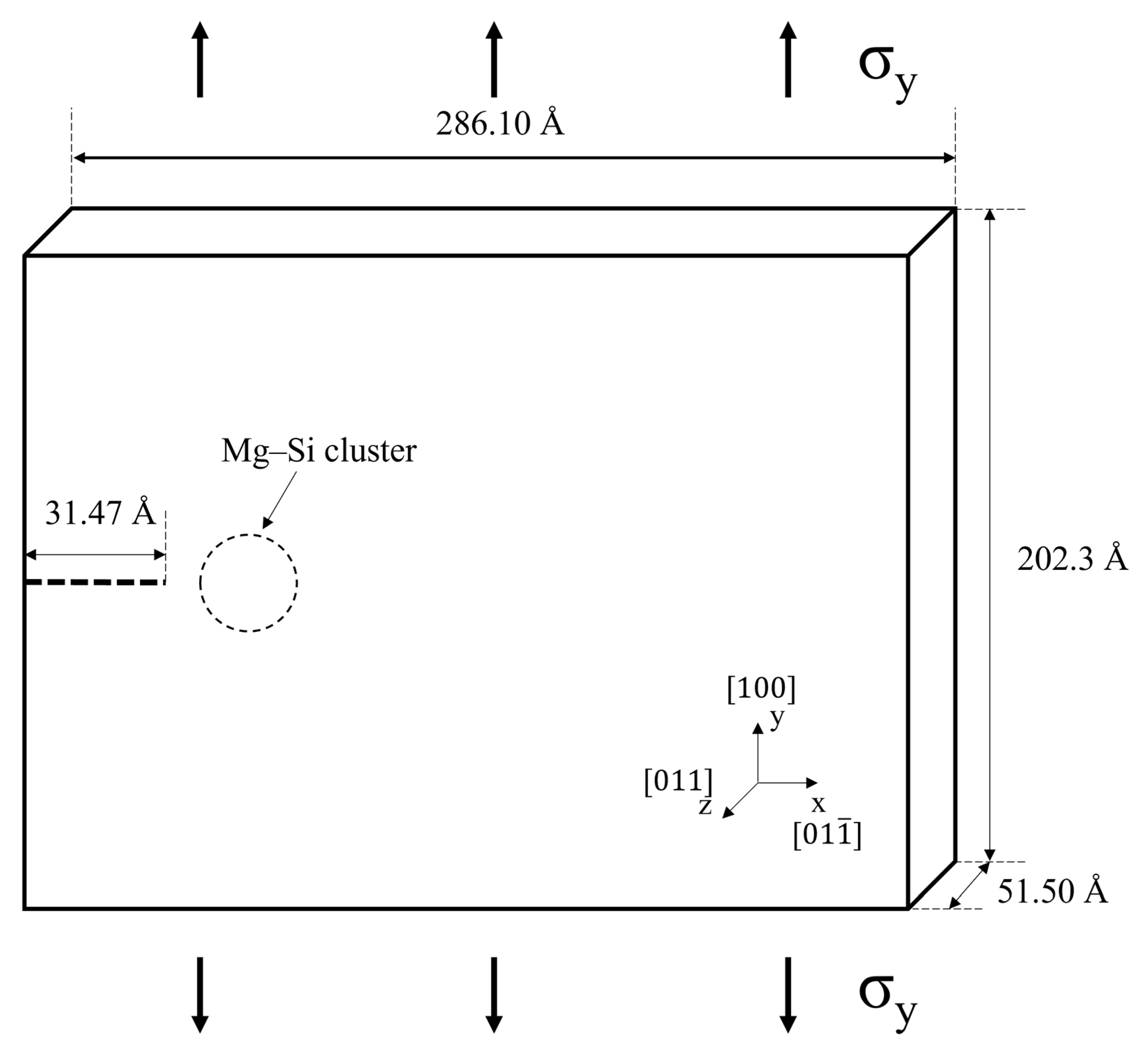
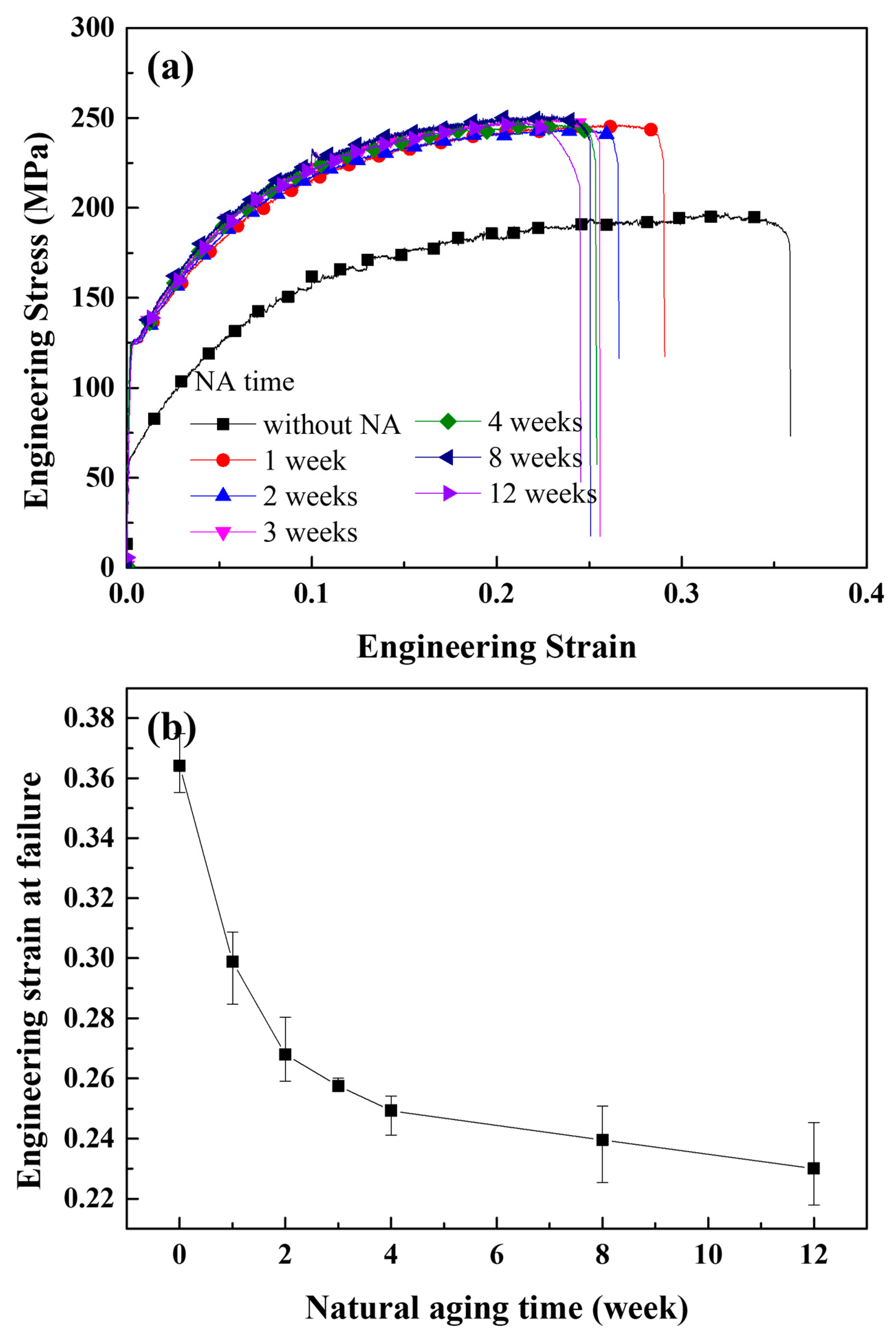
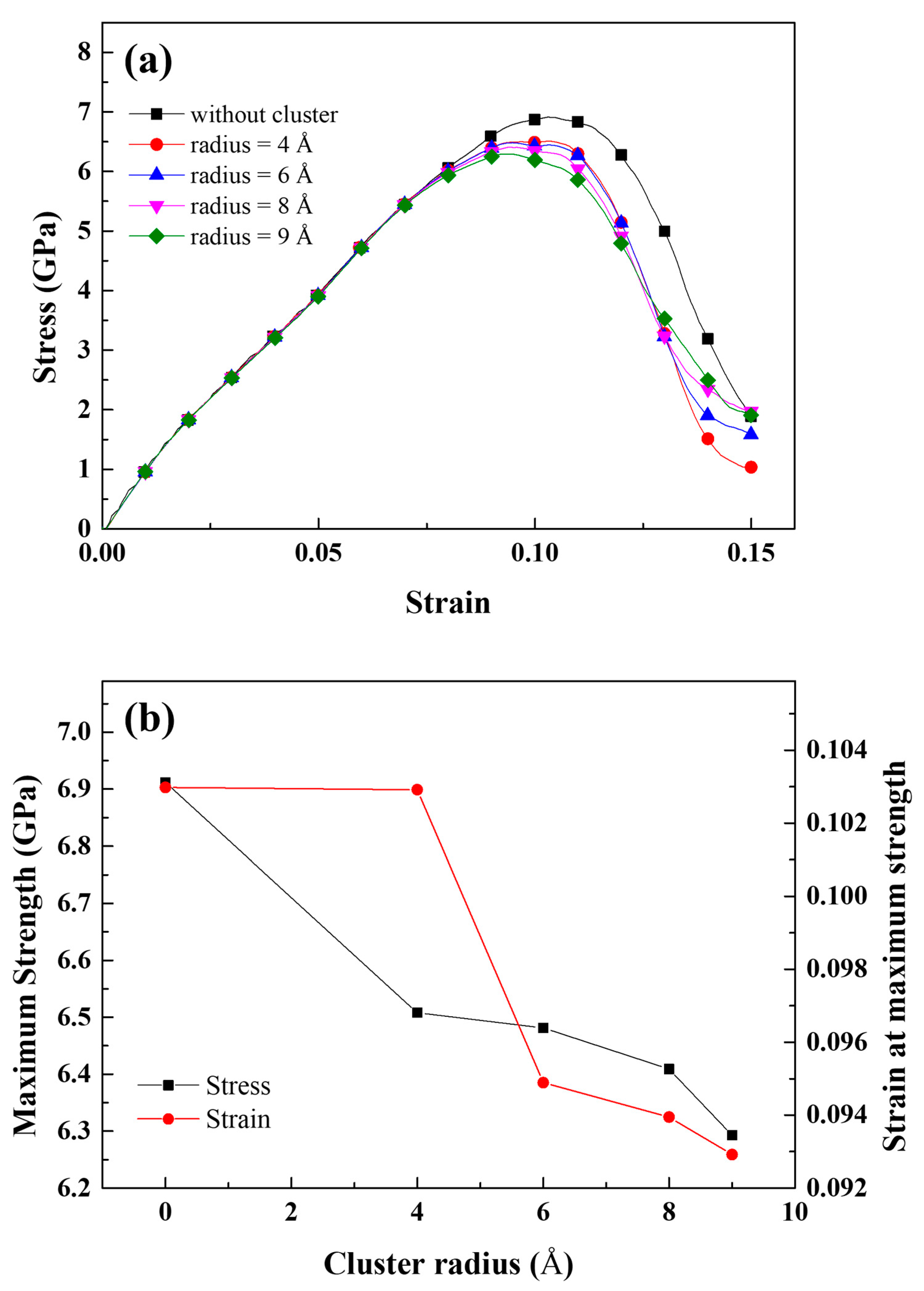
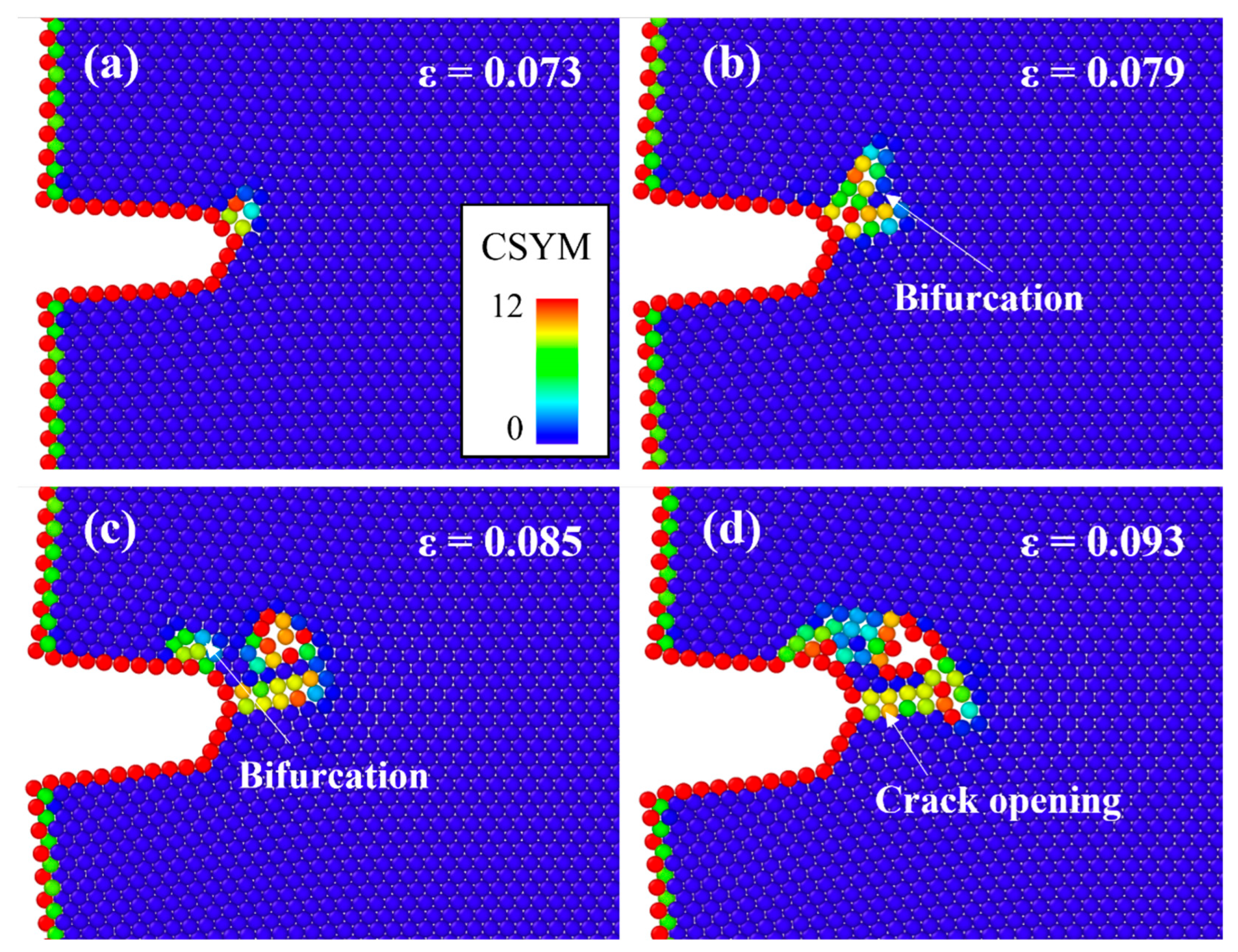
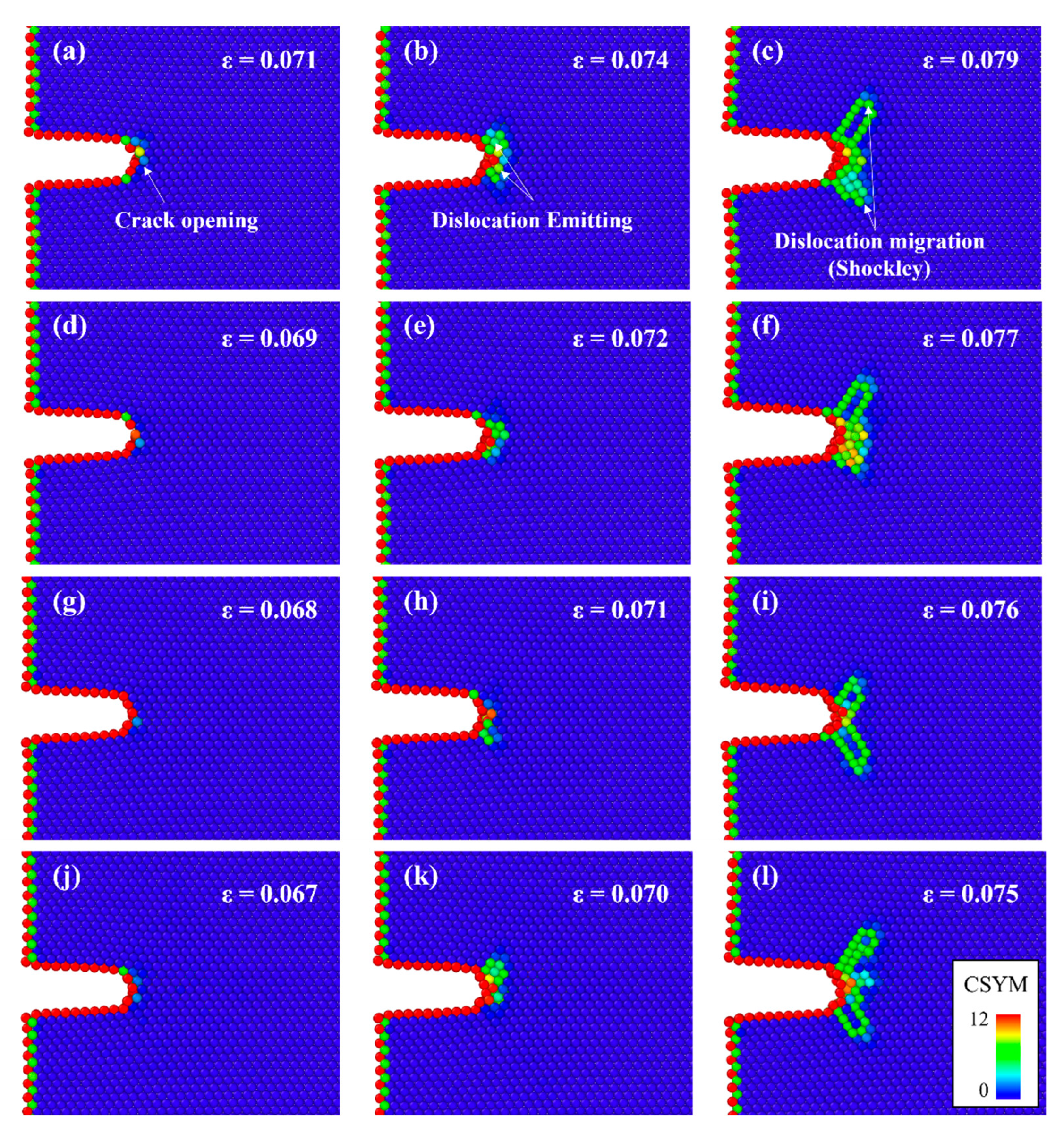
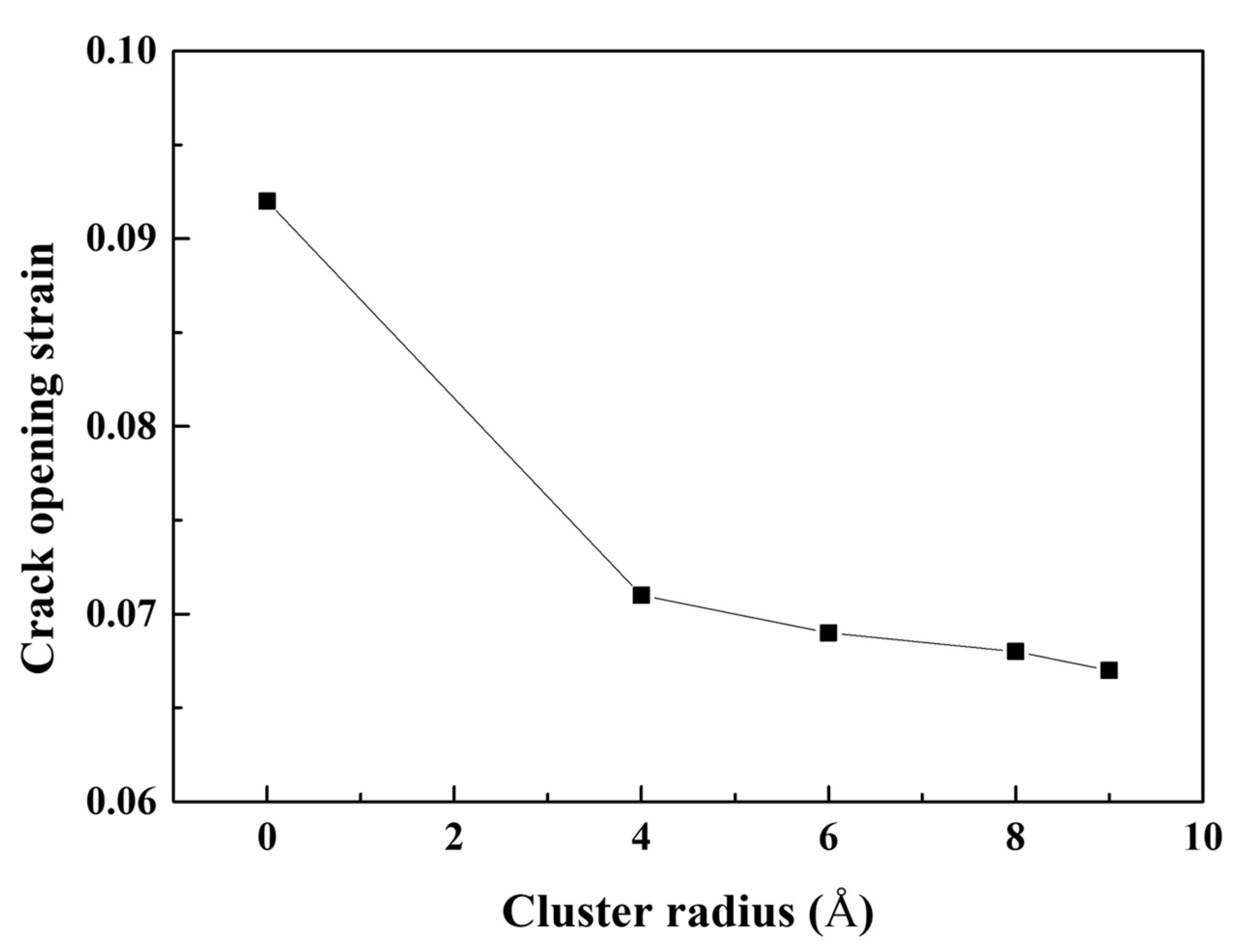
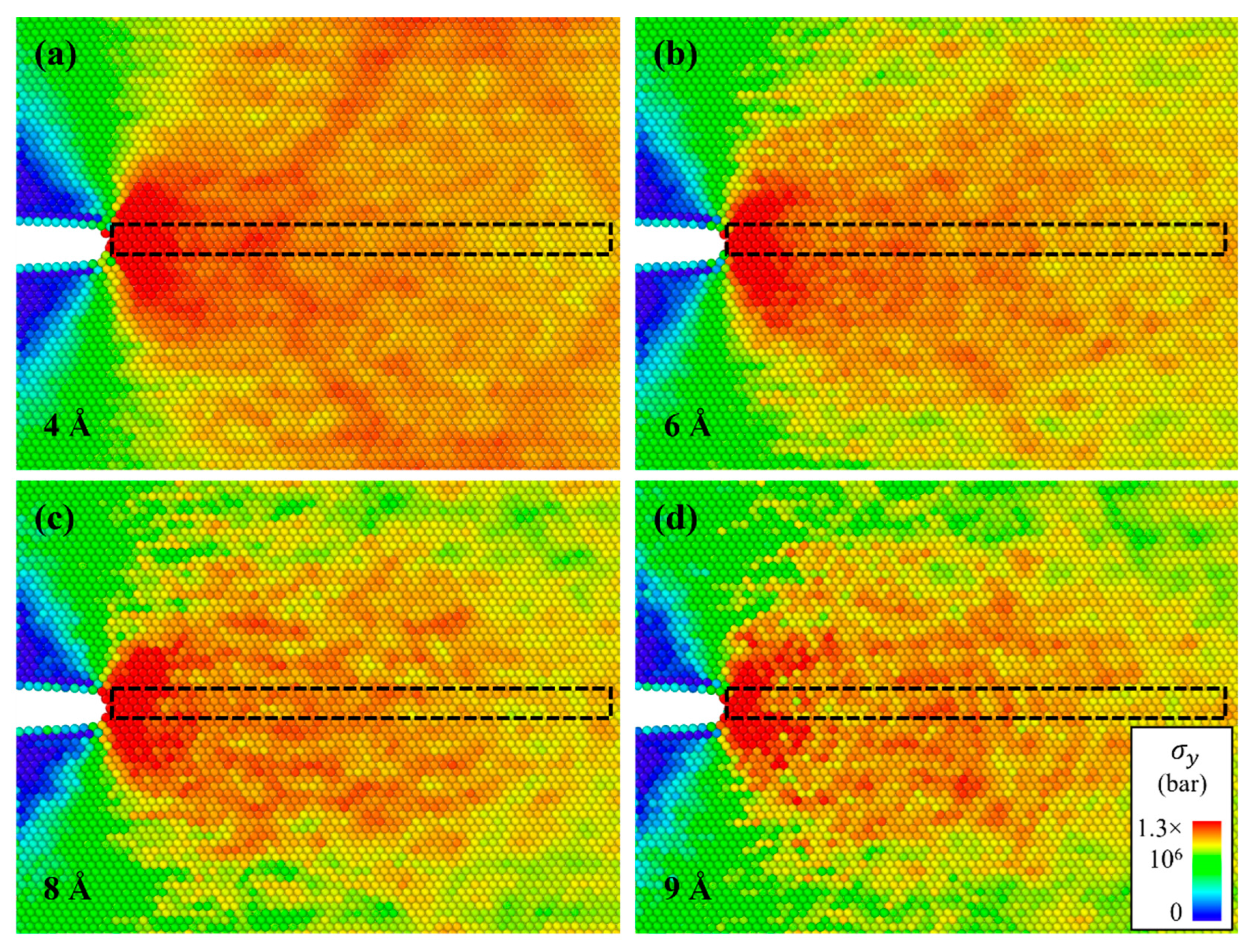
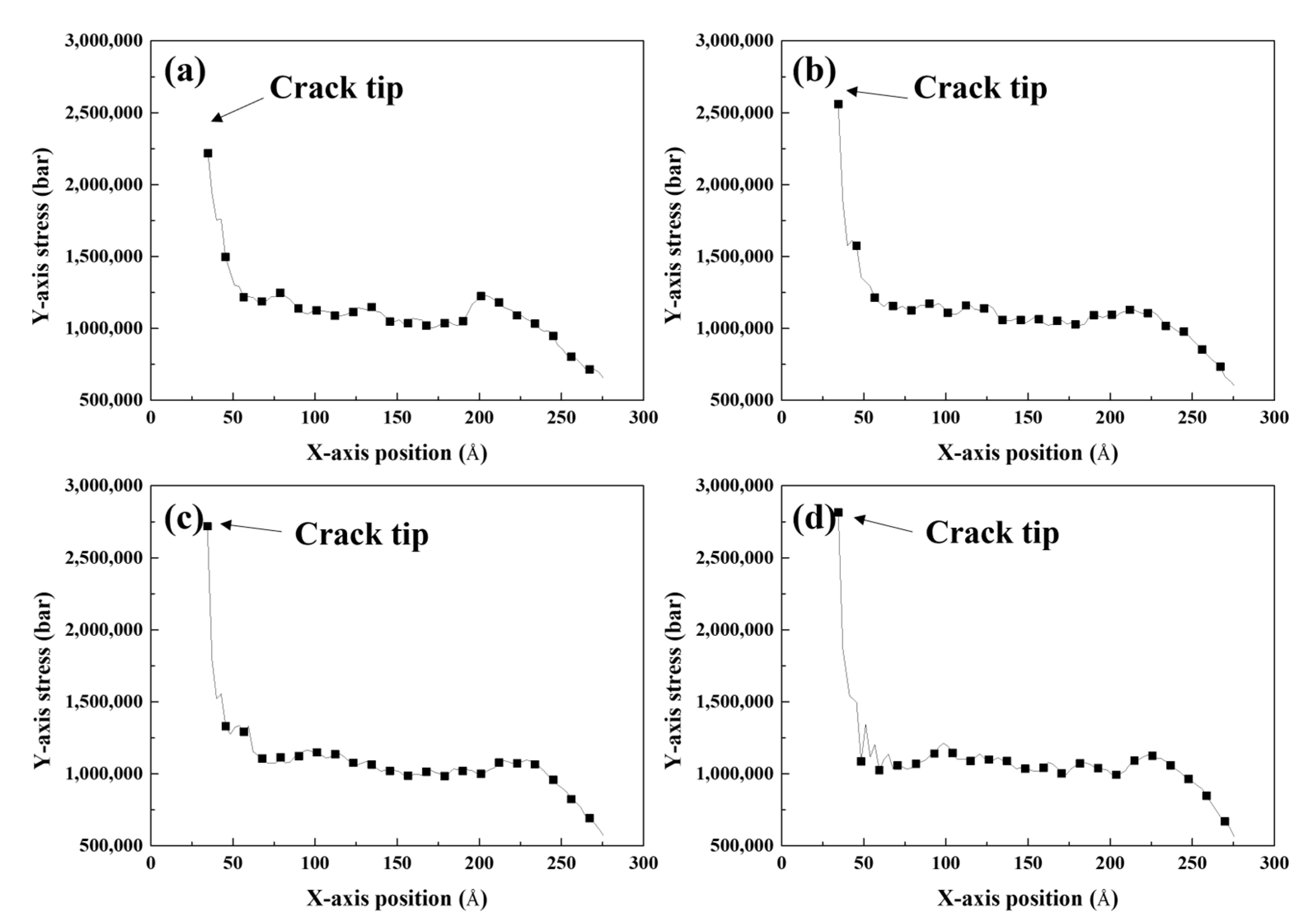



| Parameter | Value |
|---|---|
| εAl–Si (eV) | 0.75 |
| εAl–Mg (eV) | 0.3 |
| εMg–Si (eV) | 0.3 |
| σAl–Si (eV) | 1.0 |
| σAl–Mg (eV) | 1.0 |
| σMg–Si (eV) | 0.75 |
| rc (Cutoff radius for the calculation of the LJ potential) (Å) | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, S.; Kang, H.; Bae, D. Molecular Dynamics Study on Crack Propagation in Al Containing Mg–Si Clusters Formed during Natural Aging. Materials 2023, 16, 883. https://doi.org/10.3390/ma16020883
Lee S, Kang H, Bae D. Molecular Dynamics Study on Crack Propagation in Al Containing Mg–Si Clusters Formed during Natural Aging. Materials. 2023; 16(2):883. https://doi.org/10.3390/ma16020883
Chicago/Turabian StyleLee, Sangjun, Heon Kang, and Donghyun Bae. 2023. "Molecular Dynamics Study on Crack Propagation in Al Containing Mg–Si Clusters Formed during Natural Aging" Materials 16, no. 2: 883. https://doi.org/10.3390/ma16020883
APA StyleLee, S., Kang, H., & Bae, D. (2023). Molecular Dynamics Study on Crack Propagation in Al Containing Mg–Si Clusters Formed during Natural Aging. Materials, 16(2), 883. https://doi.org/10.3390/ma16020883









