Fused Deposition Modelling (FDM) of Thermoplastic-Based Filaments: Process and Rheological Properties—An Overview
Abstract
1. Introduction
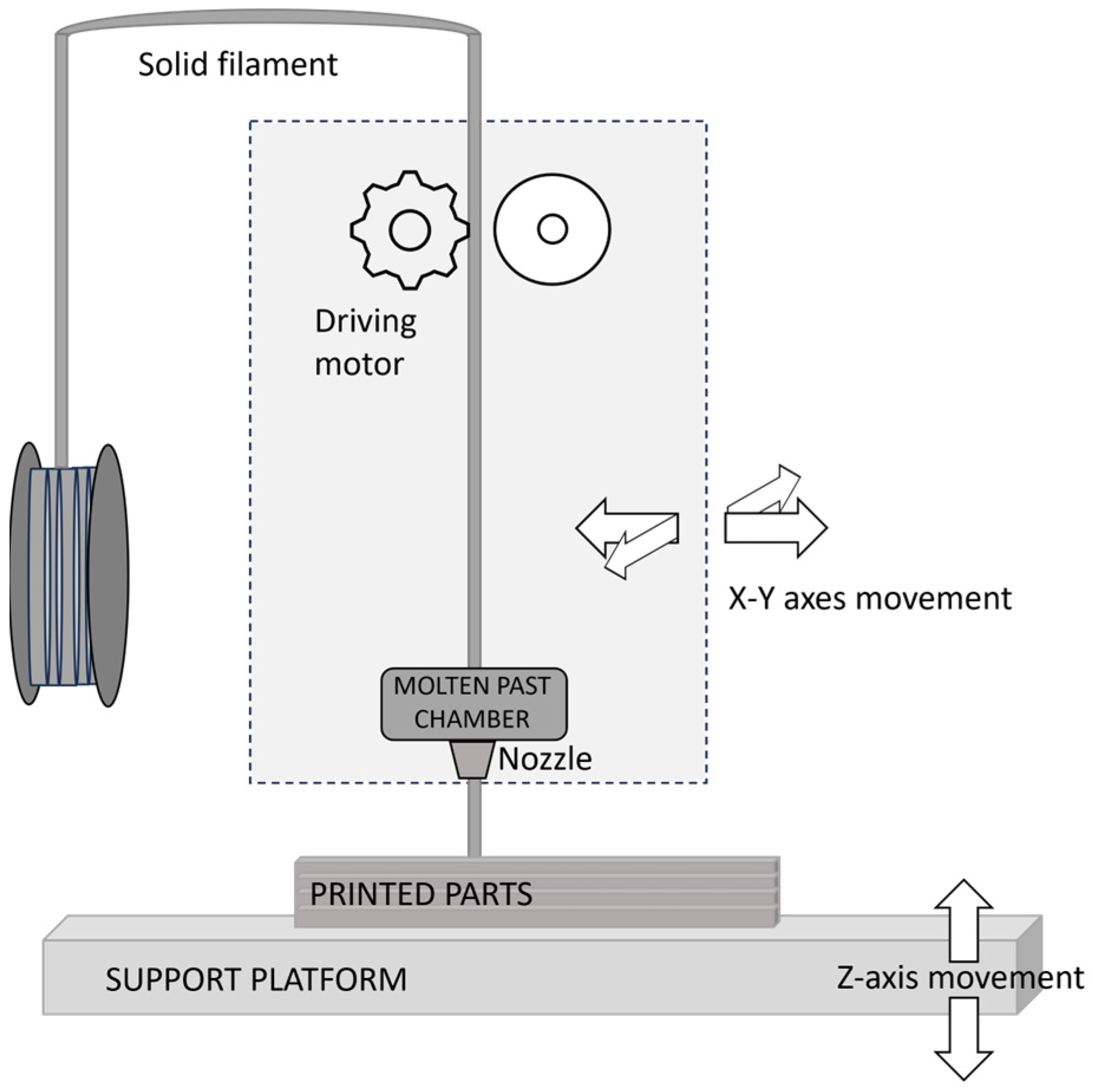
2. Fused Deposition Modelling (FDM) Technology of Thermoplastic-Based Filaments
3. Rheology in the Processing of Polymer-Based Materials
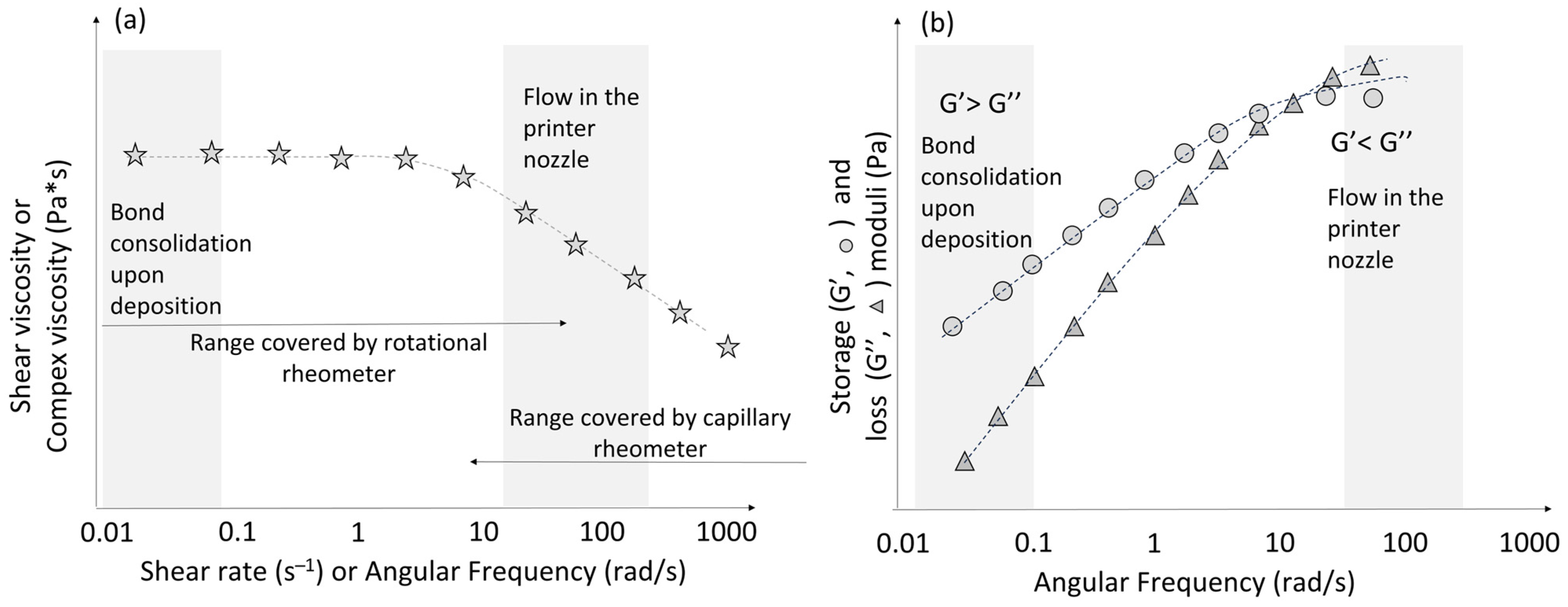
Modelling of Viscosity Data
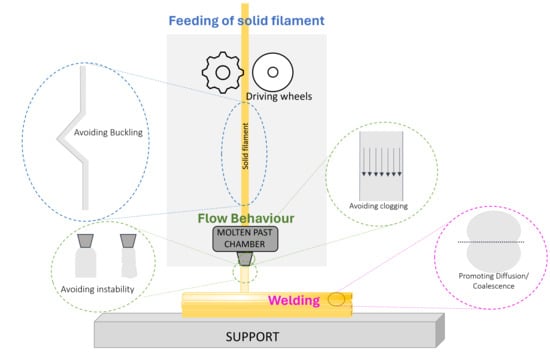
4. Rheology in the FDM Process
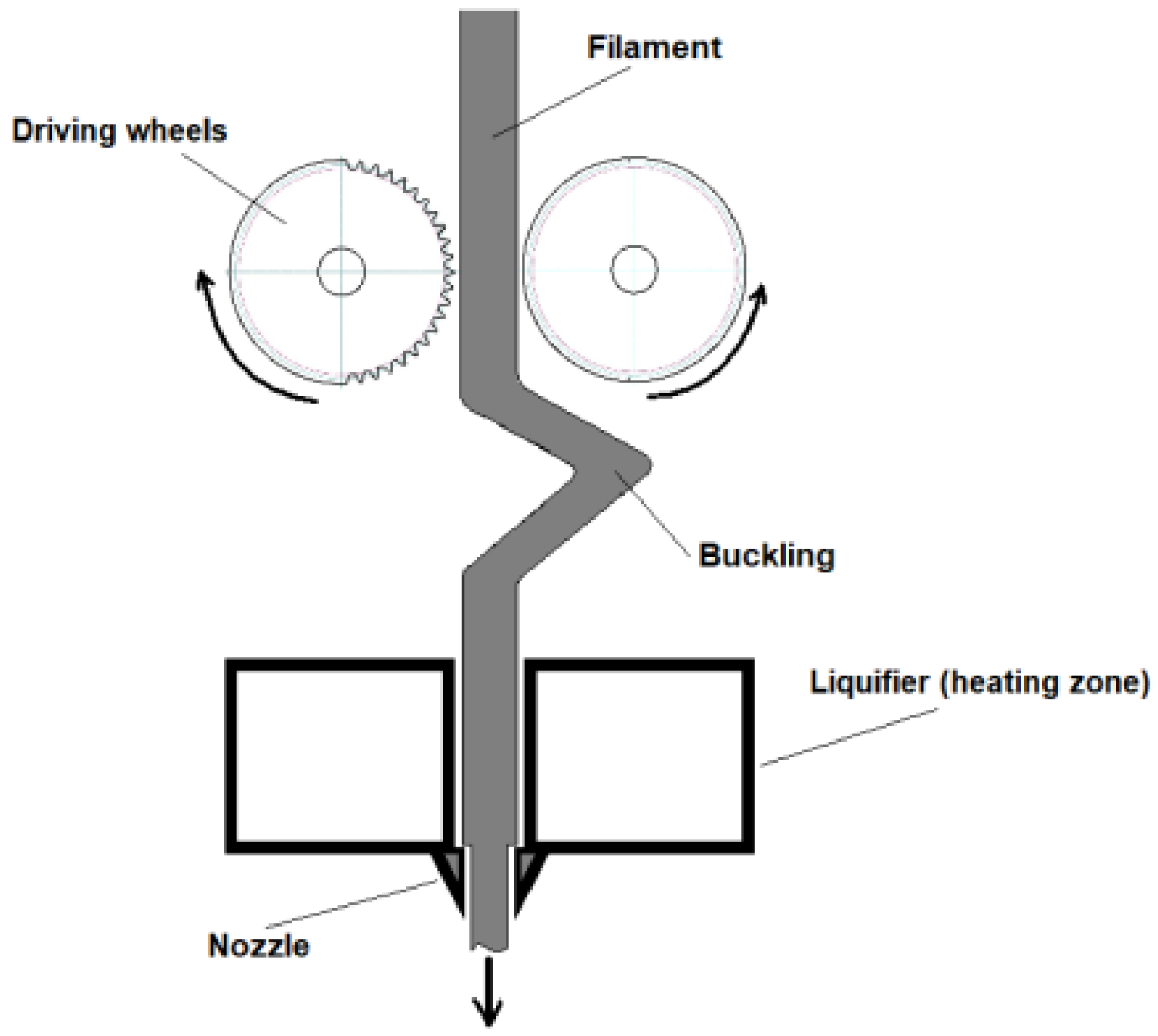
4.1. Buckling
4.2. Pressure-Driven Extrusion
4.3. Flow Instability
4.4. Welding
5. Rheological Measurements to Attest the Printability of Polymer-Based Materials
6. Challenges and Future Perspectives
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Negi, S.; Kumar Sharma, R.; Dhiman, S. Basics, applications and future of additive manufacturing technologies: A review. J. Manuf. Technol. Res. 2013, 5, 75–96. [Google Scholar]
- ISO/ASTM 52900; Additive Manufacturing—General Principles—Terminology. ASTM International: West Conshohocken, PA, USA, 2022.
- Bikas, H.; Koutsoukos, S.; Stavropoulos, P. A decision support method for evaluation and process selection of Additive Manufacturing. Procedia CIRP 2019, 81, 1107–1112. [Google Scholar] [CrossRef]
- Najmon, J.C.; Raeisi, S.; Tovar, A. Review of additive manufacturing technologies and applications in the aerospace industry. In Additive Manufacturing for the Aerospace Industry; Froes, F.H., Boyer, R., Eds.; Elsevier Inc.: Amsterdam, The Netherlands, 2019; pp. 7–31. ISBN 9780128140635. [Google Scholar]
- Al Rashid, A.; Khan, S.A.; Al-Ghamdi, S.G.; Koç, M. Additive manufacturing: Technology, applications, markets, and opportunities for the built environment. Autom. Constr. 2020, 118, 103268. [Google Scholar] [CrossRef]
- Kumar, R.; Kumar, M.; Chohan, J.S. The role of additive manufacturing for biomedical applications: A critical review. J. Manuf. Process. 2021, 64, 828–850. [Google Scholar] [CrossRef]
- Leal, R.; Barreiros, F.M.; Alves, L.; Romeiro, F.; Vasco, J.C.; Santos, M.; Marto, C. Additive manufacturing tooling for the automotive industry. Int. J. Adv. Manuf. Technol. 2017, 92, 1671–1676. [Google Scholar] [CrossRef]
- Andre, J.-C.; Le Mehaute, A.; Witte, O. De Device for Producing a Model of an Industrial Part. FR2567668A1, 17 January 1986. Available online: https://patents.google.com/patent/FR2567668A1/en (accessed on 10 December 2023).
- Charles, W. Hull Apparatus for Production of Three-Dimensional Objects by Stereolithography. U.S. Patent US4575330A, 19 December 1989. [Google Scholar]
- Ryder, M.A.; Lados, D.A.; Iannacchione, G.S.; Peterson, A.M. Fabrication and properties of novel polymer-metal composites using fused deposition modeling. Compos. Sci. Technol. 2018, 158, 43–50. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, J.; Zeng, H. Polymer processing and rheology. In Polymer Science and Nanotechnology: Fundamentals and Applications; Narain, R., Ed.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 149–178. ISBN 9780128168066. [Google Scholar]
- Mohd Pu’ad, N.A.S.; Abdul Haq, R.H.; Mohd Noh, H.; Abdullah, H.Z.; Idris, M.I.; Lee, T.C. Review on the fabrication of fused deposition modelling (FDM) composite filament for biomedical applications. Mater. Today Proc. 2020, 29, 228–232. [Google Scholar] [CrossRef]
- Solomon, I.J.; Sevvel, P.; Gunasekaran, J. A review on the various processing parameters in FDM. Mater. Today Proc. 2021, 37, 509–514. [Google Scholar] [CrossRef]
- Rajan, K.; Samykano, M.; Kadirgama, K.; Harun, W.S.W.; Rahman, M.M. Fused deposition modeling: Process, materials, parameters, properties, and applications. Int. J. Adv. Manuf. Technol. 2022, 120, 1531–1570. [Google Scholar] [CrossRef]
- Kumar, R.; Kumar, M.; Chohan, J.S. Material-specific properties and applications of additive manufacturing techniques: A comprehensive review. Bull. Mater. Sci. 2021, 44, 1–19. [Google Scholar] [CrossRef]
- Mohan, N.; Senthil, P.; Vinodh, S.; Jayanth, N. A review on composite materials and process parameters optimisation for the fused deposition modelling process. Virtual Phys. Prototyp. 2017, 12, 47–59. [Google Scholar] [CrossRef]
- Nurhudan, A.I.; Supriadi, S.; Whulanza, Y.; Saragih, A.S. Additive manufacturing of metallic based on extrusion process: A review. J. Manuf. Process. 2021, 66, 228–237. [Google Scholar] [CrossRef]
- Dadkhah, M.; Tulliani, J.M.; Saboori, A.; Iuliano, L. Additive manufacturing of ceramics: Advances, challenges, and outlook. J. Eur. Ceram. Soc. 2023, 43, 6635–6664. [Google Scholar] [CrossRef]
- Wu, H.; Fahy, W.P.; Kim, S.; Kim, H.; Zhao, N.; Pilato, L.; Kafi, A.; Bateman, S.; Koo, J.H. Recent developments in polymers/polymer nanocomposites for additive manufacturing. Prog. Mater. Sci. 2020, 111, 100638. [Google Scholar] [CrossRef]
- Sodeifian, G.; Ghaseminejad, S.; Yousefi, A.A. Preparation of polypropylene/short glass fiber composite as Fused Deposition Modeling (FDM) filament. Results Phys. 2019, 12, 205–222. [Google Scholar] [CrossRef]
- Le, A.D.; Akué Asséko, A.C.; Cosson, B.; Krawczak, P. Investigating the Effect of Interface Temperature on Molecular Interdiffusion during Laser Transmission Welding of 3D-Printed Composite Parts. Materials 2023, 16, 6121. [Google Scholar] [CrossRef] [PubMed]
- Bi, X.; Huang, R. 3D printing of natural fiber and composites: A state-of-the-art review. Mater. Des. 2022, 222, 111065. [Google Scholar] [CrossRef]
- Angelopoulos, P.M.; Samouhos, M.; Taxiarchou, M. Functional fillers in composite filaments for fused filament fabrication; a review. Mater. Today Proc. 2021, 37, 4031–4043. [Google Scholar] [CrossRef]
- Patti, A.; Acierno, S.; Cicala, G.; Zarrelli, M.; Acierno, D. Recovery of Waste Material from Biobags: 3D Printing Process and Thermo-Mechanical Characteristics in Comparison to Virgin and Composite Matrices. Polymers 2022, 14, 1943. [Google Scholar] [CrossRef]
- Candal, M.V.; Calafel, I.; Fernández, M.; Aranburu, N.; Aguirresarobe, R.H.; Gerrica-Echevarria, G.; Santamaría, A.; Müller, A.J. Study of the interlayer adhesion and warping during material extrusion-based additive manufacturing of a carbon nanotube/biobased thermoplastic polyurethane nanocomposite. Polymer 2021, 224, 123734. [Google Scholar] [CrossRef]
- Srinivas, V.; van Hooy-Corstjens, C.S.J.; Rastogi, S.; Harings, J.A.W. Promotion of molecular diffusion and/or crystallization in fused deposition modeled poly(lactide) welds. Polymer 2020, 202, 122637. [Google Scholar] [CrossRef]
- Gao, J.; Hao, M.; Wang, Y.; Kong, X.; Yang, B.; Wang, R.; Lu, Y.; Zhang, L.; Gong, M.; Zhang, L.; et al. 3D printing boron nitride nanosheets filled thermoplastic polyurethane composites with enhanced mechanical and thermal conductive properties. Addit. Manuf. 2022, 56, 102897. [Google Scholar] [CrossRef]
- Das, A.; Etemadi, M.; Davis, B.A.; McKnight, S.H.; Williams, C.B.; Case, S.W.; Bortner, M.J. Rheological investigation of nylon-carbon fiber composites fabricated using material extrusion-based additive manufacturing. Polym. Compos. 2021, 42, 6010–6024. [Google Scholar] [CrossRef]
- Vaes, D.; Van Puyvelde, P. Semi-crystalline feedstock for filament-based 3D printing of polymers. Prog. Polym. Sci. 2021, 118, 101411. [Google Scholar] [CrossRef]
- Ho, Q.B.; Kontopoulou, M. Improving the adhesion and properties in the material extrusion of polypropylene by blending with a polyolefin elastomer. Addit. Manuf. 2022, 55, 102818. [Google Scholar] [CrossRef]
- Gomes, T.E.; Cadete, M.S.; Dias-de-Oliveira, J.; Neto, V. Controlling the properties of parts 3D printed from recycled thermoplastics: A review of current practices. Polym. Degrad. Stab. 2022, 196, 109850. [Google Scholar] [CrossRef]
- Mishra, V.; Negi, S.; Kar, S. FDM-based additive manufacturing of recycled thermoplastics and associated composites. J. Mater. Cycles Waste Manag. 2023, 25, 758–784. [Google Scholar] [CrossRef] [PubMed]
- Patti, A.; Acierno, S.; Cicala, G.; Acierno, D. Changes in Chemical and Physical Properties of Bio-based Thermoplastics During Fused Deposition Modelling (FDM) Process. Chem. Eng. Trans. 2023, 100, 439–444. [Google Scholar] [CrossRef]
- Wang, P.; Zou, B.; Ding, S.; Li, L.; Huang, C. Effects of FDM-3D printing parameters on mechanical properties and microstructure of CF/PEEK and GF/PEEK. Chin. J. Aeronaut. 2021, 34, 236–246. [Google Scholar] [CrossRef]
- Wang, P.; Zou, B.; Xiao, H.; Ding, S.; Huang, C. Effects of printing parameters of fused deposition modeling on mechanical properties, surface quality, and microstructure of PEEK. J. Mater. Process. Technol. 2019, 271, 62–74. [Google Scholar] [CrossRef]
- Garcia-Leiner, M.; Streifel, B.; Başgül, C.; MacDonald, D.W.; Kurtz, S.M. Characterization of polyaryletherketone (PAEK) filaments and printed parts produced by extrusion-based additive manufacturing. Polym. Int. 2021, 70, 1128–1136. [Google Scholar] [CrossRef]
- Kennedy, Z.C.; Christ, J.F.; Fenn, M.D.; Zhong, L.; Chouyyok, W.; Arnold, A.M.; Denny, A.C.; Albrecht, A.M.; Silverstein, J.A.; Erikson, R.L.; et al. Mica filled polyetherketoneketones for material extrusion 3D printing. Addit. Manuf. 2022, 49, 102492. [Google Scholar] [CrossRef]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena; John Wiley & Sons, Inc.: New York City, NY, USA, 2002. [Google Scholar]
- Bird, R.B.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids; John Wiley & Sons, Inc.: New York City, NY, USA, 1987. [Google Scholar]
- Polychronopoulos, N.D.; Vlachopoulos, J. Polymer Processing and Rheology. In Functional Polymers; Mazumder, M.A.J., Sheardown, H., Al-Ahmed, A., Eds.; Springer: New York City, NY, USA, 2019; pp. 1–47. [Google Scholar]
- Carnicer, V.; Alcázar, C.; Orts, M.J.; Sánchez, E.; Moreno, R. Microfluidic rheology: A new approach to measure viscosity of ceramic suspensions at extremely high shear rates. Open Ceram. 2021, 5, 100052. [Google Scholar] [CrossRef]
- Vlachopoulos, J.; Polychronopoulos, N.D. Understanding Rheology and Technology of Polymer Extrusion; Polydynamics Inc.: Dundas, ON, Canada, 2019. [Google Scholar]
- Elbadawi, M. Polymeric Additive Manufacturing: The Necessity and Utility of Rheology. In Polymer Rheology; Rivera-Armenta, O.L., Cruz, B.A.S., Eds.; IntechOpen: London, UK, 2018; pp. 43–63. ISBN 978-1-78984-002-5. [Google Scholar]
- Patti, A.; Acierno, S.; Cicala, G.; Acierno, D. Predicting the Printability of Poly(Lactide) Acid Filaments in Fused Deposition Modeling (FDM) Technology: Rheological Measurements and Experimental Evidence. ChemEngineering 2022, 7, 1. [Google Scholar] [CrossRef]
- Kulicke, W.-M.; Porter, R.S. Relation between steady shear flow and dynamic rheology. Rheol. Acta 1980, 19, 601–605. [Google Scholar] [CrossRef]
- Ritzen, L.; Montano, V.; Garcia, S.J. 3D Printing of a Self-Healing Thermoplastic Polyurethane through FDM: From Polymer Slab to Mechanical Assessment. Polymers 2021, 13, 305. [Google Scholar] [CrossRef] [PubMed]
- Esposito Corcione, C.; Gervaso, F.; Scalera, F.; Montagna, F.; Sannino, A.; Maffezzoli, A. The feasibility of printing polylactic acid–nanohydroxyapatite composites using a low-cost fused deposition modeling 3D printer. J. Appl. Polym. Sci. 2017, 134, 44656. [Google Scholar] [CrossRef]
- Bermudez, D.; Quiñonez, P.A.; Vasquez, E.J.; Carrete, I.A.; Word, T.J.; Roberson, D.A. A Comparison of the physical properties of two commercial 3D printing PLA grades. Virtual Phys. Prototyp. 2021, 16, 178–195. [Google Scholar] [CrossRef]
- Candal, M.V.; Calafel, I.; Aranburu, N.; Fernández, M.; Gerrica-Echevarria, G.; Santamaría, A.; Müller, A.J. Thermo-rheological effects on successful 3D printing of biodegradable polyesters. Addit. Manuf. 2020, 36, 101408. [Google Scholar] [CrossRef]
- Das, A.; Gilmer, E.L.; Biria, S.; Bortner, M.J. Importance of Polymer Rheology on Material Extrusion Additive Manufacturing: Correlating Process Physics to Print Properties. ACS Appl. Polym. Mater. 2021, 3, 1218–1249. [Google Scholar] [CrossRef]
- Nguyen, N.A.; Bowland, C.C.; Naskar, A.K. A general method to improve 3D-printability and inter-layer adhesion in lignin-based composites. Appl. Mater. Today 2018, 12, 138–152. [Google Scholar] [CrossRef]
- Khaliq, M.H.; Gomes, R.; Fernandes, C.; Nóbrega, J.; Carneiro, O.S.; Ferrás, L.L. On the use of high viscosity polymers in the fused filament fabrication process. Rapid Prototyp. J. 2017, 23, 727–735. [Google Scholar] [CrossRef]
- Zhao, Y.Q.; Yang, J.H.; Ding, X.; Ding, X.; Duan, S.; Xu, F.J. Polycaprolactone/polysaccharide functional composites for low-temperature fused deposition modelling. Bioact. Mater. 2020, 5, 185–191. [Google Scholar] [CrossRef] [PubMed]
- Calafel, I.; Aguirresarobe, R.H.; Peñas, M.I.; Santamaria, A.; Tierno, M.; Conde, J.I.; Pascual, B. Searching for Rheological Conditions for FFF 3D Printing with PVC Based Flexible Compounds. Materials 2020, 13, 178. [Google Scholar] [CrossRef] [PubMed]
- Prasong, W.; Muanchan, P.; Ishigami, A.; Thumsorn, S.; Kurose, T.; Ito, H. Properties of 3D Printable Poly(lactic acid)/Poly(butylene adipate-co-terephthalate) Blends and Nano Talc Composites. J. Nanomater. 2020, 2020, 8040517. [Google Scholar] [CrossRef]
- Mohammed, A.H.; Kovacev, N.; Elshaer, A.; Melaibari, A.A.; Iqbal, J.; Hassanin, H.; Essa, K.; Memić, A. Preparation of Polylactic Acid/Calcium Peroxide Composite Filaments for Fused Deposition Modelling. Polymers 2023, 15, 2229. [Google Scholar] [CrossRef] [PubMed]
- Smirnov, A.; Seleznev, A.; Peretyagin, P.; Bentseva, E.; Pristinskiy, Y.; Kuznetsova, E.; Grigoriev, S. Rheological Characterization and Printability of Polylactide (PLA)-Alumina (Al2O3) Filaments for Fused Deposition Modeling (FDM). Materials 2022, 15, 8399. [Google Scholar] [CrossRef]
- Sanchez, L.C.; Beatrice, C.A.G.; Lotti, C.; Marini, J.; Bettini, S.H.P.; Costa, L.C. Rheological approach for an additive manufacturing printer based on material extrusion. Int. J. Adv. Manuf. Technol. 2019, 105, 2403–2414. [Google Scholar] [CrossRef]
- Chen, J.; Smith, D.E. Filament rheological characterization for fused filament fabrication additive manufacturing: A low-cost approach. Addit. Manuf. 2021, 47, 102208. [Google Scholar] [CrossRef]
- Ahmad, M.N.; Ishak, M.R.; Taha, M.M.; Mustapha, F.; Leman, Z. Rheological and Morphological Properties of Oil Palm Fiber-Reinforced Thermoplastic Composites for Fused Deposition Modeling (FDM). Polymers 2021, 13, 3739. [Google Scholar] [CrossRef]
- Henry, S.; Samaro, A.; Marchesini, F.H.; Shaqour, B.; Macedo, J.; Vanhoorne, V.; Vervaet, C. Extrusion-based 3D printing of oral solid dosage forms: Material requirements and equipment dependencies. Int. J. Pharm. 2021, 598, 120361. [Google Scholar] [CrossRef] [PubMed]
- Seggiani, M.; Altieri, R.; Puccini, M.; Stefanelli, E.; Esposito, A.; Castellani, F.; Stanzione, V.; Vitolo, S. Polycaprolactone-collagen hydrolysate thermoplastic blends: Processability and biodegradability/compostability. Polym. Degrad. Stab. 2018, 150, 13–24. [Google Scholar] [CrossRef]
- Patti, A.; Cicala, G.; Acierno, S. Rotational Rheology of Wood Flour Composites Based on Recycled Polyethylene. Polymers 2021, 13, 2226. [Google Scholar] [CrossRef] [PubMed]
- Lacambra-Andreu, X.; Morelle, X.P.; Maazouz, A.; Chenal, J.M.; Lamnawar, K. Rheological investigation and modeling of healing properties during extrusion-based 3D printing of poly(lactic-acid). Rheol. Acta 2023, 62, 31–44. [Google Scholar] [CrossRef]
- Balani, S.B.; Mokhtarian, H.; Salmi, T.; Coatanéa, E. An Investigation of the Influence of Viscosity and Printing Parameters on the Extrudate Geometry in the Material Extrusion Process. Polymers 2023, 15, 2202. [Google Scholar] [CrossRef] [PubMed]
- Ivanova, R.; Kotsilkova, R. Rheological study of poly(lactic) acid nanocomposites with carbon nanotubes and graphene additives as a tool for materials characterization for 3D printing application. Appl. Rheol. 2018, 28, 201854014. [Google Scholar] [CrossRef]
- Thumsorn, S.; Prasong, W.; Kurose, T.; Ishigami, A.; Kobayashi, Y.; Ito, H. Rheological Behavior and Dynamic Mechanical Properties for Interpretation of Layer Adhesion in FDM 3D Printing. Polymers 2022, 14, 2721. [Google Scholar] [CrossRef]
- Esmi, A.; Jahani, Y.; Yousefi, A.A.; Zandi, M. PMMA-CNT-HAp nanocomposites optimized for 3D-printing applications. Mater. Res. Express 2019, 6, 085405. [Google Scholar] [CrossRef]
- Stanciu, N.V.; Stan, F.; Fetecau, C.; Susac, F. On the Feasibility of Printing 3D Composite Objects Based on Polypropylene/Multi-walled Carbon Nanotubes. MATEC Web Conf. 2019, 290, 03017. [Google Scholar] [CrossRef][Green Version]
- Duty, C.; Ajinjeru, C.; Kishore, V.; Compton, B.; Hmeidat, N.; Chen, X.; Liu, P.; Hassen, A.A.; Lindahl, J.; Kunc, V. What makes a material printable? A viscoelastic model for extrusion-based 3D printing of polymers. J. Manuf. Process. 2018, 35, 526–537. [Google Scholar] [CrossRef]
- Riecker, S.; Hein, S.; Studnitzky, T.; Andersen, O.; Kieback, B. 3D printing of metal parts by means of fused filament fabrication—A non beam-based approach. In Proceedings of the Euro PM 2017 Congress & Exhibition, Milan, Italy, 1–5 October 2017. [Google Scholar]
- Olesik, P.; Godzierz, M.; Kozioł, M. Preliminary Characterization of Novel LDPE-Based Wear-Resistant Composite Suitable for FDM 3D Printing. Materials 2019, 12, 2520. [Google Scholar] [CrossRef] [PubMed]
- Shaqour, B.; Samaro, A.; Verleije, B.; Beyers, K.; Vervaet, C.; Cos, P. Production of Drug Delivery Systems Using Fused Filament Fabrication: A Systematic Review. Pharmaceutics 2020, 12, 517. [Google Scholar] [CrossRef] [PubMed]
- Achilleos, E.C.; Georgiou, G.; Hatzikiriakos, S.G. Role of processing aids in the extrusion of molten polymers. J. Vinyl Addit. Technol. 2002, 8, 7–24. [Google Scholar] [CrossRef]
- Agassant, J.F.; Arda, D.R.; Combeaud, C.; Merten, A.; Münstedt, H.; Mackley, M.R.; Robert, L.; Vergnes, B. Polymer processing extrusion instabilities and methods for their elimination or minimisation. Int. Polym. Process. 2006, 21, 239–255. [Google Scholar] [CrossRef]
- Bakrani Balani, S.; Chabert, F.; Nassiet, V.; Cantarel, A. Influence of printing parameters on the stability of deposited beads in fused filament fabrication of poly(lactic) acid. Addit. Manuf. 2019, 25, 112–121. [Google Scholar] [CrossRef]
- Wang, K. Die Swell of Complex Polymeric Systems. In Viscoelasticity from Theory to Biological Applications; de Vicente, J., Ed.; IntechOpen: London, UK, 2012; pp. 77–96. [Google Scholar]
- Colon, A.R.; Kazmer, D.O.; Peterson, A.M.; Seppala, J.E. Characterization of die-swell in thermoplastic material extrusion. Addit. Manuf. 2023, 73, 103700. [Google Scholar] [CrossRef]
- Nisticò, R. Polyethylene terephthalate (PET) in the packaging industry. Polym. Test. 2020, 90, 106707. [Google Scholar] [CrossRef]
- Zawaski, C.E.; Wilts, E.M.; Chatham, C.A.; Stevenson, A.T.; Pekkanen, A.M.; Li, C.; Tian, Z.; Whittington, A.R.; Long, T.E.; Williams, C.B. Tuning the material properties of a water-soluble ionic polymer using different counterions for material extrusion additive manufacturing. Polymer 2019, 176, 283–292. [Google Scholar] [CrossRef]
- Garzon-Hernandez, S.; Garcia-Gonzalez, D.; Jérusalem, A.; Arias, A. Design of FDM 3D printed polymers: An experimental-modelling methodology for the prediction of mechanical properties. Mater. Des. 2020, 188, 108414. [Google Scholar] [CrossRef]
- Chaunier, L.; Duchemin, B.; Couallier, E.; Valle, G.D.; Lourdin, D.; Reguerre, A.L.; Leroy, E. Fusion-bonding behavior of plasticized corn proteins in fused deposition modeling process. AIP Conf. Proc. 2019, 2065, 030055. [Google Scholar] [CrossRef]
- Bellehumeur, C.T.; Kontopoulou, M.; Vlachopoulos, J. The role of viscoelasticity in polymer sintering. Rheol. Acta 1998, 37, 270–278. [Google Scholar] [CrossRef]
- Gurrala, P.K.; Regalla, S.P. Part strength evolution with bonding between filaments in fused deposition modelling. Virtual Phys. Prototyp. 2014, 9, 141–149. [Google Scholar] [CrossRef]
- Yin, J.; Lu, C.; Fu, J.; Huang, Y.; Zheng, Y. Interfacial bonding during multi-material fused deposition modeling (FDM) process due to inter-molecular diffusion. Mater. Des. 2018, 150, 104–112. [Google Scholar] [CrossRef]
- Gao, X.; Qi, S.; Kuang, X.; Su, Y.; Li, J.; Wang, D. Fused filament fabrication of polymer materials: A review of interlayer bond. Addit. Manuf. 2021, 37, 101658. [Google Scholar] [CrossRef]
- McIlroy, C.; Olmsted, P.D. Disentanglement effects on welding behaviour of polymer melts during the fused-filament-fabrication method for additive manufacturing. Polymer 2017, 123, 376–391. [Google Scholar] [CrossRef]
- Naqi, A.; Swain, Z.; Mackay, M.E. Dual Material Fused Filament Fabrication via Core-Shell Die Design. ACS Appl. Polym. Mater. 2023, 5, 2481–2489. [Google Scholar] [CrossRef]
- Srinivas, V.; van Hooy-Corstjens, C.S.J.; Harings, J.A.W. Correlating molecular and crystallization dynamics to macroscopic fusion and thermodynamic stability in fused deposition modeling; a model study on polylactides. Polymer 2018, 142, 348–355. [Google Scholar] [CrossRef]
- Coogan, T.J.; Kazmer, D.O. Prediction of interlayer strength in material extrusion additive manufacturing. Addit. Manuf. 2020, 35, 101368. [Google Scholar] [CrossRef]
- Coogan, T.J.; Kazmer, D.O. Modeling of interlayer contact and contact pressure during fused filament fabrication. J. Rheol. 2019, 63, 655–672. [Google Scholar] [CrossRef]
- Shahriar, B.B.; France, C.; Valerie, N.; Arthur, C.; Christian, G. Toward improvement of the properties of parts manufactured by FFF (fused filament fabrication) through understanding the influence of temperature and rheological behaviour on the coalescence phenomenon. AIP Conf. Proc. 2017, 1896, 040008. [Google Scholar] [CrossRef]
- Patti, A.; Acierno, S.; Cicala, G.; Acierno, D. Evaluating Thermal Ageing of Recycled Bio-based Filament for 3D Printing as Function of Extruder Temperature. Chem. Eng. Trans. 2023, 98, 57–62. [Google Scholar] [CrossRef]
- Patti, A.; Acierno, S.; Cicala, G.; Tuccitto, N.; Acierno, D. Refining the 3D Printer Set-up to Reduce the Environmental Impact of the Fused Deposition Modelling (fdm) Technology. Chem. Eng. Trans. 2022, 91, 415–420. [Google Scholar] [CrossRef]
- Ferreira, R.T.L.; Amatte, I.C.; Dutra, T.A.; Bürger, D. Experimental characterization and micrography of 3D printed PLA and PLA reinforced with short carbon fibers. Compos. Part B Eng. 2017, 124, 88–100. [Google Scholar] [CrossRef]
- Mustapha, K.B.; Metwalli, K.M. A review of fused deposition modelling for 3D printing of smart polymeric materials and composites. Eur. Polym. J. 2021, 156, 110591. [Google Scholar] [CrossRef]
- Samy, A.A.; Golbang, A.; Harkin-Jones, E.; Archer, E.; Tormey, D.; McIlhagger, A. Finite element analysis of residual stress and warpage in a 3D printed semi-crystalline polymer: Effect of ambient temperature and nozzle speed. J. Manuf. Process. 2021, 70, 389–399. [Google Scholar] [CrossRef]
- Frunzaverde, D.; Cojocaru, V.; Ciubotariu, C.-R.; Miclosina, C.-O.; Ardeljan, D.D.; Ignat, E.F.; Marginean, G. The Influence of the Printing Temperature and the Filament Color on the Dimensional Accuracy, Tensile Strength, and Friction Performance of FFF-Printed PLA Specimens. Polymers 2022, 14, 1978. [Google Scholar] [CrossRef] [PubMed]
- Patti, A.; Acierno, S.; Cicala, G.; Acierno, D. Recycling Waste from Film Packaging to 3D Printing Applications: A Prospective Study to Identify the Processing Temperature. Chem. Eng. Trans. 2022, 96, 55–60. [Google Scholar] [CrossRef]
- Guo, Z.; Peng, X.; Sang, L.; Guo, Z.; Peng, X.; Sang, L. Enhancement of Mechanical Properties of PA6 Blending with Talcum for Fused Deposition Modeling. Macromol. Mater. Eng. 2020, 305, 2000355. [Google Scholar] [CrossRef]
- Torabi, H.; McGreal, H.; Zarrin, H.; Behzadfar, E. Effects of Rheological Properties on 3D Printing of Poly(lactic acid) (PLA) and Poly(hydroxy alkenoate) (PHA) Hybrid Materials. ACS Appl. Polym. Mater. 2023, 5, 4034–4044. [Google Scholar] [CrossRef]
- Kovalcik, A.; Smilek, J.; Machovsky, M.; Kalina, M.; Enev, V.; Dugova, H.; Cernekova, N.; Kovacova, M.; Spitalsky, Z. Properties and structure of poly(3-hydroxybutyrate-co-4-hydroxybutyrate) filaments for fused deposition modelling. Int. J. Biol. Macromol. 2021, 183, 880–889. [Google Scholar] [CrossRef]
- Kovalcik, A.; Sangroniz, L.; Kalina, M.; Skopalova, K.; Humpolíček, P.; Omastova, M.; Mundigler, N.; Müller, A.J. Properties of scaffolds prepared by fused deposition modeling of poly(hydroxyalkanoates). Int. J. Biol. Macromol. 2020, 161, 364–376. [Google Scholar] [CrossRef]
- Hou, J.; Zhong, M.; Pan, X.; Chen, L.; Wu, X.; Kong, Z.; Yuan, Y.; Yan, S.; Zhang, J.; Duan, Y. Fabricating 3D printable BIIR/PP TPV via masterbatch and interfacial compatibilization. Compos. Part B Eng. 2020, 199, 108220. [Google Scholar] [CrossRef]
- Ju, Q.; Tang, Z.; Shi, H.; Zhu, Y.; Shen, Y.; Wang, T. Thermoplastic starch based blends as a highly renewable filament for fused deposition modeling 3D printing. Int. J. Biol. Macromol. 2022, 219, 175–184. [Google Scholar] [CrossRef] [PubMed]
- Bi, H.; Ren, Z.; Guo, R.; Xu, M.; Song, Y. Fabrication of flexible wood flour/thermoplastic polyurethane elastomer composites using fused deposition molding. Ind. Crops Prod. 2018, 122, 76–84. [Google Scholar] [CrossRef]
- Xiao, X.; Chevali, V.S.; Song, P.; He, D.; Wang, H. Polylactide/hemp hurd biocomposites as sustainable 3D printing feedstock. Compos. Sci. Technol. 2019, 184, 107887. [Google Scholar] [CrossRef]
- Golbang, A.; Harkin-Jones, E.; Wegrzyn, M.; Campbell, G.; Archer, E.; McIlhagger, A. Production and characterization of PEEK/IF-WS2 nanocomposites for additive manufacturing: Simultaneous improvement in processing characteristics and material properties. Addit. Manuf. 2020, 31, 100920. [Google Scholar] [CrossRef]
- Slonov, A.; Musov, I.; Zhansitov, A.; Rzhevskaya, E.; Khakulova, D.; Khashirova, S. The Effect of Modification on the Properties of Polyetherimide and Its Carbon-Filled Composite. Polymers 2020, 12, 1056. [Google Scholar] [CrossRef] [PubMed]
- Tian, J.; Zhang, R.; Wu, Y.; Xue, P. Additive manufacturing of wood flour/polyhydroxyalkanoates (PHA) fully bio-based composites based on micro-screw extrusion system. Mater. Des. 2021, 199, 109418. [Google Scholar] [CrossRef]
- Díaz-García, Á.; Law, J.Y.; Felix, M.; Guerrero, A.; Franco, V. Functional, thermal and rheological properties of polymer-based magnetic composite filaments for additive manufacturing. Mater. Des. 2022, 219, 110806. [Google Scholar] [CrossRef]
- Murillo-Morales, G.; Sethupathy, S.; Zhang, M.; Xu, L.; Ghaznavi, A.; Xu, J.; Yang, B.; Sun, J.; Zhu, D. Characterization and 3D printing of a biodegradable polylactic acid/thermoplastic polyurethane blend with laccase-modified lignin as a nucleating agent. Int. J. Biol. Macromol. 2023, 236, 123881. [Google Scholar] [CrossRef]
- Wu, X.; Liu, Y.; Wu, H.; Duan, Y.; Zhang, J. Melt-processed poly (L-lactic acid)/cellulose nanocrystals biocomposites for 3D printing: Improved melt processibility and inter-fuse adhesion. Compos. Sci. Technol. 2022, 218, 109135. [Google Scholar] [CrossRef]
- Liu, J.; Sun, L.; Xu, W.; Wang, Q.; Yu, S.; Sun, J. Current advances and future perspectives of 3D printing natural-derived biopolymers. Carbohydr. Polym. 2019, 207, 297–316. [Google Scholar] [CrossRef] [PubMed]
- Shah, S.W.A.; Xu, Q.; Ullah, M.W.; Zahoor; Sethupathy, S.; Morales, G.M.; Sun, J.; Zhu, D. Lignin-based additive materials: A review of current status, challenges, and future perspectives. Addit. Manuf. 2023, 74, 103711. [Google Scholar] [CrossRef]
- Ateeq, M.; Shafique, M.; Azam, A.; Rafiq, M. A review of 3D printing of the recycled carbon fiber reinforced polymer composites: Processing, potential, and perspectives. J. Mater. Res. Technol. 2023, 26, 2291–2309. [Google Scholar] [CrossRef]
- Mikula, K.; Skrzypczak, D.; Izydorczyk, G.; Warchoł, J.; Moustakas, K.; Chojnacka, K.; Witek-Krowiak, A. 3D printing filament as a second life of waste plastics—A review. Environ. Sci. Pollut. Res. 2021, 28, 12321–12333. [Google Scholar] [CrossRef] [PubMed]
- Kwon, O.; Yoon, C.; Ham, S.; Park, J.; Lee, J.; Yoo, D.; Kim, Y. Characterization and Control of Nanoparticle Emission during 3D Printing. Environ. Sci. Technol. 2017, 51, 10357–10368. [Google Scholar] [CrossRef] [PubMed]
- Quanjin, M.; Rejab, M.R.M.; Idris, M.S.; Kumar, N.M.; Abdullah, M.H.; Reddy, G.R. Recent 3D and 4D intelligent printing technologies: A comparative review and future perspective. Procedia Comput. Sci. 2020, 167, 1210–1219. [Google Scholar] [CrossRef]
- Thompson, M.S. Current status and future roles of additives in 3D printing—A perspective. J. Vinyl Addit. Technol. 2022, 28, 3–16. [Google Scholar] [CrossRef]
- Ajinjeru, C.; Kishore, V.; Lindahl, J.; Sudbury, Z.; Hassen, A.A.; Post, B.; Love, L.; Kunc, V.; Duty, C. The influence of dynamic rheological properties on carbon fiber-reinforced polyetherimide for large-scale extrusion-based additive manufacturing. Int. J. Adv. Manuf. Technol. 2018, 99, 411–418. [Google Scholar] [CrossRef]
- Pricci, A.; de Tullio, M.D.; Percoco, G. Analytical and Numerical Models of Thermoplastics: A Review Aimed to Pellet Extrusion-Based Additive Manufacturing. Polymers 2021, 13, 3160. [Google Scholar] [CrossRef]
- Kantaros, A.; Soulis, E.; Petrescu, F.I.T.; Ganetsos, T. Advanced Composite Materials Utilized in FDM/FFF 3D Printing Manufacturing Processes: The Case of Filled Filaments. Materials 2023, 16, 6210. [Google Scholar] [CrossRef]

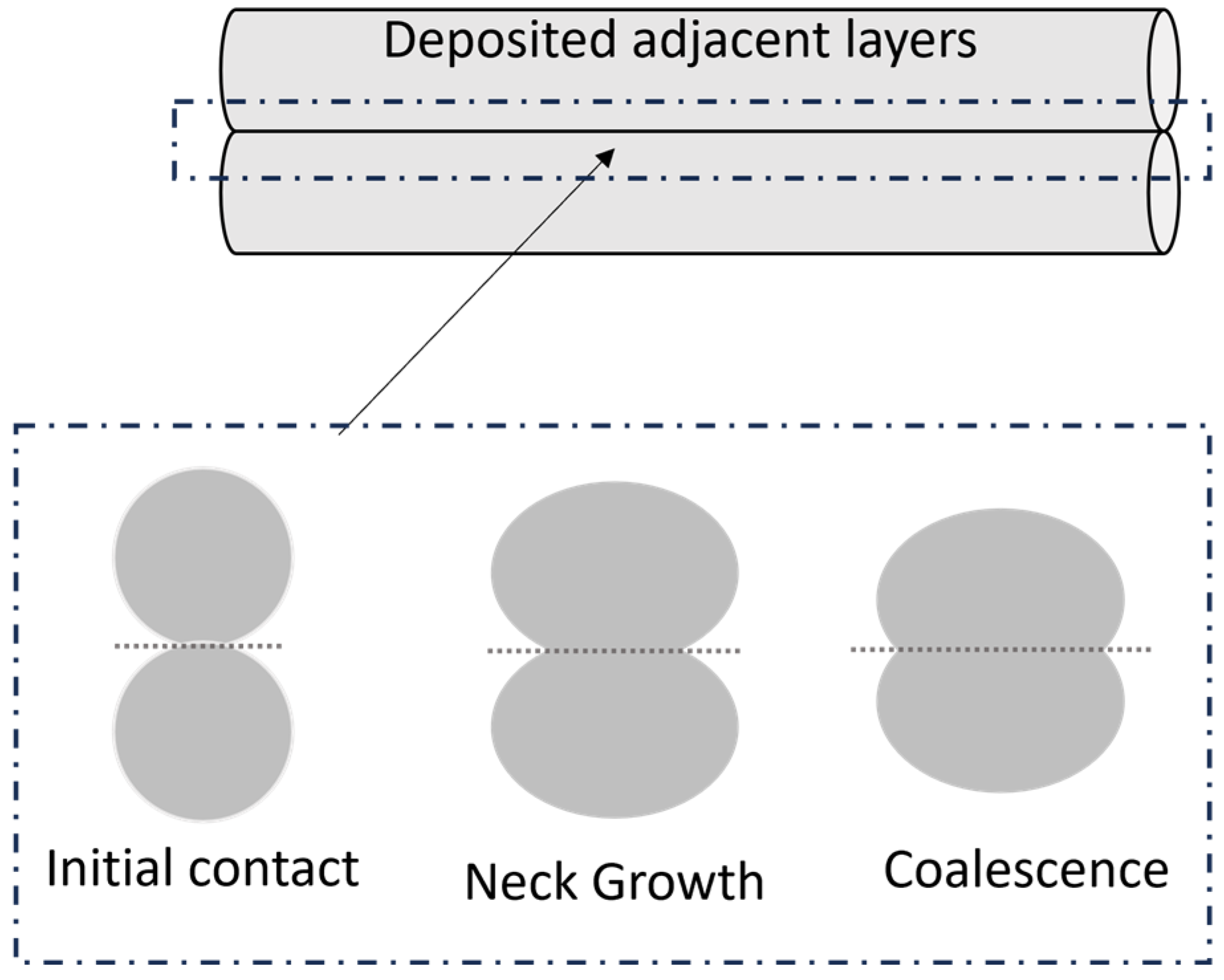
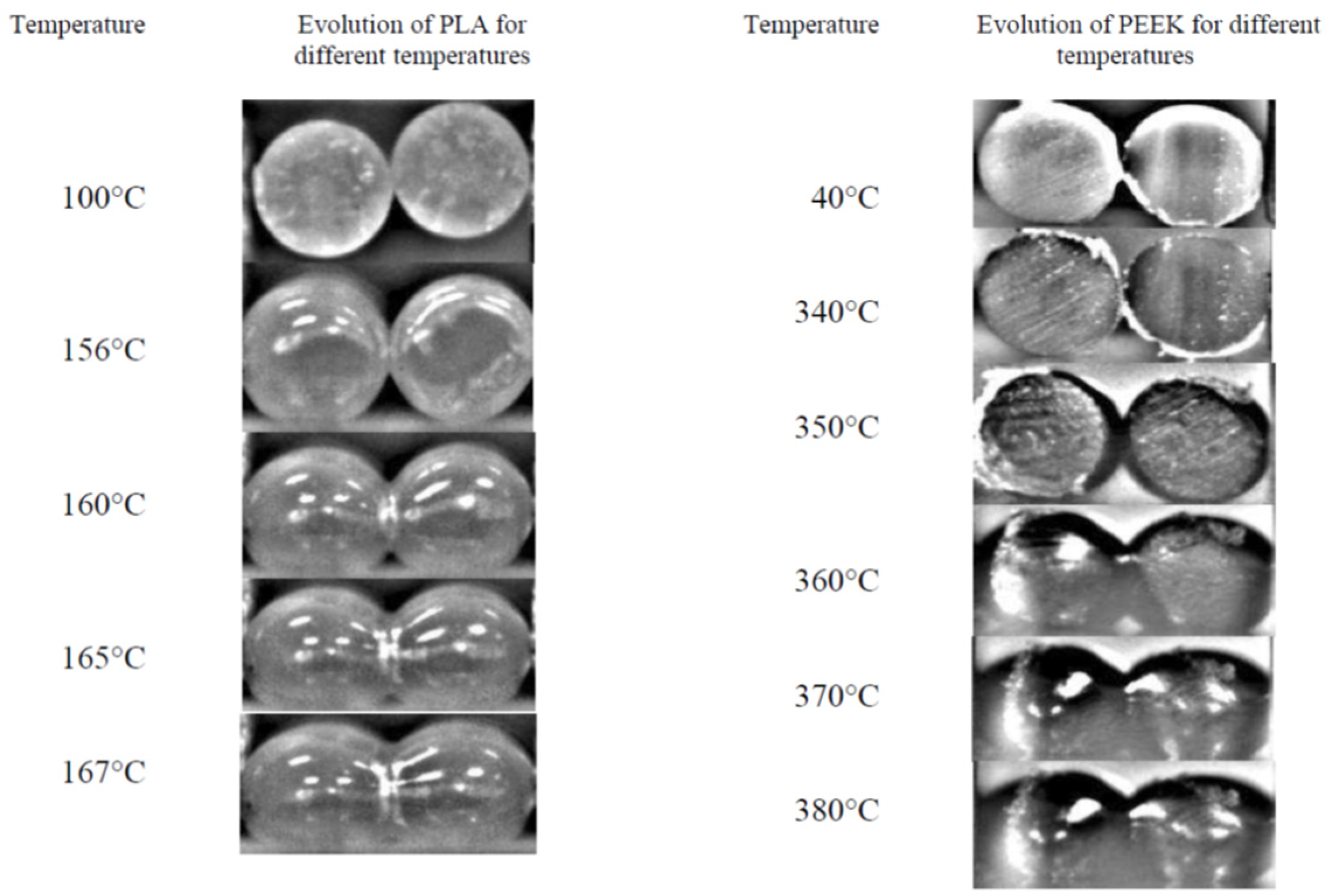
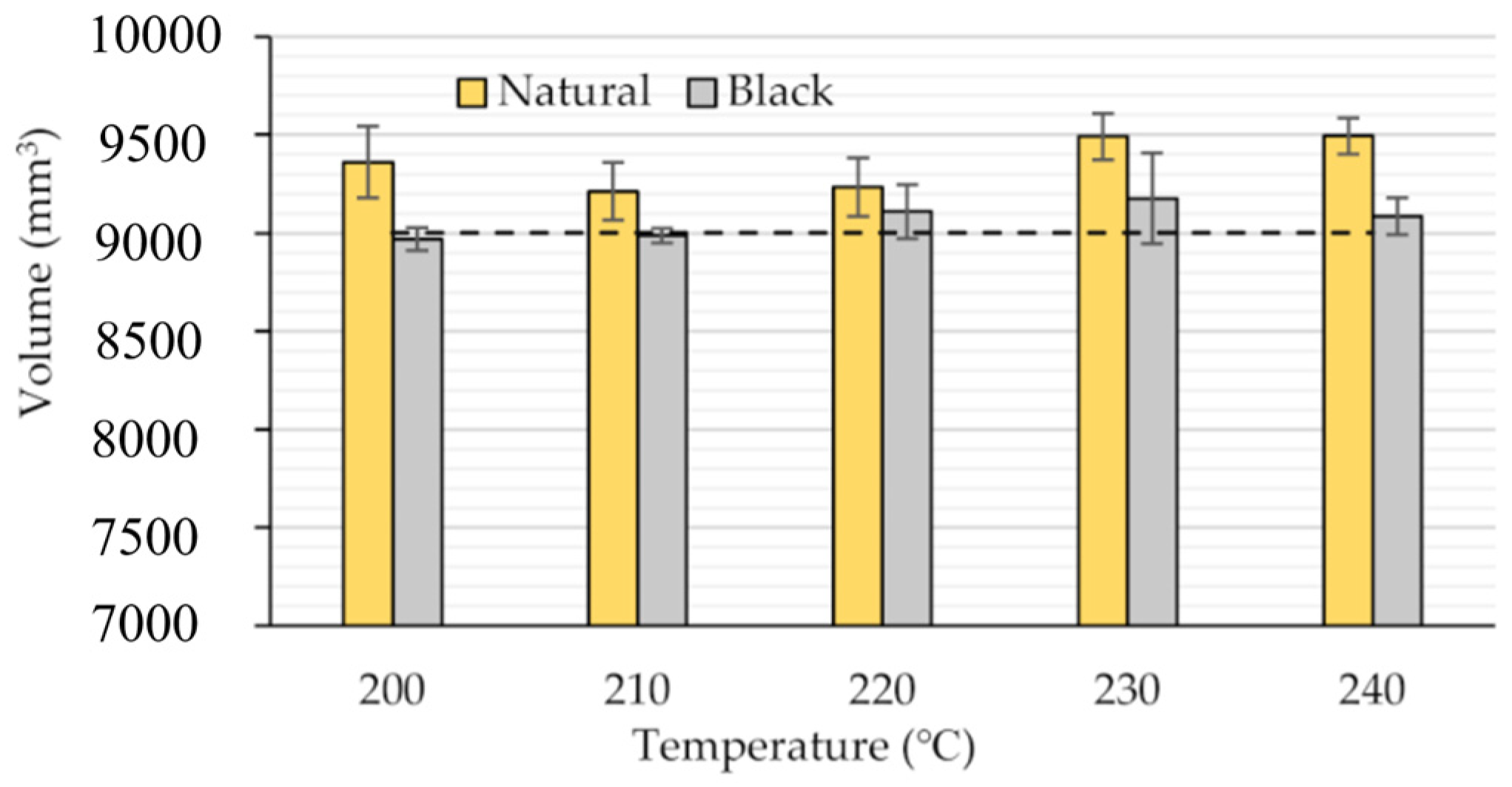
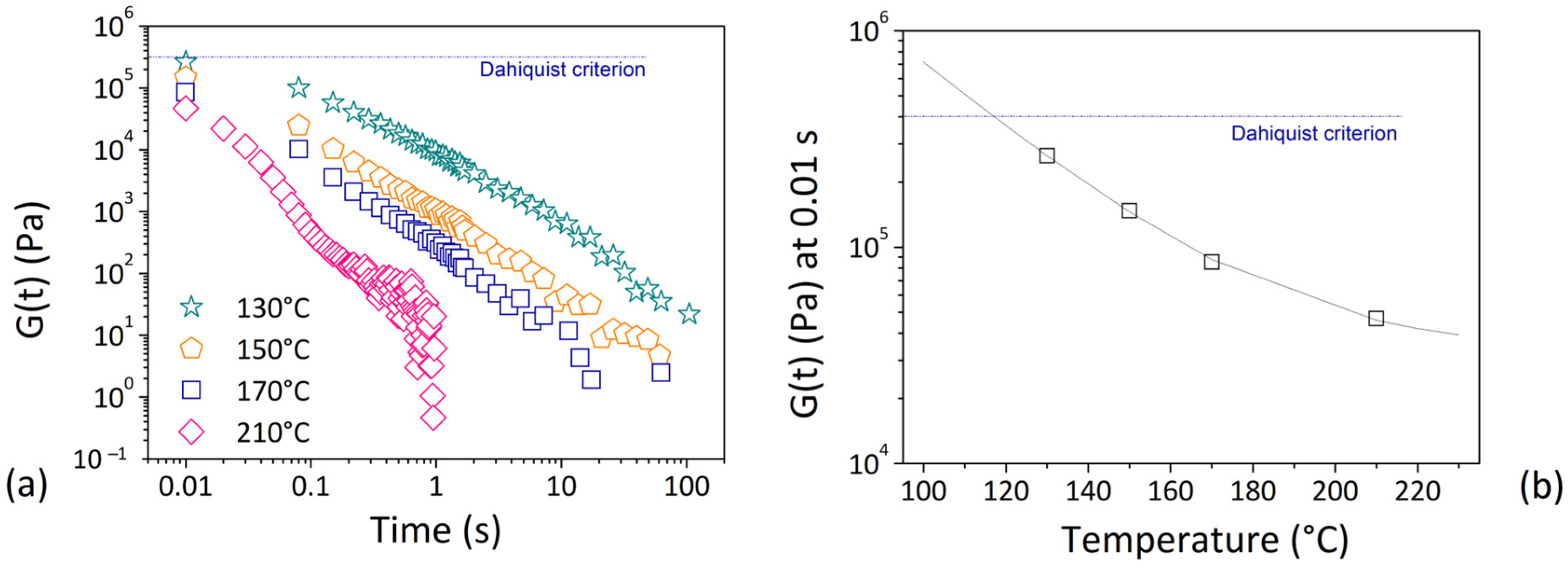
| Materials | Shear-Thinning Index (n) | Other Parameters | Fitting Models | References |
|---|---|---|---|---|
| Acrylonitrile butadiene styrene (ABS) | 0.27 | / | Carreau–Yasuda | [58] |
| 0.42–0.49 | k = 6678–12189 Pa*s | Power law | [59] | |
| ABS/carbon fibers | 0.50 | k = 8213 Pa*s | Power law | [59] |
| ABS/oil palm fiber (up to 7 wt.% in content) | 0.53–0.71 | k = 0.0024 − 0.0008 | Power law | [60] |
| Ethylene-vinyl acetate (EVA) | 0.40–0.47 | η0 = 5.65 × 102 − 2.95 × 104 Pa*s | Cross model | [61] |
| Polycaprolactone (PCL) | 0.18 | η0 = 4.55 × 103 Pa*s | Cross model | [61] |
| 0.47–0.49 | / | / | [62] | |
| PCL/ hydrolyzed collagen (30 wt.%) | 0.6–0.7 | / | / | [62] |
| Polyethylene oxide (PEO) | 0.47 | η0 = 4.18 × 104 Pa*s | Cross model | [61] |
| Polyethylene/wood flour (up to 50 wt%) | 0.4 | k = 104–4 × 104 Pa*sn | Power law | [63] |
| Poly(D,L-lactic-acid) (PDLLA) | 0.33 | η∞ = 0.1 Pa*s; η0 = 2260 Pa*s, λ = 0.0294 s | Carreau–Yasuda | [64] |
| Polylactide acid (PLA) | 0.32 | / | Power Law | [58] |
| 0.69 | η∞ = 0 Pa*s; η0 = 1945 Pa*s, λ = 0.08 s | Carreau–Yasuda | [65] | |
| 0.29–0.37 | η∞ = 0 Pa*s; η0 = 1480–9460 Pa*s, λ = 0.013–0.083 s | Cross | [44] | |
| PLA/carbon nanotubes (CNT) (1.5 to 12 wt.%) | 0.51–0.07 | / | Power law | [66] |
| PLA/ graphene nanoplates (GNP) (1.5 to 12 wt.%) | 1–0.15 | / | Power law | [66] |
| PLA/copper (66 wt.%) | 0.52 | k = 215 Pa*s | Power law | [67] |
| PLA/carbon fibers (14 wt.%) | 0.76 | k = 222 Pa*s | Power law | [67] |
| Poly(methyl methacrylate) (PMMA)/ CNT(1 wt.%)/ nano hydroxyapatite up to 10 wt.%) | 0.53–0.56 | / | / | [68] |
| Polypropylene/CNT (0.3–1 wt.%) | 0.27–0.28 | η0 = 440–509 Pa*s | Carreau–Winter | [69] |
| Thermoplastic-Based Systems | Scope | Testing | Results | Reference |
|---|---|---|---|---|
| Blends | ||||
| Polycaprolactone (PCL)/hydrolysed collagen (HC) | To produce biodegradable items for applications in agriculture and plant nurseries. | Capillary rheometer and flow curves at 130, 140, 150 °C | A decrease in the melt viscosity was observed with the addition of HC due to its plasticizing effect | Seggiani et al., 2018 [62] |
| Polybutylene succinate (PBS)/ Poly (butylene succinateran-adipate) (PBSA) | To develop semi-crystalline biodegradable filaments | Frequency sweep from 150 °C to 230 °C (TTS principle). Continuous flow measurements (Cox–Merz rule). | The viscosity values of samples lie below 104 Pa*s, which allows forecasting a suitable flow in the nozzle | Candal et al., 2020 [49] |
| Poly(lactide) blends containing low molecular weight polymers of chemically identical but enantiomerically different nature (poly(L-lactide) (PLLA) and poly(D-Lactide) (PDLA).) | To promote interfacial weld and stiffness | Oscillatory frequency sweep tests at 180 °C | Any composition possessing η0 > 5000 Pa*s is too viscous for extrusion. Any composition characterized by η0 < 500 Pa*s, could not be printed due to uncontrolled fluctuations in volume flow rate and consequential loss in print resolution | Srinivas et al., 2020 [26] |
| Brominated butyl-rubber (BIIR)/polypropylene (PP) thermoplastic vulcanizate (BIIR/PP-TPV) | To flexible FDM | Frequency sweep at 180 °C | The low viscosity of blends prepared by masterbatch procedure and interfacial compatibilization effectively improves the bonding strength between the adjacent layers of the 3D printed product. | Hou et al., 2020 [104] |
| Polypropylene (PP)/ elastomeric ethylene-octene copolymer (EOC) | To address the deficiencies of PP in melt extrusion processing (warpage and poor layer adhesion) | Frequency sweep at 190 and 210 °C | The EOC addition did not change substantially the complex viscosity of the blends | Ho and Kontopoulou 2022 [30] |
| Thermoplastic starch (TPS)/polylactic acid (PLA) /poly(butyleneadipate-co-terephthalate) (PBAT) and chain extender (CE) | Highly renewable filaments for 3D printing | Frequency sweep at 180 °C. | Complex viscosity and modulus increment of blends with the addition of chain extender | Ju et al., 2022 [105] |
| Composites | ||||
| Wood flour (WF)/thermoplastic polyurethane (TPU) and modification with ethylene-propylene-diene-monomer grafted maleic anhydride (EPDM-g-MAH) | Adding low-cost natural fibre to make a low cost, biodegradable, and ecofriendly material | Frequency sweep at 200 °C. | Moduli and complex viscosity increase in composites after the modification with EPDM-g-MAH | Bi et al., 2018 [106] |
| Polylactide/hemp hurd | To valuable reinforcement of PLA-based blend | Frequency sweep at 190 °C | The biocomposites showed shear-thinning behaviour. Composites at the highest filler loading (40 wt.%) displayed lower melt flow and lower ease of processability than other biocomposite blends. | Xiao et al., 2019 [107] |
| Inorganic Fullerene Tungsten Sulphide (IF-WS2) nanoparticles/ poly-ether-ketone-ketone (PEEK) | Nanoparticles addition to enhance the flowability of PEEK | Dynamic shear tests at 400 °C | At low shear rate, the shear viscosity of PEEK was reduced with the addition of 2 wt% IF-WS2. The difference in viscosity of samples becomes less pronounced in the higher-shear rate range. | Golbang et al., 2020 [108] |
| Carbon fibre (CF)/ polyetherimide (PEI)/oligophenylene sulfone (OPSU)/polycarbonate (PC) | Plasticizing high-performance polymers | Capillary rheometer at a temperature of 380 °C | OPSU and PC decrease the melt viscosity of carbon-filled composite preserving the mechanical properties and heat resistance at a sufficiently high level. | Slonov et al., 2020 [109] |
| Wood flour (WF)/polyhydroxyalkanoates (PHA) | To cost reduction in PHA uses | Frequency sweep at 190 °C | The fluidity of the composites decreased with the increase in WF content | Tian et al., 2021 [110] |
| Polylactic acid (PLA)/ maraging steel particles | To develop composite functional filaments with additional properties (magnetic, electrical, optical) | Frequency and flow tests at 160 °C (Cox–Merz rule) | The viscous component predominates over the elastic component for all the samples. Remarkable decrease in the viscoelastic moduli as the particle content increases | Díaz-García et al., 2022 [111] |
| Boron nitride nanosheets/thermoplastic polyurethane (TPU) | To high-power integrated electronic devices for 5 G system | Rotational rheometer in the small-amplitude shear oscillation mode at 225 °C | G′ of the composites diverged from the behaviour of the pure TPU. However, liquid-to-solid transition not significantly affected the viscosity within the shear rate range of the 3D printing process | Gao et al., 2022 [27] |
| Polylactic acid (PLA)/ thermoplastic polyurethane (TPU) blend with enzymatically modified lignin (EL) | To enhance mechanical and thermal properties of bio-based polymers | Frequency sweep at 170–200 °C depending on materials | A gradual decrease in complex viscosity at higher EL concentrations | Murillo-Morales et al., 2023 [112] |
| Polyetherketoneketone (PEKK)/mica platelets | To provide added mechanical strength to the PEKK | Frequency sweep at 360 °C and temperature ramp | Mica doping does not significantly alter the viscoelastic properties inherent to unfilled PEKK | Kennedy et al., 2022 [37] |
| Poly (L-lactic acid)/cellulose nanocrystals | To produce fully green, high-performance consumables | Capillary rheology measurements and small amplitude oscillatory shear experiments | Composites exhibited shear-thinning behaviour favourable for the stable extrusion at nozzle, and G″ > G′ beneficial to the interfuse adhesion during welding | Wu et al., 2022 [113] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acierno, D.; Patti, A. Fused Deposition Modelling (FDM) of Thermoplastic-Based Filaments: Process and Rheological Properties—An Overview. Materials 2023, 16, 7664. https://doi.org/10.3390/ma16247664
Acierno D, Patti A. Fused Deposition Modelling (FDM) of Thermoplastic-Based Filaments: Process and Rheological Properties—An Overview. Materials. 2023; 16(24):7664. https://doi.org/10.3390/ma16247664
Chicago/Turabian StyleAcierno, Domenico, and Antonella Patti. 2023. "Fused Deposition Modelling (FDM) of Thermoplastic-Based Filaments: Process and Rheological Properties—An Overview" Materials 16, no. 24: 7664. https://doi.org/10.3390/ma16247664
APA StyleAcierno, D., & Patti, A. (2023). Fused Deposition Modelling (FDM) of Thermoplastic-Based Filaments: Process and Rheological Properties—An Overview. Materials, 16(24), 7664. https://doi.org/10.3390/ma16247664