Room-Temperature Strengthening, Portevin-Le Chatelier Effect, High-Temperature Tensile Deformation Behavior, and Constitutive Modeling in a Lightweight Mg-Gd-Al-Zn Alloy
Abstract
:1. Introduction
2. Experimental Procedures
2.1. Materials Preparation
2.2. Tensile Tests at Room Temperature and Elevated Temperatures
2.3. Microstructural Characterization
3. Results
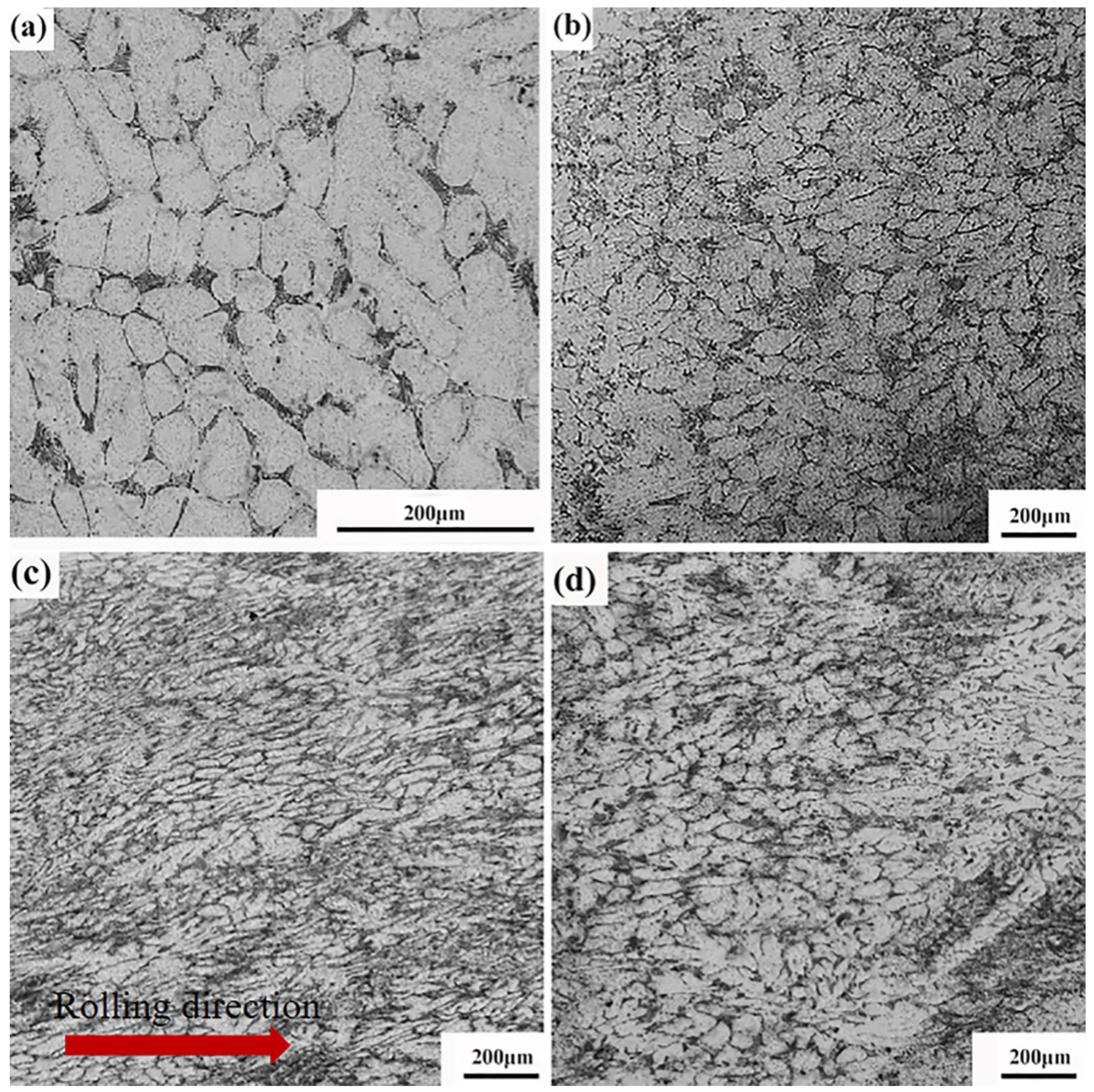
3.1. Microstructure and Mechanical Properties of the Present Alloy at Room Temperature
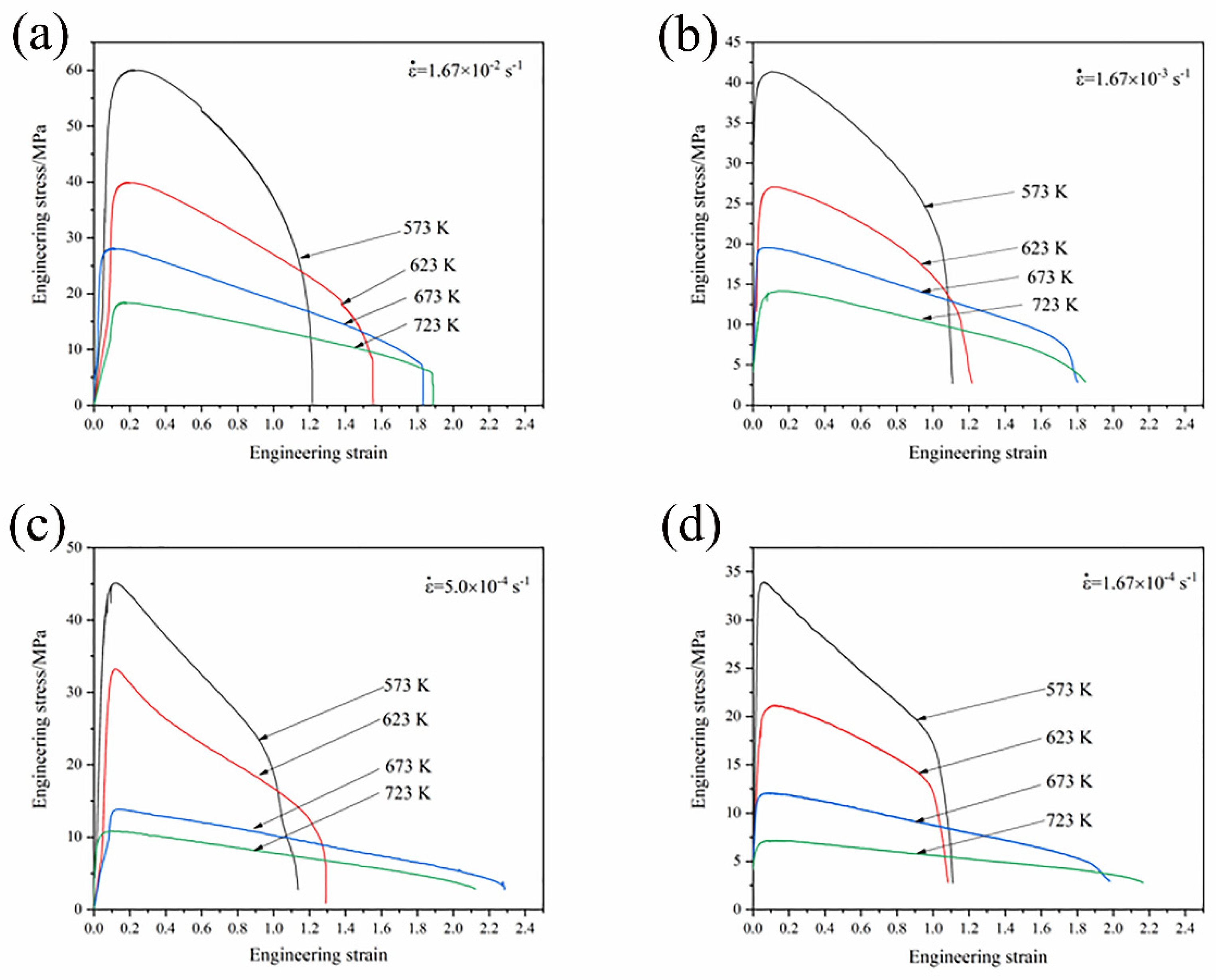
3.2. Microstructure and Stress–Strain Curves of the Present Alloy at Elevated Temperature
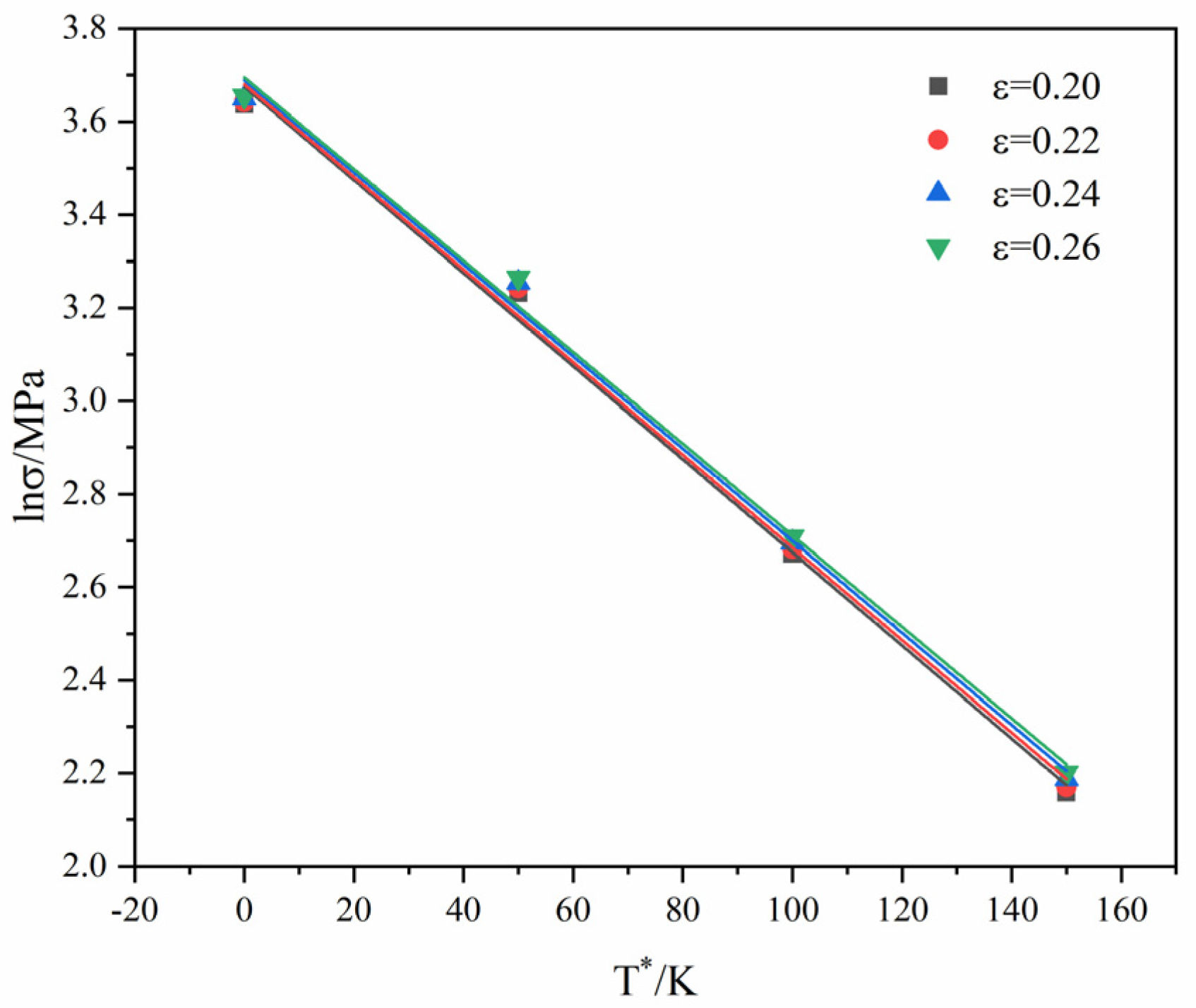
3.3. Establishment of Constitutive Models at Elevated Temperatures
3.3.1. Modified Zerilli–Armstrong Constitutive Model Incorporating the Number of Dislocations
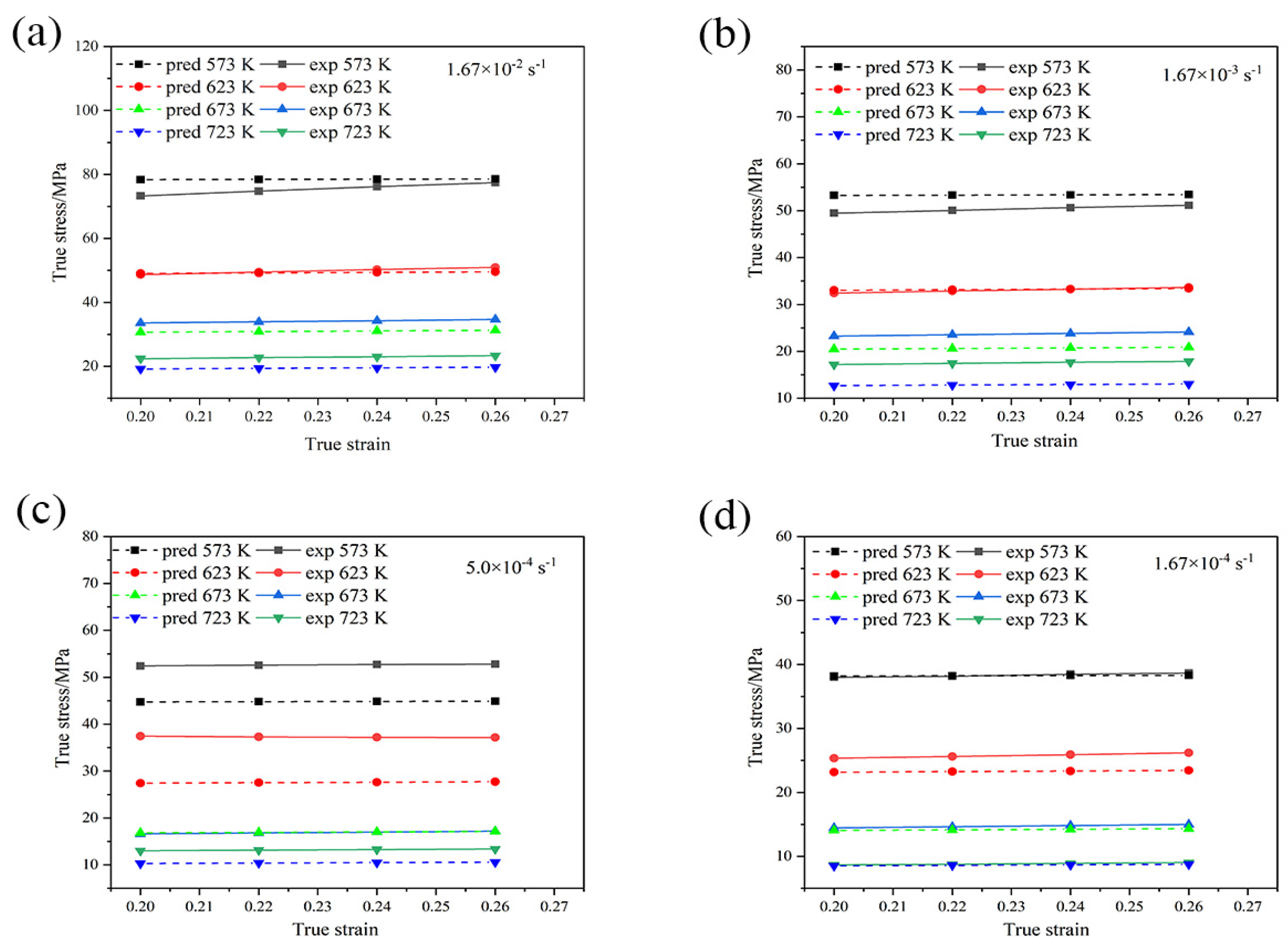
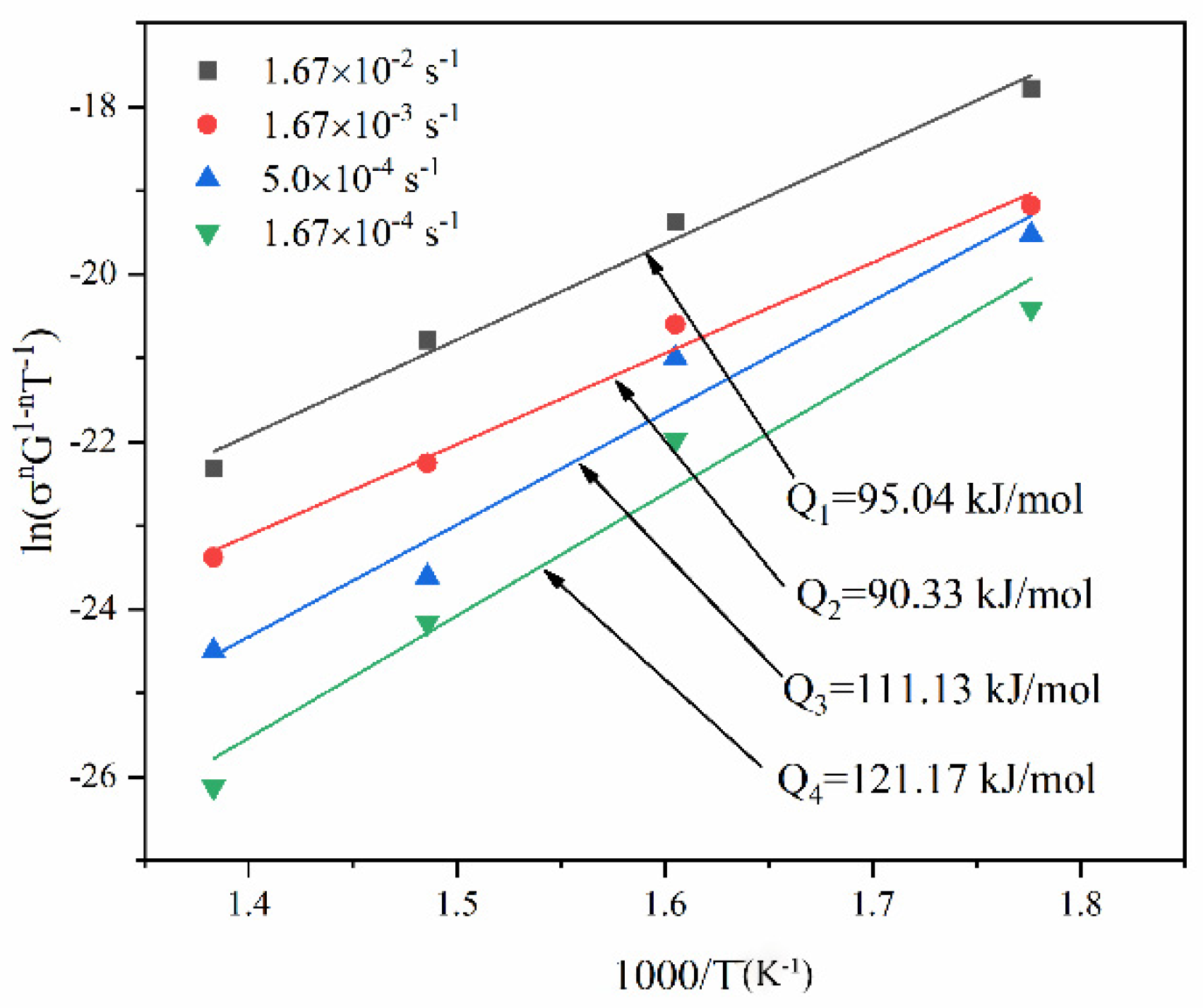
3.3.2. Power-Law Constitutive Model
4. Discussion
4.1. Estimation of Room-Temperature Strengthening and Contribution to Yield Strength Property in GAZ211 Alloy
4.2. Analysis of Type C PLC Effect at Room Temperature
4.3. Analysis of Microstructural and Flow Stress Characteristics at Elevated Temperature
4.4. Comparison of MZA and Power-Law Constitutive Models Established in This Alloy
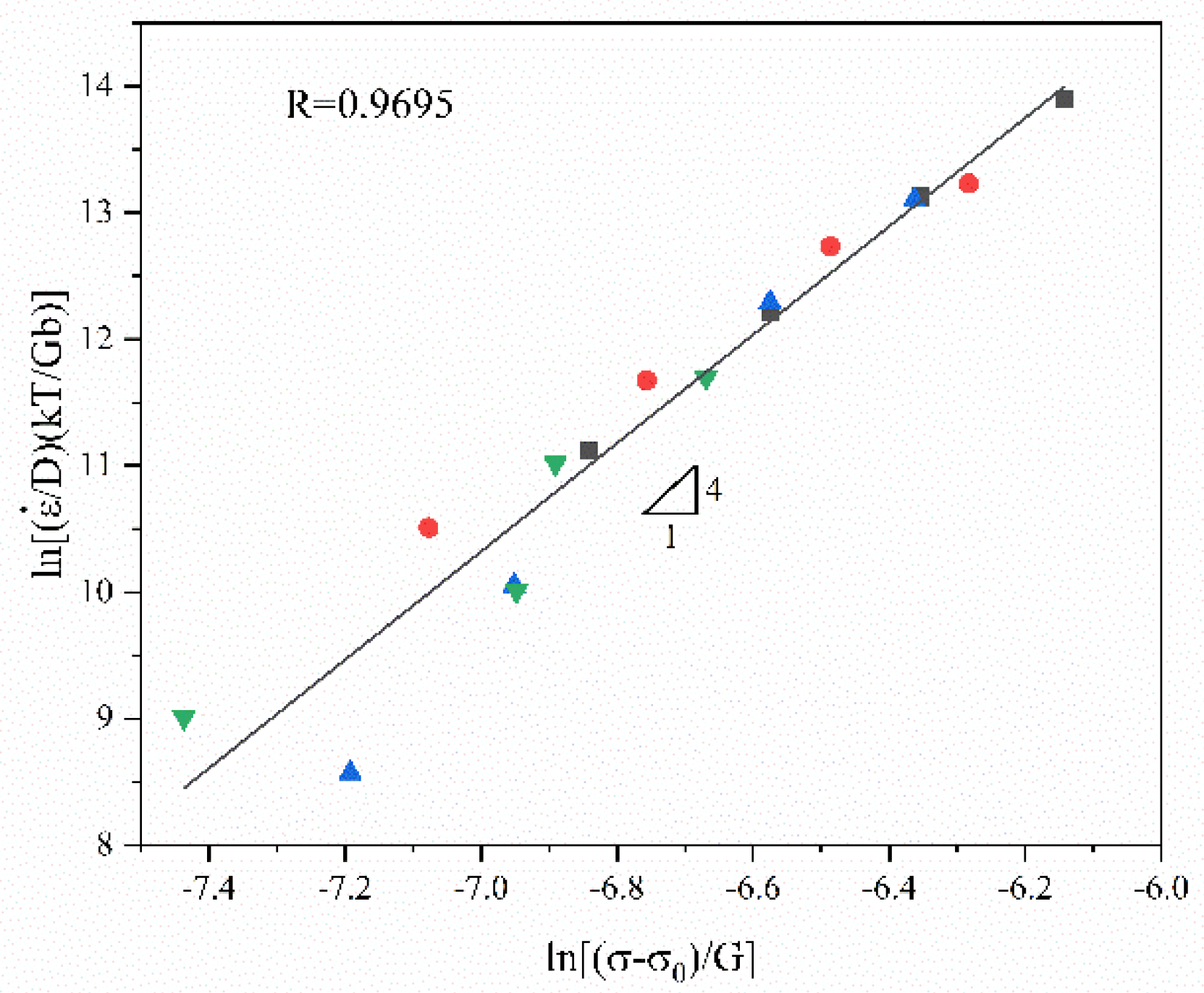
4.5. Deformation Mechanism of the Present Alloy at Elevated Temperature
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abdiyan, F.; Mahmudi, R.; Ghasemi, H.M. Effect of Mn addition on the microstructure, mechanical properties and corrosion resistance of a biodegradable Mg-Gd-Zn alloy. Mater. Chem. Phys. 2021, 271, 124878. [Google Scholar] [CrossRef]
- Luo, S.F.; Wang, N.; Wang, Y.; Chen, J.M.; Qin, H.; Kong, S.; Bai, T.; Lu, W.J.; Xiao, L.; Ma, X.K.; et al. Texture, microstructure and mechanical properties of an extruded Mg-10Gd-1Zn-0.4Zr alloy: Role of microstructure prior to extrusion. Mater. Sci. Eng. A 2022, 849, 143476. [Google Scholar] [CrossRef]
- Wu, D.; Chen, R.S.; Tang, W.N.; Han, E.H. Influence of texture and grain size on the room-temperature ductility and tensile behavior in a Mg–Gd–Zn alloy processed by rolling and forging. Mater. Des. 2012, 41, 306–313. [Google Scholar] [CrossRef]
- Deng, Q.C.; Wu, Y.J.; Zhu, W.X.; Chen, K.; Liu, D.Z.; Peng, L.M.; Ding, W.J. Effect of heat treatment on microstructure evolution and mechanical properties of selective laser melted Mg-11Gd-2Zn-0.4Zr alloy. Mater. Sci. Eng. A 2022, 829, 142139. [Google Scholar] [CrossRef]
- Pourbahari, B.; Mirzadeh, H.; Emamy, M. Toward unraveling the effects of intermetallic compounds on the microstructure and mechanical properties of Mg-Gd-Al-Zn magnesium alloys in the as-cast, homogenized, and extruded conditions. Mater. Sci. Eng. A 2017, 680, 39–46. [Google Scholar] [CrossRef]
- Pourbahari, B.; Mirzadeh, H.; Emamy, M. Elucidating the effect of intermetallic compounds on the behavior of Mg-Gd-Al-Zn magnesium alloys at elevated temperatures. J. Mater. Res. 2017, 32, 4186–4195. [Google Scholar] [CrossRef]
- Ding, Z.B.; Zhang, S.; Zhao, Y.H.; Chen, D.R.; Zou, L.H.; Chen, Z.G.; Guo, W.M.; Su, Z.; Hou, J.H. Effect of Al addition on microstructure and mechanical properties of Mg-Gd-Zn alloys. Trans. Nonferrous Met. Soc. China 2022, 32, 824–837. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Straumal, B.; Langdon, T.G. Using Severe Plastic Deformation to Produce Nanostructured Materials with Superior Properties. Annu. Rev. Mater. Res. 2022, 52, 357–382. [Google Scholar] [CrossRef]
- Lu, F.M.; Ma, A.B.; Jiang, J.H.; Yang, D.H.; Yuan, Y.C.; Zhang, L.Y. Formation of profuse long period stacking ordered microcells in Mg–Gd–Zn–Zr alloy during multipass ECAP process. J. Alloys Compd. 2014, 601, 140–145. [Google Scholar] [CrossRef]
- Langdon, T.G. Twenty-five years of ultrafine-grained materials: Achieving exceptional properties through grain refinement. Acta Mater. 2013, 61, 7035–7059. [Google Scholar] [CrossRef]
- Tang, L.L.; Zhao, Y.H.; Islamgaliev, R.K.; Valiev, R.Z.; Zhu, Y.T. Microstructure and thermal stability of nanocrystalline Mg-Gd-Y-Zr alloy processed by high pressure torsion. J. Alloys Compd. 2017, 721, 577–585. [Google Scholar] [CrossRef]
- Yang, Q.; Xiao, B.L.; Zhang, Q.; Zheng, M.Y.; Ma, Z.Y. Exceptional high-strain-rate superplasticity in Mg-Gd-Y-Zn-Zr alloy with long-period stacking ordered phase. Scr. Mater 2013, 69, 801–804. [Google Scholar] [CrossRef]
- Xia, X.S.; Chen, Q.; Zhao, Z.D.; Ma, M.L.; Li, X.G.; Zhang, K. Microstructure, texture and mechanical properties of coarse-grained Mg–Gd–Y–Nd–Zr alloy processed by multidirectional forging. J. Alloys Compd. 2015, 623, 62–68. [Google Scholar] [CrossRef]
- Jin, Z.Z.; Zha, M.; Yu, Z.Y.; Ma, P.K.; Li, Y.K.; Liu, J.M.; Jia, H.L.; Wang, H.Y. Exploring the Hall-Petch relation and strengthening of bimodal-grained Mg-Al-Zn alloys. J. Alloys Compd. 2020, 833, 155004. [Google Scholar] [CrossRef]
- Kang, Y.H.; Wang, X.X.; Zhang, N.; Yan, H.; Chen, R.S. Effect of pre-deformation on microstructure and mechanical properties of WE43 magnesium alloy. Mater. Sci. Eng. A 2017, 689, 435–445. [Google Scholar] [CrossRef]
- Tahreen, N.; Zhang, D.F.; Pan, F.S.; Jiang, X.Q.; Li, D.Y.; Chen, D.L. Strengthening mechanisms in magnesium alloys containing ternary I, W and LPSO phases. J. Mater. Sci. Technol. 2018, 34, 1110–1118. [Google Scholar] [CrossRef]
- Zhang, Y.; Jiang, H.T.; Wang, S.H.; Wang, Y.J.; Tian, S.W.; Lin, H.T.; Zhang, G.H.; Yang, Y.G.; Xu, Z. Simultaneous improvements in strength and formability of modified Mg–2Zn alloys by alloying addition and twin-roll casting process. Mater. Sci. Eng. A 2021, 804, 140566. [Google Scholar] [CrossRef]
- Jin, X.Z.; Xu, W.C.; Yang, G.J.; Shan, D.B.; Guo, B. Microstructure evolution and strengthening mechanisms of Mg-6Gd-4Y-0.5Zn-0.5Zr alloy during hot spinning and aging treatment. Mater. Sci. Eng. A 2021, 827, 142035. [Google Scholar] [CrossRef]
- Ji, Q.; Zhang, S.; Wu, R.Z.; Jin, S.Y.; Zhang, J.H.; Hou, L.G. High strength BCC magnesium-Lithium alloy processed by cryogenic rolling and room temperature rolling and its strengthening mechanisms. Mater. Charact. 2022, 187, 111869. [Google Scholar] [CrossRef]
- Wang, R.R.; Han, Y.J.; Yu, H.; Su, Q.; Li, H.; Cheng, K.M.; Zhou, J.X.; Tang, S.Q.; Ju, W. Exceptional Strengthening Efficiency and Hardness of Ti/Mg-9Al-Zn-0.3Mn Matrix Composite. Materials 2022, 15, 7075. [Google Scholar] [CrossRef]
- Jeong, H.T.; Kim, W.J. Strain hardening behavior and strengthening mechanism in Mg-rich Al–Mg binary alloys subjected to aging treatment. Mater. Sci. Eng. A 2020, 794, 139862. [Google Scholar] [CrossRef]
- Yuzbekova, D.; Mogucheva, A.; Borisova, Y.; Kaibyshev, R. On the mechanisms of nucleation and subsequent development of the PLC bands in an AlMg alloy. J. Alloys Compd. 2021, 868, 159135. [Google Scholar] [CrossRef]
- He, W.J.; Hu, R. Portevin-Le Chatelier effect, twinning-detwinning and disordering in an aged Ni–Cr–Mo alloy during large plastic deformation. Mater. Sci. Eng. A 2021, 803, 140506. [Google Scholar] [CrossRef]
- Borisova, Y.; Yuzbekova, D.; Mogucheva, A.; Xu, W. Characterization of the spatiotemporal behavior of the PLC bands in austenitic steel during deformation at elevated temperature. Mater. Lett. 2021, 304, 130584. [Google Scholar] [CrossRef]
- Cao, F.R.; Zhang, J.; Ding, X.; Xue, G.Q.; Liu, S.Y.; Sun, C.F.; Su, R.K.; Teng, X.M. Mechanical properties and microstructural evolution in a superlight Mg-6.4Li-3.6Zn-0.37Al-0.36Y alloy processed by multidirectional forging and rolling. Mater. Sci. Eng. A 2019, 760, 377–393. [Google Scholar] [CrossRef]
- Lin, Y.C.; Chen, X.M. A critical review of experimental results and constitutive descriptions for metals and alloys in hot working. Mater. Des. 2011, 32, 1733–1759. [Google Scholar] [CrossRef]
- Hoseini-Athar, M.M.; Mahmudi, R. Effect of Zn content on hot deformation behavior of extruded Mg–Gd–Zn alloys. Mater. Sci. Eng. A 2019, 759, 745–753. [Google Scholar] [CrossRef]
- Li, K.; Chen, Z.Y.; Chen, T.; Shao, J.B.; Wang, R.K.; Liu, C.M. Hot deformation and dynamic recrystallization behaviors of Mg-Gd-Zn alloy with LPSO phases. J. Alloys Compd. 2019, 792, 894–906. [Google Scholar] [CrossRef]
- Cao, F.R.; Sun, C.F.; Liu, S.Y.; Liang, J.R.; Liu, R.J.; Guo, H.Z.; Guo, N.P. Microstructures, hot tensile deformation behavior and constitutive modeling in a superlight Mg-2.76Li-3Al-2.6Zn-0.39Y alloy. J. Alloys Compd. 2022, 896, 163049. [Google Scholar] [CrossRef]
- Sakai, T.; Belyakov, A.; Kaibyshev, R.; Miura, H.; Jonas, J.J. Dynamic and post-dynamic recrystallization under hot, cold and severe plastic deformation conditions. Prog. Mater. Sci. 2014, 60, 130–207. [Google Scholar] [CrossRef] [Green Version]
- Azizi, N.; Mahmudi, R. Superplasticity of fine-grained Mg–xGd alloys processed by multi-directional forging. Mater. Sci. Eng. A 2019, 767, 138436. [Google Scholar] [CrossRef]
- Wu, Y.J.; Peng, L.M.; Zeng, X.Q.; Lin, D.L.; Ding, W.J.; Peng, Y.H. A high-strength extruded Mg–Gd–Zn–Zr alloy with superplasticity. J. Mater. Res. 2009, 24, 3596–3602. [Google Scholar] [CrossRef]
- Hoseini-Athar, M.M.; Mahmudi, R.; Babu, R.P.; Hedstrom, P. Microstructure and superplasticity of Mg–2Gd–xZn alloys processed by equal channel angular pressing. Mater. Sci. Eng. A 2021, 808, 140921. [Google Scholar] [CrossRef]
- Sarebanzadeh, M.; Roumina, R.; Mahmudi, R.; Wu, G.H.; Jafari Nodooshan, H.R. Enhancement of superplasticity in a fine-grained Mg–3Gd–1Zn alloy processed by equal-channel angular pressing. Mater. Sci. Eng. A 2015, 646, 249–253. [Google Scholar] [CrossRef]
- Sotoudeh, K.; Bate, P.S. Diffusion creep and superplasticity in aluminum alloys. Acta Mater. 2010, 58, 1909–1920. [Google Scholar] [CrossRef]
- Cao, F.R.; Teng, X.M.; Su, R.K.; Liang, J.R.; Liu, R.J.; Kong, S.T.; Guo, N.P. Room temperature strengthening mechanisms, high temperature deformation behavior and constitutive modeling in an Al-3.25Mg-0.37Zr-0.28Mn-0.19Y alloy. J. Mater. Res. Technol. 2022, 18, 962–977. [Google Scholar] [CrossRef]
- Zerilli, F.J.; Armstrong, R.W. Dislocation-Mechanics Based Constitutive Relations for Material Dynamics Calculations. J. Appl. Phys. 1987, 61, 1816–1825. [Google Scholar] [CrossRef] [Green Version]
- Zhan, H.Y.; Wang, G.; Damon, K.; Dargusch, M. Constitutive modelling of the flow behaviour of a β titanium alloy at high strain rates and elevated temperatures using the Johnson-Cook and modified Zerilli-Armstrong models. Mater. Sci. Eng. A 2014, 612, 71–79. [Google Scholar] [CrossRef] [Green Version]
- Samantaray, D.; Sumantra, M.; Borah, U.; Bhaduri, A.K.; Sivaprasad, P.V. A thermo-viscoplastic constitutive model to predict elevated-temperature flow behaviour in a titanium-modified austenitic stainless steel. Mater. Sci. Eng. A 2009, 526, 1–6. [Google Scholar] [CrossRef]
- Cao, F.R.; Xia, F.; Xue, G.Q. Hot tensile deformation behavior and microstructural evolution of a Mg-9.3Li-1.79Al-1.61Zn alloy. Mater. Des. 2016, 92, 44–57. [Google Scholar] [CrossRef]
- Taleff, E.M.; Hector, L.G.; Verma, R.; Krajewski, P.E.; Chang, J.K. Material models for simulation of superplastic Mg alloy sheet forming. J. Mater. Eng. Perform. 2010, 19, 488–494. [Google Scholar] [CrossRef]
- Kim, W.J.; Lee, B.H.; Lee, J.B.; Lee, M.J.; Park, Y.B. Synthesis of high-strain-rate superplastic magnesium alloy sheets using a high-ratio differential speed rolling technique. Scr. Mater. 2010, 63, 772–775. [Google Scholar] [CrossRef]
- Frost, H.J.; Ashby, M.F. Deformation Mechanism Maps; Pergamon Press: Oxford, UK, 1982; p. 21. [Google Scholar]
- Mukherjee, A.K. An examination of the constitutive equation for elevated temperature plasticity. Mater. Sci. Eng. A 2002, 322, 1–22. [Google Scholar] [CrossRef]
- Langdon, T.G. A unified approach to grain boundary sliding in creep and superplasticity. Acta Metall. Mater. 1994, 42, 2437–2443. [Google Scholar] [CrossRef]
- Langdon, T.G. Seventy-five years of superplasticity: Historic developments and new opportunities. J. Mater. Sci. 2009, 44, 5998–6010. [Google Scholar] [CrossRef]
- Taleff, E.M.; Ruano, O.A.; Sherby, O.D. Superplastic behavior of a fine-grained Mg-9Li materials at low homologous temperature. J. Mater. Res. 1992, 7, 2131–2135. [Google Scholar] [CrossRef] [Green Version]
- Nagarajan, D.; Caceres, C.H. The friction stress of the Hall–Petch relationship of pure Mg and solid solutions of Al, Zn, and Gd. Metall. Mater. Trans. A 2018, 49, 5288–5297. [Google Scholar] [CrossRef]
- Fernandez-Zelaia, P.; Melkote, S.; Marusich, T.; Usui, S. A microstructure sensitive grain boundary sliding and slip based constitutive model for machining of Ti-6Al-4V. Mech. Mater. 2017, 109, 67–81. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.H.; Liao, X.Z.; Jin, Z.; Valiev, R.Z.; Zhu, Y.T. Microstructures and mechanical properties of ultrafine grained 7075 Al alloy processed by ECAP and their evolutions during annealing. Acta Mater. 2004, 52, 4589–4599. [Google Scholar] [CrossRef]
- Cao, F.R.; Sun, C.F.; Shang, H.H.; Xiang, C.; Liu, R.J. Microstructure evolution and mechanical properties in an ultralight Mg-2.76Li–3Al-2.6Zn-0.39Y alloy. Mater. Sci. Eng. A 2021, 822, 141680. [Google Scholar] [CrossRef]
- Xie, J.; Chen, X.P.; Cao, Y.; Huang, G.J.; Liu, Q. Microstructure and mechanical properties in Al-Mg-Sc alloy induced by hetero-deformation. Mater. Charact. 2022, 183, 111622. [Google Scholar] [CrossRef]
- Ma, K.K.; Wen, H.M.; Hu, T.; Topping, T.D.; Isheim, D.; Seidman, D.N.; Lavernia, E.J.; Schoenung, J.M. Mechanical behavior and strengthening mechanisms in ultrafine grain precipitation-strengthened aluminum alloy. Acta Mater. 2014, 62, 141–155. [Google Scholar] [CrossRef]
- Caceres, C.H.; Lukac, P. Strain hardening behaviour and the Taylor factor of pure Mg. Philos. Mag. 2008, 88, 977–989. [Google Scholar] [CrossRef]
- Xu, M.N.; Li, N.; Sha, X.C.; Liu, Y.; Gao, B.; Xiao, L.R.; Chen, X.F.; Zhou, H. In-situ TEM observations on interaction of basal <a> dislocations and β′ phases in a Mg–Gd binary alloy. Mater. Sci. Eng. A 2022, 841, 143017. [Google Scholar]
- Mogucheva, A.; Yuzbekova, D.; Kaibyshev, R.; Lebedkina, T.; Lebyodkin, M. Effect of grain refinement on jerky flow in an Al-Mg-Sc alloy. Metall. Mater. Trans. A 2016, 47, 2093–2106. [Google Scholar] [CrossRef]
- Li, C.; Teng, J.W.; Yang, B.B.; Ye, X.J.; Huang, L.; Liu, Y.; Li, Y.P. Portevin-Le Châtelier Effect in a Powder Metallurgy Co-Ni-Based Superalloy. Materials 2022, 15, 2796. [Google Scholar] [CrossRef] [PubMed]
- Mao, W.Q.; Gong, W.; Kawasaki, T.; Harjo, S. Effect of deformation-induced martensitic transformation on nonuniform deformation of metastable austenitic steel. Mater. Sci. Eng. A 2022, 837, 142758. [Google Scholar] [CrossRef]
- Su, J.; Sanjari, M.; Kabir, A.S.H.; Jonas, J.J.; Yue, S. Static recrystallization behavior of magnesium AZ31 alloy subjected to high speed rolling. Mater. Sci. Eng. A 2016, 662, 412–425. [Google Scholar] [CrossRef]
- Doherty, R.D.; Hughes, D.A.; Humphreys, F.J.; Jonas, J.J.; Jensen, J.D.; Kassner, M.E.; King, W.E.; McNelley, T.R.; McQueen, H.J.; Rollett, A.D. Current issues in recrystallization: A review. Mater. Sci. Eng. A 1997, 238, 219–274. [Google Scholar] [CrossRef] [Green Version]
- Cao, F.R.; Yin, B.; Liu, S.Y.; Shi, L.; Wang, S.C.; Wen, J.L. Microstructural evolution, flow stress and constitutive modeling of a novel Al-1.88Mg-0.18Sc-0.084Er alloy during hot compression. Trans. Nonferrous Met. Soc. China 2021, 31, 53–73. [Google Scholar] [CrossRef]
- Mirzadeh, H.; Roostaei, M.; Parsa, M.H.; Mahmudi, R. Rate controlling mechanisms during hot deformation of Mg–3Gd–1Zn magnesium alloy: Dislocation glide and climb, dynamic recrystallization, and mechanical twinning. Mater. Des. 2015, 68, 228–231. [Google Scholar] [CrossRef]
- Barezban, M.H.; Mirzadeh, H.; Roumina, R.; Mahmudi, R. Constitutive analysis of wrought Mg-Gd magnesium alloys during hot compression at elevated temperatures. J. Alloys Compd. 2019, 791, 1200–1206. [Google Scholar] [CrossRef]
- Xiao, Y.W.; Lin, Y.C.; Jiang, Y.Q.; Zhang, X.Y.; Pang, G.D.; Wang, D.; Zhou, K.C. A dislocation density-based model and processing maps of Ti-55511 alloy with bimodal microstructures during hot compression in α + β region. Mater. Sci. Eng. A 2020, 790, 139692. [Google Scholar] [CrossRef]
- Kim, W.J.; Jeong, H.T. Construction of processing maps combined with deformation mechanism maps using creep deformation equations. J. Mater. Res. Technol. 2020, 9, 13434–13449. [Google Scholar] [CrossRef]
- Ruano, O.A.; Wadsworth, J.; Sherby, O.D. Deformation mechanisms in an austenitic stainless steel (25Cr-20Ni) at elevated temperature. J. Mater. Sci. 1985, 20, 3735–3744. [Google Scholar] [CrossRef]


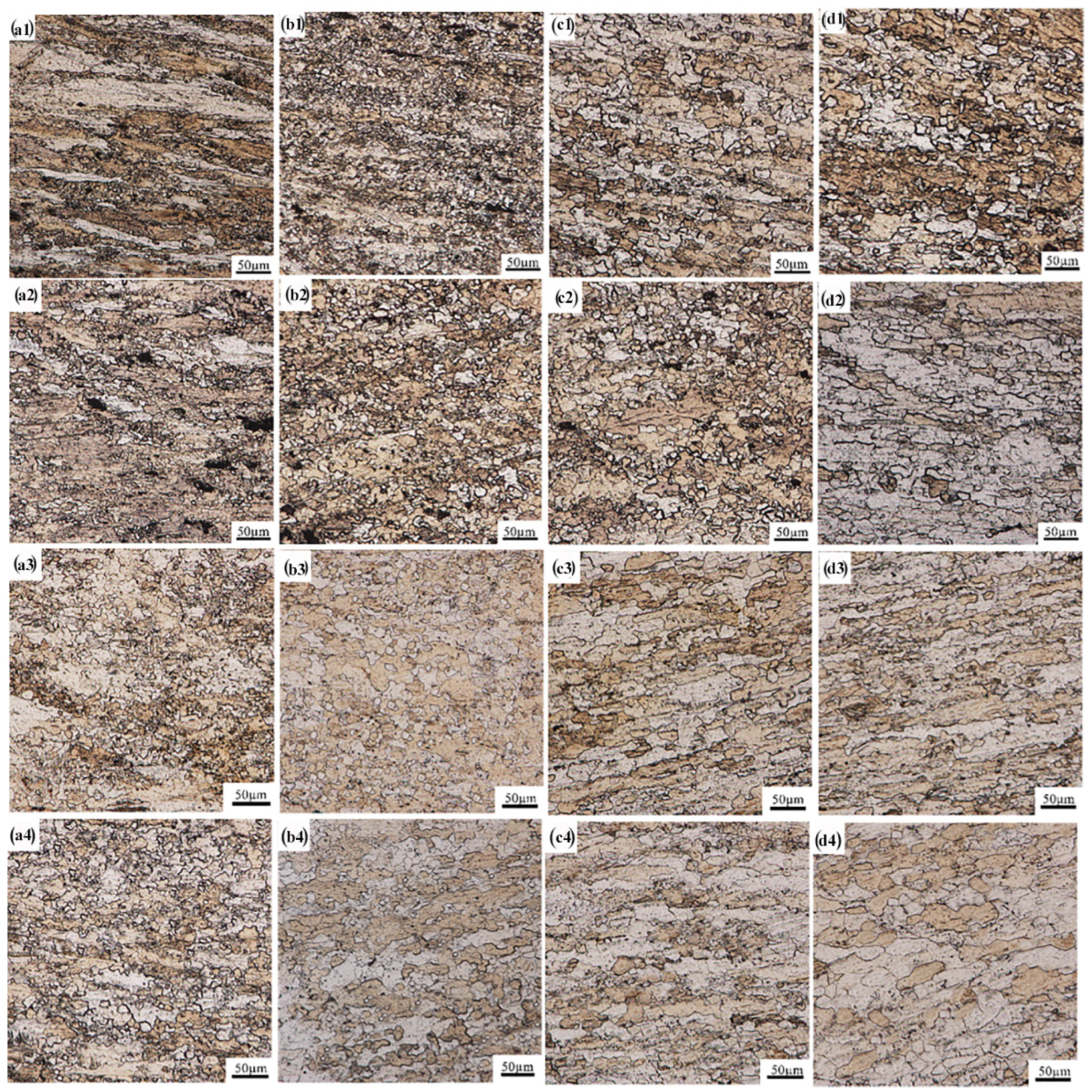

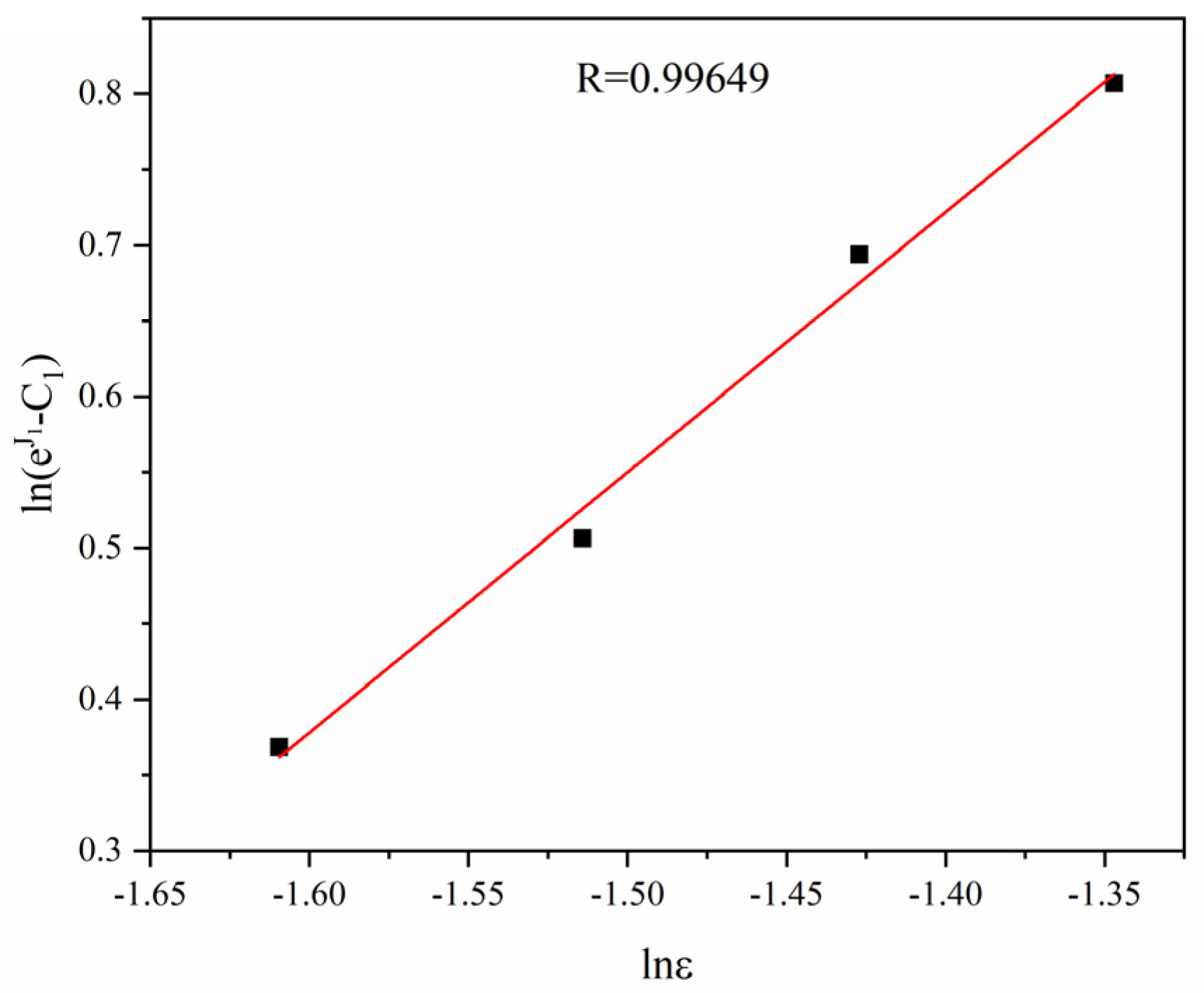
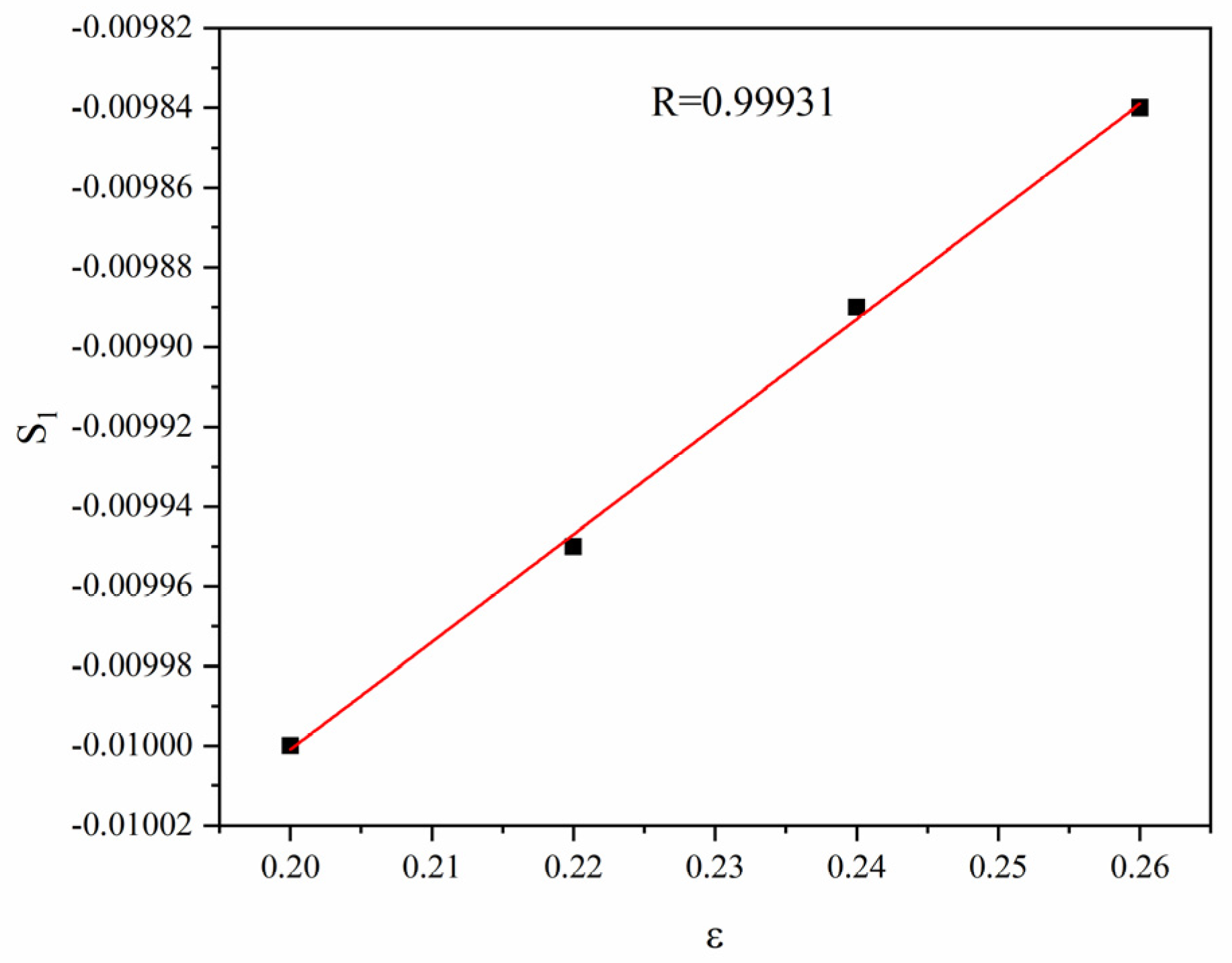
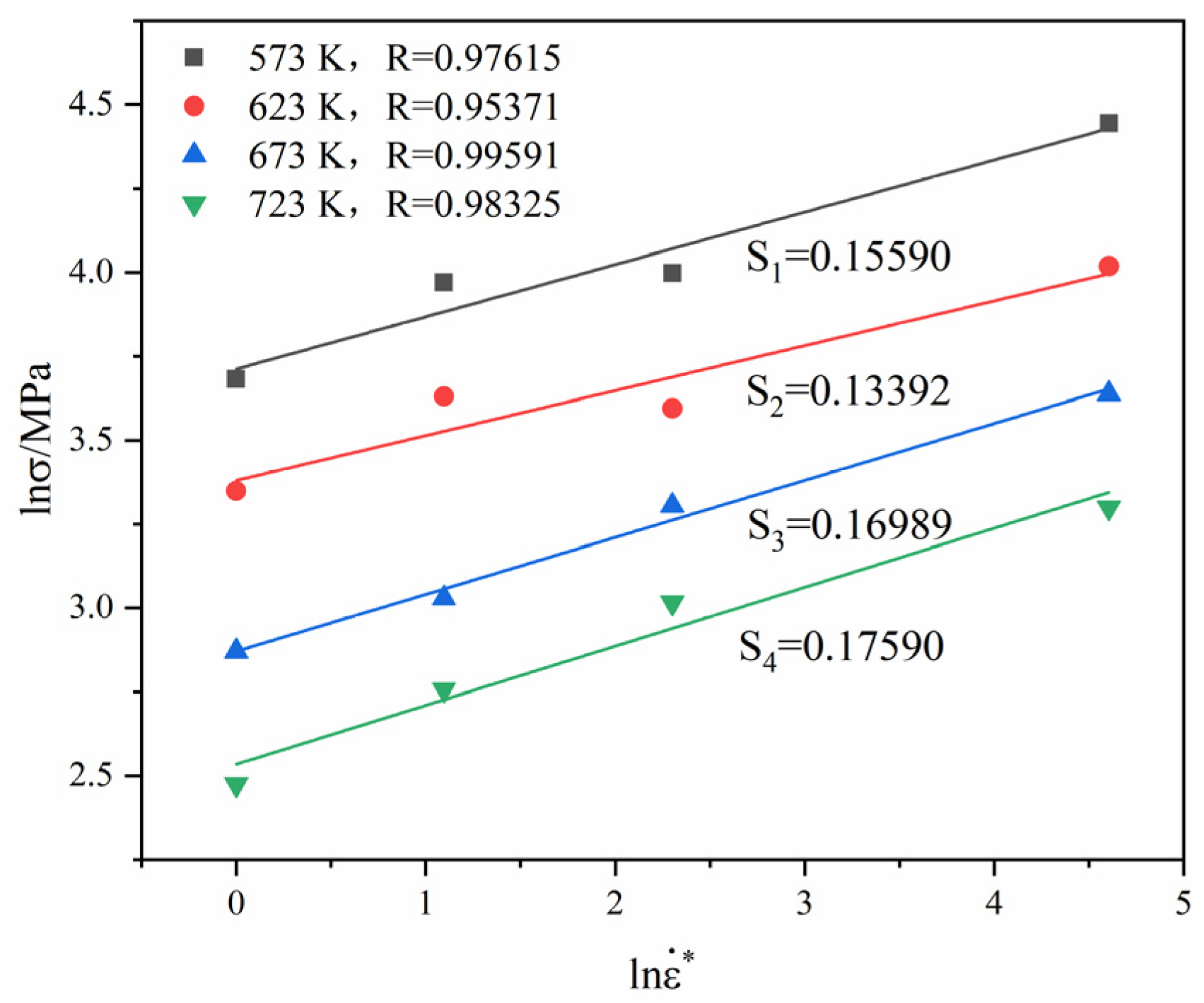
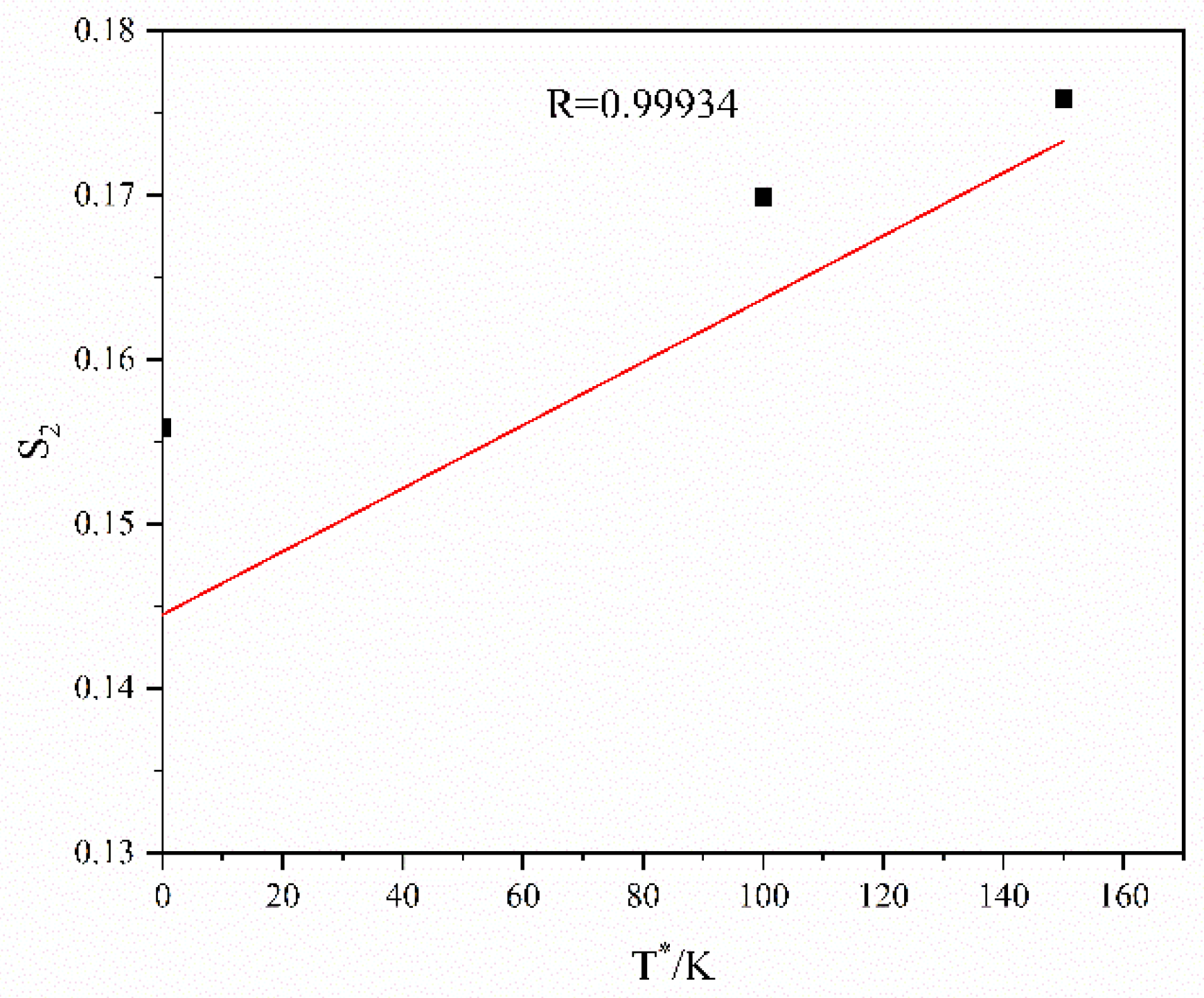


| Temperature/K | Strain Rate/s−1 | Grain Size/μm | Elongation/% |
|---|---|---|---|
| 573 K | 1.67 × 10−2 | 23 ± 4.1 | 121.6 |
| 1.67 × 10−3 | 22 ± 2.0 | 110.8 | |
| 5 × 10−4 | 17 ± 2.7 | 113.6 | |
| 1.67 × 10−4 | 19 ± 3.2 | 110.9 | |
| 623 K | 1.67 × 10−2 | 15 ± 1.5 | 155.3 |
| 1.67 × 10−3 | 19 ± 3.1 | 121.6 | |
| 5 × 10−4 | 16 ± 2.8 | 129.1 | |
| 1.67 × 10−4 | 18 ± 2.4 | 108.3 | |
| 673 K | 1.67 × 10−2 | 20 ± 3.0 | 189.1 |
| 1.67 × 10−3 | 17 ± 1.8 | 180.1 | |
| 5 × 10−4 | 34 ± 3.2 | 228.4 | |
| 1.67 × 10−4 | 38 ± 3.4 | 198.2 | |
| 723 K | 1.67 × 10−2 | 22 ± 2.9 | 190.7 |
| 1.67 × 10−3 | 35 ± 3.5 | 184.6 | |
| 5 × 10−4 | 40 ± 4.0 | 212.4 | |
| 1.67 × 10−4 | 42 ± 2.7 | 216.6 |
| Parameter | C1/MPa | C2/MPa | n | C3 | C4 | C5 | C6 |
|---|---|---|---|---|---|---|---|
| Value | 38.00 | 3.13 | 1.71943 | 0.01054 | −0.0027 | 0.15604 | 0.00013 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, F.; Guo, H.; Guo, N.; Kong, S.; Liang, J. Room-Temperature Strengthening, Portevin-Le Chatelier Effect, High-Temperature Tensile Deformation Behavior, and Constitutive Modeling in a Lightweight Mg-Gd-Al-Zn Alloy. Materials 2023, 16, 1639. https://doi.org/10.3390/ma16041639
Cao F, Guo H, Guo N, Kong S, Liang J. Room-Temperature Strengthening, Portevin-Le Chatelier Effect, High-Temperature Tensile Deformation Behavior, and Constitutive Modeling in a Lightweight Mg-Gd-Al-Zn Alloy. Materials. 2023; 16(4):1639. https://doi.org/10.3390/ma16041639
Chicago/Turabian StyleCao, Furong, Huizhen Guo, Nanpan Guo, Shuting Kong, and Jinrui Liang. 2023. "Room-Temperature Strengthening, Portevin-Le Chatelier Effect, High-Temperature Tensile Deformation Behavior, and Constitutive Modeling in a Lightweight Mg-Gd-Al-Zn Alloy" Materials 16, no. 4: 1639. https://doi.org/10.3390/ma16041639
APA StyleCao, F., Guo, H., Guo, N., Kong, S., & Liang, J. (2023). Room-Temperature Strengthening, Portevin-Le Chatelier Effect, High-Temperature Tensile Deformation Behavior, and Constitutive Modeling in a Lightweight Mg-Gd-Al-Zn Alloy. Materials, 16(4), 1639. https://doi.org/10.3390/ma16041639







