Application of the Variational Method to the Large Deformation Problem of Thin Cylindrical Shells with Different Moduli in Tension and Compression
Abstract
:1. Introduction
2. Background
3. Method and Problem
3.1. The Displacement Variational Method Based on Energy Conservation
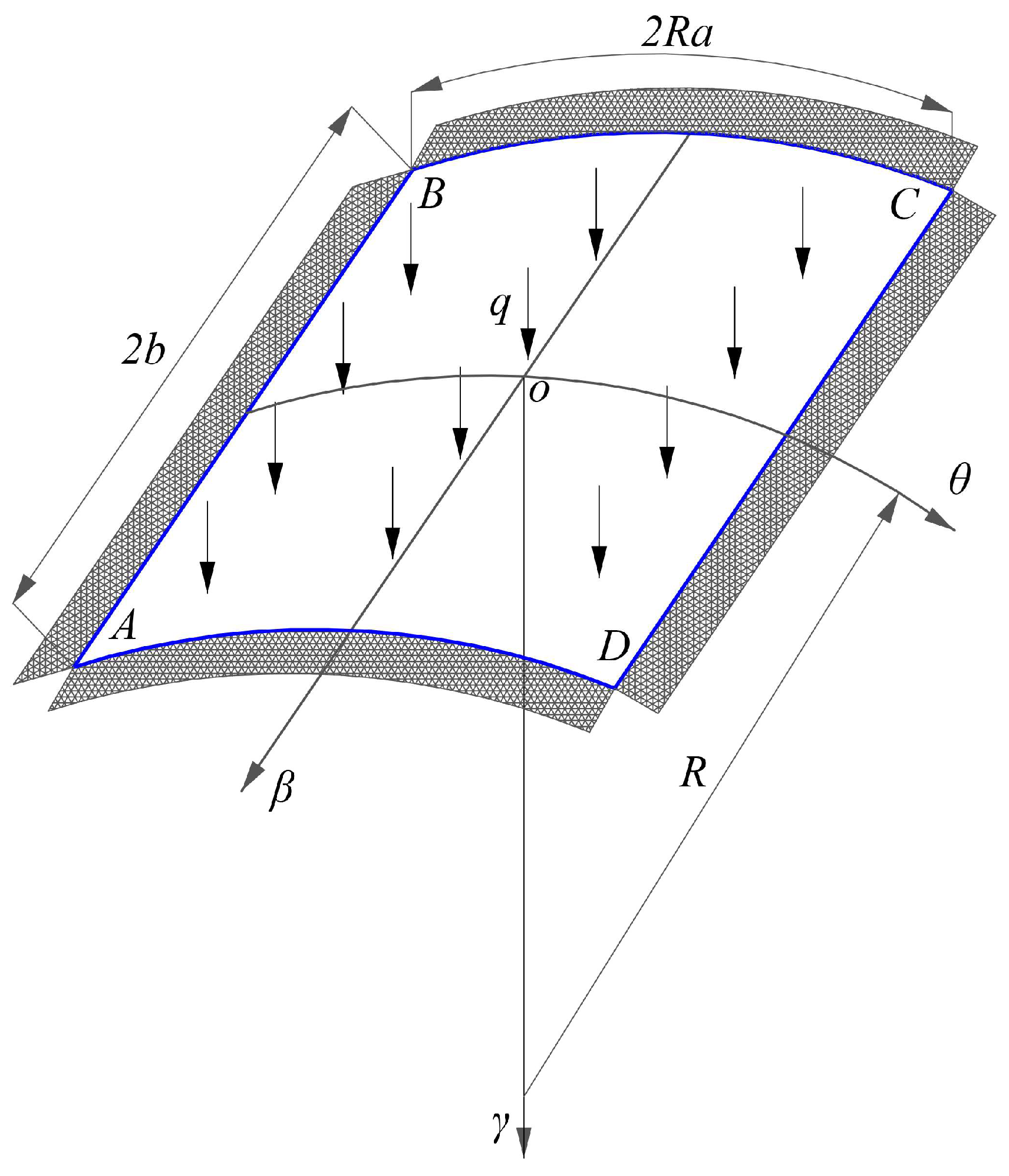
3.2. Describtion of Problem
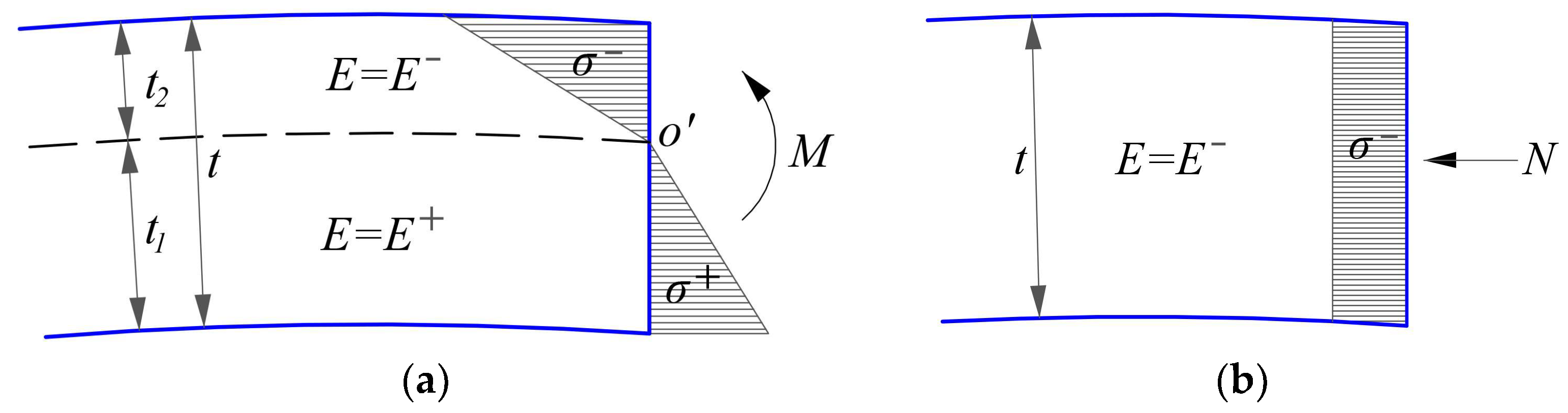
4. Physical Equations on Bimodular Materials Model
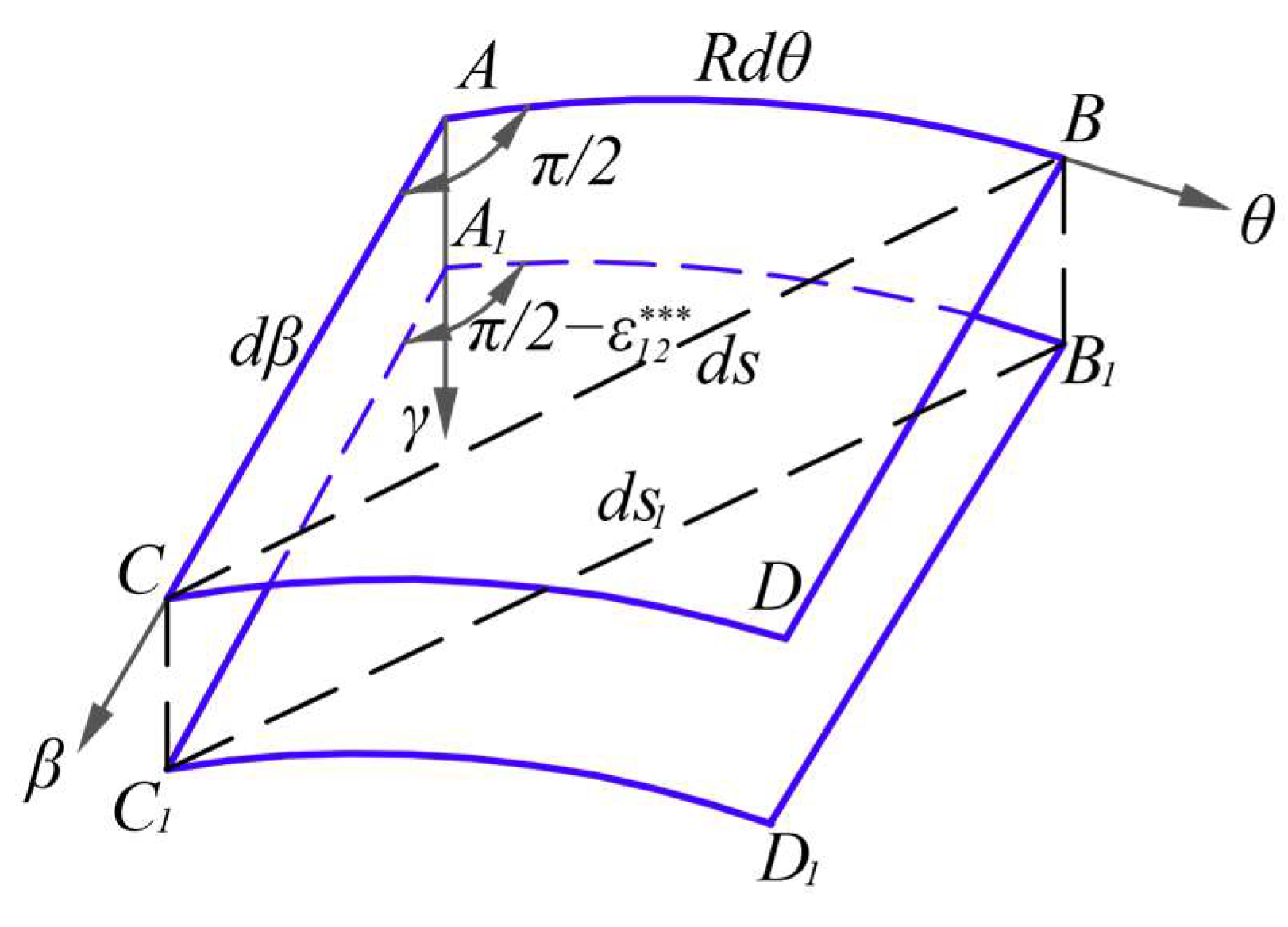
5. Geometrical Equations under Large Deformation
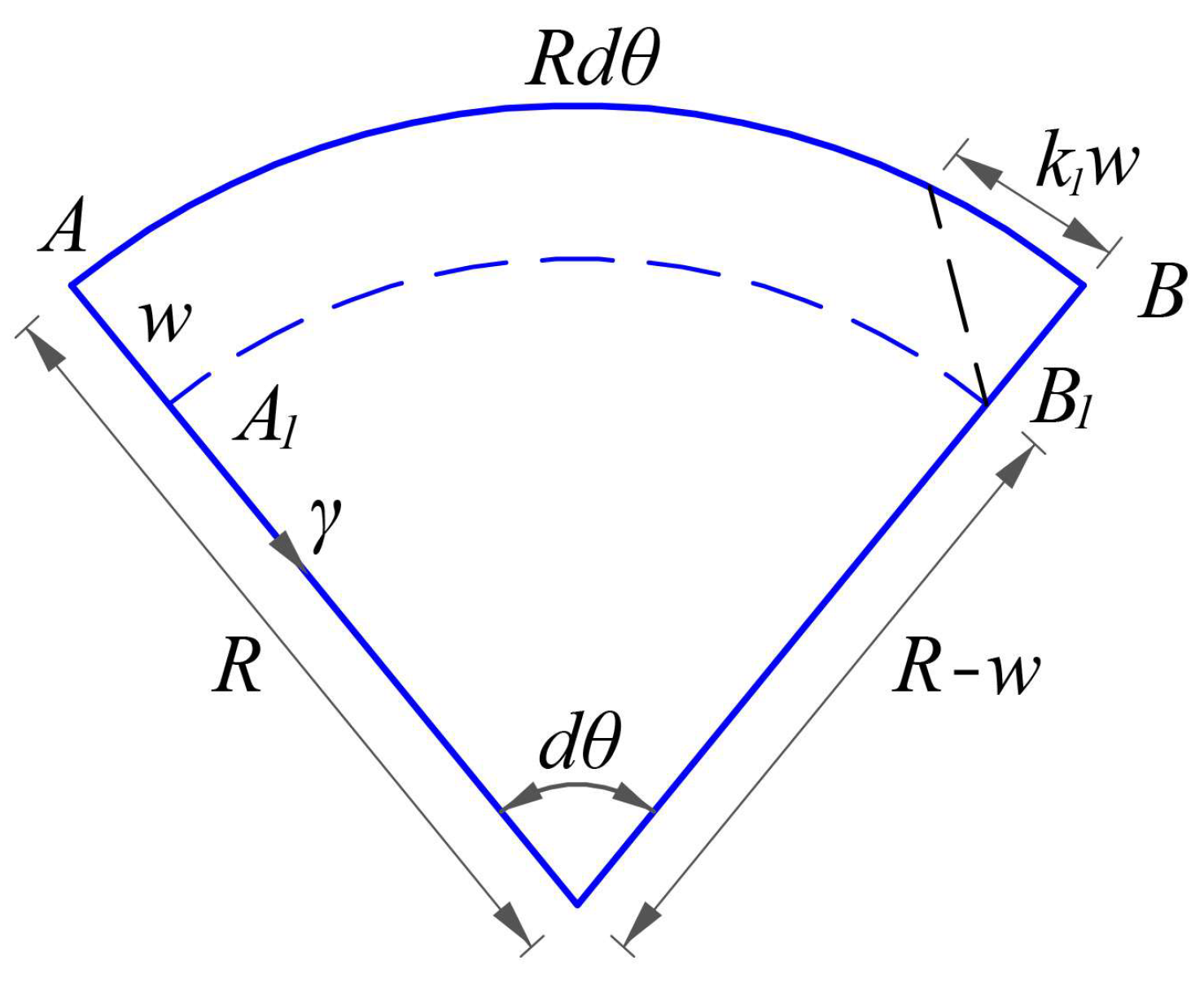
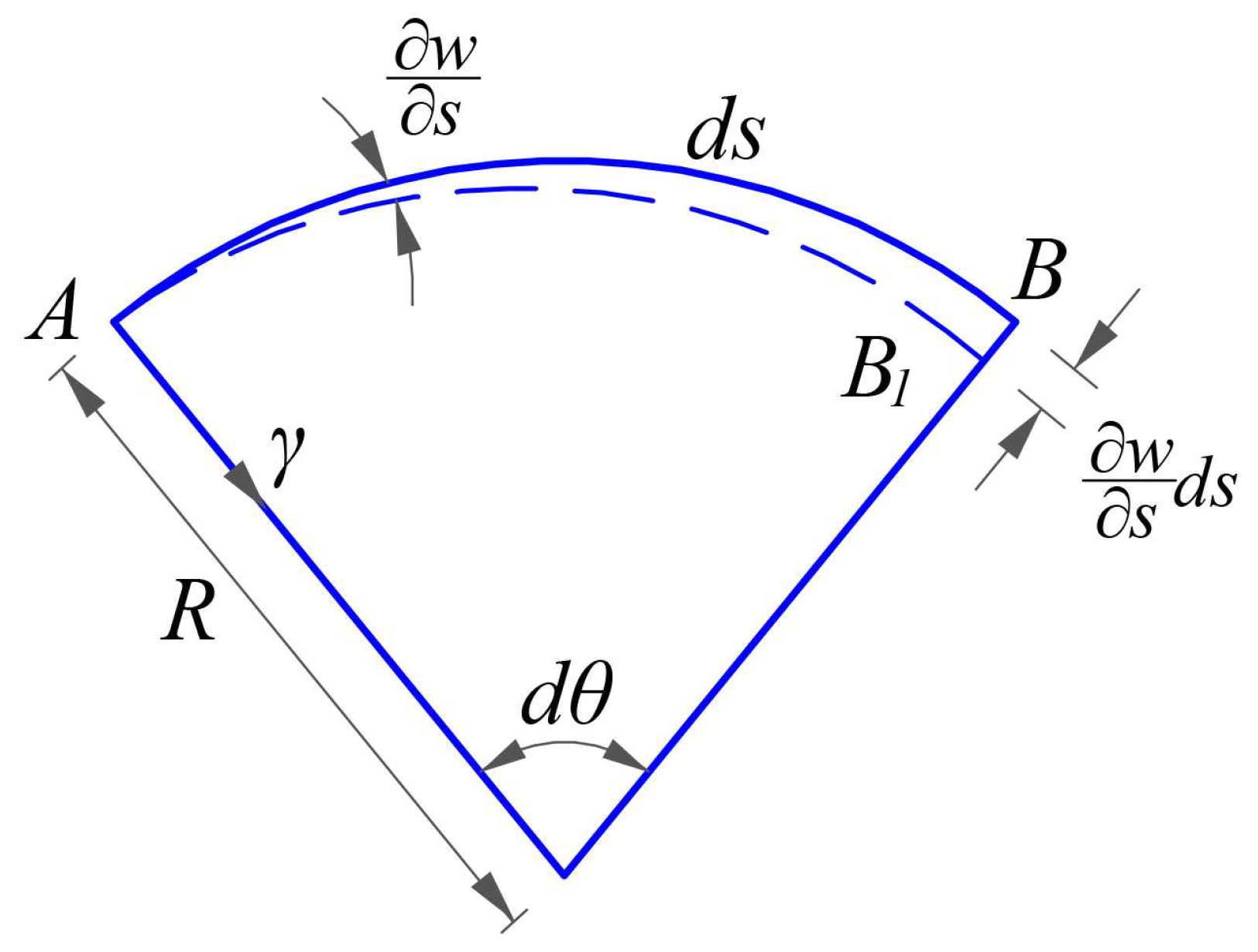
5.1. Geometrical Equation Concerning Bending and Torsion Moments
5.2. Geometrical Equation Concerning In-Plane Deformation
6. Application of the Displacement Variational Method
6.1. Total Strain Potencial Energy
6.2. The Ritz Method
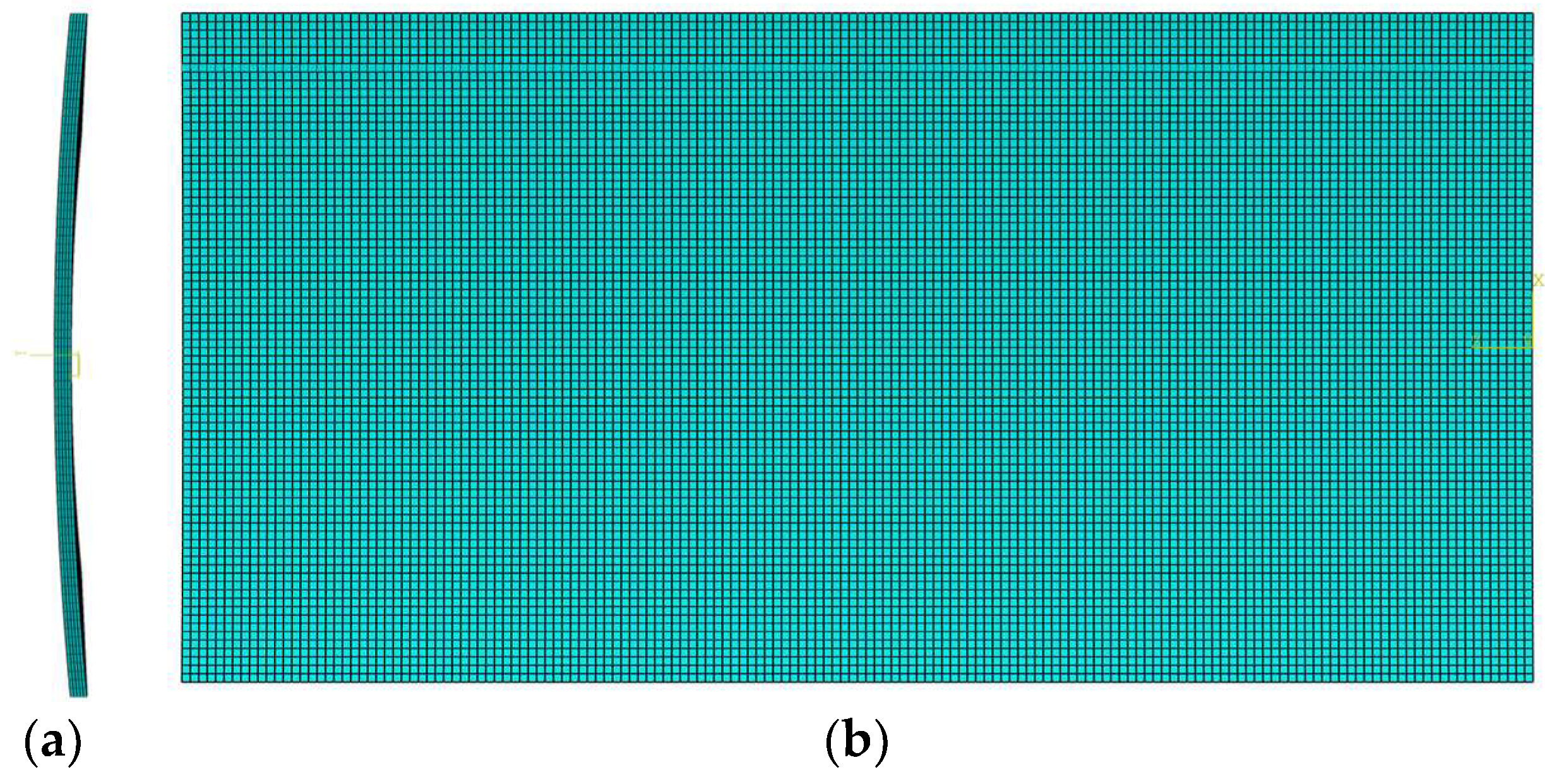
7. Numerical Simulation and Comparison with Theoretical Solution
- (i)
- First, the material is assumed to have a single modulus, and the stress and strain of each element are calculated;
- (ii)
- The principal stress and its direction for each element are thus obtained;
- (iii)
- According to principal stress obtained, the due constitutive relationship between each element is constructed, and the stiffness matrix of each element is collected to form the total stiffness matrix;
- (iv)
- According to the new constitutive relationship, once again, the stress and strain of each element is calculated, and repeat this process;
- (v)
- If reaching the stopping condition, output the final result; otherwise go to (ii).
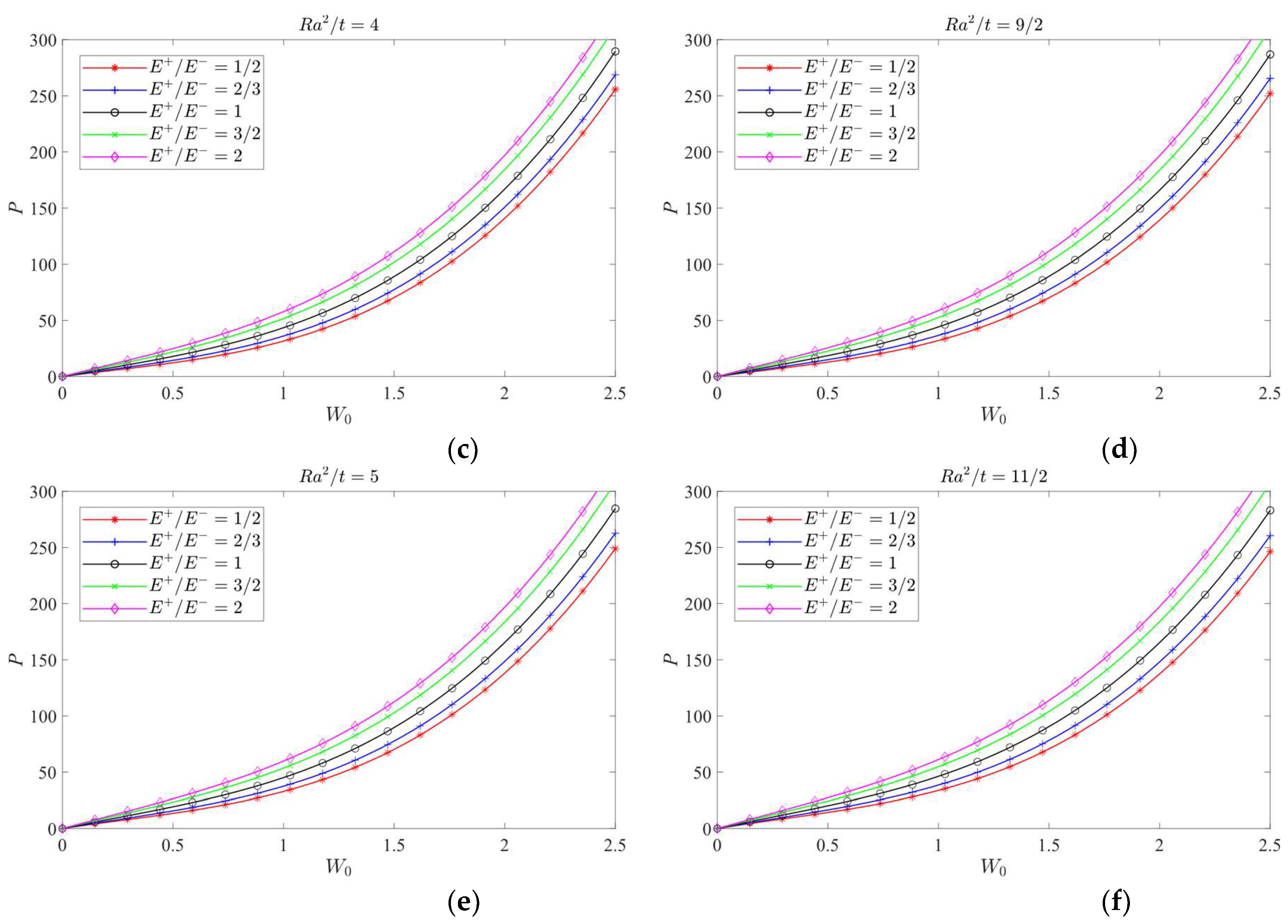
8. Results and Discussion
8.1. Load vs. Central Deflection
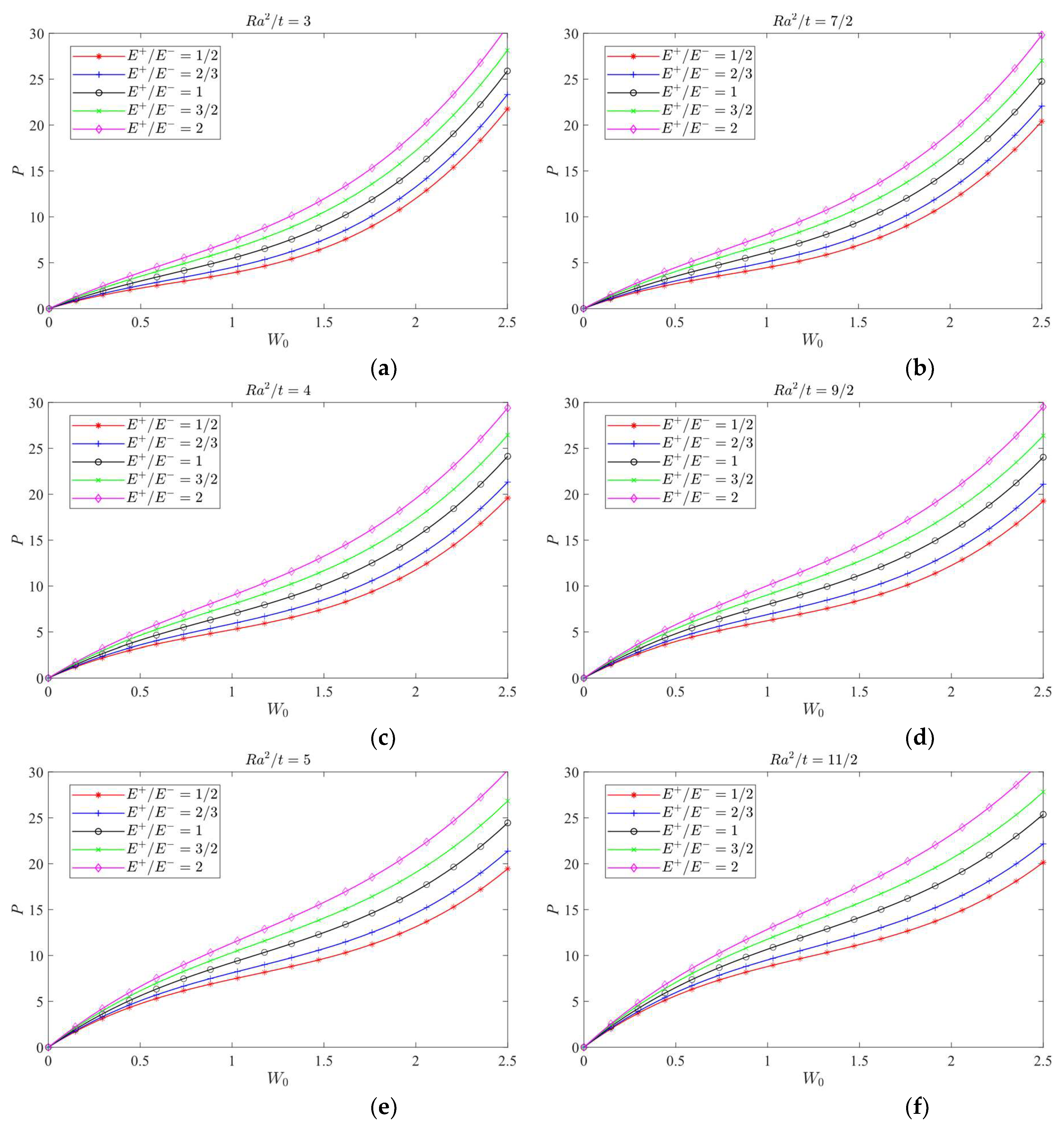
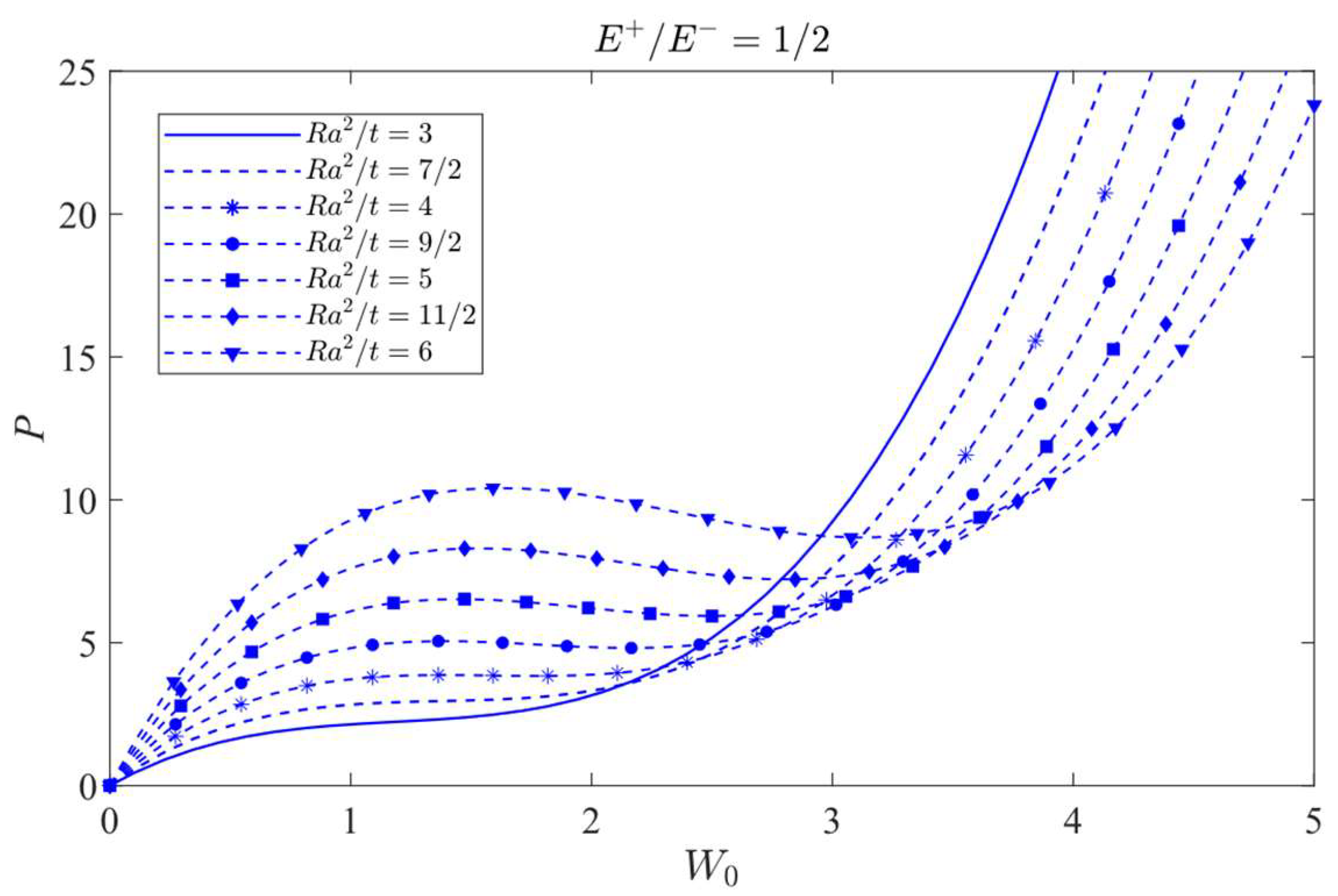
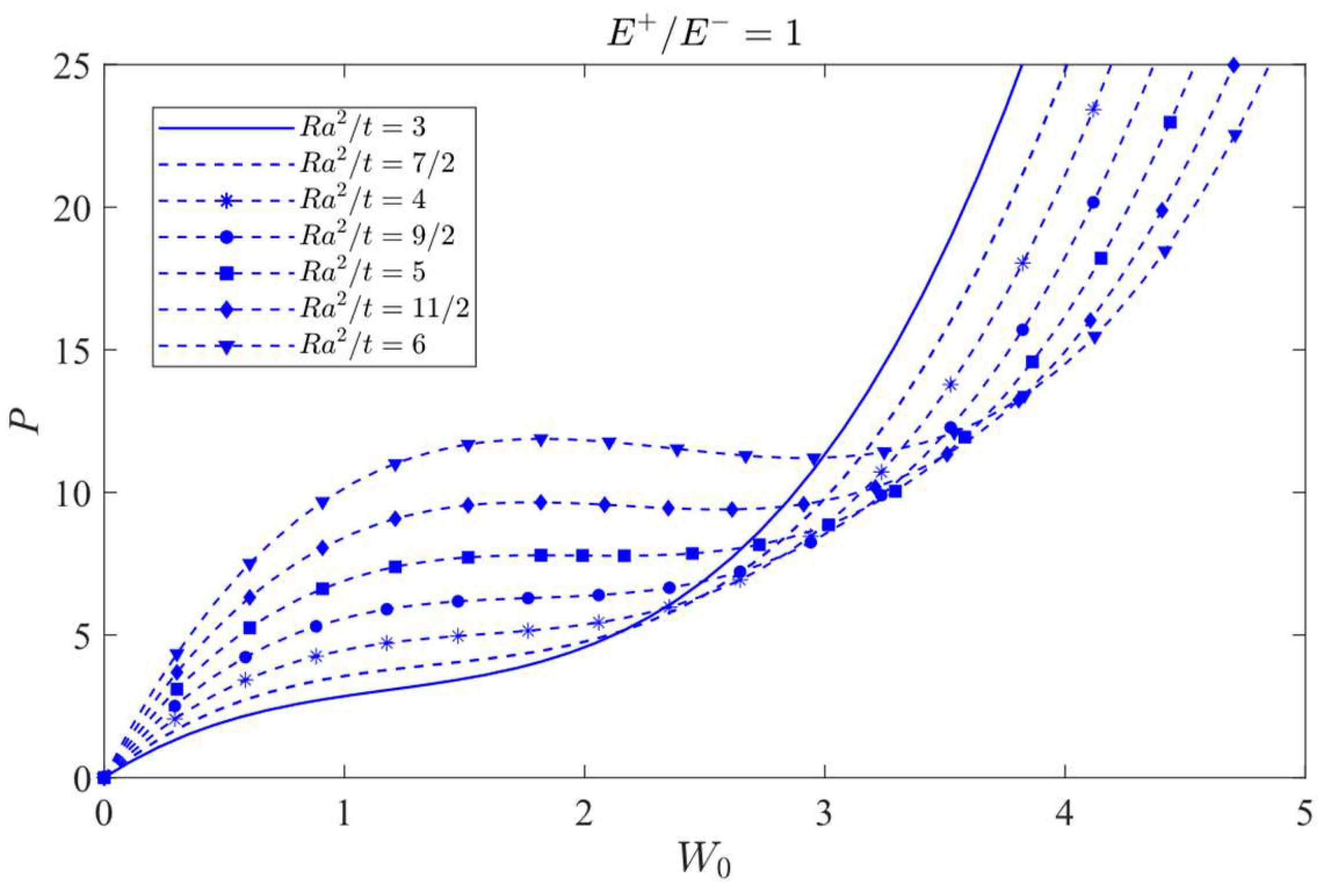
8.2. Jumping Phenomenon
9. Concluding Remarks
- (i)
- Under large deformation and using bimodular materials, the establishment of physical equations and the geometrical equation lays the foundation for the calculation of strain energy and then the application of the variational method.
- (ii)
- The bimodular effect will change the structural stiffness, thus resulting in the corresponding change in the deformation magnitude. Specifically, under the action of the same load, the central deflection of the cylindrical shell will decrease when E+ > E− while the central deflection of the cylindrical shell will increase when E+ < E−, in comparison with the central deflection when E+ = E−.
- (iii)
- When the shell is relatively thin, the bimodular effect will influence the occurrence of the jumping phenomenon of the cylindrical shell. Specifically, if λ = 1/2, that is, the arc length along the θ direction is half of line length along the β direction, the shell is a long shell, when E+/E− = 1/2, the critical value of Ra2/t is 3.80; when E+/E− = 1, the critical value of Ra2/t gives 4.89 and when E+/E− = 2, the critical value of Ra2/t changes to be 6.08.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Bakshi, K.; Chakravorty, D. Numerical study on failure of thin composite conoidal shell roofs considering geometric nonlinearity. KSCE J. Civ. Eng. 2020, 24, 913–921. [Google Scholar] [CrossRef]
- Arbocz, J.; Starnes, J.H., Jr. Future directions and challenges in shell stability analysis. Thin-Walled Struct. 2002, 40, 729–754. [Google Scholar] [CrossRef]
- Thai, H.T.; Kim, S.E. A review of theories for the modeling and analysis of functionally graded plates and shells. Compos. Struct. 2015, 128, 70–86. [Google Scholar] [CrossRef]
- Carrera, E.; Pagani, A.; Azzara, R.; Augello, R. Vibration of metallic and composite shells in geometrical nonlinear equilibrium states. Thin-Walled Struct. 2020, 157, 107131. [Google Scholar] [CrossRef]
- Ambartsumyan, S.A. Elasticity Theory of Different Moduli; Wu, R.F., Zhang, Y.Z., Translators; China Railway Publishing House: Beijing, China, 1986. [Google Scholar]
- Destrade, M.; Gilchrist, M.D.; Motherway, J.A.; Murphy, J.G. Bimodular rubber buckles early in bending. Mech. Mater. 2010, 42, 469–476. [Google Scholar] [CrossRef] [Green Version]
- Barak, M.M.; Currey, J.D.; Weiner, S.; Shahar, R. Are tensile and compressive Young’s moduli of compact bone different. J. Mech. Behav. Biomed. Mater. 2009, 2, 51–60. [Google Scholar] [CrossRef] [PubMed]
- Bertoldi, K.; Bigoni, D.; Drugan, W.J. Nacre: An orthotropic and bimodular elastic material. Compos. Sci. Technol. 2008, 68, 1363–1375. [Google Scholar] [CrossRef] [Green Version]
- Jones, R.M. Apparent flexural modulus and strength of multimodulus materials. J. Compos. Mater. 1976, 10, 342–354. [Google Scholar] [CrossRef]
- Bert, C.W. Models for fibrous composites with different properties in tension and compression. ASME J. Eng. Mater. Technol. 1977, 99, 344–349. [Google Scholar] [CrossRef]
- Reddy, J.N.; Chao, W.C. Nonlinear bending of bimodular material plates. Int. J. Solids Struct. 1983, 19, 229–237. [Google Scholar] [CrossRef]
- Zinno, R.; Greco, F. Damage evolution in bimodular laminated composite under cyclic loading. Compos. Struct. 2001, 53, 381–402. [Google Scholar] [CrossRef]
- Khan, A.H.; Patel, B.P. Nonlinear periodic response of bimodular laminated composite annular sector plates. Compos. Part B Eng. 2019, 169, 96–108. [Google Scholar] [CrossRef]
- Li, X.; Sun, J.-Y.; Dong, J.; He, X.-T. One-dimensional and two-dimensional analytical solutions for functionally graded beams with different moduli in tension and compression. Materials 2018, 11, 830. [Google Scholar] [CrossRef] [Green Version]
- He, X.-T.; Li, W.-M.; Sun, J.-Y.; Wang, Z.-X. An elasticity solution of functionally graded beams with different moduli in tension and compression. Mech. Adv. Mater. Struct. 2018, 25, 143–154. [Google Scholar] [CrossRef]
- He, X.-T.; Pei, X.-X.; Sun, J.-Y.; Zheng, Z.-L. Simplified theory and analytical solution for functionally graded thin plates with different moduli in tension and compression. Mech. Res. Commun. 2016, 74, 72–80. [Google Scholar] [CrossRef]
- Ye, Z.M.; Chen, T.; Yao, W.J. Progresses in elasticity theory with different moduli in tension and compression and related FEM. Mech. Engin. 2004, 26, 9–14. [Google Scholar]
- Sun, J.Y.; Zhu, H.Q.; Qin, S.H.; Yang, D.L.; He, X.T. A review on the research of mechanical problems with different moduli in tension and compression. J. Mech. Sci. Technol. 2010, 24, 1845–1854. [Google Scholar] [CrossRef]
- Du, Z.L.; Zhang, Y.P.; Zhang, W.S.; Guo, X. A new computational framework for materials with different mechanical responses in tension and compression and its applications. Int. J. Solids Struct. 2016, 100–101, 54–73. [Google Scholar] [CrossRef]
- Ma, J.W.; Fang, T.C.; Yao, W.J. Nonlinear large deflection buckling analysis of compression rod with different moduli. Mech. Adv. Mater. Struct. 2019, 26, 539–551. [Google Scholar] [CrossRef]
- Simitses, G.J. Buckling of moderately thick laminated cylindrical shells: A review. Compos. Part B 1996, 27, 581–587. [Google Scholar] [CrossRef]
- Zheng, J.Y.; Sun, G.Y.; Wang, L.Q. A review of development in layered vessels using flat-ribbon-wound cylindrical shells. Int. J. Pres. Ves. Pip. 1998, 75, 653–659. [Google Scholar] [CrossRef]
- Arefi, M.; Kiani Moghaddam, S.; Mohammad-Rezaei Bidgoli, E.; Kiani, M.; Civalek, O. Analysis of graphene nanoplatelet reinforced cylindrical shell subjected to thermo-mechanical loads. Compos. Struct. 2021, 255, 112924. [Google Scholar] [CrossRef]
- Li, M.; Zhu, H.; Lai, C.; Bao, W.; Han, H.; Lin, R.; He, W.; Fan, H. Recent progresses in lightweight carbon fibre reinforced lattice cylindrical shells. Prog. Aerosp. Sci. 2022, 135, 100860. [Google Scholar] [CrossRef]
- Pasternak, H.; Li, Z.; Juozapaitis, A.; Daniūnas, A. Ring stiffened cylindrical shell structures: State-of-the-art review. Appl. Sci. 2022, 12, 11665. [Google Scholar] [CrossRef]
- Wang, B.; Hao, P.; Ma, X.; Tian, K. Knockdown factor of buckling load for axially compressed cylindrical shells: State of the art and new perspectives. Acta Mech. Sin. 2022, 38, 421440. [Google Scholar] [CrossRef]
- Alshabatat, N.T. Natural frequencies optimization of thin-walled circular cylindrical shells using axially functionally graded materials. Materials 2022, 15, 698. [Google Scholar] [CrossRef]
- Zhangabay, N.; Sapargaliyeva, B.; Utelbayeva, A.; Kolesnikov, A.; Aldiyarov, Z.; Dossybekov, S.; Esimov, E.; Duissenbekov, B.; Fediuk, R.; Vatin, N.I.; et al. Experimental analysis of the stress state of a prestressed cylindrical shell with various structural parameters. Materials 2022, 15, 4996. [Google Scholar] [CrossRef]
- Sofiyev, A.H.; Fantuzzi, N.; Ipek, C.; Tekin, G. Buckling behavior of sandwich cylindrical shells covered by functionally graded coatings with clamped boundary conditions under hydrostatic pressure. Materials 2022, 15, 8680. [Google Scholar] [CrossRef]
- Chien, W.Z. Large deflection of a circular clamped plate under uniform pressure. Chin. J. Phys. 1947, 7, 102–113. [Google Scholar]
- Xu, Z.L. Elasticity, 5th ed.; Higher Education Press: Beijing, China, 2016. [Google Scholar]
- Xue, X.-Y.; Du, D.-W.; Sun, J.-Y.; He, X.-T. Application of variational method to stability analysis of cantilever vertical plates with bimodular effect. Materials 2021, 14, 6129. [Google Scholar] [CrossRef]
- He, X.-T.; Chang, H.; Sun, J.-Y. Axisymmetric large deformation problems of thin shallow shells with different moduli in tension and compression. Thin-Walled Struct. 2023, 182, 110297. [Google Scholar] [CrossRef]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Volmir, A.C. Flexible Plates and Shells; Lu, W.D., Huang, Z.Y., Lu, D.H., Translators; Science Press: Beijing, China, 1959. [Google Scholar]
- He, X.T.; Hu, X.J.; Sun, J.Y.; Zheng, Z.L. An analytical solution of bending thin plates with different moduli in tension and compression. Struct. Eng. Mech. 2010, 36, 363–380. [Google Scholar] [CrossRef]


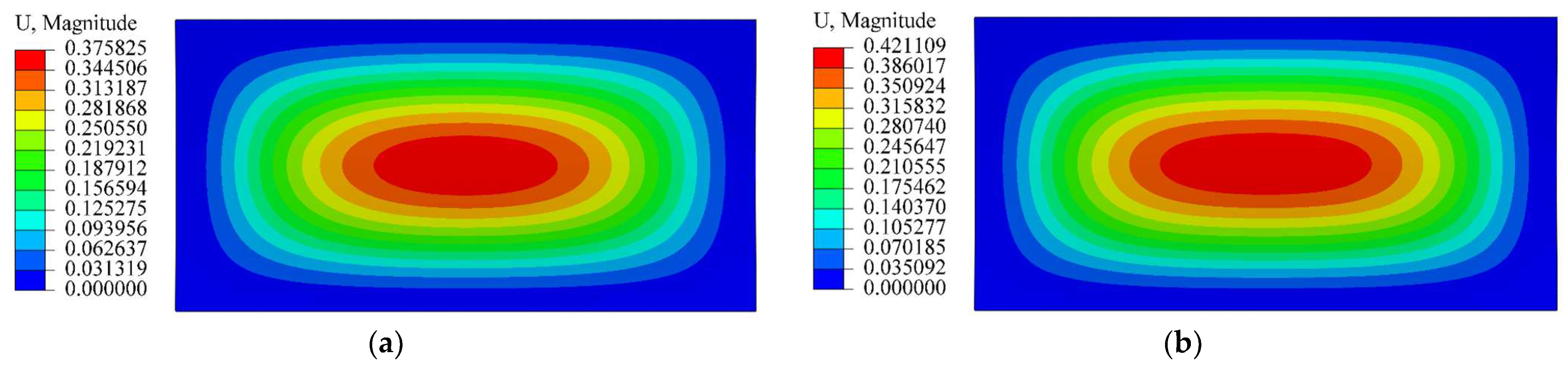





| E+/E− | q (Kpa) | Central Deflection w0 (m) | |
|---|---|---|---|
| Variational Method | FEM | ||
| 1/2 | 15 | 0.262811 | 0.227548 |
| 20 | 0.297710 | 0.290979 | |
| 25 | 0.326251 | 0.340906 | |
| 30 | 0.350656 | 0.382729 | |
| 2/3 | 15 | 0.267203 | 0.230800 |
| 20 | 0.304338 | 0.294299 | |
| 25 | 0.334660 | 0.344613 | |
| 30 | 0.360554 | 0.385766 | |
| 1 | 15 | 0.277442 | 0.295023 |
| 20 | 0.318139 | 0.345777 | |
| 25 | 0.351337 | 0.384648 | |
| 30 | 0.379657 | 0.417361 | |
| 3/2 | 15 | 0.294951 | 0.271896 |
| 20 | 0.339607 | 0.318476 | |
| 25 | 0.376050 | 0.355947 | |
| 30 | 0.407135 | 0.388520 | |
| 2 | 15 | 0.312865 | 0.275682 |
| 20 | 0.360583 | 0.325684 | |
| 25 | 0.399556 | 0.361290 | |
| 30 | 0.432812 | 0.393627 | |
| E+/E− | q (Kpa) | Central Deflection w0 (m) | |
|---|---|---|---|
| Variational Method | FEM | ||
| 1/2 | 15 | 0.294340 | 0.217232 |
| 20 | 0.331284 | 0.326770 | |
| 25 | 0.361030 | 0.404759 | |
| 30 | 0.386234 | 0.462075 | |
| 2/3 | 15 | 0.297410 | 0.221017 |
| 20 | 0.336883 | 0.320205 | |
| 25 | 0.368587 | 0.395324 | |
| 30 | 0.395397 | 0.450756 | |
| 1 | 15 | 0.306059 | 0.223635 |
| 20 | 0.349428 | 0.308476 | |
| 25 | 0.384217 | 0.375825 | |
| 30 | 0.413595 | 0.428147 | |
| 3/2 | 15 | 0.322687 | 0.296831 |
| 20 | 0.370170 | 0.368591 | |
| 25 | 0.408309 | 0.421109 | |
| 30 | 0.440528 | 0.461153 | |
| 2 | 15 | 0.340483 | 0.327177 |
| 20 | 0.391018 | 0.375792 | |
| 25 | 0.431690 | 0.428531 | |
| 30 | 0.466087 | 0.471214 | |
| E+/E− | q (Kpa) | Central Deflection w0 (m) | |
|---|---|---|---|
| Variational Method | FEM | ||
| 1/2 | 15 | 0.324920 | 0.214879 |
| 20 | 0.364591 | 0.269926 | |
| 25 | 0.395850 | 0.450152 | |
| 30 | 0.422018 | 0.545194 | |
| 2/3 | 15 | 0.326456 | 0.243589 |
| 20 | 0.369030 | 0.288025 | |
| 25 | 0.402457 | 0.470796 | |
| 30 | 0.430367 | 0.572830 | |
| 1 | 15 | 0.333323 | 0.277248 |
| 20 | 0.380188 | 0.306887 | |
| 25 | 0.416937 | 0.392184 | |
| 30 | 0.447571 | 0.511123 | |
| 3/2 | 15 | 0.349096 | 0.290966 |
| 20 | 0.400188 | 0.418038 | |
| 25 | 0.440379 | 0.500748 | |
| 30 | 0.473923 | 0.558026 | |
| 2 | 15 | 0.366912 | 0.346429 |
| 20 | 0.420965 | 0.464555 | |
| 25 | 0.463658 | 0.519060 | |
| 30 | 0.499369 | 0.561576 | |
| η | E+ | E− | E+/E− | μ+ | μ− | t1/t | t2/t | A | K |
|---|---|---|---|---|---|---|---|---|---|
| −1/3 | 2/3E | 4/3E | 1/2 | 0.2 | 0.4 | 0.6019 | 0.3981 | 0.1585 | 0.2516 |
| −1/5 | 4/5E | 6/5E | 2/3 | 0.24 | 0.36 | 0.5603 | 0.4397 | 0.1933 | 0.2665 |
| 0 | E | E | 1 | 0.3 | 0.3 | 0.5 | 0.5 | 0.25 | 0.2747 |
| 1/5 | 6/5E | 4/5E | 3/2 | 0.36 | 0.24 | 0.4397 | 0.5603 | 0.3140 | 0.2665 |
| 1/3 | 4/3E | 2/3E | 2 | 0.4 | 0.2 | 0.3981 | 0.6019 | 0.3623 | 0.2516 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, X.-T.; Wang, X.-G.; Sun, J.-Y. Application of the Variational Method to the Large Deformation Problem of Thin Cylindrical Shells with Different Moduli in Tension and Compression. Materials 2023, 16, 1686. https://doi.org/10.3390/ma16041686
He X-T, Wang X-G, Sun J-Y. Application of the Variational Method to the Large Deformation Problem of Thin Cylindrical Shells with Different Moduli in Tension and Compression. Materials. 2023; 16(4):1686. https://doi.org/10.3390/ma16041686
Chicago/Turabian StyleHe, Xiao-Ting, Xiao-Guang Wang, and Jun-Yi Sun. 2023. "Application of the Variational Method to the Large Deformation Problem of Thin Cylindrical Shells with Different Moduli in Tension and Compression" Materials 16, no. 4: 1686. https://doi.org/10.3390/ma16041686
APA StyleHe, X.-T., Wang, X.-G., & Sun, J.-Y. (2023). Application of the Variational Method to the Large Deformation Problem of Thin Cylindrical Shells with Different Moduli in Tension and Compression. Materials, 16(4), 1686. https://doi.org/10.3390/ma16041686






