Experimental Studies on the Seismic Performance of Prefabricated Circular Hollow Bridge Piers Constructed with PVA Fiber Concrete
Abstract
:1. Introduction
2. Experimental Program and Setup
2.1. Model Design and Material Properties
2.2. Loading Setup and Test Instrument
2.3. Loading Protocol
3. Test Results and Discussion
3.1. General Behavior and Mode of Failure
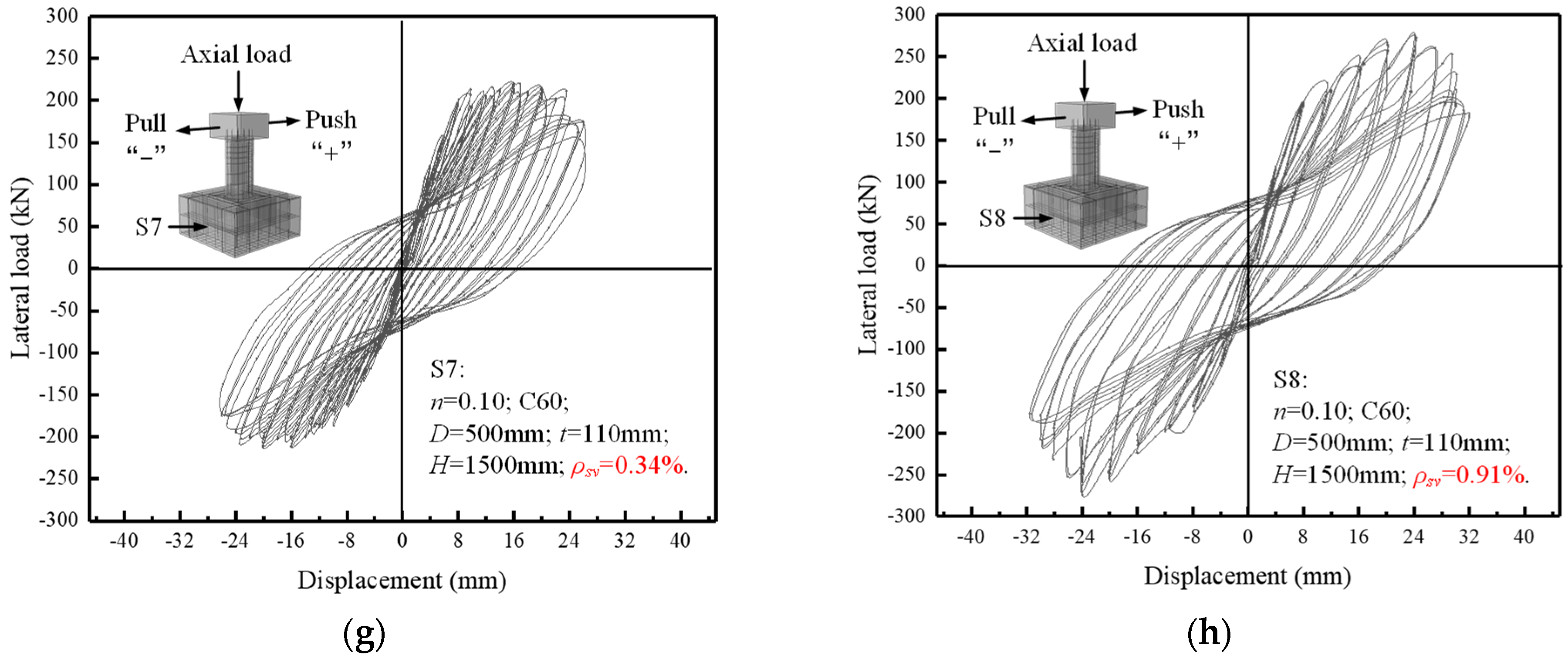
3.2. Load–Displacement Behavior
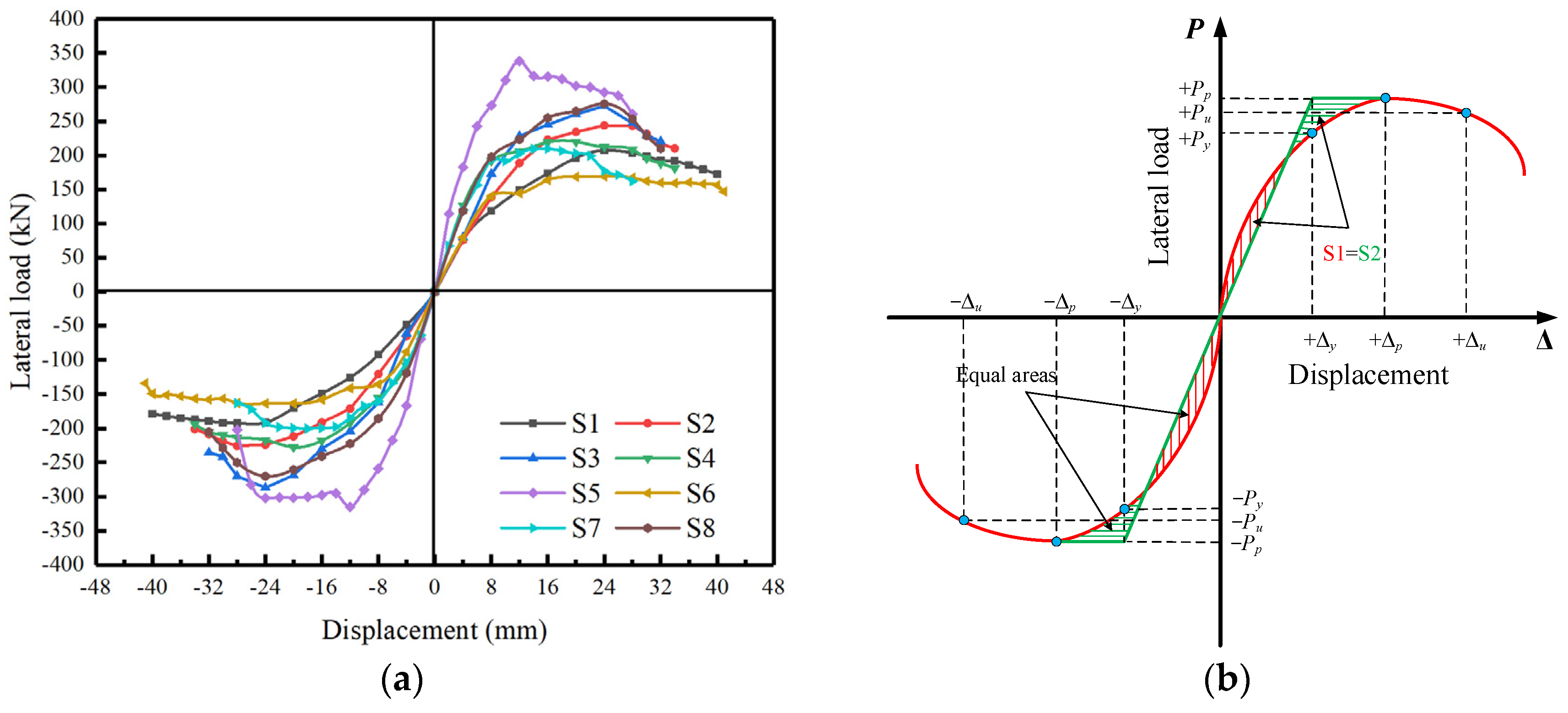
3.3. Skeleton Curve and Ductility
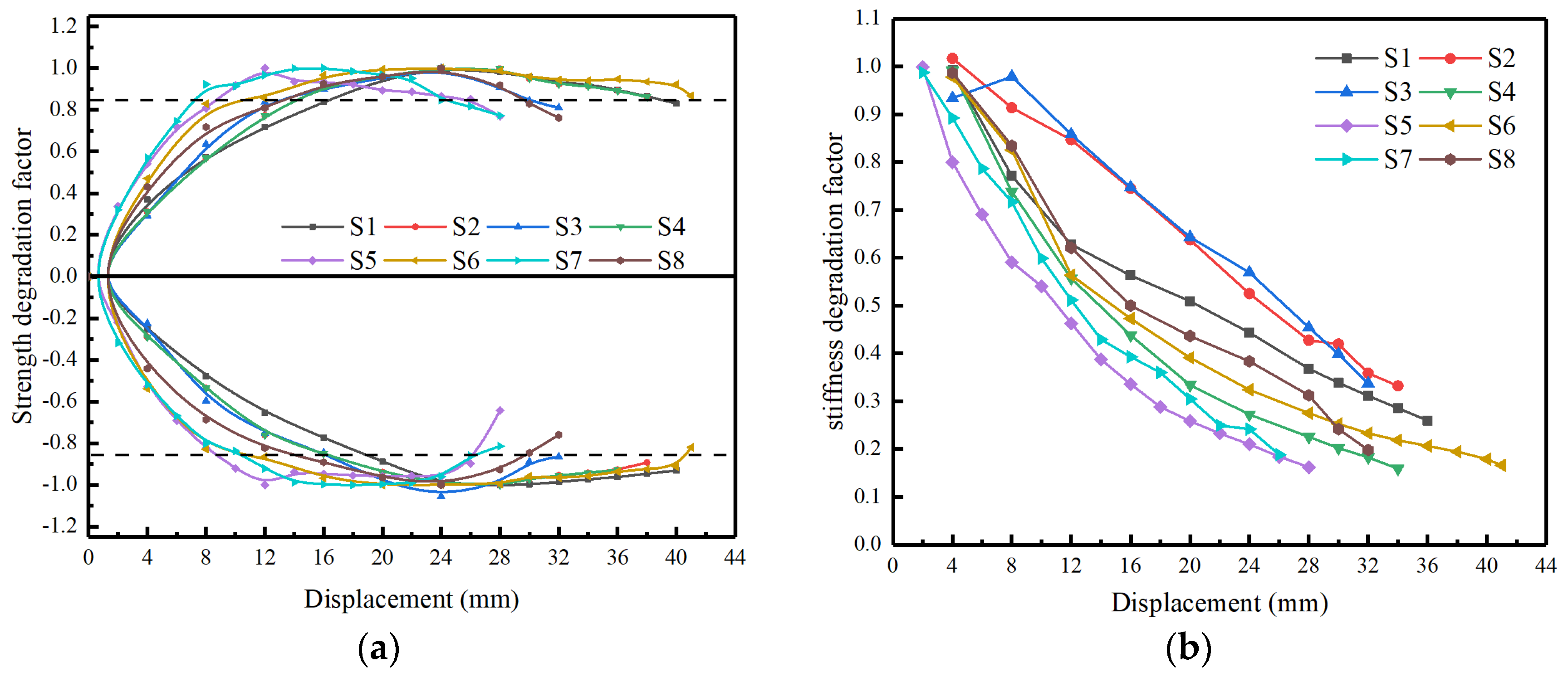
3.4. Degradation of Strength and Stiffness
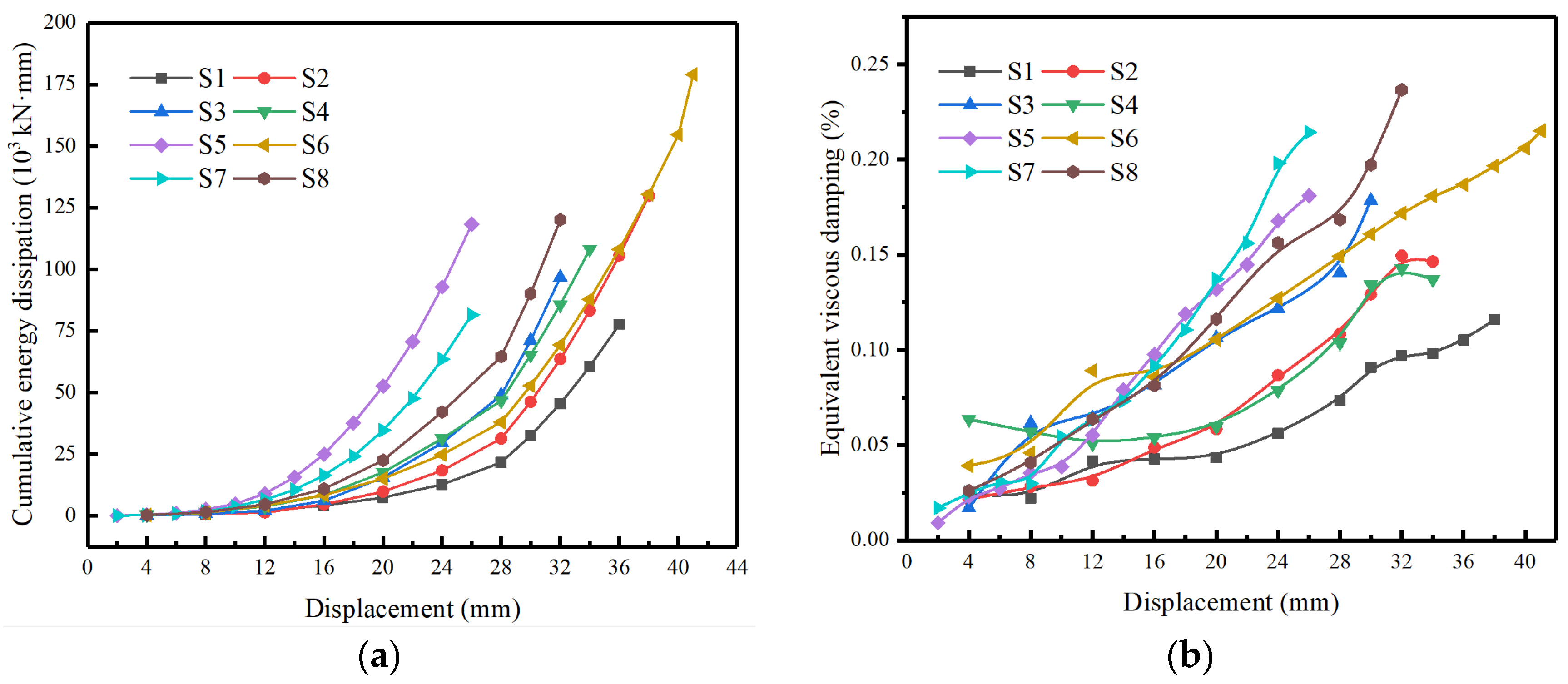
3.5. Energy Dissipation and Viscous Damping
4. Derivation of Shear Strength Model
4.1. Existing Shear Strength Models
4.2. Proposed Shear Strength Model
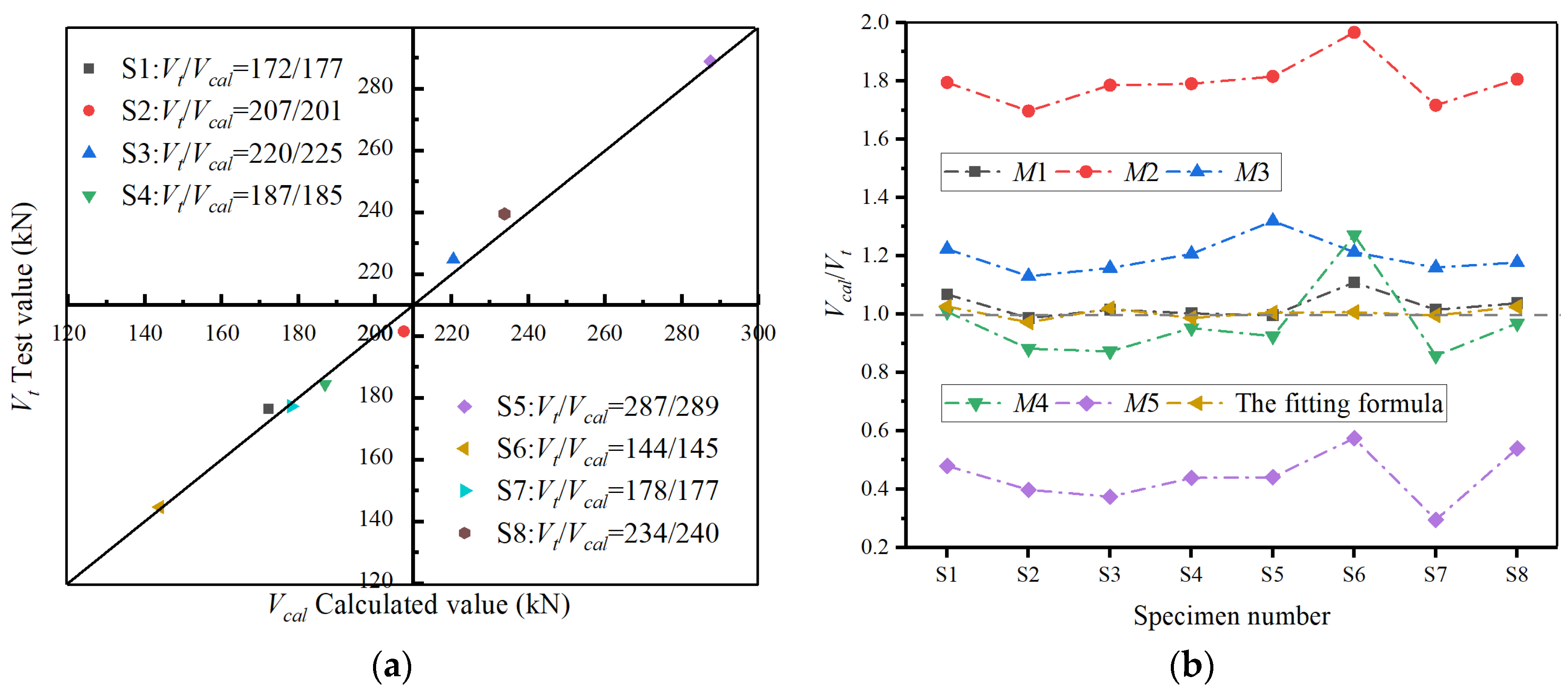
4.3. Comparison of Existing Shear Models’ Applicability
5. Conclusions
- (1)
- Excessive axial compression ratio (>15%) and stirrup ratio (>0.91%) will lead to a more significant spalling phenomenon when the specimen is damaged. The use of PVA concrete can produce finer cracks during the failure of the specimen, thus effectively improving the ductility and apparent failure morphology of the specimen.
- (2)
- The bearing capacity and energy dissipation capacity of specimens are positively correlated with the axial compression ratio (5–15%). However, the excessive axial compression ratio will lead to insufficient ductility. The shear strength of specimens is negatively correlated with the shear-span ratio and positively correlated with the stirrup ratio, and the increase in the stirrup ratio and shear-span ratio caused by the change in pile height can effectively improve the ductility of specimens.
- (3)
- In a certain range, the increase in axial compression ratio, stirrup ratio, and shear-span ratio caused by the change in pier height can significantly improve the energy dissipation characteristics of specimens, and the increase in the axial compression ratio will lead to a less apparent degradation of strength in the early stage and more significant degradation of strength in the later loading stage.
- (4)
- The shear bearing capacity formula of plastic hinge area fitted in this study can effectively predict the shear bearing capacity of the circular hollow pier. Among the existing shear bearing capacity models, the formulas of Priestley and Sezen overestimate the shear capacity of circular hollow piers while the Aschheim and ACI 318-14 formulas have good applicability for predicting the shear capacity of such specimens in the plastic hinge area.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fu, T.; Wang, K.; Zhu, Z.; Ren, X.; Li, Y.; Xu, L.; Meng, L.; Sun, Z. Seismic performance of prefabricated square hollow section piers strengthened by jacketing using UHPC and high-strength steel. Structures 2023, 47, 449–465. [Google Scholar] [CrossRef]
- Fu, T.; Zhu, Z.; Liu, Y.; Zhu, J. Study on the seismic performance of prefabricated square pier with hollow interlayer. Soil Dyn. Earthq. Eng. 2022, 158, 107312. [Google Scholar] [CrossRef]
- Culmo, M.P. Accelerated Bridge Construction—Experience in Design, Fabrication and Erection of Prefabricated Bridge Elements and Systems; Federal Highway Administration. Office of Bridge Technology: McLean, VA, USA, 2011. [Google Scholar]
- Shim, C.; Park, S.; Lee, S.; Koem, C. Cyclic Tests on Prefabricated Bridge Piers. In Proceedings of the Advances in Structural Engineering and Mechanics (ASEM15), Incheon, Republic of Korea, 25–29 August 2015. [Google Scholar]
- Echevarria, A.; Zaghi, A.E.; Christenson, R.; Accorsi, M. CFFT Bridge Columns for Multihazard Resilience. J. Struct. Eng. 2016, 142, C4015002. [Google Scholar] [CrossRef]
- Shao, C.; Qi, Q.; Wang, M.; Xiao, Z.; Wei, W.; Hu, C.; Xiao, L. Experimental study on the seismic performance of round-ended hollow piers. Eng. Struct. 2019, 195, 309–323. [Google Scholar] [CrossRef]
- Pang, Y.; Wei, K.; Yuan, W. Life-cycle seismic resilience assessment of highway bridges with fiber-reinforced concrete piers in the corrosive environment. Eng. Struct. 2020, 222, 111120. [Google Scholar] [CrossRef]
- Forcellini, D. A Resilience-Based Methodology to Assess Soil Structure Interaction on a Benchmark Bridge. Infrastructures 2020, 5, 90. [Google Scholar] [CrossRef]
- Gidaris, I.; Padgett, J.E.; Barbosa, A.R.; Chen, S.; Cox, D.; Webb, B.; Cerato, A. Multiple-Hazard Fragility and Restoration Models of Highway Bridges for Regional Risk and Resilience Assessment in the United States: State-of-the-Art Review. J. Struct. Eng. 2017, 143, 04016188. [Google Scholar] [CrossRef]
- Pantelides, C.P.; Ameli, M.J.; Parks, J.E.; Brown, D.N. Seismic evaluation of grouted splice sleeve connections for reinforced precast concrete column–to–cap beam joints in accelerated bridge construction. PCI J. 2015, 60, 80–103. [Google Scholar]
- Ameli, M.J.; Pantelides, C.P. Seismic Analysis of Precast Concrete Bridge Columns Connected with Grouted Splice Sleeve Connectors. J. Struct. Eng. 2016, 143, 04016176.1–04016176.13. [Google Scholar] [CrossRef]
- Lu, Z.; Huang, J.; Dai, S.; Liu, J.; Zhang, M. Experimental study on a precast beam-column joint with double grouted splice sleeves. Eng. Struct. 2019, 199, 109589. [Google Scholar] [CrossRef]
- Han, Y.; Dong, J.; Wang, L. Experimental Study on the Seismic Performance of Socket Bridge Piers. Adv. Civ. Eng. 2020, 2020, 8895196. [Google Scholar] [CrossRef]
- Han, Y.; Liu, Z.; Wang, L. Test for Influence of Socket Connection Structure on Dynamic Response of Prefabricated Pier under Vehicle Collision. KSCE J. Civ. Eng. 2022, 26, 1188–1202. [Google Scholar] [CrossRef]
- Liu, Y.; Li, X.; Zheng, X.; Song, Z. Experimental study on seismic response of precast bridge piers with double-grouted sleeve connections. Eng. Struct. 2020, 221, 111023. [Google Scholar] [CrossRef]
- Fu, T.; Ren, X.; Wang, K.; Zhu, Z. Seismic performance of prefabricated steel tube-confined concrete circular pier. Structures 2022, 43, 910–925. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, M.; Lei, L.; He, Y. Numerical simulation on dynamic response of prefabricated concrete pier under vehicle collision. Case Stud. Constr. Mater. 2022, 17, e01553. [Google Scholar] [CrossRef]
- Fu, T.; Zhu, Z.; Ren, X.; Wang, K.; Meng, L.; Sun, Z. Research on the seismic behaviour of hollow prefabricated square column with high-strength reinforcement using UHPC grouting materials. Case Stud. Constr. Mater. 2023, 18, e01816. [Google Scholar] [CrossRef]
- Motaref, S.; Saiidi, M.S.; Sanders, D. Shake Table Studies of Energy-Dissipating Segmental Bridge Columns. J. Bridg. Eng. 2014, 19, 186–199. [Google Scholar] [CrossRef]
- Sun, Z.; Wang, D.; Wang, T.; Wu, S.; Guo, X. Investigation on seismic behavior of bridge piers with thin-walled rectangular hollow section using quasi-static cyclic tests. Eng. Struct. 2019, 200, 109708. [Google Scholar] [CrossRef]
- Shi, K.; Zhang, M.; Zhang, T.; Xue, R.; Li, P. Analysis on the Seismic Performance of Steel Fiber-Reinforced High-Strength Concrete Beam–Column Joints. Materials 2021, 14, 4016. [Google Scholar] [CrossRef]
- Han, J.P.; Liu, W.L. Experimental investigation on seismic behavior of pva fiber reinforced concrete columns with high axial compression ratios. Eng. Mech. 2017, 34, 193–201. [Google Scholar]
- Su, J.; Hu, Q.; Liu, J. Nonlinear finite element analysis of PVA fiber reinforced high strength concrete columns under low cyclic loading. IOP Conf. Ser. Earth Environ. Sci. 2017, 61, 012096. [Google Scholar] [CrossRef]
- Cassese, P.; De Risi, M.T.; Verderame, G.M. A degrading shear strength model for R.C. columns with hollow circular cross-section. Int. J. Civ. Eng. 2018, 17, 1241–1259. [Google Scholar] [CrossRef]
- AlAjarmeh, O.; Manalo, A.; Benmokrane, B.; Karunasena, K.; Ferdous, W.; Mendis, P. Hollow concrete columns: Review of structural behavior and new designs using GFRP reinforcement. Eng. Struct. 2020, 203, 109829. [Google Scholar] [CrossRef]
- Yu, J.; Li, J.; Guo, W.; Fan, C.; Zeng, C.; Chen, X. Seismic evaluation method on shear strength models for round-end hollow piers of high-speed railway bridges. Structures 2023, 50, 23–38. [Google Scholar] [CrossRef]
- Yang, D.; Wang, Z.; Zhang, Y.; Pan, W.; Wang, J.; Shi, J. Seismic Performance of the PVA Fiber Reinforced Concrete Prefabricated Hollow Circular Piers with Socket and Slot Connection. Buildings 2022, 12, 1339. [Google Scholar] [CrossRef]
- Cai, Z.; Zhuo, W.; Wang, Z.; Briseghella, B. Compressive performance of an innovative tall pier with composite box section. J. Constr. Steel Res. 2023, 202, 107779. [Google Scholar] [CrossRef]
- Nozawa, T.; Choi, J.H.; Hattori, M.; Otsuka, H. Cyclic loading test for columns made with ultra-strength fiber-reinforced concrete and trace analyses using finite element analyses. Struct. Concr. J. FIB 2017, 18, 433–443. [Google Scholar] [CrossRef]
- Park, R. Evaluation of ductility of structures and structural assemblages from laboratory testing = Evaluation de la ductilité de structures et de liaisons à partir d’essais en laboratoire. Bull. N. Z. Natl. Soc. Earthq. Eng. 1989, 22, 155–166. [Google Scholar]
- Cai, Z.; Wang, D.; Smith, S.T.; Wang, Z. Experimental investigation on the seismic performance of GFRP-wrapped thin-walled steel tube confined RC columns—ScienceDirect. Eng. Struct. 2016, 110, 269–280. [Google Scholar] [CrossRef]
- Cassese, P.; Ricci, P.; Verderame, G.M. Experimental study on the seismic performance of existing reinforced concrete bridge piers with hollow rectangular section—ScienceDirect. Eng. Struct. 2017, 144, 88–106. [Google Scholar] [CrossRef]
- Clough, R.W.; Penzien, J. Dynamics of Structures, 2nd ed.; Computers & Structures, Inc.: Berkeley, CA, USA, 2003. [Google Scholar]
- Aschheim, M.; Moehle, J.P. Shear Strength and Deformability of RC Bridges Columns Subjected to Inelastic Cyclic Displacements; University of California Earthquake Engineering Research Central: Berkeley, CA, USA, 1992. [Google Scholar]
- Priestley, M.J.N.; Verma, R.; Xiao, Y. Seismic Shear Strength of Reinforced Concrete Columns. J. Struct. Eng. 1994, 120, 2310–2329. [Google Scholar] [CrossRef]
- Sezen, H.; Moehle, J.P. Shear Strength Model for Lightly Reinforced Concrete Columns. J. Struct. Eng. 2004, 130, 1692–1703. [Google Scholar] [CrossRef]
- Ministry of Transport of the People’s Republic of China. Guidelines for Seismic Design of Highway Bridges; Ministry of Transport of the People’s Republic of China: Beijing, China, 2008. [Google Scholar]
- ACI Committee. Building code requirements for reinforced concrete (ACI 318-77). ACI Struct. J. 2014, 59, 145–276. [Google Scholar]
- Bing, L.; Cao, T. Reinforced concrete beam analysis supplementing concrete contribution in truss models. Eng. Struct. 2008, 30, 3285–3294. [Google Scholar]

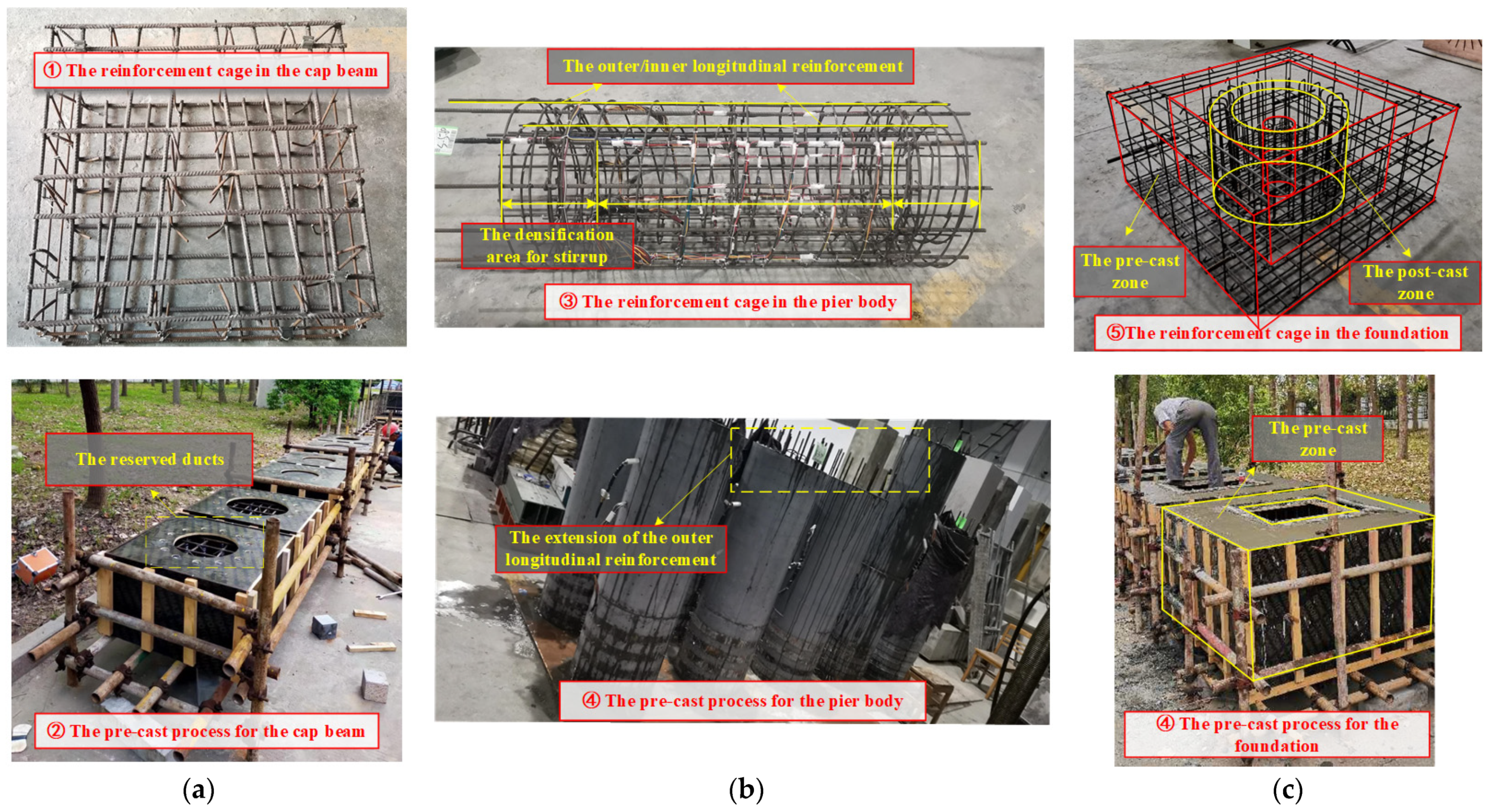

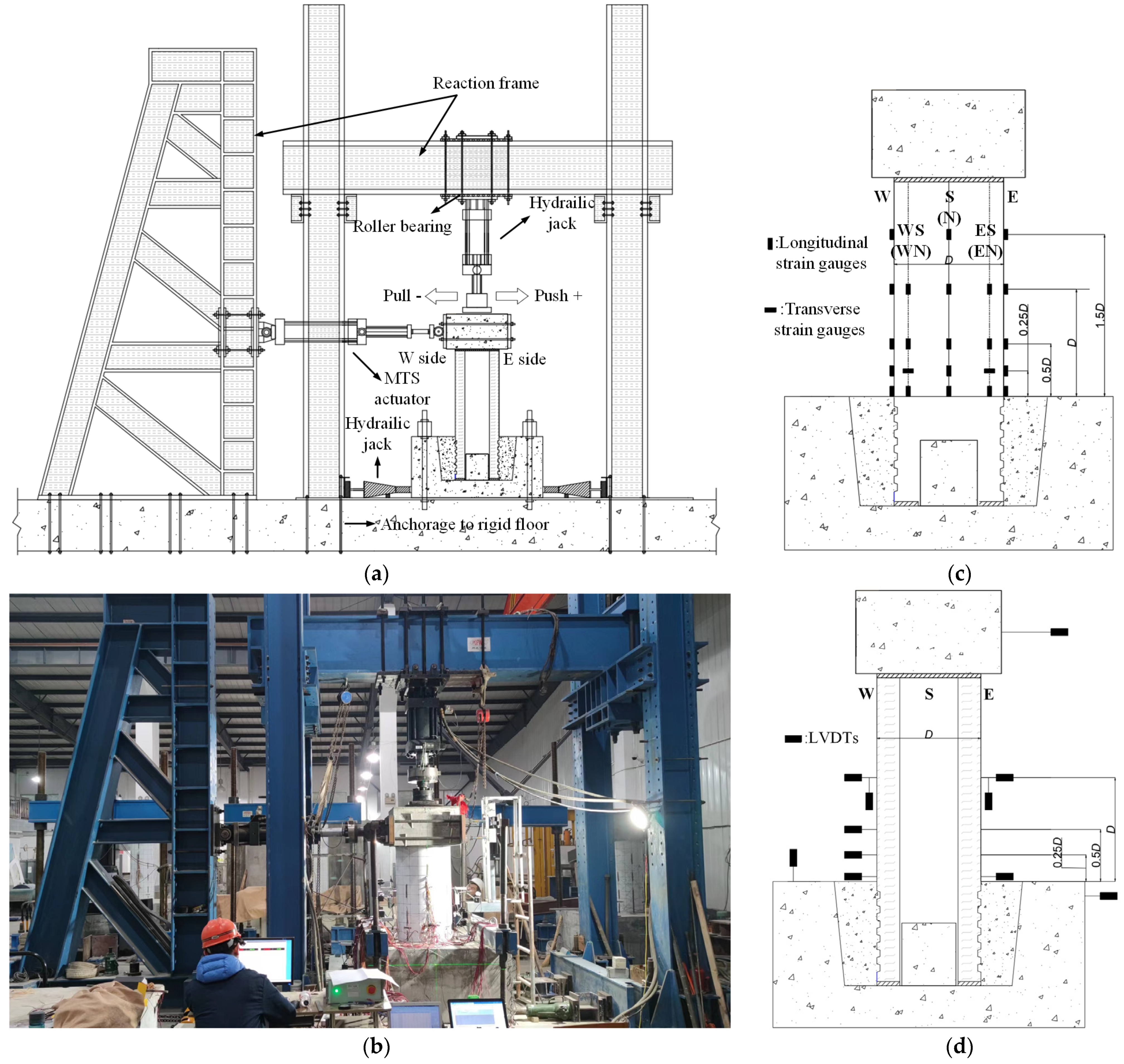

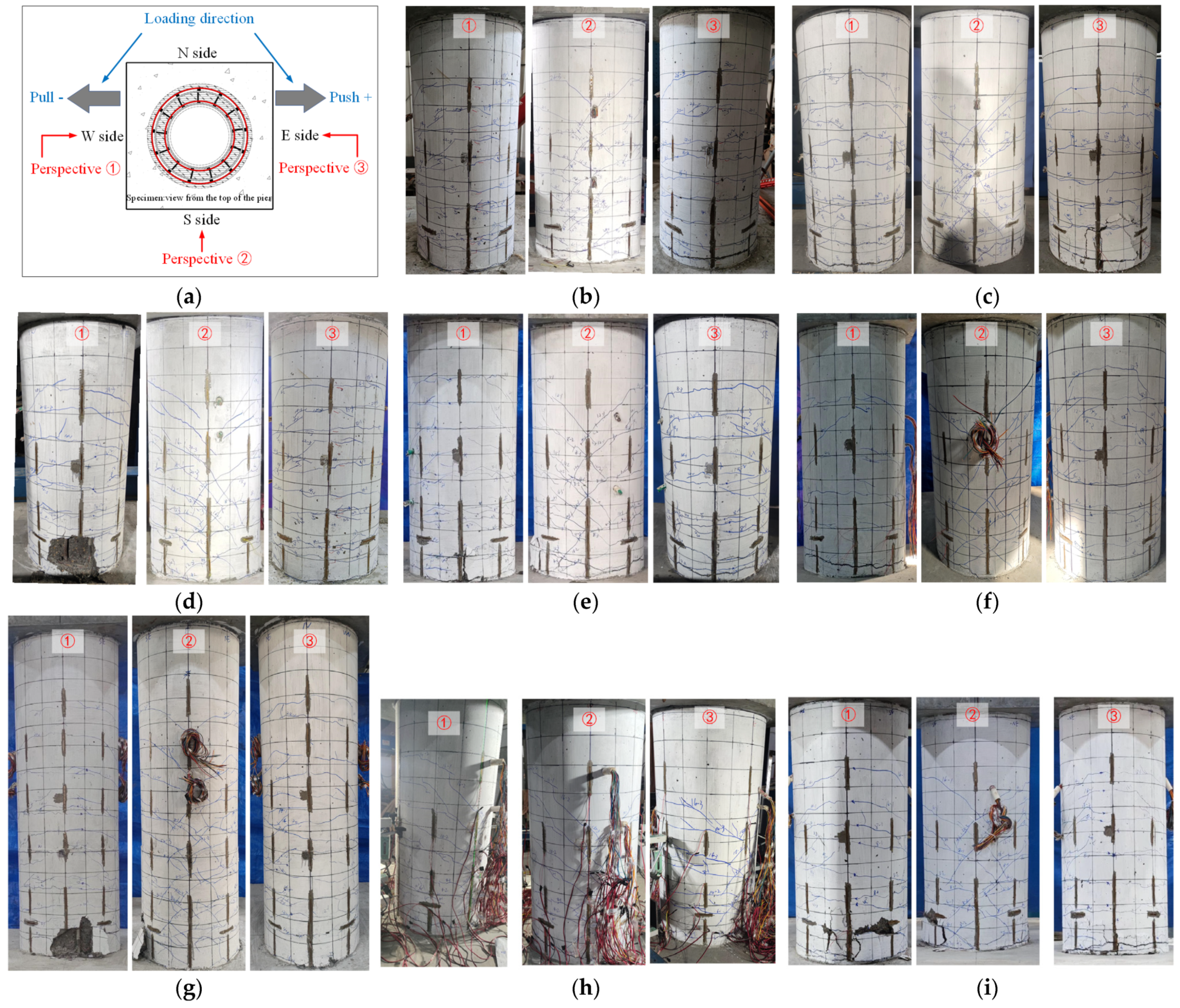
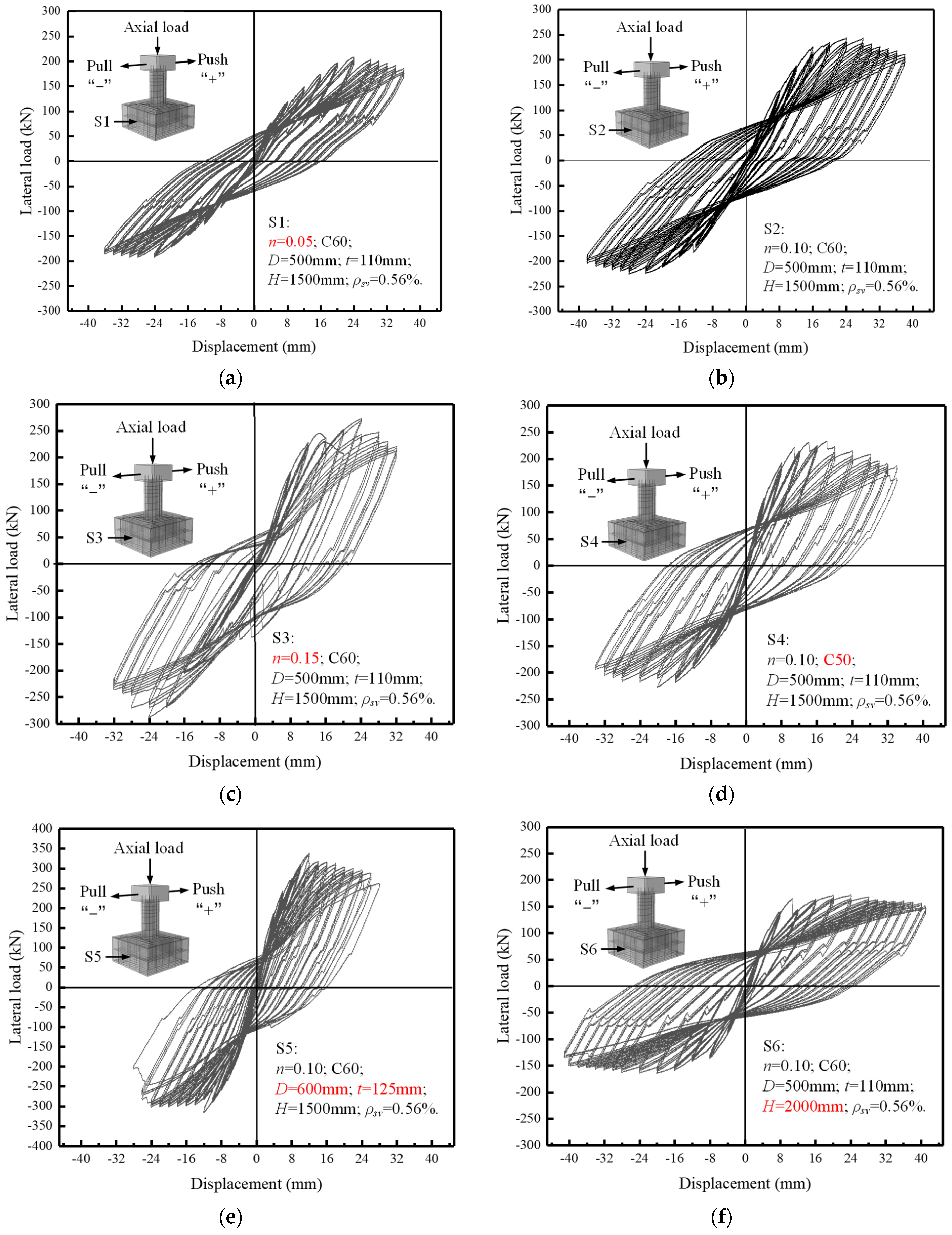
| Specimens | D (mm) | H (mm) | t (mm) | n | Pva Concrete | Longitudinal Rebar | Stirrup Rebar | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Grade | Outer/Inner Layout | ρt (%) | ρsv (%) | ds (mm) | R/R′ (mm) | L/L′ (mm) | s/s′ (mm) | |||||
| S1 | 500 | 1500 | 110 | 0.05 | C60 | 13φ12/6 | 1.36 | 0.56 | 6.0 | 220/160 | 360/720 | 90/180 |
| S2 | 500 | 1500 | 110 | 0.10 | C60 | 13φ12/6 | 1.36 | 0.56 | 6.0 | 220/160 | 360/720 | 90/180 |
| S3 | 500 | 1500 | 110 | 0.15 | C60 | 13φ12/6 | 1.36 | 0.56 | 6.0 | 220/160 | 360/720 | 90/180 |
| S4 | 500 | 1500 | 110 | 0.10 | C50 | 13φ12/6 | 1.36 | 0.56 | 6.0 | 220/160 | 360/720 | 90/180 |
| S5 | 600 | 1500 | 125 | 0.10 | C60 | 13φ13.5/8 | 1.35 | 0.56 | 6.0 | 270/195 | 350/700 | 70/140 |
| S6 | 500 | 2000 | 110 | 0.10 | C60 | 13φ12/6 | 1.36 | 0.56 | 6.0 | 220/160 | 360/1170 | 90/180 |
| S7 | 500 | 1500 | 110 | 0.10 | C60 | 13φ12/6 | 1.36 | 0.34 | 5.0 | 220/160 | 400/600 | 100/200 |
| S8 | 500 | 1500 | 110 | 0.10 | C60 | 13φ12/6 | 1.36 | 0.91 | 6.5 | 220/160 | 320/780 | 80/130 |
| Grade | Cement (kg/m3) | Water (kg/m3) | Slag Powder (kg/m3) | Silicon Powder (kg/m3) | Water Reducer (kg/m3) | Quartz Sand (kg/m3) | PVA Fiber (%) |
|---|---|---|---|---|---|---|---|
| C50 | 406 | 150.0 | 100 | 18.4 | 7.5 | 632.4 | 0.5 |
| C60 | 410 | 151.2 | 102 | 20.5 | 7.5 | 630.8 | 0.5 |
| Location of Materials | Pier Body | Foundation/Bent Cap (The Post-Cast Part) | Contact Surface | Other Pre-Cast Parts | |
|---|---|---|---|---|---|
| Material type | C50 (PVA) | C60 (PVA) | C50 (grouted material) | epoxy mortar | C60 (NC) |
| Compressive strength (MPa) | 52.6 | 63.8 | 50.8 | 54.4 (28 h) 55.7 (28 d) | 61.2 |
| Specimens | Py (kN) | Δy (mm) | Pp (kN) | Δy (mm) | Pu (kN) | Δu (mm) | |
|---|---|---|---|---|---|---|---|
| S1 | 190.00 | 17.4 | 207.66 | 24.6 | 172.13 | 39.6 | 2.28 |
| S2 | 226.01 | 15.5 | 243.75 | 24.1 | 207.48 | 34.0 | 2.21 |
| S3 | 235.27 | 13.7 | 272.40 | 23.2 | 220.42 | 29.8 | 2.17 |
| S4 | 210.73 | 13.4 | 219.94 | 20.0 | 186.95 | 32.3 | 2.42 |
| S5 | 329.75 | 11.4 | 338.23 | 12.5 | 287.47 | 26.6 | 2.33 |
| S6 | 143.64 | 13.7 | 169.17 | 24.5 | 143.79 | 41.0 | 3.01 |
| S7 | 192.02 | 11.5 | 209.62 | 14.5 | 178.18 | 24.2 | 2.10 |
| S8 | 237.38 | 13.2 | 275.80 | 24.0 | 233.75 | 30.4 | 2.31 |
| Formula Number | Source of Formula | V = Vc + (Vp) + Vs | k |
|---|---|---|---|
| M1 | Aschheim and Moehle | ||
| M2 | Priestley et al. | ||
| M3 | Sezen | ||
| M4 | CHN-08 | 0.023 | |
| M5 | ACI 318-14 | 0.167 |
| Specimens | n | fc (N/mm2) | Ae (mm2) | Asv1and2 (mm2) | s1and2 (mm) | (mm) | (mm) | N (kN) | Vt (kN) | ||
|---|---|---|---|---|---|---|---|---|---|---|---|
| S1 | 2.4 | 0.05 | 2.28 | 27.5 | 107,819 | 28.27 | 180 | 464 | 320 | 185.31 | 172.13 |
| S2 | 2.4 | 0.10 | 2.21 | 27.5 | 107,819 | 28.27 | 180 | 464 | 320 | 370.63 | 207.48 |
| S3 | 2.4 | 0.15 | 2.17 | 27.5 | 107,819 | 28.27 | 180 | 464 | 320 | 555.94 | 220.42 |
| S4 | 2.4 | 0.10 | 2.42 | 23.1 | 107,819 | 28.27 | 180 | 464 | 320 | 370.62 | 186.95 |
| S5 | 2.0 | 0.10 | 2.33 | 27.5 | 149,226 | 28.27 | 140 | 564 | 390 | 512.96 | 287.47 |
| S6 | 3.4 | 0.10 | 3.01 | 27.5 | 107,819 | 28.27 | 180 | 464 | 320 | 370.62 | 143.79 |
| S7 | 2.4 | 0.10 | 2.10 | 27.5 | 107,819 | 19.64 | 220 | 464 | 320 | 370.62 | 178.18 |
| S8 | 2.4 | 0.10 | 2.31 | 27.5 | 107,819 | 33.18 | 130 | 464 | 320 | 370.62 | 233.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, J.; Deng, Y.; Zhang, Y.; Shi, F.; Yang, J. Experimental Studies on the Seismic Performance of Prefabricated Circular Hollow Bridge Piers Constructed with PVA Fiber Concrete. Materials 2023, 16, 1981. https://doi.org/10.3390/ma16051981
Shi J, Deng Y, Zhang Y, Shi F, Yang J. Experimental Studies on the Seismic Performance of Prefabricated Circular Hollow Bridge Piers Constructed with PVA Fiber Concrete. Materials. 2023; 16(5):1981. https://doi.org/10.3390/ma16051981
Chicago/Turabian StyleShi, Jun, Yuang Deng, Yi Zhang, Feiting Shi, and Jian Yang. 2023. "Experimental Studies on the Seismic Performance of Prefabricated Circular Hollow Bridge Piers Constructed with PVA Fiber Concrete" Materials 16, no. 5: 1981. https://doi.org/10.3390/ma16051981
APA StyleShi, J., Deng, Y., Zhang, Y., Shi, F., & Yang, J. (2023). Experimental Studies on the Seismic Performance of Prefabricated Circular Hollow Bridge Piers Constructed with PVA Fiber Concrete. Materials, 16(5), 1981. https://doi.org/10.3390/ma16051981





