Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging
Abstract
1. Introduction
2. Materials and Methods
2.1. Cu/Al Composite
2.2. Plastometric Tests
2.3. Neutron Diffraction Experiments
2.4. Strain and Stress Analysis
2.5. FEM Analysis
3. Results
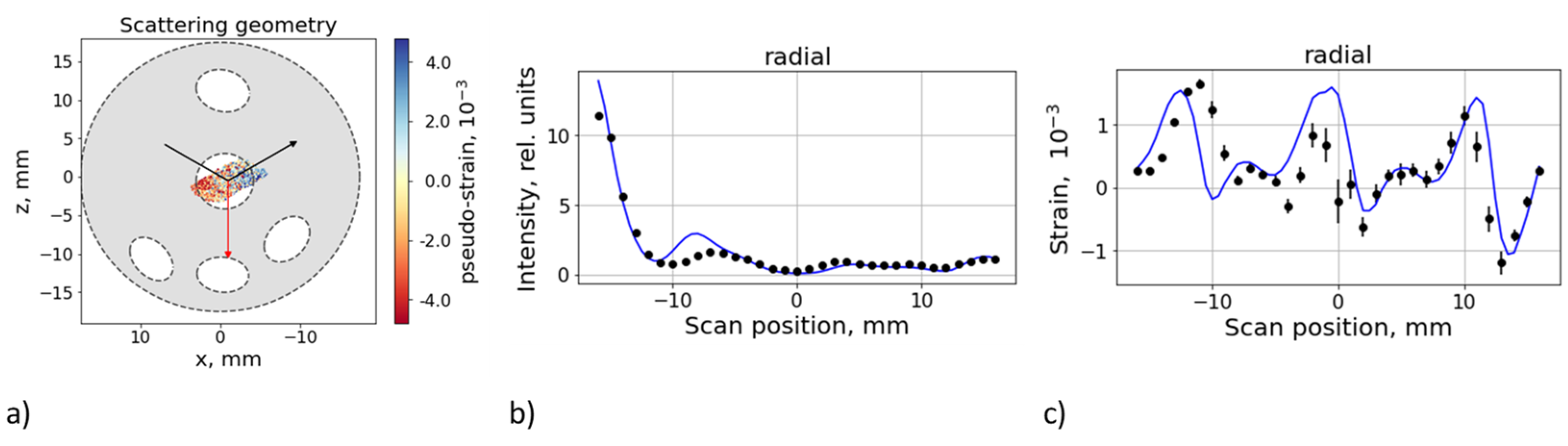
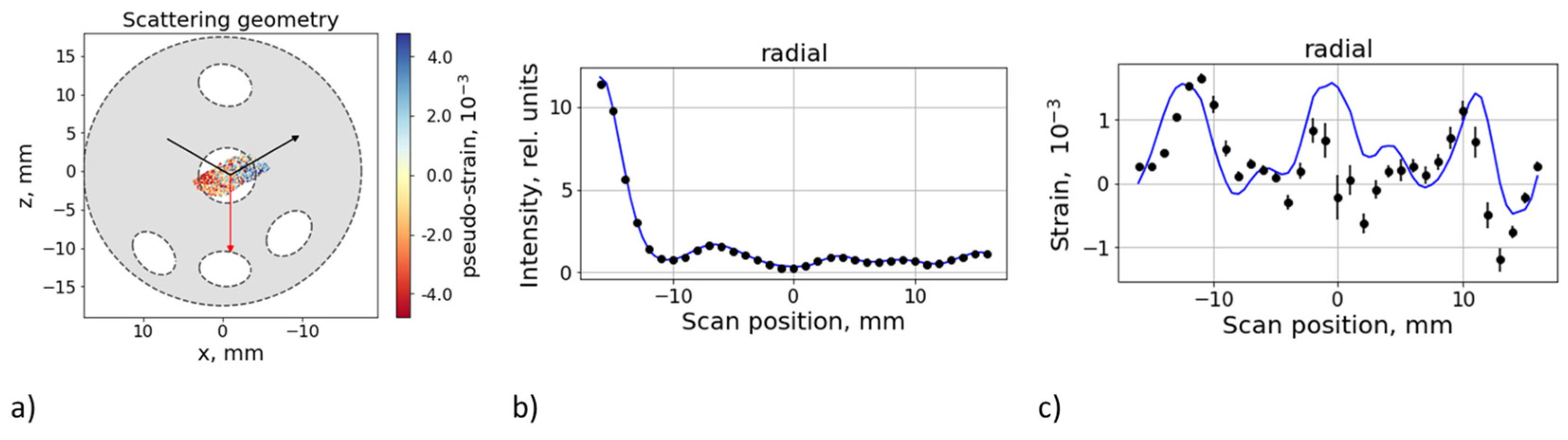
3.1. Sample Model, Measurement Geometry, and Simulation of Pseudo-Strain
- Irregularity of Al wires geometry.
- Variation of the scattering intensity due to texture gradients.
3.2. Fitting of Intrinsic Intensities
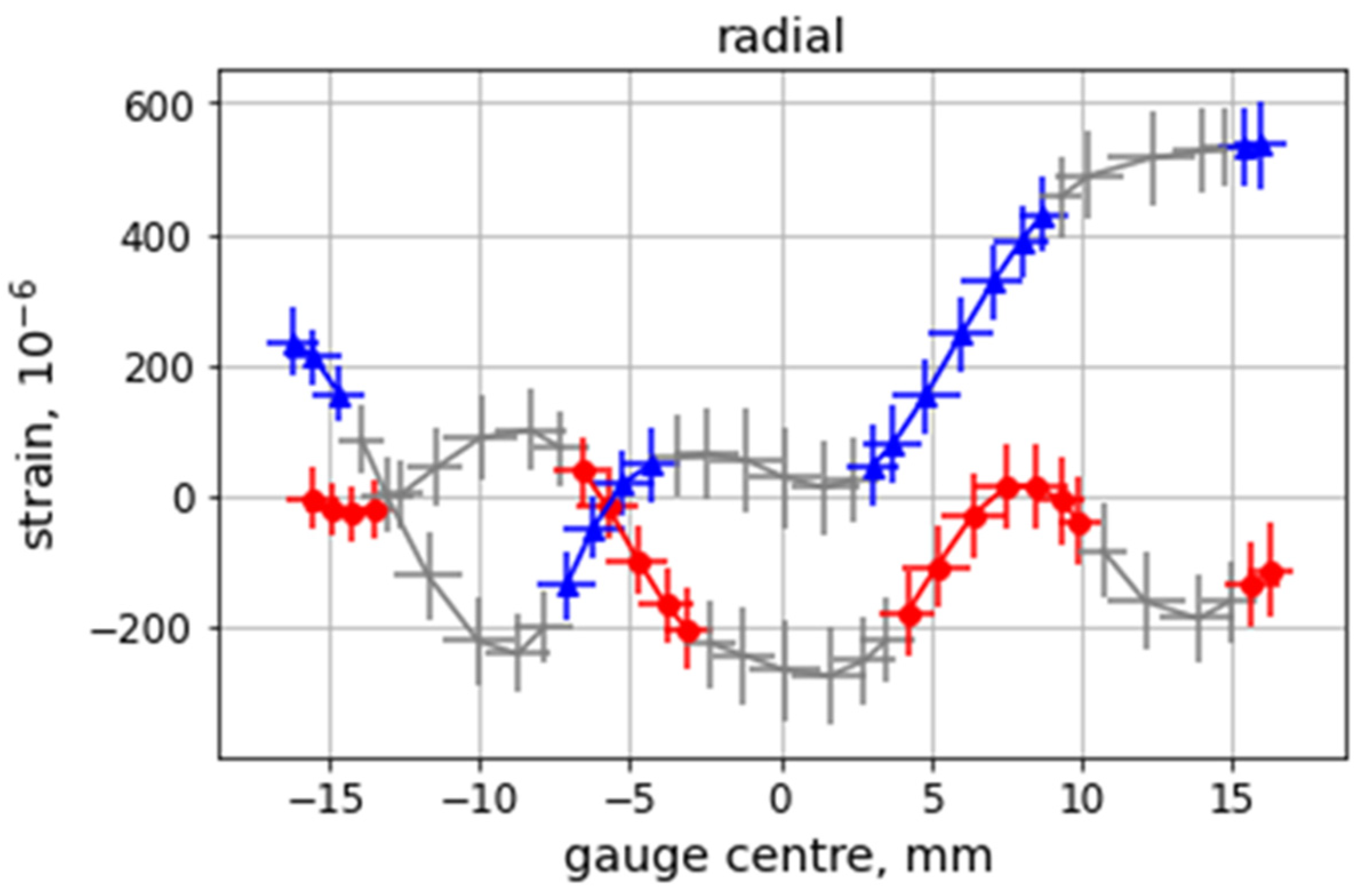
3.3. Evaluation of the Lattice Strain Distribution
3.4. d0 Problem and Stress Differences
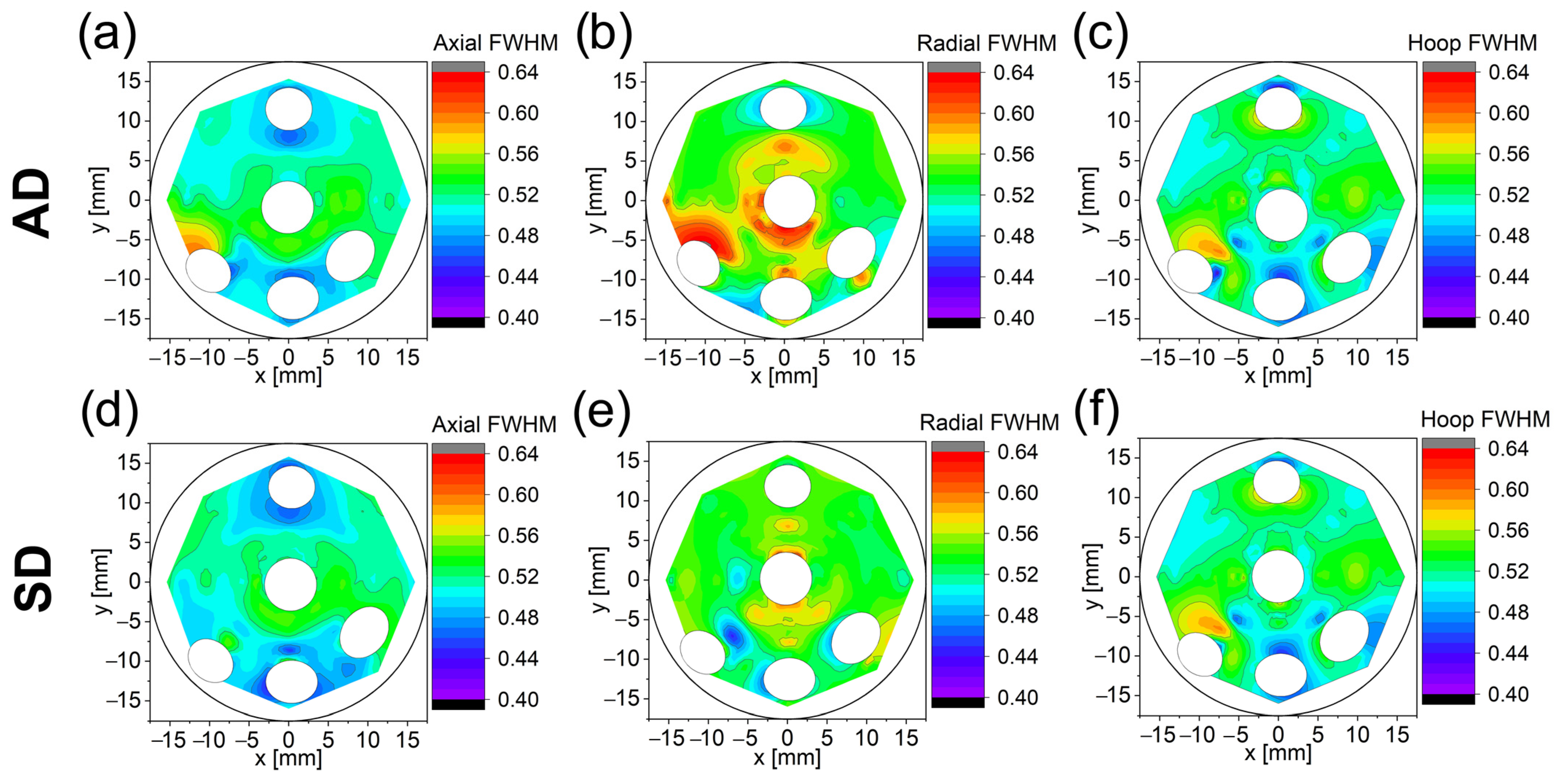
3.5. Full-Width at Half Maximum
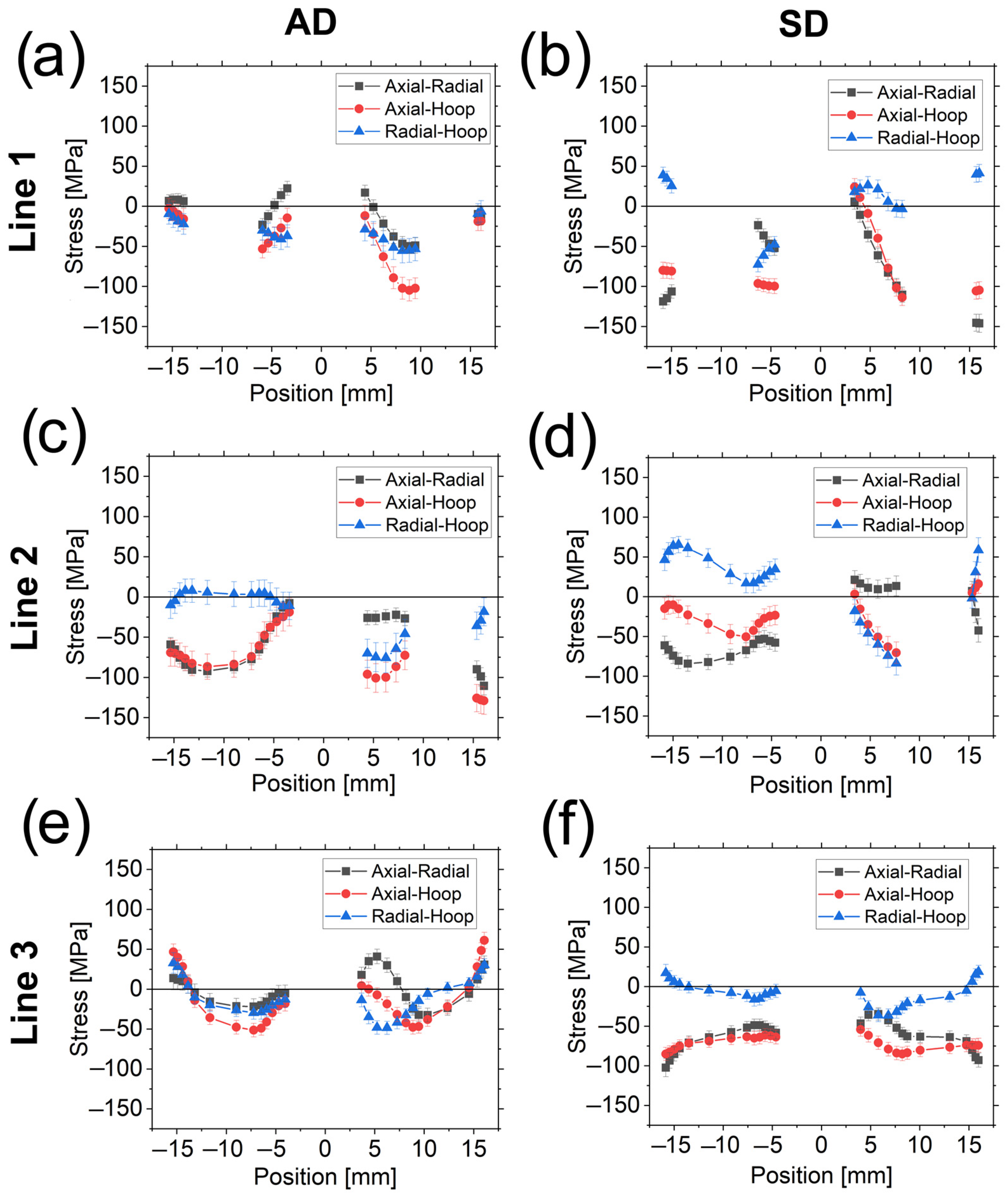
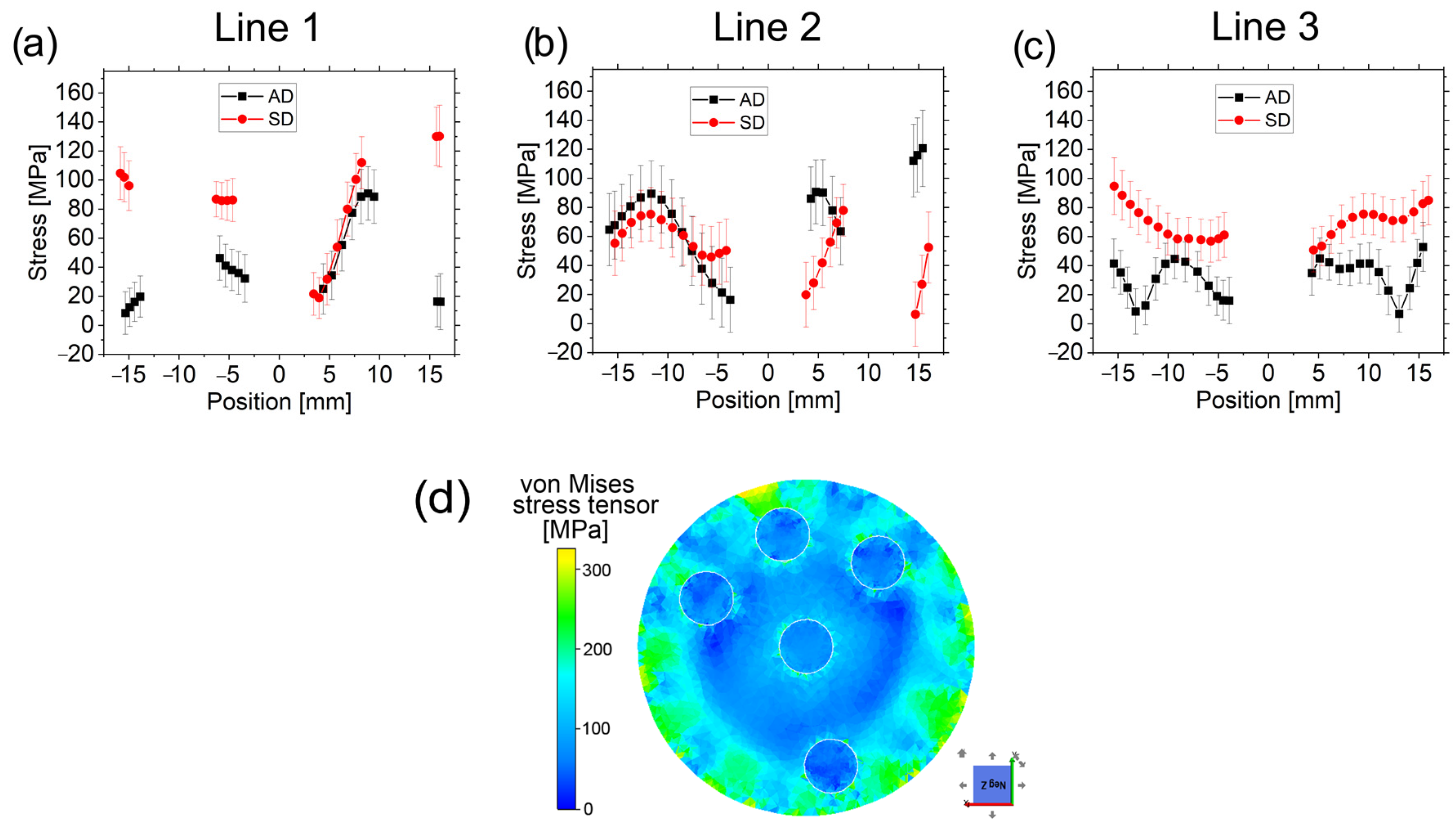
3.6. FEM Results
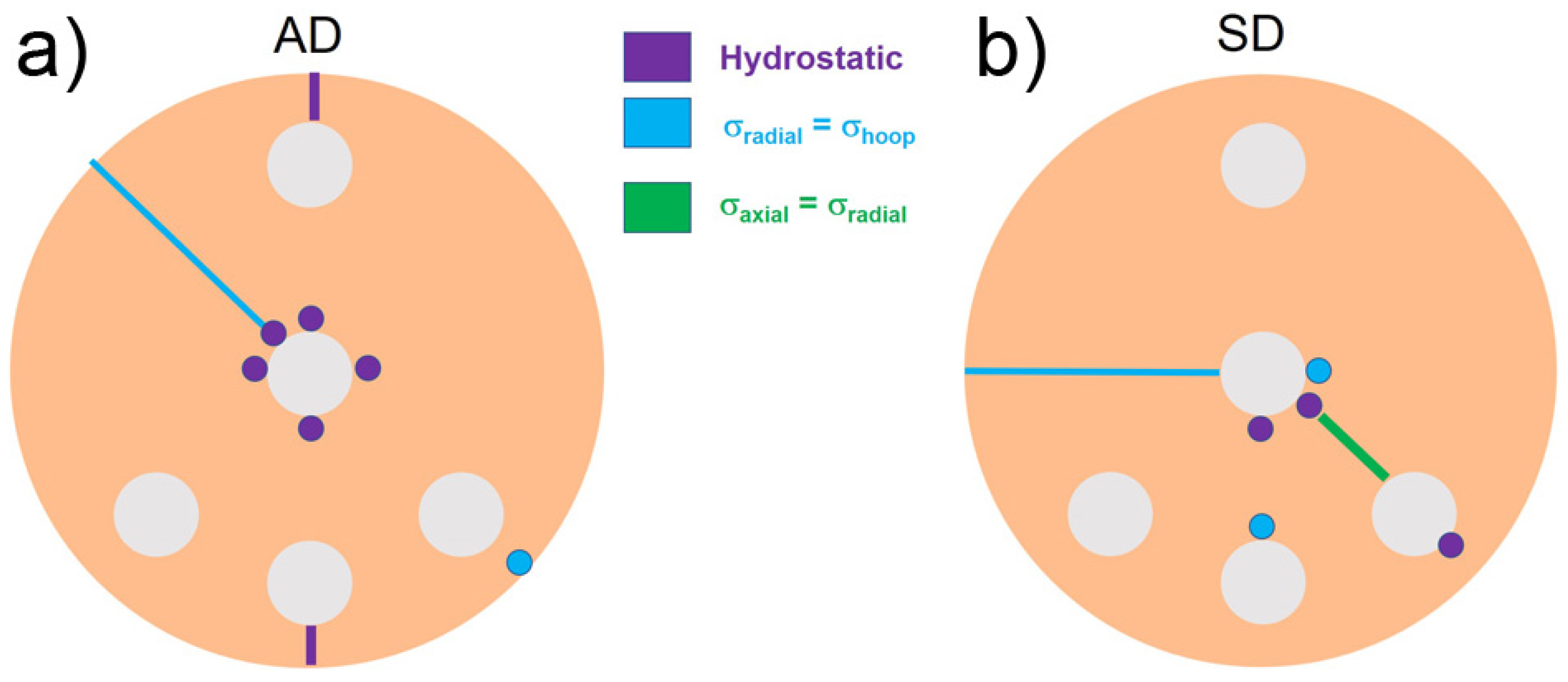
4. Discussion
- The reversal of the bar direction contributes to lowering the deviatoric stress components in Line 1 (except in L1.3 near the outer Al wire). It seems to be advantageous in order to delay material plastification and failure;
- Within the region with a high density of Al filaments, the reversal between the passes does not change significantly the overall state for both samples;
- The line delimiting one side of the high-density Al wire region (L2.3) for the AD sample is prone to failure as well as the L1.4 for the SD;
- The filament-free region exhibits a roughly symmetric behavior but with lower VM stresses for the AD sample.
5. Conclusions
- Hydrostatic stresses for the AD sample tend to be tensile in regions within the Al wires or surrounding them. Only far from the Al wires do hydrostatic stresses become compressive. The SD specimen exhibits more regions with compressive hydrostatic stresses;
- Axial deviatoric stresses are zero or compressive in most of the regions for both samples;
- The reversal of the bar direction provokes a lowering of the deviatoric stresses in the regions far from the Al wires and around the low Al-wire density regions where the stresses tend to be hydrostatic;
- The reversal of the bar direction slightly changes the overall state within the region of the high density of Al wires. The stresses calculated with the von Mises relation are at a maximum when close to the outer Al wire for both samples. In regions without Al wires, the reversal of the bar seems to be advantageous to avoid a possible plastification;
- Von Mises stresses calculated with the FEM simulations are higher than those measured with neutron diffraction; among other reasons, the presence of shear stresses may be possible;
- It was found that the reversal of the bar direction seems to be advantageous for the component properties. The reversal lowers the deviatoric stress components in a significantly larger part of the volume examined, although not in all the scanned segments;
- FEM shows that there can be a large variability of residual stress along the circumference. The residual stresses calculated using the von Mises relation from the neutron diffraction data can be approaching the yield stress of the Cu matrix near the outer surface of the component. It occurs regardless of the applied deformation mode (reversal, no reversal), although this effect seems to be more pronounced for the no-reversal component. Further optimization should aim at setting the processing parameters to still lower the stresses near the surface.
- The full-width-at-half-maximum of diffraction peaks is largest in the radial direction near the central Al wire in the region with a higher density of filaments and may initially be attributed to a predominance of microstresses in the radial direction;
- A novel evaluation procedure was successfully used for pseudo-strain treatment.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bae, J.; Rao, A.P.; Kim, K.; Kim, N.J. Cladding of Mg alloy with Al by twin-roll casting. Scr. Mater. 2011, 64, 836–839. [Google Scholar] [CrossRef]
- Uscinowicz, R. Impact of temperature on shear strength of single lap Al–Cu bimetallic joint. Compos. Part B Eng. 2013, 44, 344–356. [Google Scholar] [CrossRef]
- Kappel, E. Distortions of composite aerospace frames due to processing, thermal loads and trimming operations and an as-sessment from an assembly perspective. Compos. Struct. 2019, 220, 338–346. [Google Scholar] [CrossRef]
- Elanchezhian, C.; Ramnath, B.V.; Ramakrishnan, G.; Raghavendra, K.S.; Muralidharan, M.; Kishore, V. Review on metal matrix composites for marine applications. Mater. Today Proc. 2018, 5, 1211–1218. [Google Scholar] [CrossRef]
- Tabin, J.; Skoczeń, B.; Bielski, J. Discontinuous plastic flow in superconducting multifilament composites. Int. J. Solids Struct. 2020, 202, 12–27. [Google Scholar] [CrossRef]
- Ambli, K.G.; Dodamani, B.M.; Jagadeesh, A.; Vanarotti, M.B. Heterogeneous composites for low and medium temperature thermal insulation: A review. Energy Build. 2019, 199, 455–460. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Lowe, T.C. Advances in metals and alloys for joint replacement. Prog. Mater. Sci. 2017, 88, 232–280. [Google Scholar] [CrossRef]
- Gibson, A. The economics of copper clad aluminum bimetallic cables. Wire Cable Technol. Int. 2005, 33, 82–83. [Google Scholar]
- Chu, D.; Zhang, J.Y.; Yao, J.J.; Han, Y.Q.; Wu, C.J. Cu–Al interfacial compounds and formation mechanism of copper cladding aluminum composites. Trans. Nonferrous Met. Soc. China 2017, 27, 2521–2528. [Google Scholar] [CrossRef]
- Kunčická, L.; Macháčková, A.; Krátká, L.; Kocich, R. Analysis of Deformation Behaviour and Residual Stress in Rotary Swaged Cu/Al Clad Composite Wires. Materials 2019, 12, 3462. [Google Scholar] [CrossRef]
- Kim, W.N.; Hong, S.I. Interactive deformation and enhanced ductility of tri-layered Cu/Al/Cu clad composite. Mater. Sci. Eng. A 2016, 651, 976–986. [Google Scholar] [CrossRef]
- Jin, K.; Yuan, Q.; Tao, J.; Domblesky, J.; Guo, X. Analysis of the forming characteristics for Cu/Al bimetal tubes produced by the spinning process. Int. J. Adv. Manuf. Technol. 2018, 101, 147–155. [Google Scholar] [CrossRef]
- Lesuer, D.R.; Syn, C.K.; Sherby, O.D.; Wadsworth, J.; Lewandowski, J.J.; Hunt, W.H. Mechanical behaviour of laminated metal composites. Int. Mater. Rev. 1996, 41, 169–197. [Google Scholar] [CrossRef]
- Kocich, R.; Szurman, I.; Kursa, M.; Fiala, J. Investigation of influence of preparation and heat treatment on deformation be-haviour of the alloy NiTi after ECAE. Mater. Sci. Eng. A 2009, 512, 100–104. [Google Scholar] [CrossRef]
- Atrian, A.; Fereshteh-Saniee, F. Deep drawing process of steel/brass laminated sheets. Compos. Part B Eng. 2013, 47, 75–81. [Google Scholar] [CrossRef]
- Lee, J.; Son, H.; Oh, I.; Kang, C.; Yun, C.; Lim, S.; Kwon, H. Fabrication and characterization of Ti–Cu clad materials by indirect extrusion. J. Mater. Process. Technol. 2007, 187–188, 653–656. [Google Scholar] [CrossRef]
- Movahedi, M.; Kokabi, A.H.; Seyed Reihani, S.M. Investigation on the bond strength of Al-1100/St-12 roll bonded sheets, optimization and characterization. Mater. Des. 2011, 32, 3143–3149. [Google Scholar] [CrossRef]
- Masahashi, N.; Komatsu, K.; Watanabe, S.; Hanada, S. Microstructure and properties of iron aluminum alloy/CrMo steel composite prepared by clad rolling. J. Alloy. Compd. 2004, 379, 272–279. [Google Scholar] [CrossRef]
- Hosseini, M.; Manesh, H.D. Bond strength optimization of Ti/Cu/Ti clad composites produced by roll-bonding. Mater. Des. 2015, 81, 122–132. [Google Scholar] [CrossRef]
- Kocich, R.; Macháčková, A.; Kunčická, L.; Fojtík, F. Fabrication and characterization of cold-swaged multilayered Al–Cu clad composites. Mater. Des. 2015, 71, 36–47. [Google Scholar] [CrossRef]
- Kim, I.-K.; Hong, S.I. Effect of heat treatment on the bending behavior of tri-layered Cu/Al/Cu composite plates. Mater. Des. 2013, 47, 590–598. [Google Scholar] [CrossRef]
- Ha, J.S.; Hong, S.I. Deformation and fracture of Ti/439 stainless steel clad composite at intermediate temperatures. Mater. Sci. Eng. A 2016, 651, 805–809. [Google Scholar] [CrossRef]
- Canelo-Yubero, D.; Kocich, R.; Hervoches, C.; Strunz, P.; Kunčická, L.; Krátká, L. Neutron Diffraction Study of Residual Stresses in a W–Ni–Co Heavy Alloy Processed by Rotary Swaging at Room and High Temperatures. Met. Mater. Int. 2021, 28, 919–930. [Google Scholar] [CrossRef]
- Hutchings, M.; Withers, P.J.; Holden, T.; Lorentzen, T. Introduction to the Characterization of Residual Stress by Neutron Diffraction; Informa UK Limited: Colchester, UK, 2005. [Google Scholar]
- Šaroun, J.; Rebelo-Kornmeier, J.; Gibmeier, J.; Hofmann, M. Treatment of spatial resolution effects in neutron residual strain scanning. Phys. B Condens. Matter 2018, 551, 468–471. [Google Scholar] [CrossRef]
- Withers, P.J.; Bhadeshia, H.K.D.H. Residual stress, Part 1—Measurement techniques. Mater. Sci. Technol. 2001, 17, 355–365. [Google Scholar] [CrossRef]
- HK4—Strain Scanner. Available online: http://www.ujf.cas.cz/en/departments/department-of-neutron-physics/instruments/lvr15/HK4.html (accessed on 22 February 2023).
- Hodek, J.; Prantl, A.; Džugan, J.; Strunz, P. Determination of Directional Residual Stresses by the Contour Method. Metals 2019, 9, 1104. [Google Scholar] [CrossRef]
- Lodini, A. Calculation of residual stress from measured strain. In Analysis of Residual Stress by Diffraction Using Neutron and Synchrotron Radiation; Fitzpatrick, M.E., Lodini, A., Eds.; Taylor & Francis: London, UK, 2003; pp. 47–59. [Google Scholar]
- Kröner, E. Berechnung der elastischen Konstanten des Vielkristalls aus den Konstanten des Einkristalls. Z. Für Phys. 1958, 151, 504–518. [Google Scholar] [CrossRef]
- Saroun, J. SIMRES—Neutron Ray-Tracing Simulation Program. Available online: https://github.com/saroun/simres (accessed on 31 December 2022).
- Saroun, J. STRESSFIT—Program for Fitting of Residual Stress Distributions. Available online: https://github.com/NPLtools/stressfit (accessed on 31 December 2022).
- Strunz, P.; Kocich, R.; Canelo-Yubero, D.; Macháčková, A.; Beran, P.; Krátká, L. Texture and Differential Stress Development in W/Ni-Co Composite after Rotary Swaging. Materials 2020, 13, 2869. [Google Scholar] [CrossRef] [PubMed]
- Fernández, P.; Bruno, G.; González-Doncel, G. Macro and micro-residual stress distribution in 6061 Al-15 vol.% SiCw under different heat treatment conditions. Compos. Sci. Technol. 2006, 66, 1738–1748. [Google Scholar] [CrossRef]
- Martinez-Perez, M.; Mompean, F.; Ruiz-Hervias, J.; Borlado, C.; Atienza, J.; Garcia-Hernandez, M.; Elices, M.; Gil-Sevillano, J.; Peng, R.L.; Buslaps, T. Residual stress profiling in the ferrite and cementite phases of cold-drawn steel rods by synchrotron X-ray and neutron diffraction. Acta Mater. 2004, 52, 5303–5313. [Google Scholar] [CrossRef]
- Pintschovius, L.; Jung, V.; Macherauch, E.; Vöhringer, O. Residual stress measurements by means of neutron diffraction. Mater. Sci. Eng. 1983, 61, 43–50. [Google Scholar] [CrossRef]
- Salvati, E.; Korsunsky, A. An analysis of macro- and micro-scale residual stresses of Type I, II and III using FIB-DIC mi-cro-ring-core milling and crystal plasticity FE modelling. Int. J. Plast. 2017, 98, 123–138. [Google Scholar] [CrossRef]
- Everaerts, J.; Salvati, E.; Uzun, F.; Brandt, L.R.; Zhang, H.; Korsunsky, A.M. Separating macro- (Type I) and micro- (Type II+III) residual stresses by ring-core FIB-DIC milling and eigenstrain modelling of a plastically bent titanium alloy bar. Acta Mater. 2018, 156, 43–51. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L.; Král, P.; Strunz, P. Characterization of innovative rotary swaged Cu-Al clad composite wire con-ductors. Mater. Des. 2018, 160, 828–835. [Google Scholar] [CrossRef]
- Strunz, P.; Kunčická, L.; Beran, P.; Kocich, R.; Hervoches, C. Correlating Microstrain and Activated Slip Systems with Me-chanical Properties within Rotary Swaged WNiCo Pseudoalloy. Materials 2020, 13, 208. [Google Scholar] [CrossRef] [PubMed]
- Kocich, R.; Kuncická, L.; Dvorák, K.; Machácková, A. Rotary swaged laminated Cu-Al composites: Effect of structure on residual stress and mechanical and electric properties. Mater. Sci. Eng. A 2019, 742, 743–750. [Google Scholar]
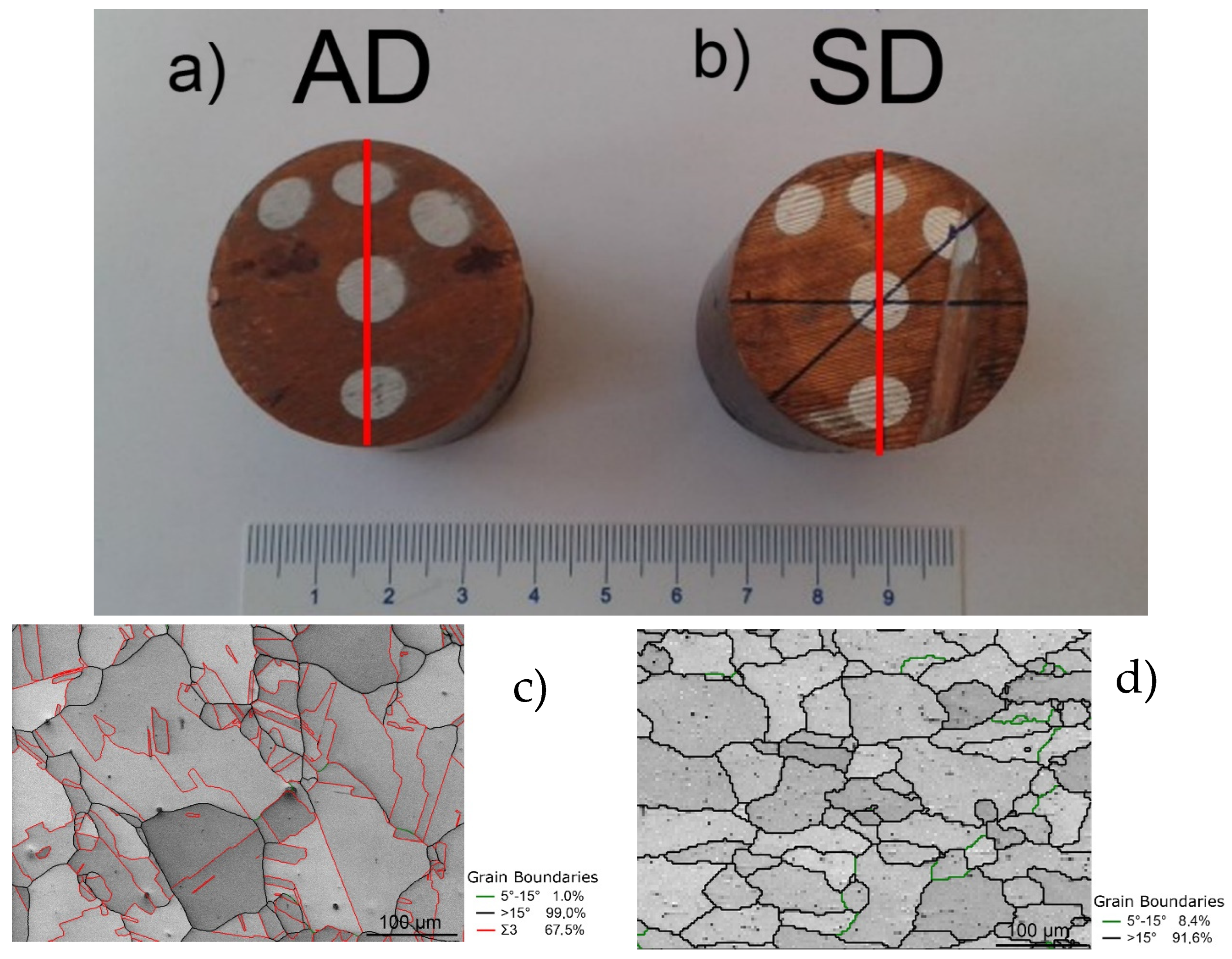
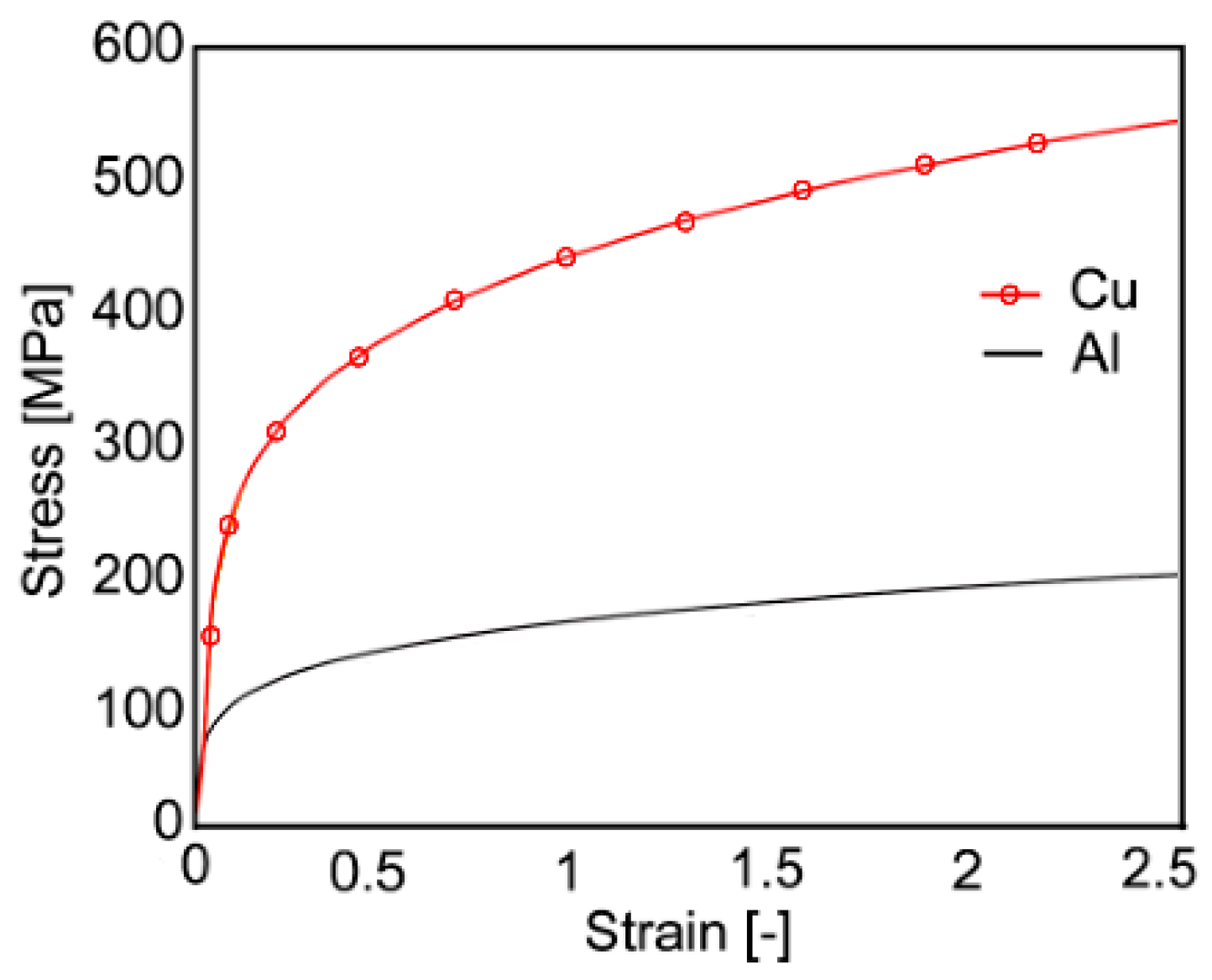

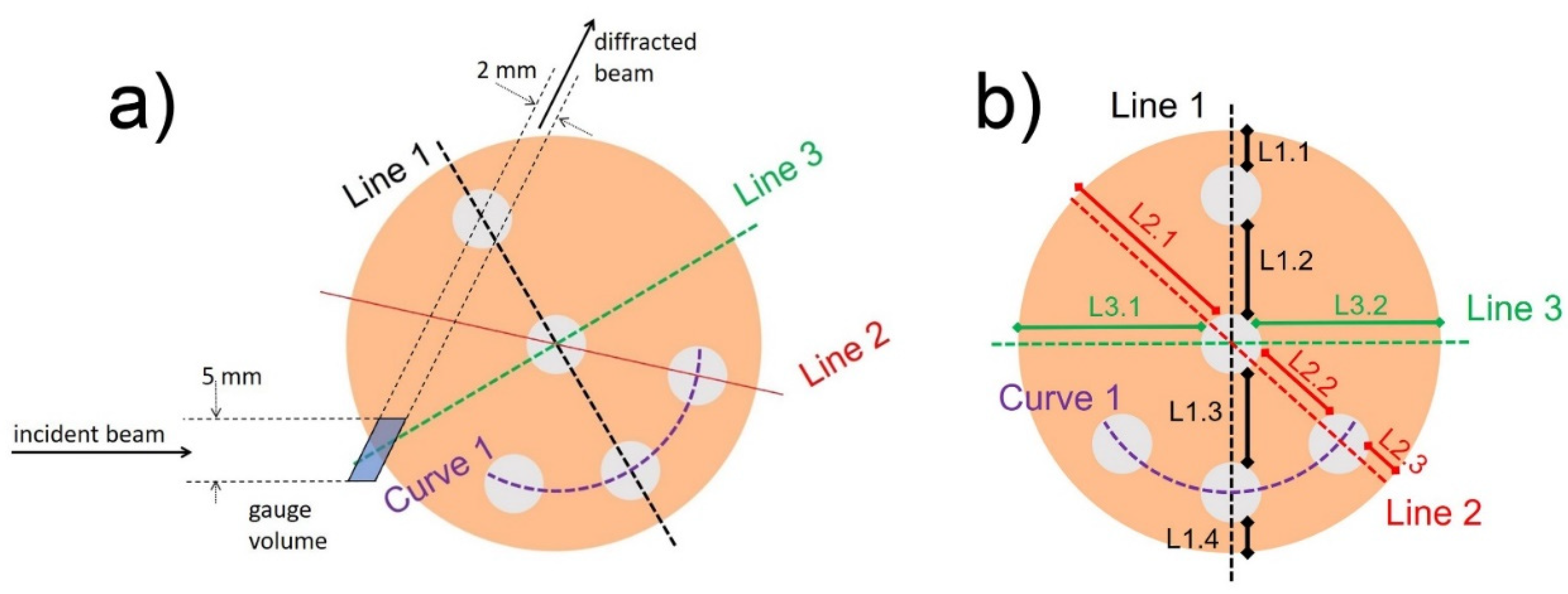
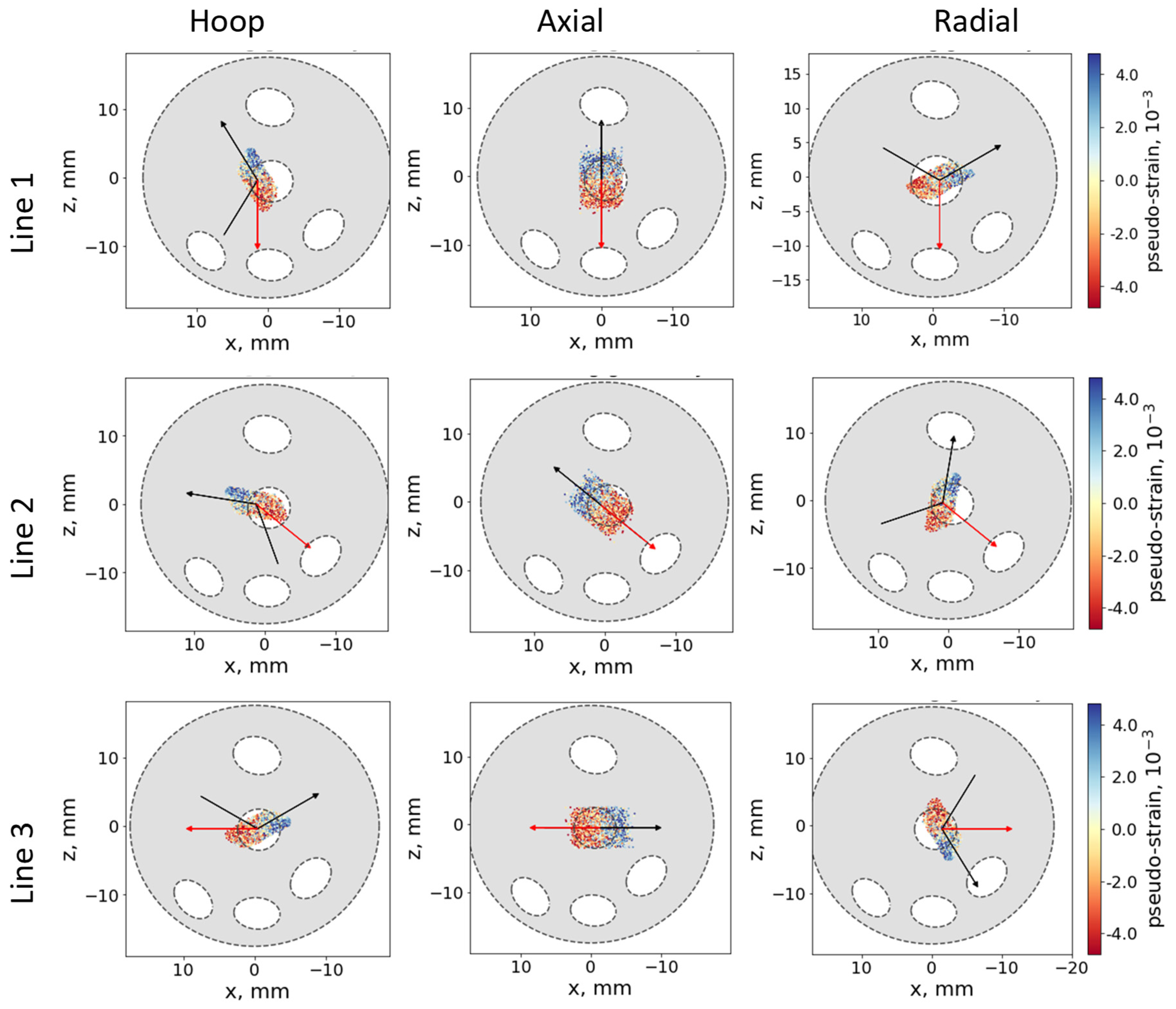


| Property | Unit | Cu | Al |
|---|---|---|---|
| Young modulus | (GPa) | 111 | 72 |
| Poisson ratio | - | 0.3 | 0.3 |
| Density | (g.cm−3) | 8.96 | 2.80 |
| Specific heat | (J.kg−1.K−1) | 398 | 1230 |
| Emissivity | - | 0.7 | 0.03 |
| Thermal expansion | (K−1) | 1.7 × 10−5 | 2.4 × 10−5 |
| Thermal conductivity | (W/(m.K)) | 394 | 250 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Canelo-Yubero, D.; Kocich, R.; Šaroun, J.; Strunz, P. Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging. Materials 2023, 16, 2102. https://doi.org/10.3390/ma16052102
Canelo-Yubero D, Kocich R, Šaroun J, Strunz P. Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging. Materials. 2023; 16(5):2102. https://doi.org/10.3390/ma16052102
Chicago/Turabian StyleCanelo-Yubero, David, Radim Kocich, Jan Šaroun, and Pavel Strunz. 2023. "Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging" Materials 16, no. 5: 2102. https://doi.org/10.3390/ma16052102
APA StyleCanelo-Yubero, D., Kocich, R., Šaroun, J., & Strunz, P. (2023). Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging. Materials, 16(5), 2102. https://doi.org/10.3390/ma16052102






