Review of Play and Preisach Models for Hysteresis in Magnetic Materials
Abstract
1. Introduction
2. Magnetic Hysteresis
Physics of Magnetic Hysteresis
- Nucleation of domains in multidomain particles meaning that there is a cost of energy of a new domain wall when each new domain is nucleated. This acts as a threshold for demagnetization into a low magnetization, since a demagnetized particle must consist of several domains with magnetic contributions that cancel out. This threshold is not present when magnetizing, since it is then an annihilation of the domain wall, and the domain wall energy becomes a power loss [19,20,21,22].
- Incoherent rotation of single-domain particles. This is the case when the particles are to small for containing several magnetic domains. There are then no domain walls and no domain wall pinning or no nucleation of the domain walls. The magnetization of the single domain can still experience hysteresis caused by the incoherent rotation when the magnetization jumps from one anisotropic easy direction to another. There are also materials described by single-domain particles or at least partially described by single-domain particles. This is the case for small grains and particles that are too small to contain several domains [23].
3. Play Models of Magnetic Hysteresis
Play Models by Sums of Play Hysterons
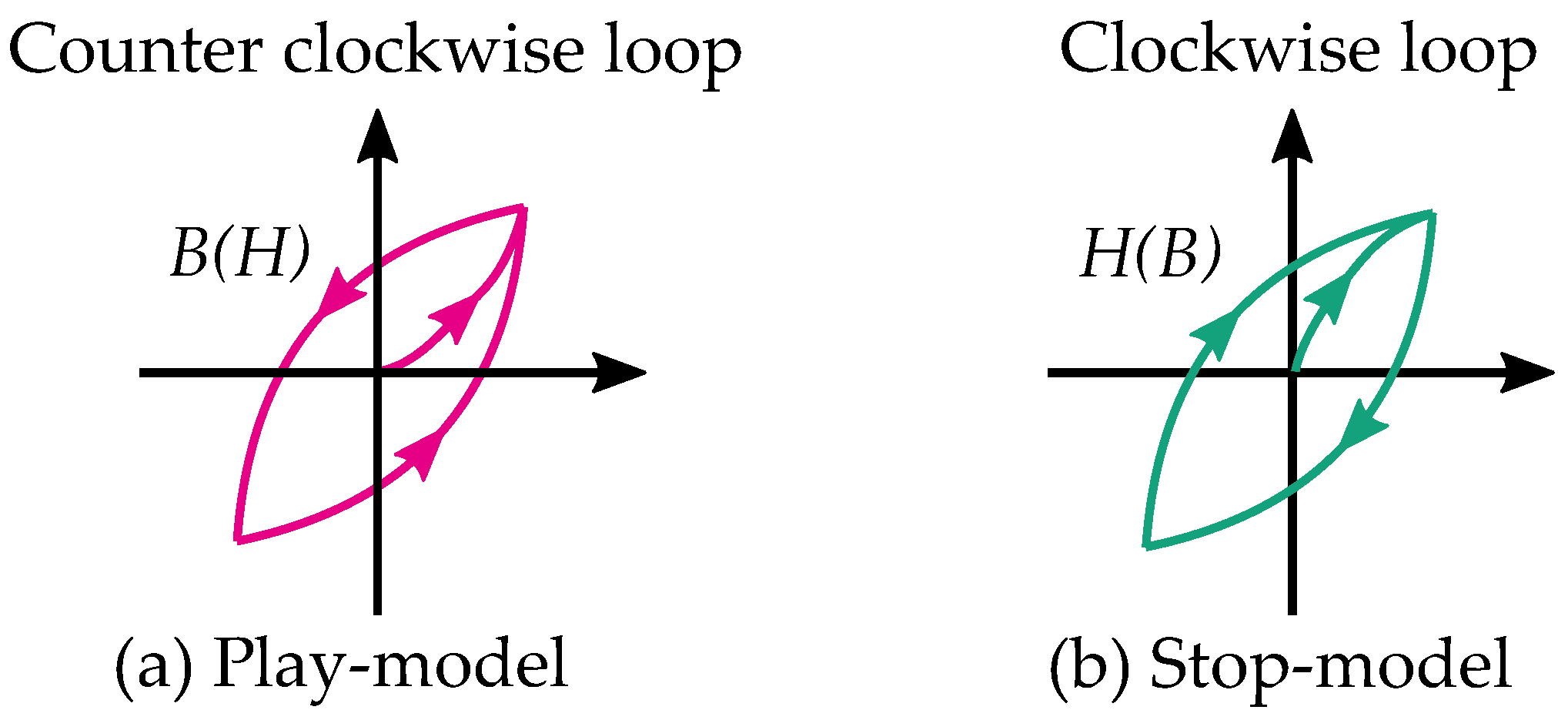
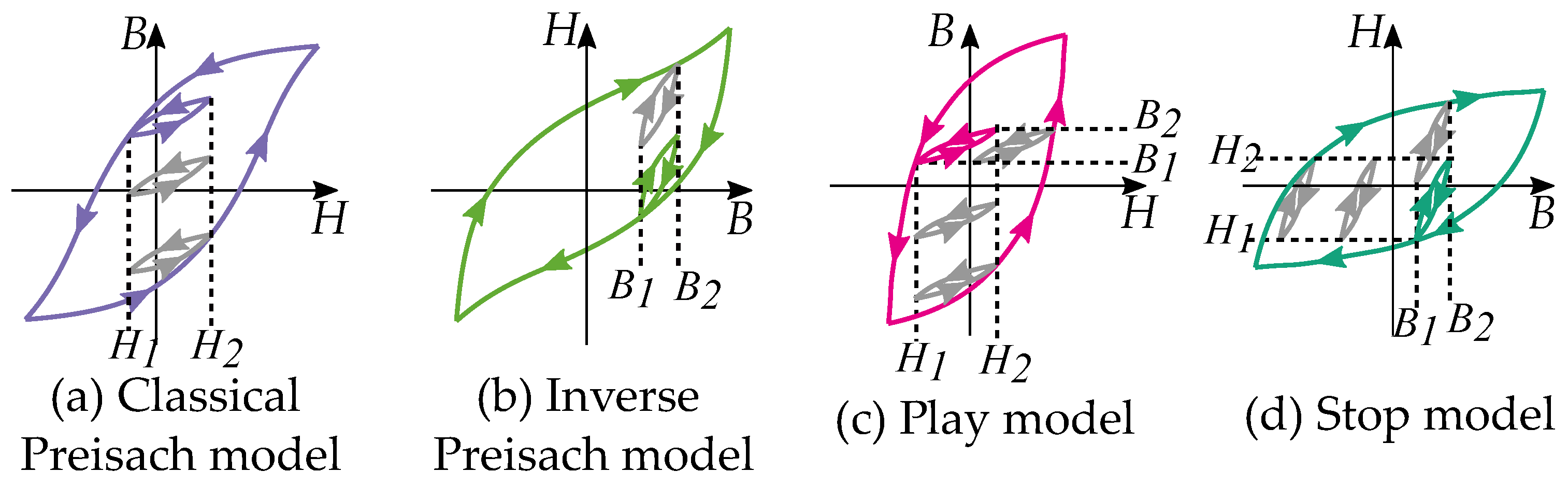
4. Stop Models
4.1. Prandtl–Ishlinskii Model
4.2. Modeling Play Models as Prandtl–Ishlinskii Models
4.3. Inconsequential Nomenclature of the Stop Model
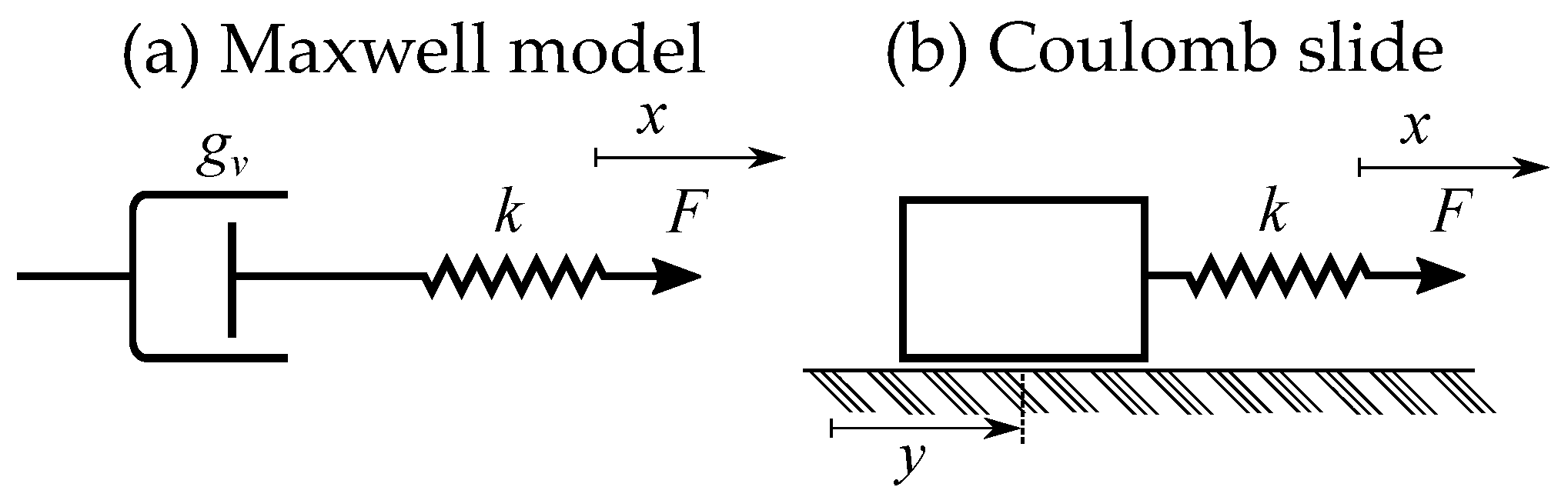
4.4. Lazan’s Generalized Maxwell Slip Model
4.5. Random Pinning of Domain Motions Seen as Friction
5. Comparison of Play and Stop
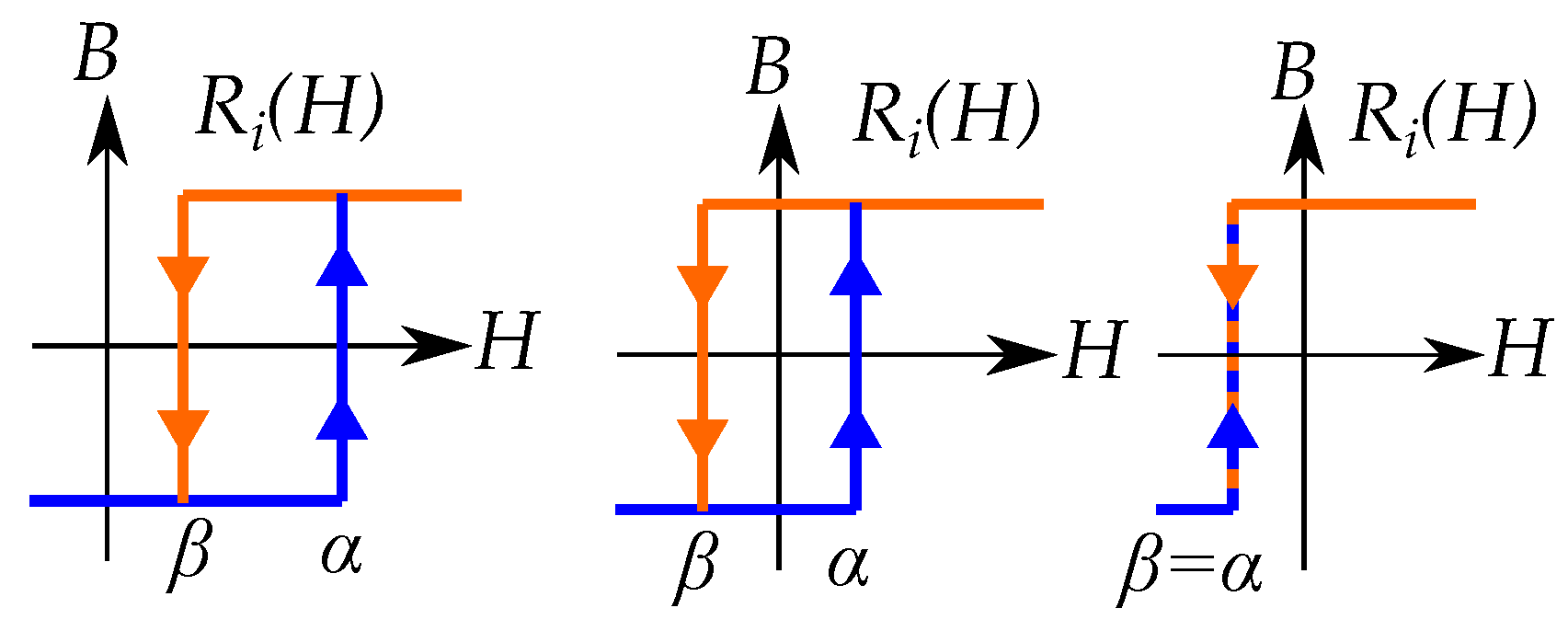
Play Hysterons Relation to Stop Hysterons
6. Shape of Play and Stop Models
6.1. Masing Model
- Loading curve: The initial curve is called a loading curve, and its shape is defined by a so-called backbone curve or skeleton curve.
- Unloading curve: All the reversal curves or loops are defined from the last reversal point. The unloading curve is always on the same shape as a loading curve but scaled with a scaling factor of two. The unloading curve also has the same initial slope as the loading curve.
- 3.
- Asymmetric cycle: The unloading and reloading curves should follow the initial loading curve if the previous maximum shear strain is exceeded.
- 4.
- Irregular cycling loads: If the current loading or unloading curve intersects the curve described by a previous loading or unloading curve, the stress–strain relationship follows the previous curve.
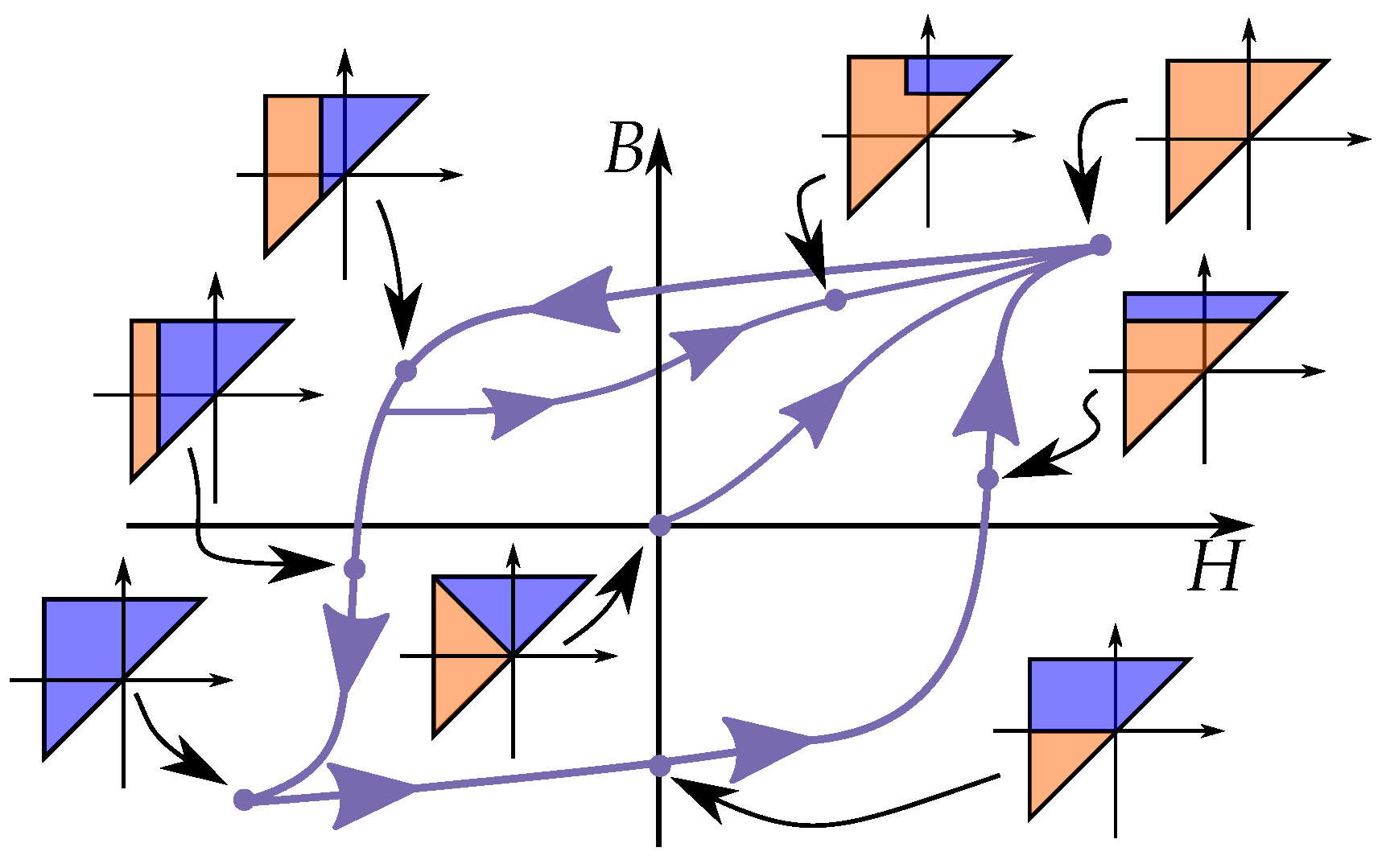
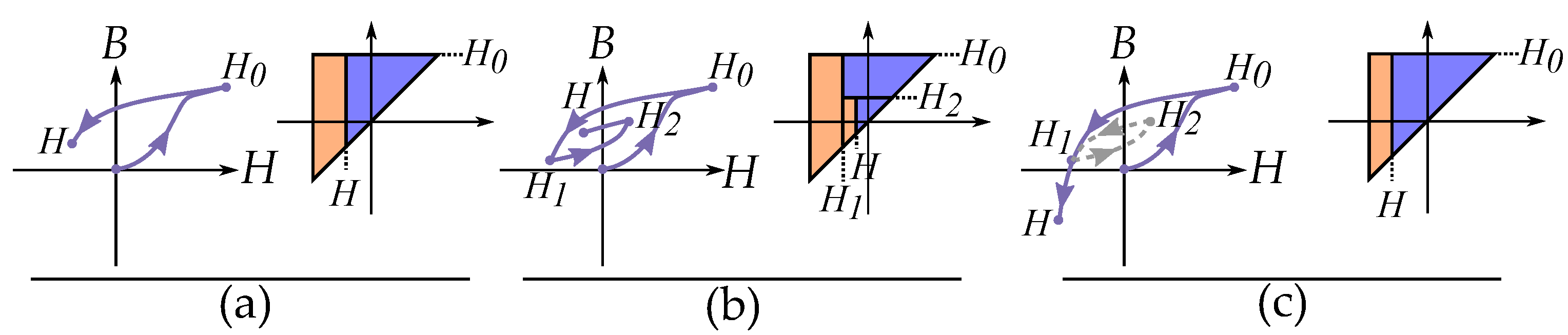
6.2. Madelung’s Rules; History by Reversal Points
- Each curve , which runs inside the hysteresis area, is clearly defined by the reversal point , from which it emerges.
- If one makes any point of this curve itself into a new reversal point , the curve defined by leads back again to the starting point of the curve .
- If the magnetization curve originating in to continues beyond , it continues as a continuation of the curve that had originally arrived at as if the cycle -- had not been present.
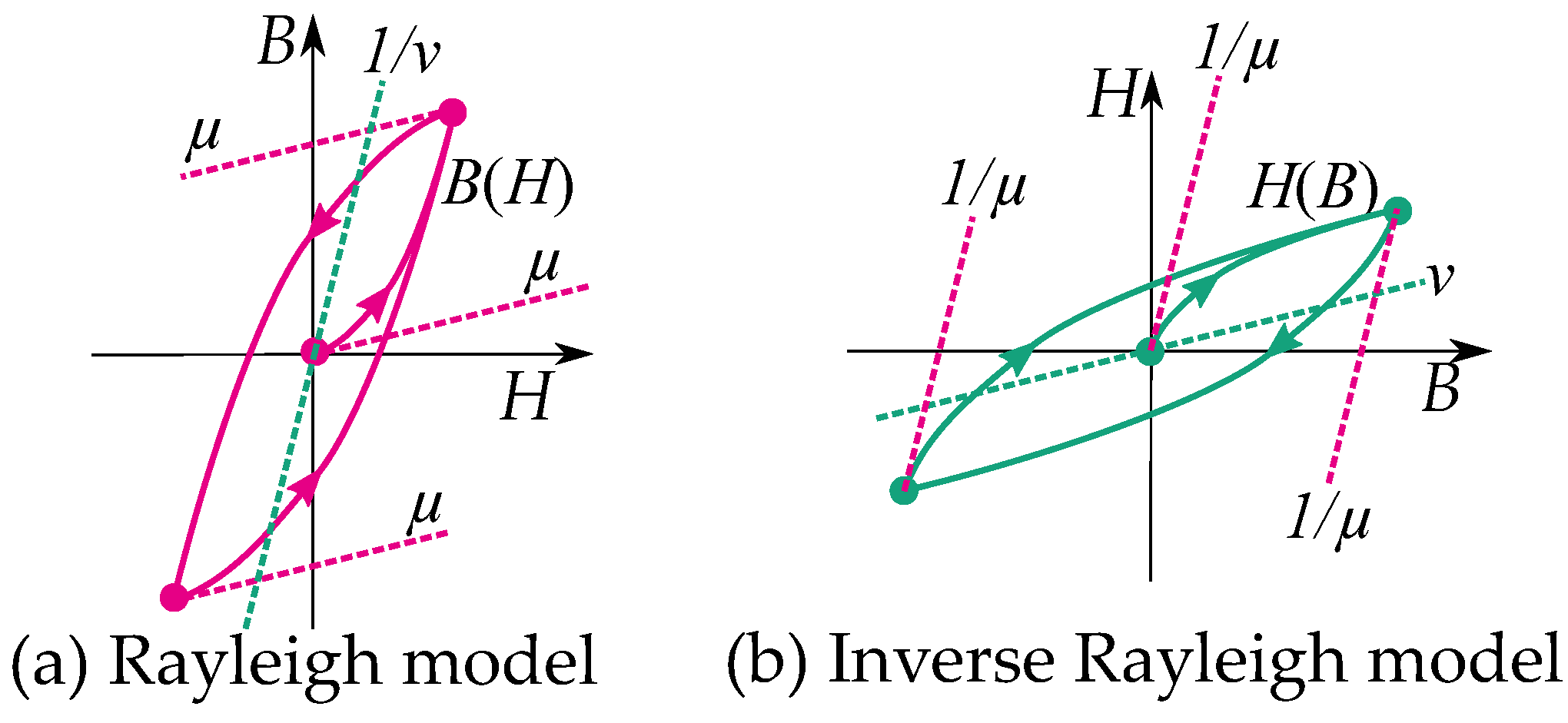
7. Example of a Play Model for the Rayleigh Model
7.1. Rayleigh Model as a Play Model
7.2. Inverse Rayleigh Model
8. Modeling for Iron Losses
8.1. Losses by the Stop Model
8.2. Steinmetz Equation as a Stop Model
8.3. Rain-Flow Modeling of Power Losses
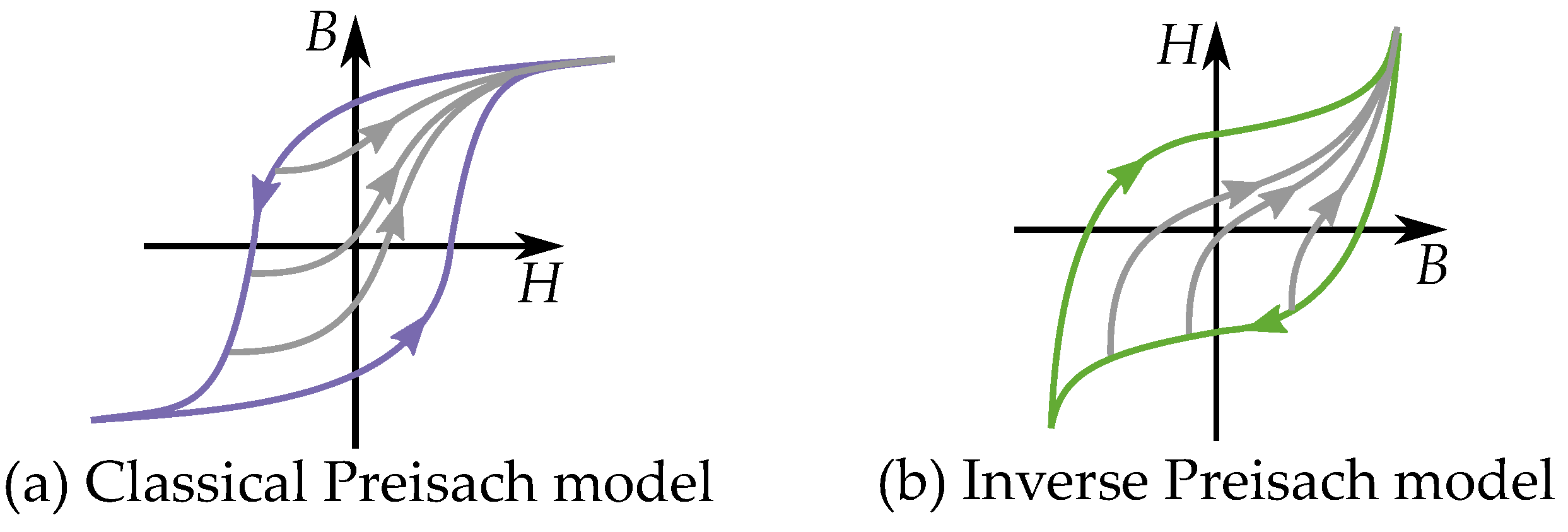
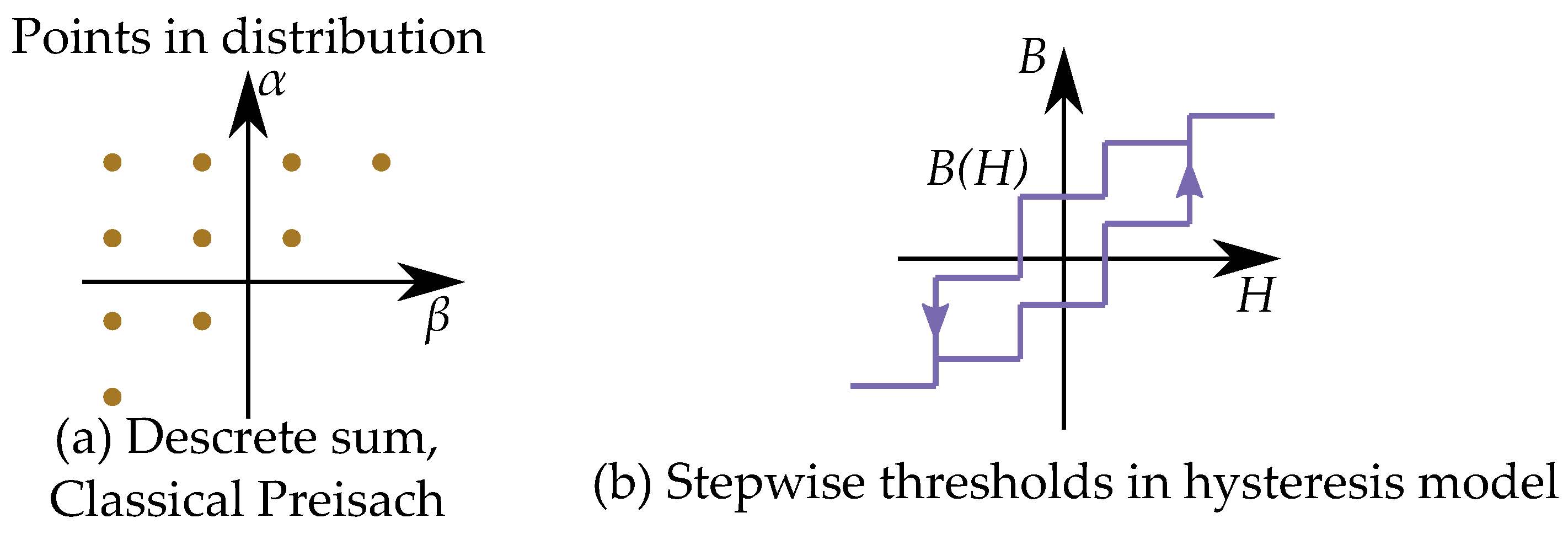
9. Preisach Model
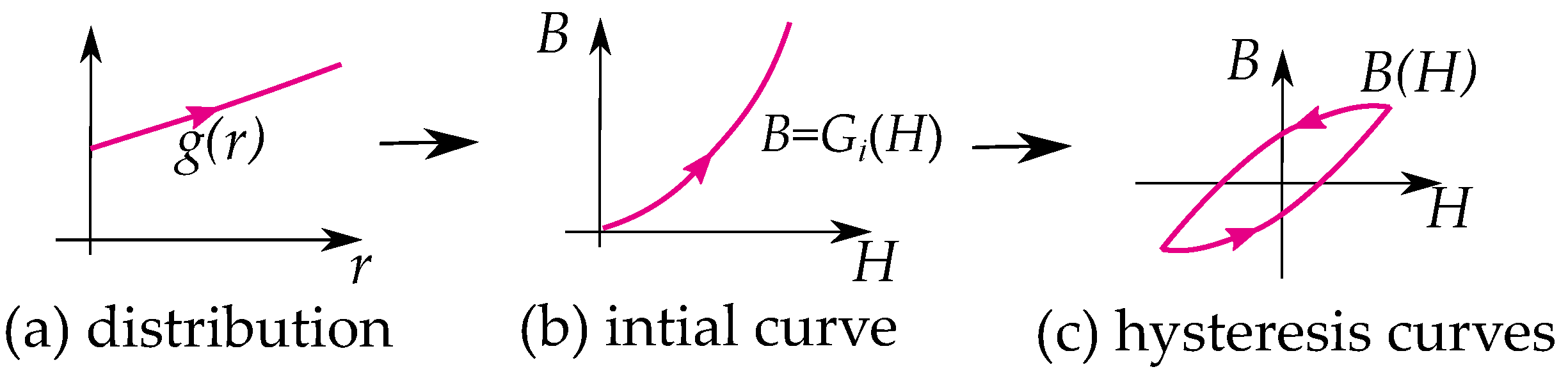
9.1. Continuous Preisach Model
9.2. Preisach Distributions
9.3. Simple Distribution for the Rayleigh Model
9.4. Distributions with Separation of Variables
9.5. Statistical Preisach Distributions
10. Changes of Magnetization
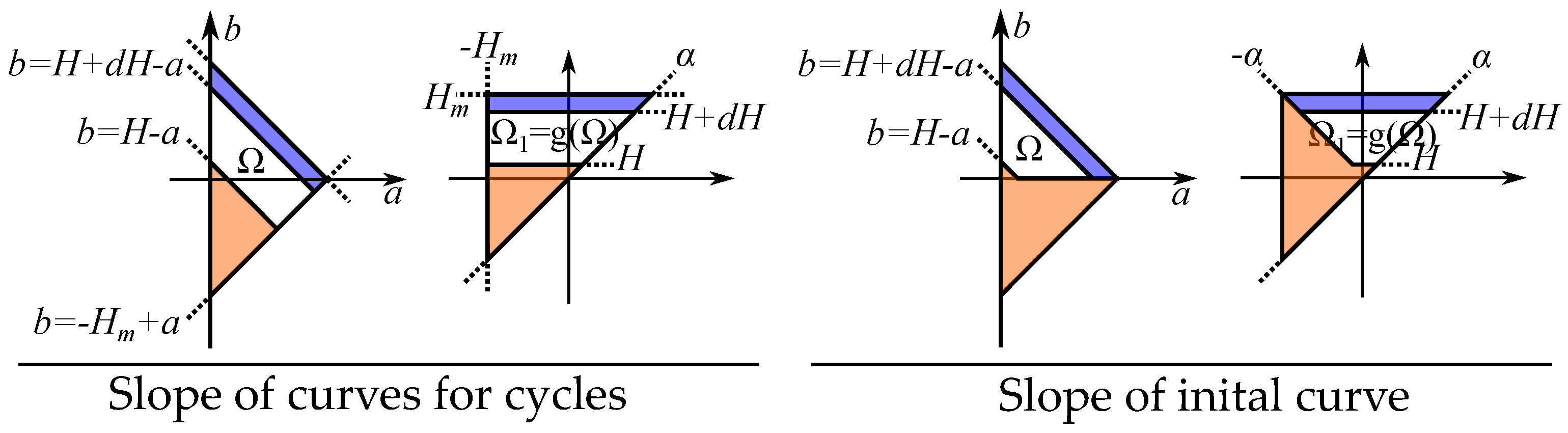
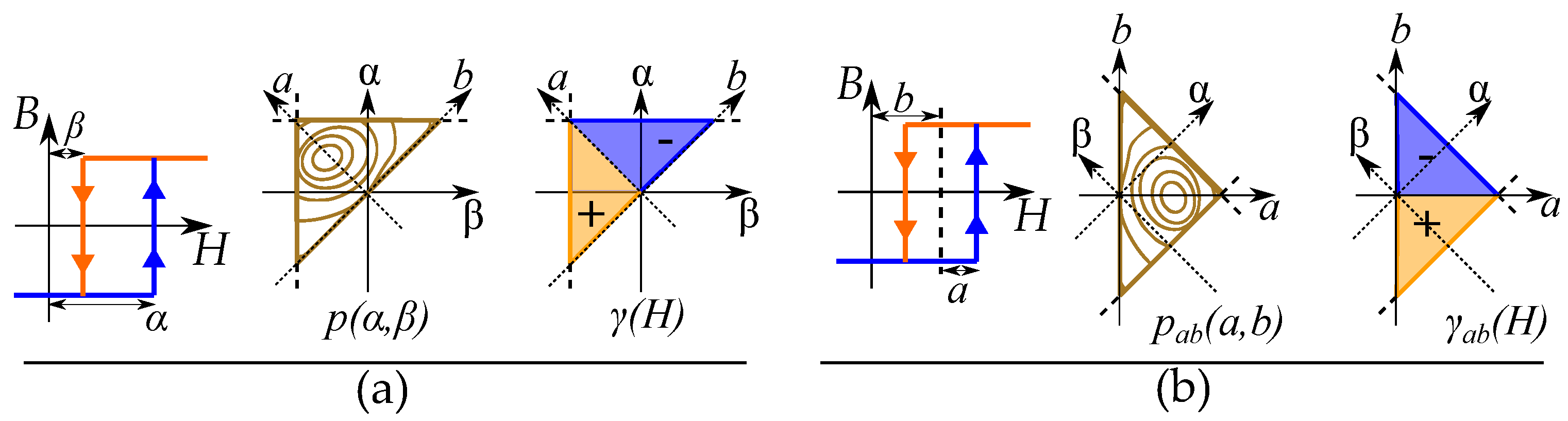
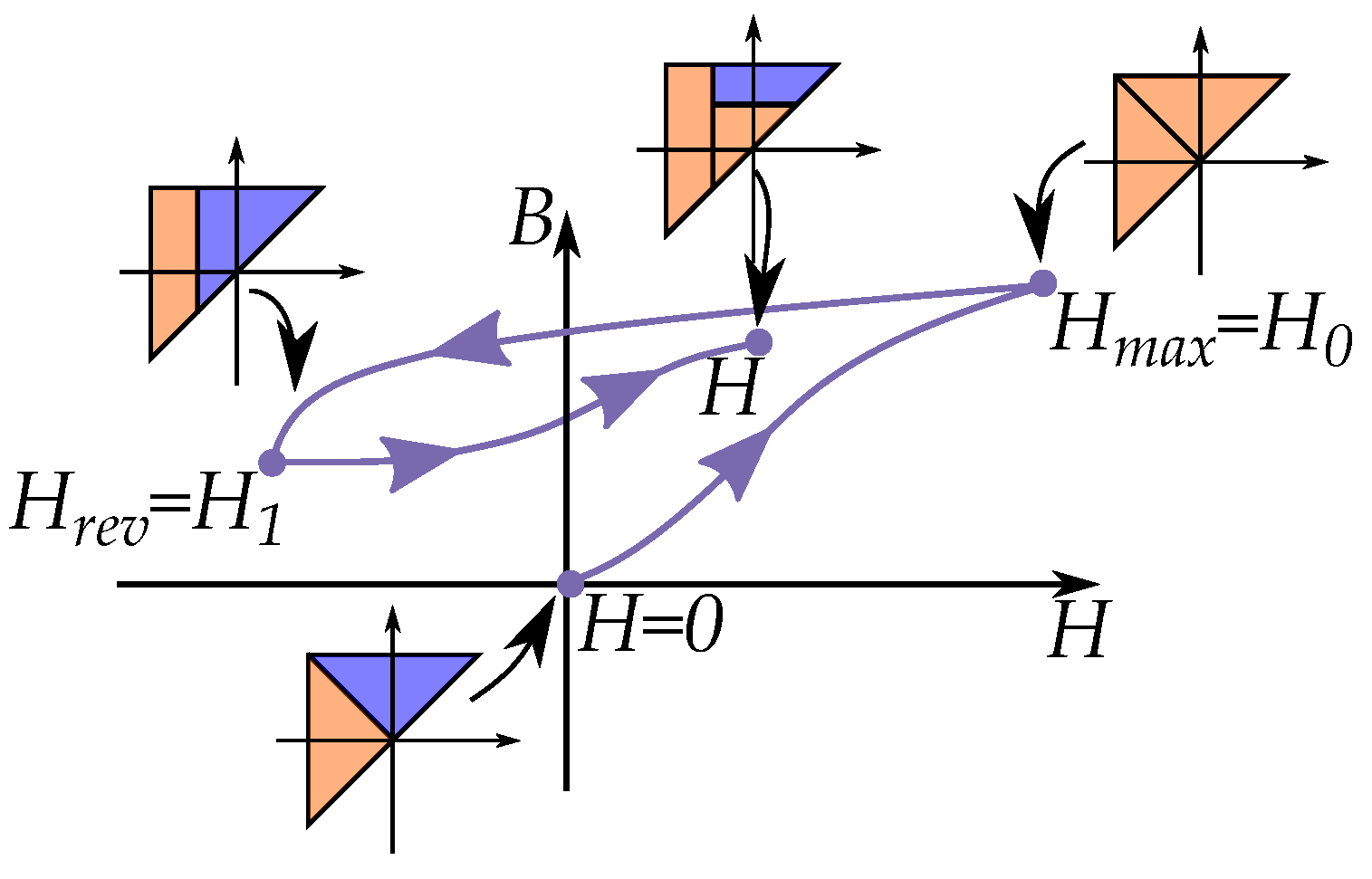
10.1. Triangular Areas in the Preisach Plane
10.2. Incremental Permeability
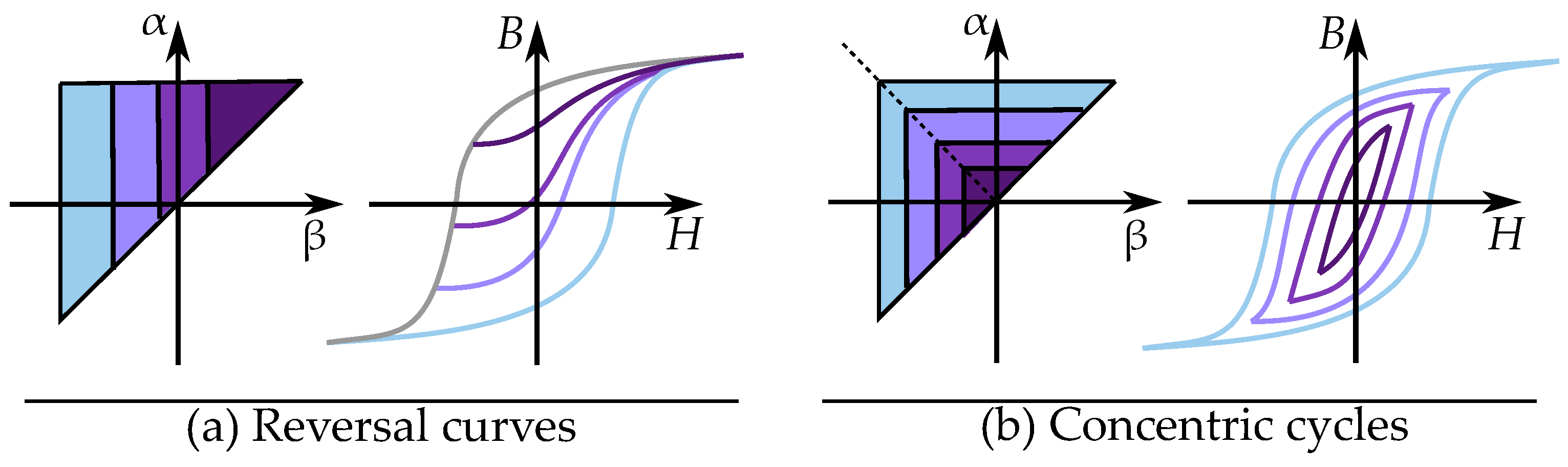
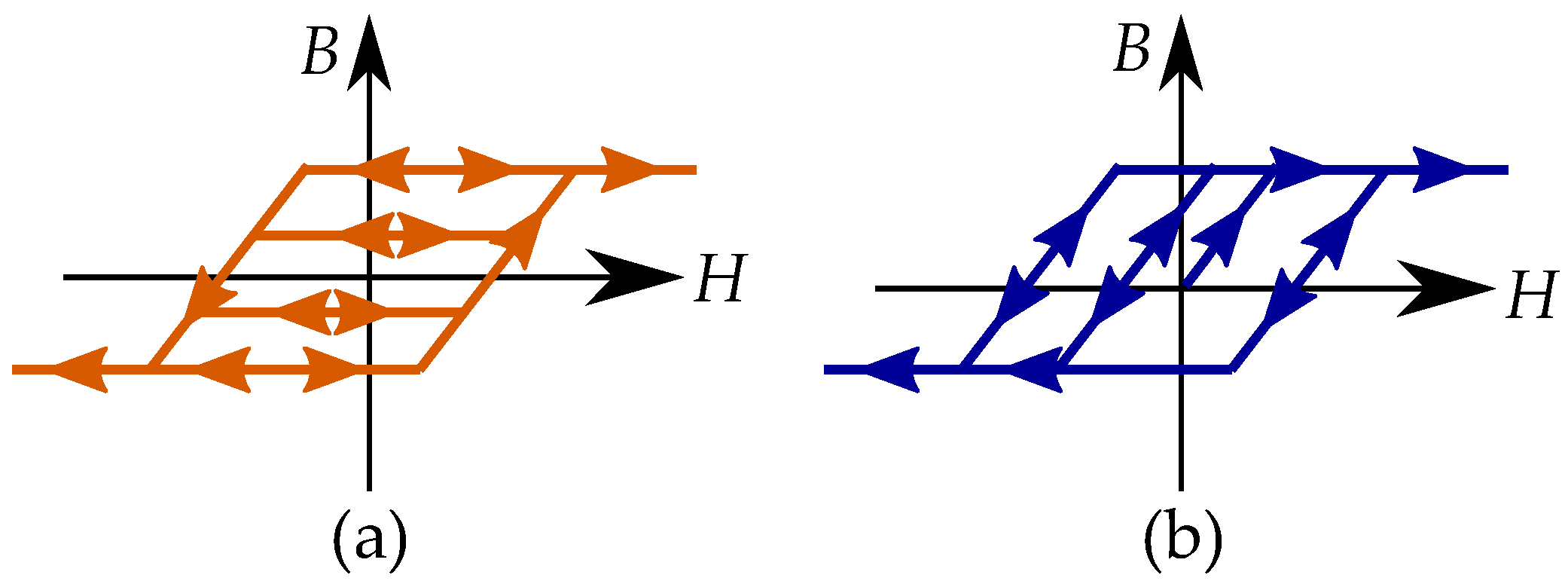
10.3. Congruency and Wiping Out Properties
11. History by Reversal Curves
11.1. Everett Functions
11.2. First-Order Reversal Curve Measurements
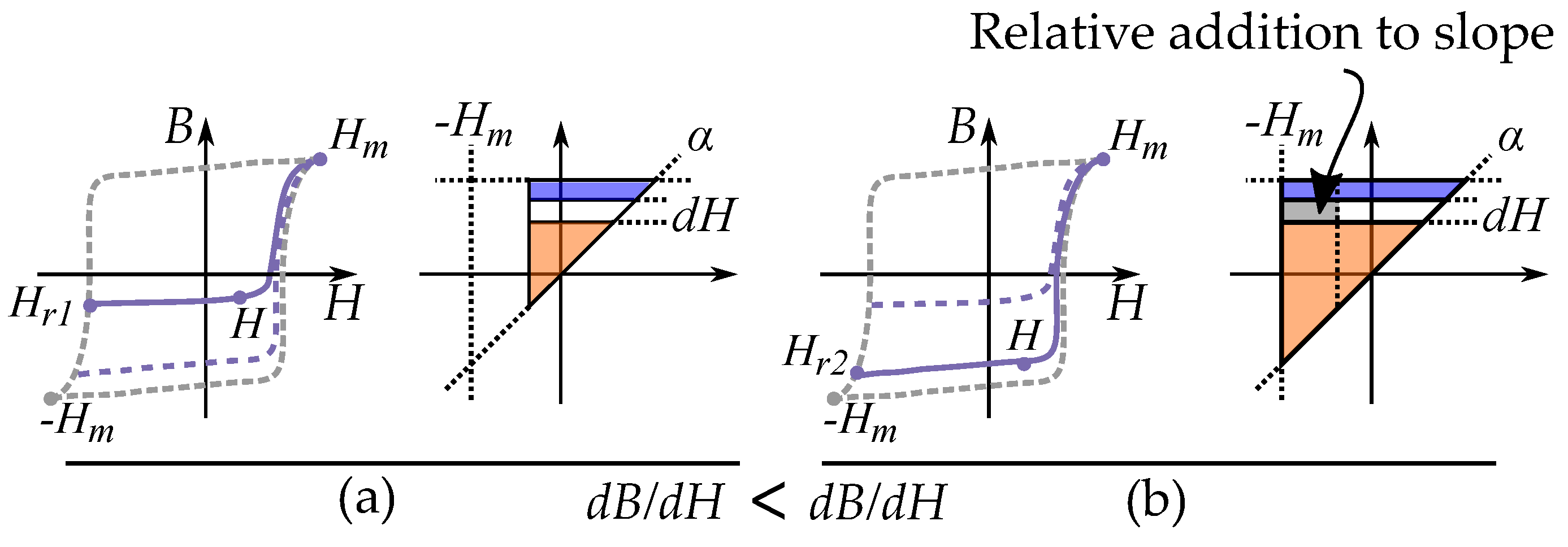
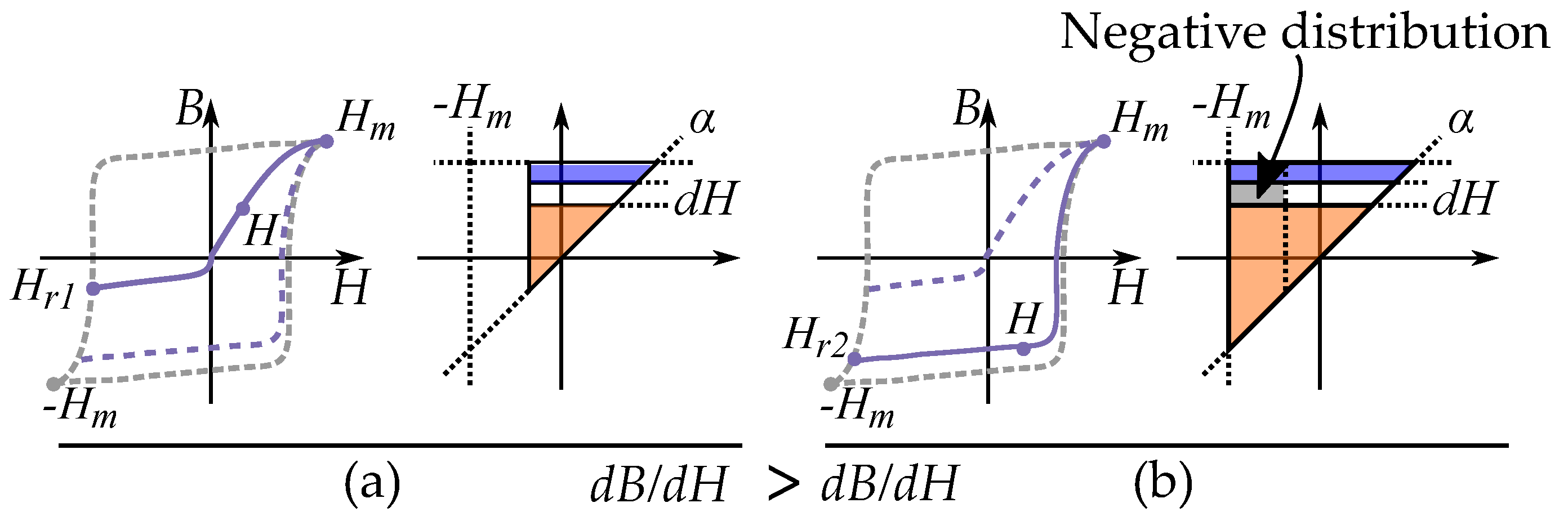
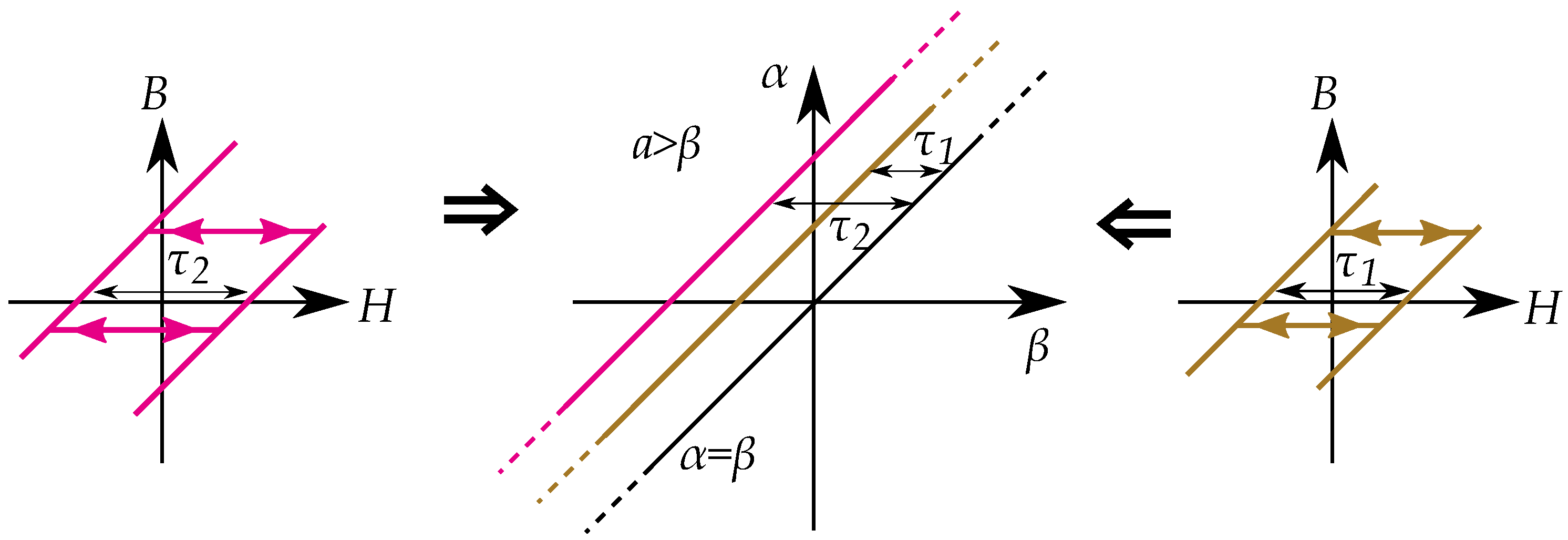
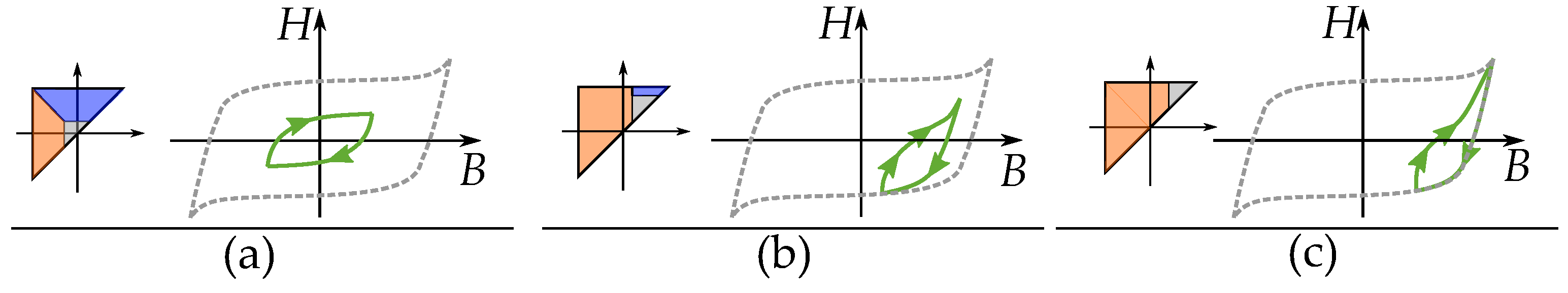
12. Negative Values in the Preisach Distribution
- This can appear in the classical Preisach model as something non-ideal in empirical measurements. When the type of material cannot be properly modeled by a Preisach model, such as hysteresis mechanisms driven by the nucleation of domains.
- It could also appear in the inverse Preisach model and is then less problematic. It is then a consequence of the clockwise hysteresis loop that appear for the inverse from, similar to a stop model.
12.1. Negative Values in the Classical Preisach Distribution
12.2. Hysteresis by Nucleation of Domains
12.3. Negative Values in the Inverted Preisach Model
13. Comparison of Hysterons
13.1. Play Model as a Preisach Model
13.2. Stop Models by Negative Preisach Distributions
14. Comparison of Congruency
Comparing Approaches to Initial Curves
15. Play Model as a Preisach Model
15.1. Writing a Single Play Operator as a Preisach Model
15.2. Case of Reversal Curves
15.3. Case of Initial Curves
15.4. The Incremental Permeability
15.5. Preisach Distribution for a Play Model
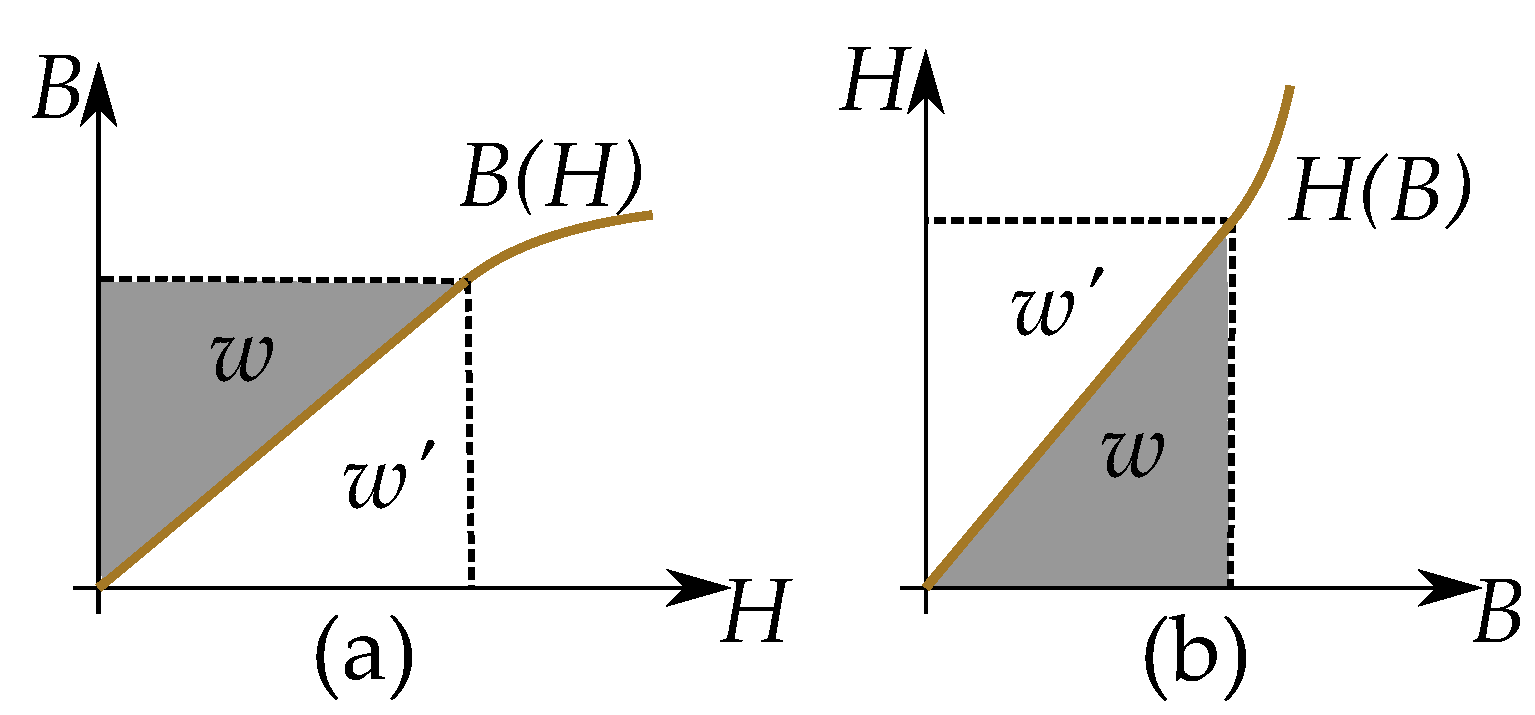
16. Comparison of Energy Losses
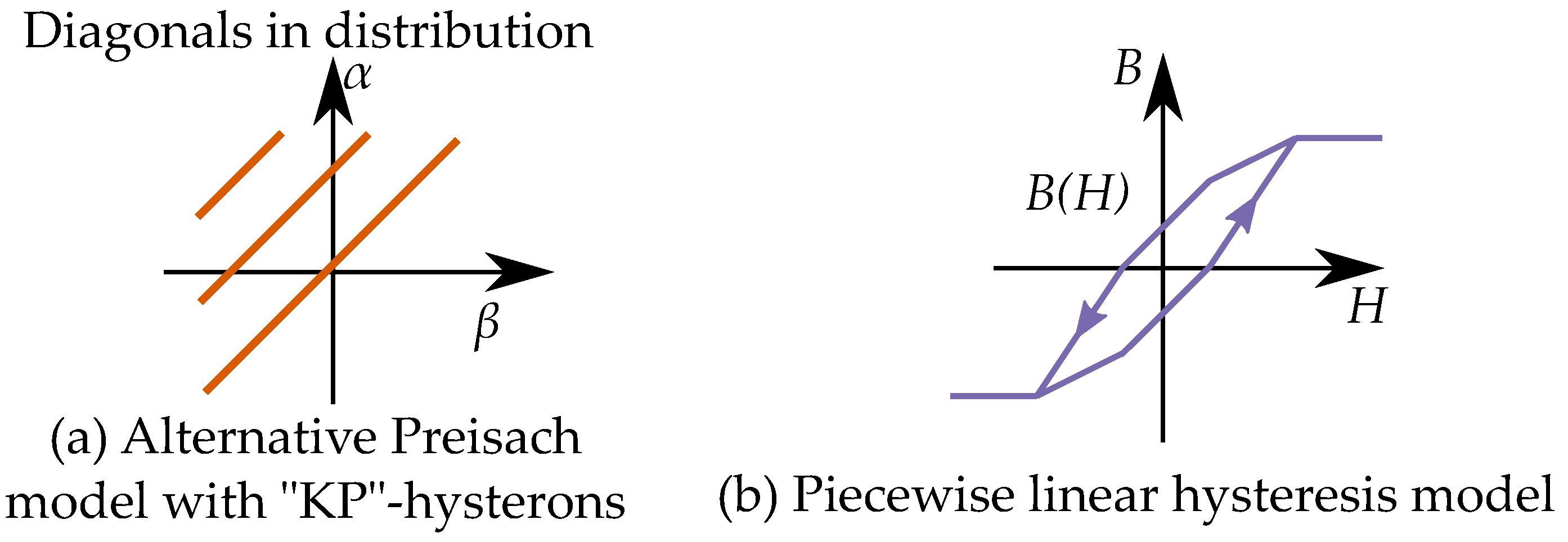
17. Alternative Hysteron Models
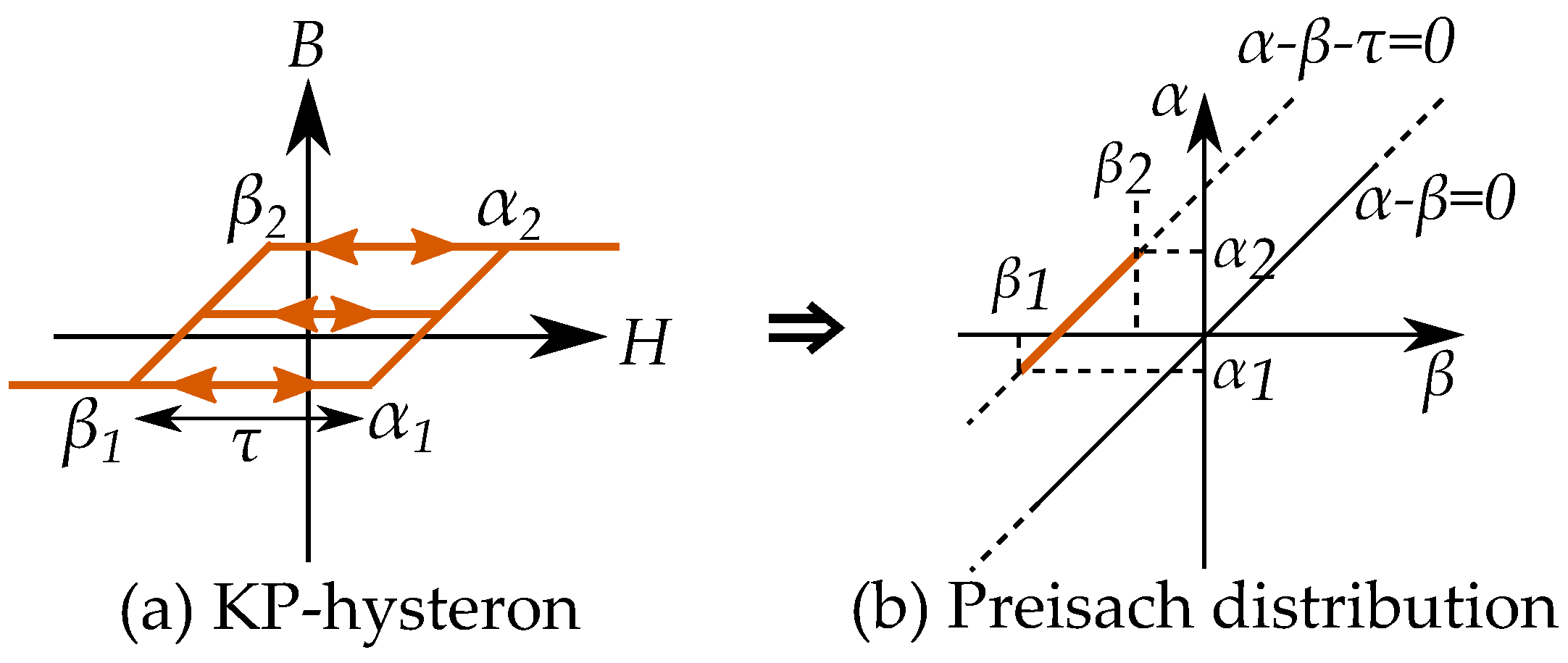
17.1. KP-Hysteron Model
17.2. Sigmoid Shaped General Play–Preisach Model
18. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Change of Coordinates
References
- Preisach, F. Über die magnetische Nachwirkung. Zeitschrift für Physik 1935, 94, 277–302. [Google Scholar] [CrossRef]
- Preisach, F. On the Magnetic Aftereffect. IEEE Trans. Magn. 2017, 53, 1–11. [Google Scholar] [CrossRef]
- Bergqvist, A.; Engdahl, G. A phenomenological differential-relation-based vector hysteresis model. J. Appl. Phys. 1994, 75, 5484–5486. [Google Scholar] [CrossRef]
- Bobbio, S.; Milano, G.; Serpico, C.; Visone, C. Models of magnetic hysteresis based on play and stop hysterons. IEEE Trans. Magn. 1997, 33, 4417. [Google Scholar] [CrossRef]
- Matsuo, T. Magnetic Hysteresis Represented by Play Model. In Motor Drive System and Magnetic Material; Fujisaki, K., Ed.; Springer: Berlin/Heidelberg, Germany, 2019; pp. 191–202. [Google Scholar] [CrossRef]
- Matsuo, T.; Osaka, Y.; Shimasaki, M. Eddy-current analysis using vector hysteresis models with play and stop hysterons. IEEE Trans. Magn. 2000, 36, 1172–1177. [Google Scholar] [CrossRef]
- Matsuo, T.; Terada, Y.; Shimasaki, M. Stop Model with Input-Dependent Shape Function and Its Identification Methods. IEEE Trans. Magn. 2004, 40, 1776–1783. [Google Scholar] [CrossRef]
- Prandtl, L. Ein Gedankenmodell zur kinetischen Theorie der festen Körper. ZAMM J. Appl. Math. Mech./Z. Angew. Math. Mech. 1928, 8, 85–106. [Google Scholar] [CrossRef]
- Krasnosel’skii, M.A.; Pokrovskii, A.V. Systems with Hysteresis, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1989. [Google Scholar] [CrossRef]
- Masing, G. Eigenspannungen und verfestigung beim messing. In Verhandlungen des 2. Internationalen Kongresses für technische Mechanik, Comptes rendus du 2ième Congrès international de méchanique appliquée, Proceedings of the Second International Congress for Applied Mechanics, Zürich, Switzerland, 12–17 September 1926; Orell Fussli: Zurich, Switzerland, 1927; pp. 332–335. [Google Scholar]
- Iwan, W.D. A Distributed-Element Model for Hysteresis and Its Steady-State Dynamic Response. J. Appl. Mech. 1966, 33, 893–900. [Google Scholar] [CrossRef]
- Jenkins, G.M. Analysis of the stress–strain relationships in reactor grade graphite. Br. J. Appl. Phys. 1962, 13, 30–32. [Google Scholar] [CrossRef]
- Krejči, P.; Lamba, H.; Melnik, S.; Rachinskii, D. Analytical solution for a class of network dynamics with mechanical and financial applications. Phys. Rev. E 2014, 90, 032822. [Google Scholar] [CrossRef]
- Liu, L.; Yang, Y. Modeling and Precision Control of Systems with Hysteresis; Elsevier Science and Technology: Oxford, UK, 2015; ISBN 9780128035672. [Google Scholar]
- Macki, J.W.; Nistri, P.; Zecca, P. Mathematical models for hysteresis. SIAM Rev. 1993, 35, 94–123. [Google Scholar] [CrossRef]
- Krejčí, P. On Maxwell equations with the Preisach hysteresis operator: The one-dimensional time-periodic case. Apl. Mat. 1989, 34, 364–374. [Google Scholar] [CrossRef]
- Krejčí, P.; Lovicar, V. Continuity of hysteresis operators in Sobolev spaces. Apl. Mat. 1990, 35, 60–66. [Google Scholar] [CrossRef]
- Visone, C. Hysteresis modelling and compensation for smart sensors and actuators. J. Phys. Conf. Ser. 2008, 138, 012028. [Google Scholar] [CrossRef]
- Livingston, J.D. A review of coercivity mechanisms (invited). J. Appl. Phys. 1981, 52, 2544–2548. [Google Scholar] [CrossRef]
- Hadjipanayis, G.; Singleton, E.; Tang, Z. Magnetization reversal in ferrite magnets. J. Magn. Magn. Mater. 1989, 81, 318–322. [Google Scholar] [CrossRef]
- Hadjipanayis, G.C.; Kim, A. Domain wall pinning versus nucleation of reversed domains in R-Fe-B magnets (invited). J. Appl. Phys. 1988, 63, 3310–3315. [Google Scholar] [CrossRef]
- Brailsford, F. Domain-wall energy and hysteresis loss in ferromagnetic material. Proc. Inst. Electr. Eng. 1970, 117, 1052–1055. [Google Scholar] [CrossRef]
- Flavio de Campos, M.; Adilson de Castro, J. An overview on nucleation theories and models. J. Rare Earths 2019, 37, 1015–1022. [Google Scholar] [CrossRef]
- Stoner, E.C.; Wohlfarth, E.P. A Mechanism of Magnetic Hysteresis in Heterogeneous Alloys. R. Soc. Lond. 1947, A240, 201–213. [Google Scholar] [CrossRef]
- De Campos, M.F.; Sampaio da Silva, F.A.; Perigo, E.A.; de Castro, J.A. Stoner–Wohlfarth model for the anisotropic case. J. Magn. Magn. Mater. 2013, 345, 147–152. [Google Scholar] [CrossRef]
- De Campos, M.F.; da Silva, F.A.; de Castro, J.A. Squareness of NdFeB Stoner-Wohlfarth Hysteresis. In Proceedings of the Advanced Powder Technology IX, Sao Paulo, Brazil, 27–29 October 2013; Materials Science Forum. Trans Tech Publications Ltd.: Bach, Switzerland, 2014; Volume 802, pp. 601–606. [Google Scholar] [CrossRef]
- Sampaio da Silva, F.A.; Castro, N.A.; de Campos, M.F. Modeling hysteresis curves of anisotropic SmCoFeCuZr magnets. J. Magn. Magn. Mater. 2013, 328, 53–57. [Google Scholar] [CrossRef]
- De Campos, M.; de Castro, J. Predicting Recoil Curves in Stoner–Wohlfarth Anisotropic Magnets. Acta Physica Polonica A 2019, 136, 737–739. [Google Scholar] [CrossRef]
- Brokate, M.; Huo, Y.; Kenmochi, N.; Müller, I.; Rodriguez, J.; Verdi, C. Phase Transitions and Hysteresis: Lectures Given at the Third Session of the Centro Internazionale Matematico Estivo (C.I.M.E.) held in Montecatini Terme, Italy, 13–21 July 1993; Visintin, Ed.; Lecture Notes in Mathematic; Springer: Berlin/Heidelberg, Germany, 1994; Volume 1584. [Google Scholar] [CrossRef]
- Bobbio, S.; Marrucci, G. A possible alternative to Preisach’s model of static hysteresis. Il Nuovo C. D 1993, 15, 723–733. [Google Scholar] [CrossRef]
- Takeda, Y.; Takahashi, Y.; Fujiwara, K.; Ahagon, A.; Matsuo, T. Iron Loss Estimation Method for Rotating Machines Taking Account of Hysteretic Property. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Alatawneh, N.; Pillay, P. Modeling of the interleaved hysteresis loop in the measurements of rotational core losses. J. Magn. Magn. Mater. 2016, 397, 157–163. [Google Scholar] [CrossRef]
- Minowa, N.; Takahashi, Y.; Fujiwara, K.; Ahagon, A.; Matsuo, T. Dynamic Hysteresis Modeling of Silicon Steel Sheet Considering Excess Eddy-current Loss. Int. J. Appl. Electromagn. Mech. 2019, 59, 217–226. [Google Scholar] [CrossRef]
- Matsuo, T.; Takahashi, Y.; Fujiwara, K. Pinning field representation using play hysterons for stress-dependent domain-structure model. J. Magn. Magn. Mater. 2020, 499, 166303. [Google Scholar] [CrossRef]
- Bergqvist, A. Magnetic vector hysteresis model with dry friction-like pinning. Phys. B Condens. Matter 1997, 233, 342–347. [Google Scholar] [CrossRef]
- Bergqvist, A.; Lundgren, A.; Engdahl, G. Experimental testing of an anisotropic vector hysteresis model. IEEE Trans. Magn. 1997, 33, 4152–4154. [Google Scholar] [CrossRef]
- Jiang, C.; Deng, M.; Inoue, A. Operator based robust control for nonlinear systems with hysteresis. In Proceedings of the SICE Annual Conference 2007, Takamatsu, Japan, 17–20 September 2007; pp. 2292–2295. [Google Scholar] [CrossRef]
- Deng, M.; Jiang, C.; Inoue, A.; Su, C.Y. Operator-based robust control for nonlinear systems with Prandtl–Ishlinskii hysteresis. Int. J. Syst. Sci. 2011, 42, 643–652. [Google Scholar] [CrossRef]
- Armin, M.; Roy, P.N.; Das, S.K. A Survey on Modelling and Compensation for Hysteresis in High Speed Nanopositioning of AFMs: Observation and Future Recommendation. Int. J. Autom. Comput. 2020, 17, 479–501. [Google Scholar] [CrossRef]
- Kuhnen, K. Modeling, Identification and Compensation of Complex Hysteretic Nonlinearities: A Modified Prandtl-Ishlinskii Approach. Eur. J. Control 2003, 9, 407–418. [Google Scholar] [CrossRef]
- Krejčí, P. On Ishlinskii’s model for non-perfectly elastic bodies. Apl. Mat. 1988, 33, 133–144. [Google Scholar] [CrossRef]
- Visintin, A. Differential Models of Hysteresis; Series: Applied Mathematical Sciences; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar] [CrossRef]
- Capecchi, D.; Masiani, R.; Vestroni, F. Periodic and Non-Periodic Oscillations of a Class of Hysteretic Two Degree of Freedom Systems. Nonlinear Dyn. 1997, 13, 309–325. [Google Scholar] [CrossRef]
- Chiang, D.Y. The generalized Masing models for deteriorating hysteresis and cyclic plasticity. Appl. Math. Model. 1999, 23, 847–863. [Google Scholar] [CrossRef]
- Segalman, D.J.; Starr, M.J. Inversion of Masing models via continuous Iwan systems. Int. J.-Non-Linear Mech. 2008, 43, 74–80. [Google Scholar] [CrossRef]
- Iwan, W.D. On a Class of Models for the Yielding Behavior of Continuous and Composite Systems. J. Appl. Mech. 1967, 34, 612–617. [Google Scholar] [CrossRef]
- Goldfarb, M.; Celanovic, N. Modeling piezoelectric stack actuators for control of micromanipulation. IEEE Control Syst. Mag. 1997, 17, 69–79. [Google Scholar] [CrossRef]
- Lazan, B.J. Damping of Materials and Members in Structural Mechanics, 1st ed.; Pergamon Press: Oxford, UK, 1968. [Google Scholar]
- Wiechert, E. Gesetze der elastischen Nachwirkung für constante Temperatur. Ann. Phys. 1893, 286, 335–348. [Google Scholar] [CrossRef]
- Popova, E.; Popov, V. The research works of Coulomb and Amontons and generalized laws of friction. Friction 2015, 3, 183–190. [Google Scholar] [CrossRef]
- Al-Bender, F.; Lampaert, V.; Swevers, J. The generalized Maxwell-slip model: A novel model for friction Simulation and compensation. IEEE Trans. Autom. Control 2005, 50, 1883–1887. [Google Scholar] [CrossRef]
- Ruderman, M.; Bertram, T. Modified Maxwell-slip Model of Presliding Friction. IFAC Proc. Vol. 2011, 44, 10764–10769. [Google Scholar] [CrossRef]
- Hassani, V.; Tjahjowidodo, T.; Do, T.N. A survey on hysteresis modeling, identification and control. Mech. Syst. Signal Process. 2014, 49, 209–233. [Google Scholar] [CrossRef]
- Gan, J.; Zhang, X. A review of nonlinear hysteresis modeling and control of piezoelectric actuators. AIP Adv. 2019, 9, 040702. [Google Scholar] [CrossRef]
- Popov, V.; Gray, J. Prandtl-Tomlinson model: History and applications in friction, plasticity, and nanotechnologies. ZAMM J. Appl. Math. Mech./Z. Angew. Math. Mech. 2012, 92, 683–708. [Google Scholar] [CrossRef]
- Reggio, A.; De Angelis, M. Modelling and identification of structures with rate-independent linear damping. Meccanica 2015, 50, 617–632. [Google Scholar] [CrossRef]
- Maxwell, J.C. The Bakerian Lecture.On the viscosity or internal friction of air and other gases. Philos. Trans. R. Soc. Lond. 1866, 156, 249–268. [Google Scholar] [CrossRef]
- Maxwell, J.C. On the Dynamical Theory of Gases. Philos. Trans. R. Soc. Lond. 1867, 157, 49–88. [Google Scholar]
- Maxwell, J.C., II. Illustrations of the dynamical theory of gases. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1860, 20, 21–37. [Google Scholar] [CrossRef]
- Maxwell, J.C., XV. On the dynamical theory of gases. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1868, 35, 129–145. [Google Scholar] [CrossRef]
- Bertotti, G. Chapter 10—Magnetization Curves. In Hysteresis in Magnetism; Bertotti, G., Ed.; Electromagnetism, Academic Press: San Diego, CA, USA, 1998; pp. 297–346. [Google Scholar] [CrossRef]
- Bertotti, G.; Mayergoyz, I.D.; Basso, V.; Magni, A. Functional integration approach to hysteresis. Phys. Rev. E 1999, 60, 1428–1440. [Google Scholar] [CrossRef] [PubMed]
- Al-Janaideh, M.; Rakotondrabe, M.; Al-Janaideh, O. Further Results on Hysteresis Compensation of Smart Micropositioning Systems With the Inverse Prandtl–Ishlinskii Compensator. IEEE Trans. Control. Syst. Technol. 2016, 24, 428–439. [Google Scholar] [CrossRef]
- Mayergoyz, I.D.; Andrei, P. Preisach modeling of clockwise hysteresis and its application to front propagation problems. J. Appl. Phys. 2002, 91, 7645–7647. [Google Scholar] [CrossRef]
- Mayergoyz, I.D. Mathematical Models of Hysteresis and Their Applications; Elsevier: Amsterdam, The Netherlands, 2003; Norm. Distr.: Ch 4, Clockwise loop: Ch 2.1, Play model: Ch 1, KP-hysteron: Ch 1.3. [Google Scholar]
- Brokate, M.; Sprekels, J. Hysteresis and Phase Transitions, 1st ed.; Springer: New York, NY, USA, 1996; Preisach type models: Ch 2.3 p 42. Rainflow counting: Ch 2, p 78, Alg 2.6.10. Masing’s law: Section 2.8, Remark 2.8.3. [Google Scholar]
- Jayakumar, P. Modeling and Identification in Structural Dynamics. Ph.D. Thesis, CalTech, Pasadena, CA, USA, 1987. PB-89-194146/AS, CaltechEERL: 1987.EERL-87-01. [Google Scholar]
- Ashrafi, S.A.; Smyth, A.W. Generalized Masing Approach to Modeling Hysteretic Deteriorating Behavior. J. Eng. Mech. 2007, 133, 495–505. [Google Scholar] [CrossRef]
- Farrokh, M.; Dizaji, M.S.; Dizaji, F.S.; Moradinasab, N. Universal Hysteresis Identification Using Extended Preisach Neural Network. arXiv 2019, arXiv:2001.01559. [Google Scholar]
- Pyke, R.M. Nonlinear Soil Models for Irregular Cyclic Loadings. J. Geotech. Eng. Div. 1979, 105, 715–726. [Google Scholar] [CrossRef]
- Rosenblueth, E.; Herrera, I. On a Kind of Hysteretic Damping. J. Eng. Mech. Div. 1964, 90, 37–48, Paper No 3999 EM4. [Google Scholar] [CrossRef]
- Herrera, I. Modelos Dinámicos para Materiales y Estructuras del Tipo de Masing. BoletíN Soc. Mex. Ing. SíSmica 1965, 3, 1–8. (In Spanish) [Google Scholar]
- Jennings, P.C. Response Of Yielding Structures To Statistically Generated Ground Motion. In Proceedings of the Third World Conference on Earthquake Engineering, Wellington, New Zealand, 22 January–1 February 1965; Volume 2, pp. 783–796. [Google Scholar]
- Jennings, P.C. Periodic Response of a General Yielding Structure. J. Eng. Mech. Div. Proc. Am. Soc. Civ. Eng. 1964, 90, 131–166. [Google Scholar] [CrossRef]
- Finn, W.D.L.; Martin, G.R.; Lee, K.W. An Effective Stress Model for Liquefaction. J. Geotech. Eng. Div. Proc. Am. Soc. Civ. Eng. 1977, 103, 517–533. [Google Scholar] [CrossRef]
- Muravskii, G. On description of hysteretic behaviour of materials. Int. J. Solids Struct. 2005, 42, 2625–2644. [Google Scholar] [CrossRef]
- Madelung, E. Über Magnetisierung durch schnellverlaufende Ströme und die Wirkungsweise des Rutherford-Marconischen Magnetdetektors. Ann. Phys. 1905, 322, 861–890. [Google Scholar] [CrossRef]
- Harrison, R.G. Positive-Feedback Theory of Hysteretic Recoil Loops in Hard Ferromagnetic Materials. IEEE Trans. Magn. 2011, 47, 175–191. [Google Scholar] [CrossRef]
- Lord Rayleigh, F.R.S. XXV. Notes on electricity and magnetism.—III. On the behaviour of iron and steel under the operation of feeble magnetic forces. Philos. Mag. Ser. 5 1887, 23, 225–245. [Google Scholar] [CrossRef]
- Kachniarz, M.; Szewczyk, R. Study on the Rayleigh Hysteresis Model and its Applicability in Modeling Magnetic Hysteresis Phenomenon in Ferromagnetic Materials. Acta Phys. Pol. A 2017, 131, 1244–1249. [Google Scholar] [CrossRef]
- Bush, H.D. Departure from the Rayleigh Law of the Magnetization of a Ferromagnetic Material. Nature 1950, 166, 401–402. [Google Scholar] [CrossRef]
- Cullity, B.; Graham, C. Introduction to Magnetic Materials, 2nd ed.; IEEE-Press and Wiley: Hoboken, NJ, USA, 2009; Rayleigh region: Ch 9.13. [Google Scholar] [CrossRef]
- Baldwin, J.A.; Revol, J.; Milstein, F. Failure of the Rayleigh hysteresis law in low magnetic fields. Phys. Rev. B 1977, 15, 426–428. [Google Scholar] [CrossRef]
- Baldwin, J. Rayleigh hysteresis-A new look at an old law. IEEE Trans. Magn. 1978, 14, 81–84. [Google Scholar] [CrossRef]
- Zapperi, S.; Magni, A.; Durin, G. Microscopic foundations of the Rayleigh law of hysteresis. J. Magn. Magn. Mater. 2002, 242–245, 987–992. [Google Scholar] [CrossRef]
- Bintachitt, P.; Jesse, S.; Damjanovic, D.; Han, Y.; Reaney, I.M.; Trolier-McKinstry, S.; Kalinin, S.V.; Nattermann, T. Collective dynamics underpins Rayleigh behavior in disordered polycrystalline ferroelectrics. Proc. Natl. Acad. Sci. USA 2010, 107, 7219–7224. [Google Scholar] [CrossRef]
- Dietzmann, G.; Schaefer, M. Rayleigh hysteresis with sinusoidal wave form of magnetic induction. J. Magn. Magn. Mater. 1992, 110, 151–160. [Google Scholar] [CrossRef]
- Ponomarev, Y.F. On the Rayleigh Law of Magnetization: A New Mathematical Model of Hysteresis Loops. Phys. Met. Metallogr. 2007, 104, 469–477. [Google Scholar] [CrossRef]
- Ponomarev, Y. On the Rayleigh law of magnetization. Symmetrical and asymmetric hysteresis loops. Experiment. Phys. Met. Metallogr. 2008, 105, 263–274. [Google Scholar] [CrossRef]
- Kaido, C. Modeling of magnetization curves in nonoriented electrical steel sheets. Electr. Eng. Jpn. 2012, 180, 1–8. [Google Scholar] [CrossRef]
- Canova, A.; Freschi, F.; Giaccone, L.; Repetto, M. Numerical Modeling and Material Characterization for Multilayer Magnetically Shielded Room Design. IEEE Trans. Magn. 2018, 54, 1–4. [Google Scholar] [CrossRef]
- Paesano, A., Jr.; Ferreira, R.F.; Schafer, D.; Alves, T.J.B.; Fortunato, J.; Ivashita, F.F. Application of the modified Rayleigh model in the mathematical analysis of Alnico II minor loops. Phys. B Condens. Matter 2021, 612, 412629. [Google Scholar] [CrossRef]
- Néel, L. Théorie des lois d’aimantation de Lord Rayleigh et les déplacements d’une paroi isolée. Cah. Phys. 1942, 12, 1–20. [Google Scholar]
- Néel, L. Some theoretical aspects of rock-magnetism. Adv. Phys. 1955, 4, 191–243. [Google Scholar] [CrossRef]
- Bertotti, G. Domain Wall Dynamics and Preisach Modeling. In Magnetic Hysteresis in Novel Magnetic Materials; Hadjipanayis, G., Ed.; NATO ASI Series (Series E: Applied Sciences); Springer: Berlin/Heidelberg, Germany, 1997; Volume 338, pp. 125–157. [Google Scholar] [CrossRef]
- Koller, A.; Pfrenger, E.; Stierstadt, K. New Interpretation of the Rayleigh Law. J. Appl. Phys. 1968, 39, 869–870. [Google Scholar] [CrossRef]
- Ramberg, W.; Osgood, W.R. Description of Stress-Strain Curves by Three Parameters; NASA Technical Reports Server: Cleveland, OH, USA, 1943; ID: 9930081614, Report Number: NACA-TN-902. [Google Scholar]
- Nazarov, V.; Kiyashko, S. Modified Davidenkov hysteresis and the propagation of sawtooth waves in polycrystals with hysteresis loss saturation. Phys. Met. Metallogr. 2016, 117, 766–771. [Google Scholar] [CrossRef]
- Marsh, G.; Wignall, C.; Thies, P.R.; Barltrop, N.; Incecik, A.; Venugopal, V.; Johanning, L. Review and application of Rainflow residue processing techniques for accurate fatigue damage estimation. Int. J. Fatigue 2016, 82, 757–765. [Google Scholar] [CrossRef]
- Sumarac, D.; Knezevic, P.; Dolicanin, C.; Cao, M. Preisach Elasto-Plastic Model for Mild Steel Hysteretic Behavior-Experimental and Theoretical Considerations. Sensors 2021, 21, 3546. [Google Scholar] [CrossRef] [PubMed]
- McKeehan, L.W.; Bozorth, R.M. Hysteresis Losses and the Area of the Hysteresis Loop. Phys. Rev. 1934, 46, 526–527. [Google Scholar] [CrossRef]
- Warburg, E.; Hönig, L. Ueber die Wärme, welche durch periodisch wechselnde magnetisirende Kräfte im Eisen erzeugt wird. Ann. Phys. 1883, 256, 814–835. [Google Scholar] [CrossRef]
- Warburg, E. Magnetische Untersuchungen. Ann. Phys. 1881, 249, 141–164. [Google Scholar] [CrossRef]
- Steinmetz, C.P. On the Law of Hysteresis. Trans. Am. Inst. Electr. Eng. 1892, IX, 1–64. [Google Scholar] [CrossRef]
- Steinmetz, C.P. On the Law of Hysteresis (Part II.) and Other Phenomena of the Magnetic Circuit. Trans. Am. Inst. Electr. Eng. 1892, IX, 619–758. [Google Scholar] [CrossRef]
- Steinmetz, C.P. On the Law of Hysteresis (Part III.), and the Theory of Ferric Inductances. Trans. Am. Inst. Electr. Eng. 1894, XI, 570–616. [Google Scholar] [CrossRef]
- Kobayashi, S.; Ishibashi, Y.; Baba, R. Re-examination of the Steinmetz law for unsymmetrical magnetic hysteresis loops. J. Magn. Magn. Mater. 2013, 330, 49–54. [Google Scholar] [CrossRef]
- Takahashi, S.; Zhang, L. Minor Hysteresis Loop in Fe Metal and Alloys. J. Phys. Soc. Jpn. 2004, 73, 1567–1575. [Google Scholar] [CrossRef]
- Takahashi, S.; Kobayashi, S.; Shishido, T. A scaling power-law relation in magnetic minor hysteresis loops in Fe and Ni metals. J. Phys. Condens. Matter 2007, 20, 035217. [Google Scholar] [CrossRef]
- Takahashi, S.; Kobayashi, S.; Kamada, Y.; Shishido, T. Similarity rules of magnetic minor hysteresis loops in Fe and Ni metals. J. Magn. Magn. Mater. 2008, 320, 2056–2061. [Google Scholar] [CrossRef]
- Endo, T.; Mitsunaga, K.; Takahashi, K.; Kobayashi, K.; Matsuishi, M. Damage evaluation of metals for random or varying loading—Three aspects of rain flow method. Mech. Behav. Mater. 1974, 1, 371–380. [Google Scholar]
- Socie, D. Rainflow Cycle Counting: A Historical Perspective. In The Rainflow Method in Fatigue; Murakami, Y., Ed.; Butterworth-Heinemann: Oxford, UK, 1992; pp. 3–10. [Google Scholar] [CrossRef]
- Antonopoulos, A.; D’Arco, S.; Hernes, M.; Peftitsis, D. Challenges and Strategies for a Real-Time Implementation of a Rainflow-Counting Algorithm for Fatigue Assessment of Power Modules. In Proceedings of the 2019 IEEE Applied Power Electronics Conference and Exposition (APEC), Anaheim, CA, USA, 17–21 March 2019; pp. 2708–2713. [Google Scholar] [CrossRef]
- Anzai, H. Algorithm Of The Rainflow Method. In The Rainflow Method in Fatigue; Murakami, Y., Ed.; Butterworth-Heinemann: Oxford, UK, 1992; pp. 11–20. [Google Scholar] [CrossRef]
- Rychlik, I. A new definition of the rainflow cycle counting method. Int. J. Fatigue 1987, 9, 119–121. [Google Scholar] [CrossRef]
- Dressler, K.; Hack, M.; Krüger, W. Stochastic Reconstruction of Loading Histories from a Rainflow Matrix. ZAMM J. Appl. Math. Mech./Z. Angew. Math. Mech. 1997, 77, 217–226. [Google Scholar] [CrossRef]
- Johannesson, P. Extrapolation of load histories and spectra. Fatigue Fract. Eng. Mater. Struct. 2006, 29, 209–217. [Google Scholar] [CrossRef]
- Sadowski, N.; Lajoie-Mazenc, M.; Bastos, J.; Ferreira da Luz, M.; Kuo-Peng, P. Evaluation and analysis of iron losses in electrical machines using the rain-flow method. IEEE Trans. Magn. 2000, 36, 1923–1926. [Google Scholar] [CrossRef]
- Visintin, A. On the Preisach model for hysteresis. Nonlinear Anal. Theory Methods Appl. 1984, 8, 977–996. [Google Scholar] [CrossRef]
- Mayergoyz, I.D.; Adly, A.A.; Friedman, G. New Preisach-type models of hysteresis and their experimental testing. J. Appl. Phys. 1990, 67, 5373–5375. [Google Scholar] [CrossRef]
- Brokate, M. Some mathematical properties of the Preisach model for hysteresis. IEEE Trans. Magn. 1989, 25, 2922–2924. [Google Scholar] [CrossRef]
- Bertotti, G. Hysteresis in Magnetism; For Physicists, Materials Scientists, and Engineers; A volume in Electromagnetism, 1st ed.; Academic Press (Imprint of Elsevier): Cambridge, MA, USA, 1998. [Google Scholar] [CrossRef]
- Coulson, M.A.; Slater, R.D.; Simpson, R.R.S. Representation of magnetic characteristic, including hysteresis, using Preisach’s theory. Proc. Inst. Electr. Eng. 1977, 124, 895–898. [Google Scholar] [CrossRef]
- Michelakis, C.; Litsardakis, G.; Samaras, D. A contribution to 2D vector Preisach modelling. J. Magn. Magn. Mater. 1996, 157–158, 347–348. [Google Scholar] [CrossRef]
- Rosu, M.; Jokinen, T.; Demeter, E. Simulation of the Magnetic Hysteresis in NdFeB Permanent Magnet Using Preisach’s Model. In Proceedings of the Optimization of Electrical and Electronic Equipments, Brasow, Romania, 14–15 May 1998; pp. 11–14. [Google Scholar] [CrossRef]
- Chiriac, H.; Lupu, N.; Stoleriu, L.; Postolache, P.; Stancu, A. Experimental and micromagnetic first-order reversal curves analysis in NdFeB-based bulk “exchange spring”-type permanent magnets. J. Magn. Magn. Mater. 2007, 316, 177–180. [Google Scholar] [CrossRef]
- Miyazawa, K.; Okamoto, S.; Yomogita, T.; Kikuchi, N.; Kitakami, O.; Toyoki, K.; Billington, D.; Kotani, Y.; Nakamura, T.; Sasaki, T.; et al. First-order reversal curve analysis of a Nd-Fe-B sintered magnet with soft X-ray magnetic circular dichroism microscopy. Acta Mater. 2019, 162, 1–9. [Google Scholar] [CrossRef]
- Groß, F.; Martínez-García, J.C.; Ilse, S.E.; Schütz, G.; Goering, E.; Rivas, M.; Gräfe, J. gFORC: A graphics processing unit accelerated first-order reversal-curve calculator. J. Appl. Phys. 2019, 126, 163901. [Google Scholar] [CrossRef]
- Ilse, S.E.; Groß, F.; Schütz, G.; Gräfe, J.; Goering, E. Understanding the interaction of soft and hard magnetic components in NdFeB with first-order reversal curves. Phys. Rev. B 2021, 103, 024425. [Google Scholar] [CrossRef]
- Cornejo, D.R.; Missell, F.P. Preisach analysis of reversible magnetization in SmCo/Fe films. J. Appl. Phys. 2000, 87, 4741–4743. [Google Scholar] [CrossRef]
- Rahman, M.; Poloujadoff, M.; Jackson, R.; Perard, J.; Gowda, S. Improved algorithms for digital simulation of hysteresis processes in semi hard magnetic materials. IEEE Trans. Magn. 1981, 17, 3253–3255. [Google Scholar] [CrossRef]
- Barker, J.A.; Schreiber, D.E.; Huth, B.G.; Everett, D.H. Magnetic hysteresis and minor loops: Models and experiments. Proc. R. Scoiety A 1983, 386, 251–261. [Google Scholar] [CrossRef]
- Vajda, F.; Della Torre, E. Efficient numerical implementation of complete-moving-hysteresis models. IEEE Trans. Magn. 1993, 29, 1532–1537. [Google Scholar] [CrossRef]
- Ramirez-Laboreo, E.; Roes, M.G.L.; Sagues, C. Hybrid Dynamical Model for Reluctance Actuators Including Saturation, Hysteresis, and Eddy Currents. IEEE/ASME Trans. Mechatron. 2019, 24, 1396–1406. [Google Scholar] [CrossRef]
- Velázquez, Y.; Guerrero, A.; Martínez, J.; Araujo, E.; Tabasum, M.R.; Nysten, L.P.B.; Encinas, A. Relation of the average interaction field with the coercive and interaction field distributions in First order reversal curve diagrams of nanowire arrays. Nat. Sci. Rep. 2020, 10, 21396. [Google Scholar] [CrossRef]
- Atherton, D.; Szpunar, B.; Szpunar, J. A new approach to Preisach diagrams. IEEE Trans. Magn. 1987, 23, 1856–1865. [Google Scholar] [CrossRef]
- Atherton, D.; Schonbachler, M. Measurements of reversible magnetization component. IEEE Trans. Magn. 1988, 24, 616–620. [Google Scholar] [CrossRef]
- Szpunar, B.; Atherton, D.; Schonbachler, M. An extended Preisach model for hysteresis processes. IEEE Trans. Magn. 1987, 23, 3199–3201. [Google Scholar] [CrossRef]
- Szpunar, J.; Atherton, D.; Szpunar, B. Analysis of the irreversible processes of magnetization in steel. IEEE Trans. Magn. 1987, 23, 300–304. [Google Scholar] [CrossRef]
- Ohteru, S. On Expressions of Magnetic Hysteresis Characteristics. Trans. Am. Inst. Electr. Eng. Part III Power Appar. Syst. 1959, 78, 1809–1815. [Google Scholar] [CrossRef]
- Füzi, J. Analytical approximation of Preisach distribution functions. IEEE Trans. Magn. 2003, 39, 1357–1360. [Google Scholar] [CrossRef]
- Takács, J. A phenomenological mathematical model of hysteresis. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2001, 20, 1002–1015. [Google Scholar] [CrossRef]
- Roberts, A.P.; Pike, C.R.; Verosub, K.L. First-order reversal curve diagrams: A new tool for characterizing the magnetic properties of natural samples. J. Geophys. Res. Solid Earth 2000, 105, 28461–28475. [Google Scholar] [CrossRef]
- Carvallo, C.; Dunlop, D.J.; Özdemir, Ö. Experimental comparison of FORC and remanent Preisach diagrams. Geophys. J. Int. 2005, 162, 747–754. [Google Scholar] [CrossRef]
- Cao, Y.; Xu, K.; Jiang, W.; Droubay, T.; Ramuhalli, P.; Edwards, D.; Johnson, B.R.; McCloy, J. Hysteresis in single and polycrystalline iron thin films: Major and minor loops, first order reversal curves, and Preisach modeling. J. Magn. Magn. Mater. 2015, 395, 361–375. [Google Scholar] [CrossRef]
- Bertotti, G.; Basso, V.; Durin, G. Random free energy model for the description of hysteresis. J. Appl. Phys. 1996, 79, 5764–5766. [Google Scholar] [CrossRef]
- Néel, L. Sur les effets d’un couplage entre grains ferromagnétiques doués d’hystérésis. Comptes Rendus Hebd. Seances Acad. Sci. 1958, 246, 2313–2319, HALid: hal-02878452. [Google Scholar]
- Pescetti, D. Hysteresis Modelling. J. Phys. Colloq. 1988, 49, C8-1923–C8-1924, HAL-ID: jpa-00229133. [Google Scholar] [CrossRef]
- Damjanovic, D. Chapter 4—Hysteresis in Piezoelectric and Ferroelectric Materials. In The Science of Hysteresis; Bertotti, G., Mayergoyz, I.D., Eds.; Academic Press: Oxford, UK, 2006; pp. 337–465. [Google Scholar] [CrossRef]
- Robert, G.; Damjanovic, D.; Setter, N.; Turik, A.V. Preisach modeling of piezoelectric nonlinearity in ferroelectric ceramics. J. Appl. Phys. 2001, 89, 5067–5074. [Google Scholar] [CrossRef]
- Biorci, G.; Pescetti, D. Analytical theory of the behaviour of ferromagnetic materials. Il Nuovo C. 1958, 7, 829–842. [Google Scholar] [CrossRef]
- Wiesen, K.; Charap, S.H. Vector Preisach modeling. J. Appl. Phys. 1987, 61, 4019–4021. [Google Scholar] [CrossRef]
- Zeinali, R.; Krop, D.C.J.; Lomonova, E.; Ertan, H.B. Improved Preisach Model for Modelling Magnetic Hysteresis Effect in Non-Oriented Steels. In Proceedings of the 2018 XIII International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 1031–1036. [Google Scholar] [CrossRef]
- Naidu, S. Simulation of the hysteresis phenomenon using Preisach’s theory. IEE Proc. (Phys. Sci. Meas. Instrumentation, Manag. Educ.) 1990, 137, 73–79. [Google Scholar] [CrossRef]
- Naidu, S.R.; Filho, H.S.R.; Fernandes, A.B. A software package for the simulation of magnetic hysteresis. In Proceedings of the PESC Record. 27th Annual IEEE Power Electronics Specialists Conference, Baveno, Italy, 23–27 June 1996; Volume 2, pp. 1441–1446. [Google Scholar] [CrossRef]
- Hui, S.; Zhu, J. Magnetic hysteresis modeling and simulation using the Preisach theory and TLM technique. In Proceedings of the 1994 Power Electronics Specialist Conference—PESC’94, Taipei, Taiwan, 20–25 June 1994; Volume 2, pp. 837–842. [Google Scholar] [CrossRef]
- Dunlop, D.J.; Westcott-Lewis, M.F.; Bailey, M.E. Preisach diagrams and anhysteresis: Do they measure interactions? Phys. Earth Planet. Inter. 1990, 65, 62–77. [Google Scholar] [CrossRef]
- Pasquale, M.; Basso, V.; Bertotti, G.; Jiles, D.C.; Bi, Y. Domain-wall motion in random potential and hysteresis modeling. J. Appl. Phys. 1998, 83, 6497–6499. [Google Scholar] [CrossRef]
- Pasquale, M.; Bertotti, G.; Jiles, D.C.; Bi, Y. Application of the Preisach and Jiles–Atherton models to the simulation of hysteresis in soft magnetic alloys. J. Appl. Phys. 1999, 85, 4373–4375. [Google Scholar] [CrossRef]
- Stancu, A.; Stoleriu, L.; Postolache, P.; Tanasa, R. New Preisach model for structured particulate ferromagnetic media. J. Magn. Magn. Mater. 2005, 290–291, 490–493. [Google Scholar] [CrossRef]
- Basso, V.; Bertotti, G. Hysteresis models for the description of domain wall motion. IEEE Trans. Magn. 1996, 32, 4210–4212. [Google Scholar] [CrossRef]
- Basso, V. Hysteresis models for magnetization by domain wall motion. IEEE Trans. Magn. 1998, 34, 2207–2212. [Google Scholar] [CrossRef]
- Pike, C.R.; Roberts, A.P.; Verosub, K.L. Characterizing interactions in fine magnetic particle systems using first order reversal curves. J. Appl. Phys. 1999, 85, 6660–6667. [Google Scholar] [CrossRef]
- Dupré, L.R.; Van Keer, R.; Melkebeek, J.A.A. Identification of the relation between the material parameters in the Preisach model and in the Jiles–Atherton hysteresis model. J. Appl. Phys. 1999, 85, 4376–4378. [Google Scholar] [CrossRef]
- Hussain, S.; Lowther, D.A. Establishing a Relation between Preisach and Jiles–Atherton Models. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Li, Y.; Zhu, J.; Li, Y.; Zhu, L. A hybrid Jiles–Atherton and Preisach model of dynamic magnetic hysteresis based on backpropagation neural networks. J. Magn. Magn. Mater. 2022, 544, 168655. [Google Scholar] [CrossRef]
- Jiles, D.C.; Atherton, D.L. Theory of ferromagnetic hysteresis (invited). J. Appl. Phys. 1984, 55, 2115–2120. [Google Scholar] [CrossRef]
- Jiles, D.; Atherton, D. Theory of ferromagnetic hysteresis. J. Magn. Magn. Mater. 1986, 61, 48–60. [Google Scholar] [CrossRef]
- Monnor, T.; Yimnirun, R.; Kanchiang, K.; Laosiritaworn, Y. Hysteresis Scaling by Defined Hysteron Pattern in Preisach Model. In Proceedings of the World Congress on Engineering and Computer Science 2012 Vol. II, WCECS 2012, San Francisco, CA, USA, 24–26 October 2012; Volume 2, pp. 1283–1286. [Google Scholar]
- Sari, Z.; Ivanyi, A. Statistical approach of hysteresis. Phys. Condens. Matter 2006, 372, 45–48. [Google Scholar] [CrossRef]
- Bertotti, G.; Fiorillo, F.; Soardo, G. Dependence of power losses on peak magnetization and magnetization frequency in grain-oriented and non-oriented 3% SiFe. IEEE Trans. Magn. 1987, 23, 3520–3522. [Google Scholar] [CrossRef]
- Henze, O.; Rucker, W.M. Identification procedures of Preisach model. IEEE Trans. Magn. 2002, 38, 833–836. [Google Scholar] [CrossRef]
- Azzerboni, B.; Cardelli, E.; Della Torre, E.; Finocchio, G. Reversible magnetization and Lorentzian function approximation. J. Appl. Phys. 2003, 93, 6635–6637. [Google Scholar] [CrossRef]
- Vajda, F.; Della Torre, E. Measurements of output-dependent Preisach functions. IEEE Trans. Magn. 1991, 27, 4757–4762. [Google Scholar] [CrossRef]
- De Wulf, M.; Vandevelde, L.; Maes, J.; Dupre, L.; Melkebeek, J. Computation of the Preisach distribution function based on a measured Everett map. IEEE Trans. Magn. 2000, 36, 3141–3143. [Google Scholar] [CrossRef]
- Consolo, G.; Finocchio, G.; Carpentieri, M.; Cardelli, E.; Azzerboni, B. About identification of Scalar Preisach functions of soft magnetic materials. IEEE Trans. Magn. 2006, 42, 923–926. [Google Scholar] [CrossRef]
- Pruksanubal, P.; Binner, A.; Gonschorek, K.H. Determination of distribution functions and parameters for the Preisach hysteresis model. In Proceedings of the 2006 17th International Zurich Symposium on Electromagnetic Compatibility, Singapore, 28 February–3 March 2006; pp. 258–261. [Google Scholar] [CrossRef]
- Basso, V.; Berlotti, G.; Infortuna, A.; Pasquale, M. Preisach model study of the connection between magnetic and microstructural properties of soft magnetic materials. IEEE Trans. Magn. 1995, 31, 4000–4005. [Google Scholar] [CrossRef]
- Sutor, A.; Rupitsch, S.; Lerch, R. A Preisach-based hysteresis model for magnetic and ferroelectric hysteresis. Appl. Phys. A 2010, 100, 425–430. [Google Scholar] [CrossRef]
- Eichler, J.; Novak, M.; Kosek, M. Experimental Determination of the Preisach Model for Grain Oriented Steel. Acta Phys. Pol. A 2019, 136, 713–719. [Google Scholar] [CrossRef]
- Biorci, G.; Pescetti, D. Some consequences of the analytical theory of the ferromagnetic hysteresis. J. Phys. Radium 1959, 20, 233–236. [Google Scholar] [CrossRef]
- Biorci, G.; Ferro, A. Hysteresis losses along open transformations. J. Phys. Radium 1959, 20, 237–240, ID: jpa-00236024, v1. [Google Scholar] [CrossRef]
- Everett, D.H.; Smith, F.W. A general approach to hysteresis. Part 2: Development of the domain theory. Trans. Faraday Soc. 1954, 50, 187–197. [Google Scholar] [CrossRef]
- Everett, D.H. A general approach to hysteresis. Part 4. An alternative formulation of the domain model. Trans. Faraday Soc. 1955, 51, 1551–1557. [Google Scholar] [CrossRef]
- Mayergoyz, I.D. Mathematical models of hysteresis. IEEE Trans. Magn. 1986, 22, 603–608. [Google Scholar] [CrossRef]
- Everett, D.H. A general approach to hysteresis. Part 3.-A formal treatment of the independent domain model of hysteresis. Trans. Faraday Soc. 1954, 50, 1077–1096. [Google Scholar] [CrossRef]
- Pescetti, D. Some remarks on Preisach modeling. J. Appl. Phys. 1991, 69, 4605. [Google Scholar] [CrossRef]
- Everett, D.H.; Whitton, W.I.A. A general apporach to hysteresis. Trans. Faraday Soc. 1952, 48, 749–757. [Google Scholar] [CrossRef]
- Del Vecchio, R. An efficient procedure for modeling complex hysteresis processes in ferromagnetic materials. IEEE Trans. Magn. 1980, 16, 809–811. [Google Scholar] [CrossRef]
- Park, G.-S.; Hahn, S.-Y.; Lee, K.-S.; Jung, H.-K. Implementation of hysteresis characteristics using the Preisach model with M-B variables. IEEE Trans. Magn. 1993, 29, 1542–1545. [Google Scholar] [CrossRef]
- Stenzel, J.; Weber, T. Model representing the nonlinear behaviour of three-phase transformers. Arch. Elektrotechnik 1994, 77, 143–150. [Google Scholar] [CrossRef]
- Yamaguchi, T.; Ueda, F.; Yamamoto, E. Simulation of Hysteresis Characteristics of Core Materials Using the Everett Function. IEEE Transl. J. Magn. Jpn. 1989, 4, 353–359. [Google Scholar] [CrossRef]
- Enderby, J.A. The domain model of hysteresis. Part 1.-Independent domains. Trans. Faraday Soc. 1955, 51, 835–848. [Google Scholar] [CrossRef]
- Enderby, J.A. The domain model of hysteresis. Part 2.-Interacting domains. Trans. Faraday Soc. 1956, 52, 106–120. [Google Scholar] [CrossRef]
- Sjöström, M. Differentiation and power loss computation of classical Preisach model. Phys. B Condens. Matter 2004, 343, 96–100. [Google Scholar] [CrossRef]
- Kádár, G. On the product Preisach model of hysteresis. Phys. B Condens. Matter 2000, 275, 40–44. [Google Scholar] [CrossRef]
- Stoleriu, L.; Stancu, A. Using Experimental FORC Distribution as Input for a Preisach-Type Model. IEEE Trans. Magn. 2006, 42, 3159–3161. [Google Scholar] [CrossRef]
- Finocchio, G.; Carpentieri, M.; Cardelli, E.; Azzerboni, B. Analytical solution of Everett integral using Lorentzian Preisach function approximation. J. Magn. Magn. Mater. 2006, 300, 451–470. [Google Scholar] [CrossRef]
- Włodarski, Z. Modeling hysteresis by analytical reversal curves. Phys. B Condens. Matter 2007, 398, 159–163. [Google Scholar] [CrossRef]
- Renhart, W.; Bíró, O.; Magele, C.; Preis, K.; Rabel, A. Inrush current computations using an optimised analytical hysteresis model. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2017, 36, 1568–1576. [Google Scholar] [CrossRef]
- Iyer, R.; Shirley, M. Hysteresis parameter identification with limited experimental data. IEEE Trans. Magn. 2004, 40, 3227–3239. [Google Scholar] [CrossRef]
- Mayergoyz, I.D. Hysteresis models from the mathematical and control theory points of view. J. Appl. Phys. 1985, 57, 3803–3805. [Google Scholar] [CrossRef]
- Daniel, E.D.; Levine, I. Experimental and Theoretical Investigation of the Magnetic Properties of Iron Oxide Recording Tape. J. Acoust. Soc. Am. 1960, 32, 1–15. [Google Scholar] [CrossRef]
- Woodward, J.G.; Torre, E.D. Particle Interaction in Magnetic Recording Tapes. J. Appl. Phys. 1961, 32, 126–127. [Google Scholar] [CrossRef]
- Bate, G. Statistical Stability of the Preisach Diagram for Particles of γ-Fe2O3. J. Appl. Phys. 1962, 33, 2263–2269. [Google Scholar] [CrossRef]
- Hejda, P.; Zelinka, T. Modelling of hysteresis processes in magnetic rock samples using the Preisach diagram. Phys. Earth Planet. Inter. 1990, 63, 32–40. [Google Scholar] [CrossRef]
- Pike, C.R. First-order reversal-curve diagrams and reversible magnetization. Phys. Rev. B 2003, 68, 104424. [Google Scholar] [CrossRef]
- Zhao, X.; Roberts, A.P.; Heslop, D.; Paterson, G.A.; Li, Y.; Li, J. Magnetic domain state diagnosis using hysteresis reversal curves. J. Geophys. Res. Solid Earth 2017, 122, 4767–4789. [Google Scholar] [CrossRef]
- Pohlit, M.; Eibisch, P.; Akbari, M.; Porrati, F.; Huth, M.; Müller, J. First order reversal curves (FORC) analysis of individual magnetic nanostructures using micro-Hall magnetometry. Rev. Sci. Instruments 2016, 87, 113907. [Google Scholar] [CrossRef] [PubMed]
- Bernard, Y.; Mendes, E.; Ren, Z. Determination of the distribution function of Preisach’s model using centred cycles. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2000, 19, 997–1006. [Google Scholar] [CrossRef]
- Szabó, Z.; Tugyi, I.; Kádár, G.; Füzi, J. Identification procedures for scalar Preisach model. Phys. Condens. Matter 2004, 343, 142–147. [Google Scholar] [CrossRef]
- Dlala, E.; Saitz, J.; Arkkio, A. Hysteresis modeling based on symmetric minor loops. IEEE Trans. Magn. 2005, 41, 2343–2348. [Google Scholar] [CrossRef]
- Koh, C.S.; Eum, Y.H.; ki Hong, S.; seok Shin, P. Comparison of the Identification Methods of Preisach Model For a Grain-Oriented Electrical Steel Sheet. In Proceedings of the 2006 12th Biennial IEEE Conference on Electromagnetic Field Computation, Miami, FL, USA, 30 April–3 May 2006; p. 99. [Google Scholar] [CrossRef]
- Zeinali, R.; Krop, D.; Lomonova, E. Weight-Function Identification for the Preisach Model of Laminated Steels Using Concentric Hysteresis Loops. In ELECTRIMACS 2019: Selected Papers-Volume 2; Springer International Publishing: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Lin, D.; Zhou, P.; Lu, C.; Chen, N.; Rosu, M. Construction of magnetic hysteresis loops and its applications in parameter identification for hysteresis models. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 1050–1055. [Google Scholar] [CrossRef]
- Mayergoyz, I.D. The classical Preisach model of hysteresis and reversibility. J. Appl. Phys. 1991, 69, 4602. [Google Scholar] [CrossRef]
- Parker, S.; Faunce, C.; Grundy, P.; Maylin, M.; Ludlow, J.; Lane, R. Preisach modelling of magnetisation changes in steel. J. Magn. Magn. Mater. 1995, 145, 51–56. [Google Scholar] [CrossRef]
- Zirka, S.E.; Moroz, Y.I.; Marketos, P.; Moses, A.J. Congruency-based hysteresis models for transient simulation. IEEE Trans. Magn. 2004, 40, 390–399. [Google Scholar] [CrossRef]
- Stancu, A.; Pike, C.; Stoleriu, L.; Postolache, P.; Cimpoesu, D. Micromagnetic and Preisach analysis of the First Order Reversal Curves (FORC) diagram. J. Appl. Phys. 2003, 93, 6620–6622. [Google Scholar] [CrossRef]
- Newell, A.J. A high-precision model of first-order reversal curve (FORC) functions for single-domain ferromagnets with uniaxial anisotropy. Geochem. Geophys. Geosyst. 2005, 6. [Google Scholar] [CrossRef]
- Church, N.S.; Fabian, K.; McEnroe, S.A. Nonlinear Preisach maps: Detecting and characterizing separate remanent magnetic fractions in complex natural samples. J. Geophys. Res. Solid Earth 2016, 121, 8373–8395. [Google Scholar] [CrossRef]
- Durst, K.D.; Kronmüller, H. The coercive field of sintered and melt-spun NdFeB magnets. J. Magn. Magn. Mater. 1987, 68, 63–75. [Google Scholar] [CrossRef]
- Tang, X.; Chen, R.; Wang, Z.; Jin, C.; Li, M.; Yin, W.; Lee, D.; Yan, A. The Understanding of Reversed Domain Nucleation and Pinning Mechanism in Hot Deformed Nd2Fe14B Magnets. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Takahashi, N.; Miyabara, S.H.; Fujiwara, K. Problems in practical finite element analysis using Preisach hysteresis model. IEEE Trans. Magn. 1999, 35, 1243–1246. [Google Scholar] [CrossRef]
- Dlala, E.; Saitz, J.; Arkkio, A. Inverted and Forward Preisach Models for Numerical Analysis of Electromagnetic Field Problems. IEEE Trans. Magn. 2006, 42, 1963–1973. [Google Scholar] [CrossRef]
- Bernard, Y.; Mendes, E.; Santandrea, L.; Bouillault, F. Inverse Preisach model in finite elements modelling. Eur. Phys. J. AP 2000, 12, 117–121. [Google Scholar] [CrossRef]
- Al-Janaideh, M.; Visone, C.; Davino, D.; Krejčí, P. The generalized Prandtl-Ishlinskii model: Relation with the preisach nonlinearity and inverse compensation error. In Proceedings of the 2014 American Control Conference, Portland, OR, USA, 4–6 June 2014; pp. 4759–4764. [Google Scholar] [CrossRef]
- Al-Janaideh, M.; Davino, D.; Krejčí, P.; Visone, C. Comparison of Prandtl–Ishlinskii and Preisach modeling for smart devices applications. Phys. Condens. Matter 2016, 486, 155–159. [Google Scholar] [CrossRef]
- Iyer, R.; Tan, X.; Krishnaprasad, P. Approximate inversion of the Preisach hysteresis operator with application to control of smart actuators. IEEE Trans. Autom. Control 2005, 50, 798–810. [Google Scholar] [CrossRef]
- Della Torre, E. Energy considerations in hysteresis models. In Proceedings of the 1992 IEEE International Magnetics Conference (INTERMAG), St. Louis, MO, USA, 13–16 April 1992; p. 209. [Google Scholar] [CrossRef]
- Dupré, L.; Keer, R.V.; Melkebeek, J. Modelling and identification of iron losses in nonoriented steel laminations using Preisach theory. IEE Proc. Electr. Power Appl. 1997, 144, 227–234. [Google Scholar] [CrossRef]
- Dupre, L.; Van Keer, R.; Melkebeek, J. An iron loss model for electrical machines using the Preisach theory. IEEE Trans. Magn. 1997, 33, 4158–4160. [Google Scholar] [CrossRef]
- Bottauscio, O.; Chiampi, M.; Chiarabaglio, D. Iron losses in soft magnetic materials under periodic non-sinusoidal supply conditions. Phys. Condens. Matter 2000, 275, 191–196. [Google Scholar] [CrossRef]
- Zhao, X.; Yang, L.; Xu, H.; Huang, K.; Liu, L.; Du, Z. Dynamic Hysteresis and Loss Modeling of Grain-oriented Silicon Steel under High-frequency Sinusoidal Excitation. IEEE Trans. Magn. 2022, 58, 7300805. [Google Scholar] [CrossRef]
- Chubb, L.W.; Spooner, T. The effect of displaced magnetic pulsations on the hysteresis loss of sheet steel. Proc. Am. Inst. Electr. Eng. 1915, 34, 2321–2342. [Google Scholar] [CrossRef]
- Chubb, L.W.; Spooner, T. The Effect of Displaced Magnetic Pulsations on the Hysteresis Loss of Sheet Steel. Trans. Am. Inst. Electr. Eng. 1915, 34, 2671–2692. [Google Scholar] [CrossRef]
- Ball, J.D. The unsymmetrical hysteresis loop. Proc. Am. Inst. Electr. Eng. 1915, 34, 2275–2297. [Google Scholar] [CrossRef]
- Sidhu, S.S. Formulas for magnetic hysteresis losses. Electr. Eng. 1933, 52, 625–630. [Google Scholar] [CrossRef]
- Simao, C.; Sadowski, N.; Batistela, N.J.; Bastos, J.P.A. Evaluation of Hysteresis Losses in Iron Sheets Under DC-biased Inductions. IEEE Trans. Magn. 2009, 45, 1158–1161. [Google Scholar] [CrossRef]
- Lancarotte, M.S.; Goldemberg, C.; Penteado, A.D.A. Estimation of FeSi Core Losses Under PWM or DC Bias Ripple Voltage Excitations. IEEE Trans. Energy Convers. 2005, 20, 367–372. [Google Scholar] [CrossRef]
- Zhu, S.; Cheng, M.; Dong, J.; Du, J. Core Loss Analysis and Calculation of Stator Permanent-Magnet Machine Considering DC-Biased Magnetic Induction. IEEE Trans. Ind. Electron. 2014, 61, 5203–5212. [Google Scholar] [CrossRef]
- Xue, S.; Feng, J.; Guo, S.; Chen, Z.; Peng, J.; Chu, W.Q.; Huang, L.R.; Zhu, Z.Q. Iron Loss Model Under DC Bias Flux Density Considering Temperature Influence. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Xue, S.; Chu, W.; Feng, J.; Guo, S.; Chen, Z.; Peng, J. Evaluation of Iron Loss Models in Electrical Machines. IEEE Trans. Ind. Appl. 2019, 55, 1461–1472. [Google Scholar] [CrossRef]
- Rens, J.; Vandenbossche, L.; Dorez, O. Iron Loss Modelling of Electrical Traction Motors for Improved Prediction of Higher Harmonic Losses. World Electr. Veh. J. 2020, 11, 24. [Google Scholar] [CrossRef]
- Ewart, D.N. Digital Computer Simulation Model of a Steel-Core Transformer. IEEE Trans. Power Deliv. 1986, 1, 174–183. [Google Scholar] [CrossRef]
- Dick, E.; Watson, W. Transformer Models for Transient Studies Based on Field Measurements. IEEE Trans. Power Appar. Syst. 1981, PAS-100, 409–419. [Google Scholar] [CrossRef]
- Curland, N.; Speliotis, D. An iterative hysteretic model for digital magnetic recording. IEEE Trans. Magn. 1971, 7, 538–543. [Google Scholar] [CrossRef]
- Zhu, X.; Quan, L.; Chen, D.; Cheng, M.; Hua, W.; Sun, X. Electromagnetic Performance Analysis of a New Stator-Permanent-Magnet Doubly Salient Flux Memory Motor Using a Piecewise-Linear Hysteresis Model. IEEE Trans. Magn. 2011, 47, 1106–1109. [Google Scholar] [CrossRef]
- Parodi, M.; Storace, M.; Cincotti, S. Static and dynamic hysteretic features in a PWL circuit. Int. J. Circuit Theory Appl. 1996, 24, 183–199. [Google Scholar] [CrossRef]
- Cannas, B.; Cincotti, S. Higher Order Reversal Hysteresis Curves Approximation by a Piecewise Linear Circuit Model of Hysteresis. IEEE Trans. Magn. 2003, 39, 1349–1352. [Google Scholar] [CrossRef]
- Cannas, B.; Cincotti, S.; Daneri, I. A generalization of a piece-wise linear circuit model of hysteresis. IEEE Trans. Magn. 2002, 38, 901–904. [Google Scholar] [CrossRef]
- Cincotti, S.; Daneri, I. A non-linear circuit model of hysteresis. IEEE Trans. Magn. 1999, 35, 1247–1250. [Google Scholar] [CrossRef]
- Matsuo, T.; Shimasaki, M. Representation Theorems for stop and play models with input-dependent shape functions. IEEE Trans. Magn. 2005, 41, 1548–1551. [Google Scholar] [CrossRef]
- Iyer, R.V.; Tan, X. Control of hysteretic systems through inverse compensation. IEEE Control Syst. Mag. 2009, 29, 83–99. [Google Scholar] [CrossRef]
- Webb, G.V.; Lagoudas, D.C.; Kurdila, A.J. Hysteresis Modeling of SMA Actuators for Control Applications. J. Intell. Mater. Syst. Struct. 1998, 9, 432–448. [Google Scholar] [CrossRef]
- Banks, H.T.; Kurdila, A.J.; Webb, G. Identification of hysteretic control influence operators representing smart actuators part I: Formulation. Math. Probl. Eng. 1997, 3, 723495. [Google Scholar] [CrossRef]
- Tan, X.; Bennani, O. Fast inverse compensation of Preisach-type hysteresis operators using field-programmable gate arrays. In Proceedings of the 2008 American Control Conference, Seattle, WA, USA, 11–13 June 2008; pp. 2365–2370. [Google Scholar] [CrossRef]
- Riccardi, L.; Naso, D.; Janocha, H.; Turchiano, B. A precise positioning actuator based on feedback-controlled magnetic shape memory alloys. Mechatronics 2012, 22, 568–576. [Google Scholar] [CrossRef]
- Zhou, M.; He, S.; Hu, B.; Zhang, Q. Modified KP Model for Hysteresis of Magnetic Shape Memory Alloy Actuator. IETE Tech. Rev. 2015, 32, 29–36. [Google Scholar] [CrossRef]
- Hay, J.L.; Chaplin, R.I. Dynamic simulation of ferromagnetic hysteretic behaviour by digital computer. Simulation 1975, 25, 185–191. [Google Scholar] [CrossRef]
- Serpico, C.; d’Aquino, M.; Visone, C.; Davino, D. A new class of Preisach-type isotropic vector model of hysteresis. Phys. B Condens. Matter 2004, 343, 117–120. [Google Scholar] [CrossRef]
- Curland, N.; Speliotis, D. A theoretical study of an isolated transition using an iterative hysteretic model. IEEE Trans. Magn. 1970, 6, 640–646. [Google Scholar] [CrossRef]
- Janssens, N. Mathematical modelling of Magnetic Hysteresis. In Proceedings of the First Compumag Conference, Oxford, UK, 31 March–2 April 1976; pp. 190–195. Available online: https://www.compumag.org/wp/conferences/proceedings/ (accessed on 20 January 2023).





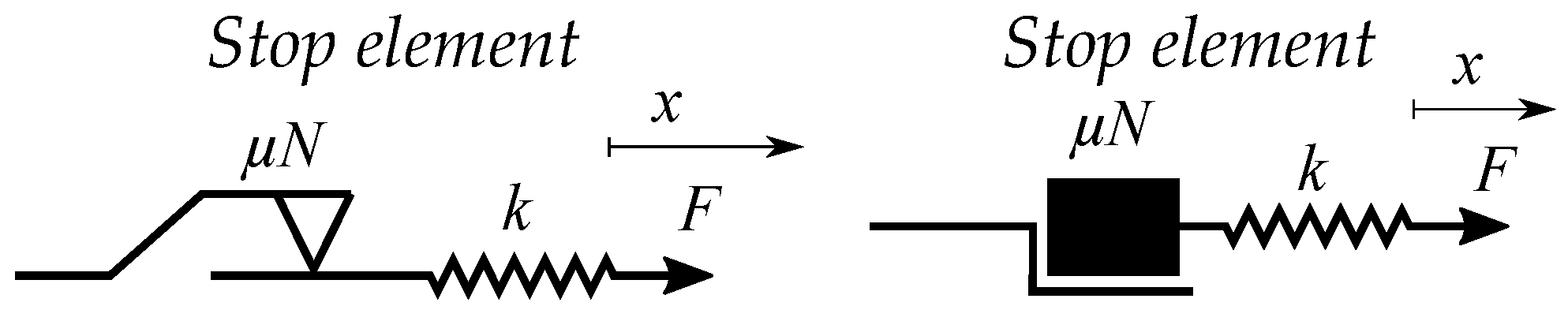
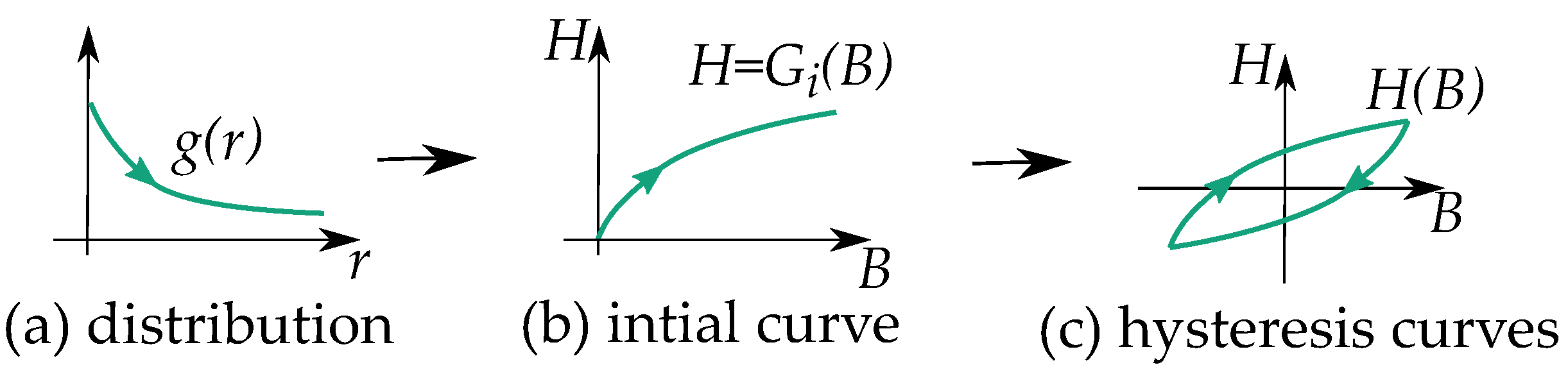
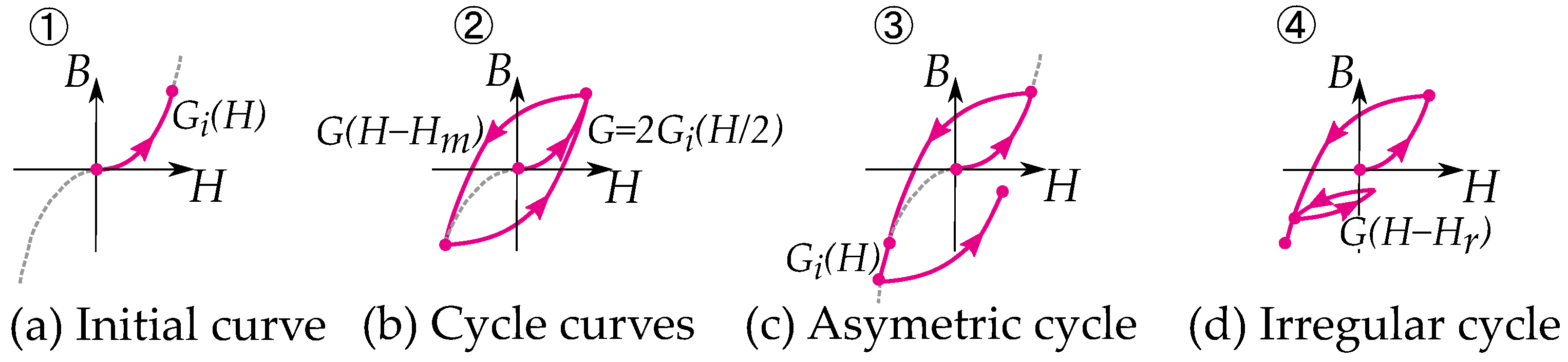

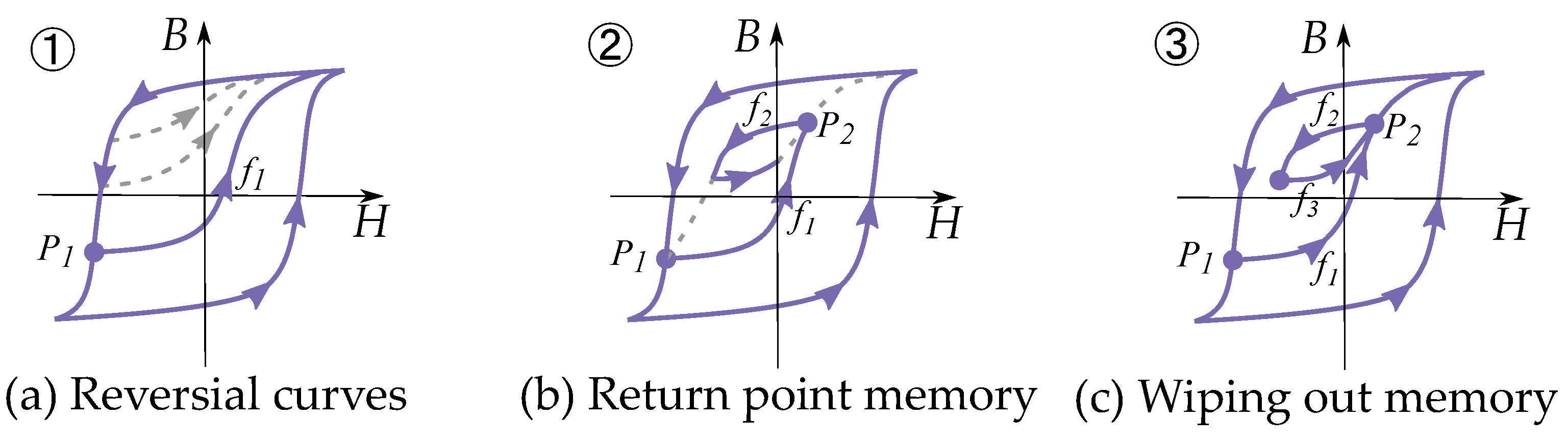

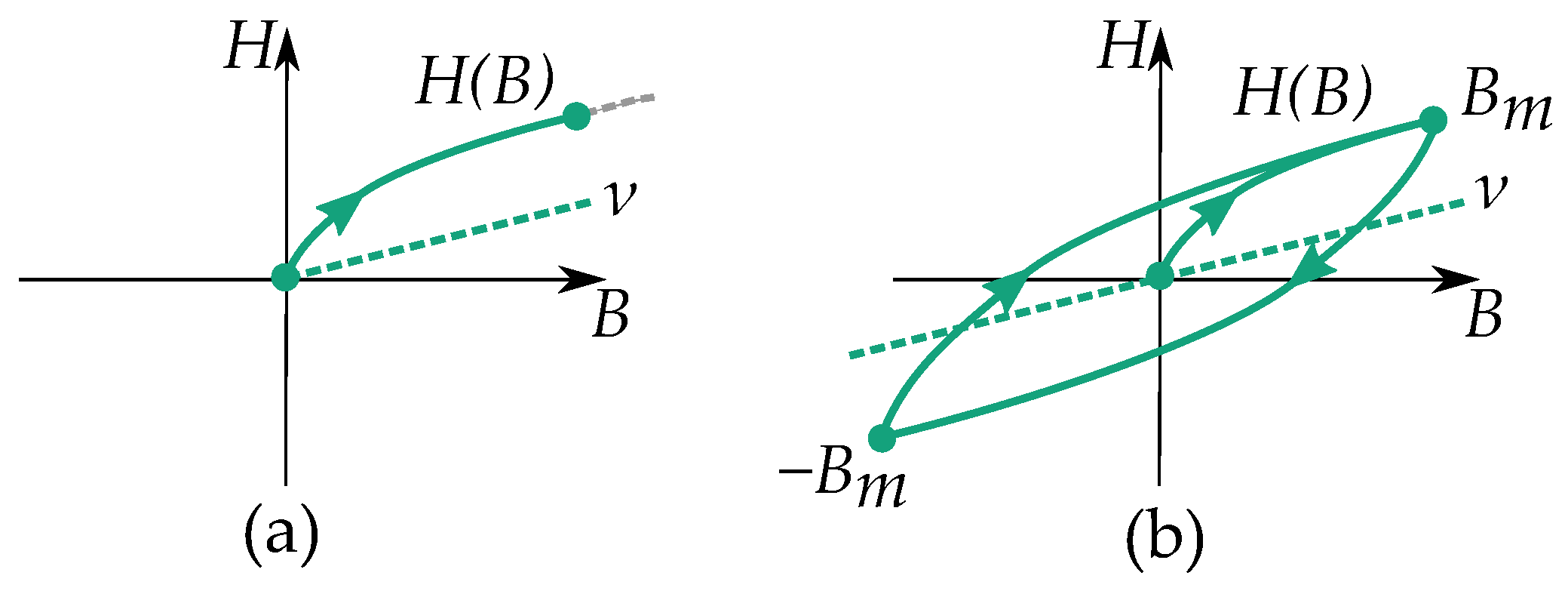
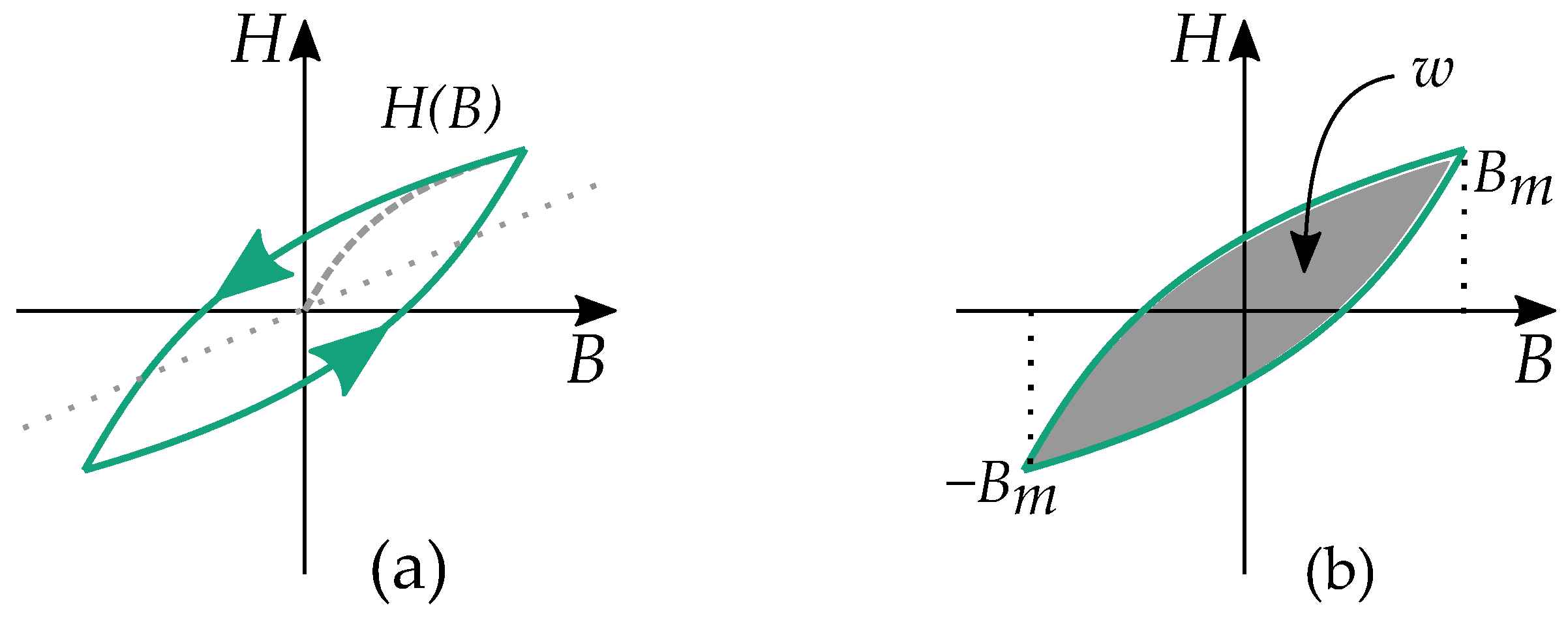


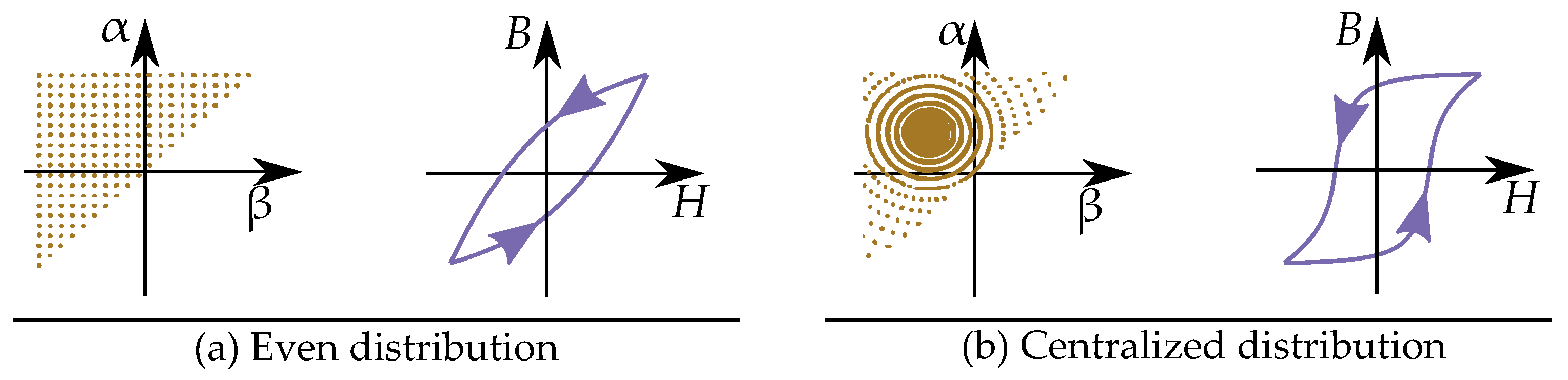

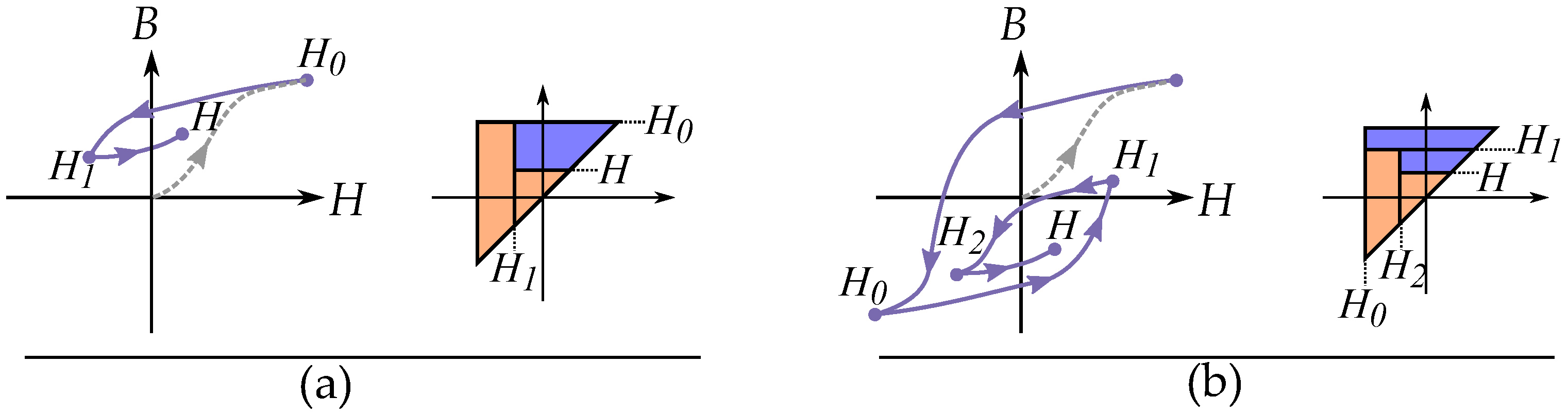
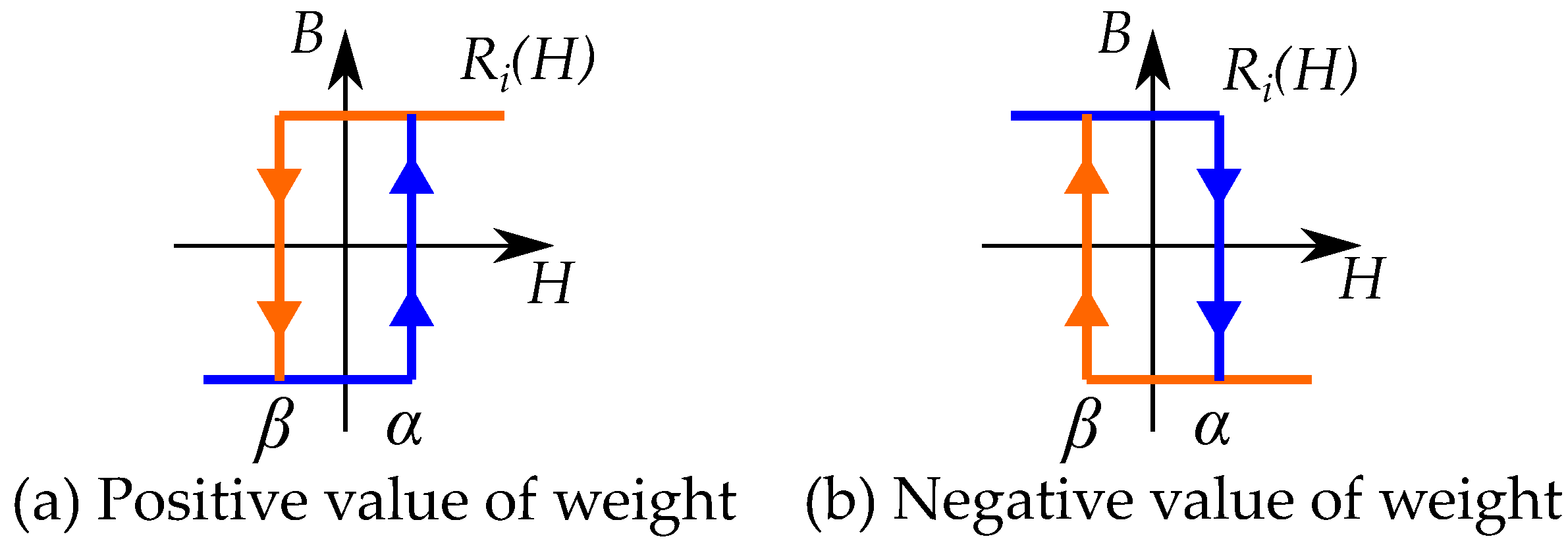

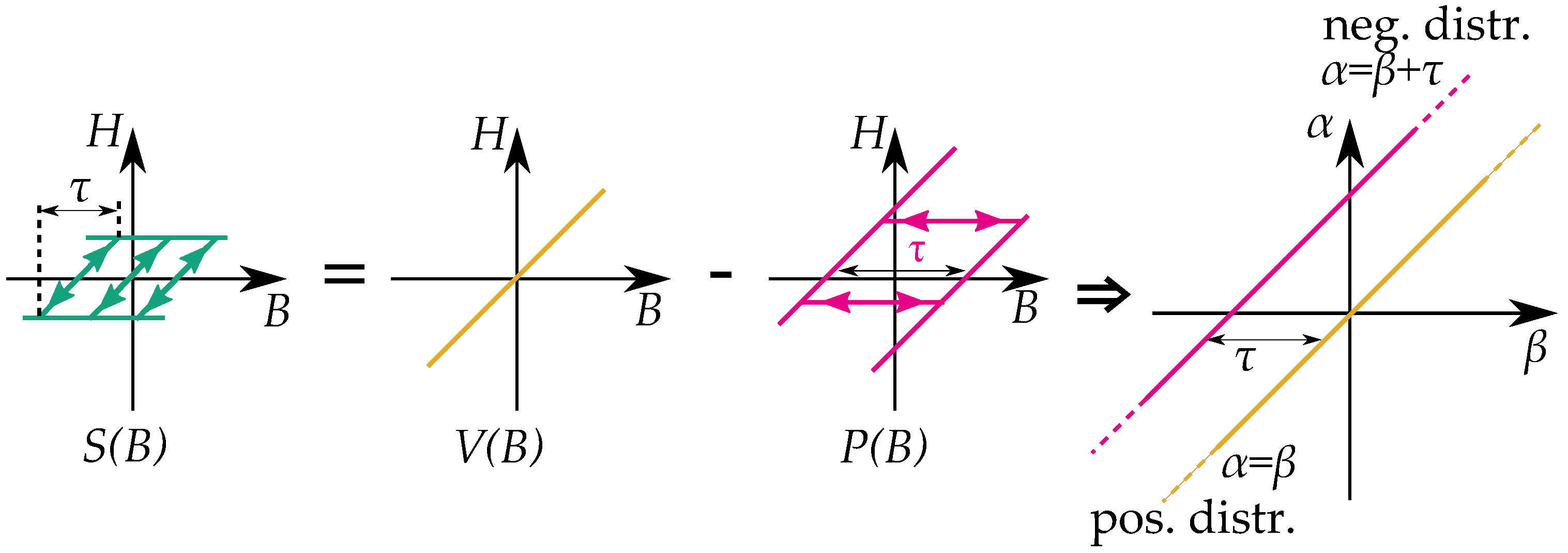
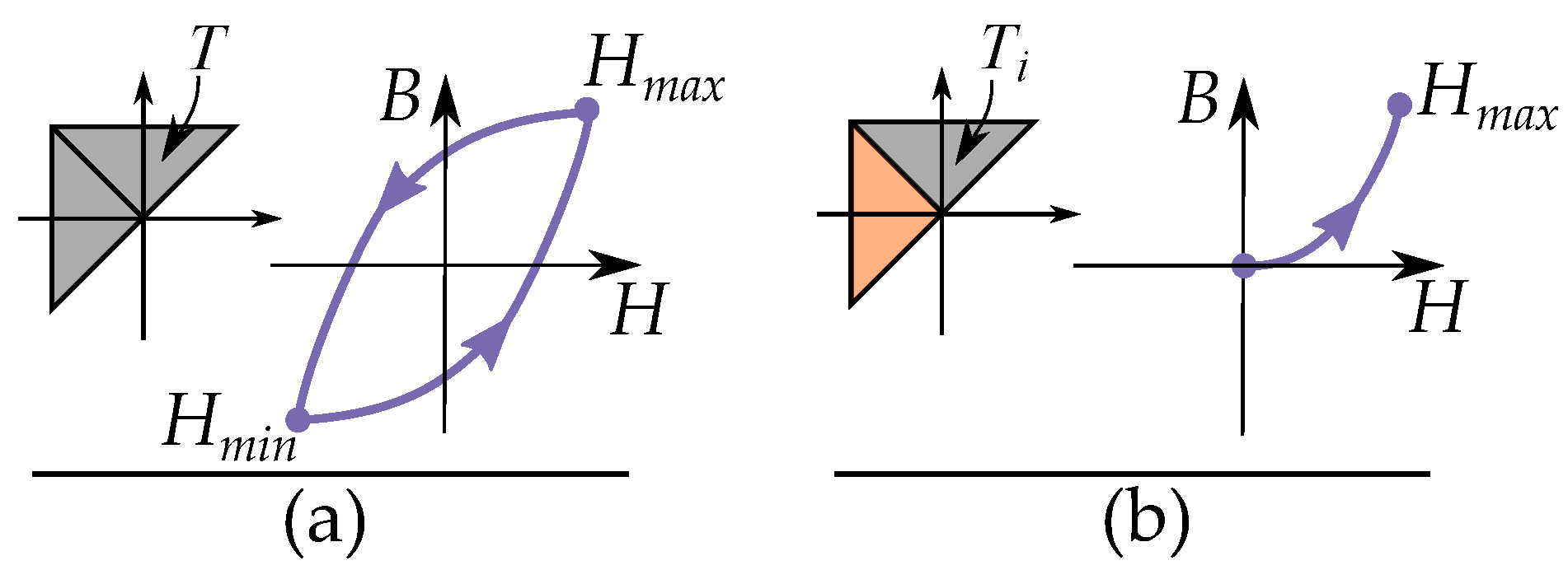
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mörée, G.; Leijon, M. Review of Play and Preisach Models for Hysteresis in Magnetic Materials. Materials 2023, 16, 2422. https://doi.org/10.3390/ma16062422
Mörée G, Leijon M. Review of Play and Preisach Models for Hysteresis in Magnetic Materials. Materials. 2023; 16(6):2422. https://doi.org/10.3390/ma16062422
Chicago/Turabian StyleMörée, Gustav, and Mats Leijon. 2023. "Review of Play and Preisach Models for Hysteresis in Magnetic Materials" Materials 16, no. 6: 2422. https://doi.org/10.3390/ma16062422
APA StyleMörée, G., & Leijon, M. (2023). Review of Play and Preisach Models for Hysteresis in Magnetic Materials. Materials, 16(6), 2422. https://doi.org/10.3390/ma16062422






