Theory, Method and Practice of Metal Deformation Instability: A Review
Abstract
1. Introduction
2. Definition of Deformation Instability
- (1)
- Hart’s instability criterion [60]
- (2)
- Jona’s instability criterion [61]
- (3)
- Semiatin’s instability criterion [62]
- (4)
- Dynamic Material Model (DMM) criterion [63]
- (5)
- Gegel’s and Alexander’s instability criterion [68]
- (6)
- Metallurgical instability criterion [69]
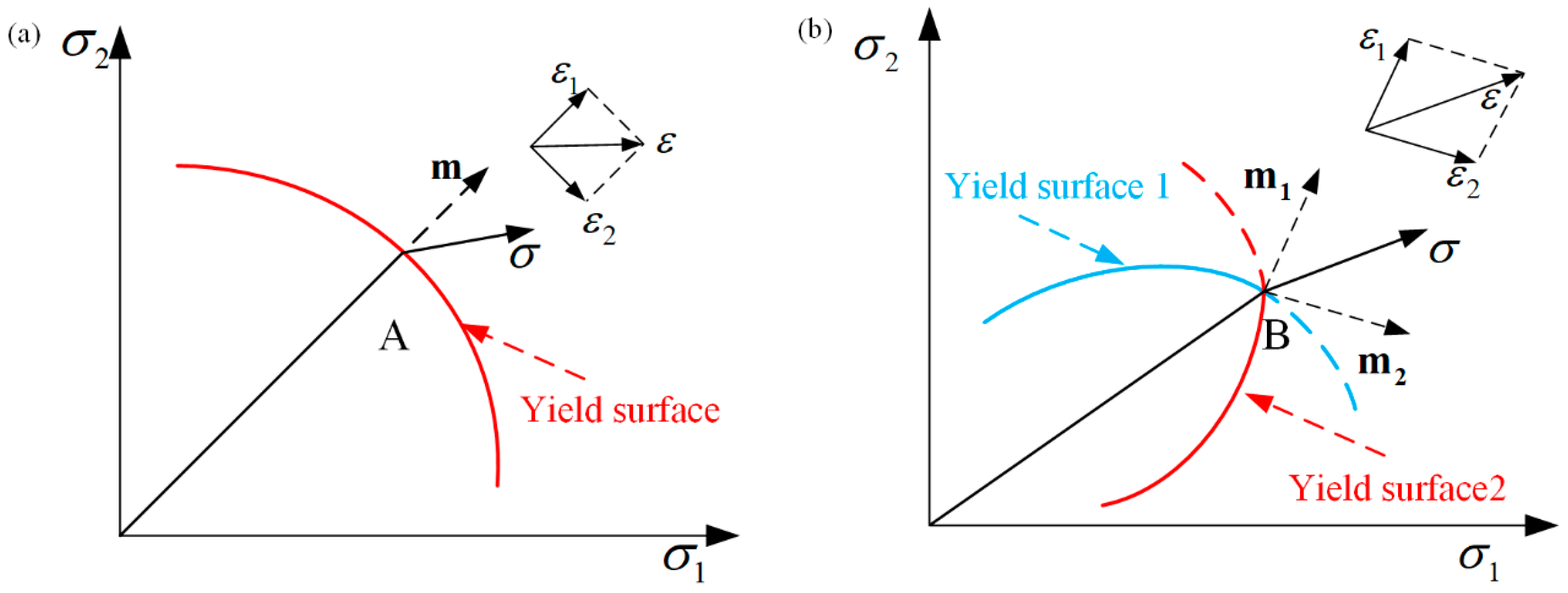
3. Deformation Instability Induced by Characteristics of Material
3.1. Deformation Instability in Superplastics of Materials
- (1)
- Load instability criterion are as follows:
- (2)
- The geometric instability criterion is as follows:
3.2. Deformation Instability in Hot Forming Process
4. Deformation Instability Induced by the Structural Geometry of Materials
4.1. Deformation Instability of Sheet Metal
4.2. Deformation Instability of Tubes
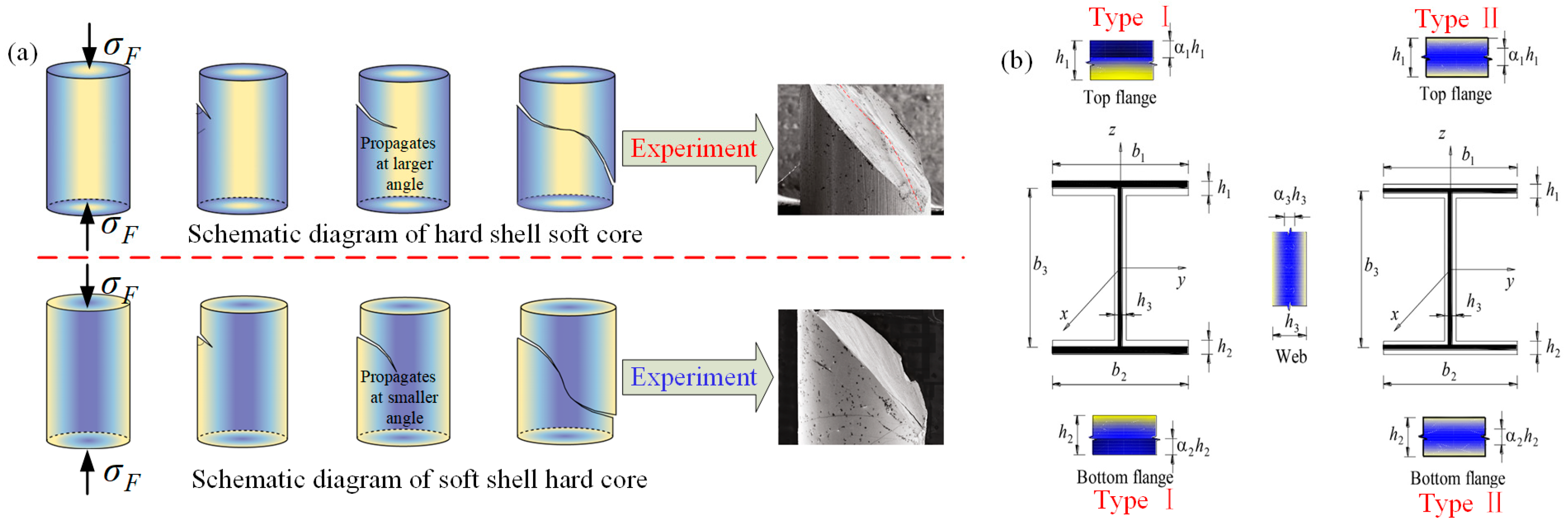
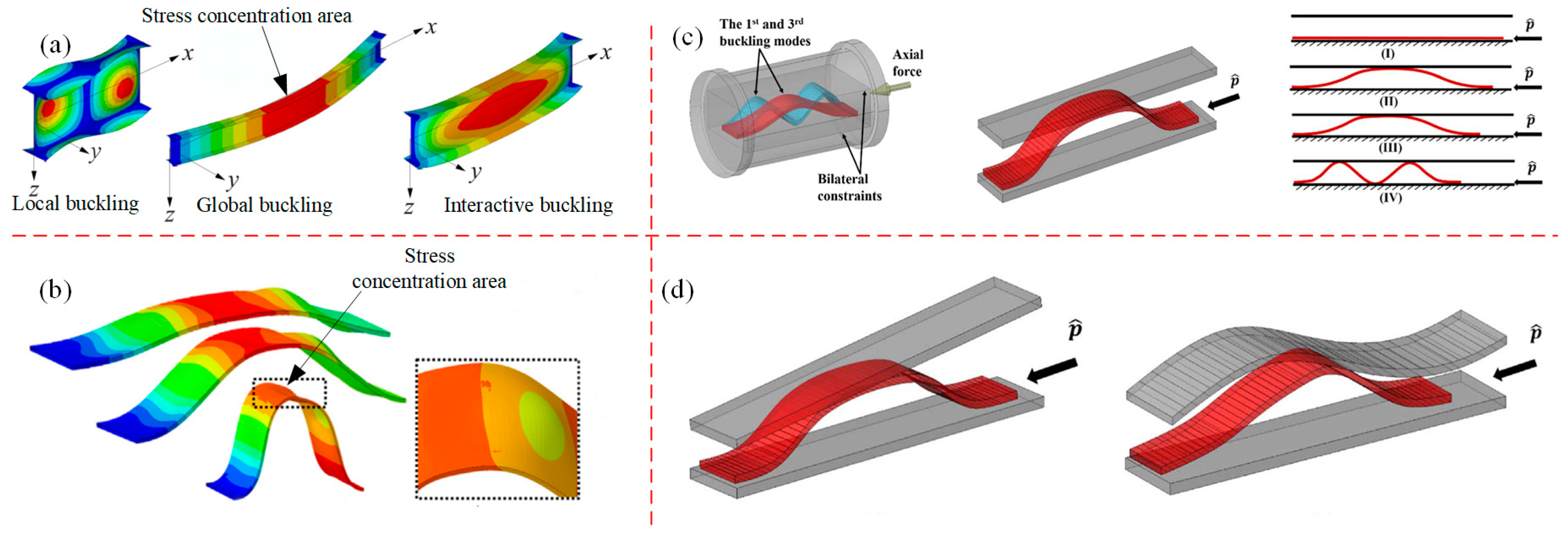
4.3. Deformation Instability of Beams
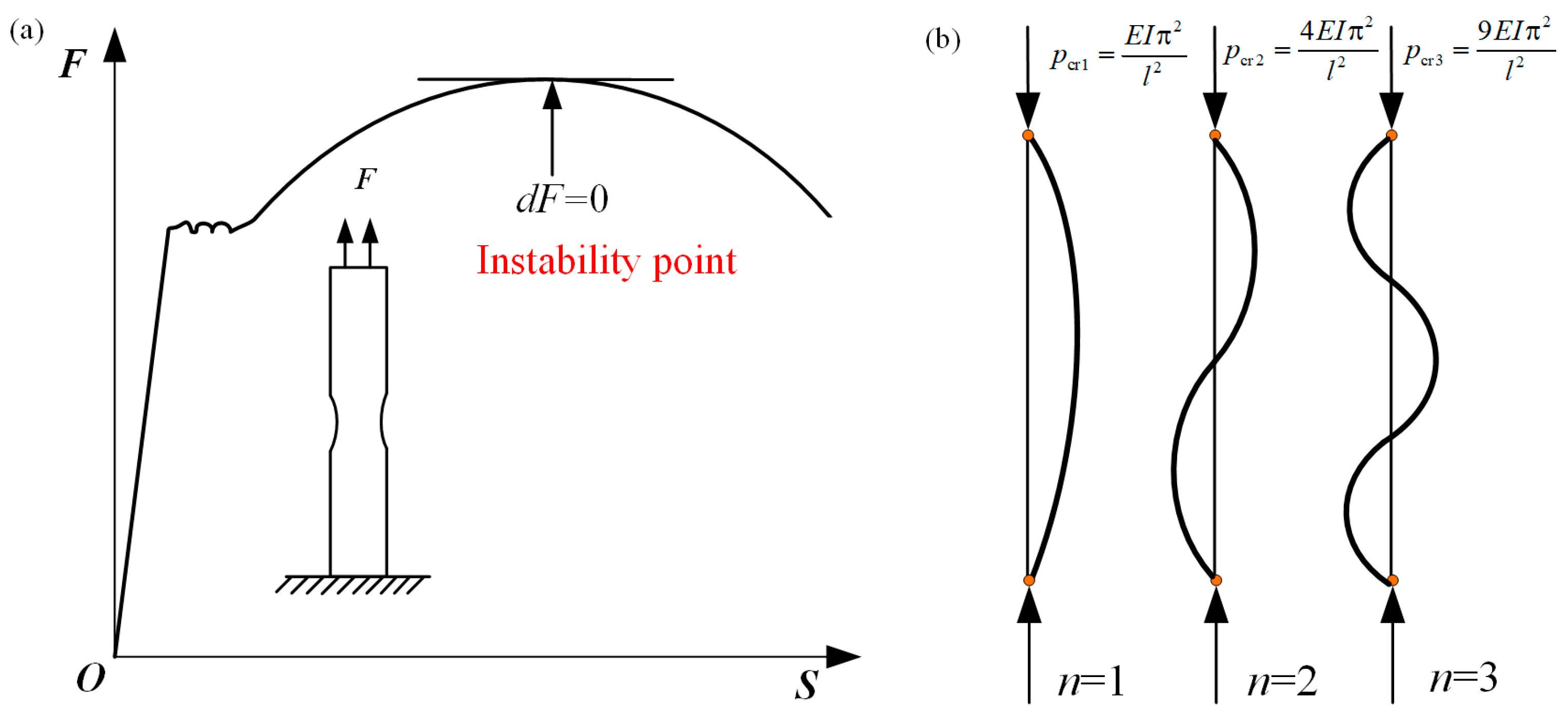
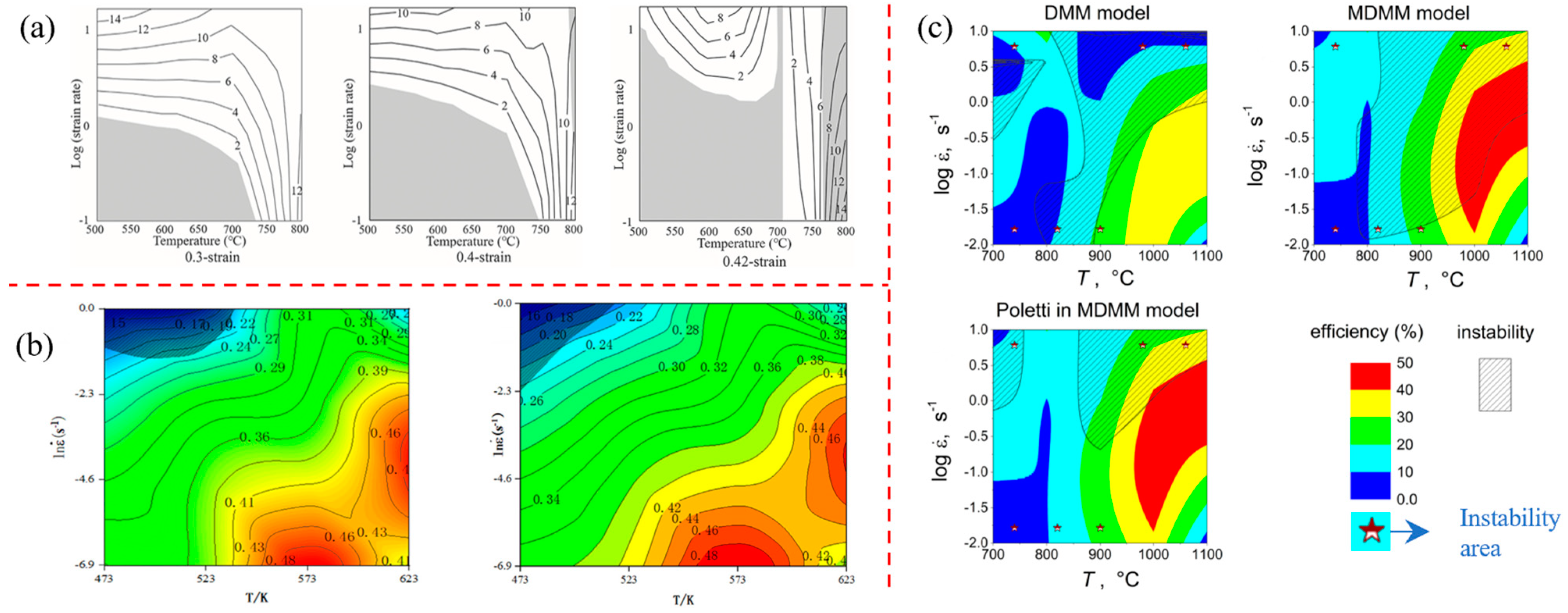
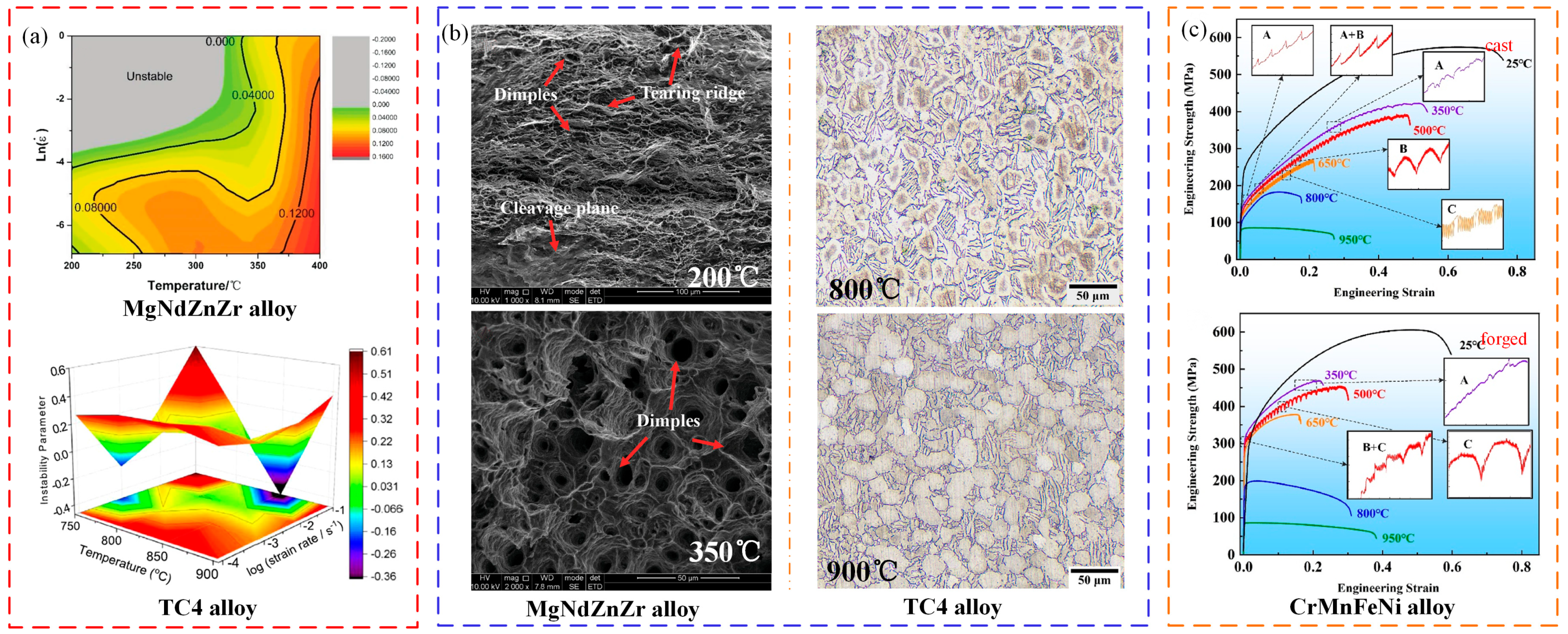
5. Analytical Methods of Deformation Instability
5.1. Theory Analysis
5.2. FE Simulation and Experiment
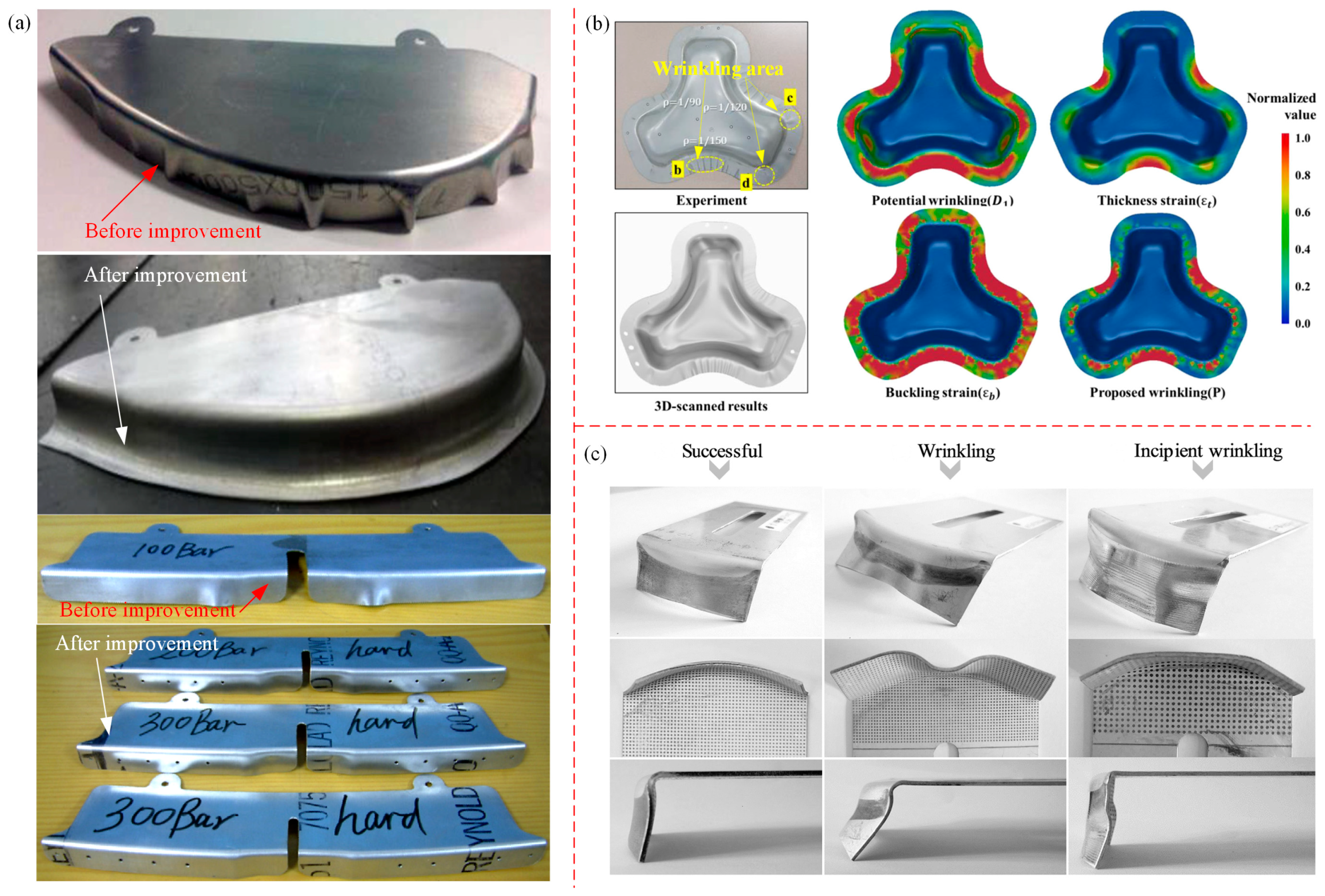
6. Engineering Applications of Deformation Instability
7. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, Z.; Cao, J.; Huang, L.; Yin, Z.; Zheng, L. Buckling behavior of AA6061 circular tube under axial compression by considering contact condition of tube end. Int. J. Lightweight Mater. Manuf. 2021, 4, 383–392. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Staker, M.R.; Jonas, J.J. Plastic instability and flow localization in shear at high rates of deformation. Acta Met. 1984, 32, 1347–1354. [Google Scholar] [CrossRef]
- Wang, Z.; Guines, D.; Chu, X.; Leotoing, L. Prediction and characterization of forming limits at necking from shear to equi-biaxial loading using the biaxial tensile testing method: Feasibility study and application to AA6061-T4. Mech. Mater. 2023, 179, 104598. [Google Scholar] [CrossRef]
- Xie, Z.; Chen, Y.; Wang, W. Stress-state-dependency of post-necking hardening rule and its influence on ductile fracture prediction. J. Constr. Steel Res. 2023, 202, 107797. [Google Scholar] [CrossRef]
- He, J.; Feng, Y. Determining localized necking in polycrystalline sheet metals using the bifurcation phenomenon in strain evolution. Crystals 2023, 13, 272. [Google Scholar] [CrossRef]
- Yang, Z.; Li, G.; Bradford, M. Local buckling and hysteretic behavior of Q690 high-strength steel box-section beam–columns. Thin-Walled Struct. 2023, 182, 110225. [Google Scholar] [CrossRef]
- Guo, M.; Arvin, H. Nonlinear thermal buckling instability analysis of a rotating nanocomposite beam reinforced with graphene platelet via the Chebyshev–Ritz scheme. Eng. Anal. Bound. Elem. 2023, 146, 241–251. [Google Scholar] [CrossRef]
- Carvalho, A.S.; Rossi, A.; Martins, C.H. Assessment of lateral–torsional buckling in steel I-beams with sinusoidal web openings. Thin-Walled Struct. 2022, 175, 109242. [Google Scholar] [CrossRef]
- Chen, G.; Chu, G.; Liu, P.; Gao, J. Analysis of the wall thickness distortion in tube hydro-forging. Int. J. Adv. Manuf. Technol. 2023, 1–10. [Google Scholar] [CrossRef]
- Chen, Y.; Zhao, S.; Wang, H.; Li, J.; Hua, L. Theoretical analysis and verification on plastic deformation behavior of rocket nozzle using a novel tube upsetting-bulging method. Materials 2023, 16, 1680. [Google Scholar] [CrossRef]
- Zhang, T.; Li, D.; Xu, T.; Sui, Y.; Han, X. Local Buckling-Induced Forming Method to Produce Metal Bellows. Chin. J. Mech. Eng. 2023, 36, 16. [Google Scholar] [CrossRef]
- Ji, S.M.; Choi, J.M.; Joun, M.S. Evaluation of the macroscopic formability of metallic materials using a cylinder compression test. J. Mater. Res. Technol. 2023, 23, 2798–2809. [Google Scholar] [CrossRef]
- Xue, T.; Yu, J.; Wang, Z.; Lin, P.; Sun, D.; Yan, B.; Zhang, Y.; Jiang, S. Investigation on hot workability of Fe-6.5Si-2Cr-12Ni high-silicon steel based on processing map and microstructural evolution. Metall. Mater. Trans. 2023, 1–17. [Google Scholar] [CrossRef]
- Raja, N.; Sinha, M.; Gautam, G. Dynamic response and microstructural characterization of Al3Zr/Al composite using 3D processing map. J. Mater. Eng. Perform. 2023, 1–12. [Google Scholar] [CrossRef]
- Zhao, M.; Xu, Y.; Guo, J.; Liao, X.; Zhang, D.; Han, P. Hot deformation behavior and processing map of Cu-Bearing ship plate steels. J. Mater. Eng. Perform. 2023, 1–8. [Google Scholar] [CrossRef]
- Mu, T.; Zhang, K.; Li, Y. Study on thermal compression deformation behavior and constitutive model of homogenized Mg–5Zn–1Mn alloy. J. Mater. Res. Technol. 2023, 22, 838–852. [Google Scholar] [CrossRef]
- Fani, M.; Taheri Behrooz, F. Analytical study of thermal buckling and post-buckling behavior of composite beams reinforced with SMA by Reddy Bickford theory. J. Intell. Mater. Syst. Struct. 2021, 33, 121–135. [Google Scholar] [CrossRef]
- Adin, H.; Ergün, R.K.; Adin, M.Ş. Computer aided numerical damage analysis of the axle shaft. Eur. Mech. Sci. 2022, 6, 201–206. [Google Scholar] [CrossRef]
- Adin, M.Ş.; Ergün, R.K.; Adin, H. Finite element analysis of safety pin in snowplow equipment. Eur. J. Technol. 2022, 12, 89–92. [Google Scholar] [CrossRef]
- Nguyen, X.T.; Park, J.S. Design equations for buckling strength of steel I-beam under non-uniform heating condition. Fire Saf. J. 2022, 127, 103464. [Google Scholar] [CrossRef]
- Islam, A.; Imanpour, A. Seismic stability of steel wide-flange columns in ductile moment-resisting frames: Out-of-plane response and design recommendations. Bull. Earthq. Eng. 2023, 20, 1–27. [Google Scholar] [CrossRef]
- Zhang, L.; Harrison, W.; Mehraban, S.; Brown, S.G.R.; Lavery, N.P. Size effect on the post-necking behaviour of dual-phase 800 steel: Modelling and experiment. Materials 2023, 16, 1458. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Su, X.; Geng, N. Experimental research on seismic behaviors of RC columns considering buckling of HRB600 longitudinal steel bars. Eng. Struct. 2023, 277, 115384. [Google Scholar] [CrossRef]
- Felippa, C.A.; Oñate, E. Accurate timoshenko beam elements for linear elastostatics and LPB stability. Arch. Comput. Methods Eng. 2021, 28, 2021–2080. [Google Scholar] [CrossRef]
- Abrahamson, G.R.; Goodier, J.N. Dynamic flexural buckling of rods within an axial plastic compression wave. J. Appl. Mech. 1966, 33, 241–247. [Google Scholar] [CrossRef]
- Hillier, M.J. Tensile plastic instability of thin tubes. Int. J. Mech. Sci. 1964, 7, 531–538. [Google Scholar] [CrossRef]
- Kim, J.B.; Yang, D.Y.; Yoon, J.W. The effect of plastic anisotropy on compressive instability in sheet metal forming. Int. J. Plast. 2000, 16, 649–676. [Google Scholar] [CrossRef]
- Swift, H.W. Plastic instability under plane stress. J. Mech. Phys. Solids 1952, 1, 1–18. [Google Scholar] [CrossRef]
- Hill, R. On discontinuous plastic states, with special reference to localized necking in thin sheets. J. Mech. Phys. Solids 1952, 1, 19–30. [Google Scholar] [CrossRef]
- Marciniak, Z.; Kuczynski, K. Limit strains in the processes of stretch-forming sheet metal. Int. J. Mech. Sci. 1967, 9, 609–620. [Google Scholar] [CrossRef]
- Tang, H.; Wen, T.; Zhou, Y.; Yang, F.; Zheng, Y. Finite element modeling of tensile instability of sheet metals considering nonuniform mechanical properties. J. Mater. Eng. Perform. 2022, 31, 3753–3762. [Google Scholar] [CrossRef]
- Seshacharyulu, K.; Mahalle, G.; Kotkunde, N.; Singh, S.K.; Balu Naik, B. High temperatures deformation and formability behavior of DP590 steel: Mechanical characterization and modeling. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 472. [Google Scholar] [CrossRef]
- Li, X.; Dong, H.; Wang, H.; Guo, G.; Li, D. Effect of strain rate difference between inside and outside groove in M-K model on prediction of forming limit curve of Ti6Al4V at elevated temperatures. Trans. Nonferrous Met. Soc. China 2020, 30, 405–416. [Google Scholar] [CrossRef]
- Mohebbi, M.S.; Akbarzadeh, A. Prediction of formability of tailor welded blanks by modification of MK model. Int. J. Mech. Sci. 2012, 61, 44–51. [Google Scholar] [CrossRef]
- Wang, X.; Fan, X.; Chen, X.; Yuan, S. Forming limit of 6061 aluminum alloy tube at cryogenic temperatures. J. Mater. Process. Technol. 2022, 3, 117649. [Google Scholar] [CrossRef]
- Wang, X.; Fan, X.; Chen, X.; Yuan, S. Cryogenic deformation behavior of 6061 aluminum alloy tube under biaxial tension condition. J. Mater. Process. Technol. 2022, 3, 117532. [Google Scholar] [CrossRef]
- Yu, K.; Li, Q.; Wu, Y.; Guo, M.; Li, D.; Liu, C.; Zhuang, L.; Wu, P. An improved Marciniak-Kuczynski approach for predicting sheet metal formability. Int. J. Mech. Sci. 2022, 2, 107200. [Google Scholar] [CrossRef]
- Yu, K.; Hou, L.; Guo, M.; Li, D.; Huang, D.; Zhuang, L.; Zhang, J.; Wu, P. A method for determining r-value of aluminum sheets with the Portevin-Le Chatelier effect. Mater. Sci. Eng. A 2021, 8, 141246. [Google Scholar] [CrossRef]
- Bong, H.J.; Lee, J. Crystal plasticity finite element-Marciniak-Kuczynski approach with surface roughening effect in predicting formability of ultra-thin ferritic stainless steel sheets. Int. J. Mech. Sci. 2021, 19, 106066. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C.; Wang, Y.; Zhao, G.; Chen, L. An investigation on the anisotropic plastic behavior and forming limits of an Al-Mg-Li alloy sheet. J. Mater. Eng. Perform. 2021, 30, 8224–8234. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C.; Yang, Y.; Fan, S.; Wang, G.; Zhao, G.; Chen, L. The integration of through-thickness normal stress and friction stress in the M-K model to improve the accuracy of predicted FLCs. Int. J. Plast. 2019, 120, 147–163. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C.; Yang, Y.; Wang, Y.; Zhao, G.; Chen, L. The identification of improved Johnson-Cook constitutive model in a wide range of temperature and its application in predicting FLCs of Al-Mg-Li sheet. J. Mater. Res. Technol. 2020, 9, 3782–3795. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, C. The determination of friction coefficient in Nakazima test and its application in predicting FLCs with modified M-K model. IOP Conf. Ser. Mater. Sci. Eng. 2020, 967, 012056. [Google Scholar] [CrossRef]
- Li, X.; Chen, Y.; Lang, L.; Xiao, R. A modified M-K method for accurate prediction of FLC of aluminum alloy. Metals 2021, 11, 394. [Google Scholar] [CrossRef]
- Hu, Q.; Li, X.; Chen, J. Forming limit evaluation by considering through-thickness normal stress: Theory and modeling. Int. J. Mech. Sci. 2019, 155, 187–196. [Google Scholar] [CrossRef]
- Hu, Q.; Li, X.; Chen, J. New robust algorithms for Marciniak–Kuczynski model to calculate the forming limit diagrams. Int. J. Mech. Sci. 2018, 148, 293–306. [Google Scholar] [CrossRef]
- He, Z.; Wang, Z.; Lin, Y.; Zhu, H.; Yuan, S. A modified Marciniak–Kuczynski model for determining the forming limit of thin-walled tube extruded with initial eccentricity. Int. J. Mech. Sci. 2019, 151, 715–723. [Google Scholar] [CrossRef]
- Storen, S.; Rice, J.R. Localized necking in thin sheets. J. Mech. Phys. Solids 1975, 23, 421–441. [Google Scholar] [CrossRef]
- Zajkani, A.; Bandizaki, A. An efficient model for diffuse to localized necking transition in rate-dependent bifurcation analysis of metallic sheets. Int. J. Mech. Sci. 2017, 133, 794–803. [Google Scholar] [CrossRef]
- Pham, Q.T.; Mac, T.B.; Kim, Y.S.; Nguyen, D.T. A comparative investigation on theoretical models for forming limit diagram prediction of automotive sheet metals. Mech. Based Des. Struct. Mach. 2021, 1–15. [Google Scholar] [CrossRef]
- Long, B.T.; Kim, Y.H.; Ishizaki, K.; Toan, N.D.; Parinov, I.A.; Vu, N.P. Proceedings of the 2nd Annual International Conference on Material, Machines and Methods for Sustainable Development; Spring Nature: New York, NY, USA, USA, 2021; Volume 12, pp. 123–128. [Google Scholar]
- Bressan, J.D.; Willams, J.A. The use of a shear instability criterion to predict local necking in sheet metal deformation. Int. J. Mech. Sci. 1983, 25, 155–168. [Google Scholar] [CrossRef]
- Thomson, P.F.; Nayak, P.U. The effect of plastic deformation on the roughening of free surfaces of sheet metal. Int. J. Mach. Tool Des. Res. 1980, 20, 73–86. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Jonas, J.J. Formability and workability of metals: Plastic instability and flow localization. Am. Soc. Met. 1984, 299. [Google Scholar]
- Song, Y.; Guan, Z.; Wang, M.; Song, J. Mechanical analysis of temperature impact on stability during superplastic tensile deformation. Sci. China Ser. E Technol. Sci. 2006, 49, 641–654. [Google Scholar] [CrossRef]
- Song, Y.Q.; Suo, Z.L.; Guan, Z.P. Mechanical analysis of material parameters’ impact on tensile instability. Acta Metall. Sin. 2006, 42, 337–340. [Google Scholar]
- Song, Y.Q. Mechanical analysis of superplastic tensile deformation. Chin. J. Mech. Eng. 2003, 39, 67–72. [Google Scholar] [CrossRef]
- Li, Y.P.; Zhu, X.F.; Zhang, G.P.; Tan, J.; Wang, W.; Wu, B. Investigation of deformation instability of Au/Cu multilayers by indentation. Philos. Mag. 2010, 90, 3049–3067. [Google Scholar] [CrossRef]
- Li, Y.P.; Zhu, X.F.; Tan, J.; Wu, B.; Zhang, G.P. Two different types of shear-deformation behaviour in Au–Cu multilayers. Philos. Mag. Lett. 2009, 89, 66–74. [Google Scholar] [CrossRef]
- Hart, E.W. Theory of the tensile test. Acta Metall. 1967, 15, 351–355. [Google Scholar] [CrossRef]
- Jonas, J.J.; Holt, R.A.; Coleman, C.E. Plastic stability in tension and compression. Acta Metall. 1976, 24, 911–918. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Lahoti, G.D. Deformation and unstable flow in hot forging of Ti-6Ai-2Sn-4Zr-2Mo-0.1 Si. Metall. Trans. A 1981, 12, 1705–1717. [Google Scholar] [CrossRef]
- Ziegler, H. Some extremum principles in irreversible thermodynamics, with application to continuum mechanics. Prog. Solid Mech. 1963, 4, 93–193. [Google Scholar]
- Chen, G.; Jin, Y.; Wang, J.; Zhang, C.; Chen, Q.; Zhang, H.; Zhao, X.; Li, Z.; Xie, C.; Du, Z. Dislocation density-based constitutive model and processing map for T2 copper during isothermal and time-variant deformation. Met. Mater. Int. 2022, 28, 2134–2145. [Google Scholar] [CrossRef]
- Zheng, X.; Luo, P.; Han, Z. Hot deformation behavior and processing map of high-strength Mg-2.5Nd-0.2Zn-1.0Zr alloy. J. Mater. Eng. Perform. 2021, 31, 1222–1230. [Google Scholar] [CrossRef]
- Zheng, X.; Luo, P.; Chu, Z.; Xu, J.; Wang, F. Plastic flow behavior and microstructure characteristics of light-weight 2060 Al-Li alloy. Mater. Sci. Eng. A 2018, 736, 465–471. [Google Scholar] [CrossRef]
- Liu, A.; Wang, L.; Cheng, X.; Ning, Z.; Pan, L. Study on hot deformation behavior of as-cast 22%W high-density steel. J. Mater. Res. Technol. 2022, 17, 2355–2368. [Google Scholar] [CrossRef]
- Alexander, J.M. Mapping dynamic material behaviour. In Modelling Hot Deformation of Steels; Springer: Berlin, Germany, 1989; pp. 101–115. [Google Scholar]
- Murty, S.V.S.N.; Rao, B.N. On the development of instability criteria during hotworking with reference to IN 718. Mater. Sci. Eng. 1998, 254, 76–82. [Google Scholar] [CrossRef]
- Xing, H.L.; Wang, C.W.; Zhang, K.F. Recent development in the mechanics of superplasticity and its applications. J. Mater. Process. Technol. 2004, 151, 196–202. [Google Scholar] [CrossRef]
- Giuliano, G. Simulation of instability during superplastic deformation using finite element method. Mater. Des. 2005, 26, 373–376. [Google Scholar] [CrossRef]
- Giuliano, G. Failure analysis in superplastic materials. Int. J. Mach. Tools Manuf. 2006, 46, 1604–1609. [Google Scholar] [CrossRef]
- Zhang, W.; Gao, Y.; Feng, Z.; Wang, X.; Zhang, S.; Huang, L.; Huang, Z.; Jiang, L. Ductility limit diagrams for superplasticity and forging of high temperature polycrystalline materials. Acta Mater. 2020, 194, 378–386. [Google Scholar] [CrossRef]
- Sheinerman, A.G. Effect of the specimen size on necking development in metals and alloys during superplastic deformation. Mater. Phys. Mech. 2020, 46, 1–6. [Google Scholar]
- Jafarian, H.R.; Mousavi Anijdan, S.H.; Miyamoto, H. Observation of low temperature superplasticity in an ultrafine grained AA6063 alloy. Mater. Sci. Eng. A 2020, 795, 140015. [Google Scholar] [CrossRef]
- Jafarian, H.R.; Anijdan, S.H.M.; Eivani, A.R. A comprehensive study of microstructure development and its corresponding tensile properties in nano/ultrafine-grained metastable austenitic steel during accumulative roll bonding (ARB). Mater. Sci. Eng. A 2017, 703, 196–204. [Google Scholar] [CrossRef]
- Li, S.; Huang, Z.; Jin, S. Superplastic behavioral characteristics of fine-grained 5A70 Aluminum alloy. Metals 2019, 9, 62. [Google Scholar] [CrossRef]
- Malik, A.; Wang, Y.; Huanwu, C.; Bhatti, T.M.; Nazeer, F. Superplastic behavior of fine-grained extruded ZK61 Mg alloy. Results Phys. 2021, 20, 103731. [Google Scholar] [CrossRef]
- Nazeer, F.; Long, J.; Yang, Z.; Li, C. Superplastic deformation behavior of Mg alloys: A-review. J. Magnes. Alloy. 2022, 10, 97–109. [Google Scholar] [CrossRef]
- Demirel, M.Y.; Karaağaç, İ. High-speed superplastic formability and deformation mechanisms of Ti6Al4V sheets. Mater. Sci. Eng. A 2023, 866, 144652. [Google Scholar] [CrossRef]
- Bobruk, E.V.; Murashkin, M.Y.; Ramazanov, I.A.; Kazykhanov, V.U.; Valiev, R.Z. Low-temperature superplasticity and high strength in the Al 2024 alloy with ultrafine grains. Materials 2023, 16, 727. [Google Scholar] [CrossRef]
- Myshlyaev, M.; Korznikova, G.; Konkova, T.; Korznikova, E.; Aletdinov, A.; Khalikova, G.; Raab, G.; Mironov, S. Microstructural evolution during superplastic deformation of Al-Mg-Li alloy: Dynamic recrystallization or grain-boundary sliding? J. Alloys Compd. 2023, 936, 168302. [Google Scholar] [CrossRef]
- Mochugovskiy, A.G.; Mosleh, A.O.; Kotov, A.D.; Khokhlov, A.V.; Kaplanskaya, L.Y.; Mikhaylovskaya, A.V. Microstructure evolution, constitutive modelling, and superplastic forming of experimental 6XXX-type alloys processed with different thermomechanical treatments. Materials 2023, 16, 445. [Google Scholar] [CrossRef] [PubMed]
- Furjan, A.; Yang, Y.; Farrokhian, A.; Shen, X.; Kolahchi, R.; Rajak, D.K. Dynamic instability of nanocomposite piezoelectric-leptadenia pyrotechnica rheological elastomer-porous functionally graded materials micro viscoelastic beams at various strain gradient higher-order theories. Polym. Compos. 2021, 43, 282–298. [Google Scholar] [CrossRef]
- Ghiamy, A.; Amoushahi, H. Dynamic stability of different kinds of sandwich plates using third order shear deformation theory. Thin-Walled Struct. 2022, 172, 108822. [Google Scholar] [CrossRef]
- Ghanavati, R.; Naffakh-Moosavy, H. Additive manufacturing of functionally graded metallic materials: A review of experimental and numerical studies. J. Mater. Res. Technol. 2021, 13, 1628–1664. [Google Scholar] [CrossRef]
- Saleh, B.; Jiang, J.; Fathi, R.; Al-hababi, T.; Xu, Q.; Wang, L.; Song, D.; Ma, A. 30 Years of functionally graded materials: An overview of manufacturing methods, applications and future challenges. Compos. Part B Eng. 2020, 201, 108376. [Google Scholar] [CrossRef]
- Tang, Y.; Zhou, H.; Lu, H.; Wang, X.; Cao, Q.; Zhang, D.; Yang, W.; Jiang, J.Z. Extra plasticity governed by shear band deflection in gradient metallic glasses. Nat. Commun. 2022, 13, 2120. [Google Scholar] [CrossRef]
- Nguyen, N.D.; Vo, T.P.; Nguyen, T.K. An improved shear deformable theory for bending and buckling response of thin-walled FG sandwich I-beams resting on the elastic foundation. Compos. Struct. 2020, 254, 112823. [Google Scholar] [CrossRef]
- Lukaszek Solek, A.; Sleboda, T.; Lisiecki, L.; Krawczyk, J. Hot deformation behavior of 4130 high-strength steel. Materials 2022, 15, 7817. [Google Scholar] [CrossRef]
- Narayana Murty, S.V.S.; Nageswara, R.B.; Kashyap, B.P. Instability criteria for hot deformation of materials. Int. Mater. Rev. 2013, 45, 15–26. [Google Scholar] [CrossRef]
- Zhao, T.; Zhang, B.; Zhang, Z.; Chen, L.; Xu, Y.; Cai, J.; Wang, K. Deformation behavior and microstructural evolution of pure Ti produced by hot compressing. J. Mater. Res. Technol. 2022, 21, 2383–2399. [Google Scholar] [CrossRef]
- Culver, R.S. Thermal instability strain in dynamic plastic deformation. In Metallurgical Effects at High Strain Rates; Springer: Boston, MA, USA, 1973; pp. 519–530. [Google Scholar]
- Ji, S.M.; Jang, S.M.; Lee, Y.S.; Kwak, H.M.; Choi, J.M.; Joun, M.S. Characterization of Ti-6Al-4V alloy in the temperature range of warm metal forming and fracture analysis of the warm capping process. J. Mater. Res. Technol. 2022, 18, 1590–1606. [Google Scholar] [CrossRef]
- Xia, Y.F.; Jiang, W.; Cheng, Q.; Jiang, L.; Jin, L. Hot deformation behavior of Ti-6Al-4V-0.1Ru alloy during isothermal compression. Trans. Nonferrous Met. Soc. China 2020, 30, 134–146. [Google Scholar] [CrossRef]
- Ma, Z.; Li, G.; Su, Z.; Wei, G.; Huang, Y.; Hort, N.; Hadadzadeh, A.; Wells, M.A. Hot deformation behavior and microstructural evolution for dual-phase Mg-9Li-3Al alloys. J. Mater. Res. Technol. 2022, 19, 3536–3545. [Google Scholar] [CrossRef]
- Ling, K.; Mo, W.; Deng, P.; Chen, J.; Luo, B.; Bai, Z. Hot deformation behavior and dynamic softening mechanisms of hot-extruded Al-Cu-Mg-Ag-Mn-Zr-Ti alloy. Mater. Today Commun. 2023, 34, 105300. [Google Scholar] [CrossRef]
- Singh, P.P.; Mula, S.; Malakar, A.; Ghosh, S. Strain-rate dependent workability and plastic flow instability of a (Nb+V) stabilized microalloyed steel. Mater. Today Commun. 2022, 31, 103519. [Google Scholar] [CrossRef]
- Singh, P.P.; Ghosh, S.; Mula, S. Flow stress modeling and microstructural characteristics of a low carbon Nb-V microalloyed steel. Mater. Today Commun. 2022, 30, 103156. [Google Scholar] [CrossRef]
- Zheng, X.; Luo, P.; Dong, J.; Wang, S. The effect of casting speed on microstructure, microsegregation, and mechanical properties of high-strength Mg-Nd-Zn-Zr alloy. J. Mater. Eng. Perform. 2019, 28, 1753–1761. [Google Scholar] [CrossRef]
- Imran, S.M.; Li, C.; Lang, L.; Guo, Y.; Mirza, H.A.; Haq, F.; Alexandrova, S.; Jiang, J.; Han, H. An investigation into Arrhenius type constitutive models to predict complex hot deformation behavior of TC4 alloy having bimodal microstructure. Mater. Today Commun. 2022, 31, 103622. [Google Scholar] [CrossRef]
- Yang, T.; Li, X.; Zhang, L.; Wang, J.; Jin, P. Hot tensile deformation mechanism and microstructure evolution of Mg 2Nd alloy with heterostructure. Mater. Charact. 2022, 186, 111792. [Google Scholar] [CrossRef]
- Sun, J.; Zhao, W.; Yan, P.; Li, S.; Dai, Z.; Jiao, L.; Qiu, T.; Wang, X. High temperature tensile properties of as-cast and forged CrMnFeCoNi high entropy alloy. Mater. Sci. Eng. A 2022, 850, 143570. [Google Scholar]
- Shabani, A.; Toroghinejad, M.R.; Aminaei, M. Hot deformation behavior of FeCrCuMnNi high entropy alloy. Mater. Chem. Phys. 2023, 295, 127213. [Google Scholar] [CrossRef]
- Singh, V.; Srirangam, P.; Chakrabarti, D.; Roy, G.G. Hot deformation behavior of EN30B forged steels in the presence of non-metallic inclusions. J. Mater. Eng. Perform. 2023, 1–13. [Google Scholar] [CrossRef]
- Jeong, H.Y.; Park, J.; Kim, Y.; Shin, S.Y.; Kim, N. Processing parameters optimization in hot forging of AISI 4340 steel using instability map and reinforcement learning. J. Mater. Res. Technol. 2023, 23, 1995–2009. [Google Scholar] [CrossRef]
- Azizi, A.; Zangeneh, S.; Mojtahedi, M.; Rasaee, S. Hot deformation behavior and microstructure of Ni-Cu rich Al-Si AA4032 alloy. J. Alloys Compd. 2023, 940, 168934. [Google Scholar] [CrossRef]
- Yang, Z.; Dong, Y.; Li, W.; Liu, X.; Feng, H. Effect of Sr on hot deformation behavior and microstructure of Al-4.6Mg alloy. J. Alloys Compd. 2023, 930, 167300. [Google Scholar] [CrossRef]
- Lin, X.; Huang, H.; Yuan, X.; Wang, Y.; Zheng, B.; Zuo, X.; Zhou, G. Study on hot deformation behavior and processing map of a Ti-47.5Al-2.5V-1.0Cr-0.2Zr alloy with a fully lamellar microstructure. J. Alloys Compd. 2022, 901, 163648. [Google Scholar] [CrossRef]
- Yang, G.; Xu, W.; Jin, X.; Wang, Z.; Shan, D.; Guo, B. Hot deformation behavior and microstructure evolution of the spray deposited and secondary hot extruded 2195 Al-Li alloy. J. Mater. Res. Technol. 2022, 20, 2784–2798. [Google Scholar] [CrossRef]
- Qiao, L.; Ramanujan, R.V.; Zhu, J. Optimized hot working parameters of Fe2.5Ni2.5CrAl multi-principal element alloys. J. Alloys Compd. 2022, 925, 166594. [Google Scholar] [CrossRef]
- Ghosh, S.; Hamada, A.; Patnamsetty, M.; Borek, W.; Gouda, M.; Chiba, A.; Ebied, S. Constitutive modeling and hot deformation processing map of a new biomaterial Ti-14Cr alloy. J. Mater. Res. Technol. 2022, 20, 4097–4113. [Google Scholar] [CrossRef]
- Yi, H.; Ding, J.; Ni, C.; Dai, J.; Tang, Y.; Chen, X.; Song, K.; Xia, X. Hot compression deformation behavior and processing maps of Al-0.5Mg-0.4Si-0.1Cu alloy. J. Mater. Res. Technol. 2022, 19, 4890–4904. [Google Scholar] [CrossRef]
- Cai, G.; Fu, J.; Zhang, D.; Yang, J.; Yuan, Y.; Lang, L.; Alexandrov, S. A novel approach to predict wrinkling of aluminum alloy during warm/hot sheet hydroforming based on an improved yoshida buckling test. Materials 2020, 13, 1165. [Google Scholar] [CrossRef] [PubMed]
- Du, B.; Yang, H.; Wang, J.; Liang, J.; Li, H.; Zhao, C. Establishment of the unified critical wrinkling limit curve of thin-walled parts forming. Eng. Fail. Anal. 2022, 140, 106605. [Google Scholar] [CrossRef]
- Tang, H.; Wen, T.; Zhou, Y.; You, J.; Ma, D. Study on the wrinkling behavior of perforated metallic plates using uniaxial tensile tests. Thin-Walled Struct. 2021, 167, 108132. [Google Scholar] [CrossRef]
- Li, R.; Zheng, Z.; Zhan, M.; Zhang, H.; Cui, X.; Lei, Y. Fracture prediction for metal sheet deformation under different stress states with uncoupled ductile fracture criteria. J. Manuf. Process. 2022, 73, 531–543. [Google Scholar] [CrossRef]
- Li, F.F.; Fang, G. Stress-state dependency of ductile fracture in an extruded magnesium alloy and its underlying mechanisms. Int. J. Plast. 2022, 152, 103258. [Google Scholar] [CrossRef]
- Du, B.; Xie, J.; Li, H.; Zhao, C.; Zhang, X.; Yuan, X. Determining factors affecting sheet metal plastic wrinkling in response to nonuniform tension using wrinkling limit diagrams. Thin-Walled Struct. 2020, 147, 106535. [Google Scholar] [CrossRef]
- Tang, H.; Wen, T.; Hong, J.; Zhou, Y.; Zhang, L. Analysis of shear stress wrinkling of asymmetric sheet specimen under offset loading. J. Mech. Sci. Technol. 2022, 36, 1451–1457. [Google Scholar] [CrossRef]
- Chen, L.; Bai, Y.; Jiang, Z.; Chen, H.; Wu, C.; Wang, Q. Numerical and experimental studies on wrinkling control methods of sheet metal part with high curvature and large flange in rubber forming. Adv. Mech. Eng. 2019, 11, 16878. [Google Scholar] [CrossRef]
- Chen, L.; Chen, H.; Wang, Q.; Li, Z. Studies on wrinkling and control method in rubber forming using aluminium sheet shrink flanging process. Mater. Des. 2015, 65, 505–510. [Google Scholar] [CrossRef]
- Won, C.; Kim, H.; Lee, S.; Kim, D.; Park, S.; Yoon, J. Wrinkling prediction for GPa-grade steels in sheet metal forming process. Int. J. Adv. Manuf. Technol. 2019, 102, 3849–3863. [Google Scholar] [CrossRef]
- López-Fernández, J.A.; Centeno, G.; Vallellano, C. Wrinkling in shrink flanging by single point incremental forming. Int. J. Mech. Sci. 2023, 240, 107930. [Google Scholar] [CrossRef]
- López-Fernández, J.A.; Centeno, G.; Martínez-Donaire, A.J.; Morales-Palma, D.; Vallellano, C. Stretch-flanging of AA2024-T3 sheet by single-stage SPIF. Thin-Walled Struct. 2021, 160, 107338. [Google Scholar] [CrossRef]
- Haley, J.A.; Kyriakides, S. Bending induced wrinkling and creasing in axially crushed aluminum tubes. Int. J. Solids Struct. 2020, 202, 368–383. [Google Scholar] [CrossRef]
- Huang, X.; Guan, B.; Zang, Y.; Wang, B. Investigation of defect behavior during the stamping of a thin-walled semicircular shell with bending angle. J. Manuf. Process. 2023, 87, 231–244. [Google Scholar] [CrossRef]
- Li, J.; Wang, Z.; Zhang, S.; Lin, Y.; Wang, L.; Sun, C.; Tan, J. A novelty mandrel supported thin-wall tube bending cross-section quality analysis: A diameter-adjustable multi-point contact mandrel. Int. J. Adv. Manuf. Technol. 2023, 124, 4615–4637. [Google Scholar] [CrossRef]
- Safdarian, R. Investigation of tube fracture in the rotary draw bending process using experimental and numerical methods. Int. J. Mater. Form. 2019, 13, 493–516. [Google Scholar] [CrossRef]
- Safdarian, R. Experimental and numerical investigation of wrinkling and tube ovality in the rotary draw bending process. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 5568–5584. [Google Scholar] [CrossRef]
- Jiang, L.; Lin, Y.; Li, H.; Zhang, S.; Feng, Y.; Wang, Y.; Sun, M. A new mandrel design with mandrel ball thickness variation for the bending process of aviation ultra-thin-walled tubes. Int. J. Adv. Manuf. Technol. 2022, 122, 1805–1819. [Google Scholar] [CrossRef]
- Jia, Q.; An, N.; Ma, X.; Zhou, J. A dynamic finite element procedure for bending collapse of composite thin-walled lenticular tubes. Compos. Struct. 2022, 287, 115364. [Google Scholar] [CrossRef]
- Jia, Q.; An, N.; Ma, X.; Zhou, J. Exploring the design space for nonlinear buckling of composite thin-walled lenticular tubes under pure bending. Int. J. Mech. Sci. 2021, 207, 106661. [Google Scholar] [CrossRef]
- Naderi, G.; Moussavi Torshizi, S.E.; Dibajian, S.H. Experimental-numerical study of wrinkling in rotary-draw bending of Tight Fit Pipes. Thin-Walled Struct. 2023, 183, 110428. [Google Scholar] [CrossRef]
- Zhu, Y.; Wan, M.; Chen, W.; Wang, Y.; Tu, W.; Xu, F. Influence of mandrel-cores filling on size effect of cross-section distortion of bimetallic thin-walled composite bending tube. Chin. J. Aeronaut. 2022, 36, 421–435. [Google Scholar] [CrossRef]
- Zhu, Y.X.; Wan, M.M.; Wang, Y.; Tu, W.B.; Cheng, Y.F. Size effect mechanism of cross-section deformation and section hollow coefficient-bending degree of the thin-walled composite bending tube. Mater. Des. 2021, 212, 110274. [Google Scholar] [CrossRef]
- Sun, H.; Li, H.; Gong, F.; Liu, Y.; Li, G.; Fu, M. Filler parameters affected wrinkling behavior of aluminum alloy double-layered gap tube in rotary draw bending process. Int. J. Adv. Manuf. Technol. 2022, 119, 5261–5276. [Google Scholar] [CrossRef]
- Li, H.; Sun, H.; Liu, H.; Liu, N. Loading conditions constrained wrinkling behaviors of thin-walled sheet/tube parts during metal forming. J. Mater. Process. Technol. 2021, 296, 117199. [Google Scholar] [CrossRef]
- Liu, H.; Liu, Y.; Zhang, P.; Du, X. Effect of weld zone and corner with cold bending effect on wrinkling of rectangular welded tube in rotary draw bending. Thin-Walled Struct. 2020, 157, 107115. [Google Scholar] [CrossRef]
- Li, H.; Liu, H.R.; Liu, N.; Sun, H.; Yang, H.; Liu, B.Y. Towards sensitive prediction of wrinkling instability in sheet metal forming by introducing evolution of triple nonlinearity: Tube forming. Int. J. Mech. Sci. 2019, 161–162, 105054. [Google Scholar] [CrossRef]
- Sun, H.; Li, H.; Yi, L.; Liu, Y.; Du, J.; Fu, M.W. Prediction and control of bending quality of double-layered gap tube. Int. J. Mech. Sci. 2022, 228, 107474. [Google Scholar] [CrossRef]
- Li, H.; Ma, J.; Liu, B.Y.; Gu, R.J.; Li, G.J. An insight into neutral layer shifting in tube bending. Int. J. Mach. Tools Manuf. 2018, 126, 51–70. [Google Scholar] [CrossRef]
- Yu, G.; Qi, S.; Cao, H.; Zhou, K. A novel cold stamping process of large extrados single-welded elbow suitable for internal high pressure. Int. J. Adv. Manuf. Technol. 2022, 122, 2293–2306. [Google Scholar]
- Tao, R.; Xu, X.; Fan, Y.; Xiao, J.; Wang, Y.; Wei, L. Differential flow velocities control method for push-bending of the thin-walled tube with a 0.9D bending radius by differential lubrication. Int. J. Adv. Manuf. Technol. 2022, 124, 3359–3369. [Google Scholar] [CrossRef]
- Xiao, J.; Xu, X.; Zuo, D.; Wu, S.; Huang, L. Effect of contact states in the tube on the push bending with low-melting-point alloy as filling medium. Int. J. Adv. Manuf. Technol. 2022, 123, 4277–4291. [Google Scholar] [CrossRef]
- Österreicher, J.A.; Nebeling, D.; Grabner, F.; Cerny, A.; Zickler, G.A.; Eriksson, J.; Wikström, G.; Suppan, W.; Schlögl, C.M. Secondary ageing and formability of an Al-Cu-Mg alloy (2024) in W and under-aged tempers. Mater. Des. 2023, 226, 111634. [Google Scholar] [CrossRef]
- Cheng, X.; Zhao, Y.; Abd El-Aty, A.; Guo, X.; You, S. Deformation behavior of convolute thin-walled AA6061-T6 rectangular tubes manufactured by the free bending forming technology. Int. J. Adv. Manuf. Technol. 2021, 118, 2933–2948. [Google Scholar] [CrossRef]
- Wang, W.; Cheng, C.; Wan, L.; Tao, J.; Guo, X.; Liu, C. Effect of die structure parameters on deformation behavior of bent tube in free bending process. J. Iron Steel Res. Int. 2022, 1–17. [Google Scholar] [CrossRef]
- Hu, S.; Cheng, C.; El-Aty, A.A.; Zheng, S.; Guo, X.; Liu, C.; Tao, J. Influence of deformation zone length on bending radius of SS304 tubes with small diameters manufactured via free bending-based active motion. Chin. J. Aeronaut. 2022, in press. [CrossRef]
- Cheng, Z.; Li, S.; Cheng, C.; Guo, X.; Shi, Y.; Shu, S. Investigation on variation characteristics of bent tube axis and determination of bending die motion trajectory in free bending process. Int. J. Adv. Manuf. Technol. 2022, 124, 3371–3389. [Google Scholar] [CrossRef]
- Yang, Q.; Liu, C.; Cheng, C.; Tao, J.; Bai, X.; Ma, Z.; Guo, X. Six-axis free bending and twisting analysis of spiral square tube. Int. J. Mech. Sci. 2022, 228, 107459. [Google Scholar] [CrossRef]
- Jiao, P.; Borchani, W.; Lajnef, N. Large deformation solutions to post-buckled beams confined by movable and flexible constraints: A static and dynamic analysis. Int. J. Solids Struct. 2017, 128, 85–98. [Google Scholar] [CrossRef]
- Jiao, P.; Borchani, W.; Hasni, H.; Lajnef, N. Enhancement of quasi-static strain energy harvesters using non-uniform cross-section post-buckled beams. Smart Mater. Struct. 2017, 26, 085045. [Google Scholar] [CrossRef]
- Eyvazian, A.; Zhang, C.; Alkhedher, M.; Muhsen, S.; Elkotb, M.A. Thermal buckling and post-buckling analyses of rotating Timoshenko microbeams reinforced with graphene platelet. Compos. Struct. 2023, 304, 116358. [Google Scholar] [CrossRef]
- Mittelstedt, S.; Mittelstedt, C. Mixed-mode buckling of shear-deformable composite laminated I-beams. Int. J. Mech. Sci. 2020, 169, 105332. [Google Scholar] [CrossRef]
- Jiao, P.; Alavi, A.H. Size-dependent buckling instability and recovery of beam-like, architected microstructures. Mater. Des. 2019, 162, 405–417. [Google Scholar] [CrossRef]
- Jiao, P.; Borchani, W.; Hasni, H.; Lajnef, N. Static and dynamic post-buckling analyses of irregularly constrained beams under the small and large deformation assumptions. Int. J. Mech. Sci. 2017, 124–125, 203–215. [Google Scholar] [CrossRef]
- Mhada, K.; Bourihane, O. A multi-scale model for global buckling and local wrinkling interaction with application to sandwich beams. Structures 2021, 32, 1398–1407. [Google Scholar] [CrossRef]
- Mhada, K.; Braikat, B.; Hu, H.; Damil, N.; Potier-Ferry, M. About macroscopic models of instability pattern formation. Int. J. Solids Struct. 2012, 49, 2978–2989. [Google Scholar] [CrossRef]
- Salem, T.; Jiao, P.; Zaabar, I.; Li, X.; Zhu, R.; Lajnef, N. Functionally graded materials beams subjected to bilateral constraints: Structural instability and material topology. Int. J. Mech. Sci. 2021, 194, 106218. [Google Scholar] [CrossRef]
- Yang, Z.; Zhao, S.; Yang, J.; Liu, A.; Fu, J. Thermomechanical in-plane dynamic instability of asymmetric restrained functionally graded graphene reinforced composite arches via machine learning-based models. Compos. Struct. 2023, 308, 116709. [Google Scholar] [CrossRef]
- Yang, Z.; Liu, A.; Lai, S.; Safaei, B.; Lv, J.; Huang, Y.; Fu, J. Thermally induced instability on asymmetric buckling analysis of pinned-fixed FG-GPLRC arches. Eng. Struct. 2022, 250, 113243. [Google Scholar] [CrossRef]
- Wu, Z.; Zhang, S.; Xiao, Y.; Liu, Z.; Ren, X. Buckling and wrinkling of sandwich structures reinforced by functionally graded carbon nanotubes. J. Aerosp. Eng. 2023, 36, 04022127. [Google Scholar] [CrossRef]
- Chawla, H.; Singh, S.B. Stability and failure characterization of fiber reinforced pultruded beams with different stiffening elements, part 2: Analytical and numerical studies. Thin-Walled Struct. 2019, 141, 606–626. [Google Scholar] [CrossRef]
- Singh, S.B.; Chawla, H. Stability and failure characterization of fiber reinforced pultruded beams with different stiffening elements, Part I: Experimental investigation. Thin-Walled Struct. 2019, 141, 593–605. [Google Scholar] [CrossRef]
- Pozorski, Z.; Pozorska, J.; Kreja, I.; Smakosz, Ł. On wrinkling in sandwich panels with an orthotropic core. Materials 2021, 14, 5043. [Google Scholar] [CrossRef] [PubMed]
- Pozorski, Z.; Wojciechowski, S. The influence of symmetrical boundary conditions on the structural behaviour of sandwich panels subjected to torsion. Symmetry 2020, 12, 2093. [Google Scholar] [CrossRef]
- Wang, M.; Jin, L.; Fu, Y. Axisymmetric necking versus Treloar–Kearsley instability in a hyperelastic sheet under equibiaxial stretching. Math. Mech. Solids 2022, 27, 1610–1631. [Google Scholar] [CrossRef]
- Wang, M.; Fu, Y. Necking of a hyperelastic solid cylinder under axial stretching: Evaluation of the infinite-length approximation. Int. J. Eng. Sci. 2021, 159, 103432. [Google Scholar] [CrossRef]
- Sabri, F.; Meguid, S.A. Wrinkling prediction of laminated composite panels under in-plane shear deformation. Acta Mech. 2020, 232, 57–72. [Google Scholar] [CrossRef]
- Huang, J.; Jin, J.; Deng, L.; Wang, X.; Gong, P.; Zhang, M.; Gao, C. Theoretical prediction of flange wrinkling in the first-pass conventional spinning of dual-metal sheets. J. Manuf. Process. 2021, 62, 368–377. [Google Scholar] [CrossRef]
- He, Q.; Li, W.; Wan, M.; Li, C.; Cui, C. Efficient approach to plastic wrinkling prediction for multi-intersecting stiffened panels in spherical die forming. Thin-Walled Struct. 2022, 173, 108967. [Google Scholar] [CrossRef]
- Li, X.; Guo, L.; Wang, F. On a plastic instability criterion for ultra-large radial-axial ring rolling process with four guide rolls. Chin. J. Aeronaut. 2022, 35, 391–406. [Google Scholar] [CrossRef]
- Li, X.; Guo, L.; Wang, Y.; Liang, L. Rolling paths design assisted by target-temperature driven intelligent FE simulation of radial-axial ring rolling. Manuf. Rev. 2019, 6, 20. [Google Scholar] [CrossRef]
- Miyajima, Y.; Yamada, T.; Fujii, T. Plastic instability criterion based on new necking parameters for Cu-Al, Cu-A5052, and Cu-A5083 roll-bonded laminated metal composites fabricated without post-annealing. J. Mater. Process. Technol. 2022, 306, 117634. [Google Scholar] [CrossRef]
- Malik, A.; Wang, Y.; Huanwu, C.; Nazeer, F.; Ahmed, B.; Khan, M.A.; Mingjun, W. Constitutive analysis, twinning, recrystallization, and crack in fine-grained ZK61 Mg alloy during high strain rate compression over a wide range of temperatures. Mater. Sci. Eng. A 2020, 771, 138649. [Google Scholar] [CrossRef]
- Malik, A.; Yangwei, W.; Huanwu, C.; Khan, M.A.; Nazeer, F.; Rui, A.; Jiawei, B.; Mingjun, W. Fracture behavior of twin induced ultra-fine grained ZK61 magnesium alloy under high strain rate compression. J. Mater. Res. Technol. 2019, 8, 3475–3486. [Google Scholar] [CrossRef]
- Malik, A.; Yangwei, W.; Huanwu, C.; Nazeer, F.; Khan, M.A.; Mingjun, W. Microstructural evolution of ultra-fine grained Mg-6.62Zn-0.6Zr alloy on the basis of adiabatic rise in temperature under dynamic loading. Vacuum 2019, 168, 108810. [Google Scholar] [CrossRef]
- Chen, X.; Si, Y.; Bai, R.; Zhang, X.; Li, Z. Hot formability study of Cr5 alloy steel by integration of FEM and 3D processing maps. Materials 2022, 15, 4801. [Google Scholar] [CrossRef]
- Wu, R.; Li, M.; Hu, Q. Evaluation and simulation on deformation instability mechanism in laminated sheet incremental forming. Procedia Manuf. 2019, 29, 36–44. [Google Scholar] [CrossRef]
- Shuai, Y.; Wang, X.; Feng, C.; Zhu, Y.; Wang, C.; Sun, T.; Han, J.; Cheng, Y. A novel strain-based assessment method of compressive buckling of X80 corroded pipelines subjected to bending moment load. Thin-Walled Struct. 2021, 167, 108172. [Google Scholar] [CrossRef]
- Nieto-Fuentes, J.C.; Jacques, N.; Marvi-Mashhadi, M.; N’souglo, K.E.; Rodríguez-Martínez, J.A. Modeling dynamic formability of porous ductile sheets subjected to biaxial stretching: Actual porosity versus homogenized porosity. Int. J. Plast. 2022, 158, 103418. [Google Scholar] [CrossRef]
- Dal, S.R.; Darendeliler, H. Analysis of side-wall wrinkling in deep drawing processes. Key Eng. Mater. 2022, 926, 732–743. [Google Scholar] [CrossRef]
- Więckowski, W.; Motyka, M.; Adamus, J.; Lacki, P.; Dyner, M. Numerical and experimental analysis of titanium sheet forming for medical instrument parts. Materials 2022, 15, 1735. [Google Scholar] [CrossRef]
- Chen, K.; Carter, A.J.; Korkolis, Y.P. Flange wrinkling in deep-drawing: Experiments, simulations and a reduced-order model. J. Manuf. Mater. Process. 2022, 6, 76. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, W.; Luo, Q.; Zheng, G.; Li, Q.; Sun, G. A novel failure criterion based upon forming limit curve for thermoplastic composites. Compos. Part B Eng. 2020, 202, 108320. [Google Scholar] [CrossRef]
- Du, B.; Wang, X.; Wang, J.; Yang, H.; Chen, X.; Yang, Z. The establishment of critical wrinkling judgment line for shear wrinkling instability of thin plate and its influencing factors. Thin-Walled Struct. 2022, 175, 109226. [Google Scholar] [CrossRef]
- Lin, G.; Li, F.; Zhang, Q.; Chen, P.; Sun, W.; Saikov, I.; Shcherbakov, V.; Alymov, M. Dynamic instability of fiber composite cylindrical shell with metal liner subjected to internal pulse loading. Compos. Struct. 2022, 280, 114906. [Google Scholar] [CrossRef]
- Jiao, P.; Chen, Z.; Ma, H.; Ge, P.; Gu, Y.; Miao, H. Buckling behaviors of thin-walled cylindrical shells under localized axial compression loads, Part 1: Experimental study. Thin-Walled Struct. 2021, 166, 108118. [Google Scholar] [CrossRef]
- Li, J.; Qiu, W.; Huang, L.; Su, H.; Tao, H.; Li, P. Gradient electromagnetic forming (GEMF): A new forming approach for variable-diameter tubes by use of sectional coil. Int. J. Mach. Tools Manuf. 2018, 135, 65–77. [Google Scholar] [CrossRef]
- Chu, G.; Wang, Q.; Zhu, M.; Sun, L.; Ling, C.; Liu, X. Analysis on corner wrinkling for axial hydro-forging of variable-diameter tube. Int. J. Adv. Manuf. Technol. 2023, 1–10. [Google Scholar] [CrossRef]
- Chu, G.; Sun, L.; Wang, G.; Fan, Z.; Li, H. Axial hydro-forging sequence for variable-diameter tube of 6063 aluminum alloy. J. Mater. Process. Technol. 2019, 272, 87–99. [Google Scholar] [CrossRef]
- Yuan, S. Hydroforming of Variable Diameter Tubular Parts. In Modern Hydroforming Technology; Springer Nature Singapore: Singapore, 2022; Volumes 27–70. [Google Scholar]
- Haley, J.A. On the Axial Crushing and Failure of Aluminum Alloy Tubes: Experiments and Numerical Simulations. Doctoral Dissertation, The University of Texas at Austin, Austin, TX, USA, 2020. [Google Scholar] [CrossRef]
- Saritaş, F.; Bedirhanoglu, I.; Konak, A.; Keskin, M.S. Effect of seismic isolation on the performance of high-rise buildings with torsional instability. Sustainability 2022, 15, 36. [Google Scholar] [CrossRef]
- Tay, Y.Y.; Flores, P.; Lankarani, H. Crashworthiness analysis of an aircraft fuselage section with an auxiliary fuel tank using a hybrid multibody/plastic hinge approach. Int. J. Crashworthiness 2019, 25, 95–105. [Google Scholar] [CrossRef]
- Li, X.; Wang, Q.; Ma, G. Mechanical properties of a novel plastic hinge seismic fuse based on frictional energy dissipation to avoid brittle failures in beam-to-column moment-resistant joints. Arab. J. Sci. Eng. 2019, 45, 3695–3706. [Google Scholar] [CrossRef]
- Wang, X.; Zhu, S.; Xi, X. Research on crashworthiness of a civil transport aircraft. In Proceedings of the 2022 8th International Conference on Mechanical Engineering and Automation Science (ICMEAS), Wuhan, China, 14–16 October 2022; pp. 281–286. [Google Scholar]
- Mirkarimi, S.P.; Mohammadi Dehcheshmeh, E.; Broujerdian, V. Investigating the progressive collapse of steel frames considering vehicle impact dynamics. Iran. J. Sci. Technol. Trans. Civ. Eng. 2022, 46, 4463–4479. [Google Scholar] [CrossRef]
- Yuan, Y.; Wang, W.; Huang, H. Deformation mechanism analysis of steel artificial controllable plastic hinge in prefabricate frame. J. Constr. Steel Res. 2023, 201, 107735. [Google Scholar] [CrossRef]
- Yuan, Y.; Huang, H.; Ye, Y.; Li, M.; Sun, H. Performance coordination design method applied to replaceable artificial controllable plastic hinge for precast concrete beam-column joints. J. Build. Eng. 2022, 47, 103863. [Google Scholar] [CrossRef]
- Feng, D.; Cetiner, B.; Azadi Kakavand, M.R.; Taciroglu, E. Data-driven approach to predict the plastic hinge length of reinforced concrete columns and its application. J. Struct. Eng. 2021, 147, 04020332. [Google Scholar] [CrossRef]
- Feng, D.; Ren, X. Enriched force-based frame element with evolutionary plastic hinge. J. Struct. Eng. 2017, 143, 06017005. [Google Scholar] [CrossRef]
- Wei, W.; Zhang, F.; Xing, Y.; Wang, H.; Liu, R. Research on mechanical properties of origami aluminum honeycomb for automobile energy absorbing box. Materials 2022, 16, 41. [Google Scholar] [CrossRef]
- Zheng, D.; Zhang, J.; Lu, B.; Zhang, T.; Xie, J. Energy absorption performance of fully clamped curved tubes under transverse loading. Thin-Walled Struct. 2022, 175, 109240. [Google Scholar] [CrossRef]
- Zheng, D.; Zhang, J.; Lu, B.; Zhang, T. Energy absorption of fully clamped multi-cell square tubes under transverse loading. Thin-Walled Struct. 2021, 169, 108334. [Google Scholar] [CrossRef]
- Scheglov, G.A.; Lukovkin, R.O. Dynamic crushing of spacecraft landing gear with crash legs. J. Phys. Conf. Ser. 2019, 1348, 012080. [Google Scholar] [CrossRef]
- Yang, X.; Ma, J.; Sun, Y.; Yang, J. An internally nested circular-elliptical tube system for energy absorption. Thin-Walled Struct. 2019, 139, 281–293. [Google Scholar] [CrossRef]
- Yang, X.; Ma, J.; Wen, D.; Yang, J. Crashworthy design and energy absorption mechanisms for helicopter structures: A systematic literature review. Prog. Aerosp. Sci. 2020, 114, 100618. [Google Scholar] [CrossRef]
- Chiariello, A.; Orlando, S.; Vitale, P.; Linari, M.; Longobardi, R.; Di Palma, L. Development of a morphing landing gear composite door for high speed compound rotorcraft. Aerospace 2020, 7, 88. [Google Scholar] [CrossRef]
- Essa, A.; Abeyrathna, B.; Rolfe, B.; Weiss, M. A novel incremental sheet metal forming process for long and open section profiles. IOP Conf. Ser. Mater. Sci. Eng. 2022, 1238, 012080. [Google Scholar] [CrossRef]
- Agrawal, A.K.; Narayanan, R.G.; Kailas, S.V. Formability and instability evaluation of friction stir processed AA6063-T6 tubes during end forming and numerical prediction. J. Mater. Eng. Perform. 2021, 30, 973–993. [Google Scholar] [CrossRef]
- Liu, N.; Yang, H.; Li, H.; Li, Z.J. A hybrid method for accurate prediction of multiple instability modes in in-plane roll-bending of strip. J. Mater. Process. Technol. 2014, 214, 1173–1189. [Google Scholar] [CrossRef]
- Li, H.; Zhou, X.; Zhang, X. Modeling of forming instability in in-plane roll-bending process by considering multi-modal geometric micro-defects of strip. Procedia Eng. 2017, 207, 1611–1616. [Google Scholar] [CrossRef]
- Li, Z.J.; Yang, H.; Li, H.; Liu, J. Sideways movement of strip in in-plane roll-bending process with conical rollers. Adv. Mater. Res. 2010, 154–155, 1419–1422. [Google Scholar] [CrossRef]
- Chen, Y.Z.; Liu, W.; Zhang, Z.C.; Xu, Y.C.; Yuan, S.J. Analysis of wrinkling during sheet hydroforming of curved surface shell considering reverse bulging effect. Int. J. Mech. Sci. 2017, 120, 70–80. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, W.; Xu, Y.; Yuan, S. Analysis and experiment on wrinkling suppression for hydroforming of curved surface shell. Int. J. Mech. Sci. 2015, 104, 112–125. [Google Scholar] [CrossRef]
- Nahak, B.; Kumar, A.; Yadav, A. Investigation of deformation behaviour of steel, aluminium and copper alloys during hydro-mechanical drawing. Arch. Mech. Eng. 2022, 69, 1–15. [Google Scholar] [CrossRef]
- Liang, Z.; Liu, Y.; Zou, T.; Li, D.; Ding, S.; Xiao, H.; Shi, L.; Peng, Y. Analysis and suppression of flange wrinkling in AHSS chain-die forming channels with a curved axis. J. Manuf. Process. 2021, 71, 70–84. [Google Scholar] [CrossRef]
- Tanaka, Y.; Wang, Z.G.; Hakoyama, T. Finding the pad load to suppress wrinkling in free bending of ultra-high tensile strength steel sheets. CIRP Ann. 2022, 71, 213–216. [Google Scholar] [CrossRef]
- Atxaga, G.; Arroyo, A.; Canflanca, B. Hot stamping of aerospace aluminium alloys: Automotive technologies for the aeronautics industry. J. Manuf. Process. 2022, 81, 817–827. [Google Scholar] [CrossRef]




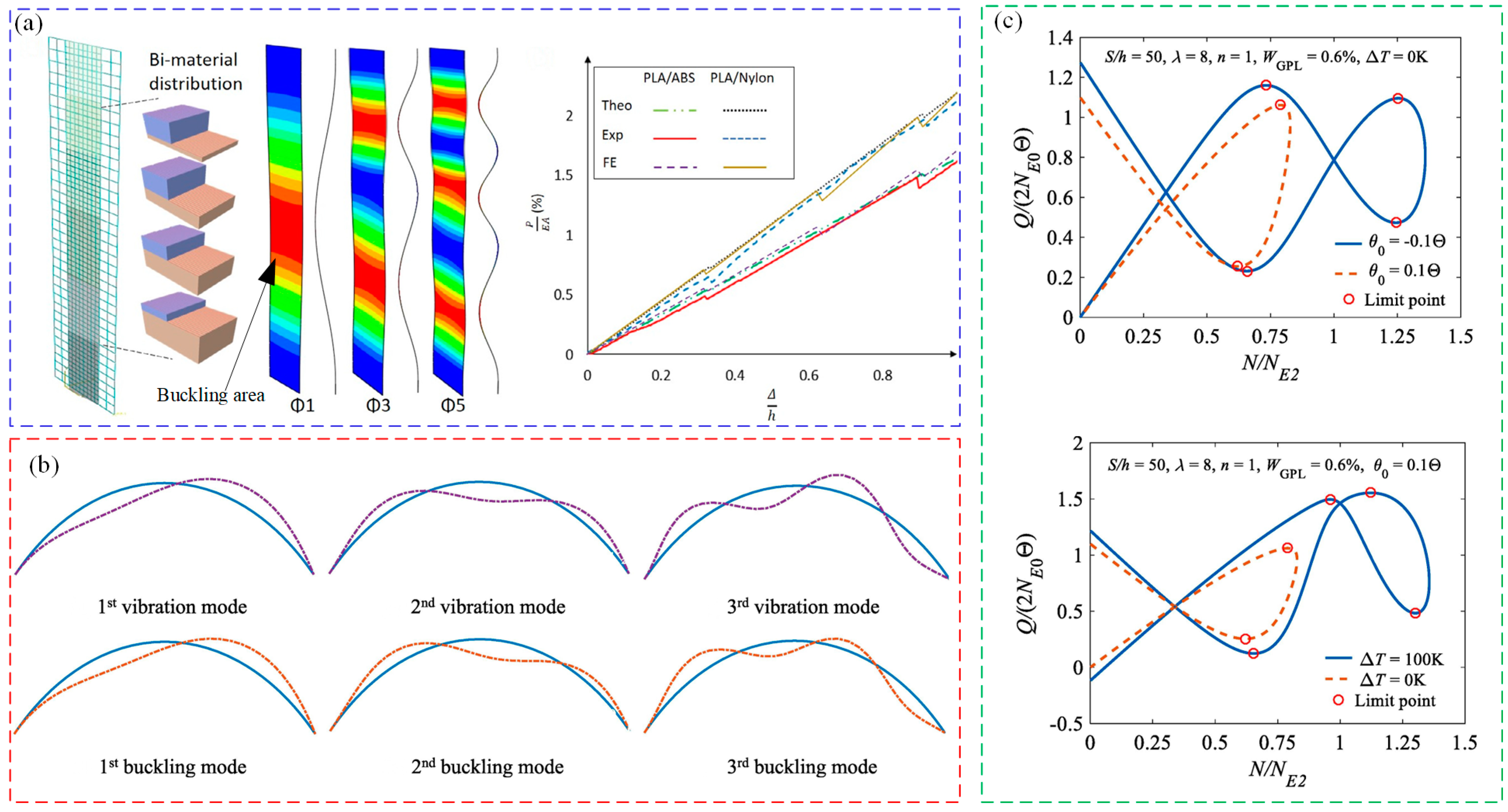




| Authors | Materials | Modified M–K Model | Representative Figures |
|---|---|---|---|
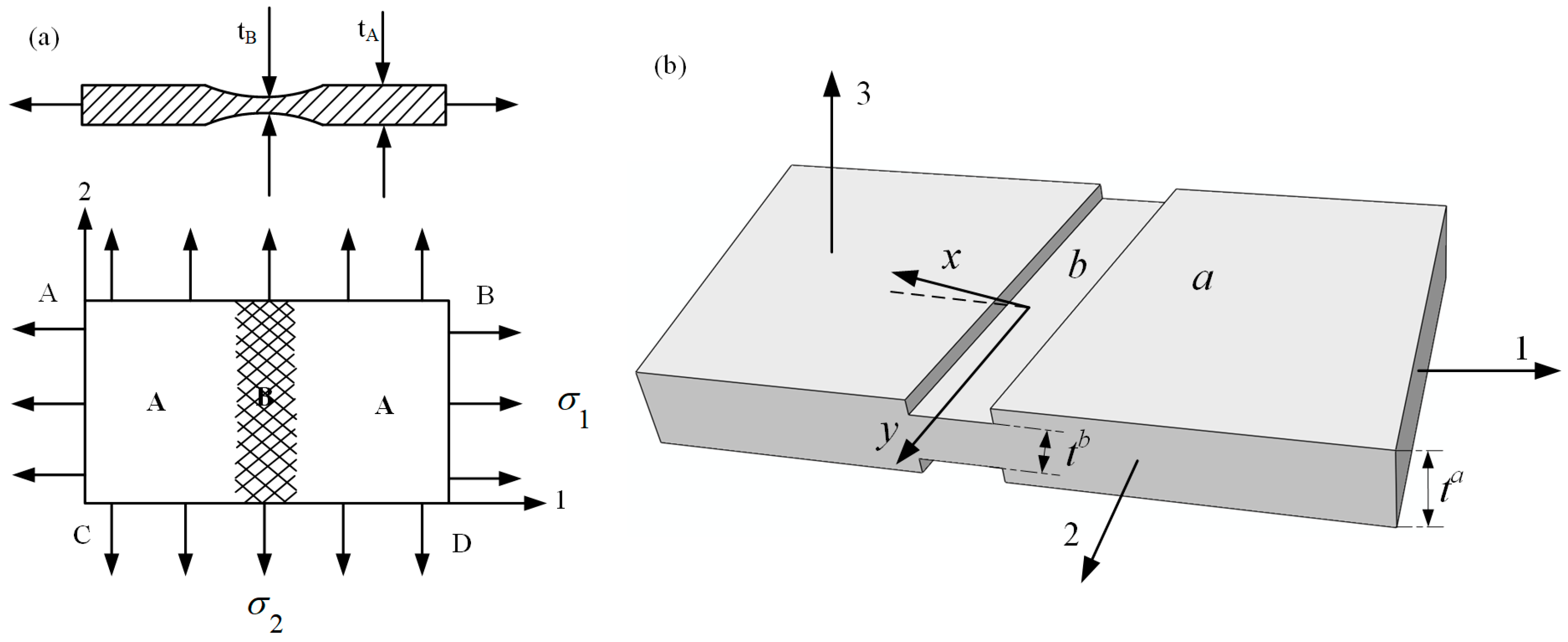
| Wang et al. [35,36] | 6061 Aluminum Alloy | where , , n is real-time strain hardening exponents, and A and B are zone-A and zone-B, respectively. | 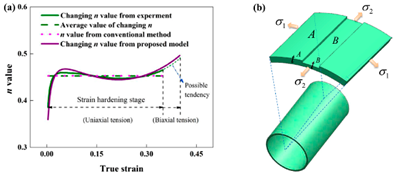 |
| Yu et al. [37,38] | AA5182O Sheet | where is critical groove angle, is strain routes, is degree of anisotropy, and is maximum r-value. |  |
| Hyuk et al. [39] | Ferritic Stainless Steel (FSS) Sheets | where t is thick, t0 is initial thick, R is surface roughness, and and are the stresses of REV-A and B, respectively. | 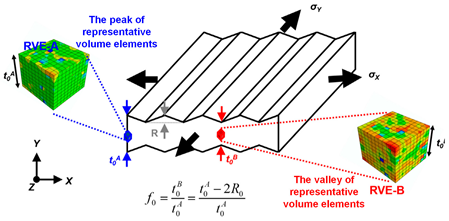 |
| Wang et al. [40,41,42,43] | Al-Mg-Li Alloy Sheet | where f0 is imperfection coefficient and and are initial thick of a and b, respectively. | 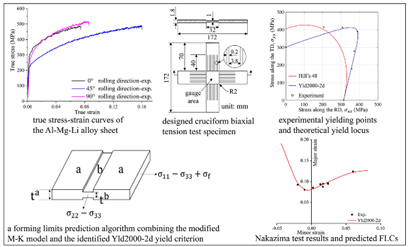 |
| Li et al. [44] | Aluminum Alloy |  | |
| Hu et al. [45,46] | AA5754 Aluminm Alloy | where ; . |  |
| He et al. [47] | AA6061-F Tube | where tmin is minimum thickness on the tube, is angle, and are the thicknesses of zone-A and zone-B, Δd is the eccentric distance of extrusion mandrel, and R1 and R2 are the radius of outer and inner profiles of the tube, respectively. | 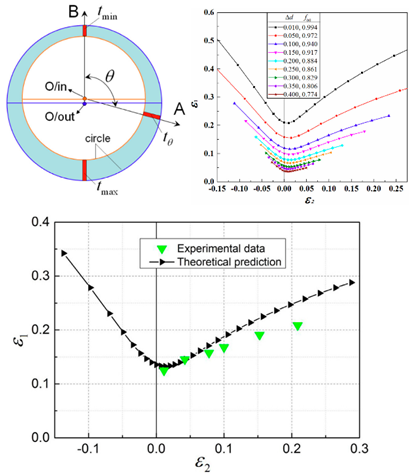 |
| Author | Year | Material | Main Points |
|---|---|---|---|
| Demirel et al. [80] | 2023 | Ti6Al4V | For high-temperature superplastic formation of Ti alloys, the main causes of deformation instability are grain boundary slip (GBS) and creep mechanisms. |
| Bobruk et al. [81] | 2023 | 2021Al | For ultrafine grained (UFG) Al alloys, according to the analysis of strain rate sensitivity, they showed stable superplastic behavior at the test temperature of 240~270 °C. |
| Myshlyaev et al. [82] | 2023 | Al-Mg-Li | The important role of intra-grain slip during superplastic flow was demonstrated through experimental analysis of strain hardening, the formation of typical deformation textures, and the increase of dislocation density within grains. Superplastic materials exhibited pronounced porosity near the instability point. |
| Mochugovskiy et al. [83] | 2023 | Al-Mg-Si-Cu | When the strain rate was low, the residual cavitation after superplastic forming was relatively large; the impurity particles inside the grains also caused the surrounding cavities to increase, which would easily lead to superplastic deformation instability. |
| Authors | Year | Material | The Conditions of Deformation Instability | |
|---|---|---|---|---|
| Temperature/°C | Strain Rate/s−1 | |||
| Shabani et al. [104] | 2023 | FeCrCuMnNi | 750~850 | 0.1, 0.01, 0.001 |
| Singh et al. [105] | 2023 | EN30B Steel | 1000~1150 | 0.1~0.8 |
| Jeong et al. [106] | 2023 | AlSi4340 Steel | 1000~1100 | 0.1, 0.2, 0.9, 1.0 |
| Azizi et al. [107] | 2023 | AlSiAA4032 | 427~527 | 0.01~0.1 |
| Yang et al. [108] | 2023 | Al4.6Mg0.2Sr | 300~400, 400~450 | 0.018~1, 0.018~0.1 |
| Lin et al. [109] | 2022 | Ti47.5Al2.5V1.0Cr0.2Zr | 1050~1140, 1180~1200 | 0.006~1 |
| Yang et al. [110] | 2022 | 215AlLi | 390~520 | 0.1~10 |
| Qiao et al. [111] | 2022 | Fe2.5Ni2.5CrAl | 1020~1100 | 0.01~1 |
| Ghosh et al. [112] | 2022 | Ti14Cr | 850~950 | 0.01 |
| Yi et al. [113] | 2022 | Al0.5Mg0.4Si0.1Cu | 350~500 | 0.316~10 |
| Authors | Materials | Types of Forming | Conditions of Instability |
|---|---|---|---|
| Yu et al. [143] | ST12 | Push bending | Gap between punch and U/O die, and excessive stock at the end of elbow causing wrinkling. |
| Tao et al. [144] | 5A02 Al Alloy | Push bending | Due to the tangential tensile stress concentration at the front end of the tube, the smaller the relative bending radius, the easier it is to have instability. |
| Xiao et al. [145] | 5A02 Al Alloy | Push bending | The stress distribution on the compression side is greater than the tension side, indicating that inner side of the tube is more prone to instability. |
| Österreicher et al. [146] | AA2024 | Three-roll-push bending | Only solution-annealed material leads to a wrinkle-free bend. |
| Cheng et al. [147] | AA6061-T6 | Free bending | When t0 < 0.8 mm, plastic instability and wrinkling occurred in the inner flange, and the smaller the wall thickness, the more obvious the wrinkling. |
| Wang, Hu and Cheng et al. [148,149,150] | Stainless Steel SS304 | Free bending | The smaller the distance between the center point of the bending die and the front end of guide, the easier it is for the tube to wrinkle. |
| Yang et al. [151] | SS304 | Free bending | The inner side of the rectangular tube is subjected to uneven compressive stress, which makes the material flow unevenly, resulting in increased wall thickness on the inner side of the tube, and wrinkled instability. |
| Authors | Equation | Explanation | |
|---|---|---|---|
| Chawla et al. [164] | (31) | are longitudinal compressive bending stress, transverse compressive stress and shear stress, respectively; , and are bending compressive strength, transverse compressive strength and shear strength, respectively. | |
| Pozorski et al. [166] | (32) | EC and GC are the modulus of elasticity and shear modulus of the isotropic core material; EF is the modulus of elasticity of the isotropic facing material. | |
| He et al. [172] | (33) | D is the plastic modulus; k is a coefficient related to the flange width and Poisson’s ratio, k = 1.5; are coefficients related to the geometrical parameters of the material; are the coefficients representing the increase in the moment of inertia caused by the shift of the neutral surface after stiffening; . | |
| Li et al. [173] | (34) | is the maximum section bending moment; Wy is section modulus in bending; h and b are the width and height of the section, respectively; Rt is the radius; is section bending moment factor; is guide forces. | |
| (35) | is equivalent strain; h and s are hard and soft floor, respectively; B < 0 indicates necking progression, larger absolute values. | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wan, M.; Li, F.; Yao, K.; Song, G.; Fan, X. Theory, Method and Practice of Metal Deformation Instability: A Review. Materials 2023, 16, 2667. https://doi.org/10.3390/ma16072667
Wan M, Li F, Yao K, Song G, Fan X. Theory, Method and Practice of Metal Deformation Instability: A Review. Materials. 2023; 16(7):2667. https://doi.org/10.3390/ma16072667
Chicago/Turabian StyleWan, Miaomiao, Fuguo Li, Kenan Yao, Guizeng Song, and Xiaoguang Fan. 2023. "Theory, Method and Practice of Metal Deformation Instability: A Review" Materials 16, no. 7: 2667. https://doi.org/10.3390/ma16072667
APA StyleWan, M., Li, F., Yao, K., Song, G., & Fan, X. (2023). Theory, Method and Practice of Metal Deformation Instability: A Review. Materials, 16(7), 2667. https://doi.org/10.3390/ma16072667