Construction of 2-2 Type Cement-Based Piezoelectric Composites’ Mechanic–Electric Relationship Based on Strain Rate Dependence
Abstract
:1. Introduction
2. Methods
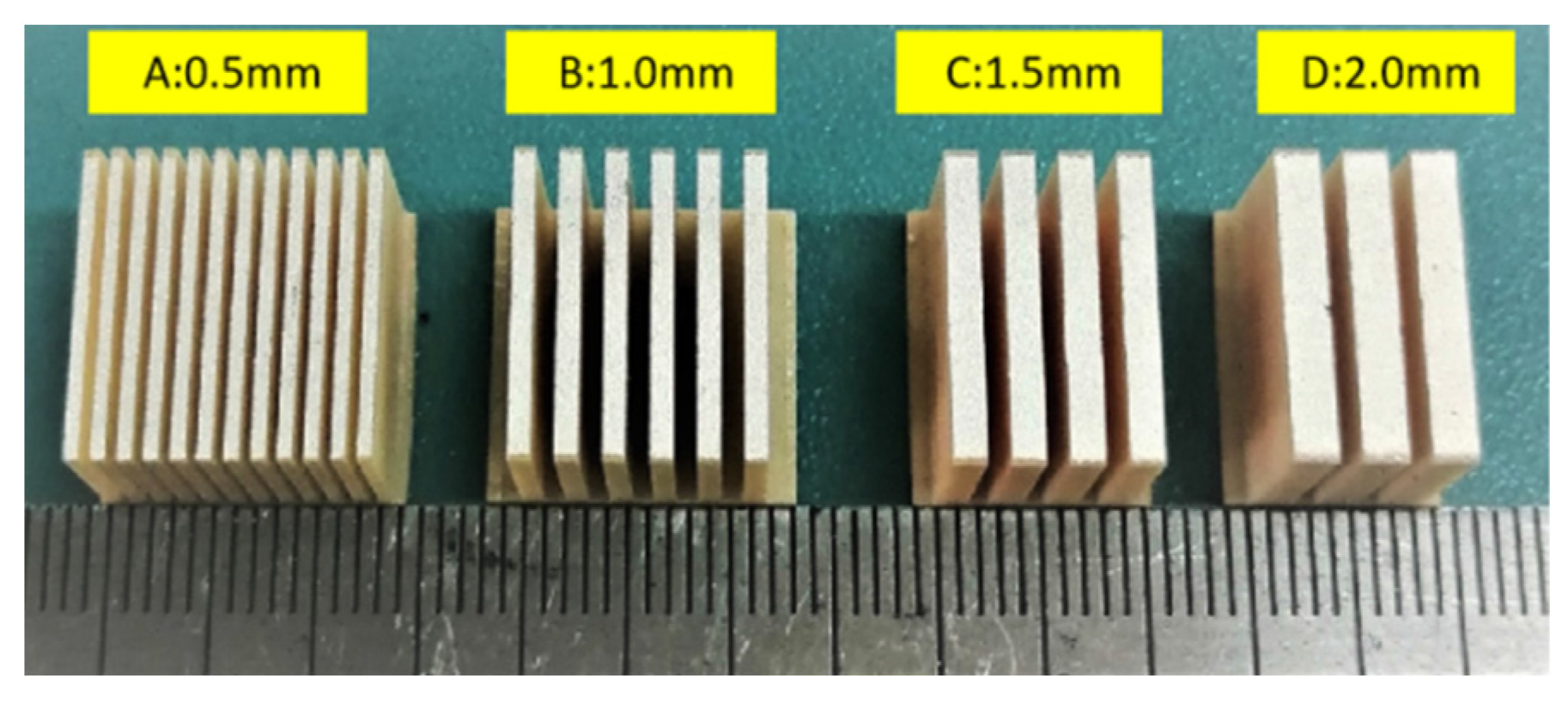
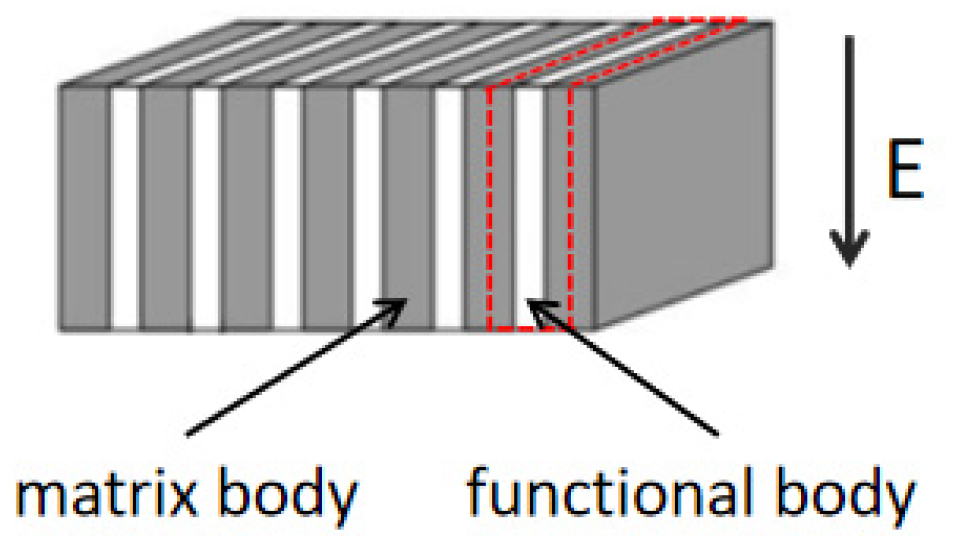
2.1. Sample Preparation
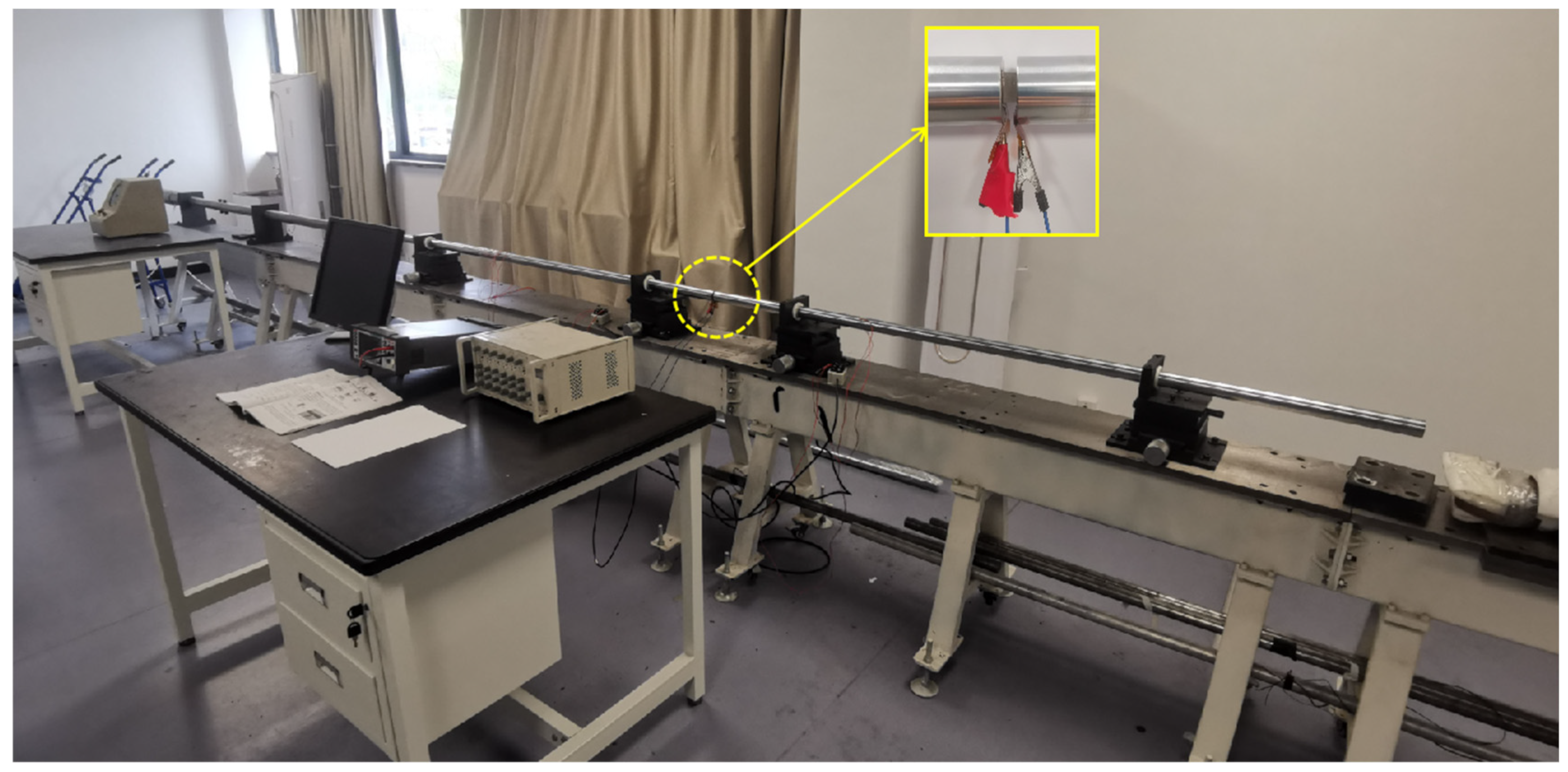
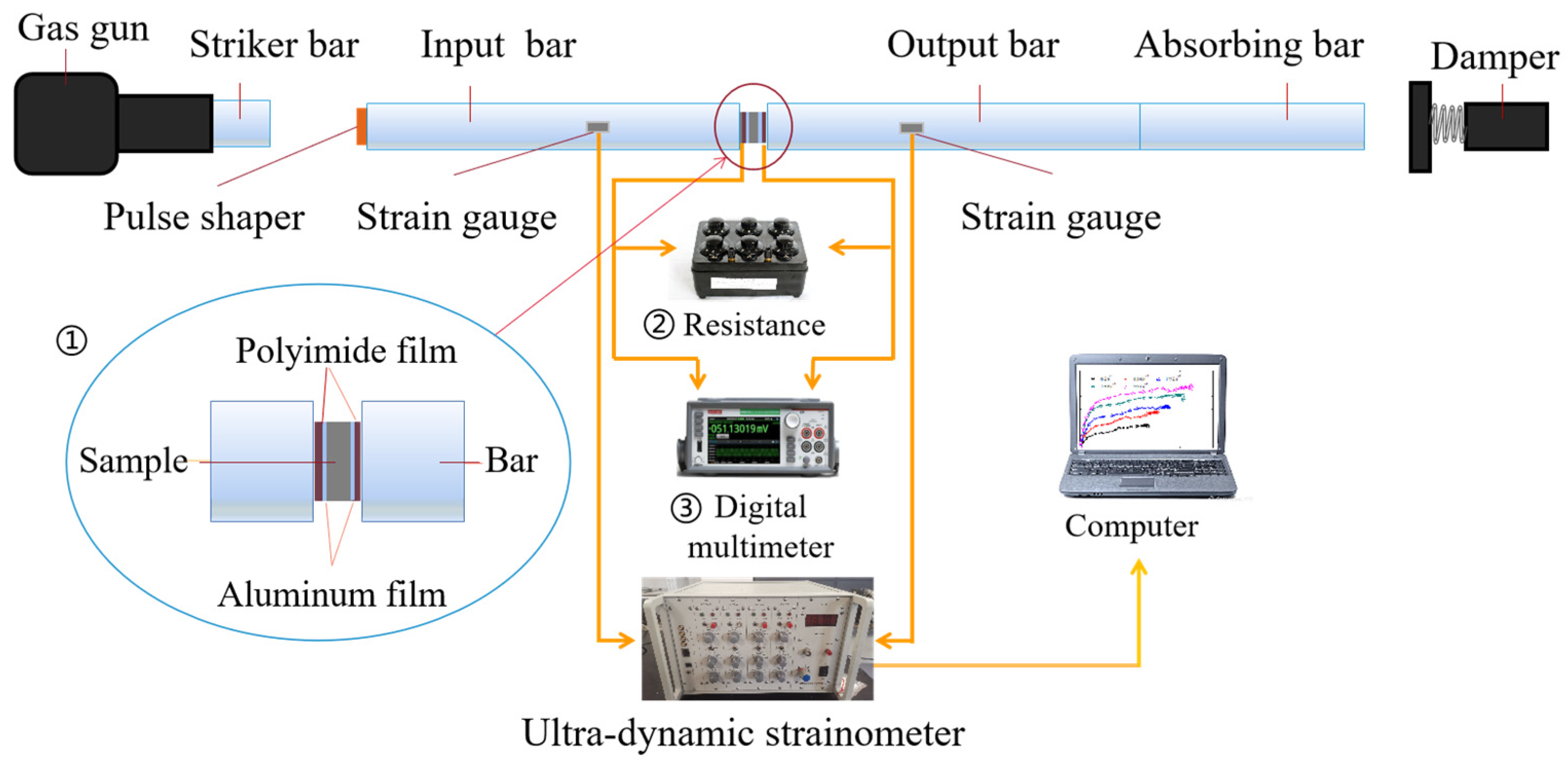
2.2. Test Method
3. Results and Discussion
3.1. Damage Evolution
3.2. Enhancement Effect of Piezoelectric Ceramics
3.3. Dynamic Damage Physical Equation and Verification Analysis
3.4. Verification of the Dynamic Stress–Strain Relationship
3.5. Mechanical and Electrical Response Characteristics
4. Conclusions
- (1)
- The compressive strength of the sample increased with an increase in the loading strain rate, and the material had an obvious strain rate effect.
- (2)
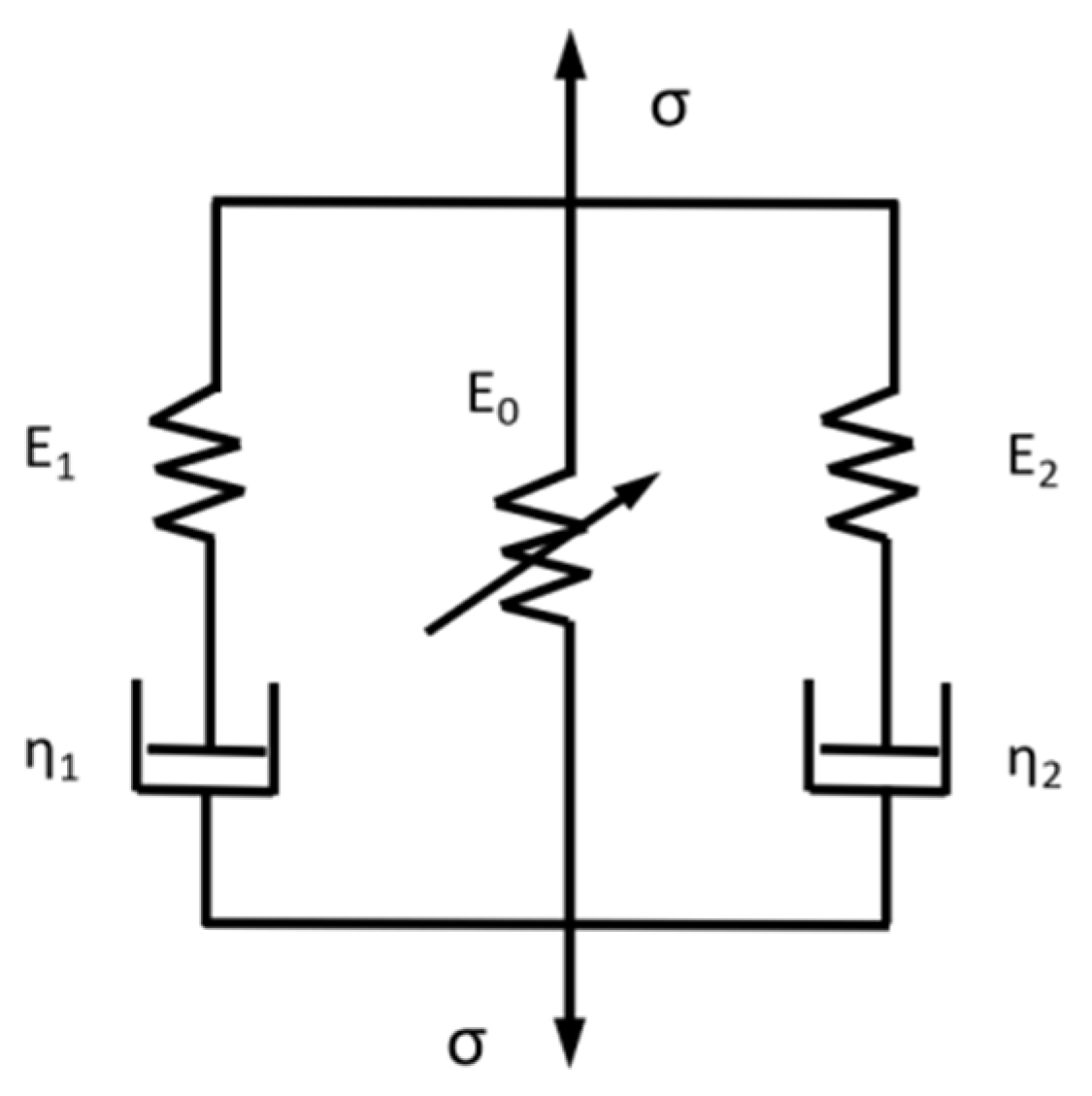
- It was assumed that the damage occurred only inside the cement paste. The modified nonlinear viscoelastic ZWT equation and the nonlinear stress–electric displacement equation of the 2-2 type cement-based piezoelectric composite were established.
- (3)
- The stress–strain curves of the 2-2 type cement-based piezoelectric composites under different strain rates were obtained using the SHPB device. The experimental results were fitted using the modified ZWT equation, and the fitting parameters of the equation were determined. With the strengthening coefficient G considering the piezoelectric ceramic term, the fitting result showed that the G value was <1 (=0.9780). The results show that piezoelectric ceramics have a certain weakening effect on the stress–strain behavior of a cement matrix when the strain rate is in the range of 150–450 S−1.
- (4)
- The stress–electric displacement curve was measured using an additional electrical signal acquisition device, and the dynamic sensitivity and linear threshold of the sample were obtained by data fitting. We compared four samples A–D; the linear sensitivity of sample B was 343 pC/N, and the linear threshold value of sample C was 85 MPa, which were the largest. The curve after the linear threshold was a nonlinear response curve of a cubic polynomial. Therefore, the nonlinear relationship of the strain–stress–electric displacement of 2-2 type cement-based piezoelectric composites was established.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tasuji, M.E. Stress-stain Response and Fracture of Concrete under Multiaxial Conditions. ACI J. Proc. 1978, 75, 306–312. [Google Scholar]
- Chen, J.K.; Jiang, M.Q. Long-term evolution of delayed ettringite and gypsum in Portland cement mortars under sulfate erosion. Constr. Build. Mater. 2008, 23, 812–816. [Google Scholar]
- Zhao, X.Y. Structural Health Monitoring and Damage Detection Based on Piezoelectric Ceramic Transducers; Dalian University of Technology: Dalian, China, 2008. [Google Scholar]
- Marc, K.; Michael, R. Diagnosis of concrete structures: The influence of sampling parameters on the accuracy of chloride profiles. Mater. Struct. 2018, 51, 75. [Google Scholar]
- Farrar, C.R.; Worden, K. An introduction to structural health monitoring. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2007, 365, 303–315. [Google Scholar]
- Brunner, A.J.; Barbezat, M.; Huber, C.P.H. The potential of active fiber composites made from piezoelectric fibers for actuating and sensing applications in structural health monitoring. Mater. Struct. 2005, 38, 561–567. [Google Scholar]
- Roh, Y.S.; Chang, F.K. Built in Diagnostics for Identifying an Anomaly in Plates Using Wave Scattering; California Stanford University: Stanford, CA, USA, 1999; pp. 44–68. [Google Scholar]
- Liang, C.; Sun, F.P.; Rogers, C.A. An Impedance Method for Dynamic Analysis of Active Material Systems. J. Intell. Mater. Syst. Struct. 1997, 8, 323–334. [Google Scholar]
- Ou, J.P.; Han, B.G. Piezoresistive Cement-based Strain Sensors and Self-sensing Concrete Components. Intell. Mater. Syst. Struct. 2009, 20, 329–336. [Google Scholar]
- Sha, F.; Xu, D.; Huang, S. Mechanical Sensing Properties of Embedded Smart Piezoelectric Sensor for Structural Health Monitoring of Concrete. Res. Nondestruct. Eval. 2021, 32, 88–112. [Google Scholar]
- Sikora, P.; Chung, S.Y. Cement-Based Composites: Advancements in Development and Characterization. Crystals 2020, 10, 832. [Google Scholar]
- Newnham, R.E.; Skinner, D.P.; Cross, L.E. Connectivity and Piezoelectric-Pyroelectric Composite. Mater. Res. Bull. 1978, 13, 525–536. [Google Scholar]
- Li, Z.J.; Zhang, D.; Wu, K.R. Cement matrix 2-2 piezoelectric composite-Part 1. Sensory effect. Mater. Struct. 2001, 34, 506–512. [Google Scholar]
- Zhang, D.; Wu, K.R.; Li, Z.J. Study on 2-2 Cement Based Piezoelectric Composite. Piezoelectr. Acoustoopt. 2022, 24, 217–220+231. [Google Scholar]
- Chen, X. Cement-Based Piezoelectric Composites and Their Applications; Science Press: Beijing, China, 2018. [Google Scholar]
- Pan, H.H.; Lai, T.Z.; Chaipanich, A.; Wittinanon, T. Effect of graphene on the piezoelectric properties of cement-based piezoelectric composites. Sens. Actuators A Phys. 2022, 346, 113882. [Google Scholar]
- Aodkeng, S.; Sinthupinyo, S.; Chamnankid, B.; Hanpongpun, W.; Chaipanich, A. Effect of carbon nanotubes/clay hybrid composite on mechanical properties, hydration heat and thermal analysis of cement-based materials. Constr. Build. Mater. 2022, 320, 126212. [Google Scholar]
- Pan, H.H.; Guan, J.C. Stress and strain behavior monitoring of concrete through electromechanical impedance using piezoelectric cement sensor and PZT sensor. Constr. Build. Mater. 2022, 324, 126685. [Google Scholar]
- Lei, T.; Chen, G.; He, Y.B.; Ling, S.K. Dynamic Behavior of PZT-5 Piezoelectric Ceramics under Impact Loading. Chin. J. High Press. Phys. 2019, 5, 131–137. [Google Scholar]
- Chure, M.C.; Wu, L.; Wu, K.K.; Tung, C.C.; Lin, J.S.; Ma, W.C. Power generation characteristics of PZT piezoelectric ceramics using drop weight impact techniques effect of dimensional size. Ceram. Int. 2014, 40, 341–345. [Google Scholar] [CrossRef]
- Wang, R.Z.; Tang, E.L.; Yang, G.L.; Sun, Q.Z.; Han, Y.F.; Chen, C. Construction of PZT-5H mechano-electric model based on strain rate dependence and its numerical simulation in overload igniter application. Mech. Mater. 2021, 157, 103837. [Google Scholar]
- Han, R.; Shi, Z.F. Dynamic analysis of sandwich cement-based piezoelectric composites. Compos. Sci. Technol. 2012, 72, 894–901. [Google Scholar]
- Zhang, T.; Zhang, K.; Liu, W.; Liao, Y. Impact Mechanical Response of a 2-2 Cement-Based Piezoelectric Sensor Considering the Electrode Layer Effect. Sensors 2017, 17, 2035. [Google Scholar]
- Zhang, F.; Feng, P.J.; Wang, T.; Chen, J.Y. Mechanical-electric response characteristics of 1–3 cement based piezoelectric composite under impact loading. Constr. Build. Mater. 2019, 228, 116781. [Google Scholar] [CrossRef]
- Xu, X.Y.; Zhou, W. Study on dynamic properties of 1-3 cement-based piezoelectric composites. Constr. Build. Mater. 2022, 316, 125797. [Google Scholar] [CrossRef]
- Hopkinson, B. A method of measuring the pressure produced in the detonation of high explosives or by the impact of bullets. Proc. R. Soc. Lond. A Contain. Pap. Math. Phys. Character 1914, 89, 437–456. [Google Scholar]
- Kolsky, H. An investigation of the mechanical properties of materials at very high rates of loading. Proc. Phys. Soc. Sect. B 1949, 62, 676–700. [Google Scholar] [CrossRef]
- Wang, L.L.; Hu, S.S.; Yang, L.M.; Dong, X.L.; Wang, H. Talk about dynamic strength and damage evolution. Explos. Shock Waves 2017, 37, 169–179. [Google Scholar]
- Mohammed, A.; Pankaj; Kaushik, S.K. Mechanical behavior of ferrocement composites: An experimental investigation. Cem. Concr. Compos. 1992, 21, 301–312. [Google Scholar]
- Ye, J.Q.; Wu, Z.J. Micro-mechanical analysis of splitting failure in concrete reinforced with fiber reinforced plastic rods. Cem. Concr. Compos. 2000, 22, 243–251. [Google Scholar] [CrossRef]
- Haselwander, B.; Jonas, W.; Riech, H. Material equations for steel fiber reinforced concrete members. Nucl. Eng. Des. 1995, 156, 235–248. [Google Scholar] [CrossRef]
- Shang, L.; Ning, J.G.; Sun, Y.X. The constitutive relationship of reinforced concrete subjected to shock loading. Acta Mech. Solid Sin. 2005, 26, 175–181. [Google Scholar]
- Curran, D.R.; Shockey, D.A.; Seaman, L. Dynamic fracture criteria for a polycarbonate. J. Appl. Phys. 1973, 44, 4025–4038. [Google Scholar] [CrossRef]
- Shen, W. Damage Mechanics; Huazhong Science and Technology Press: Wuhan, China, 1989. [Google Scholar]
- Chu, F.; Reaney, I.; Setter, N. Role of defects in the ferroelectric relaxor Lead Scandium Tantalate. J. Am. Ceram. Soc. 1995, 78, 1947–1952. [Google Scholar] [CrossRef]
- Hagimura, A.; Uchino, K. Impurity doping effect on electrostrictive properties of (Pb,Ba)(Zr,Ti)O3. Ferroelectrics 1989, 93, 373–378. [Google Scholar] [CrossRef]
- Zhou, F.H.; Wang, L.L.; Hu, S.S. A damage-modified nonlinear visco-elastic constitutive relation and failure criterion of PMMA at high strain-rates. Explos. Shock Waves 1992, 12, 333–342. [Google Scholar]
- Qian, L.; Zhang, F.; Xu, X.Y.; Chen, J.Y. Researches of PZT5 in domain switching under impact loading. J. Funct. Mater. Devices 2017, 48, 93–97. [Google Scholar]
- Khan, A.S.; Proud, W.G. Temperature and strain rate effects on the piezoelectric charge production of PZT 95/5. AIP Conf. Proc. 2017, 1793, 100037. [Google Scholar]



| 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|
| D0 | a | γ | f | EP/GPa |
| 0.02 | 0.0916 | 0.2594 | 31.14% | 60.90 |
| 6 | 7 | 8 | 9 | 10 |
| Ec/GPa | α/GPa | β/GPa | E2/GPa | θ2/μs |
| 17.00 | 316.50 | −937.13 | 22.50 | 15.03 |
| No. | Sample | Linearity Range /MPa | Linearity Sensitivity/(pC/N) | Dynamic Sensitivity Parameters | |||
|---|---|---|---|---|---|---|---|
| K1 | K2 | K3 | K4 | ||||
| 1 | A | 0–60 | 311 | 0.00734 | 0.000311 | 1.50 × 10−6 | −2.65 × 10−8 |
| 2 | B | 0–70 | 343 | 0.00731 | 0.000343 | 4.79 × 10−7 | −9.33 × 10−9 |
| 3 | C | 0–85 | 294 | 0.00829 | 0.000294 | 1.43 × 10−6 | −1.70 × 10−8 |
| 4 | D | 0–80 | 229 | 0.0139 | 0.000229 | 9.25 × 10−7 | −1.20 × 10−8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, H.; Li, Z.; Zhu, Z.; Li, Y.; Cheng, W.; Chen, J. Construction of 2-2 Type Cement-Based Piezoelectric Composites’ Mechanic–Electric Relationship Based on Strain Rate Dependence. Materials 2023, 16, 2702. https://doi.org/10.3390/ma16072702
Dong H, Li Z, Zhu Z, Li Y, Cheng W, Chen J. Construction of 2-2 Type Cement-Based Piezoelectric Composites’ Mechanic–Electric Relationship Based on Strain Rate Dependence. Materials. 2023; 16(7):2702. https://doi.org/10.3390/ma16072702
Chicago/Turabian StyleDong, Haiwei, Zhe Li, Ziye Zhu, Yi Li, Wenjie Cheng, and Jiangying Chen. 2023. "Construction of 2-2 Type Cement-Based Piezoelectric Composites’ Mechanic–Electric Relationship Based on Strain Rate Dependence" Materials 16, no. 7: 2702. https://doi.org/10.3390/ma16072702
APA StyleDong, H., Li, Z., Zhu, Z., Li, Y., Cheng, W., & Chen, J. (2023). Construction of 2-2 Type Cement-Based Piezoelectric Composites’ Mechanic–Electric Relationship Based on Strain Rate Dependence. Materials, 16(7), 2702. https://doi.org/10.3390/ma16072702





