Analysis of Critical Current Dependence on Specimen Length and Crack Size Distribution in Cracked Superconductor

Abstract

In order to describe the dependence of critical current on specimen length and crack size distribution in the superconducting tape with cracks of different sizes, a Monte Carlo simulation and a model analysis were carried out, employing the model specimens of various lengths constituted of multiple short sections with a crack per each. The model analysis was carried out to evaluate the effects of the two factors on the critical current of a specimen. Factor 1 is the size of the largest crack in a specimen, and Factor 2 is the difference in crack size among all sections at the critical voltage of critical current. Factors 1 and 2 were monitored by the smallest ligament parameter among all sections constituting the specimen and by the number of sections equivalent to the section containing the largest crack at the critical voltage of the critical current of the specimen, respectively. The research using the monitoring method revealed quantitatively that the critical current-reducing effect with increasing specimen length is caused by the increase in the size of the largest crack (Factor 1), and also, the critical current-raising effect is caused by the increase in the difference of crack size (Factor 2). As the effect of Factor 1 is larger than that of Factor 2, the critical current decreases with increasing specimen length. With the present approach, the critical current reducing and raising effects under various crack size distributions were evaluated quantitatively as a function of specimen length, and the specimen length-dependence of critical current obtained by the Monte Carlo simulation was described well.

1. Introduction

Critical current I_c (estimated by application of the electrical field criterion E_c = 1 µV/cm to the voltage V—current I curve) and n-value (estimated as the index n of the approximated I ∝ Iⁿ curve in the electrical field range of E = 0.1~10 µV/cm) are reduced under high electromagnetic and mechanic stresses, as have been reported for the superconducting oxide (such as RE(Y, Sm, Dy, Gd,···)Ba₂Cu₃O_7-δ: REBCCO) layer-coated tape [1,2,3,4,5,6,7,8,9,10,11,12,13,14], and Bi2223 [15,16,17,18,19,20]-, MgB₂ [21,22,23,24,25]- and Nb₃Sn [26,27,28,29,30,31]-filamentary types. In this work, we conduct a fundamental study on the influences of cracks in superconducting layer-coated tape on I_c. Usually, the cracking takes place non-uniformly, and hence, the I_c/n-values vary by specimen [3,7,13,18] and along the length of the specimen [7,13,14]. Not limited to the stress-induced cracks, the non-uniformly distributed defects introduced during the fabrication process also cause a reduction in I_c and n-values [11,13]. For safety design, it is required to reveal the relation of non-uniformly distributed defects/cracks to superconducting property [32].

In studying the effect of crack size distribution and specimen length on I_c and n-values, the authors used a Monte Carlo simulation method [13,14] combined with a current shunting model of cracks [17]. From the simulation results using the model specimens consisting of multiple short sections with cracks of different sizes, the following results have been obtained: (i) The section with the largest crack contributes most significantly to the synthesis of the V–I curve of the specimen. (ii) The I_c-value of a specimen is determined by the size of the largest crack in the specimen as a first approximation when the specimen is short but not when the specimen is long [9]. The size of the largest crack determines the I_c value of the specimen as a first approximation when the specimen is short but not when the specimen is long [13]. This result suggests that the I_c value is determined not only by the effect of the largest crack but also by an effect whose contribution to I_c becomes greater the longer the specimen.

Motivated by the results above, we attempted to describe the specimen length-dependence of the I_c value based on the effects of two factors. Factor 1 is the size of the largest crack in the specimen. Factor 2 is the difference in size among cracks at the critical voltage of the critical current of the specimen. As the research tools, we used the monitoring method for numerical estimation of the effect of Factor 1 and Factor 2, together with the Monte Carlo simulation method [13,14] and Gumbel’s extreme value distribution function [33].

2. Materials and Methods

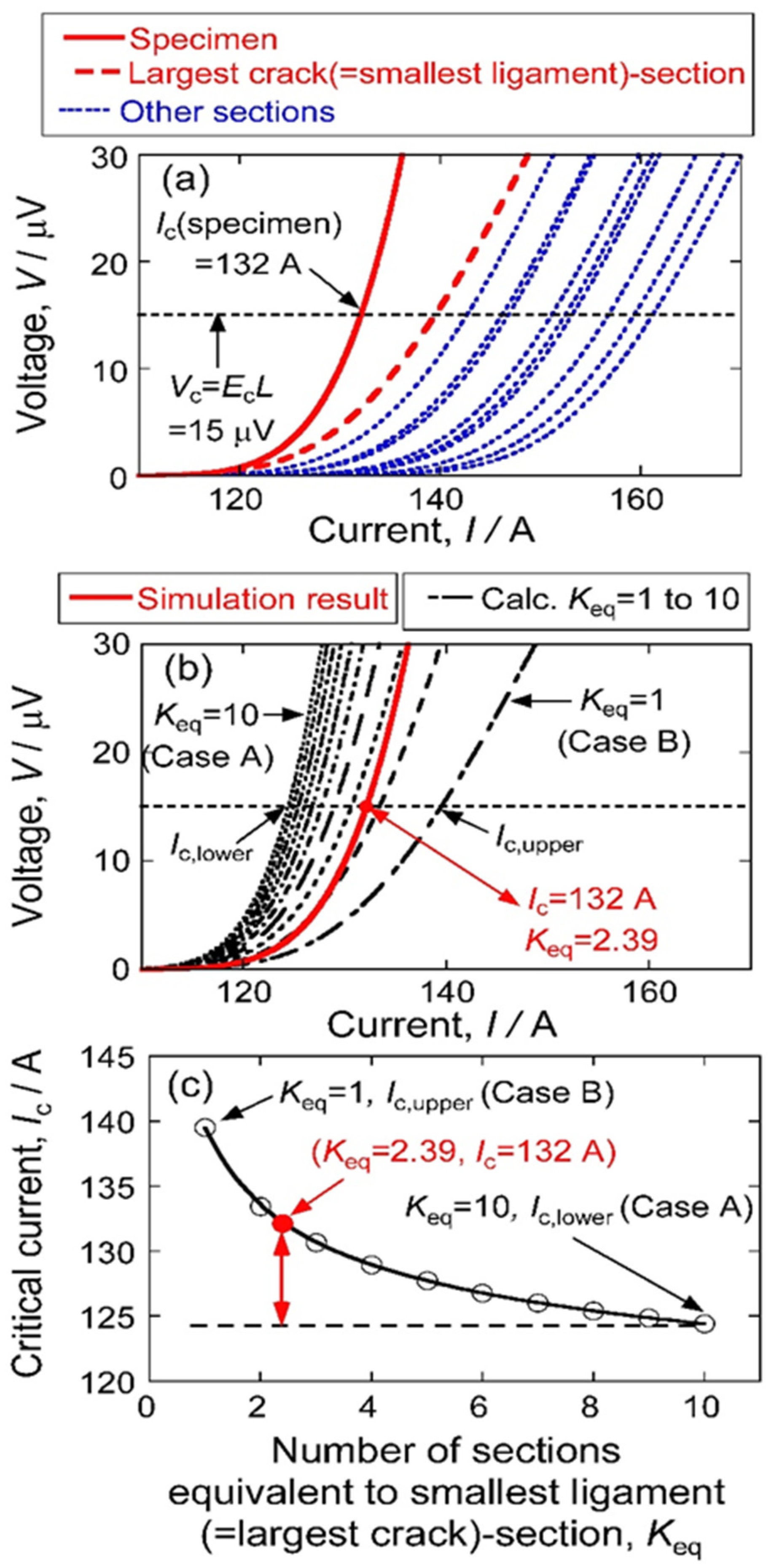
2.1. Simulation to Obtain Critical Current Values under Various Specimen Lengths and Various Distribution Widths of Crack Size

Figure 1 shows (a) a model specimen of a superconducting layer such as REBCCO-layer-coated tape, containing N local sections with a length L₀ = 1.5 cm and one crack per each, (b) heterogeneously cracked superconducting layer, (c) extreme Case A, (d) extreme Case B and (e) array of the largest crack in the intermediate case between Cases A and B, which were used as the tools to estimate the effects of Factor 1 and Factor 2 on I_c. as follows:

The simulation was carried out in the following procedure. Then, the simulation results were analyzed to clarify the effect of Factor 1 (size of the largest crack) and Factor 2 (difference of crack size at the critical voltage of critical current of the specimen) on the I_c of the specimen by applying the method and procedure in Section 2.2.

The following technical terms were used. “f and 1 − f: ratios of cross-sectional area of the cracked- and ligament-parts to the total cross-sectional area of the superconducting layer, respectively”, “I_RE: current transported by the superconducting layer in the ligament part”, “I_s: crack-induced shunting current”, “R_t: electrical resistance of the shunting circuit”, “V_RE: voltage developed at the ligament part that transports current I_RE, being equal to the voltage vs. (=I_sR_t) developed at the cracked part by shunting current I_s, since the cracked part and the ligament part constitute a parallel electrical circuit”, “I_c0 and n₀: critical current and n-value of the sections in the non-cracked state, respectively”, “E_c (=1 μV/cm): critical electrical field for determination of the critical current value”, “s (<<L₀): current transfer length”, “L_p: ligament parameter of section, given by L_p = (1 − f)(L₀/s)^1/n0, which was derived by the authors [13,14] through a modification of the formulations of Fang et al. [17]”, and “ΔL_p: standard deviation of the ligament parameter L_p”.

The cross-sectional area of ligament 1 − f and crack f have a one-to-one relation; the smaller the ligament, the larger the crack. The ligament parameter L_p, which is proportional to 1 − f, was used as a monitor of 1 − f and f. Accordingly, the size of the largest crack is expressed by the smallest ligament parameter L_p,smallest. The standard deviation of 1 − f and f is the same. Thus, the standard deviation ΔL_p of the ligament area fraction 1 − f is the same as that of the crack area fraction f. Hence, the standard deviation of the ligament parameter, ΔL_p, was used as a monitor of the distribution width of crack size and ligament size. As well as that of crack size, the larger the ΔL_p value, the wider the distribution of both the crack size- and ligament size.

The distribution of L_p was formulated by the normal distribution function as an example. Noting the average of L_p values as L_p,ave, the cumulative probability F(L_p) and density probability f(L_p) are expressed by:

$$F(L_{\mathrm{p}})=\frac{1}{2}\left\{1+\mathrm{erf}\left(\frac{L_{\mathrm{p}}-L_{\mathrm{p},\mathrm{ave}}}{\sqrt{2}\Delta L_{\mathrm{p}}}\right)\right\}$$

(1)

$$f(L_{\mathrm{p}})=\frac{1}{\sqrt{2\pi }\Delta L_{\mathrm{p}}}\exp \left\{-\frac{(L_{\mathrm{p}}-L_{\mathrm{p},\mathrm{ave}}){}^2}{2(\Delta L_{\mathrm{p}}){}^2}\right\}$$

(2)

In the Monte Carlo simulation, the L_p value for each section was set by the following process. The L_p,ave was taken to be 0.667, which refers to a situation where the average crack size is ≈1/3. The standard deviation of L_p, ΔL_p was set to be 0.01, 0.025, 0.05, 0.10, and 0.15. Using the values mentioned above, the L_p value was given for each cracked section by generating a random value in the range of 0~1, setting F(L_p) = generated random value, and substituting the values of L_p,ave (=0.667) and ΔL_p (0.01–0.15) in Equation (1).

For the section without crack, the voltage (I)—current (I) relation is expressed by:

$$V=E_{\mathrm{c}}{L}_0\left(\frac{I}{I_{\mathrm{c}0}}\right){}^{n_0}$$

(3)

The transport current in a cracked section is the sum (I = I_s + I_RE) of the shunting current I_s in the cracked part and superconducting layer (for instance, REBCO)-transported current I_RE in the ligament part. The V–I curve of the cracked section is expressed as:

$$V=E_{\mathrm{c}}{L}_0\left(\frac{I}{I_{\mathrm{c}0}}\right){}^{n_0}+V_{\mathrm{RE}}$$

(4)

$$I=I_{\mathrm{RE}}+I_{\mathrm{s}}=I_{\mathrm{c}0}{L}_{\mathrm{p}}\left[\frac{V_{\mathrm{RE}}}{E_{\mathrm{c}}{L}_0}\right]{}^{1/n_0}+\frac{V_{\mathrm{RE}}}{R_{\mathrm{t}}}$$

(5)

We calculated the V–I curve of the non-cracked section by substituting L₀ = 1.5 cm, I_c0 = 200 A, and n₀ = 40 taken from the experimental result of DyBCO coated conductor [3], into Equation (3), and also V–I curve of the cracked section by substituting the L_p value given in the Monte Carlo method and the values mentioned above into Equations (4) and (5).

Since the specimen consists of a series electrical circuit of N sections (Figure 1a,b), the current I in the specimen is the same as that in all sections (Equation (6)), and the voltage of the specimen is the sum of the voltages of all sections (Equation (7)).

I = I_Si (i = 1 to N)

(6)

$$V={\displaystyle \sum _{i=1}^N{V}_{{\mathrm{S}}_i}}$$

(7)

The V–I curves of the specimens were synthesized with the V–I curves of the sections using Equations (6) and (7). Using the V–I curves of the sections and specimens, the I_c values of the sections and specimens were obtained using the electrical field criterion of E_c = 1 μV/cm.

2.2. Model Analysis of the Specimen Length (L)-Dependence of Critical Current (I_c) under Various Distribution-Widths of Crack Size (ΔL_p)

A series of sections with cracks of different sizes (Figure 1b) constructs a specimen.

With increasing applied current, the superconductivity of the specimen is lost first in the section with the largest crack since the voltage developed at the largest crack section is highest among all sections, and hence, it contributes most to the voltage of the specimen. The ligament parameter of the largest crack section is the smallest among all sections. It is noted as L_p,smallest. Factor 1 (largest crack in the specimen) was monitored by L_p,smallest. When the L_p,smallest value is known, the lower and upper bounds of critical current can be calculated using the extreme Case A and Case B [13,14], as follows.

Case A is an extreme case where the crack size is the same as the largest of all sections, as shown in Figure 1c, and the V–I curves of all sections are the same. Accordingly, the voltage of the specimen, given by the sum of the crack size of the voltages of all sections, corresponds to the upper bound of the voltage of the specimen, V_upper. As V_upper reaches V_c at the lowest current, the I_c value in Case A is the lower bound, I_c,lower.

Case B is another extreme case where the crack size of one section is far larger than that of the other sections, as shown in Figure 1d, and the voltages developed at the cracks in the other sections are too low to contribute to the voltage of the specimen. This case gives the lower bound of the voltage of specimen V_lower. As V_lower reaches V_c at the highest current, the I_c value in Case B is the higher bound, I_c,upper, under the given largest crack size, monitored by L_p,smallest.

In this way, the V_upper–I curve and I_c,lower are given by Case A, and the V_lower–I curve and I_c,upper are given by Case B due to the difference in the number of the largest cracks; N in Case A and 1 in Case B.

As shown in Figure 1b, cracks of various sizes exist in practical specimens. Accordingly, the largest crack section and the other sections contribute to the voltage of the specimen. The extent of the contribution of the other sections is seen in the positional relation among the V–I curves of the sections, reflecting the distribution-width of crack size ΔL_p; when ΔL_p is small, the V–I curves of sections exist near each other and hence the voltage of the specimen (=sum of the voltages of sections) tends to be high, while, when ΔL_p is large, the V–I curves of sections exist apart to each other and hence voltage of the specimen tends to be low. This means that the difference in the size of cracks is an important factor in determining the I_c value.

The effect of the difference in size among cracks (Factor 2) on the I_c value of the specimen can be obtained by expressing the sum of the voltages of all sections (=the voltage of the specimen) as the sum of the voltages of several K_eq sections equivalent to the largest crack-section [14] and N–K_eq sections without cracks. A large K_eq value corresponds to a small difference in crack size. In this direction, Factor 2 (size difference among cracks) is monitored by Keq; the smaller the size difference of cracks, the larger the Keq. The K_eq value can be estimated as follows.

Using the smallest ligament parameter L_p,smallest, the V–I curve of the largest crack section is expressed by Equations (8) and (9), which are the modified forms of Equations (4) and (5), respectively.

$$V\left(\mathrm{smallest}\mathrm{ligament}\text{-}\mathrm{section}\right)=E_{\mathrm{c}}{L}_0\left(\frac{I}{I_{\mathrm{c}0}}\right){}^{n_0}+V_{\mathrm{RE}}$$

(8)

$$I=I_{\mathrm{RE}}+I_{\mathrm{s}}=I_{\mathrm{c}0}{L}_{\mathrm{p},\mathrm{smallest}}\left[\frac{V_{\mathrm{RE}}}{E_{\mathrm{c}}{L}_0}\right]{}^{1/n_0}+\frac{V_{\mathrm{RE}}}{R_{\mathrm{t}}}$$

(9)

The V–I curve of the specimen, containing a number of K_eq sections equivalent to the largest crack (smallest ligament)-section and the number of N − K_eq sections without cracks, having original critical current I_c0, is expressed by:

$$V(\mathrm{specimen})=E_{\mathrm{c}}L\left(\frac{I}{I_{\mathrm{c}0}}\right){}^{n_0}+K_{\mathrm{eq}}{V}_{\mathrm{RE}}$$

(10)

The K_eq value is between 1 and N, as shown in Figure 1c–e.

Figure 2 shows the relation of K_eq to I_c and the procedure to estimate the K_eq value from the estimated values of I_c and L_p,smallest values. Figure 2a shows the V–I curves of the specimen with 10 sections (solid curve), and V–I curve of the largest crack section (broken curve), and the V–I curves of the other 9 sections whose cracks are smaller than the largest crack (dotted curves), obtained in advance by the present work. L_p,smallest, was 0.617, and I_c was 132 A in this example.

Figure 2b compares the V–I curve of the specimen obtained by simulation and the V–I curves of the specimen calculated with L_p,smallest = 0.617 for K_eq = 1 to 10. At V = V_c = 15 μV, the I_c value of the specimen obtained by simulation was 132 A. This value is between the I_c values calculated with K_eq = 2 and 3. It is noted that not only the integers but also the decimal places can be used as the K_eq value for the calculation of the V–I curve and I_c of the specimen since Equations (8)–(10) hold mathematically for positive real numbers, including decimal places. Using Equations (8)–(10), the K_eq value that describes the simulation result of I_c = 132 A at V = V_c = 15 μV for L_p,smallest = 0.617 was 2.39, as shown in Figure 2b.

Figure 2c shows the relation of the I_c value to K_eq value for L_p,smallest = 0.617 in 15 cm-specimen, obtained from the V–I curves for K_eq = 1 to 10 in Figure 2b. It is shown that the lower bound of critical current I_c,lower corresponding to K_eq = 10 in this example, is determined by the effect of Factor 1 (size of the largest crack, which is monitored by L_p,smallest) and the effect of Factor 2 (size difference among cracks, which is monitored by K_eq) plays a role in raising I_c from I_c,lower. In this way, the effects of Factor 1 and Factor 2 on I_c could be evaluated separately.

3. Results

The number of sections equivalent to the smallest ligament section K_eq, smallest ligament parameter among all sections L_p,smallest, lower bound of critical current I_c,lower and critical current I_c with an increase in specimen length L (1.5~60 cm) were investigated for a wide standard deviation of ligament parameter values ΔL_p = 0.01 to 0.15. Figure 3 shows the plots of K_eq, L_p,smallest, I_c,lower, and I_c against L, obtained for ΔL_p = 0.01, 0.05, and 0.15. The K_eq values were obtained by analysis of the simulation results with the approach in Section 2.2. The L_p,smallest, I_c,lower, and I_c values were obtained by simulation. The value of each specimen is shown with a circle (○) and the average value with a square (□). While the K_eq, L_p,smallest, I_c,lower, and I_c values are different from specimen to specimen, the average values (K_eq,ave, L_{p,smallest,ave}, I_c,lower,ave, and I_c,ave) show their correlation to L and ΔL_p.

Figure 4 shows the plots of an average of (a) number of sections equivalent to the largest crack section, K_eq,ave, (b) smallest ligament parameter, L_{p,smallest,ave}, (c) lower bound of critical current, I_c,lower,ave, and (d) critical current, I_c,ave, with an increase in L in each case of ΔL_p = 0.01, 0.025, 0.05, 0.1, and 0.15. The open symbols for K_eq,ave, L_{p,smallest,ave}, I_c,lower,ave, and I_c,ave, show the results obtained by simulation. The closed symbols in Figure 4b–d show the L_{p,smallest,ave}, I_c,lower,ave, and I_c,ave values obtained by calculation, whose method is shown in Section 4.1.

The following features are read in Figure 4:

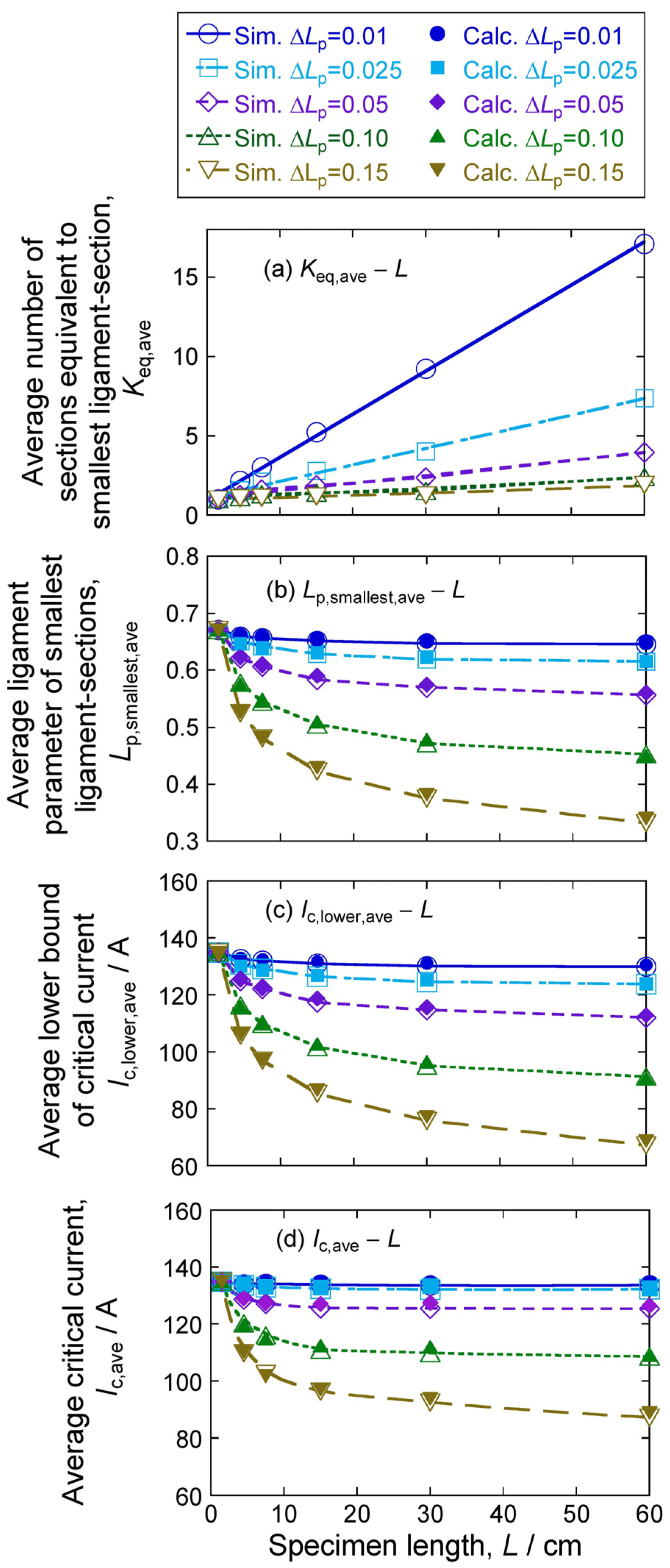
• The average smallest ligament parameter L_{p,smallest,ave} decreases with an increase in L. Namely, the average size of the largest crack increases with an increase in L. The extent of the increment of the size of the largest crack with L is enhanced with an increase in ΔL_p;
• Average critical current I_c,ave decreases with an increase in specimen length L. The extent of the decrease in I_c,ave with L increases with the increase in the standard deviation of the ligament size (=standard deviation of crack size) ΔL_p;
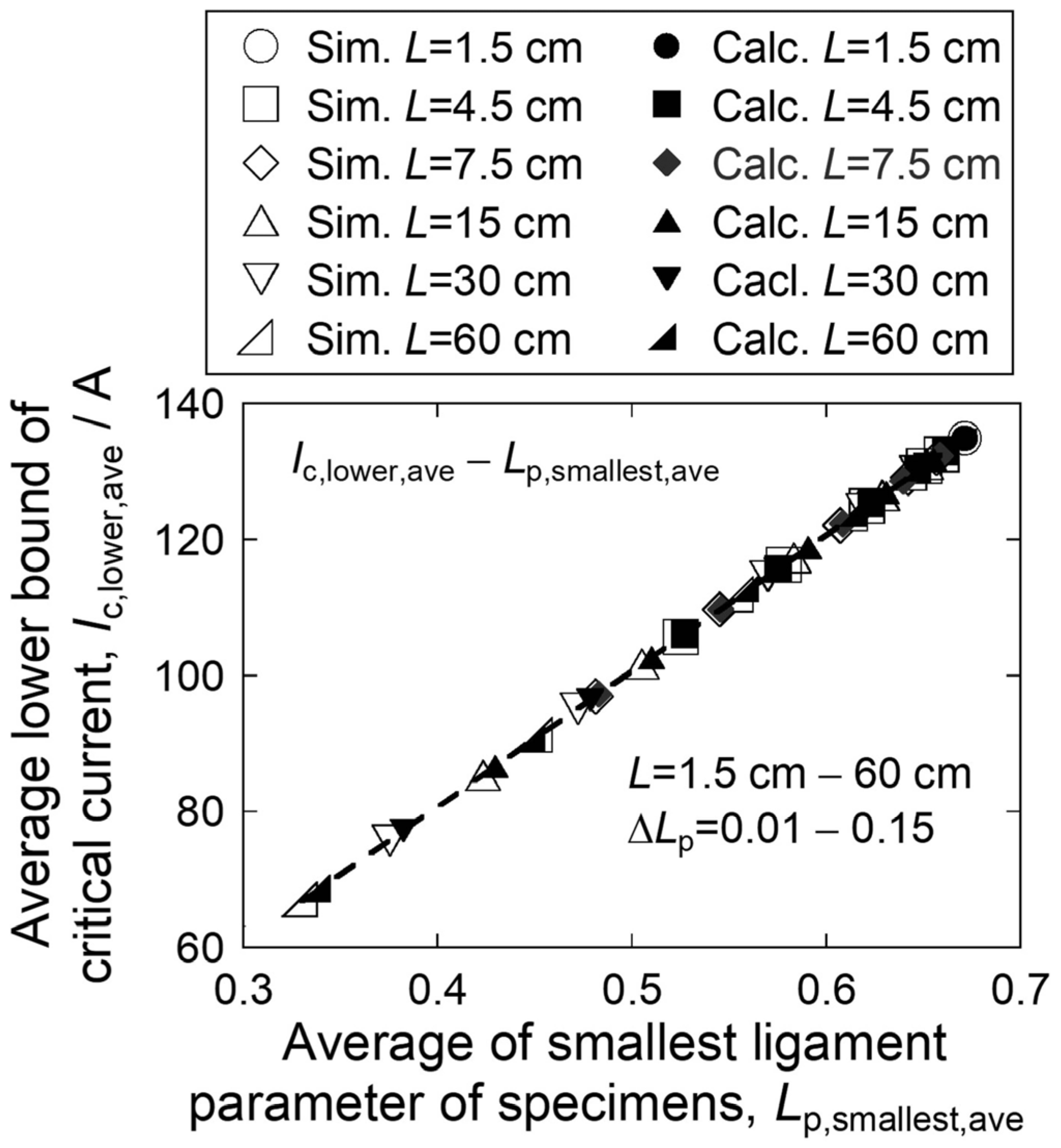
• The change in the average of critical current I_c,ave and the average of the lower bound of critical) current I_c,lower,ave with an increase in specimen length L is similar to the change in L_{p,smallest,ave}. This result suggests that the decrease in L_{p,smallest,ave}, namely the increase in the size of the largest crack, has a significant influence on the determination of critical current.

The features mentioned above will be analyzed numerically from the viewpoint of the effects of Factor 1 and Factor 2 on I_c.

4. Discussion

4.1. Calculation of Average Values of the Smallest Ligament Parameter, Lower Bound of Critical Current, Critical Current, and the Correlation between the Smallest Ligament Parameter and Lower Bound of Critical Current

Gumbel’s extreme value distribution function [33] describes the distribution of the extreme values of the smallest ligament (largest crack) parameter L_p,smallest in the present specimens, and the calculation results are compared with the present simulation and analyzed results. The cumulative probability of L_p,smallest value, ϕ (L_p,smallest), and the average L_p,smallest value, L_{p,smallest,ave}, for each set of ΔL_p and L values under the given value of L_p,ave (=0.667 in this work) are expressed as Equations (11)–(14).

$$\Phi (L_{\mathrm{p},\mathrm{smallest}})=1-\exp \left\{-\exp \left(\frac{L_{\mathrm{p},\mathrm{smallest}}-\lambda }{\alpha }\right)\right\}$$

(11)

L_{p,smallest,ave} = λ − αγ

(12)

λ and α are the positional and scale parameters, respectively, and γ is the Euler–Mascheroni constant (=0.5772). The values of λ and α under given values of L and ΔL_p are obtained by using Equation (1) (cumulative distribution function of L_p: F(L_p)) and Equation (2) probability density function L_p: f(L_p)) and the following relational expressions:

F(λ) = 1/N

(13)

α = 1/{N f(λ)}

(14)

We can calculate L_{p,smallest,ave} for given values of L and ΔL_p with Equations (1), (2), (12)–(14). Then, we can calculate the V_upper,ave–I curve (Case A), which gives the average lower bound for critical current using Equations (9) and (10). From the calculated V_upper,ave–I curve, we can obtain the I_c,lower,ave value for a given combination pair of L value and ΔL_p value with the criterion of E_c = 1 μV/cm. By substituting L_p,smallest = L_{p,smallest,ave} in Equation (9) and K_eq value (Figure 4a) in Equation (10) and applying the E_c = 1 μV/cm criterion, we can calculate the I_c,ave values for L = 1.5 to 60 cm and ΔL_p = 0.01 to 0.15.

The calculation results (closed symbols) of the average values of L_{p,smallest,ave}: smallest ligament parameter, I_c,ave: critical current, and I_c,lower,ave: lower bound of critical current in comparison with the simulation results (open symbols) are shown in Figure 4b–d.

The simulation results of L_{p,smallest,ave}, I_c,lower,ave, and I_c,ave values were described well by calculation. With the estimated valuers above, the effects of Factor 1 and Factor 2 on the specimen length-dependence of critical current can be estimated separately, as shown below.

4.2. Separate Assessment of Effects of Factor 1 and Factor 2 on Specimen Length-Dependence of Critical Current

The changes of the average critical current I_c,ave of specimens with increasing specimen length were described with Factor 1 monitored by L_{p,smallest,ave} and Factor 2 monitored by K_eq,ave, as shown in Figure 4b. It is noted that, as in Figure 4c, the I_c,lower,ave values of the simulation results were well described by calculation using Case A in which all sections have the same L_{p,smallest,ave} value (K_eq = N). In this case, Factor 1 is zero, and hence, this effect does not arise. Namely, the I_c,lower,ave value reflects just Factor 1. Plotting the I_c,lower,ave values in Figure 4c against the corresponding L_{p,smallest,ave} values in Figure 4b, we have Figure 5. It is clearly shown that the I_c,lower,ave has almost one to one relation to the L_{p,smallest,ave} for any specimen length L and any crack size difference ΔL_p. Using this feature, the effects of Factor 1 and Factor 2 on the specimen length-dependence of critical current can be assessed separately in the following manner:

Figure 6 shows the plots of I_c,ave and I_c,lower,ave, obtained by simulation under the condition of (a) ΔL_p = 0.15 and L = 1.5 cm to 60 cm, and (b) ΔL_p = 0.01 to 0.15 and L = 60 cm, against L_{p,smallest,ave}. The solid and broken lines show the relations of I_c,ave to L_{p,smallest,ave} and I_c,lower,ave to L_{p,smallest,ave}, respectively. The average critical current of the sections for any length is noted as I_c,ave* (=135 A). The value of I_c,ave* = 135 A is equal to the critical current value that appears if uniform cracking takes place under the condition of ΔL_p = 0 and L_p,ave = 0.667. This value is kept for any specimen length under ΔL_p = 0. The change of critical current ΔI_c,ave (1) caused by the increase in Factor 1 and the change of critical current ΔI_c,ave (2) caused by the increase in Factor 2 at the critical voltage with increasing L and ΔL_p are indicated with arrows.

Evidently, with a decreasing average of the smallest ligament parameter L_{p,smallest,ave}, namely with increasing size of largest crack, values of I_c,ave and I_c,lower,ave decrease, ΔI_c,ave (1) increases more in a minus direction caused by decreased I_c,lower,ave, and ΔI_c,ave (2) increases caused by an increase of the difference between I_c,ave and I_c,lower,ave. In this way, an increase in specimen length L and an increase in width of crack size distribution ΔL_p lead to an increase in Factor 1, which reduces critical current, and an increase in Factor 2, which raises the critical current by ΔI_c,ave (2).

Figure 7 shows I_c,ave, I_c,lower,ave, ΔI_c,ave (1) and ΔI_c,ave (2) plotted against specimen length L for ΔL_p = (a) 0.05 and (b) 0.15. As stated above, if cracking occurs uniformly (ΔL_p = 0) as the geometry of Case A (K_eq = N), the critical current value does not vary with specimen length, and it is kept to be I_c,ave* (=135 A). In practical specimens, cracking occurs heterogeneously (ΔL_p > 0), and hence, the critical current value decreases from the I_c,ave* value with increasing specimen length L and width of crack size distribution ΔL_p.

As shown in Figure 7, with increasing L, the I_c,ave is reduced from I_c,ave* to I_c,lower,ave by ΔI_c,ave (1) caused by the effect of increase in Factor 1. The critical current I_c,ave is given by the sum of the I_c,lower,ave, and effect of Factor 2, ΔI_c,ave (2), as shown in Figure 2c, Figure 6 and Figure 7. The relations of ΔI_c,ave (1) and ΔI_c,ave (2) to I_c,ave*, I_c,lower,ave, and I_c,ave, shown in Figure 6 and Figure 7, are expressed below.

The size of the largest crack is different from specimen to specimen. The average size of the largest crack in non-uniform cracking is monitored by L_{p,smallest,ave} in the present approach, which can be calculated by Equations (12)–(14). Setting L_p,smallest to be equal to the calculated L_{p,smallest,ave} value and K_eq = N in Equations (8)–(10), we can calculate I_c,lower,ave value. We can calculate the I_c,ave values by substituting L_p,smallest = L_{p,smallest,ave,} and K_eq,ave values shown in Figure 4a in Equations (8)–(10). From the value of I_c,ave* = 135 A and the simulated and calculated I_c,lower,ave and I_c,ave values as a function of L for each ΔL_p value (0.01~0.15), the ΔI_c,ave (1) and ΔI_c,ave (2) can be estimated by Equations (15) and (16) from the simulation result and also from the calculation result.

ΔI_c,ave (1) = I_c,lower,ave − I_c,ave*

(15)

ΔI_c,ave (2) = I_c,ave − I_c,lower,ave

(16)

Figure 8 shows specimen length dependence (a) ΔI_c,ave (1) and (b) ΔI_c,ave (2) for ΔL_p = 0.01 to 0.15, estimated by simulation (open symbols) and by calculation (closed symbols). The following features are read:

(1)

ΔI_c,ave (1), arising from the increase in the size of the largest crack (Factor 1), increases in minus direction with increasing specimen length L and standard deviation of crack-size distribution ΔL_p;

(2)

ΔI_c,ave (2), arising from the difference in crack size (Factor 2), increases in the plus direction with increasing L and ΔL_p;

(3)

From Figure 7, the effect of I_c,ave (1) and ΔI_c,ave (2) on I_c,ave with increasing specimen length;

(4)

The change in the minus (ΔI_c,ave (1))—and plus (ΔI_c,ave (2))—effects with specimen length becomes moderate in long specimens.

L is expressed as

I_c,ave = I_c,lower,ave + ΔI_c,ave (2) = I_c,ave* + ΔI_c,ave (1) + ΔI_c,ave (2)

(17)

In this work, I_c,ave* is 135 A for any L. Accordingly, whether I_c,ave increases or decreases with increasing L depends on the value of ΔI_c,ave (1) + ΔI_c,ave (2). Figure 8a,b demonstrates that the I_c,ave decreases with increasing specimen length L since the minus effect ΔI_c,ave (1) arising from Factor 1 is larger than the plus effect ΔI_c,ave (2) arising from Factor 2.

5. Conclusions

Using a model analysis and a Monte Carlo simulation method, critical current dependence on specimen length was studied by monitoring the effects of Factor 1: size of the largest crack, and Factor 2: size difference among cracks in cracked superconductor. The following results were obtained.

(1)

The calculation- and simulation-results showed the following features of the specimen length-dependence of the critical current. (a) Large Factor 1 plays a role in reducing critical current. (b) Large Factor 2 plays a role in raising critical current under a given size of the largest crack. (c) The effect of Factor 1 is larger than that of Factor 2, and hence, the critical current decreases with increasing specimen length. This feature is enhanced with increasing standard deviation of crack size;

(2)

For a quantitative description of the results mentioned in (1), the effect of Factor 1 on the critical current of the specimen was formulated by combining the smallest ligament parameter, corresponding to the largest crack section, with a shunting of the current model at the crack. The effect of Factor 2 on critical current was formulated using the number of sections equivalent to the largest crack section at the critical voltage of the specimen’s critical current. With the application of the present approach to the calculation and simulation results, the following results were obtained: (a) The critical current-reducing effect caused by an increase in Factor 1 and the critical current-raising effect caused by the increase in Factor 2 were assessed separately, and the critical current of the specimen was described as a function of specimen length. (b) The features mentioned in (1) were quantitatively described.

Present methods of simulation and calculation are simple, and reliable data are obtained. Thus, the methods and the results in this work are expected to be used for analysis and confirmation of experimental data concerning the relation between critical current, specimen length, and crack size distribution, which provides useful design information to ensure safety and reliability in the practical applications of superconductors.