Mechanical Behavior of 3D-Printed Thickness Gradient Honeycomb Structures
Abstract
:1. Introduction
2. Materials and Methods
2.1. Design and Modelling of the Model
2.2. Specimen Preparation
2.3. Raw Material Mechanical Properties
3. Experiments
4. Analytical Structure
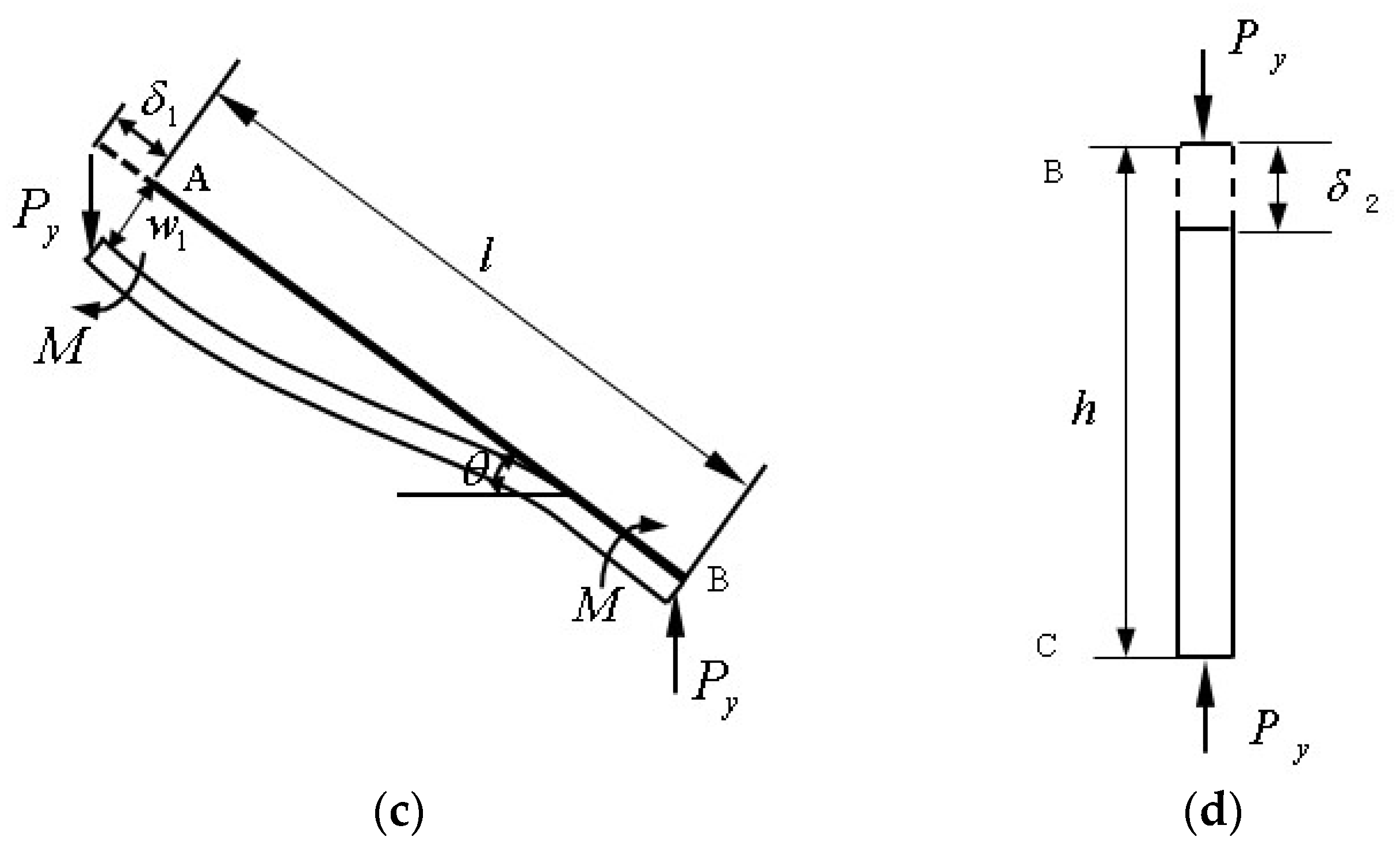
4.1. Theoretical Analysis
- M—the bending moment of the cellular node of the honeycomb, ;
- —the external force of the cellular node of the honeycomb, ;
- —the cross-sectional area of the honeycomb cell under stress in the Y direction, .
4.2. Structural Compressive Behavior
4.3. Finite Element Analysis
5. Results and Discussion
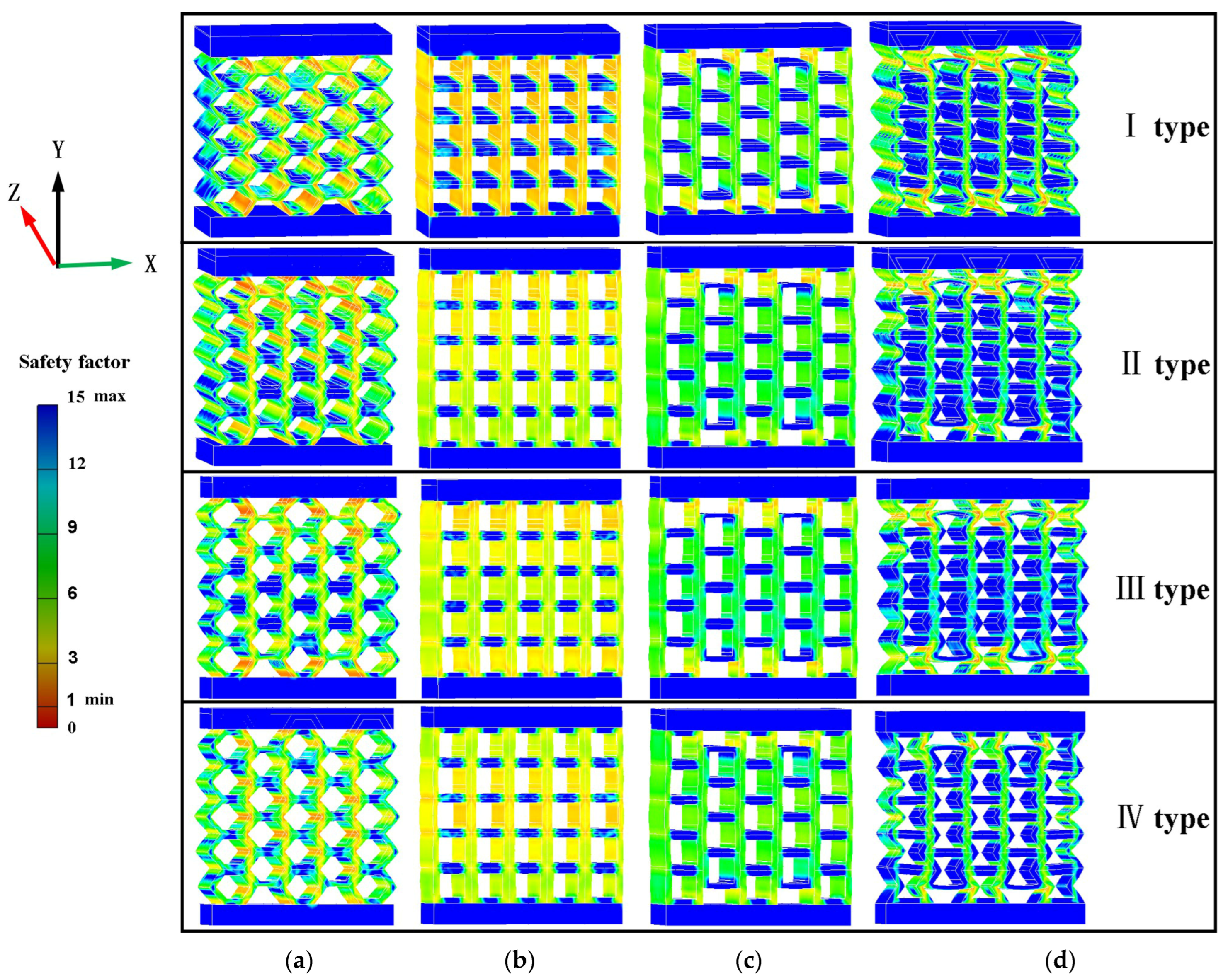
5.1. Structural Failure Process
5.2. Gradient Honeycomb Structure
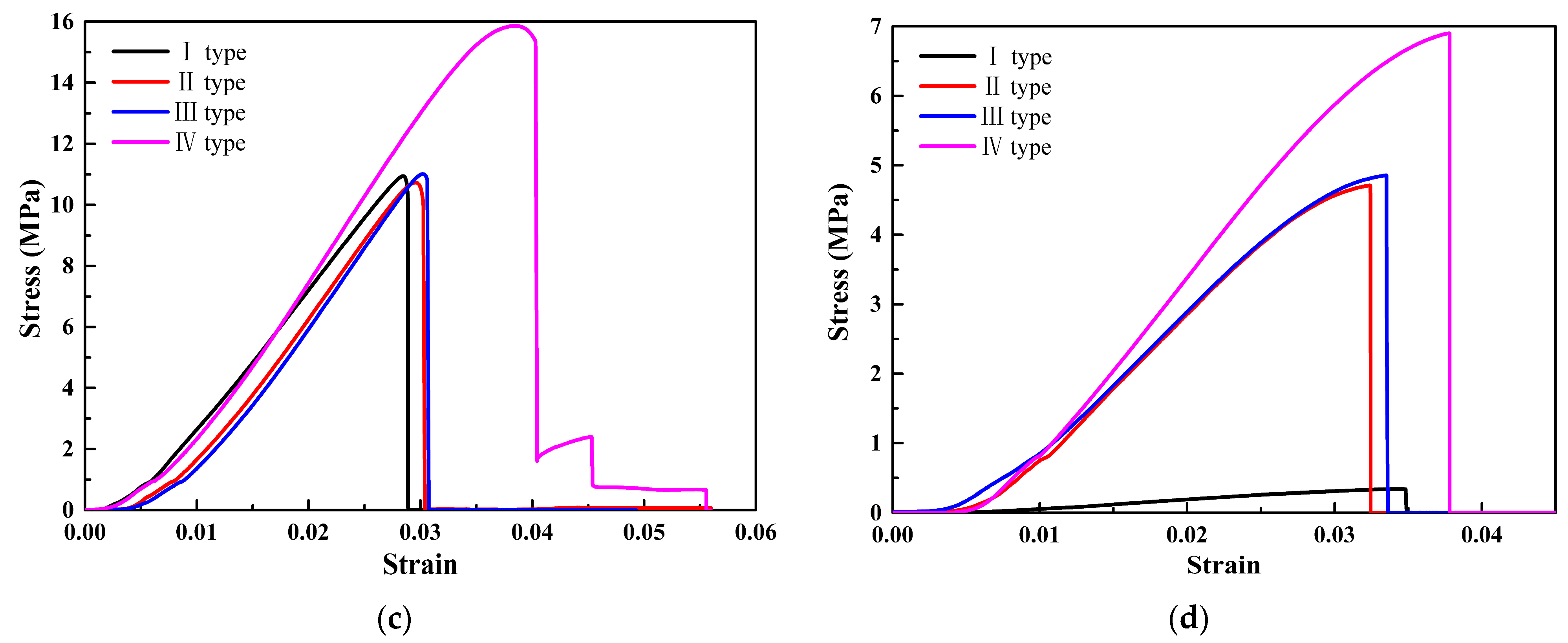
5.3. Uniaxial Compression Results
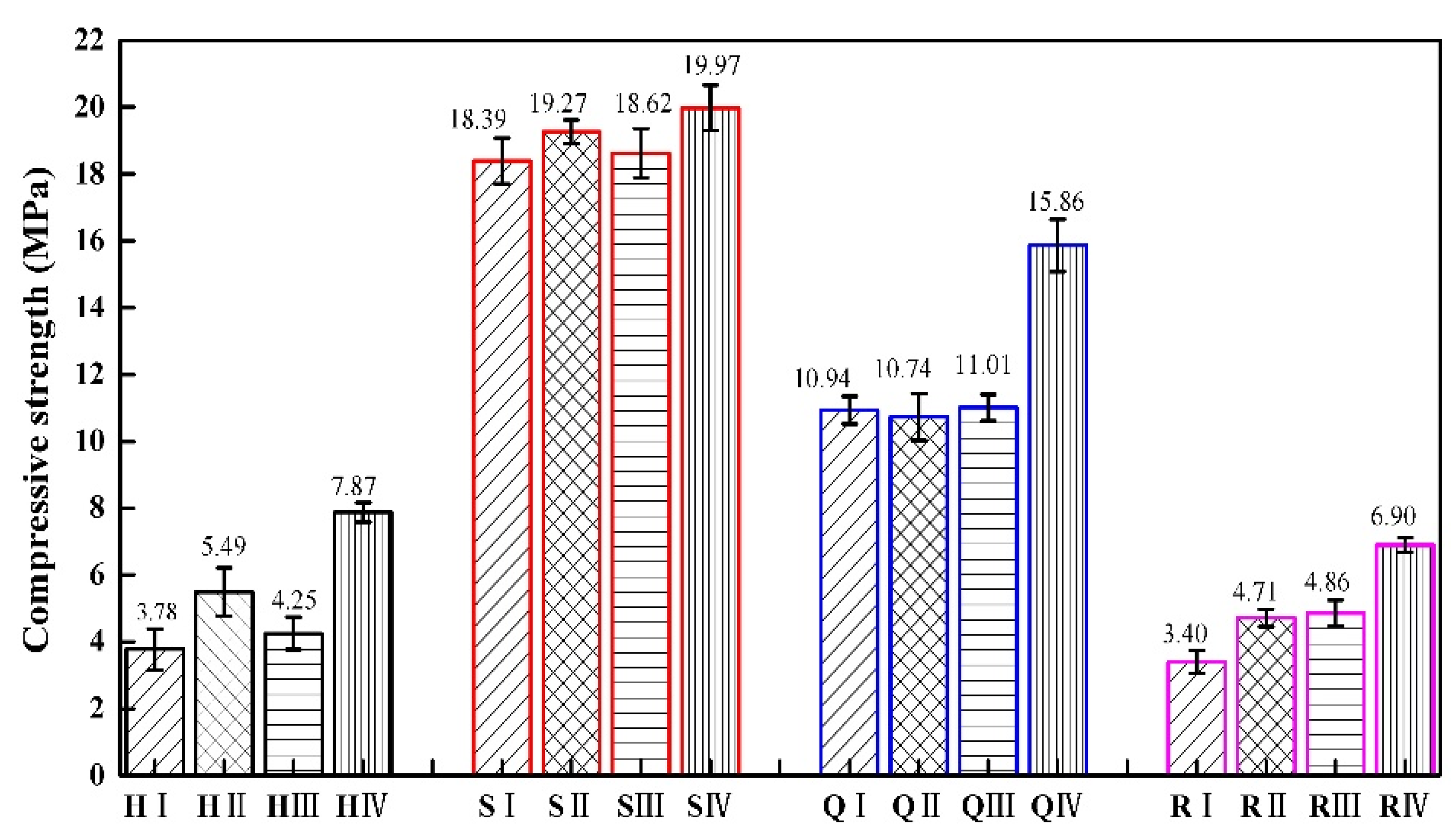
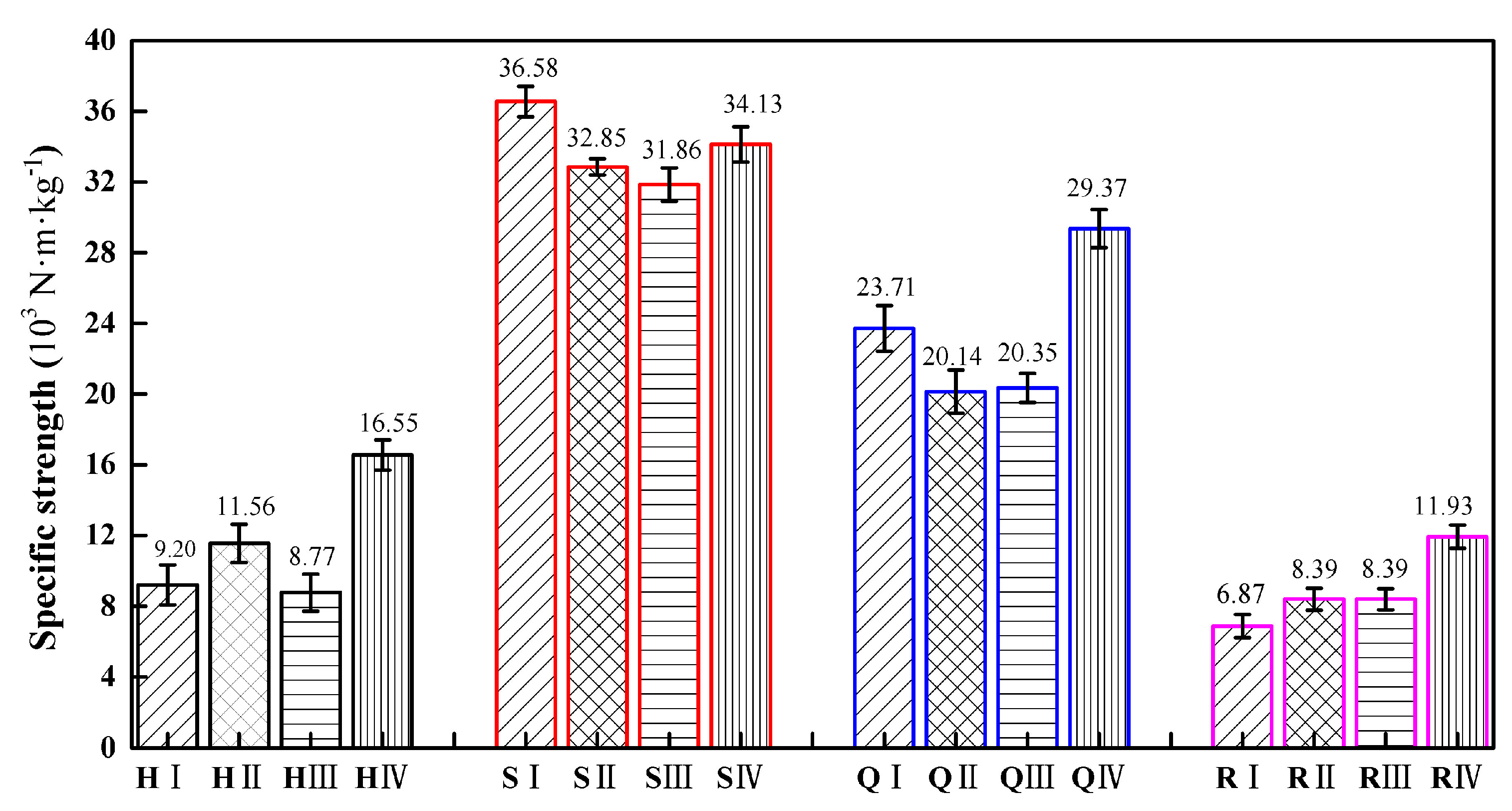
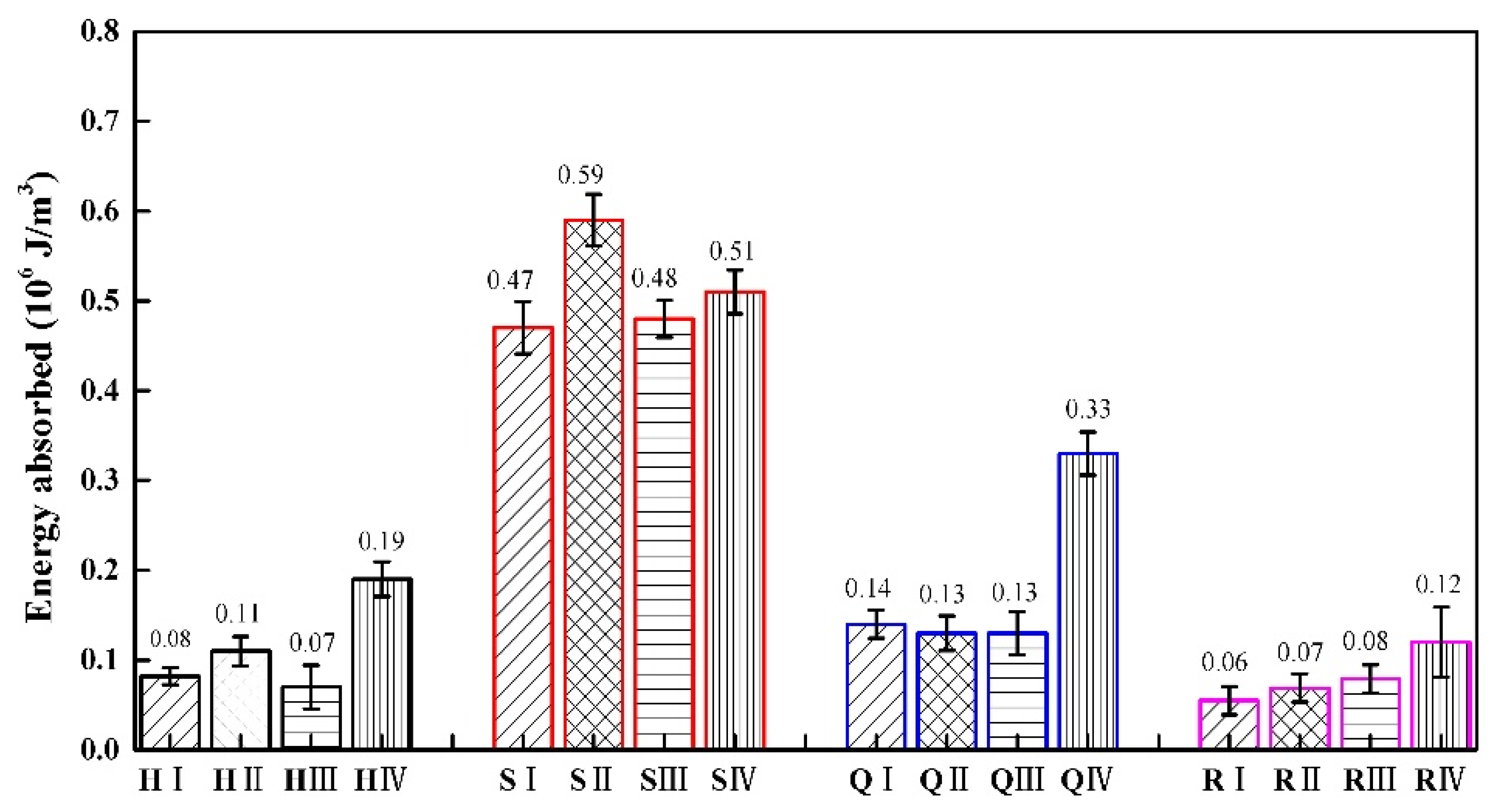
5.4. Compression Performance
6. Conclusions
- (1)
- Among the four kinds of thickness gradient honeycomb structures with periodic regular structures, the thickness gradient honeycomb structure with square cells has the best overall mechanical properties and the highest bending flexibility under pressure. By changing the thickness of the cellular wall and the arrangement order of the cells, the corresponding mechanical properties will also change. Increasing the thickness of the cellular wall at the upper and lower ends of the thickness gradient honeycomb structure can effectively improve the bearing capacity of the structure.
- (2)
- The thickness of the cellular wall in the honeycomb structure directly affects the overall stiffness of the gradient honeycomb structure. The thicker the cellular wall, the stronger the deformation resistance of the honeycomb structure under external force, that is, the greater the stiffness of the structure. Therefore, increasing the thickness of the cellular wall can improve the overall stiffness of the honeycomb structure, so as to improve its mechanical strength.
- (3)
- The order of the cell arrangement will directly affect the mass distribution, center of gravity position, stress distribution, and transmission path of the honeycomb structure. Optimizing the arrangement of the cells can reduce the position of the center of gravity of the structure, reduce the overturning moment under the load state, and make the stress more evenly distributed among the cells, which can effectively improve the bearing capacity of the honeycomb structure and cause it to have a higher structural stability.
- (4)
- The mechanical properties of structures with different Poisson’s ratios are significantly different. The hexagonal thickness gradient honeycomb structure is a positive-Poisson’s-ratio structural material, and its compressive strength is related to the structural configuration and material density; the square gradient honeycomb structure specimen and the quasi-square gradient honeycomb structure specimen are zero-Poisson’s-ratio structural materials, which have a high compressive strength, and the cell configuration of the structure can effectively disperse and resist external pressure; however, the re-entrant thickness gradient honeycomb structure specimen is a negative-Poisson’s-ratio structural material, and its compressive strength is low in the flat compression state. At the same time, its bearing capacity and compressive strength are more easily affected by the internal cell configuration of the structure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- San Ha, N.; Lu, G. A review of recent research on bio inspired structures and materials for energy absorption applications. Compos. Part B Eng. 2020, 181, 107496. [Google Scholar]
- Zhong, R.; Ren, X.; Zhang, X.Y.; Luo, C.; Zhang, Y.; Xie, Y.M. Mechanical properties of concrete composites with auxetic single and layered honeycomb structures. Constr. Build. Mater. 2022, 322, 126453. [Google Scholar] [CrossRef]
- Alsubari, S.; Zuhri, M.Y.M.; Sapuan, S.M.; Ishak, M.R.; Ilyas, R.A.; Asyraf, M.R.M. Potential of Natural Fiber Reinforced Polymer Composites in Sandwich Structures: A Review on Its Mechanical Properties. Polymers 2021, 13, 423–430. [Google Scholar] [CrossRef] [PubMed]
- Schaedler, T.A.; Carter, W.B. Architected Cellular Materials. Annu. Rev. Mater. Res. 2016, 46, 187–210. [Google Scholar] [CrossRef]
- Wang, Z. Recent advances in nover metallic honeycomb structure. Compos. Part B Eng. 2019, 166, 731–741. [Google Scholar] [CrossRef]
- Ge, Y.; Xue, J.; Liu, L.; Wan, H.; Yang, Y. Advances in multiple assembly acoustic structural design strategies for honeycomb composites: A Review. Mater. Today Commun. 2024, 38, 108013. [Google Scholar] [CrossRef]
- Pan, C.; Han, Y.; Lu, J. Design and Optimization of Lattice Structures: A Review. Appl. Sci. 2020, 10, 6374. [Google Scholar] [CrossRef]
- Zhao, J.; Zhu, S. Analysis of mechanical properties of 3D printing honeycomb in temporary construction. J. Harbin Inst. Technol. 2020, 52, 98–104. [Google Scholar]
- Yuan, M.; Xu, F.; Gong, M. In plane impact performance of honeycomb material with gradient thickness and negative Poisson’s ratio. J. Plast. Eng. 2021, 28, 192–199. [Google Scholar]
- Sun, G.; Chen, D.; Zhu, G.; Li, Q. Lightweight hybrid materials and structures for energy absorption: A state of the art review and outlook. Thin Walled Struct. 2022, 172, 332–400. [Google Scholar] [CrossRef]
- Zhang, Q.; Yang, X.; Li, P.; Huang, G.; Feng, S.; Shen, C.; Han, B.; Zhang, X.; Jin, F.; Xu, F.; et al. Bioinspired engineering of honeycomb structure Using nature to inspire human innovation. Prog. Mater. Sci. 2015, 74, 332–400. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, J.; Han, X. Honeycomb sandwich composite and research progress. New Chem. Mater. 2021, 49, 253–258. [Google Scholar]
- Feng, Y.; Qiu, H.; Gao, Y.; Zheng, H.; Tan, J. Creative design for sandwich structures: A review. Int. J. Adv. Robot. Syst. 2020, 17, 1729881420921327. [Google Scholar] [CrossRef]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Xu, M.; Liu, D.; Wang, P.; Zhang, Z.; Jia, H.; Lei, H.; Fang, D. In plane compression behavior of hybrid honeycomb metastructures: Theoretical and experimental studies. Aerosp. Sci. Technol. 2020, 106, 106081. [Google Scholar] [CrossRef]
- Yin, H.F.; Zhang, W.Z.; Zhu, L.C.; Meng, F.B.; Liu, J.E.; Wen, G.L. Review on lattice structures for energy absorption properties. Compos. Struct. 2022, 304, 145–157. [Google Scholar] [CrossRef]
- Luo, Y.; Yuan, K.; Shen, L.; Liu, J. Sandwich panel with in-plane honeycombs in different Poisson’s ratio under low to medium impact loads. Rev. Adv. Mater. Sci. 2021, 14, 145–157. [Google Scholar] [CrossRef]
- Gong, X.; Ren, C.; Liu, Y.; Sun, J.; Xie, F. Impact Response of the Honeycomb Sandwich Structure with Different Poisson’s Ration. Materials 2022, 15, 6982. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, Y.; Guo, Z.; Li, D.; Zhao, S.; Xie, W. Analyzing in-plane mechanics of a novel honeycomb structure with zero Poisson’s ratio. Thin Walled Struct. 2023, 192, 111134. [Google Scholar] [CrossRef]
- Lira, C.; Scarpa, F. Transverse shear stiffness of thickness gradient honeycombs. Compos. Sci. Technol. 2010, 70, 930–936. [Google Scholar] [CrossRef]
- Del Broccolo, S.; Laurenzi, S.; Scarpa, F. AUXHEX—A Kirigami inspired zero Poisson’s ratio cellular structure. Compos. Struct. 2017, 176, 433–441. [Google Scholar] [CrossRef]
- Zheng, B.B.; Liu, F.M.; Hu, L.L.; Fu, M.H.; Chen, Y. An auxetic honeycomb structure with series coneected parallograms. Int. J. Mech. Sci. 2019, 161, 105073. [Google Scholar] [CrossRef]
- Jiang, W.; Ren, X.; Wang, S.L.; Zhang, X.G.; Zhang, X.Y.; Luo, C.; Xie, Y.M.; Scarpa, F.; Alderson, A.; Evans, K.E. Manufacturing, characteristics and applications of auxetic foams: A state of the art review. Compos. Part B Eng. 2022, 235, 109733. [Google Scholar] [CrossRef]
- Kothandaraman, L.; Balasubramanian, N.K. Optimization of FDM printing parameters for square lattice structures: Improving mechanical characteristics. Mater. Today Proc. 2024, 4, 1–6. [Google Scholar]
- Khan, S.; Joshi, K.; Deshmukh, S. A comprehensive review on effect of printing parameters on mechanical properties of FDM printed parts. Mater. Today Proc. 2022, 50, 2119–2127. [Google Scholar] [CrossRef]
- Ritter, T.; McNiffe, E.; Higgins, T.; Sam-Daliri, O.; Flanagan, T.; Walls, M.; Ghabezi, P.; Finnegan, W.; Mitchell, S.; Harrison, N.M. Design and Modification of a Material Extrusion 3D Printer to Manufacture Functional Gradient PEEK Components. Polymers 2023, 15, 3825. [Google Scholar] [CrossRef]
- ISO 604; Plastics—Determination of Compressive Properties. ISO (International Organization for Standardization): Geneva, Switzerland, 2002.
- ISO 178-2010; Plastics—Determination of Flexural Properties. ISO (International Organization for Standardization): Geneva, Switzerland, 2010.
- ASTM D638-2014; Standard Test Method for Tensile Properties of Plastics. ASTM: New York, NY, USA, 2014.
- Li, S.; Qin, J.; Wang, B.; Zheng, T.; Hu, Y. Design and Compressive Behavior of a Photosensitive Resin Based 2D Lattice Structure with Variable Cross Section Core. Polymers 2019, 11, 186. [Google Scholar] [CrossRef] [PubMed]
- ASTM C365-16; Standard Test Method for Flatwise Compressive Properties of Sandwich Cores. ASTM: New York, NY, USA, 2011.
- Gibson, L.J.; Ashby, M.F.; Schajer, G.S.; Robertson, C.I. The mechanics of two-dimensional cellular materials. Proc. R. Soc. Lond. A Math. Phys. Sci. 1982, 382, 25–42. [Google Scholar]
- Fu, M.; Xu, O.; Chen, Y. Review of Research on4quivalent Parameters of Honeycomb Core. Mater. Guide 2015, 29, 127–134. [Google Scholar]
- Li, X. Lightweight Design of Load-Bearing Sandwich Composites. Doctoral Dissertation, Wuhan University, Wuhan, China, 2011. [Google Scholar]
- Li, X.; Wang, Y.; Yang, Z.; Chen, B.; To, G. Research on Poisson’s Ratio of Quasi-Square Honeycomb Sandwich Structure’s Core. J. China Three Gorges Univ. 2019, 41, 91–96. [Google Scholar]
- Chen, S.; Tan, X.; Hu, J.; Zhu, S.; Wang, B.; Wang, L.; Jin, Y.; Wu, L. A novel gradient negative stiffness honeycomb for recoverable energy absorption. Compos. Part B 2021, 215, 108745. [Google Scholar] [CrossRef]
- Amada, S. Hierarchical functionally gradient structures of bamboo, barley, and corn. MRS Bull. 1995, 20, 35–36. [Google Scholar] [CrossRef]
- Schuster, P.J. Current trends in bumper design for pedestrian impact. In Proceedings of the SAE 2006 World Congress & Exhibition, Detroit, MI, USA, 3–6 April 2006; pp. 1–18. [Google Scholar]
- Guo, Z.; Xiao, J. In plane impact dynamics of thickness gradient honeycomb structures with negative Poisson’s ratio multi-arc concave cells. Mech. Adv. Mater. Struct. 2024, 31, 2386–2397. [Google Scholar] [CrossRef]
- Liu, M. Finite element analysis and optimal design of vehicle seat plastic based on ABAQUS. J. Plast. Eng. 2011, 18, 116–119. [Google Scholar]
- Zaharia, S.M.; Enescu, L.A.; Pop, M.A. Mechanical Performances of lightweight sandwich structures produced by material extrusion-based additive manufacturing. Polymers 2020, 12, 1740. [Google Scholar] [CrossRef] [PubMed]
- Nath, S.D.; Nilufar, S. Performance Evaluation of Sandwich Structures Printed by Vat Photopolymerization. Polymers 2022, 14, 1513. [Google Scholar] [CrossRef]
- Xu, S.C.; Chen, N.; Qin, H.Y.; Wang, R.X.; Yang, X.; Song, J.F. Research on Impact Resistance of Aluminum Alloy New Rotating Thin-Walled Structures. Biomimetics 2023, 8, 590. [Google Scholar] [CrossRef]
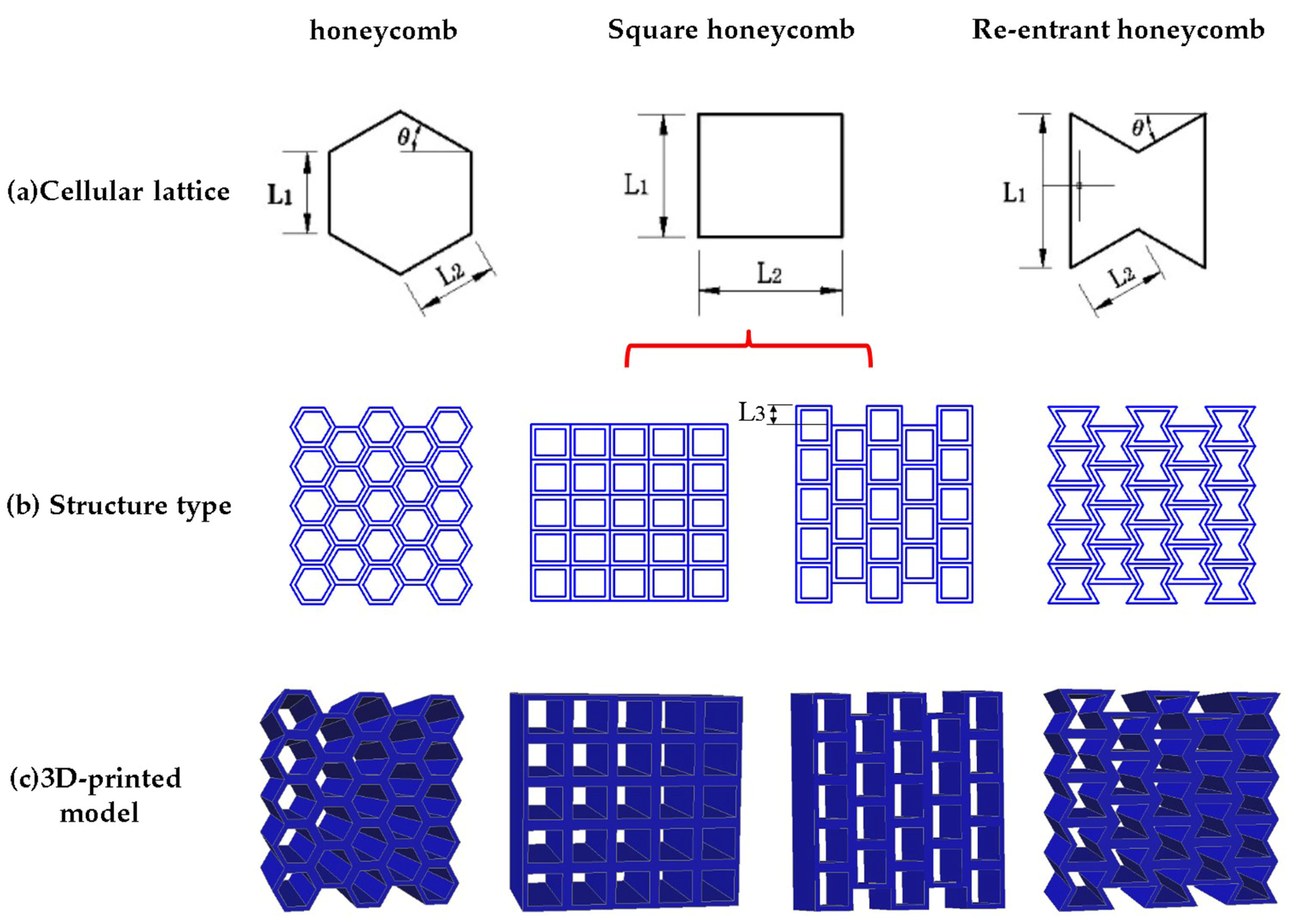




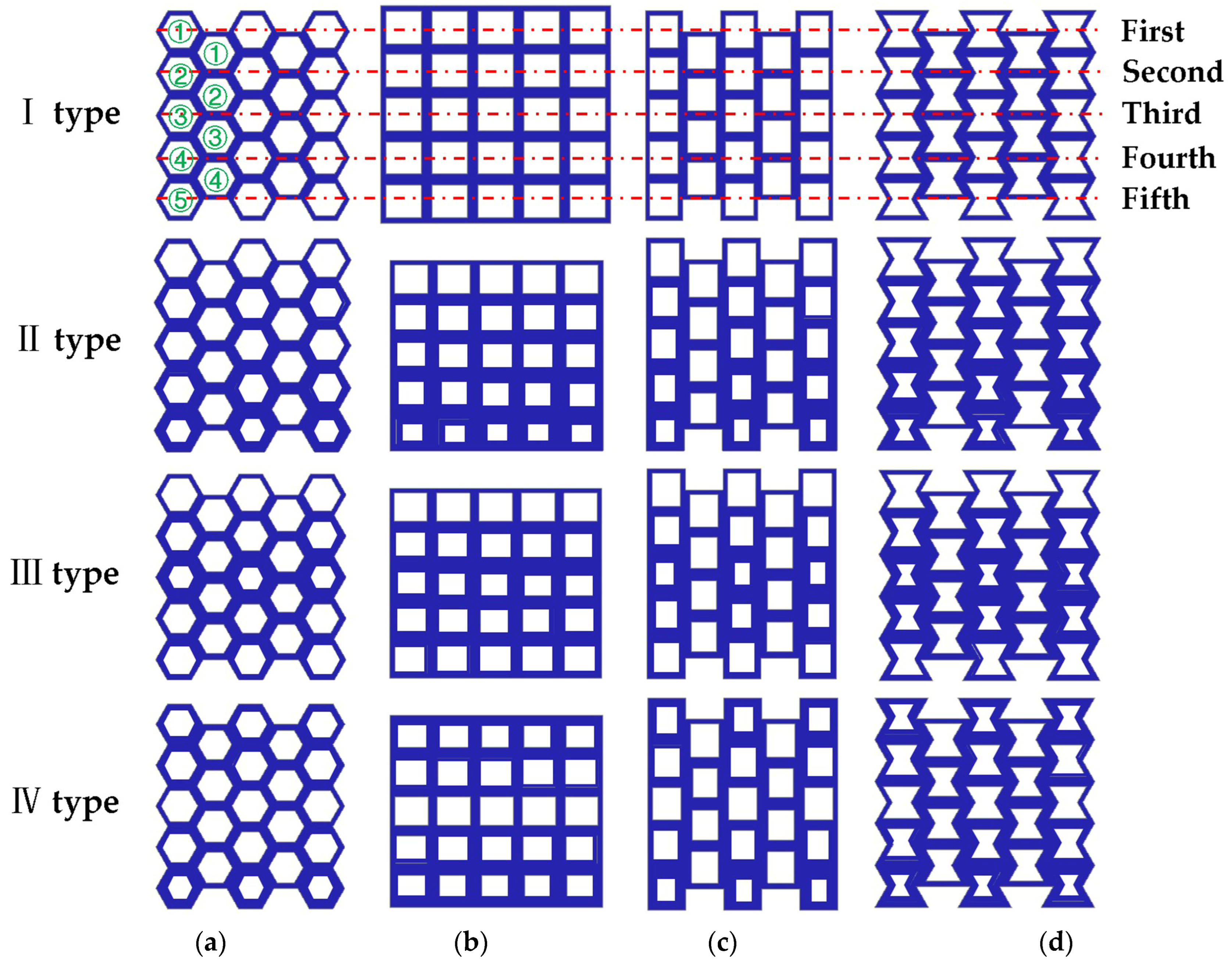


| Unit Cell | Side Length L1/mm | Side Length L2/mm | Included Angle θ/(°) |
|---|---|---|---|
| Honeycomb | 4.00 | 4.00 | 30.00 |
| Square honeycomb | 6.00 | 6.93 | 90.00 |
| Re-entrant honeycomb | 8.00 | 4.00 | 30.00 |
| Name | Height (h) mm | Width (w) mm | Length (l) mm |
|---|---|---|---|
| Honeycomb | 89.28 | 82.48 | 50.00 |
| Square honeycomb | 80.00 | 89.30 | 50.00 |
| Quasi-square honeycomb | 89.30 | 80.00 | 50.00 |
| Re-entrant honeycomb | 89.28 | 94.02 | 50.00 |
| Serial Number | Parameter | Value |
|---|---|---|
| 1 | Laser wavelength | 354.7 nm |
| 2 | Recoating thickness | 0.1 mm |
| 3 | Spot diameter | 0.15 mm |
| 4 | Part scanning speed | 6.0 m/s |
| 5 | Jumping speed of parts | 10.0 m/s |
| 6 | Repetitive positioning accuracy | ±0.01 mm |
| 7 | Temperature | 20 °C |
| 8 | Volume of resin vat | 200 kg |
| Compressive Modulus | Compressive Strength | Flexural Modulus | Flexural Strength | Tensile Modulus | Tensile Strength |
|---|---|---|---|---|---|
| 1699.97 ± 169.08 | 53.17 ± 7.28 | 1897.30 ± 134.24 | 59.29 ± 7.11 | 1205.02 ± 213.02 | 36.46 ± 2.66 |
| Wall Thickness | Honeycomb Type | |||
|---|---|---|---|---|
| I | II | III | IV | |
| First | 2.00 | 2.00 | 2.00 | 2.60 |
| Second | 2.00 | 2.20 | 2.40 | 2.20 |
| Third | 2.00 | 2.40 | 2.80 | 2.00 |
| Fourth | 2.00 | 2.60 | 2.60 | 2.40 |
| Fifth | 2.00 | 2.80 | 2.20 | 2.80 |
| Type | Compressive Strength (MPa) | Specific Strength (MPa) | ||
|---|---|---|---|---|
| Mean Value ± Standard Deviation | Coefficient of Variation (%) | Mean Value ± Standard Deviation | Coefficient of Variation (%) | |
| H I | 3.78 ± 0.61 | 16.14 | 9.20 ± 1.12 | 12.17 |
| H II | 5.49 ± 0.72 | 13.11 | 11.56 ± 1.09 | 9.43 |
| H III | 4.25 ± 0.48 | 11.29 | 8.77 ± 1.04 | 11.86 |
| H IV | 7.87 ± 0.29 | 3.68 | 16.55 ± 0.87 | 5.26 |
| S I | 18.39 ± 0.69 | 3.75 | 36.58 ± 0.87 | 2.38 |
| S II | 19.27 ± 0.35 | 1.82 | 32.85 ± 0.46 | 1.40 |
| S III | 18.62 ± 0.68 | 3.65 | 31.86 ± 0.94 | 2.95 |
| S IV | 19.97 ± 0.74 | 3.71 | 34.13 ± 1.01 | 2.96 |
| Q I | 10.94 ± 0.40 | 3.66 | 23.71 ± 1.29 | 5.44 |
| Q II | 10.74 ± 0.70 | 6.52 | 20.14 ± 1.23 | 6.11 |
| Q III | 11.01 ± 0.39 | 3.54 | 20.35 ± 0.82 | 4.03 |
| Q IV | 15.86 ± 0.78 | 4.92 | 29.37 ± 1.09 | 3.71 |
| R I | 3.40 ± 0.34 | 10.00 | 6.87 ± 0.65 | 9.46 |
| R II | 4.71 ± 0.26 | 5.52 | 8.39 ± 0.63 | 7.51 |
| R III | 4.86 ± 0.78 | 16.05 | 8.39 ± 0.59 | 7.15 |
| R IV | 6.90 ± 0.22 | 3.19 | 11.93 ± 0.67 | 5.62 |
| Type | Load–Mass Ratio (N·g−1) | Energy Absorbed (106 J/m3) | ||
|---|---|---|---|---|
| Mean Value ± Standard Deviation | Coefficient of Variation (%) | Mean Value ± Standard Deviation | Coefficient of Variation (%) | |
| H I | 102.98 ± 12.01 | 11.66 | 0.08 ± 0.0093 | 11.63 |
| H II | 129.46 ± 7.73 | 5.97 | 0.11 ± 0.0161 | 14.64 |
| H III | 98.29 ± 15.22 | 15.48 | 0.07 ± 0.0241 | 34.40 |
| H IV | 185.53 ± 9.02 | 4.86 | 0.19 ± 0.0192 | 10.13 |
| S I | 457.38 ± 8.04 | 1.76 | 0.47 ± 0.0291 | 6.19 |
| S II | 410.73 ± 12.18 | 2.97 | 0.59 ± 0.0286 | 4.85 |
| S III | 398.56 ± 9.18 | 2.30 | 0.48 ± 0.0207 | 4.31 |
| S IV | 426.87 ± 4.89 | 1.15 | 0.51 ± 0.0241 | 4.73 |
| Q I | 265.69 ± 15.61 | 5.88 | 0.14 ± 0.0158 | 11.29 |
| Q II | 225.57 ± 10.05 | 4.46 | 0.13 ± 0.0192 | 14.77 |
| Q III | 228.04 ± 11.19 | 4.91 | 0.13 ± 0.0241 | 18.54 |
| Q IV | 329.11 ± 15.71 | 4.77 | 0.33 ± 0.0241 | 7.27 |
| R I | 76.82 ± 16.88 | 21.97 | 0.06 ± 0.0158 | 26.33 |
| R II | 93.97 ± 9.13 | 9.72 | 0.07 ± 0.0156 | 22.29 |
| R III | 93.90 ± 8.12 | 8.65 | 0.08 ± 0.0155 | 19.38 |
| R IV | 133.47 ± 13.77 | 10.32 | 0.12 ± 0.0389 | 32.42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, D.; Guo, L.; Fan, C. Mechanical Behavior of 3D-Printed Thickness Gradient Honeycomb Structures. Materials 2024, 17, 2928. https://doi.org/10.3390/ma17122928
Yang D, Guo L, Fan C. Mechanical Behavior of 3D-Printed Thickness Gradient Honeycomb Structures. Materials. 2024; 17(12):2928. https://doi.org/10.3390/ma17122928
Chicago/Turabian StyleYang, Dongxia, Lihua Guo, and Changsheng Fan. 2024. "Mechanical Behavior of 3D-Printed Thickness Gradient Honeycomb Structures" Materials 17, no. 12: 2928. https://doi.org/10.3390/ma17122928
APA StyleYang, D., Guo, L., & Fan, C. (2024). Mechanical Behavior of 3D-Printed Thickness Gradient Honeycomb Structures. Materials, 17(12), 2928. https://doi.org/10.3390/ma17122928






