Synthesis, Characterization, and Magnetocaloric Properties of the Ternary Boride Fe2AlB2 for Caloric Applications
Abstract
:1. Introduction
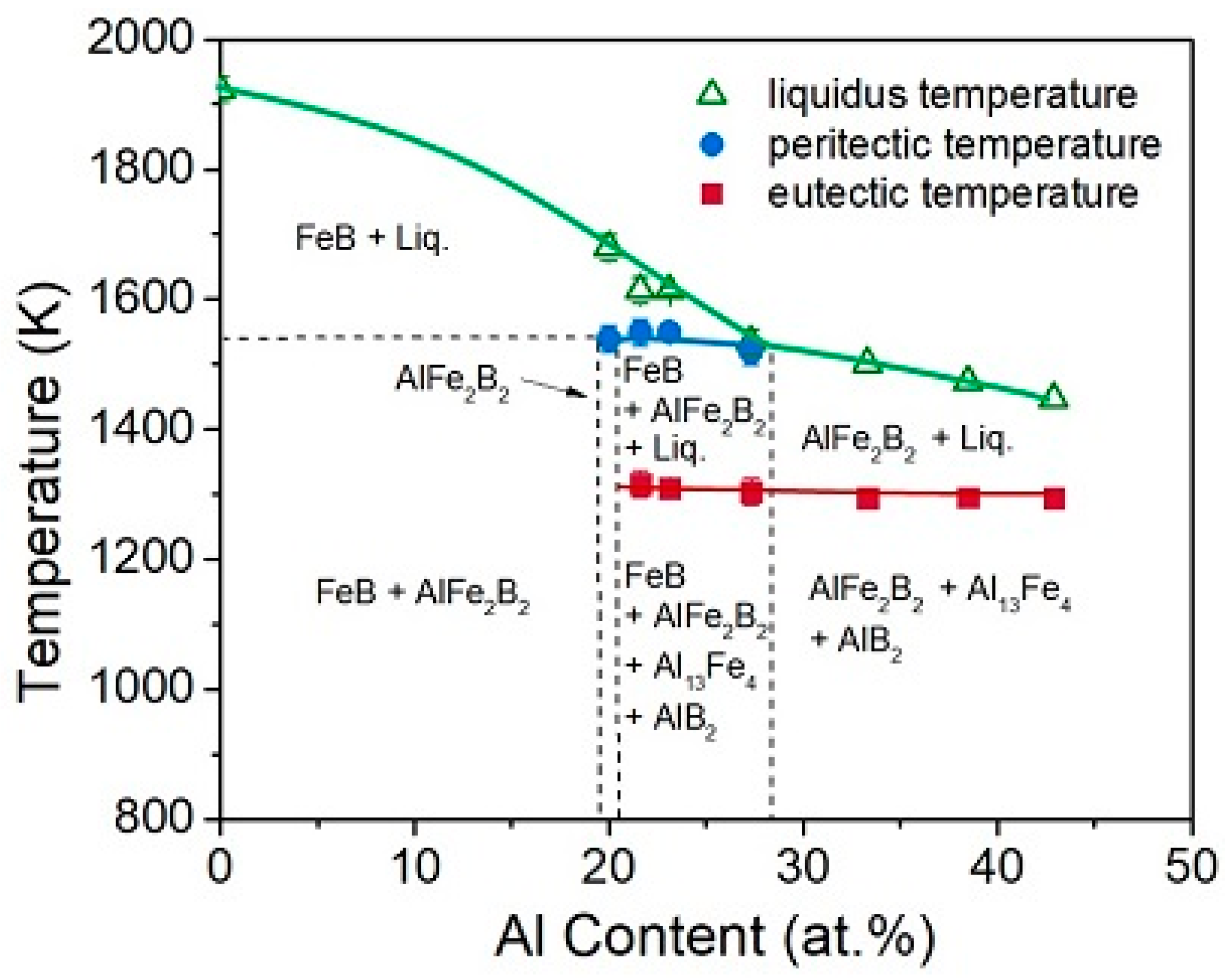
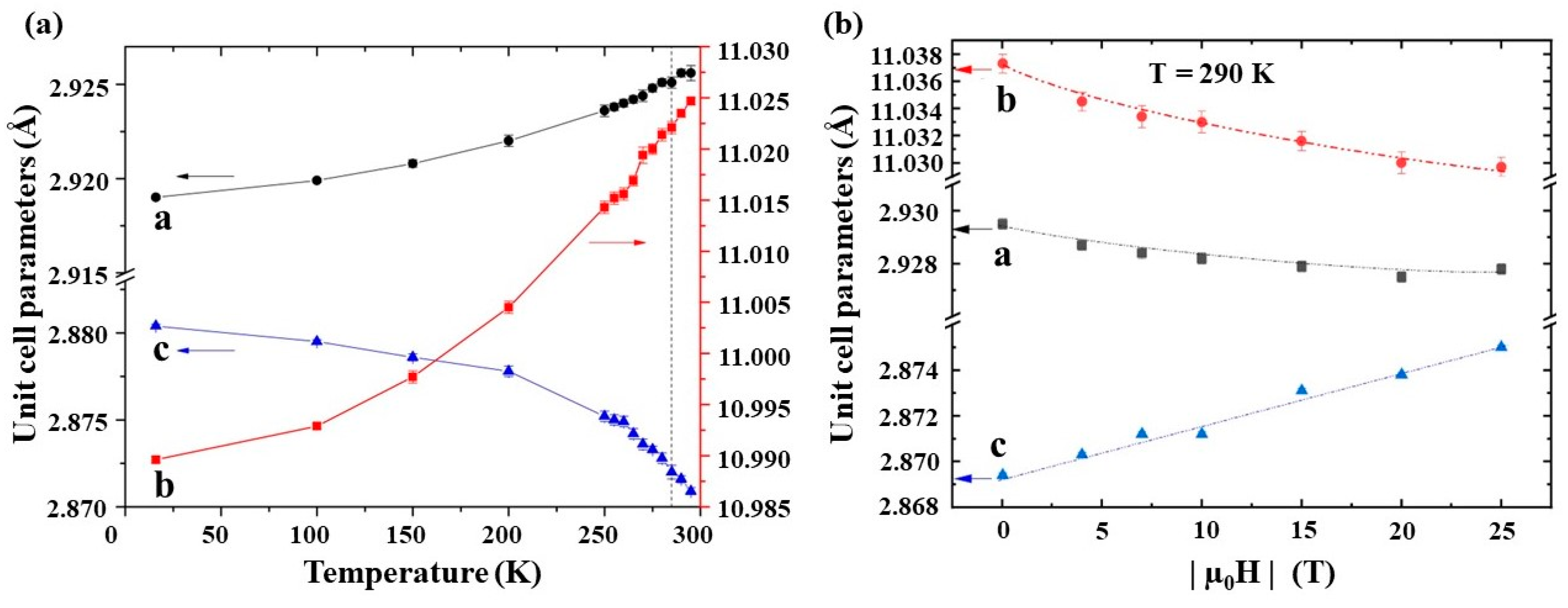
2. Fundamentals
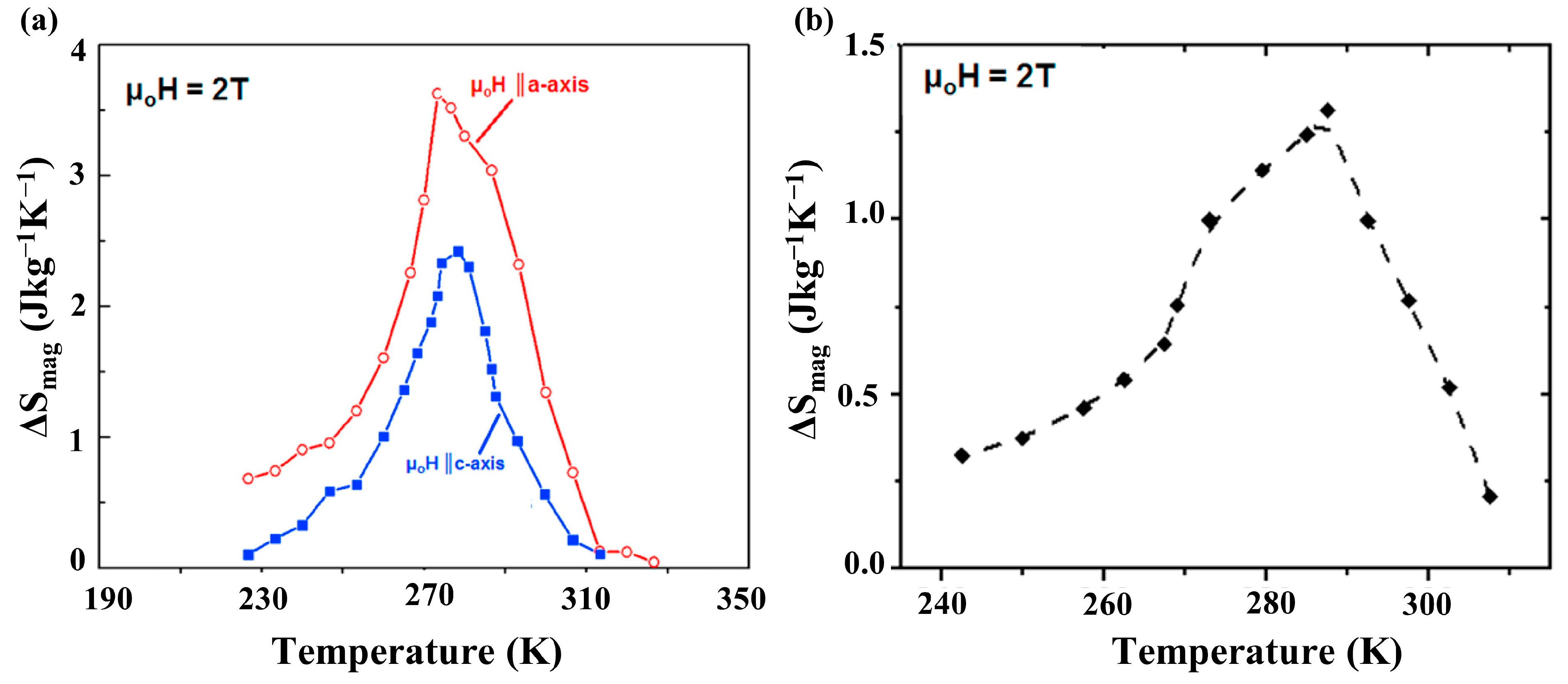
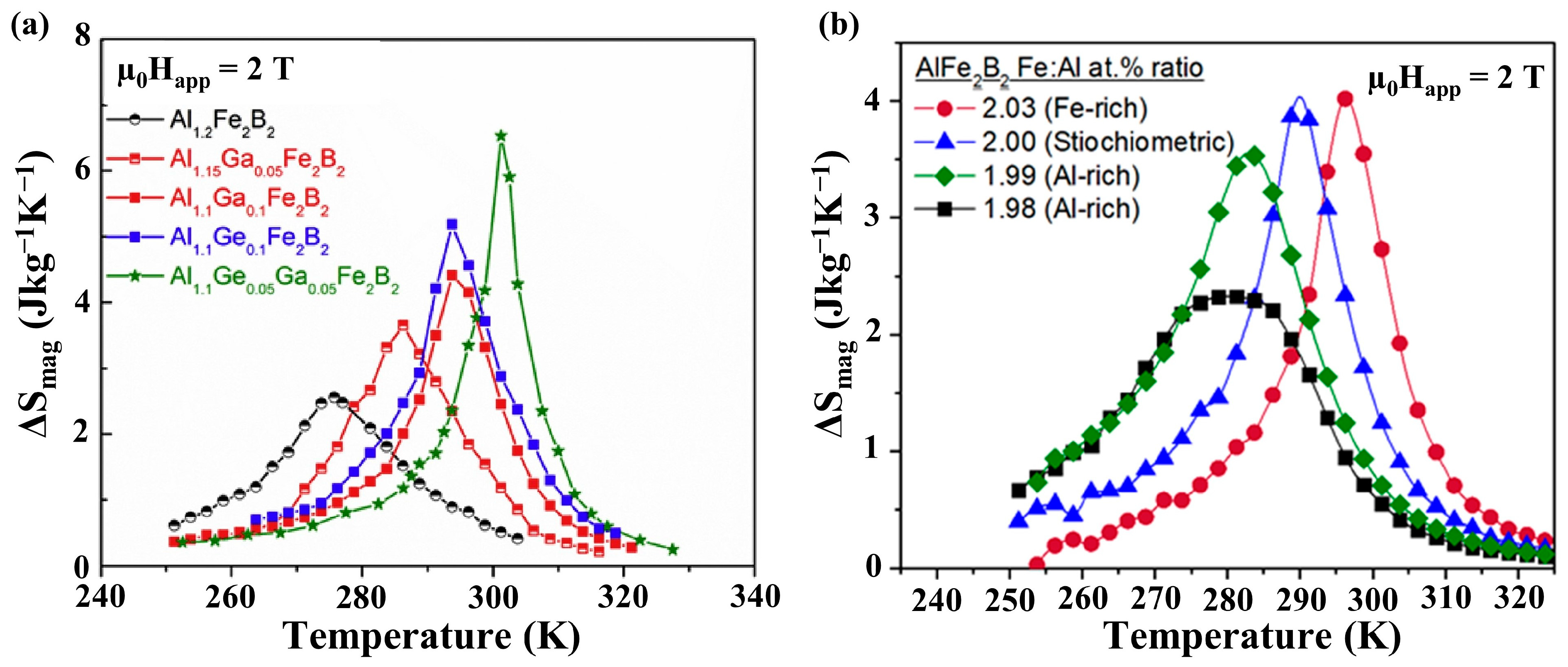
3. Magnetofunctional Response of Fe2AlB2 and Its Compositional Variants
4. Thermal and Electrical Transport Properties
5. Mechanical and Chemical Stability
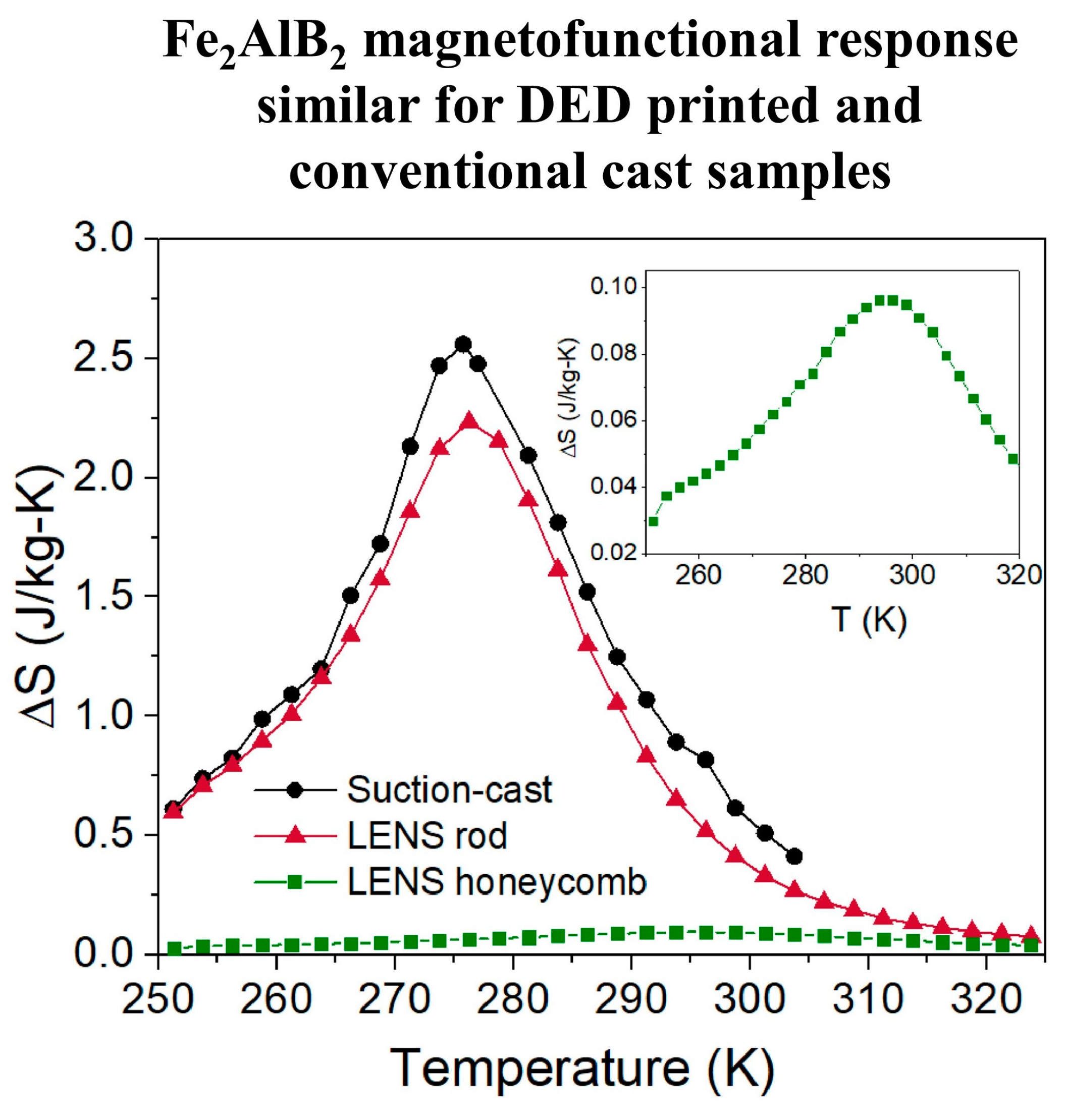
6. Amenability to Shaping into Heat Exchange Structures
7. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
References
- Kota, S.; Sokol, M.; Barsoum, M.W. A Progress Report on the MAB Phases: Atomically Laminated, Ternary Transition Metal Borides. Int. Mater. Rev. 2020, 65, 226–255. [Google Scholar] [CrossRef]
- Tan, X.; Chai, P.; Thompson, C.M.; Shatruk, M. ChemInform Abstract: Magnetocaloric Effect in AlFe2B2: Toward Magnetic Refrigerants from Earth-Abundant Elements. ChemInform 2013, 135, 9553–9557. [Google Scholar] [CrossRef]
- Jeitschko, W. The Crystal Structure of Fe2AlB2. Acta Crystallogr. Sect. B Struct. Crystallogr. Cryst. Chem. 1969, 25, 163–165. [Google Scholar] [CrossRef]
- Lejeune, B.T.; Schlagel, D.L.; Jensen, B.A.; Lograsso, T.A.; Kramer, M.J.; Lewis, L.H. Effects of Al and Fe Solubility on the Magnetofunctional Properties of AlFe2B2. Phys. Rev. Mater. 2019, 3, 094411. [Google Scholar] [CrossRef]
- Du, Q.; Chen, G.; Yang, W.; Song, Z.; Hua, M.; Du, H.; Wang, C.; Liu, S.; Han, J.; Zhang, Y.; et al. Magnetic Properties of AlFe2B2 and CeMn2Si2 Synthesized by Melt Spinning of Stoichiometric Compositions. Jpn. J. Appl. Phys. 2015, 54, 053003. [Google Scholar] [CrossRef]
- Sivaprahasam, D.; Kumar, A.; Jayachandran, B.; Gopalan, R. Thermoelectric Properties of Sb Doped AlFe2B2. arXiv 2022, arXiv:2212.01193. [Google Scholar] [CrossRef]
- Barua, R.; Lejeune, B.T.; Jensen, B.A.; Ke, L.; McCallum, R.W.; Kramer, M.J.; Lewis, L.H. Enhanced Room-Temperature Magnetocaloric Effect and Tunable Magnetic Response in Ga-and Ge-Substituted AlFe2B2. J. Alloys Compd. 2019, 777, 1030–1038. [Google Scholar] [CrossRef]
- Levin, E.M.; Jensen, B.A.; Barua, R.; Lejeune, B.; Howard, A.; McCallum, R.W.; Kramer, M.J.; Lewis, L.H. Effects of Al Content and Annealing on the Phases Formation, Lattice Parameters, and Magnetization of AlxFe2B2 (x = 1.0, 1.1, 1.2) Alloys. Phys. Rev. Mater. 2018, 2, 034403. [Google Scholar] [CrossRef]
- Lamichhane, T.N.; Xiang, L.; Lin, Q.; Pandey, T.; Parker, D.S.; Kim, T.H.; Zhou, L.; Kramer, M.J.; Bud’Ko, S.L.; Canfield, P.C. Magnetic Properties of Single Crystalline Itinerant Ferromagnet AlFe2B2. Phys. Rev. Mater. 2018, 2, 084408. [Google Scholar] [CrossRef]
- Han, K.; Li, M.; Gao, M.; Wang, X.; Huo, J.; Wang, J.Q. Improved Magnetocaloric Effects in AlFe2B2 Intermetallics through the Enhancement of Magnetoelastic Coupling. J. Alloys Compd. 2022, 908, 164663. [Google Scholar] [CrossRef]
- Lejeune, B.T.; Barua, R.; Simsek, E.; McCallum, R.W.; Ott, R.T.; Kramer, M.J.; Lewis, L.H. Towards Additive Manufacturing of Magnetocaloric Working Materials. Materialia 2021, 16, 101071. [Google Scholar] [CrossRef]
- Hirt, S.; Yuan, F.; Mozharivskyj, Y.; Hillebrecht, H. AlFe2−XCoxB2 (x = 0–0.30): TC Tuning through Co Substitution for a Promising Magnetocaloric Material Realized by Spark Plasma Sintering. Inorg. Chem. 2016, 55, 9677–9684. [Google Scholar] [CrossRef] [PubMed]
- Mann, D.K.; Wang, Y.X.; Marks, J.D.; Strouse, G.F.; Shatruk, M. Microwave Synthesis and Magnetocaloric Effect in AlFe2B2. Inorg. Chem. 2020, 59, 12625–12631. [Google Scholar] [CrossRef] [PubMed]
- Elmassalami, M.; Oliveira, D.D.S.; Takeya, H. On the Ferromagnetism of AlFe2B2. J. Magn. Magn. Mater. 2011, 323, 2133–2136. [Google Scholar] [CrossRef]
- Cedervall, J.; Andersson, M.S.; Sarkar, T.; Delczeg-Czirjak, E.K.; Bergqvist, L.; Hansen, T.C.; Beran, P.; Nordblad, P.; Sahlberg, M. Magnetic Structure of the Magnetocaloric Compound AlFe2B2. J. Alloys Compd. 2016, 664, 784–791. [Google Scholar] [CrossRef]
- Cedervall, J.; Häggström, L.; Ericsson, T.; Sahlberg, M. Mössbauer Study of the Magnetocaloric Compound AlFe2B2. Hyperfine Interact. 2016, 237, 47. [Google Scholar] [CrossRef]
- Barua, R.; Lejeune, B.T.; Ke, L.; Hadjipanayis, G.; Levin, E.M.; McCallum, R.W.; Kramer, M.J.; Lewis, L.H. Anisotropic Magnetocaloric Response in AlFe2B2. J. Alloys Compd. 2018, 745, 505–512. [Google Scholar] [CrossRef]
- Lejeune, B.T.; Du, X.; Barua, R.; Zhao, J.C.; Lewis, L.H. Anisotropic Thermal Conductivity of Magnetocaloric AlFe2B2. Materialia 2018, 1, 150–154. [Google Scholar] [CrossRef]
- Sharma, V.; Dey, M.; Duong, A.; Gupta, S.; Barua, R. Magnetofunctional Response of AlFe2B2 Powders Synthesized in Open Air via Molten Salt Shielded/Sintering Method. MRS Commun. 2023, 13, 574–580. [Google Scholar] [CrossRef]
- Bennett, S.P.; Kota, S.; ElBidweihy, H.; Parker, J.F.; Hanner, L.A.; Finkel, P.; Barsoum, M.W. Magnetic and Magnetocaloric Properties of Fe2AlB2 Synthesized by Single-Step Reactive Hot Pressing. Scr. Mater. 2020, 188, 244–248. [Google Scholar] [CrossRef]
- Oey, Y.M.; Bocarsly, J.D.; Mann, D.; Levin, E.E.; Shatruk, M.; Seshadri, R. Structural Changes upon Magnetic Ordering in Magnetocaloric AlFe2B2. Appl. Phys. Lett. 2020, 116, 212403. [Google Scholar] [CrossRef]
- Sharma, S.; Kovalev, A.E.; Rebar, D.J.; Mann, D.; Yannello, V.; Shatruk, M.; Suslov, A.V.; Smith, J.H.; Siegrist, T. Magnetostriction of AlFe2B2 in High Magnetic Fields. Phys. Rev. Mater. 2021, 5, 064409. [Google Scholar] [CrossRef]
- Lamichhane, T.N.; Rana, K.; Lin, Q.; Bud’Ko, S.L.; Furukawa, Y.; Canfield, P.C. Near Room Temperature Antiferromagnetic Ordering with a Potential Low-Dimensional Magnetism in AlMn2B2. Phys. Rev. Mater. 2019, 3, 064415. [Google Scholar] [CrossRef]
- Cedervall, J.; Andersson, M.S.; Iuşan, D.; Delczeg-Czirjak, E.K.; Jansson, U.; Nordblad, P.; Sahlberg, M. Magnetic and Mechanical Effects of Mn Substitutions in AlFe2B2. J. Magn. Magn. Mater. 2019, 482, 54–60. [Google Scholar] [CrossRef]
- Lejeune, B.T.; Barua, R.; Mudryk, Y.; Kramer, M.J.; McCallum, R.W.; Pecharsky, V.; Lewis, L.H. Borderline First-Order Magnetic Phase Transition in AlFe2B2. J. Alloys Compd. 2021, 886, 161150. [Google Scholar] [CrossRef]
- Scheibel, F.; Gottschall, T.; Taubel, A.; Fries, M.; Skokov, K.P.; Terwey, A.; Keune, W.; Ollefs, K.; Wende, H.; Farle, M.; et al. Hysteresis design of magnetocaloric materials—From basic mechanisms to applications. Energy Technol. 2018, 6, 1397–1428. [Google Scholar] [CrossRef]
- Wang, S.; Liu, P.; Chen, J.; Cui, W. Substitution Effects on the Magnetic Phase Transition and Magnetocaloric Effects in Nanolaminated AlFe2B2 alloys. AIP Adv. 2022, 12, 035235. [Google Scholar] [CrossRef]
- Du, Q.; Chen, G.; Yang, W.; Wei, J.; Hua, M.; Du, H.; Wang, C.; Liu, S.; Han, J.; Zhang, Y.; et al. Magnetic Frustration and Magnetocaloric Effect in AlFe2−XMnxB2 (x = 0–0.5) Ribbons. J. Phys. D. Appl. Phys. 2015, 48, 335001. [Google Scholar] [CrossRef]
- Chai, P.; Stoian, S.A.; Tan, X.; Dube, P.A.; Shatruk, M. Investigation of Magnetic Properties and Electronic Structure of Layered-Structure Borides AlT2B2 (T = Fe, Mn, Cr) and AlFe2−XMnxB2. J. Solid State Chem. 2015, 224, 52–61. [Google Scholar] [CrossRef]
- Lejeune, B.T.; Jensen, B.A.; Barua, R.; Stonkevitch, E.; McCallum, R.W.; Kramer, M.J.; Lewis, L.H. Lattice-Driven Magnetic Transitions in Al(Fe,T) 2 X 2 Compounds. J. Magn. Magn. Mater. 2019, 481, 262–267. [Google Scholar] [CrossRef]
- Zhang, Z.; Yao, G.; Zhang, L.; Jia, P.; Fu, X.; Cui, W.; Wang, Q. Magnetic Phase Transition and Room-Temperature Magnetocaloric Effects in (Al,M)Fe2B2 (M = Si, Ga) Compounds. J. Magn. Magn. Mater. 2019, 484, 154–158. [Google Scholar] [CrossRef]
- Stillwell, K.; Kramer, N.; Birch, B.; Reese, B.; Pathak, A.K.; Khan, M. The Magnetic and Magnetocaloric Properties of Al-Rich Al0.85+XSi0.15Fe2B2 compounds Prepared by Drop-Casting. AIP Adv. 2023, 13, 025132. [Google Scholar] [CrossRef]
- Beckmann, B.; El-Melegy, T.A.; Koch, D.; Wiedwald, U.; Farle, M.; Maccari, F.; Snyder, J.; Skokov, K.P.; Barsoum, M.W.; Gutfleisch, O. Reactive single-step hot-pressing and magnetocaloric performance of polycrystalline Fe2Al1.15−xB2GexGax (x = 0, 0.05) MAB phases. J. Appl. Phys. 2023, 133, 173903. [Google Scholar] [CrossRef]
- Kitanovski, A. Energy Applications of Magnetocaloric Materials. Adv. Energy Mater. 2020, 10, 1903741. [Google Scholar] [CrossRef]
- Cheng, Y.; Lv, Z.L.; Chen, X.R.; Cai, L.C. Structural, Electronic and Elastic Properties of AlFe2B 2: First-Principles Study. Comput. Mater. Sci. 2014, 92, 253–257. [Google Scholar] [CrossRef]
- Kádas, K.; Iuşan, D.; Hellsvik, J.; Cedervall, J.; Berastegui, P.; Sahlberg, M.; Jansson, U.; Eriksson, O. AlM2B2 (M = Cr, Mn, Fe, Co, Ni): A Group of Nanolaminated Materials. J. Phys. Condens. Matter 2017, 29, 155402. [Google Scholar] [CrossRef] [PubMed]
- Qin, K.; Qi, X.; Gao, J.; He, X.; Yin, H.; Yin, W.; Song, G.; Zheng, Y.; Bai, Y. Improving the Density Functional Theory Prediction Accuracy for Elastic Moduli and Thermal Expansion of MoAlB, Fe2AlB2, and Mn2AlB2. J. Am. Ceram. Soc. 2024, 107, 5313–5325. [Google Scholar] [CrossRef]
- Atalay, A.S.; Derin, B. Mechanical Effects of Cr and V Substitutions in AlFe2B2 by First-Principles Calculations. Comput. Mater. Sci. 2024, 239, 112960. [Google Scholar] [CrossRef]
- Liu, Y.Z.; Sun, L.; Zheng, B.C.; Yi, Y.L.; Zhai, W.Y.; Peng, J.H.; Li, W. Anisotropic Elastic, Thermal Properties and Electronic Structures of M2AlB2 (M = Fe, Cr, and Mn) Layer Structure Ceramics. Ceram. Int. 2021, 47, 1421–1428. [Google Scholar] [CrossRef]
- Wang, R.; Tao, X.; Ouyang, Y.; Chen, H.; Peng, Q. Suggest a New Approach to Fabricate AlFe2B2. Comput. Mater. Sci. 2020, 171, 109239. [Google Scholar] [CrossRef]
- Wang, Q.; Ding, H.; Tian, F. Temperature Dependent Mechanical Properties of MAB Phase Fe2AlB2. Comput. Condens. Matter 2023, 34, e00786. [Google Scholar] [CrossRef]
- Aydin, S.; Şimşek, M. Pressure-Induced Magnetic Phase Transitions of Intermetallic Fe2AlB2. J. Magn. Magn. Mater. 2020, 502, 166453. [Google Scholar] [CrossRef]
- Pugh, S.F. XCII. Relations between the Elastic Moduli and the Plastic Properties of Polycrystalline Pure Metals. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1954, 45, 823–843. [Google Scholar] [CrossRef]
- Bai, Y.; Sun, D.; Li, N.; Kong, F.; Qi, X.; He, X.; Wang, R.; Zheng, Y. High-Temperature Mechanical Properties and Thermal Shock Behavior of Ternary-Layered MAB Phases Fe2AlB2. Int. J. Refract. Met. Hard Mater. 2019, 80, 151–160. [Google Scholar] [CrossRef]
- Liu, J.; Li, S.; Yao, B.; Hu, S.; Zhang, J.; Yu, W.; Zhou, Y. Rapid Synthesis and Characterization of a Nanolaminated Fe2AlB2 Compound. J. Alloys Compd. 2018, 766, 488–497. [Google Scholar] [CrossRef]
- Liu, J.; Li, S.; Yao, B.; Zhang, J.; Lu, X.; Zhou, Y. Thermal Stability and Thermal Shock Resistance of Fe2AlB2. Ceram. Int. 2018, 44, 16035–16039. [Google Scholar] [CrossRef]
- Li, N.; Bai, Y.; Wang, S.; Zheng, Y.; Kong, F.; Qi, X.; Wang, R.; He, X.; Duff, A.I. Rapid Synthesis, Electrical, and Mechanical Properties of Polycrystalline Fe2AlB2 Bulk from Elemental Powders. J. Am. Ceram. Soc. 2017, 100, 4407–4411. [Google Scholar] [CrossRef]
- Verger, L.; Kota, S.; Roussel, H.; Ouisse, T.; Barsoum, M.W. Anisotropic Thermal Expansions of Select Layered Ternary Transition Metal Borides: MoAlB, Cr2AlB2, Mn2AlB2, and Fe2AlB2. J. Appl. Phys. 2018, 124, 205108. [Google Scholar] [CrossRef]
- Zhang, X.; Lejeune, B.T.; Barua, R.; McCallum, R.W.; Lewis, L.H. Estimating the In-Operando Stabilities of AlFe2B2-Based Compounds for Magnetic Refrigeration. J. Alloys Compd. 2020, 823, 153693. [Google Scholar] [CrossRef]
- Funk, A.; Zeilinger, M.; Miehe, A.; Sopu, D.; Eckert, J.; Dötz, F.; Waske, A. MnFePSi-Based Magnetocaloric Packed Bed Regenerators: Structural Details Probed by X-ray Tomography. Chem. Eng. Sci. 2018, 175, 84–90. [Google Scholar] [CrossRef]
- Lei, T.; Engelbrecht, K.; Nielsen, K.K.; Veje, C.T. Study of Geometries of Active Magnetic Regenerators for Room Temperature Magnetocaloric Refrigeration. Appl. Therm. Eng. 2017, 111, 1232–1243. [Google Scholar] [CrossRef]
- Sharma, V.; Balderson, L.; Heo, R.; Bishop, O.; Hunt, C.S.M.; Carpenter, E.E.; Hadimani, R.L.; Zhao, H.; Barua, R. Room-Temperature Polymer-Assisted Additive Manufacturing of Microchanneled Magnetocaloric Structures. J. Alloys Compd. 2022, 920, 165891. [Google Scholar] [CrossRef]
- Mugica, I.; Poncet, S.; Bouchard, J. Detailed Numerical Simulations of a Single Stage of Rotatory Active Magnetic Regenerators: Influence of the Pin Geometry. Int. J. Therm. Sci. 2020, 149, 106198. [Google Scholar] [CrossRef]
- Joo, J.H.; Kang, B.S.; Kang, K.J. Experimental Studies on Friction Factor and Heat Transfer Characteristics through Wire-Woven Bulk Kagome Structure. Exp. Heat Transf. 2009, 22, 99–116. [Google Scholar] [CrossRef]
- Pulko, B.; Tušek, J.; Moore, J.D.; Weise, B.; Skokov, K.; Mityashkin, O.; Kitanovski, A.; Favero, C.; Fajfar, P.; Gutfleisch, O.; et al. Epoxy-Bonded La-Fe-Co-Si Magnetocaloric Plates. J. Magn. Magn. Mater. 2015, 375, 65–73. [Google Scholar] [CrossRef]
- Mohamed, A.E.M.A.; Jeong, M.; Sheridan, R.S.; Attallah, M.M. Enabling High Efficiency Magnetic Refrigeration Using Laser Powder Bed Fusion of Porous LaCe(Fe,Mn,Si)13 Structures. Addit. Manuf. 2022, 51, 102620. [Google Scholar] [CrossRef]
- Nilsén, F.; Ituarte, I.F.; Salmi, M.; Partanen, J.; Hannula, S.P. Effect of Process Parameters on Non-Modulated Ni-Mn-Ga Alloy Manufactured Using Powder Bed Fusion. Addit. Manuf. 2019, 28, 464–474. [Google Scholar] [CrossRef]
- Laitinen, V.; Salminen, A.; Ullakko, K. First Investigation on Processing Parameters for Laser Powder Bed Fusion of Ni-Mn-Ga Magnetic Shape Memory Alloy. J. Laser Appl. 2019, 31, 022303. [Google Scholar] [CrossRef]
- Laitinen, V.; Sozinov, A.; Saren, A.; Salminen, A.; Ullakko, K. Laser Powder Bed Fusion of Ni-Mn-Ga Magnetic Shape Memory Alloy. Addit. Manuf. 2019, 30, 100891. [Google Scholar] [CrossRef]
- Imaizumi, K.; Fujita, A.; Suzuki, A.; Kobashi, M.; Kato, M. Additive Manufacturing for 3D Microchannel Structure Using La(FexSi1−x)13 Magnetic Refrigerant via Laser Powder Bed Fusion. Addit. Manuf. 2024, 83, 104076. [Google Scholar] [CrossRef]
- Zhong, S.; Qian, M.; Zhang, J.; Zhang, Q.; Sun, L.; Shen, P.; Zhang, X.; Geng, L. Microstructure and Magnetocaloric Effect in Nonequilibrium Solidified Ni-Mn-Sn-Co Alloy Prepared by Laser Powder Bed Fusion. Addit. Manuf. 2024, 79, 103941. [Google Scholar] [CrossRef]
- Sun, K.; Mohamed, A.E.M.A.; Li, S.; Jeong, M.; Head, J.; Attallah, M.M. Laser Powder Bed Fusion of the Ni-Mn-Sn Heusler Alloy for Magnetic Refrigeration Applications. Addit. Manuf. 2023, 69, 103536. [Google Scholar] [CrossRef]
- Lu, X.; Miao, L.; Zhang, Y.; Wang, Z.; Zhang, H.; Li, G.; Liu, J. First-Order Phase Transition La-Fe-Si Bulk Materials with Small Hysteresis by Laser Powder Bed Fusion: Microstructure and Magnetocaloric Effect. Scr. Mater. 2023, 232, 115479. [Google Scholar] [CrossRef]
- Stevens, E.; Kimes, K.; Salazar, D.; Mostafaei, A.; Rodriguez, R.; Acierno, A.; Lázpita, P.; Chernenko, V.; Chmielus, M. Mastering a 1.2 K Hysteresis for Martensitic Para-Ferromagnetic Partial Transformation in Ni-Mn(Cu)-Ga Magnetocaloric Material via Binder Jet 3D Printing. Addit. Manuf. 2021, 37, 101560. [Google Scholar] [CrossRef]
- Gokuldoss, P.K.; Kolla, S.; Eckert, J. Additive Manufacturing Processes: Selective Laser Melting, Electron Beam Melting and Binder Jetting-Selection Guidelines. Materials 2017, 10, 672. [Google Scholar] [CrossRef] [PubMed]
- Sharma, V. Extrusion-Based Additive Manufacturing of Magnetic Heat Exchange Structures for Caloric Applications. Ph.D. Thesis, Virginia Commonwealth University, Richmond, VA, USA, 2024. [Google Scholar]
- Taylor, S.L.; Shah, R.N.; Dunand, D.C. Ni-Mn-Ga Micro-Trusses via Sintering of 3D-Printed Inks Containing Elemental Powders. Acta Mater. 2018, 143, 20–29. [Google Scholar] [CrossRef]
- Mostafaei, A.; Rodriguez De Vecchis, P.; Stevens, E.L.; Chmielus, M. Sintering Regimes and Resulting Microstructure and Properties of Binder Jet 3D Printed Ni-Mn-Ga Magnetic Shape Memory Alloys. Acta Mater. 2018, 154, 355–364. [Google Scholar] [CrossRef]
- Dri, K.D.N.; Charpentier, N.; Hirsinger, L.; Gilbin, A.; Barriere, T.; Dri, K.D.N.; Charpentier, N.; Hirsinger, L.; Gilbin, A.; Highly, T.B. Highly Loaded Magnetocaloric Composites by La(Fe,Si)13H Powder Dedicated to Extrusion-Based Additive Manufacturing Applications. Powder Technol. 2023, 425, 118616. [Google Scholar] [CrossRef]
- Wieland, S.; Petzoldt, F. Powder-Extrusion and Sintering of Magnetocaloric LaCe(FeMnSi)13 Alloy. J. Alloys Compd. 2017, 719, 182–188. [Google Scholar] [CrossRef]
- Stevens, E.; Kimes, K.; Chernenko, V.; Lázpita, P.; Wojcik, A.; Maziarz, W.; Toman, J.; Chmielus, M. Effect of Homogenization on the Microstructure and Magnetic Properties of Direct Laser-Deposited Magnetocaloric Ni43Co7Mn39Sn11. J. Manuf. Sci. Eng. Trans. ASME 2020, 142, 071006. [Google Scholar] [CrossRef]
- Toman, J.; Müllner, P.; Chmielus, M. Properties of As-Deposited and Heat-Treated Ni-Mn-Ga Magnetic Shape Memory Alloy Processed by Directed Energy Deposition. J. Alloys Compd. 2018, 752, 455–463. [Google Scholar] [CrossRef]
- Miao, X.; Wang, W.; Liang, H.; Qian, F.; Cong, M.; Zhang, Y.; Muhammad, A.; Tian, Z.; Xu, F. Printing (Mn,Fe)2(P,Si) Magnetocaloric Alloys for Magnetic Refrigeration Applications. J. Mater. Sci. 2020, 55, 6660–6668. [Google Scholar] [CrossRef]
- Moore, J.D.; Klemm, D.; Lindackers, D.; Grasemann, S.; Träger, R.; Eckert, J.; Löber, L.; Scudino, S.; Katter, M.; Barcza, A.; et al. Selective Laser Melting of La(Fe,Co,Si)13 Geometries for Magnetic Refrigeration. J. Appl. Phys. 2013, 114, 043907. [Google Scholar] [CrossRef]
- Navickaitė, K.; Liang, J.; Bahl, C.; Wieland, S.; Buchenau, T.; Engelbrecht, K. Experimental Characterization of Active Magnetic Regenerators Constructed Using Laser Beam Melting Technique. Appl. Therm. Eng. 2020, 174, 115297. [Google Scholar] [CrossRef]
- Wieland, S.; Goossens, N.; Weck, C.; Hein, S.B. Additive Manufacturing of Functional Metallic Materials; Euro PM2019 Congress & Exhibition: Maastricht, The Netherlands, 2019. [Google Scholar]
- Nikitin, S.A.; Skokov, K.P.; Koshkid’Ko, Y.S.; Pastushenkov, Y.G.; Ivanova, T.I. Giant Rotating Magnetocaloric Effect in the Region of Spin-Reorientation Transition in the NdCo5 Single Crystal. Phys. Rev. Lett. 2010, 105, 137205. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Zhang, M.; Ouyang, Y.; Liu, J.; Zhang, H. Enhancement of Rotating Magnetocaloric Effect by Fe Substitution in NdCo5-XFex Alloys. Intermetallics 2020, 118, 106676. [Google Scholar] [CrossRef]
- Hu, Y.; Hu, Q.B.; Wang, C.C.; Cao, Q.Q.; Gao, W.L.; Wang, D.H.; Du, Y.W. Large Room-Temperature Rotating Magnetocaloric Effect in NdCo4Al Polycrystalline Alloy. Solid State Commun. 2017, 250, 45–48. [Google Scholar] [CrossRef]
- Almeida, R.; Freitas, S.C.; Fernandes, C.R.; Kiefe, R.; Araújo, J.P.; Amaral, J.S.; Ventura, J.O.; Belo, J.H.; Silva, D.J. Rotating Magnetocaloric Effect in Polycrystals—Harnessing the Demagnetizing Effect. J. Phys. 2024, 6, 015020. [Google Scholar] [CrossRef]
- Sousa, J.B.; Pinto, R.P.; Amado, M.M.; Pinheiro, M.F.; Moreira, J.M.; Braga, M.E. Critical Behaviour of the Thermal Conductivity Near the Curie Point of Gadolinium. J. Phys. Paris 1980, 41, 573–578. [Google Scholar] [CrossRef]
- Szade, J.; Skorek, G. Electronic Structure and Magnetism of Gds (Si, Ge) 4 Compounds. J. Magn. Magn. Mater. 1999, 197, 699–700. [Google Scholar] [CrossRef]
- Brück, E. Developments in Magnetocaloric Refrigeration. J. Phys. D Appl. Phys. 2005, 38, R381. [Google Scholar] [CrossRef]
- Palstra, T.T.M.; Mydosh, J.A.; Nieuwenhuys, G.J.; van der Kraan, A.M.; Buschow, K.H.J. Study of the Critical Behaviour of the Magnetization and Electrical Resistivity in Cubic La(Fe, Si)13 Compounds. J. Magn. Magn. Mater. 1983, 36, 290–296. [Google Scholar] [CrossRef]
- Court, C.J.; Jain, A.; Cole, J.M. Inverse Design of Materials That Exhibit the Magnetocaloric Effect by Text-Mining of the Scientific Literature and Generative Deep Learning. Chem. Mater. 2021, 33, 7217–7231. [Google Scholar] [CrossRef]
- Hartnett, T.Q.; Sharma, V.; Garg, S.; Barua, R.; Balachandran, P.V. Accelerated Design of MTX Alloys with Targeted Magnetostructural Properties through Interpretable Machine Learning. Acta Mater. 2022, 231, 117891. [Google Scholar] [CrossRef]
- Maiorino, A.; Del Duca, M.G.; Tušek, J.; Tomc, U.; Kitanovski, A.; Aprea, C. Evaluating Magnetocaloric Effect in Magnetocaloric Materials: A Novel Approach Based on Indirect Measurements Using Artificial Neural Networks. Energies 2019, 12, 1871. [Google Scholar] [CrossRef]
- Ali, T.; Khan, M.N.; Ahmed, E.; Ali, A. Phase Analysis of AlFe2B2 by Synchrotron X-Ray Diffraction, Magnetic and Mössbauer Studies. Prog. Nat. Sci. Mater. Int. 2017, 27, 251–256. [Google Scholar] [CrossRef]
- Lee, J.W.; Song, M.S.; Cho, K.K.; Cho, B.K.; Nam, C. Magnetocaloric Properties of AlFe2B2 Including Paramagnetic Impurities of Al13Fe4. J. Korean Phys. Soc. 2018, 73, 1555–1560. [Google Scholar] [CrossRef]
- Wang, S.; Liu, P.; Chen, J.; Cui, W. Magnetostriction and Heat-Capacity Study on the Metamagnetic Phase Transition of Dy2In1-XAlxalloys. AIP Adv. 2022, 12, 1–5. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharma, V.; Barua, R. Synthesis, Characterization, and Magnetocaloric Properties of the Ternary Boride Fe2AlB2 for Caloric Applications. Materials 2024, 17, 3886. https://doi.org/10.3390/ma17163886
Sharma V, Barua R. Synthesis, Characterization, and Magnetocaloric Properties of the Ternary Boride Fe2AlB2 for Caloric Applications. Materials. 2024; 17(16):3886. https://doi.org/10.3390/ma17163886
Chicago/Turabian StyleSharma, Vaibhav, and Radhika Barua. 2024. "Synthesis, Characterization, and Magnetocaloric Properties of the Ternary Boride Fe2AlB2 for Caloric Applications" Materials 17, no. 16: 3886. https://doi.org/10.3390/ma17163886
APA StyleSharma, V., & Barua, R. (2024). Synthesis, Characterization, and Magnetocaloric Properties of the Ternary Boride Fe2AlB2 for Caloric Applications. Materials, 17(16), 3886. https://doi.org/10.3390/ma17163886





