Low-Cycle Fatigue Damage Mechanism and Life Prediction of High-Strength Compacted Graphite Cast Iron at Different Temperatures
Abstract
:1. Introduction
2. Experimental Materials and Procedures
2.1. Research Material
2.2. Microstructure Analysis
2.3. Mechanical Properties
3. Results
3.1. Tensile Properties
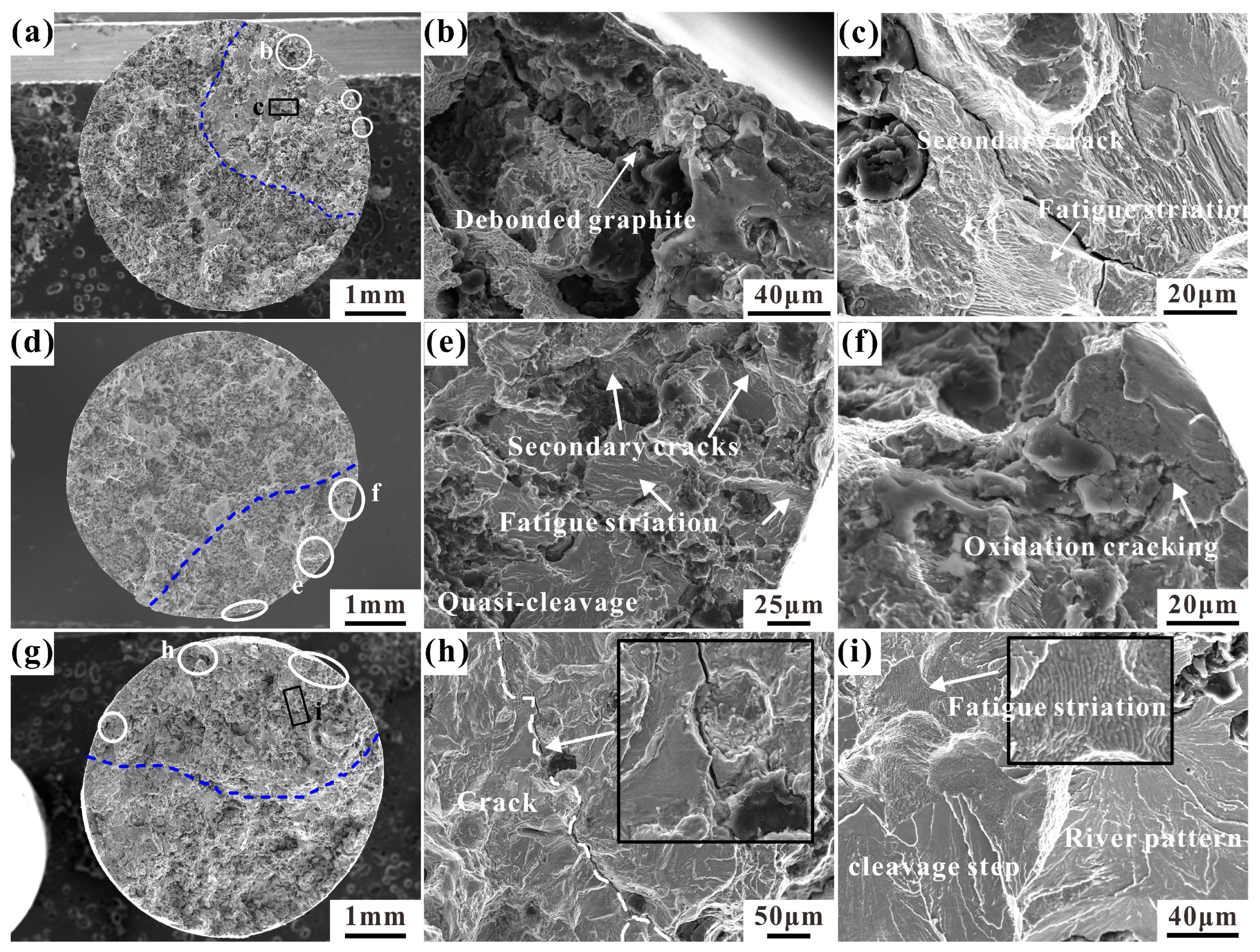
3.2. Tensile Fracture Morphologies
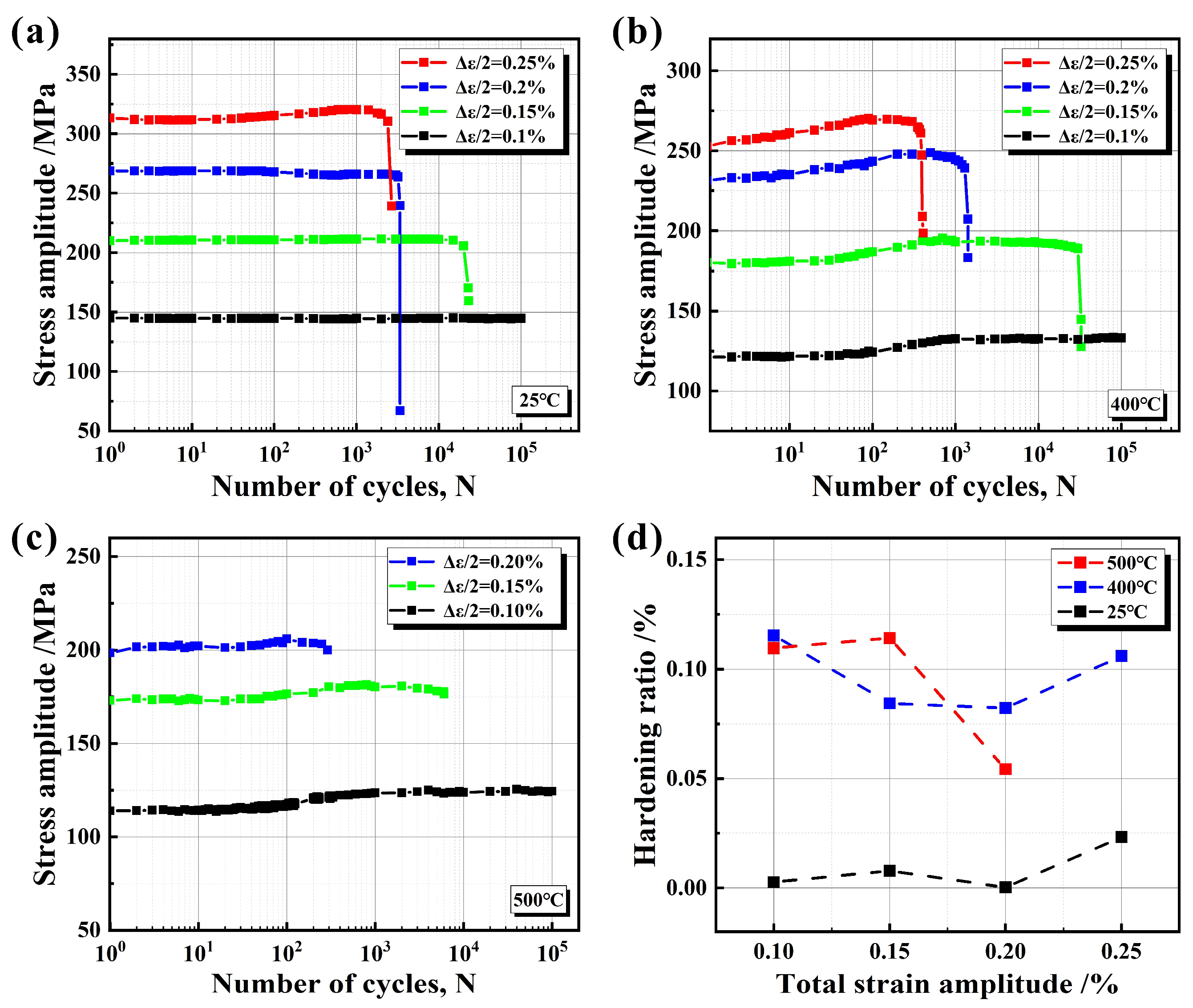
3.3. LCF Properties
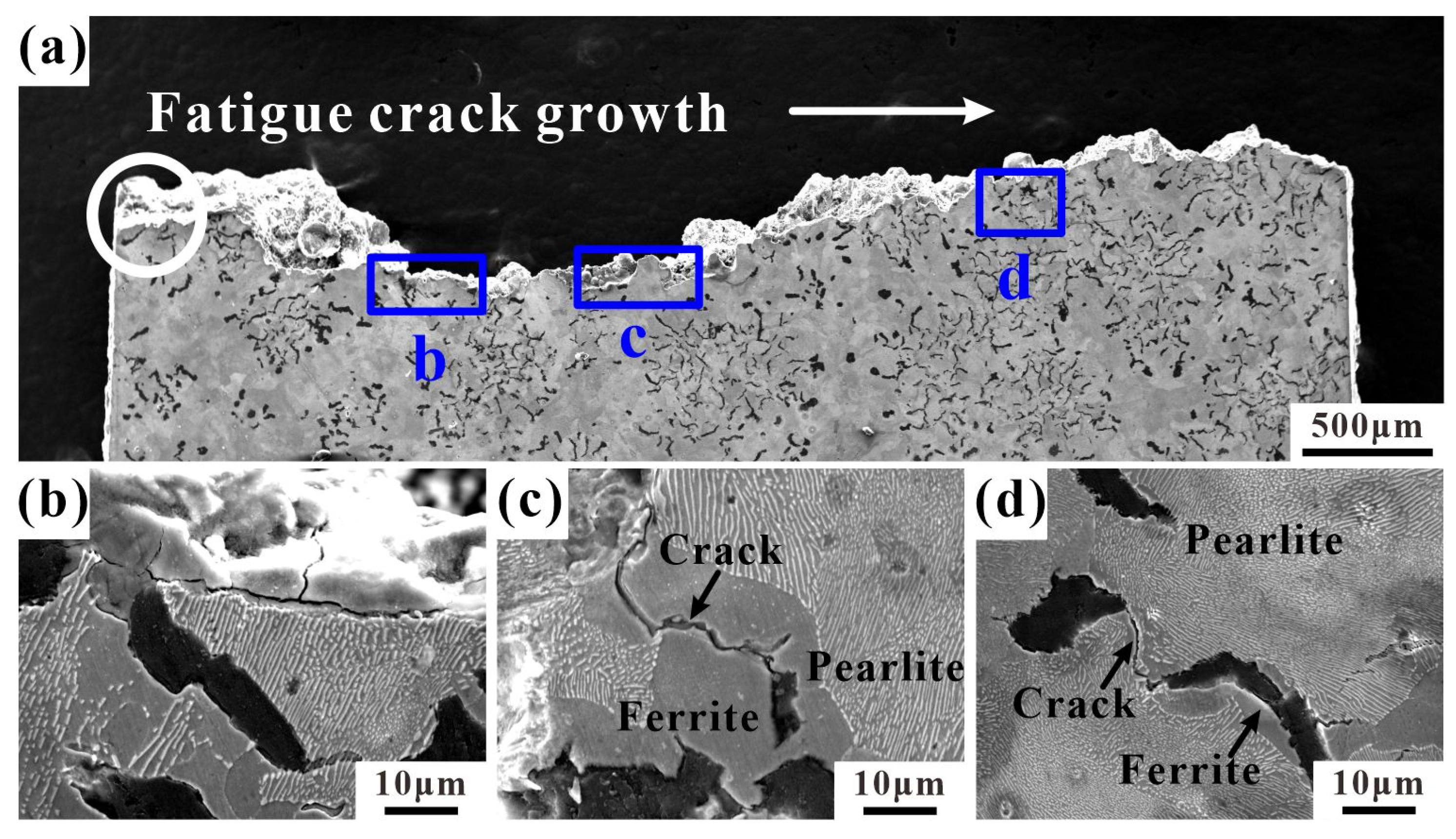
3.4. Fatigue Fracture Morphology
4. Discussion
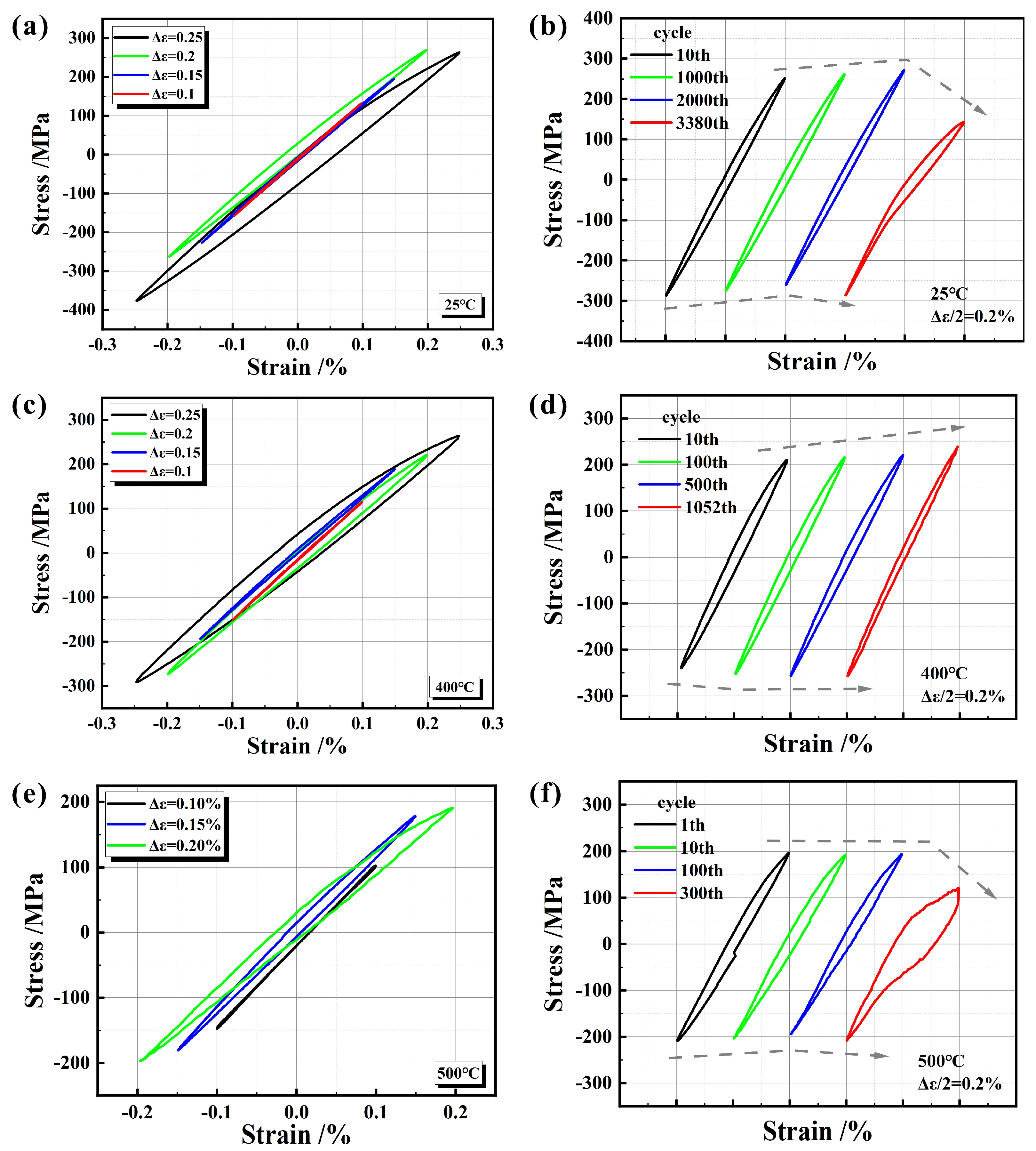
4.1. Cyclic Stress Response Curves
4.2. Hysteresis Loops
4.3. Crack Growth and Fracture Profiles
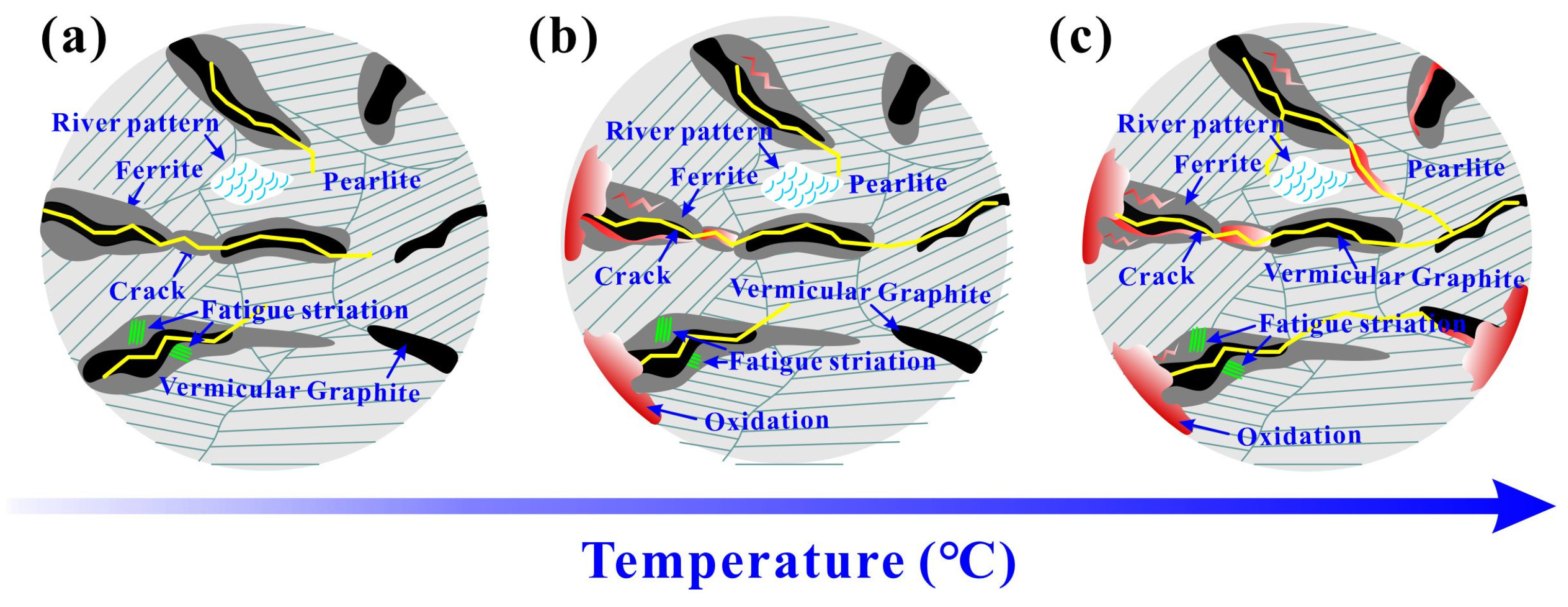
4.4. Damage Mechanism
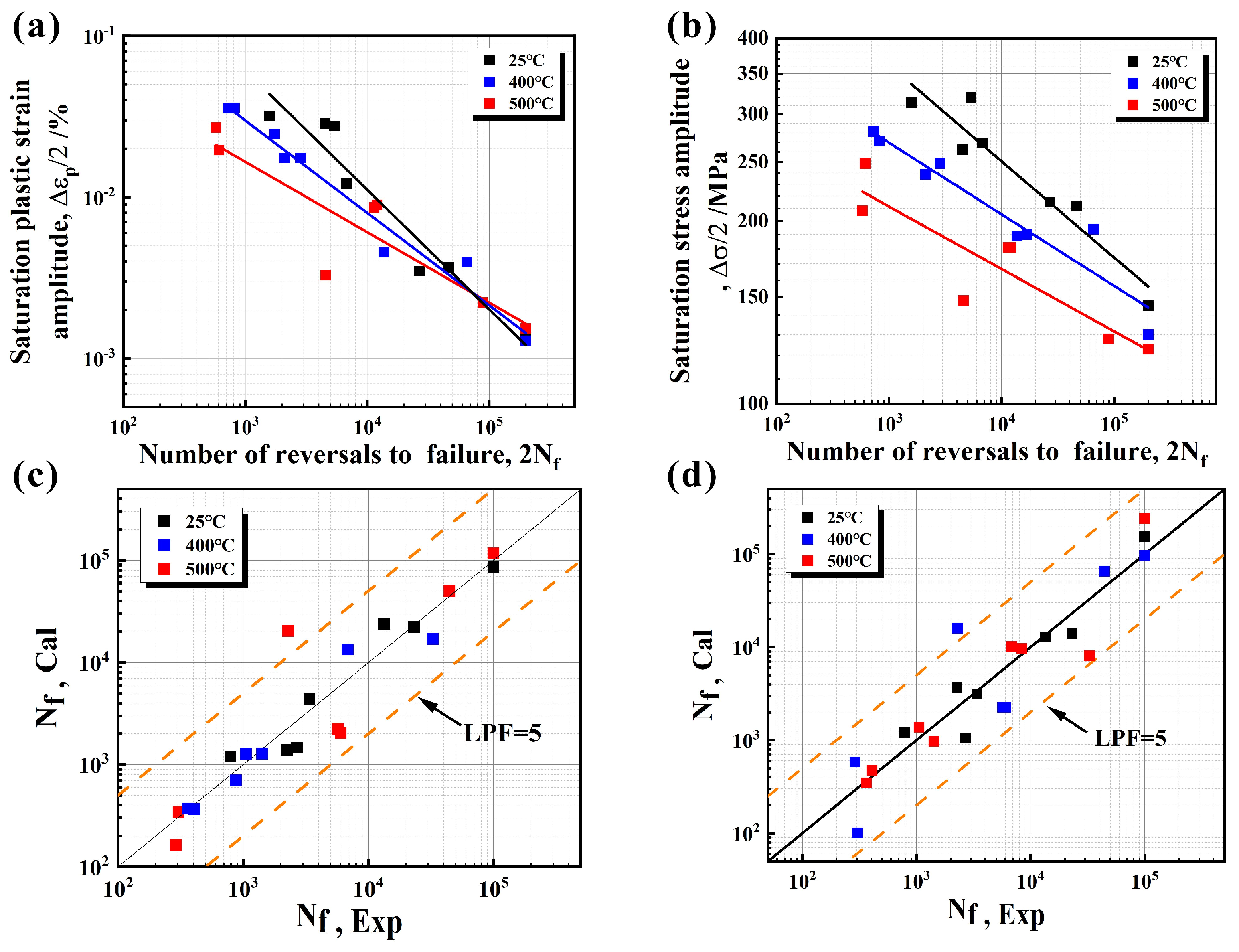
4.5. Prediction of LCF Life
5. Conclusions
- With the increase in experimental temperature, the yield ratio of RuT450 increases, and dimples appear in the tensile fracture at high temperatures. The decreasing trend of LCF life at 500 °C was significantly higher than those at 25 °C and 400 °C.
- At 25 °C, the fatigue crack initiates from the graphite tip and propagates along the inside or edge of the ferrite. At 400 °C, a layer of oxide film appears at the edge of the sample and around the crack, and the crack propagates along the ferrite and pearlite. At 500 °C, the oxidation cracking of graphite and matrix is obvious, and the dependence of crack propagation on ferrite greatly reduces.
- The LCF life prediction model, based on the linear relationships of the energy method parameters, can more effectively predict the LCF life of CGI compared with the other two models.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, Y.; Liu, J.; Zhong, G.; Huang, W.; Zou, R. Analysis of a Diesel Engine Cylinder Head Failure Caused by Casting Porosity Defects. Eng. Fail. Anal. 2021, 127, 105498. [Google Scholar] [CrossRef]
- Norman, V.; Skoglund, P.; Moverare, J. Damage Evolution in Compacted Graphite Iron during Thermomechanical Fatigue Testing. Int. J. Cast Met. Res. 2016, 29, 26–33. [Google Scholar] [CrossRef]
- Pierce, D.; Haynes, A.; Hughes, J.; Graves, R.; Maziasz, P.; Muralidharan, G.; Shyam, A.; Wang, B.; England, R.; Daniel, C. High Temperature Materials for Heavy Duty Diesel Engines: Historical and Future Trends. Prog. Mater. Sci. 2019, 103, 109–179. [Google Scholar] [CrossRef]
- Lee, Y.S.; Kong, J.P.; Lee, J.O.; Lee, H.S.; Kim, J.H.; Jun, J.T.; Kim, C.G. A Study on the Fatigue Analysis of Heavy Duty Diesel Engine. Mod. Phys. Lett. B 2008, 22, 929–934. [Google Scholar] [CrossRef]
- Hosdez, J.; Limodin, N.; Najjar, D.; Witz, J.-F.; Charkaluk, E.; Osmond, P.; Forré, A.; Szmytka, F. Fatigue Crack Growth in Compacted and Spheroidal Graphite Cast Irons. Int. J. Fatigue 2020, 131, 105319. [Google Scholar] [CrossRef]
- Sharifi, S.M.H.; Saeidi Googarchin, H.; Forouzesh, F. Three Dimensional Analysis of Low Cycle Fatigue Failure in Engine Part Subjected to Multi-Axial Variable Amplitude Thermo-Mechanical Load. Eng. Fail. Anal. 2016, 62, 128–141. [Google Scholar] [CrossRef]
- Natesan, E.; Eriksson, S.; Ahlström, J.; Persson, C. Deformation and Fatigue Behaviour of A356-T7 Cast Aluminium Alloys Used in High Specific Power Ic Engines. Materials 2019, 12, 3033. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Huang, W.; Liu, J.; Ren, P.; Zuo, Z.; Zhang, L. Study on Scatter in Mechanical Properties of Cylinder Head with Complex Structure Characters. Eng. Fail. Anal. 2022, 135, 106114. [Google Scholar] [CrossRef]
- Li, Y.; Liu, J.; Zhang, Q.; Huang, W. Casting Defects and Microstructure Distribution Characteristics of Aluminum Alloy Cylinder Head with Complex Structure. Mater. Today Commun. 2021, 27, 102416. [Google Scholar] [CrossRef]
- Kihlberg, E.; Norman, V.; Skoglund, P.; Schmidt, P.; Moverare, J. On the Correlation between Microstructural Parameters and the Thermo-Mechanical Fatigue Performance of Cast Iron. Int. J. Fatigue 2021, 145, 106112. [Google Scholar] [CrossRef]
- Lim, C.H.; Goo, B.C. Development of Compacted Vermicular Graphite Cast Iron for Railway Brake Discs. Met. Mater. Int. 2011, 17, 199–205. [Google Scholar] [CrossRef]
- Šamec, B.; Potrč, I.; Šraml, M. Low Cycle Fatigue of Nodular Cast Iron Used for Railway Brake Discs. Eng. Fail. Anal. 2011, 18, 1424–1434. [Google Scholar] [CrossRef]
- Zhang, Q.; Zuo, Z.; Liu, J. Failure Analysis of a Diesel Engine Cylinder Head Based on Finite Element Method. Eng. Fail. Anal. 2013, 34, 51–58. [Google Scholar] [CrossRef]
- Ma, Z.J.; Tao, D.; Yang, Z.; Guo, Y.C.; Li, J.P.; Liang, M.X.; Yeung, L.T.L. The Effect of Vermicularity on the Thermal Conductivity of Vermicular Graphite Cast Iron. Mater. Design 2016, 93, 418–422. [Google Scholar] [CrossRef]
- Dawson, S. Compacted Graphita Iron-a Material Solution for Modern Diesel Engine Cylinder Block and Heads. China Foundry 2009, 6, 241–246. [Google Scholar]
- Lacaze, J.; Moussa, C.; Thebault, Y.; Guesser, W. Extrema of Micro-Hardness in Fully Pearlitic Compacted Graphite Cast Iron. Int. J. Cast Met. Res. 2020, 33, 218–225. [Google Scholar] [CrossRef]
- Gonzaga, R.A. Influence of Ferrite and Pearlite Content on Mechanical Properties of Ductile Cast Irons. Mater. Sci. Eng. A 2013, 567, 1–8. [Google Scholar] [CrossRef]
- Metzloff, K.E.; Loper, C.R. The Effect of Metallurgical Factors and Heat Treatment on the Elastic Modulus and Damping in Ductile and Compacted Graphite Irons. Int. J. Cast Met. Res. 2003, 16, 239–244. [Google Scholar] [CrossRef]
- Lyu, Y.; Sun, Y.; Liu, S.; Zhao, J. Effect of Tin on Microstructure and Mechanical Properties of Compacted Graphite Iron. Int. J. Cast Met. Res. 2015, 28, 263–268. [Google Scholar] [CrossRef]
- Tao, D.; Li, J.P.; Yang, Z.; Yang, T.; Ma, Z.J.; Wu, Y.; Li, Y. Effect of Pearlite Content on High-Temperature Mechanical Properties and Thermophysical Properties of Vermicular Graphite Cast Iron. Trans. Mater. Heat Treat. 2018, 39, 83–90. [Google Scholar] [CrossRef]
- Wu, X.; Quan, G.; MacNeil, R.; Zhang, Z.; Sloss, C. Failure Mechanisms and Damage Model of Ductile Cast Iron under Low-Cycle Fatigue Conditions. Metall. Mater. Trans. A 2014, 45, 5085–5097. [Google Scholar] [CrossRef]
- Bartošák, M.; Horváth, J. Isothermal Low-Cycle Fatigue, Fatigue–Creep and Thermo-Mechanical Fatigue of SiMo 4.06 Cast Iron: Damage Mechanisms and Life Prediction. Eng. Fract. Mech. 2023, 288, 109316. [Google Scholar] [CrossRef]
- Qiu, Y.; Pang, J.C.; Li, S.X.; Yang, E.N.; Fu, W.Q.; Liang, M.X.; Zhang, Z.F. Influence of Thermal Exposure on Microstructure Evolution and Tensile Fracture Behaviors of Compacted Graphite Iron. Mater. Sci. Eng. A 2016, 664, 75–85. [Google Scholar] [CrossRef]
- Pevec, M.; Oder, G.; Potrč, I.; Šraml, M. Elevated Temperature Low Cycle Fatigue of Grey Cast Iron Used for Automotive Brake Discs. Eng. Fail. Anal. 2014, 42, 221–230. [Google Scholar] [CrossRef]
- Coffin, L.F., Jr. A Study of the Effects of Cyclic Thermal Stresses on a Ductile Metal. Ryūmachi 1954, 76, 937–950. [Google Scholar] [CrossRef]
- Manson, S.S. Behavior of Materials under Conditions of Thermal Stress. Nasa Tnd. 1954, 7, 661–665. [Google Scholar]
- Deng, J.L.; Yang, P.; Chen, Y. Low-Cycle Fatigue Crack Initiation Life of Hull-Notched Plate Considering Short Crack Effect and Accumulative Plastic Damage. Appl. Ocean Res. 2017, 68, 65–76. [Google Scholar] [CrossRef]
- Korsunsky, A.; Dini, D.; Dunne, F.; Walsh, M. Comparative Assessment of Dissipated Energy and Other Fatigue Criteria☆. Int. J. Fatigue 2007, 29, 1990–1995. [Google Scholar] [CrossRef]
- Saiprasertkit, K.; Hanji, T.; Miki, C. Local Strain Estimation Method for Low- and High-Cycle Fatigue Strength Evaluation. Int. J. Fatigue 2012, 40, 1–6. [Google Scholar] [CrossRef]
- Pang, J.C.; Nie, L.L.; Zhu, L.B.; Zhang, Z.J.; Yao, G.; Mao, Y.X.; Chen, M.; Zhang, Z.F. Low-Cycle Fatigue Behavior and Life Prediction of Copper Busbar. Adv. Eng. Mater. 2016, 18, 2018–2027. [Google Scholar] [CrossRef]
- Liu, R.; Zhang, Z.J.; Zhang, P.; Zhang, Z.F. Extremely-Low-Cycle Fatigue Behaviors of Cu and Cu–Al Alloys: Damage Mechanisms and Life Prediction. Acta Mater. 2015, 83, 341–356. [Google Scholar] [CrossRef]
- Zhu, Q.; Xu, Z.; Zou, Y.; Yao, W. Low-Temperature Fatigue Behavior and Damage Analysis of Al-7Zn-2Mg-1.5Cu Alloy Based on Hysteretic Energy. Mater. Today Commun. 2023, 35, 105855. [Google Scholar] [CrossRef]
- GB/T 26656−2023; Metallographic Test for Compacted (Vermicular) Graphite Cast Irons. SAC: Beijing, China, 2023.
- Selin, M. Comparing Three Equations Used for Modeling the Tensile Flow Behavior of Compacted Graphite Cast Irons at Elevated Temperatures. Metall. Mater. Trans. A 2010, 41, 2805–2815. [Google Scholar] [CrossRef]
- Zou, C.L.; Chen, L.J.; Pang, J.C.; Wang, M.; Qiu, Y.; Li, S.X.; Zhang, Z.F. The Low-Cycle Fatigue, Fracture and Life Prediction of Compacted Graphite Iron: Influence of Temperature. Mater. Sci. Eng. A 2019, 763, 138101. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, T.; Gong, X.; Li, Q.; Liu, Y.; Wang, Q.; Zhang, H.; Wang, Q. Low Cycle Fatigue Properties, Damage Mechanism, Life Prediction and Microstructure of MarBN Steel: Influence of Temperature. Int. J. Fatigue 2021, 144, 106070. [Google Scholar] [CrossRef]
- Wang, M.; Pang, J.C.; Li, S.X.; Zhang, Z.F. Low-Cycle Fatigue Properties and Life Prediction of Al-Si Piston Alloy at Elevated Temperature. Mater. Sci. Eng. A 2017, 704, 480–492. [Google Scholar] [CrossRef]
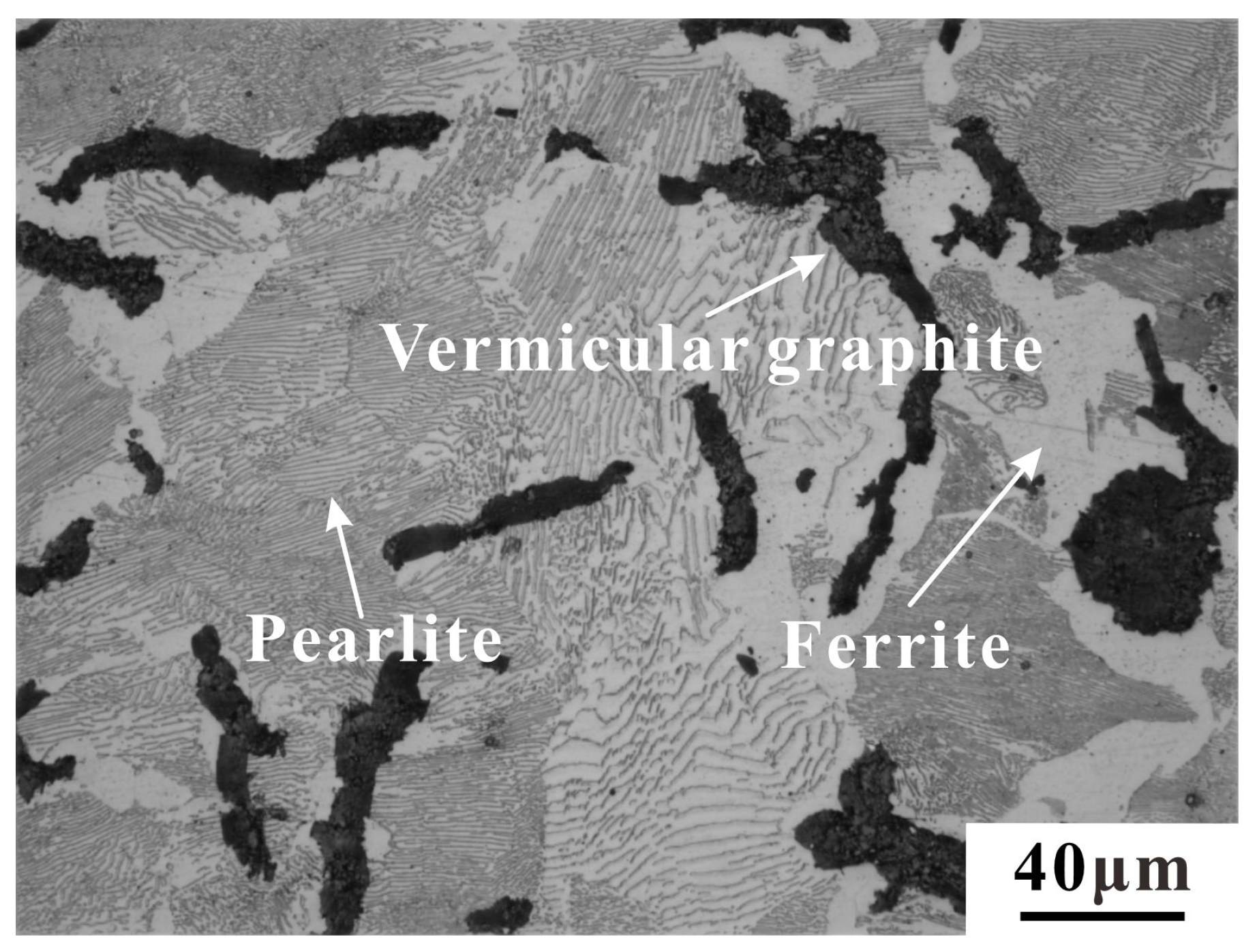
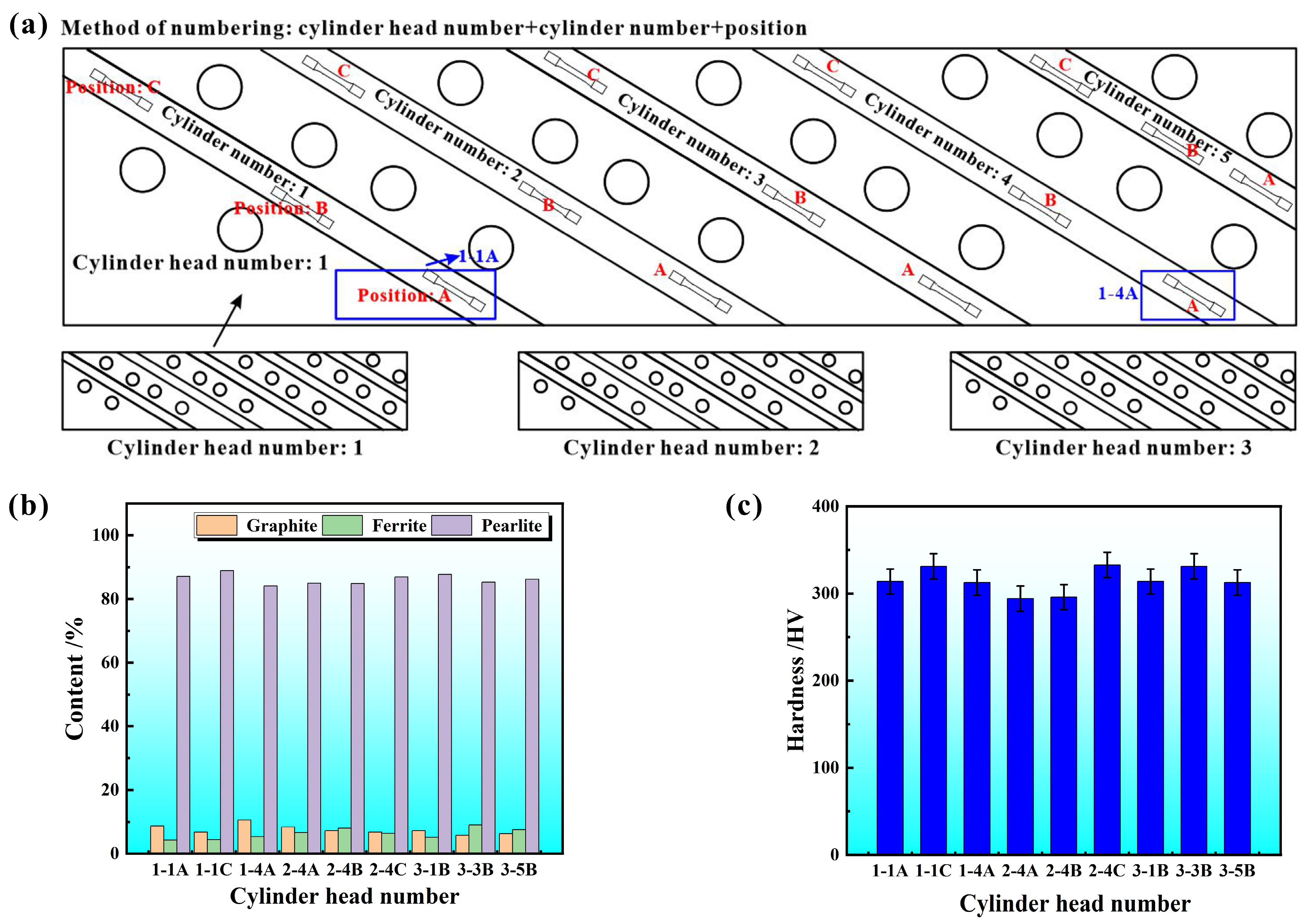







| C | S | Si | Mn | Cu | Cr | Sn | Mo | Re | Mg |
|---|---|---|---|---|---|---|---|---|---|
| 3.81 | 0.01 | 2.20 | 0.32 | 0.80 | 0.05 | 0.09 | 0.001 | 0.02 | 0.017 |
| Test Temperature/°C | Total Strain Amplitude ∆εt/2/% | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.15 | 0.2 | 0.25 | ||||||
| 25 | 105 | - | 13,427 | 23,136 | - | 3382 | 2254 | 2682 | 791 |
| 400 | 105 | - | 32,739 | 6833 | 8387 | 1053 | 1421 | 363 | 409 |
| 500 | 105 | 44,508 | 5707 | 6030 | 2291 | 289 | 305 | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Q.; Tan, B.; Pang, J.; Shi, F.; Jiang, A.; Zou, C.; Zhang, Y.; Li, S.; Zhang, Y.; Li, X.; et al. Low-Cycle Fatigue Damage Mechanism and Life Prediction of High-Strength Compacted Graphite Cast Iron at Different Temperatures. Materials 2024, 17, 4266. https://doi.org/10.3390/ma17174266
Wu Q, Tan B, Pang J, Shi F, Jiang A, Zou C, Zhang Y, Li S, Zhang Y, Li X, et al. Low-Cycle Fatigue Damage Mechanism and Life Prediction of High-Strength Compacted Graphite Cast Iron at Different Temperatures. Materials. 2024; 17(17):4266. https://doi.org/10.3390/ma17174266
Chicago/Turabian StyleWu, Qihua, Bingzhi Tan, Jianchao Pang, Feng Shi, Ailong Jiang, Chenglu Zou, Yunji Zhang, Shouxin Li, Yanyan Zhang, Xiaowu Li, and et al. 2024. "Low-Cycle Fatigue Damage Mechanism and Life Prediction of High-Strength Compacted Graphite Cast Iron at Different Temperatures" Materials 17, no. 17: 4266. https://doi.org/10.3390/ma17174266
APA StyleWu, Q., Tan, B., Pang, J., Shi, F., Jiang, A., Zou, C., Zhang, Y., Li, S., Zhang, Y., Li, X., & Zhang, Z. (2024). Low-Cycle Fatigue Damage Mechanism and Life Prediction of High-Strength Compacted Graphite Cast Iron at Different Temperatures. Materials, 17(17), 4266. https://doi.org/10.3390/ma17174266








