Next Article in Journal
Stress–Dilatancy Behavior of Highly Elastic Rubber-Added Cohesionless Materials
Previous Article in Journal
Evaluation of 3-Point and 4-Point Bending Tests for Tensile Strength Assessment of GFRP Bars
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Materials
Volume 17
Issue 21
10.3390/ma17215263
materials-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Effect of Plastic Deformation on Thermal Properties in Twinning-Induced Plasticity Steel

by
Joong-Ki Hwang
School of Mechatronics Engineering, Korea University of Technology & Education, Cheonan 31253, Republic of Korea
Materials 2024, 17(21), 5263; https://doi.org/10.3390/ma17215263
Submission received: 6 October 2024 / Revised: 22 October 2024 / Accepted: 24 October 2024 / Published: 29 October 2024
Browse Figures
Versions Notes

Abstract

:
The effect of plastic deformation induced by wire drawing on thermal properties in twinning-induced plasticity (TWIP) steel has been investigated. The investigation on the relationship between thermal conductivity (k) and the microstructure in the drawn TWIP steel wire was systematically performed to accurately understand the behavior of the k of a metal during wire drawing. The yield and tensile strengths linearly increased with drawing strain owing to the deformation twins and dislocations that were generated during wire drawing. However, the total elongation sharply decreased with drawing strain. The linear thermal expansion coefficient of the TWIP steel exhibited a similar value regardless of drawing strain. The density decreased linearly with temperature, and it was independent of the drawing strain. k increased initially and then decreased after reaching its maximum value with increasing drawing strains. At a nominal drawing strain of 0.26, k increased compared with the state of hot rolling because the increase in k due to grain elongation was greater than the decrease in k due to dislocations generated during wire drawing. However, as the amount of drawing step increased further, the influence of dislocations on k increased more than that of grain elongation, causing k to decrease.

1. Introduction

The weight reduction of automobiles has been continuously demanded to improve the energy efficiency of cars and prevent environmental pollution. Recently, the demand for the weight reduction of cars has become stronger owing to the maturity of electric vehicles. In this situation, researchers in steel industries are constantly studying to develop stronger and lighter steels. In particular, researchers’ efforts are accelerating to avoid losing market share to other materials in car industries. This trend is also apparent in steel long products industries such as wire, rod, and bar industries, which produce springs, tie rods, bearings, bolts, tire cords, etc. for automobiles. Practically, the most effective way to increase the strength of steel is to control its chemical composition. Accordingly, research and development on steels has been directed toward increasing the chemical composition of steels to effectively use their strengthening mechanisms such as solid solution hardening, precipitation strengthening, and strengthening with heat treatments. However, as the chemical composition of steels increases, the thermophysical properties of steels tend to deteriorate. This may cause some issues during the manufacturing process and/or under service. For instance, Hwang et al. [1,2] have suggested twinning-induced plasticity (TWIP) steels as good candidates to replace existing steels for wire, rod, and bar products because TWIP steels satisfy the products’ property requirements such as high strength, ductility, and resistance to hydrogen-delayed fracture [3,4,5,6]. The excellent mechanical properties of TWIP steels are attributed to deformation twins and/or dynamic strain aging (DSA) occurring during plastic deformation [7,8,9]. In TWIP steels, 10–30% manganese is used to stabilize the austenite phase. This high alloy tends to deteriorate the thermophysical properties of steels [10,11], which can worsen the formability of TWIP steels, in particular the wire drawability for wire and rod products. The wire drawing process is almost an essential process for producing wire and rod products such as springs, bolts, tie rods, tire cords, and bearings [12]. During the process, the temperature of the wire increases because the drawing speed is significantly high for productivity and wire drawing is performed through direct frictional contact between the wire and die [13,14].
The quality and productivity of products are closely related to the heat generated in the material during wire drawing. For example, temperature rises and thermal gradients in the materials can worsen the formability of the wire, and finally may cause wire breakages during the drawing process in plain carbon steels due to strain aging of the drawn wire [15]. The heat and thermal gradient in a material generated during wire drawing is highly correlated, not only with process conditions, but also with the thermal properties of the material [16]. For example, when the thermal conductivity (k) of a wire is low, the drawing speed should be reduced and more attention should be paid to cooling the wire during the drawing process. This is because the surface temperature of the wire increases with decreasing k and the thermal gradient in the wire increases. This means that process conditions for wire drawing should be modified according to the k of a wire and the productivity of the wire drawing process is dependent on the k of a wire. Therefore, to effectively design the wire drawing process, we need to accurately understand the thermal properties of metals, especially k.
In addition, since most wire, rod, and bar products are used after the wire drawing process or undergo additional processes after the wire drawing process, it is of great importance to understand the thermal properties of the drawn steel wire [1]. Consequently, it is necessary to investigate the thermal properties of the drawn TWIP steel wire to improve the drawability and productivity of wire products. To the best of the author’s knowledge, no studies have been conducted on the thermal properties of drawn TWIP steel, although there is considerable research on the drawing process conditions and mechanical properties such as drawing speed, drawing direction, die angle, drawing force, strength, ductility of materials [13,15,17,18,19,20,21,22,23,24] and electrical conductivity in Cu and Al alloys [25,26]. Meanwhile, it is known that the thermophysical properties of metals such as thermal diffusivity (α), specific heat capacity (cp), linear thermal expansion coefficient (β), density (ρ), and k are strongly dependent on the temperature [11,27,28,29,30].
Consequently, this study investigated the thermophysical properties of TWIP steel wire, especially k, during wire drawing. The thermophysical properties of the TWIP steel such as α, cp, and β were measured with temperature and drawing strain. Subsequently, ρ and k were calculated based on the measured thermophysical properties. The investigation on the relationship between k and the microstructures in the drawn TWIP steel was systematically performed to accurately understand the behavior of the k of a metal during wire drawing.

2. Experimental Procedures

2.1. Material Preparation

An ingot of 50 kg was cast using induction melting for the specimen preparation of Fe-Mn-C-Al TWIP steel. The alloy component of the ingot is listed in Table 1. Although there is an excellent combination of strength and elongation in TWIP steels, great efforts have been put into further improving the formability and resistance to delayed fractures in TWIP steels. Researchers showed that the formability and resistance to delayed fractures in TWIP steels increased with Al addition in Fe-Mn-C TWIP steels [31,32,33,34,35]. The addition of Al contents in TWIP steel improved the yield strength (YS) through the strengthening mechanism of solid solution, formability by suppressing the DSA, and resistance to delayed fractures. Meanwhile, C stabilizes austenite and inhibits martensite transformation by increasing the stacking fault energy (SFE) in TWIP steels and the addition of C contents in TWIP steel dramatically increases the YS through solid solution strengthening [36,37]. Therefore, Fe-17Mn-0.7C-1.5Al TWIP steel was selected as the test material in this study as listed in Table 1.
To suppress manganese segregation in the ingot, the cast specimen was heated to 1200 °C and homogenized for 12 h using a furnace. Then it was directly hot-rolled into the plate with the thickness of 20 mm. The rolling temperatures ranged from 950 °C to 1200 °C. Subsequently, the specimen was cooled in an atmospheric environment at a temperature of 21 °C. For a wire drawing test, several 15 mm-diameter round rods were machined from the hot-rolled plate using a lathe. The SFE of the present TWIP steel, calculated using the thermodynamic model proposed by Saeed-Akbari et al. [38] and Dumay et al. [39], was approximately 31.2 mJ/m2. Accordingly, this TWIP steel is expected to be deformed by a dislocation glide and form a deformation twin during the plastic deformation at room temperature (RT). In contrast, martensitic transformation is strongly suppressed during deformation due to the relatively high SFE [40,41,42].

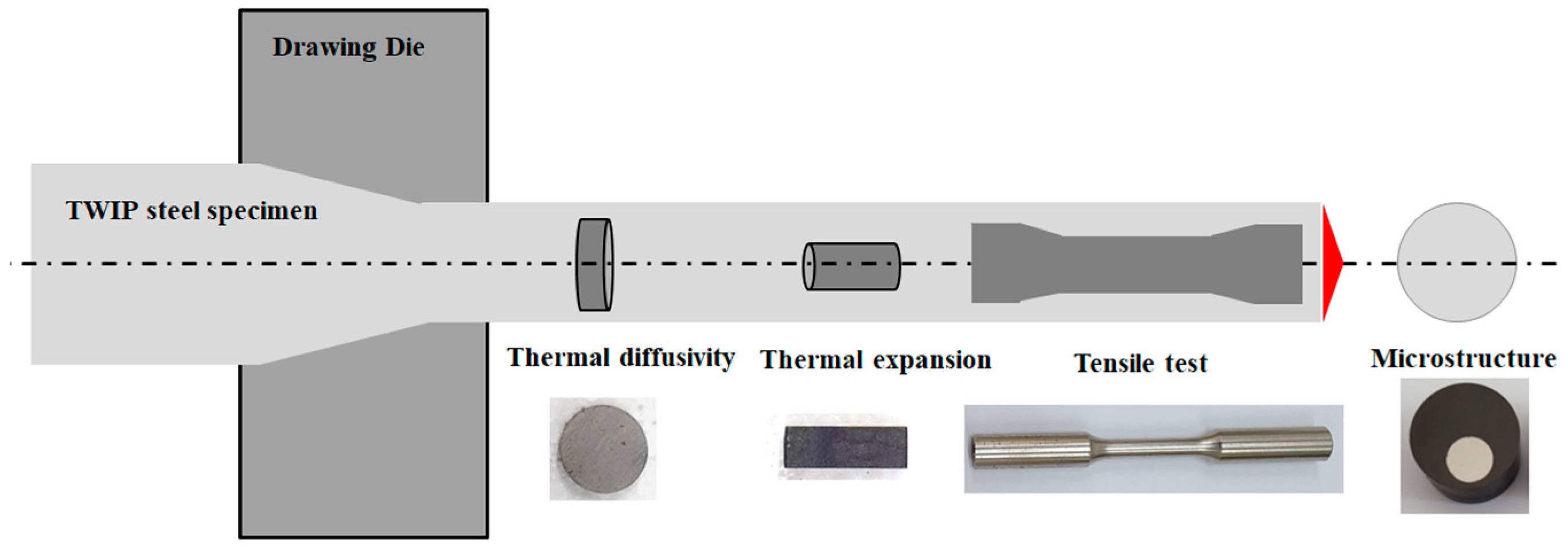
2.2. Wire Drawing Test

The TWIP steel wire was drawn at a velocity of 8.3 mm/s using a draw bench machine with a single pass at RT. To clean the surface of the specimens, pickling was conducted with 12.5% HCl before the test. A MoS2 spray-type lubricant was utilized to decrease the frictional stress and improve wire quality during the drawing process. The specific die designs used in this test are summarized in Table 2. The reduction in area (RA) is calculated as follows, and the RA per pass was designed to be approximately 10%.
R A = A i − A f A i × 100   ( % )
Here Ai and Af are the initial and final cross-sectional areas of the wire, respectively. The nominal drawing strain (εN) of the specimen is obtained as follows and is summarized in Table 2.
ε N = l n A i A f

2.3. Measurement of Microstructure and Mechanical Properties

The samples were sectioned perpendicular to the radial direction of the hot-rolled and drawn wires using a cutting machine to observe their microstructures as shown in Figure 1. Microstructures were evaluated using electron backscatter diffraction (EBSD). Based on the EBSD techniques, the grain size and orientation, phase, density of deformation twin in the TWIP steel and the geometrically necessary dislocation (GND), etc. can be easily analyzed. In this study, we investigated the comprehensive relationship between k and the microstructure in the TWIP steel wire with applied strain induced by wire drawing. Therefore, EBSD measurements are the most suitable method for microstructural evolution in this study compared with a scanning electron microscope or an optical microscope. For the specimen preparation, the samples were progressively ground using SiC papers with water up to 2000 grit. Grounded samples were polished using diamond compound pastes from 6 to 1 μm; subsequently, a colloidal silica suspension was utilized for approximately 1.2 ks. A field-emission scanning electron microscopy with a TexSEM Laboratories (TSL) EBSD system was utilized to gather the EBSD data at an acceleration voltage of 20 kV. The measured area of the EBSD data was 180 μm × 180 μm with a 0.1 μm step size. The sample tilt angle was approximately 70°. The obtained EBSD data were evaluated using orientation imaging microscopy software.
For the tensile test, cylindrical-type specimens with a length of 25 mm and diameter of 5 mm were machined using a lathe along the hot rolling and drawing directions as shown in Figure 1. Tensile specimens were pulled at an initial strain rate of 10−3 s−1 with an Instron machine at RT. Ductility of the specimen was measured using a mechanical extensometer during the straining.

2.4. Measurement of Thermophysical Properties

The thermophysical properties of metals such as ρ, cp, α, k, and β are dependent on the temperature and applied strain. Therefore, the α, cp, and β of the TWIP steel were measured with temperatures and εN. Then, ρ and k were calculated based on the measured thermophysical properties. The α was measured with laser flash analysis (LFA), Netzsch LFA 467 HT, Germany. The LFA specimen with a disk shape in a diameter of 10.0 mm and thickness of 2.5 mm was utilized as shown in Figure 1 [43]. During the test, argon gas was used to suppress surface oxidation of the specimen. Measurements were conducted at the interval of 100 °C from RT to 1000 °C.
To calculate ρ using temperatures based on ρ0, the thermal expansion ratio (ΔL/L0) was measured with thermomechanical analysis (TMA), TA Instruments TMA Q400, USA [44]. ρ0 indicates ρ at RT, and it was measured using the Archimedes’ principle at RT. L indicates the length of the TMA specimen. The subscript o indicates the values at RT. The specimen with a cylindrical shape in a diameter of 5.0 mm and thickness of 12.0 mm was utilized as shown in Figure 1. Nitrogen gas was utilized to suppress air intrusion during the test at a heating rate of 5 °C/min. Measurements were conducted from RT to 950 °C, and β was mathematically calculated using the following simple equation [45].
β ( T ) = ∆ L L 0 1 ∆ T
To calculate ρ, the volume of a specimen (V) can be mathematically calculated as follows.
V = L 3 = ( L 0 + ∆ L ) 3 = L 0 3 ( 1 + ∆ L L 0 ) 3
At a small ΔL/L0, Equation (4) can be approximated as follows using the Taylor series expansion.
V = V 0 ( 1 + 3 ∆ L L 0 )
Accordingly, ρ can be obtained based on Equations (3) and (5), and it can be expressed using the measured ρ0 and β as follows.
ρ = m V = m V 0 ( 1 + 3 ∆ L L 0 )   = ρ 0 1 1 + 3 β ∆ T
The cp was measured with simultaneous thermal analysis (STA), Netzsch STA449 F5 Jupiter, Germany [46] from RT to 400 °C. To suppress surface oxidation of the STA specimen, argon gas was used during the test. Finally, k was obtained using the cp, α, and ρ as follows, according to the definition of k [47].
k T = α ( T ) ρ ( T ) c p ( T )
As explained above, α and cp were measured using LFA and STA, respectively, and ρ was calculated based on Equation (7).

3. Results

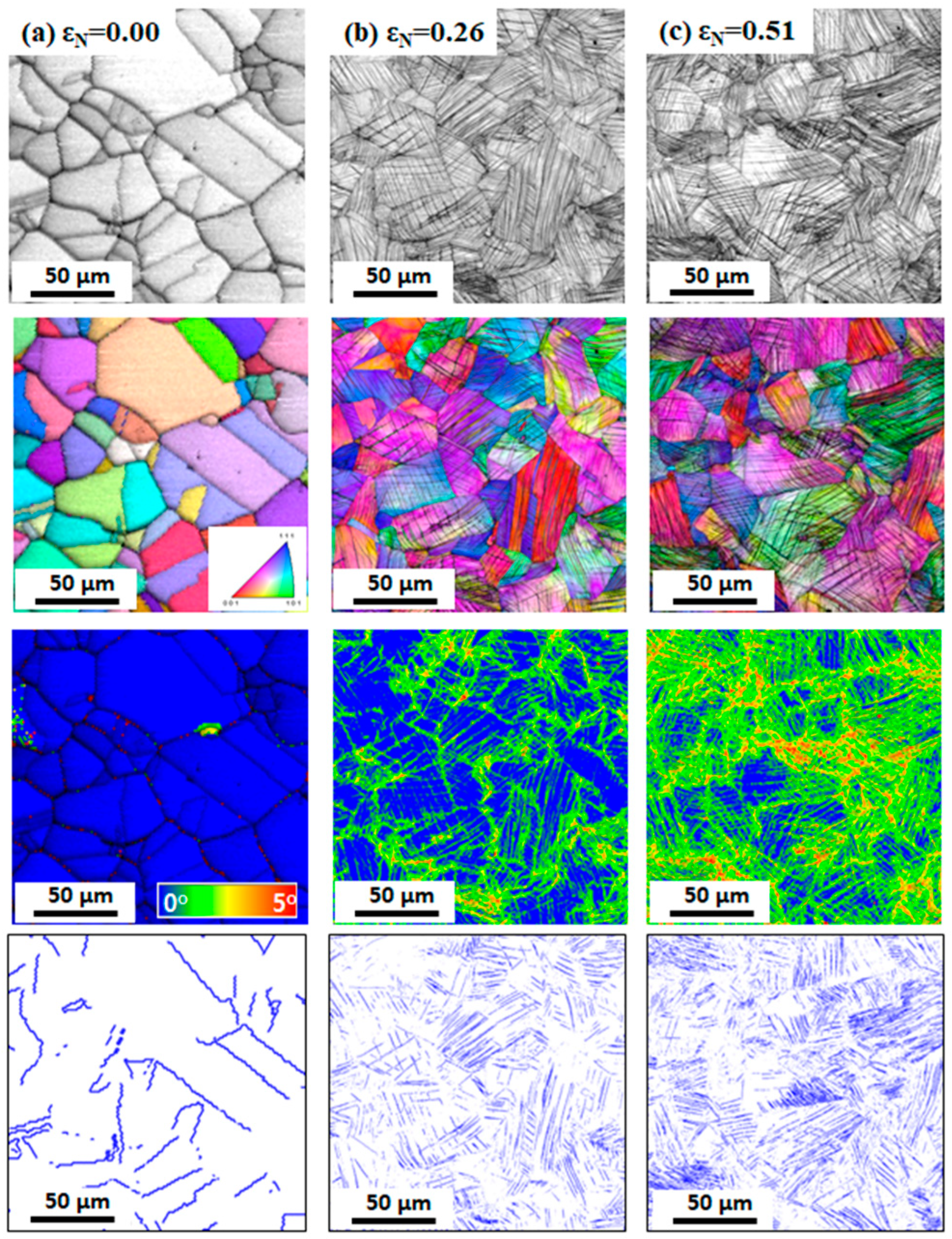
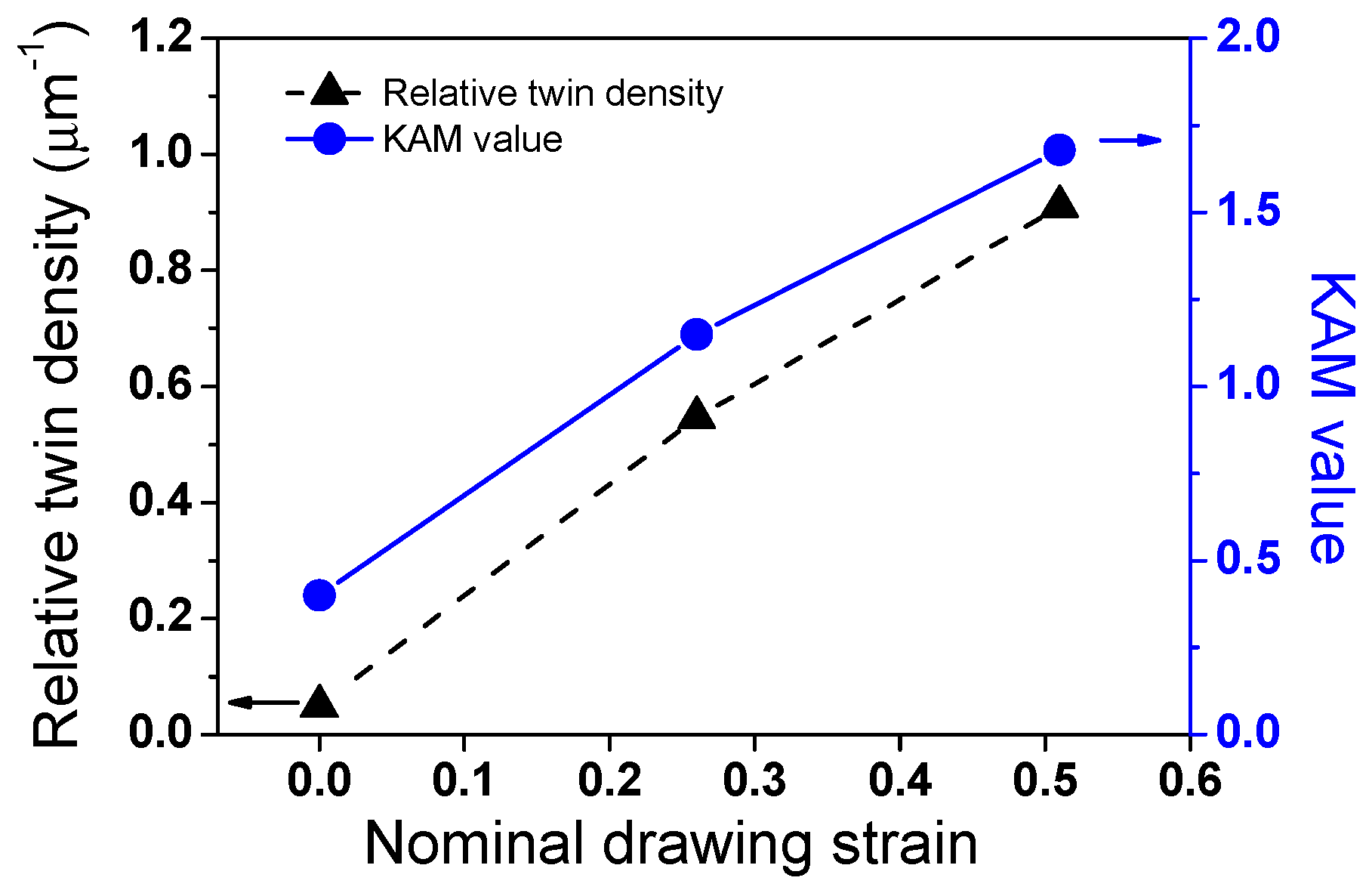
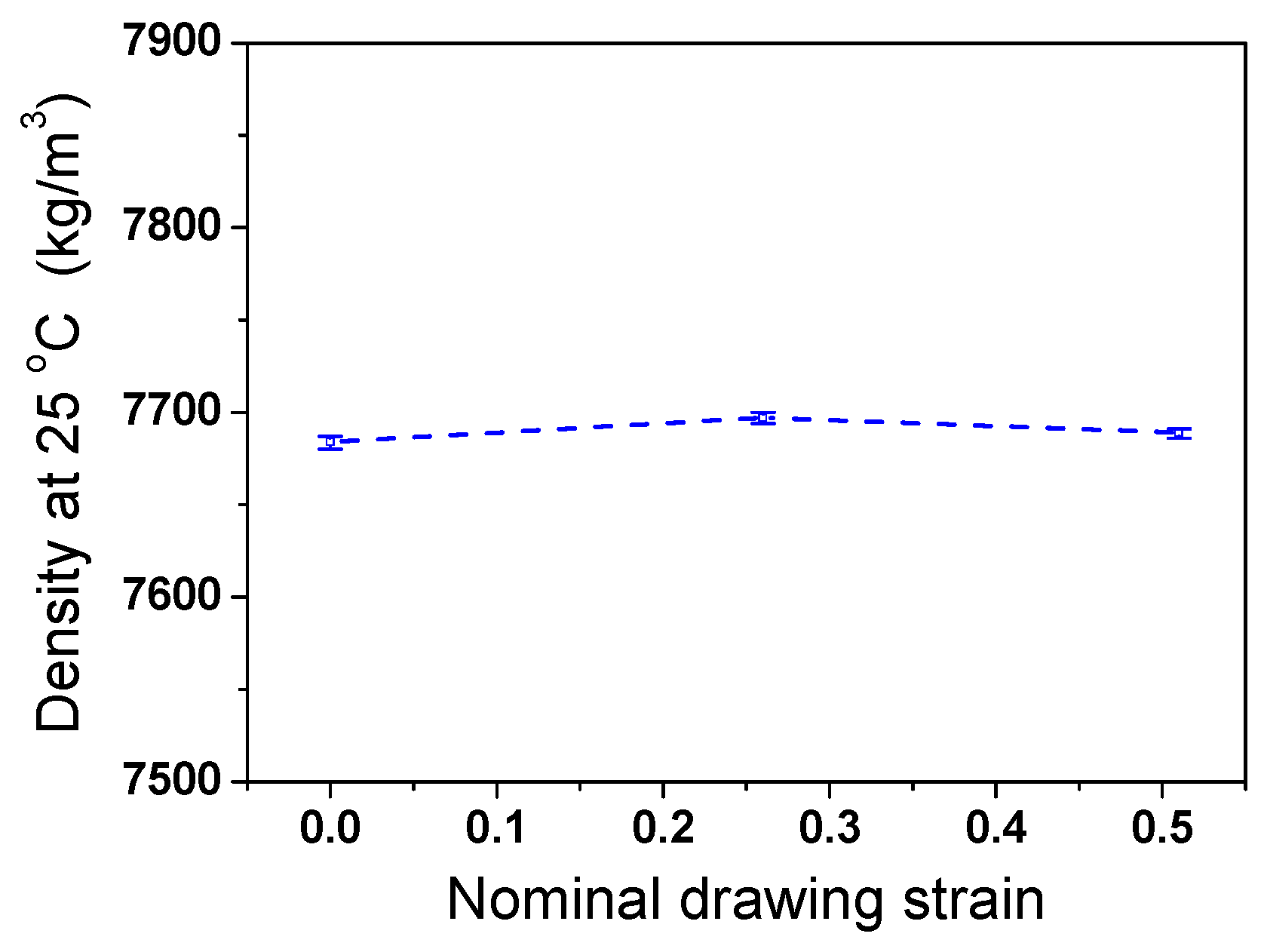
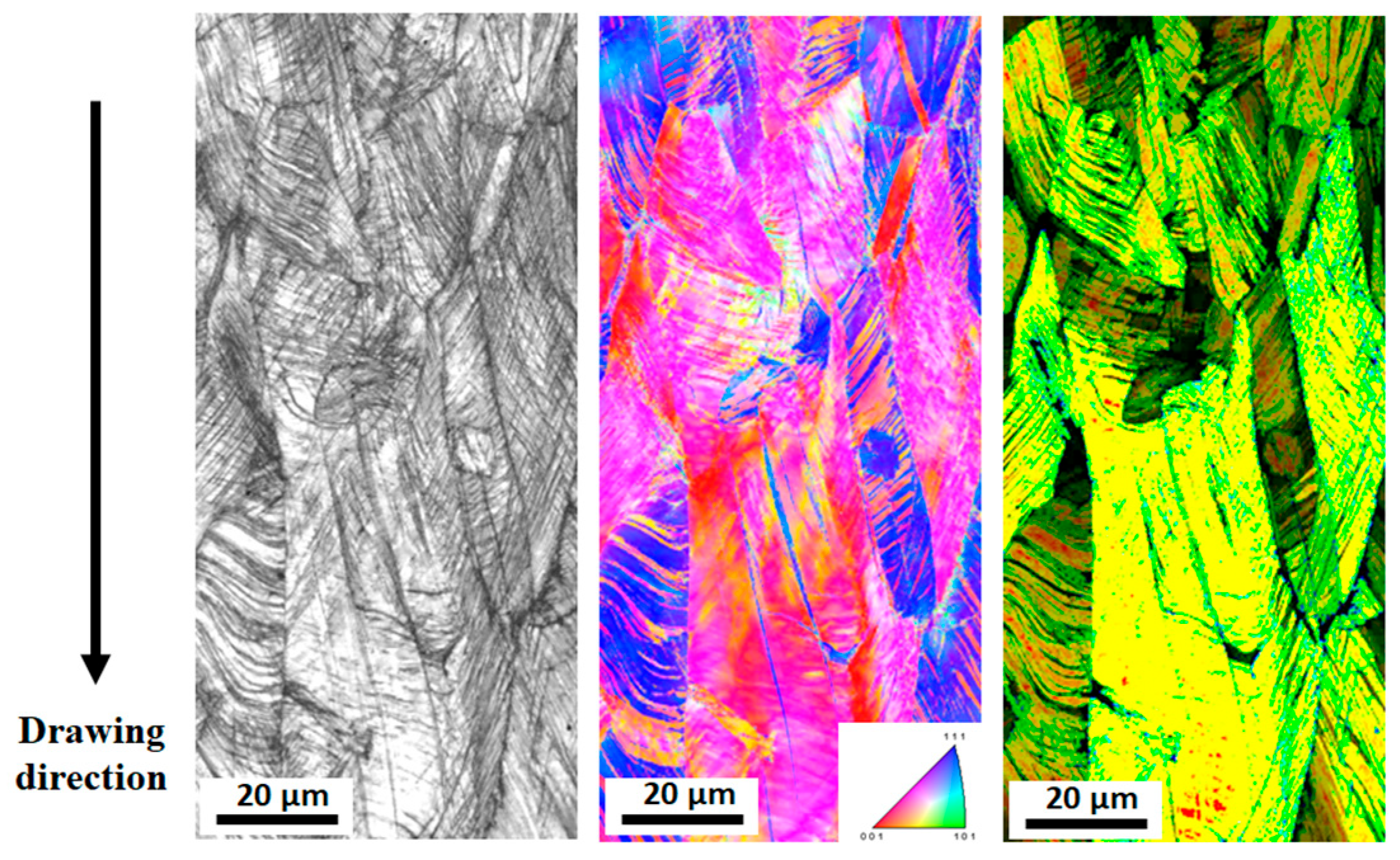
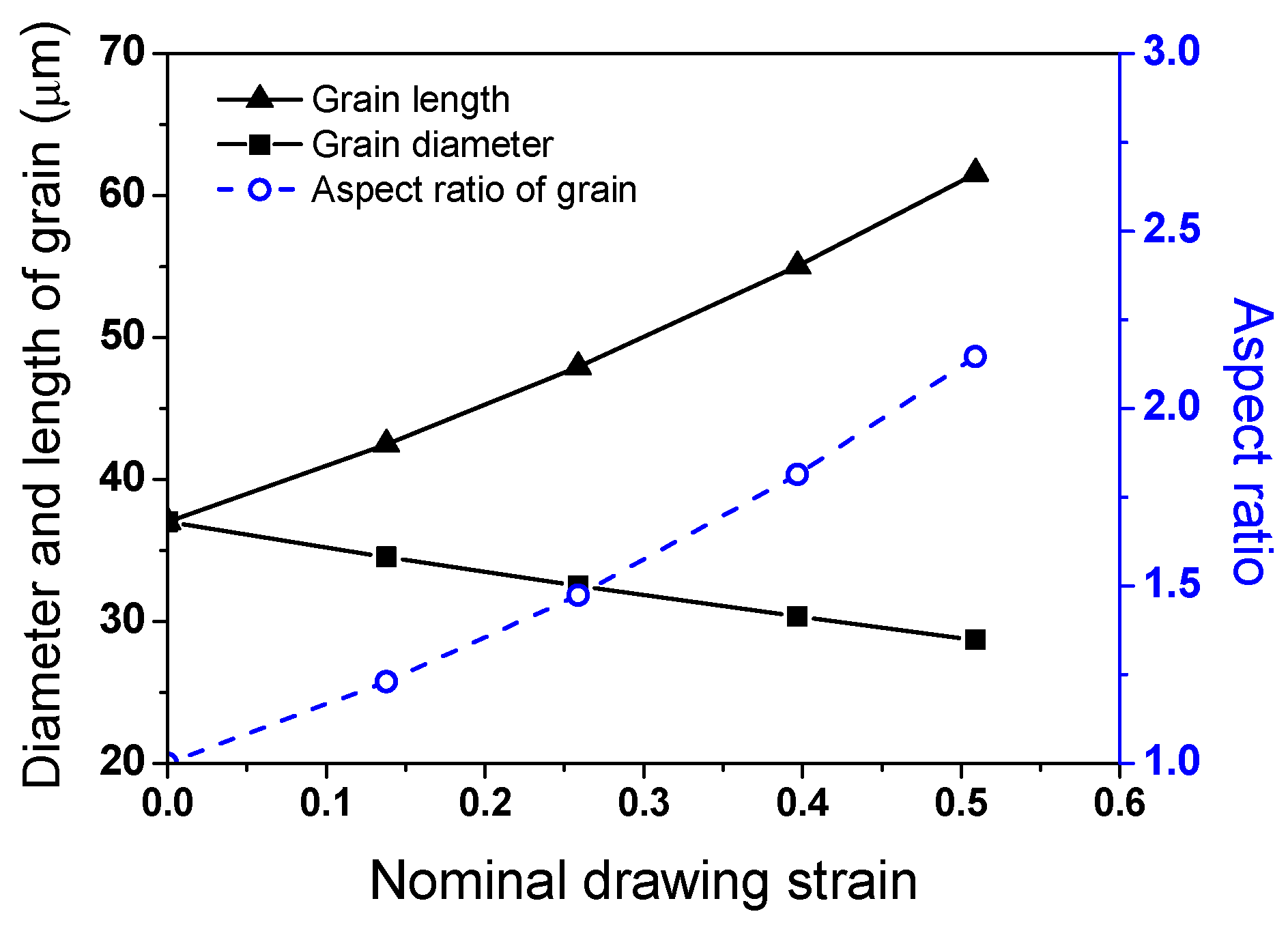
3.1. Microstructure

Figure 2 shows the comparison of microstructures of the hot-rolled and drawn TWIP steel specimens based on the EBSD analysis. The EBSD image quality (IQ), inverse pole figure (IPF), kernel average misorientation (KAM) and twin boundaries maps are compared with εN. In twin boundaries maps, twin boundaries are defined as the misorientation angles of 58° < θ < 62°, and they are presented using blue lines. In the hot-rolled specimen (εN = 0.00), fully recrystallized grains with no significant textures and no GNDs were observed. It is known that the KAM value highly depends on the GND density [48,49]. Annealing twins were observed, but no deformation twins were seen. The average grain size was approximately 37 μm. At an εN of 0.26, most of the grains exhibited deformation twins, and the GND density increased compared with the hot-rolled specimen. The amount of deformation twins and GNDs increased at an εN of 0.51. In particular, deformation twins with multi-variants were highly observed at this drawing strain. It is generally accepted that deformation twins with more variants effectively hinder the dislocation glide due to the strong dynamic Hall–Petch effect, resulting in the outstanding work hardening rate of TWIP steels [50,51].
Figure 3 shows the comparison of relative twin density (Dtwin) and the KAM values with εN for a more quantitative analysis of the microstructures. To calculate Dtwin, the total length of twins (ltwin) was obtained based on the EBSD technique, and it was divided by the measured area (Am). Accordingly, Dtwin can be obtained using the following equation.
D t w i n = ∑ l t w i n A m   ( μ m − 1 )
It is clearly shown that the Dtwin and GND density increased with increasing εN. The KAM and Dtwin values showed a similar trend with εN, meaning that the GND density is related to the twinning behaviors. Unfortunately, we cannot determine the specific pattern of growth in the KAM and Dtwin with εN because only three points of εN were measured in this study.

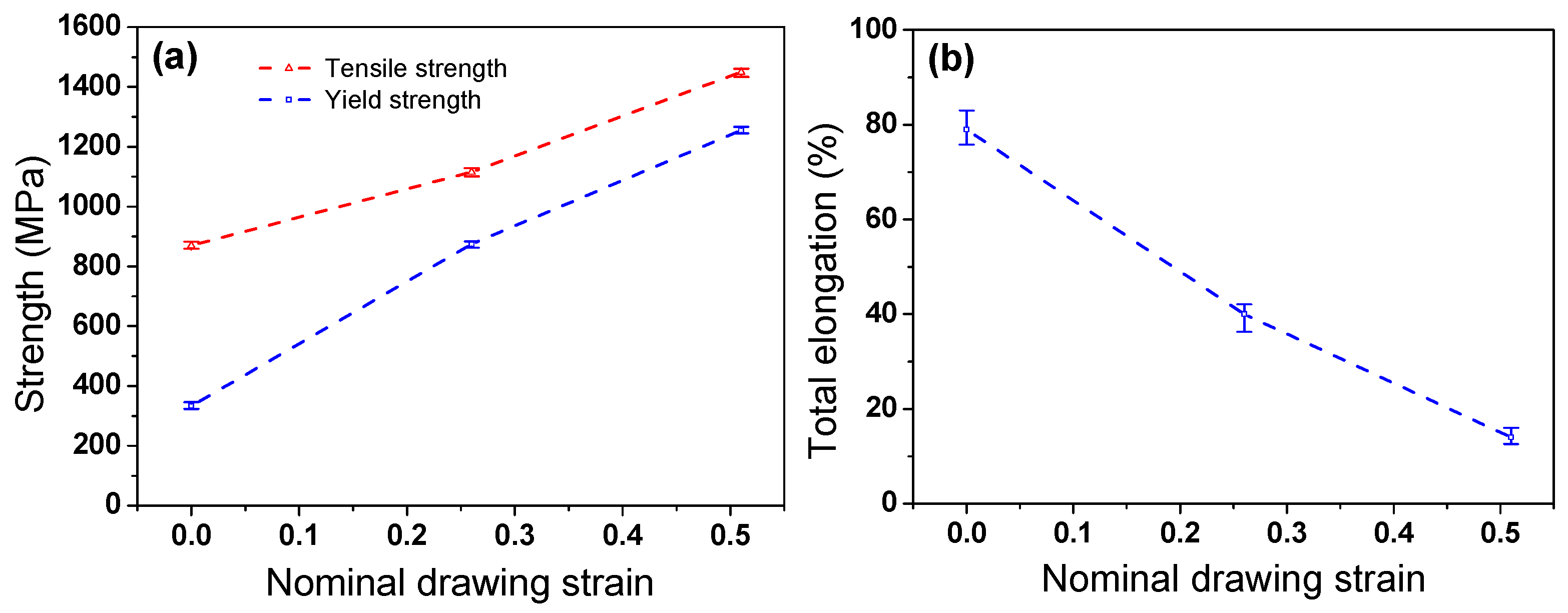
3.2. Mechanical Properties

Figure 4 presents the tensile curve of the hot-rolled TWIP steel. The steel exhibited an excellent combination of tensile properties: the tensile strength (TS) is 870 MPa and the total elongation (TE) is approximately 80%. However, a small YS compared with TS and a small post-necking elongation compared with TE were observed. These are typical characteristics of TWIP steels [3]. The tensile curve also exhibited a significant serration flow resulting from the DSA [52,53,54].
Figure 5 compares the YS, TS, and TE of the TWIP steel with εN. The YS and TS increased due to the deformation twins and dislocations generated during plastic deformation with εN. However, TE decreased sharply with εN. The difference between YS and TS was reduced with an increasing εN. The specific pattern of increase in strength and the decrease in TE with increasing εN cannot be determined in this study because the tensile test was performed at only three εN of 0.00, 0.26, and 0.51.

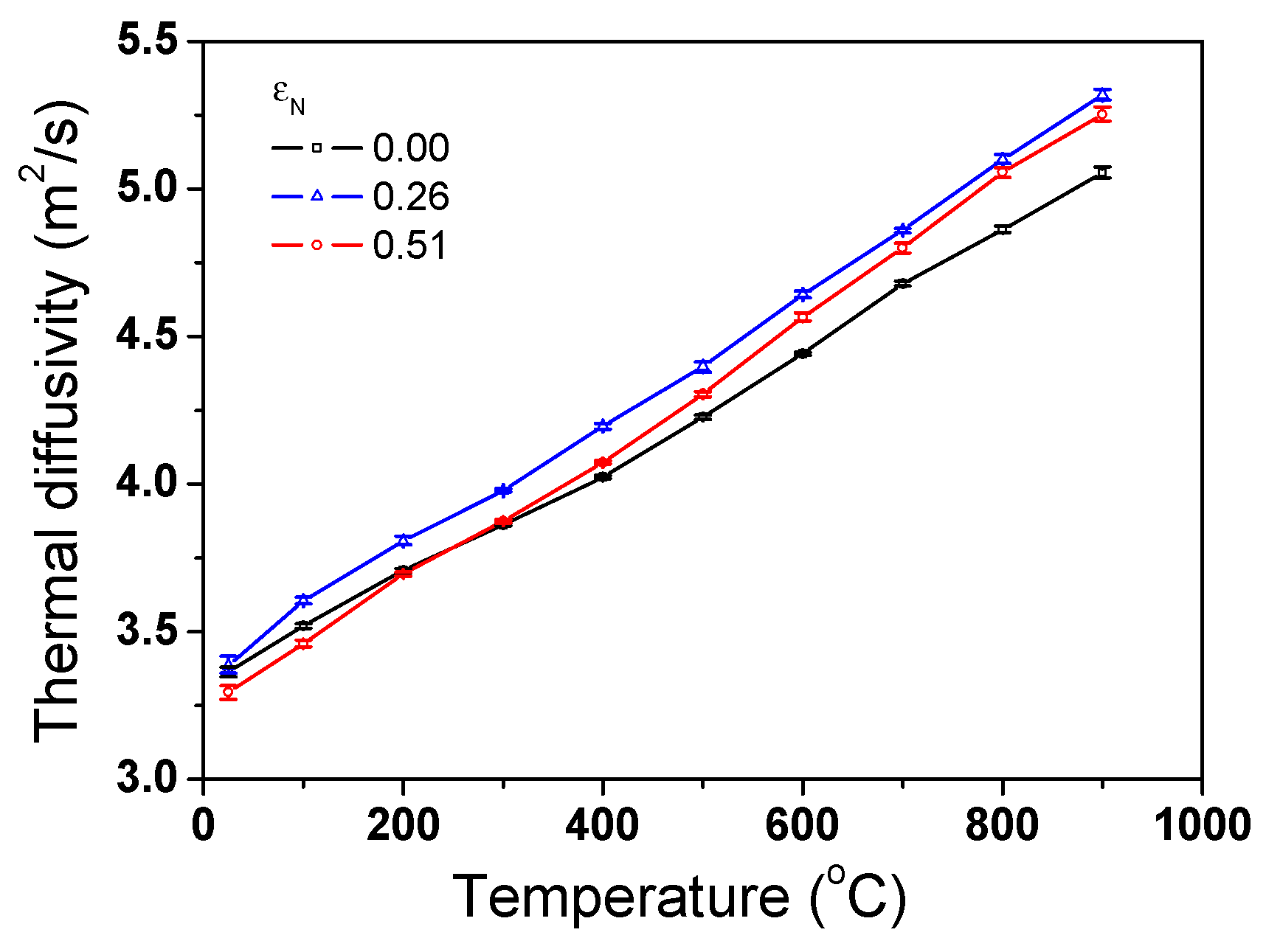
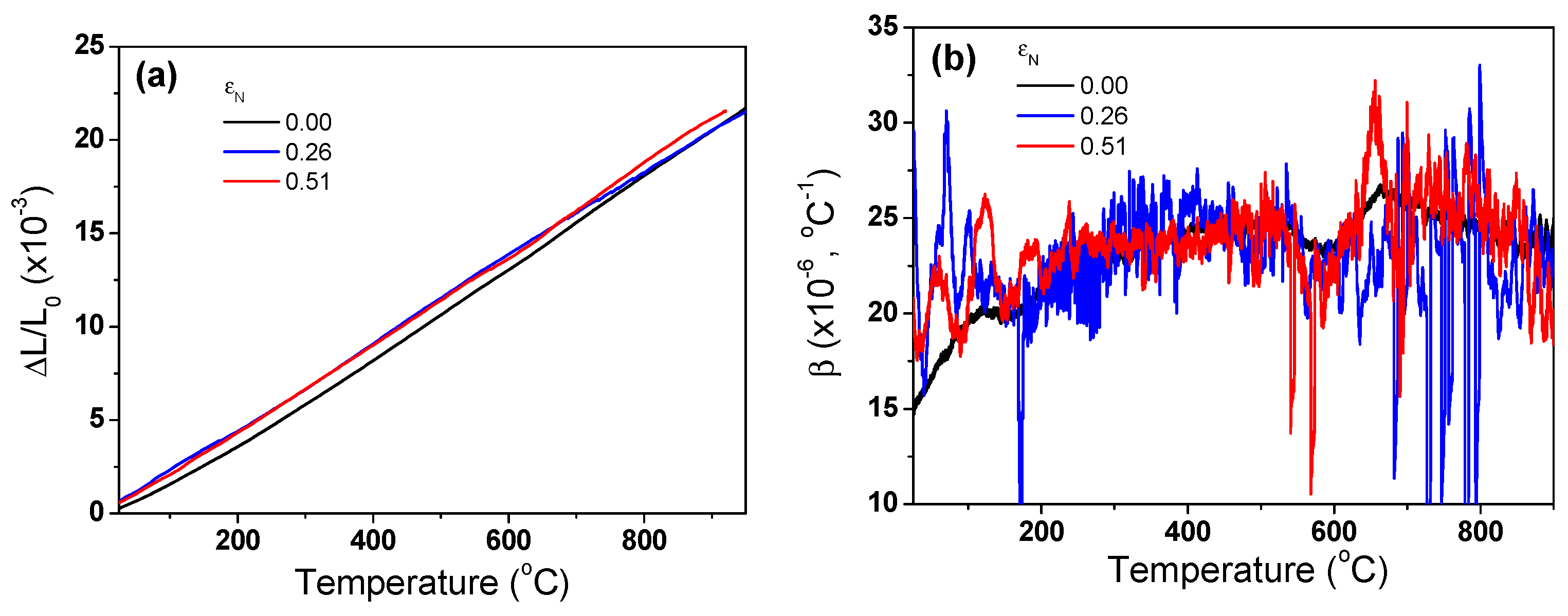
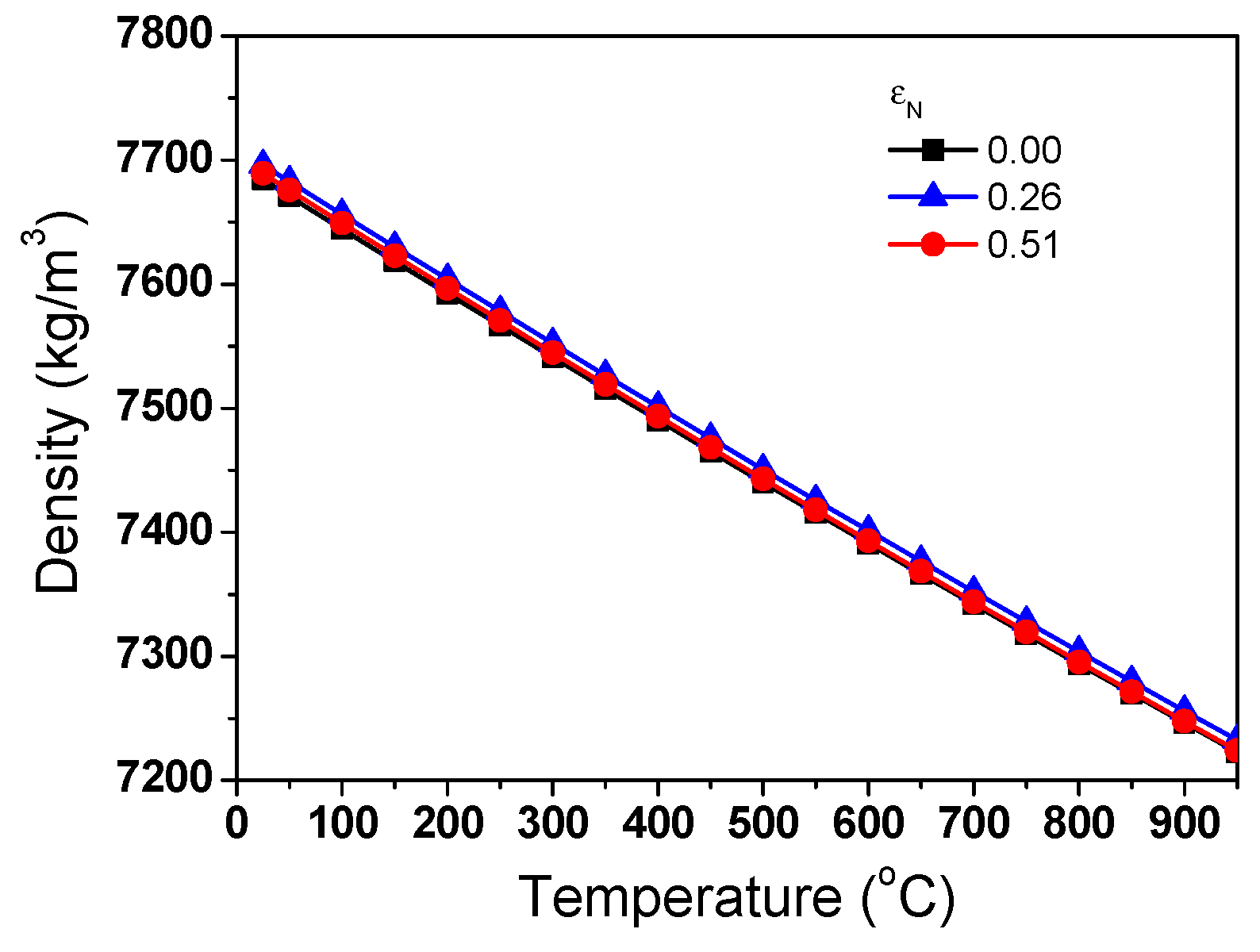
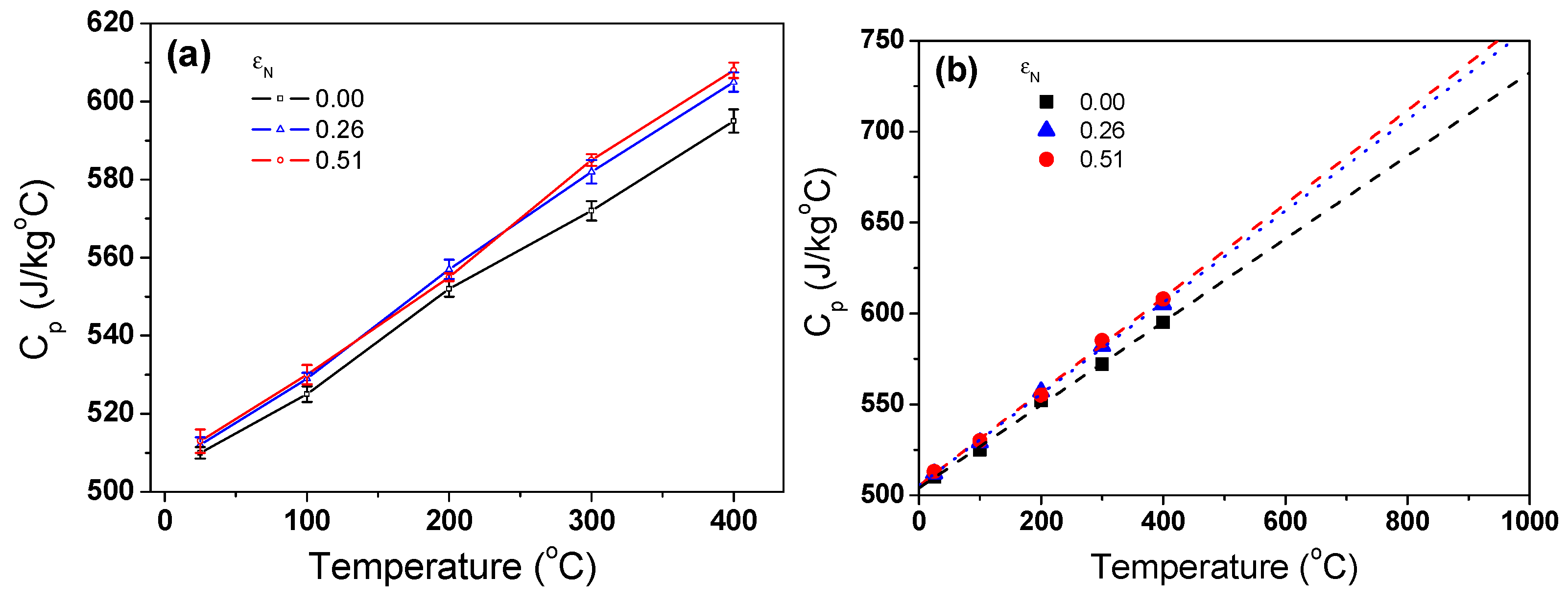
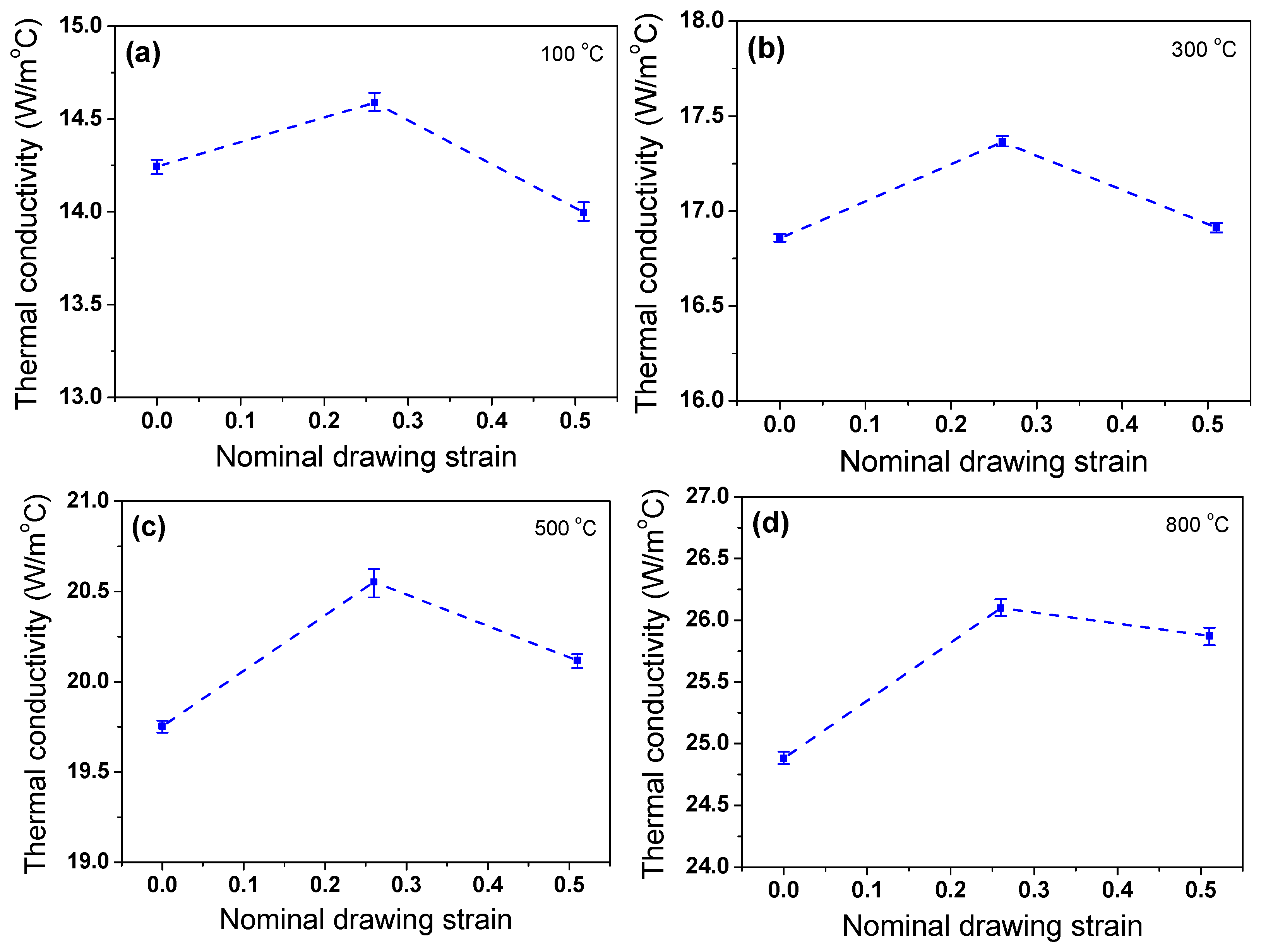
3.3. Thermophysical Properties

Figure 6 compares the measured α of the TWIP steel as functions of temperature and εN. Five repeated tests were performed to obtain the one measurement point and error bars were also displayed on the graph. It can be seen that the deviation of α values for the repeated measurements is small. It can be clearly observed that α increased with temperature. However, α exhibited complex behaviors depending on εN. For example, the α at an εN of 0.25 exhibited the highest value, regardless of temperature.
Figure 7a compares the measured ΔL/L0 of the TWIP steel as functions of temperature and εN. It can be seen that the length of the specimen expanded as the temperature increased. All the specimens showed a similar ΔL/L0, regardless of εN. The β of the TWIP steel was calculated based on Equation (3) as shown in Figure 7b. It was found that β exhibited considerable fluctuations with temperature changes due to the significantly small sampling interval. In this measurement, ΔL of the specimen was measured every 0.05 °C. This phenomenon appears to be caused by the sensitivity of the probe measuring the ΔL in the TMA equipment because this phenomenon was reduced by decreasing the sampling interval and performing a smoothing technique during the data analysis. However, in this study, most of the analyses were conducted focusing on the raw data as shown in Figure 7b to gain more insights on the thermophysical properties. Overall, the β of TWIP steel showed no specific patterns with temperature and exhibited similar values, regardless of temperature and εN. From another perspective, the overall curves showed a slight increasing trend with temperature. The average β of the TWIP steel was approximately 23.5 × 10−6 °C−1. Based on Refs. [55,56,57,58], the β of the TWIP steels was higher than those of plain carbon steels (13–15 × 10−6 °C−1) and austenitic stainless steels (18–21 × 10−6 °C−1). Figure 8 shows the measured ρ0 with εN. ρ0 exhibited similar values, regardless of εN. Figure 9 compares the calculated ρ using Equation (6). ρ decreased with temperature. This result clearly showed that the ρ of the TWIP steel was almost independent of εN.
The cp of the TWIP steel increased with temperature as shown in Figure 10a, which is consistent with previous results with stainless steels with no change of crystal structure and magnetic properties [59,60]. Despite some minor fluctuations, the cp of the TWIP steel primarily increased with temperature. Therefore, cp over the temperature of 400 °C was obtained through linear fitting of the measured values as shown in Figure 10b.
The k of the specimen with temperature and εN is shown in Figure 11a based on Equation (7). The k of the TWIP steel linearly increased with increasing temperature (Figure 11b). This pattern is consistent with the results of stainless steels [10,60], Mg alloys with high impurity [61], and AlCoCrFeNi high entropy alloys [62]. Interestingly, the drawn wire with an εN of 0.26 exhibited the maximum k value, regardless of temperature; whereas, hot-rolled wire showed the minimum k value, meaning that there is an optimal εN with high k. To understand the above phenomenon more effectively, k was displayed as a function of εN at a fixed temperature as shown in Figure 12. k increased with drawing strain until an εN of 0.26; subsequently, it decreased with εN, indicating that k exhibited the peak value at an εN of 0.26. Since we only measured k value at three εN, it is not clear which εN gives the highest k value. In other words, the maximum k value was known to be around an εN of 0.26, but the exact value is unknown. This is a limitation of this study; therefore, further research is necessary.

4. Discussion

It is known that the k of metals deteriorates as the amount of plastic deformation increases because the defects, such as dislocations, induced by plastic deformation impede heat flow in the metals [63]. However, it was found that k increased in the drawn TWIP steel wire compared with the hot-rolled specimen as shown in Figure 12. And then, k decreased again as the amount of deformation increased further. In metals, the energy transport is primarily conducted due to free electrons and lattice waves (phonons) [64]. Therefore, k can be expressed as thermal conductivity depending on the movement of free electrons (ke) and thermal conductivity depending on the influence of phonons (kp) as follows:
k = ke + kp
As the temperature of metals increases, the greater lattice vibration scatters the free electrons, although the energy of the free electrons and lattice vibrations increase. Under the influence of these two contradictions, the complex behaviors of k are observed depending on the temperature of the metals. Dislocations, grain boundaries, solute atoms, and precipitates are the prime scattering centers for free electrons and phonons in metals, which generally lead to the deterioration of k. In high-alloyed metals such as stainless steels and TWIP steels, the k increased with increasing temperatures, as shown in Figure 11, because the influence of kp was stronger compared with ke with increasing temperatures. As the amount of strain increases, dislocations accumulate inside the metals, which act as scattering sources for free electrons and phonons, causing ke and kp to decrease [65]. In this study, the increase in k with increasing εN can be explained by the elongation of grain during wire drawing. Figure 13 shows theehEBSD IQ, IPF, and grain shape major axis maps of the present TWIP steel at an εN of 0.51. It was measured by cutting the drawn specimen along the longitudinal direction of wire. It was clearly observed that the grains are elongated along the wire drawing direction. Figure 14 represents the mathematically calculated diameter (Dgrain), length (Lgrain), and aspect ratio (ARgrain) of grains in drawn wire with εN. ARgrain is defined as follows:
A R g r a i n = L g r a i n D g r a i n
As the wire drawing process progressed, the Lgrain increased and the Dgrain decreased, leading to an increase in ARgrain. The ARgrain had a value greater than 2.0 at. an εN of 0.51 as shown in Figure 14. This is confirmed by the elongated grains in Figure 13. These elongated grains can increase k as the amount of drawing steps increases. Since the grain boundaries along the heat flow increase, the scattering sources of free electrons and phonons increase, leading to the decrease in k [66,67]. Meanwhile, Sun et al. [25] and Wang et al. [26] revealed that the electrical conductivity increased in Cu wire and Al-Y alloy by wire drawing, respectively. They explained this mechanism in terms of grain elongation and orientation during wire drawing. Figure 15 shows the schematic description of the heat flow in drawn wire with εN. At an εN of 0.26, k increased compared with the hot-rolled steel because the increase in k due to the grain elongation was greater than the decrease in k due to the dislocations generated during wire drawing. However, as the amount of drawing step increased further, the influence of dislocations on k increased more than that of the grain elongation, causing k to decrease.
Meanwhile, it should be noted that the k of TWIP steel was much lower than that of plain carbon steels [11,29,68,69,70,71] owing to the high-alloy contents in the TWIP steel as listed in Table 1. In particular, the difference in k between the TWIP and plain carbon steels was extremely large in low temperature ranges. For example, at 100 °C, the k of plain carbon steels was approximately 45 W/m°C; in contrast, that of TWIP steel was approximately 14.2 W/m°C as shown in Figure 11. However, all steels showed a similar k in high temperature ranges where face centered cubic structure forms. For example, at 1000 °C, the k values of TWIP and plain carbon steels showed a similar value, i.e., 28 W/m °C.
Figure 16 shows the balance of k and strength of the TWIP steel with εN. It can be seen that there is an appropriate amount of drawing strain that increases both strength and k. When the k of materials is considered an important factor under service or during the manufacturing process, selecting an appropriate amount of drawing strain can lead to an effective material or process design, which could be of industrial benefit. In addition, based on the present study, care should be taken with the thermal cracks generated in TWIP steels when manufacturing products with TWIP steels using high heat flux processes such as hot working, welding, and heat treatment. The low k and high β of TWIP steels, compared with plain carbon steels, can induce thermal cracks during high heat flux processes. Accordingly, welding, heat treatment, and hot working processes for TWIP steels must be conducted using different process conditions that should be set appropriately for plain carbon steels.

5. Conclusions

Comprehensive study of the relationship between thermal conductivity and the microstructures in TWIP steel wire was performed with strain induced by wire drawing and the results are summarized as follows:
  • The YS and TS increased linearly with increasing drawing strain owing to the deformation twins and dislocations generated during wire drawing. However, TE decreased sharply with drawing strain.
  • The β of TWIP steel exhibited a similar value regardless of drawing strain. ρ decreased linearly with temperature, and it was almost independent of the drawing strain.
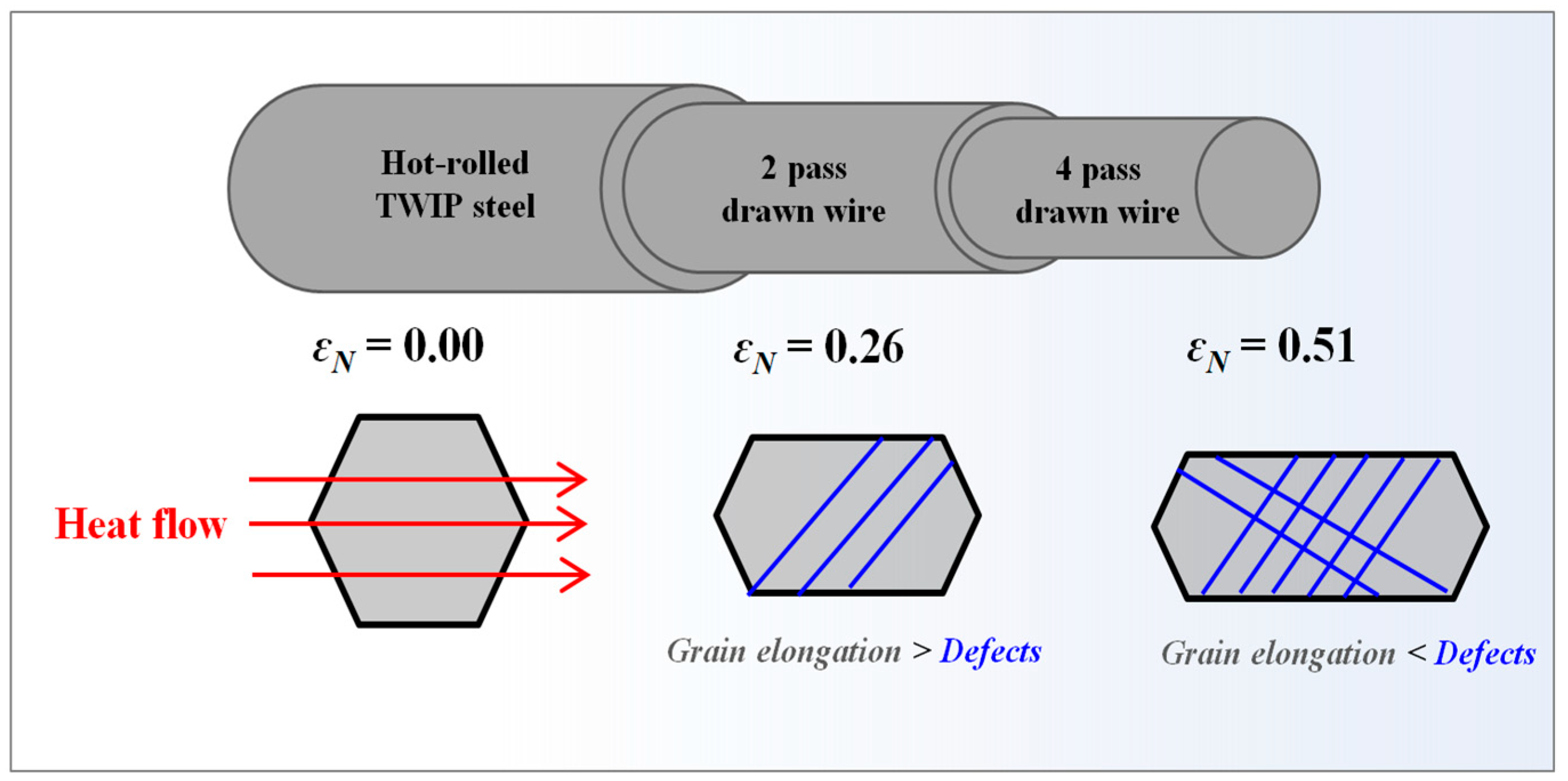
  • k initially increased and then decreased after reaching its maximum value with increasing drawing strain. At a nominal drawing strain of 0.26, k increased compared with the state of hot rolling because the increase in k due to grain elongation was greater than the decrease in k due to dislocations during wire drawing. However, as the amount of drawing step increased further, the influence of dislocations on k increased more than that of the grain elongation, causing k to decrease.

Funding

This research was supported by the “Regional Innovation Strategy (RIS)” through the National Research Foundation of Korea (NRF) funded by the Ministry of Education (MOE) (2021RIS-004).

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The original contributions presented in the study are included in the article, further inquiries can be directed to the corresponding author.

Conflicts of Interest

The author declares no conflicts of interest.

References

  1. Kim, S.J.; Lee, T.; Hwang, J.K. High-strength bolt manufactured by an extrusion-based forming process using twinning-induced plasticity steel. J. Manuf. Process 2020, 59, 33–42. [Google Scholar] [CrossRef]
  2. Hwang, J.K. The microstructure dependence of drawability in ferritic, pearlitic and TWIP steels during wire drawing. J. Mater. Sci. 2019, 54, 8743–8759. [Google Scholar] [CrossRef]
  3. Bouaziz, O.; Allain, S.; Scott, C.P.; Cugy, P.; Barbier, D. High manganese austenitic twinning induced plasticity steels: A review of the microstructure properties relationships. Curr. Opin. Solid State Mater. Sci. 2011, 15, 141–168. [Google Scholar] [CrossRef]
  4. Grassel, O.; Kruger, L.; Frommeyer, G.; Meyer, L.W. High strength Fe-Mn-(Al, Si) TRIP/TWIP steels development-properties-application. Int. J. Plast. 2000, 16, 1391–1409. [Google Scholar] [CrossRef]
  5. Chun, Y.S.; Lee, J.; Bae, C.M.; Prak, K.T.; Lee, C.S. Caliber-rolled TWIP steel for high-strength wire rods with enhanced hydrogen-delayed fracture resistance. Scripta Mater. 2012, 67, 681–684. [Google Scholar] [CrossRef]
  6. So, K.H.; Kim, J.S.; Chun, Y.S.; Park, K.T.; Lee, Y.K.; Lee, C.S. Hydrogen delayed fracture properties and internal hydrogen behavior of a Fe-18Mn-1.5Al-0.6C TWIP steel. ISIJ Int. 2009, 49, 1952–1959. [Google Scholar] [CrossRef]
  7. De Cooman, B.C.; Estrin, Y.; Kim, S.K. Twinning-induced plasticity (TWIP) steels. Acta Mater. 2018, 142, 283–362. [Google Scholar] [CrossRef]
  8. Lee, Y.K. Microstructural evolution during plastic deformation of twinning-induced plasticity steels. Scripta Mater. 2012, 66, 1002–1006. [Google Scholar] [CrossRef]
  9. Luo, Z.C.; Huang, M.X. The role of interstitial carbon atoms on the strain-hardening rate of twinning-induced plasticity steels. Scripta Mater. 2020, 178, 264–268. [Google Scholar] [CrossRef]
  10. Peet, M.J.; Hasan, H.S.; Bhadeshia, H. Prediction of thermal conductivity of steel. Int. J. Heat Mass Transf. 2011, 54, 2602–2608. [Google Scholar] [CrossRef]
  11. Xing, Y.; Wang, W.; Al-azzani, H. Assessment of thermal properties of various types of high-strength steels at elevated temperatures. Fire Saf. J. 2021, 122, 103348. [Google Scholar] [CrossRef]
  12. Wright, R.N. Wire Technology: Process Engineering and Metallurgy; Butterworth-Heinemann: Oxford, UK, 2011. [Google Scholar]
  13. El-Domiaty, A.; Kassab, S.Z. Temperature rise in wire-drawing. J. Mater. Process. Technol. 1998, 83, 72–78. [Google Scholar] [CrossRef]
  14. Kemp, I.P.; Pollard, G.; Bramley, A.N. Temperature distributions in the high speed drawing of high strength steel wire. Int. J. Mech. Sci. 1985, 27, 803–811. [Google Scholar] [CrossRef]
  15. Lee, S.K.; Ko, D.C.; Kim, B.M. Pass schedule of wire drawing process to prevent delamination for high strength steel cord wire. Mater. Des. 2009, 30, 2919–2927. [Google Scholar] [CrossRef]
  16. Hwnag, J.K. Comparison of temperature distribution between TWIP and plain carbon steels during wire drawing. Materials 2022, 15, 8696. [Google Scholar] [CrossRef]
  17. Vega, G.; Haddi, A.; Imad, A. Temperature effects on wire-drawing process: Experimental investigation. Int. J. Mater. Form. 2009, 2, 229–232. [Google Scholar] [CrossRef]
  18. Haddi, A.; Imad, A.; Vega, G. Analysis of temperature and speed effects on the drawing stress for improving the wire drawing process. Mater. Des. 2011, 32, 4310–4315. [Google Scholar] [CrossRef]
  19. Hwang, J.K. Effect of drawing speed on microstructure distribution and drawability in twinning-induced plasticity steel during wire drawing. J. Iron Steel Res. Int. 2020, 27, 577–587. [Google Scholar] [CrossRef]
  20. Suliga, M.; Kruzel, R.; Garstka, T.; Gazdowicz, J. The influence of drawing speed on structure changes in high carbon steel wires. Metalurgija 2015, 54, 161–164. [Google Scholar]
  21. Nemec, I.; Golis, B.; Pilarczyk, J.W.; Budzik, R.; Waszkielewicz, W. Effect of high-speed drawing on properties of high-carbon steel wires. Wire J. Int. 2007, 40, 63–68. [Google Scholar]
  22. De Castro, A.L.R.; Campos, H.B.; Cetlin, P.R. Influence of die semi-angle on mechanical properties of single and multiple pass drawn copper. J. Mater. Process. Technol. 1996, 60, 179–182. [Google Scholar] [CrossRef]
  23. Masse, T.; Fourment, L.; Montmitonnet, P.; Bobadilla, C.; Foissey, S. The optimal die semi-angle concept in wire drawing, examined using automatic optimization techniques. Int. J. Mater. Form. 2013, 6, 377–389. [Google Scholar] [CrossRef]
  24. Kraft, F.F.; Chakkingal, U.; Baker, G.; Wright, R.N. The effects of die angle on texture and annealing response of ETP copper wire. J. Mater. Process. Technol. 1996, 60, 171–178. [Google Scholar] [CrossRef]
  25. Sun, P.F.; Zhang, P.L.; Hou, J.P.; Wang, Q.; Zhang, Z.F. Quantitative mechanisms behind the synchronous increase of strength and electrical conductivity of cold-drawing oxygen-free Cu wires. J. Alloys Compd. 2021, 863, 158759. [Google Scholar] [CrossRef]
  26. Wang, M.; Lv, H.; Zhang, C.; Li, M.; Gao, H.; Wang, J.; Sun, B. High strength high electrical conductivity ultrafine-grained Al–Y alloy processed via cold drawing. Mater. Sci. Eng. A 2020, 772, 138824. [Google Scholar] [CrossRef]
  27. Denis, S.; Sjöström, S.; Simon, A. Coupled temperature, stress, phase transformation calculation. Metall. Mater. Trans. A 1987, 18, 1203–1212. [Google Scholar] [CrossRef]
  28. Li, K.; Tian, H. Integrated scheduling of reheating furnace and hot rolling based on improved multiobjective differential evolution. Complexity 2018, 2018, 1919438. [Google Scholar] [CrossRef]
  29. Wang, K.Y.; Jin, Y.J.; Xu, M.J.; Chen, J.S.; Lu, H. Estimation of heat transfer coefficient and phase transformation latent heat by modified pattern search method. Int. Commun. Heat Mass Transf. 2015, 68, 14–19. [Google Scholar] [CrossRef]
  30. Faini, F.; Attanasio, A.; Ceretti, E. Experimental and FE analysis of void closure in hot rolling of stainless steel. J. Mater. Process. Technol. 2018, 259, 235–242. [Google Scholar] [CrossRef]
  31. Chin, K.G.; Kang, C.Y.; Shin, S.Y.; Hong, S.; Lee, S.; Kim, H.S.; Kim, K.; Kim, N.J. Effects of Al addition on deformation and fracture mechanisms in two high manganese TWIP steels. Mater. Sci. Eng. A 2011, 528, 2922–2928. [Google Scholar] [CrossRef]
  32. Hong, S.; Shin, S.Y.; Kim, H.S.; Lee, S.; Kim, S.; Chin, K.G.; Kim, N.J. Effects of aluminum addition on tensile and cup forming properties of three Twinning Iduced Plasticity steels. Metall. Mater. Trans. A 2012, 43A, 1870–1883. [Google Scholar] [CrossRef]
  33. Han, D.K.; Kim, Y.M.; Han, H.N.; Bhadeshia, H.K.D.H.; Suh, D.W. Hydrogen and aluminium in high-manganese twinning-induced plasticity steel. Scripta Mater. 2014, 80, 9–12. [Google Scholar] [CrossRef]
  34. Jin, J.E.; Lee, Y.K. Effect of Al on microstructure and tensile properties of C-bearing high Mn TWIP steel. Acta Mater. 2012, 60, 1680–1688. [Google Scholar] [CrossRef]
  35. Yang, H.K.; Zhang, Z.J.; Zhang, Z.F. Comparison of work hardening and deformation twinning evolution in Fe-22Mn-0.6C-(1.5Al) twinning-induced plasticity steels. Scripta Mater. 2013, 68, 992–995. [Google Scholar] [CrossRef]
  36. Liu, S.; Qian, L.; Meng, J.; Ma, P.; Zhang, F. On the more persistently-enhanced strain hardening in carbon-increased Fe-Mn-C twinning-induced plasticity steel. Mater. Sci. Eng. A 2015, 639, 425–430. [Google Scholar] [CrossRef]
  37. Kusakin, P.; Belyakov, A.; Molodov, D.A.; Kaibyshev, R. On the effect of chemical composition on yield strength of TWIP steels. Mater. Sci. Eng. A 2017, 687, 82–84. [Google Scholar] [CrossRef]
  38. Saeed-Akbari, A.; Imlau, J.; Prahl, U.; Bleck, W. Derivation and variation in composition-dependent stacking fault energy maps based on subregular solution model in high-manganese steels. Metall. Mater. Trans. A 2009, 40, 3076–3090. [Google Scholar] [CrossRef]
  39. Dumay, A.; Chateau, J.P.; Allain, S.; Migot, S.; Bouaziz, O. Influence of addition elements on the stacking-fault energy and mechanical properties of an austenite Fe-Mn-C steel. Mater. Sci. Eng. A 2008, 483–484, 184–187. [Google Scholar] [CrossRef]
  40. Allain, S.; Chateau, J.P.; Bouaziz, O.; Migot, S.; Guelton, N. Correlations between the calculated stacking fault energy and the plasticity mechanisms in Fe-Mn-C alloys. Mater. Sci. Eng. A 2004, 387–389, 158–162. [Google Scholar] [CrossRef]
  41. Ghasri-Khouzani, M.; McDermid, J.R. Effect of carbon content on the mechanical properties and microstructure evolution of Fe-22Mn-C steels. Mater. Sci. Eng. A 2015, 621, 118–127. [Google Scholar] [CrossRef]
  42. Hwang, J.K. Deformation behaviors of various Fe–Mn–C twinning-induced plasticity steels: Effect of stacking fault energy and chemical composition. J. Mater. Sci. 2020, 55, 1779–1795. [Google Scholar] [CrossRef]
  43. Thomsen, E.G.; Yang, C.T.; Bierbower, T.B. An experimental investigation of the mechanics of plastic deformation of metals. Univ. Calif. Publ. Eng. 1954, 5, 89–144. [Google Scholar]
  44. Prieler, R.; Mayr, B.; Demuth, M.; Holleis, B.; Hochenauer, C. Numerical analysis of the transient heating of steel billets and the combustion process under air-fired and oxygen enriched conditions. Appl. Therm. Eng. 2016, 103, 252–263. [Google Scholar] [CrossRef]
  45. Steinboeck, A.; Graichen, K.; Wild, D.; Kiefer, T.; Kugi, A. Model-based trajectory planning, optimization, and open-loop control of a continuous slab reheating furnace. J. Process Control 2011, 21, 279–292. [Google Scholar] [CrossRef]
  46. Jang, Y.J.; Kim, S.W. An estimation of a billet temperature during reheating furnace operation. Int. J. Control Autom. 2007, 5, 43–50. [Google Scholar]
  47. Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer, 7th ed.; John Wiley and Sons Ltd.: Chichester, UK, 2012. [Google Scholar]
  48. Park, J.; Kang, M.; Sohn, S.S.; Kim, S.H.; Kim, K.S.; Kim, N.J.; Lee, S. Quasi-static and dynamic deformation mechanisms interpreted by microstructural evolution in TWinning Induced Plasticity (TWIP) steel. Mater. Sci. Eng. A 2017, 684, 54–63. [Google Scholar] [CrossRef]
  49. Badji, R.; Chauveau, T.; Bacroix, B. Texture, misorientation and mechanical anisotropy in a deformed dual phase stainless steel weld joint. Mater. Sci. Eng. A 2013, 575, 94–103. [Google Scholar] [CrossRef]
  50. Renard, K.; Jacques, P.J. On the relationship between work hardening and twinning rate in TWIP steels. Mater. Sci. Eng. A 2012, 542, 8–14. [Google Scholar] [CrossRef]
  51. Idrissi, H.; Renard, K.; Schryvers, D.; Jacques, P.J. On the relationship between the twin internal structure and the work hardening rate of TWIP steels. Scripta Mater. 2010, 63, 961–964. [Google Scholar] [CrossRef]
  52. Chen, L.; Kim, H.S.; Kim, S.K.; DeCooman, B.C. Localized deformation due to Portevin-LeChatelier effect in 18Mn-0.6C TWIP austenitic steel. ISIJ Int. 2007, 47, 1804–1812. [Google Scholar] [CrossRef]
  53. Dastur, Y.N.; Leslie, W.C. Mechanism of work hardening in Hadfield manganese steel. Metall. Trans. A 1981, 12, 749–759. [Google Scholar] [CrossRef]
  54. Qian, L.; Guo, P.; Zhang, F.; Meng, J.; Zhang, M. Abnormal room temperature serrated flow and strain rate dependence of critical strain of a Fe-Mn-C twin-induced plasticity steel. Mater. Sci. Eng. A 2013, 561, 266–269. [Google Scholar] [CrossRef]
  55. Ebert, H.P.; Braxmeier, S.; Neubert, D. Intercomparison of thermophysical property measurements on iron and steels. Int. J. Thermophys. 2019, 40, 96. [Google Scholar] [CrossRef]
  56. Xu, G.; Deng, P.; Wang, G.X.; Cao, L.F.; Liu, F. Measurement of expansion coefficients of four steel types. Ironmak. Steelmak. 2013, 40, 613–618. [Google Scholar] [CrossRef]
  57. Tripathy, H.; Rai, A.K.; Hajra, R.N.; Vijaya Shanthi, N.; Subramanian, R.; Saibaba, S. High-temperature thermophysical properties of 18Cr–9Ni–2.95 Cu–0.58 Nb–0.1 C (mass%) austenitic stainless steel. J. Therm. Anal. Calorim. 2018, 131, 2749–2761. [Google Scholar] [CrossRef]
  58. Rudtsch, S.; Ebert, H.P.; Hemberger, F.; Barth, G.; Brandt, R.; Groß, U.; Hohenauer, W.; Jaenicke-Roessler, K.; Kaschnitz, E.; Pfaff, E.; et al. Intercomparison of thermophysical property measurements on an austenitic stainless steel. Int. J. Thermophys. 2005, 26, 855–867. [Google Scholar] [CrossRef]
  59. Ahsan, F.; Razmi, J.; Ladani, L. Experimental measurement of thermal diffusivity, conductivity and specific heat capacity of metallic powders at room and high temperatures. Powder Technol. 2020, 374, 648–657. [Google Scholar] [CrossRef]
  60. Mayrhofer, M.; Koller, M.; Seemann, P.; Prieler, R.; Hochenauer, C. CFD investigation of a vertical annealing furnace for stainless steel and non-ferrous alloys strips–A comparative study on air-staged & MILD combustion. Therm. Sci. Eng. Prog. 2022, 28, 101056. [Google Scholar]
  61. Ying, T.; Zheng, M.Y.; Li, Z.T.; Qiao, X.G. Thermal conductivity of as-cast and as-extruded binary Mg–Al alloys. J. Alloys Compd. 2014, 608, 19–24. [Google Scholar] [CrossRef]
  62. Chou, H.P.; Chang, Y.S.; Chen, S.K.; Yeh, J.W. Microstructure, thermophysical and electrical properties in AlxCoCrFeNi (0≤ x≤ 2) high-entropy alloys. Mater. Sci. Eng. B 2009, 163, 184–189. [Google Scholar] [CrossRef]
  63. Mora-Barzaga, G.; Miranda, E.N.; Bringa, E.M. Do dislocations always decrease thermal conductivity? Int. J. Therm. Sci. 2023, 193, 108474. [Google Scholar] [CrossRef]
  64. Tritt, T.M. Thermal Conductivity: Theory, Properties, and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2005; pp. 21–91. [Google Scholar]
  65. Kemp, W.R.G.; Klemens, P.G.; Tainsh, R.J. The lattice thermal conductivity of copper alloys: Effect of plastic deformation and annealing. Philos. Mag. 1959, 4, 845–857. [Google Scholar] [CrossRef]
  66. Kenfaui, D.; Chateigner, D.; Gomina, M.; Noudem, J.G.; Ouladdiaf, B.; Dauscher, A.; Lenoir, B. Volume texture and anisotropic thermoelectric properties in Ca3Co4O9 bulk materials. Mater. Today 2015, 2, 637–646. [Google Scholar] [CrossRef]
  67. Liu, Y.; Bian, Y.; Chernatynskiy, A.; Han, Z. Effect of grain boundary angle on the thermal conductivity of nanostructured bicrystal ZnO based on the molecular dynamics simulation method. Int. J. Heat Mass Transf. 2019, 145, 118791. [Google Scholar] [CrossRef]
  68. Mayr, B.; Prieler, R.; Demuth, M.; Moderer, L.; Hochenauer, C. CFD analysis of a pusher type reheating furnace and the billet heating characteristic. Appl. Therm. Eng. 2017, 115, 986–994. [Google Scholar] [CrossRef]
  69. Li, H.; Zaho, G.; Niu, S.; Huang, C. FEM simulation of quenching process and experimental verification of simulation results. Mater. Sci. Eng. A 2007, 452–453, 705–714. [Google Scholar]
  70. Fakir, R.; Barka, N.; Brousseau, J. Case study of laser hardening process applied to 4340 steel cylindrical specimens using simulation and experimental validation. Case Stud. Therm. Eng. 2018, 11, 15–25. [Google Scholar] [CrossRef]
  71. Casal, J.M.; Porteiro, J.; Míguez, J.L.; Vázquez, A. New methodology for CFD three-dimensional simulation of a walking beam type reheating furnace in steady state. Appl. Therm. Eng. 2015, 86, 69–80. [Google Scholar] [CrossRef]
Materials 17 05263 g001
Figure 1. Schematic description of specimen preparation in drawn wire and a photograph of specimens for evaluation of thermal properties, tensile properties, and microstructure.
Figure 1. Schematic description of specimen preparation in drawn wire and a photograph of specimens for evaluation of thermal properties, tensile properties, and microstructure.
Materials 17 05263 g001
Materials 17 05263 g002
Figure 2. EBSD image quality, inverse pole figure, kernel average misorientation, and twin boundary maps of TWIP steel at nominal drawing strains of (a) 0.00, (b) 0.26, and (c) 0.51.
Figure 2. EBSD image quality, inverse pole figure, kernel average misorientation, and twin boundary maps of TWIP steel at nominal drawing strains of (a) 0.00, (b) 0.26, and (c) 0.51.
Materials 17 05263 g002
Materials 17 05263 g003
Figure 3. Comparison of relative twin density and kernel average misorientation values with nominal drawing strain.
Figure 3. Comparison of relative twin density and kernel average misorientation values with nominal drawing strain.
Materials 17 05263 g003
Materials 17 05263 g004
Figure 4. (a) Engineering and (b) true stress–strain curves of hot-rolled TWIP steel.
Figure 4. (a) Engineering and (b) true stress–strain curves of hot-rolled TWIP steel.
Materials 17 05263 g004
Materials 17 05263 g005
Figure 5. Comparison of (a) yield and tensile strengths and (b) total elongation of TWIP steel with nominal drawing strain.
Figure 5. Comparison of (a) yield and tensile strengths and (b) total elongation of TWIP steel with nominal drawing strain.
Materials 17 05263 g005
Materials 17 05263 g006
Figure 6. Variations in measured thermal diffusivity of TWIP steel with nominal drawing strain and temperature.
Figure 6. Variations in measured thermal diffusivity of TWIP steel with nominal drawing strain and temperature.
Materials 17 05263 g006
Materials 17 05263 g007
Figure 7. Variations in measured (a) thermal expansion ratio of length and (b) thermal expansion coefficient of TWIP steel as functions of nominal drawing strain and temperature.
Figure 7. Variations in measured (a) thermal expansion ratio of length and (b) thermal expansion coefficient of TWIP steel as functions of nominal drawing strain and temperature.
Materials 17 05263 g007
Materials 17 05263 g008
Figure 8. Comparison of measured density of TWIP steel at room temperature with nominal drawing strain.
Figure 8. Comparison of measured density of TWIP steel at room temperature with nominal drawing strain.
Materials 17 05263 g008
Materials 17 05263 g009
Figure 9. Comparison of calculated density of TWIP steel as functions of nominal drawing strain and temperature.
Figure 9. Comparison of calculated density of TWIP steel as functions of nominal drawing strain and temperature.
Materials 17 05263 g009
Materials 17 05263 g010
Figure 10. Variations in (a) measured and (b) linearly fitted specific heat capacity of TWIP steel with nominal drawing strain and temperature.
Figure 10. Variations in (a) measured and (b) linearly fitted specific heat capacity of TWIP steel with nominal drawing strain and temperature.
Materials 17 05263 g010
Materials 17 05263 g011
Figure 11. (a) Variations in calculated thermal conductivity of TWIP steels with nominal drawing strain and temperature and (b) their linear fitted lines.
Figure 11. (a) Variations in calculated thermal conductivity of TWIP steels with nominal drawing strain and temperature and (b) their linear fitted lines.
Materials 17 05263 g011
Materials 17 05263 g012
Figure 12. Comparison of thermal conductivity of TWIP steel with nominal drawing strain at temperatures of (a) 100 °C, (b) 300 °C, (c) 500 °C, and (d) 800 °C.
Figure 12. Comparison of thermal conductivity of TWIP steel with nominal drawing strain at temperatures of (a) 100 °C, (b) 300 °C, (c) 500 °C, and (d) 800 °C.
Materials 17 05263 g012
Materials 17 05263 g013
Figure 13. EBSD image quality, inverse pole figure, and grain shape major axis maps of TWIP steel at a nominal drawing strain of 0.51. It was measured by cutting the specimen along the longitudinal direction of drawn wire.
Figure 13. EBSD image quality, inverse pole figure, and grain shape major axis maps of TWIP steel at a nominal drawing strain of 0.51. It was measured by cutting the specimen along the longitudinal direction of drawn wire.
Materials 17 05263 g013
Materials 17 05263 g014
Figure 14. Variations in calculated diameter, length, and aspect ratio of grain in the drawn wire with nominal drawing strain.
Figure 14. Variations in calculated diameter, length, and aspect ratio of grain in the drawn wire with nominal drawing strain.
Materials 17 05263 g014
Materials 17 05263 g015
Figure 15. Schematic description of heat flow at grains in drawn wire with nominal drawing strain.
Figure 15. Schematic description of heat flow at grains in drawn wire with nominal drawing strain.
Materials 17 05263 g015
Materials 17 05263 g016
Figure 16. Balance of (a) thermal conductivity and yield strength and (b) thermal conductivity and tensile strength of drawn TWIP steel wire.
Figure 16. Balance of (a) thermal conductivity and yield strength and (b) thermal conductivity and tensile strength of drawn TWIP steel wire.
Materials 17 05263 g016
Table 1. Main chemical composition and stacking fault energy of test TWIP steel.
Table 1. Main chemical composition and stacking fault energy of test TWIP steel.
Chemical Compositions (wt.%)Stack Fault Energy in RT (mJ/m2)
CMnAlFe
0.70 17.18 1.50 Bal.31.2
Table 2. Process condition of dies for drawing test in the present study.
Table 2. Process condition of dies for drawing test in the present study.
No. of PassWire Diameter
(mm)		Die Angle
(°)		RA Per Pass
(%)		Total RA
(%)		Nominal
Drawing Strain
015.00 --0.00
114.001212.912.90.14
213.181211.422.80.26
312.301212.932.80.40
411.631210.639.90.51
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Hwang, J.-K. Effect of Plastic Deformation on Thermal Properties in Twinning-Induced Plasticity Steel. Materials 2024, 17, 5263. https://doi.org/10.3390/ma17215263

AMA Style

Hwang J-K. Effect of Plastic Deformation on Thermal Properties in Twinning-Induced Plasticity Steel. Materials. 2024; 17(21):5263. https://doi.org/10.3390/ma17215263

Chicago/Turabian Style

Hwang, Joong-Ki. 2024. "Effect of Plastic Deformation on Thermal Properties in Twinning-Induced Plasticity Steel" Materials 17, no. 21: 5263. https://doi.org/10.3390/ma17215263

APA Style

Hwang, J.-K. (2024). Effect of Plastic Deformation on Thermal Properties in Twinning-Induced Plasticity Steel. Materials, 17(21), 5263. https://doi.org/10.3390/ma17215263

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop