Analysis of the Deburring Efficiency of EN-AW 7075 Aluminum Alloy Parts with Complex Geometric Shapes Considering the Tool Path Strategy During Multi-Axis Brushing
Abstract
1. Introduction
2. Materials and Methods
2.1. Material and Shape of the Samples
2.2. General Methodology
2.3. Tools
2.4. Brushing Conditions
- nc—the number of square points
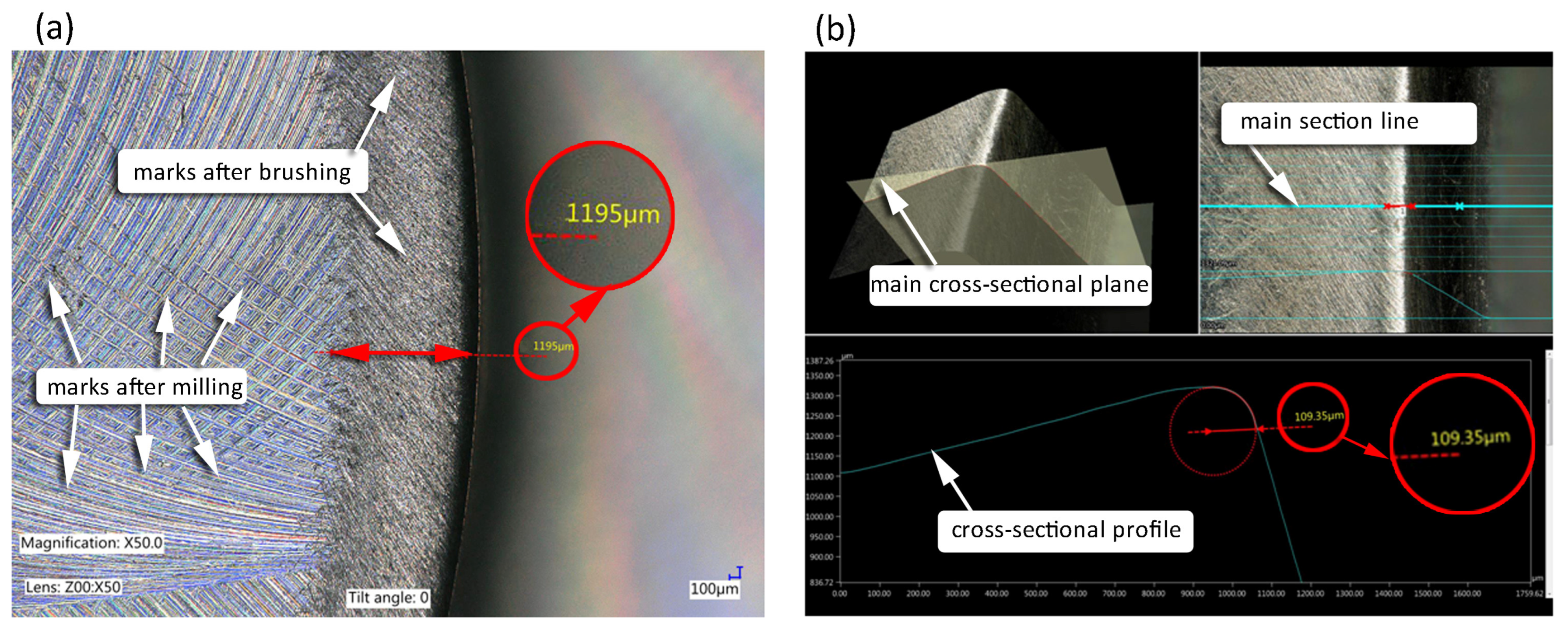
2.5. Measurements Methodology
3. Results
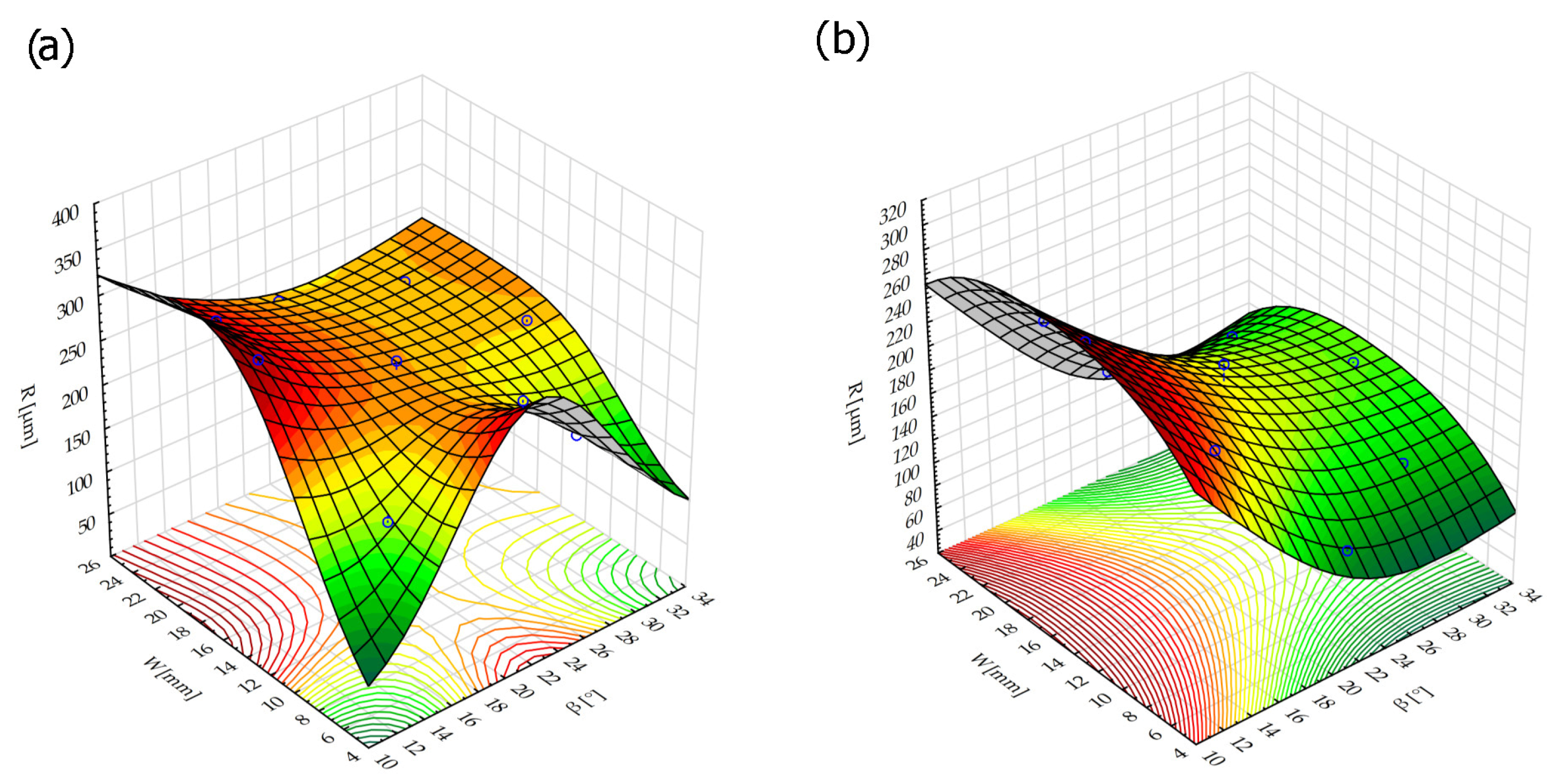
3.1. Surface Roughness
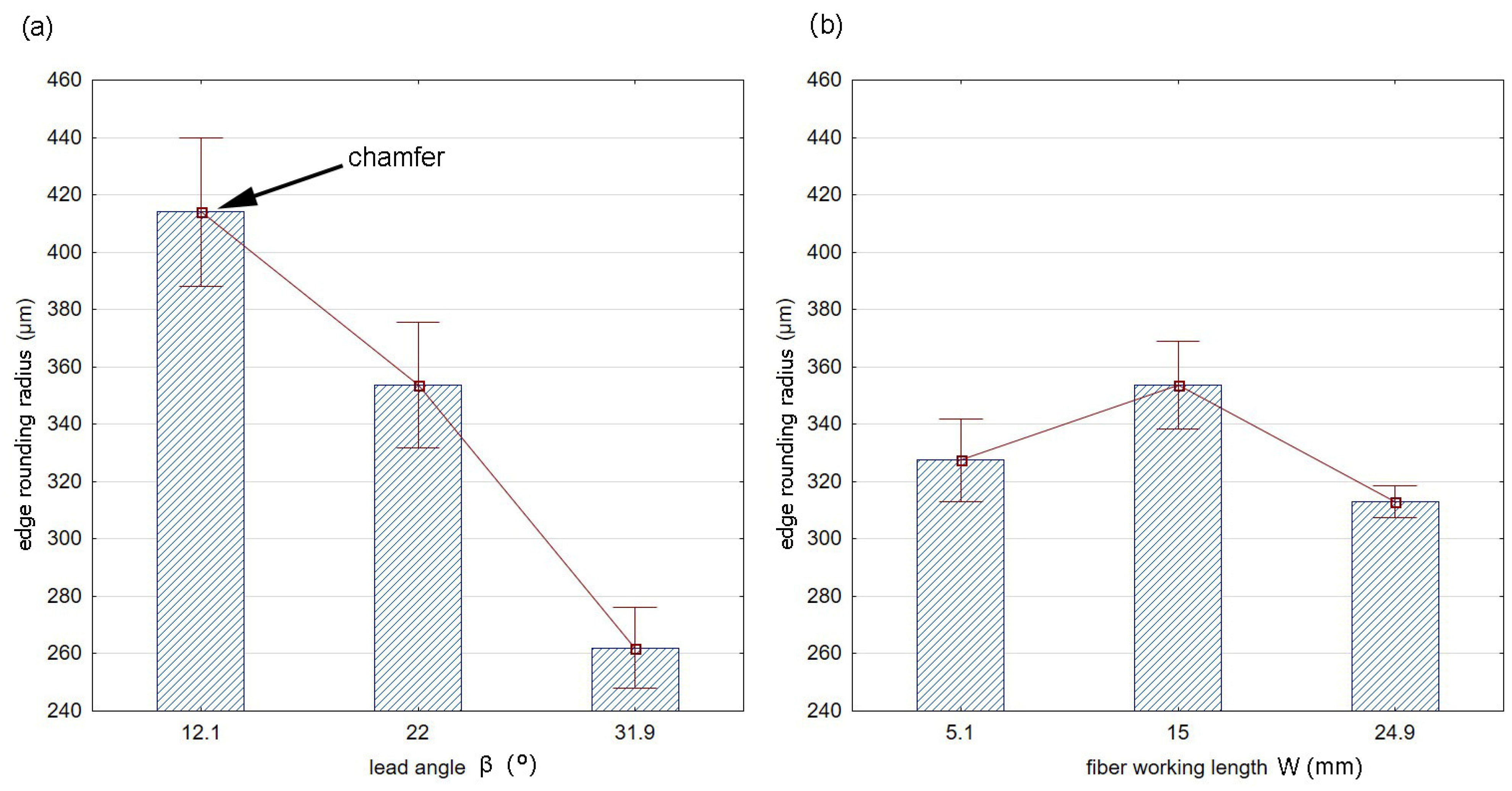
- β—lead angle
- W—fiber working length
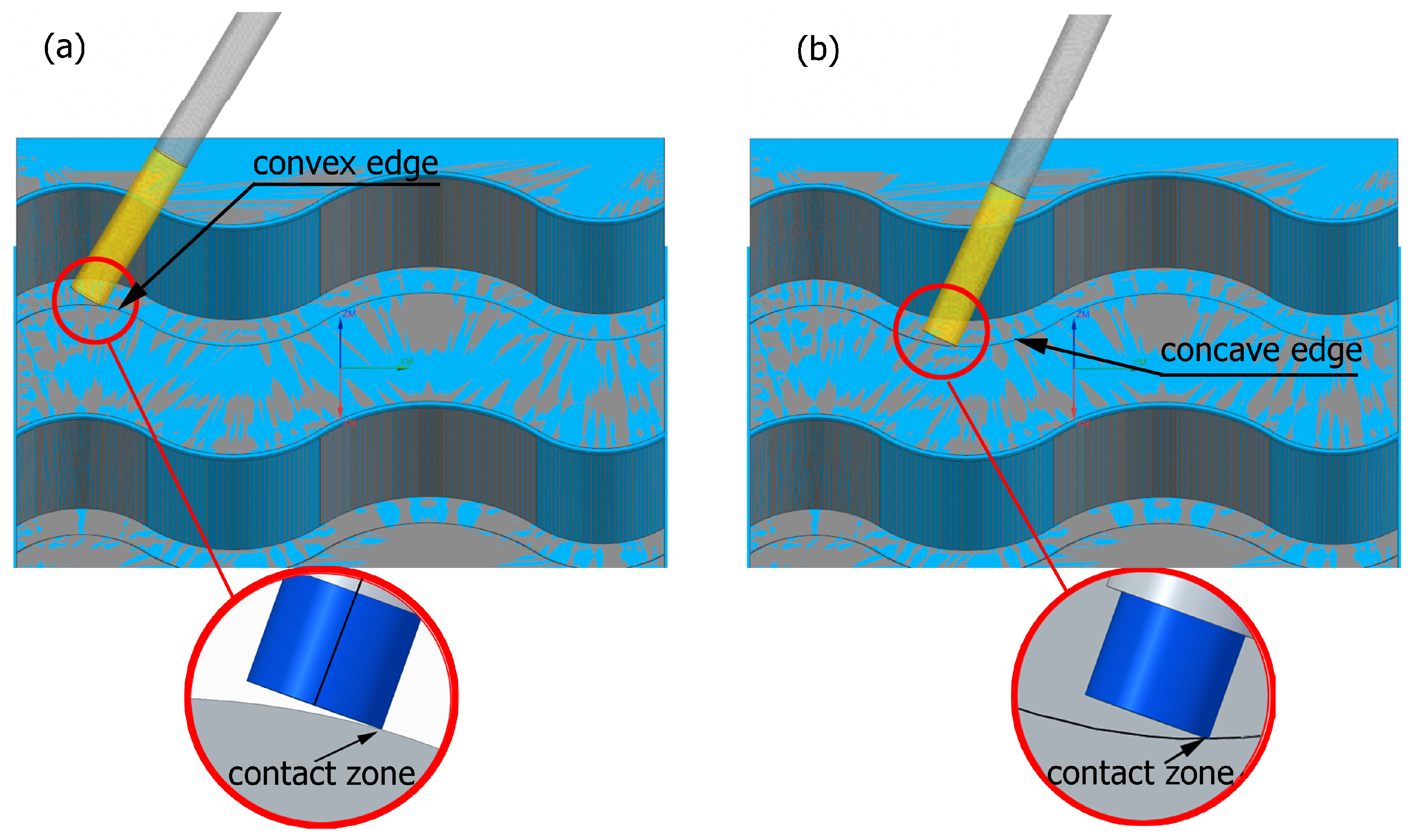
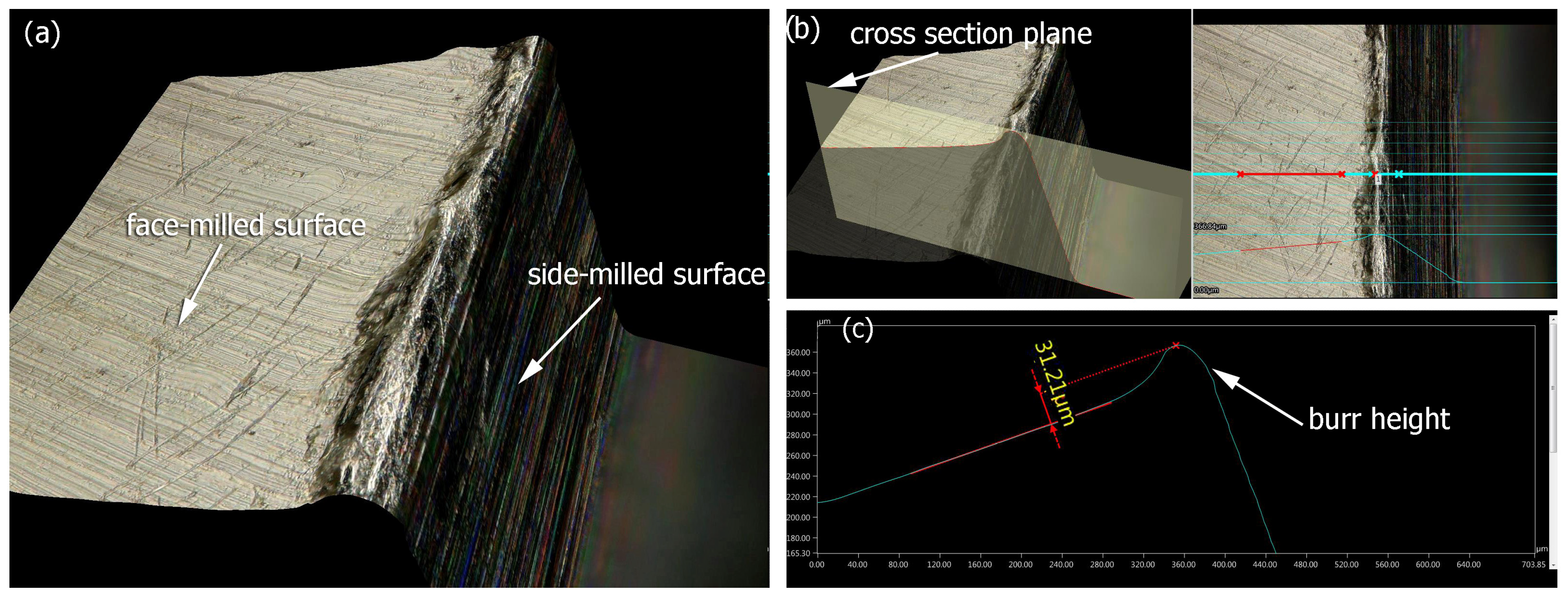
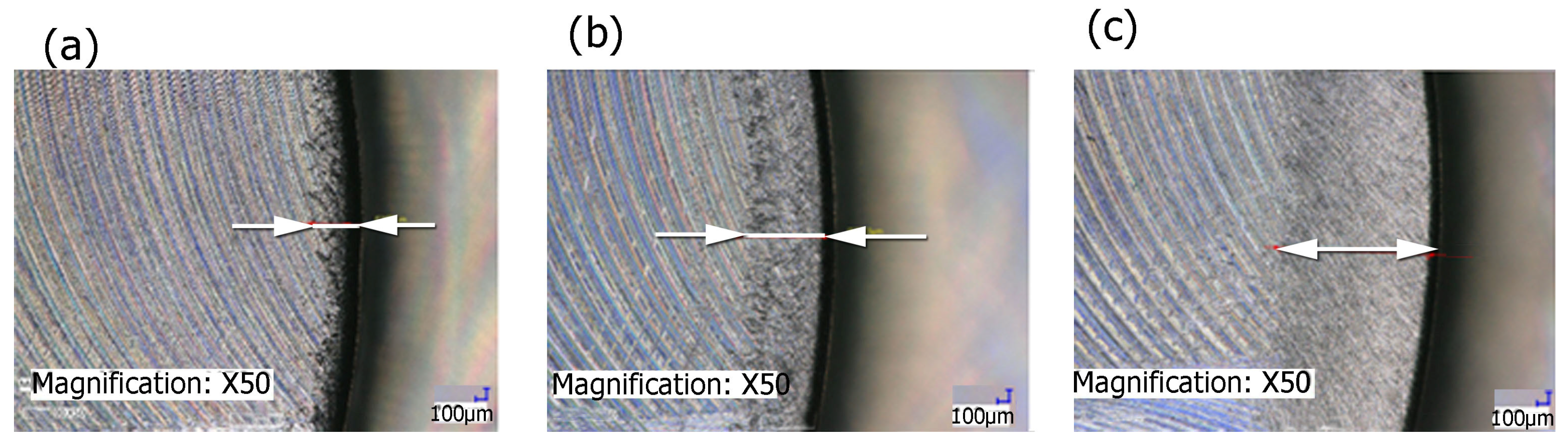
3.2. Edge States
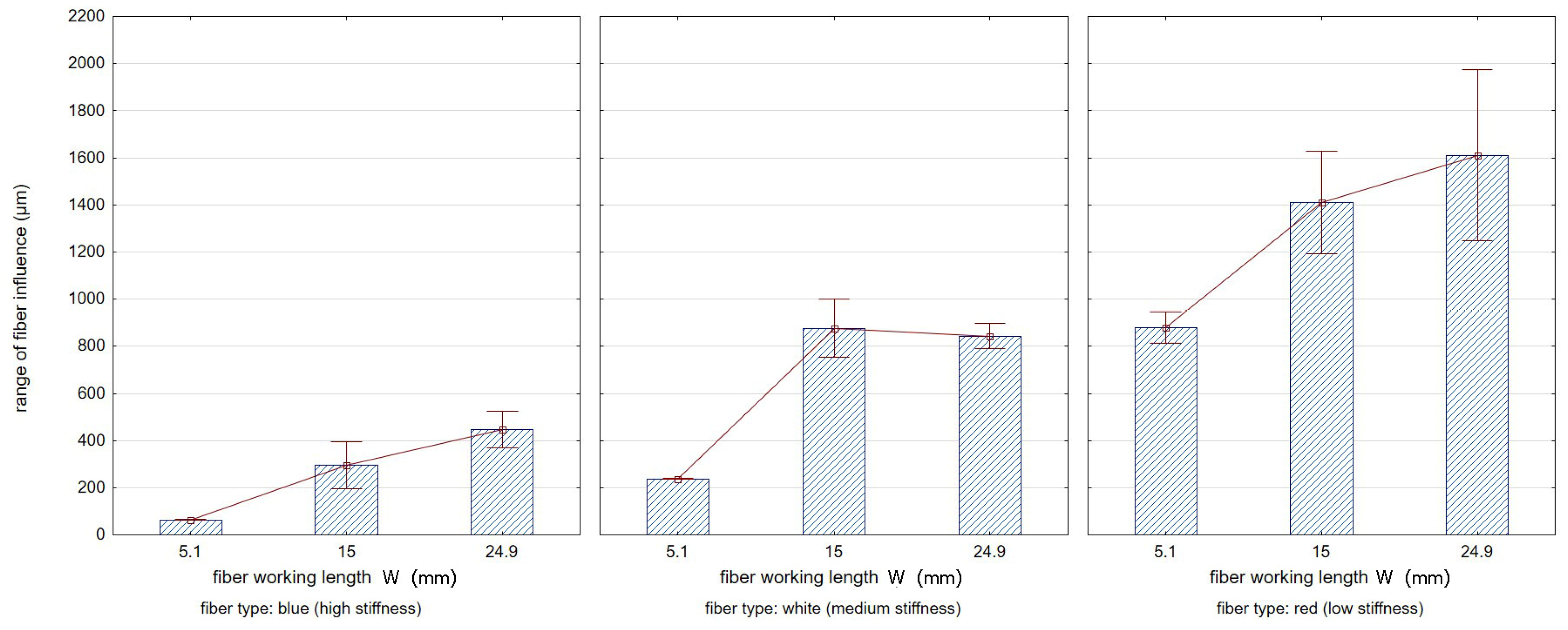
3.3. Range of Fiber Influence
4. Discussion
5. Conclusions
- -
- for the blue (high stiffness) and white (medium stiffness) fibers, the roughness increased after the brushing process compared to the roughness after milling (from Sa = 0.484 µm after milling to Sa = 1.07 µm for the blue and Sa = 0.83 µm for the white fibers);
- -
- for the red (low stiffness) fibers, the roughness remained the same (Sa = 0.46 µm) as after the milling process, which is important in terms of surface quality;
- -
- the brushing treatment led to changes in the arrangement of micro-irregularities (removal of milling marks);
- -
- after the milling process, burrs appeared on the edges, which demonstrates the necessity of using further processing to resolve unfavorable edge states;
- -
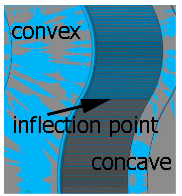
- a change in the edge curvature (convex and concave) influenced the change in the contact conditions between the tool and the workpiece for a lead angle of β = 0;
- -
- the use of a lead angle of β = 0 led to a change in the chamfer width along the processed edge, depending on the edge curvature;
- -
- a machining operation conducted with a lead angle of β > 0 ensured a stable and repeatable edge radius regardless of the edge curvature;
- -
- as the fiber working length and the lead angle increased, the range of fiber influence on surfaces forming an edge increased too.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hashimura, M.; Ueda, K.; Dornfeld, D.; Manabe, K. Analysis of Three-Dimensional Burr Formation in Oblique Cutting. CIRP Ann.—Manuf. Technol. 1995, 44, 27–30. [Google Scholar] [CrossRef]
- Hashimura, M.; Hassamontr, J.; Dornfeld, D.A. Effect of In-Plane Exit Angle and Rake Angles on Burr Height and Thickness in Face Milling Operation. J. Manuf. Sci. Eng. 1999, 121, 13–19. [Google Scholar] [CrossRef]
- Chern, G.-L. Study on Mechanisms of Burr Formation and Edge Breakout near the Exit of Orthogonal Cutting. J. Mater. Process. Technol. 2006, 176, 152–157. [Google Scholar] [CrossRef]
- Toropov, A.A.; Ko, S.L.; Lee, J.M. A New Burr Formation Model for Orthogonal Cutting of Ductile Materials. CIRP Ann.—Manuf. Technol. 2006, 55, 55–58. [Google Scholar] [CrossRef]
- Gillespie, L.K.; Blotter, P.T. The Formation and Properties of Machining Burrs. J. Eng. Ind. 1976, 98, 66–74. [Google Scholar] [CrossRef]
- Lin, T.-R. Experimental Study of Burr Formation and Tool Chipping in the Face Milling of Stainless Steel. J. Mater. Process. Technol. 2000, 108, 12–20. [Google Scholar] [CrossRef]
- Biermann, D.; Hartmann, H. Reduction of Burr Formation in Drilling Using Cryogenic Process Cooling. Procedia CIRP 2012, 3, 85–90. [Google Scholar] [CrossRef]
- Kim, J.; Min, S.; Dornfeld, D.A. Optimization and Control of Drilling Burr Formation of AISI 304 L and AISI 4118 Based on Drilling Burr Control Charts. Int. J. Mach. Tools Manuf. 2001, 41, 923–936. [Google Scholar] [CrossRef]
- Ko, S.-L.; Chang, J.-E.; Yang, G.-E. Burr Minimizing Scheme in Drilling. J. Mater. Process. Technol. 2003, 140, 237–242. [Google Scholar] [CrossRef]
- Mahdy, A.M. Economic Drilling Conditions for a given Deburring Radius. J. Mater. Process. Technol. 2001, 110, 197–205. [Google Scholar] [CrossRef]
- Min, S.; Kim, J.; Dornfeld, D.A. Development of a Drilling Burr Control Chart for Low Alloy Steel, AISI 4118. J. Mater. Process. Technol. 2001, 113, 4–9. [Google Scholar] [CrossRef]
- Kim, Y.; Kim, J.; Lee, S.-K. Investigation of Surface Uniformity Machined by Ceramic Brush. Int. J. Adv. Manuf. Technol. 2018, 94, 2593–2603. [Google Scholar] [CrossRef]
- Aurich, J.C.; Dornfeld, D.; Arrazola, P.J.; Franke, V.; Leitz, L.; Min, S. Burrs—Analysis, Control and Removal. CIRP Ann. 2009, 58, 519–542. [Google Scholar] [CrossRef]
- Chu, C.-H.; Dornfeld, D. Tool Path Planning for Avoiding Exit Burrs. J. Manuf. Process. 2000, 2, 116–123. [Google Scholar] [CrossRef]
- Chu, C.-H.; Dornfeld, D. Geometric Approaches for Reducing Burr Formation in Planar Milling by Avoiding Tool Exits. J. Manuf. Process. 2005, 7, 182–195. [Google Scholar] [CrossRef]
- Hassamontr, J.; Blondaz, L.; Dornfeld, D.A. Avoiding Exit Burr in CNC End Milling by an Adapted Tool Path. In Proceedings of the ASME 1998 International Mechanical Engineering Congress and Exposition, Anaheim, CA, USA, 15–20 November 1998; Volume 16066, pp. 497–510. [Google Scholar]
- Gdula, M.; Burek, J. Cutting Layer and Cutting Forces in a 5-Axis Milling of Sculptured Surfaces Using the Toroidal Cutter. J. Mach. Eng. 2017, 4, 98–122. [Google Scholar] [CrossRef]
- Gdula, M.; Mrówka-Nowotnik, G. Analysis of Tool Wear, Chip and Machined Surface Morphology in Multi-Axis Milling Process of Ni-Based Superalloy Using the Torus Milling Cutter. Wear 2023, 520–521, 204652. [Google Scholar] [CrossRef]
- Zaleski, K.; Matuszak, J.; Zyśko, A. Highly Efficient Milling on the Example of Selected Machining Strategies. Adv. Sci. Technol. Res. J. 2020, 14, 167–177. [Google Scholar] [CrossRef] [PubMed]
- Szajna, A.; Bazan, A.; Tymczyszyn, J.; Kawalec, M.; Rydzak, T.; Kubik, P. The Effect of Milling Parameters of Vanadis 4 Extra Steel on Cutting Force Values and Roughness of Machined Surface. Adv. Sci. Technol. Res. J. 2024, 18, 181–190. [Google Scholar] [CrossRef]
- Żurawski, K.; Żurek, P.; Kawalec, A.; Bazan, A.; Olko, A. Modeling of Surface Topography after Milling with a Lens-Shaped End-Mill, Considering Runout. Materials 2022, 15, 1188. [Google Scholar] [CrossRef]
- Gancarczyk, K.; Albrecht, R.; Kawalec, M.; Kościelniak, B.; Gradzik, A.; Szeliga, D.; Kawalec, A.; Ziaja, W.; Motyka, M. The Effect of Re Content on Microstructure and Creep Resistance of Single Crystal Castings Made of Nickel-Based Superalloys. Adv. Sci. Technol. Res. J. 2024, 18, 291–305. [Google Scholar] [CrossRef]
- Dąbrowski, L.; Marciniak, M.; Wieczorek, W. Advancement of abrasive flow machining using an anodic solution. J. New Mater. Electrochem. Syst. 2006, 9, 439–445. [Google Scholar]
- Yin, S.; Shinmura, T. Vertical Vibration-Assisted Magnetic Abrasive Finishing and Deburring for Magnesium Alloy. Int. J. Mach. Tools Manuf. 2004, 44, 1297–1303. [Google Scholar] [CrossRef]
- Fang, L.; Zhao, J.; Sun, K.; Zheng, D.; Ma, D. Temperature as Sensitive Monitor for Efficiency of Work in Abrasive Flow Machining. Wear 2009, 266, 678–687. [Google Scholar] [CrossRef]
- Jeong, Y.H.; HanYoo, B.; Lee, H.U.; Min, B.-K.; Cho, D.-W.; Lee, S.J. Deburring Microfeatures Using Micro-EDM. J. Mater. Process. Technol. 2009, 209, 5399–5406. [Google Scholar] [CrossRef]
- Bähre, D.; Brünnet, H.; Swat, M. Investigation of One-Way Abrasive Flow Machining and In-Process Measurement of Axial Forces. Procedia CIRP 2012, 1, 419–424. [Google Scholar] [CrossRef]
- Swat, M.; Brünnet, H.; Lyubenova, N.; Schmitt, J.; Diebels, S.; Bähre, D. Improved Process Control and Model of Axial Forces of One-Way Abrasive Flow Machining. Procedia CIRP 2014, 14, 19–24. [Google Scholar] [CrossRef][Green Version]
- Kwon, B.C.; Kim, K.H.; Ko, S.L. New Abrasive Deburring Method Using Suction for Micro Burrs at Intersecting Holes. Ann. CIRP 2016, 65, 145–148. [Google Scholar] [CrossRef]
- Halladay, J. Practical Applications of Thermal Deburring and Electro-Chemical Deburring; Society of Manufacturing Engineers: Southfield, MI, USA, 1985. [Google Scholar]
- Sarkar, S.; Mitra, S.; Bhattacharyya, B. Mathematical Modeling for Controlled Electrochemical Deburring (ECD). J. Mater. Process. Technol. 2004, 147, 241–246. [Google Scholar] [CrossRef]
- Prathap, R. A Case Study to Setup Optimum Process Parameters in Thermal Deburring for Removing the Burr in Barrel of Fuel Injector Pump Using Taguchi Approach. ResearchGate 2012, 2, 26–35. [Google Scholar] [CrossRef]
- Matuszak, J.; Klonica, M.; Zagorski, I. Effect of Brushing Conditions on Axial Forces in Ceramic Brush Surface Treatment. In Proceedings of the 2019 IEEE 5th International Workshop on Metrology for AeroSpace (MetroAeroSpace), Torino, Italy, 19–21 June 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 644–648. [Google Scholar]
- Matuszak, J.; Zaleski, K.; Ciecieląg, K.; Skoczylas, A. Analysis of the Effectiveness of Removing Surface Defects by Brushing. Materials 2022, 15, 7833. [Google Scholar] [CrossRef]
- Matuszak, J. Comparative Analysis of the Effect of Machining with Wire and Ceramic Brushes on Selected Properties of the Surface Layer of EN AW-7075 Aluminium Alloy. Adv. Sci. Technol. Res. J. 2022, 16, 50–56. [Google Scholar] [CrossRef]
- Skoczylas, A.; Zaleski, K. Study on the Surface Layer Properties and Fatigue Life of a Workpiece Machined by Centrifugal Shot Peening and Burnishing. Materials 2022, 15, 6677. [Google Scholar] [CrossRef] [PubMed]
- Skoczylas, A.; Kłonica, M. Selected Properties of the Surface Layer of C45 Steel Samples after Slide Burnishing. Materials 2023, 16, 6513. [Google Scholar] [CrossRef] [PubMed]
- Ciecieląg, K. Machinability Measurements in Milling and Recurrence Analysis of Thin-Walled Elements Made of Polymer Composites. Materials 2023, 16, 4825. [Google Scholar] [CrossRef] [PubMed]
- Ciecieląg, K.; Zaleski, K.; Kęcik, K. Effect of Milling Parameters on the Formation of Surface Defects in Polymer Composites. Mater. Sci. 2022, 57, 882–893. [Google Scholar] [CrossRef]
- Cariapa, V.; Stango, R.J.; Chen, L.; Hermann, R. Aspects of Process Model for Automatic Control of Edge-Deburring with Filamentary Brush. J. Eng. Ind. 1992, 114, 294–300. [Google Scholar] [CrossRef]
- Stango, R.J.; Chen, L.; Cariapa, V. Automated Deburring with a Filamentary Brush: Prescribed Burr Geometry. J. Manuf. Sci. Eng. 1999, 121, 385–392. [Google Scholar] [CrossRef]
- Kumar, S.; Dornfeld, D. Basic Approach to a Prediction System for Burr Formation in Face Milling. J. Manuf. Process. 2003, 5, 127–142. [Google Scholar] [CrossRef]
- Onstein, I.F.; Bjerkeng, M.; Martinsen, K. Automated Tool Trajectory Generation for Robotized Deburring of Cast Parts Based on 3D Scans. Procedia CIRP 2023, 118, 507–512. [Google Scholar] [CrossRef]
- Stango, R.J.; Cariapa, V.; Zuzanski, M. Contact Zone Force Profile and Machining Performance of Filamentary Brush. J. Manuf. Sci. Eng. 2005, 127, 217–226. [Google Scholar] [CrossRef]
- Stango, R.J.; Shia, C.-Y. Analysis of Filament Deformation for a Freely Rotating Cup Brush. J. Manuf. Sci. Eng. 1997, 119, 298–306. [Google Scholar] [CrossRef]
- Matuszak, J.; Zaleski, K. Analysis of Deburring Effectiveness and Surface Layer Properties around Edges of Workpieces Made of 7075 Aluminium Alloy. Aircr. Eng. Aerosp. Technol. 2018, 90, 515–523. [Google Scholar] [CrossRef]
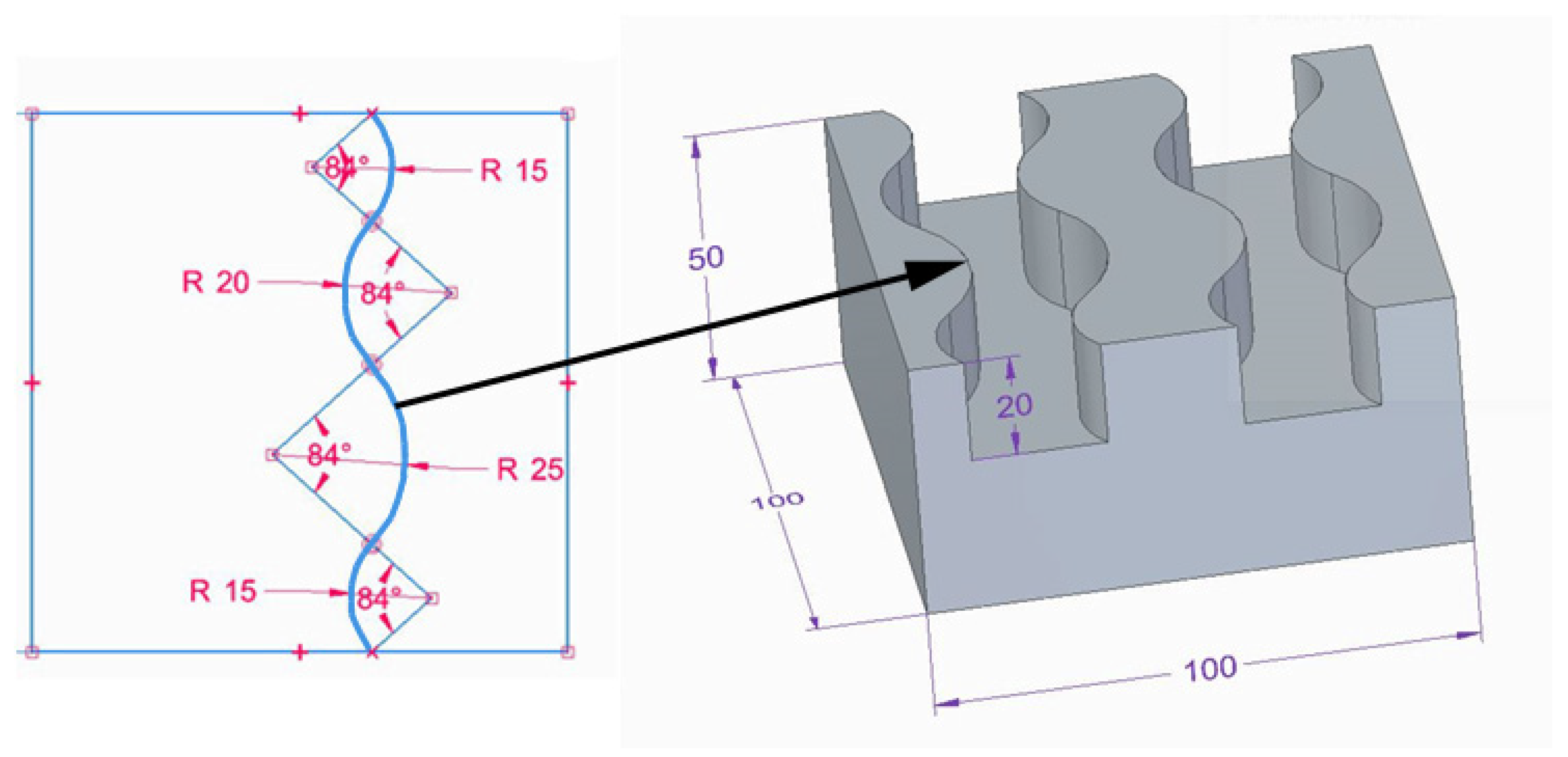

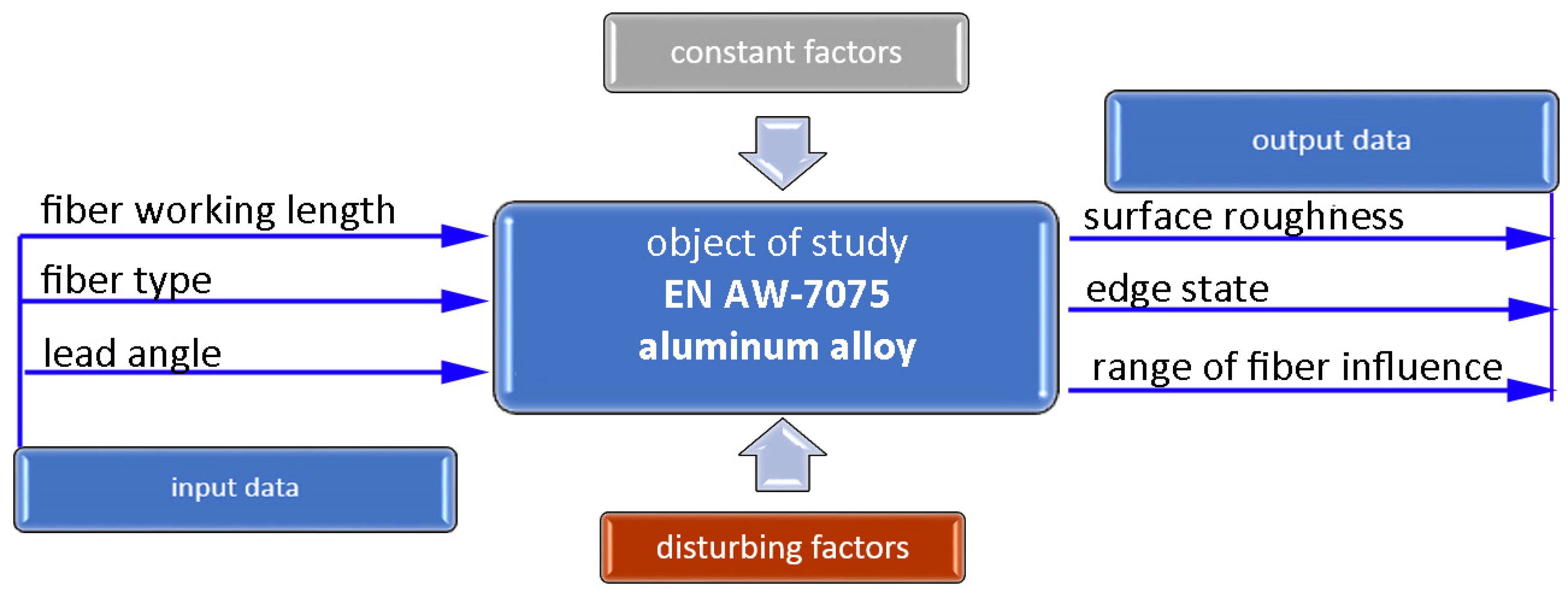

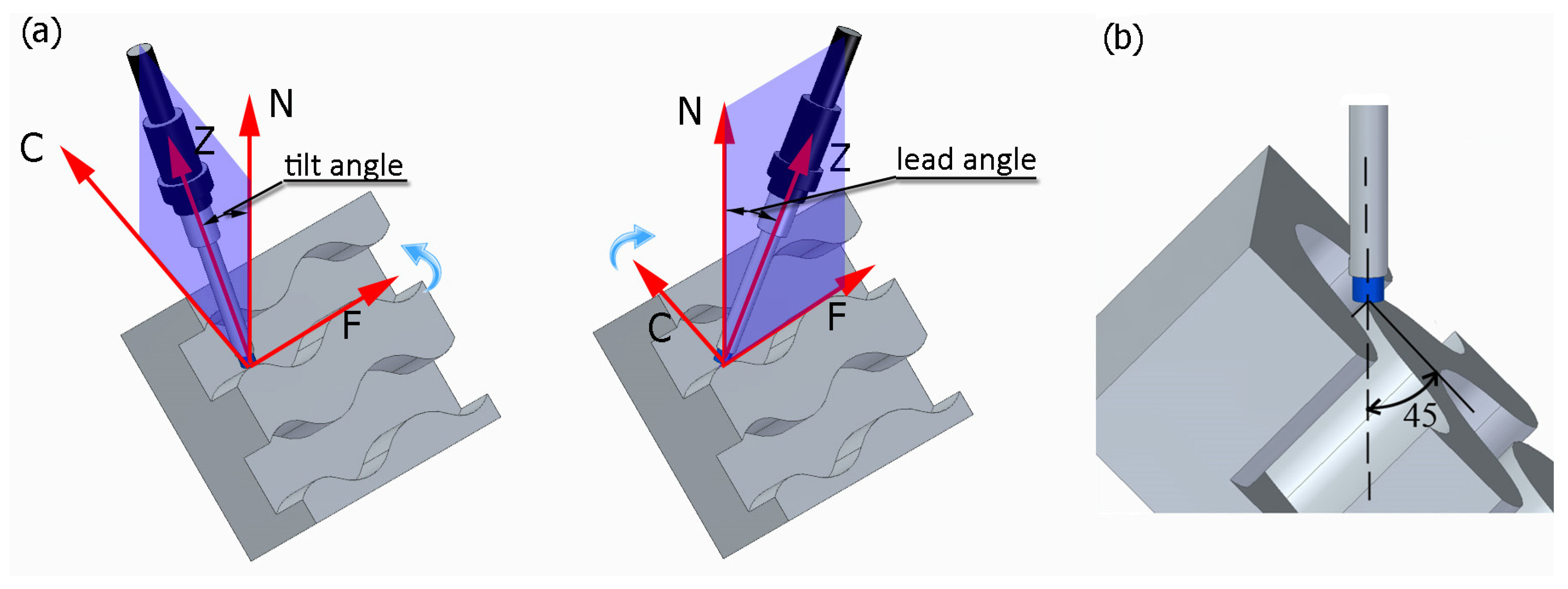




| Chemical Composition, wt. % | Physical Properties | Specimen | ||
|---|---|---|---|---|
| Cu | 1.59 | Rm | 559 MPa |  |
| Mn | 0.01 | |||
| Mg | 2.56 | |||
| Cr | 0.18 | Rp0.2 | 448 MPa | |
| Zn | 5.78 | |||
| Si | 0.07 | |||
| Fe | 0.13 | HB | 172 | |
| Ti | 0.05 | |||
| Al | Rest | |||
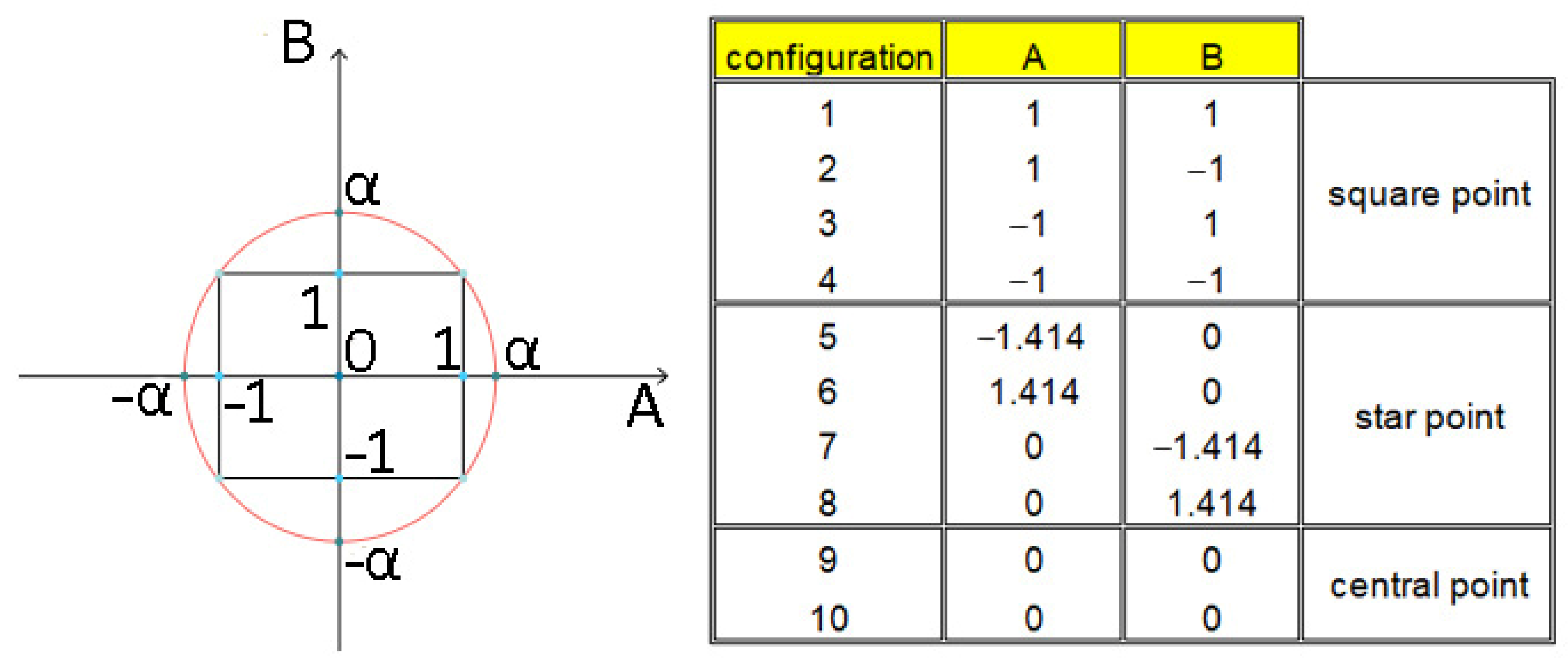
| Configuration | Lead Angle β (°) | Fiber Working Length W (mm) |
|---|---|---|
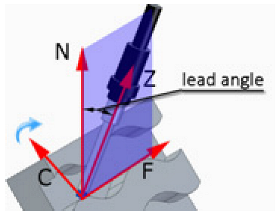 |  | |
| 1 | 15 | 8 |
| 2 | 15 | 22 |
| 3 | 29 | 8 |
| 4 | 29 | 22 |
| 5 | 12.1 | 15 |
| 6 | 31.9 | 15 |
| 7 | 22 | 5.1 |
| 8 | 22 | 24.9 |
| 9 | 22 | 15 |
| 10 | 22 | 15 |
| Sa = 0.484 ± 0.03 µm | Sa = 1.07 ± 0.09 µm |
|---|---|
After milling | Brushing (blue fiber)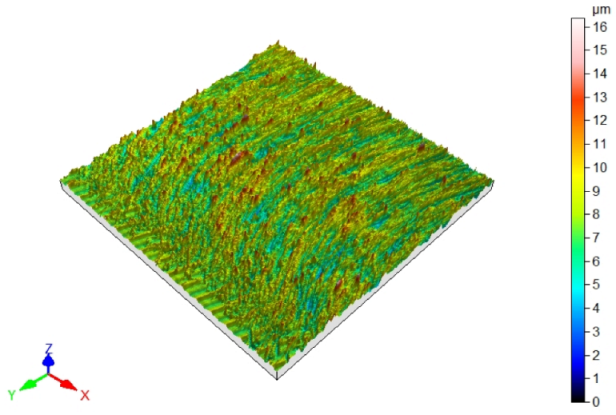 |
| Sa = 0.83 ± 0.11 µm | Sa = 0.46 ± 0.05 µm |
Brushing (white fiber)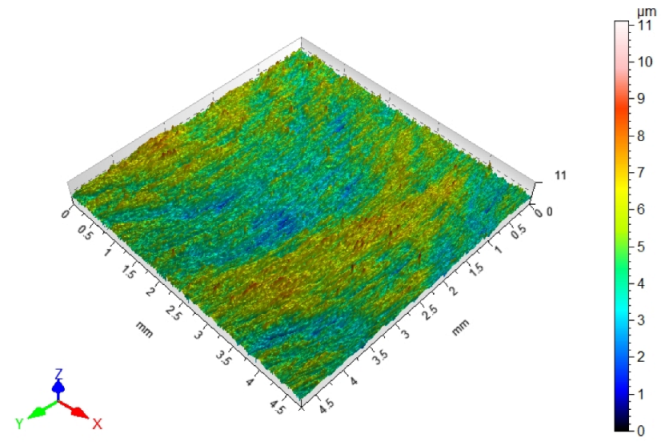 | Brushing (red fiber)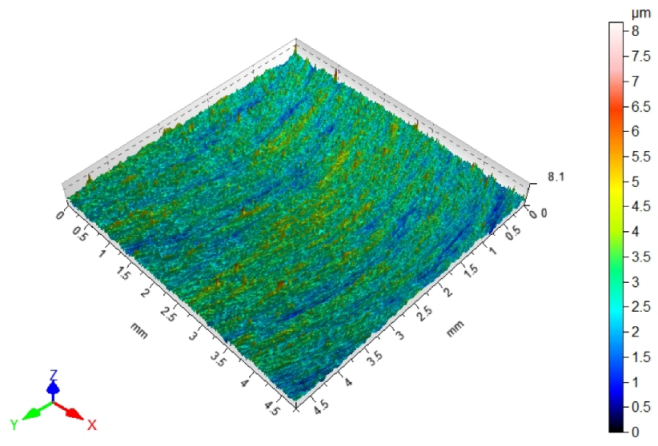 |
| Convex Edge | Concave Edge | Inflection Point |
|---|---|---|
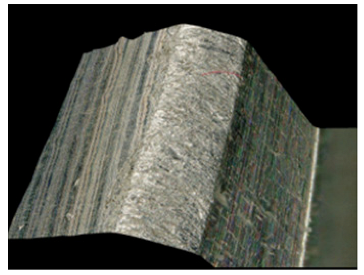 | 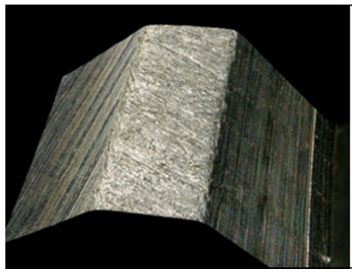 | 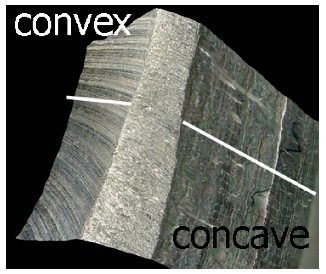 |
 | 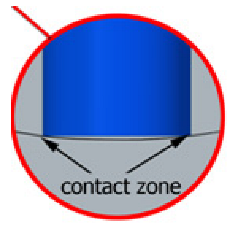 |  |
| Config. | Lead Angle β (°) | Fiber Working Length W (mm) | Edge State | ||
|---|---|---|---|---|---|
| Blue Fiber (High Stiffness) | White Fiber (Medium Stiffness) | Red Fiber Low Stiffness | |||
| 1 | 15 | 8 | chamfered | rounded | rounded |
| 2 | 15 | 22 | chamfered | rounded | rounded |
| 3 | 29 | 8 | rouned | rounded | rounded |
| 4 | 29 | 22 | rounded | rounded | rounded |
| 5 | 12.1 | 15 | chamfered | rounded | rounded |
| 6 | 31.9 | 15 | rounded | rounded | rounded |
| 7 | 22 | 5.1 | rounded | rounded | rounded |
| 8 | 22 | 24.9 | rounded | rounded | rounded |
| 9 | 22 | 15 | rounded | rounded | rounded |
| 10 | 22 | 15 | rounded | rounded | rounded |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matuszak, J.; Kawalec, A.; Gdula, M. Analysis of the Deburring Efficiency of EN-AW 7075 Aluminum Alloy Parts with Complex Geometric Shapes Considering the Tool Path Strategy During Multi-Axis Brushing. Materials 2024, 17, 6267. https://doi.org/10.3390/ma17246267
Matuszak J, Kawalec A, Gdula M. Analysis of the Deburring Efficiency of EN-AW 7075 Aluminum Alloy Parts with Complex Geometric Shapes Considering the Tool Path Strategy During Multi-Axis Brushing. Materials. 2024; 17(24):6267. https://doi.org/10.3390/ma17246267
Chicago/Turabian StyleMatuszak, Jakub, Andrzej Kawalec, and Michał Gdula. 2024. "Analysis of the Deburring Efficiency of EN-AW 7075 Aluminum Alloy Parts with Complex Geometric Shapes Considering the Tool Path Strategy During Multi-Axis Brushing" Materials 17, no. 24: 6267. https://doi.org/10.3390/ma17246267
APA StyleMatuszak, J., Kawalec, A., & Gdula, M. (2024). Analysis of the Deburring Efficiency of EN-AW 7075 Aluminum Alloy Parts with Complex Geometric Shapes Considering the Tool Path Strategy During Multi-Axis Brushing. Materials, 17(24), 6267. https://doi.org/10.3390/ma17246267








