Axial Crushing Theory and Optimization of Lattice-Filled Multicellular Square Tubes
Abstract
1. Introduction
2. Experiments on Axial Compression
2.1. Preparation of Specimen
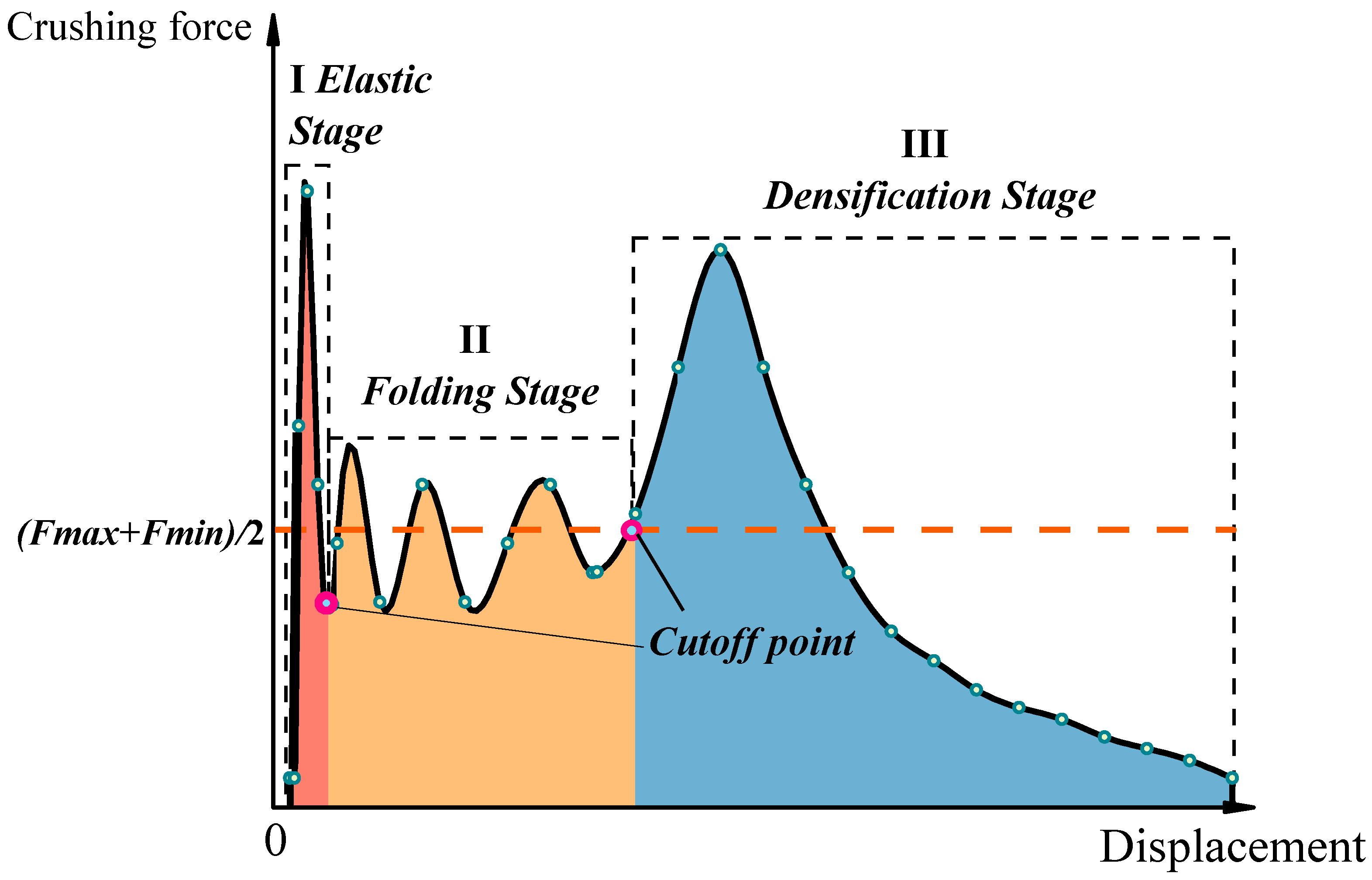
2.2. Energy Consumption Indicators
2.3. Quasi-Static Compression Tests
3. Verification of the Finite-Element Model
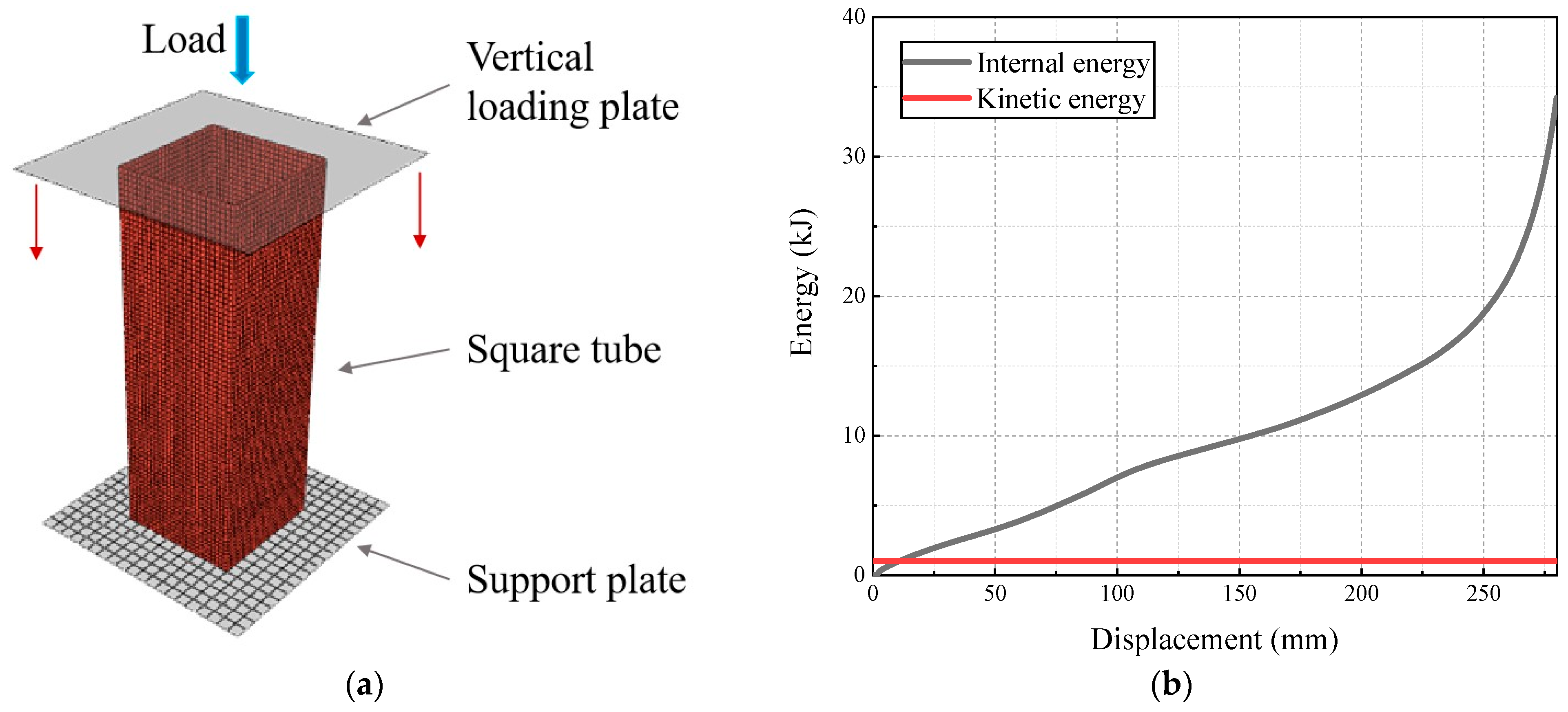
3.1. Model Parameters
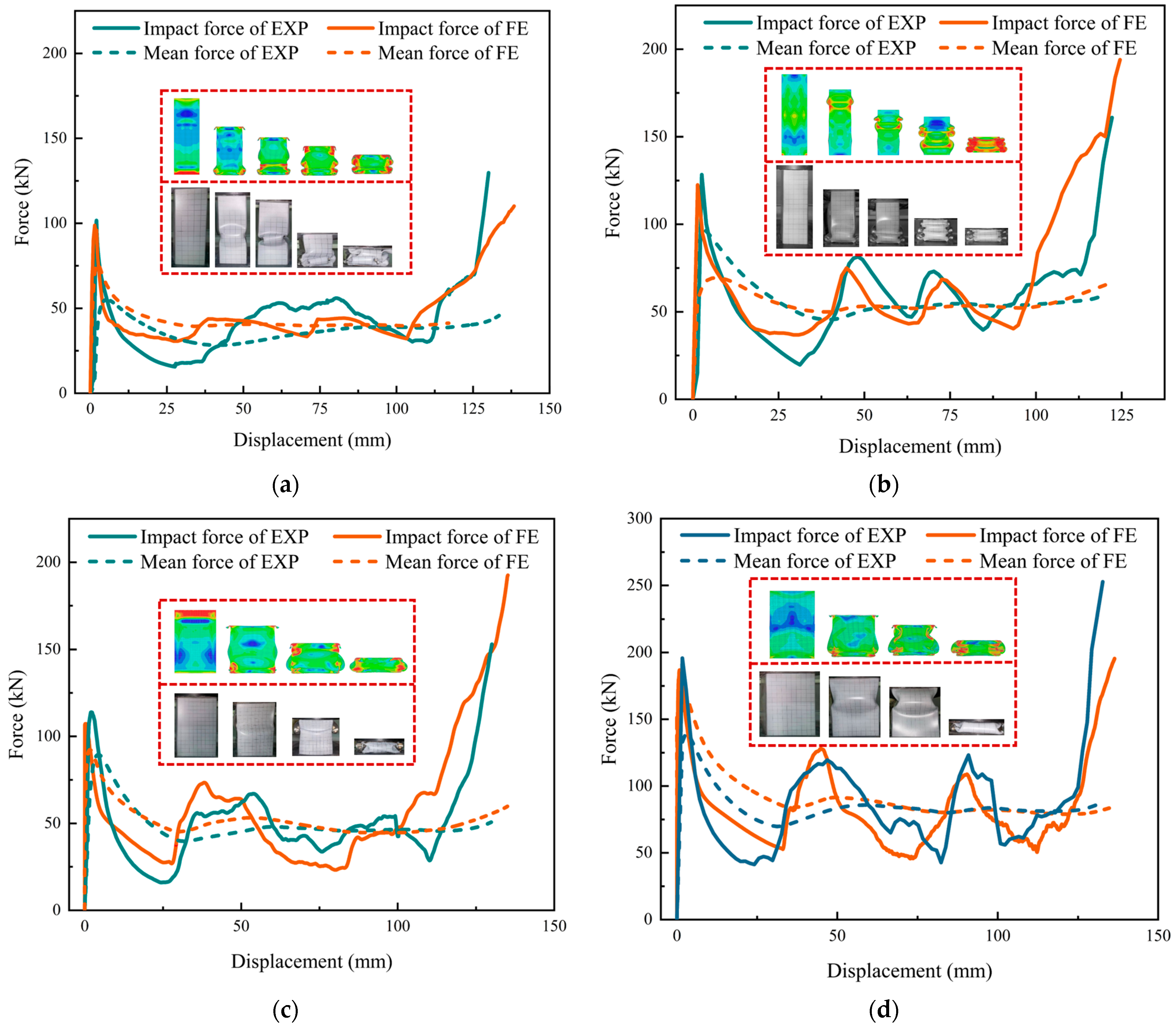
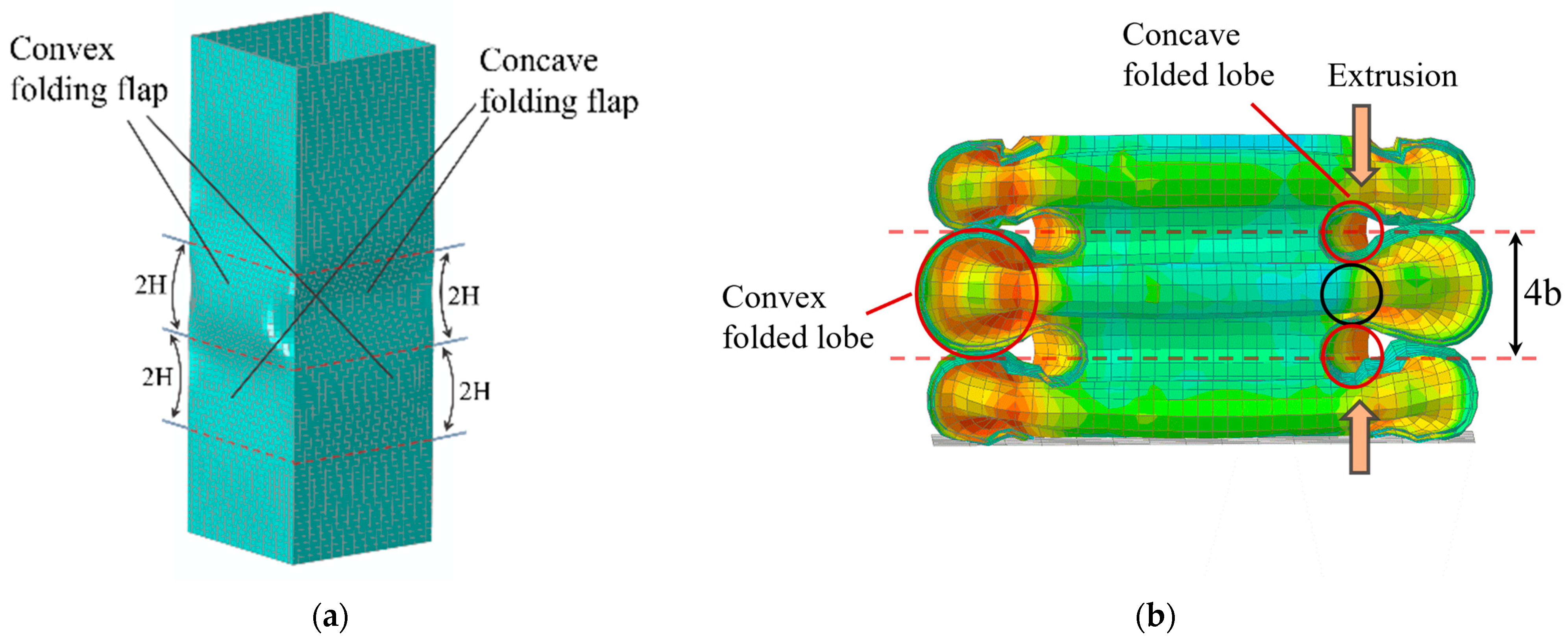
3.2. Model Validation
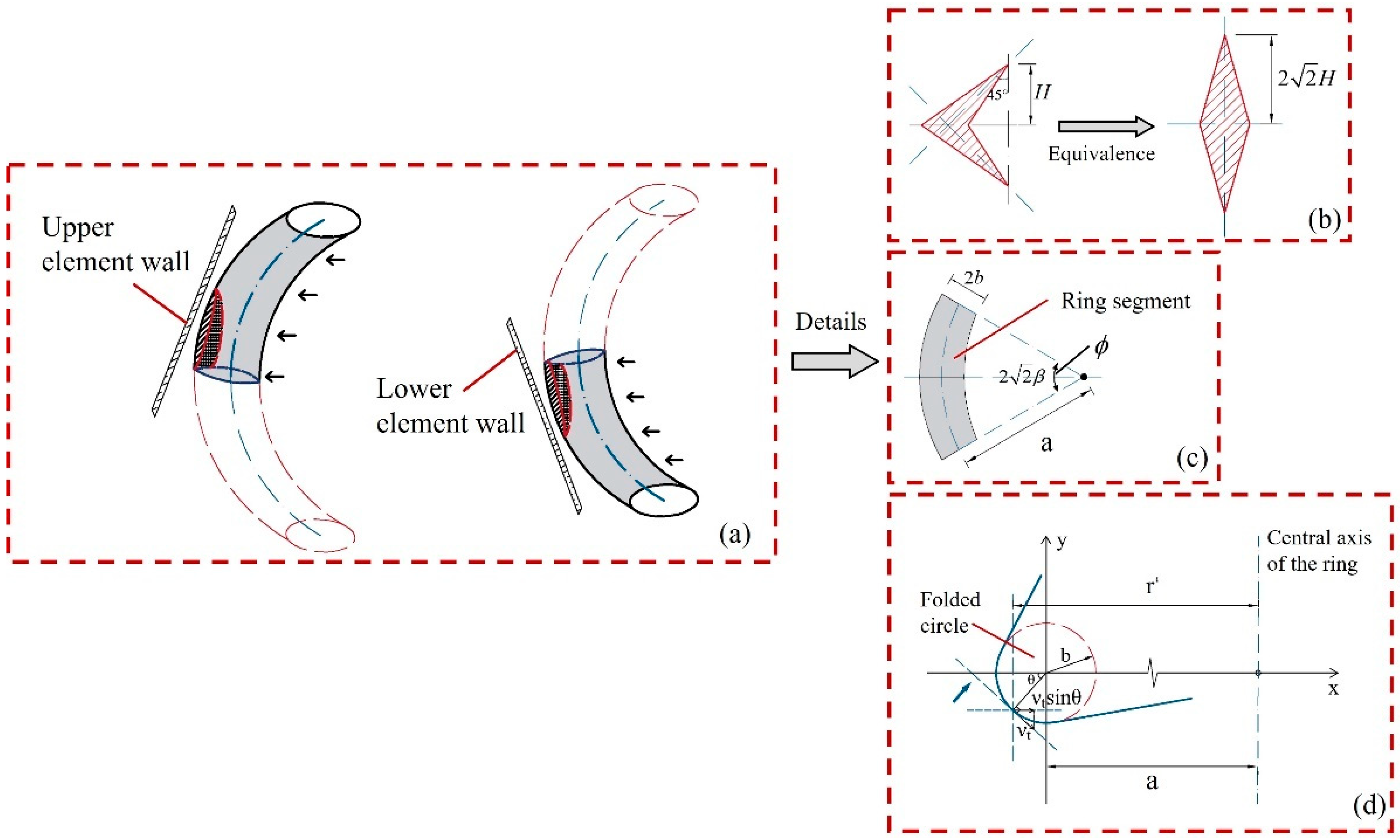
4. Improved Folding Model for Corner Elements
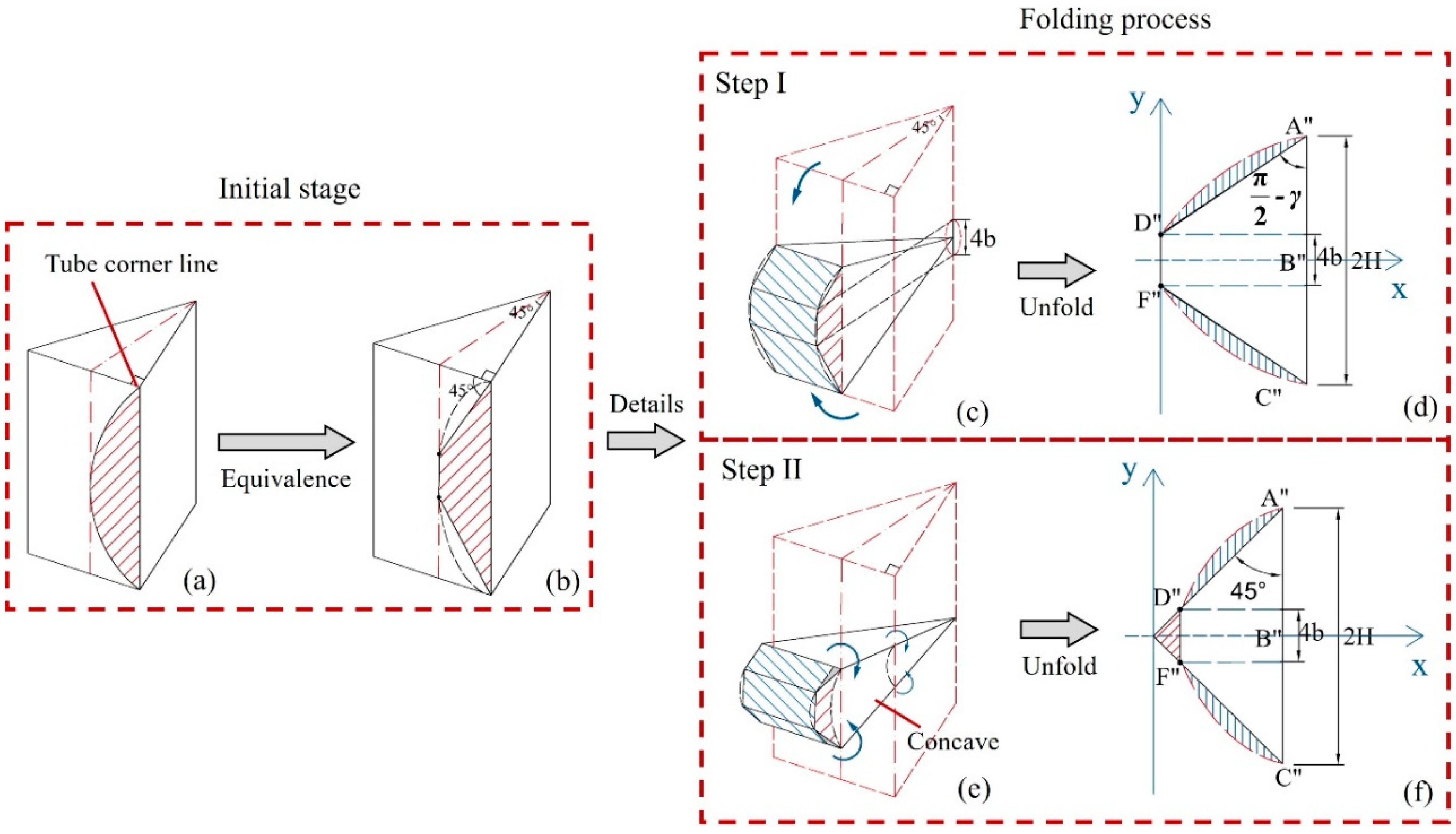
4.1. Corner Element Theory Considering Convex Panel Stretching
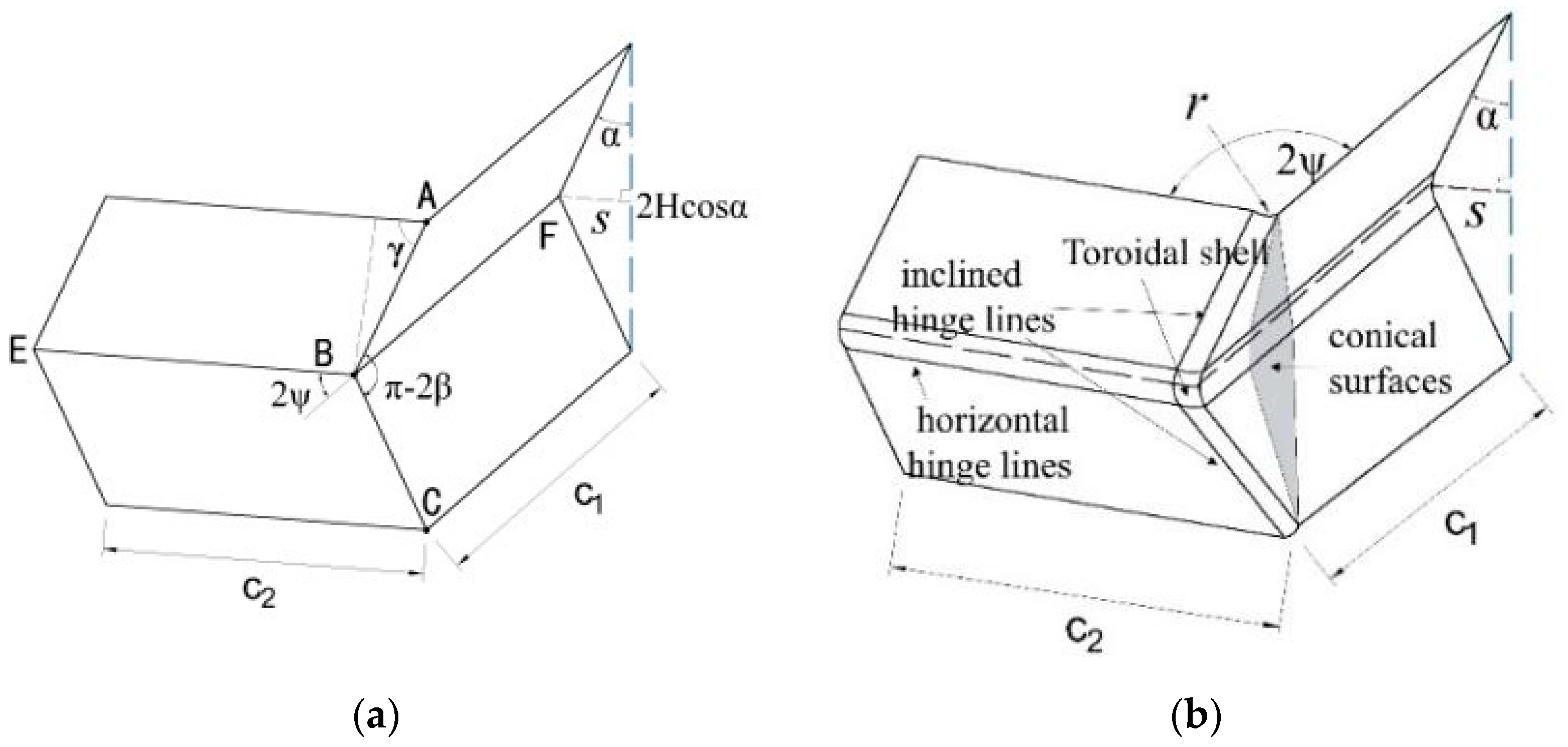
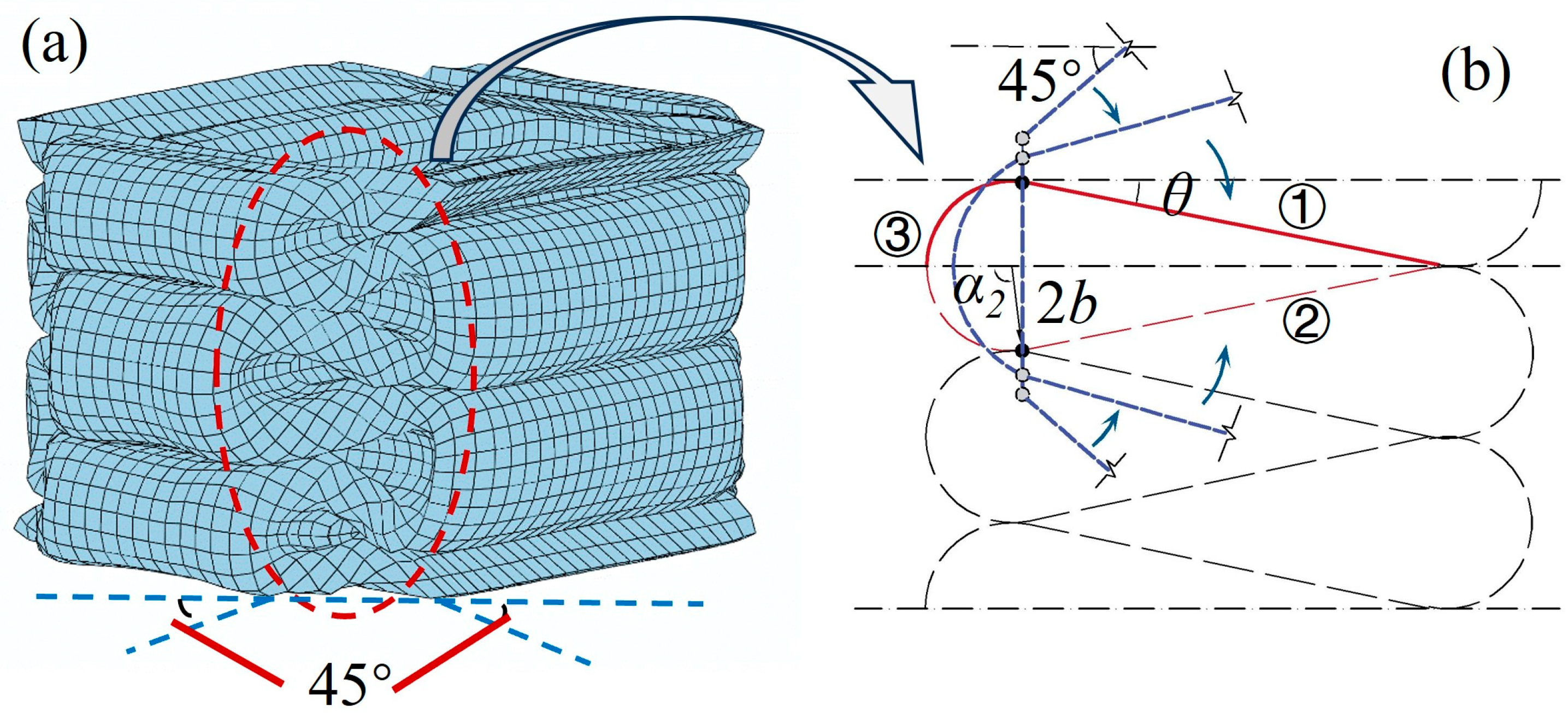
4.2. Folded-Lobe Model on Reference Plane I
4.3. Double-Hinged-Line Model on Reference Plane II
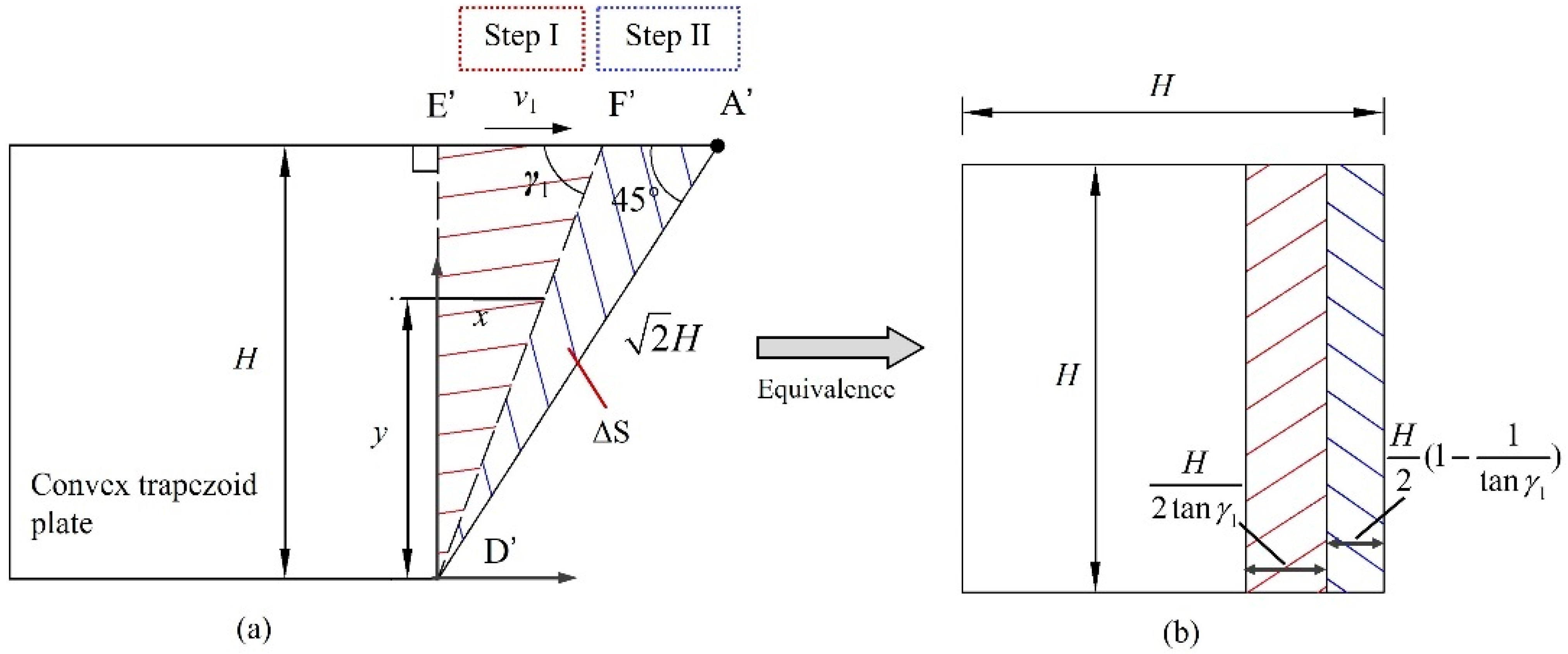
4.3.1. Stretching of Convex Panels
4.3.2. Stretching of Toroidal Shell Surface
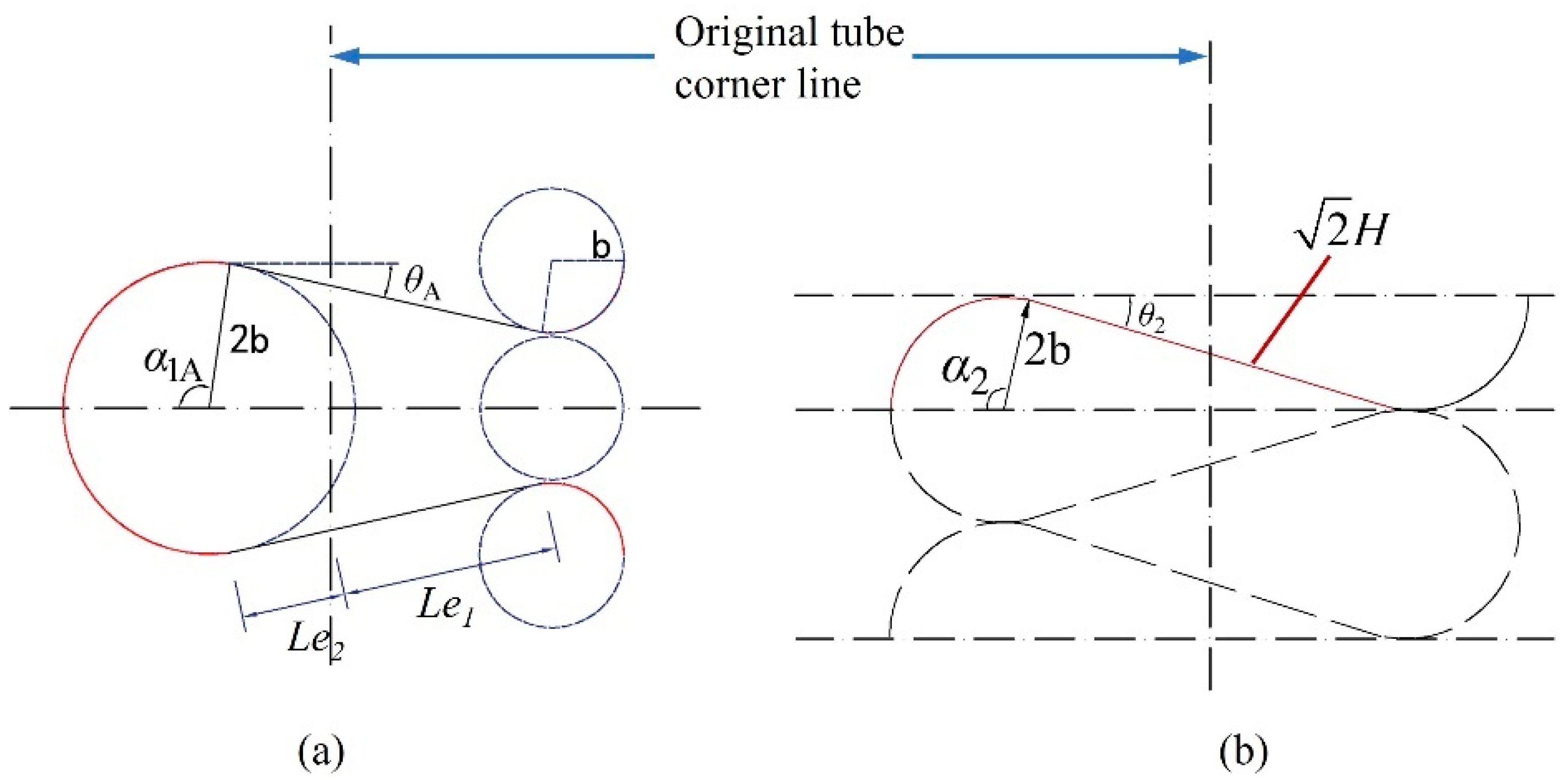
5. Formula for Folding of Square Lattice Multicellular Tubes
5.1. Calculation Model
5.2. Number of Quantitative Parameters on Areas Divided by Element Type
5.3. Integration of Element Energy Consumption
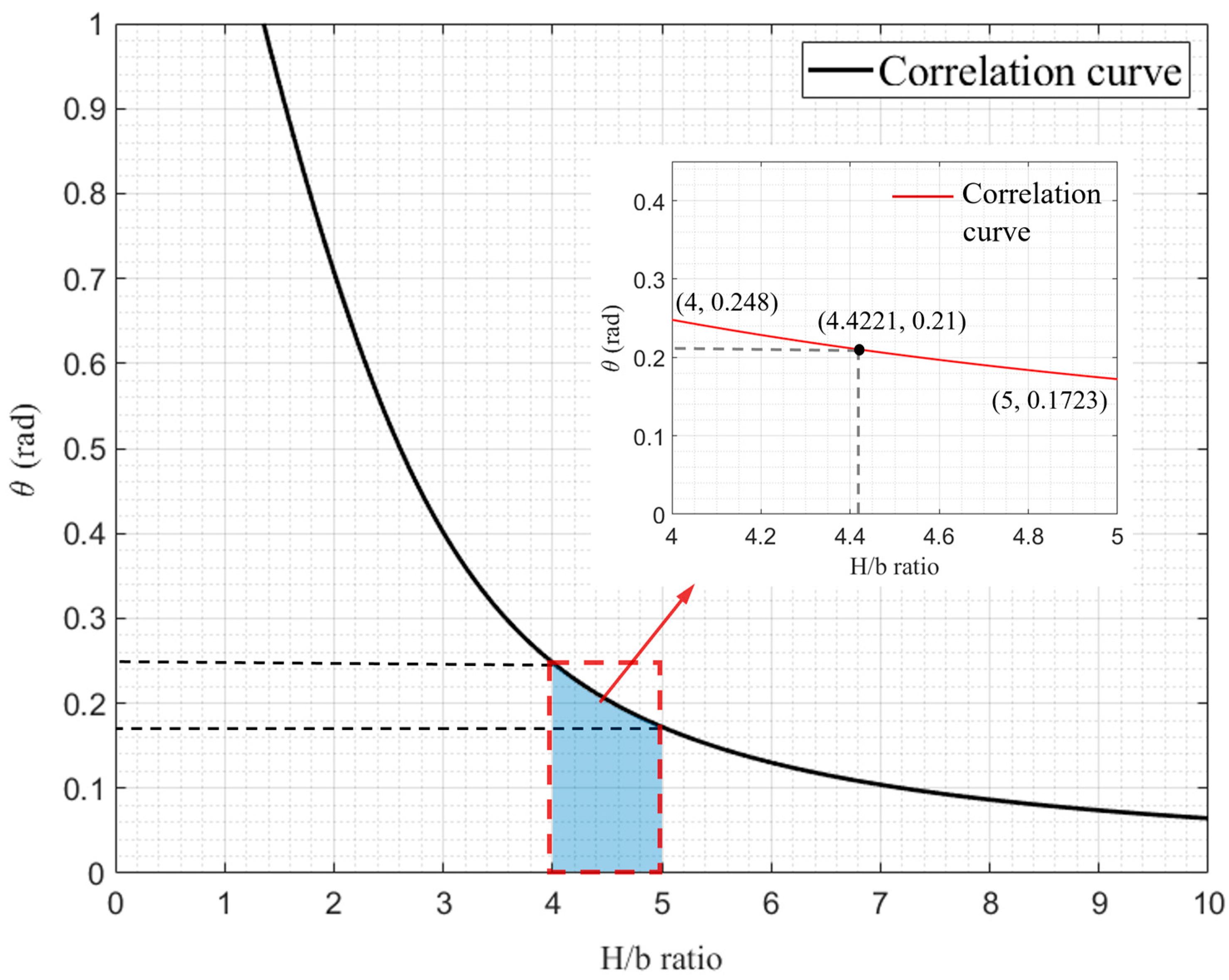
5.4. Solution of Folding Angle and Average Force
6. Formula for Peak Initial Crushing Force
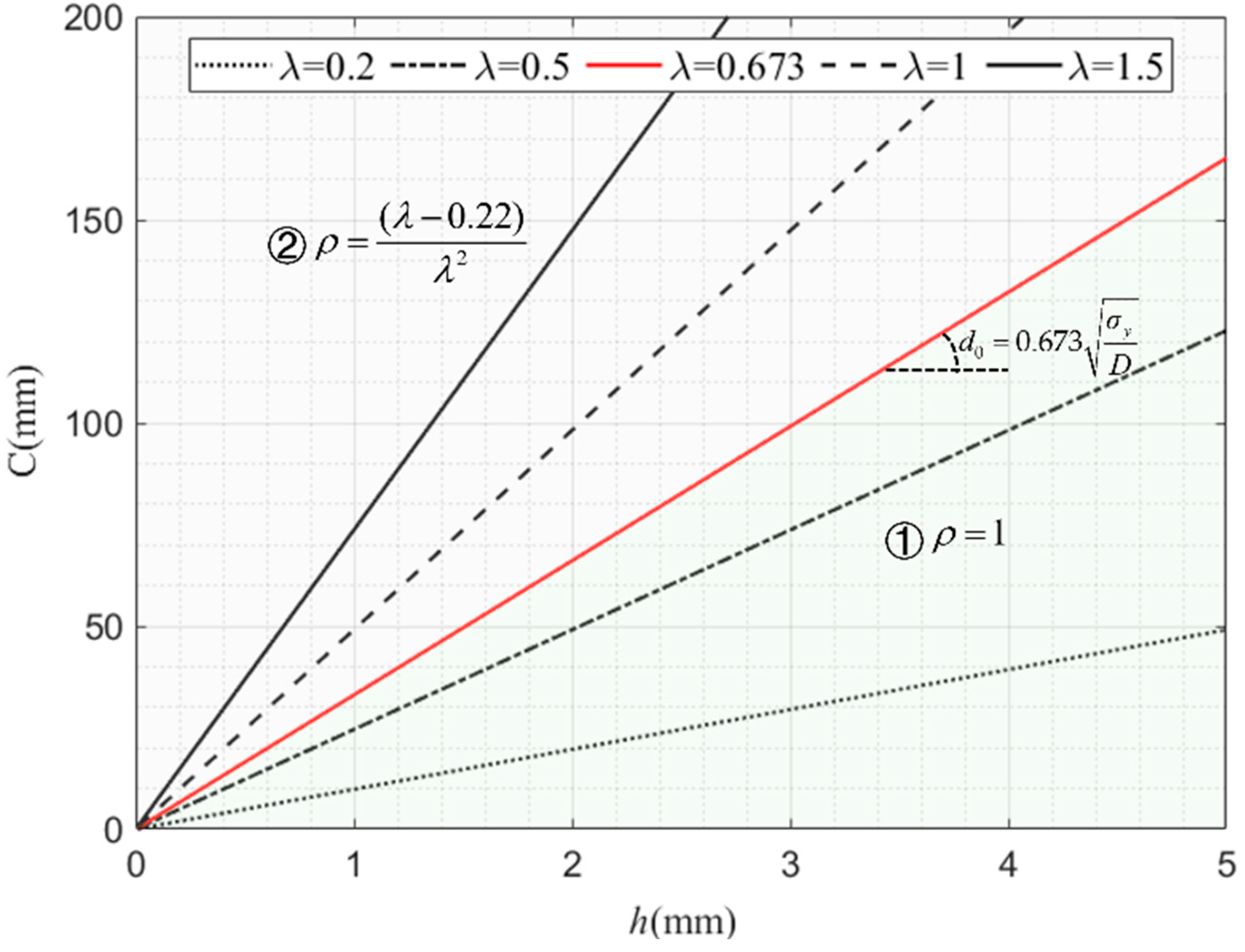
6.1. Specification of Critical Force
6.2. Critical Force in the Axial Direction of Multicellular Thin-Walled Tubes
7. Discussion
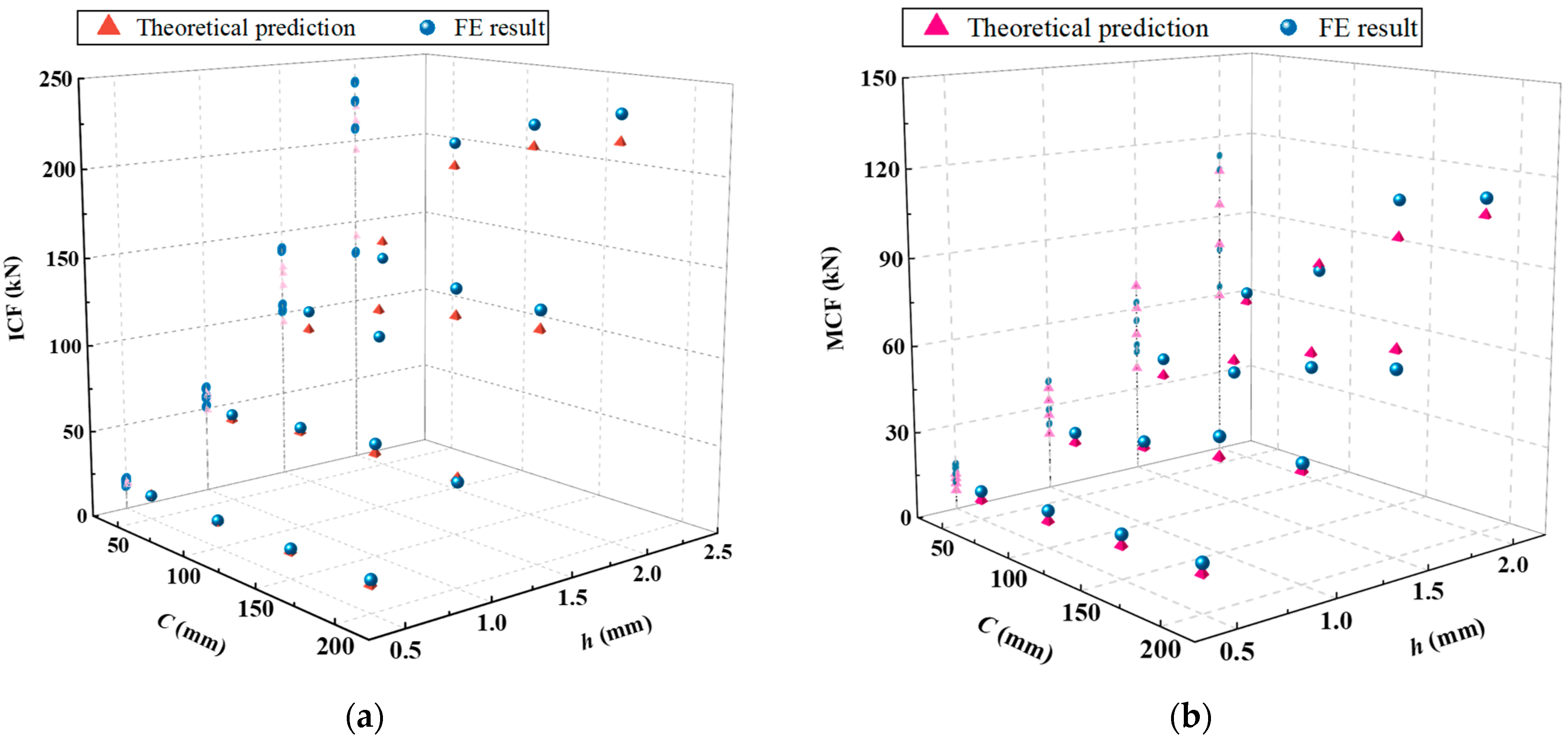
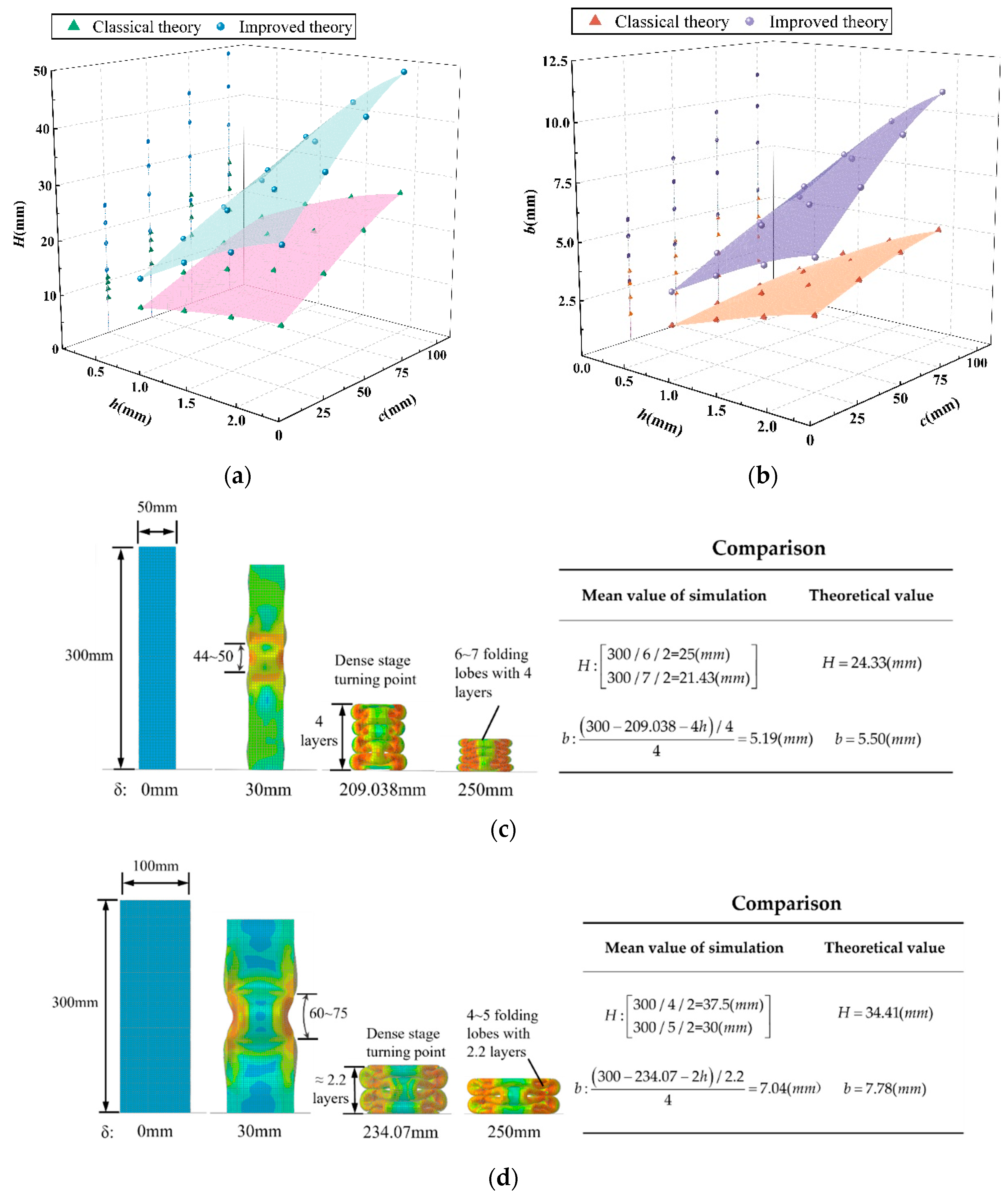
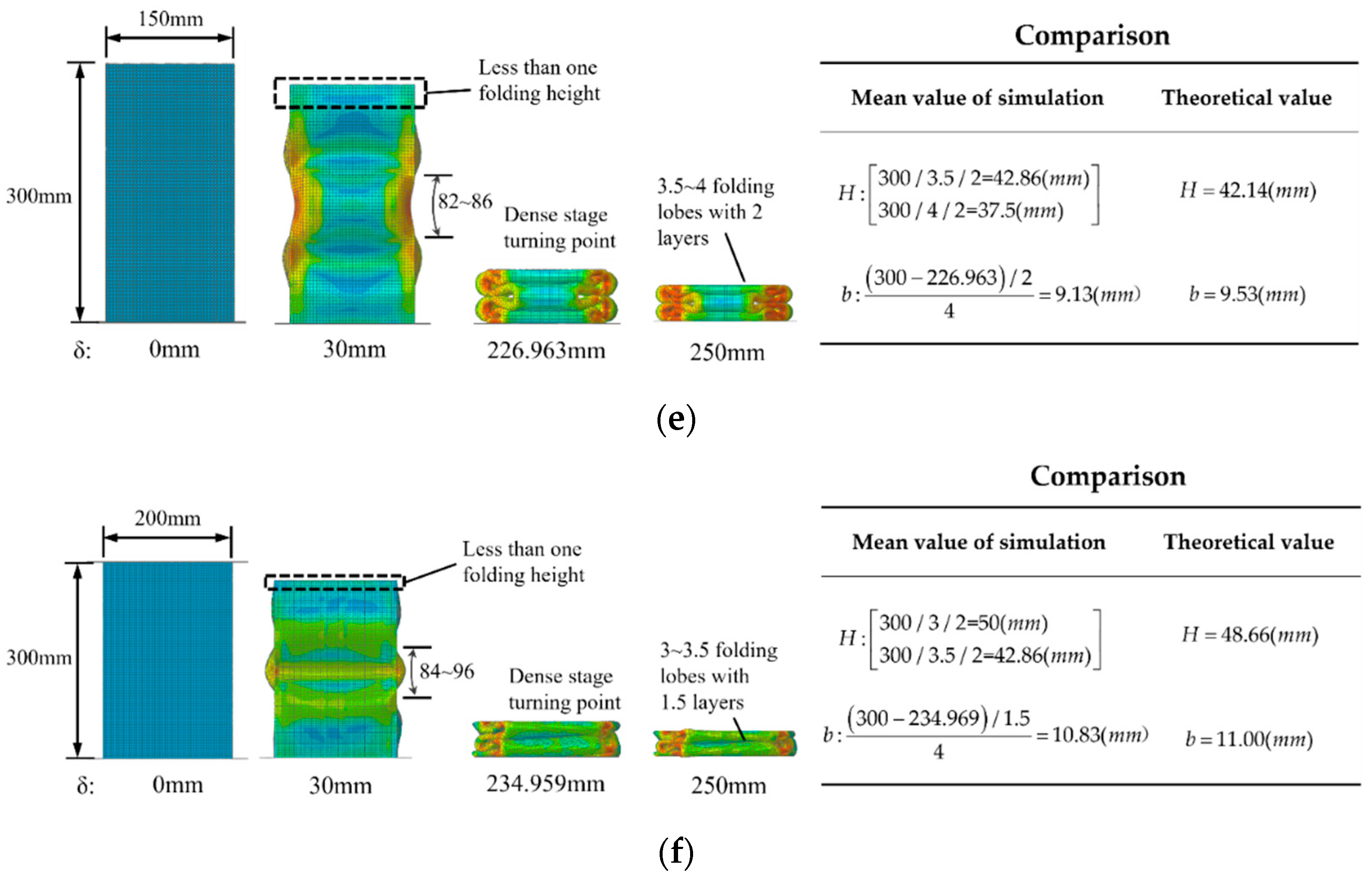
7.1. Verification of Axial Compression Theory
7.2. Optimization of Variables N and h
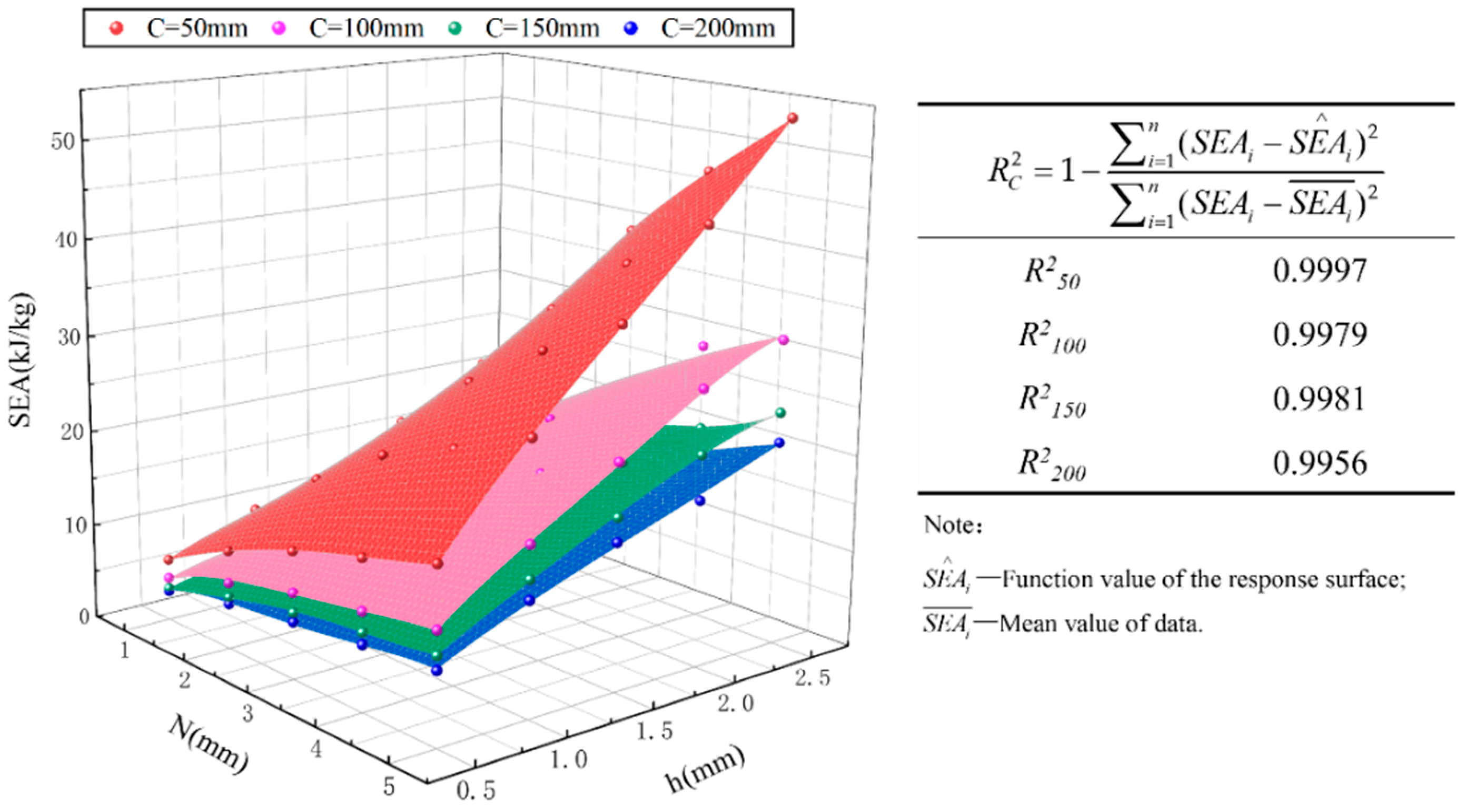
7.2.1. Construction of Response Surface
7.2.2. Pareto Front
8. Conclusions
- (1)
- A super folding element model that considers convex panel stretching is proposed based on the classic super folding element model and the extended folding element model. Meanwhile, the folded-lobe model and double-hinged-line model are introduced, and the corresponding reference planes are set. Moreover, the partition problem of the calculation of the cross section of energy consumption’s area has been solved, making the calculation of energy consumption of the tube more convenient.
- (2)
- A comparison and analysis of the critical crushing force calculation formulas in AISI and GB50018-2002 specifications is made, which takes into consideration the stability coefficient of the plate group in the effective width calculation. Meanwhile, the critical force formulas based on the influence of the width-to-thickness ratio are summarized. In the expansion of multicellular tubes, the width-to-thickness ratio of cells and the number of cells are taken as parameters so as to obtain the calculation formula for multicellular tubes.
- (3)
- Additionally, the folding element in square lattice multicellular cross-sectional tubes is divided into the following types based on the folding characteristics: L-shaped element, T-shaped element, and crisscross element. According to the differences in the folded-lobe model, the folding element is divided into Mode A and Mode B. Ultimately, the average collapse response value of multicellular tubes is calculated for the participation of five energy-consuming regions in the three element types.
- (4)
- Based on the NSGAII method, SEA and ICF are optimized by regarding N and h as variables, and the NBI method is used to obtain knee points, aiming to explore the influence of N and h on the optimized square lattice multicellular tubes under different Ctotal values. Finally, optimization directions and references are provided in this study for future research.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Garai, F.; Beres, G.; Weltsch, Z. Development of tubes filled with aluminium foams for lightweight vehicle manufacturing. Mater. Sci. Eng. A 2020, 790, 139743. [Google Scholar] [CrossRef]
- Gao, Z.; Ruan, D. Axial crushing of novel hierarchical multi-cell square tubes. Eng. Struct. 2023, 286, 116141. [Google Scholar] [CrossRef]
- Pratama, A.A.; Prabowo, A.R.; Muttaqie, T.; Muhayat, N.; Ridwan, R.; Cao, B.; Laksono, F.B. Hollow tube structures subjected to compressive loading: Implementation of the pitting corrosion effect in nonlinear FE analysis. J. Braz. Soc. Mech. Sci. 2023, 45, 143. [Google Scholar] [CrossRef]
- Patel, V.; Tiwari, G.; Dumpala, R. Review of the crushing response of collapsible tubular structures. Front. Mech. Eng. 2020, 15, 438–474. [Google Scholar] [CrossRef]
- Xie, S.; Yang, W.; Li, H.; Wang, N. Impact characteristics and crashworthiness of multi-cell, square, thin-walled, structures under axial loads. Int. J. Crashworth. 2017, 22, 503–517. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Abramowicz, W. On the crushing mechanics of thin-walled structures. J. Appl. Mech. 1983, 50, 727–734. [Google Scholar] [CrossRef]
- Malekshahi, A.; Shirazi, K.; Shishehsaz, M. Axial crushing of prismatic multi-corner metal columns considering plastic hardening and curvature. J. Mech. 2019, 35, 315–326. [Google Scholar] [CrossRef]
- Trongnhan, T.; Hou, S.; Han, X.; Chau, M. Crushing analysis and numerical optimization of angle element structures under axial impact loading. Compos. Struct. 2015, 119, 422–435. [Google Scholar]
- Singace, A.A. Axial crushing analysis of tubes deforming in the multi-lobe mode. Int. J. Mech. Sci. 1999, 41, 865–890. [Google Scholar] [CrossRef]
- Wierzbicki, T. Crushing analysis of metal honeycombs. Int. J. Impact. Eng. 1983, 1, 157–174. [Google Scholar] [CrossRef]
- Mahmoudabadi, M.Z.; Sadighi, M. A theoretical and experimental study on metal hexagonal honeycomb crushing under quasi-static and low velocity impact loading. Mater. Sci. Eng. A 2011, 528, 4958–4966. [Google Scholar] [CrossRef]
- Yao, S.; Tan, B.; Xu, P.; Che, Q. A three-hinge-line method for calculating the deformation process of thin plates under quasi-static axial loading. Int. J. Crashworth. 2020, 25, 164–174. [Google Scholar] [CrossRef]
- Abramowicz, W.; Jones, N. Dynamic progressive buckling of circular and square tubes. Int. J. Impact. Eng. 1986, 4, 243–270. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Bhat, S.U.; Abramowicz, W.; Brodkin, D. Alexander revisited—A two folding elements model of progressive crushing of tubes. Int. J. Solids Struct. 1992, 29, 3269–3288. [Google Scholar] [CrossRef]
- Abramowicz, W. The macro element approach in crash calculations. Crashworth. Transp. Syst. Struct. Impact Occupant Prot. 1997, 332, 291–320. [Google Scholar]
- Abramowicz, W. Thin-walled structures as impact energy absorbers. Thin-Walled Struct. 2003, 41, 91–107. [Google Scholar] [CrossRef]
- Ha, N.S.; Lu, G. Thin-walled corrugated structures: A review of crashworthiness designs and energy absorption characteristics. Thin Wall Struct. 2020, 157, 106995. [Google Scholar] [CrossRef]
- Winter, G. Commentary on the 1968 Edition of the Specification for the Design of Cold-Formed Steel Structural Members; AISI: New York, NY, USA, 1970; p. 104. [Google Scholar]
- Schager, B.W. Progress on the direct strength method. In Proceedings of the CCFSS Proceedings of International Specialty Conference on Cold-Formed Steel Structures, Orlando, FL, USA, 17–18 October 2002; University of Missouri: Rolla, MI, USA. [Google Scholar]
- Trongnhan, T.; Hou, S.; Han, X.; Nguyen, N.; Chau, M. Theoretical prediction and crashworthiness optimization of multi-cell square tubes under oblique impact loading. Int. J. Mech. Sci. 2014, 89, 177–193. [Google Scholar]
- Zhang, X.; Cheng, G.; Zhang, H. Theoretical prediction and numerical simulation of multi-cell square thin-walled structures. Thin Wall Struct. 2006, 44, 1185–1191. [Google Scholar] [CrossRef]
- Wang, Z.G.; Liu, J.F.; Yao, S. On folding mechanics of multi-cell thin-walled square tubes. Compos. B Eng. 2018, 132, 17–27. [Google Scholar] [CrossRef]
- Quach, W.; Teng, J.G.; Chung, K.F. Three-stage full-range stress-strain model for stainless steels. J. Struct. Eng. 2008, 134, 1518–1527. [Google Scholar] [CrossRef]
- Ramberg, W.; Osgood, W.R. Description of Stress-Strain Curves by Three Parameters; No. NACA-TN-902; NASA: Washington, DC, USA, 1943. [Google Scholar]
- ASTM E8/E8M–09; Standard Test Methods for Tension Testing of Metallic Materials. ASTM International: West Conshohocken, PA, USA, 2009.
- Mingzhu, J.; Xiuhui, H.; Guansheng, Y.; Guansheng, Y.; Jianguo, G.; Zichen, D. Improving the crashworthiness of bio-inspired multi-cell thin-walled tubes under axial loading: Experimental, numerical, and theoretical studies. Thin Wall Struct. 2022, 177, 109415. [Google Scholar]
- Abramowicz, W.; Jones, N. Dynamic axial crushing of square tubes. Int. J. Impact. Eng. 1984, 2, 179–208. [Google Scholar] [CrossRef]
- Malekshahi, A.; Shirazi, K.H.; Shishesaz, M.; Hosseini, M. A novel theoretical approach for estimation of axial crushing behavior of polygonal hollow sections. J. Appl. Mech. 2020, 12, 2050008. [Google Scholar] [CrossRef]
- Zhou, J.; Qin, R.; Chen, B. Energy absorption properties of multi-cell thin-walled tubes with a double surface gradient. Thin Wall Struct. 2019, 145, 106386. [Google Scholar] [CrossRef]
- Ye, L.; Lu, G.X. Axial crushing of thin walled cylindrical shell with foam core. Mater. Res. Innov. 2011, 15, S3–S6. [Google Scholar] [CrossRef]
- AISI S100-16; North American Specification for the Design of Cold-Formed Steel Structural Members. American Iron & Steel Institute, Committee of Steel Plate Producers & Committee of Stainless Steel Producers: New York, NY, USA, 2007.
- GB50018-2002; Technical Code of Cold-Formed Thin-Wall Steel Structures. Ministry of Construction of the People’s Republic of China. China Planning Press: Beijing, China, 2002.
- Yao, R.; Pang, T.; He, S.; Li, Q.; Zhang, B.; Sun, G. A bio-inspired foam-filled multi-cell structural configuration for energy absorption. Compos. B Eng. 2022, 238, 109801. [Google Scholar] [CrossRef]
- Jin, M.Z.; Yin, G.S.; Hao, W.Q.; Tuo, H.L.; Yao, R.Y. Energy absorption characteristics of multi-cell tubes with different cross-sectional shapes under quasi-static axial crushing. Int. J. Crashworth. 2022, 27, 565–580. [Google Scholar] [CrossRef]
- Hou, S.; Li, Q.; Long, S.; Yang, X.; Li, W. Multiobjective optimization of multi-cell sections for the crashworthiness design. Int. J. Impact. Eng. 2008, 35, 1355–1367. [Google Scholar] [CrossRef]
- Xiang, Y.; Yu, T.; Yang, L. Comparative analysis of energy absorption capacity of polygonal tubes, multi-cell tubes and honeycombs by utilizing key performance indicators. Mater. Des. 2016, 89, 689–696. [Google Scholar] [CrossRef]
- Usta, F.; Eren, Z.; Kurtaran, H.; Türkmen, H.S.; Kazancı, Z.; Mecitoglu, Z. Crashworthiness Optimization of Nested and Concentric Circular Tubes Using Response Surface Methodology and Genetic Algorithm. Lat. Am. J. Solids Struct. 2018, 15. [Google Scholar] [CrossRef]
- Khalkhali, A.; Mostafapour, M.; Tabatabaie, S.M.; Ansari, B. Multi-objective crashworthiness optimization of perforated square tubes using modified NSGAII and MOPSO. Struct. Multidiscipl. Optim. 2016, 54, 45–61. [Google Scholar] [CrossRef]
- Rad, M.A.; Khalkhali, A. Crashworthiness multi-objective optimization of the thin-walled tubes under probabilistic 3D oblique load. Mater. Des. 2018, 156, 538–557. [Google Scholar]
- Das, I. On characterizing the “knee” of the Pareto curve based on normal-boundary intersection. Struct. Multidiscipl. Optim. 1999, 18, 107–115. [Google Scholar] [CrossRef]
- Tang, J.; Wang, H.; Xiong, L. Surrogate-assisted multi-objective optimization via knee-oriented Pareto front estimation. Swarm Evol. Comput. 2023, 77, 101252. [Google Scholar] [CrossRef]








| Material | Young’s Modulus E0 (MPa) | Yield Strength σ0.2 (MPa) | Ultimate Strength σu (MPa) | Hardening Coefficient n | Poisson’s Ratio ν | Density (kg/mm3) |
|---|---|---|---|---|---|---|
| 304 Austenitic Stainless Steel | 210,000 | 315 | 632.58 | 6 | 0.3 | 7.93 × 10−6 |
| Specimen Number | Dimensions of Square Tube cross Section (mm × mm) | Thickness of Tube Surface (mm) | ICF (kN) | MCF (kN) | ||||
|---|---|---|---|---|---|---|---|---|
| Value of the Experiment | FE Analysis | Error | Value of the Experiment | FE Analysis | Error | |||
| T1 | 50 × 50 | 1.5 | 101.65 | 96.01 | 5.54% | 40.22 | 39.86 | 0.90% |
| T2 | 50 × 50 | 2 | 128.32 | 122.49 | 4.54% | 54.58 | 52.74 | 3.37% |
| T3 | 100 × 100 | 1.5 | 113.89 | 107.30 | 5.83% | 46.16 | 44.61 | 3.35% |
| T4 | 100 × 100 | 2 | 195.79 | 186.87 | 4.56% | 81.60 | 80.53 | 1.31% |
| Energy Consumption Zone of Folding Element | Reference Plane I | Reference Plane II |
|---|---|---|
| Area of inclined traveling hinge | √ | — |
| Area of horizontal fixed hinge | √ | — |
| Area of conical surface stretching | √ | — |
| Area of convex panel stretching | — | √ |
| Area of the toroidal shell surface stretching | — | √ |
| Type | Number of Zones | ||||
|---|---|---|---|---|---|
| Horizontal Fixed Hinge Line mH | Oblique Traveling Hinge Line mL | Stretch Zone of Conical Surface mC | Stretch Zone of Convex Panel mP | Toroidal Shell Surface mS | |
| L-shaped element | 2 | 2 | 1 | 1 | 1 |
| T-shaped element | 3 | 2 | 2 | 1 | 2 |
| Cross-shaped element | 4 | 4 | 2 | 2 | 2 |
| Type | Folded-Lobe Model | Double-Hinged-Line Model | |
|---|---|---|---|
| Type A | Type B | ||
| L-shaped element | 8 | 0 | 4 |
| T-shaped element | |||
| Crisscross-shaped element | 0 | ||
| Number | Dimensions of Square Tube cross Section (mm × mm) | Thickness of Tube Surface (mm) | ICF (kN) | MCF (kN) | Theoretical Calculation of H (mm) | Theoretical Calculation of b (mm) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Theory | FE | Diff (%) | Theory | FE | Diff (%) | |||||
| S1-1 | 50 × 50 | 0.5 | 13.77 | 13.81 | −0.11 | 6.70 | 9.98 | −32.87 | 12.16 | 2.75 |
| S1-2 | 50 × 50 | 1.0 | 48.50 | 51.55 | −7.72 | 19.87 | 23.54 | −15.59 | 17.20 | 3.89 |
| S1-3 | 50 × 50 | 1.5 | 94.33 | 105.09 | −10.24 | 37.79 | 44.83 | −15.70 | 21.07 | 4.76 |
| S1-4 | 50 × 50 | 2.0 | 126.54 | 130.64 | −3.14 | 59.84 | 66.62 | −10.18 | 24.33 | 5.50 |
| S2-1 | 100 × 100 | 0.5 | 14.59 | 15.48 | −5.75 | 9.16 | 12.52 | −26.84 | 17.20 | 3.89 |
| S2-2 | 100 × 100 | 1.0 | 55.08 | 57.63 | −4.43 | 26.81 | 29.64 | −9.55 | 24.33 | 5.50 |
| S2-3 | 100 × 100 | 1.5 | 116.53 | 101.4 | 14.92 | 50.54 | 46.48 | 8.73 | 29.80 | 6.74 |
| S2-4 | 100 × 100 | 2.0 | 194.01 | 207.7 | −6.59 | 79.48 | 75.54 | 5.22 | 34.41 | 7.78 |
| S3-1 | 150 × 150 | 0.5 | 14.87 | 16.59 | −10.37 | 11.04 | 15.12 | −26.98 | 21.07 | 4.76 |
| S3-2 | 150 × 150 | 1.0 | 57.27 | 62.67 | −8.62 | 32.14 | 38.45 | −16.41 | 29.80 | 6.74 |
| S3-3 | 150 × 150 | 1.5 | 123.93 | 139.64 | −11.25 | 60.32 | 55.61 | 8.47 | 36.49 | 8.25 |
| S3-4 | 150 × 150 | 2.0 | 211.55 | 224.33 | −5.70 | 94.54 | 110.61 | −14.53 | 42.14 | 9.53 |
| S4-1 | 200 × 200 | 0.5 | 15.00 | 17.84 | −15.92 | 12.63 | 16.21 | −22.09 | 24.33 | 5.50 |
| S4-2 | 200 × 200 | 1.0 | 58.37 | 56.98 | 2.44 | 36.63 | 39.59 | −7.48 | 34.41 | 7.78 |
| S4-3 | 200 × 200 | 1.5 | 127.63 | 138.51 | −7.86 | 68.57 | 61.66 | 11.21 | 42.14 | 9.53 |
| S4-4 | 200 × 200 | 2.0 | 220.32 | 235.97 | −6.63 | 107.24 | 112.44 | −4.62 | 48.66 | 11.00 |
| Number of Cells N × N | h (mm) | Section Width Ctotal (mm) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 50 | 100 | 150 | 200 | ||||||
| δe (mm) | SEA (kJ/kg) | δe (mm) | SEA (kJ/kg) | δe (mm) | SEA (kJ/kg) | δe (mm) | SEA (kJ/kg) | ||
| 1 × 1 | 0.5 | 227.98 | 6.49 | 233.76 | 4.52 | 219.77 | 3.41 | 230.98 | 3.07 |
| 2 × 2 | 0.5 | 219.46 | 10.28 | 223.16 | 6.92 | 220.69 | 5.43 | 224.46 | 4.70 |
| 3 × 3 | 0.5 | 219.65 | 13.12 | 227.56 | 8.88 | 223.94 | 6.89 | 225.65 | 5.89 |
| 4 × 4 | 0.5 | 216.92 | 15.33 | 220.22 | 10.07 | 223.63 | 8.02 | 223.92 | 6.79 |
| 5 × 5 | 0.5 | 218.63 | 17.62 | 220.17 | 11.38 | 222.03 | 8.97 | 221.21 | 7.53 |
| 1 × 1 | 1 | 229.76 | 9.79 | 227.98 | 6.49 | 213.14 | 4.83 | 233.76 | 4.52 |
| 2 × 2 | 1 | 216.16 | 15.69 | 219.46 | 10.28 | 222.88 | 8.18 | 234.14 | 7.27 |
| 3 × 3 | 1 | 219.56 | 20.72 | 219.65 | 13.12 | 220.24 | 10.23 | 227.56 | 8.88 |
| 4 × 4 | 1 | 210.22 | 23.80 | 216.92 | 15.33 | 215.48 | 11.76 | 220.22 | 10.07 |
| 5 × 5 | 1 | 209.17 | 27.34 | 213.21 | 17.18 | 222.97 | 13.80 | 227.43 | 11.75 |
| 1 × 1 | 1.5 | 211.77 | 11.56 | 210.14 | 7.55 | 227.98 | 6.49 | 217.77 | 5.27 |
| 2 × 2 | 1.5 | 208.69 | 19.91 | 218.88 | 13.20 | 227.51 | 10.66 | 217.69 | 8.57 |
| 3 × 3 | 1.5 | 209.94 | 26.41 | 215.24 | 16.72 | 219.65 | 13.12 | 231.81 | 11.57 |
| 4 × 4 | 1.5 | 207.63 | 31.71 | 209.48 | 19.40 | 210.99 | 14.91 | 219.63 | 12.91 |
| 5 × 5 | 1.5 | 204.03 | 36.35 | 216.97 | 23.07 | 218.63 | 17.62 | 226.69 | 15.13 |
| 1 × 1 | 2 | 221.98 | 14.54 | 229.76 | 9.79 | 215.77 | 7.24 | 203.97 | 5.80 |
| 2 × 2 | 2 | 209.46 | 24.49 | 228.14 | 16.56 | 214.69 | 12.02 | 235.56 | 11.03 |
| 3 × 3 | 2 | 207.65 | 32.42 | 219.56 | 20.72 | 228.81 | 16.45 | 219.65 | 13.12 |
| 4 × 4 | 2 | 202.92 | 38.87 | 210.22 | 23.80 | 215.63 | 18.44 | 228.79 | 16.17 |
| 5 × 5 | 2 | 197.21 | 44.48 | 217.43 | 28.42 | 222.69 | 21.81 | 213.21 | 17.18 |
| 1 × 1 | 2.5 | 211.19 | 16.01 | 220.41 | 10.75 | 204.77 | 7.83 | 245.79 | 7.95 |
| 2 × 2 | 2.5 | 216.90 | 29.91 | 218.08 | 18.36 | 235.36 | 15.18 | 226.90 | 12.20 |
| 3 × 3 | 2.5 | 211.32 | 39.35 | 207.95 | 22.93 | 218.82 | 18.24 | 208.58 | 14.38 |
| 4 × 4 | 2.5 | 203.80 | 47.02 | 217.59 | 28.97 | 203.56 | 20.27 | 218.80 | 17.91 |
| 5 × 5 | 2.5 | 195.74 | 53.67 | 205.04 | 31.69 | 211.45 | 24.22 | 225.64 | 21.14 |
| Section Width Ctotal | Optimal Parameters Based on PF Knee Points | SEA (kJ/kg) | ICF (kN) | CFE | |
|---|---|---|---|---|---|
| N | h (mm) | ||||
| 50 | 4 | 0.56 | 19.94 | 115.29 | 42.75% |
| 100 | 4 | 0.57 | 10.95 | 151.48 | 44.70% |
| 150 | 5 | 0.5 | 9.01 | 190.21 | 45.00% |
| 200 | 3 | 0.96 | 8.59 | 288.85 | 43.10% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; Liu, J.; Rong, W.; Wu, B. Axial Crushing Theory and Optimization of Lattice-Filled Multicellular Square Tubes. Materials 2024, 17, 1245. https://doi.org/10.3390/ma17061245
Zhou X, Liu J, Rong W, Wu B. Axial Crushing Theory and Optimization of Lattice-Filled Multicellular Square Tubes. Materials. 2024; 17(6):1245. https://doi.org/10.3390/ma17061245
Chicago/Turabian StyleZhou, Xiwu, Jingdong Liu, Weifeng Rong, and Benying Wu. 2024. "Axial Crushing Theory and Optimization of Lattice-Filled Multicellular Square Tubes" Materials 17, no. 6: 1245. https://doi.org/10.3390/ma17061245
APA StyleZhou, X., Liu, J., Rong, W., & Wu, B. (2024). Axial Crushing Theory and Optimization of Lattice-Filled Multicellular Square Tubes. Materials, 17(6), 1245. https://doi.org/10.3390/ma17061245





