Rotating Sonotrode Design for Ultrasonic-Assisted Arc Welding of Metal Materials
Abstract
1. Introduction
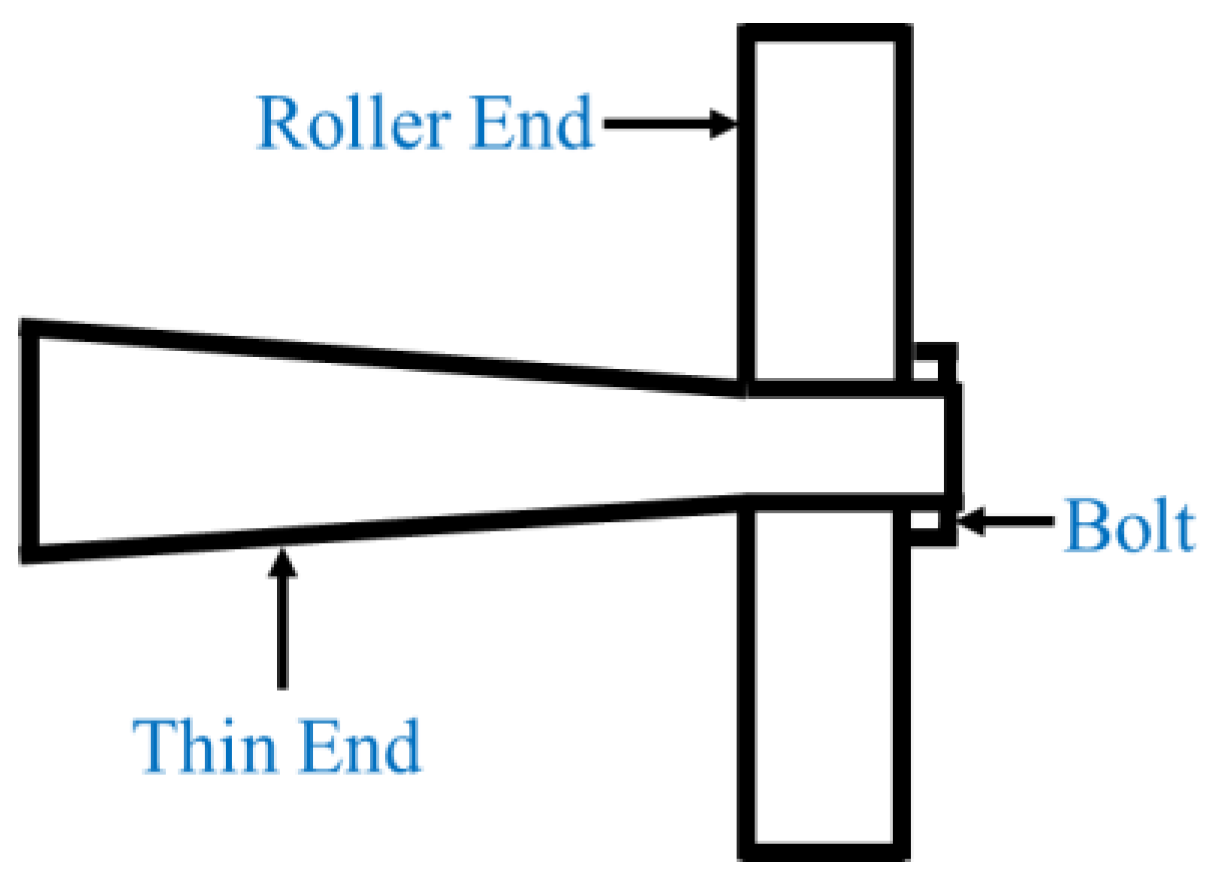
2. The Design Route of Double Thin-End Rotating Sonotrodes
3. Design Theory and the Results of the Unslotted Rotating Sonotrodes
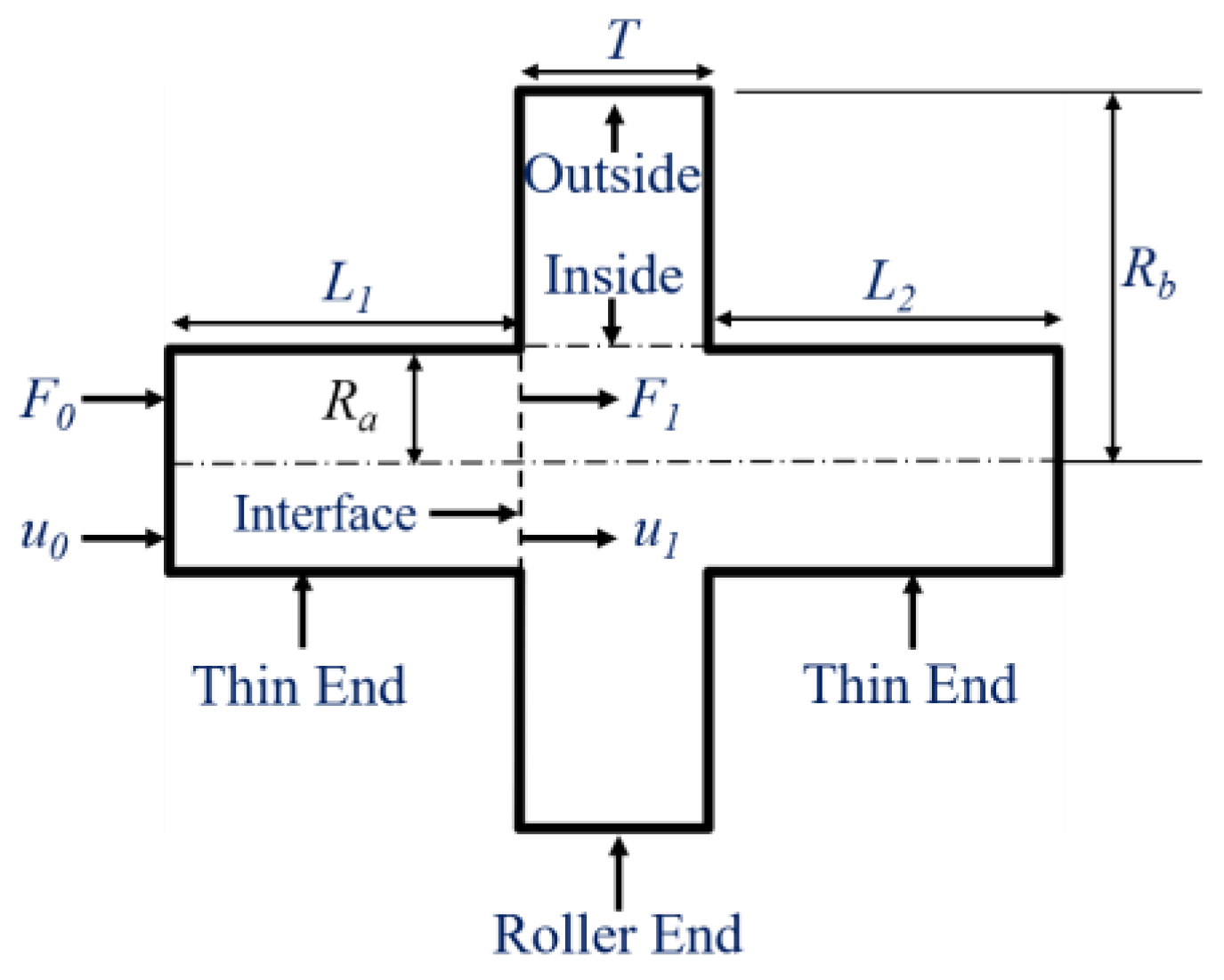
3.1. Mathematical Model and Boundary Conditions
3.2. Relevant Parameters and Calculation Methods
3.3. The Size and Characteristic Frequency of the Unslotted Rotating Sonotrode
4. Design and Optimization of the Slotted Rotating Sonotrode
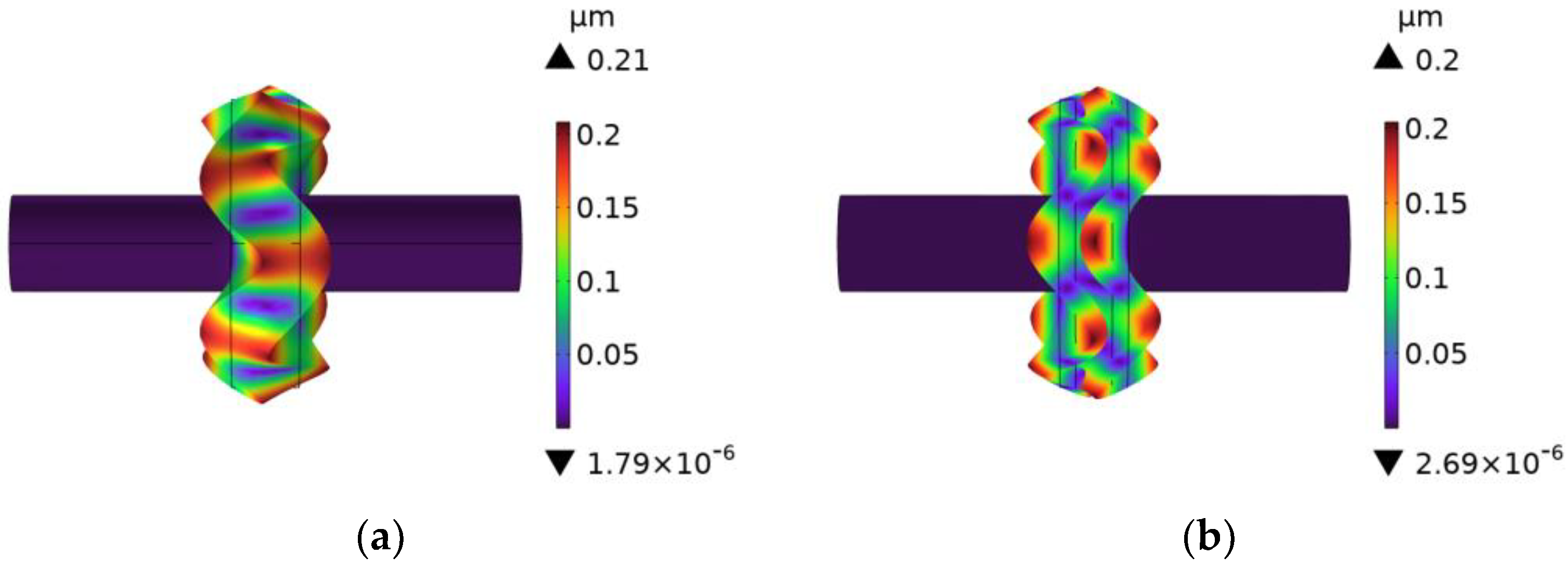
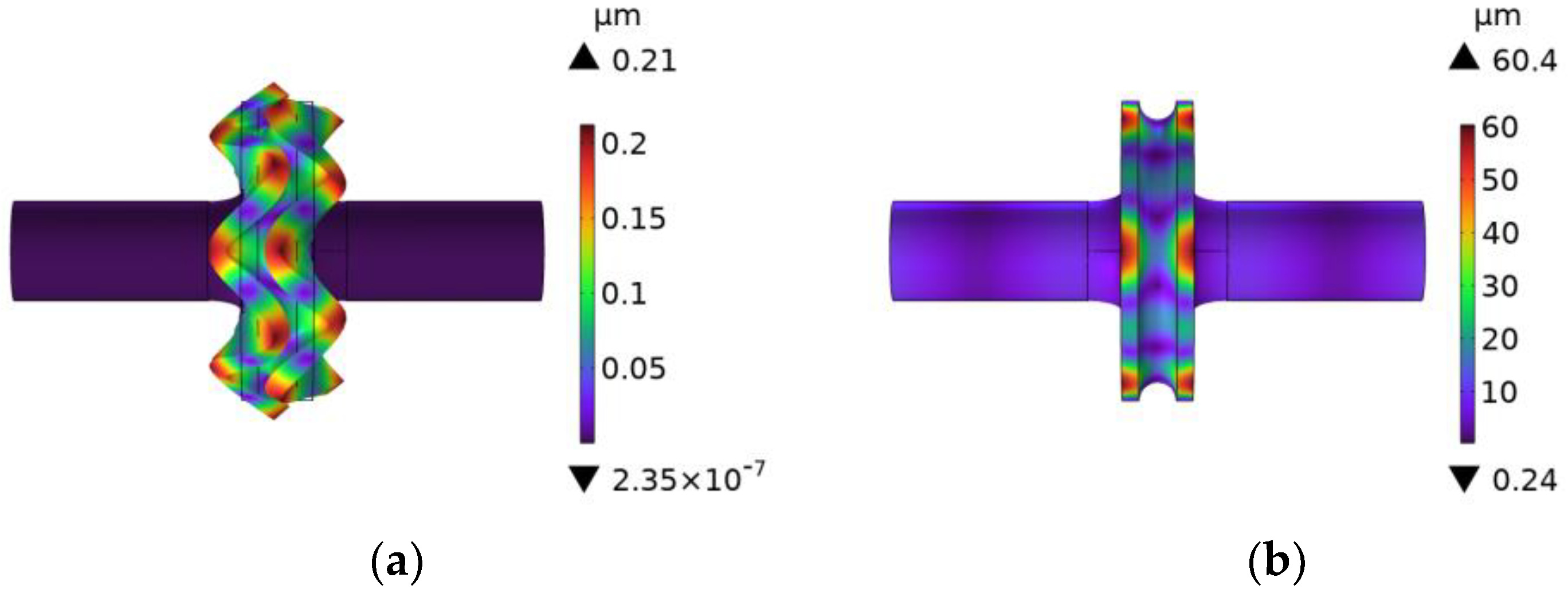
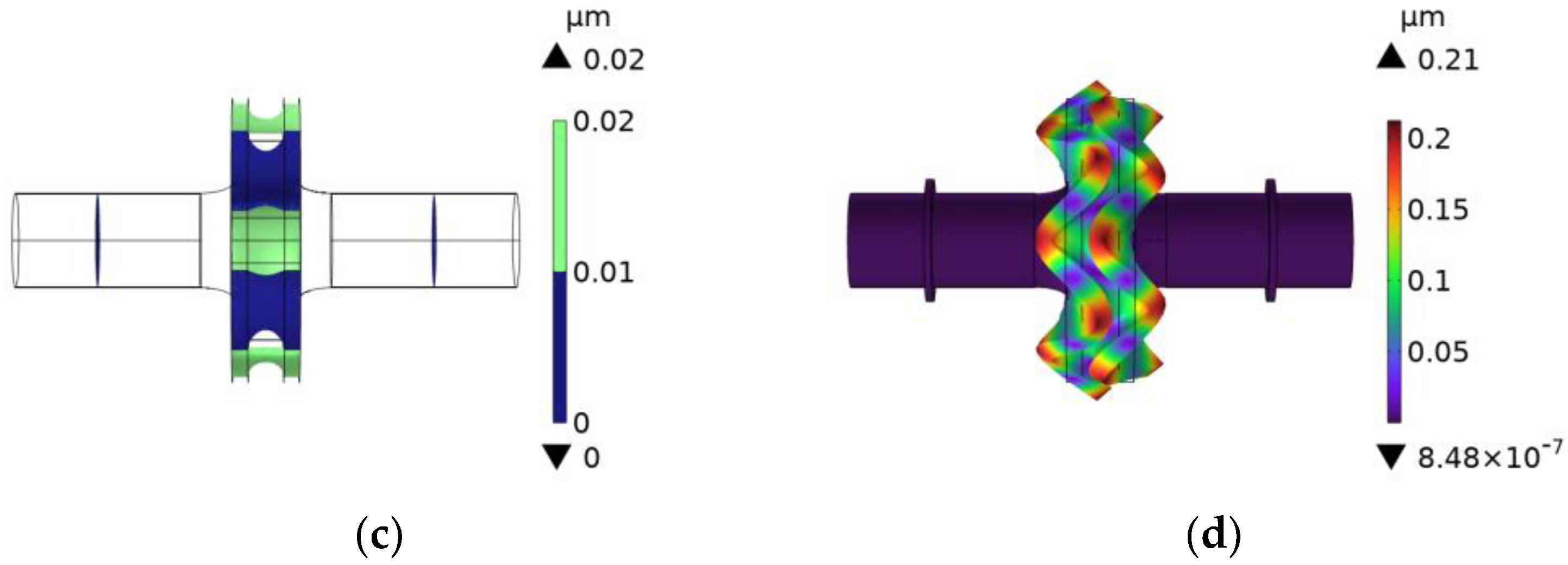
4.1. The Influence of Slotting on the Vibration Mode and Characteristic Frequency
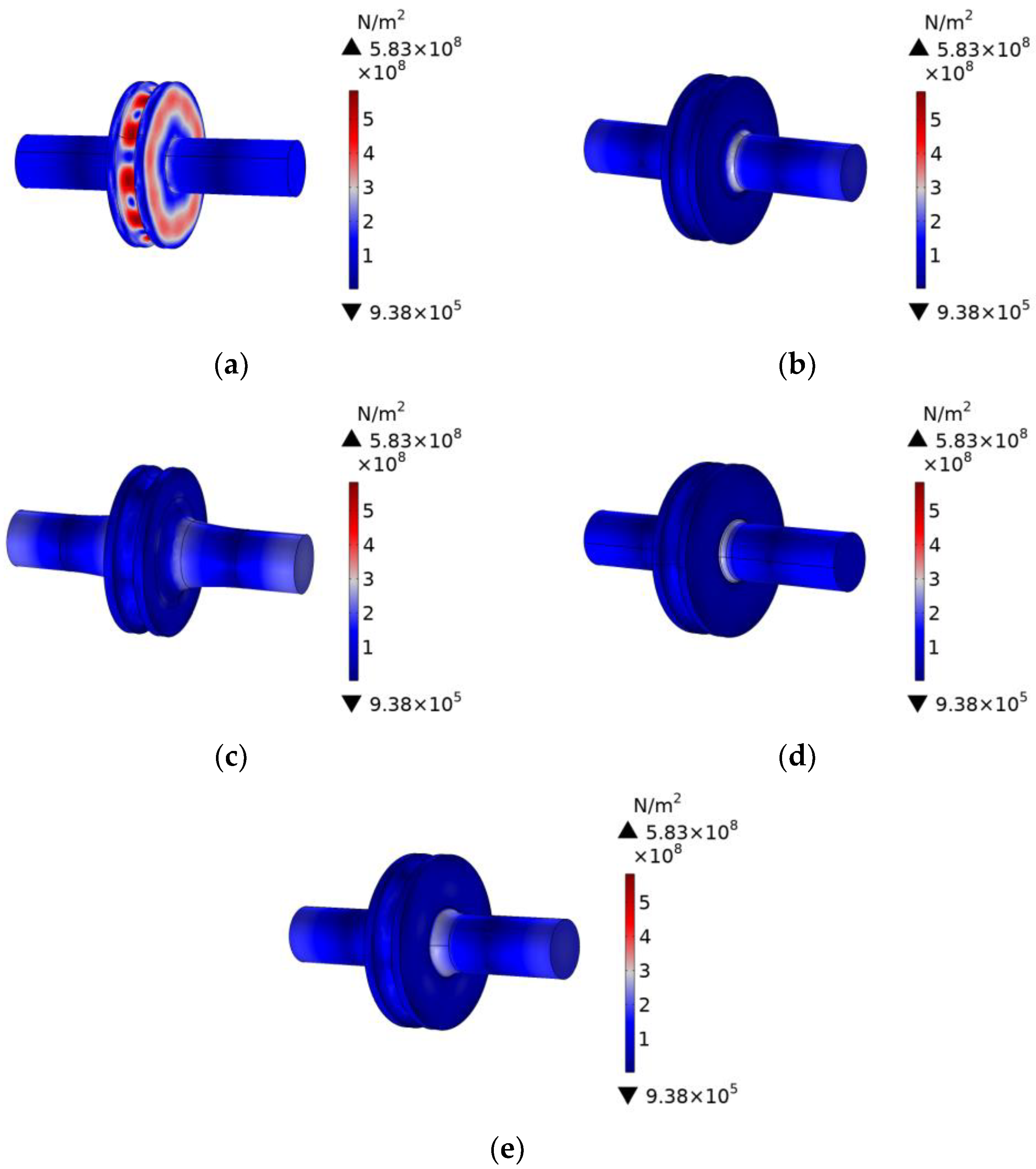
4.2. The Influence of the Transitional Form on the Stress Distribution
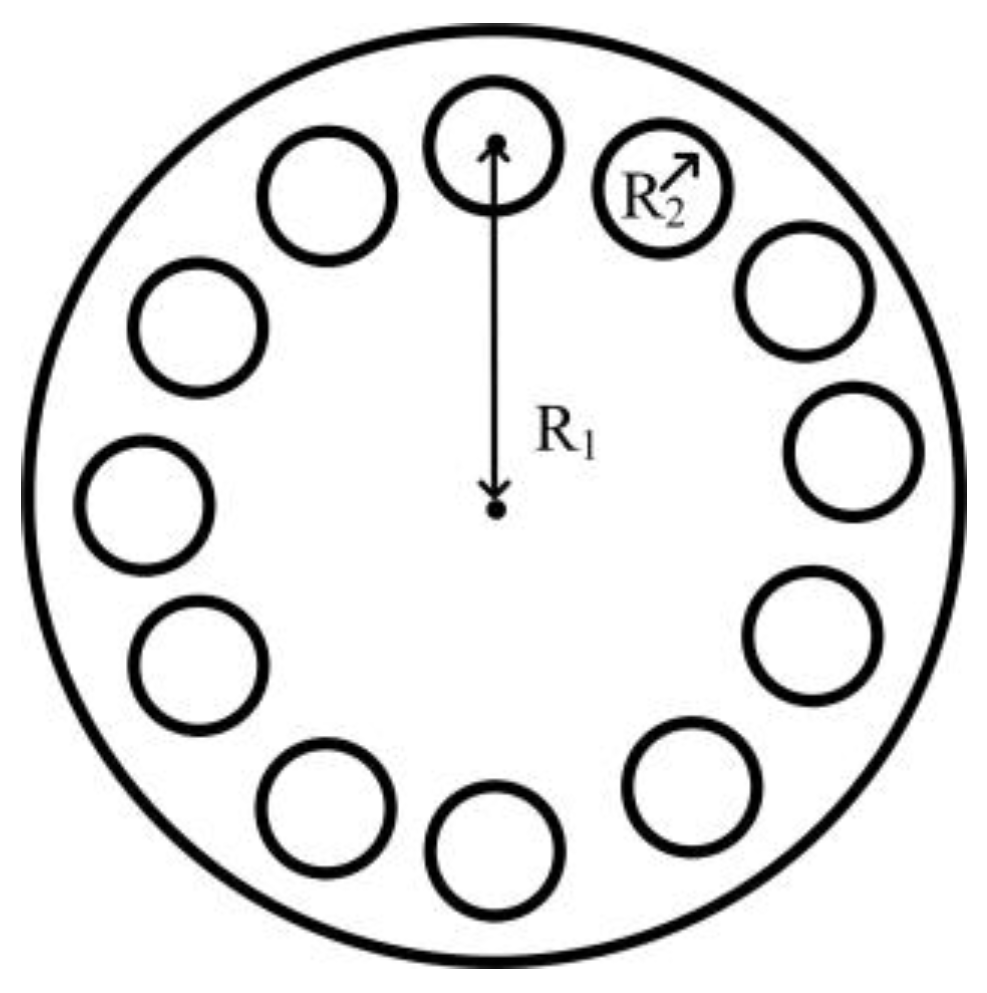
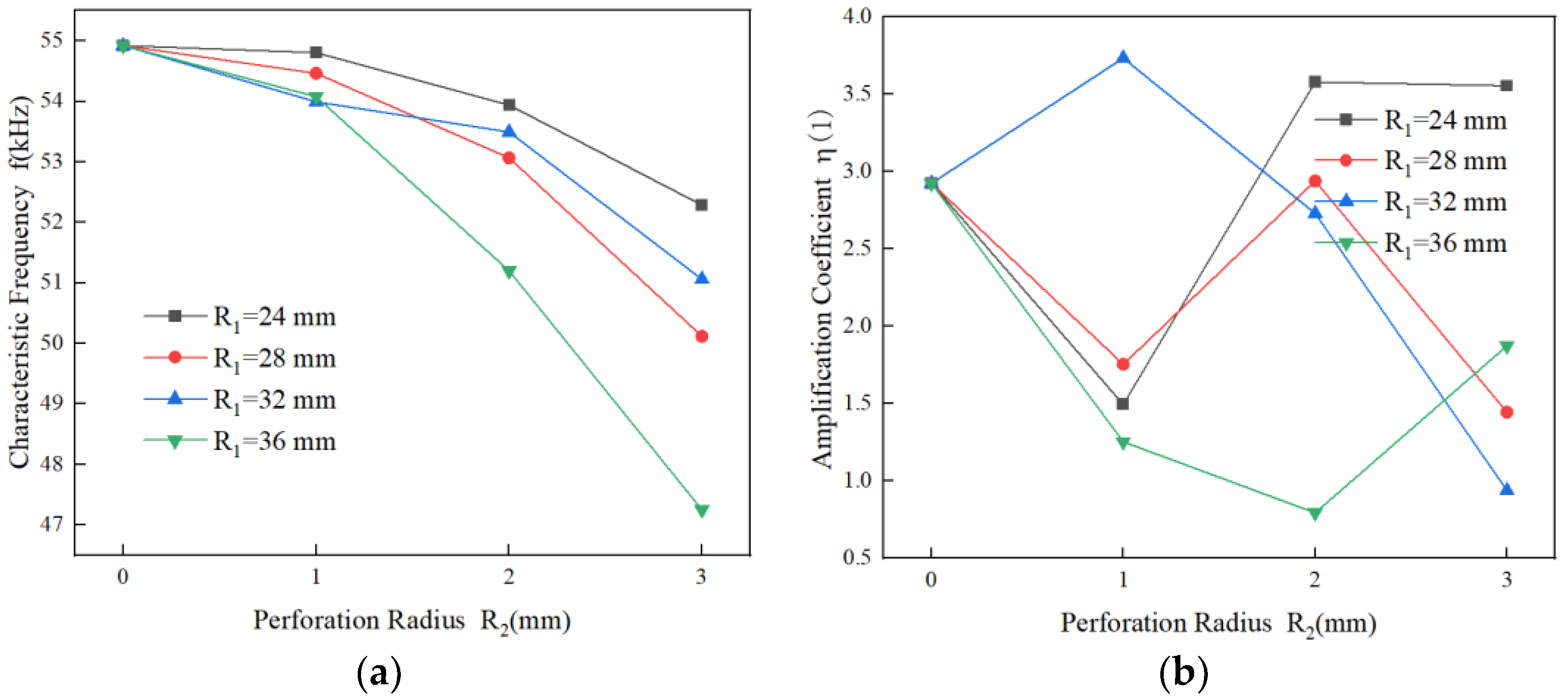
4.3. The Influence of the Size and Position of the Perforation on the Characteristic Frequency and Amplification Coefficient
4.4. The Influence of the Double-Position Joint Perforation on the Characteristic Frequency and Amplification Coefficient
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, S.; Ji, H.; Zhao, W.; Hu, C.; Wang, J.; Li, H.; Wang, J.; Lei, Y. Evaluation of Arc Signals, Microstructure and Mechanical Properties in Ultrasonic-Frequency Pulse Underwater Wet Welding Process with Q345 Steel. Metals 2022, 12, 2119. [Google Scholar] [CrossRef]
- Da Cunha, T.V.; Bohόrquez, C.E. Ultrasound in arc welding: A review. Ultrasonics 2015, 56, 201–209. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.; Wu, C.; Padhy, G.; Ding, W. Application of ultrasonic vibrations in welding and metal processing: A status review. J. Manuf. Process. 2017, 26, 295–322. [Google Scholar] [CrossRef]
- Liu, X.B.; Zhou, Z.K.; Li, Y.N.; Peng, Z.L. Microstructure and mechanical properties of ultrasonic assisted TIG surfacing weld. Hot Process. Technol. 2018, 47, 23–26. [Google Scholar]
- Abbas, P.; Hamid, S.; Hamid, R.R.A.; Yahya, C. A comprehensive study of ultrasonic-assisted gas metal arc welding of high-strength steel. Ultrasonics 2024, 138, 107242. [Google Scholar]
- Chen, Q.H.; Lin, S.B.; Yang, C.L.; Fan, C.L. Effect of Ultrasonic Impact on the Microstructure of Welded Joint of 2195 Al–Li Alloy. Acta Metall. Sin. (Engl. Lett.) 2016, 29, 367–372. [Google Scholar] [CrossRef]
- Chen, Q.H.; Wang, C.C.; Xie, Z.Y.; Mao, X.Y.; Lin, S.B. Formation and properties of lamellar structural weld of aluminum alloy under ultrasonic impact. Chin. J. Nonferrous Met. 2023, 33, 2441–2455. [Google Scholar]
- Yazdian, A.; Karafi, M.R. An analytical approach to design horns and boosters of ultrasonic welding machines. SN Appl. Sci. 2022, 4, 166. [Google Scholar] [CrossRef]
- Hamed, R.; Mohammad, K.; Iman, F.G. Analysis of free and forced vibrations of ultrasonic vibrating tools, case study: Ultrasonic assisted surface rolling process. Int. J. Adv. Manuf. Technol. 2019, 103, 2725–2737. [Google Scholar]
- Hornišová, K.; Billik, P. Some properties of horn equation model of ultrasonic system vibration and of transfer matrix and equivalent circuit methods of its solution. Ultrasonics 2014, 54, 330–342. [Google Scholar] [CrossRef] [PubMed]
- Yahya, C.; Reza, R.M.; Mohsen, K.; Mohammadreza, K. Design and development of ultrasonic assisted-rotational magnetorheological abrasive flow finishing (UA-RMRAFF) process. Appl. Acoust. 2022, 197, 18950. [Google Scholar]
- Lokesh, K.P.; Aswani, K.S.; Varun, S.; Prateek, K. Analysis of a hybrid ultrasonic horn profile using finite element analysis. Mater. Today Proc. 2020, 41, 772–779. [Google Scholar]
- Bikash, B.; Subhadip, P.; Somnath, D.; Arindam, C.; Debabrata, D. Horn design and analysis in ultrasonic machining process using ANSYS. Adv. Mater. Process. Technol. 2022, 8, 1359–1372. [Google Scholar]
- Kartheek, G.; Krishna, P.V. Design and analysis of different horn profiles using FEM for vibration assisted machining. Multiscale Multidiscip. Model. Exp. Des. 2022, 5, 135–155. [Google Scholar] [CrossRef]
- Singh, P.D.; Mishra, S.; Porwal, K.R. Modal Analysis of Ultrasonic Horn using Finite Element Method. Mater. Today Proc. 2019, 18, 3617–3623. [Google Scholar] [CrossRef]
- Zeng, F.F.; Wang, S.Y.; Lv, M. Dynamic analysis for ladder type ultrasonic transformers arc transition. Mech. Des. Manuf. 2012, 210–212. [Google Scholar]
- González-Mendoza, M.J.; Alcántara-Montes, S.; Silva-Lomelí, J.D.J.; Cruz-Alejo, C.D.L.; Ocampo-Ramírez, A. Size optimization of shoulder filleted shafts with relief grooves for improving their fatigue life. Ing. E Investig. 2017, 37, 85–91. [Google Scholar] [CrossRef]
- Tu, X.X.; Xu, Y.J. Shaft shoulder transition curve design and stress concentration analysis. Ship Sci. Technol. 2017, 39, 38–41. [Google Scholar]
- Hannemann, R.; Sander, M. Effect of Specimen Geometry and Press-Fit on the Stress Intensity Factor Solution for Scaled Wheelset Axles under Bending. Procedia Struct. Integr. 2016, 2, 22527–22534. [Google Scholar] [CrossRef][Green Version]
- Mehran, A.; Behrooz, A. Optimal design and experimental validation of ultrasonic wide blade horn. Appl. Acoust. 2021, 182, 108254. [Google Scholar]
- Satpathy, M.P.; Sahoo, S.K. Experimental and numerical studies on ultrasonic welding of dissimilar metals. Int. J. Adv. Manuf. Technol. 2017, 93, 2531–2545. [Google Scholar] [CrossRef]
- Hameed, K.M.; Mahmood, A.M.Q.; Farhan, S.R. Novel ultrasonic horn design for machining advanced brittle composites: A step forward towards green and sustainable manufacturing. Environ. Technol. Innov. 2021, 101652. [Google Scholar]
- Dubravko, R.; Firšt, S.R.; Željko, K.; Siniša, F. New Method for Determining the Machine-Hand Welding Times Using Ultrasonic Welding Machines with Rotary Sonotrode. Machines 2021, 9, 330. [Google Scholar] [CrossRef]
- Dils, C.; Hohner, S.; SchneiderRamelow, M. Use of Rotary Ultrasonic Plastic Welding as a Continuous Interconnection Technology for Large-Area e-Textiles. Textiles 2023, 3, 66–87. [Google Scholar] [CrossRef]
- Thanh-Hai, N.; Thanh, Q.L.; Loc, H.N.; Khoa, T.D. Design of a radial ultrasonic horn for plastic welding using finite element analysis. Jpn. J. Appl. Phys. 2021, 60, 096502. [Google Scholar]
- Camacho, L.M.; Antonio, G.O.; López, D.A.S.; López, M.A.; Ramírez, C.N. Factors influencing droplet size in pneumatic and ultrasonic atomization and its application in food processing. Discov. Food 2023, 3, 23. [Google Scholar] [CrossRef]
- Kustron, P.; Korzeniowski, M.; Sajbura, A.; Piwowarczyk, T.; Kaczynski, P.; Sokolowski, P. Development of High-Power Ultrasonic System Dedicated to Metal Powder Atomization. Appl. Sci. 2023, 13, 8984. [Google Scholar] [CrossRef]
- Zhang, X.; Li, X.H.; He, X.H. Simulation and design of transformer in ultrasonic rolling device. Manuf. Technol. Mach. Tools 2013, 69–73. [Google Scholar]
- Ahmad, S.; Zhang, J.F.; Feng, P.F.; Yu, D.; Wu, Z.J. Experimental study on rotary ultrasonic machining (RUM) characteristics of Nomex honeycomb composites (NHCs) by circular knife cutting tools. J. Manuf. Process. 2020, 58, 58524–58535. [Google Scholar] [CrossRef]
- Mughal, K.H.; Jamil, M.F.; Qureshi, M.A.M.; Qaiser, A.A.; Khalid, F.A.; Maqbool, A.; Raza, S.F.; Ahmad, S.; Zhang, J.F.; Abbas, S.Z. Investigation of rotary ultrasonic vibration assisted machining of Nomex honeycomb composite structures. Int. J. Adv. Manuf. Technol. 2023, 129, 5541–5560. [Google Scholar] [CrossRef]
- Ahmad, S.; Zhang, J.; Feng, P.; Yu, D.W.; Wu, Z.J. Processing technologies for Nomex honeycomb composites (NHCs): A critical review. Compos. Struct. 2020, 250, 112545. [Google Scholar] [CrossRef]
- Harpreet, S.; Pramod, K.J. Study on ultrasonic-assisted electrochemical honing of bevel gears. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2018, 232, 705–712. [Google Scholar]
- Zhang, N.N. Dynamics research and design of ultrasonic gear honing composite horn. Mech. Transm. 2016, 40, 92–96. [Google Scholar]
- Zhou, M.N.; Zhang, N.N.; Hou, Y.P. Design of ultrasonic gear honing amplitude transformer based on transfer matrix method. Machinery 2018, 56, 21–24. [Google Scholar]
- Li, Y.; Lv, M.; Wang, S.Y.; Qin, H.B. A 3D Free Vibration Analysis of the Horn-Gear System through Chebyshev–Ritz Method in Ultrasonic Gear Honing. Shock. Vib. 2021, 2021, 6639345. [Google Scholar] [CrossRef]
- Wu, X.; Qin, H.B.; Zhu, X.J.; Feng, Y.; Zhou, R.F. Investigation of the longitudinal-flexural resonating spindle in ultrasound-assisted grinding of SiCp/Al composites. Int. J. Adv. Manuf. Technol. 2022, 121, 3511–3526. [Google Scholar] [CrossRef]
- Fu, J.F.; Qin, H.B.; Lv, M. Design and experiment of ultrasonic longitudinal-flexural resonant transformer based on Mindlin theory. Vib. Shock 2018, 37, 259–266. [Google Scholar]
- Mathew, W.; Margaret, L. The effect of Ti-6Al-4V microstructure on the performance of ultrasonic soft tissue cutting tips. Proc. Meet. Acoust. 2018, 32, 200. [Google Scholar]
- Heinz, S.; Balle, F.; Wagner, G.; Dietmar, E. Analysis of fatigue properties and failure mechanisms of Ti6Al4V in the very high cycle fatigue regime using ultrasonic technology and 3D laser scanning vibrometry. Ultrasonics 2013, 53, 1433–1440. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.L.; Liu, S.Q. A study on longitudinal vibration characteristics of a radially perforated ultrasonic horn by finite element method. J. Zhejiang Norm. Univ. (Nat. Sci. Ed.) 2020, 43, 264–268. [Google Scholar]
- Takuya, A.; Hikaru, M. Design and experimental investigation of stepped horn with a hollow part to allow individual design of amplitude amplification factors for longitudinal-torsional vibration source. Jpn. J. Appl. Phys. 2022, 61, SG1074. [Google Scholar]




| Parameter Name/Symbol | Numerical Value |
|---|---|
| The length of the thin ends on both sides of the rotating sonotrode/L1 and L2 | 61 mm |
| Rotating sonotrode radius/Ra | 10–15 mm |
| Roller end radius of rotating sonotrode/Rb | 40 mm |
| Thickness of the roller end of rotating sonotrode/T | 16–20 mm |
| Density of the TC4 titanium alloy/ρ | 4.43 g/cm3 |
| Elastic modulus of the TC4 titanium alloy/E | 110 G Pa |
| Poisson’s ratio of the TC4 titanium alloy/μ | 0.34 |
| Number of Classes | |||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| Thin-end radius (mm) | 10.8735 | 11.6395 | 12.9620 | 13.5615 | 14.1484 |
| Radius of the roller end (mm) | 37.9179 | 39.6248 | 37.8928 | 41.5578 | 43.1557 |
| Thickness of the roller end (mm) | 20.2326 | 17.0428 | 20.3324 | 19.9792 | 16 |
| Entry 4 characteristic frequency (kHz) | 55.23 | 49.026 | 55.368 | 48.667 | 54.073 |
| Frequency error (%) | 10.46 | 1.948 | 10.736 | 2.666 | 8.146 |
| Number of Classes | |||||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | |
| Thin-end radius (mm) | 11.6 | 13.3 | 13.3 | 13.3 | 13.5 | 13.6 | 13.8 |
| Radius of the roller end (mm) | 39.6 | 40 | 40 | 40 | 39.5 | 41.6 | 39.4 |
| Thickness of the roller end (mm) | 17.1 | 18.6 | 18.7 | 18.8 | 18.5 | 20 | 18.5 |
| Characteristic frequency (kHz) | 48.621 | 49.998 | 50.101 | 50.187 | 50.778 | 48.621 | 50.949 |
| Frequency error (%) | 2.758 | 0.004 | 0.202 | 0.374 | 1.556 | 2.758 | 1.898 |
| Transition Mode | Characteristic Frequency (kHz) |
|---|---|
| No transition | 45.093 |
| Single-curvature arc transition | 45.282 |
| Double-curvature arc transition | 48.172 |
| Three-curvature arc transition | 45.217 |
| Elliptical transition | 45.226 |
| Interface | No Transition | Single-Curvature Arc | Double-Curvature Arc | Three-Curvature Arc | Ellipse | |
|---|---|---|---|---|---|---|
| Peak stress (N/m2) | Inner side | 2.74 × 108 | 1.14 × 108 | 1.89 × 108 | 4.22 × 107 | 6.36 × 107 |
| Outer side | 2.4 × 108 | 3.54 × 107 | 1.46 × 108 | 1.71 × 108 | ||
| Minimum stress (N/m2) | Inner side | 1.93 × 108 | 8.55 × 107 | 1.26 × 108 | 8.03 × 106 | 3.76 × 107 |
| Outer side | 2.2 × 108 | 2.86 × 107 | 1.43 × 108 | 1.65 × 108 | ||
| Mean stress (N/m2) | Inner side | 2.23 × 108 | 9.98 × 107 | 1.55 × 108 | 2.58 × 107 | 5.04 × 107 |
| Outer side | 2.28 × 108 | 3.2 × 107 | 1.45 × 108 | 1.67 × 108 |
| Inner Perforation | Outer Perforation | Characteristic Frequency (kHz) | Amplification Coefficient |
|---|---|---|---|
| The perforation radius was 2 mm. | The perforation radius was 2 mm. | 51.988 | 3.18 |
| The perforation radius was 3 mm. | 49.598 | 3.185 | |
| The perforation radius was 3 mm. | The perforation radius was 2 mm. | 49.721 | 3.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mao, X.; Yang, Z.; Chen, Q.; Hu, M.; Gan, T. Rotating Sonotrode Design for Ultrasonic-Assisted Arc Welding of Metal Materials. Materials 2024, 17, 1599. https://doi.org/10.3390/ma17071599
Mao X, Yang Z, Chen Q, Hu M, Gan T. Rotating Sonotrode Design for Ultrasonic-Assisted Arc Welding of Metal Materials. Materials. 2024; 17(7):1599. https://doi.org/10.3390/ma17071599
Chicago/Turabian StyleMao, Xinyu, Zhidong Yang, Qihao Chen, Mingzhu Hu, and Tian Gan. 2024. "Rotating Sonotrode Design for Ultrasonic-Assisted Arc Welding of Metal Materials" Materials 17, no. 7: 1599. https://doi.org/10.3390/ma17071599
APA StyleMao, X., Yang, Z., Chen, Q., Hu, M., & Gan, T. (2024). Rotating Sonotrode Design for Ultrasonic-Assisted Arc Welding of Metal Materials. Materials, 17(7), 1599. https://doi.org/10.3390/ma17071599






