Investigation of the Penetration Performance of the Radial Forging Process for Wrought Aluminium Alloy
Abstract
:1. Introduction
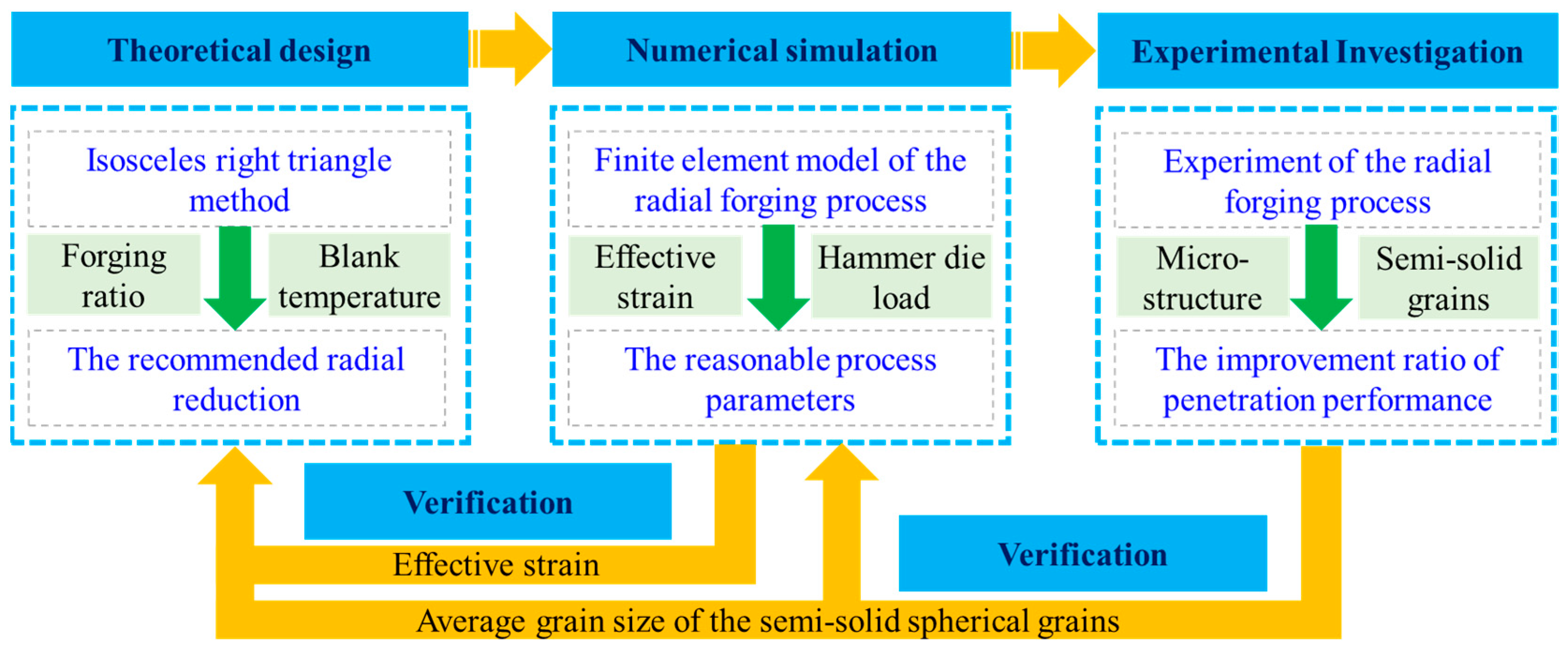
2. Materials and Methods
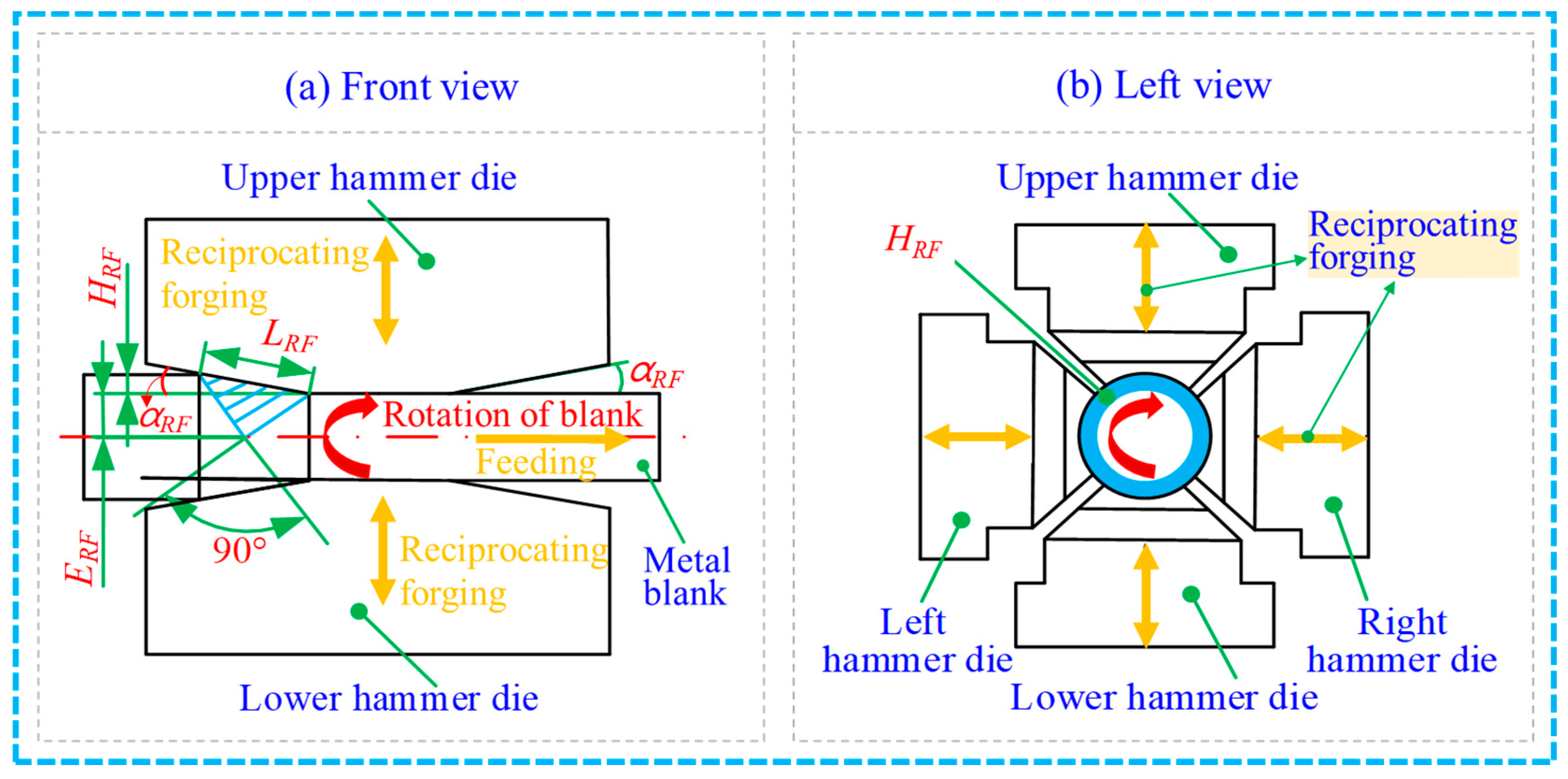
2.1. Theoretical Design for the Penetration Performance of the RF Process
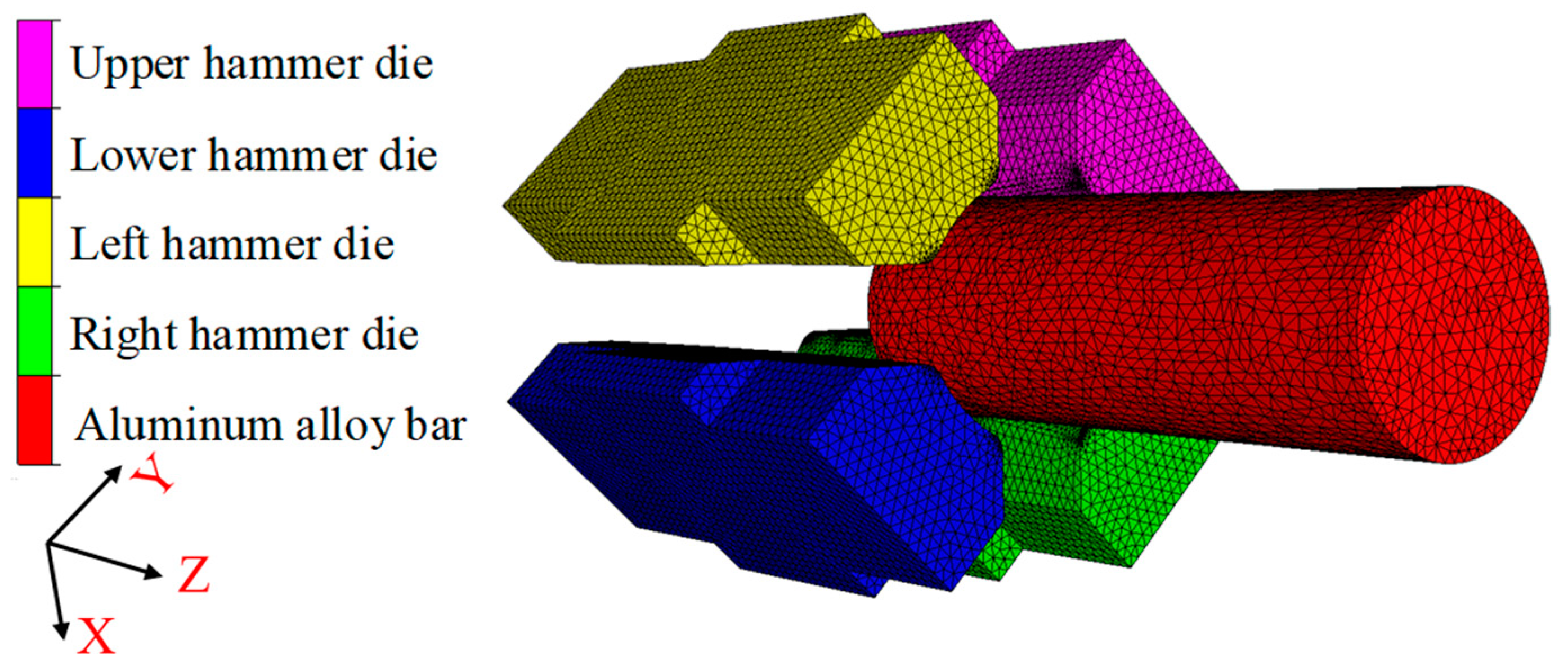
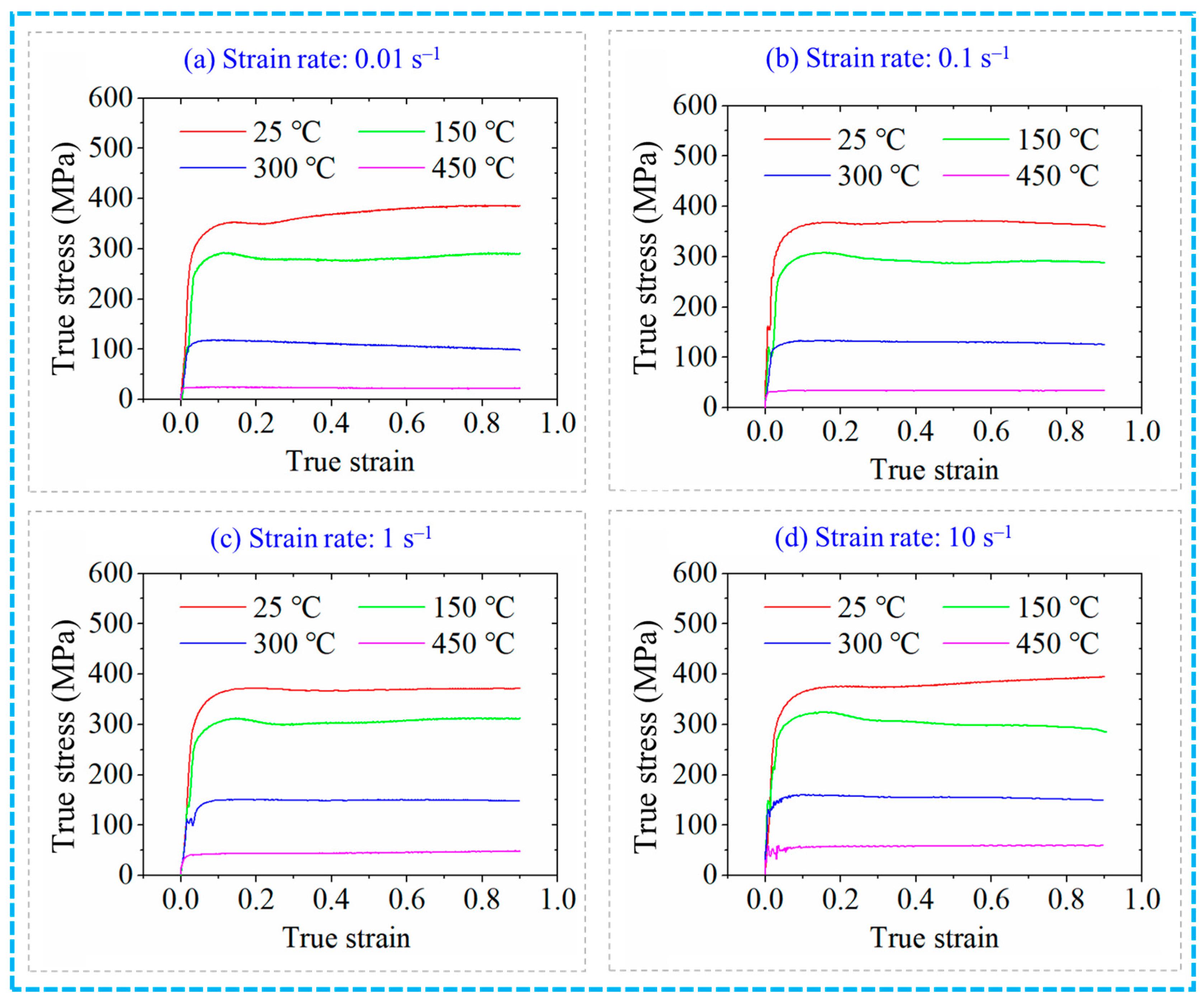
2.2. Simulation Setting
- (1)
- The hammer die is regarded as a rigid component in the process of RF deformation, as it has a significantly smaller deformation.
- (2)
- The elastic deformation in the metal blank is ignored in the process of RF deformation, as its value is significantly low compared to plastic deformation, which has a limited effect on the deformation.
- (3)
- Defects and density change in the material are ignored.
- (4)
- The weight of the material and the hammer dies are negligible.
- (5)
- The initial material has an isotropic and uniform structure.
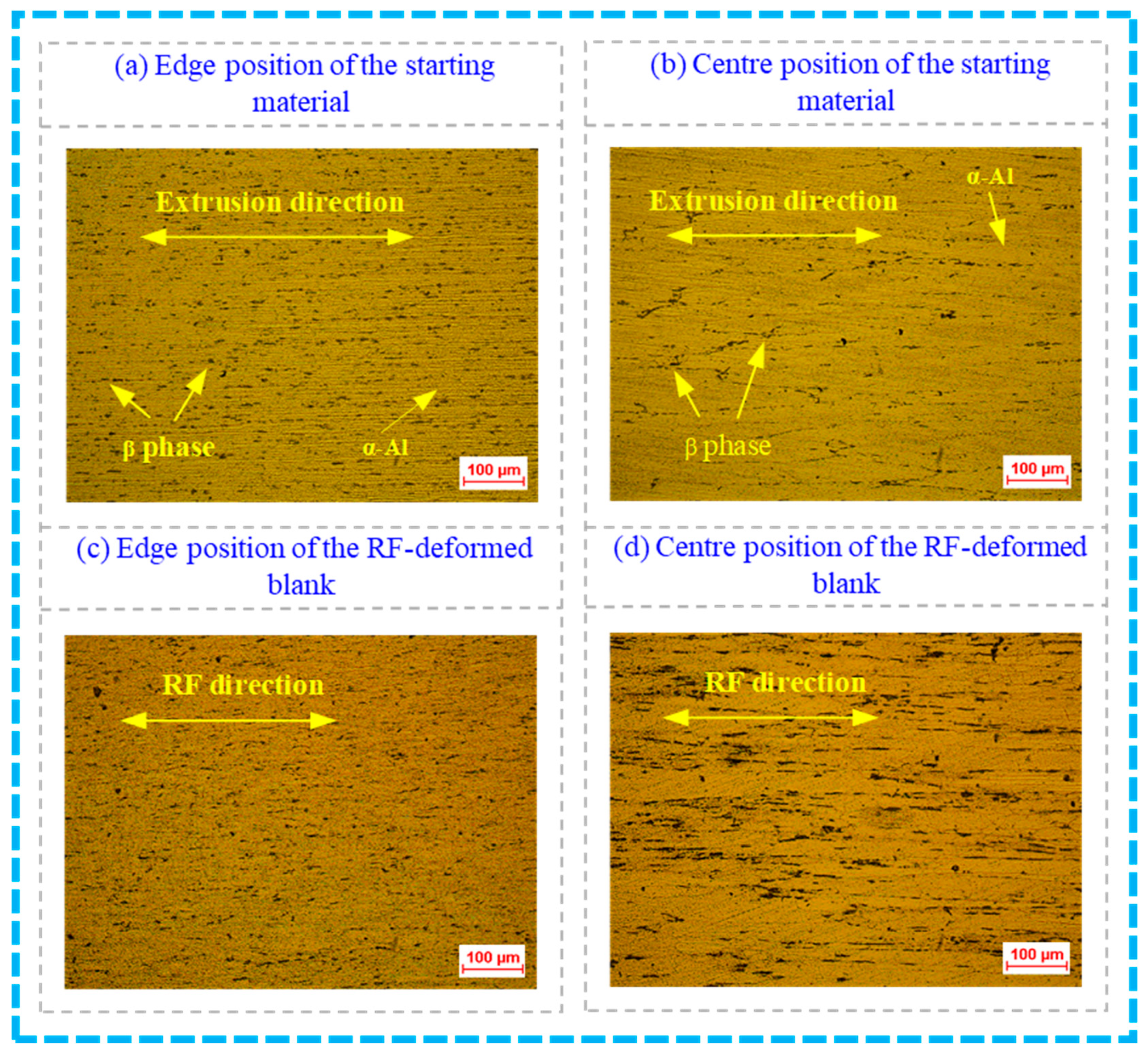
2.3. Experimental Setup and Microstructure Analysis
3. Results
3.1. Simulation Results and Discussion for the Radial Forging Process
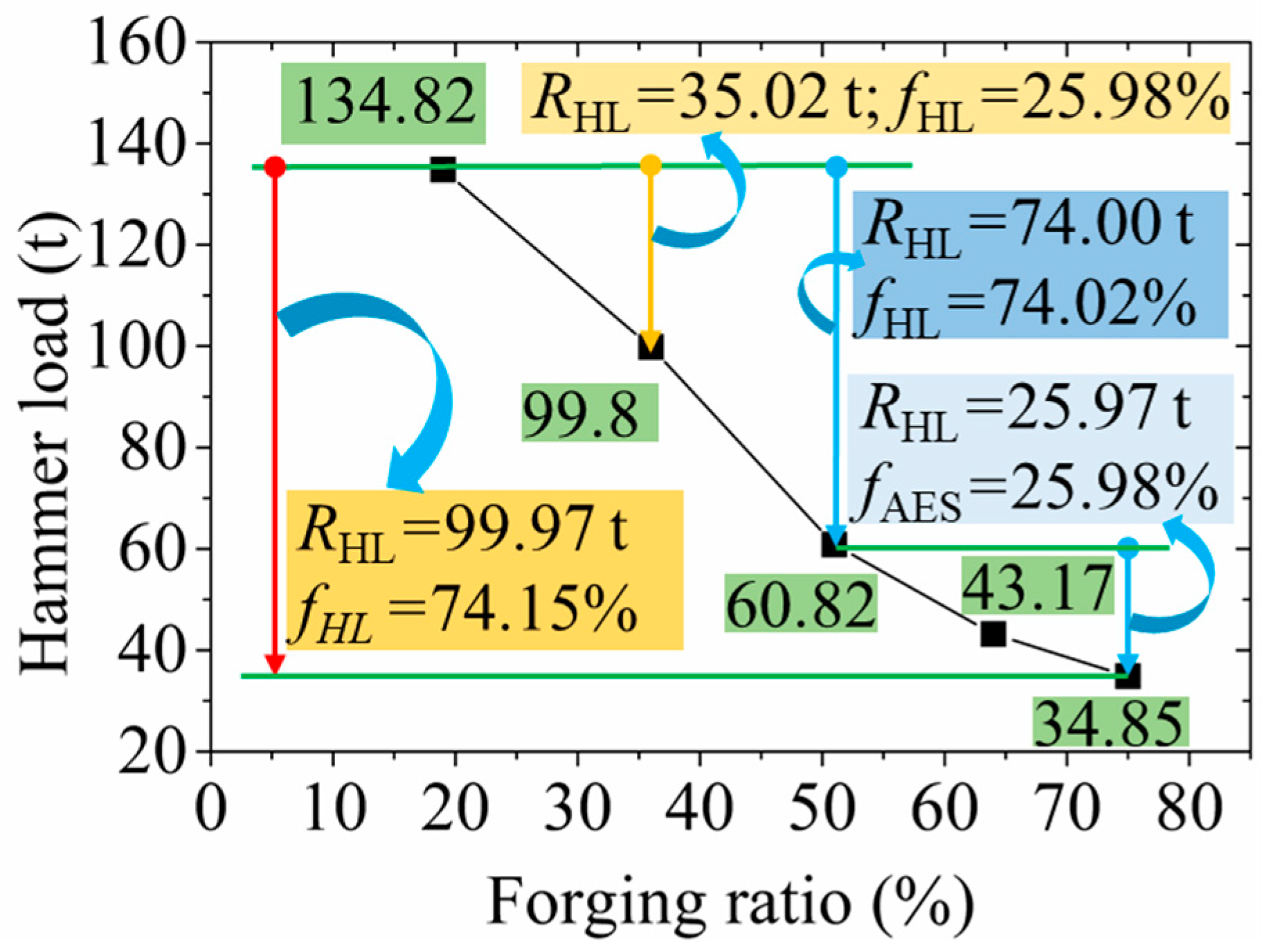
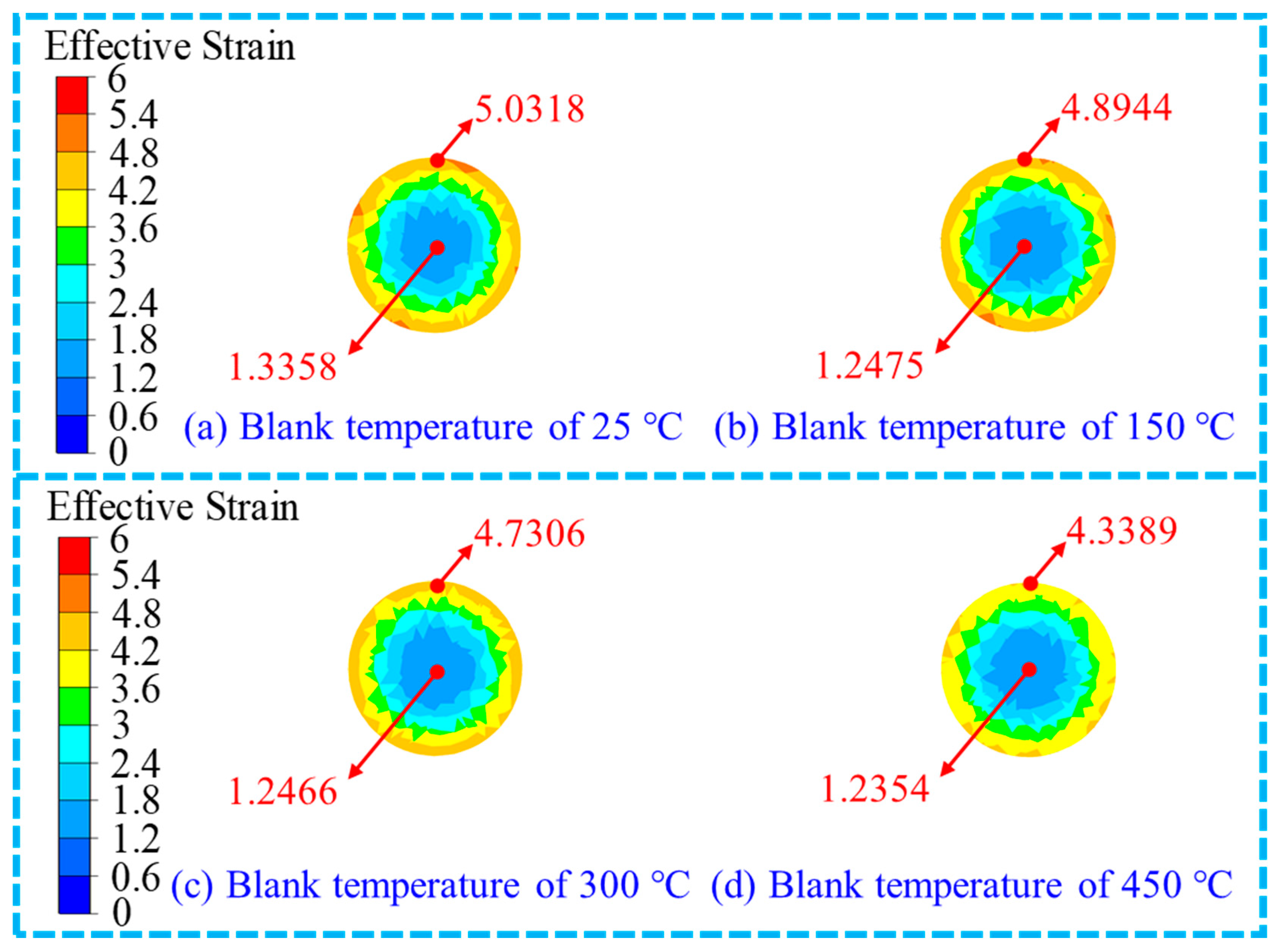
3.1.1. Effect of FR on the RF Process
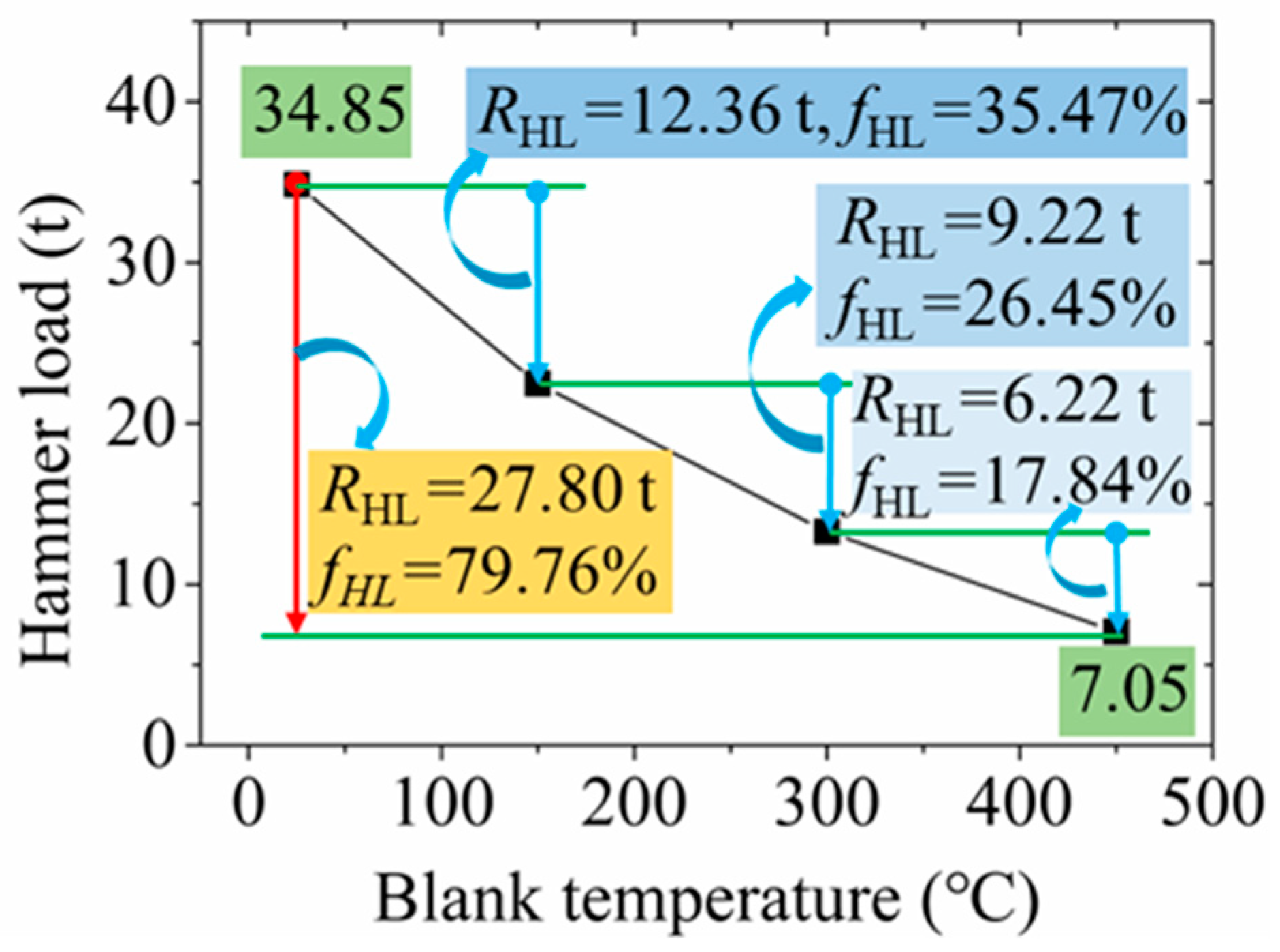
3.1.2. Effect of Blank Temperature on the RF Process
3.2. Experimental Results and Discussion for the Radial Forging Process
4. Conclusions
- (1)
- With the increase in FR, the effective strains at the edge and the centre positions of the RF-deformed blank gradually increase. With an increase in the FR from 19% to 36%, 36% to 51%, 51% to 64% and 64% to 75%, the fAES is obtained as 90.78%, 52.56%, 30.14% and 2.47%, respectively. The reduction in hammer load is 99.98 t with an increase in the FR from 19% to 75%, along with a reduction ratio of 74.15%. Moreover, with the increase in the FR from 19% to 51% and 64% to 75%, the reduction in hammer load was observed as 74.00 t and 25.98 t, respectively.
- (2)
- With an increase in blank temperature, the effective strain at the edge position decreases slightly, while the change degree of the effective strain at the centre position is not obvious. The fAES is 2.15%, 2.42%, and 3.75% with the increase in the blank temperature from 25 °C to 150 °C, 150 °C to 300 °C and 300 °C to 450 °C, respectively. The hammer load significantly reduces when increasing the blank temperature. The reduction in hammer load is found to be 12.36 t, 9.22 t and 6.22 t with changes in blank temperature from 25 °C to 150 °C to 300 °C and 300 °C to 450 °C, respectively.
- (3)
- The selection principle of process parameters for the RF process of the wrought aluminium alloy is concluded as follows: process parameters with low temperature and large FR should be selected as far as possible. The appropriate process parameters for the RF process in this work are then selected as an FR of 64% and a blank temperature of 25 °C.
- (4)
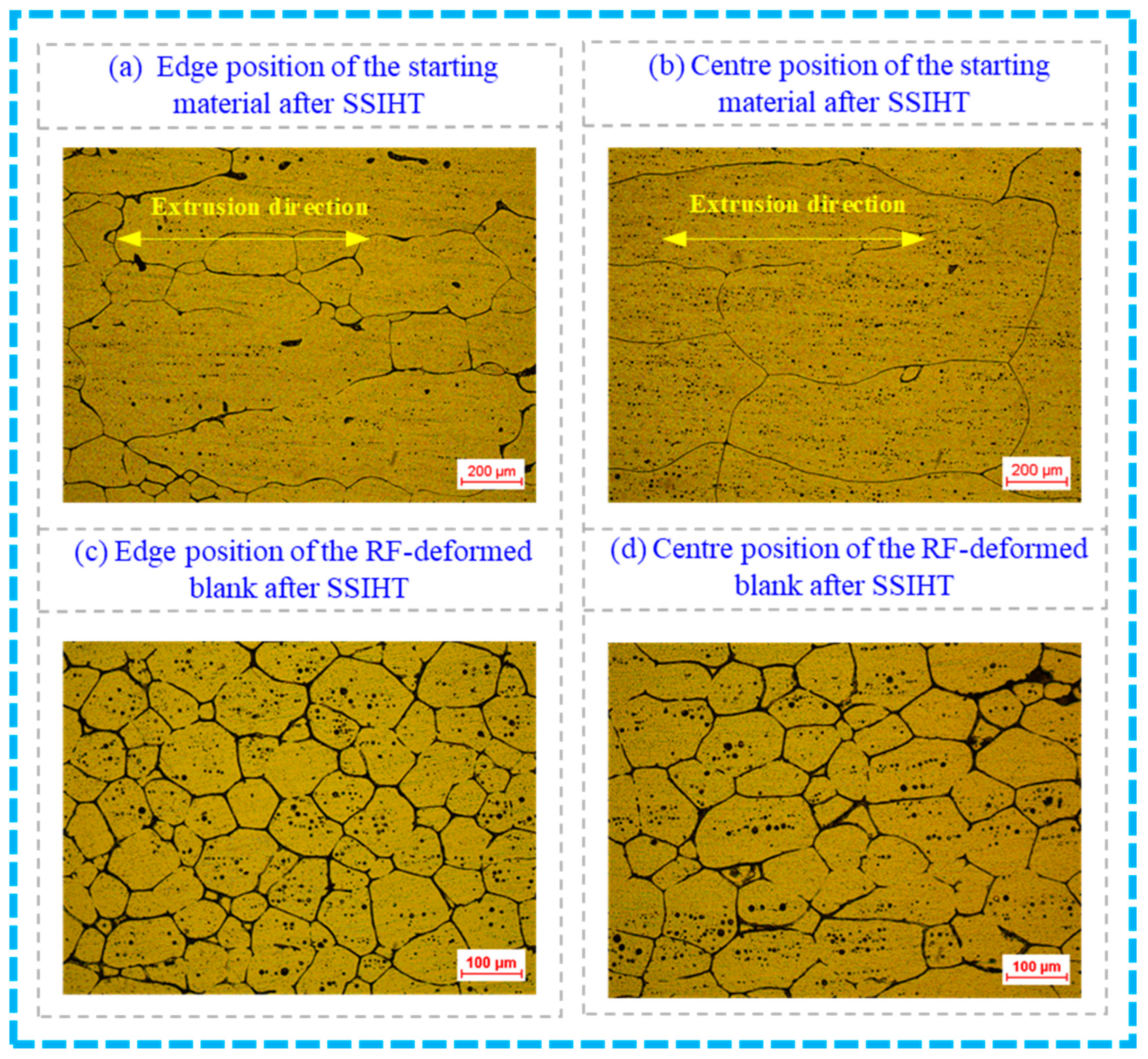
- The semi-solid microstructure with the AGS of 71.65 μm can be obtained by applying the SSIHT process to an RF-deformed blank at 630 °C for 10 min. Compared with the starting material, the improvement ratio of the penetration performance is obtained as 91.19% for the RF-deformed blank with an FR of 64% and a blank temperature of 25 °C.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Becker, M.; Kuball, A.; Ghavimi, A.; Adam, B.; Busch, R.; Gallino, I.; Balle, F. Solid State Joining of a Cold Rolled Zr-Based Bulk Metallic Glass to a Wrought Aluminum Alloy by Power Ultrasonics. Materials 2022, 15, 7673. [Google Scholar] [CrossRef] [PubMed]
- Shen, T.; Zhang, S.; Liu, Z.; Yu, S.; Jiang, J.; Tao, X.; Akopyan, T.; Belov, N.; Yao, Z. Convert Harm into Benefit: The Role of the Al10CaFe2 Phase in Al-Ca Wrought Aluminum Alloys Having High Compatibility with Fe. Materials 2023, 16, 7488. [Google Scholar] [CrossRef] [PubMed]
- Ha, S.-H.; Shin, Y.-C. Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging. Materials 2024, 17, 968. [Google Scholar] [CrossRef]
- Chen, G.-C.; Li, X. Effect of TiC nano-treating on the fluidity and solidification behavior of aluminum alloy 6063. J. Mater. Process. Technol. 2024, 324, 118241. [Google Scholar] [CrossRef]
- Özen, Z.T.; Sarı, İ.A.; Siyah, İ.Y.; Tanses, A.; Özçelik, G.; Nurten, A.H.; Özdemir, B.; Caylak, M. Enhancing Recycling Efficiency and Critical Raw Material Substitution in 6xxx Alloys’ Production with Respect to Their Extrusion Feasibility and Mechanical Properties. In TMS Annual Meeting & Exhibition; Springer Nature: Cham, Switzerland; pp. 215–225.
- Guo, Y.; Wang, Y.; Zhao, S. Experimental Investigation and Optimization of the Semisolid Multicavity Squeeze Casting Process for Wrought Aluminum Alloy Scroll. Materials 2020, 13, 5278. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Zhang, X.; Wang, L.; Qiao, J. The Effect of Extrusion and Heat Treatment on the Microstructure and Tensile Properties of 2024 Aluminum Alloy. Materials 2022, 15, 7566. [Google Scholar] [CrossRef]
- Moshtaghi, M.; Safyari, M.; Kuramoto, S.; Hojo, T. Unraveling the effect of dislocations and deformation-induced boundaries on environmental hydrogen embrittlement behavior of a cold-rolled Al–Zn–Mg–Cu alloy. Int. J. Hydrogen Energy 2021, 46, 8285–8299. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, S.; Zhang, C. Microstructural evolution of semisolid 6063 aluminum alloy prepared by recrystallization and partial melting process. J. Mater. Eng. Perform. 2017, 26, 4354–4363. [Google Scholar] [CrossRef]
- Chang, Z.; Liu, L.; Sui, Z.; Yan, X.; Li, Y.; Zhang, Y.; Zhang, Y.; Yang, M. Effect of Aging Temperature on Pitting Corrosion of AA6063 Aluminum Alloy. Met. Mater. Int. 2024, 1–15. [Google Scholar] [CrossRef]
- Hovden, S.; Kronsteiner, J.; Arnoldt, A.; Horwatitsch, D.; Kunschert, G.; Österreicher, J.A. Parameter study of extrusion simulation and grain structure prediction for 6xxx alloys with varied Fe content. Mater. Today Commun. 2024, 38, 108128. [Google Scholar] [CrossRef]
- Negozio, M.; Segatori, A.; Pelaccia, R.; Reggiani, B.; Donati, L. Experimental investigation and numerical prediction of the peripheral coarse grain (PCG) evolution during the extrusion of different AA6082 aluminum alloy profiles. Mater. Charact. 2024, 113723. [Google Scholar] [CrossRef]
- Zou, J.; Ma, L.; Zhu, Y.; Qin, L.; Yuan, Y. Gradient microstructure and superior strength–ductility synergy of AZ61 magnesium alloy bars processed by radial forging with different deformation temperatures. J. Mater. Sci. Technol. 2024, 170, 65–77. [Google Scholar] [CrossRef]
- Xu, W.; Zhang, J.; Zhong, R.; Yang, J.; Han, D.; Ji, P.; Lian, Y.; Zhang, B.; Liu, B. Residual Stress Relaxation and Microstructure Evolution in Cold Radial Forged High-Strength Steel Tube Using Thermal-Vibratory Stress Relief Technique. J. Mater. Eng. Perform. 2023, 33, 2064–2076. [Google Scholar] [CrossRef]
- Feng, F.; Lian, Y.; Wang, J.; Song, J.; Yan, B.; Liu, X. Mechanical properties and thermal shock performance of high-energy-rate-forged W-1%TaC alloy. Crystals 2022, 12, 1047. [Google Scholar] [CrossRef]
- Sanjari, M.; Taheri, A.K.; Movahedi, M. An optimization method for radial forging process using ANN and Taguchi method. Int. J. Adv. Manuf. Technol. 2009, 40, 776–784. [Google Scholar] [CrossRef]
- Zou, J.; Ma, L.; Jia, W.; Le, Q.; Qin, G.; Yuan, Y. Microstructural and mechanical response of ZK60 magnesium alloy subjected to radial forging. J. Mater. Sci. Technol. 2021, 83, 228–238. [Google Scholar] [CrossRef]
- Du, Z.; Xu, W.; Wang, Z.; Zhu, X.; Wang, J.; Wang, H. Multi-objective optimization of concave radial forging process parameters based on response surface methodology and genetic algorithm. Int. J. Adv. Manuf. Technol. 2024, 130, 5025–5044. [Google Scholar] [CrossRef]
- Yang, Y.-Z.; Xu, C.; Fan, L.-X. Evolution of inner wall wrinkle defects in the sinking zone of a thick-walled steel tube during radial forging. Adv. Manuf. 2023, 1–13. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, S.; Zhang, C. Grain Refinement of Aluminum Alloy Bar by a Modified RAP Process for Semi-Solid Forming. Mater. Trans. 2017, 58, 176–181. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, S.; Guo, Y.; Liu, K.; Zheng, S. Deformation Characteristics and Constitutive Equations for the Semi-Solid Isothermal Compression of Cold Radial Forged 6063 Aluminium Alloy. Materials 2021, 14, 194. [Google Scholar] [CrossRef]
- Zhou, X.-d.; Liu, X.-r.; Xing, J.-b. Forging penetration efficiency of steel H13 stepped shaft radial forging with GFM forging machine. J. Shanghai Jiaotong Univ. Sci. 2012, 17, 315–318. [Google Scholar] [CrossRef]
- Xue, Z.; Lixia, F.; Heci, Z. Analysis of Forging Penetration of Barrel Rifling in Precision Radial Forging Process. Acta Armamentarii 2019, 40, 473. [Google Scholar]
- Han, X.; Zeng, F.; Zhuang, W.; Hua, L.; Hu, Y.; Wang, Z. An innovative rotary rolling-forging process for manufacturing fork ring with extreme geometry. J. Mater. Process. Technol. 2023, 322, 118160. [Google Scholar] [CrossRef]
- Yang, X.; Dong, X.; Wu, Y. An upper bound solution of forging load in cold radial forging process of rectangular cross-section billet. Int. J. Adv. Manuf. Technol. 2017, 92, 2765–2776. [Google Scholar] [CrossRef]
- Li, H.-x.; Wang, K.; Luo, R.; Zhu, Z.-z.; Deng, S.; Luo, R.; Zhang, J.-y.; Fang, F.-s. Influence of radial forging process on strain inhomogeneity of hollow gear shaft using finite element method and orthogonal design. J. Cent. South Univ. 2020, 27, 1666–1677. [Google Scholar] [CrossRef]
- Gautam, S.K.; Singh, B.K. Investigation on the effects of isothermal holding temperature and time on the coarsening mechanism and rheological properties of ADC12 Al semi-solid slurry. Mater. Chem. Phys. 2024, 314, 128813. [Google Scholar] [CrossRef]
- Wang, J.; Wang, Z.; Xu, W.; Du, Z.; Wang, H. Multi-objective optimization of forging surface structure parameters of radial forging die with cycloidal. Int. J. Adv. Manuf. Technol. 2023, 129, 5709–5727. [Google Scholar] [CrossRef]
- Liu, X.R.; Zhou, X.D. The forging penetration efficiency of C45 steel stepped shaft radial forging with GFM forging machine. Adv. Mater. Res. 2011, 154, 593–596. [Google Scholar] [CrossRef]
- Shabestari, S.G.; Abdi, M.; Naghdali, S. Effect of thixoforming and precipitation hardening on microstructure and mechanical properties of Al-10.5Si–3Cu-0.2Mg alloy produced by strain induced melt activation process. J. Mater. Res. Technol. 2021, 15, 4981–4992. [Google Scholar] [CrossRef]
- Jiang, H.; An, L.; Li, F.; Zhang, P.; Dong, P.; Li, S.; Wang, Y.; Zhao, S. Numerical and experimental study on the rheo-extrusion process of 2A50 aluminium alloy miniature gear. J. Mater. Res. Technol. 2023, 24, 1468–1482. [Google Scholar] [CrossRef]
- Zhang, Q.Q.; Cao, Z.Y.; Zhang, Y.F.; Su, G.H.; Liu, Y.B. Effect of compression ratio on the microstructure evolution of semisolid AZ91D alloy. J. Mater. Process. Technol. 2007, 184, 195–200. [Google Scholar] [CrossRef]
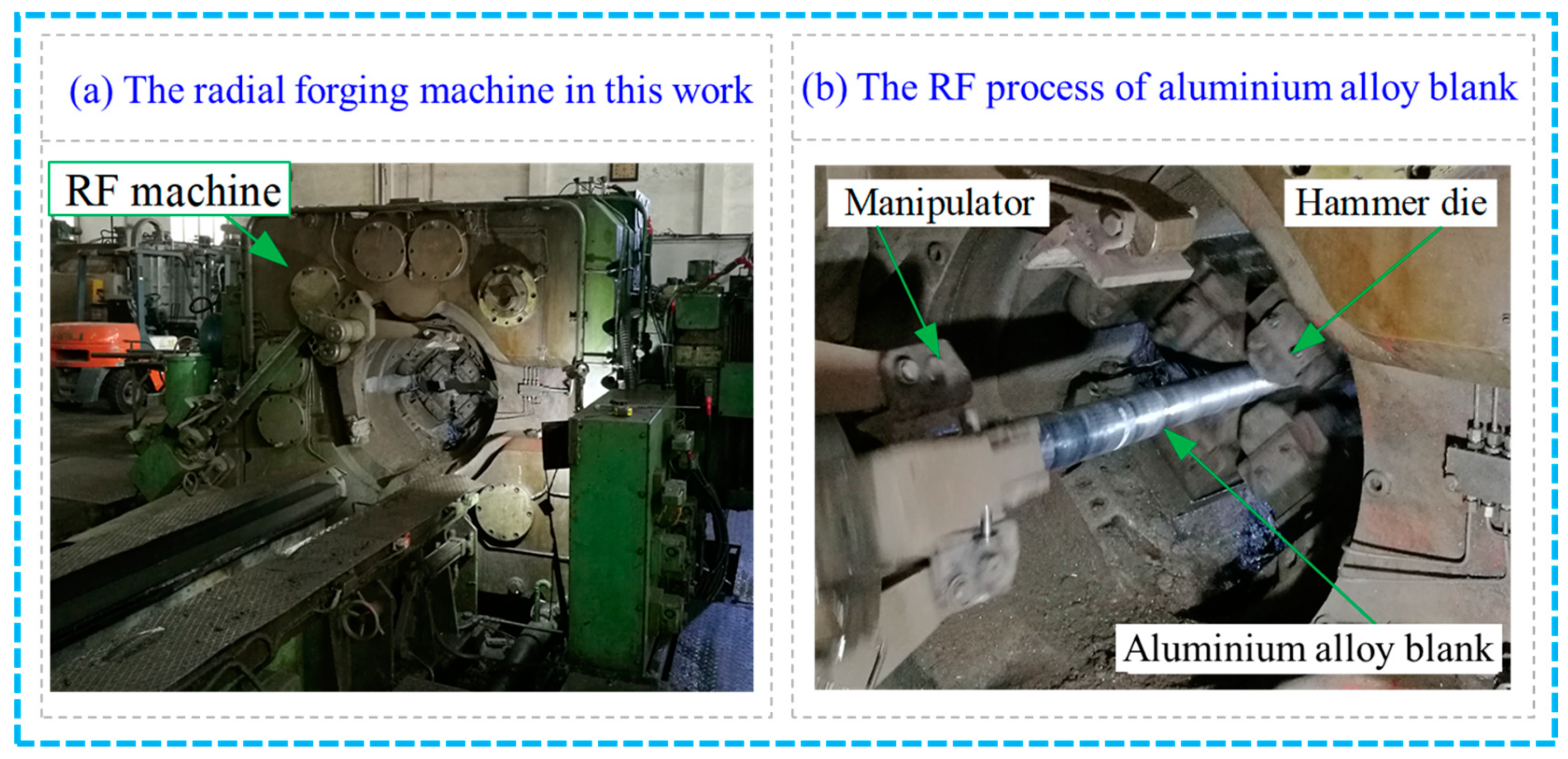




| Si | Fe | Cu | Mn | Mg | Zn | Ti | Cr | Al |
|---|---|---|---|---|---|---|---|---|
| 0.38 | 0.15 | 0.023 | 0.018 | 0.65 | 0.02 | <0.1 | <0.1 | Bal. |
| Constant Process Parameters | Specific Values | |
|---|---|---|
| Dimension of the starting material | A commercial 6063 wrought aluminium extruded bar with 100 mm in diameter and 1000 mm in length under T6 state | |
| Radial reduction value | 10 mm/stroke | |
| Frequency for RF | 580 stroke/min | |
| Feed speed of hammer die | 50 mm/s | |
| Feed speed of blank | 580 mm/min | |
| Rotation speed of blank | 19.3 r/min | |
| Relative feed rate | 1 mm/stroke | |
| Relative angle of rotation | 12 °/stroke | |
| Variable process parameters | ||
| Group No. | FR (RF-deformed diameter) | Blank temperature |
| 1 | 19% (90 mm), 36% (80 mm), 51% (70 mm), 64% (60 mm), 75% (50 mm) | 25 °C |
| 2 | 75% (50 mm) | 25 °C, 150 °C, 300 °C, 450 °C |
| Process Method | AGS at the Edge Position (μm) | AGS at the Centre Position (μm) | Difference | Improvement Ratio |
|---|---|---|---|---|
| IHT of the starting material | 294.39 | 480.82 | 186.43 | 91.19% |
| IHT of the RF-deformed blank | 64.10 | 79.19 | 15.09 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Xiong, L.; Feng, D.; Zhao, S.; Guo, Y. Investigation of the Penetration Performance of the Radial Forging Process for Wrought Aluminium Alloy. Materials 2024, 17, 2065. https://doi.org/10.3390/ma17092065
Wang Y, Xiong L, Feng D, Zhao S, Guo Y. Investigation of the Penetration Performance of the Radial Forging Process for Wrought Aluminium Alloy. Materials. 2024; 17(9):2065. https://doi.org/10.3390/ma17092065
Chicago/Turabian StyleWang, Yongfei, Linhua Xiong, Dongxiao Feng, Shengdun Zhao, and Yi Guo. 2024. "Investigation of the Penetration Performance of the Radial Forging Process for Wrought Aluminium Alloy" Materials 17, no. 9: 2065. https://doi.org/10.3390/ma17092065
APA StyleWang, Y., Xiong, L., Feng, D., Zhao, S., & Guo, Y. (2024). Investigation of the Penetration Performance of the Radial Forging Process for Wrought Aluminium Alloy. Materials, 17(9), 2065. https://doi.org/10.3390/ma17092065










