Analytical Model of Temperature-Induced Deformation for Tunable Thermal Expansion Metamaterial
Abstract
:1. Introduction
2. Models and Methods
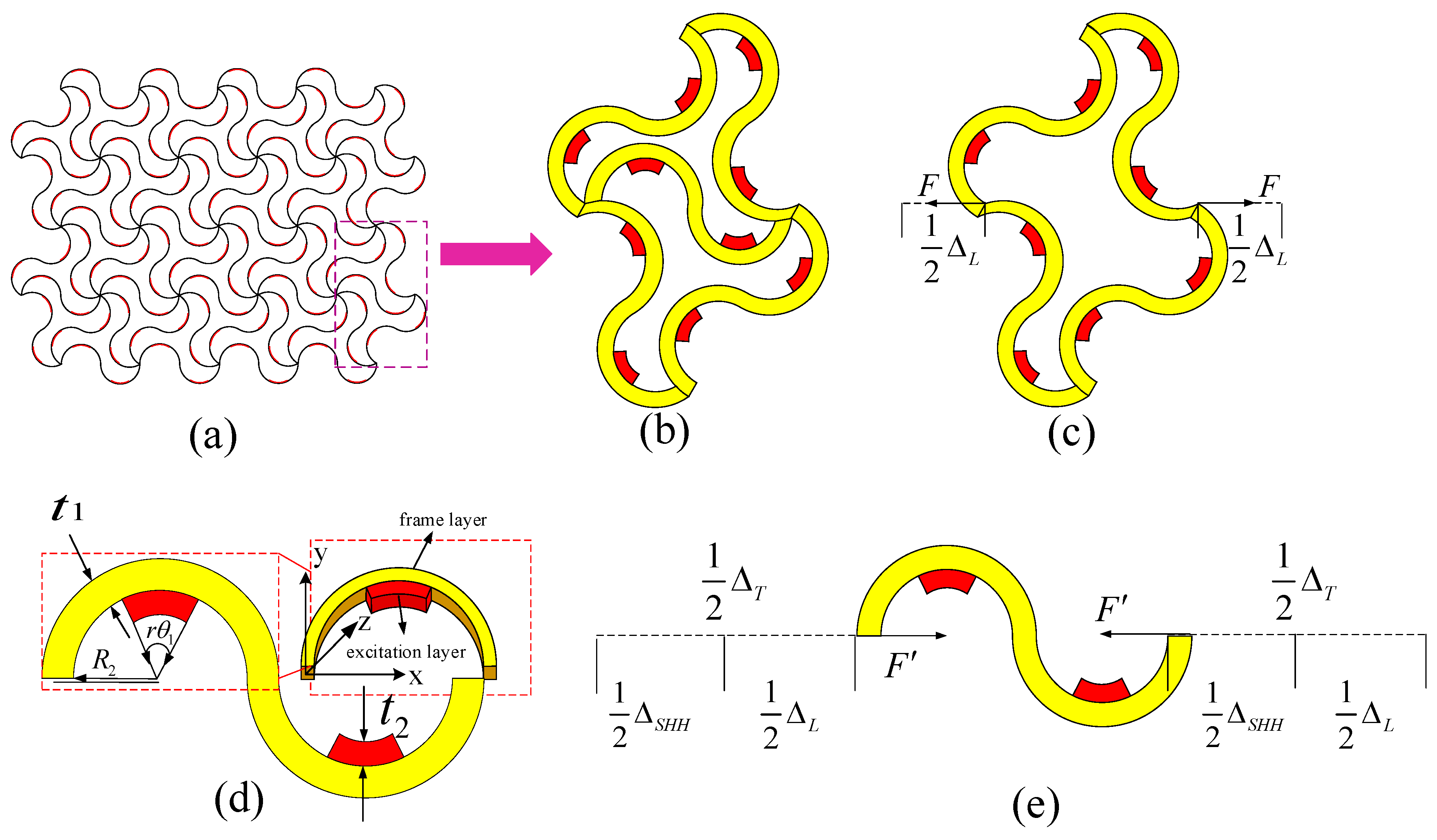
2.1. Geometry of the TTEM
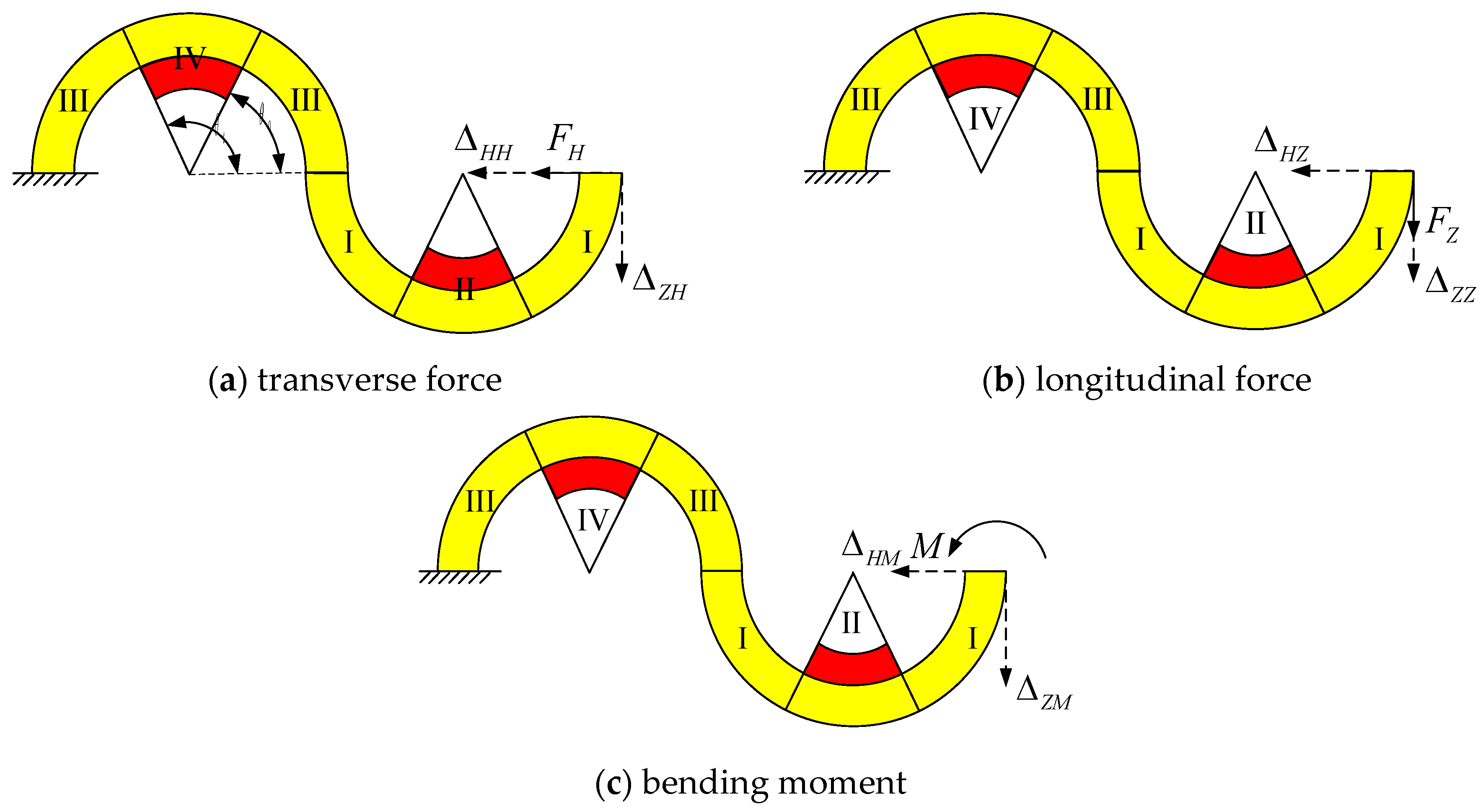
2.2. Displacement Functions for the Bi-Material Serpentine Unit
2.3. Displacement Functions for the Lattice Structure
2.4. Deformation Coordination Relationship for the TTEM
3. Results and Discussion
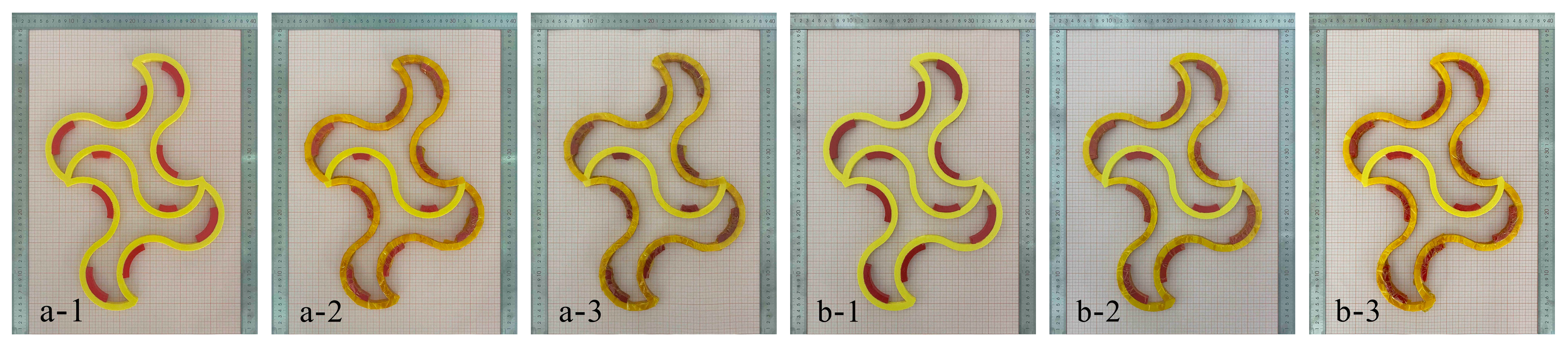
3.1. Material Preparation
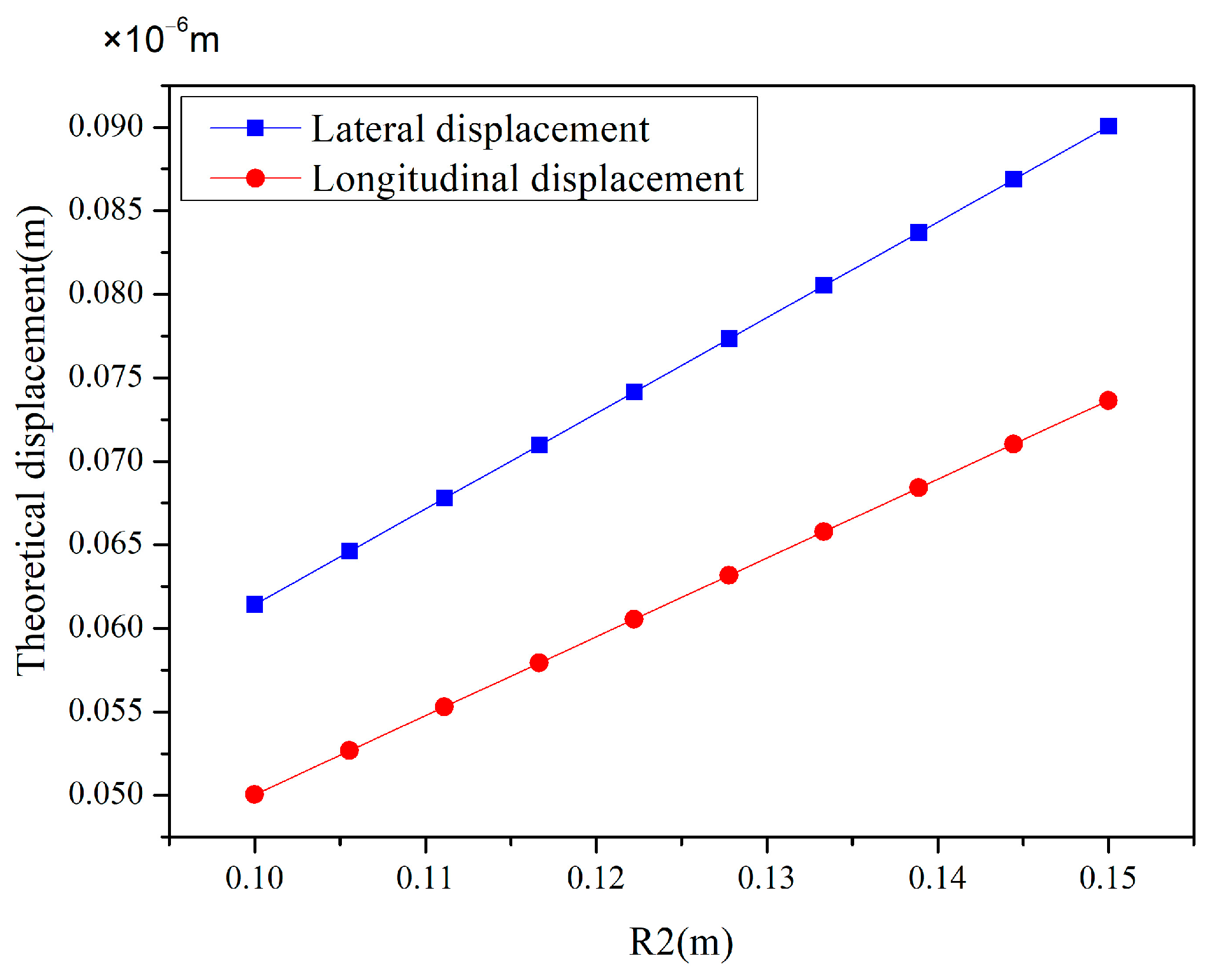
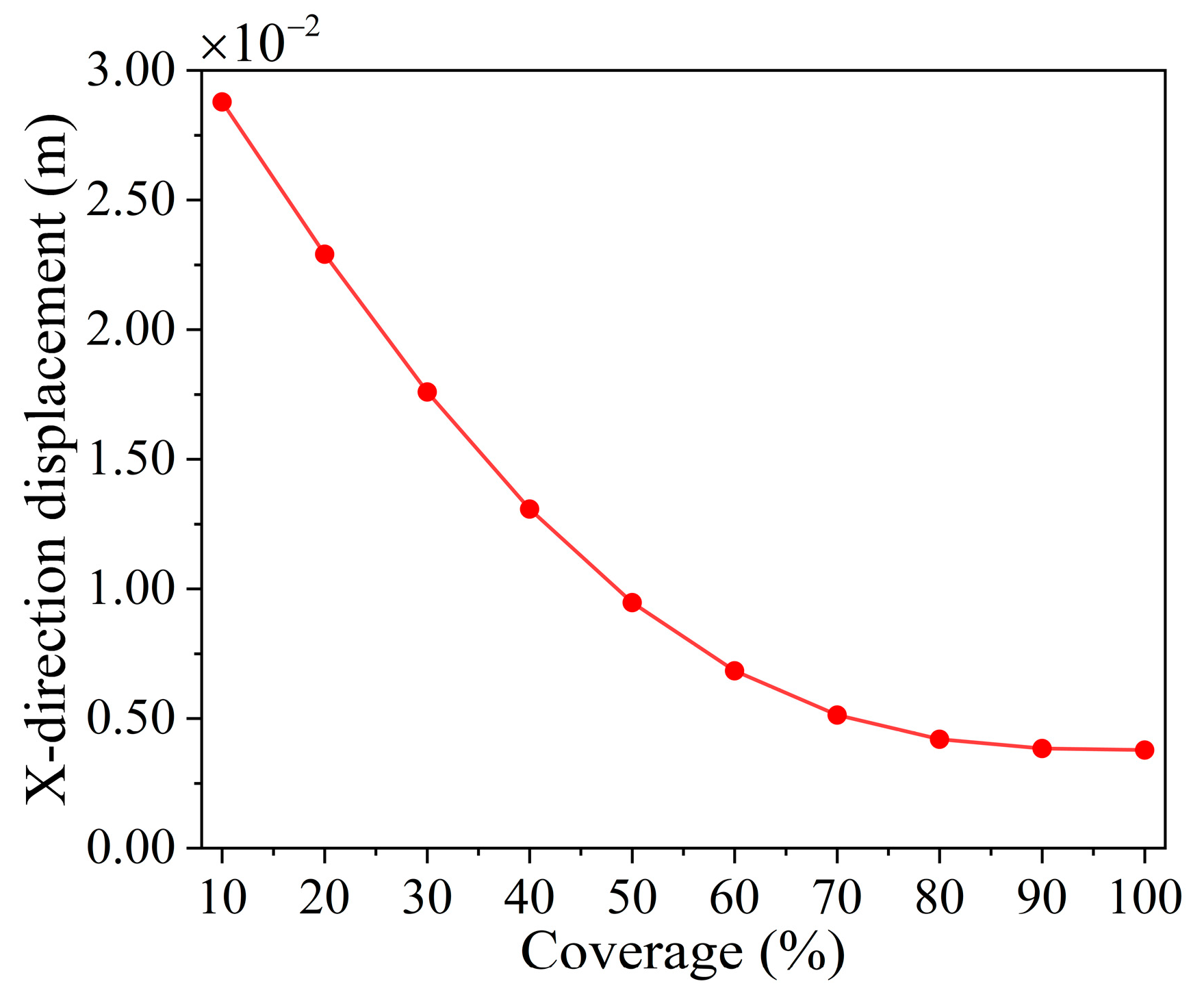
3.2. Deformation Coordination Relationship
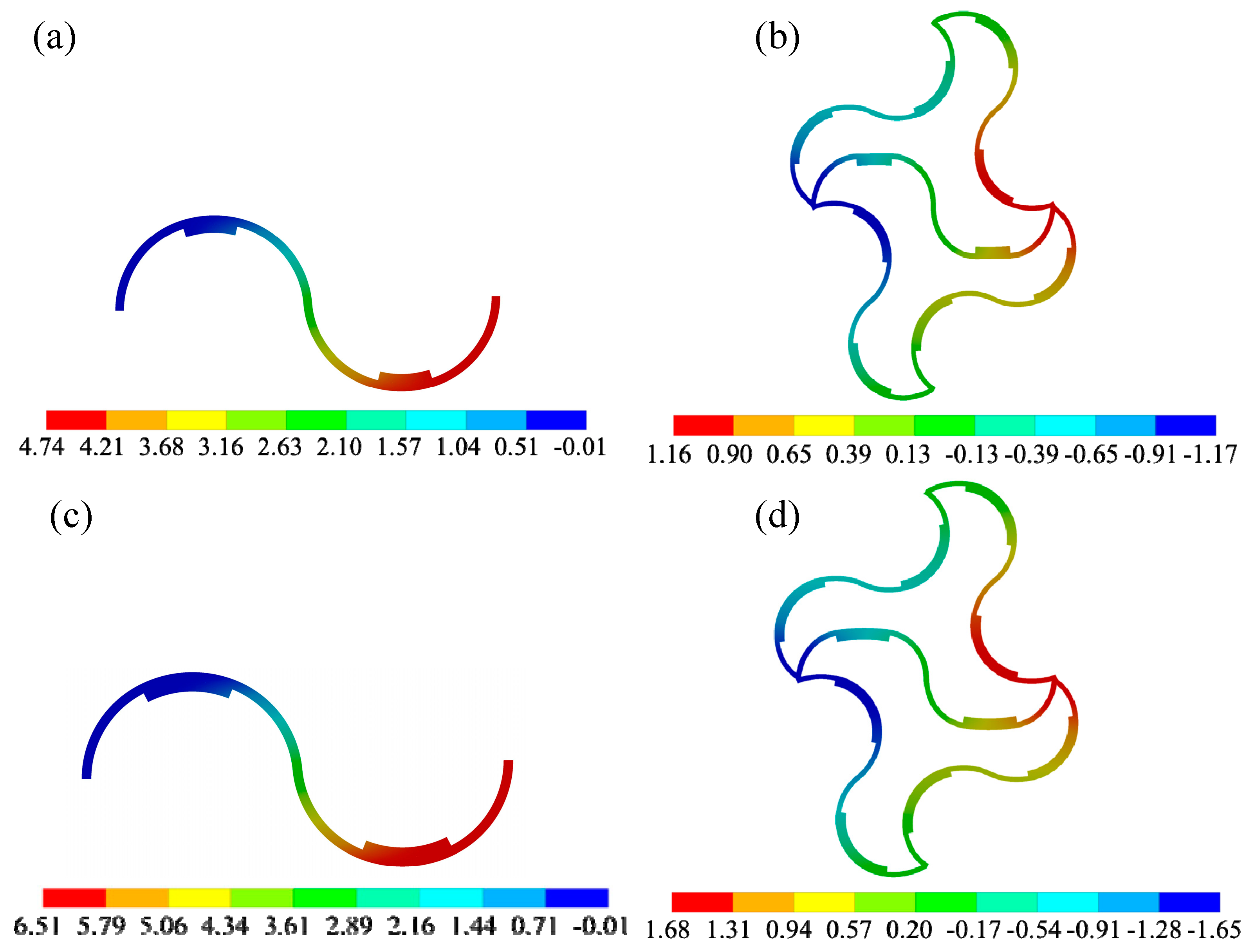
3.3. Simulation Verification
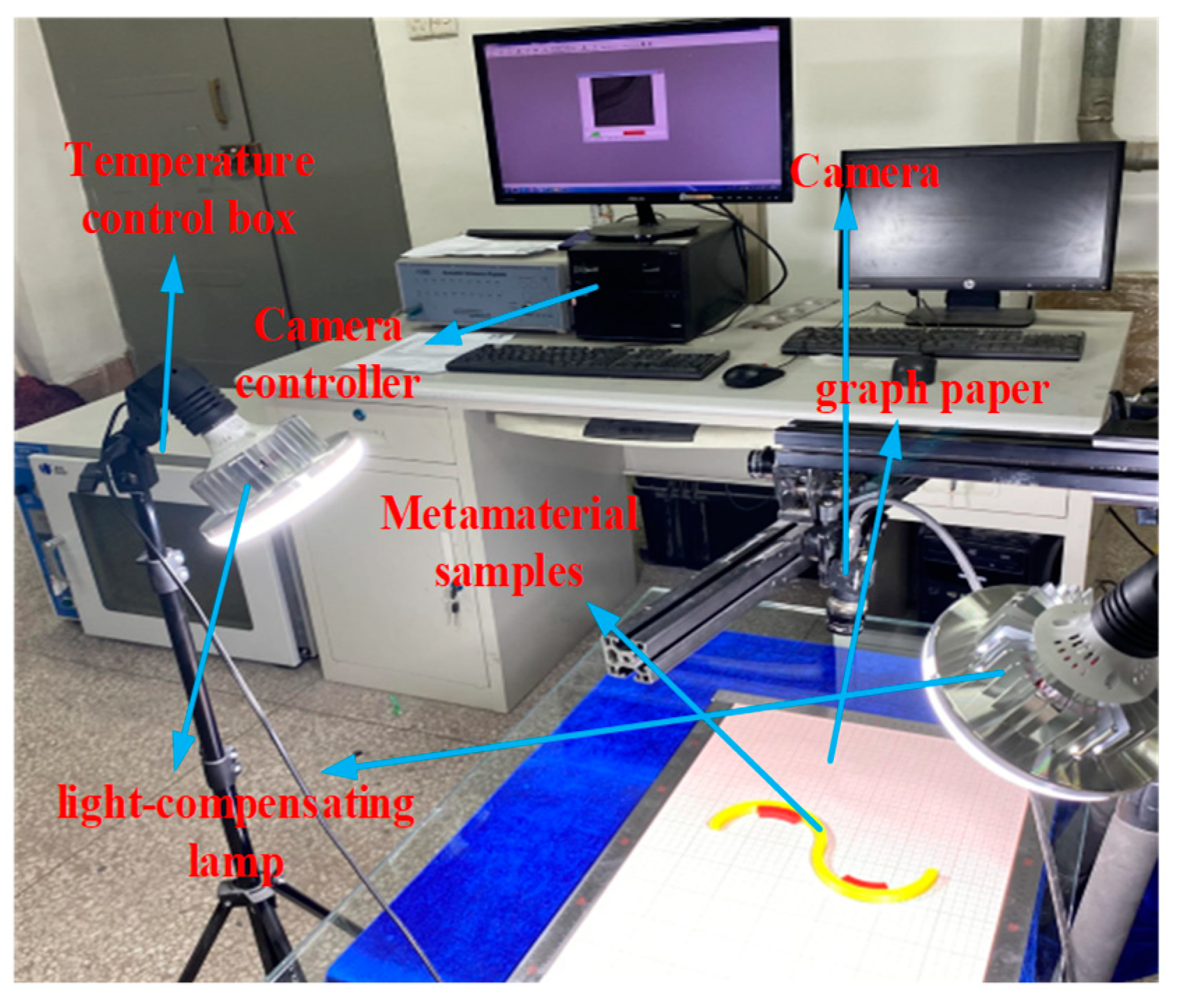
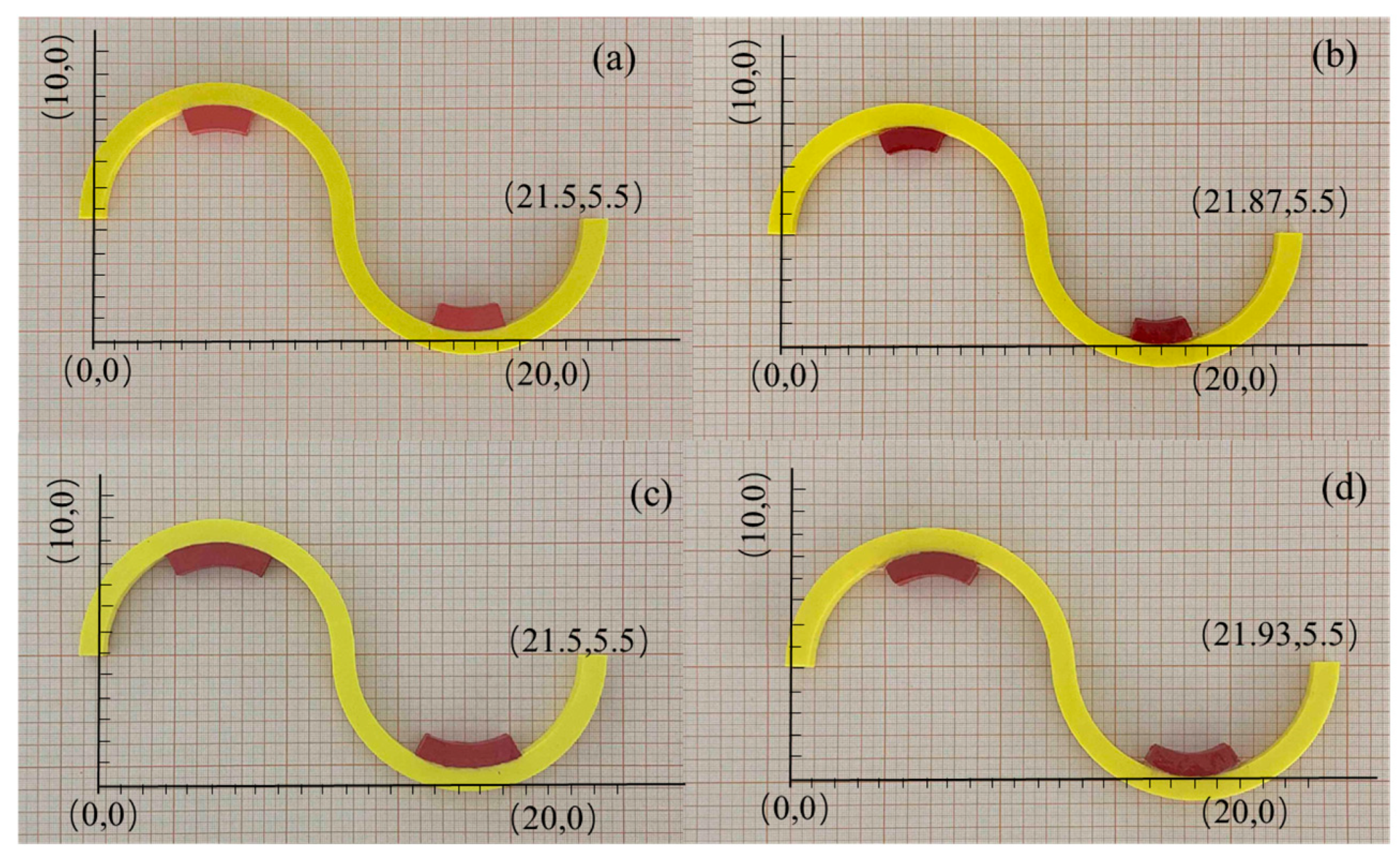
3.4. Experimental Verification
4. Conclusions
- Displacement flexibility functions for the serpentine units and lattice structure within tunable thermal expansion metamaterials are formulated using the principle of virtual work and the method of superposition.
- It is discovered that the internal serpentine unit and the external lattice structure exhibit equivalent flexibility when the final deformation of the serpentine unit is half of its temperature-induced deformation. Based on this finding, this study presents a method for analyzing the deformation coordination relationship between the lattice and serpentine unit coverage rates in metamaterials.
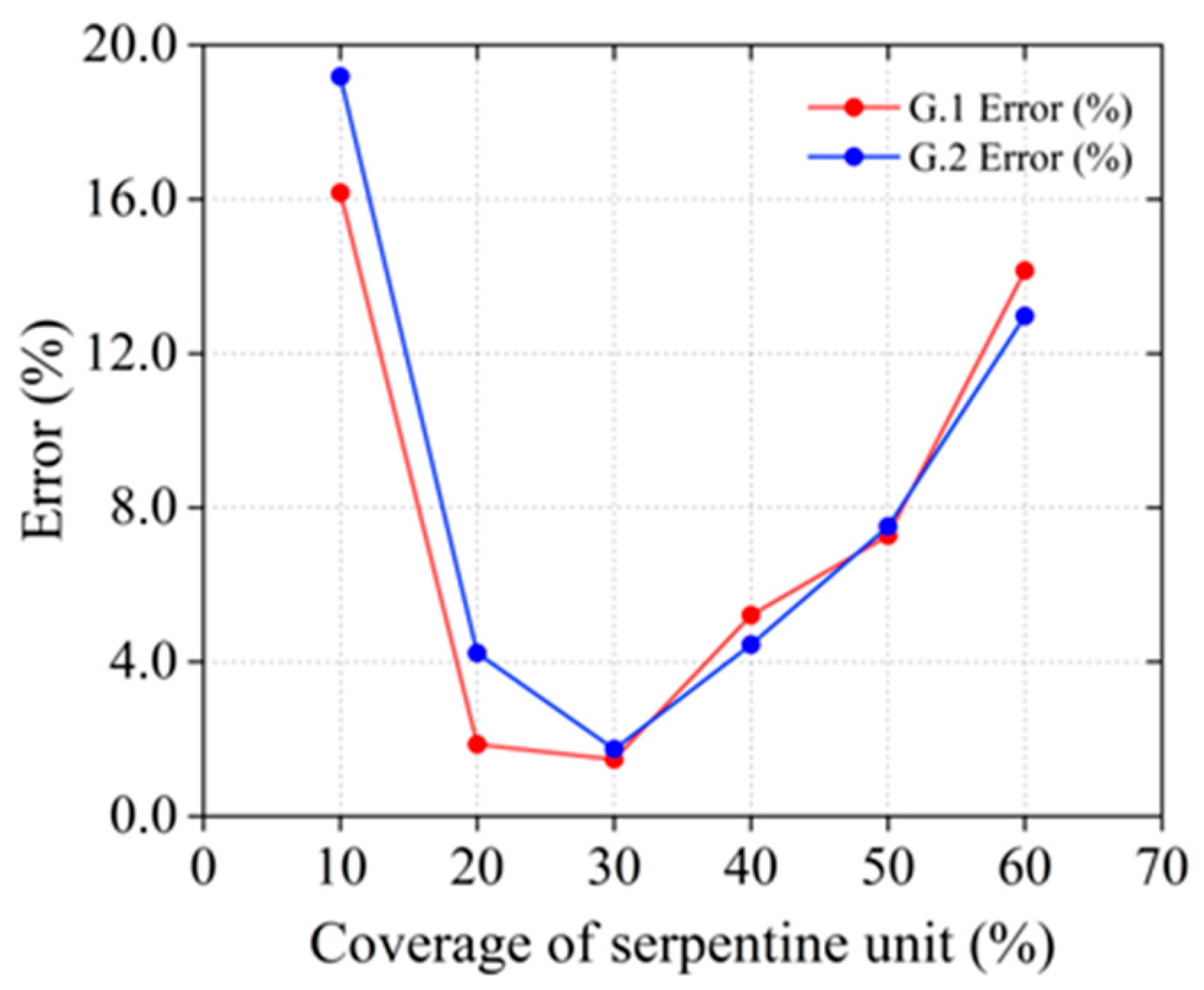
- The proposed deformation coordination relationship is validated through finite element analysis and experimental methods. With an internal unit coverage rate of 30% and an external lattice structure coverage rate of 52.06%, the prediction error is maintained within 1.47%. These research outcomes provide a theoretical foundation and design guidance for precise temperature-induced deformation control of bi-material serpentine network structural units.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, T.; Deng, R.; Zhang, Y.; Yang, J.; Cai, J. Composite negative stiffness structure with tunable and temperature-dependent properties induced by viscoelastic and shape-memory materials. Compos. Commun. 2024, 48, 101937. [Google Scholar] [CrossRef]
- Liu, S.; Li, F.; Peng, J.; Zhang, X. Universal model describing the negative thermal expansion coefficients of bending-type two-dimensional metamaterials with chiral/anti-chiral structures. Compos. Commun. 2023, 39, 101559. [Google Scholar] [CrossRef]
- Wei, K.; Peng, Y.; Wang, K.; Duan, S.; Yang, X.; Wen, W. Three dimensional lightweight lattice structures with large positive, zero and negative thermal expansion. Compos. Struct. 2018, 188, 287–296. [Google Scholar] [CrossRef]
- He, X.; Yu, J.; Xie, Y. Bi-material re-entrant triangle cellular structures incorporating tailorable thermal expansion and tunable Poisson’s ratio. J. Mech. Robot. 2019, 11, 061003. [Google Scholar] [CrossRef]
- Wei, K.; Peng, Y.; Qu, Z.; Duan, S.; Yang, X.; Wen, W. Lightweight composite lattice cylindrical shells with novel character of tailorable thermal expansion. Int. J. Mech. Sci. 2018, 137, 77–85. [Google Scholar] [CrossRef]
- Timoshenko, S. Analysis of bi-metal thermostats. Josa 1925, 11, 233–255. [Google Scholar] [CrossRef]
- Lakes, R. Cellular solid structures with unbounded thermal expansion. J. Mater. Sci. Lett. 1996, 15, 475–477. [Google Scholar] [CrossRef]
- Lakes, R. Cellular solids with tunable positive or negative thermal expansion of unbounded magnitude. Appl. Phys. Lett. 2007, 90, 359. [Google Scholar] [CrossRef]
- Zheng, B.B.; Fu, M.H.; Li, W.H.; Hu, L.L. A novel re-entrant honeycomb of negative thermal expansion. Smart Mater. Struct. 2018, 27, 085005. [Google Scholar] [CrossRef]
- Peng, X.L.; Bargmann, S. A novel hybrid-honeycomb structure: Enhanced stiffness, tunable auxeticity and negative thermal expansion. Int. J. Mech. Sci. 2021, 190, 106021. [Google Scholar] [CrossRef]
- Peng, Y.; Wei, K.; Mei, M.; Yang, X.; Fang, D. Simultaneously program thermal expansion and Poisson’s ratio in three dimensional mechanical metamaterial. Compos. Struct. 2021, 262, 113365. [Google Scholar] [CrossRef]
- Liu, H.T.; Xu, N.; Wang, Y.B.; Wang, L. Three-dimensional enhanced star-shaped honeycombs with negative thermal expansion. Compos. Struct. 2022, 279, 114772. [Google Scholar] [CrossRef]
- Liu, K.J.; Liu, H.T.; Zhen, D. Mechanical and bandgap properties of 3D bi-material triangle re-entrant honeycomb. Int. J. Mech. Sci. 2024, 261, 108664. [Google Scholar] [CrossRef]
- Giannopoulos, G.I.; Georgantzinos, S.K. A tunable metamaterial joint for mechanical shock applications inspired by carbon nanotubes. Appl. Sci. 2021, 11, 11139. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, X.; Shen, Z.; Zhang, Y. Imperfection sensitivity of mechanical properties in soft network materials with horseshoe microstructures. Acta Mech. Sin. 2021, 37, 1050–1062. [Google Scholar] [CrossRef]
- Liu, G.; Zheng, Z.; Zhao, R.; Yue, X. Horseshoe Lattice Property-Structure Inverse Design Based on Deep Learning. Mater. Trans. 2024, 65, 308–317. [Google Scholar] [CrossRef]
- Zhou, J.; Chang, J.; Song, X.; Zhang, Y. Bio-inspired design and unusual mechanical properties of 3D horseshoe-shaped soft network metamaterials. Compos. Part B Eng. 2024, 275, 111284. [Google Scholar] [CrossRef]
- Widlund, T.; Yang, S.; Hsu, Y.Y.; Lu, N. Stretchability and compliance of freestanding serpentine-shaped ribbons. Int. J. Solids Struct. 2014, 51, 4026–4037. [Google Scholar] [CrossRef]
- Chen, J.; Wang, H.; Wang, K.; Wei, Z.; Xu, W.; Wei, K. Mechanical performances and coupling design for the mechanical metamaterials with tailorable thermal expansion. Mech. Mater. 2022, 165, 104176. [Google Scholar] [CrossRef]
- Zhang, Q.; Sun, Y. Novel metamaterial structures with negative thermal expansion and tunable mechanical properties. Int. J. Mech. Sci. 2024, 261, 108692. [Google Scholar] [CrossRef]
- Li, J.; Liu, H.T.; Zhang, Z.Y. Stiffness characteristics for bi-directional tunable thermal expansion metamaterial based on bi-material triangular unit. Int. J. Mech. Sci. 2023, 241, 107983. [Google Scholar] [CrossRef]
- Liu, J.; Yan, D.; Zhang, Y. Mechanics of unusual soft network materials with rotatable structural nodes. J. Mech. Phys. Solids 2021, 146, 104210. [Google Scholar] [CrossRef]
- Zhang, X.; Han, Y.; Zhu, M.; Chu, Y.; Li, W.; Zhang, Y.; Zhang, Y.; Luo, J.; Tao, R.; Qi, J. Bio-inspired 4D printed intelligent lattice metamaterials with tunable mechanical property. Int. J. Mech. Sci. 2024, 272, 109198. [Google Scholar] [CrossRef]
- Han, Z.; Xiao, X.; Chen, J.; Wei, K.; Wang, Z.; Yang, X.; Fang, D. Bifunctional metamaterials incorporating unusual geminations of Poisson’s ratio and coefficient of thermal expansion. ACS Appl. Mater. Interfaces 2022, 14, 50068–50078. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Jiang, W.Z.; Pan, Y.; Teng, X.C.; Xu, H.H.; Yan, H.; Ni, X.H.; Dong, J.; Han, D.; Chen, W.Q.; et al. Temperature-responsive metamaterials made of highly sensitive thermostat metal strips. Sci. Adv. 2024, 10, eads0892. [Google Scholar] [CrossRef]
- Hernández-Acosta, M.A.; Martines-Arano, H.; Soto-Ruvalcaba, L.; Martínez-González, C.L.; Martínez-Gutiérrez, H.; Torres-Torres, C. Fractional thermal transport and twisted light induced by an optical two-wave mixing in single-wall carbon nanotubes. Int. J. Therm. Sci. 2020, 147, 106136. [Google Scholar] [CrossRef]
- Wang, D.; Xu, H.; Wang, J.; Jiang, C.; Zhu, X.; Ge, Q.; Gu, G. Design of 3D printed programmable horseshoe lattice structures based on a phase-evolution model. ACS Appl. Mater. Interfaces 2020, 12, 22146–22156. [Google Scholar] [CrossRef] [PubMed]
- Ni, X.; Guo, X.; Li, J.; Huang, Y.; Zhang, Y.; Rogers, J.A. 2D mechanical metamaterials with widely tunable unusual modes of thermal expansion. Adv. Mater. 2019, 31, 1905405. [Google Scholar] [CrossRef]

| Coverage of the Serpentine Unit (%) | Lattice Structure Coverage (%) | Temperature Deformation of the Serpentine Unit (mm) | Simulation Value of the Final Deformation of the Serpentine Unit (mm) | Analytic Model of the Final Deformation of the Serpentine Unit (mm) | |||
|---|---|---|---|---|---|---|---|
| G.1 | G.2 | G.1 | G.2 | G.1 | G.2 | ||
| 10 | 36.97 | 2.93 | 2.88 | 1.26 | 1.16 | 1.47 | 1.44 |
| 20 | 44.16 | 4.74 | 3.78 | 2.33 | 1.81 | 2.37 | 1.89 |
| 30 | 52.06 | 6.51 | 4.67 | 3.30 | 2.29 | 3.26 | 2.34 |
| 40 | 61.62 | 8.18 | 5.51 | 4.32 | 2.85 | 4.09 | 2.75 |
| 50 | 74.16 | 9.62 | 6.53 | 5.19 | 3.35 | 4.81 | 3.11 |
| 57.24 | 100 | 10.60 | 6.71 | 4.64 | 2.92 | 5.30 | 3.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, L.; Yao, Y.; Chen, S.; Lai, M.; Zhu, G. Analytical Model of Temperature-Induced Deformation for Tunable Thermal Expansion Metamaterial. Materials 2025, 18, 532. https://doi.org/10.3390/ma18030532
Xiao L, Yao Y, Chen S, Lai M, Zhu G. Analytical Model of Temperature-Induced Deformation for Tunable Thermal Expansion Metamaterial. Materials. 2025; 18(3):532. https://doi.org/10.3390/ma18030532
Chicago/Turabian StyleXiao, Ling, Yaxin Yao, Shuai Chen, Mengting Lai, and Guanghong Zhu. 2025. "Analytical Model of Temperature-Induced Deformation for Tunable Thermal Expansion Metamaterial" Materials 18, no. 3: 532. https://doi.org/10.3390/ma18030532
APA StyleXiao, L., Yao, Y., Chen, S., Lai, M., & Zhu, G. (2025). Analytical Model of Temperature-Induced Deformation for Tunable Thermal Expansion Metamaterial. Materials, 18(3), 532. https://doi.org/10.3390/ma18030532






