Study on Damage Mechanism of Fiber Concrete with Initial Pores
Abstract
:1. Introduction
2. The Establishment of Two-Dimensional Mesoscopic Model
2.1. Aggregate Grading Theory and Its Generation Criteria
- (1)
- It must accurately reflect the gradation of aggregates.
- (2)
- The size, geometry, and positioning of the aggregates should be random and uniformly distributed, and the size and distribution of pores must also be random.
- (1)
- Input the characteristic parameters of the model, including the sample size of the concrete, and proceed to mesh the specimen. Subsequently, determine the aggregates’ particle sizes and input the percentage of each particle size following the grading curve.
- (2)
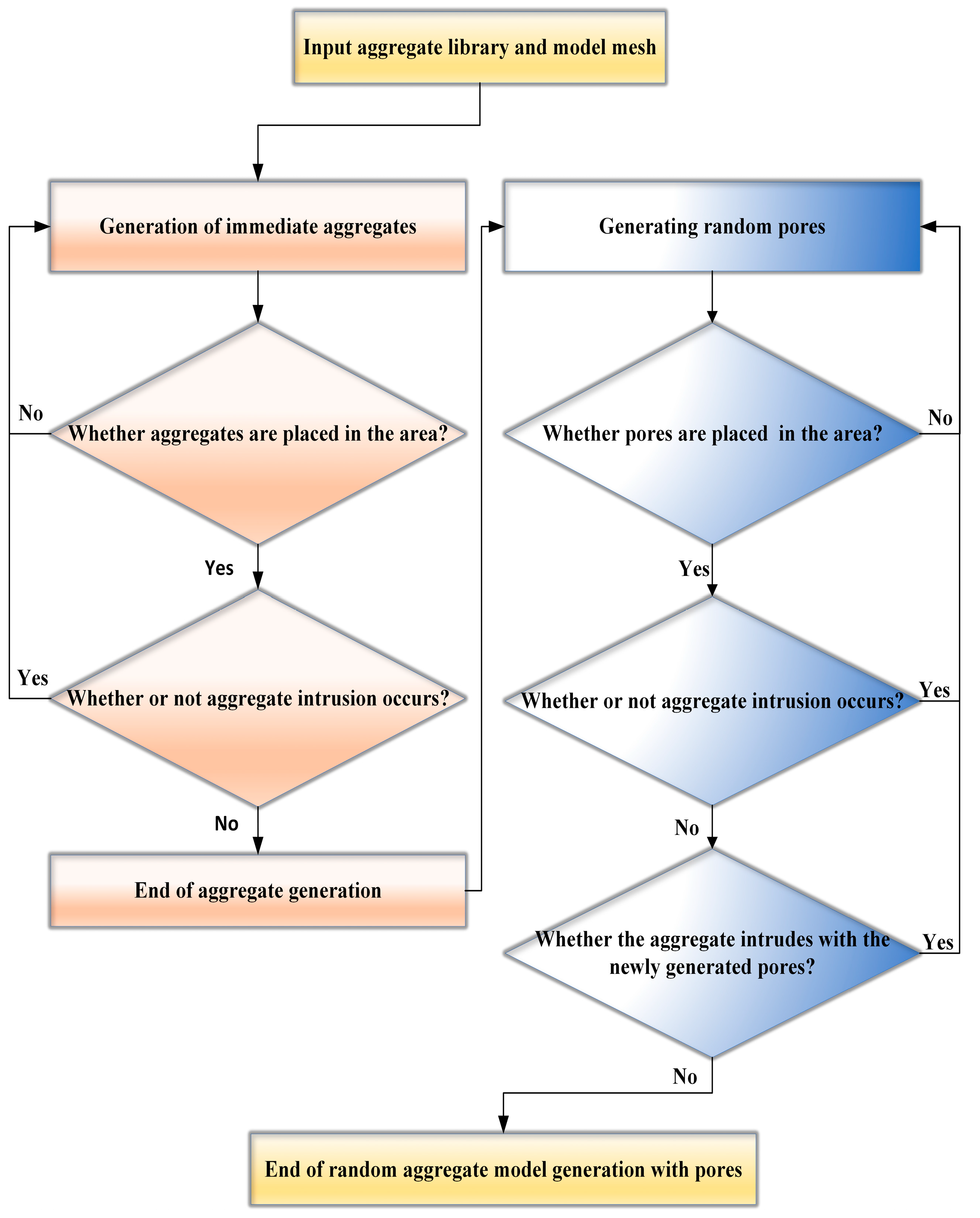
- The generation of random aggregates begins with the assignment of random locations for the aggregates, which are primarily governed by the coordinates of a randomly selected geometric center point. Following this, the random generation function in MATLAB is employed to produce a substantial number of random point coordinates within the designated simulation area. For each generated aggregate, it is essential to verify whether it overlaps with any previously generated aggregates or extends beyond the simulation boundaries. Overlap detection can be accomplished by calculating the distance between two spheres; if this distance is less than the sum of their respective radii, overlap is deemed to occur. For instance, if the coordinates of the centers of the spheres are (x1, y1, z1) and (x2, y2, z2) with radii r1 and r2, the overlap is confirmed if the following condition is satisfied.
- (3)
- Generation of random pores. Similar to the generation of the aggregates, random points are generated in the pore space, which are used as the intermediate positions of the pores. It is essential to assess whether these newly generated pores intersect with the previously established aggregates and other pores. This judgment is divided into the judgment of pore and aggregate and the judgment of intrusion between pores. In pore generation, the first step is to complete the contact judgement between the new pore and the old aggregate. If there is no contact, then continue to judge whether the newly generated pore and the old generated pore are in contact with each other. If not, the pore generation is successful. If the contact is found, then return to the initial step to re-generate the pore. The specific process is shown in Figure 1 below:
2.2. Fiber-Reinforced Concrete Constitutive Equation
2.3. Material Parameters
2.4. Modeling
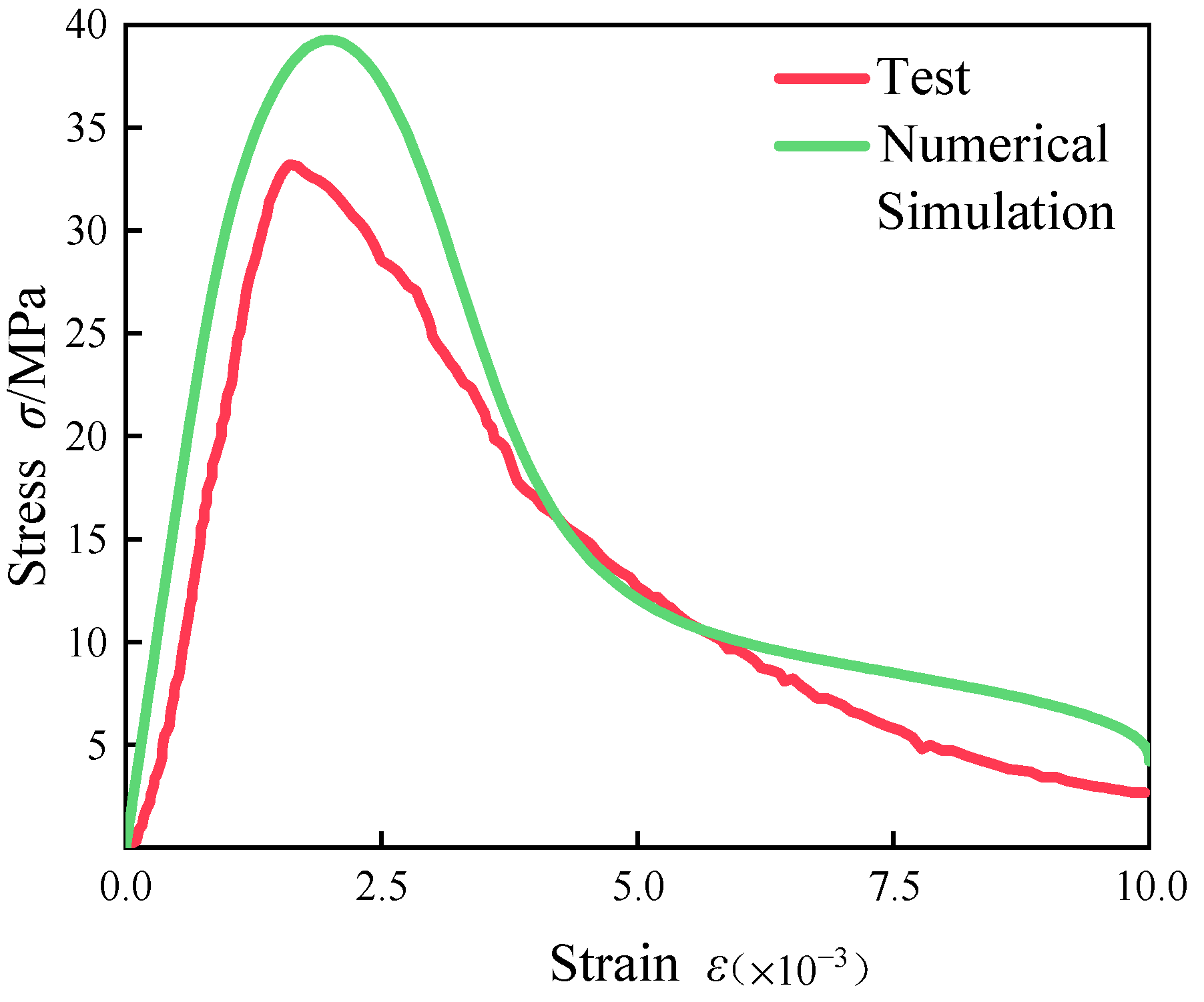
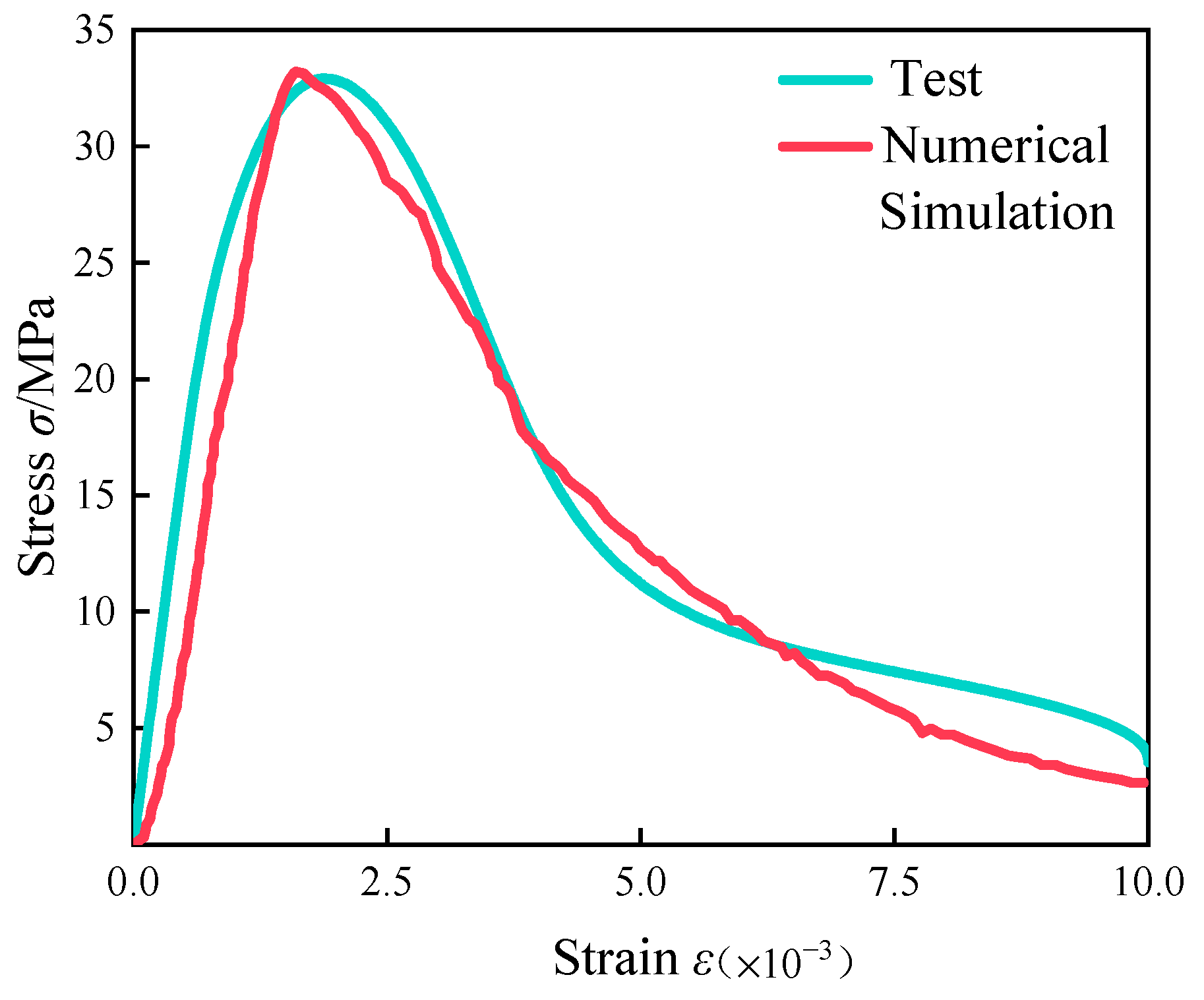
2.5. Finite Element Validation and Curve Fitting
3. Simulation Results
3.1. Mechanical Properties of Fiber Concrete with Different Porosities
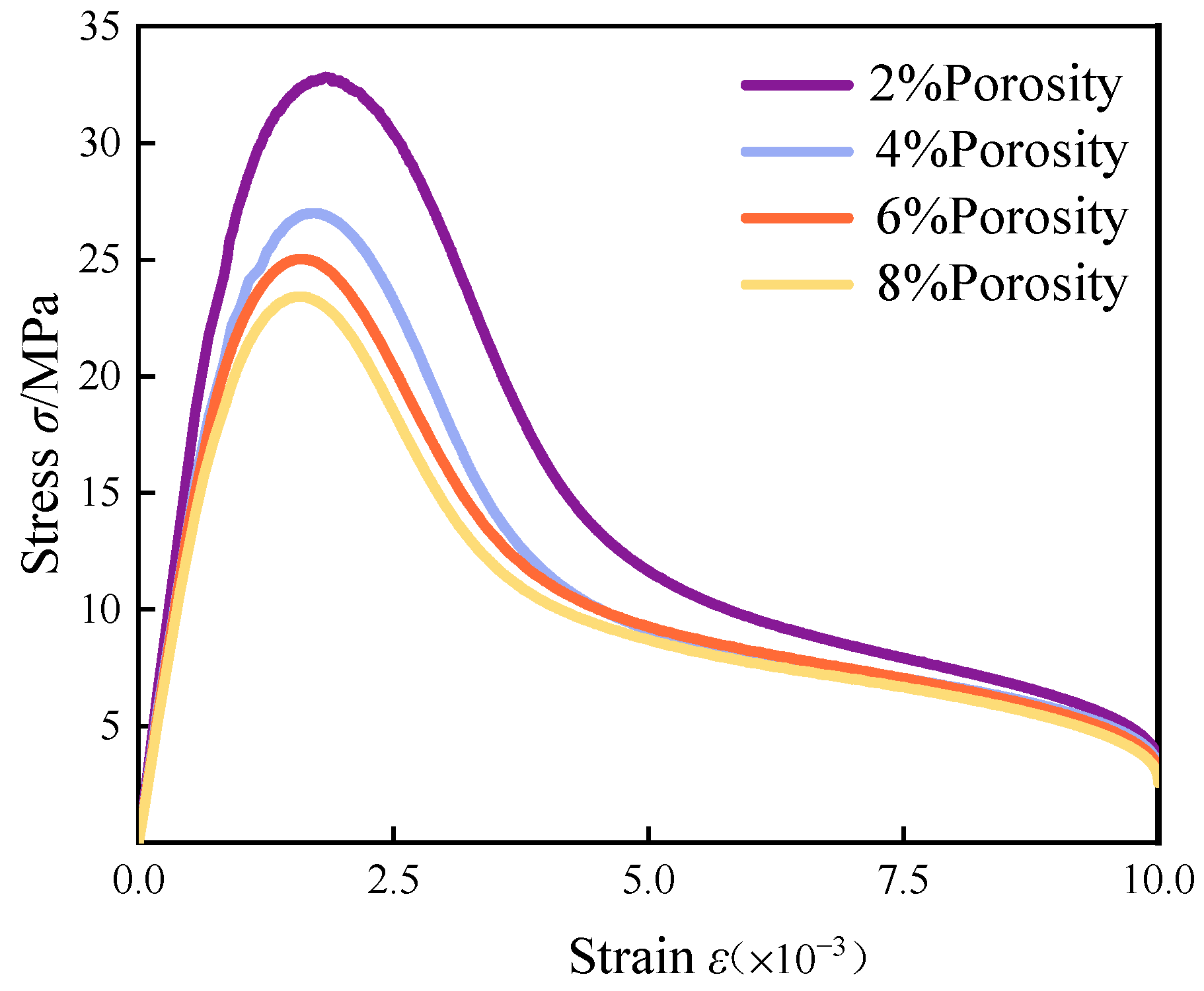
3.1.1. Stress–Strain Curve
3.1.2. Displacement Distribution
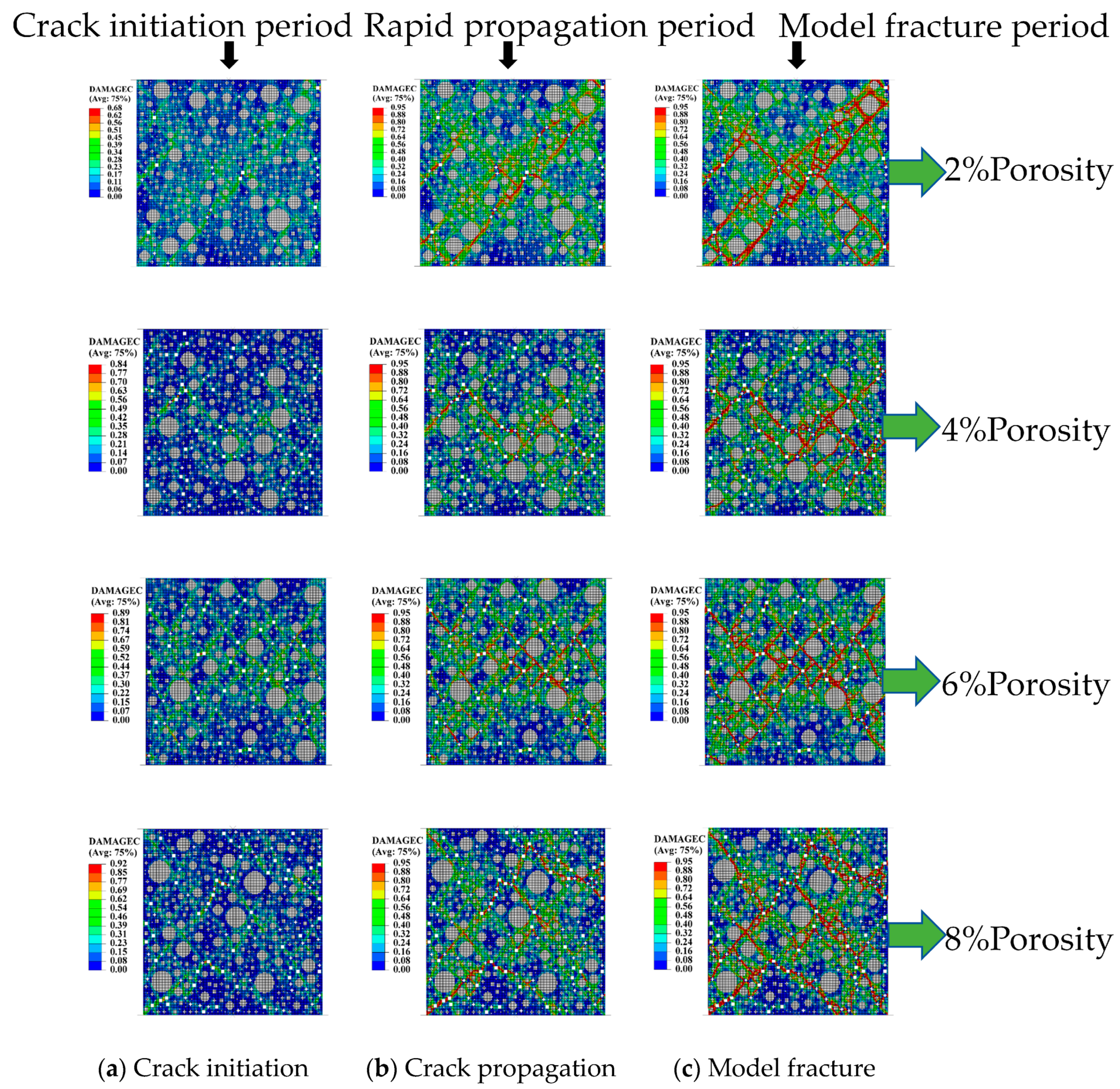
3.1.3. Macroscopic Crack Development Process
- (1)
- The initial stage, known as crack initiation, as shown in column a, typically occurs at the material’s surface or at sites of internal stress concentration. During this phase, a small amount of unit damage occurred at localized locations of stress concentrations. Multiple microcracks were produced at the edges of several pores. Cracks tended to form around pores, and interface layers began to emerge.
- (2)
- The subsequent stage is characterized as stable expansion, as shown in column b. During this period, the crack gradually developed to the main crack region, causing localized damage, and the unit damage was concentrated near the main crack region. The main crack sprouted a large number of small cracks, the number of damage units increased rapidly, and the length and width of the main crack region increased.
- (3)
- The final stage, referred to as destabilized expansion, was marked by a rapid increase in crack length, leading to a swift and irreversible process that culminated in the formation of a macroscopic penetration crack, ultimately resulting in the specimen’s inability to sustain load. These observations align with the experimental results obtained in column c.
3.2. Uniaxial Compression Damage Analysis Under Different Loading Rates
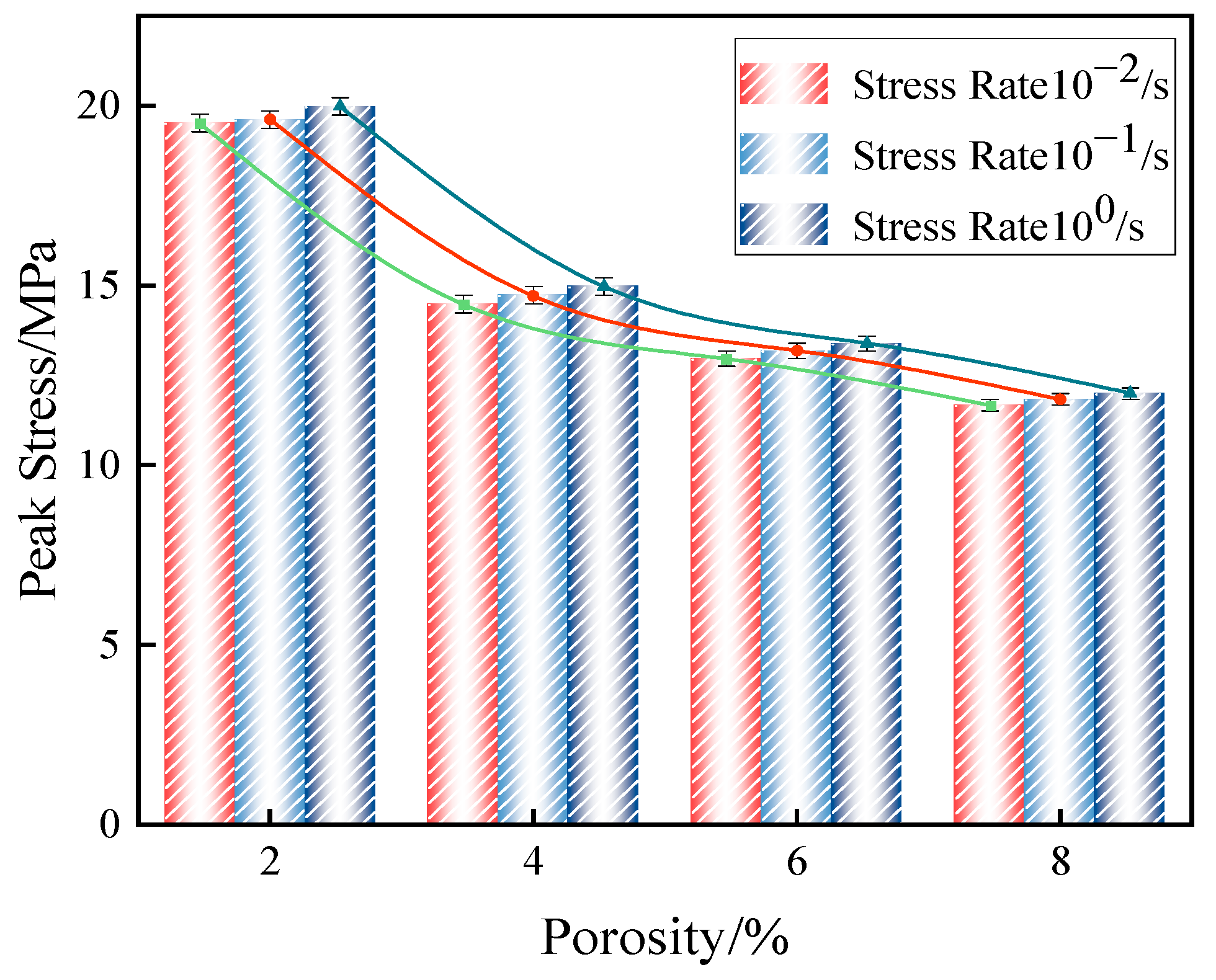
3.2.1. Peak Stress Analysis
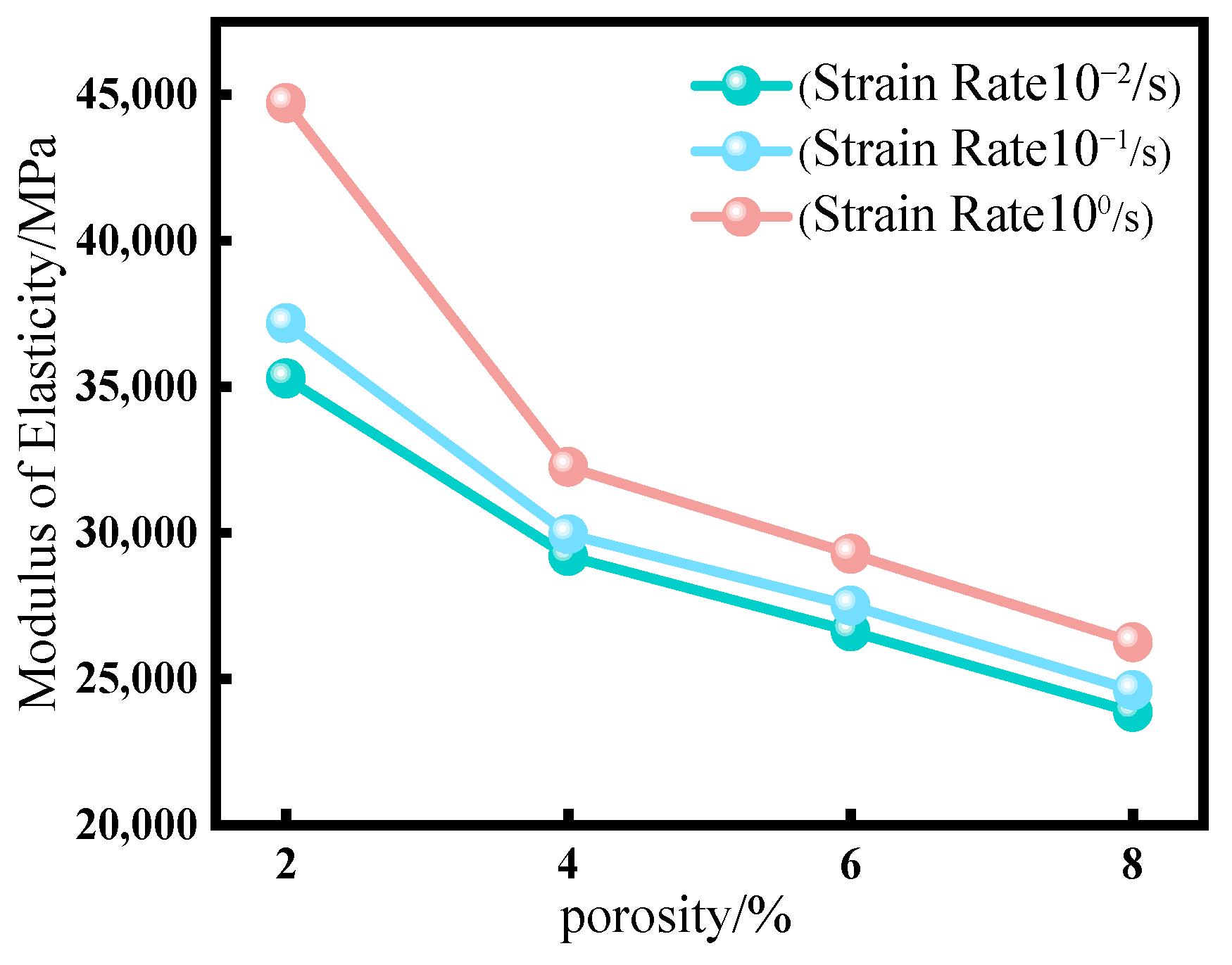
3.2.2. Modulus of Elasticity Analysis
4. Discussion
5. Conclusions
- (1)
- After modifying the constitutive equation using the least squares method, the numerical simulation results showed significant improvement compared to previous results and were in good agreement with experimental data. The error in peak stress between the numerical simulation and physical test was reduced from 18% to about 1%. This result validates the rationality of the modified constitutive equation.
- (2)
- The uniaxial compression calculation results of fiber-reinforced concrete with different porosities indicated that with the increase in porosity, the vertical displacement also increased from 1.374 mm at the time of destruction to 1.598 mm. The growth rate was as high as 16.3% during this period. The peak stress and elastic modulus of fiber-reinforced concrete with initial porosity gradually decreased as porosity increased. The peak stresses were 98.6%, 81.3%, 75.4%, and 70.5% for nonporous concrete. This characteristic was consistent with the performance of the fiber-reinforced concrete’s mechanical properties during construction and concrete production.
- (3)
- In the damage evolution process of cracks, it was found that concrete with a high porosity exhibited a wider and greater number of crack distributions upon failure. In contrast, concrete with a low porosity had a more concentrated stress distribution and fewer cracks compared to high-porosity concrete. This result can provide a basis for structural safety in engineering, ensuring the long-term safety of buildings.
- (4)
- After considering the combined effects of strain rate and porosity, it was found that the strain rate significantly affected the elastic modulus of fiber-reinforced concrete, particularly at a strain rate of 100, where it increased by a factor of 1.26 compared to a strain rate of 10−2, while its impact on the reduction of concrete strength was not obvious. Therefore, for high strain rates such as in explosions, it is particularly important to consider the effect of strain rate on fiber-reinforced concrete.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mufti, A.A.; Bakht, B.; Banthia, N.; Benmokrane, B.; Desgagné, G.; Eden, R.; Erki, M.A.; Karbhari, V.; Kroman, J.; Lai, D.; et al. New Canadian highway bridge Design code design provisions for fibre-reinforced structures. Can. J. Civil. Eng. 2007, 34, 267–283. [Google Scholar] [CrossRef]
- Behfarnia, K.; Behravan, A. Application of high performance polypropylene fibers in concrete lining of water tunnels. Mater. Des. 2014, 55, 274–279. [Google Scholar] [CrossRef]
- Ghadban, A.A.; Wehbe, N.I.; Underberg, M. Effect of Fiber Type and Dosage on Flexural Performance of Fiber-Reinforced Concrete for Highway Bridges. ACI Mater. J. 2018, 115, 413–424. [Google Scholar] [CrossRef]
- Liu, Y.W.; Cho, S.W. Study on application of fiber-reinforced concrete in sluice gates. Constr. Build. Mater. 2018, 176, 737–746. [Google Scholar] [CrossRef]
- An, D.; Chen, Z.; Meng, L.H.; Cui, G.Y. Application of fiber-reinforced concrete lining for fault-crossing tunnels in meizoseismal area to improving seismic performance. Adv. Mech. Eng. 2020, 12, 1687814020944023. [Google Scholar] [CrossRef]
- Li, F.R.; Chen, G.X.; Xu, G.Z.; Wu, Y.Y. An Experimental Study on the Compressive Dynamic Performance of Polypropylene Fiber Reinforced Concrete for Retaining Structure under Automobile Collision Magnitude. Adv. Civ. Eng. 2020, 2020, 8826006. [Google Scholar] [CrossRef]
- Ryan, C.; Garcia-Taengua, E. Fibre-Reinforced Concrete Is Sustainable and Cost-Effective for Water-Retaining Structures. Sustainability 2021, 13, 11479. [Google Scholar] [CrossRef]
- Ruiz, R.; Todisco, L.; Corres, H. Application of high-performance fibre reinforced concrete to precast girders for road bridges: Conceptual considerations and numerical analyses. Struct. Concr. 2023, 24, 4645–4659. [Google Scholar] [CrossRef]
- Hájek, M.; Decký, M.; Walter, S. Objectification of Modulus Elasticity of Foam Concrete Poroflow 17-5 on the Subbase Layer. Civ. Environ. Eng. 2016, 12, 55–62. [Google Scholar] [CrossRef]
- Zingg, L.; Briffaut, M.; Baroth, J.; Malecot, Y. Influence of cement matrix porosity on the triaxial behavior of concrete. Cem. Concr. Res. 2016, 80, 52–59. [Google Scholar] [CrossRef]
- Zhu, H.B.; Yan, M.Z.; Li, C. Analysis of the Influence of Porosity of Macroscopic Pore on Concrete Strength by Image Method. J. Build. Mater. 2015, 18, 275–280. [Google Scholar]
- Shi, R. The Effect of Mechanical Properties of Rcc with Initial Pores. Master’s Thesis, Xi’an University of Technology, Xi’an, China, 2020. [Google Scholar]
- Zhao, C.; Li, Q.J.; Zhong, X.G.; Zhang, T.Y. Experimental study on basic mechanical properties of porous metal-concrete. Constr. Build. Mater. 2021, 304, 124663. [Google Scholar] [CrossRef]
- Chen, X.D.; Xu, L.Y.; Wu, S.X. Influence of Pore Structure on Mechanical Behavior of Concrete under High Strain Rates. J. Mater. Civil. Eng. 2016, 28, 04015110. [Google Scholar] [CrossRef]
- Liu, G.Z.; Hou, Z.B.; Liao, L.; Lu, Y.B.; Li, Z.D.; Wang, Y.M. Analysis of Microscopic Pore Structure of Pervious Concrete. China Concr. Cem. Prod. 2022, 7, 40–44. [Google Scholar] [CrossRef]
- Liu, D.W.; Zhang, W.M.; Jian, Y.H.; Tang, Y.; Cao, K.P. Damage precursors of sulfate erosion concrete based on acoustic emission multifractal characteristics and b-value. Constr. Build. Mater. 2024, 419, 135380. [Google Scholar] [CrossRef]
- Li, S.H.; Liu, H.Q. Coal Gangue Concrete Mechanical Properties Under Porosity Influence. Non Met. Mines 2017, 40, 44–46. [Google Scholar]
- Xie, C.; Yuan, L.J.; Zhao, M.; Jia, Y.H. Study on failure mechanism of porous concrete based on acoustic emission and discrete element method. Constr. Build. Mater. 2020, 235, 117409. [Google Scholar] [CrossRef]
- Nitka, M.; Tejchman, J. Meso-mechanical modelling of damage in concrete using discrete element method with porous ITZs of defined width around aggregates. Eng. Fract. Mech. 2020, 231, 107029. [Google Scholar] [CrossRef]
- Wen, F.S.; Fan, H.F.; Zhai, S.T.; Zhang, K.Q.; Liu, F.S. Pore characteristics analysis and numerical seepage simulation of antifreeze permeable concrete. Constr. Build. Mater. 2020, 255, 119310. [Google Scholar] [CrossRef]
- Zhang, G.H.; Wei, X.; Yang, Z.D.; Gu, Y.S.; Wang, M.M. Influence of Mesoscopic Initial Pore Defects on Mechanical Properties of Concrete. J. Build. Mater. 2024, 27, 425–431. [Google Scholar]
- Guo, Y.; Wu, X.T.; Wang, B.Z.; Cheng, C.Z.; Feng, X.K. Numerical simulation of dynamic compression of concrete with pores. J. Hefel Univ. Technol. Nat. Sci. 2023, 46, 1388–1393+1400. [Google Scholar]
- Khodayari, A.; Sadeghnejad, A.; Azizinamini, A. Numerical Investigation of a UHPC Connection Detail for Simple for Dead Load and Continuous for Live Load Steel Bridges in Seismic Areas. Constr. Mater. 2024, 4, 2673–7108. [Google Scholar] [CrossRef]
- Liu, J.Y.; Li, Y. Analysis of the explosion resistance of an I-shaped steel-concrete composite structure under contact explosion. Adv. Struct. Eng. 2023, 26, 1453–1467. [Google Scholar] [CrossRef]
- Zha, Z.X.; Wang, T.; Wu, K.W.; Peng, W.; Wu, Z.H. Experimental study on collapse modes of reinforced concrete wallboards under earthquake action. J. Asian Archit. Build. 2023, 22, 256–273. [Google Scholar] [CrossRef]
- Ozbek, A.S.A.; Weerheijm, J.; Schlangen, E.; van Brengel, K. Dynamic behavior of porous concretes under drop weight impact testing. Cem. Concr. Comp. 2013, 39, 1–11. [Google Scholar] [CrossRef]
- Bai, E.L.; Xu, J.Y.; Lu, S.; Lin, K.X.; Zhang, Y.M. Comparative study on the dynamic properties of lightweight porous concrete. RSC Adv. 2018, 8, 14454–14461. [Google Scholar] [CrossRef]
- Baalbaki, W.; Benmokrane, B.; Chaallal, O.; Aietcin, P.C. Influence of coarse aggregate on elastic properties of high-performance concrete. ACI Mater. J. 1991, 88, 499–503. [Google Scholar]
- Wittmann, F.H.; Roelfstra, P.E.; Sadouki, H. Simulation and analysis of composite structures. Mater. Sci. Eng. 1985, 68, 239–248. [Google Scholar] [CrossRef]
- Walraven, J.C.; Reinhardt, H.W. Theory and Experiments on the Mechanical Behaviour of Cracks in Plain and Reinforced Concrete Subjected to Shear Loading. Heron 1981, 26, 3–68. [Google Scholar]
- Xu, L.H.; Huang, B.; Li, B.; Chi, Y.; Li, C.L.; Shi, Y.C. Study on the stress-strain relation of polypropylene fiber reinforced concrete under cyclic compression. China Civil. Eng. J. 2019, 52, 1–12. [Google Scholar] [CrossRef]
- GB 50010-2010; Code for Design of Concrete Structures. China Architecture & Building Press: Beijing, China, 2010. (In Chinese)
- Zhou, X.Q.; Hao, H. Mesoscale modelling of concrete tensile failure mechanism at high strain rates. Comput. Struct. 2008, 86, 2013–2026. [Google Scholar] [CrossRef]
- Hu, X.Y.; Pang, J.Y.; Lei, C.X. Study on pore structure and permeability of basalt fiber reinforced concrete under confining pressure. Compos. Sci. Eng. 2023, 1, 94–99. [Google Scholar] [CrossRef]
- Zhao, J.; Li, X.F.; Guo, L. Study on fractal dimension and mechanical properties of basalt polypropylene fiber concrete pore structure. Compos. Sci. Eng. 2023, 8, 78–84. [Google Scholar] [CrossRef]
- Liu, B.; Li, D.; Fu, Q.; He, L.; Mai, T.R. Applicability of fractal models for characterising pore structure of hybrid basalt-polypropylene fibre-reinforced concrete. Rev. Adv. Mater. Sci. 2023, 62, 20220272. [Google Scholar] [CrossRef]
- Wu, S.X.; Chen, X.D.; Zhou, J.K. Influence of strain rate and water content on mechanical behavior of dam concrete. Constr. Build. Mater. 2012, 36, 448–457. [Google Scholar] [CrossRef]
- Zheng, Q.Q.; Hu, H.; Xu, Y.; Zhang, T. Damage evolution models of static pre-loaded concrete under impact load based on the Weibull distribution. Case Stud. Constr. Mat. 2022, 17, e01604. [Google Scholar] [CrossRef]
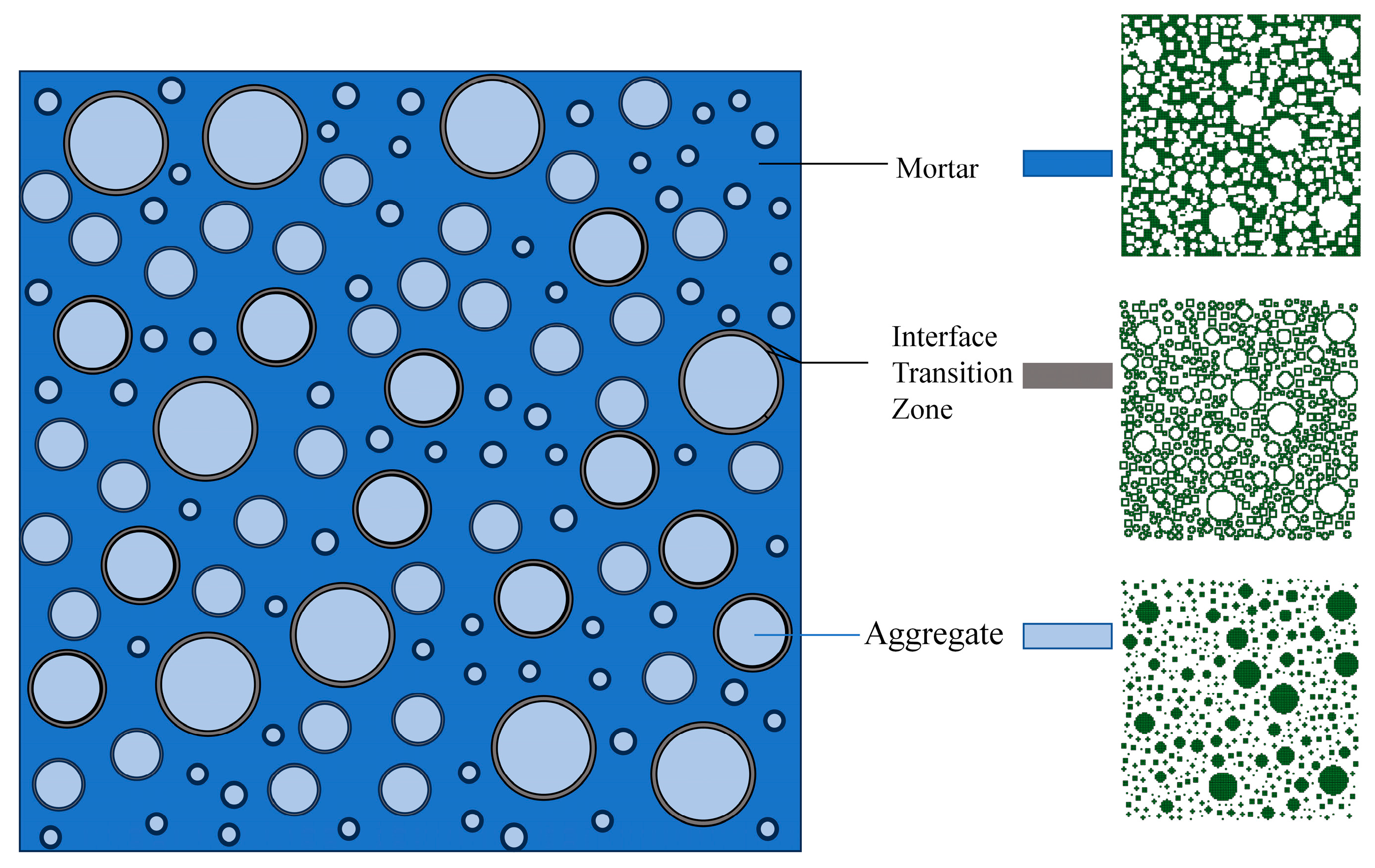

| Density | Modulus of Elasticity | Poisson’s Ratio | Expansion Angle | Eccentricity | fbo/fco | K | Coefficient of Viscosity | |
|---|---|---|---|---|---|---|---|---|
| Unit | kg/m3 | MPa | / | ° | / | / | / | / |
| Aggregate | 2690 | 70,000 | 0.2 | 30 | 0.1 | 1.16 | 0.6667 | 0.006 |
| Interface Layer | 2390 | 24,000 | 0.2 | |||||
| Mortar | 2390 | 30,000 | 0.2 |
| Case | Porosity (%) | Strain Rate (s−1) |
|---|---|---|
| 1 | 2% | 10−2 |
| 2 | 4% | 10−2 |
| 3 | 6% | 10−2 |
| 4 | 8% | 10−2 |
| 5 | 2% | 10−1 |
| 6 | 4% | 10−1 |
| 7 | 6% | 10−1 |
| 8 | 8% | 10−1 |
| 9 | 2% | 100 |
| 10 | 4% | 100 |
| 11 | 6% | 100 |
| 12 | 8% | 100 |
| Porosity | Stress Value/MPa | ||||
|---|---|---|---|---|---|
| Strain Rate/s−1 | 2% | 4% | 6% | 8% | |
| 10−2 | 19.52 | 14.48 | 12.96 | 11.67 | |
| 10−1 | 19.61 | 14.73 | 13.18 | 11.83 | |
| 100 | 19.98 | 14.97 | 13.38 | 11.99 | |
| Porosity | Stress Value/MPa | ||||
|---|---|---|---|---|---|
| Strain Rate/s−1 | 2% | 4% | 6% | 8% | |
| 10−2 | 35,288 | 29,205 | 26,633 | 23,870 | |
| 10−1 | 37,172 | 29,955 | 27,520 | 24,614 | |
| 100 | 44,713 | 32,254 | 29,283 | 26,256 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, A.; Du, X.; Wang, F.; Li, J.; Zhang, T.; Li, Y. Study on Damage Mechanism of Fiber Concrete with Initial Pores. Materials 2025, 18, 916. https://doi.org/10.3390/ma18050916
Hu A, Du X, Wang F, Li J, Zhang T, Li Y. Study on Damage Mechanism of Fiber Concrete with Initial Pores. Materials. 2025; 18(5):916. https://doi.org/10.3390/ma18050916
Chicago/Turabian StyleHu, Ankui, Xinyu Du, Fei Wang, Junjie Li, Tianlong Zhang, and Yajing Li. 2025. "Study on Damage Mechanism of Fiber Concrete with Initial Pores" Materials 18, no. 5: 916. https://doi.org/10.3390/ma18050916
APA StyleHu, A., Du, X., Wang, F., Li, J., Zhang, T., & Li, Y. (2025). Study on Damage Mechanism of Fiber Concrete with Initial Pores. Materials, 18(5), 916. https://doi.org/10.3390/ma18050916







