The Effect of Specimen Size on the Results of Concrete Adiabatic Temperature Rise Test with Commercially Available Equipment
Abstract
:1. Introduction
2. Experimental Plan and Method
2.1. Experimental Plan
2.2. Materials and Mixing
| Binder Type | Adiabatic Specimen Volume (L) | W/B (%) | Slump (mm) | Air (%) | S/a (%) | Unit Weight (kg/m3) | Test Items | |||
|---|---|---|---|---|---|---|---|---|---|---|
| Water | Binder | Sand | Gravel | |||||||
| OPC | (1) 6
(2) 30 (3) 50 | 53.3 | 150 ± 25 | 4.5 ± 1.5 | 47 | 160 | 300 | 870 | 1011 | —Adiabatic temperature rise (Q∞, r); —Correlation between the 6 L, 30 L and the 50 L adiabatic temperature rise tests |
| 40.0 | 46 | 160 | 400 | 814 | 985 | |||||
| 32.0 | 45 | 160 | 500 | 759 | 956 | |||||
| LPC | 53.3 | 47 | 160 | 300 | 873 | 1014 | ||||
| 40.0 | 46 | 160 | 400 | 817 | 989 | |||||
| 32.0 | 45 | 160 | 500 | 763 | 961 | |||||
| TBC | 53.3 | 47 | 160 | 300 | 858 | 997 | ||||
| 40.0 | 46 | 160 | 400 | 798 | 966 | |||||
| 32.0 | 45 | 160 | 500 | 740 | 932 | |||||
| EBC | 53.3 | 47 | 160 | 300 | 858 | 997 | ||||
| 40.0 | 46 | 160 | 400 | 798 | 966 | |||||
| 32.0 | 45 | 160 | 500 | 740 | 932 | |||||
| Material | Specific gravity | Blaine (cm3/g) | Ignition loss |
|---|---|---|---|
| Ordinary Portland cement | 3.15 | 3475 | 2.15 |
| Low heat Portland cement | 3.22 | 3500 | 1.90 |
| Ternary blended cement | 2.88 | 3810 | 1.20 |
| Early strength low heat blended cement | 2.89 | 3802 | 0.07 |
| Material | Type | Diameter (mm) | F.M | S.G |
|---|---|---|---|---|
| Fine aggregate | River sand | 5 | 3.06 | 2.59 |
| Coarse aggregate | Crushed stone | 25 | 7.06 | 2.67 |
| Material | Type | pH | S.G |
|---|---|---|---|
| Admixture 1 | Polynaphthalene sulfonates based | 6.0 | 1.20 |
| Admixture 2 | Polycarboxylic acid based | 6.5 | 1.05 |

2.3. Adiabatic Temperature Rise Test

3. Results and Discussion
3.1. Influence of Binder Types on Adiabatic Temperature History



3.2. Influence of Specimen Volume on Adiabatic Temperature History





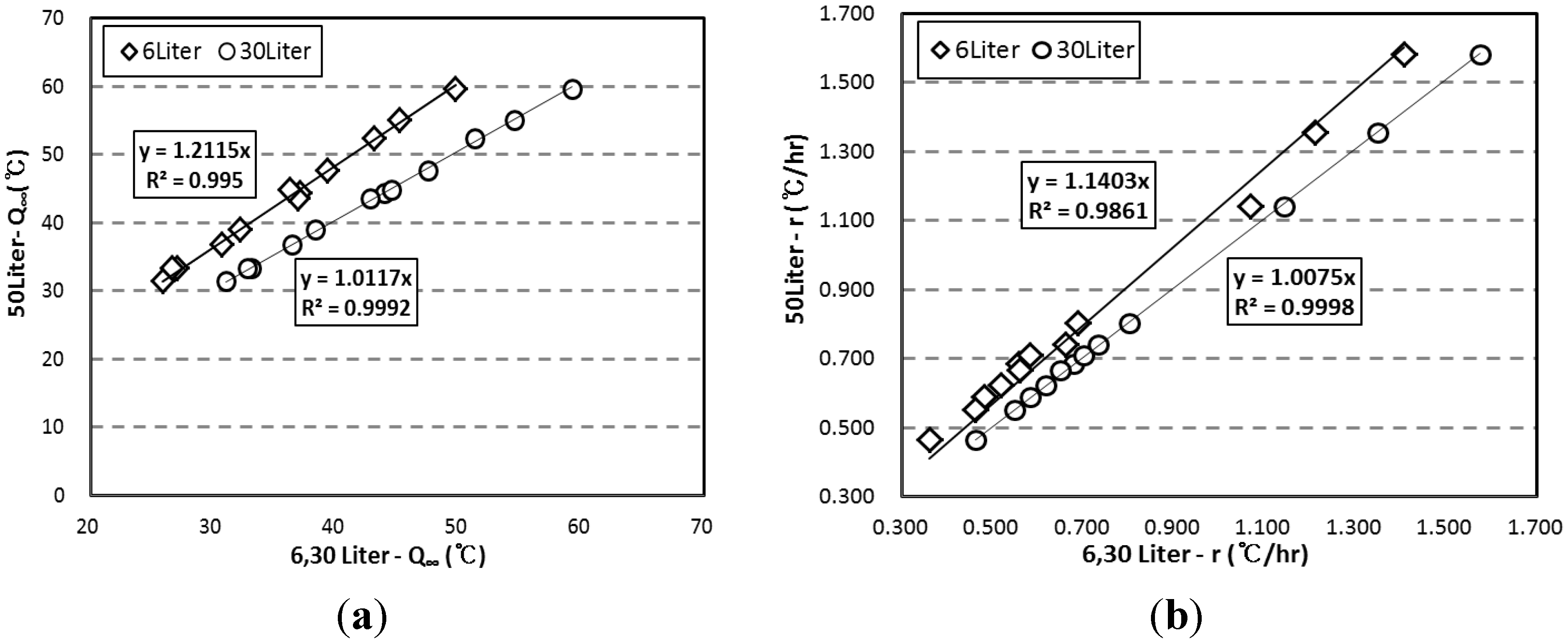
3.3. Correlation of the Adiabatic Temperature Rises and the Specimen Volume
4. Conclusions
- (1)
- The adiabatic temperature rise test showed that for every mixture, the Q∞ and the r increased in proportion to the unit weight of binder. Of the mixtures, the EBC mixture had the lowest Q∞ and the TBC mixture had the lowest r.
- (2)
- Even though the experiment was conducted using an adiabatic calorimeter satisfying the minimum requirement of temperature loss, test results show that the volume of samples influences the adiabatic test results. For every mixture, the maximum temperature increase (Q∞) and reaction factor (r) of the 50 L specimens were about 17.5% and 12.5% higher than those of the 6 L specimens. However, there are only little differences (Q∞ 1.27%, r 1.30%) between the results of the 30 L and 50 L specimens. This proves that even a small temperature loss can affect the adiabatic temperature history of a small size of specimen. In this experiment, a 4 L sample size was not appropriate for an adiabatic temperature rise test.
- (3)
- Based on the test results, correlations are proposed for the compensation of temperature loss with small-size specimens. By using the proposed correlation depending on adiabatic specimen volume, the adiabatic temperature rise of the 50 L specimen could be predicted based on the results of the 6 L and 30 L specimens. Therefore, it is thought that this correlation can be used as baseline data for on-site concrete quality control and research purposes.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kang, S.H.; Jeong, H.J.; Park, C.L. Evaluation on external restraint stress in mass concrete. J. Korea Concr. Inst. 1996, 8, 111–122. [Google Scholar]
- Korean Concrete Standard Specification, 7th ed.; Korea Concrete Institute: Seoul, Korea, 2009.
- Baek, D.I.; Kim, M.S. Application of heat pipe for hydration heat control of mass concrete. J. Korea Concr. Inst. 2008, 20, 157–164. [Google Scholar] [CrossRef]
- Cha, S.W.; Jang, B.S. Thermal stresses of roller compacted concrete dam considering construction sequence and seasonal temperature. J. Korean Soc. Civil Eng. 2008, 28, 881–891. [Google Scholar]
- Park, C.W.; Sim, J.S.; Lee, J.R. Fundamental property of chloride inhibiting and low heat cement for marine concrete structures. J. Adv. Concr. Technol. 2009, 7, 135–142. [Google Scholar] [CrossRef]
- Evsukoff, A.G.; Fairbairn, E.M.R.; Faria, É.F.; Silvoso, M.M.; Toledo Filho, R.D. Modeling adiabatic temperature rise during concrete hydration. Comput. Struct. 2006, 84, 2351–2362. [Google Scholar] [CrossRef]
- Yoon, D.Y.; Yang, O.B.; Min, C.S. Finite Element Analysis on Heat of Hydration with Reinforcing Steel Bars. J. Korea Concr. Inst. 2005, 17, 43–49. [Google Scholar] [CrossRef]
- Lee, K.C.; Cho, J.W.; Jung, S.H.; Jang, J.H. Study on hydration heat of blended belite binder. J. Korea Concr. Inst. 2011, 23, 145–150. [Google Scholar] [CrossRef]
- Kwon, Y.H. An experimental study on the required performances of roof concrete placed in the In-ground LNG storage tank. J. Korea Concr. Inst. 2013, 25, 339–345. [Google Scholar] [CrossRef]
- Kwak, Y.J.; Son, H.J.; Kim, K.M.; Park, S.J.; Han, M.C.; Han, C.G. Engineering properties of high early strength type low heat cement concrete. Proc. Korea Concr. Inst. 2012, 24, 349–350. [Google Scholar]
- Song, H.W.; Lee, C.H.; Lee, K.C.; Kim, J.H.; Ann, K.Y. Chloride penetration resistance of ternary blended concrete and discussion for durability. J. Korea Concr. Inst. 2008, 20, 439–449. [Google Scholar] [CrossRef]
- Morabito, P. Methods to determine the heat of hydration of concrete. In Prevention of the Thermal Cracking in Concrete at Early Ages; Springenschmid, R., Ed.; E&FN Spon: London, UK, 1998; pp. 1–25. [Google Scholar]
- Schutter, G.D. Hydration and temperature development of concrete made with blast-furnace slag cement. Cem. Concr. Res. 1999, 29, 143–149. [Google Scholar] [CrossRef]
- Liwu, M.; Min, D. Thermal behavior of cement matrix with high-volume mineral admixtures at early hydration age. Cem. Concr. Res. 2006, 36, 1992–1998. [Google Scholar] [CrossRef]
- Atis, C.D. Heat evolution of high-volume fly ash concrete. Cem. Concr. Res. 2002, 32, 751–756. [Google Scholar] [CrossRef]
- Schutter, G.D. General hydration model for Portland cement and blast furnace slag cement. Cem. Concr. Res. 1995, 25, 593–604. [Google Scholar] [CrossRef]
- Tanaka, I.; Suzuki, N.; Ono, Y.; Koishi, M. A comparison of the fluidity of spherical cement with that of broad cement and a study of the properties of fresh concrete using spherical cement. Cem. Concr. Res. 1999, 29, 553–560. [Google Scholar] [CrossRef]
- Hagiwara, S.; Sakai, E.; Sato, H.; Asaga, K. Development of high performance adiabatic temperature rise measuring system for long term measurement. Netsu Bussei 2009, 23, 21–26. [Google Scholar] [CrossRef]
- Branco, F.A.; Mendes, P.; Mirambell, E. Heat of hydration effects in concrete structures. Am. Concr. Inst. Mater. J. 1992, 89, 139–145. [Google Scholar]
- Hyun, J.H. Development of Experimental Equipment for Thermal Stress in Mass Concrete. Master’s Thesis, Korea Advanced Institute of Science and Technology, Daejeon, Korea, February 2000. [Google Scholar]
- Du, L.; Chen, X.; Liao, B. Dynamic model of temperature rise caused by cementitious materials hydration. Cem. Concr. Res. 2005, 35, 1609–1612. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, B.J.; Bang, J.W.; Shin, K.J.; Kim, Y.Y. The Effect of Specimen Size on the Results of Concrete Adiabatic Temperature Rise Test with Commercially Available Equipment. Materials 2014, 7, 7861-7874. https://doi.org/10.3390/ma7127861
Lee BJ, Bang JW, Shin KJ, Kim YY. The Effect of Specimen Size on the Results of Concrete Adiabatic Temperature Rise Test with Commercially Available Equipment. Materials. 2014; 7(12):7861-7874. https://doi.org/10.3390/ma7127861
Chicago/Turabian StyleLee, Byung Jae, Jin Wook Bang, Kyung Joon Shin, and Yun Yong Kim. 2014. "The Effect of Specimen Size on the Results of Concrete Adiabatic Temperature Rise Test with Commercially Available Equipment" Materials 7, no. 12: 7861-7874. https://doi.org/10.3390/ma7127861
APA StyleLee, B. J., Bang, J. W., Shin, K. J., & Kim, Y. Y. (2014). The Effect of Specimen Size on the Results of Concrete Adiabatic Temperature Rise Test with Commercially Available Equipment. Materials, 7(12), 7861-7874. https://doi.org/10.3390/ma7127861





