Failure Progress of 3D Reinforced GFRP Laminate during Static Bending, Evaluated by Means of Acoustic Emission and Vibrations Analysis
Abstract
:1. Introduction
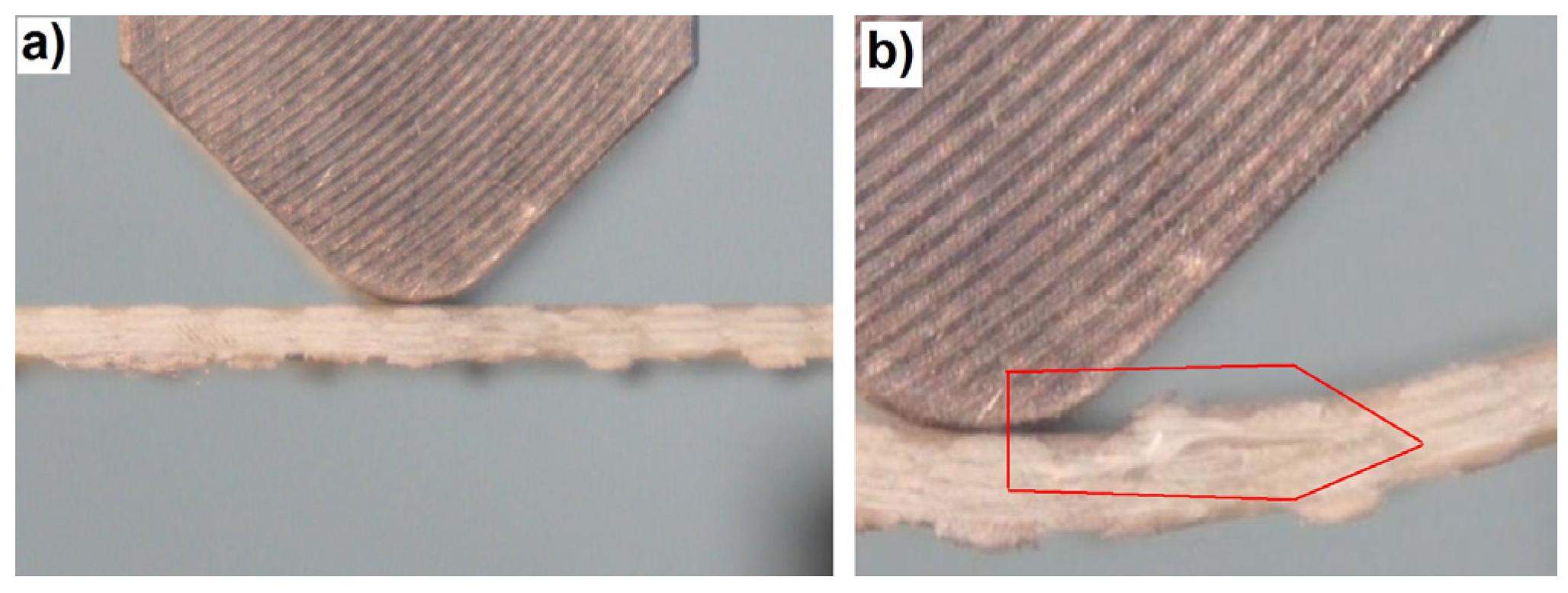
2. Experimental Section
- Classic preform (only for AE tests, comparison purposes): 10 layers of plain-woven glass fabric (E glass), produced by KROSGLASS (Krosno, Poland), areal mass 350 g/m2 (total of 3500 g/m2), preform thickness 2.65 ± 0.02 mm; preform for classic laminate.
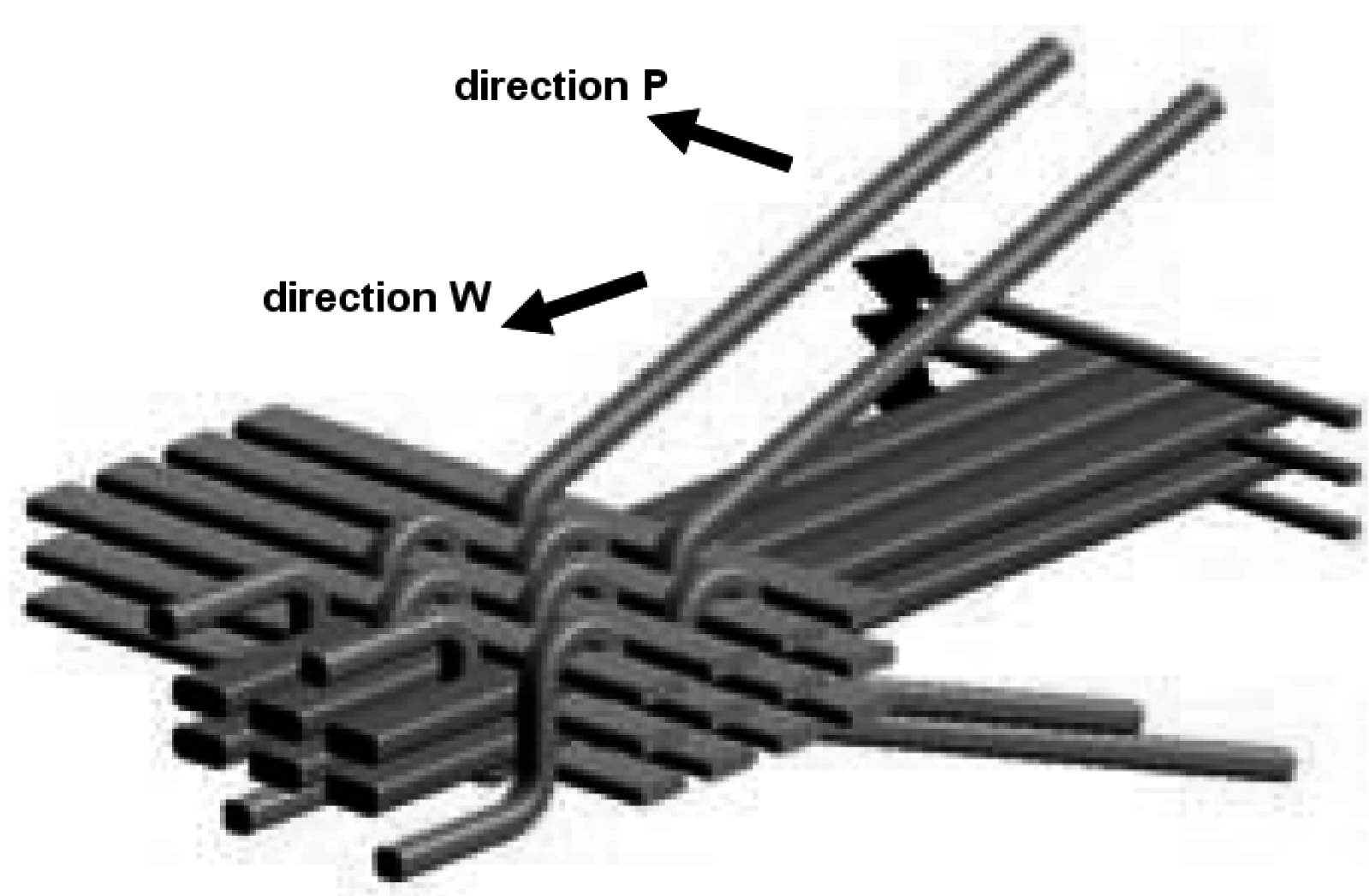
- 3D preform (non-crimp, E glass), produced by 3TEX (Rutherfordton, NC, USA), woven of 7 layers: 4 in the weft direction and 3 in the warp direction, areal mass 3280 g/m2, fabric thickness 2.65 ± 0.02 mm. The preliminary analysis showed that the structural model of this fabric is the most appropriate from among the currently available free trade fabrics for the comparison with the classic preform; preform for 3D laminate.

3. Results and Discussion
3.1. Acoustic Emission Results








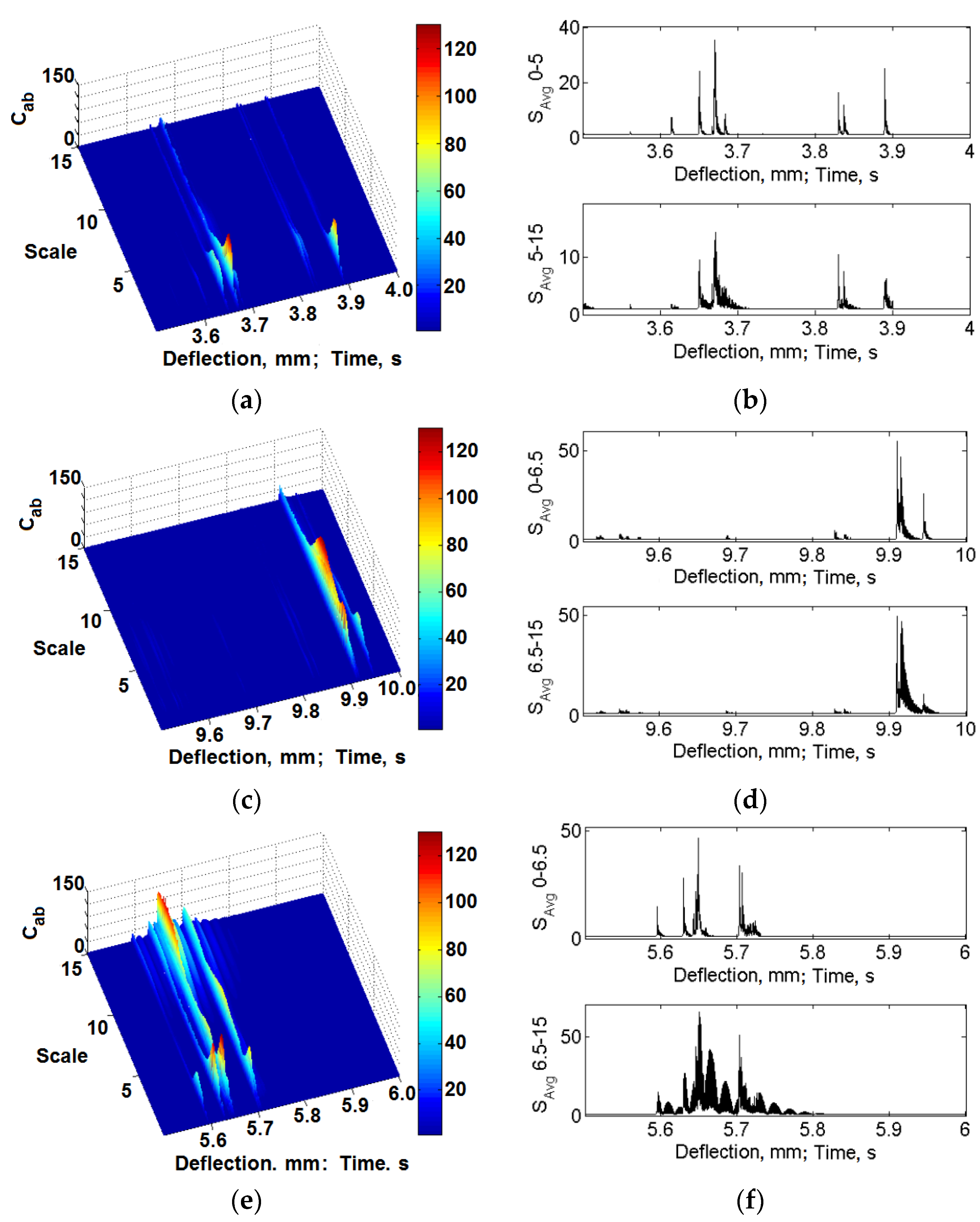
3.2. Vibrations Signal Results





4. Conclusions
- The applied innovative method of separating the acoustic emission signal coming from fiber fracture and the one coming from matrix fracture with the use of the acoustic event energy proves very good in the analysis of the main laminate failure. A problematic issue is the detection of a simultaneous fracture of small amounts of elementary fibers, which makes the separative analysis impossible at an earlier stage of failure. At the same time, emission remains a good tool for the detection of initial failure processes in the case when there is no need to separate the signal.
- The method of vibration signal analysis is more precise than the method of acoustic emission, due to its higher frequency of sampling and higher sensitivity of the measuring system. It is very useful in the detection and analysis of the signal originating from the effects occurring in the initial stage of material failure, which significantly precedes the main failure. In this respect, vibration analysis is a valuable complementation, or alternative, for acoustic emission.
- The energy approach to signal separation can be effectively implemented with the use of the kurtosis of the signal progress.
- In the comparison of the test results obtained in separate bending tests by the two different methods of signal registration and analysis, one can state that the results are similar, both with respect of the general laminate failure progress and the occurrence of separated signals of fiber fracture and matrix fracture.
- The strength of the 3D laminate is comparable with that of the classic laminate. This results from the overlap of the beneficial “non-crimp” effect and the negative effect of the “lattice” construction.
- The strength of the 3D laminate is slightly lower in the direction perpendicular to the translaminar interweave strands than in the direction parallel to them.
- The 3D laminate is characterized as having a much higher deformability than the classic laminate, especially in the direction perpendicular to the translaminar interweave strands.
- The failure progress of the 3D laminate is “safer” than that of the classic laminate, especially in the direction perpendicular to the translaminar interweave strands. It exhibits a milder failure model, with less rapid load capacity drops. The results of the acoustic emission also show a higher fraction of fiber fracture, already before the occurrence of the basic material failure. This is a very beneficial failure model, which proves the occurrence of effort, that is the work of the fibers during the load shift.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Karczewski, R.; Golebiowski, L.; Molak, R.; Plowiec, J.; Spychalski, W.L. Acoustic emission in monitoring composite bridge structures. Compos. Theory Pract. 2015, 15, 112–116. [Google Scholar]
- Altenbach, H.; Becker, W. (Eds.) Modern Trends in Composite Laminates Mechanics; Series: CISM International Centre for Mechanical Sciences; Springer-Verlag Wien: Vienna, Austria, 2003; Volume 448.
- Giordano, M.; Calabro, A.; Esposito, C.; D’Amore, A.; Nicolais, L. An acoustic-emission characterization of the failure modes in polymer-composite materials. Compos. Sci. Technol. 1998, 58, 1923–1928. [Google Scholar] [CrossRef]
- Singh, C.V.; Talreja, R. Evolution of ply cracks in multidirectional composite laminates. Int. J. Solids Struct. 2010, 47, 1338–1349. [Google Scholar] [CrossRef]
- Oleksiak, B.; Koziol, M.; Wieczorek, J.; Krupa, M.; Folega, P. Strength of briquettes made of Cu concentrate and carbon-bearing materials. Metalurgija 2015, 54, 95–97. [Google Scholar]
- Labaj, J.; Jodkowski, M.; Szeja, W.; Helhmann, J.; Koziol, M. Altrnative binder for copper concentrate briquetting. Metalurgija 2015, 54, 646–648. [Google Scholar]
- Galina, H. (Ed.) Physics of Polymer Materials; WNT: Warszawa, Poland, 2008. (In Polish)
- Myalski, J.; Wieczorek, J.; Plachta, A. Physical and mechanical properties of composites with aluminum alloy matrix designed for metal forming. Solid State Phenom. 2014, 212, 59–62. [Google Scholar] [CrossRef]
- Szkliniarz, A.; Blacha, L.; Szkliniarz, W.; Labaj, J. Characteristics of plasticity of hot deformed Cu-Ti alloys. Arch. Metall. Mater. 2014, 59, 1307–1312. [Google Scholar]
- Hejna, A.; Formela, K.; Saeb, M.R. Processing, mechanical and thermal behavior assessments ofpolycaprolactone/agricultural wastes biocomposites. Ind. Crops Prod. 2015, 76, 725–733. [Google Scholar] [CrossRef]
- Korol, J.; Lenza, J.; Formela, K. Manufacture and research of TPS/PE biocomposites properties. Compos. B 2015, 68, 310–316. [Google Scholar] [CrossRef]
- Brauner, C.; Pereira, G.C.; Herrmann, A.S.J. Effects of fabric-based unbalances on process-induced distortions of composite materials. J. Compos. Mater. 2015, 49, 1291–1300. [Google Scholar] [CrossRef]
- Koziol, M.; Bogdan-Wlodek, A.; Myalski, J.; Wieczorek, J. Influence of wet chemistry treatment on the mechanical performance of natural fibres. Pol. J. Chem. Technol. 2011, 13, 21–27. [Google Scholar] [CrossRef]
- Rydarowski, H.; Koziol, M. Repeatability of glass fiber reinforced polymer laminate panels manufactured by hand lay-up and vacuum-assisted resin infusion. J. Compos. Mater. 2015, 49, 573–586. [Google Scholar] [CrossRef]
- Landowski, M.; Budzik, M.; Imielinska, K. Water absorption and blistering of glass fibre-reinforced polymer marine laminates with nanoparticle-modified coatings. J. Compos. Mater. 2015, 48, 2805–2813. [Google Scholar] [CrossRef]
- Priess, T.; Sause, M.G.R.; Fischer, D.; Middendorf, P. Detection of delamination onset in laser-cut carbon fiber transverse crack tension specimens using acoustic emission. J. Compos. Mater. 2015, 49, 2639–2647. [Google Scholar] [CrossRef]
- Wrobel, G.; Rojek, M.; Szymiczek, M. Mechanical properties evaluation and ultrasound diagnosis of unsaturated polyester-glass pipes. Pol. J. Chem. Technol. 2015, 17, 77–83. [Google Scholar] [CrossRef]
- Johnston, R.E.; Bache, M.R.; Amos, M.; Liaptsis, D.; Lancaster, R.; Lewis, R.; Andrews, P.; Edmonds, I. Investigation of non-destructive evaluation methods applied to oxide/oxide fiber reinforced ceramic matrix composite. In Book Series: Ceramic Engineering and Science Proceedings; Singh, D., Salem, J., Halbig, M., Mathur, S., Eds.; Wiley: Hoboken, NJ, USA, 2013; pp. 57–67. [Google Scholar]
- Jakubczak, P.; Bienias, J.; Surowska, B. Impact damage live-time analysis of modern composite materials using thermography. Compo. Theory Pract. 2014, 14, 219–223. [Google Scholar]
- Lomov, S.V.; Karahan, M.; Bogdanovich, A.E.; Verpoest, I. Monitoring of acoustic emission damage during tensile loading of 3D woven carbon/epoxy composites. Text. Res. J. 2014, 84, 1373–1384. [Google Scholar] [CrossRef]
- Blazejewski, W.; Gasior, P.; Kaleta, J.; Rybczynski, R. Application of optical fiber sensors for damage accumulation monitoring in composite structures. Key Eng. Mater. 2014, 598, 1–6. [Google Scholar] [CrossRef]
- Blazejewski, W.; Czulak, A.; Gasior, P.; Kaleta, J.; Mech, R. SMART composite high pressure vessels with integrated Optical Fiber Sensors. In Book Series: Proceedings of SPIE-The International Society for Optical Engineering; Tomizuka, M., Yun, C.B., Giurgiutiu, V., Lynch, J.P., Eds.; Publisher: Bellingham, WA, USA, 2010; Volume 7647. [Google Scholar]
- Katunin, A.; Dragan, K.; Dziendzikowski, M. Low-velocity impact damage identification of aircraft laminated structures based on wavelet analysis of modal shapes. Compo. Theory Pract. 2015, 15, 39–43. [Google Scholar]
- Moghaddam, M.K.; Breede, A.; Brauner, C.; Lang, W. Embedding piezoresistive pressure sensors to obtain online pressure profiles inside fiber composite laminates. Sensors 2015, 15, 7499–7511. [Google Scholar] [CrossRef] [PubMed]
- Sorrentino, L.; Bellini, C.; Capriglione, D.; Ferrigno, L. Local monitoring of polymerization trend by an interdigital dielectric sensor. Int. J. Adv. Manuf. Technol. 2015, 79, 1007–1016. [Google Scholar] [CrossRef]
- Seyhan, A.T.; De la Vega, A.; Tanoglu, M.; Schulte, K. Thermal curing behavior of MWCNT modified vinyl ester-polyester resin suspensions prepared with 3-roll milling technique. J. Polym. Sci. B 2009, 47, 1511–1522. [Google Scholar] [CrossRef]
- Wieczorek, J. Tribological properties and a wear model of aluminium matrix composites-SiC particles designed for metal forming. Arch. Metall. Mater. 2015, 60, 111–115. [Google Scholar] [CrossRef]
- Paszkiewicz, S.; Pilawka, R.; Zenker, M.; Czulak, A.; Roslaniec, Z. Detailed study on interfacial interactions in epoxy composites cured with 1-buthylimidazole containing functionalized carbon nanotubes. Compos. Interfaces 2015, 22, 629–649. [Google Scholar] [CrossRef]
- Haniszewski, T.; Gaska, D. Overhead traveling crane vibration research using experimental wireless measuring system. Transp. Probl. 2013, 8, 57–66. [Google Scholar]
- Dransfield, K.; Baillie, C.; Mai, Y.W. Improving the delamination resistance of CFRP by stitching—A review. Compos. Sci. Technol. 1994, 50, 305–317. [Google Scholar] [CrossRef]
- Cox, B.N.; Dadkhah, M.S.; Morris, W.L.; Flintoff, J.G. Failure mechanisms of 3D woven composites in tension, compression, and bending. Acta Metall. Mater. 1994, 42, 3967–3984. [Google Scholar] [CrossRef]
- Oskouei, A.R.; Zucchelli, A.; Ahmadi, M.; Minak, G. An integrated approach based on acoustic emission and mechanical information to evaluate the delamination fracture toughness at mode I in composite laminate. Mater. Des. 2011, 32, 1444–1455. [Google Scholar] [CrossRef]
- Callus, P.J.; Mouritz, A.P.; Bannister, M.K.; Leong, K.H. Tensile properties and failure mechanisms of 3D woven GRP composites. Compos. A 1999, 30, 1277–1287. [Google Scholar] [CrossRef]
- Cox, B.N.; Dadkhah, M.S.; Morris, W.L. On the tensile failure of 3D woven composites. Compos. A 1996, 27, 447–458. [Google Scholar] [CrossRef]
- Wojcicka, A.; Mroczka, K.; Kurtyka, P.; Binkowski, M.; Wrobel, Z. X-ray microtomography analysis of the aluminum alloy composite reinforced by SiC after friction stir processing. J. Mater. Eng. Perform. 2014, 23, 3215–3221. [Google Scholar] [CrossRef]
- Budzik, M.; Jumel, J.; Imielinska, K.; Shanahan, M.E.R. Effect of adhesive compliance in the assessment of soft adhesives with the wedge test. J. Adhes. Sci. Technol. 2011, 25, 131–149. [Google Scholar] [CrossRef]
- Rohwer, K. Predicting fiber composite damage and failure. J. Compos. Mater. 2015, 49, 2673–2683. [Google Scholar] [CrossRef]
- Lopresto, V.; Caprino, G.; Leone, C.; Langella, A. A damage index of indentation sensitivity in low velocity impact conditions. Polym. Compos. 2015, 36, 987–991. [Google Scholar] [CrossRef]
- Herwan, J.; Kondo, A.; Morooka, S.; Watanabe, N. Effects of stitch density and stitch thread thickness on mode II delamination properties of Vectran stitched composites. Plast. Rubber Compos. 2014, 43, 300–308. [Google Scholar] [CrossRef]
- Arani, A.G.; Abdollahian, M.; Kolahchi, R. Nonlinear vibration of embedded smart composite microtube conveying fluid based on modified couple stress theory. Polym. Compos. 2015, 36, 1314–1324. [Google Scholar] [CrossRef]
- Cokaygil, Z.; Banar, M.; Seyhan, A.T. Orange peel-derived pectin jelly and corn starch-based biocomposite film with layered silicates. J. Appl. Polym. Sci. 2014, 131, 40654. [Google Scholar] [CrossRef]
- Gray, V.; Whittaker, M. Development and assessment of a new empirical model for predicting full creep curves. Materials 2015, 8, 4582–4592. [Google Scholar] [CrossRef]
- Jones, J.; Whittaker, M.; Lancaster, R.; Williams, S. Lifing the thermo-mechanical fatigue (TMF) behaviour of the polycrystalline nickel-based superalloy RR1000. In MATEC Web of Conferences; Guedou, J.Y., Chone, J., Eds.; EDP Sciences: Les Ulis, France, 2014; Volume 14. [Google Scholar]
- Whittaker, M.; Lancaster, R.; Harrison, W.; Pretty, C.; Williams, S. An empirical approach to correlating thermo-mechanical fatigue behaviour of a polycrystalline Ni-base superalloy. Materials 2013, 6, 5275–5290. [Google Scholar] [CrossRef]
- Virk, A.S.; Hall, W.; Summerscales, J. Microstructural characterisation of jute/epoxy quasi-unidirectional composites. Appl. Compos. Mater. 2014, 21, 885–903. [Google Scholar] [CrossRef]
- Thakur, V.K.; Kessler, M.R. Self-healing polymer nanocomposite materials: A review. Polymer 2015, in press. [Google Scholar] [CrossRef]
- Pappu, A.; Patil, V.; Jain, S.; Mahindrakar, A.; Haque, R.; Thakur, V.K. Advances in industrial prospective of cellulosic macromolecules enriched banana biofibre resources: A review. Int. J. Biol. Macromol. 2015, 79, 449–458. [Google Scholar] [CrossRef] [PubMed]
- Posmyk, A.; Myalski, J. Producing of composite materials with aluminium alloy matrix containing solid lubricants. Solid State Phenom. 2012, 191, 67–74. [Google Scholar] [CrossRef]
- Jiang, L.D.; Pandraud, G.; French, P.J.; Spearing, S.M.; Kraft, M. A novel method for nanoprecision alignment in wafer bonding applications. J. Micromech. Microeng. 2007, 17, S61–S67. [Google Scholar] [CrossRef]
- Prasanna, S.; Spearing, S.M. Materials selection and design of microelectrothermal bimaterial actuators. J. Microelectromech. Syst. 2007, 16, 248–259. [Google Scholar] [CrossRef]
- Kim, K.J.; Yu, W.R.; Lee, J.S.; Gao, L.; Thostenson, E.T.; Chou, T.W.; Byun, J.H. Damage characterization of 3D braided composites using carbon nanotube-based in situ sensing. Compos. A 2010, 41, 1531–1537. [Google Scholar] [CrossRef]
- Caneva, C.; Olivieri, S.; Santulli, C.; Bonifazi, G. Impact damage evaluation on advanced stitched composites by means of acoustic emission and image analysis. Compos. Struct. 1993, 25, 121–128. [Google Scholar] [CrossRef]
- Koziol, M.; Figlus, T. Evaluation of the failure progress in the static bending of GFRP laminates reinforced with a classic plain-woven fabric and a 3D fabric, by means of the vibrations analysis. Polym. Compos. 2015. [Google Scholar] [CrossRef]
- Figlus, T.; Koziol, M. Evaluation of failure progress in glass- and jute-fibre reinforced polymer laminates by analysis of vibration and noise. J. Vibroeng. 2014, 16, 3449–3468. [Google Scholar]
- Koziol, M.; Sleziona, J. Failure progress of stitched reinforced polyester-glass laminate at static bending. Polimery 2008, 53, 876–882. [Google Scholar]
- De Groot, P.J.; Wijnen, P.A.M.; Janssen, R.B.F. Real-time frequency determination of acoustic emission for different fracture mechanisms in carbon/epoxy composites. Compos. Sci. Technol. 1995, 55, 405–412. [Google Scholar] [CrossRef]
- Dabrowski, Z.; Zawisza, M. The choice of vibroacoustic signal measures, in mechanical fault diagnosis of diesel engines. Solid State Phenom. 2015, 236, 220–227. [Google Scholar] [CrossRef]
- Dabrowski, Z.; Zawisza, M. Investigations of the vibroacoustic signals sensitivity to mechanical defects not recognised by the OBD system in diesel engines. Solid State Phenom. 2011, 180, 194–199. [Google Scholar] [CrossRef]
- Polish Committee for Standardization. Fibre-Reinforced Plastic Composites—Determination of Flexural Properties; PN-EN-ISO 14125: 1998; Polish Committee for Standardization: Warsaw, Poland, 1998. [Google Scholar]
- Figlus, T.; Koziol, M. Method for evaluating destruction of composite materials, involves measuring instantaneous sound pressure level in vicinity of sample located at supports of body in direction perpendicular or parallel to axis of movement of mandrel. Patent Number: PL406442-A1, 22 June 2015. [Google Scholar]
- Dybala, J.; Zimroz, R. Rolling bearing diagnosing method based on empirical mode decomposition of machine vibration signal. Appl. Acoust. 2014, 77, 195–203. [Google Scholar] [CrossRef]
- Dziurdz, J. Transformation of nonstationary signals into pseudostationary signals for the needs of vehicle diagnostics. Acta Phys. Pol. A 2010, 118, 49–53. [Google Scholar]
- Cho, H.K.; Rowlands, R.E. Enhancing buckling performance of perforated composite laminates by manipulating fiber direction using a genetic algorithm. J. Mech. Sci. Technol. 2015, 29, 3727–3737. [Google Scholar] [CrossRef]
- Fattahi, A.M.; Moaddab, E.; Bibishahrbanoei, N. Thermo-mechanical stress analysis in platelet reinforced composites with bonded and debonded platelet end. J. Mech. Sci. Technol. 2015, 29, 2067–2072. [Google Scholar] [CrossRef]
- 3TEX Inc. Product Data Sheet for 96oz E Glass 3WEAVE fabric. 2012. Available online: http://www.3tex.com/sites/default/files/96oz%20P3W-GE044.pdf (accessed on 8 December 2015).
- Figlus, T.; Koziol, M. Diagnosis of early-stage damage to polymer—Glass fibre composites using non-contact measurement of vibration signals. J. Mech. Sci. Technol. 2015. submitted. [Google Scholar]
- Figlus, T.; Wilk, A. Application of a continuous wavelet transform for the diagnosing of excessive valve clearance of the combustion engine. Solid State Phenom. 2015, 236, 153–160. [Google Scholar] [CrossRef]
- Czech, P. Diagnosis of industrial gearboxes condition by vibration and time-frequency, scale-frequency, frequency-frequency analysis. Metalurgija 2012, 51, 521–524. [Google Scholar]
- Resnikoff, H.L.; Wells, R.O., Jr. Wavelet Analysis; Springer: Berlin, Germany, 1960. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koziol, M.; Figlus, T. Failure Progress of 3D Reinforced GFRP Laminate during Static Bending, Evaluated by Means of Acoustic Emission and Vibrations Analysis. Materials 2015, 8, 8751-8767. https://doi.org/10.3390/ma8125490
Koziol M, Figlus T. Failure Progress of 3D Reinforced GFRP Laminate during Static Bending, Evaluated by Means of Acoustic Emission and Vibrations Analysis. Materials. 2015; 8(12):8751-8767. https://doi.org/10.3390/ma8125490
Chicago/Turabian StyleKoziol, Mateusz, and Tomasz Figlus. 2015. "Failure Progress of 3D Reinforced GFRP Laminate during Static Bending, Evaluated by Means of Acoustic Emission and Vibrations Analysis" Materials 8, no. 12: 8751-8767. https://doi.org/10.3390/ma8125490
APA StyleKoziol, M., & Figlus, T. (2015). Failure Progress of 3D Reinforced GFRP Laminate during Static Bending, Evaluated by Means of Acoustic Emission and Vibrations Analysis. Materials, 8(12), 8751-8767. https://doi.org/10.3390/ma8125490






