Compressive Behavior of Fiber-Reinforced Concrete with End-Hooked Steel Fibers
Abstract
:1. Introduction
| Researchers | Models |
|---|---|
| †Ezeldin and Balaguru [11] | where, ;; ; ; , , and are the compressive strength, corresponding strain, and elastic modulus of plain concrete, respectively. |
| Hsu and Hsu [12] | where for for where, is the strain at in the descending; is the measured compressive strength; ; ; where, , , , and are constant. |
| †Someh and Saeki [13] | where, is the measured compressive strength; ; and . |
| †Mansur et al. [14] | for for where, is the measured compressive strength; ; ; . |
| †Nataraja et al. [15] | where, ; ; ; and are the compressive strength of plain concrete and corresponding strain, respectively. |
2. Test Program for the Compressive Behavior of SFRC
| Type | Length lf [mm] | Diameter df [mm] | Tensile strength σfu [MPa] | Aspect ratio lf/df |
|---|---|---|---|---|
| F1: RL-4550-BN * | 50 | 1.05 | 1000 | 47.6 |
| F2: RC-6535-BN * | 35 | 0.55 | 1100 | 63.6 |
| F3: RC-8030-BP * | 30 | 0.38 | 2300 | 78.9 |

| Concrete strength | Water to binder ratio | Water [kg/m3] | Cement [kg/m3] | Silica fume [kg/m3] | Sand [kg/m3] | Coarse aggregate [kg/m3] | Super-plasticizer [kg/m3] |
|---|---|---|---|---|---|---|---|
| Normal strength (N) | 0.35 | 200 | 572 | - | 798 | 627 | 1.430 |
| High strength (H) | 0.25 | 200 | 737 | 64 | 667 | 569 | 6.008 |
3. Test Results and Investigations
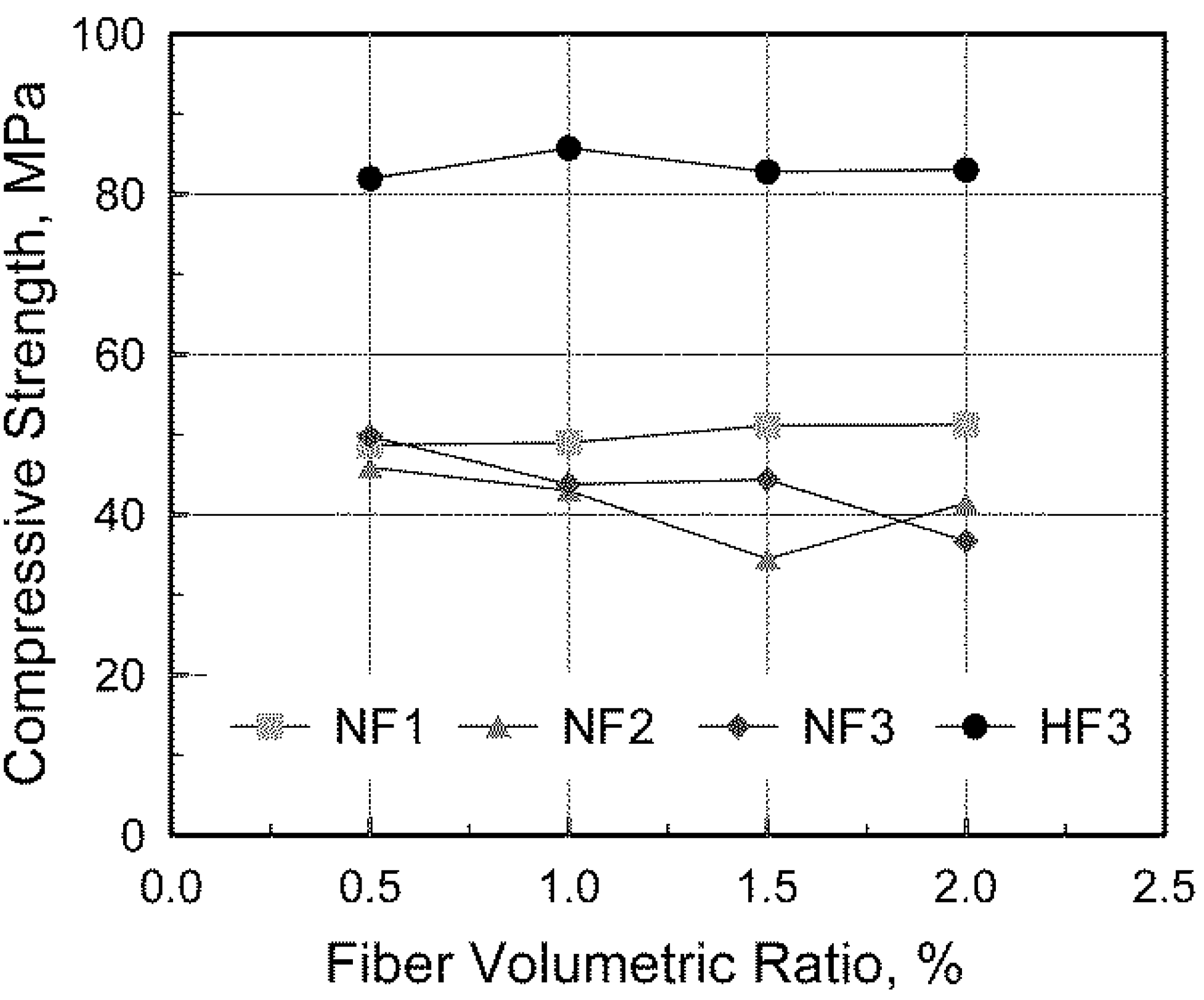
3.1. Pre-Peak Compressive Behavior
| Specimen. | Target strength [MPa] | [%] | [MPa] | [] | [MPa] | Slump [mm] | |
|---|---|---|---|---|---|---|---|
| NF1V1 | 50 | 45 | 0.5 | 48.7 | 3,137 | 25,406 | 146 |
| NF1V2 | 1.0 | 49.0 | 3,047 | 25,781 | 154 | ||
| NF1V3 | 1.5 | 51.1 | 3,190 | 25,187 | 129 | ||
| NF1V4 | 2.0 | 51.2 | 3,244 | 26,091 | 111 | ||
| NF2V1 | 65 | 0.5 | 45.9 | 2,701 | 29,987 | 113 | |
| NF2V2 | 1.0 | 43.0 | 3,150 | 22,812 | 135 | ||
| NF2V3 | 1.5 | 34.5 | 2,289 | 26,107 | 141 | ||
| NF2V4 | 2.0 | 41.4 | 3,187 | 21,758 | 83 | ||
| NF3V1 | 80 | 0.5 | 49.8 | 3,078 | 24,373 | 111 | |
| NF3V2 | 1.0 | 43.8 | 3,100 | 26,661 | 114 | ||
| NF3V3 | 1.5 | 44.4 | 3,422 | 20,640 | 83 | ||
| NF3V4 | 2.0 | 36.7 | 3,499 | 22,880 | 39 | ||
| HF3V1 | 80 | 80 | 0.5 | 81.9 | 3,191 | 31,328 | 225 |
| HF3V2 | 1.0 | 85.7 | 3,424 | 33,581 | 193 | ||
| HF3V3 | 1.5 | 82.8 | 3,288 | 33,855 | 164 | ||
| HF3V4 | 2.0 | 83.0 | 3,922 | 28,246 | 118 |


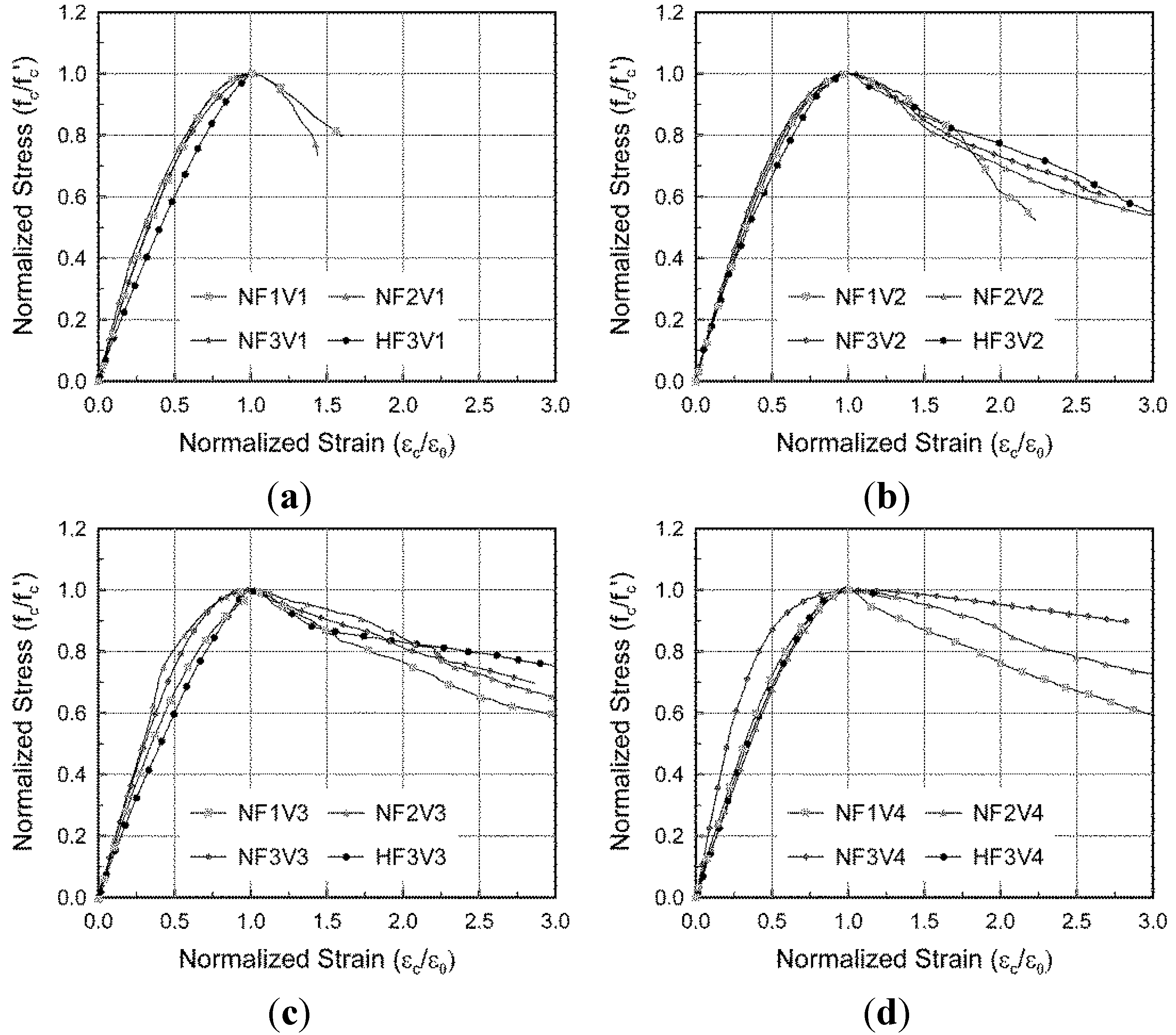
3.2. Post-Peak Compressive Behavior

4. Model for the Compressive Behavior of SFRC Members
4.1. Strain at the Compressive Strength

4.2. Elastic Modulus

4.3. Compressive Stress-Strain Relationship


5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ashour, S.A.; Hasanain, G.S.; Wafa, F.F. Shear behavior of high-strength fiber reinforced concrete beams. ACI Struct. J. 1992, 89, 176–184. [Google Scholar]
- Casanova, P.; Rossi, P.; Schaller, I. Can steel fibers replace transverse reinforcement in reinforced concrete beams? ACI Mater. J. 1997, 94, 341–354. [Google Scholar]
- Noghabai, K. Beams of fibrous concrete in shear and bending: Experiment and model. ASCE J. Struct. Eng. 2000, 126, 243–251. [Google Scholar] [CrossRef]
- Meda, A.; Plizzari, G.A. New design approach for steel fiber-reinforced concrete slabs-on-ground based on fracture mechanics. ACI Struct. J. 2004, 101, 298–303. [Google Scholar]
- Minelli, F.; Vechcio, F.J. Compression field modeling of fiber-reinforced concrete members under shear loading. ACI Struct. J. 2006, 103, 244–252. [Google Scholar]
- Dinh, H.H.; Parra-Montesinos, G.J.; Wight, J.K. Shear behavior of steel fiber-reinforced concrete beams without stirrup reinforcement. ACI Struct. J. 2010, 107, 597–606. [Google Scholar]
- Susetyo, J.; Gauvreau, P.; Vecchio, F.J. Effectiveness of steel fiber as minimum shear reinforcement. ACI Struct. J. 2011, 108, 488–496. [Google Scholar]
- Hwang, J.-H.; Lee, D.H.; Ju, H.; Kim, K.S.; Seo, S.-Y.; Kang, J.-W. Shear behavior models of steel fiber reinforced concrete beams modifying softened truss model approaches. Materials 2013, 6, 4847–4867. [Google Scholar] [CrossRef]
- Deluce, J.R.; Lee, S.-C.; Vecchio, F.J. Crack model for steel fiber-reinforced concrete members containing conventional reinforcement. ACI Struct. J. 2014, 111, 93–102. [Google Scholar]
- Shahnewaz, M.; Alam, M.S. Improved shear equations for steel fiber-reinforced concrete deep and slender beams. ACI Struct. J. 2014, 111, 851–860. [Google Scholar]
- Ezeldin, A.S.; Balaguru, P.N. Normal and high strength fiber reinforced concrete under compression. ASCE J. Mater. Civil Eng. 1992, 4, 415–429. [Google Scholar] [CrossRef]
- Hsu, L.S.; Hsu, C.T.T. Stress-strain behavior of steel-fiber high-strength concrete under compression. ACI Struct. J. 1994, 91, 448–457. [Google Scholar]
- Someh, A.K.; Saeki, N. Prediction for the stress-strain curve of steel fiber reinforced concrete. Proc. Jpn. Concr. Inst. 1994, 18, 1149–1154. [Google Scholar]
- Mansur, M.A.; Chin, M.S.; Wee, T.H. Stress-strain relationship of high-strength fiber concrete in compression. ASCE J. Mater. Civil Eng. 1999, 11, 21–29. [Google Scholar] [CrossRef]
- Nataraja, M.; Dhang, N.; Gupta, A. Stress-strain curves for steel-fiber reinforced concrete under compression. Cement Concr. Compos. 1999, 21, 383–390. [Google Scholar] [CrossRef]
- Bencardino, F.; Rizzuti, L.; Spadea, G.; Swamy, R.N. Stress-strain behavior of steel fiber-reinforced concrete in compression. J. Mater. Civil Eng. 2008, 20, 255–263. [Google Scholar] [CrossRef]
- Rizzuti, L.; Bencardino, F. Effects of fibre volume fraction on the compressive and flexural experimental behavior of SFRC. Contemp. Eng. Sci. 2014, 7, 379–390. [Google Scholar]
- Carreira, D.J.; Chu, K.H. Stress-strain relationship for plain concrete in compression. ACI J. 1985, 82, 797–804. [Google Scholar]
- Lee, S.-C.; Cho, J.-Y.; Vecchio, F.J. Diverse embedment model for steel fiber-reinforced concrete in tension: Model development. ACI Mater. J. 2011, 107, 516–525. [Google Scholar]
- Lee, S.-C.; Cho, J.-Y.; Vecchio, F.J. Diverse embedment model for steel fiber-reinforced concrete in tension: Model verification. ACI Mater. J. 2011, 107, 526–535. [Google Scholar]
- Oh, J.-H. Uniaxial Behavior of Steel Fiber Reinforced Concrete. Master’s Thesis, Seoul National University, Seoul, Korea, 28 August 2011. [Google Scholar]
- Susetyo, J. Fibre Reinforcement for Shrinkage Crack Control in Prestressed, Precast Segmental Bridges. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2009. [Google Scholar]
- ASTM C 469 Standard Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression; Annual Book of ASTM Standards; American Society for Testing and Materials: West Conshohocken, PA, USA, 2002.
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, S.-C.; Oh, J.-H.; Cho, J.-Y. Compressive Behavior of Fiber-Reinforced Concrete with End-Hooked Steel Fibers. Materials 2015, 8, 1442-1458. https://doi.org/10.3390/ma8041442
Lee S-C, Oh J-H, Cho J-Y. Compressive Behavior of Fiber-Reinforced Concrete with End-Hooked Steel Fibers. Materials. 2015; 8(4):1442-1458. https://doi.org/10.3390/ma8041442
Chicago/Turabian StyleLee, Seong-Cheol, Joung-Hwan Oh, and Jae-Yeol Cho. 2015. "Compressive Behavior of Fiber-Reinforced Concrete with End-Hooked Steel Fibers" Materials 8, no. 4: 1442-1458. https://doi.org/10.3390/ma8041442
APA StyleLee, S.-C., Oh, J.-H., & Cho, J.-Y. (2015). Compressive Behavior of Fiber-Reinforced Concrete with End-Hooked Steel Fibers. Materials, 8(4), 1442-1458. https://doi.org/10.3390/ma8041442






