Use of Time-of-Flight Ultrasound to Measure Wave Speed in Poplar Seedlings
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
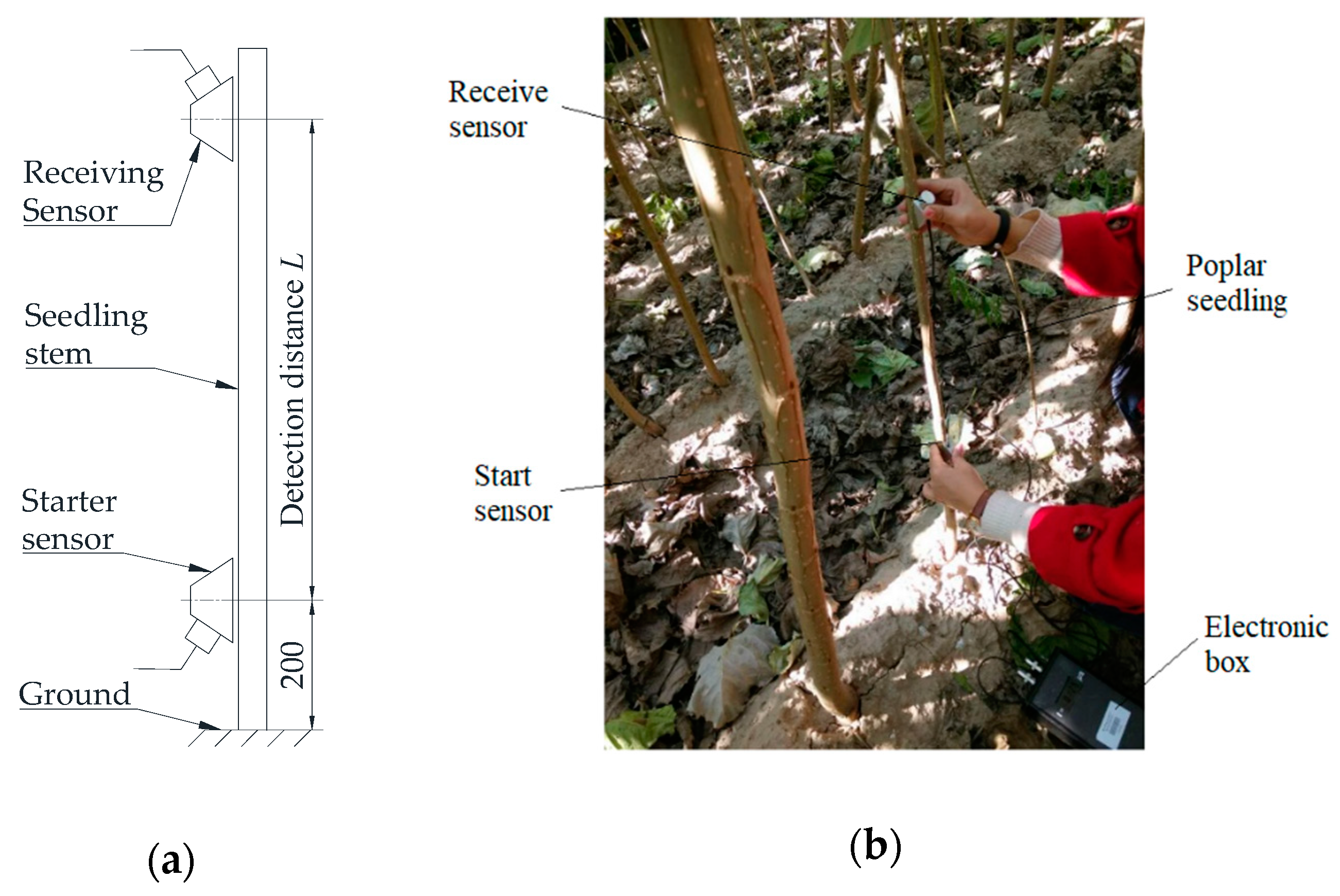
2.2.1. Ultrasonic Method
2.2.2. Acoustic Resonance Method
2.2.3. Density Measurement
3. Results and Discussion
3.1. Proper Detection Distance for Ultrasonic Tests
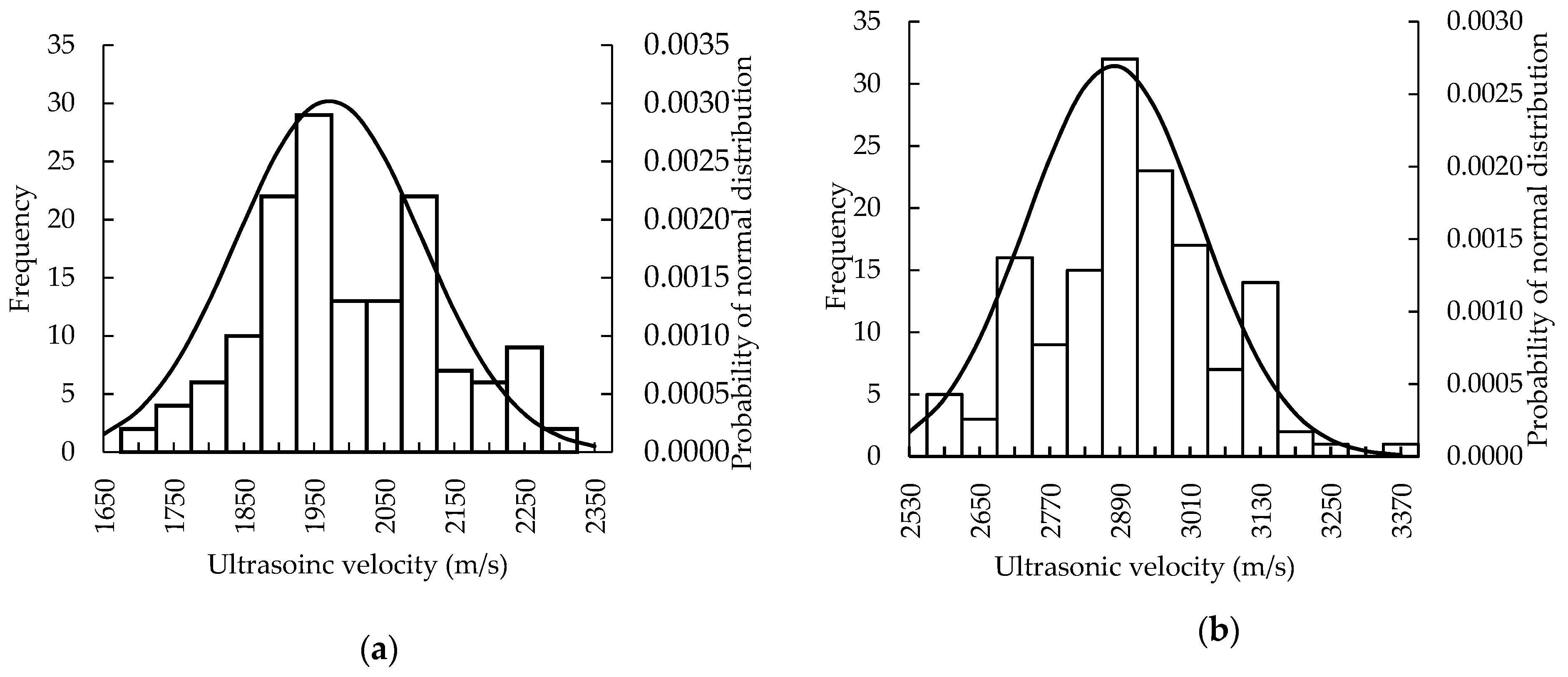
3.2. Ultrasonic Velocity and Root-Collar Diameters of Poplar Seedlings
3.3. Relationships Between Ultrasonic Velocity and Influencing Factors
3.3.1. Growth Days
3.3.2. Root-Collar Diameter
3.3.3. Density
3.3.4. Microfibril Angle
3.4. Comparison with the Results of Acoustic and Ultrasonic Tests
4. Conclusions
- The proper detection distance for the ultrasonic method to be applied to poplar seedlings is ranged from 265 mm to 665 mm, and 365 mm was used in this study.
- There were good correlations (R2 = 0.99) between the average ultrasonic velocities and growth days. Ultrasonic speed increased with growth days, within 209 growing days. However, almost no relationship was found between the ultrasonic velocities and the root-collar diameters of seedlings, i.e., ultrasonic speed does not seem to increase with increasing root-collar diameter.
- Even though ultrasonic velocity, in general, decreases with increasing density, the density of seedlings showed a weak influence on ultrasonic speed due to the low correlation between them (R2 = 0.26). However, ultrasonic velocity significantly decreased with the increasing microfibril angle of seedlings. The relations between ultrasonic speed and MFA are of great interest for the two sample seedlings, and MFA may have an impact on ultrasonic speed in poplar seedlings. More seedling specimens are definitely needed to verify this relationship and confirm the effect of MFA on ultrasonic velocity.
- There was a significant relationship between ultrasonic velocity and acoustic velocity, and a similar result was also found in the dynamic MOE values derived from the acoustic resonance test and the ultrasonic test, respectively.
- Other influencing factors that were excluded in this paper—such as MFA, temperature, and moisture content—need to be studied in future research to investigate their effect on ultrasonic velocity in seedlings.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Emms, G.W.; Nanayakkara, B.; Harrington, J.J. Application of longitudinal-wave time-of-flight sound speed measurement to Pinus radiata seedlings. Can. J. For. Res. 2013, 43, 750–756. [Google Scholar]
- Huang, Y.H.; Zhao, R.J.; Fei, B.H. Variation patterns of microfibril angle for Chinese fir wood. J. Northwest For. Univ. 2007, 22, 119–122. [Google Scholar]
- Sun, D.Y.; Yang, W.X.; Liu, Q.L.; Fang, S.Z. A study on geographic variation in wood microfibril angle of Cyclocarya paliurus. J. Nanjing For. Univ. Nat. Sci. Ed. 2018, 42, 81–85. [Google Scholar]
- Donaldson, L.A.; Burdon, R.D. Clonal variation and repeatability of microfibril angle in Pinus radiata. N. Z. J. For. Sci. 1995, 25, 164–174. [Google Scholar]
- Dungey, H.S.; Matheson, A.C.; Kain, D.; Evans, R. Genetics of wood stiffness and its component traits in Pinus radiata. Can. J. For. Res. 2006, 36, 1165–1178. [Google Scholar]
- Nakada, R. Within-tree variation of wood characteristics in conifers and the anatomical characteristics specific to very young trees. In The Compromised Wood Workshop 2007; University of Canterbury: Christchurch, New Zealand, 2007; pp. 51–67. [Google Scholar]
- Watt, M.S.; Sorensson, C.; Cown, D.J.; Dungey, H.S.; Evans, R. Determining the main and interactive effect of age and clone on wood density, microfibril angle, and modulus of elasticity for Pinus radiata. Can. J. For. Res. 2010, 40, 1550–1557. [Google Scholar]
- Emms, G.; Harrington, J.J. A novel technique for non-damaging measurement of sound speed in seedlings. Eur. J. For. Res. 2012, 131, 1449–1459. [Google Scholar]
- Hearmon, R.F.S. The influence of shear and rotatory inertia on the free flexural vibration of wooden beams. Br. J. Appl. Phys. 1958, 9, 381–388. [Google Scholar] [CrossRef]
- Bucur, V. Acoustics of Wood; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Yin, Y.F.; Nagao, H.; Liu, X.L. Mechanical properties assessment of Cunninghamia lanceolata plantation wood with three acoustic-based nondestructive methods. J. Wood Sci. 2010, 56, 33–40. [Google Scholar]
- Lachenbruch, B.; Johnson, G.R.; Downes, G.M.; Evans, R. Relationships of density, microfibril angle, and sound velocity with stiffness and strength in mature wood of Douglas-fir. Can. J. For Res. 2010, 40, 55–64. [Google Scholar]
- Todoroki, C.L.; Lowell, E.C.; Dykstra, D. Automated knot detection with visual post-processing of Douglas-fir veneer images. Comput. Electron. Agric. 2010, 70, 163–171. [Google Scholar]
- Chauhan, S.S.; Walker, J.C.F. Variations in acoustic velocity and density with age, and their interrelationships in radiata pine. For. Ecol. Manag. 2006, 229, 388–394. [Google Scholar]
- Toulmin, M.J.; Raymond, C.A. Developing a sampling strategy for measuring acoustic velocity in standing Pinus radiata using the treetap time of flight tool. N. Z. J. For Sci. 2007, 37, 96–111. [Google Scholar]
- Lindstrom, H.; Reale, M.; Grekin, M. Using non-destructive testing to assess modulus of elasticity of Pinus sylvestris trees. Scand. J. For. Res. 2009, 24, 247–257. [Google Scholar]
- Gerhards, C. Longitudinal stress-waves for lumber stress grading: Factors affecting applications: State of the art. For. Prod. J. 1982, 32, 20–25. [Google Scholar]
- Tsehaye, A.; Buchanan, A.H.; Walker, J.C.F. Sorting of logs using acoustics. Wood Sci. Technol. 2000, 34, 337–344. [Google Scholar]
- Carter, P.; Chauhan, S.S.; Walker, J.C.F. Sorting logs and lumber for stiffness using director HM200. Wood Fibre Sci. 2006, 38, 49–54. [Google Scholar]
- Huang, C.L.; Lambeth, C.C. Methods for Determining Potential Characteristics of a Specimen Based on Stress Wave Velocity Measurements. U.S. Patent Application No. 7340958, 11 March 2008. [Google Scholar]
- Divos, F. Acoustic tools for seedling, tree and log selection. Presented at the The Future of Quality Control for Wood & Wood Products—COST Action E53, Edinburgh, UK, 4–7 May 2010. [Google Scholar]
- ASTM G2395-17. Standard Test Methods for Density and Specific Gravity (Relative Density) of Wood and Wood-Based Materials; ASTM International: West Conshohocken, PA, USA, 2017. [Google Scholar]
- GB/T1933-2009. Method for Determination of the Density of Wood; Standardization Administration of China: Beijing, China, 2009. [Google Scholar]
- Ultrasonic Timer User’s Guide; FAKOPP: Budapest, Hungary, 2008.
- Wang, X.P.; Ross, R.J.; Carter, P. Acoustic evaluation of wood quality in standing trees. Part I. Acoustic wave behavior. Wood Fiber Sci. 2007, 39, 28–38. [Google Scholar]
- Chauhan, S.S.; Entwistle, K.M.; Walker, J.C.F. Differences in acoustic velocity by resonance and transit-time methods in an anisotropic laminated wood medium. Holzforschung 2005, 59, 428–434. [Google Scholar]
- Isik, F.; Mora, C.R.; Schimleck, L.R. Genetic variation in Pinus taeda wood properties predicted using non-destructive techniques. Ann. For. Sci. 2011, 68, 283–293. [Google Scholar]
- Hasegawa, M.; Takata, M.; Matsumura, J.; Oda, K. Effect of wood properties on within-tree variation in ultrasonic wave velocity in softwood. Ultrasonics 2011, 51, 296–302. [Google Scholar] [CrossRef] [PubMed]
- Krauss, A.; Kudela, J. Ultrasonic wave propagation and Young’s modulus of elasticity along the grain of Scots pine wood (Pinus Sylvestris L.) varying with distance from the pith. Wood Res. 2011, 56, 479–488. [Google Scholar]
- Chen, Z.Q.; Karlsson, B.; Lundqvist, S.O.; Maria, R.G.G.; Olsson, L.; Wu, H.X. Estimating solid wood properties using Pilodyn and acoustic velocity on standing trees of Norway spruce. Ann. For. Sci. 2015, 72, 499–508. [Google Scholar] [Green Version]
- Blackburn, D.; Farrell, R.; Hamilton, M.; Volker, P.; Harwood, C. Genetic improvement for pulpwood and peeled veneer in Eucalyptus nitens. Can. J. For. Res. 2012, 42, 1724–1732. [Google Scholar]
- Ribeiro, P.G.; Goncalez, J.C.; Goncalves, R.; Teles, R.F.; Souza, F.D. Ultrasound waves for assessing the technological properties of Pinus caribaea var hondurensis and Eucalyptus grandis wood. Maderas Cienc. Tecnol. 2013, 15, 195–204. [Google Scholar]
- Cown, D.J.; Hebert, J.; Ball, R. Modelling Pinus radiata lumber characteristics. Part 1: Mechanical properties of small clears. N. Z. J. For. Sci. 1999, 29, 203–213. [Google Scholar]











| Date | Root-Collar Diameter | Number | Average Root-Collar Diameter | Maximum Root-Collar Diameter | Minimum Root-Collar Diameter | Average Velocity | Maximum Velocity | Minimum Velocity |
|---|---|---|---|---|---|---|---|---|
| (mm) | (mm) | (mm) | (mm) | (m/s) | (m/s) | (m/s) | ||
| 24 July | d ≤ 10 | 15 | 8.4(1.5) | 10.0 | 4.9 | 1921(219) | 2241 | 1675 |
| 10 < d ≤ 20 | 101 | 15.4(2.9) | 20.0 | 10.3 | 1995(123) | 2269 | 1680 | |
| 20 < d ≤ 30 | 29 | 21.9(1.4) | 25.0 | 20.1 | 1919(122) | 2087 | 1748 | |
| 2 September | d ≤ 10 | 5 | 8.0(1.5) | 9.4 | 5.7 | 2435(116) | 2520 | 2283 |
| 10 < d ≤ 20 | 56 | 15.4(2.6) | 20.0 | 10.2 | 2518(153) | 2747 | 2136 | |
| 20 < d ≤ 30 | 79 | 24.9(2.7) | 29.9 | 20.2 | 2315(122) | 2707 | 1996 | |
| d > 30 | 5 | 31.6(1.1) | 33.3 | 30.5 | 2145(88) | 2283 | 2041 | |
| 20 September | d ≤ 10 | 5 | 8.0(1.6) | 9.7 | 5.7 | 2509(137) | 2667 | 2404 |
| 10 < d ≤ 20 | 49 | 15.4(2.6) | 19.5 | 10.2 | 2703(125) | 2923 | 2327 | |
| 20 < d ≤ 30 | 73 | 25.1(2.7) | 29.6 | 20.1 | 2484(134) | 2833 | 2228 | |
| d > 30 | 18 | 31.8(1.6) | 35.0 | 30.1 | 2328(57) | 2404 | 2188 | |
| 5 November | d ≤ 0 | 5 | 8.4(1.4) | 9.9 | 6.8 | 2669(281) | 2855 | 2555 |
| 10 < d ≤ 20 | 48 | 15.6(2.6) | 19.9 | 10.2 | 2953(107) | 3234 | 2707 | |
| 20 < d ≤ 30 | 71 | 25.4(2.8) | 29.8 | 20.5 | 2884(133) | 3353 | 2629 | |
| d > 30 | 21 | 32.5(1.7) | 36.3 | 30.1 | 2741(92) | 2971 | 2573 |
| Date | Number of Trees | Growth Days | Average Velocity | Standard Deviation | Upper Limit of Velocity | Lower Limit of Velocity |
|---|---|---|---|---|---|---|
| (m/s) | (m/s) | (m/s) | ||||
| 24 July | 145 | 105 | 1972 | 132 | 2368 | 1576 |
| 2 September | 145 | 145 | 2365 | 166 | 2865 | 1866 |
| 20 September | 145 | 165 | 2540 | 183 | 3087 | 1992 |
| 5 November | 145 | 209 | 2879 | 148 | 3323 | 2434 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Xu, P.; Zhang, H.; Guan, C.; Feng, D.; Wang, X. Use of Time-of-Flight Ultrasound to Measure Wave Speed in Poplar Seedlings. Forests 2019, 10, 682. https://doi.org/10.3390/f10080682
Liu F, Xu P, Zhang H, Guan C, Feng D, Wang X. Use of Time-of-Flight Ultrasound to Measure Wave Speed in Poplar Seedlings. Forests. 2019; 10(8):682. https://doi.org/10.3390/f10080682
Chicago/Turabian StyleLiu, Fenglu, Pengfei Xu, Houjiang Zhang, Cheng Guan, Dan Feng, and Xiping Wang. 2019. "Use of Time-of-Flight Ultrasound to Measure Wave Speed in Poplar Seedlings" Forests 10, no. 8: 682. https://doi.org/10.3390/f10080682
APA StyleLiu, F., Xu, P., Zhang, H., Guan, C., Feng, D., & Wang, X. (2019). Use of Time-of-Flight Ultrasound to Measure Wave Speed in Poplar Seedlings. Forests, 10(8), 682. https://doi.org/10.3390/f10080682




