Assessment of Effective Wind Loads on Individual Plantation-Grown Forest Trees
Abstract
:1. Introduction
2. Material and Methods
2.1. Workflow
2.2. Research Site and Forest Characteristics
2.3. Airflow Measurements
2.4. Stem Tilt Measurements
2.5. Non-Destructive Tree Pulling
2.6. Processing and Analysis of Stem Displacement Data
2.7. Calculation of Effective Wind Load
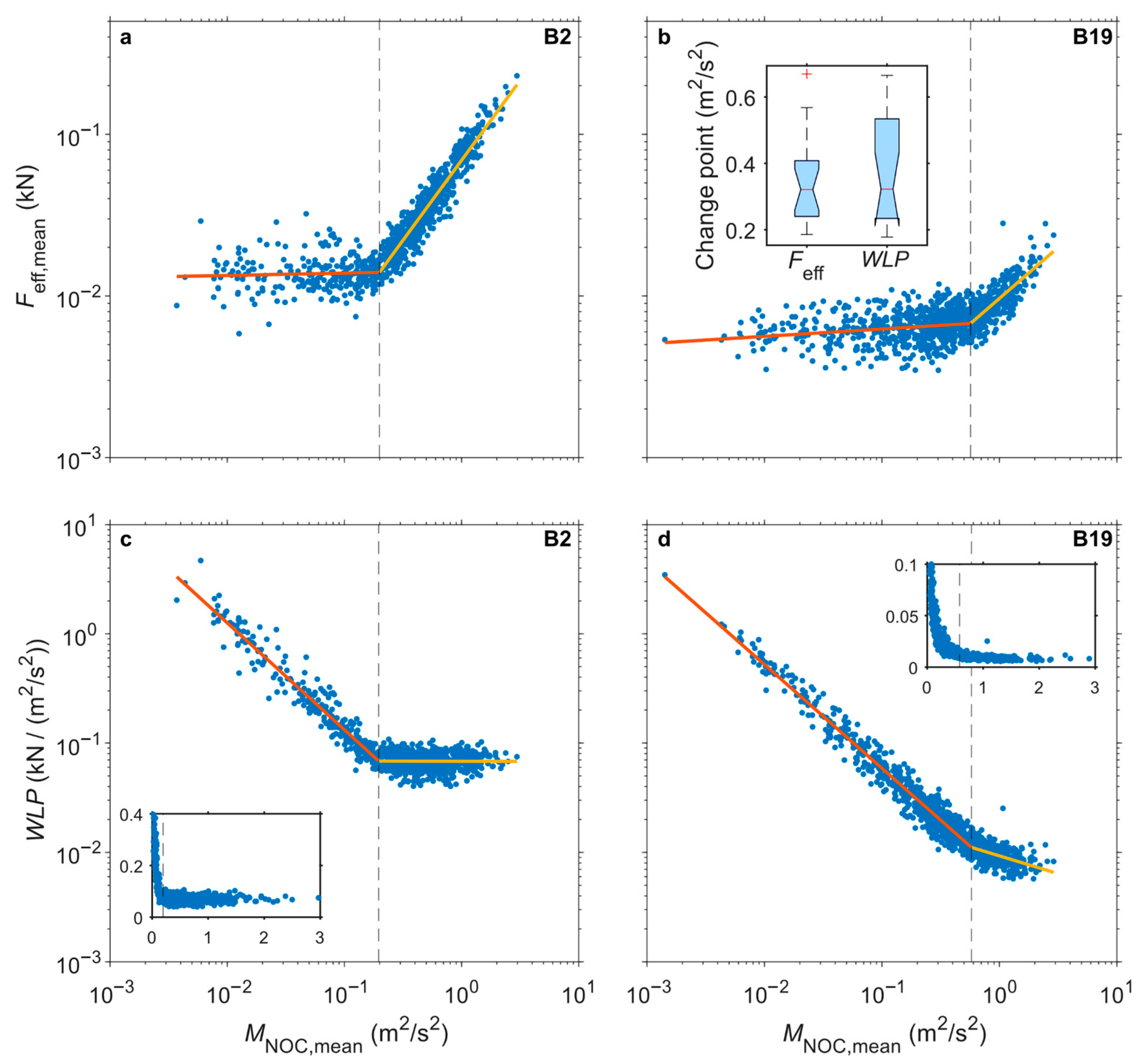
2.8. Change Point Analysis
3. Results and Discussion
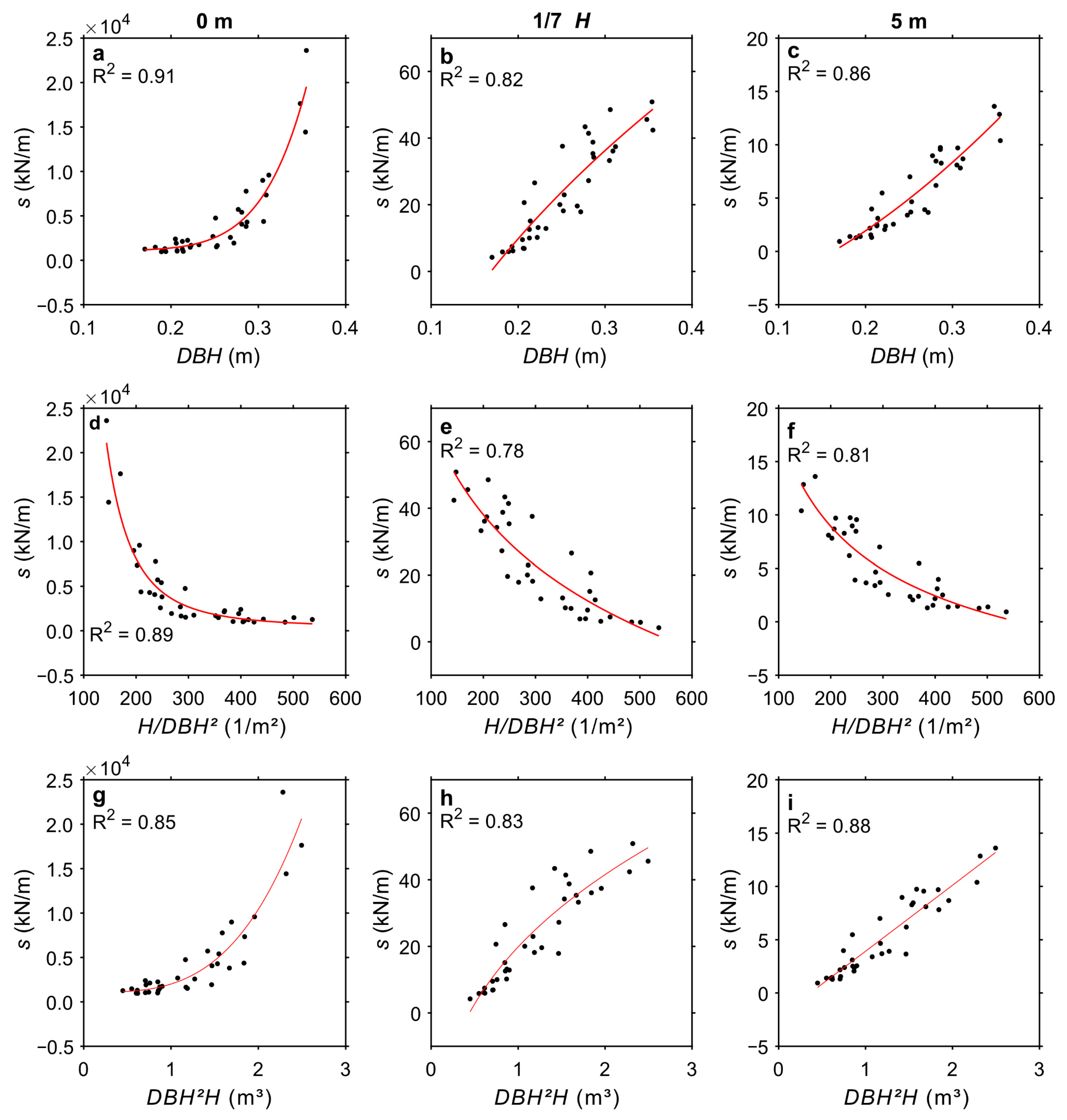
3.1. Non-Destructive Tree Pulling
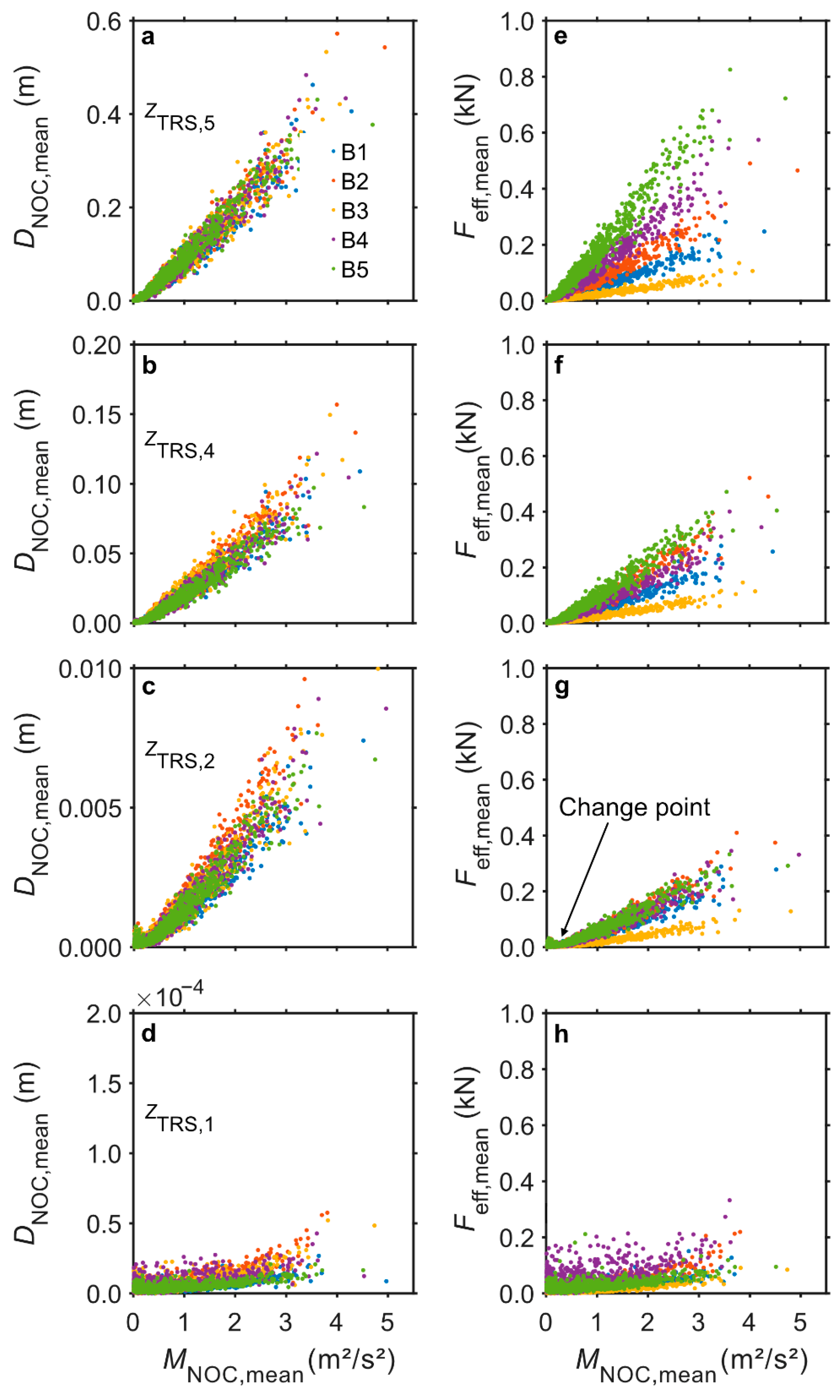
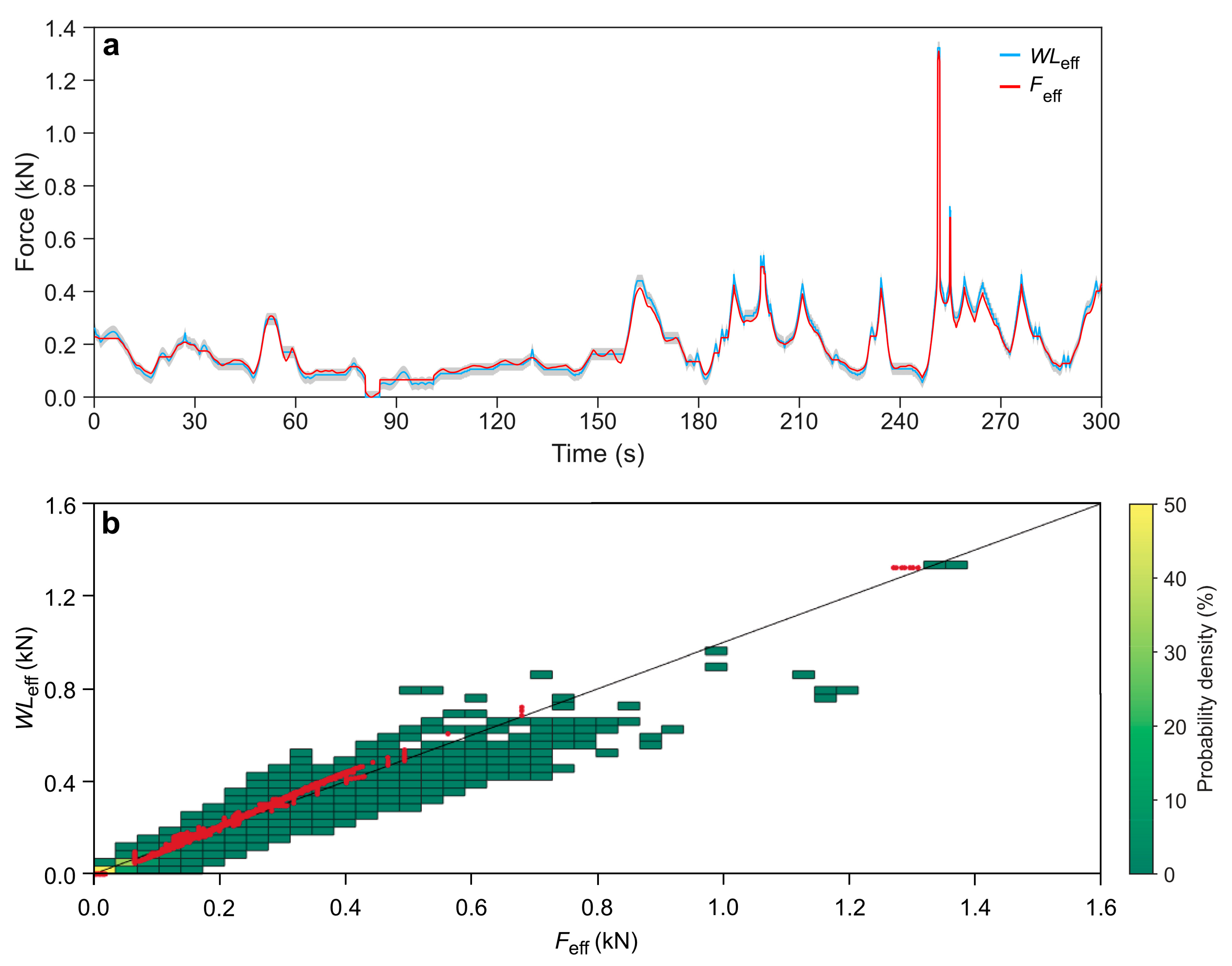
3.2. Tree Response under Natural Wind Conditions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Acronyms | Description |
| α | significance level |
| c | above-ground tree characteristic |
| CA | crown area (m2) |
| D | stem displacement (m) |
| DNOC | non-oscillatory component of stem displacement (m) |
| DNOC,mean | 10 min mean value of DNOC |
| DOC | oscillatory component of stem displacement (m) |
| DLP | spline-smoothed stem displacement during tree pulling tests (m) |
| DBH | diameter at breast height (cm) |
| Fa | force applied along the rope into the pulling direction (N) |
| Feff | effective force (N) |
| Feff,mean | 10 min mean value of Feff |
| FP | horizontal component of the pulling force at pulling rope attachment height during tree pulling tests (N) |
| FPLP | Low-pass filtered (SSA) bending moment (N) |
| FZV | effective load during pulling test (N) |
| H | tree height (m) |
| M | above-canopy momentum flux density (m²/s²) |
| MNOC | low-pass filtered component of above-canopy momentum flux (m²/s²) |
| MNOC,mean | 10 min mean value of MNOC |
| p | p-value of the applied regression analyses |
| r | correlation coefficient |
| r2 | coefficient of determination |
| RA | pulling rope angle between za and anchorage point at the ground (°) |
| s | slope of the regression line determined between DLP and FPLP during tree pulling (N/m) |
| tx | stem tilt in x direction (east-west) (°) |
| ty | stem tilt in y direction (north-south) (°) |
| u | horizontal wind vector component in east-west direction (m/s) |
| v | horizontal wind vector component in north-south direction (m/s) |
| w | vertical wind vector component (m/s) |
| WLeff | effective wind load (N) |
| WLC | wind load coefficient (kN/(m2/s2)) |
| WLP | wind load parameter (kN/(m2/s2)) |
| za | attachment height of the pulling rope (m) |
| zPTQ | measurement height above ground of TreeQinetic sensors (m) |
| zTRS | measurement height above ground of the Tree Response Sensor (m) |
| Abbreviations | Description |
| PTQ | Picus TreeQinect |
| S | ultrasonic anemometer |
| SSA | singular spectrum analysis |
| B | sample tree |
| TLS | terrestrial laser scanning |
| TRS | tree response sensor |
| TreeMMoSys | tree motion monitoring system |
References
- Schindler, D.; Bauhus, J.; Mayer, H. Wind effects on trees. Eur. J. For. Res. 2012, 131, 159–163. [Google Scholar] [CrossRef] [Green Version]
- Bonnesoeur, V.; Constant, T.; Moulia, B.; Fournier, M. Forest trees filter chronic wind-signals to acclimate to high winds. New Phytol. 2016, 210, 850–860. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gardiner, B. Wind damage to forests and trees: A review with an emphasis on planted and managed forests. J. For. Res. 2021, 26, 248–266. [Google Scholar] [CrossRef]
- Jackson, T.D.; Sethi, S.; Dellwik, E.; Angelou, N.; Bunce, A.; van Emmerik, T.; Duperat, M.; Ruel, J.-C.; Wellpott, A.; Van Bloem, S.; et al. The motion of trees in the wind: A data synthesis. Biogeosciences 2021, 18, 4049–4072. [Google Scholar] [CrossRef]
- Mayer, H. Wind-induced tree sways. Trees 1987, 1, 195–206. [Google Scholar] [CrossRef]
- Gardiner, B.A. The interactions of wind and tree movement in forest canopies. In Wind and Trees; Coutts, M.P., Grace, J., Eds.; Cambridge University Press: Cambridge, UK, 1995; pp. 41–59. [Google Scholar] [CrossRef]
- Gardiner, B.; Berry, P.; Moulia, B. Review: Wind impacts on plant growth, mechanics and damage. Plant. Sci. 2016, 245, 94–118. [Google Scholar] [CrossRef]
- De Langre, E. Plant vibrations at all scales: A review. J. Exp. Bot. 2019, 70, 3521–3531. [Google Scholar] [CrossRef]
- Gardiner, B.; Byrne, K.; Hale, S.; Kamimura, K.; Mitchell, S.J.; Peltola, H.; Ruel, J.-C. A review of mechanistic model-ling of wind damage risk to forests. Forestry 2008, 81, 447–463. [Google Scholar] [CrossRef] [Green Version]
- Jackson, T.; Shenkin, A.; Wellpott, A.; Calders, K.; Origo, N.; Disney, M.; Burt, A.; Raumonen, P.; Gardiner, B.; Herold, M.; et al. Finite element analysis of trees in the wind based on terrestrial laser scanning data. Agric. For. Meteorol. 2019, 265, 137–144. [Google Scholar] [CrossRef]
- Usbeck, T.; Wohlgemuth, T.; Dobbertin, M.; Pfister, C.; Bürgi, A.; Rebetez, M. Increasing storm damage to forests in Switzerland from 1858 to 2007. Agric. For. Meteorol. 2010, 150, 47–55. [Google Scholar] [CrossRef]
- Valta, H.; Lehtonen, I.; Laurila, T.K.; Venäläinen, A.; Laapas, M.; Gregow, H. Communicating the amount of windstorm induced forest damage by the maximum wind gust speed in Finland. Adv. Sci. Res. 2019, 16, 31–37. [Google Scholar] [CrossRef] [Green Version]
- Albrecht, A.; Jung, C.; Schindler, D. Improving empirical storm damage models by coupling with high-resolution gust speed data. Agric. For. Meteorol. 2019, 268, 23–31. [Google Scholar] [CrossRef]
- Schindler, D.; Jung, C.; Buchholz, A. Using highly resolved maximum gust speed as predictor for forest storm damage caused by the high-impact winter storm Lothar in Southwest Germany. Atmos. Sci. Lett. 2016, 17, 462–469. [Google Scholar] [CrossRef] [Green Version]
- Ancelin, P.; Courbaud, B.; Fourcaud, T. Development of an individual tree-based mechanical model to predict wind damage within forest stands. For. Ecol. Manag. 2004, 203, 101–121. [Google Scholar] [CrossRef]
- Dupont, S.; Ikonen, V.-P.; Väisänen, H.; Peltola, H. Predicting tree damage in fragmented landscapes using a wind risk model coupled with an airflow model. Can. J. For. Res. 2015, 45, 1065–1076. [Google Scholar] [CrossRef]
- Gardiner, B.; Peltola, H.; Kellomäki, S. Comparison of two models for predicting the critical winds required to damage coniferous trees. Ecol. Model. 2000, 129, 1–23. [Google Scholar] [CrossRef]
- Peltola, H.; Kellomäki, S.; Vaisanen, H.; Ikonen, V.-P. A mechanistic model for assessing the risk of wind and snow damage to single trees and stands of Scots pine, Norway spruce, and birch. Can. J. For. Res. 1999, 29, 647–661. [Google Scholar] [CrossRef]
- Quine, C.P.; Gardiner, B.A.; Moore, J. Wind disturbance in forests: The process of wind created gaps, tree overturning, and stem breakage. In Plant Disturbance Ecology—The Process and the Response; Johnson, E.A., Miyanishi, K., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 117–184. [Google Scholar] [CrossRef]
- Seidl, R.; Schelhaas, M.J.; Lexer, M.J. Unraveling the drivers of intensifying forest disturbance regimes in Europe. Glob. Chang. Biol. 2011, 17, 2842–2852. [Google Scholar] [CrossRef]
- Schelhaas, M.-J.; Nabuurs, G.-J.; Schuck, A. Natural disturbances in the European forests in the 19th and 20th centuries. Glob. Chang. Biol. 2003, 9, 1620–1633. [Google Scholar] [CrossRef]
- Ulanova, N.G. The effects of windthrow on forests at different spatial scales: A review. For. Ecol. Manag. 2000, 135, 155–167. [Google Scholar] [CrossRef]
- Lindroth, A.; Lagergren, F.; Grelle, A.; Klemendtsson, L.; Langvall, O.; Weslien, P.; Tuulik, J. Storms can cause Europe-wide reduction in forest carbon sink. Glob. Chang. Biol. 2009, 15, 346–355. [Google Scholar] [CrossRef]
- Magnabosco Marra, D.; Trumbore, S.E.; Higuchi, N.; Ribeiro, G.H.P.M.; Negrón-Juárez, R.I.; Holzwarth, F.; Rifai, S.W.; dos Santos, J.; Lima, A.J.N.; Kinupp, V.F.; et al. Windthrows control biomass patterns and functional composition of Amazon forests. Glob. Chang. Biol. 2018, 24, 5867–5881. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Reyer, C.P.O.; Bathgate, S.; Blennow, K.; Borges, J.G.; Bugmann, H.; Delzon, S.; Faias, S.P.; Garcia-Gonzalo, J.; Gardiner, B.; Gonzalez-Olabarria, J.R.; et al. Are forest disturbances amplifying or canceling out climate change-induced productivity changes in European forests? Environ. Res. Lett. 2017, 12, 034027. [Google Scholar] [CrossRef] [PubMed]
- Dahle, A.G.; James, K.R.; Kane, B.; Grabosky, J.C.; Detter, A. A review of factors that affect the static load-bearing capacity of urban trees. Arboricul. Urban For. 2017, 43, 89–106. [Google Scholar] [CrossRef]
- Abetz, P.; Künstle, E. Zur Druckholzbildung bei Fichte. Allg. Forst Und Jagdztg. 1982, 153, 117–127. [Google Scholar]
- Duncker, P.; Spiecker, H. Compression wood formation and pith eccentricity in Picea abies L. depending on selected site-related factors: Detection of compression wood by its spectral properties in reflected light. In Proceedings of the TRACE—Tree Rings in Archaeology, Climatology and Ecology, Bormernsdorf, Switzerland, 22–24 April 2004; pp. 150–158. [Google Scholar]
- Schindler, D.; Vogt, R.; Fugmann, H.; Rodriguez, M.; Schönborn, J.; Mayer, H. Vibration behavior of plantation-grown Scots pine trees in response to wind excitation. Agric. For. Meteorol. 2010, 150, 984–993. [Google Scholar] [CrossRef]
- Schindler, D.; Mohr, M. Non-oscillatory response to wind loading dominates movement of Scots pine trees. Agric. For. Meteorol. 2018, 250, 209–216. [Google Scholar] [CrossRef]
- Schindler, D.; Mohr, M. No resonant response of Scots pine trees to wind excitation. Agric. For. Meteorol. 2019, 265, 227–244. [Google Scholar] [CrossRef]
- Schindler, D.; Kolbe, S. Assessment of the response of a Scots pine tree to effective wind loading. Forests 2020, 11, 145. [Google Scholar] [CrossRef] [Green Version]
- Angelou, N.; Dellwik, E.; Mann, J. Wind load estimation on an open-grown European oak tree. Forestry 2019, 92, 381–392. [Google Scholar] [CrossRef]
- Kane, B.; Clouston, P. Tree pulling tests of large shade trees in the genus Acer. Arboricul. Urban For. 2008, 34, 101–109. [Google Scholar] [CrossRef]
- Moore, J. Differences in maximum resistive bending moments of Pinus radiata trees grown on a range of soil types. For. Ecol. Manag. 2000, 135, 63–71. [Google Scholar] [CrossRef]
- Nicoll, B.C.; Gardiner, B.A.; Rayner, B.; Peace, A.J. Anchorage of coniferous trees in relation to species, soil type, and rooting depth. Can. J. For. Res. 2006, 36, 1871–1883. [Google Scholar] [CrossRef]
- Rahardjo, H.; Harnas, F.R.; Indrawan, I.G.B.; Leong, E.C.; Tan, P.Y.; Fong, Y.K.; Ow, L.F. Understanding the stability of Samanea saman trees through tree pulling, analytical calculations and numerical models. Urban For. Urban Green. 2014, 13, 355–364. [Google Scholar] [CrossRef]
- Duperat, M.; Gardiner, B.; Ruel, J.-C. Testing an individual tree wind damage risk model in a naturally regenerated balsam fir stand: Potential impact of thinning on the level of risk. Forestry 2021, 94, 141–150. [Google Scholar] [CrossRef]
- Peltola, H. Mechanical stability of trees under static loads. Am. J. Bot. 2006, 93, 1501–1511. [Google Scholar] [CrossRef]
- Kolbe, S.; Schindler, D. TreeMMoSys: A low cost sensor network to measure wind-induced tree response. HardwareX 2021, 9, e00180. [Google Scholar] [CrossRef]
- EM (Environmental Meteorology, University of Freiburg, Germany). Hartheim Forest Research Site. Available online: https://www.meteo.uni-freiburg.de/en/infrastructure/hartheim-forest-research-site (accessed on 31 December 2021).
- CloudCompare (version 2.12) (GPL software). 3D Point Cloud and Mesh Processing Software Open Source Project. Available online: http://www.cloudcompare.org/ (accessed on 31 December 2021).
- Stull, R.B. An Introduction to Boundary Layer Meteorology; Springer: Dordrecht, The Netherlands, 2009. [Google Scholar] [CrossRef]
- Detter, A.; Rust, C.; Maybaum, G. Determining strength limits for standing tree stems from bending tests. In Proceedings of the 18th International Nondestructive Testing and Evaluation of Wood Symposium, Madison, WI, USA, 24–27 September 2013; US Department of Agriculture, Forest Service, Forest Products Laboratory: Madison, WI, USA. Available online: https://www.tree-consult.org/upload/mediapool/pdf/baumstatik_und_biomechanik/detter_etal-ndt-proceedings-2014.pdf (accessed on 13 June 2022).
- Detter, A.; Rust, S. Findings of recent research on the pulling test method. In Jahrbuch der Baumpflege; Dujesiefken, D., Ed.; Haymarket Media GmbH Buch- und Zeitschriftenverlage: Braunschweig, Germany, 2013; pp. 87–100. [Google Scholar]
- Broomhead, D.; King, G. Extracting qualitative dynamics from experimental data. Phys. D 1986, 20, 217–236. [Google Scholar] [CrossRef]
- Vautard, R.; Ghil, M. Singular spectrum analysis in nonlinear dynamics with applications to paleoclimatic time series. Phys. D 1989, 35, 395–424. [Google Scholar] [CrossRef]
- Sellier, D.; Brunet, Y.; Fourcaud, T. A numerical model of tree aerodynamic response to a turbulent airflow. Forestry 2008, 81, 279–297. [Google Scholar] [CrossRef] [Green Version]
- Schindler, D.; Schönborn, J.; Fugmann, H.; Mayer, H. Responses of an individual deciduous broadleaved tree to wind excitation. Agric. For. Meteorol. 2013, 177, 69–82. [Google Scholar] [CrossRef]
- Sakoe, H.; Chiba, S. Dynamic programming algorithm optimization for spoken word recognition. IEEE Trans. Acoust. Speech 1978, 26, 43–48. [Google Scholar] [CrossRef] [Green Version]
- Paliwal, K.K.; Agarwal, A.; Sinha, S.S. A modification over Sakoe and Chiba’s dynamic time warping algorithm for isolated word recognition. Signal. Process. 1982, 4, 329–333. [Google Scholar] [CrossRef]
- Krišāns, O.; Čakša, L.; Matisons, R.; Rust, S.; Elferts, D.; Seipulis, A.; Jansons, Ā. A Static Pulling Test Is a Suitable Method for Comparison of the Loading Resistance of Silver Birch (Betula pendula Roth.) between Urban and Peri-Urban Forests. Forests 2022, 13, 127. [Google Scholar] [CrossRef]
- Atanasov, D. Two-Phase Linear Regression Model. Available online: https://www.mathworks.com/matlabcentral/fileexchange/26804-two-phase-linear-regression-model MATLAB Central File Exchange (accessed on 10 March 2022).
- Papesch, A.J.G.; Moore, J.R.; Hawke, A.E. Mechanical stability of Pinus Radiata trees at Eyrewell Forest investigated using static tests. N. Z. J. For. Sci. 1997, 27, 188–204. [Google Scholar]
- Peltola, H.; Nykänen, M.-L.; Kellomäki, S. Model computations on the critical combination of snow loading and windspeed for snow damage of scots pine, Norway spruce and Birch sp. at stand edge. For. Ecol. Manag. 1997, 95, 229–241. [Google Scholar] [CrossRef]
- Moore, J.R.; Maguire, D.A. Natural sway frequencies and damping ratios of trees: Concepts, review and synthesis of previous studies. Trees 2004, 18, 195–203. [Google Scholar] [CrossRef]
- Moore, J.R.; Maguire, D.A. Natural sway frequencies and damping ratios of trees: Influence of crown structure. Trees 2005, 19, 363–373. [Google Scholar] [CrossRef]
- Fredericksen, T.S.; Hedden, R.L.; Williams, S.A. Testing loblolly pine wind firmness with simulated wind stress. Can. J. For. Res. 1993, 23, 1760–1765. [Google Scholar] [CrossRef]
- Hale, S.E.; Gardiner, B.A.; Wellpott, A.; Nicoll, B.C.; Achim, A. Wind loading of trees: Influence of tree size and competition. Eur. J. Forest. Res. 2012, 131, 203–217. [Google Scholar] [CrossRef]
- Hale, S.E.; Gardiner, B.A.; Peace, A.; Nicoll, B.; Taylor, P.; Pizzirani, S. Comparison and validation of three versions of a forest wind risk model. Environ. Model. Softw. 2015, 68, 27–41. [Google Scholar] [CrossRef] [Green Version]
- Peltola, H.; Kellomäki, S.; Hassinen, A.; Granander, M. Mechanical stability of Scots pine, Norway spruce and birch: An analysis of tree-pulling experiments in Finland. For. Ecol. Manag. 2000, 135, 143–153. [Google Scholar] [CrossRef]

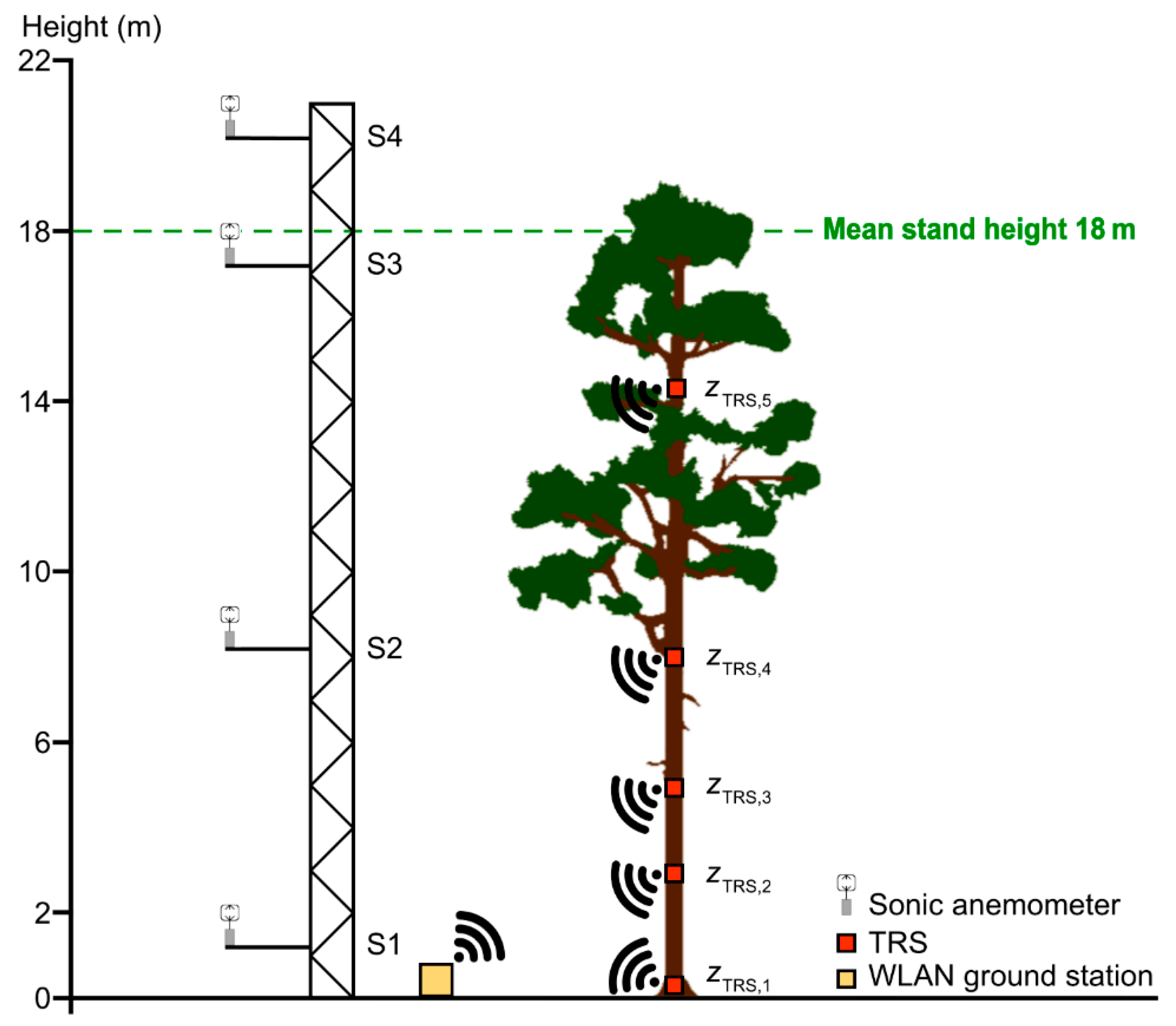



| Sample Tree | H | DBH | CA | za | RA | zTRS,1 | zTRS,2 | zTRS,3 | zTRS,4 | zTRS,5 |
|---|---|---|---|---|---|---|---|---|---|---|
| B1 | 18.5 | 25.1 | 26.0 | 11.2 | 18.5 | 0.1 | 2.7 | 5.0 | 8.2 | 13.7 |
| B2 | 20.4 | 28.6 | 31.2 | 13.4 | 17.9 | 0.1 | 2.9 | 5.0 | 8.6 | 14.3 |
| B3 | 16.7 | 23.2 | 18.0 | 9.7 | 16.6 | 0.1 | 2.6 | 5.0 | 7.8 | 12.9 |
| B4 | 19.4 | 28.6 | 15.9 | 11.2 | 18.4 | 0.1 | 2.8 | 5.0 | 8.4 | 13.9 |
| B5 | 18.5 | 27.7 | 27.0 | 11.9 | 22.7 | 0.1 | 2.7 | 5.0 | 8.1 | 13.5 |
| B6 | 17.7 | 26.8 | 27.3 | 11.7 | 26.8 | 0.1 | 2.5 | 5.0 | ||
| B7 | 19.6 | 30.6 | 40.4 | 10.6 | 19.2 | 0.1 | 2.8 | 5.0 | ||
| B8 | 17.7 | 21.9 | 26.6 | 9.7 | 22.5 | 0.1 | 2.5 | 5.0 | ||
| B9 | 17.5 | 22.3 | 27.0 | 9.6 | 27.9 | 0.1 | 2.5 | 5.0 | ||
| B10 | 16.5 | 20.7 | 17.9 | 9.5 | 22.3 | 0.1 | 2.4 | 5.0 | ||
| B11 | 18.5 | 21.4 | 24.0 | 9.8 | 19.6 | 0.1 | 2.6 | 5.0 | ||
| B12 | 18.7 | 25.2 | 33.3 | 11.5 | 23.4 | 0.1 | 2.7 | 5.0 | ||
| B13 | 19.6 | 28.1 | 31.4 | 11.6 | 27.8 | 0.1 | 2.8 | 5.0 | ||
| B14 | 18.8 | 21.3 | 21.3 | 10.8 | 26.2 | 0.1 | 2.7 | 5.0 | ||
| B15 | 19.8 | 27.2 | 24.5 | 11.5 | 27.5 | 0.1 | 2.8 | 5.0 | ||
| B16 | 17.6 | 22.2 | 24.9 | 11.6 | 27.8 | 0.1 | 2.5 | 5.0 | ||
| B17 | 17.4 | 20.7 | 23.5 | 10.7 | 25.9 | 0.1 | 2.5 | 5.0 | ||
| B18 | 18.3 | 25.3 | 19.8 | 11.1 | 32.4 | 0.1 | 2.6 | 5.0 | ||
| B19 | 16.5 | 19.3 | 18.1 | 10.7 | 29.7 | 0.1 | 2.4 | 5.0 | ||
| B20 | 17.5 | 24.8 | 22.8 | 10.4 | 30.5 | 0.1 | 2.5 | 5.0 | ||
| B21 | 18.2 | 30.5 | 34.5 | 10.5 | 27.5 | 0.1 | 2.6 | 5.0 | ||
| B22 | 20.6 | 34.8 | 53.8 | 12.8 | 32.6 | 0.1 | 2.9 | 5.0 | ||
| B23 | 18.1 | 35.5 | 47.6 | 12.3 | 39.2 | 0.1 | 2.6 | 5.0 | ||
| B24 | 16.8 | 20.6 | 14.0 | 10.7 | 25.5 | 0.1 | 2.4 | 5.0 | ||
| B25 | 16.7 | 21.3 | 19.4 | 11.6 | 21.1 | 0.1 | 2.4 | 5.0 | ||
| B26 | 15.5 | 17.0 | 11.1 | 11.8 | 30.5 | 0.1 | 2.2 | 5.0 | ||
| B27 | 18.5 | 35.4 | 40.8 | 11.9 | 29.9 | 0.1 | 2.6 | 5.0 | ||
| B28 | 16.8 | 20.5 | 14.3 | 10.7 | 39.9 | 0.1 | 2.4 | 5.0 | ||
| B29 | 20.1 | 31.2 | 32.1 | 10.8 | 29.0 | 0.1 | 2.9 | 5.0 | ||
| B30 | 16.0 | 19.4 | 13.1 | 11.2 | 39.9 | 0.1 | 2.3 | 5.0 | ||
| B31 | 17.3 | 18.9 | 14.7 | 11.5 | 35.0 | 0.1 | 2.5 | 5.0 | ||
| B32 | 16.6 | 18.2 | 13.1 | 10.0 | 25.8 | 0.1 | 2.4 | 5.0 | ||
| B33 | 18.6 | 28.1 | 28.5 | 11.0 | 28.8 | 0.1 | 2.7 | 5.0 | ||
| B34 | 18.6 | 28.7 | 37.6 | 12.2 | 29.7 | 0.1 | 2.7 | 5.0 | ||
| B35 | 19.3 | 30.9 | 40.2 | 12.0 | 36.2 | 0.1 | 2.8 | 5.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolbe, S.; Rentschler, F.; Frey, J.; Seifert, T.; Gardiner, B.; Detter, A.; Schindler, D. Assessment of Effective Wind Loads on Individual Plantation-Grown Forest Trees. Forests 2022, 13, 1026. https://doi.org/10.3390/f13071026
Kolbe S, Rentschler F, Frey J, Seifert T, Gardiner B, Detter A, Schindler D. Assessment of Effective Wind Loads on Individual Plantation-Grown Forest Trees. Forests. 2022; 13(7):1026. https://doi.org/10.3390/f13071026
Chicago/Turabian StyleKolbe, Sven, Felix Rentschler, Julian Frey, Thomas Seifert, Barry Gardiner, Andreas Detter, and Dirk Schindler. 2022. "Assessment of Effective Wind Loads on Individual Plantation-Grown Forest Trees" Forests 13, no. 7: 1026. https://doi.org/10.3390/f13071026
APA StyleKolbe, S., Rentschler, F., Frey, J., Seifert, T., Gardiner, B., Detter, A., & Schindler, D. (2022). Assessment of Effective Wind Loads on Individual Plantation-Grown Forest Trees. Forests, 13(7), 1026. https://doi.org/10.3390/f13071026







