Friction Coefficients of Chestnut (Castanea sativa Mill.) Sawn Timber for Numerical Simulation of Timber Joints
Abstract
:1. Introduction
1.1. Literature Review: Timber-to-Timber Friction
1.2. Literature Review: Timber-to-Steel Friction
2. Materials and Methods
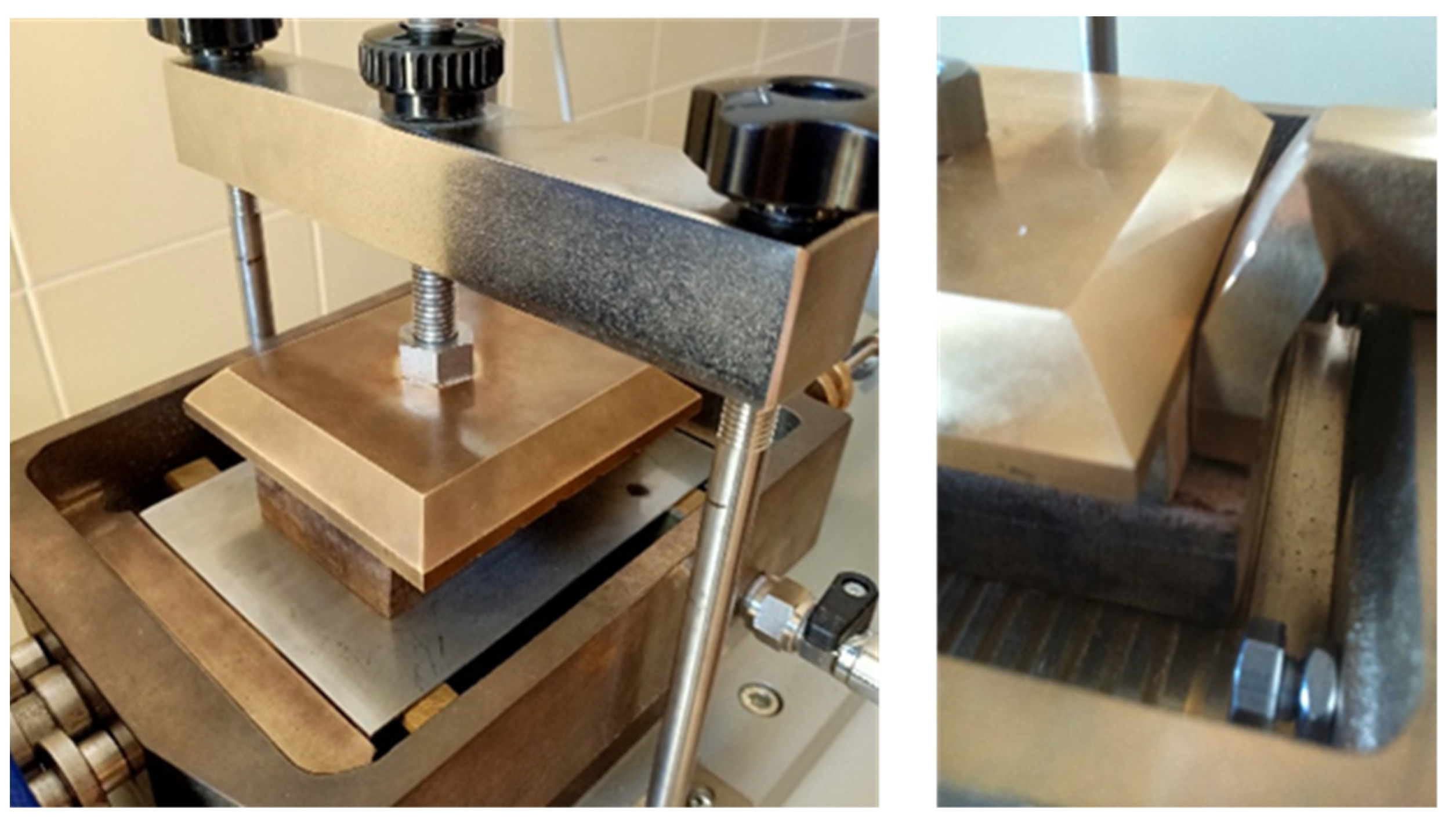
2.1. Test Implementation
2.2. Materials
- -
- Radial sliding direction, following the radius of the annular rings, (A).
- -
- Tangential sliding direction, tangential to the annular rings, (B).
- -
- Sliding direction parallel to the grain, (C).
- -
- Sliding direction perpendicular to the grain, (D).
- -
- Sliding direction parallel to the grain, in tangential, (E).
- -
- Sliding direction perpendicular to the grain, (F).
3. Results and Discussion
3.1. Timber-to-Timber Friction Tests
3.2. Timber-to-Steel Friction Tests
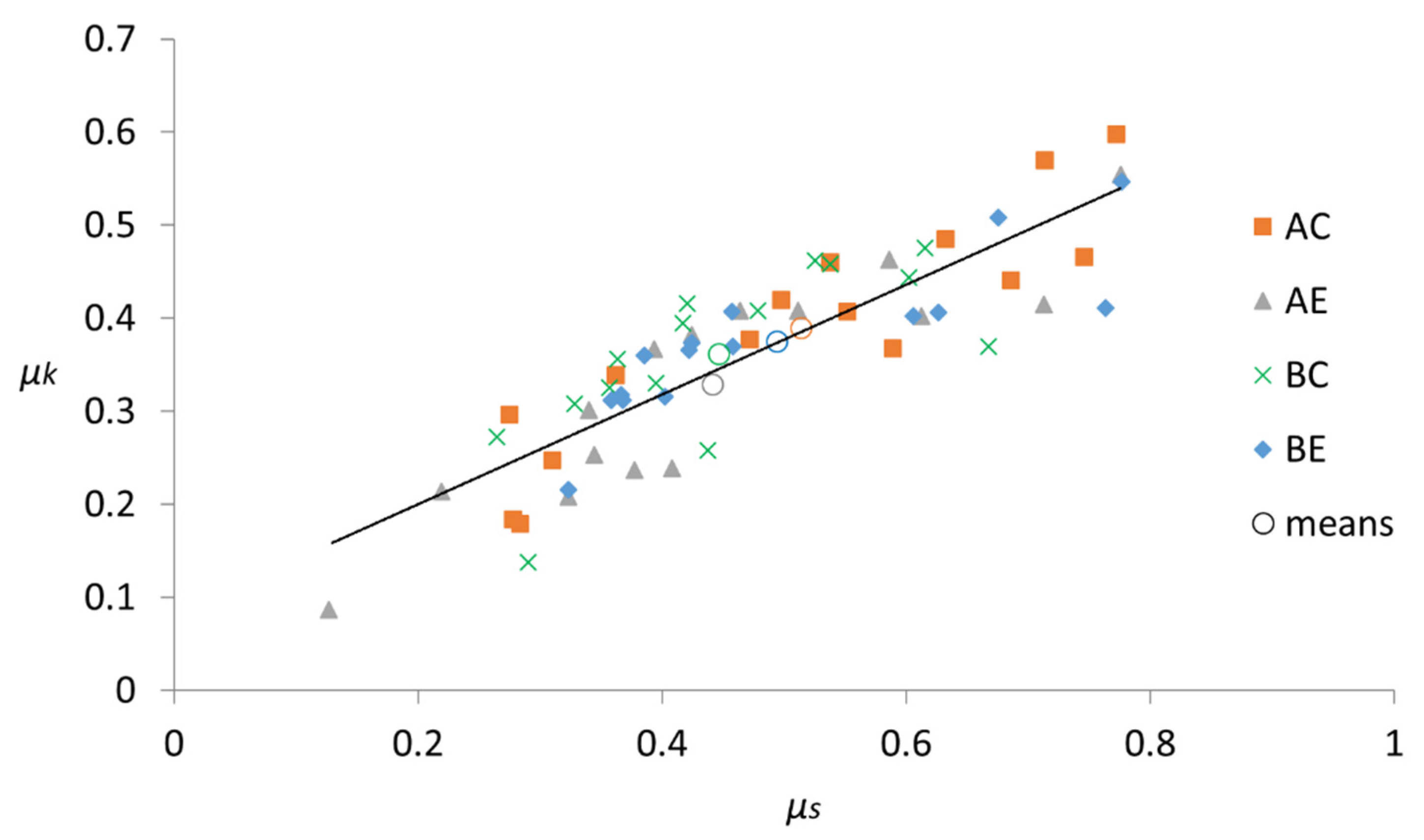
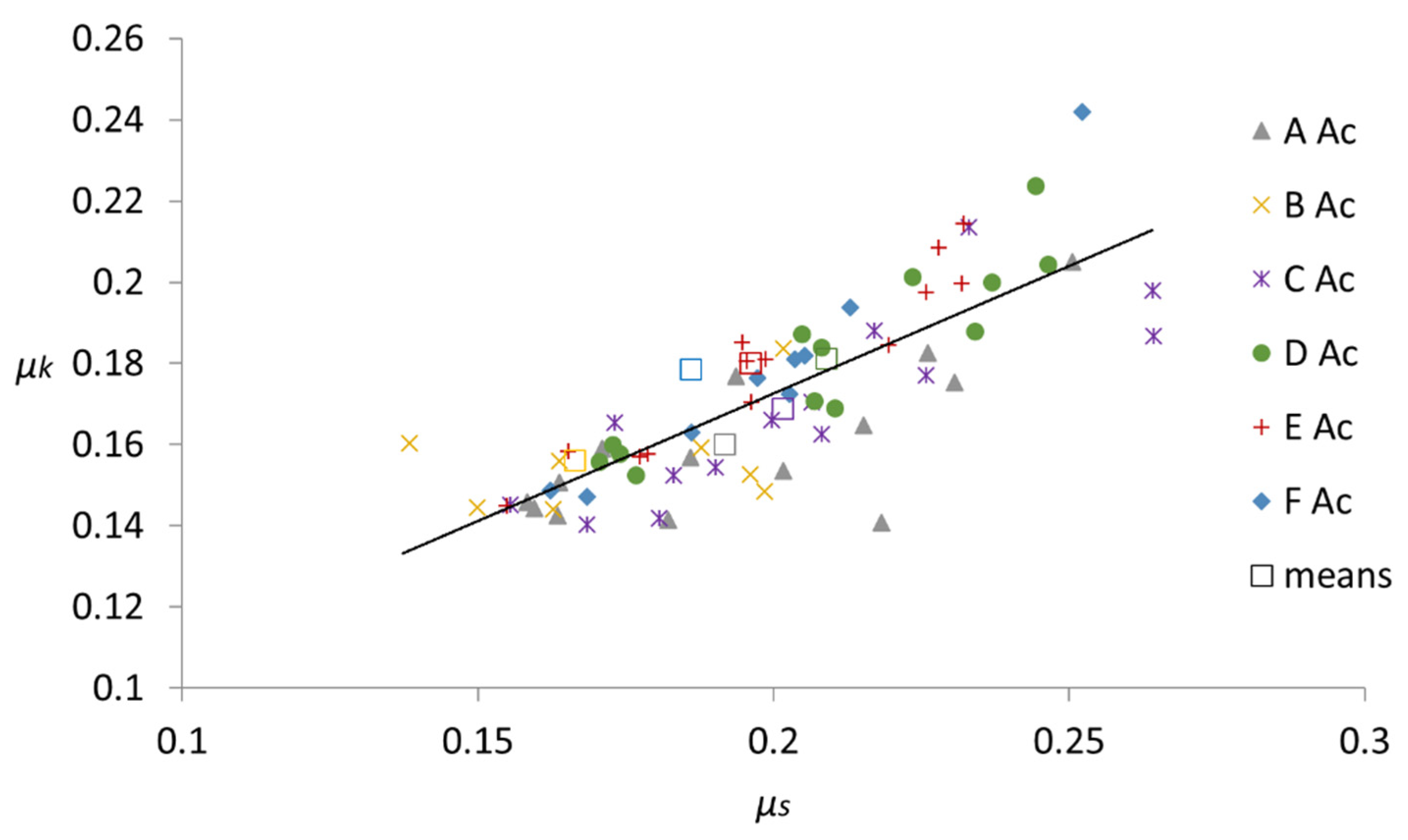
3.3. Correlation between μs and μk
4. Patents
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thaler, N.; Žlahtič, M.; Humar, M. Performance of Recent and Old Sweet Chestnut (Castanea Sativa) Wood. Int. Biodeterior. Biodegrad. 2014, 94, 141–145. [Google Scholar] [CrossRef]
- Topaloglu, E.; Ustaomer, D.; Ozturk, M.; Pesman, E. Changes in Wood Properties of Chestnut Wood Structural Elements with Natural Aging. Maderas. Cienc. Tecnol. 2021, 23, 1–12. [Google Scholar] [CrossRef]
- Carbone, F.; Moroni, S.; Mattioli, W.; Mazzocchi, F.; Romagnoli, M.; Portoghesi, L. Competitiveness and Competitive Advantages of Chestnut Timber Laminated Products. Ann. For. Sci. 2020, 77, 51. [Google Scholar] [CrossRef]
- UNE 56546; Visual Grading for Structural Sawn Timber. Hardwood Timber. AENOR: Madrid, Spain, 2013.
- FAO; UNECE. Forest Products-Annual Market Review 2020–2021; United Nations Economic Commission for Europe: Geneva, Switzerland, 2021; ISBN 978-92-1-117279-9. [Google Scholar]
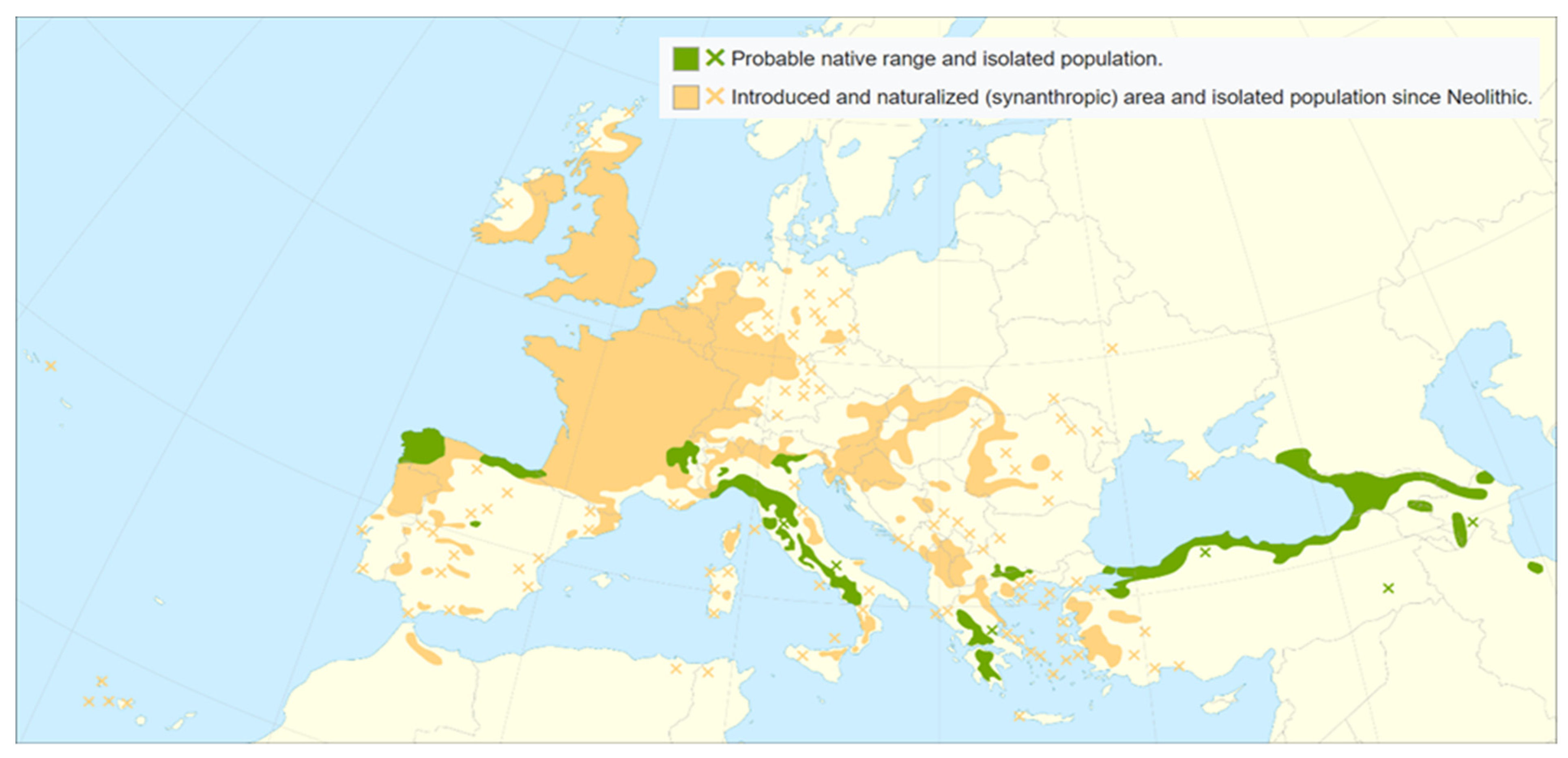
- Conedera, M.; Manetti, M.C.; Giudici, F.; Amorini, E. Distribution and Economic Potential of the Sweet Chestnut (Castanea Sativa Mill.) in Europe. Ecol. Mediterr. 2004, 30, 179–193. [Google Scholar] [CrossRef]
- Ministerio para la Transición Ecológica y el Reto Demográfico. In Informe Sobre El Estado Del Patrimonio Natural y de La Biodiversidad En España a 2020; Ministerio para la Transición Ecológica y el Reto Demográfico (MITECO): Madrid, Spain, 2021.
- Caudullo, G.; Welk, E.; San-Miguel-Ayanz, J. Chorological Maps for the Main European Woody Species. Data Brief 2017, 12, 662–666. [Google Scholar] [CrossRef] [PubMed]
- Beccaro, G.; Alma, A.; Bounous, G.; Gomes-Laranjo, J. (Eds.) The Chestnut Handbook: Crop & Forest Management; CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2020; ISBN 978-0-429-44560-6. [Google Scholar]
- Feio, A.O.; Lourenço, P.B.; Machado, J.S. Non-Destructive Evaluation of the Mechanical Behavior of Chestnut Wood in Tension and Compression Parallel to Grain. Int. J. Archit. Herit. 2007, 1, 272–292. [Google Scholar] [CrossRef] [Green Version]
- Mancini, M.; Leoni, E.; Nocetti, M.; Urbinati, C.; Duca, D.; Brunetti, M.; Toscano, G. Near Infrared Spectroscopy for Assessing Mechanical Properties of Castanea Sativa Wood Samples. J. Agric. Eng. 2019, 50, 191–197. [Google Scholar] [CrossRef]
- Vega, A.; Dieste, A.; Guaita, M.; Majada, J.; Baño, V. Modelling of the Mechanical Properties of Castanea Sativa Mill. Structural Timber by a Combination of Non-Destructive Variables and Visual Grading Parameters. Eur. J. Wood Prod. 2012, 70, 839–844. [Google Scholar] [CrossRef]
- Faggiano, B.; Grippa, M.R.; Marzo, A.; Mazzolani, F.M. Experimental Study for Non-Destructive Mechanical Evaluation of Ancient Chestnut Timber. J. Civil Struct. Health Monit. 2011, 1, 103–112. [Google Scholar] [CrossRef]
- Vega, A.; González, L.; Fernández, I.; González, P. Grading and Mechanical Characterization of Small-Diameter Round Chestnut (Castanea Sativa Mill.) Timber from Thinning Operations. Wood Mater. Sci. Eng. 2019, 14, 81–87. [Google Scholar] [CrossRef]
- Ay, N.; Şahin, H. Some Mechanical Properties of Chestnut (Castanea Sativa, Mill.) Wood Obtained from Maçka-Çatak Region. Artvin Çoruh Üniversitesi Orman Fakültesi Derg. 2011, 3, 87–95. [Google Scholar]
- Clair, B.; Ruelle, J.; Thibaut, B. Relationship Between Growth Stress, Mechanical-Physical Properties and Proportion of Fibre with Gelatinous Layer in Chestnut (Castanea Sativa Mill.). Holzforschung 2003, 57, 189–195. [Google Scholar] [CrossRef]
- Marini, F.; Manetti, M.C.; Corona, P.; Portoghesi, L.; Vinciguerra, V.; Tamantini, S.; Kuzminsky, E.; Zikeli, F.; Romagnoli, M. Influence of Forest Stand Characteristics on Physical, Mechanical Properties and Chemistry of Chestnut Wood. Sci. Rep. 2021, 11, 1549. [Google Scholar] [CrossRef] [PubMed]
- Tamantini, S.; Bergamasco, S.; Portoghesi, L.; Vettraino, A.M.; Zikeli, F.; Mugnozza, G.S.; Romagnoli, M. Detection, Description, and Technological Properties of Colour Aberration in Wood of Standards and Shoots from a Chestnut (Castanea Sativa Mill.) Coppice Stand. Eur. J. Forest Res. 2022, 1–16. [Google Scholar] [CrossRef]
- EN 350; Durability of Wood and Wood-Based Products—Testing and Classification of the Durability to Biological Agents of Wood and Wood-Based Materials. CEN: Brussels, Belgium, 2016.
- Villar, J.R.; Guaita, M.; Vidal, P.; Arriaga, F. Analysis of the Stress State at the Cogging Joint in Timber Structures. Biosyst. Eng. 2007, 96, 79–90. [Google Scholar] [CrossRef]
- Villar-García, J.R.; Crespo, J.; Moya, M.; Guaita, M. Experimental and Numerical Studies of the Stress State at the Reverse Step Joint in Heavy Timber Trusses. Mater. Struct. 2018, 51, 17. [Google Scholar] [CrossRef]
- Villar, J.R.; Guaita, M.; Vidal, P.; Argüelles, R. Numerical Simulation of Framed Joints in Sawn-Timber Roof Trusses. Span. J. Agric. Res. 2008, 6, 508–520. [Google Scholar] [CrossRef] [Green Version]
- Aira, J.R.; Íñiguez-González, G.; Guaita, M.; Arriaga, F. Load Carrying Capacity of Halved and Tabled Tenoned Timber Scarf Joint. Mater. Struct. 2016, 49, 5343–5355. [Google Scholar] [CrossRef]
- Koch, H.; Eisenhut, L.; Seim, W. Multi-Mode Failure of Form-Fitting Timber Connections—Experimental and Numerical Studies on the Tapered Tenon Joint. Eng. Struct. 2013, 48, 727–738. [Google Scholar] [CrossRef]
- Villar-García, J.R.; Vidal-López, P.; Crespo, J.; Guaita, M. Analysis of the Stress State at the Double-Step Joint in Heavy Timber Structures. Mater. Construcción 2019, 69, 196e. [Google Scholar] [CrossRef] [Green Version]
- Dorn, M.; Habrova, K.; Koubek, R.; Serrano, E. Determination of Coefficients of Friction for Laminated Veneer Lumber on Steel under High Pressure Loads. Friction 2021, 9, 367–379. [Google Scholar] [CrossRef]
- Sjodin, J.; Serrano, E.; Enquist, B. An Experimental and Numerical Study of the Effect of Friction in Single Dowel Joints. Holz Als Roh-Und Werkst. 2008, 66, 363–372. [Google Scholar] [CrossRef]
- Crespo, J.; Regueira, R.; Soilan, A.; Díez, M.R.; Guaita, M. Methodology to Determine the Coefficients of Both Static and Dynamic Friction Apply to Different Species of Wood. In Proceeding of the 1st Ibero-Latin American Congress on Wood in Construction. CIMAD, Coimbra, Portugal, 11 June 2011. [Google Scholar]
- Hu, W.; Liu, N. Numerical and Optimal Study on Bending Moment Capacity and Stiffness of Mortise-and-Tenon Joint for Wood Products. Forests 2020, 11, 501. [Google Scholar] [CrossRef]
- Wilkinson, G.; Augarde, C. A Serviceability Investigation of Dowel-Type Timber Connections Featuring Single Softwood Dowels. Eng. Struct. 2022, 260, 114210. [Google Scholar] [CrossRef]
- Li, G.; Liu, Z.; Tang, W.; He, D.; Shan, W. Experimental and Numerical Study on the Flexural Performance of Assembled Steel-Wood Composite Slab. Sustainability 2021, 13, 3814. [Google Scholar] [CrossRef]
- Friedrich, K.; Akpan, E.I.; Wetzel, B. On the Tribological Properties of Extremely Different Wood Materials. Eur. J. Wood Wood Prod. 2021, 79, 977–988. [Google Scholar] [CrossRef]
- Tipler, P.A. Physics for Scientists and Engineers, 6th ed.; W.H. Freeman: New York, NY, USA, 2008; ISBN 9781429202657/1429202653. [Google Scholar]
- Serway, R.A.; Jewett, J.W. Physics for Scientists and Engineers with Modern Physics; Cengage Learning: New York, NY, USA, 2013; ISBN 9781133954057. [Google Scholar]
- Villar-García, J.R.; Vidal-López, P.; Corbacho, A.J.; Moya, M. Determination of the Friction Coefficients of Chestnut (Castanea Sativa Mill.) Sawn Timber. Int. Agrophysics 2020, 34, 65–77. [Google Scholar] [CrossRef]
- CEN EN 1995-2:2016; Eurocode 5: Design of Timber Structures—Part. 2. Bridges. European Committee for Standardisation: Brussels, Belgium, 2016.
- Argüelles, R.; Arriaga, F.; Esteban, M.; Iñíguez, G.; Argüelles Bustillo, R. Timber Structures. Joints; AITIM; Technical Research Association of the Wood and Cork Industries: Madrid, Spain, 2015; ISBN 9788487381485. (In Spanish) [Google Scholar]
- McKenzie, W.M.; Karpovich, H. The Frictional Behaviour of Wood. Wood Sci. Technol. 1968, 2, 139–152. [Google Scholar] [CrossRef]
- Kollmann, F. Wood Technology and Its Applications. Technologie Des Loses Und Der Holzwerkstoffe; Instituto Forestal de Investigaciones y experiencias y servicio de la madera; Ministerio de Agricultura: Madrid, Spain, 1959. [Google Scholar]
- Aira, J.R.; Arriaga, F.; Iniguez-Gonzalez, G.; Crespo, J. Static and Kinetic Friction Coefficients of Scots Pine (Pinus Sylvestris L.), Parallel and Perpendicular to Grain Direction. Mater. De Constr. 2014, 64, e030. [Google Scholar] [CrossRef] [Green Version]
- Fu, W.; Guan, H.; Chen, B. Investigation on the Influence of Moisture Content and Wood Section on the Frictional Properties of Beech Wood Surface. Tribol. Trans. 2021, 64, 830–840. [Google Scholar] [CrossRef]
- Xu, M.; Li, L.; Wang, M.; Luo, B. Effects of Surface Roughness and Wood Grain on the Friction Coefficient of Wooden Materials for Wood–Wood Frictional Pair. Tribol. Trans. 2014, 57, 871–878. [Google Scholar] [CrossRef]
- McMillin, C.W.; Lemoine, T.J.; Manwiller, F.G. Friction Coefficient of Oven-Dry Spruce Pine on Steel, as Related to Temperature and Wood Properties. Wood Fiber Sci. 1970, 197, 6–11. [Google Scholar]
- USDA. Forest Products Laboratory Wood Handbook: Wood as an Engineering Material. General Technical Report FPL-GTR-190; USDA: Madison, WI, USA, 2010. [Google Scholar]
- Seki, M.; Sugimoto, H.; Miki, T.; Kanayama, K.; Furuta, Y. Wood Friction Characteristics during Exposure to High Pressure: Influence of Wood/Metal Tool Surface Finishing Conditions. J. Wood Sci. 2013, 59, 10–16. [Google Scholar] [CrossRef]
- ASTM International ASTM G115-10(2018); Standard Guide for Measuring and Reporting Friction Coefficients. Am. Soc. Testing Materials: West Conshohocken, PA, USA, 2018.
- Villar García, J.R.; Vidal López, P.Y.; Moya Ignacio, M. Device to Perform Friction Tests between Solid Bodies. Utility Model U 201932027. 2020. Available online: https://patentscope.wipo.int/search/en/detail.jsf?docId=ES289318649 (accessed on 7 July 2022).
- EN 338; Structural Timber—Strength Classes. CEN: Brussels, Belgium, 2016.
- CEN EN 1995-1-1:2016; Eurocode 5: Design of Timber Structures—Part. 1.1 General. Common Rules and Rules for Buildings. European Committee for Standardisation: Brussels, Belgium, 2016.
- CEN EN 408:2011+A1 2012; Timber Structures—Structural Timber and Glued Laminated Timber—Determination of Some Physical and Mechanical Properties. European Committee for Standardization: Brussels, Belgium, 2012.
- EN 13183-1; Moisture Content of a Piece of Sawn Timber—Part 1: Determination by Oven Dry Method. CEN: Brussels, Belgium, 2002.
- EN 13183-2; Moisture Content of a Piece of Sawn Timber—Part 2: Estimation by Electrical Resistance Method. CEN: Brussels, Belgium, 2002.
- Mohler, K.; Herroder, W. Range of the coefficient of friction of spruce wood rough from sawing. Holz Als Roh-Und Werkst. 1979, 37, 27–32. [Google Scholar] [CrossRef]
- Li, L.; Gong, M.; Li, D.G. Evaluation of the Kinetic Friction Performance of Modified Wood Decking Products. Constr. Build. Mater. 2013, 40, 863–868. [Google Scholar] [CrossRef]






| Property | Range |
|---|---|
| Density in green condition | 700–1100 kg/m3 |
| Density at 12% MC | 470–700 kg/m3 (Average: 580 kg/m3) |
| Total linear shrinkage | Axial: 0.6% Radial:4.1% Tangential: 6.1% (Average volumetric: 10.8%) |
| T/R anisotropy ratio | Around 1.5 |
| Compression strength | 21–64 N/mm2 (Average: 51 N/mm2) |
| Bending strength | 50–140 N/mm2 (Average: 86 N/mm2) |
| Modulus of elasticity on bending | 8450–14,400 N/mm2 (Average: 11,380 N/mm2) |
| Shear strength | 5.7 to 9.2 N/mm2 (Average: 7.3 N/mm2) |
| Shock resistance and hardness | Low to medium |
| Durability to basidiomycetes | Class 2 [19] |
| Durability to xylophagous insects | Class D [19] |
| Durability to termites | Class M [19] |
| Treatability | Class 4—extremely difficult |
| Use class complying with natural durability | Class 3—for external use (but not in ground contact or with permanent humidification) |
| Specimen | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | Average (CoV %) | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A–C | μs | 0.31 | 0.28 | 0.69 | 0.59 | 0.53 | 0.55 | 0.28 | 0.28 | 0.77 | 0.71 | 0.36 | 0.63 | 0.54 | 0.75 | 0.47 | 0.51 (34.6) |
| μk | 0.25 | 0.30 | 0.44 | 0.37 | 0.42 | 0.41 | 0.18 | 0.18 | 0.60 | 0.57 | 0.34 | 0.49 | 0.46 | 0.47 | 0.38 | 0.39 (32.1) | |
| A–E | μs | 0.41 | 0.38 | 0.13 | 0.46 | 0.42 | 0.22 | 0.51 | 0.39 | 0.34 | 0.61 | 0.71 | 0.34 | 0.78 | 0.32 | 0.59 | 0.44 (39.7) |
| μk | 0.24 | 0.24 | 0.09 | 0.41 | 0.38 | 0.21 | 0.41 | 0.37 | 0.25 | 0.40 | 0.42 | 0.30 | 0.55 | 0.21 | 0.46 | 0.33 (37.0) | |
| B–C | μs | 0.33 | 0.39 | 0.29 | 0.44 | 0.60 | 0.67 | 0.42 | 0.36 | 0.36 | 0.62 | 0.42 | 0.53 | 0.54 | 0.48 | 0.26 | 0.45 (27.3) |
| μk | 0.31 | 0.33 | 0.14 | 0.26 | 0.44 | 0.37 | 0.39 | 0.32 | 0.35 | 0.48 | 0.40 | 0.46 | 0.46 | 0.40 | 0.27 | 0.36 (25.6) | |
| B–E | μs | 0.77 | 0.37 | 0.40 | 0.63 | 0.76 | 0.36 | 0.37 | 0.39 | 0.32 | 0.46 | 0.68 | 0.46 | 0.61 | 0.42 | 0.42 | 0.49 (31.0) |
| μk | 0.55 | 0.32 | 0.32 | 0.41 | 0.41 | 0.31 | 0.31 | 0.36 | 0.22 | 0.41 | 0.51 | 0.37 | 0.40 | 0.36 | 0.37 | 0.37 (21.6) | |
| Specimen | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | Average (CoV %) | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A-S | μs | 0.25 | 0.22 | 0.18 | 0.20 | 0.23 | 0.23 | 0.19 | 0.16 | 0.19 | 0.16 | 0.16 | 0.15 | 0.22 | 0.17 | 0.16 | 0.192 (16) |
| μk | 0.20 | 0.14 | 0.14 | 0.15 | 0.18 | 0.18 | 0.18 | 0.18 | 0.14 | 0.16 | 0.14 | - | 0.16 | 0.16 | 0.15 | 0.16 (11.8) | |
| B-S | μs | 0.14 | 0.16 | 0.20 | 0.15 | 0.20 | 0.14 | 0.19 | 0.13 | 0.20 | 0.15 | 0.15 | 0.16 | 0.16 | 0.16 | - | 0.16 (14.0) |
| μk | - | 0.14 | 0.15 | 0.14 | 0.15 | 0.16 | 0.16 | - | 0.18 | - | - | 0.15 | 0.16 | 0.14 | - | 0.15 (8.5) | |
| C-S | μs | 0.21 | 0.18 | 0.18 | 0.15 | 0.19 | 0.16 | 0.21 | 0.17 | 0.23 | 0.26 | 0.26 | 0.22 | 0.23 | 0.17 | 0.20 | 0.20 (17.3) |
| μk | 0.17 | 0.14 | 0.15 | - | 0.15 | 0.15 | 0.16 | 0.16 | 0.21 | 0.19 | 0.20 | 0.19 | 0.18 | 0.14 | 0.17 | 0.17 (13.0) | |
| D-S | μs | 0.24 | 0.24 | 0.18 | 0.25 | 0.17 | 0.21 | 0.17 | 0.20 | 0.22 | 0.20 | 0.22 | 0.23 | 0.21 | 0.21 | 0.17 | 0.21 (12.6) |
| μk | 0.20 | 0.22 | 0.15 | 0.20 | 0.16 | 0.17 | 0.16 | 0.19 | - | - | 0.20 | 0.19 | 0.18 | 0.17 | 0.16 | 0.18 (12.3) | |
| E-S | μs | 0.23 | 0.18 | 0.23 | 0.15 | 0.18 | 0.19 | 0.20 | 0.20 | 0.22 | 0.20 | 0.23 | 0.23 | 0.20 | 0.17 | 0.15 | 0.20 (14.0) |
| μk | 0.21 | 0.16 | 0.21 | - | 0.16 | 0.19 | 0.18 | - | 0.18 | 0.18 | 0.20 | 0.20 | 0.17 | 0.16 | 0.15 | 0.18 (12.0) | |
| F-S | μs | 0.19 | 0.21 | 0.17 | 0.19 | 0.13 | 0.16 | 0.20 | 0.13 | 0.25 | 0.19 | 0.21 | 0.20 | 0.19 | 0.20 | - | 0.19 (18.9) |
| μk | 0.18 | 0.19 | 0.15 | 0.16 | - | 0.15 | 0.17 | - | 0.24 | - | 0.18 | 0.18 | 0.17 | 0.18 | - | 0.18 (15.1) | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Villar-García, J.R.; Vidal-López, P.; Rodríguez-Robles, D.; Moya Ignacio, M. Friction Coefficients of Chestnut (Castanea sativa Mill.) Sawn Timber for Numerical Simulation of Timber Joints. Forests 2022, 13, 1078. https://doi.org/10.3390/f13071078
Villar-García JR, Vidal-López P, Rodríguez-Robles D, Moya Ignacio M. Friction Coefficients of Chestnut (Castanea sativa Mill.) Sawn Timber for Numerical Simulation of Timber Joints. Forests. 2022; 13(7):1078. https://doi.org/10.3390/f13071078
Chicago/Turabian StyleVillar-García, José Ramón, Pablo Vidal-López, Desirée Rodríguez-Robles, and Manuel Moya Ignacio. 2022. "Friction Coefficients of Chestnut (Castanea sativa Mill.) Sawn Timber for Numerical Simulation of Timber Joints" Forests 13, no. 7: 1078. https://doi.org/10.3390/f13071078
APA StyleVillar-García, J. R., Vidal-López, P., Rodríguez-Robles, D., & Moya Ignacio, M. (2022). Friction Coefficients of Chestnut (Castanea sativa Mill.) Sawn Timber for Numerical Simulation of Timber Joints. Forests, 13(7), 1078. https://doi.org/10.3390/f13071078






