Impact of Relaxation Time on Electrochemical Impedance Spectroscopy Characterization of the Most Common Lithium Battery Technologies—Experimental Study and Chemistry-Neutral Modeling
Abstract
:1. Introduction
- Both high energy and high-power battery chemistries are considered along with the different aging (pristine and aged) states. To the best of the authors’ knowledge, such studies on the most common technologies have not been performed before. Moreover, investigation of aged cells is also a rare study that has been included in this work. All the batteries that are aged, used similar cycling conditions for more than 2 years. Impedance measurements are done following an in-house developed methodology for another 6 months.
- Variable (low to high) temperatures and SoC ranges are considered for the study of the impedance change, covering a wide range of experimental conditions.
- From no relaxation to ten different rest periods (up to 5 h) are employed.
- The relaxation impact on the ohmic impedance is modeled and a standard technology-neutral rest time is proposed that is a unique approach to avoid individual technology-specific study.
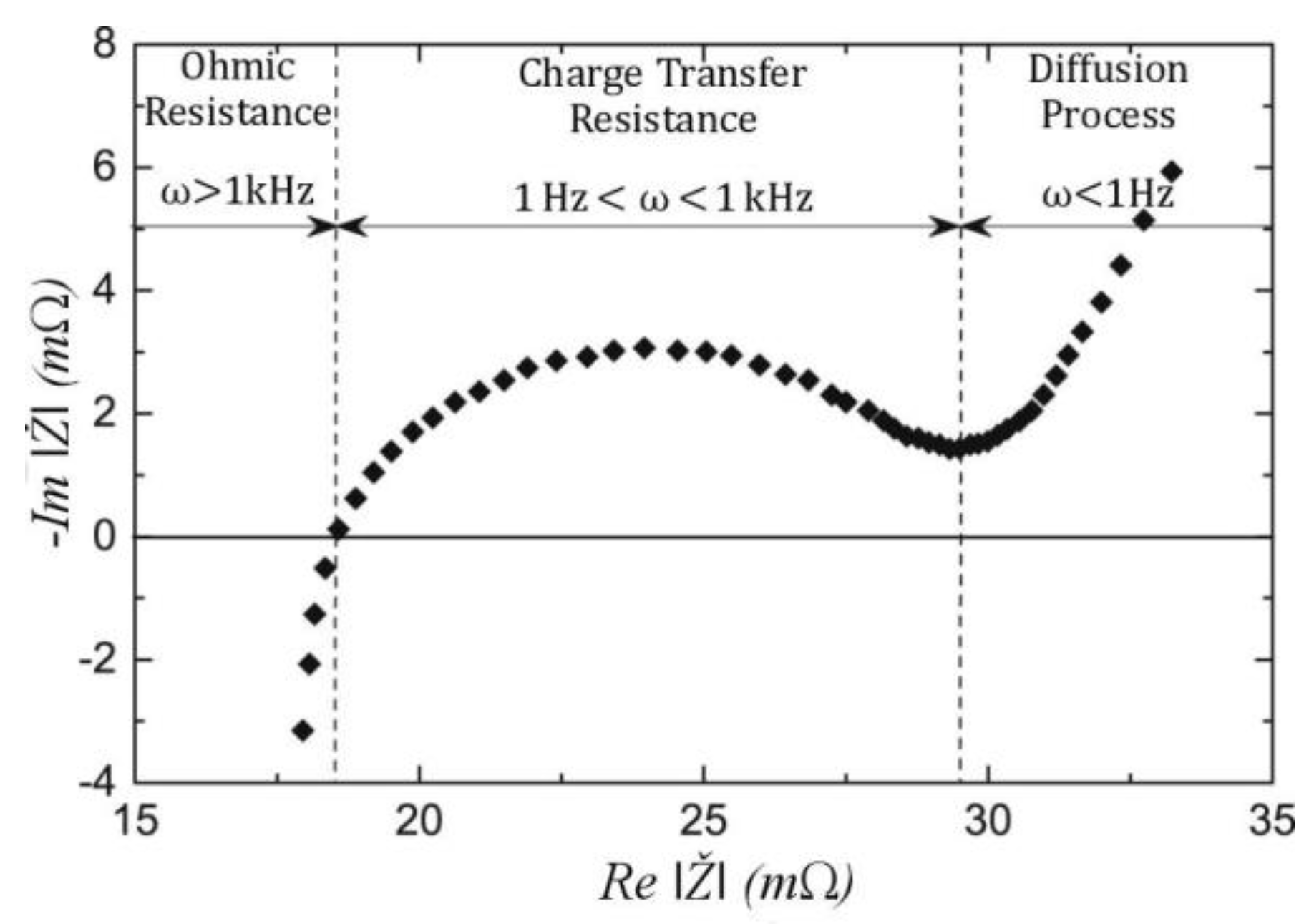
2. Electrochemical Impedance Spectroscopy
2.1. Battery Impedance (Theory)
2.2. EIS Experimental Method
2.3. EIS Modeling
3. Experimental Study
4. Results and Discussion
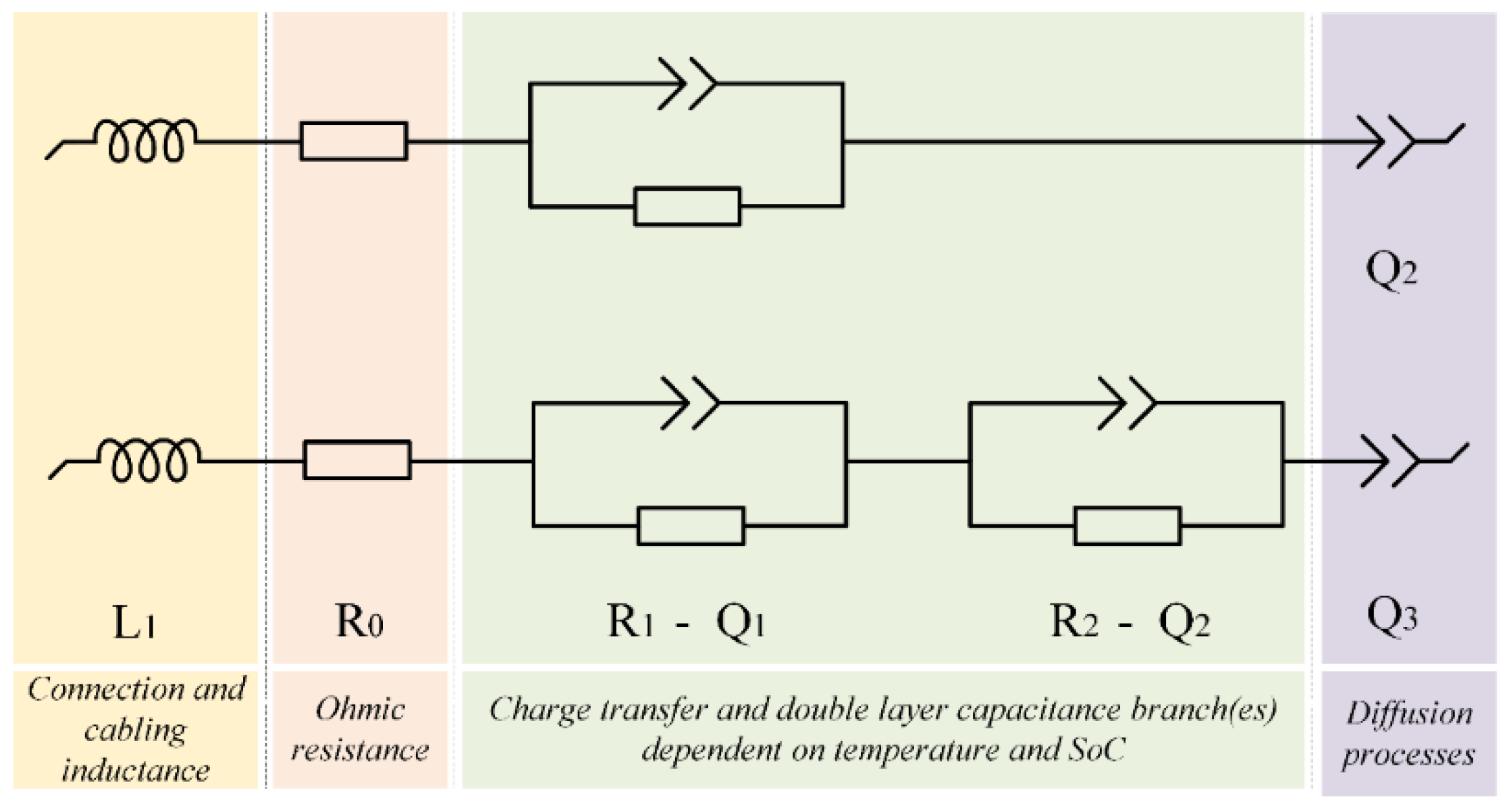
4.1. Equivalent Circuit Selection and Test Conditions
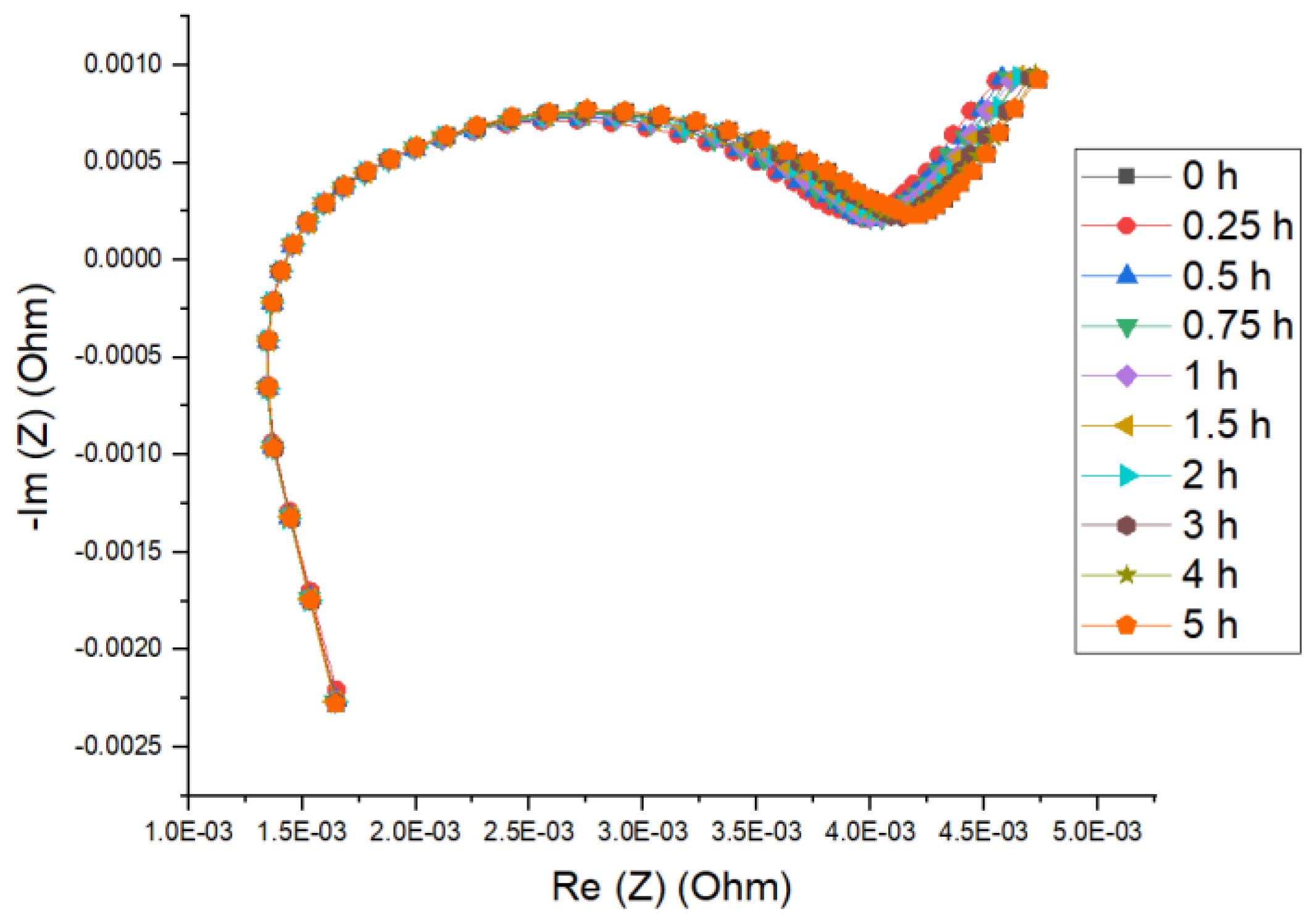
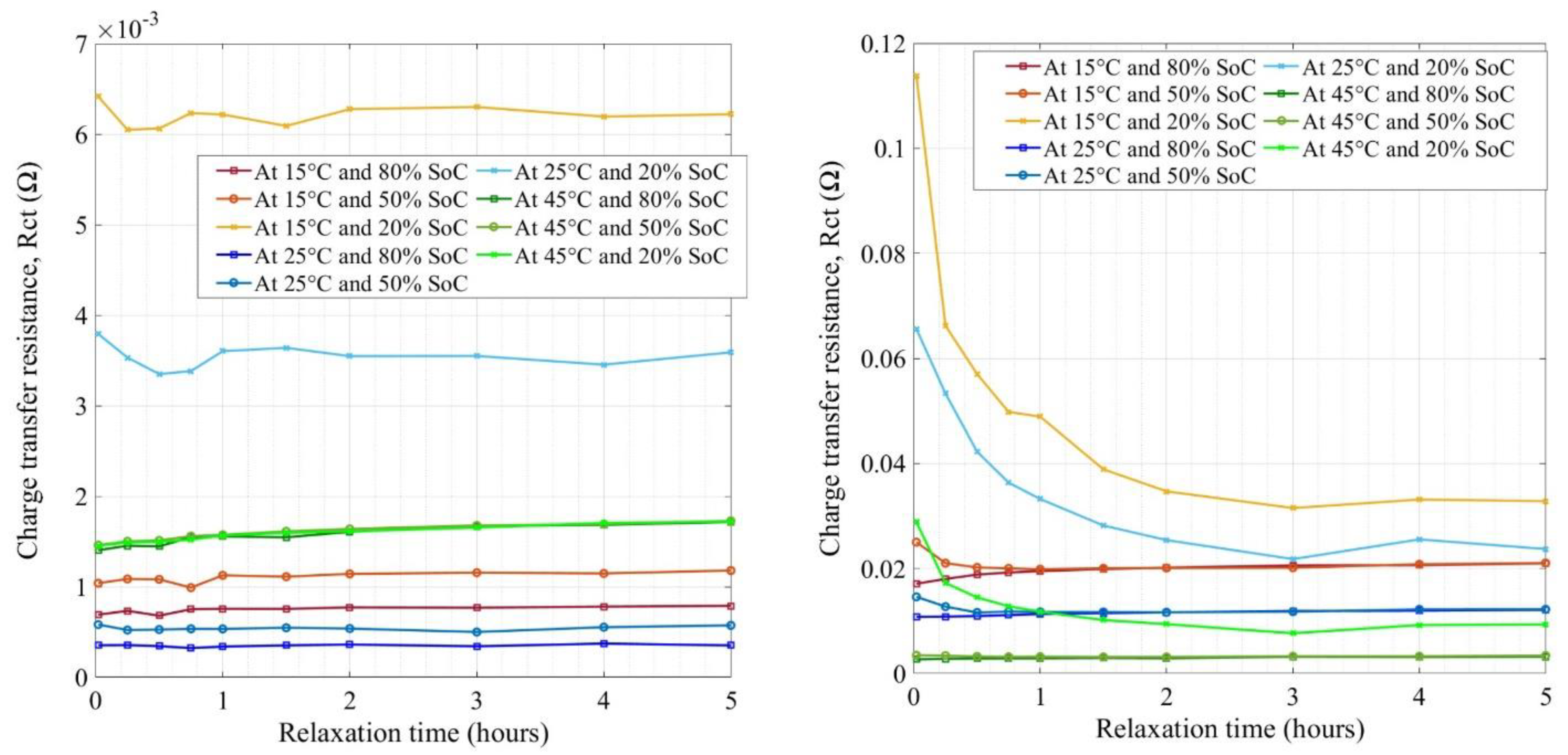
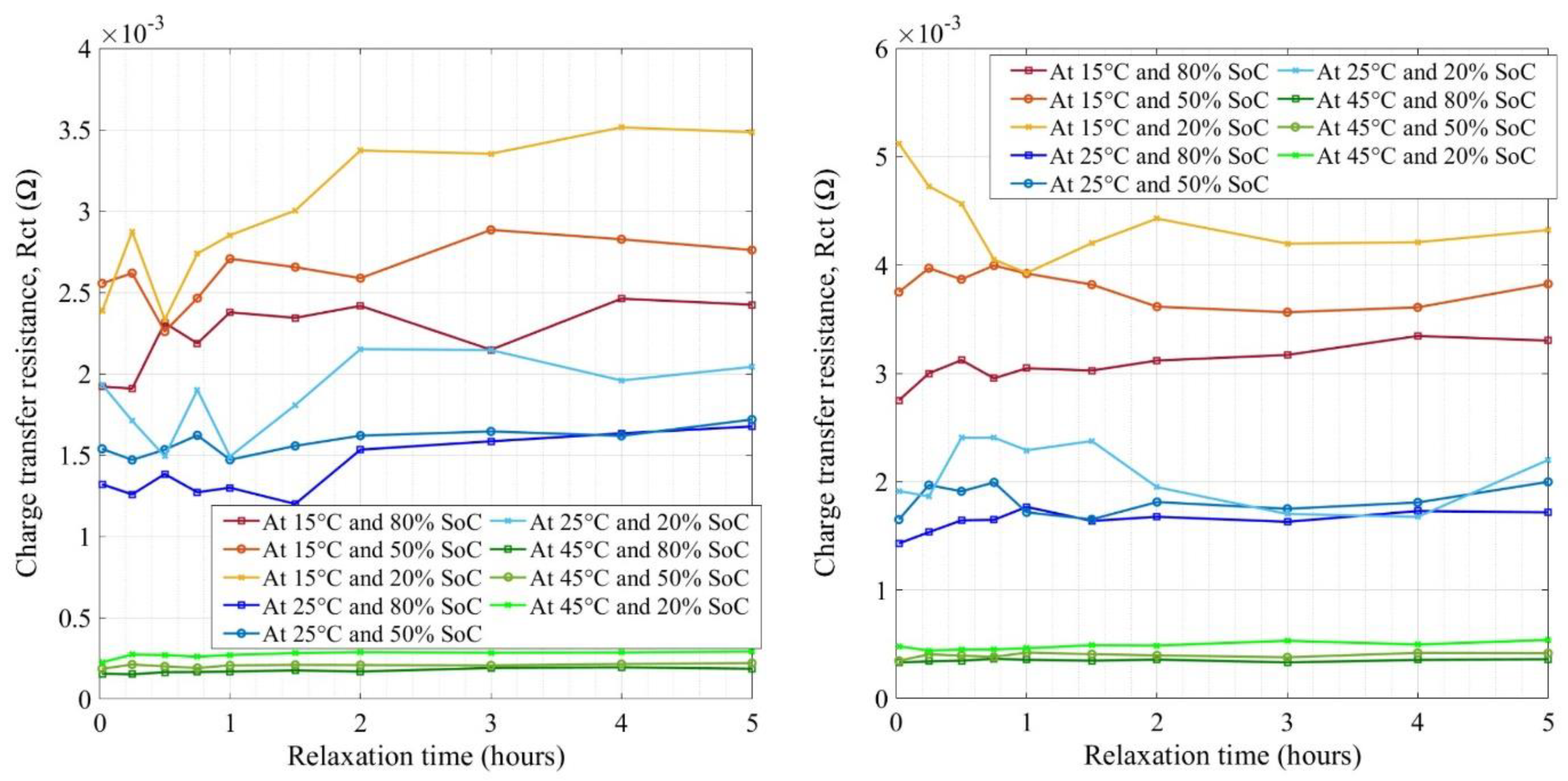
4.2. Impedance Measurement of NMC Cells
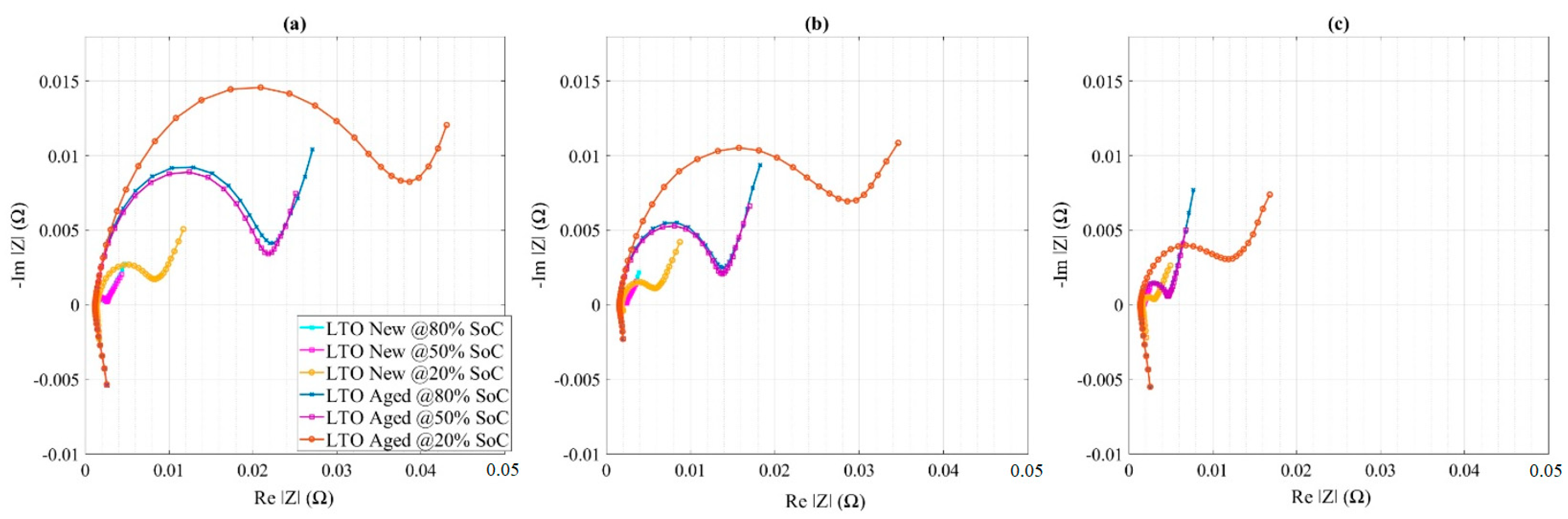
4.3. Impedance Measurement of LTO Cells
4.4. Impedance Measurement of LFP Cells
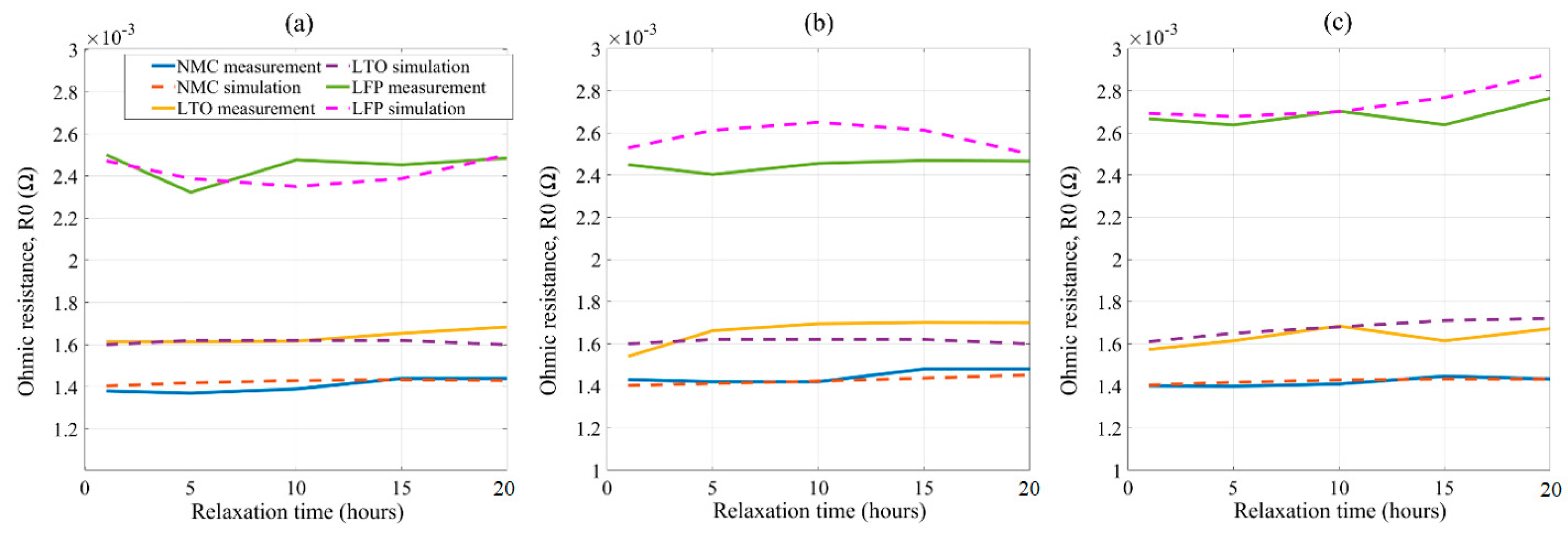
5. Model Development and Validation
5.1. Fitting Polynomial Model
5.2. Validation
5.3. Limitations and Potential Study
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmed, R.; El Sayed, M.; Arasaratnam, I.; Tjong, J.; Habibi, S. Reduced-Order Electrochemical Model Parameters Identification and State of Charge Estimation for Healthy and Aged Li-Ion Batteries—Part II: Aged Battery Model and State of Charge Estimation. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 678–690. [Google Scholar] [CrossRef]
- Andre, D.; Meiler, M.; Steiner, K.; Walz, H.; Soczka-Guth, T.; Sauer, D. Characterization of high-power lithium-ion batteries by electrochemical impedance spectroscopy. II: Modelling. J. Power Sources 2011, 196, 5349–5356. [Google Scholar] [CrossRef]
- Barai, A.; Chouchelamane, G.H.; Guo, Y.; McGordon, A.; Jennings, P. A study on the impact of lithium-ion cell relaxation on electrochemical impedance spectroscopy. J. Power Sources 2015, 280, 74–80. [Google Scholar] [CrossRef]
- Evgenij, B.; Macdonald, J.R. Impedance Spectroscopy: Theory, Experiment, and Applications. Impedance Spectroscopy: Theory, Experiment, and Applications; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar] [CrossRef]
- BATTLE—IWT SB Project. 2014. Available online: http://etec.vub.ac.be/battle/index.php (accessed on 20 April 2021).
- Berecibar, M.; Gandiaga, I.; Villarreal, I.; Omar, N.; Van Mierlo, J.; van den Bossche, P. Critical review of state of health estimation methods of Li-ion batteries for real applications. Renew. Sustain. Energy Rev. 2016, 56, 572–587. [Google Scholar] [CrossRef]
- Braun, P.V.; Cho, J.; Pikul, J.H.; King, W.P.; Zhang, H. High power rechargeable batteries. Curr. Opin. Solid State Mater. Sci. 2012, 16, 186–198. [Google Scholar] [CrossRef]
- Cho, H.-M.; Choi, W.-S.; Go, J.-Y.; Bae, S.-E.; Shin, H.-C. A study on time-dependent low temperature power performance of a lithium-ion battery. J. Power Sources 2012, 198, 273–280. [Google Scholar] [CrossRef]
- Costard, J.; Ender, M.; Weiss, M.; Ivers-Tiffée, E. Three-Electrode Setups for Lithium-Ion Batteries. J. Electrochem. Soc. 2017, 164, A80–A87. [Google Scholar] [CrossRef]
- Robert, D.; Fichtner, M.; Otuszewski, T.; Punckt, C.; Tarascon, J.; Vegge, T.; Dominko, R.; Edström, K.; Fichtner, M.; Punckt, C. BATTERY 2030+ Roadmap 1. 2020. Available online: https://battery2030.eu/ (accessed on 20 April 2021).
- Samadani, E.; Farhad, S.; Scott, W.; Mastali, M.; Gimenez, L.E.; Fowler, M.; Fraser, R.A. Empirical Modeling of Lithium-ion Batteries Based on Electrochemical Impedance Spectroscopy Tests. Electrochim. Acta 2015, 160, 169–177. [Google Scholar] [CrossRef]
- Ecker, M.; Nieto, N.; Käbitz, S.; Schmalstieg, J.; Blanke, H.; Warnecke, A.; Sauer, D.U. Calendar and cycle life study of Li(NiMnCo)O2-based 18650 lithium-ion batteries. J. Power Sources 2014, 248, 839–851. [Google Scholar] [CrossRef]
- Eddahech, A.; Briat, O.; Bertrand, N.; Delétage, J.-Y.; Vinassa, J.-M. Behavior and state-of-health monitoring of Li-ion batteries using impedance spectroscopy and recurrent neural networks. Int. J. Electr. Power Energy Syst. 2012, 42, 487–494. [Google Scholar] [CrossRef]
- Kindermann, F.M.; Noel, A.; Erhard, S.V.; Jossen, A. Long-term equalization effects in Li-ion batteries due to local state of charge inhomogeneities and their impact on impedance measurements. Electrochim. Acta 2015, 185, 107–116. [Google Scholar] [CrossRef]
- Farmann, A.; Waag, W.; Marongiu, A.; Sauer, D.U. Critical review of on-board capacity estimation techniques for lithium-ion batteries in electric and hybrid electric vehicles. J. Power Sources 2015, 281, 114–130. [Google Scholar] [CrossRef]
- Farmann, A.; Waag, W.; Sauer, D.U. Application-specific electrical characterization of high power batteries with lithium titanate anodes for electric vehicles. Energy 2016, 112, 294–306. [Google Scholar] [CrossRef]
- Fleischer, C.; Waag, W.; Heyn, H.-M.; Sauer, D.U. On-line adaptive battery impedance parameter and state estimation considering physical principles in reduced order equivalent circuit battery models. J. Power Sources 2014, 260, 276–291. [Google Scholar] [CrossRef]
- Fu, R.; Choe, S.-Y.; Agubra, V.; Fergus, J. Development of a physics-based degradation model for lithium ion polymer batteries considering side reactions. J. Power Sources 2015, 278, 506–521. [Google Scholar] [CrossRef]
- Fuller, T.F.; Doyle, M.; Newman, J. Relaxation Phenomena in Lithium-Ion-Insertion Cells. J. Electrochem. Soc. 1994, 141, 982–990. [Google Scholar] [CrossRef] [Green Version]
- Gopalakrishnan, R.; Li, Y.; Smekens, J.; Barhoum, A.; Van Assche, G.; Omar, N.; Van Mierlo, J. Electrochemical impedance spectroscopy characterization and parameterization of lithium nickel manganese cobalt oxide pouch cells: Dependency analysis of temperature and state of charge. Ionics 2018, 25, 111–123. [Google Scholar] [CrossRef]
- Gordon, I.J.; Grugeon, S.; Takenouti, H.; Tribollet, B.; Armand, M.; Davoisne, C.; Débart, A.; Laruelle, S. Electrochemical Impedance Spectroscopy response study of a commercial graphite-based negative electrode for Li-ion batteries as function of the cell state of charge and ageing. Electrochim. Acta 2017, 223, 63–73. [Google Scholar] [CrossRef]
- He, H.; Xiong, R.; Fan, J. Evaluation of Lithium-Ion Battery Equivalent Circuit Models for State of Charge Estimation by an Experimental Approach. Energies 2011, 4, 582–598. [Google Scholar] [CrossRef]
- Hosen, S.; Karimi, D.; Kalogiannis, T.; Pirooz, A.; Jaguemont, J.; Berecibar, M.; Van Mierlo, J. Electro-aging model development of nickel-manganese-cobalt lithium-ion technology validated with light and heavy-duty real-life profiles. J. Energy Storage 2020, 28, 101265. [Google Scholar] [CrossRef]
- Ivers-Tiffée, E.; Weber, A. Evaluation of electrochemical impedance spectra by the distribution of relaxation times. J. Ceram. Soc. Jpn. 2017, 125, 193–201. [Google Scholar] [CrossRef] [Green Version]
- Leng, F.; Tan, C.M.; Pecht, M. Effect of Temperature on the Aging rate of Li Ion Battery Operating above Room Temperature. Sci. Rep. 2015, 5, 12967. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ran, L.; Junfeng, W.; Haiying, W.; Gechen, L. Prediction of State of Charge of Lithium-Ion Rechargeable Battery with Electrochemical Impedance Spectroscopy Theory. In Proceedings of the 2010 5th IEEE Conference on Industrial Electronics and Applications, ICIEA, Taichung, Taiwan, 15–17 June 2010. [Google Scholar] [CrossRef]
- Li, S.E.; Wang, B.; Peng, H.; Hu, X. An electrochemistry-based impedance model for lithium-ion batteries. J. Power Sources 2014, 258, 9–18. [Google Scholar] [CrossRef]
- Li, X.; Ahmadi, M.; Collins, L.; Kalinin, S.V. Deconvolving distribution of relaxation times, resistances and inductance from electrochemical impedance spectroscopy via statistical model selection: Exploiting structural-sparsity regularization and data-driven parameter tuning. Electrochim. Acta 2019, 313, 570–583. [Google Scholar] [CrossRef]
- Lohmann, N.; Weßkamp, P.; Haußmann, P.; Melbert, J.; Musch, T. Electrochemical impedance spectroscopy for lithium-ion cells: Test equipment and procedures for aging and fast characterization in time and frequency domain. J. Power Sources 2015, 273, 613–623. [Google Scholar] [CrossRef]
- Luka, Z. Evaluation of Model and Dispersion Parameters and Their Effects on the Formation of Constant-Phase Ele-ments in Equivalent Circuits. J. Electroanal. Chem. 1999, 464, 68–75. [Google Scholar]
- Ma, S.; Jiang, M.; Tao, P.; Song, C.; Wu, J.; Wang, J.; Deng, T.; Shang, W. Temperature effect and thermal impact in lithium-ion batteries: A review. Prog. Nat. Sci. 2018, 28, 653–666. [Google Scholar] [CrossRef]
- Maheshwari, A.; Heck, M.; Santarelli, M. Cycle aging studies of lithium nickel manganese cobalt oxide-based batteries using electrochemical impedance spectroscopy. Electrochim. Acta 2018, 273, 335–348. [Google Scholar] [CrossRef]
- Orazem, M.E.; Tribollet, B. Electrical Circuits. In Electrochemical Impedance Spectroscopy; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar] [CrossRef]
- Momma, T.; Matsunaga, M.; Mukoyama, D.; Osaka, T. Ac impedance analysis of lithium ion battery under temperature control. J. Power Sources 2012, 216, 304–307. [Google Scholar] [CrossRef]
- Murbach, M.D.; Schwartz, D.T. Analysis of Li-Ion Battery Electrochemical Impedance Spectroscopy Data: An Easy-to-Implement Approach for Physics-Based Parameter Estimation Using an Open-Source Tool. J. Electrochem. Soc. 2018, 165, A297–A304. [Google Scholar] [CrossRef]
- Noh, H.-J.; Youn, S.; Yoon, C.S.; Sun, Y.-K. Comparison of the structural and electrochemical properties of layered Li[NixCoyMnz]O2 (x = 1/3, 0.5, 0.6, 0.7, 0.8 and 0.85) cathode material for lithium-ion batteries. J. Power Sources 2013, 233, 121–130. [Google Scholar] [CrossRef]
- Nyman, A.; Zavalis, T.G.; Elger, R.; Behm, M.; Lindbergh, G. Analysis of the Polarization in a Li-Ion Battery Cell by Numerical Simulations. J. Electrochem. Soc. 2010, 157, A1236–A1252. [Google Scholar] [CrossRef]
- Olofsson, Y.; Groot, J.; Katrašnik, T.; Tavčar, G. Impedance Spectroscopy Characterisation of Automotive NMC/Graphite Li-Ion Cells Aged with Realistic PHEV Load Profile Quantification of Cell Properties vs. Temperature at Different Stages of Ageing; IEEE International Electric Vehicle Conference (IEVC): Florence, Italy, 2014. [Google Scholar] [CrossRef]
- Osaka, T.; Mukoyama, D.; Nara, H. Review—Development of Diagnostic Process for Commercially Available Batteries, Especially Lithium Ion Battery, by Electrochemical Impedance Spectroscopy. J. Electrochem. Soc. 2015, 162, A2529–A2537. [Google Scholar] [CrossRef]
- Ovejas, V.J.; Cuadras, A. Impedance Characterization of an LCO-NMC/Graphite Cell: Ohmic Conduction, SEI Transport and Charge-Transfer Phenomenon. Batteries 2018, 4, 43. [Google Scholar] [CrossRef] [Green Version]
- Palacín, M.R. Understanding ageing in Li-ion batteries: A chemical issue. Chem. Soc. Rev. 2018, 47, 4924–4933. [Google Scholar] [CrossRef] [PubMed]
- Petzl, M.; Danzer, M.A. Nondestructive detection, characterization, and quantification of lithium plating in commercial lithium-ion batteries. J. Power Sources 2014, 254, 80–87. [Google Scholar] [CrossRef]
- Paul, R.; Bonomi, D. Growing The Battery Storage Market 2018: Exploring Four Key Issues. Energy Storage World Forum, no. January. 2018. Available online: http://www.egedec.com/sites/default/files/documents/eswf_whitepaper_-_growing_the_battery_storage_market.pdf (accessed on 15 May 2021).
- Schmidt, J.P.; Chrobak, T.; Ender, M.; Illig, J.; Klotz, D.; Ivers-Tiffée, E. Studies on LiFePO4 as cathode material using impedance spectroscopy. J. Power Sources 2011, 196, 5342–5348. [Google Scholar] [CrossRef]
- Schmitt, J.; Maheshwari, A.; Heck, M.; Lux, S.; Vetter, M. Impedance change and capacity fade of lithium nickel manganese cobalt oxide-based batteries during calendar aging. J. Power Sources 2017, 353, 183–194. [Google Scholar] [CrossRef]
- Schweikert, N.; Heinzmann, R.; Eichhöfer, A.; Hahn, H.; Indris, S. Electrochemical impedance spectroscopy of Li4Ti5O12 and LiCoO2 based half-cells and Li4Ti5O12/LiCoO2 cells: Internal interfaces and influence of state-of-charge and cycle number. Solid State Ionics 2012, 226, 15–23. [Google Scholar] [CrossRef]
- Scrosati, B. History of lithium batteries. J. Solid State Electrochem. 2011, 15, 1623–1630. [Google Scholar] [CrossRef]
- Stroe, D.I.; Swierczynski, M.; Stan, A.I.; Knap, V.; Teodorescu, R.; Andreasen, S.J. Diagnosis of Lithium-Ion Batteries State-of-Health Based on Electrochemical Impedance Spectroscopy Technique; IEEE Energy Conversion Congress and Exposition (ECCE): Pittsburgh, PA, USA, 2014; pp. 4576–4582. [Google Scholar]
- De Sutter, L.; Firouz, Y.; De Hoog, J.; Omar, N.; Van Mierlo, J. Battery aging assessment and parametric study of lithium-ion batteries by means of a fractional differential model. Electrochim. Acta 2019, 305, 24–36. [Google Scholar] [CrossRef]
- US DOE Global Energy Storage Database. 2020. Available online: https://www.sandia.gov/ess-ssl/global-energy-storage-database-home/ (accessed on 20 April 2021).
- Vetter, J.; Novák, P.; Wagner, M.; Veit, C.; Möller, K.-C.; Besenhard, J.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Vyroubal, P.; Kazda, T. Equivalent circuit model parameters extraction for lithium ion batteries using electrochemical impedance spectroscopy. J. Energy Storage 2018, 15, 23–31. [Google Scholar] [CrossRef]
- Waag, W.; Fleischer, C.; Sauer, D.U. Critical review of the methods for monitoring of lithium-ion batteries in electric and hybrid vehicles. J. Power Sources 2014, 258, 321–339. [Google Scholar] [CrossRef]
- Waag, W.; Käbitz, S.; Sauer, D.U. Experimental investigation of the lithium-ion battery impedance characteristic at various conditions and aging states and its influence on the application. Appl. Energy 2013, 102, 885–897. [Google Scholar] [CrossRef]
- Waldmann, T.; Wilka, M.; Kasper, M.; Fleischhammer, M.; Wohlfahrt-Mehrens, M. Temperature dependent ageing mechanisms in Lithium-ion batteries—A Post-Mortem study. J. Power Sources 2014, 262, 129–135. [Google Scholar] [CrossRef]
- Xie, Y.; Li, J.; Yuan, C. Mathematical modeling of the electrochemical impedance spectroscopy in lithium ion battery cycling. Electrochim. Acta 2014, 127, 266–275. [Google Scholar] [CrossRef]
- Zhu, X.; Macía, L.F.; Jaguemont, J.; De Hoog, J.; Nikolian, A.; Omar, N.; Hubin, A. Electrochemical impedance study of commercial LiNi0.80Co0.15Al0.05O2 electrodes as a function of state of charge and aging. Electrochim. Acta 2018, 287, 10–20. [Google Scholar] [CrossRef]
- Zhu, Y.-R.; Xie, Y.; Zhu, R.-S.; Shu, J.; Jiang, L.-J.; Qiao, H.-B.; Yi, T.-F. Kinetic study on LiFePO4-positive electrode material of lithium-ion battery. Ionics 2011, 17, 437–441. [Google Scholar] [CrossRef]
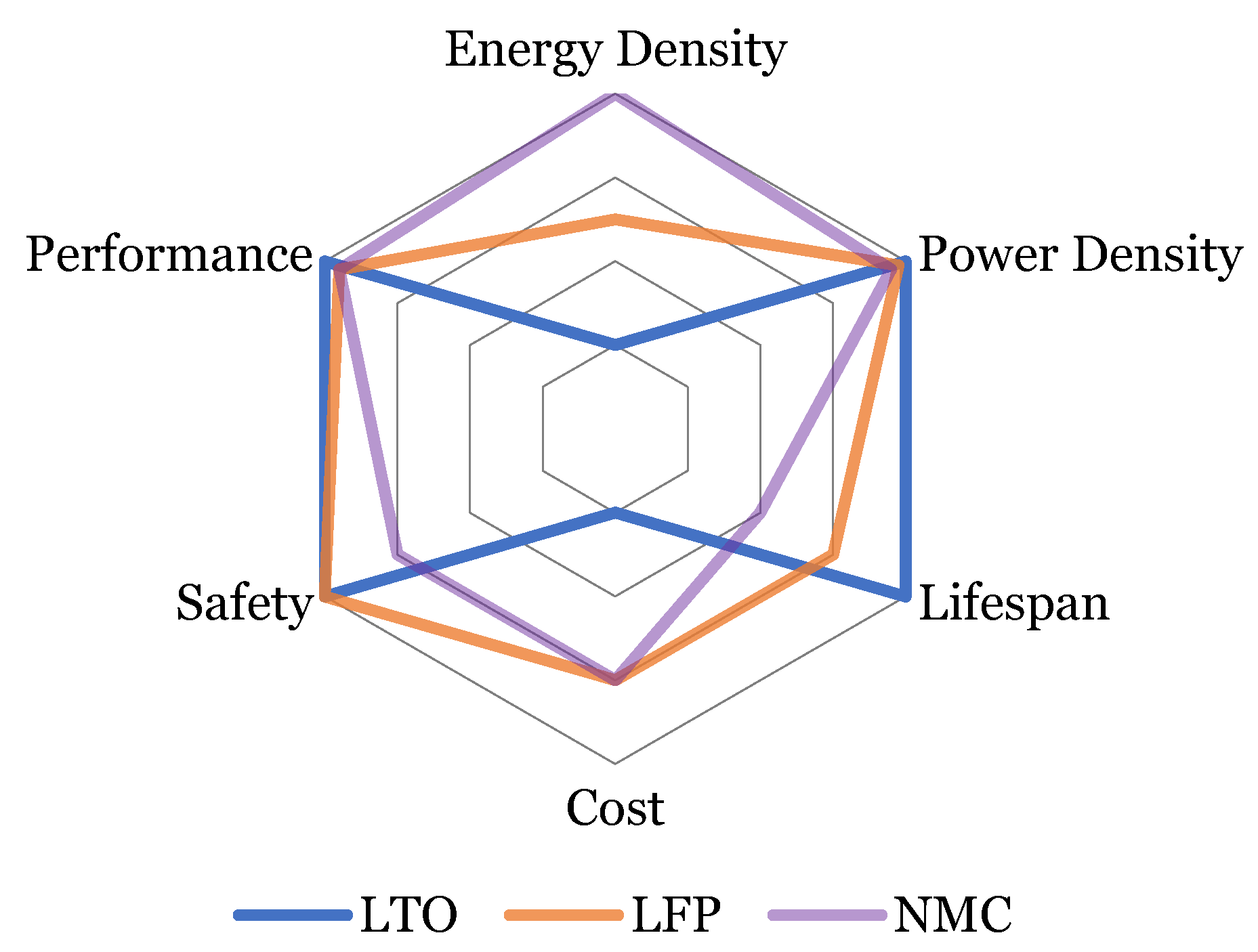

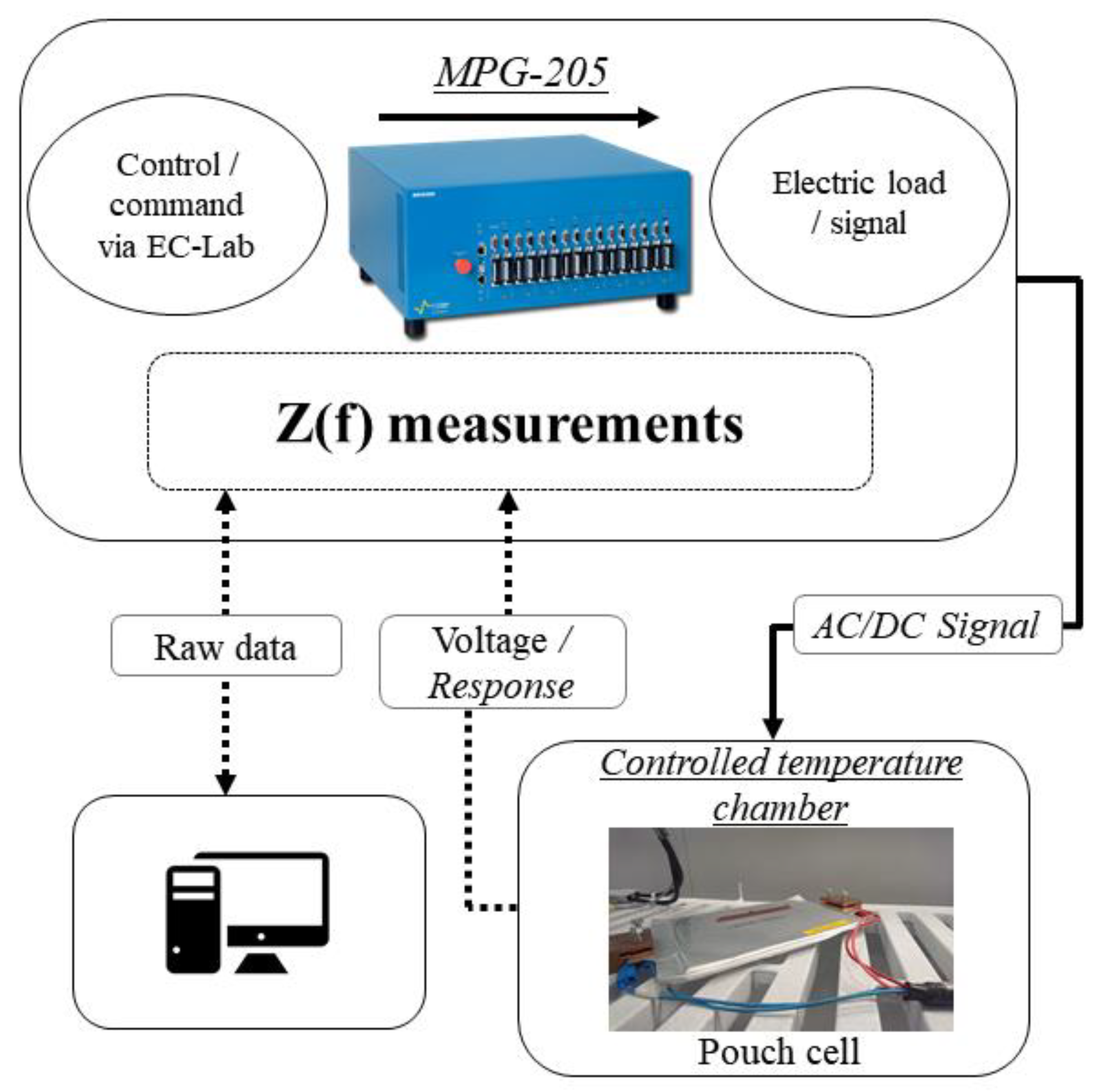






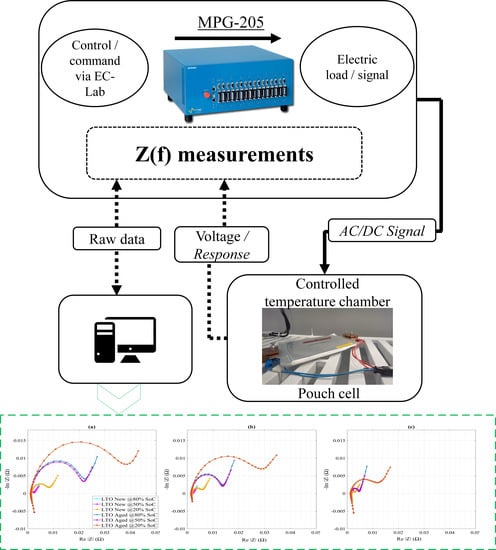
| Cell Technology | Nominal Capacity | Nominal Voltage | AC Impedance |
|---|---|---|---|
| NMC/graphite | 20 Ah | 3.65 V | <3 mΩ |
| NCA/LTO | 5 Ah | 2.2 V | 0.7 mΩ |
| LFP/graphite | 14 Ah | 3.2 V | <5 mΩ |
| Cell No. | Cycling History | SoH | ||||
|---|---|---|---|---|---|---|
| DoD | Temperature | Charge C-Rate | Discharge C-Rate | Performed FECs (#) | ||
| NMC New | New Cell | 100% | ||||
| NMC Aged | 90% | 45 °C | 0.5 C | 1 C | 2400 | 80.76% |
| LTO New | New Cell | 100% | ||||
| LTO Aged | 90% | 45 °C | 0.5 C | 3 C | 1500 | 71.60% |
| LFP New | New Cell | 100% | ||||
| LFP Aged | 90% | 45 °C | 0.5 C | 1 C | 1800 | 78.01% |
| Fitting Equation | NMC | LTO | LFP | ||||||
|---|---|---|---|---|---|---|---|---|---|
| a | b | g | a | b | g | a | b | g | |
| R0 (80% SoC) = a1 × t2 + b1 × t + g1 × 0.8 | −1.5 × 10−7 | 4.5 × 10−6 | 1.8 × 10−3 | 1.0 × 10−8 | 4.0 × 10−6 | 2.0 × 10−3 | 1.5 × 10−6 | −3.0 × 10−5 | 3.1 × 10−3 |
| R0 (50% SoC) = a2 × t2 + b2 × t + g2 × 0.5 | 3.0 × 10−8 | 2.0 × 10−6 | 2.8 × 10−3 | −2.0 × 10−7 | 4.0 × 10−6 | 3.2 × 10−3 | −2.0 × 10−6 | 3.0 × 10−5 | 5.0 × 10−3 |
| R0 (20% SoC) = a3 × t2 + b3 × t + g3 × 0.2 | −1.2 × 10−7 | 4.0 × 10−6 | 7.0 × 10−3 | −2.0 × 10−7 | 1.0 × 10−5 | 8.0 × 10−3 | 9.0 × 10−7 | −9.0 × 10−6 | 1.4 × 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hosen, M.S.; Gopalakrishnan, R.; Kalogiannis, T.; Jaguemont, J.; Van Mierlo, J.; Berecibar, M. Impact of Relaxation Time on Electrochemical Impedance Spectroscopy Characterization of the Most Common Lithium Battery Technologies—Experimental Study and Chemistry-Neutral Modeling. World Electr. Veh. J. 2021, 12, 77. https://doi.org/10.3390/wevj12020077
Hosen MS, Gopalakrishnan R, Kalogiannis T, Jaguemont J, Van Mierlo J, Berecibar M. Impact of Relaxation Time on Electrochemical Impedance Spectroscopy Characterization of the Most Common Lithium Battery Technologies—Experimental Study and Chemistry-Neutral Modeling. World Electric Vehicle Journal. 2021; 12(2):77. https://doi.org/10.3390/wevj12020077
Chicago/Turabian StyleHosen, Md Sazzad, Rahul Gopalakrishnan, Theodoros Kalogiannis, Joris Jaguemont, Joeri Van Mierlo, and Maitane Berecibar. 2021. "Impact of Relaxation Time on Electrochemical Impedance Spectroscopy Characterization of the Most Common Lithium Battery Technologies—Experimental Study and Chemistry-Neutral Modeling" World Electric Vehicle Journal 12, no. 2: 77. https://doi.org/10.3390/wevj12020077
APA StyleHosen, M. S., Gopalakrishnan, R., Kalogiannis, T., Jaguemont, J., Van Mierlo, J., & Berecibar, M. (2021). Impact of Relaxation Time on Electrochemical Impedance Spectroscopy Characterization of the Most Common Lithium Battery Technologies—Experimental Study and Chemistry-Neutral Modeling. World Electric Vehicle Journal, 12(2), 77. https://doi.org/10.3390/wevj12020077