Temperature Field Calculation of the Hybrid Heat Pipe Cooled Permanent Magnet Synchronous Motor for Electric Vehicles Based on Equivalent Thermal Network Method
Abstract
:1. Introduction
2. Hybrid Heat-Pipe Cooling PMSM for EVs
2.1. Motor Model
2.2. The Equivalent Thermal Conductivity of Hybrid Heat Pipe
3. Thermal Network Analysis of Hybrid Heat-Pipe Cooling Motor
3.1. Thermal Network Model Construction
- The motor’s cooling effect and temperature distribution are circumferentially symmetrical.;
- All the heat generated in the motor is regarded as all derived from the coolant, and the coolant temperature is regarded as the external node;
- Due to the heat pipe’s relatively large thermal conductivity, heat pipe’s additional heat path is regarded as heat conduction only through the heat pipe.
- All the losses of the rotor and the permanent magnet are transmitted from the heat pipe to the coolant in the hollow shaft.
3.2. Thermal Resistance Calculation of Hybrid Heat-Pipe Cooling Structure
- The thermal resistance from the end winding to the annular fixed heat pipe is R87 and R1011. Taking R87 as an example:where, dcudb is the radial thickness of the end winding, and λhp is the static thermal conductivity of the heat pipe.
- The thermal resistance of the rotor yoke through the rotating heat pipe to the cooling water inside the hollow shaft includes R1828, R1928 and R2028. The thermal resistance R1828 is analyzed as an example:where, αq is the convective heat transfer coefficient between the heat pipe and the cooling water, and S1828 is the equivalent heat transfer area between the rotating heat pipe and the cooling water.
- The thermal resistance of the annular fixed heat pipe at the end of the winding and the rotating heat pipe at the rotor yoke can be obtained by the temperature difference between the evaporation section and the condensation section of the heat pipe obtained by the CFD simulation above, namely:where, Te is the steady-state temperature of the evaporation section of the heat pipe, Tc is the steady-state temperature of the condensation section of the heat pipe, P is the heat load of the evaporation section of the heat pipe, and R is the equivalent thermal resistance of the heat pipe.
3.3. Thermal Resistance Calculation of Other Parts
3.3.1. The Stator Yoke
- The thermal resistance from the stator yoke to the winding in the slot is R18, R29 and R310. Considering the consistency of thermal resistance, R18 is taken as the analysis object:where, λstj is the radial thermal conductivity of the silicon–steel sheet in the stator yoke, λjy is the thermal conductivity of insulation in the slot, λcu is the thermal conductivity of the winding’’s copper wire, dde is the radial thickness of the stator yoke, dcu is the radial thickness of the winding, S18 is the equivalent heat-transfer area between node 1 and node 8, and the same below.
- Taking the thermal resistance from the stator yoke to shell R133 from node 1 to node 33 as an example:where, λSh is the shell thermal conductivity, rden is the inner diameter of the stator yoke, rdw is the outer diameter of the stator yoke, and rsh is the outer diameter of the motor shell.
- The thermal resistance from the stator yoke to the potting material is R131 and R132, taking R131 as an example:where, R12 is the axial thermal resistance of stator yoke, λgf is the thermal conductivity of the potting adhesive, and S131 is the equivalent heat-transfer area between the stator yoke and potting material.
- The thermal resistances from the stator yoke to stator teeth are R14, R25 and R36. Taking R14 as an example:where, rdn is the inner diameter of the stator.
- The axial thermal resistance of the stator yoke is R12 and R23. Taking R12 as an example:where, λstz is the axial thermal conductivity of the stator silicon–steel sheet, and l is the axial length of the stator.
3.3.2. The Winding
- The thermal resistance from the winding to the stator yoke is, taking R81 between node 8 and node 1 as an example:
- The thermal resistance from the winding to the stator teeth includes R84, R95, and R106, taking R84 as an example:where, ddc is the radial thickness of stator teeth.
- The axial thermal resistance of the winding includes R89 and R910, taking R89 as an example:
3.3.3. The Stator Teeth
- The thermal resistance from the stator teeth to the stator yoke includes R41, R51, and R63. Taking R41 as an example for calculation:
- The thermal resistance from the stator teeth to the winding includes R48, R59, and R610, taking R48 as an example:
- The axial thermal resistance of stator teeth includes R45 and R56. Taking R45 as an example:
- The thermal resistance from stator teeth to air gap includes R426, R526 and R626. Taking R426 as an example:where, αcq is the convective heat transfer coefficient between the stator teeth and the air-gap.
3.3.4. The Rotor Boot
- The thermal resistance from the rotor boot to the air gap includes R1226, R1326 and R1426. Taking R1226 as an example:where, rrw is the outer diameter of the rotor, rre is the outer diameter of the rotor yoke, and αcq is the convective heat-transfer coefficient between the rotor boot and the air gap.
- The thermal resistance from rotor boot to the permanent magnet includes R1215, R1316 and R1417. The thermal resistance R1215 is analyzed as an example:where, λpm is the thermal conductivity of the permanent magnet and dpm is the thickness of the permanent magnet.
- The thermal resistance from the rotor boot to the rotor yoke includes R1218, R1319 and R1420. Taking R1218 as an example for analysis:where, rm is the inner diameter of the rotor.
- The axial thermal resistance inside the rotor boot includes R1213 and R1314. Taking R1213 as an example for analysis:
3.3.5. The Permanent Magnet
- The thermal resistance from permanent magnet to rotor boot includes R1512, R1613 and R1714. Taking R1512 as an example for analysis:
- The thermal resistance from the permanent magnet to the rotor yoke includes R1518, R1619 and R1720. The thermal resistance R1518 is analyzed as an example:
- The axial thermal resistance of permanent magnet includes R1516 and R1617. Taking R1516 as an example for analysis:
3.3.6. The Rotor Yoke
- The thermal resistance from the rotor yoke to the rotor boot includes R1812, R1913 and R2014. The thermal resistance R1812 is analyzed as an example:
- The thermal resistance from the rotor yoke to the permanent magnet includes R1815, R1916 and R2017. The thermal resistance R1815 is analyzed as an example:
- The axial thermal resistance of the rotor yoke includes R1819 and R1920. Taking R1819 as an example for analysis:
3.4. Solution of Equivalent Thermal Network Model
4. The Temperature Field Analysis of Hybrid Heat-Pipe Cooling PMSM for EVs
4.1. Hybrid Heat-Pipe Cooling PMSM for Evs
4.2. Equivalent Thermal Conductivity Experiment of Heat Pipe
4.3. Equivalent Thermal Conductivity Experiment of Heat Pipe
5. Conclusions
- The equivalent thermal resistance of the rotating heat pipe decreases first, then increases, and finally decreases with the increase of the rotor rotation speed when the thermal load is constant. The thermal resistance is low at the rated speed of 4000 rpm, and the maximum equivalent thermal conductivity can reach 76,804.9W/m·k;
- By establishing an equivalent thermal network for the motor, the temperature of each node was analyzed and calculated. When comparing the calculation results with the CFD simulation results, it was found that the temperature error of each node was within 5%, which is within the acceptable range. In view of the error, the analysis in this paper was mainly due to the complex shape of the cooling structure of the rotating heat pipe and the cooling structure of the heat pipe at the end winding, and the existence of potting glue between the end winding and the fixed heat pipe, which affects the accuracy of the thermal resistance calculation, so there is a certain error in the calculation results;
- In this paper, by comparing the temperature field calculation method of hybrid heat-pipe cooling motor based on equivalent thermal network with the traditional CFD simulation time, it was found that the iteration time of the proposed calculation method is within 194 s, which greatly shortens the temperature field calculation time of the hybrid heat-pipe cooling PMSM for EVs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, X.; Li, B.; Gerada, D.; Huang, K.; Stone, I.; Worrall, S.; Yan, Y. A critical review on thermal management technologies for motors in electric cars. Appl. Therm. Eng. 2022, 117758, 1359–4311. [Google Scholar] [CrossRef]
- Gronwald, P.O.; Kern, T.A. Traction Motor Cooling Systems: A Literature Review and Comparative Study. IEEE Trans. Transp. Electrif. 2021, 7, 2892–2913. [Google Scholar] [CrossRef]
- Dan, D.; Yao, C.; Zhang, Y.; Zhang, H.; Zeng, Z.; Xu, X. Dynamic thermal behavior of micro heat pipe array-air cooling battery thermal management system based on thermal network model. Appl. Therm. Eng. 2019, 162, 114183. [Google Scholar] [CrossRef]
- Vese, I.; Marignetti, F.; Radulescu, M.M. Multiphysics approach to numerical modeling of a permanent-magnet tubular linear motor. IEEE Trans. Ind. Electron. 2010, 57, 320–326. [Google Scholar] [CrossRef]
- Boglietti, A.; Cavagnino, A.; Staton, D.; Shanel, M.; Mueller, M.; Mejuto, C. Evolution and modern approaches for thermal analysis of electrical machines. IEEE Trans. Ind. Electron. 2009, 56, 871–882. [Google Scholar] [CrossRef]
- Tessarolo, A.; Bruzzese, C. Computationally efficient thermal analysis of a low-speed high-thrust linear electric actuator with a three-dimensional thermal network approach. IEEE Trans. Ind. Electron. 2015, 62, 1410–1420. [Google Scholar] [CrossRef]
- Nerg, J.; Rilla, M.; Pyrhonen, J. Thermal Analysis of Radial-Flux Electrical Machines with a High Power Density. IEEE Trans. Ind. Electron. 2008, 55, 3543–3554. [Google Scholar] [CrossRef]
- Si, J.; Zhao, S.; Feng, H.; Hu, Y.; Cao, W. Analysis of temperature field for a surface-mounted and interior permanent magnet synchronous motor adopting magnetic-thermal coupling method. CES Trans. Electr. Mach. Syst. 2018, 2, 166–174. [Google Scholar] [CrossRef]
- Li, L.; Zhang, J.; Zhang, C.; Yu, J. Research on Electromagnetic and Thermal Issue of High-Efficiency and High-Power-Density Outer-Rotor Motor. IEEE Trans. Appl. Supercond. 2016, 26, 5204805. [Google Scholar] [CrossRef]
- Zhang, B.; Qu, R.; Xu, W.; Wang, J.; Chen, Y. Thermal model of totally enclosed water-cooled permanent magnet synchronous machines for electric vehicle applications. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 2205–2211. [Google Scholar] [CrossRef]
- Xiao, S.; Griffo, A. Online thermal parameter identification for permanent magnet synchronous machines. Electr. Power Appl. 2020, 14, 2340–2347. [Google Scholar] [CrossRef]
- Huang, J.; Naini, S.S.; Miller, R.; Rizzo, D.; Sebeck, K.; Shurin, S.; Wagner, J. A Hybrid Electric Vehicle Motor Cooling System—Design, Model, and Control. IEEE Trans. Veh. Technol. 2019, 68, 4467–4478. [Google Scholar] [CrossRef]
- Liang, D.; Zhu, Z.Q.; Zhang, Y.; Feng, J.; Guo, S.; Li, Y.; Wu, J.; Zhao, A. A hybrid lumped-parameter and two-dimensional analytical thermal model for electrical machines. IEEE Trans. Ind. Appl. 2020, 57, 246–258. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, X.; Zhao, F.; Gerada, D.; Li, L. Improvements on Permanent Magnet Synchronous Motor by Integrating Heat Pipes into Windings for Solar Unmanned Aerial Vehicle. Green Energy Intell. Transp. 2022, 1, 100011. [Google Scholar] [CrossRef]
- Liu, L.; Tan, G.; Zhou, F.; Sun, M.; Liu, Z.; Yang, C. A Study on Heat Dissipation of Electric Vehicle Motor Based on Heat-Pipe Heat Transfer Analysis. SAE Technical Paper, 6 April 2021. [Google Scholar]
- Sun, D.; Xu, J.; Chen, Q. Modeling of the evaporation and condensation phase-change problems with FLUENT. Numer. Heat Transf. Part B Fundam. 2014, 66, 326–342. [Google Scholar] [CrossRef]
- Sun, Y.; Zhang, S.; Chen, G.; Tang, Y.; Liang, F. Experimental and numerical investigation on a novel heat pipe based cooling strategy for permanent magnet synchronous motors. Appl. Therm. Eng. 2020, 170, 114970. [Google Scholar] [CrossRef]
- Liang, F.; Gao, J.; Li, F.; Xu, L.; Wang, Z.; Jiang, H. A central cooling structure for motorized spindles: Principle and application. In Proceedings of the 2019 18th IEEE Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems (ITherm), Las Vegas, NV, USA, 28–31 May 2019; pp. 1204–1211. [Google Scholar]
- Chang, S.W.; Hsieh, M.F.; Wu, P.S.; Cai, W.L. Convective heat transfer motivated by liquid-to-vapor density difference in centrifugal force field of axially rotating loop thermosyphons. Processes 2021, 9, 1909. [Google Scholar] [CrossRef]
- Wan, Z.; Sun, B.; Wang, X.; Wen, W.; Tang, Y. Improvement on the heat dissipation of permanent magnet synchronous motor using heat pipe. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2020, 234, 1249–1259. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Pang, Y.; Howe, D.; Iwasaki, S.; Deodhar, R.; Pride, A. Analysis of electromagnetic performance of flux-switching permanent-magnet machines by nonlinear adaptive lumped parameter magnetic circuit model. IEEE Trans. Magn. 2005, 41, 4277–4287. [Google Scholar] [CrossRef]
- Mellor, P.H.; Roberts, D.; Turner, D.R. Lumped parameter thermal model for electrical machines of TEFC design. IEE Proc. B (Electr. Power Appl.) 1991, 138, 205–218. [Google Scholar] [CrossRef]
- Demetriades, G.D.; de la Parra, H.Z.; Andersson, E.; Olsson, H. A real-time thermal model of a permanent-magnet synchronous motor. IEEE Trans. Power Electron. 2010, 25, 463–474. [Google Scholar] [CrossRef]
- Park, J.B.; Moosavi, M.; Toliyat, H.A. Electromagnetic-thermal coupled analysis method for interior PMSM. In Proceedings of the 2015 IEEE International Electric Machines & Drives Conference (IEMDC), Coeur d’Alene, ID, USA, 10–13 May 2015; pp. 1209–1214. [Google Scholar]
- Menter, F. Two-equation Eddy-viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Howey, D.A.; Childs PR, N.; Holmes, A.S. Air-gap convection in rotating electrical machines. IEEE Trans. Ind. Electron. 2010, 59, 1367–1375. [Google Scholar] [CrossRef]
- Wang, H.; Liu, X.; Kang, M.; Guo, L.; Li, X. Oil injection cooling design for the IPMSM applied in electric vehicles. IEEE Trans. Transp. Electrif. 2022, 8, 3427–3440. [Google Scholar] [CrossRef]
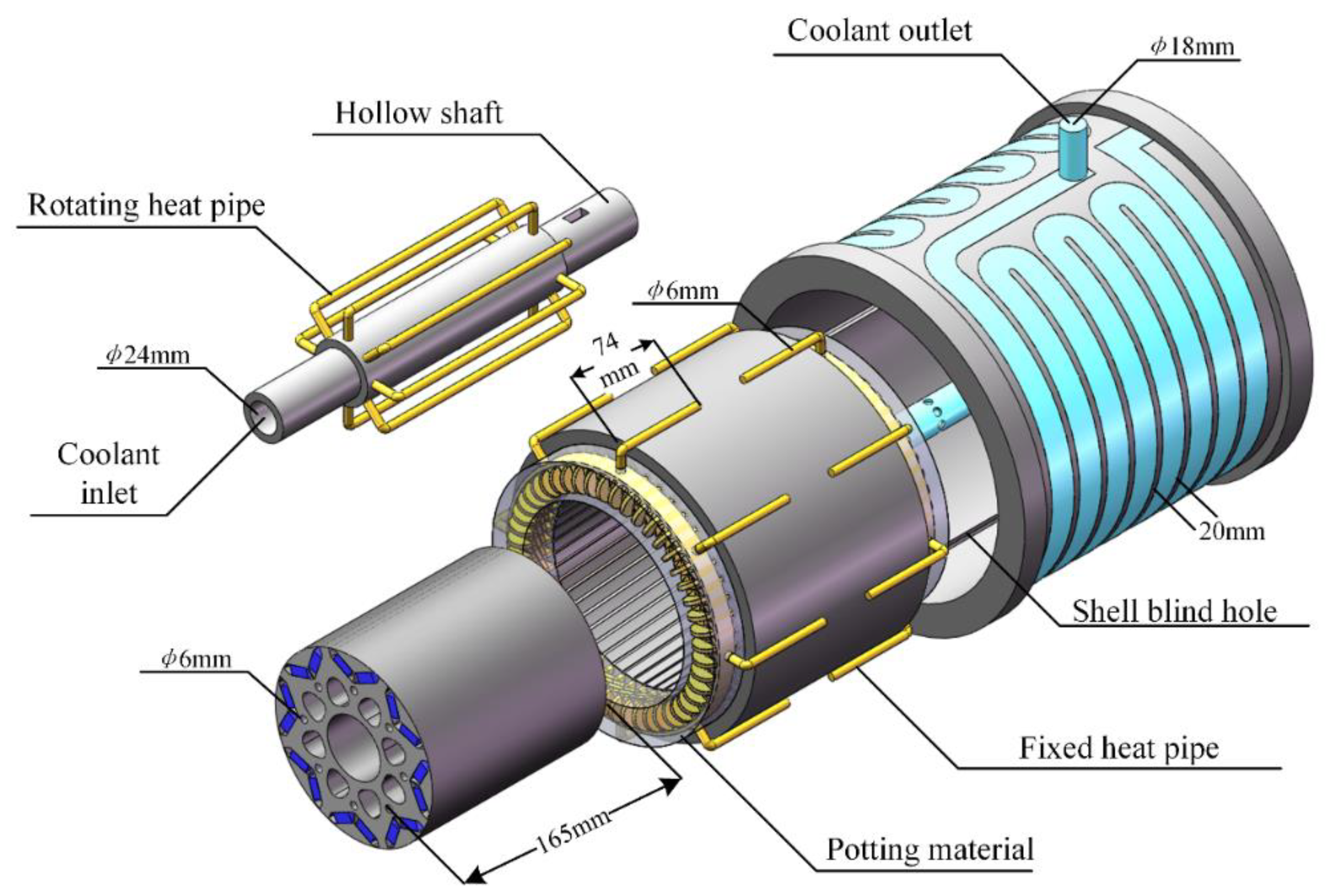




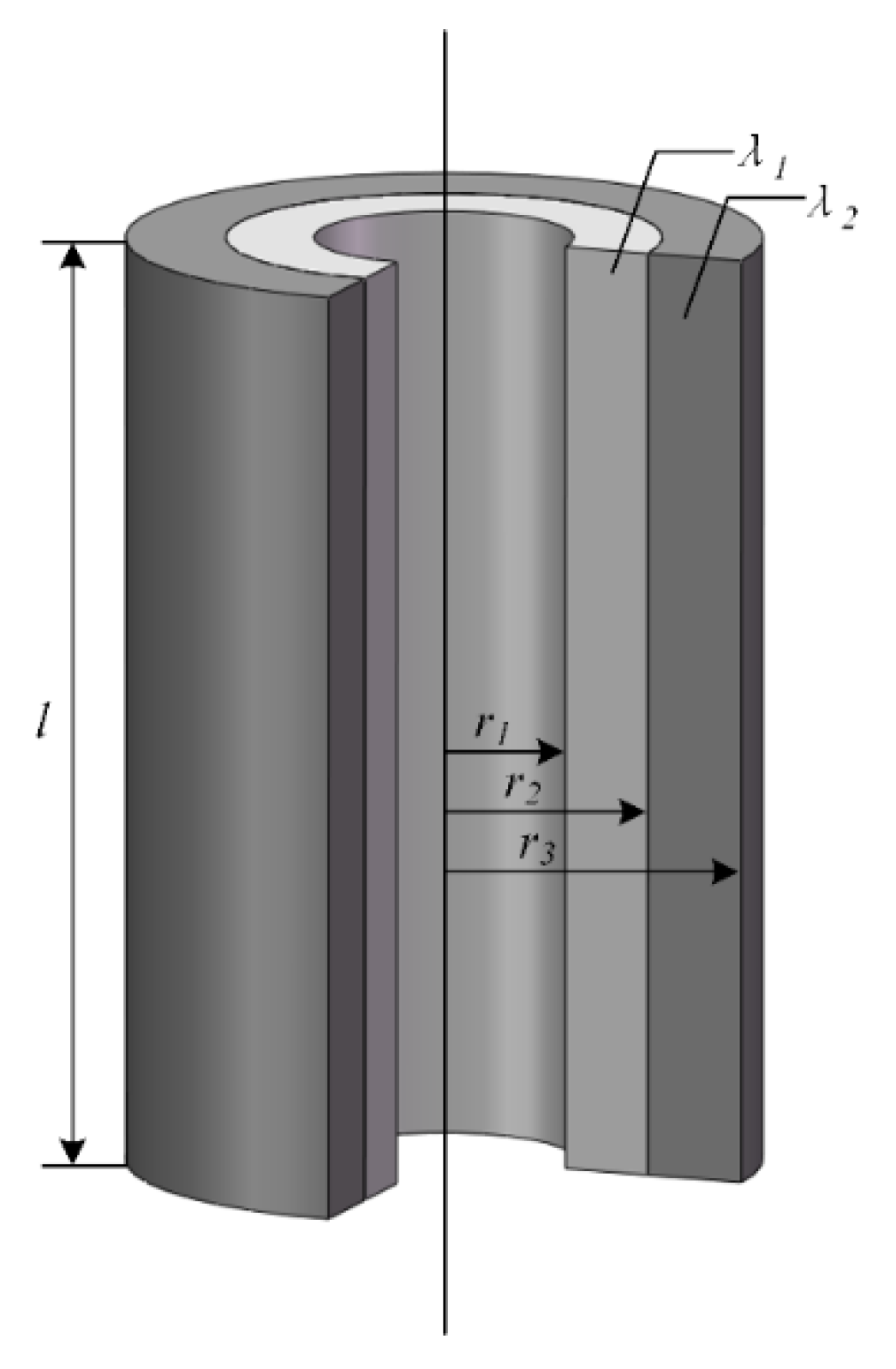

| Parameter | Date | Parameter | Date |
|---|---|---|---|
| Rated power | 90 kW | Pole-Slot number | 8p48s |
| Rated torque | 215 Nm | Pitch | 5 |
| Rated speed | 4000 r/min | Stator yoke diameter | 144 mm |
| Peak Power | 160 kW | Rotor yoke diameter | 48 mm |
| Maximum torque | 380 Nm | Air gap length | 0.5 mm |
| Maximum speed | 12000 r/min | Polar arc coefficient | 0.64 |
| DC Bus Voltage | 320 V | Insulation level | H |
| Motor Speed (rpm) | Thermal Resistance (°C/W) | Thermal Conductivity (W/m·k) |
|---|---|---|
| 0 | 0.018 | 23,560.3 |
| 600 | 0.023 | 18,723.8 |
| 4000 | 0.006 | 74,628.2 |
| Property | Unit | Date |
|---|---|---|
| Thermal conductivity | W/m·k | 3.2–3.4 |
| Breakdown voltage | kV/mm | ≥15 |
| Working temperature | °C | −55–180 |
| Modeling Area | Material | Thermal Conductivity (W/m·k) |
|---|---|---|
| Shell | Iron | 39.2 |
| Stator yoke (radial/axial) | Silicon steel sheet | 38.7/3.7 |
| Rotor (radial/axial) | Silicon steel sheet | 38.7/3.7 |
| Winding | Copper | 385 |
| Slot insulation | Insulating paper | 0.2 |
| Permanent magnet | Nd-Fe-B | 9 |
| Shaft | Steel | 42 |
| Bearing | Steel | 42 |
| Cavity | Air | 0.026 |
| Node | Loss at Rated Condition (W) | Loss at Maximum Torque Condition (W) | Loss at Maximum Speed Condition (W) |
|---|---|---|---|
| 1–3 | 254.26 | 23.1 | 2147.53 |
| 7–11 | 6749.9 | 15,835.8 | 6145.6 |
| 4–6 | 484.57 | 123 | 766.97 |
| 12–14 | 32.4 | 12.5 | 126.75 |
| 18–20 | 52.4 | 11.1 | 200.7 |
| 15–17 | 11.1 | 5.8 | 29.3 |
| 21–22 | 126.1 | 18.9 | 432 |
| Motor Speed (rpm) | Temperature (°C) | Thermal Resistance (°C/W) | Thermal Conductivity (W/m·k) | |
|---|---|---|---|---|
| Tc | Te | |||
| 0 | 109.78 | 113.37 | 0.018 | 25,601.6 |
| 600 | 16.95 | 21.62 | 0.023 | 20,036.1 |
| 1200 | 22.71 | 25.12 | 0.012 | 38,402.5 |
| 1800 | 24.96 | 28.45 | 0.017 | 27,107.6 |
| 2400 | 26.40 | 30.31 | 0.019 | 24,254.2 |
| 3000 | 30.41 | 33.62 | 0.016 | 28,801.8 |
| 3600 | 32.59 | 34.96 | 0.012 | 38,402.5 |
| 4000 | 35.14 | 36.40 | 0.006 | 76,804.9 |
| Speed (rpm) | Equivalent Experiments (W/m·k) | CFD Calculations (W/m·k) |
|---|---|---|
| 0 | 25,601.6 | 23,560.3 |
| 4000 | 76,804.9 | 74,628.2 |
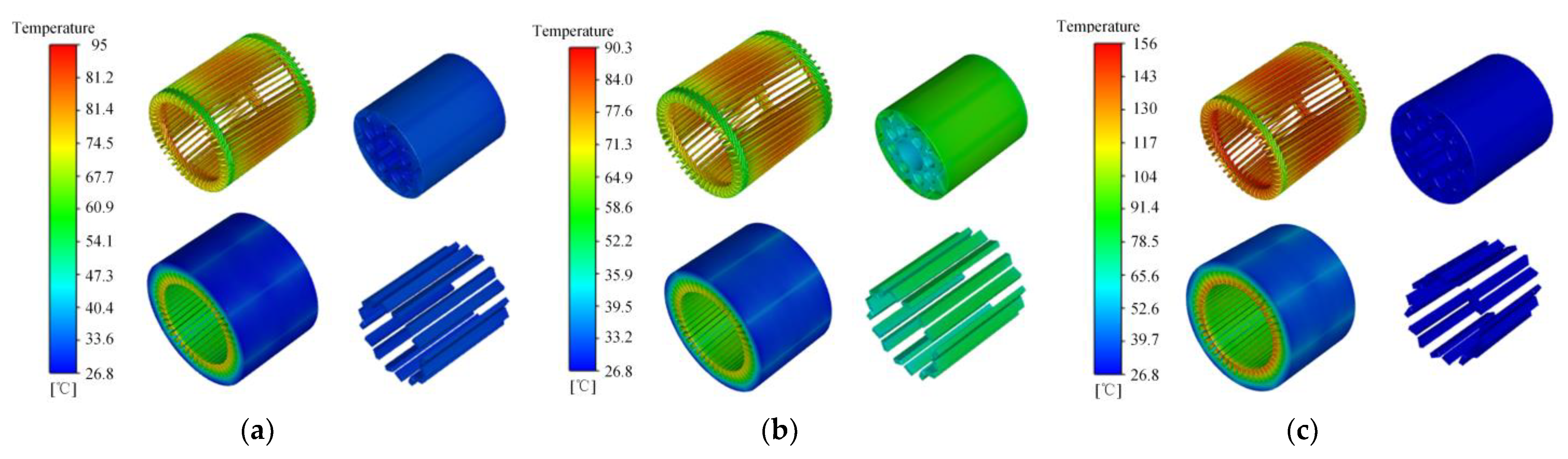
| Operating Conditions | Calculation Method | Winding | Stator | PM | Rotor |
|---|---|---|---|---|---|
| Rated condition | Thermal network | 89.34 °C | 44.32 °C | 35.12 °C | 33.98 °C |
| CFD | 86.48 °C | 45.4 °C | 35.54 °C | 34.33 °C | |
| Error | 3.2% | 2.4% | 1.2% | 1% | |
| Maximum torque condition | Thermal network | 141.82 | 45.62 | 27.12 | 27.09 |
| CFD | 138.77 | 46.55 | 27.8 | 27.62 | |
| Error | 2.2% | 2% | 2.4% | 2% | |
| Maximum speed condition | Thermal network | 87.62 | 49.27 | 37.11 | 33.98 |
| CFD | 85.26 | 49.81 | 37.72 | 34.59 | |
| Error | 2.7% | 1.1% | 1.6% | 1.8% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Zhang, C.; Guo, L.; Chen, W.; Zhang, Z. Temperature Field Calculation of the Hybrid Heat Pipe Cooled Permanent Magnet Synchronous Motor for Electric Vehicles Based on Equivalent Thermal Network Method. World Electr. Veh. J. 2023, 14, 141. https://doi.org/10.3390/wevj14060141
Wang H, Zhang C, Guo L, Chen W, Zhang Z. Temperature Field Calculation of the Hybrid Heat Pipe Cooled Permanent Magnet Synchronous Motor for Electric Vehicles Based on Equivalent Thermal Network Method. World Electric Vehicle Journal. 2023; 14(6):141. https://doi.org/10.3390/wevj14060141
Chicago/Turabian StyleWang, Huimin, Chujie Zhang, Liyan Guo, Wei Chen, and Zhen Zhang. 2023. "Temperature Field Calculation of the Hybrid Heat Pipe Cooled Permanent Magnet Synchronous Motor for Electric Vehicles Based on Equivalent Thermal Network Method" World Electric Vehicle Journal 14, no. 6: 141. https://doi.org/10.3390/wevj14060141
APA StyleWang, H., Zhang, C., Guo, L., Chen, W., & Zhang, Z. (2023). Temperature Field Calculation of the Hybrid Heat Pipe Cooled Permanent Magnet Synchronous Motor for Electric Vehicles Based on Equivalent Thermal Network Method. World Electric Vehicle Journal, 14(6), 141. https://doi.org/10.3390/wevj14060141







