Research on the Multi-Mode Composite Braking Control Strategy of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles
Abstract
1. Introduction
- (a)
- Research on modeling and analysis of braking performance of multi-axle vehicles.
- (b)
- Braking response characteristics of multi-axle vehicles.
- (c)
- Anti-lock control of brakes on multi-axle vehicles.
2. Analysis and Modeling of Brake Dynamics for Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles
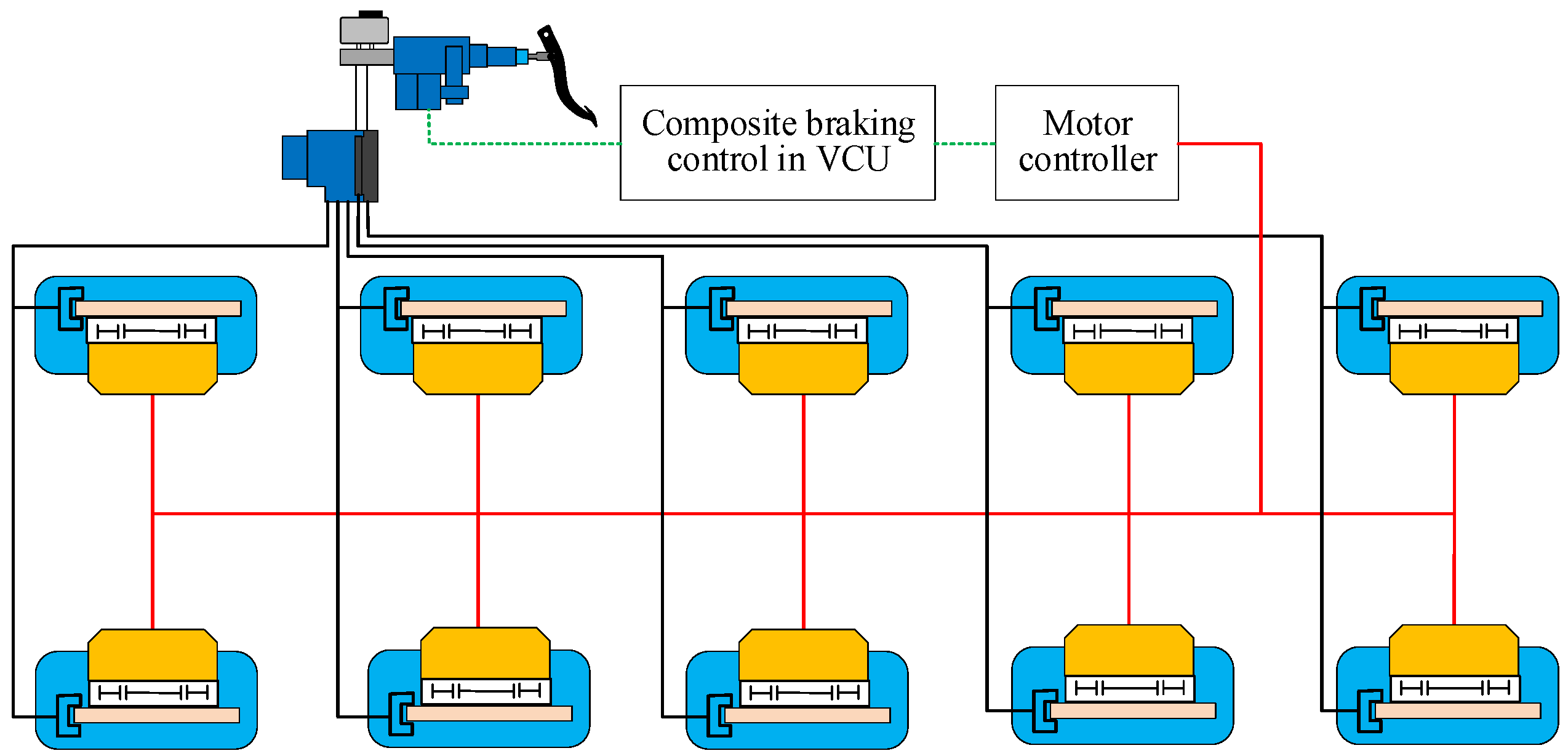
2.1. Structure and Characterization of Composite Braking System for Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles
2.2. Braking Force Analysis of Multi-Axle Vehicles
2.3. Motor Braking Characteristics
2.4. Battery Characteristics
3. Multi-Mode Composite Braking Control Strategy Based on Braking Dynamics of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles
3.1. Composite Braking Control Strategy Architecture and Process
- (1)
- Calculate the total required braking torque Tbreq according to the vehicle velocity and brake pedal opening. Then, judge electric braking based on battery SOC. That is, when SOC ≥ 90%, the hub motor regenerative braking can not be activated because the battery’s power is too high, and when SOC < 90%, the electric braking can be activated, and enters the next step of judgment.
- (2)
- According to the current velocity, the maximum regenerative braking torque of individual motors on each axle is calculated. Then, the total maximum braking torque of the motors is compared with the total required braking torque. When , regenerative braking of the hub motors can satisfy the total required braking torque, and the vehicle enters into the electric braking state; correspondingly, when , regenerative braking of the hub motors can not satisfy the total required braking torque that the deficient part needs to be supplemented by hydraulic braking, and the vehicle enters into the hybrid electro-hydraulic braking state.
- (3)
- Under the fully electric braking state, according to the instantaneous braking energy recovery power of motor braking, the regenerative braking force distribution ratio of each axle motor is optimized with the goal of optimal braking energy recovery, and this optimized ratio is used as the braking force distribution ratio of each axle to improve the braking energy recovery.
- (4)
- Under the hybrid electro-hydraulic braking state, the motor brake and hydraulic brake work together. In order to make full use of the ground adhesion, the braking torque distribution strategy based on the proportion of axle loads is adopted. The braking torque of each axle is preferentially distributed to the motor braking. The insufficient portion is supplemented by the hydraulic braking to achieve the purpose of improving the recovery rate of braking energy.
- (5)
- When the velocity decreases to the low-speed threshold of motor braking, in order to avoid the motor braking from regenerative braking to energy-consumption braking, the torque correction factor is introduced with reference to Formulas (8) and (9), and the motor braking is gradually withdrawn after reaching the velocity threshold, thereby improving the braking energy recovery efficiency at low velocities and enhancing the braking stability.
3.2. Brake Force Distribution Strategy for Fully Electric Braking State Based on Optimal Instantaneous Brake Energy Recovery
3.2.1. Construction of Regenerative Braking Optimization Function Based on Instantaneous Braking Energy Recovery
3.2.2. Optimization Constraints
- (a)
- Motor torque constraints
- (b)
- ECE regulations
3.2.3. Optimization Models
3.3. Braking Torque Distribution Strategy for Hybrid Electro-Hydraulic Braking State Based on Axle–Load Ratio
4. Hardware-in-the-Loop Simulation and Analysis of Test Results
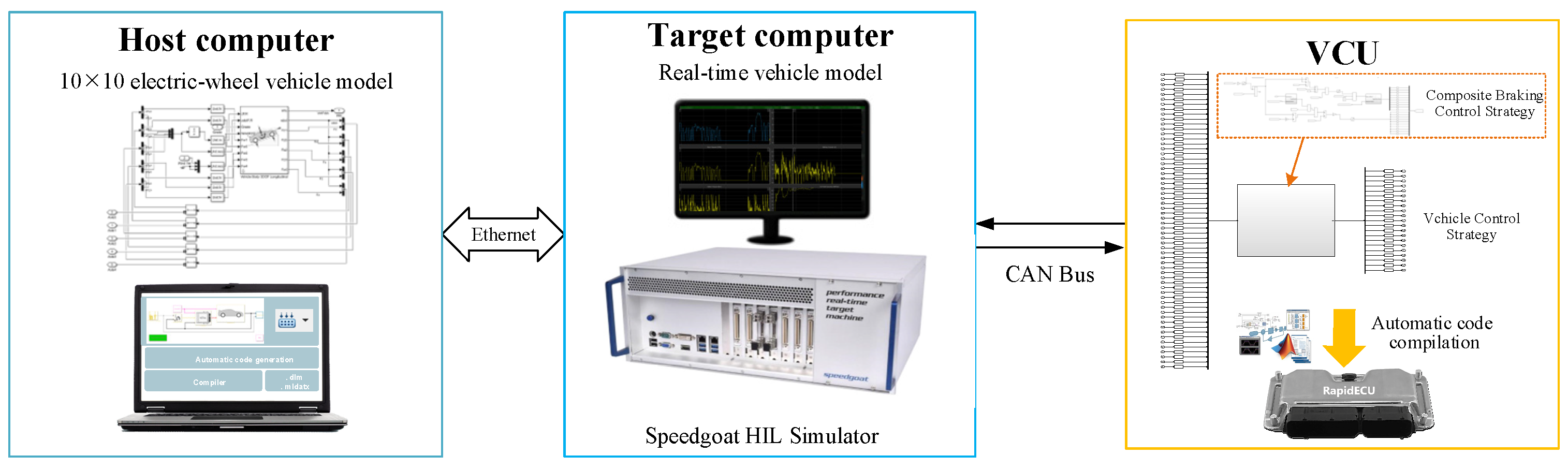
4.1. Hardware-in-the-Loop Simulation Platform
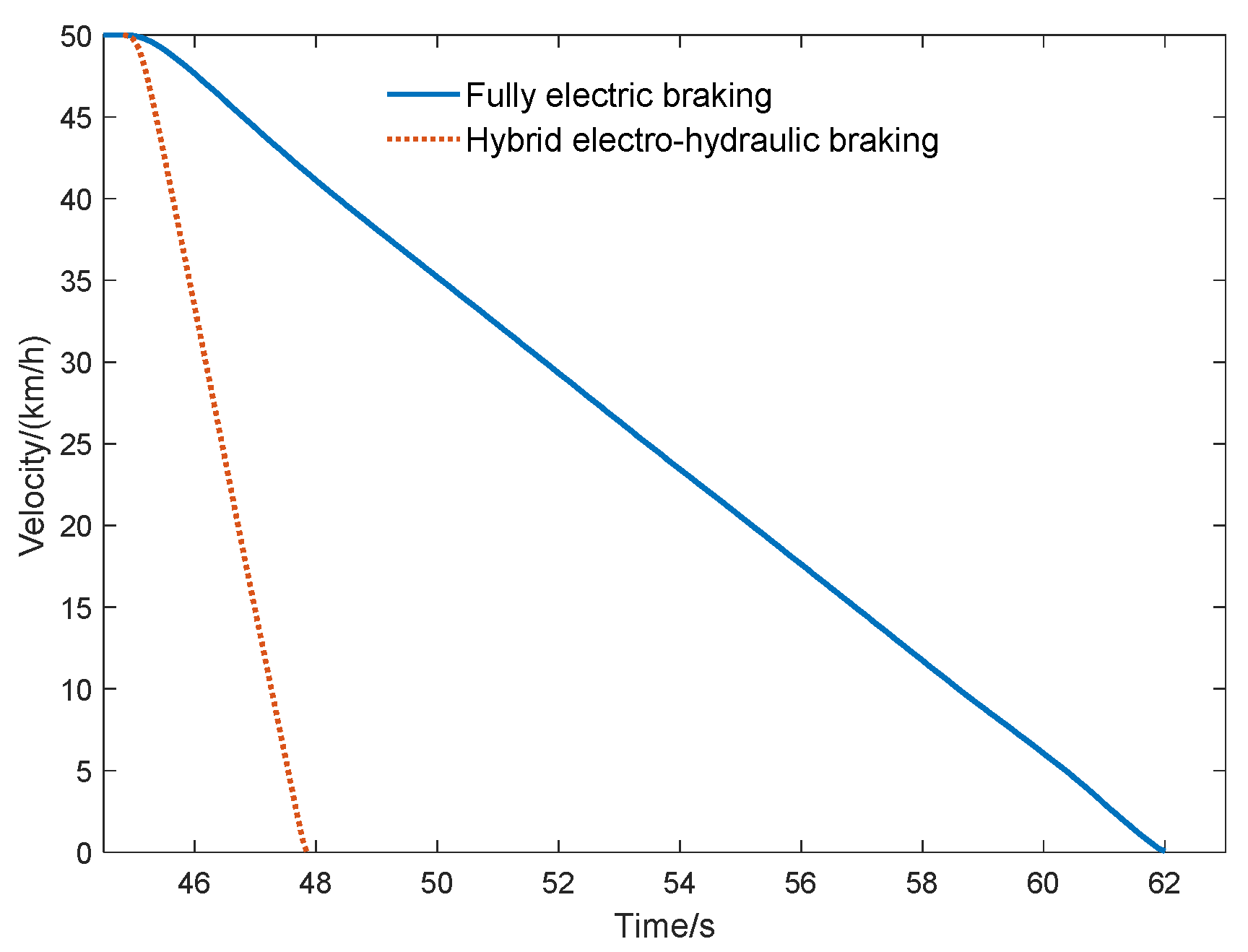
4.2. Simulation Results Analysis of the Single-Stop Braking Condition
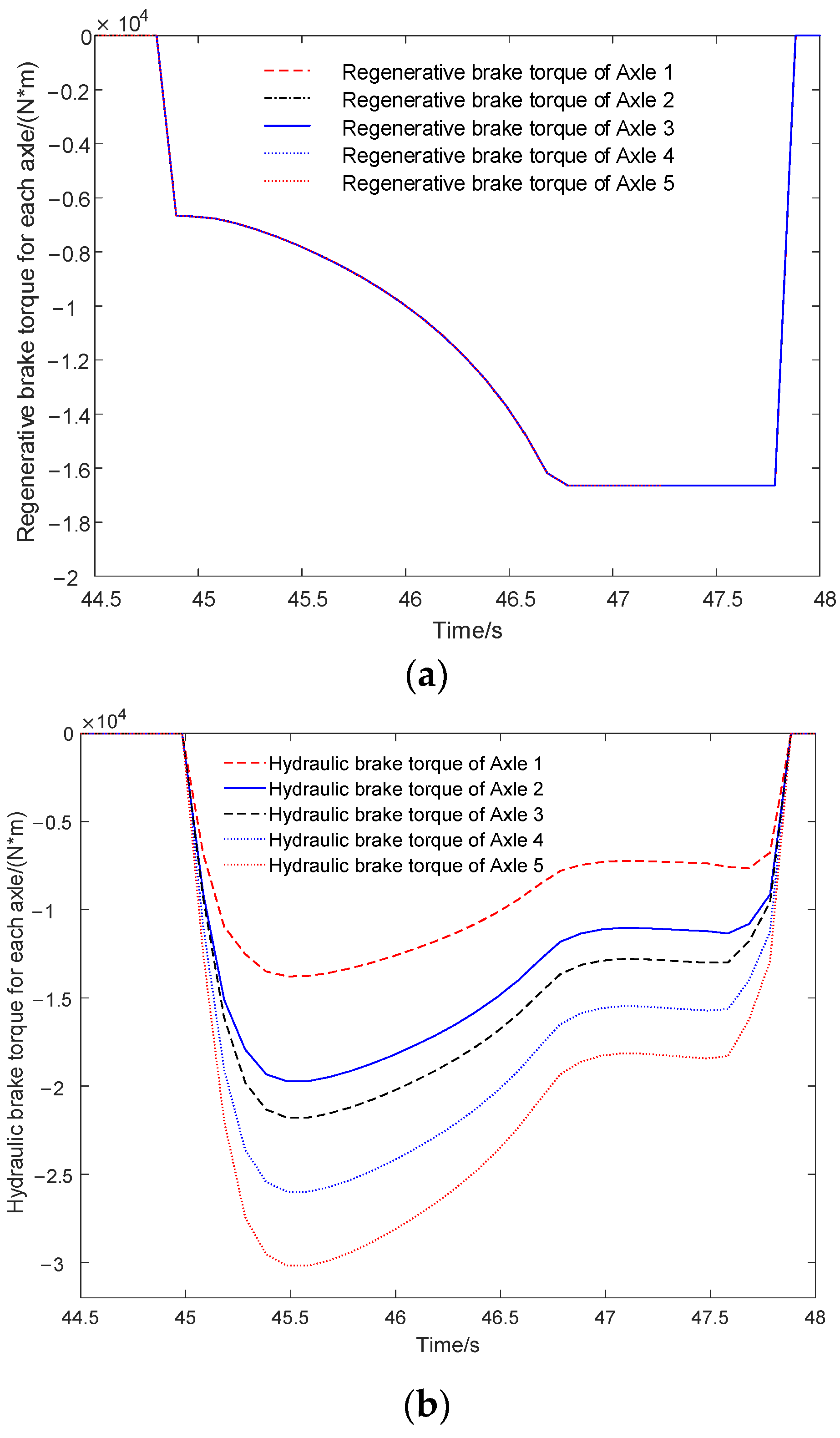
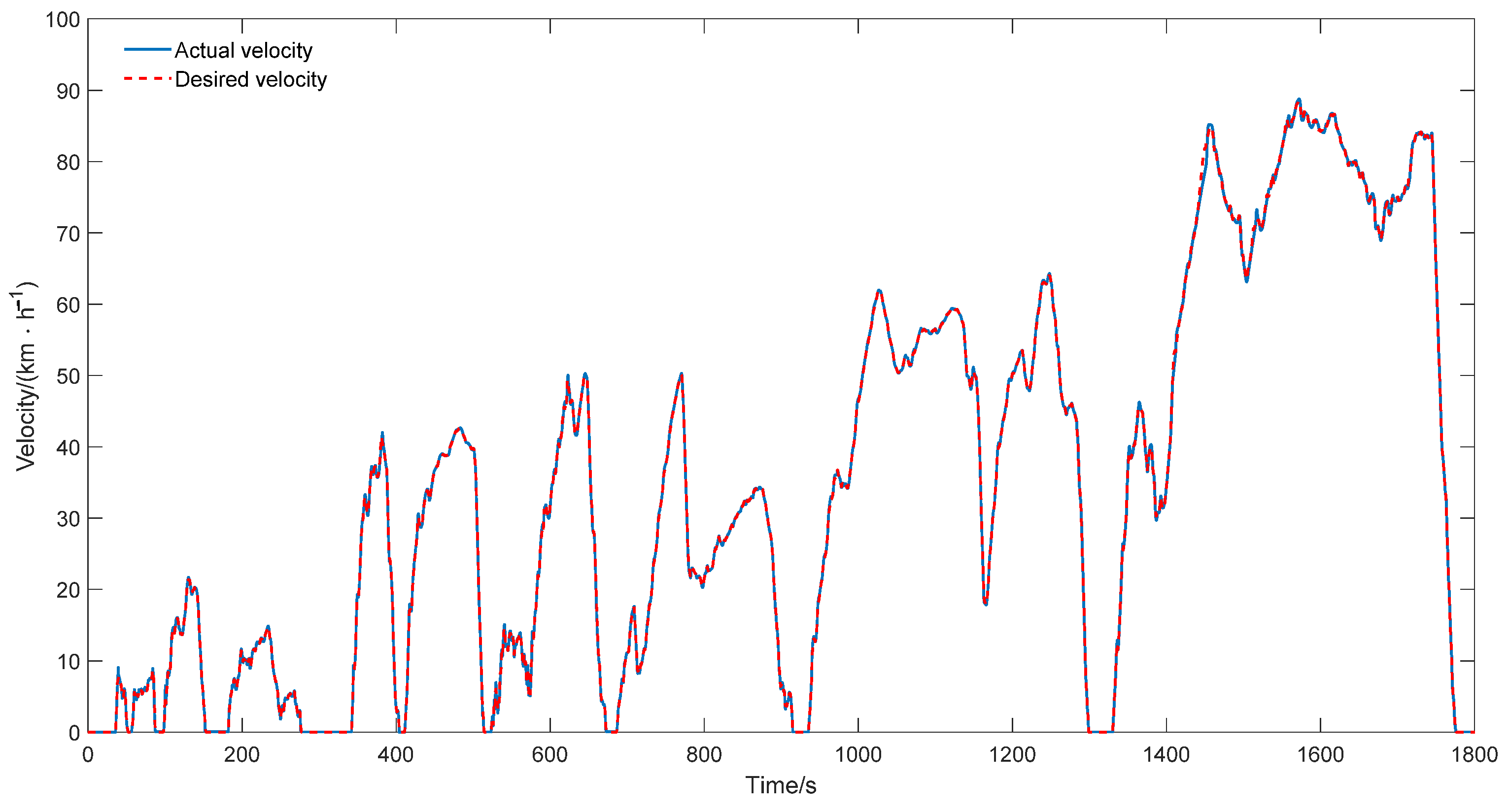
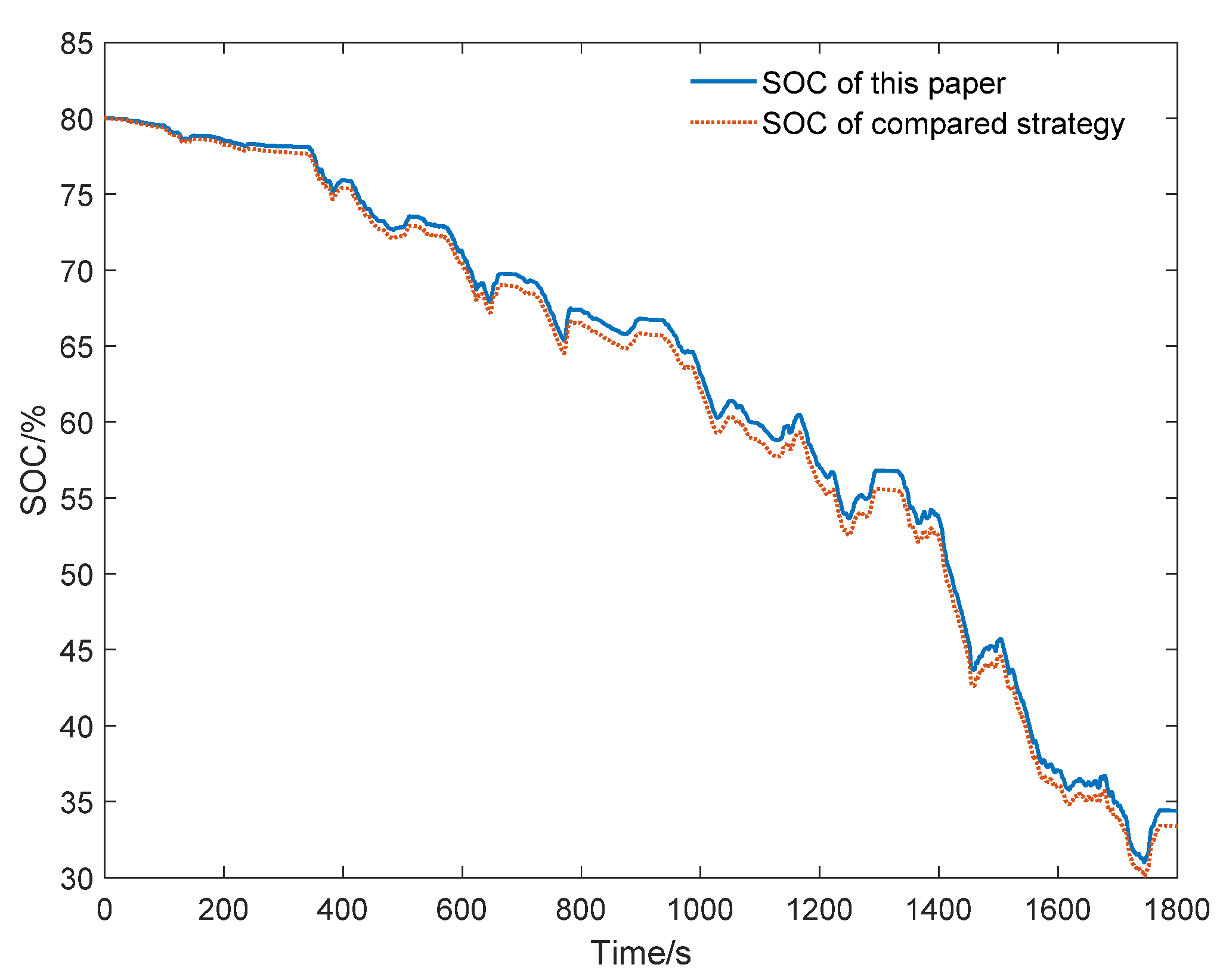
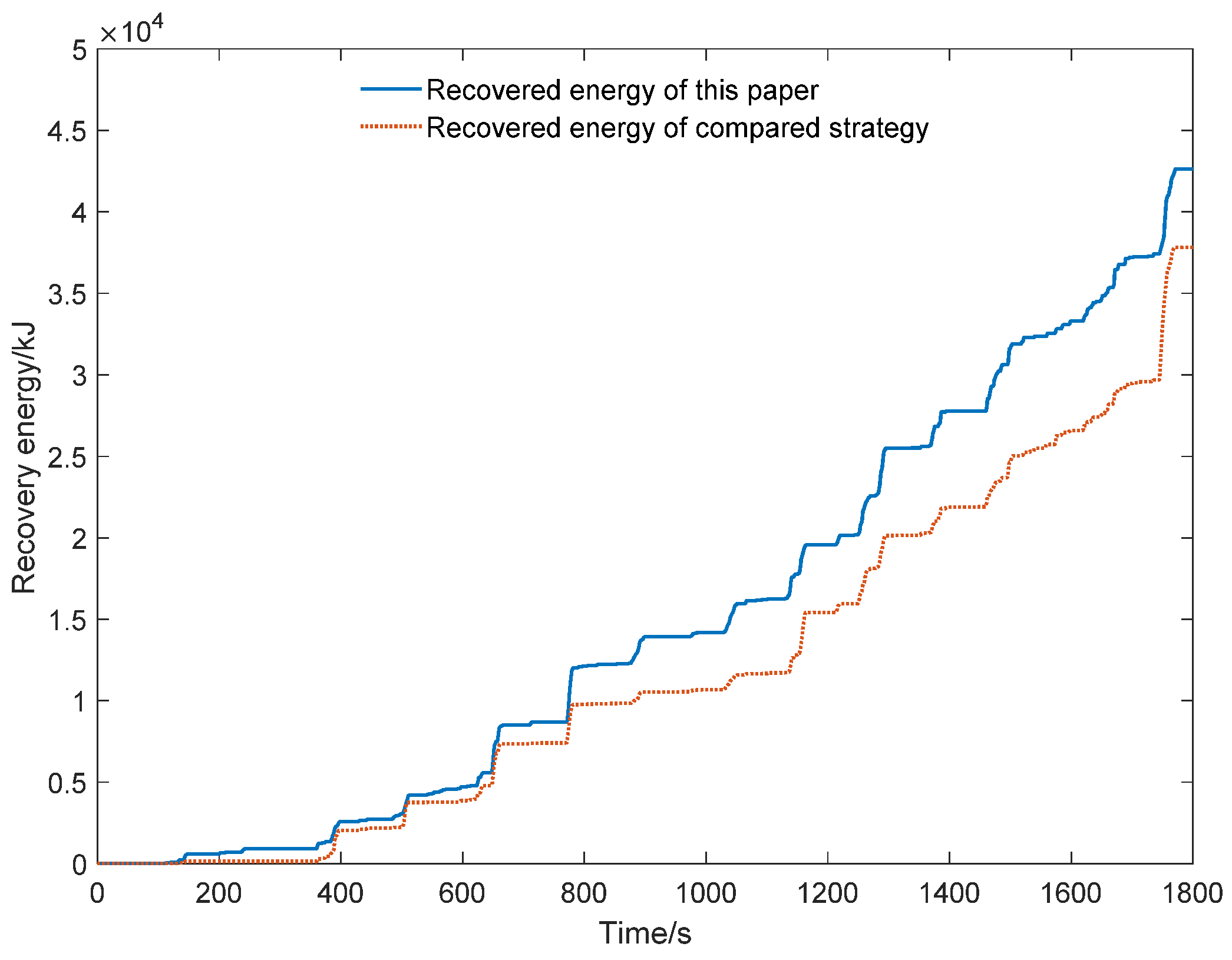
4.3. Simulation Results and Analysis of the Cycle Condition
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Burke, A.F.; Zhao, J.; Miller, M.R.; Sinha, A.; Fulton, L.M. Projections of the Costs of Medium- and Heavy-Duty Battery-Electric and Fuel Cell Vehicles (2020–2040) and Related Economic Issues. Energy Sustain. Dev. 2023, 77, 101343. [Google Scholar] [CrossRef]
- Zhang, X.; Lin, Z.; Crawford, C.; Li, S. Techno-Economic Comparison of Electrification for Heavy-Duty Trucks in China by 2040. Transp. Res. Part D Transp. Environ. 2022, 102, 103152. [Google Scholar] [CrossRef]
- Li, L.; Wang, H.; Pi, D.; Wang, X.; Wang, E.; Chen, Y. Research on Torque Distribution Control of High-Performance Distributed Drive Multi-Axle Special Vehicle. In Proceedings of the 2022 6th CAA International Conference on Vehicular Control and Intelligence (CVCI), Nanjing, China, 28–30 October 2022; pp. 1–8. [Google Scholar]
- Liu, Z.-q.; Lin, K.-l. An Experimental Study on the Brake Performance of Large Multi-Axle Construction Vehicles. J. Jiangsu Univ. Sci. Technol. (Nat. Sci.) 2000, 21, 20–23. [Google Scholar]
- Wang, Z.; Qian, Y.; Guo, C. The match design of braking system for a triaxial mid-sized-truck. J. HeFei Univ. Technol. 2007, 30, 16–18. [Google Scholar]
- Hou, X. Study on Modeling and Simulation of Air-Hydraulic Brake System. Master’s Thesis, Wuhan University of Technology, Wuhan, China, 2014. (In Chinese). [Google Scholar]
- Wang, Z.; Zhou, X.; Yang, C.; Hu, B. Effects of Driver’s Braking Intention on Hysteresis Characteristics of Emergency Braking in Multi-axle Vehicle. Automot. Eng. 2018, 40, 1185–1191. [Google Scholar] [CrossRef]
- Bartlett, W.D. Calculation of Deceleration Rates for S-Cam Air-Braked Heavy Trucks Equipped with Anti-Lock Brake Systems; SAE International: Warrendale, PA, USA, 2007. [Google Scholar]
- Chen, S. Study on Braking Delay Characteristic of Associated Braking System of Heavy Equipment Transporter. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2019. [Google Scholar]
- He, X.; Cheng, H.; Liu, Z.; Yang, J.; Huang, T. Comparative Study on Anti-Lock Braking Control Strategies Based on Heavy-Duty Multi-Axle Special Vehicles. In Proceedings of the 2021 IEEE 4th Advanced Information Management, Communicates, Electronic and Automation Control Conference (IMCEC), Chongqing, China, 18–20 June 2021; Volume 4, pp. 780–785. [Google Scholar]
- Zhang, W. Research of ABS Algorithm for Multi-Axle Vehicles and HIL Test. Doctor Thesis, Wuhan University of Technology, Wuhan, China, 2015. [Google Scholar]
- Wang, D.-F.; Ji, F.; Chen, S.-M.; Su, L.-L.; Hao, H. Simulation analysis and experiment of pneumatic ABS performance for multi-axles heavy-duty truck. J. Jilin Univ. (Eng. Technol. Ed.) 2012, 42, 7–12. [Google Scholar]
- Pennycott, A.; De Novellis, L.; Gruber, P.; Sorniotti, A. Optimal Braking Force Allocation for a Four-Wheel Drive Fully Electric Vehicle. Proc. Inst. Mech. Eng. Part I J. Syst. Control. Eng. 2014, 228, 621–628. [Google Scholar] [CrossRef]
- Yan, Y.; Zhang, J.; Xu, X.; Huang, L. Research on Regenerative Braking Control Strategy of Dual-Motor Driven Electric Vehicle. Automob. Technol. 2019, 6, 1–7. [Google Scholar] [CrossRef]
- Wu, T.; Wang, F.; Ye, P. Regenerative Braking Strategy of Dual-Motor EV Considering Energy Recovery and Brake Stability. World Electr. Veh. J. 2023, 14, 19. [Google Scholar] [CrossRef]
- Yu, D.; Wang, W.; Zhang, H.; Xu, D. Research on Anti-Lock Braking Control Strategy of Distributed-Driven Electric Vehicle. IEEE Access 2020, 8, 162467–162478. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, C.; Yang, S.; Tang, X. Study on Top Hierarchy Control Strategy of AEBS over Regenerative Brake and Hydraulic Brake for Hub Motor Drive BEVs. Energies 2022, 15, 8382. [Google Scholar] [CrossRef]
- Song, X. Design and Control Research on the Electric Wheel of 320 Tons Multi-Axis Mine Truck. Master’s Thesis, Wuhan University of Technology, Wuhan, China, 2015. [Google Scholar]
- Zhang, W. Research on Technologies of Energy Management and Energy Saving for Electric Mining Truck. Doctor Thesis, University of Science and Technology Beijing, Beijing, China, 2019. [Google Scholar]
- Hu, X. Design and Research on Joint Electromechanical Brake System of Electric Drive Mining Dump Truck. Coal Mine Mach. 2015, 36, 64–68. [Google Scholar] [CrossRef]
- Shen, Y.; Zhu, M.; Liu, X.; Li, L. Study on topological configuration and performance of composite braking systemof distributed driving multi-axis vehicle. J. Dyn. Control 2021, 19, 31–37. [Google Scholar]
- Li, X.; Ma, J.; Zhao, X.; Wang, L. Study on Braking Energy Recovery Control Strategy for Four-Axle Battery Electric Heavy-Duty Trucks. Int. J. Energy Res. 2023, 2023, 1868528. [Google Scholar] [CrossRef]
- Wan, Z.; Gao, F.; Ding, J.; Wu, X. Dynamics model and braking performance analysis of multi-axle vehicle. China Mech. Eng. 2008, 19, 365–369. [Google Scholar]
- Heydari, S.; Fajri, P.; Rasheduzzaman, M.; Sabzehgar, R. Maximizing Regenerative Braking Energy Recovery of Electric Vehicles Through Dynamic Low-Speed Cutoff Point Detection. IEEE Trans. Transp. Electrif. 2019, 5, 262–270. [Google Scholar] [CrossRef]
- Han, J.; Park, Y.; Park, Y. Cooperative Regenerative Braking Control for Front-Wheel-Drive Hybrid Electric Vehicle Based on Adaptive Regenerative Brake Torque Optimization Using under-Steer Index. Int. J. Automot. Technol. 2014, 15, 989–1000. [Google Scholar] [CrossRef]
- Panagiotidis, M.; Delagrammatikas, G.; Assanis, D. Development and use of a regenerative braking model for a parallel hybrid electric vehicle. SAE Trans. 2000, 109, 1180–1191. [Google Scholar]







| Parameter | Value | Unit | |
|---|---|---|---|
| Vehicle parameters | Curb weight/m0 | 19,000 | kg |
| Max. weight/M | 47,000 | kg | |
| Wind resistance coefficient/Cd | 0.29 | - | |
| Windward area/A | 7.58 | m2 | |
| Tire radius/r | 0.59 | m | |
| Rolling resistance coefficient/f | 0.02 | - | |
| Distance of each axle to 1st axis | 0/3.3/6.7/9.1/11.5 | m | |
| Max. velocity | 90 | km/h | |
| Reduction ratio/i0 | 10.81 | - | |
| Hub motor | Peak power | 110 | kW |
| Rated power | 80 | N∙m | |
| Max. torque | 1100 | N∙m | |
| Peak speed | 5000 | r∙min−1 |
| Braking Condition | Electric Braking | Hybrid Electro-Hydraulic Braking | |
|---|---|---|---|
| Performance | |||
| Braking time/s | 17.2 | 3.1 | |
| Braking distance/m | 121 | 23 | |
| Recovery energy/kJ | 2407 | 1698 | |
| Braking energy consumption/kJ | 6170 | 6170 | |
| Braking energy recovery rate/% | 39 | 27.5 | |
| Braking Strategies | Control Strategy in This Paper | Comparison Strategy | |
|---|---|---|---|
| Performance | |||
| Driving distance/m | 17,317 | ||
| Initial SOC/% | 80 | ||
| Terminal SOC/% | 35.36 | 34.39 | |
| Consumed energy/kJ | 138,500 | ||
| Recovered braking energy/kJ | 42,632 | 37,824 | |
| Braking energy recovery rate/% | 30.78 | 27.31 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, S.; Zhang, X.; Jiao, Y.; Wei, L.; He, J.; Zeng, X. Research on the Multi-Mode Composite Braking Control Strategy of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles. World Electr. Veh. J. 2024, 15, 83. https://doi.org/10.3390/wevj15030083
Xu S, Zhang X, Jiao Y, Wei L, He J, Zeng X. Research on the Multi-Mode Composite Braking Control Strategy of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles. World Electric Vehicle Journal. 2024; 15(3):83. https://doi.org/10.3390/wevj15030083
Chicago/Turabian StyleXu, Shiwei, Xiaopeng Zhang, Yuan Jiao, Lulu Wei, Jingjing He, and Xinyu Zeng. 2024. "Research on the Multi-Mode Composite Braking Control Strategy of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles" World Electric Vehicle Journal 15, no. 3: 83. https://doi.org/10.3390/wevj15030083
APA StyleXu, S., Zhang, X., Jiao, Y., Wei, L., He, J., & Zeng, X. (2024). Research on the Multi-Mode Composite Braking Control Strategy of Electric Wheel-Drive Multi-Axle Heavy-Duty Vehicles. World Electric Vehicle Journal, 15(3), 83. https://doi.org/10.3390/wevj15030083






