Actual and Balanced Stand Structure: Examples from Beech-Fir-Spruce Old-Growth Forests in the Area of the Dinarides in Bosnia and Herzegovina
Abstract
:1. Introduction
2. Materials and Methods
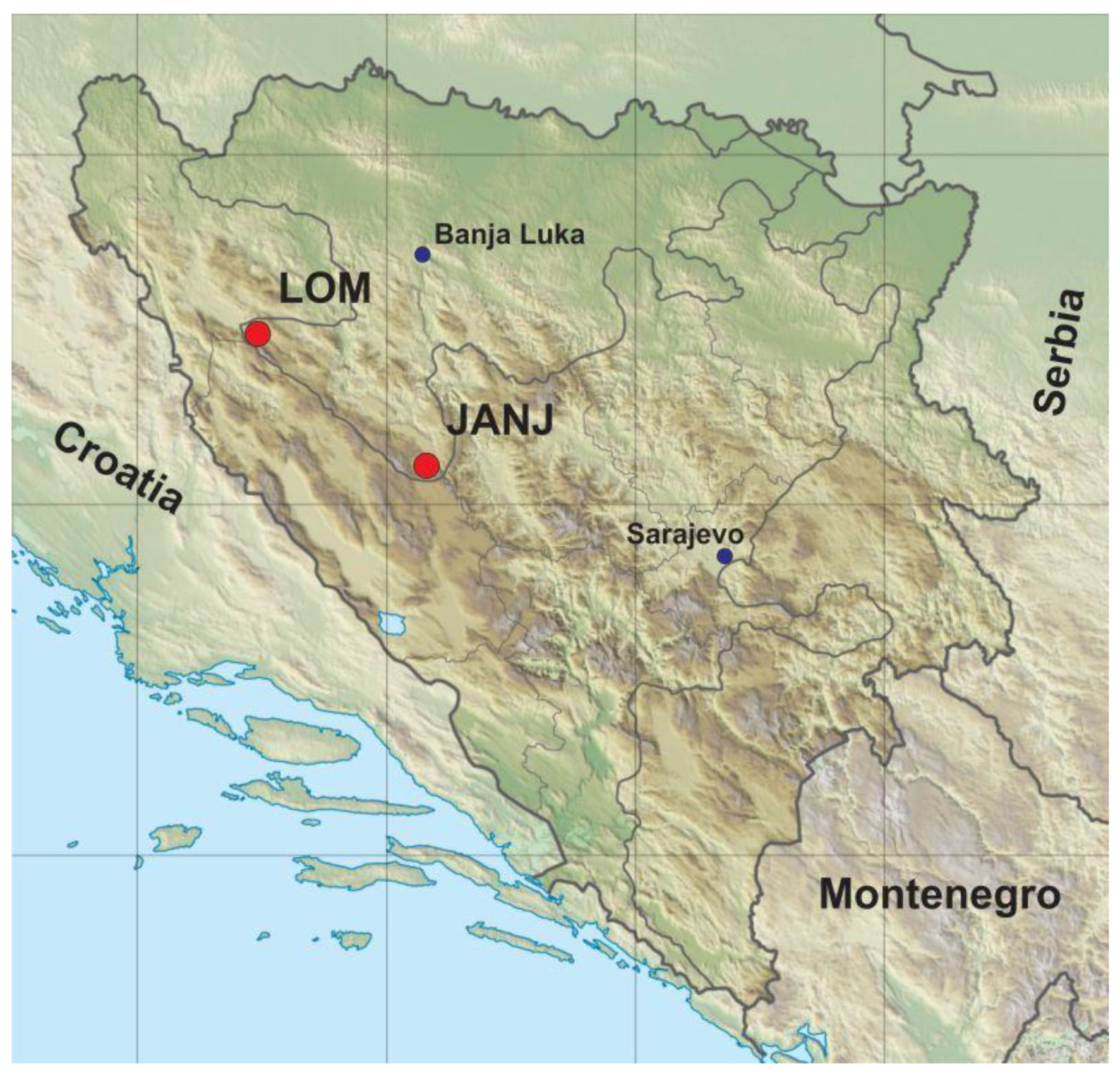
2.1. Study Site
2.2. Analysis
3. Results
3.1. The Quality of Stands
3.2. Actual and Balanced Tree Diameter Distributions
4. Discussion
5. Conclusions
Author Contributions
Conflicts of Interest
References and Notes
- von Carlowitz, H.C. Sylvicultura Oeconomica, oder Haußwirthliche Nachricht und Naturmäßige Anweisung zur Wilden Baum Zucht; Oekom Verlag: Leipzig, Germany, 1713. [Google Scholar]
- Hartig, G.L. Anweisung für die Taxation der Forste oder zur Bestimmung des Hokertrags der Wälder; Heger Verlag: Giessen, Germany, 1795. [Google Scholar]
- Schabel, H.G.; Palmer, S.L. The Dauerwald: Its role in the Restoration of Natural Forests. J. For. 1999, 97, 20–25. [Google Scholar]
- Schütz, J.P.; Saniga, M.; Diaci, J.; Vrška, T. Comparing close-to-nature silviculture with processes in pristine forests: Lessons from Central Europe. Ann. For. Sci. 2016, 73, 1–13. [Google Scholar] [CrossRef]
- Klepac, D. Onekim normalama u jelovim prebirnim šumama. Šumarski List 1961, 3–4, 85–90. [Google Scholar]
- Klepac, D. Normalne frekvencijske krivulje broja stabala u prebornoj šumi. Šumarski List 1961, 9–10, 333–341. [Google Scholar]
- Flury, Ph. Üeber den Aufbau des Plenterwaldes. Mittgl. D. Schw. Anstalt f. d. Forstl. Versuchswesen 1929, 15, 305–357. [Google Scholar]
- Flury, Ph. Üeber Bildung von Starkeklassen bei der Forsteinrichtung. Schweizerische Zeitschrift für Forstwesen 1932, 83, 78–84. [Google Scholar]
- Biolley, H.E. L’aménagement des forêts par la méthode expérimentale et spécialement la méthode du contrôle; Attinger Frères: Paris, France, 1920; p. 85. [Google Scholar]
- Biolley, H.E. Die Forsteinrichtung auf der Grundlage der Erfahrung und insbesondere das Kontrollverfahren in Deutsche übersetzung von Eberbach. Karlsruhe 1922, 1–72. [Google Scholar]
- Prodan, M. Die theoretische Bestimmung des Gleichgewichtszustandes im Plenterwalde. Schweiz Z. Forstwes. 1949, 100, 81–99. [Google Scholar]
- Mitscherlich, G. Der Tannen-Fichten-(Buchen)-Plenterwald: eine ertragskundliche Studie. Schriftenreiche der Badischen Forstlichen Versuchanstalt 1952, 8, 1–42. [Google Scholar]
- de Liocourt, F. De l'aménagement des sapinières. Bulletin Trimestriel, Société Forestière de Franche-Comté et Belfort. Julliet 1898, 4, 396–409. [Google Scholar]
- Kerr, G. The management of silver fir forests: De Liocourt (1898) revisited. Forestry 2014, 87, 29–38. [Google Scholar] [CrossRef]
- Bončina, A. History, current status and future prospects of uneven-aged forest management in the Dinaric region: An overview. Forestry 2011, 84, 467–468. [Google Scholar] [CrossRef]
- Leak, W.B. The J-shaped probability distribution. For. Sci. 1965, 11, 405–409. [Google Scholar]
- Meyer, H.A. Structure, growth, and drain in balanced uneven-aged forests. J. For. 1952, 50, 85–92. [Google Scholar]
- Ducey, M.J.; Gunn, J.S.; Whitman, A.A. Late-Successional and Old-Growth Forests in the Northeastern United States: Structure, Dynamics, and Prospects for Restoration. Forests 2013, 4, 1055–1086. [Google Scholar] [CrossRef]
- Mlinsek, D. Der naturnahe Waldbau—Sein kognitiver Weg—Eine Herausforderung. Der Dauerwald 1994, 10, 35–43. [Google Scholar]
- Hayashi, T.; Sawauchi, D.; Kunii, D. Forest Maintenance Practices and Wood Energy Alternatives to Increase Uses of Forest Resources in a Local Initiative in Nishiwaga, Iwate, Japan. Sustainability 2017, 9, 1–13. [Google Scholar] [CrossRef]
- Keren, S.; Diaci, J.; Motta, R.; Govedar, Z. Stand structural complexity of mixed old-growth and adjacent selection forests in the Dinaric Mountains of Bosnia and Herzegovina. For. Ecol. Manag. 2017, 400, 531–541. [Google Scholar] [CrossRef]
- Parviainen, J. Virgin and natural forests in the temperate zone of Europe. For. Snow Landsc. Res. 2005, 79, 9–18. [Google Scholar]
- Ford, S.E.; Keeton, W.S. Enhanced carbon storage through management for old-growth characteristics in northern hardwood-conifer forests. Ecosphere 2017, 8. [Google Scholar] [CrossRef]
- Nagel, T.A.; Svoboda, M. Gap disturbance regime in an old-growth Fagus-Abies forest in the Dinaric Mountains, Bosnia-Herzegovina. Can. J. For. Res. 2008, 38, 2728–2737. [Google Scholar] [CrossRef]
- Kimmins, J.P.; Rempel, R.; Welham, C.; Seely, B.; Rees, V.K. Biophysical sustainability, process-based monitoring and forest ecosystem management decision support systems. For. Chron. 2007, 83, 502–514. [Google Scholar] [CrossRef]
- Schütz, J.P. Die Plenterung und ihre unterschiedlichen Formen. In Skript zu Vorlesung Waldbau II und Waldbau IV; ETH Zentrum: Zürich, Switzerland, 2002; pp. 1–126. [Google Scholar]
- Božić, M.; Čavlović, J.; Goršić, E.; Teslak, K. Dinamika uspostave preborne strukture u bukovo-jelovim sastojinama na Papuku. Croat. J. For. Eng. 2011, 32, 287–300. [Google Scholar]
- Horvat, I. Šumske zajednice Jugoslavije. Šumarska enciklopedija II, Jugoslavenski leksikografski zavod, Zagreb, 1962, 560–590.
- Stefanović, V.; Beus, V. Šume bukve i jele (Abieti-Fagetum sens.lat.) Dinarida sa aspekta ekološko-vegetacijske rejonizacije Bosne i Hercegovine. In Mijušković, M., Lakušić, R., Eds. Prirodne i društvene vrijednosti NP “Biogradska gora”, Crnogorska akademija nauka i umjetnosti, Titograd 1991, 168–175.
- Stefanović, V.; Beus, V.; Burlica, Č.; Dizdarević, H.; Vukorep, I. Ekološko-vegetacijska rejonizacija Bosne i Hercegovine. Posebna izdanja Šumarskog fakulteta u Sarajevu 1983, 17, 1–51. [Google Scholar]
- Banković, S.; Pantić, D. Dendrometrija [Dendrometry]. Univerzitet u Beogradu Šumarski fakultet 2006, 1–556. [Google Scholar]
- Drinić, P.; Matić, V.; Pavlić, J.; Prolić, N.; Stojanović, O.; Vukmirović, V. Tablice taksacionih elemenata visokih i izdanačkih šuma u BiH. Posebna izdanja Šumarskog fakulteta u Sarajevu 1980, 13, 1–222. [Google Scholar]
- Meyer, H.A. Eine mathematisch-statistische Untersuchung über den Aufbau des Plenterwaldes. Schweizerische Zeitschrift für Forstwesen 1933, 84, 33–46. [Google Scholar]
- Meyer, H.A.; Stevenson, D.D. The structure and growth of virgin beech-birch-maple-hemlock forests in northern Pennsylvania. J. Agric. Res. 1943, 67, 465–484. [Google Scholar]
- Susmel, L. Leggi di variazione dei parametri della Foresta disetanea normale. L’Italia Forestale e Montana, Firenze 1956, 3, 105–116.
- Colette, L. Trente années de contrle en hêtraie jardinée. Ministère de l’Agriculture, Administration des Eaux et Forêts, Station de Recherches de Groenendaal-Hoeilaart, 1960, Travaux-Série B, 25, p44.
- O’Toole, A.L. Elementary Practical Statistics; Allendorfer Undergraduate Series; The Mecmilan Company: Basingstoke, UK, 1964; pp. 1–416. [Google Scholar]
- Kotar, M. Prebiralni gozd in prebiralno gospodarjenje v Sloveniji. Gozd. Vestn. 2002, 60, 7–9. [Google Scholar]
- Miletić, Ž. Dalja istraživanja prinosne snage teoriјske normalne prebirne sastojine. Glasnik šumarskog fakulteta 1953, 6, 105–125. [Google Scholar]
- Tregubov, V.S. Les forêts vierges montagnardes des Alpes Dinariques—Massif de Klekovatcha-Guermetch: Étude Botanique et Forestière. Causse Graille et Castelnau Montpellier 1941, 78, 1–118. [Google Scholar]
- Drinić, P. Taksacioni elementi sastojina jele, smrče i bukve prašumskog tipa u Bosni. Radovi Poljoprivredno-šumarskog fakulteta Sarajevo 1956, 1, 107–160. [Google Scholar]
- Motta, R.; Berretti, R.; Castagneri, D.; Dukić, V.; Garbarino, M.; Govedar, Z.; Lingua, E.; Maunaga, Z.; Meloni, F. Toward a definition of the range of variability of central European mixed Fagus-Abies-Picea forests: The nearlysteady-state forest of Lom (Bosnia and Herzegovina). Can. J. For. Res. 2011, 41, 1871–1884. [Google Scholar] [CrossRef]
- Burger, H. Untersuchungen uber das Hohen-wachstum verschiedener Holzarten. Mitt. Schweiz. Cent. Frst Vers. 1926, 14, 1–158. [Google Scholar]
- Leibundgut, H. Waldbauliche Untersuchungen uber den Aufbau von Plenterwalden. Mittgl. D. Schw. 1945, 24, 96–219. [Google Scholar]
- Šafar, J. Problem nadiranja i širenja bukve u arealu jele. Poseban otisak iz knjige. Anali Instituta za eksperimentalno šumarstvo Jugoslavenske akademije 1955, 1, 147–189. [Google Scholar]
- Mlinšek, D. Rast in sposobnost reagiranja pragozdne bukve. Zb. Bioteh. Fak. Ljubl. 1967, 15, 63–79. [Google Scholar]
- Fukarek, P. Prašuma Perućica nekad i danas. Posebni Otisak—Separatum Narodni Šumar 1965, 19, 39–50. [Google Scholar]
- Bottero, A.; Garbarino, M.; Dukic, V.; Govedar, Z.; Lingua, E.; Nagel, A.T.; Motta, R. Gap-Phase Dynamics in the Old-Growth Forest of Lom, Bosnia and Herzegovina. Silva Fenn. 2011, 45, 875–887. [Google Scholar] [CrossRef]
- Garbarino, M.; Mondino, E.B.; Lingua, E.; Nagel, T.A.; Dukic, V.; Govedar, Z.; Motta, R. Gap disturbances and regeneration patterns in a Bosnian old-growth forest: A multispectral remote sensing and ground-based approach. Ann. For. Sci. 2012, 69, 617–625. [Google Scholar] [CrossRef]
- Schütz, J.P. Opportunities and strategies of transforming regular forests to irregular forests. For. Ecol. Manag. 2001, 151, 87–94. [Google Scholar] [CrossRef]
- Keren, S.; Motta, R.; Govedar, Z.; Lucic, R.; Medarevic, M.; Diaci, J. Comparative Structural Dynamics of the Janj Mixed Old-Growth Mountain Forest in Bosnia and Herzegovina: Are Conifers in a Long-Term Decline? Forests 2014, 5, 1243–1266. [Google Scholar] [CrossRef]
- Boncina, А.; Cavlovic, J.; Curovic, M.; Govedar, Z.; Klopcic, M.; Medarevic, M. A comparative analysis of recent changes in Dinaric uneven-aged forests of the NW Balkans. J. For. Res. 2014, 87, 1–14. [Google Scholar] [CrossRef]
- Keren, S. Complexity of Stand Structures in Two Mixed Mountain Old-Growth Forests and Adjacent Managed Forests in Bosnia and Herzegovina. Ph.D. Thesis, University of Ljubljana, Ljubljana, Slowenien, 2015; pp. 1–147. [Google Scholar]
- Stojanović, L.J.; Krstić, M.; Medarević, M.; Bjelanović, I. Prebirno gazdovanja u mešovitim šumama jele, smrče i bukve na Zlataru. Šumarstvo 2008, 3, 31–52. [Google Scholar]
- Cestar, D.; Hren, V.; Kovačević, Z.; Martinović, J.; Pelcer, Z. Instructions for preparation of the map of ecological-management types of mountainous area (I) of the Republic of Croatia. Rad. Sumar. Inst. Izvanr. Izd. 1986, 4, 1–125. [Google Scholar]
- Keeton, W.S.; Chernyavskyy, M.; Gratzer, G.; Main-Knorn, M.; Shpylchak, M.; Bihun, Y. Structural characteristics and aboveground biomass of old-growth spruce fir stands in the eastern Carpathian mountains, Ukraine. Plant Biosyst. 2010, 144, 148–159. [Google Scholar] [CrossRef]
- Yoshida, T.; Naito, S.; Nagumo, M.; Hyodo, N.; Inoue, T.; Umegane, H.; Yamazaki, H.; Miya, H.; Nakamura, F. Structural Complexity and Ecosystem Functions in a Natural Mixed Forest under a Single-Tree Selection Silviculture. Sustainability 2017, 9, 1–15. [Google Scholar] [CrossRef]
- Schütz, J.P. Der Plenterwald und weitere Formen strukturierter und gemischter Wälder; Verlag Eugen Ulmer; Auflage: Berlin, Germany, 2011. [Google Scholar]
- Leibundgut, H. Über Waldbau auf naturgesetzliche Grundlage. Beiheft zu den Zeitschriften des Schweizerischen Forstvereins Nr 21, Zürich, 1943, 141–155.
- Korpel, S. Die Urwalder der Westkarpaten; Gustav Fischer Verlang: Jena, Germany, 1995; pp. 1–310. [Google Scholar]
- Govedar, Z.; Krstić, M. Natural regeneration of beech (Fagus sylvatica L.) in the virgin forest Lom in the west part of the Republic of Srpska. In Proceedings of the International Conference on Beech Silviculture in Europe,s Larges Beech Country, Brasov, Romania, 4–8 September 2006; pp. 36–38. [Google Scholar]
- Govedar, Z.; Stanivukovic, Z.; Cukovic, D.; Lazendic, Z. Basic taxation characteristics of mixed stands of beech, fir and spruce in “Lom” virgin forest in the area of western part of the Republika Srpska. In Proceedings “Management of Forest Eco Systems of the National Parks and Other Protected Areas”; Faculty of Forestry University of Banja Luka, 2006; pp. 285–295. [Google Scholar]
- Li, F.; Lu, S.; Sun, Y.; Li, X.; Xi, B.; Liu, W. Integrated Evaluation and Scenario Simulation for Forest Ecological Security of Beijing Based on System Dynamics Model. Sustainability 2015, 7, 13631–13659. [Google Scholar] [CrossRef]
- Gain, D.; Watanabe, T. Expert Evaluation of Subsidies for the Management of Fragmented Private Forest in Regards to National Biodiversity Goals—The Case of Kochi Prefecture, Japan. Sustainability 2017, 9, 1–15. [Google Scholar] [CrossRef]
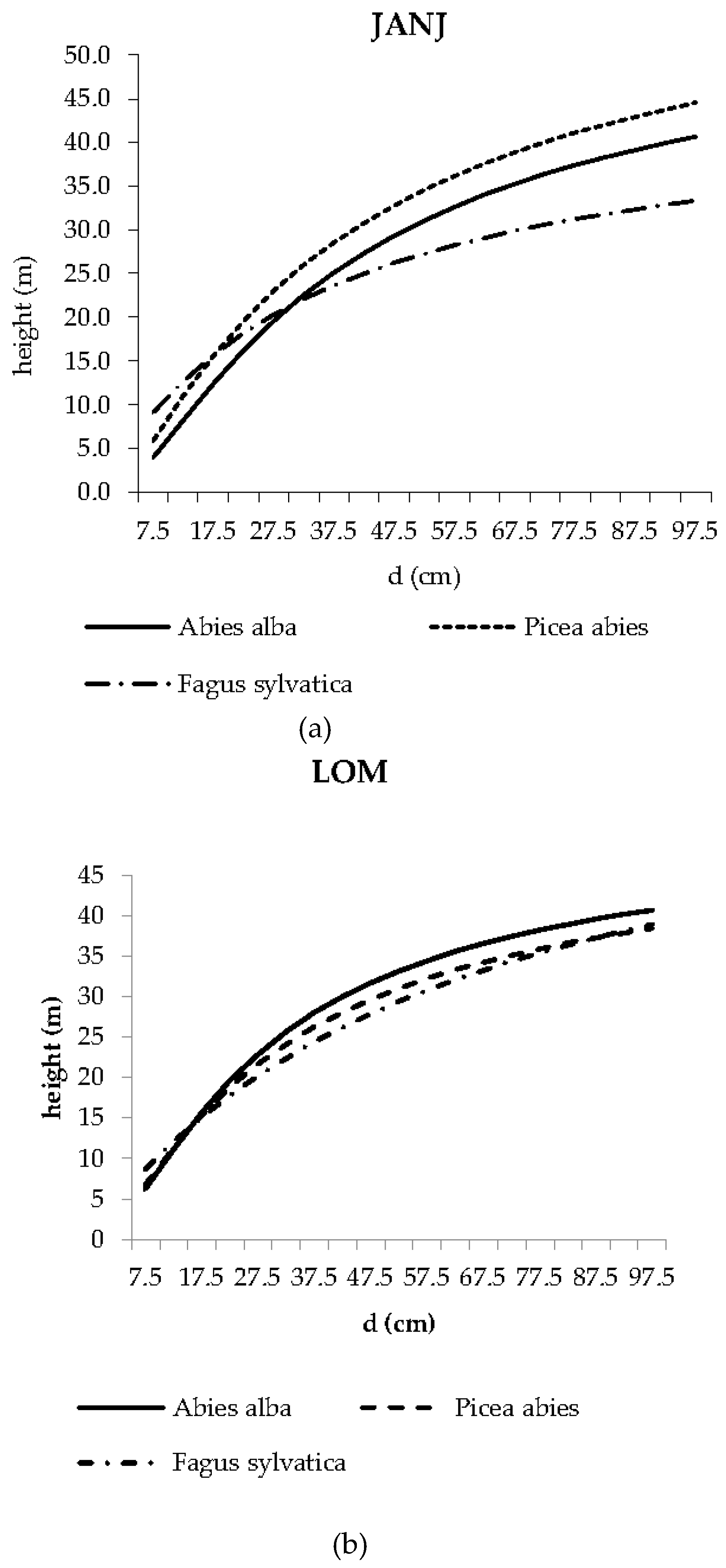


| Stand | Species | a | b | c | R2 | Se (m) | Site Class |
|---|---|---|---|---|---|---|---|
| Janj | Fir | 5.5046 | 0.6630 | 0.0202 | 98.5 | 1.14 | II |
| Spruce | 9.5387 | 0.2340 | 0.0228 | 89.6 | 2.86 | II | |
| Beech | −0.9180 | 0.7823 | 0.0220 | 75.6 | 3.01 | III | |
| Lom | Fir | 6.8039 | 0.4840 | 0.0197 | 71.4 | 4.37 | I |
| Spruce | 4.5473 | 0.6313 | 0.0200 | 83.7 | 3.88 | II | |
| Beech | −1.2380 | 1.0639 | 0.0158 | 79.0 | 4.07 | II |
| Stand | Hdom. | Dmax. | Gnorm. | Vnorm. | q | |
|---|---|---|---|---|---|---|
| Janj | Fir | 38.1 | 100.3 | 36.9 35.9 | 483.9 458.8 | 1.28 |
| Spruce | 37.1 | 97.7 | 1.29 | |||
| Beech | 36.0 | 83.4 | 26.6 | 305.6 | 1.37 | |
| Lom | Fir | 42.2 | 110.9 | 40.9 35.0 | 593.6 434.4 | 1.24 |
| Spruce | 36.1 | 95.0 | 1.30 | |||
| Beech | 35.0 | 81.6 | 25.5 | 288.9 | 1.39 |
| d | Experimental Number of Trees Per Hectare | Theoretical Number of Trees Per Hectare | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| JANJ | LOM | JANJ | LOM | |||||||||||||
| cm | A.a. | P.a. | F.s. | Total | A.a. | P.a. | F.s. | Total | A.a. | P.a. | F.s. | Total | A.a. | P.a. | F.s. | Total |
| 7.5 | 93 | 23 | 69 | 185 | 88 | 18 | 105 | 211 | 49 | 40 | 29 | 118 | 38 | 42 | 30 | 110 |
| 12.5 | 14 | 7 | 36 | 57 | 5 | 11 | 22 | 38 | 38 | 31 | 21 | 91 | 31 | 32 | 22 | 85 |
| 17.5 | 21 | 7 | 21 | 50 | 5 | 5 | 16 | 27 | 30 | 24 | 15 | 70 | 25 | 25 | 16 | 65 |
| 22.5 | 14 | 14 | 24 | 53 | 18 | 7 | 25 | 51 | 23 | 19 | 11 | 53 | 20 | 19 | 11 | 50 |
| 27.5 | 14 | 5 | 14 | 33 | 7 | 4 | 11 | 22 | 18 | 14 | 8 | 41 | 16 | 15 | 8 | 39 |
| 32.5 | 5 | 9 | 4 | 18 | 9 | 3 | 18 | 30 | 14 | 11 | 6 | 31 | 13 | 11 | 6 | 30 |
| 37.5 | 13 | 11 | 9 | 32 | 7 | 5 | 15 | 27 | 11 | 9 | 4 | 24 | 11 | 9 | 4 | 23 |
| 42.5 | 5 | 13 | 7 | 25 | 7 | 1 | 18 | 26 | 9 | 7 | 3 | 19 | 9 | 7 | 3 | 18 |
| 47.5 | 13 | 7 | 9 | 29 | 5 | 4 | 15 | 24 | 7 | 5 | 2 | 14 | 7 | 5 | 2 | 14 |
| 52.5 | 8 | 6 | 4 | 17 | 7 | 1 | 6 | 14 | 5 | 4 | 2 | 11 | 6 | 4 | 2 | 11 |
| 57.5 | 6 | 6 | 3 | 15 | 2 | 2 | 4 | 9 | 4 | 3 | 1 | 9 | 5 | 3 | 1 | 9 |
| 62.5 | 7 | 5 | 5 | 17 | 8 | 6 | 2 | 16 | 3 | 2 | 1 | 7 | 4 | 2 | 1 | 7 |
| 67.5 | 6 | 5 | 3 | 14 | 4 | 3 | 1 | 8 | 3 | 2 | 1 | 5 | 3 | 2 | 1 | 5 |
| 72.5 | 8 | 6 | 1 | 14 | 4 | 5 | 2 | 12 | 2 | 1 | 3 | 2 | 1 | 4 | ||
| 77.5 | 6 | 3 | 1 | 10 | 4 | 3 | 8 | 2 | 1 | 3 | 2 | 1 | 3 | |||
| 82.5 | 2 | 3 | 5 | 4 | 5 | 8 | 1 | 1 | 2 | 2 | 1 | 2 | ||||
| 87.5 | 2 | 2 | 4 | 1 | 3 | 4 | 1 | 1 | 2 | 1 | 1 | 2 | ||||
| 92.5 | 2 | 1 | 3 | 2 | 2 | 4 | 1 | 1 | 1 | 1 | 1 | |||||
| 97.5 | 1 | 1 | 2 | 1 | 2 | 3 | 1 | 1 | 1 | 1 | ||||||
| 102.5 | 1 | 1 | 2 | 1 | 1 | 2 | 1 | 1 | ||||||||
| 107.5 | 1 | 1 | 2 | 1 | 1 | 1 | 1 | |||||||||
| 112.5 | 1 | 1 | ||||||||||||||
| 117.5 | 1 | 1 | ||||||||||||||
| 122.5 | 1 | 1 | ||||||||||||||
| Total | 243 | 136 | 209 | 588 | 194 | 93 | 261 | 548 | 223 | 177 | 105 | 505 | 195 | 180 | 106 | 481 |
| Old-Growth Forests | k | a | max|Fn − Ft| | |
|---|---|---|---|---|
| Janj | 84.67 | 0.03 | 0.126 | 0.056 |
| Lom | 57.62 | 0.02 | 0.079 | 0.058 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Govedar, Z.; Krstić, M.; Keren, S.; Babić, V.; Zlokapa, B.; Kanjevac, B. Actual and Balanced Stand Structure: Examples from Beech-Fir-Spruce Old-Growth Forests in the Area of the Dinarides in Bosnia and Herzegovina. Sustainability 2018, 10, 540. https://doi.org/10.3390/su10020540
Govedar Z, Krstić M, Keren S, Babić V, Zlokapa B, Kanjevac B. Actual and Balanced Stand Structure: Examples from Beech-Fir-Spruce Old-Growth Forests in the Area of the Dinarides in Bosnia and Herzegovina. Sustainability. 2018; 10(2):540. https://doi.org/10.3390/su10020540
Chicago/Turabian StyleGovedar, Zoran, Milun Krstić, Srđan Keren, Violeta Babić, Brane Zlokapa, and Branko Kanjevac. 2018. "Actual and Balanced Stand Structure: Examples from Beech-Fir-Spruce Old-Growth Forests in the Area of the Dinarides in Bosnia and Herzegovina" Sustainability 10, no. 2: 540. https://doi.org/10.3390/su10020540
APA StyleGovedar, Z., Krstić, M., Keren, S., Babić, V., Zlokapa, B., & Kanjevac, B. (2018). Actual and Balanced Stand Structure: Examples from Beech-Fir-Spruce Old-Growth Forests in the Area of the Dinarides in Bosnia and Herzegovina. Sustainability, 10(2), 540. https://doi.org/10.3390/su10020540






