Performance Analysis of an Integrated Solar Dehumidification System with HVAC in A Typical Corner Store in the USA

Abstract

Food deserts have emerged in underserved urban and rural areas throughout the United States. Corner markets have filled the food voids, but generally without offering residents access to healthy food. The economics for doing so are prohibitive. The purpose of the study is to investigate an opportunity for reducing corner store energy costs in order to make possible retail of fresh produce and meat. Given the typical dominance of refrigeration to the energy cost in such stores, an integrated solar dehumidification system with heating, ventilation, and air conditioning (HVAC) is considered. A typical corner store baseline reliant upon conventional refrigeration and HVAC equipment is defined to serve as a basis for comparison. MATLAB Simulink dynamic models are developed for the posed system and baseline model. The results show energy reduction in the refrigerated cabinets of maximally 28%, 27%, and 20%, respectively, in Dayton, OH, Phoenix, AZ, and Pine Bluff, AR. The respective HVAC energy savings are respectively 28%, 56%, and 4%. Collectively these correspond to total annual energy savings of 43%, 51%, and 53%, translating to annual energy cost savings of greater than $12K in all locations.

1. Introduction

1.1. Research Problem

Poor urban and rural areas throughout the United States have increasingly seen large groceries and supermarkets go out of business or move. Created in the aftermath of these closings are what has been termed food deserts, where people in large sections of cities do not have access to healthier food. According to the United States Department of Agriculture (USDA), 23.5 million Americans live in communities where access to fresh produce and meat is inadequate [1]. The social disparity in health is extreme, as has been remarkably seen during the COVID-19 pandemic, where the urban poor death rate is substantially greater than the population at large due to the higher preponderance of pre-existing conditions in these communities. For example, the death rate from COVID-19 in food deserts account for more than 70% of coronavirus deaths in the city of Chicago [2]. In Chicago, the death rate from diabetes in a food desert is twice that of regions with access to healthier food [3].

Corner stores of various varieties have moved into these food deserts to offset the food gaps which have resulted from the grocery departures or closures. These stores include dollar stores, mini-marts, and gas station connected food marts. These stores are located to be accessible to nearly all residents and ideally within walking distance. However, they in general have not provided access to healthier foods, e.g., fresh produce and meat.

Over the years, there have been continuous initiatives, both from local and national organizations, to encourage corner markets to sell healthy foods [4]. However, there have also been challenges to fully implement said initiatives. For one, it has been difficult to establish a healthy food corner market in areas where a generation or more of people have not been exposed to healthy diets or lack awareness of how to prepare healthy meals. Often corner store customers are not open to the purchase of healthier foods [5]. This trend is also observed in food pantries where fresh produce free for the taking is left to remain on the shelves.

A second barrier, and the sole focus of this paper, is the cost for businesses to purchase healthier foods and sell them at an affordable price. The small volume of sales dissuades interest by healthy food distributors in selling to corner markets. Often corner market owners who sell fresh produce purchase their food from the groceries they can access. Collectively, these two barriers virtually prohibit healthy food sells in corner markets.

In general, corner store markets have very slim profit margins [6]. Any additional cost burden such as the inclusion of fresh produce and meat likely is enough to push them into the red. This study is predicated on the idea that if the economic position of corner markets can be improved, then there at least is a possibility of adding healthier food to their product inventories. Therefore, this study aims to assess opportunities for corner stores to increase their profit margin through affordable investments aimed at reduced energy costs.

1.2. Previous Research in Grocery Store Energy Consumption

Many reported studies have investigated the performance of typical supermarket refrigerated display cases relative to the indoor store environment. For example, Howell and Adams developed a model to predict the energy consumption of (i) medium temperature, single shelf, (ii) medium temperature, multi shelf, and (iii) low temperature reach-in (open) storage cabinets in a 4656 ${\mathrm{m}}^2$ food store in Tampa, Florida, US [7]. Their results showed a savings of 10% in annual display case operating costs with a 5% reduction in store relative humidity. Another study explored experimentally the effect of reducing the internal store environmental relative humidity on annual energy consumption of eight supermarkets in Florida, US [8]. Controls were set in place to maintain a minimum relative humidity value in all stores which reached as low of a value of 37% in the month of March. The estimated annual food refrigeration energy savings for all of stores from this intervention was 45%. In Sweden, field measurement of temperature variations in refrigerated display case cabinets was conducted in three supermarkets. The study found that the energy consumption of the display case cabinets was influenced by the outdoor climate condition. They observed that electrical consumption by the display cabinets increased 55% during summer because of an increase in the store ambient humidity [9].

A number of studies have investigated the energy savings of closed relative to open refrigerated display cases [10]. For example, an experimental investigation of the effects of operating conditions including door opening frequency, ambient air temperature, and refrigerated case temperature distributions was performed [11]. This study found that closed display cabinets achieve better thermal homogeneity and less energy consumption than open display cabinet regardless of the door opening frequency.

Additionally, many studies have assessed the benefit of air curtains used in open display cases on energy consumption [12]. The variation of the thickness, width, height, and velocity of the air curtain along with its pressure and temperature difference on the performance of the display case refrigerated was investigated by Ge and Tassou [13].

Last of all, a few studies considered investigated phase change material (PCM) integration into refrigerated display cases to buffer transient impacts which often require the case to be maintained colder than needed. Potential energy savings have been documented. One study demonstrated a 6% reduction in energy for display cabinets outfitted with this technology for store environmental conditions of 25 °C and 60% RH [14].

Nearly all of the previous studies have mainly focused on large supermarkets. Corner stores with an area of 500 ${\mathrm{m}}^2$ or less have received very little attention, despite the fact that they are deemed to be the worst performing building type from an energy perspective [15]. There do exist a few benchmarks for corner market energy intensity. These are summarized in

Table 1. Benchmark for electric consumption in corner store.

Benchmark	Energy Intensity	Description
CBECS	$532.8 \mathrm{kWh}/{\mathrm{m}}^2-\mathrm{year}$	Benchmark evaluation of wholesale food that include food market, gas station with a corner store, and corner store [17]
Minnesota Department of Commerce	$1,173.3 \mathrm{kWh}/{\mathrm{m}}^2-\mathrm{year}$	Benchmark estimation for chain ownership [16]
Minnesota Department of Commerce	$764.2 \mathrm{kWh}/{\mathrm{m}}^2-\mathrm{year}$	Benchmark assessment for local owners [16]
Benchmarks for Sustainable Retail Stores	346 to 700$\mathrm{kWh}/{\mathrm{m}}^2-\mathrm{year}$	Retail Food [18]

below. Energy intensities in the range of 346 kWh/m²-year for what has been termed a sustainable corner markets to as high as 1172.3 kWh/m²-year have been documented. Most interesting are the findings from the Minnesota Department of Commerce [16]. Their comprehensive study of corner markets in Minnesota revealed a significant difference in energy intensity for chain owned (1173.3 kWh/m²-year) versus local owned (764.2 kWh/m²-year). The benchmark energy intensity associated with typical corner stores are shown in Table 1.

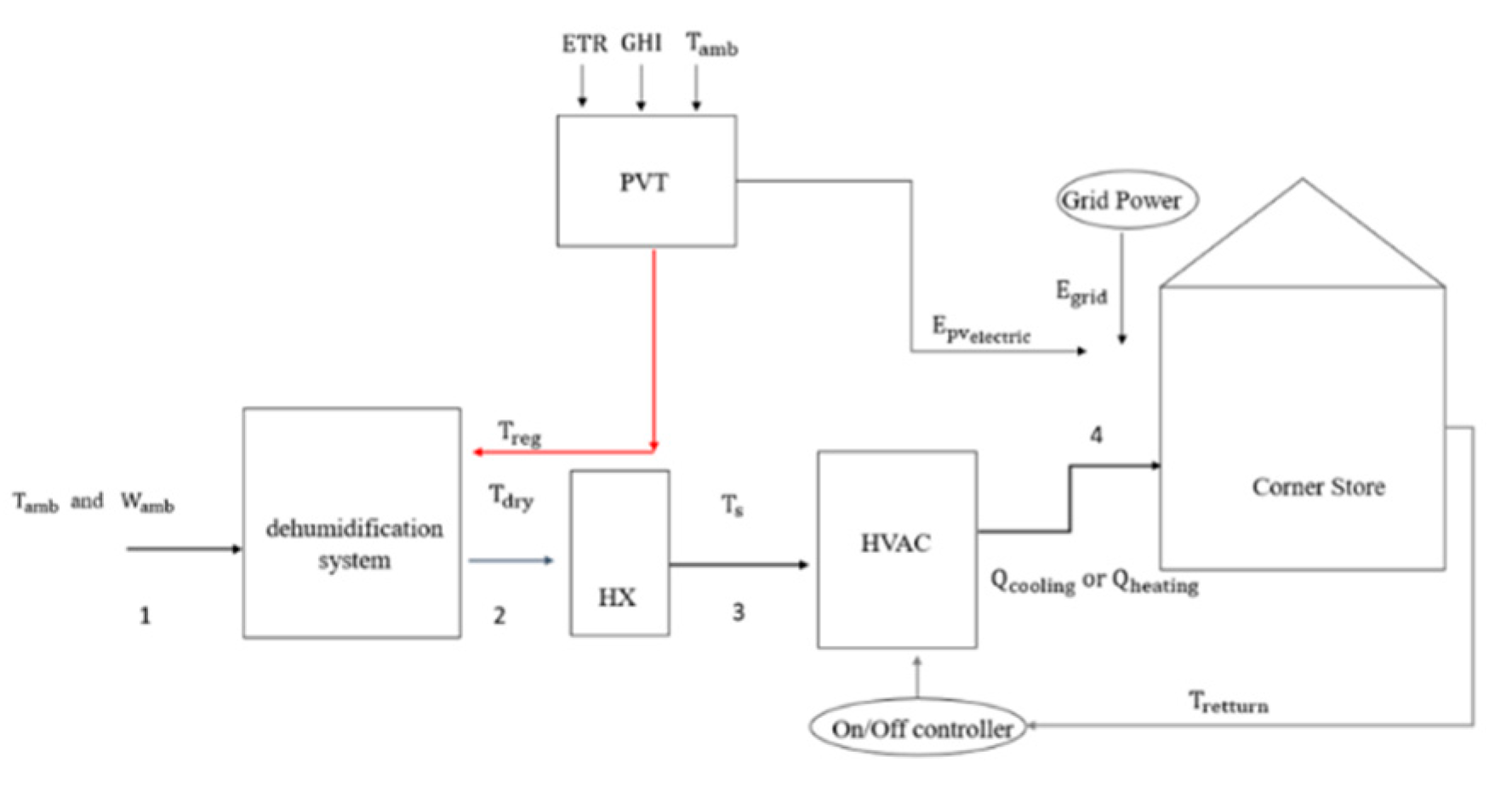
This research uniquely poses use of a solar dehumidification system integrated into a corner store heating, ventilation, and air conditioning (HVAC) systems. Several studies have considered use of these systems in commercial and residential buildings. However, no known study has addressed the problem of high energy consumption in corner markets. This study seeks to demonstrate the economic feasibility of this technology in three different cities in the United States. These cities (Dayton, OH, Pine Bluff, AR, and Phoenix, AZ) are representative of cooling dominated/relatively hot and humid summers; cooling dominated with extreme summer heat and humidity; and cooling dominated with extreme summer heat but moderate humidity.

The following describes the structure for this paper. First, in Section 2 the model section describes the models required to estimate annual energy consumption in typical corner stores and in corner stores reliant about the posed solar dehumidification system. Included in both cases are models for estimating heat loss/gain to/from the external environment, refrigeration case models, and HVAC system models. The posed system also includes models for the solar dehumidification system. Section 3 shows the predictions for the baseline and posed configurations for a typical corner store located in the three cities studied. The prediction results aim to elucidate the annual energy and cost savings. Section 4 shows the economic analysis used to estimate payback from the capital investment of the posed solar dehumidification system. Section 5 discusses the significance of the findings and the future work needed to validate results. Lastly, Section 6 offers summary conclusions.

2. Model

The model development is organized as follows. First, a model of a typical corner market is established, relying upon geometrical and energy characteristics typical of such stores. Second, the baseline model elements are developed to permit estimation of the energy performance of the defined typical store. These elements include modeling of the market building loads related to heating and cooling, the HVAC system, and the refrigeration systems. Lastly, a detailed model of the posed system is provided. This model augments the baseline model with solar dehumidification system. The dehumidification system is considered to evaluate its impact on the HVAC and refrigeration energy.

2.1. Typical Corner Store Model: Baseline Case

A baseline model is developed in this paper for two reason. One, it serves to help validate the models developed herein. The estimated energy intensity for this baseline system should reflect the benchmark energy intensities documented in Table 1. The energy use predicted from this baseline model also serves as a reference for energy consumption for the proposed system. Thus, the energy savings of the proposed system can be estimated through a comparison with the baseline model.

The establishment of this baseline model relies upon various sources to define the typical corner store geometrical, and energy (envelope, refrigeration systems, and HVAC systems) characteristics.

Table 2. Geometrical and energy characteristics for baseline typical corner store model.

Characteristics	Base Case (Parameters)	Source
Plan shape	Rectangular	Eric Richman, building energy code 1999–2007 [19]
Number of floors	1	Assumption
Floor height	6.10 m	Assumption
Floor area	330 ${\mathrm{m}}^2$	Advances in building energy research [20]
Floor dimensions	24 m × 14 m	Assumption
Window area	7% of the total gross wall area	[21]
Overall heat transfer coefficient for windows ($U_{win})$	2.84 $\mathrm{W}/{\mathrm{m}}^2.\mathrm{K}$	The American Society of Heating, Refrigerating and Air-Conditioning (ASHRAE) 2007 [22]
Solar heat gain coefficient (SHGC)	0.4
Lighting power density	15 W/${\mathrm{m}}^2$	ASHRAE Releases 90.1-2010–Part 1: Design, scope, administrative requirements [23]
Ventilation	0.20${ \mathrm{m}}^3/\mathrm{s}-{\mathrm{m}}^2$	ASHRAE 2007 [22]
Weather file	Dayton TMY3	National Solar Radiation Database (NSRDB) [24]
Thermostat	ON/Off	Assumption
Solar absorbancfor exterior surfaces	0.55	ASHRAE [25]
Overall heat transfer coefficient for exterior walls ($U_{walls}$)	0.78 $\mathrm{W}/{\mathrm{m}}^2.\mathrm{K}$	National Renewable Energy Laboratory (NREL) [26]
Overall heat transfer coefficient for roof ($U_{roof}$)	0.287 $\mathrm{W}/{\mathrm{m}}^2.\mathrm{K}$	[21]
Thermostat setting	T = $24 ℃$ and RH = 55%	Ref [7]
HVAC unit size	32 ${\mathrm{m}}^2$ of floor area per ton	HVAC refresher—facilities standard for the building Services [27]
Operating hours	6 AM to 10 PM	[28]
Peak electric plug load	5.4 W/${\mathrm{m}}^2$	NREL [26]
Occupancy	150 W/person	NREL [26]
Air conditioning system coefficient of performance (COP)	3.33	ASHRAE 2007 [22]
Moisture generation rate inside store	0.70 kg of water /kg of air per hour	Assumption based on [29]

summarizes these characteristics.

2.2. Baseline Model

In this section, models for the baseline corner store are developed to include models to permit prediction of the building heat gains/losses with the external environment and the associated heating/cooling requirements, and estimation of the energy consumption from typical refrigeration cases.

2.2.1. HVAC Model

Modeling the dynamic behavior of a typical corner store is necessary to estimate cooling and heating loads. This is done here by calculating the heat gain or lost through the store envelope, along consideration of with internal heat gains from occupants, lighting, and appliances.

Some assumptions have been made to simplify the problem. It is presumed that the store temperature is uniform so that the method of lumped capacitance can be implemented. In this approach, the dynamic temperature inside the store can be calculated as Equation (1):

$$\begin{array}{l}\mathrm{V} \ast {\mathsf{\rho }}_{\mathrm{a}} \ast {\mathrm{C}}_{\mathrm{pa}}\frac{{\mathrm{dT}}_{\mathrm{store}}}{\mathrm{dt}}\\ ={\dot{\mathrm{Q}}}_{{\mathrm{conduction}}_{\mathrm{wall}}} + {\dot{\mathrm{Q}}}_{{\mathrm{convection}}_{\mathrm{wall}}} + {\dot{\mathrm{Q}}}_{{\mathrm{conduction}}_{\mathrm{windows}}}\\ +{\dot{\mathrm{Q}}}_{{\mathrm{convection}}_{\mathrm{windows}}} + {\dot{\mathrm{Q}}}_{{\mathrm{conduction}}_{\mathrm{roof}}} + {\dot{\mathrm{Q}}}_{{\mathrm{convection}}_{\mathrm{roof}}} + {\dot{\mathrm{Q}}}_{\mathrm{lighting}}\\ +{\dot{\mathrm{Q}}}_{\mathrm{ocuppancy}} + {\dot{\mathrm{Q}}}_{\mathrm{equipment}} + {\dot{\mathrm{Q}}}_{\mathrm{inflitration}} - {\dot{\mathrm{Q}}}_{\mathrm{cooling}}\end{array}$$

(1)

For this equation, the rate of heat gain to the store through the various envelope components can be expressed as:

$${\dot{\mathrm{Q}}}_{\mathrm{x}}=\frac{\left({\mathrm{T}}_{\mathrm{ambient}}-{\mathrm{T}}_{\mathrm{store}}\right)}{{\mathrm{R}}_{\mathrm{x}}}, \mathrm{x} =\left[\mathrm{wall}, \mathrm{windows}, \mathrm{roof}, \mathrm{infiltration}\right]$$

where

$$\begin{gathered} {\dot{\mathrm{Q}}}_{\mathrm{x}}=\mathrm{the} \mathrm{rate} \mathrm{of} \mathrm{heat} \mathrm{gain}/\mathrm{loss} \mathrm{to}/ \\ \mathrm{from} \mathrm{the} \mathrm{store} \mathrm{and} \mathrm{x} \mathrm{is} \mathrm{associated} \mathrm{with} \mathrm{the} \mathrm{type} \mathrm{of} \mathrm{heat} \mathrm{transfer}; \\ \mathrm{i}.\mathrm{e}., \mathrm{conduction} \mathrm{or} \mathrm{convection} \mathrm{kW}. \\ {\mathrm{R}}_{\mathrm{x}}={\mathrm{thermal} \mathrm{resistance} \mathrm{of} \mathrm{an} \mathrm{envelope} \mathrm{feature} \mathrm{m}}^2.\mathrm{K}/\mathrm{kW} \end{gathered}$$

Additionally, the following defines the other terms used in Equation (1).

$$\begin{gathered} {\mathrm{V}}_{\mathrm{a}}={\mathrm{volume} \mathrm{flow} \mathrm{rate} \mathrm{m}}^3 \\ {\mathsf{\rho }}_{\mathrm{a}}=\mathrm{air} \mathrm{density}\frac{{\mathrm{m}}^3}{\mathrm{kg}} \\ {\mathrm{c}}_{\mathrm{pa}}=\mathrm{specific} \mathrm{heat} \mathrm{at} \mathrm{constant} \mathrm{pressure} \mathrm{of} \mathrm{air} \mathrm{kJ}/\mathrm{kg}-\mathrm{K} \\ \mathrm{t}= \mathrm{time}, \mathrm{s} \end{gathered}$$

A water vapor mass balance inside the store is calculated by considering ventilation, infiltration, and internal moisture generation from humans and cooking. The humidity ratio of the air inside the store can be calculated as Equation (2) [30]:

$${\mathsf{\rho }}_{\mathrm{a}}{\mathrm{V}}_{\mathrm{a}}\frac{{\mathrm{dw}}_{\mathrm{a}}}{\mathrm{dt}}=\left({\dot{\mathrm{m}}}_{\inf }+{\dot{\mathrm{m}}}_{\mathrm{vent}}\right)\left({\mathrm{w}}_{\mathrm{ex}}-{\mathrm{w}}_{\mathrm{a}}\right)+\mathrm{M}$$

(2)

where

$$\begin{gathered} {\mathrm{W}}_{\mathrm{a}}=\mathrm{moisture} \mathrm{content} \mathrm{inside} \mathrm{store}\frac{{\mathrm{kg}}_{\mathrm{v}}}{{\mathrm{kg}}_{\mathrm{a} }\mathrm{hr}} \\ {\dot{\mathrm{m}}}_{\inf }=\mathrm{inflitration} \mathrm{rate} \frac{\mathrm{kg}}{\mathrm{hr}} \\ {\dot{\mathrm{m}}}_{\mathrm{vent}}=\mathrm{ventilation} \mathrm{rate} \frac{\mathrm{kg}}{\mathrm{hr}} \\ {\mathrm{w}}_{\mathrm{ex}}=\mathrm{outdoor} \mathrm{mositure}\frac{{\mathrm{kg}}_{\mathrm{v}}}{{\mathrm{kg}}_{\mathrm{a} }\mathrm{hr} } \\ \mathrm{M}=\mathrm{moisture} \mathrm{generation} \mathrm{inside} \mathrm{store}\frac{\mathrm{kg}}{\mathrm{hr}} \end{gathered}$$

The heat gain through building envelope, along with the internal heat generation must be dissipated to maintain the comfort environment during the cooling season. To maintain internal comfort, the HVAC system must account for both thermal heat gain and humidity control according to Equation (3):

$${\dot{\mathrm{Q}}}_{\mathrm{cooling}}={\dot{\mathrm{m}} \ast \mathrm{C}}_{\mathrm{pa}}\ast \left({\mathrm{T}}_{\mathrm{out}}-{\mathrm{T}}_{\mathrm{store}}\right)+{\dot{\mathrm{m}} \ast \mathrm{h}}_{\mathrm{fg}}\ast \left({\mathrm{W}}_{\mathrm{ex}}-{\mathrm{W}}_{\mathrm{in}}\right)$$

(3)

where

$$\begin{gathered} {\dot{\mathrm{Q}}}_{\mathrm{cooling}}= \mathrm{cooling} \mathrm{load} \mathrm{kW} \\ \dot{\mathrm{m}}= \mathrm{mass} \mathrm{flow} \mathrm{rate} \mathrm{of} \mathrm{the} \mathrm{sum} \mathrm{of} \mathrm{infiltration} \mathrm{and} \mathrm{ventilation} \mathrm{flows} \mathrm{kg}/\mathrm{hr} \\ { \mathrm{C}}_{\mathrm{pa}}= \mathrm{specific} \mathrm{heat} \mathrm{kJ}/\mathrm{kg}-\mathrm{K} \\ { \mathrm{h}}_{\mathrm{fg}}= \mathrm{latent} \mathrm{heat} \mathrm{of} \mathrm{water} \mathrm{kJ}/\mathrm{kg} \\ {\mathrm{W}}_{\mathrm{a}}=\mathrm{moisture} \mathrm{content} \mathrm{inside} \mathrm{store}\frac{{\mathrm{kg}}_{\mathrm{v}}}{{\mathrm{kg}}_{\mathrm{a} }} \\ {\mathrm{w}}_{\mathrm{ex}}=\mathrm{outdoor} \mathrm{mositure}\frac{{\mathrm{kg}}_{\mathrm{v}}}{{\mathrm{kg}}_{\mathrm{a} }} \end{gathered}$$

An on/off controller is considered for controlling the internal temperature (and humidity level) of the store. The temperature band for the thermostat is assumed to be ± 1 ℃. A typical relative humidity level inside the store is found to be 55% RH [7]. In the baseline model, the relative humidity is set to this value.

2.2.2. Baseline Refrigeration Display Case Model

Open Display Case Refrigeration

To predict the energy consumption of a refrigerated display case, the mathematical model developed by Ge and Tassou is used [13]. In this model, the major parameters that influence energy usage are the store dry-bulb temperature, the store enthalpy, and the relative humidity of the store. Bahman leveraged Ge and Tassou’s model to predict the heat and mass transfer of horizontal and vertical multi-shelf refrigerated display cases [31]. The following empirical equations (Equations (4) and (5)) are used to predict energy consumption for display case refrigerants [13].

$$\begin{gathered} {\dot{\mathrm{Q}}}_{\mathrm{ref}}=\left[{\mathrm{c}}_1{\mathrm{h}}_{\mathrm{space}}^2+{\mathrm{c}}_2{\mathrm{h}}_{\mathrm{space}}+{\mathrm{c}}_3{\left({\mathrm{T}}_{\mathrm{case}}+\Delta \mathrm{T}\right)}^2+{\mathrm{c}}_4\left({\mathrm{T}}_{\mathrm{case}}+\Delta \mathrm{T}\right)+{\mathrm{c}}_5 \\ {\ast \mathrm{h}}_{\mathrm{space}}\left({\mathrm{T}}_{\mathrm{case}}+\Delta \mathrm{T}\right)+{\mathrm{c}}_6\right]\dot{\mathrm{m}}/{ \mathrm{L}}^{{\mathrm{c}}_7} \end{gathered}$$

(4)

$${\mathrm{h}}_{\mathrm{space}}=1.0 \mathrm{Tspace}+{\mathrm{w}}_{\mathrm{space}}\left(2501.3+1.86{\mathrm{T}}_{\mathrm{space}}\right)$$

(5)

where

$$\begin{gathered} {\dot{\mathrm{Q}}}_{\mathrm{ref}}= \mathrm{load} \mathrm{on} \mathrm{the} \mathrm{cooling} \mathrm{coil} \mathrm{of} \mathrm{the} \mathrm{vertical} \mathrm{multi}-\mathrm{shelf} \mathrm{display} \mathrm{cabinet} \mathrm{kW} \\ {\mathrm{h}}_{\mathrm{space}}=\mathrm{enthalpy} \mathrm{inside} \mathrm{the} \mathrm{store}\mathrm{kJ}/\mathrm{kg} \\ {\mathrm{T}}_{\mathrm{case}}=\mathrm{temperature} \mathrm{of} \mathrm{the} \mathrm{display} \mathrm{case} \mathrm{refrigerant} \mathrm{K} \\ {\mathrm{T}}_{\mathrm{space}}=\mathrm{store} \mathrm{temperature} °\mathrm{C} \\ \Delta \mathrm{T}= \mathrm{temperature} \mathrm{difference} \mathrm{across} \mathrm{air} \mathrm{curtain} \mathrm{K} \\ {\mathrm{w}}_{\mathrm{space}}={\mathrm{humidity} \mathrm{ratio} \mathrm{in} \mathrm{the} \mathrm{store} \mathrm{kg}}_{\mathrm{v}}/{\mathrm{kg}}_{\mathrm{air}} \\ \mathrm{L} = \mathrm{length} \mathrm{of} \mathrm{the} \mathrm{display} \mathrm{cabinet} \mathrm{m} \end{gathered}$$

Values for the constants, ${\mathrm{c}}_1$ through ${\mathrm{c}}_7$, are shown in

Table 3. Coefficients used in Equation (4) [13].

${\mathbf{c}}_{\mathbf{1}}$	${\mathbf{c}}_{\mathbf{2}}$	${\mathbf{c}}_{\mathbf{3}}$	${\mathbf{c}}_{\mathbf{4}}$	${\mathbf{c}}_{\mathbf{5}}$	${\mathbf{c}}_{\mathbf{6}}$	${\mathbf{c}}_{\mathbf{7}}$
−0.18	303.18	−0.781	216.309	−0.448	509.975	0.252

.

Typically, there are three types of vertical multi-shelf display cabinets in a supermarket, i.e., multi-shelf dairy, multi-shelf deli, and multi-shelf frozen food [13]. The model developed by Ge and Tassou aims to satisfy the operational conditions of these cabinets. The model includes the possibility of utilizing air curtains in open displace cases. For this condition, the air curtain velocity for vertical refrigerated cabinets has been classified as ‘MIN’, ‘MIDMB’, ‘BASE ’, ‘MIDBM’, and ‘MAX’ in order to cover a wide range of operating conditions, and the values of these classifications have been reported [32].

Closed Display Case Refrigeration

A simulation model for the general display case developed by Howell is adopted in order to determine the evaporator load on the closed display case (Equations (6) and (7)) [32].

$${\dot{\mathrm{Q}}}_{\mathrm{case} }={\dot{\mathrm{Q}}}_{\mathrm{wall}}+{\dot{\mathrm{Q}}}_{\mathrm{sen}}+{\dot{\mathrm{Q}}}_{\mathrm{lat}}+{\dot{\mathrm{Q}}}_{\mathrm{lighting}}+{\dot{\mathrm{Q}}}_{\mathrm{ASW}}+{\dot{\mathrm{Q}}}_{\mathrm{def}}$$

(6)

where

$${\dot{\mathrm{Q}}}_{\mathrm{wall}}=\mathrm{UA}\left({\mathrm{T}}_{\mathrm{store}}-{\mathrm{T}}_{\mathrm{case}}\right)$$

(7)

$$\begin{gathered} {\dot{\mathrm{Q}}}_{\mathrm{wall}}= \mathrm{heat} \mathrm{transfer} \mathrm{through} \mathrm{case} \mathrm{walls} \mathrm{kW} \\ \mathrm{U} = \mathrm{equivalent} \mathrm{overall} \mathrm{heat} \mathrm{transfer} \mathrm{coefficient} \mathrm{for} \mathrm{the} \mathrm{case} \mathrm{walls} \mathrm{kW}/\left({\mathrm{m}}^2. ℃\right) \\ \mathrm{A}={\mathrm{case} \mathrm{wall} \mathrm{area} \mathrm{m}}^2 \\ {\dot{\mathrm{Q}}}_{\mathrm{sen}}= \mathrm{sensible} \mathrm{heat} \mathrm{transfer} \mathrm{to} \mathrm{the} \mathrm{case} \mathrm{due} \mathrm{to} \mathrm{the} \mathrm{air} \mathrm{curtain} \mathrm{across} \mathrm{the} \mathrm{door} \mathrm{opining}, \\ 1.1 \mathrm{CFM}\left(\mathrm{T}\_\mathrm{store}-\mathrm{T}\_\mathrm{case} \right), \mathrm{kW} \\ \mathrm{CFM} = \mathrm{air} \mathrm{exchange} \mathrm{due} \mathrm{to} \mathrm{door} \mathrm{openings} \mathrm{per} \mathrm{hour} \\ {\dot{\mathrm{Q}}}_{\mathrm{lat}}= \mathrm{latent} \mathrm{heat} \mathrm{transfer} \mathrm{to} \mathrm{the} \mathrm{case} \mathrm{due} \mathrm{to} \mathrm{the} \mathrm{air} \mathrm{curtain} \mathrm{across} \mathrm{the} \mathrm{door} \mathrm{opining}, \\ 4840 \mathrm{CFM}\left(\mathrm{W}\_\mathrm{store}-\mathrm{W}\_\mathrm{case} \right), \mathrm{kW} \\ {\dot{\mathrm{Q}}}_{\mathrm{ASW}}=\mathrm{anti}-\mathrm{sweat} \mathrm{heater} \mathrm{load} \mathrm{kW} \\ {\dot{\mathrm{Q}}}_{\mathrm{def}}=\mathrm{defrost} \mathrm{energy} \mathrm{load} \mathrm{kW} \\ {\mathrm{W}}_{\mathrm{store}}=\mathrm{humidity} \mathrm{ratio} \mathrm{of} \mathrm{the} \mathrm{store} \mathrm{air}\frac{{\mathrm{kg}}_{\mathrm{w}}}{{\mathrm{kg}}_{\mathrm{a}}} \\ {\mathrm{W}}_{\mathrm{case}}=\mathrm{humidity} \mathrm{ratio} \mathrm{of} \mathrm{the} \mathrm{case} \mathrm{air}\frac{{\mathrm{kg}}_{\mathrm{w}}}{{\mathrm{kg}}_{\mathrm{a}}} \end{gathered}$$

The characteristics of the standard display case cabinets considered in this study are shown in

Table 4. Characteristics of the baseline open refrigeration [31].

	Multi-Shelf Medium Temperature
Case Temperature $℃$	2.15 (35.87)
Air curtain velocity m/s	0.35
Air curtain thickness m	0.0351
Case length (m)	8
EER	7
Orientation	Vertical

and

Table 5. Characteristics of the baseline cooler [33].

	Walk-in Cooler
Floor size $\left(m^{\mathbf{2}}\right)$	22
Width $(m$)	3.04
Depth $\left(m\right)$	2.5
Height $\left(m\right)$	2.5
Wall thickness (m)	0.025
Wall R-value $W/m^{\mathbf{2}}.K$	5.04

. Two display cabinets have been considered in this study: vertical glass door reach in multi-shelf walk-in cooler and open vertical multi-shelf medium temperature. These are the most common display cases in corner stores.

2.3. Posed System: Integrated Solar Dehumidification System

A framework for integrating solar dehumidification system with HVAC for a typical corner store is established. A dynamic model for the ‘improved’ model is developed. A comparison of the energy consumption is made to the benchmark results in order to estimate the energy benefits of this lowest energy model. A description of the proposed system and mathematical modeling is presented in the next sections.

2.3.1. Lowest Energy Model Description

In this study, the impact of a solar dehumidification system integrated into the corner store HVAC system is theoretically investigated. This proposed system is illustrated in Figure 1. It contains five sub-systems and one control unit. These sub-systems are the photovoltaic/solar thermal (PVT), a desiccant wheel for conditioning make-up air, the HVAC system, a heat exchanger (HX) for pre-cooled supply air in summer season, and the corner store thermal model. The dehumidification system is considered to evaluate its impact on HVAC and refrigeration energy. Thus, the dehumidification system aims to control the humidity level inside the store to ensure that the case refrigeration systems use the least amount of energy possible. For each of these systems, a MATLAB Simulink dynamic model is developed individually and in an integrated mode.

Figure 1 shows the input and output variables for each sub-system. The outputs of the PVT are the electric energy $({\mathrm{E}}_{{\mathrm{PV}}_{\mathrm{electric}}})$ produced and the solar heated air temperature (${\mathrm{T}}_{\mathrm{reg}}$). The input variables are the hourly extraterrestrial radiation (ETR), global horizontal radiation (GHI), and the ambient temperature (${\mathrm{T}}_{\mathrm{amb}}$). The electric energy is dispatched to the store and the regenerative hot air stream is delivered to the dehumidification sub-system to remove moisture in the desiccant material. During operation, in cooling mode, outdoor air enters the desiccant wheel (stage 1). Some of the moisture in this outdoor air is absorbed in the desiccant within the wheel. Additionally, there is heat added. The existing air from the heat exchanger (stage 3) may experience additional cooling in the HVAC system. An on/off controller for controlling the HVAC system is considered. The controller measures the temperature of the store and sends signals to the HVAC unit when the temperature of the store exceeds the targeted setpoint temperature plus the setpoint temperature spread (consider to be 1 °C).

2.3.2. Refrigeration Display Case Cabinets for Lowest Energy Model

The case refrigeration systems model is constructed to evaluate the impact of store humidity on the performance of the lowest energy display case cabinets. For reasonable comparison, the refrigeration system model for the lowest energy configuration mirrors that employed for the baseline case. Thus, the impact of lowering store relative humidity can be evaluated and the annual energy saving of the display case cabinets can be determined. In addition, another approach has been adopted to evaluate the influence of indoor relative humidity on the performance of vertical and horizontal display case. Herewith, the influence of indoor relative humidity on the refrigeration load is evaluated based on a correction factor (TP). The correction factor is defined as the energy required by the display case cabinets when operating at relative humidity other than 55% (Equations (8) and (9)) [32].

$$\mathrm{TP}={\mathrm{Q}}_{\mathrm{RH}}/{\mathrm{Q}}_{\mathrm{R}}$$

(8)

$$\mathrm{TP}=1-\left(55-\mathrm{RH}\right)\left(\mathrm{D}+\mathrm{E}\ast \mathrm{TC}+{\mathrm{F}\ast \mathrm{TC}}^2+{\mathrm{G}\ast \mathrm{TC}}^3\right)$$

(9)

where

TP = correction factor

$$\begin{gathered} {\mathrm{Q}}_{\mathrm{R}}=\mathrm{display} \mathrm{case} \mathrm{energy} \mathrm{requirement} \mathrm{at} \mathrm{the} \mathrm{design} \mathrm{value} \mathrm{of} \mathrm{relative} \mathrm{humidity} \mathrm{of} 55\% \mathrm{kW} \\ {\mathrm{Q}}_{\mathrm{RH}}=\mathrm{display} \mathrm{case} \mathrm{energy} \mathrm{requirement} \mathrm{at} \mathrm{given} \mathrm{relative} \mathrm{humidity} \mathrm{kW} \end{gathered}$$

RH = relative humidity %

$$\mathrm{TC}=\mathrm{temperature} \mathrm{of} \mathrm{the} \mathrm{display} \mathrm{case} \mathrm{cabinet} ℃$$

The coefficients D, E, F, and G have been defined previously [32] and tabulated in

Table 6. Coefficients used in Equation (9) [32].

Coefficients	Vertical Display Cases	Horizontal Display Cases
D	7.38 × 10−3	6.57 × 10−3
E	6.51 × 10−5	4.88 × 10−5
F	−4.61 × 10−7	5.35 × 10−7
G	7.24 × 10−8	3.73 × 10−9

.

2.3.3. Desiccant Dehumidification System

The main goal of the desiccant dehumidification system is to absorb moisture from the supply air, leveraging the regenerative solar heated air. Driven by the vapor pressure difference between process air and desiccant material, water vapor is absorbed, and air leaves the desiccant at a lower humidity.

The following governing equations are taken from [34], which describes the heat and mass transfer in the dehumidification system.

• Conservation of water mass in the air stream

Equation (10) shows the water mass conservation for the supply air stream

$${\mathrm{d}}_{\mathrm{e}}{ \mathsf{\rho }}_{\mathrm{a}} \left(\frac{\partial {\mathrm{Y}}_{\mathrm{a}}}{\partial \mathrm{t}}+\mathrm{u} \frac{\partial {\mathrm{Y}}_{\mathrm{a}}}{\partial \mathrm{z}}\right)={\mathrm{K}}_{\mathrm{y}} \left({\mathrm{Y}}_{\mathrm{d}}-{\mathrm{Y}}_{\mathrm{a}}\right)$$

(10)

The first and second terms on the left-hand side (LHS) of Equation (10) represent the moisture storage and the rate of moisture variation in the air, respectively. The right-hand side (RHS) of the above equation expresses the impact of the convective mass transfer in the desiccant wheel.

• Energy conservation for the air stream

Conservation of energy applied to the air stream is given by Equation (11) below.

$${\mathrm{d}}_{\mathrm{e}}{ \mathsf{\rho }}_{\mathrm{a}}{ \mathrm{c}}_{\mathrm{pa}}\left(\frac{\partial {\mathrm{T}}_{\mathrm{a}}}{\partial \mathrm{t}}+\mathrm{u} \frac{\partial {\mathrm{T}}_{\mathrm{a}}}{\partial \mathrm{z}}-\frac{{\mathrm{K}}_{\mathrm{a}}}{\mathrm{cpa} \mathsf{\rho }\mathrm{a}} \frac{{\partial }^2{\mathrm{T}}_{\mathrm{a}}}{\partial {\mathrm{z}}^2}\right)=\mathrm{h}\left({\mathrm{T}}_{\mathrm{d}}-{\mathrm{T}}_{\mathrm{a}}\right)+{ \mathrm{c}}_{\mathrm{pv}}{\mathrm{K}}_{\mathrm{y}} \left({\mathrm{Y}}_{\mathrm{d}}-{\mathrm{Y}}_{\mathrm{a}}\right)\left({\mathrm{T}}_{\mathrm{d}}-{\mathrm{T}}_{\mathrm{a}}\right)$$

(11)

The first and second terms on the LHS of Equation (11) express the energy storage in the humid air and the rate of advection of energy. The third term expresses heat conduction in the air. On the RHS, the first term indicates convective heat transfer between air and desiccant, while the second term represents the sensible heat transfer between air and desiccant.

• Water mass conservation in the desiccant

Equation (12) shows the water mass conservation in the desiccant wheel

$${\mathsf{\delta }\mathrm{d} \mathsf{\rho }}_{\mathrm{d}}\left(\frac{\partial \mathrm{W}}{\partial \mathrm{t}}-\mathrm{De} \frac{{\partial }^2\mathrm{W}}{\partial {\mathrm{z}}^2}\right)={\mathrm{K}}_{\mathrm{y}} \left({\mathrm{Y}}_{\mathrm{d}}-{\mathrm{Y}}_{\mathrm{a}}\right)$$

(12)

The first and second terms on the LHS of this equation represent the moisture storage in the desiccant and mass diffusion within the desiccant. The RHS of Equation (12) represents convective mass transfer between the regenerative and supply air within the desiccant.

• Energy conservation in the desiccant

Conservation of energy applied to the regenerative air stream is given by Equation (13) below

$${\mathsf{\delta }\mathrm{d} \mathsf{\rho }}_{\mathrm{d}}{ \mathrm{c}}_{\mathrm{pd}}\left(\frac{\partial {\mathrm{T}}_{\mathrm{d}}}{\partial \mathrm{t}}-\frac{{\mathrm{K}}_{\mathrm{d}}}{{\mathrm{c}}_{\mathrm{pd}}{ \mathsf{\rho }}_{\mathrm{d}}} \frac{{\partial }^2{\mathrm{T}}_{\mathrm{d}}}{\partial {\mathrm{z}}^2}\right)=\mathrm{h}\left({\mathrm{T}}_{\mathrm{a}}-{\mathrm{T}}_{\mathrm{d}}\right)+{\mathrm{K}}_{\mathrm{y}}\left({\mathrm{Y}}_{\mathrm{a}}-{\mathrm{Y}}_{\mathrm{d}}\right){\mathrm{q}}_{\mathrm{st}}+{ \mathrm{c}}_{\mathrm{pv}}{\mathrm{K}}_{\mathrm{y}} \left({\mathrm{Y}}_{\mathrm{d}}-{\mathrm{Y}}_{\mathrm{a}}\right)\left({\mathrm{T}}_{\mathrm{d}}-{\mathrm{T}}_{\mathrm{a}}\right)$$

(13)

The first and second terms in LHS of Equation (13) are the energy storage of desiccant and the heat transfer due to heat conduction within desiccant. The first term in the RHS expresses convective heat transfer between solid desiccant and air while the second term represents adsorption heat transfer. The last term expresses the sensible heat transfer between desiccant and air.

Auxiliary Equations (14)–(21) are needed to find the thermodynamic properties of the dehumidification system [34]

$${\mathrm{P}}_{\mathrm{vs}}=\exp (23.196-\frac{3816.44}{{\mathrm{T}}_{\mathrm{d}}-46.13})$$

(14)

$${\mathrm{q}}_{\mathrm{st}}={\mathrm{h}}_{\mathrm{fg}}\left[1.0+0.23843\exp \left(-10.28\mathrm{W}\right)\right]$$

(15)

$${\mathrm{RH}}_{\mathrm{sg}}=0.0078-0.05759\mathrm{W}+24.16553{\mathrm{W}}^2-124.478{\mathrm{W}}^3+204.226{\mathrm{W}}^4$$

(16)

$$\mathrm{h}=\frac{\mathrm{NuKP}}{4\mathrm{A}}$$

(17)

$$\mathrm{Nu} =1.1791\left[1+2.7701\ast \mathsf{\alpha }-3.1901{\ast \mathsf{\alpha }}^2+1.9975{\ast \mathsf{\alpha }}^3-0.4966{\ast \mathsf{\alpha }}^4\right]$$

(18)

$$\mathsf{\alpha }=\frac{\mathrm{a}}{\mathrm{b}}$$

(19)

$$\mathrm{Ky}=\frac{{\mathsf{\rho }}_{\mathrm{A}}{\mathrm{ShD}}_{\mathrm{A}}\mathrm{P}}{4\mathrm{A}}$$

(20)

$${\mathrm{D}}_{\mathrm{A}}=2.302\ast \frac{{10}^{-5}{\mathrm{P}}_0}{{\mathrm{P}}_{\mathrm{a}}}$$

(21)

where

$$\begin{gathered} {\mathrm{P}}_{\mathrm{vs}}=\mathrm{pressure} \mathrm{of} \mathrm{saturated} \mathrm{water} \mathrm{vapor} \mathrm{Pa} \\ {\mathrm{q}}_{\mathrm{st}}= \mathrm{heat} \mathrm{of} \mathrm{sorption} \mathrm{J}/\mathrm{kg} \\ {\mathrm{c}}_{\mathrm{pd}}=\mathrm{specific} \mathrm{heat} \mathrm{of} \mathrm{the} \mathrm{desiccant} \mathrm{J}/\mathrm{kg}.\mathrm{K} \\ {\mathrm{RH}}_{\mathrm{sg}}=\mathrm{relative} \mathrm{humidity} \% \\ \mathrm{h}= \mathrm{air}-\mathrm{side} \mathrm{convective} \mathrm{heat} \mathrm{transfer} \mathrm{coefficient}\mathrm{W}/{\mathrm{m}}^2\mathrm{K} \\ \mathrm{Ky}=\mathrm{mass} \mathrm{transfer} \mathrm{coefficient} \\ \mathrm{Do}=\mathrm{ordinary} \mathrm{diffusivity} {\mathrm{m}}^2/\mathrm{s} \\ \mathrm{a}=\mathrm{hight} \mathrm{of} \mathrm{the} \mathrm{flow} \mathrm{passage} \mathrm{m} \\ \mathrm{b}=\mathrm{width} \mathrm{of} \mathrm{the} \mathrm{flow} \mathrm{passage} \mathrm{m} \end{gathered}$$

Table 7. Geometrical and thermodynamic properties and key inputs of the desiccant wheels used in the simulation model [35].

Height of the Flow Passage a (cm)	0.002
Area of cross-section, A (m2)	4.90 × 10−6
Perimeter of air flow passage, C (m)	7.10 × 10−3
Thickness of wheel, L (m)	0.1
$\mathrm{Specific} \mathrm{heat} \mathrm{of} \mathrm{LiCl} c_{ph}$ (J/kg K)	3000
Thermal conduction of LiCl, (W/m K)	0.65
Density of LiCl, (kg/m3)	1200
Width of the flow passage, b (m)	0.002
$\mathrm{Thickness} \mathrm{of} \mathrm{the} \mathrm{wall}, \mathsf{\delta }d$ (m)	0.00045

shows the geometrical characteristics and thermodynamics properties of the desiccant wheels.

2.3.4. Photovoltaic Thermal Hybrid (PVT) Solar Collectors

Calculating the solar radiation incident to an inclined surface is necessary for evaluating the PVT performance. The solar radiation landing on the inclined surface of the PVT consists of three components; direct, beam, and diffuse radiation. The mathematical equations describing these components are taken from [36]. The hybrid photovoltaic thermal (PVT) system is capable of producing electrical and thermal energy simultaneously leading to a higher overall efficiency. PVT overcomes the drawback that is associated with low conversion efficiency in PV panels.

The layout for the PVT sub-system is presented in Figure 2. The system is composed of the PVT modules and a thermal storage tank. The hourly extraterrestrial radiation (ETR) and global horizontal radiation (GHI) are the main inputs to the solar field (PVT), while the output is the thermal and electric energy produced within the PVT.

The thermal energy of the system will be used to cover part of the thermal load in the dehumidification system to remove moisture in the desiccant material. The mathematical model for the PVT and storage tank is adopted from prior research (Equations (22)–(25)) [37].

The total thermal and electric energy are given by the following equations.

$${\dot{\mathrm{Q}}}_{\mathrm{thermal}}\left(\mathrm{t}\right)={ \mathsf{\eta }}_{\mathrm{th}}{ \mathrm{A}}_{\mathrm{pvt}}\ast \mathrm{I}\left(\mathrm{t}\right)$$

(22)

$${\dot{\mathrm{Q}}}_{\mathrm{electric}}\left(\mathrm{t}\right)={ \mathsf{\eta }}_{\mathrm{el}}{ \mathrm{A}}_{\mathrm{pvt}}\ast \mathrm{I}\left(\mathrm{t}\right)$$

(23)

$${\mathsf{\eta }}_{\mathrm{el}} \left(\mathrm{t}\right)={ \mathsf{\eta }}_{\mathrm{ref}}\left(1-{ \mathrm{B}}_{\mathrm{ref}} \left({\mathrm{T}}_{\mathrm{c} \mathrm{PVT} \left(\mathrm{t}\right)}-{ \mathrm{T}}_{\mathrm{ref}} \right)\right)$$

(24)

$${\mathsf{\eta }}_{\mathrm{th}}={ \mathsf{\eta }}_0-{ \mathrm{a}}_1\left({\mathrm{T}}_{\mathrm{in}}-{\mathrm{T}}_{\mathrm{amb}}\right)/\mathrm{I}\left(\mathrm{t}\right)$$

(25)

where

$$\begin{gathered} {\mathrm{T}}_{\mathrm{c} \mathrm{PVT} \left(\mathrm{t}\right)}= \mathrm{photovoltaic} \mathrm{temperature} \mathrm{K} \\ {\mathrm{T}}_{\mathrm{ref}}= \mathrm{reference} \mathrm{temperature}, 25 ℃ \\ \mathrm{I}\left(\mathrm{t}\right)=\mathrm{solar} \mathrm{radiation} \mathrm{W} \\ {\mathrm{T}}_{\mathrm{amb}}=\mathrm{ambient} \mathrm{temperature} ℃ \\ {\mathrm{T}}_{\mathrm{in}}=\mathrm{fluid} \mathrm{temperature} \mathrm{to} \mathrm{the} \mathrm{PVT} ℃ \end{gathered}$$

A global energy balance on the thermal storage tank yields the following given equation (Equation (26)). This equation will be used to determine the tank temperature.

$${\mathrm{c}}_{\mathrm{pw}}{\ast \mathrm{V}\ast \mathsf{\rho }}_{\mathrm{w}}\frac{{\mathrm{dT}}_{\tan \mathrm{k}}}{\mathrm{dt}}={\dot{\mathrm{Q}}}_{\mathrm{thermal}}\left(\mathrm{t}\right)+{\dot{\mathrm{Q}}}_{\mathrm{aux}}\left(\mathrm{t}\right)-{\dot{\mathrm{Q}}}_{\mathrm{losses}}\left(\mathrm{t}\right)$$

(26)

To ensure effective operation of the desiccant wheel, auxiliary heat from an electric resistance heater powered by the PV system within the PVT is required to maintain the temperature of the tank above a specific set point. The heat loss from the storage tank to the environment is calculated as Equation (27):

$${\dot{\mathrm{Q}}}_{\mathrm{losses}}=\mathrm{UA}\left({\mathrm{T}}_{\tan \mathrm{k}}-{\mathrm{T}}_{\mathrm{amb}}\right)$$

(27)

where

$$\begin{gathered} \mathrm{U} = \mathrm{overall} \mathrm{loss} \mathrm{coefficient} \mathrm{W}/\left({\mathrm{m}}^2. ℃\right) \\ \mathrm{A} ={ \tan \mathrm{k} \mathrm{surface} \mathrm{area} \mathrm{m}}^2 \\ \mathrm{V}={\mathrm{storage} \tan \mathrm{k} \mathrm{volume} \mathrm{m}}^3 \\ {\dot{\mathrm{Q}}}_{\mathrm{thermal}}=\mathrm{thermal} \mathrm{energy} \mathrm{produced} \mathrm{by} \mathrm{PVT} \mathrm{kW} \\ {\dot{\mathrm{Q}}}_{\mathrm{losses}}=\mathrm{thermal} \mathrm{energy} \mathrm{loses} \mathrm{from} \mathrm{the} \tan \mathrm{k} \mathrm{to} \mathrm{the} \mathrm{envirnment} \mathrm{kW} \\ {\mathrm{T}}_{\tan \mathrm{k}}=\tan \mathrm{k} \mathrm{temperature} ℃ \\ {\mathrm{c}}_{\mathrm{pw}}=\mathrm{specific} \mathrm{heat} \mathrm{of} \mathrm{water} \mathrm{kJ}/\left(\mathrm{kg}.\mathrm{K}\right) \\ {\mathsf{\rho }}_{\mathrm{w}}=\mathrm{water} \mathrm{density} \mathrm{kg}/{\mathrm{m}}^3 \end{gathered}$$

An on/off controller is employed to maintain the tank at specify set point temperature.

Table 8. PVT System parameters [37].

No	Symbol	Description	Value
1	${\mathsf{\eta }}_{\mathrm{ref}}$	Electrical efficiency at temperature ${\mathrm{T}}_{\mathrm{ref}}$(-)	0.144
2	${\mathsf{\beta }}_{\mathrm{ref}}$	Efficiency correction coefficient for temperature (1/K)	0.005
3	$\mathrm{P}$	Electric power of one PVT module (W)	200
4	${\mathrm{A}}_{\mathrm{PVT}}$	Area of one PVT module (${\mathrm{m}}^2$)	1.305
5	${\mathsf{\eta }}_0$	Optical efficiency of thermal part in PVT	0.715
6	${\mathrm{a}}_1$	Heat loss coefficient (w/${\mathrm{m}}^2$ k)	7.98
7	$\dot{\mathrm{Q}}\mathrm{aux}$	Auxiliary heating (kW)	4.5
8	$\mathrm{N}$	Number of PVT modules (-)	8
9	${\mathsf{\eta }}_{\mathrm{th}}$	Thermal efficiency of the PVT	Range from 75% to 80%
10	${\mathsf{\eta }}_{\mathrm{el}}$	Electric efficiency of the PVT	Range from 15% to 22%

lists the main parameters for the analyzed PVT sub-system.

3. Result

3.1. Baseline Results

In order to evaluate the potential value of the proposed system for energy savings and improved income to corner markets, an estimation of the hourly power demand is made for a typical corner store for a typical weather year. Equations (1)–(7) were implemented in MATLAB Simulink. Figure 3 shows results for three cities located in different US cities representing three different climates: one cold dominated (Dayton, OH, USA); one hot and dry (Phoenix, AZ, USA); and the other hot and humid (Pine Bluff, AR, USA). It is clear from this figure that during the summer season, i.e., from June to August, the power demand for the city of Pine Bluff is larger due to a higher ambient temperature and humidity, while that of Dayton is lowest because of the more moderate temperatures there. The simulation results show also that the daily power demand requirements in Dayton in January is respectively 14% and 8% higher than for Phoenix and Pine Bluff. In the months of March and November, the average daily power demand for Dayton is stable; 95% of the power demand in these months is driven by the baseline loads from refrigeration, lighting, and plug loads.

The hourly simulated results for all months have been averaged separately and are illustrated in Figure 4. The annual energy consumption is 224,350 kWh/year, 217,810 kWh/year, and 229,230 kWh/year in respectively Dayton OH, Phoenix AZ, and Pine Bluff AR. The energy intensities are respectively 679 kWh/${\mathrm{m}}^2$-year, 660 kWh/${\mathrm{m}}^2$- year., and 694 kWh/${\mathrm{m}}^2$- year. The baseline energy consumption inside the store which represents more than 80% of the total energy consumption is independent of the outdoor conditions. This baseline energy consumption is the same for all cities. Thus, the difference between the annual energy consumption among these cities is only due to the outdoor climate conditions, mainly due to HVAC energy requirements. These results are well agreed with the benchmark illustrated in Table 1.

3.2. Lowest Energy Corner Market Results

Since the proposed system effectively integrates models for the PVT system, desiccant dehumidification system, refrigeration cases, HVAC system, and the corner market thermal loads, a detailed description of an integrated solution approach is provided. The following sub-sections describe this approach.

3.2.1. Dehumidification System

A numerical scheme is adopted to solve the coupled equations given by Equations (10)–(13) discretized into the finite difference in which the governing equations are replaced with a set of algebraic equations. Auxiliary equations, Equations (14)–(21) are needed to solve the coupled equations and to find the thermodynamic properties of the dehumidification system.

The derivatives in each Equations (10)–(13) are replaced by finite difference formulas using the composite finite difference scheme (CFDS) [38]. The idea of CFDS is to use forward finite formulae for the first two steps in space, central differences for the inner space, and backward differences for the last two steps in space. Experimental and simulation result are adopted for validation. The current model predicts the same performance of the simulated and experimental result that was being published in [39] for variant humidity ratio.

The validity of the developed model, the physical model based on Equation (10)–(13) and using the desiccant wheel parameters defined in Table 7 has been solved numerically to evaluate and permit validation relative to prior result published in [39]. A comparison of the Alahmera et al.’s simulation result and the numerical solution considered in this study is illustrated in

Table 9. Model validation for the dehumidification sub-system.

Inlet Humidity Ratio g/kg	Outlet Humidity Ratio g/kg		Relative Error %
	Numerical Solution for This Study	Alahmera et al. Simulation
10.63	7.38	6.9	7.03
12.63	8.21	8.00	2.71
13	8.37	8.5	1.52
13.63	8.63	8.80	1.90
14.3	8.84	9.00	1.77
14.63	9.04	9.40	3.7

. The validation has been performed to the specific inlet humidity ratio illustrated in Table 9.

Figure 5 shows the humidity ratio of the outdoor climate along with the simulated humidity ratio that leaves the dehumidification system for three cities. Overall, there are wide variations of the humidity ratio of the dehumidified air during the year for each of these cities. During winter, the humidity ratio of the dehumidified air is fairly stable and at lower values than present during the summer months.

A closer look at Figure 5 reveals that Pine Bluff, AR has the highest dehumidified air humidity in the summer and transitional months, maxing out in July. Figure 5 also reveals energy saving opportunities for all cities when the dehumidification system is utilized, as evidenced by a significant drop in the humidity ratio of the air moving across the desiccant dehumidification system. As will be seen shortly, the lower humidity ratio in the make-up air as a result of dehumidification will reduce both air conditioning and refrigeration energy requirements.

3.2.2. Photovoltaic/Solar Thermal (PVT)

The PVT energy model defined by Equations (22)–(27) were implemented in MATLAB Simulink. Figure 6 shows the hourly thermal and electric energy produced from the PVT for all cities throughout an entire year. As mentioned previously, the electric energy is dispatched to the store and the thermal energy is used to remove moisture in the desiccant material.

3.2.3. Simulation of Corner Market Internal Environment Humidity Ratio

Figure 7 shows the hourly humidity ratio inside the store for the simulations completed for the three US cities with solar dehumidification. A reduction of the humidity ratio for all cities is observed in this figure. This demonstrates that the solar dehumidification system is successfully removing moisture from the air.

Based on a benchmark result reported in [7], relative humidity in the typical store is 55% which is corresponding to $0.0108{ \mathrm{kg}}_{\mathrm{w}}/{\mathrm{kg}}_{\mathrm{a}}$. A reduction of the humidity ratio of all cities is illustrated where Pin Bluff city shows a higher moisture ratio inside the store among the other cities.

It is important to note that the difference between humidity ratio inside the store and the benchmark for the Pine Bluff, AR case is small, the savings are nevertheless high. The reason for this is that the outdoor relative humidity in this time of the year in AR is near 95%. Relying upon the HVAC system alone to dehumidify ambient air consumes a lot of energy. The solar dehumidification system supplants this energy requirement.

3.2.4. Total Annual Energy Savings from Use of Solar Dehumidification for A Typical Corner Store

Simulation of Corner Market Refrigerated Case Demand

Figure 8 shows the total refrigerated case average monthly demand in kW for the three cities relative to demand at 55% relative humidity. The blue straight line represents the energy consumption when the store relative humidity is kept at the typical 55% RH [8]. For all cases, the store temperature in the simulation is set at 24 °C with ± 1 °C fluctuation. Clear from this figure is that the refrigerated cases energy consumption during the summer season is higher for all cities because of the higher humidity ratio present in the stores. The trend of the energy consumption of the display case cabinets is consistent with the field measurements conducted by Axell and Lindberg, who found that electrical consumption increased 55% during summer because of the variations in the ambient humidity [9]. Here with the utilization of solar dehumidification, the summer increases seen respectively in Dayton, OH, Phoenix, AZ, and Pine Bluff, AR were respectively 30%, 15%, and 26%.

Most importantly, Figure 9 shows that lowering the store humidity reduces the refrigeration system energy consumption regardless of the type refrigerated cabinets present. This is illustrated in Figure 9 which shows the percentage of energy savings for vertical refrigerated cases (left figure) and horizontal refrigerated cases (right figure). The results represented in these demonstrate up to 30% energy savings during the winter season. Savings are much less in the summer (7%–16%). Apparently from this figure, utilizing vertical refrigerated display case, which is the case for this study, is more energy efficient.

Table 10. Display cases refrigeration annual energy requirements kWh.

City	RH 55%	At Design Condition	Percent Saving
Dayton OH	141,150	102,550	27.5%
Pine Bluff AR	141,150	113,296	19.9%
Phoenix AZ	141,150	103,660	26.8%

shows the annual total energy requirements for a typical corner store and one with solar dehumidification for the three cities investigated. The first case represents the typical annual energy usage of the display cases when the humidity level inside the store is 55%, which is the typical humidity level found in the literature for the typical store. The second case represents the annual consumption of the display cases in the proposed system in which the dynamic humidity variation in the outdoor along with moisture generation inside the store are taking into account.

HVAC Energy Systems for Solar Dehumidification System

In this section, the HVAC system energy savings for the proposed system relative to the base case are provided.

Table 11. Latent load requirements and HVAC system savings relative to typical store for solar dehumidification for corner store in three targeted cities.

City	Latent Load kW	Percent Saving in HVAC When Utilizing Dehumidification System
Dayton OH	7.1	28.4 %
Pine Bluff AR	14.17	56.2 %
Phoenix AZ	1.05	4.2 %

shows the energy savings of the HVAC system for all cities, ranging from 56.2% in Pine Bluff, AR to 4.2% in Phoenix, AZ. Clearly, greater savings are realized in the HVAC system for more humid environments. Reliance on the HVAC system to dehumidify the air is not an energy efficient method. This is mainly because air must be cooled below its dew point in the cooling coil and subsequently heated to the setpoint temperature. This process consumes a great amount of energy. The solar dehumidification system eliminates this energy requirement completely.

A Comparison of Energy Demand for Existing Typical Store Relative to the Proposed System

Prior research had revealed corner market intensities ranging from 346 to 700 kWh/${\mathrm{m}}^2$/yr [18], with the lower bound realized from best practice operations. Here, the simulations estimated baseline energy intensities for the typical corner market of 679, 660, and 694 kWh/${\mathrm{m}}^2$-yr in respectively Dayton OH, Phoenix AZ, and Pine Bluff AR. These values fall in the higher range of previous observations as shown in Figure 10. The proposed system with solar dehumidification saws energy intensities 373, 330, and 387 kWh/yr.-${ \mathrm{m}}^2$.

4. Economic Analysis

An economic analysis of the proposed solar dehumidification system is conducted in order to assess its economic feasibility for corner markets.

Table 12. Capital and operating costs for a typical corner store for three cities.

Components	Dayton OH	Pine Bluff AR	Phoenix AZ	Source
HVAC size (ton)	5	6	7	Simulation Results
Capital Cost HVAC System ($)	5740	6890	7690	[40]
Total energy consumption of the store (kWh/ year)	224,350	229,230	217,810	Simulation Results
Operation cost ($/year)	24,678	25,215	23,959	Simulation Results

lists the components of the system and the associated capital and energy costs in a typical corner store for the locations studied. The initial investment cost depends on the equipment capacity. In practice, the HVAC system would likely be downsized some for the proposed system.

Table 13. Estimation of the investment and operating cost of the propose system.

Components	Dayton OH	Pine Bluff AR	Phoenix AZ	Source
HVAC size (ton)	4	4	7	Simulation Results
Capital Cost HVAC System ($)	4550	3590	7690	[40]
Dehumidification system ($)	3000	3000	3000	[41]
PVT ($)	5058.18	5058.18	5058.18	Benchmark [42]
Storage tank ($)	800	800	800
Water pump ($)	2000	2000	2000	[43]
Electric water heater, cost of operation ($/year)	3637.26	2473.2	1881.9	Simulation results
Installation cost of the PVT ($)	10,000	10,000	10,000	[44]
Maintenance cost of the solar system ($/year)	150	150	150	1% for solar thermal [38]
Heat exchanger to pre cooled supply air ($)	1700	1700	1700	[45]
Operation cost ($/year)	13,965.75	11,679.29	11,729.04	Simulation results
Total investment cost ($)	47,661	43,251	46,809
Annual energy saving ($)	11,614.59	15,527.49	13,340.3	Simulation results
Simple payback (years)	4	3	3.5	Simulation results

shows component prices and the operation cost of the proposed system. Some reduction in HVAC capacity is included in the price estimates. The initial investment of the system is varied between the selected cities. These variations are due to the size of the HVAC, availability of the solar resources, and the required auxiliary energy. This table also shows an estimation of the investment and operating cost of the propose system.

The proposed system is characterized by a high initial cost and low operating cost. Determining the life cycle cost of the system through the lifespan of the system is necessary. The life cycle cost is calculated by taking into account the present value of the money. A positive value indicates that the proposed system is worth the extra expenditure.

In this study, an initial investment cost C is needed. In order to make a saving S in the yearly energy bill, the market discount (d) and inflated (e) rates are taken to be 0.08 and 0.05, respectively. The lifespan of the system N is taken as 20 years. The life cost cycle is given by Equations (28) and (29) [46].

$$\mathrm{LCS}=-\mathrm{C}+\mathrm{PWF} \left(\mathrm{N},\mathrm{e},\mathrm{d}\right)\mathrm{S}$$

(28)

$$\mathrm{PWF}\left(\mathrm{N},\mathrm{e},\mathrm{d}\right)={\displaystyle \sum }_{\mathrm{j}=1}^{\mathrm{N}} [{\left(1+\mathrm{e}\right)}^{\mathrm{j}-1}/ {\left(1+\mathrm{d}\right)}^{\mathrm{j}}]=\left\{\begin{array}{c}\left[\frac{1}{\mathrm{d}-\mathrm{e}}\left(1-{\left(\frac{1+\mathrm{e}}{1+\mathrm{d}}\right)}^{\mathrm{n}}\right) \mathrm{if} \mathrm{e}\ne \mathrm{d}\right]\\ \frac{\mathrm{N}}{1+\mathrm{e}} \mathrm{if} \mathrm{e}=\mathrm{d}\end{array}\right\}$$

(29)

Figure 11 illustrates the variation of the life cycle saving with the life cycle period for all cities. The payback period of the proposed system is 4, 3.5, and 3 years in Dayton OH, Phoenix AZ, and Pine Bluff AR, respectively. The initial investment of the system varies between the selected cities. These variations are due to the size of the HVAC, availability of the solar resources, and the required auxiliary energy.

Figure 12 shows a comparison of the annual energy cost for the typical corner store and the proposed system in the respective cities. The study reveals that energy savings in the proposed system are 45%, 51%, and 55% in Dayton OH, Phoenix AZ, and Pine Bluff AR, respectively. Given the slim profit margins in these types of stores, the savings realized here could help corner store owners invest in healthy food options for customers.

5. Discussion

This study presented a theoretical approach aimed at developing a lowest energy corner store. The best contribution of this study to the knowledge is in designing an economic feasible corner market that could be planted in low income areas. As expected, augmenting a typical corner store with solar dehumidification would decrease the operation cost of the refrigeration and HVAC systems. The proposed system is effectively capable of controlling the indoor relative humidity leading to low energy consumption. Results indicated that there can be significant economically viable savings of up to 50%. Moreover, the observed savings in the refrigerated display cases as a result of lowering store relative humidity are in line with what Bahman et al. predicted in [31]. They showed that a 5% reduction in store relative humidity reduces the refrigeration load by 9.25%.

Our research has two main limitations. The first limitation is related to the data availability. Real data on energy consumption for a typical corner store was not available. In this case, a base case energy model for a typical corner store is established in order to estimate energy usage from primarily the HVAC and refrigeration systems. In the base case model, the indoor relative humidity was set at 55%. This assumption permitted a comparison to a prior study [9], but in practice real stores see the relative humidity fluctuating throughout the year. The second limitation is that the savings and conclusions drawn are based upon theoretical analyses only.

Future work should validate the savings and payback experimentally. In addition, system configuration plays a major role in determining the overall performance. Future studies may consider the utilization of other sources of renewable energy such as geothermal. Moreover, future studies should consider the interaction between the store HVAC system and lighting when it comes to overall energy consumption. For example, previous researchers have shown reductions in HVAC energy from use of cold lighting [47]. This would reduce the HVAC and refrigeration energy consumption due to lower heat generation by the lighting system inside the store.

6. Conclusions

The prevalence of food deserts is a nationwide problem that needs immediate attention. The social disparity in health has been especially apparent with COVID-19, where death rates among poor people are by far the greatest. The underlying problem appears to be lack of access to healthier food.

For several years, this problem has been an issue for scholars, politicians, social activists, and even the government. Several concepts have been introduced to address this problem. Many solutions from different perspectives and different disciplines have been suggested. There have likewise been programs, projects and activities that were initiated by the government, nonprofit organizations, and different social groups. However, this problem still exists and is gradually spreading.

The transition to a sustainable energy source is indispensable in the contemporary world, especially for small businesses. Interestingly, a previous study shows that family businesses are more willing to move to more sustainable options when their community faces challenges [48]. This study attempts to address the high energy consumption in corner stores, which many of these stores are family owned. Aside from the environmental benefits of such transition to more sustainable use of energy, the overarching goal has been to improve the energy cost-effectiveness of such stores in order to make healthy food sales economically feasible.

This research is driven by the fact that poor areas in the United States have increasingly seen large groceries and supermarkets go out of business. Created in the aftermath is what has been termed food deserts where people do not have access to healthy food. Therefore, the problem is when these supermarkets close, people start to rely on corner store for food shopping. Generally, these small stores do not sell healthy food for two main reasons. The first reason is the difficulty in purchasing healthy food at reasonable price given the small demands which exist. The second reason is the energy cost for operation of these stores, which is the worst in comparison to all other types of commercial buildings. Thus, the overarching goal has been to improve the energy cost effectiveness of such stores in order to make healthy food sales economically feasible.

The study investigates the potential energy saving of an integrated solar dehumidification with HVAC system in a typical corner store in the USA. A dynamic model was built in MATLAB Simulink, and the performance was analytically investigated. The system was analysis for three different cities in the US located at different climate conditions.

The study shows the potential of solar integrated dehumidification in corner markets of reducing energy requirements by nearly 50%; translating to annual cost savings of nearly $12,000. Such savings could go a long way in helping corner store operators test sales of healthier food options for their customers.