A Joint Stochastic/Deterministic Process with Multi-Objective Decision Making Risk-Assessment Framework for Sustainable Constructions Engineering Projects—A Case Study
Abstract
1. Introduction
2. Literature Survey
3. The Suggested Methodological Framework
4. Application of the Suggested Methodological Framework on the Constructions Sector
4.1. Application of the PRAT and TSP Processes
4.2. Application of the Typical-AHP and the Fuzzy-Extended-AHP MCDM-Processes
4.3. Application of the FTA Process
5. Discussion and Conclusions
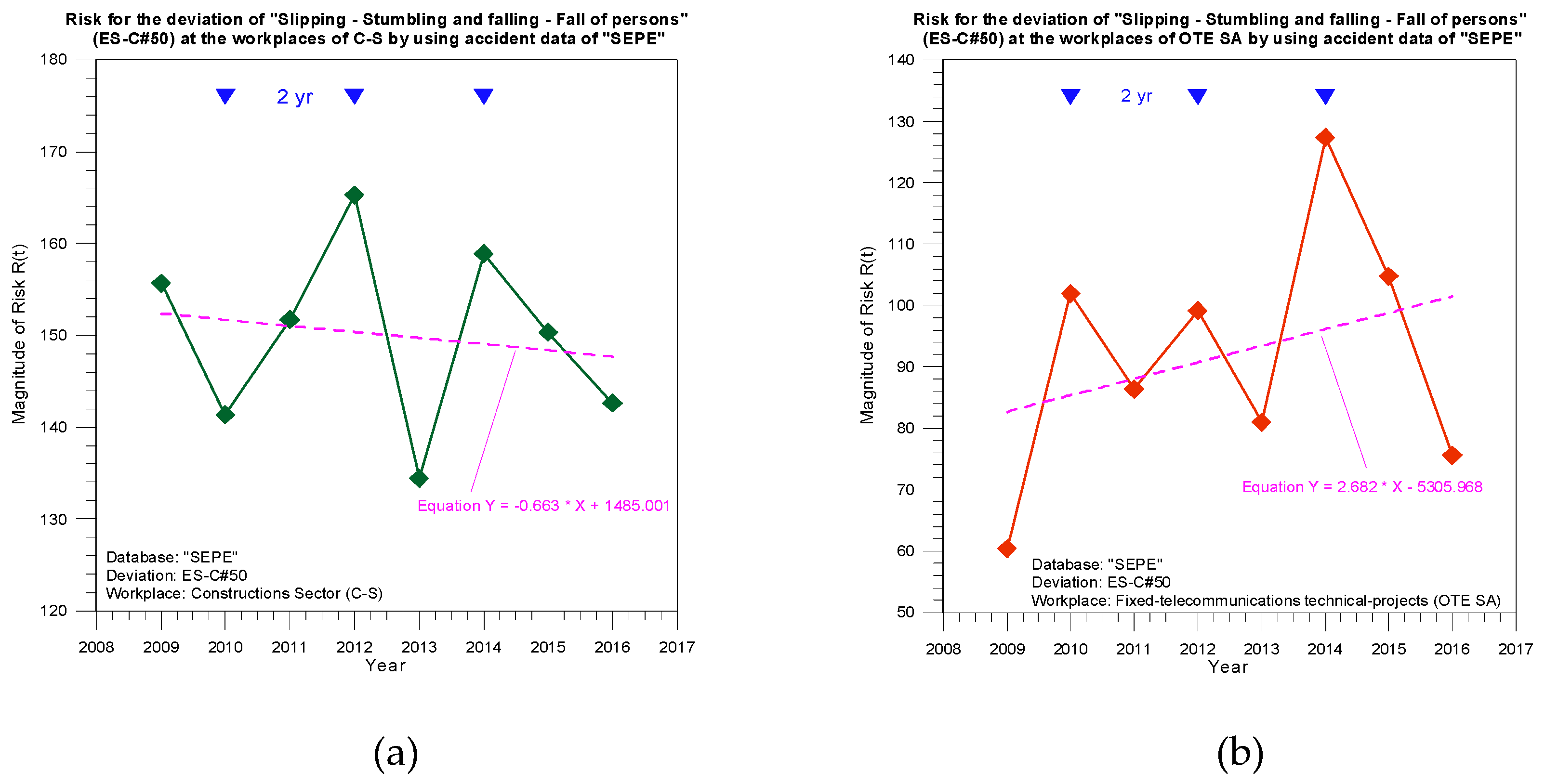
- The most significant hazard-source at the worksites of the C-S and OTE SA is the ES-C#50 one, according to the “SEPE” database (during 2009–2016).
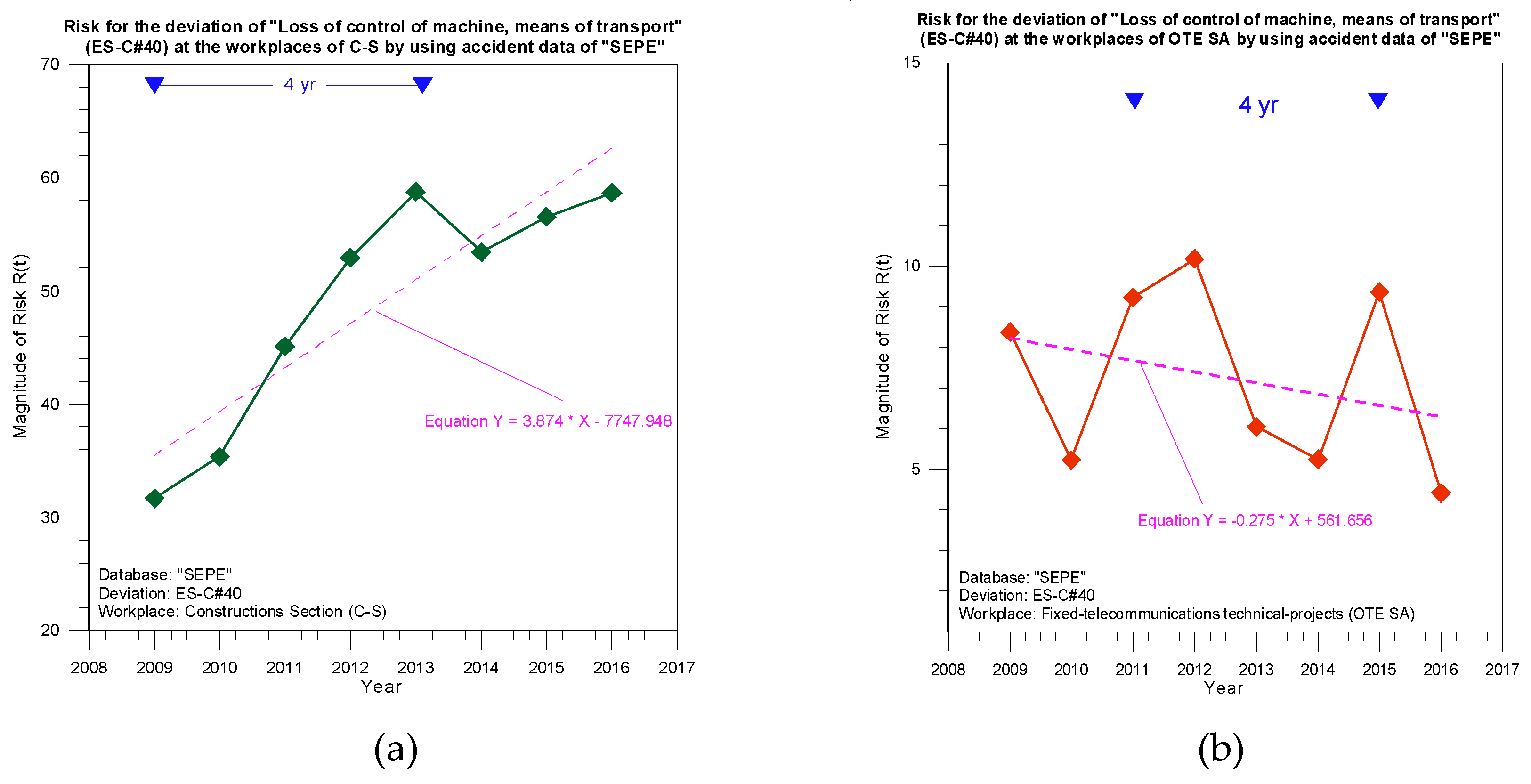
- The most significant hazard-source at the worksites of the C-S and OTE SA is the ES-C#40 one, according to “IKA” database (during 2009–2016).
- The resulting hazard-sources ranking based on the calculated risk-value R is, on the one hand: (i) ES-C#50 & ES-C#40, in accordance with the “SEPE” database, and on the other hand, (ii) ES-C#40 & ES-C#50, consistent with the “IKA” database.
- The maximum value (through 2009–2016) of the magnitude R of the hazard sources, calculated by means of the “SEPE” and “IKA” databases, is about 200.0, that means compulsory measures must be taken earlier than 1.0 year, according to the work of Marhavilas and Koulouriotis (2012b) [10], in order to demote the chance of arising fatal accidents.
- There are other considerable hazard sources which present a risk value higher than 100.0, and according to the work of Marhavilas and Koulouriotis (2012b) [10], long-term actions are necessary for the extinction of their possible dangers.
- Besides, other hazard sources present a risk value smaller than 100.0, and according to the previous referenced paper [10], compulsory actions are not essential except for surveillance of the events.
- The time-profiles of the ES-C#50 deviation show, in almost all cases, the appearance of a trend factor with a negative inclination in the curve of averaging i.e., the average risk-value decreases at the C-S workplaces (during 2009–2016).
- Instead, the profile of ES-C#50 presents a positive inclination (or a soft increase) in the curve of the risk-average, only at the workplaces of OTE SA.
- Besides, the analysis of the time-variations of the ES-C#40 hazard-source displays in its profiles the presence of a tendency factor with a negative slope in the curve of averaging, that means the average risk-value decreases at the workplaces of the C-S and OTE SA (during 2009–2016).
- Instead, the profile of ES-C#40 indicates a positive slope (or an enhancement) in the curve of the risk-average, only at the workplaces of the C-S.
- The analysis of the time-profiles of the magnitude R, concerning the ES-C#50, shows the existence of a periodic fluctuation with a periodicity of ~2 years, which seems to be a permanent feature in the “behavior” of the ES-C#50 deviation at the workplaces of the C-S and OTE SA, according the “SEPE” and “IKA” database.
- Likewise, the time-profiles of R, concerning the deviation of ES-C#40, illustrate the appearance of a periodic fluctuation with a periodicity of ~4 years (i.e., a harmonic of ~2years).
- The AHP theory tries to measure the relative importance of alternatives with respect to each criterion by using pairwise comparisons. In order to do pairwise comparisons, experts use a conversion scale with crisp values, for expressing their ideas. So, an important limitation of AHP is the usage of crisp values to reflect human thinking.
- Another limitation is coming from CR, which is a verification of the rational judgment performed by the experts.
- Moreover, an additional limitation concerns the pairwise comparisons of criteria, which are carried out by several experts with a required significant working-experience in occupational safety.
- As a classic multicriteria decision support tool, AHP is a subjective process, and the resulting results, either rankings or weights, are dependent on the way that the judgments are imposed and the criteria are compared to the rest. In other words, another decision maker (or a group of experts) could make “different” judgments regarding the relative importance of each pair of criteria, resulting in different resulting weights for the criteria and/or different rankings. This drawback leads to the need for applying group-decision-making processes for merging different judgments.
- Besides, when there are slight differences between the criteria’s weights, it is possible that the AHP could lead to the selection of a suboptimal alternative, instead of the optimal one. This phenomenon could be reduced by considering many criteria to be pairwise compared. A review of the AHP drawbacks can be found in the study of Whitaker (2007) [75].
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| AHP | Analytical Hierarchy-Process |
| C-S | Constructions Sector |
| DET | Deterministic process |
| ETA | Event Tree Analysis |
| ESAW | European Statistics on Accidents at Work |
| ES-C | ESAW-Code |
| FEAHP | Fuzzy Extended Analytical Hierarchy-Process |
| FMEA | Failure Mode and Effect Analysis |
| FTA | Fault Tree Analysis |
| HAZOP | Hazard and Operability |
| IKA | Social Insurance Institution, Hellenic/Greek Ministry of Health |
| MCDM | Multi-Criteria Decision-Making |
| OSHA | Occupational Safety & Health Administration |
| OSH | Occupational Safety and Health |
| OTE | OTE SA—the Greek Telecommunications Organization |
| PRAT | Proportional Risk Assessment Technique |
| RA | Risk Assessment |
| RAA | Risk Analysis and Assessment |
| SEPE | Labor Inspectorate, Hellenic/Greek Ministry of Employment |
| STO | Stochastic process |
| TSP | Time Series Processes |
| WHO | World Health Organization |
References
- WHO Global Strategy on Occupational Health for All: The Way to Health at Work. 1994. Available online: https://www.who.int/occupational_health/publications/globstrategy/en/index3.html (accessed on 5 April 2020).
- Boileau, P.-E. Sustainability and prevention in occupational health and safety. Ind. Health. 2016, 54, 293–295. [Google Scholar] [CrossRef]
- Koulinas, G.K.; Demesouka, O.E.; Marhavilas, P.K.; Vavatsikos, A.P.; Koulouriotis, D.E. Risk Assessment Using Fuzzy TOPSIS and PRAT for Sustainable Engineering Projects. Sustainability 2019, 11, 615. [Google Scholar] [CrossRef]
- OSHA Sustainability in the Workplace: A New Approach for Advancing Worker Safety and Health. 2016. Available online: https://www.osha.gov/sustainability/docs/OSHA_sustainability_paper.pdf (accessed on 5 April 2020).
- Marhavilas, P.K.; Koulouriotis, D.E.; Nikolaou, I.; Tsotoulidou, S. International Occupational Health and Safety Management-Systems Standards as a Frame for the Sustainability: Mapping the Territory. Sustainability 2018, 10, 3663. [Google Scholar] [CrossRef]
- Soares, C.G.; Teixeira, A.P. Risk assessment in maritime transportation. Reliab. Eng. Syst. Saf. 2001, 74, 299–309. [Google Scholar] [CrossRef]
- Zheng, X.; Liu, M. An overview of accident forecasting methodologies. J. Loss Prev. Process Ind. 2009, 22, 484–491. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E.; Gemeni, V. Risk Analysis and Assessment Methodologies in the Work Sites: On a Review, Classification and Comparative Study of the Scientific Literature of the Period 2000-2009. J. Loss Prev. Process Ind. 2011, 24, 477–523. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E. Developing a new alternative risk assessment framework in the work sites by including a stochastic and a deterministic process: A case study for the Greek Public Electric Power Provider. Saf. Sci. 2012, 50, 448–462. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E. A combined usage of stochastic and quantitative risk assessment methods in the worksites: Application on an electric power provider. Reliab. Eng. Syst. Saf. 2012, 97, 36–46. [Google Scholar] [CrossRef]
- Lassarre, S. Analysis of progress in road safety in ten European countries. Accid. Anal. Prev. 2001, 33, 743–751. [Google Scholar] [CrossRef]
- Reniers, G.L.L.; Dullaert, W.; Ale, B.J.M.; Soudan, K. The use of current risk analysis tools evaluated towards preventing external domino accidents. J. Loss Prev. Process Ind. 2005, 18, 119–126. [Google Scholar] [CrossRef]
- Verlinden, S.; Deconinck, G.; Coupé, B. Hybrid reliability model for nuclear reactor safety system. Reliab. Eng. Syst. Saf. 2012, 101, 35–47. [Google Scholar] [CrossRef]
- Whiteside, M.B.; Pinho, S.T.; Robinson, P. Stochastic failure modeling of unidirectional composite ply failure. Reliab. Eng. Syst. Saf. 2012, 108, 1–9. [Google Scholar] [CrossRef]
- Guikema, S.D.; Quiring, S.M. Hybrid data mining-regression for infrastructure risk assessment based on zero-inflated data. Reliab. Eng. Syst. Saf. 2012, 99, 178–182. [Google Scholar] [CrossRef]
- Stewart, M.G.; O’Connor, A. Probabilistic risk assessment and service life performance management of load bearing biomedical implants. Reliab. Eng. Syst. Saf. 2012, 108, 49–55. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E.; Spartalis, S.H. Harmonic Analysis of Occupational-Accident Time-Series as a Part of the Quantified Risk Evaluation in Worksites: Application on Electric Power Industry and Construction Sector. Reliab. Eng. Syst. Saf. Elsevier 2013, 112, 8–25. [Google Scholar] [CrossRef]
- Da Costa, M.A.B.; Brandão, A.L.T.; Santos, J.G.F.; Pinto, J.C.; Nele, M. Quantitative FTA using Monte Carlo analyses in a pharmaceutical plant. Eur. J. Pharm. Sci. 2020, 146, 105265. [Google Scholar] [CrossRef]
- Gul, M.; Celik, E. Fuzzy rule-based Fine–Kinney risk assessment approach for rail transportation systems. Hum. Ecol. Risk Assess. 2018, 24, 1786–1812. [Google Scholar] [CrossRef]
- AriamuthuVenkidasalapathy, J.; Mannan, M.S.; Kravaris, C. A quantitative approach for optimal alarm identification. J. Loss Prev. Process Ind. 2018, 55, 213–222. [Google Scholar] [CrossRef]
- Ozkan, N.F.; Uluta, B.H. A novel to approach to quantify the risk probabilities for a risk analysis methodology. Adv. Intell. Syst. Comput. 2017, 487, 341–347. [Google Scholar] [CrossRef]
- Athar, M.; Shariff, A.M.; Buang, A. A review of inherent assessment for sustainable process design. J. Clean. Prod. 2019, 233, 242–263. [Google Scholar] [CrossRef]
- Sanmiquel-Pera, L.; Bascompta, M.; Anticoi, H.F. Analysis of a historical accident in a Spanish coal mine. Int. J. Environ. Res. Public Health 2019, 16, 3615. [Google Scholar] [CrossRef] [PubMed]
- Domínguez, C.R.; Martínez, I.V.; Piñón Peña, P.M.; Rodríguez Ochoa, A. Analysis and evaluation of risks in underground mining using the decision matrix risk-assessment (DMRA) technique, in Guanajuato, Mexico. J. Sustain. Min. 2019, 18, 52–59. [Google Scholar] [CrossRef]
- Kharzi, R.; Chaib, R.; Verzea, I.; Akni, A. Safe and sustainable development in a hygiene and healthy company: Using the decision matrix risk assessment (DMRA) technique: A Case study. J. Min. Environ. 2020. [Google Scholar] [CrossRef]
- Cinar, U.; Cebi, S. A hybrid risk assessment method for mining sector based on QFD, fuzzy logic and AHP. Adv. Intell. Syst. Comput. 2020, 1029, 1198–1207. [Google Scholar] [CrossRef]
- Mutlu, N.G.; Altuntas, S. Risk analysis for occupational safety and health in the textile industry: Integration of FMEA, FTA, and BIFPET methods. Int. J. Ind. Ergon. 2019, 72, 222–240. [Google Scholar] [CrossRef]
- Bora, S.; Bhalerao, Y.; Goyal, A.; Niu, X.; Garg, A. Computation of safety design indexes of industry vehicle operators based on the reach angle, the distance from elbow to ground and the popliteal height. Int. J. Ind. Ergon. 2019, 71, 155–164. [Google Scholar] [CrossRef]
- Kamsu-Foguem, B.; Tiako, P. Risk information formalisation with graphs. Comput. Ind. 2017, 85, 58–69. [Google Scholar] [CrossRef]
- Zheng, X.-Z.; Wang, F.; Zhou, J.-L. A Hybrid Approach for Evaluating Faulty Behavior Risk of High-Risk Operations Using ANP and Evidence Theory. Math. Probl. Eng. 2017, 2017, 7908737. [Google Scholar] [CrossRef]
- Mentes, A.; Ozen, E. A hybrid risk analysis method for a yacht fuel system safety. Saf. Sci. 2015, 79, 94–104. [Google Scholar] [CrossRef]
- Fine, W.T.; Kinney, W.D. Mathematical evaluation for controlling hazards. J. Saf. Res. 1971, 3, 157–166. [Google Scholar]
- Hammer, R.W. Handbook of System and Products Safety; Prentice-Hall, Inc.: Englewood Cliffs, NJ, USA, 1972. [Google Scholar]
- Kinney, G.F.; Wiruth, A.D. Practical Risk Analysis for Safety Management; NTIS report number NWC-TP-5865; Naval Weapons Center: China Lake, CA, USA, 1976. [Google Scholar]
- Woodruff, J.M. Consequence and likelihood in risk estimation: A matter of balance in UK health and safety risk assessment practice. Saf. Sci. 2005, 43, 345–353. [Google Scholar] [CrossRef]
- Reniers, G.L.L.; Dullaert, W.; Ale, B.J.M.; Soudan, K. Developing an external domino prevention framework: Hazwim. J. Loss Prev. Process Ind. 2005, 18, 127–138. [Google Scholar] [CrossRef]
- Van der Hoeven, E.T. CE-Marking: Creating a model for applying the EMC, LVD, and Machinery Directive. MSc Thesis, University of Twente, School of Management and Governance, Enschede, The Netherlands, 2012. [Google Scholar]
- Gul, M.; Guven, B.; Guneri, A.F. A new Fine-Kinney-based risk assessment framework using FAHP-FVIKOR incorporation. J. Loss Prev. Process Ind. 2018, 53, 3–16. [Google Scholar] [CrossRef]
- Kokangül, A.; Polat, U.; Dağsuyu, C. A new approximation for risk assessment using the AHP and Fine Kinney methodologies. Saf. Sci. 2017, 91, 24–32. [Google Scholar] [CrossRef]
- Marhavilas, P.K. Risk Assessment Techniques in the Worksites of Occupational Health-Safety Systems with Emphasis on Industries and Constructions. Ph.D. Thesis, Department of Production and Management Engineering, Democritus University of Thrace, Xanthi, Greece, 2015. Available online: http://hdl.handle.net/10442/hedi/35612 (accessed on 10 April 2020).
- Marhavilas, P.K.; Koulouriotis, D.E.; Mitrakas, C. On the development of a new hybrid risk assessment process using occupational accidents data: Application on the Greek Public Electric Power Provider. J. Loss Prev. Process Ind. 2011, 24, 671–687. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E. A risk estimation methodological framework using quantitative assessment techniques and real accidents’ data: Application in an aluminum extrusion industry. J. Loss Prev. Process Ind. 2008, 21, 596–603. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E.; Mitrakas, C. Fault and Event-Tree techniques in occupational health-safety systems-Part I: Integrated risk-evaluation scheme. Environ. Eng. Manag. J. 2014, 13, 2097–2108. [Google Scholar] [CrossRef]
- Marhavilas, P.K.; Koulouriotis, D.E.; Mitrakas, C. Fault and Event-Tree techniques in occupational health-safety systems-Part II: Statistical analysis. Environ. Eng. Manag. J. 2014, 13, 2371–2386. [Google Scholar] [CrossRef]
- Babaei, M.; Roozbahani, A.; Shahdany, S.M.H. Risk Assessment of Agricultural Water Conveyance and Delivery Systems by Fuzzy Fault Tree Analysis Method. Water Resour. Manag. 2018, 32, 4079–4101. [Google Scholar] [CrossRef]
- Yasli, F.; Bolat, B. A risk analysis model for mining accidents using a fuzzy approach based on fault tree analysis. J. Enterp. Inf. Manag. 2018, 31, 577–594. [Google Scholar] [CrossRef]
- Gul, M.; Ak, M.F. A comparative outline for quantifying risk ratings in occupational health and safety risk assessment. J. Clean. Prod. 2018, 196, 653–664. [Google Scholar] [CrossRef]
- Fuentes-Bargues, J.L.; González-Cruz, M.C.; González-Gaya, C.; Baixauli-Pérez, M.P. Risk analysis of a fuel storage terminal using HAZOP and FTA. Int. J. Environ. Res. Public Health 2017, 14, 705. [Google Scholar] [CrossRef] [PubMed]
- Hanea, D.M.; Jagtman, H.M.; Ale, B.J.M. Analysis of the Schiphol Cell Complex fire using a Bayesian belief net based model. Reliab. Eng. Syst. Saf. 2012, 100, 115–124. [Google Scholar] [CrossRef]
- Lisnianski, A.; Elmakias, D.; Laredo, D.; Ben Haim, H. A multi-state Markov model for a short-term reliability analysis of a power generating unit. Reliab. Eng. Syst. Saf. 2012, 98, 1–6. [Google Scholar] [CrossRef]
- Khakzad, N.; Khan, F.; Amyotte, P. Risk-based design of process systems using discrete-time Bayesian networks. Reliab. Eng. Syst. Saf. 2013, 109, 5–17. [Google Scholar] [CrossRef]
- Wang, F.; Ding, L.Y.; Luo, H.B.; Love, P.E.D. Probabilistic risk assessment of tunneling-induced damage to existing properties. Expert Syst. Appl. 2014, 41, 951–961. [Google Scholar] [CrossRef]
- Biyikli, O.; Aydogan, E.K. A new model suggestion to estimate the probability value in occupational health and safety risk assessment. Appl. Math. Inf. Sci. 2016, 10, 663–671. [Google Scholar] [CrossRef]
- Zheng, G.; Zhu, N.; Tian, Z.; Chen, Y.; Sun, B. Application of a trapezoidal fuzzy AHP method for work safety evaluation and early warning rating of hot and humid environments. Saf. Sci. 2012, 50, 228–239. [Google Scholar] [CrossRef]
- Fattahi, R.; Khalilzadeh, M. Risk evaluation using a novel hybrid method based on FMEA, extended MULTIMOORA, and AHP methods under fuzzy environment. Saf. Sci. 2018, 102, 290–300. [Google Scholar] [CrossRef]
- Mete, S. Assessing occupational risks in pipeline construction using FMEA-based AHP-MOORA integrated approach under Pythagorean fuzzy environment. Hum. Ecol. Risk Assess. 2019, 25, 1645–1660. [Google Scholar] [CrossRef]
- Oturakci, M. A new fuzzy-based approach for environmental risk assessment. Hum. Ecol. Risk Assess. 2019, 25, 1718–1728. [Google Scholar] [CrossRef]
- Yucesan, M.; Kahraman, G. Risk evaluation and prevention in hydropower plant operations: A model based on Pythagorean fuzzy AHP. Energy Policy 2019, 126, 343–351. [Google Scholar] [CrossRef]
- Boral, S.; Howard, I.; Chaturvedi, S.K.; McKee, K.; Naikan VN, A. An integrated approach for fuzzy failure modes and effects analysis using fuzzy AHP and fuzzy MAIRCA. Eng. Fail. Anal. 2020, 108. [Google Scholar] [CrossRef]
- Marhavilas, P.K. Risk Estimation in the Greek Constructions’ Worksites by using a Quantitative Assessment Technique and Statistical Information of Occupational Accidents. J. Eng. Sci. Technol. Res. (JESTR) 2009, 2, 51–55. [Google Scholar] [CrossRef]
- Ardeshir, A.; Maknoon, R.; Rekab Islamizadeh, M.; Jahantab, Z. Safety risk assessment effective on occupational health in high-rise building construction projects with fuzzy approach. Iran Occup. Health 2014, 11, 82–95. [Google Scholar]
- Anil Kumar, C.N.; Sakthivel, M.; Elangovan, R.K.; Arularasu, M. Analysis of Material Handling Safety in Construction Sites and Countermeasures for Effective Enhancement. Sci. World J. 2015, 742084. [Google Scholar] [CrossRef] [PubMed]
- Marhavilas, P.K.; Vrountas, P.T. Risk Assessment in the Constructions Sector of EU Countries: Application of a Methodological Framework using Quantitative Techniques and Occupational Accidents’ Data throughout the period 1996–2011. J. Eng. Sci. Technol. Res. (JESTR) 2018, 11, 66–73. [Google Scholar] [CrossRef]
- Koulinas, G.K.; Marhavilas, P.K.; Demesouka, O.E.; Vavatsikos, A.P.; Koulouriotis, D.E. Risk Analysis and Assessment in the worksites using the Fuzzy-Analytical Hierarchy Process and a Quantitative Technique – A case study for the Greek Construction sector. Saf. Sci. 2019, 112, 96–104. [Google Scholar] [CrossRef]
- European Union (EU). European Statistics on Accidents at Work (ESAW)-Summary Methodology; EUROSTAT, European Commission: Luxembourg, 2013; ISBN 978-92-79-28419-9. [Google Scholar] [CrossRef]
- Saaty, T.L. How to make a decision: The analytic hierarchy process. Eur. J. Oper. Res. 1990, 48, 9–26. [Google Scholar] [CrossRef]
- Chang, D.-Y. Applications of the extent analysis method on fuzzy AHP. Eur. J. Oper. Res. 1996, 95, 649–655. [Google Scholar] [CrossRef]
- Lamata, M.T. Ranking of alternatives with ordered weighted averaging operators. Int. J. Intell. Syst. 2004, 19, 473–482. [Google Scholar] [CrossRef]
- Zimmermann, H.J. Fuzzy Set Theory—And Its Applications; Springer Science & Business Media: New York, NY, USA, 2001. [Google Scholar]
- Chan, H.K.; Sun, X.; Chung, S.-H. When should fuzzy analytic hierarchy process be used instead of analytic hierarchy process? Decis. Support Syst. 2019, 125. [Google Scholar] [CrossRef]
- Haasl, D.F.; Roberts, N.H.; Vesely, W.E.; Goldberg, F.F. Fault Tree Handbook; NUREG-0492, TRN: 82-003645; U.S. Nuclear Regulatory Commission: Washington, DC, USA, 1981; p. 20555. Available online: https://www.nrc.gov/docs/ML1007/ML100780465.pdf (accessed on 10 April 2020).
- ICST&D. International Conference on Sustainable Technology and Development. Shenzhen, China, 22–25 August 2020; Available online: https://www.elsevier.com/events/conferences/international-conference-on-sustainable-technology-and-development (accessed on 10 April 2020).
- Marhavilas, P.K.; Filippidis, M.; Koulinas, G.K.; Koulouriotis, D.E. A HAZOP with MCDM Based Risk-Assessment Approach: Focusing on the Deviations with Economic/Health/Environmental Impacts in a Process Industry. MDPI Sustainability, section: Sustainable Engineering and Science, Special Issue: Decision Support Systems and Knowledge Management for Sustainable Engineering. Sustainability 2020, 12, 993. [Google Scholar] [CrossRef]
- Holeček, P.; Talašová, J. A free software tool implementing the fuzzy AHP method. In Proceedings of the 34th International Conference on Mathematical Methods in Economics 2016, Liberec, Czech Republic, 6–9 September 2016; pp. 266–271, ISBN 978-80-7494-296-9. [Google Scholar]
- Whitaker, R. Criticisms of the Analytic Hierarchy Process: Why they often make no sense. Math. Comput. Modeling 2007, 46, 948–961. [Google Scholar] [CrossRef]




| 1st Level of Hazards Type of Deviation | ESAW-Code (ES-C) | 2nd Level of Hazards Description of Hazards (or Injuries) |
|---|---|---|
| (a) | (b) | (c) |
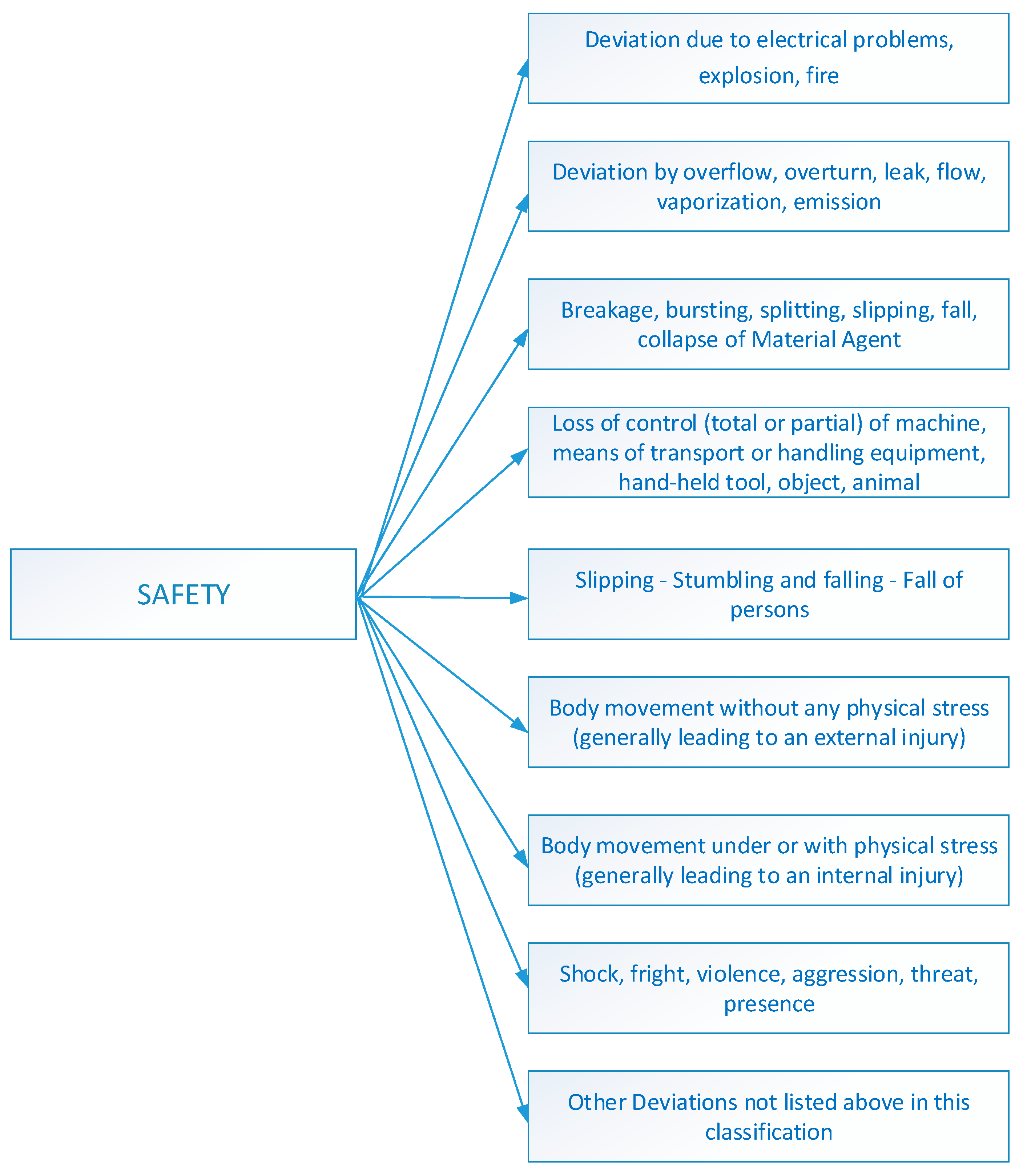
| Working Environment & Processes | 10 | Deviation due to electrical problems, explosion, fire [e.g., (i) exposure to or contact with extreme temperature levels, (ii) exposure to or contact with electric current, etc.] |
| Working Environment & Processes | 20 | Deviation by overflow, overturn, leak, flow, vaporization, emission (e.g., exposure to or contact with hazardous substances or radiation, etc.) |
| Working Environment & Processes | 30 | Breakage, bursting, splitting, slipping, fall, collapse of material agent (e.g., slipping, collapse and being struck by falling objects) |
| Psychological/Human | 40 | Loss of control (total or partial) of machine, means of transport or handling equipment, hand-held tool, object, animal [e.g., (i) collision with an immobile object and falling against or being struck by moving objects, (ii) trapping, being crushed-inside or between objects, etc.] |
| Physical Activity | 50 | Slipping—stumbling and falling—fall of persons [e.g., (i) falling of person from a height, (ii) falling of person-on the same level] |
| Physical Activity | 60 | Body movement without any physical stress (generally leading to an external injury) |
| Physical Activity | 70 | Body movement under or with physical stress (generally leading to an internal injury) [e.g., physical strain—over-exertion] |
| Psychological/Human | 80 | Shock, fright, violence, aggression, threat, presence |
| Working Environment & Processes | 99 | Other deviations not listed above in this classification |
| Importance of Factor i over Factor j | Fuzzy Number |
|---|---|
| Equal | [1,1,1] |
| Equal to Moderate | [1,2,3] |
| Moderate | [2,3,4] |
| Moderate to Strong | [3,4,5] |
| Strong | [4,5,6] |
| Strong to Very Strong | [5,6,7] |
| Very Strong | [6,7,8] |
| Very Strong to Extremely | [7,8,9] |
| Extremely | [8,9,9] |
| Safety ESAW Codes (ES-C#) | [10] | [20] | [30] | [40] | [50] | [60] | [70] | [80] | [99] |
|---|---|---|---|---|---|---|---|---|---|
| [10] | 1 | 5 | 1/3 | 1/4 | 1/5 | 3 | 2 | 5 | 5 |
| [20] | 1 | 1/5 | 1/6 | 1/7 | 1/2 | 1/3 | 2 | 2 | |
| [30] | 1 | 1/2 | 1/3 | 4 | 3 | 6 | 6 | ||
| [40] | 1 | 1/2 | 5 | 4 | 7 | 7 | |||
| [50] | 1 | 6 | 5 | 8 | 8 | ||||
| [60] | 1 | 1/2 | 3 | 3 | |||||
| [70] | 1 | 4 | 4 | ||||||
| [80] | 1 | 2 | |||||||
| [99] | 1 |
| Hazards’ Weights (Typical-AHP) | Hazards’ Ranking (Typical-AHP) | Hazards’ Weights (FEAHP) | Hazards’ Ranking (FEAHP) | ||||
|---|---|---|---|---|---|---|---|
| [10] | 10.04% | [50] | 31.51% | [10] | 12.59% | [50] | 25.72% |
| [20] | 3.24% | [40] | 22.63% | [20] | 3.95% | [40] | 20.99% |
| [30] | 16.06% | [30] | 16.06% | [30] | 16.67% | [30] | 16.67% |
| [40] | 22.63% | [10] | 10.04% | [40] | 20.99% | [10] | 12.59% |
| [50] | 31.51% | [70] | 7.08% | [50] | 25.72% | [70] | 8.98% |
| [60] | 4.83% | [60] | 4.83% | [60] | 6.21% | [60] | 6.21% |
| [70] | 7.08% | [20] | 3.24% | [70] | 8.98% | [20] | 3.95% |
| [80] | 2.49% | [80] | 2.49% | [80] | 2.86% | [80] | 2.86% |
| [99] | 2.13% | [99] | 2.13% | [99] | 2.03% | [99] | 2.03% |
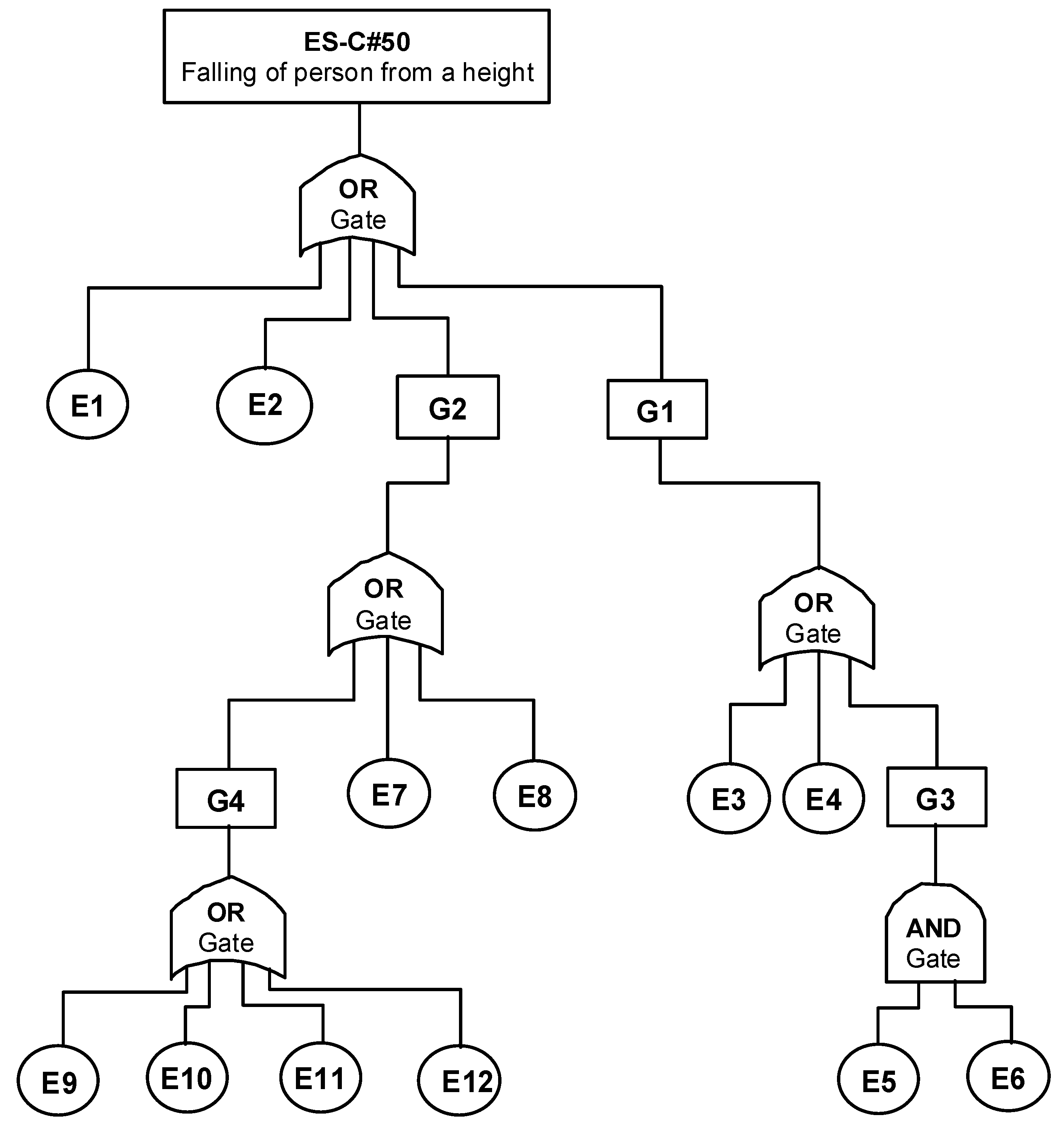
| Base-Events | Description of “Base-Events” | Estimated Probability (P) |
|---|---|---|
| E1 | Improper use of tools/equipment | 20.0% |
| E2 | Strong winds | 1.0% |
| E3 | Slippery shoes | 0.1% |
| E4 | Worn seat belts | 5.0% |
| E5 | Lack of helmet | 10.0% |
| E6 | Contact of materials with the head | 10.0% |
| E7 | Pathological causes | 0.1% |
| E8 | Heat/cold | 0.1% |
| E9 | Overtime/repetitive work | 10.0% |
| E10 | Guard | 10.0% |
| E11 | Standing | 10.0% |
| E12 | Intense stress | 10.0% |
| Intermediate Events | Description of “Intermediate-Events” | Calculated Probability (P) |
|---|---|---|
| G1 | Improper use of personal protective or damaged equipment | 6.0% |
| G2 | Loss of consciousness/concentration | 34.5% |
| G3 | Negligence | 1.0% |
| G4 | Fatigue | 34.4% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marhavilas, P.K.; Tegas, M.G.; Koulinas, G.K.; Koulouriotis, D.E. A Joint Stochastic/Deterministic Process with Multi-Objective Decision Making Risk-Assessment Framework for Sustainable Constructions Engineering Projects—A Case Study. Sustainability 2020, 12, 4280. https://doi.org/10.3390/su12104280
Marhavilas PK, Tegas MG, Koulinas GK, Koulouriotis DE. A Joint Stochastic/Deterministic Process with Multi-Objective Decision Making Risk-Assessment Framework for Sustainable Constructions Engineering Projects—A Case Study. Sustainability. 2020; 12(10):4280. https://doi.org/10.3390/su12104280
Chicago/Turabian StyleMarhavilas, Panagiotis K., Michael G. Tegas, Georgios K. Koulinas, and Dimitrios E. Koulouriotis. 2020. "A Joint Stochastic/Deterministic Process with Multi-Objective Decision Making Risk-Assessment Framework for Sustainable Constructions Engineering Projects—A Case Study" Sustainability 12, no. 10: 4280. https://doi.org/10.3390/su12104280
APA StyleMarhavilas, P. K., Tegas, M. G., Koulinas, G. K., & Koulouriotis, D. E. (2020). A Joint Stochastic/Deterministic Process with Multi-Objective Decision Making Risk-Assessment Framework for Sustainable Constructions Engineering Projects—A Case Study. Sustainability, 12(10), 4280. https://doi.org/10.3390/su12104280








