Sectoral Decomposition of Korea’s Energy Consumption by Global Value Chain Dimensions
Abstract
:1. Introduction
2. Literature Survey
3. Methodology and Data
3.1. Measuring International Fragmentation on GVCs
3.2. LMDI Decomposition from the GVC Perspective
3.3. Data Construction
4. Empirical Results
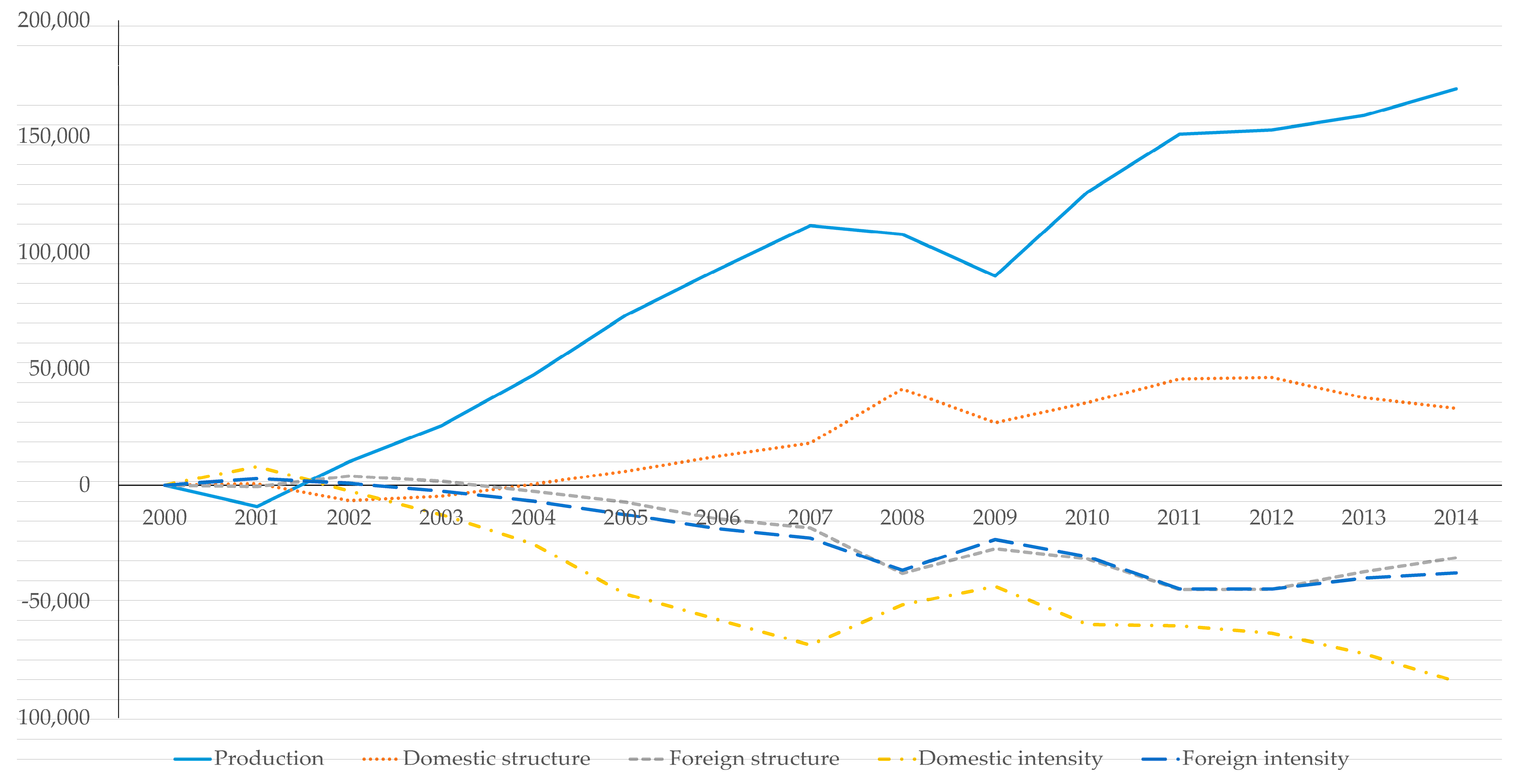
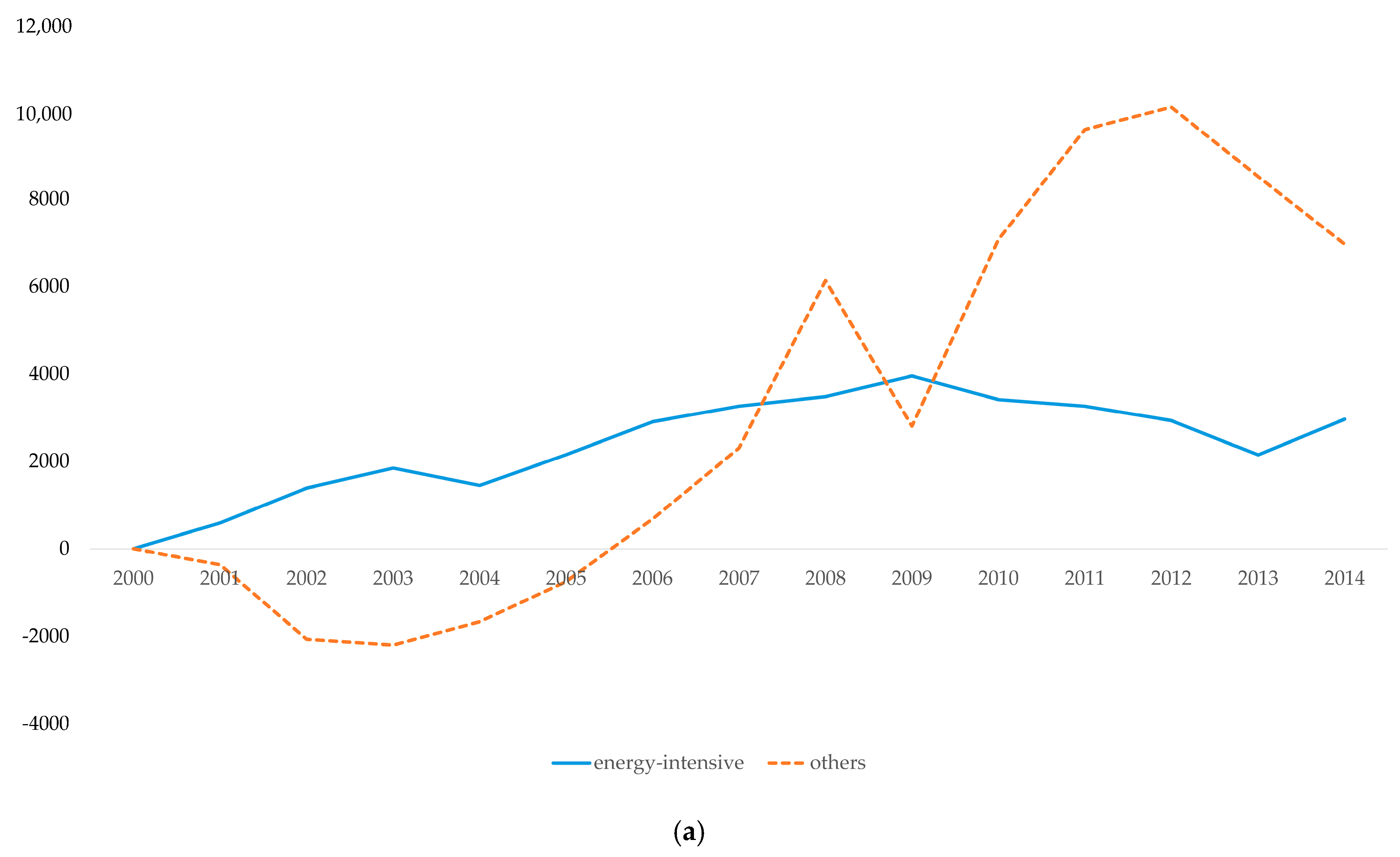
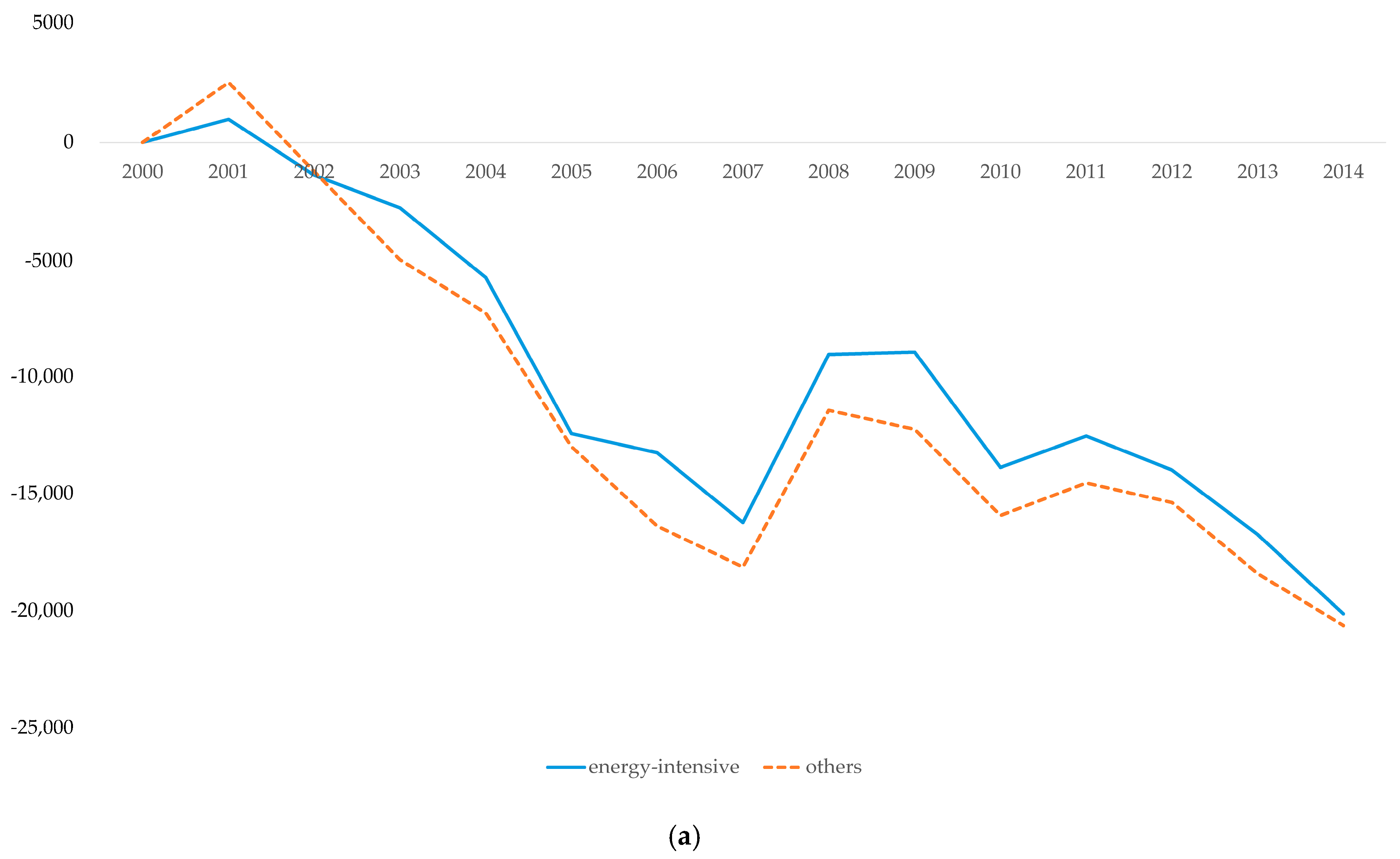
4.1. Aggregate Energy Decomposition
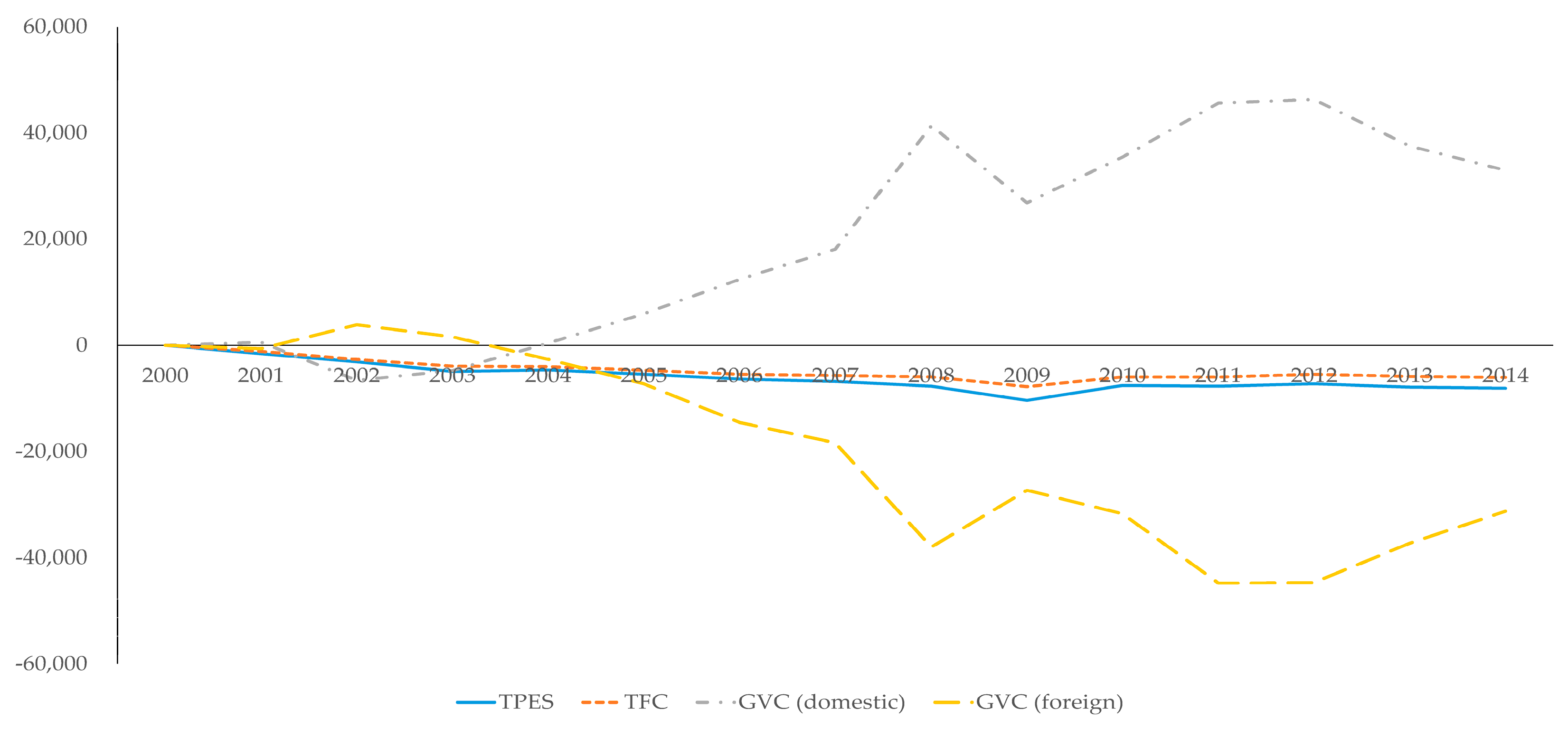
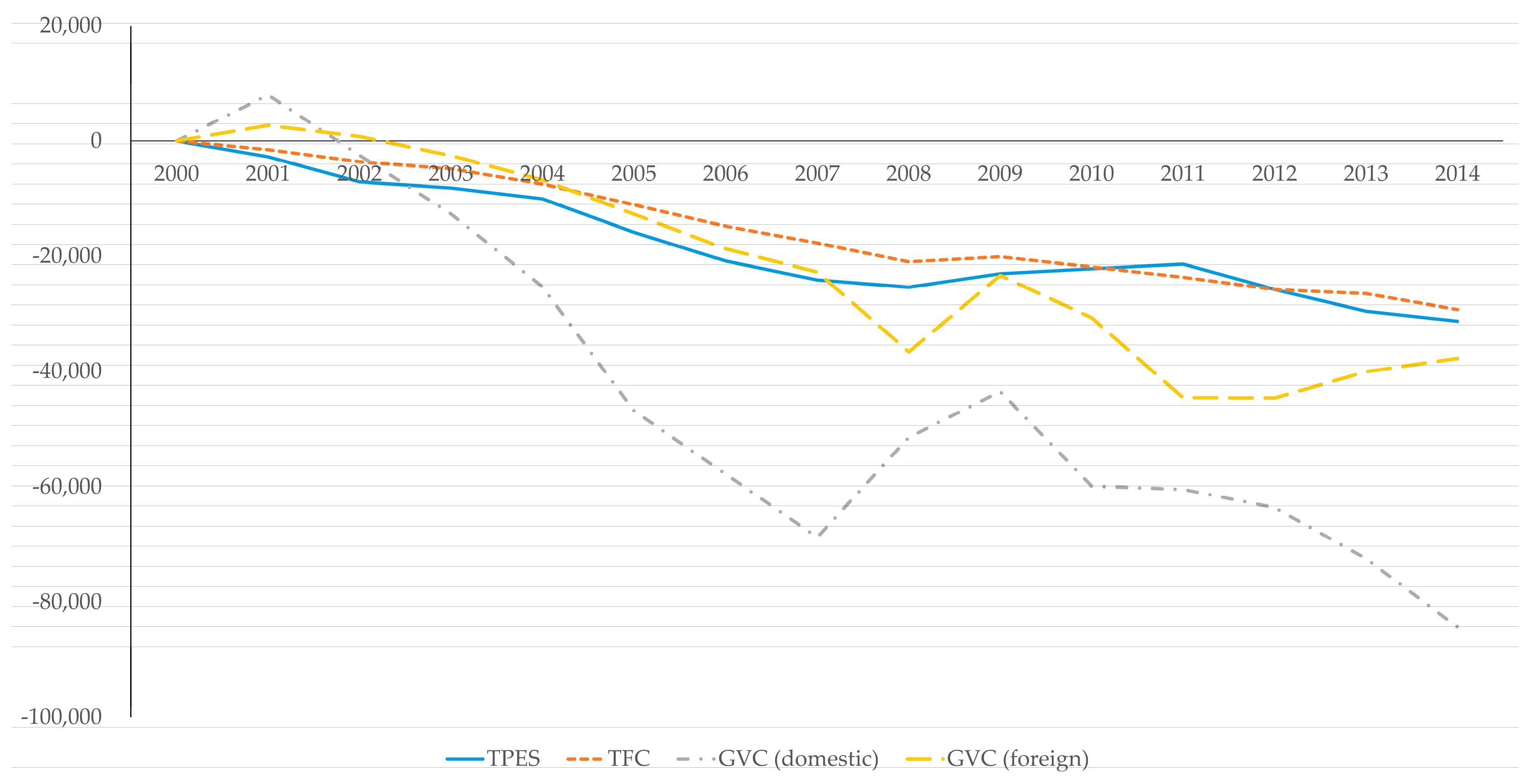
4.2. Decomposition Results of Energy-Intensive and Other Industries
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| IEA (2019) | KOSIS 1 (2020) | WIOT |
|---|---|---|
| Iron and steel/non-ferrous metals | Basic metals | Basic metals |
| Fabricated metal products | Fabricated metal products, except machinery and equipment | |
| Chemical and petrochemical | Coke, briquette, and refined petroleum products | Rubber and plastic products |
| Coke and refined petroleum products | ||
| Chemicals and chemical products | Chemicals and chemical products | |
| Basic pharmaceutical products and pharmaceutical preparations | ||
| Non-metallic minerals | Other non-metallic mineral products | Other non-metallic mineral products |
| Transport equipment | Transport equipment | Motor vehicles, trailers, and semi-trailers |
| Other transport equipment | ||
| Machinery | Electrical equipment | Electrical equipment |
| Computer, electronic, and optical instrument | Computer, electronic, and optical products | |
| Other machinery and equipment | Machinery and equipment n.e.c. | |
| Mining and quarrying | Mining and quarrying | Mining and quarrying |
| Food and tobacco | Food and tobacco products | Food products, beverages, and tobacco products |
| Paper, pulp, and printing/wood and wood products | Wood, pulp, paper, and paper products | Paper and paper products |
| Wood and of products of wood and cork, except furniture; articles of straw and plaiting materials | ||
| Printing and reproduction of recorded media | ||
| Construction | Construction | Construction |
| Textile and leather | Textile and leather | Textiles, wearing apparel, and leather products |
| Non-specified (industry) | Other manufacturing | Furniture; other manufacturing |
| Repair and installation of machinery and equipment | ||
| Transport | Transport | Land transport and transport via pipelines |
| Water transport | ||
| Air transport | ||
| Warehousing and support activities for transportation | ||
| Commercial and public services | Wholesale and retail/accommodation and food services | Wholesale and retail trade and repair of motor vehicles and motorcycles |
| Wholesale trade, except of motor vehicles and motorcycles | ||
| Retail trade, except of motor vehicles and motorcycles | ||
| Postal and courier activities | ||
| Accommodation and food service activities | ||
| Human health and social work | Human health and social work activities | |
| Electricity, gas, steam, and air conditioning supply | ||
| Water collection, treatment, and supply | ||
| Sewerage; waste collection, treatment, and disposal activities; materials recovery; remediation activities and other waste management services | ||
| Financial and insurance | Financial service activities, except insurance and pension funding | |
| Insurance, reinsurance, and pension funding, except compulsory social security | ||
| Activities auxiliary to financial services and insurance activities | ||
| Real estate | Real estate activities | |
| Business service | Telecommunications | |
| Computer programming, consultancy, and related activities; information service activities | ||
| Motion picture, video and television program production, sound recording, and music publishing activities; programming and broadcasting activities | ||
| Legal and accounting activities; activities of head offices; management consultancy activities | ||
| Architectural and engineering activities; technical testing and analysis | ||
| Scientific research and development | ||
| Advertising and market research | ||
| Other professional, scientific, and technical activities; veterinary activities | ||
| Administrative and support service activities | ||
| Public administration and defense | Public administration and defense; compulsory social security | |
| Education | Education | |
| Culture and other services | Publishing activities | |
| Other service activities | ||
| Activities of households as employers; undifferentiated goods- and services-producing activities of households for own use | ||
| Activities of extraterritorial organizations and bodies | ||
| Agriculture/forestry/fishing | Agriculture, forestry, and fishing | Crop and animal production, hunting, and related service activities |
| Fishing and aquaculture | ||
| Forestry and logging |
References
- Linden, G.; Kenneth, K.L.; Dedrick, J. Who captures value in a global innovation network? The case of Apple’s iPod. Commun. ACM. 2009, 52, 140–144. [Google Scholar] [CrossRef]
- Los, B.; Timmer, M.P.; de Vries, G.J. How global are global value chains? A new approach to measure international fragmentation. J. Reg. Sci. 2015, 55, 66–92. [Google Scholar] [CrossRef]
- UNFCC. The Paris Agreement. Available online: https://unfccc.int/process-and-meetings/the-paris-agreement/the-paris-agreement (accessed on 9 September 2020).
- Choi, B. Productivity and misallocation of energy resources: Evidence from Korea’s manufacturing Sector. Resour. Energy Econ. 2020, 61, 101184. [Google Scholar] [CrossRef]
- Timmer, M.P.; Azeez Erumban, A.; Los, B.; Stehrer, R.; de Vries, G.J. Slicing Up Global Value Chains. J. Econ. Perspect. 2014, 28, 99–118. [Google Scholar] [CrossRef] [Green Version]
- Sun, J.W. Changes in energy consumption and energy intensity: A complete decomposition model. Energy Econ. 1998, 20, 85–100. [Google Scholar] [CrossRef]
- Ang, B. Decomposition of industrial energy consumption. The energy intensity approach. Energy Econ. 1994, 16, 163–174. [Google Scholar] [CrossRef]
- Ang, B.W.; Choi, K.H. Decomposition of aggregate energy and gas emission intensities for industry: A refined divisia index method. Energy J. 1997, 18, 59–73. [Google Scholar] [CrossRef]
- Ang, B. The LMDI approach to decomposition analysis: A practical guide. Energy Policy 2005, 33, 867–871. [Google Scholar] [CrossRef]
- Kim, J. A Review on energy decomposition analysis in Korea. Korean Energy Econ. Rev. 2015, 14, 265–291. [Google Scholar] [CrossRef]
- British Petroleum (BP). BP Statistical Review-2019; British Petroleum (BP): London, UK, 2019. [Google Scholar]
- Xu, J.H.; Fleiter, T.; Eichhammer, W.; Fan, Y. Energy consumption and CO2 emissions in China’s cement industry: A perspective from LMDI decomposition analysis. Energy Policy 2012, 50, 821–832. [Google Scholar] [CrossRef]
- Wang, W.; Liu, X.; Zhang, M.; Song, X. Using a new generalized LMDI (logarithmic mean Divisia index) method to analyze China’s energy consumption. Energy 2014, 67, 617–622. [Google Scholar] [CrossRef]
- Wang, M.; Feng, C. Decomposing the change in energy consumption in China’s nonferrous metal industry: An empirical analysis based on the LMDI method. Renew. Sustain. Energy Rev. 2018, 82, 2652–2663. [Google Scholar] [CrossRef]
- Sun, X.; Liu, X. Decomposition analysis of debt’s impact on China’s energy consumption. Energy Policy 2020, 146, 111802. [Google Scholar] [CrossRef]
- Achour, H.; Belloumi, M. Decomposing the influencing factors of energy consumption in Tunisian transportation sector using the LMDI method. Transp. Policy 2016, 52, 64–71. [Google Scholar] [CrossRef]
- Akyürek, Z. LMDI decomposition analysis of energy consumption of Turkish manufacturing industry: 2005–2014. Energy Effic. 2020, 13, 649–663. [Google Scholar] [CrossRef]
- Kim, S. LMDI Decomposition analysis of energy consumption in the Korean manufacturing sector. Sustainability 2017, 9, 202. [Google Scholar] [CrossRef] [Green Version]
- Bianco, V. Analysis of electricity consumption in the tourism sector. A decomposition approach. J. Clean. Prod. 2020, 248, 119286. [Google Scholar] [CrossRef]
- Fernández González, P.; Landajo, M.; Presno, M.J. Multilevel LMDI decomposition of changes in aggregate energy consumption. A cross country analysis in the EU-27. Energy Policy 2014, 68, 576–584. [Google Scholar] [CrossRef]
- Chen, X.; Shuai, C.; Zhang, Y.; Wu, Y. Decomposition of energy consumption and its decoupling with economic growth in the global agricultural industry. Environ. Impact Assess. Rev. 2020, 81, 106364. [Google Scholar] [CrossRef]
- Feenstra, R.C.; Hanson, G.H. The impact of outsourcing and high-technology capital on wages: estimates for the united states, 1979–1990. Q. J. Econ. 1999, 114, 907–940. [Google Scholar] [CrossRef] [Green Version]
- Hummels, D.; Ishii, J.; Yi, K.M. The nature and growth of vertical specialization in world trade. J. Int. Econ. 2001, 54, 75–96. [Google Scholar] [CrossRef]
- Johnson, R.C.; Noguera, G. Accounting for intermediates: Production sharing and trade in value added. J. Int. Econ. 2012, 86, 224–236. [Google Scholar] [CrossRef] [Green Version]
- Timmer, M.P.; Los, B.; Stehrer, R.; de Vries, G.J. Fragmentation, incomes and jobs: An analysis of European competitiveness. Econ. Policy 2013, 28, 613–661. [Google Scholar] [CrossRef]
- Koopman, R.; Wang, Z.; Wei, S.J. Tracing value-added and double counting in gross exports. Am. Econ. Rev. 2014, 104, 459–494. [Google Scholar] [CrossRef] [Green Version]
- IEA. World Energy Balances; IEA: Paris, France, 2019. [Google Scholar]
- Korean Statistical Information Service (KOSIS) GDP and GNI by Economic Activity. Available online: https://kosis.kr (accessed on 11 August 2020).
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
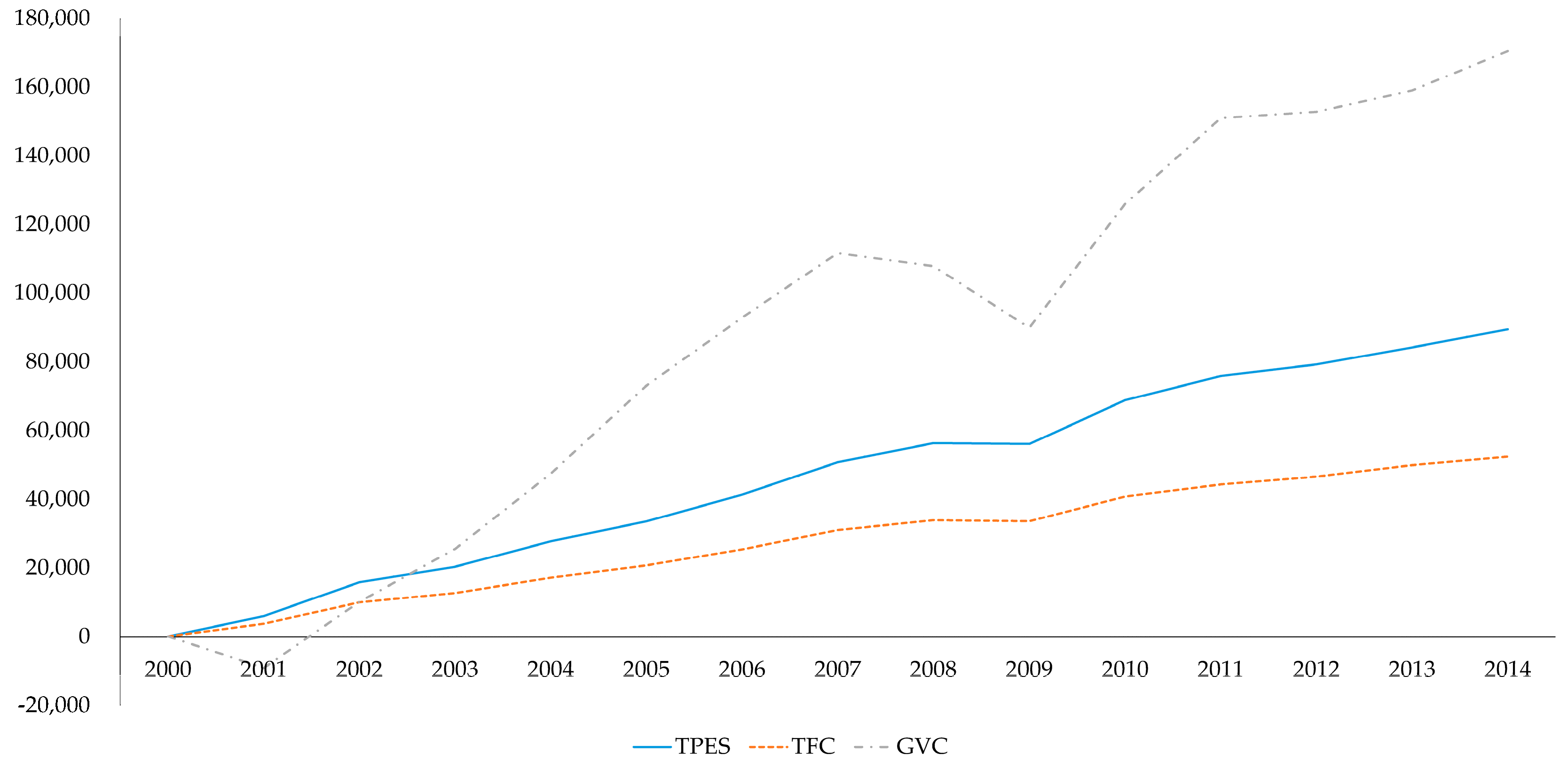



| Intermediate Use (S Columns per Country) | Final Use (C Columns per Country) | Total | |||||
|---|---|---|---|---|---|---|---|
| 1 | … | N | 1 | … | N | ||
| S industries, country 1 | |||||||
| … | |||||||
| S industries, country 43 | |||||||
| Value added | |||||||
| Output | |||||||
| Variables | Mean | St.dev | Max | Min | Units |
|---|---|---|---|---|---|
| TPES | 11,412.68 | 11,743.93 | 49,077.49 | 336.60 | ktoe |
| TFC | 6691.33 | 7889.60 | 31,867.00 | 134.00 | ktoe |
| Value-added (GVC) | 52,788.63 | 71,675.87 | 398,065.05 | 488.31 | Millions USD |
| Real GDP | 86,479.84 | 168,579.72 | 846,274.80 | 2260.40 | Billions KRW |
| Sector | TPES (%) | TFC (%) | GVC (%) | Real GDP (%) | Energy Intensity |
|---|---|---|---|---|---|
| Iron and steel/non-ferrous metals | 63.34 | 67.04 | 291.14 | 52.63 | 0.03 |
| Chemical and petrochemical | 36.58 | 45.74 | 261.30 | 74.63 | −0.07 |
| Non-metallic minerals | 6.50 | 2.66 | 158.65 | 45.91 | −0.25 |
| Transport equipment | 75.06 | 28.62 | 283.66 | 94.84 | −0.02 |
| Machinery | 160.60 | 167.88 | 178.18 | 155.01 | 0.00 |
| Mining and quarrying | 46.72 | 53.73 | 106.35 | −29.88 | 0.11 |
| Food and tobacco | 32.30 | 13.34 | 102.97 | 21.43 | 0.02 |
| Paper, pulp and printing/wood and wood products | 4.79 | 2.94 | 96.71 | 23.12 | −0.07 |
| Construction | 67.92 | 67.92 | 131.09 | 17.70 | 0.00 |
| Textile and leather | −45.09 | −52.51 | 96.99 | 18.78 | −0.32 |
| Non-specified (industry) | −39.72 | −46.57 | 391.60 | 51.24 | −0.55 |
| Transport | 20.83 | 21.32 | 177.15 | 69.10 | −0.23 |
| Commercial and public services | 54.02 | 16.90 | 195.22 | 77.87 | −0.01 |
| Agriculture/forestry/fishing | 9.83 | −26.52 | 84.65 | 23.04 | −0.02 |
| Total | 36.66 | 19.92 | 192.40 | 75.63 | −0.03 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, T.; Choi, B. Sectoral Decomposition of Korea’s Energy Consumption by Global Value Chain Dimensions. Sustainability 2020, 12, 8483. https://doi.org/10.3390/su12208483
Jin T, Choi B. Sectoral Decomposition of Korea’s Energy Consumption by Global Value Chain Dimensions. Sustainability. 2020; 12(20):8483. https://doi.org/10.3390/su12208483
Chicago/Turabian StyleJin, Taeyoung, and Bongseok Choi. 2020. "Sectoral Decomposition of Korea’s Energy Consumption by Global Value Chain Dimensions" Sustainability 12, no. 20: 8483. https://doi.org/10.3390/su12208483
APA StyleJin, T., & Choi, B. (2020). Sectoral Decomposition of Korea’s Energy Consumption by Global Value Chain Dimensions. Sustainability, 12(20), 8483. https://doi.org/10.3390/su12208483





