Game Analysis and Coordination of a Closed-Loop Supply Chain: Perspective of Components Reuse Strategy
Abstract
1. Introduction
2. Literature Review
2.1. The Development of Closed-Loop Supply Chain Network Design
2.2. The Game Theory Models in Closed-Loop Supply Chain
2.3. Research Gaps and Contributions
3. Description of the Close-Loop Supply Chain System and Assumptions
3.1. Notations
3.2. Description and Assumptions
4. Three-Stage Game Models Under Decentralized Decision
4.1. Decision Model
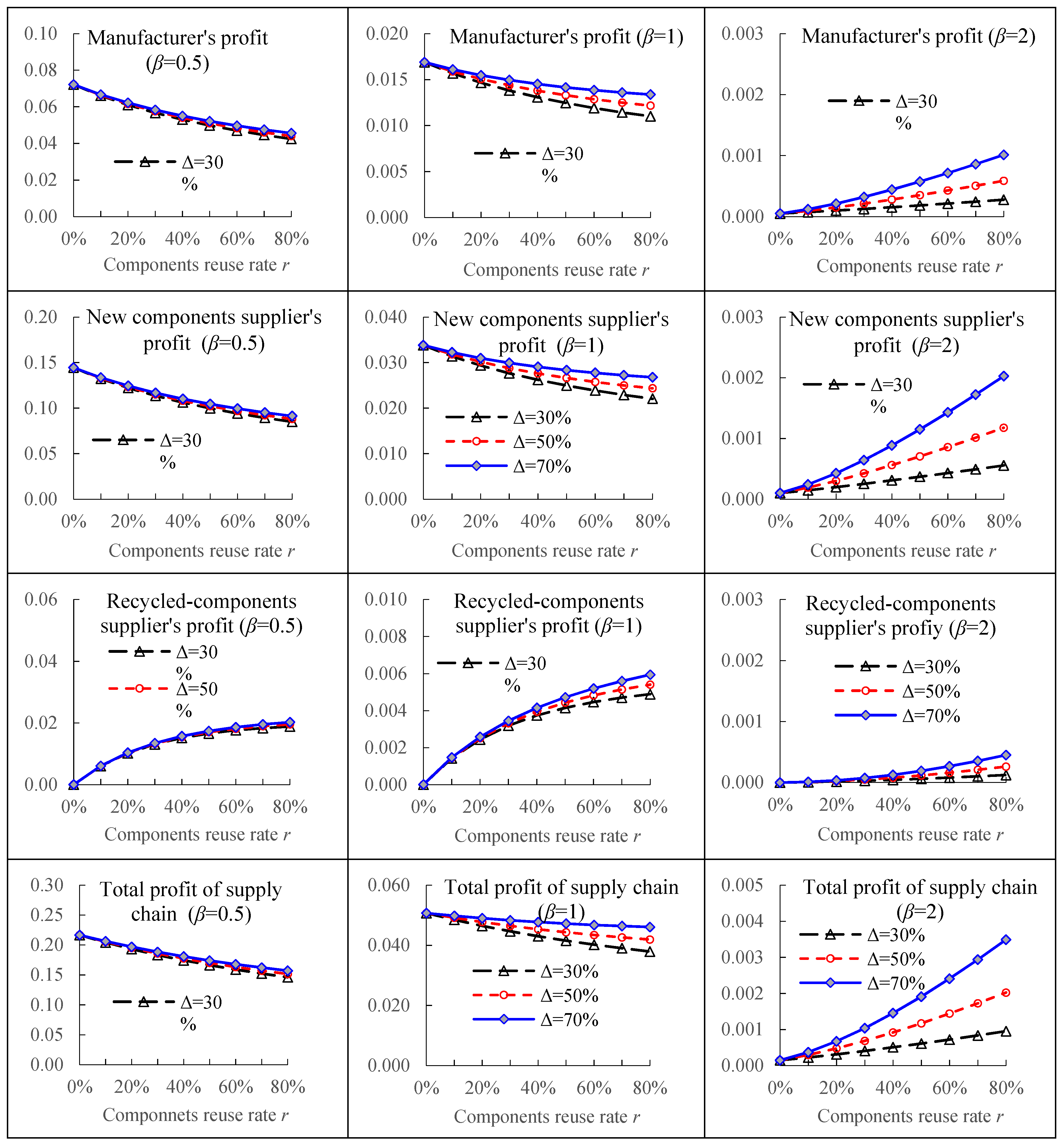
4.2. Numerical Analysis
5. The Model under Centralized Decision
6. Contract Coordination Mechanism
7. Analysis on the Effect of Wholesale Price Coordination Contracts
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Govindan, K.; Mina, H.; Esmaeili, A.; Gholami-Zanjani, S.M. An integrated hybrid approach for circular supplier selection and closed loop supply chain network design under uncertainty. J. Clean. Prod. 2020, 242, 118317. [Google Scholar] [CrossRef]
- Guide, V.D.R.; Van Wassenhove, L.N. OR FORUM-The evolution of closed-loop supply chain research. Oper. Res. 2009, 57, 10–18. [Google Scholar] [CrossRef]
- Gupta, S.; Palsule-Desai, O.D. Sustainable supply chain management: Review and research opportunities. IIMB Manag. Rev. 2011, 23, 234–245. [Google Scholar] [CrossRef]
- Peng, H.; Shen, N.; Liao, H.; Xue, H.; Wang, Q. Uncertainty factors, methods, and solutions of closed-loop supply chain—A review for current situation and future prospects. J. Clean. Prod. 2020, 254. [Google Scholar] [CrossRef]
- Schenkel, M.; Caniëls, M.C.J.; Krikke, H.; Van Der Laan, E. Understanding value creation in closed loop supply chains-Past findings and future directions. J. Manuf. Syst. 2015, 37, 729–745. [Google Scholar] [CrossRef]
- Fleischmann, M.; Bloemhof-Ruwaard, J.M.; Dekker, R.; Van der Laan, E.; Van, J.A.; Van Wassenhove, L.N. Quantitative models for reverse logistics: A review. Eur. J. Oper. Res. 1997, 103, 1–17. [Google Scholar] [CrossRef]
- Rubio, S.; Chamorro, A.; Miranda, F.J. Characteristics of the research on reverse logistics (1995–2005). Int. J. Prod. Res. 2008, 46, 1099–1120. [Google Scholar] [CrossRef]
- Govindan, K.; Soleimani, H.; Kannan, D. Reverse logistics and closed-loop supply chain: A comprehensive review to explore the future. Eur. J. Oper. Res. 2015, 240, 603–626. [Google Scholar] [CrossRef]
- Jayaraman, V.; Guide, V.D.R., Jr.; Srivastava, R. A closed-loop logistics model for remanufacturing. J. Oper. Res. Soc. 1999, 50, 497–508. [Google Scholar] [CrossRef]
- Fleischmann, M.; Beullens, P.; Bloemhof-Ruwaard, J.M.; Wassenhove, L.N. The impact of product recovery on logistics network design. Prod. Oper. Manag. 2001, 10, 156–173. [Google Scholar] [CrossRef]
- Pati, R.K.; Vrat, P.; Kumar, P. A goal programming model for paper recycling system. Omega 2008, 36, 405–417. [Google Scholar] [CrossRef]
- Amin, S.H.; Zhang, G. An integrated model for closed-loop supply chain configuration and supplier selection: Multi-objective approach. Expert Syst. Appl. 2012, 39, 6782–6791. [Google Scholar] [CrossRef]
- Chen, Y.W.; Wang, L.C.; Wang, A.; Chen, T.L. A particle swarm approach for optimizing a multi-stage closed loop supply chain for the solar cell industry. Robot. Comput. Integr. Manuf. 2017, 43, 111–123. [Google Scholar] [CrossRef]
- Ma, H.; Li, X. Closed-loop supply chain network design for hazardous products with uncertain demands and returns. Appl. Soft. Comput. 2018, 68, 889–899. [Google Scholar] [CrossRef]
- He, L.; Mao, J.; Hu, C.; Xiao, Z. Carbon emission regulation and operations in the supply chain supernetwork under stringent carbon policy. J. Clean. Prod. 2019, 238, 117652. [Google Scholar] [CrossRef]
- Samuel, C.N.; Venkatadri, U.; Diallo, C.; Khatab, A. Robust closed-loop supply chain design with presorting, return quality and carbon emission considerations. J. Clean. Prod. 2020, 247, 119086. [Google Scholar] [CrossRef]
- Yu, H.; Solvang, W.D. A fuzzy-stochastic multi-objective model for sustainable planning of a closed-loop supply chain considering mixed uncertainty and network flexibility. J. Clean. Prod. 2020, 266, 121702. [Google Scholar] [CrossRef]
- Huang, M.; Song, M.; Lee, L.H.; Ching, W.K. Analysis for strategy of close-loop supply chain with dual recycling channel. Int. J. Prod. Econ. 2013, 144, 510–520. [Google Scholar] [CrossRef]
- Wei, J.; Zhao, J. Pricing and remanufacturing decisions in two competing supply chains. Int. J. Prod. Res. 2015, 53, 258–278. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, C.; Xu, L. Decision for pricing, service, and recycling of closed-loop supply chains considering different remanufacturing roles and technology authorizations. Comput. Ind. Eng. 2019, 132, 59–73. [Google Scholar] [CrossRef]
- Gao, J.; Teng, J.; Hou, L.; Liu, X. Pricing strategy of closed-loop supply chain considering competition under uncertain demand. J. Syst. Eng. 2017, 32, 78–88. (In Chinese) [Google Scholar]
- Chen, J.M.; Chang, C.I. The co-opetitive strategy of a closed-loop supply chain with remanufacturing. Transp. Res. Part E 2012, 48, 387–400. [Google Scholar] [CrossRef]
- Zhang, H.; Gan, X.; Lai, M. Coordination of closed-loop supply chain with the optimal price and recycle effort incentive contract. J. Syst. Eng. 2015, 30, 201–209. (In Chinese) [Google Scholar]
- Panda, S.; Modak, N.M.; Cardenas-Barron, L.E. Coordinating a socially responsible closed-loop supply chain with product recycling. Int. J. Prod. Econ. 2017, 188, 11–21. [Google Scholar] [CrossRef]
- Yang, R.; Tang, W.; Dai, R.; Zhang, J. Contract design in reverse recycling supply chain with waste cooking oil under asymmetric cost information. J. Clean. Prod. 2018, 201, 61–77. [Google Scholar] [CrossRef]
- Hosseini-Motlagh, S.M.; Ebrahimi, S.; Zirakpourdehkordi, R. Coordination of dual-function acquisition price and corporate social responsibility in a sustainable closed-loop supply chain. J. Clean. Prod. 2020, 251, 119629. [Google Scholar] [CrossRef]
- Li, X.; Zhu, Q. Contract Design for Enhancing Green Food Material Production Effort with Asymmetric Supply Cost Information. Sustainability 2020, 12, 2119. [Google Scholar] [CrossRef]
- Hong, I.-H.; Yeh, J.-S. Modeling closed-loop supply chains in the electronics industry: A retailer collection application. Transp. Res. Part E 2012, 48, 817–829. [Google Scholar] [CrossRef]
- Atasu, A.; Toktay, L.B.; Van Wassenhove, L.N. How Collection Cost Structure Drives Manufacturer’s Reverse Channel Choice. Prod. Oper. Manag. 2013, 22, 1089–1102. [Google Scholar] [CrossRef]
- Feng, L.; Govindan, K.; Li, C. Strategic planning: Design and coordination for dual-recycling channel reverse supply chain considering consumer behavior. Eur. J. Oper. Res. 2017, 260, 601–612. [Google Scholar] [CrossRef]
- Gong, Y.; Chen, M.; Zhuang, Y. Decision-Making and Performance Analysis of Closed-Loop Supply Chain under Different Recycling Modes and Channel Power Structures. Sustainability 2019, 11, 6413. [Google Scholar] [CrossRef]
- Yi, Y.; Liang, J. Hybrid recycling modes for closed-loop supply chain under premium and penalty mechanism. Comput. Integr. Manuf. Syst. 2014, 20, 215–223. (In Chinese) [Google Scholar]
- Zhao, X.; Lin, Y.; Su, C. Performance analysis of S-M closed-loop supply chain under different channel power structures. Chin. J. Manag. Sci. 2012, 20, 78–86. (In Chinese) [Google Scholar]
- Xiong, Y.; Zhou, Y.; Li, G.; Chan, H.-K.; Xiong, Z. Don’t forget your supplier when remanufacturing. Eur. J. Oper. Res. 2013, 230, 15–25. [Google Scholar] [CrossRef]
- He, Y. Acquisition pricing and remanufacturing decisions in a closed-loop supply chain. Int. J. Prod. Econ. 2015, 163, 48–60. [Google Scholar] [CrossRef]
- Yi, Y. Closed-loop supply chain game models with product remanufacturing in a duopoly retailer channel. J. Manag. Sci. China 2009, 12, 45–54. (In Chinese) [Google Scholar]
- Cachon, G.P.; Lariviere, M.A. Supply chain coordination with revenue-sharing contracts: Strengths and limitations. Manag. Sci. 2005, 51, 30–44. [Google Scholar] [CrossRef]
- Govindan, K.; Popiuc, M.N.; Diabat, A. Overview of coordination contracts within forward and reverse supply chains. J. Clean. Prod. 2013, 47, 319–334. [Google Scholar] [CrossRef]
- Institute of Internet Industry. 2018 White Paper on Intelligent Remanufacturing Industry; Tsinghua University: Beijing, China, 2018. [Google Scholar]

| Products with High Price Elasticity | Products with Low Price Elasticity | |
|---|---|---|
| Coordination Principles | ||
| Conditions of Coefficient |
| Products with High Price Elasticity | Products with Low Price Elasticity | |
|---|---|---|
| Contract between the Manufacturer and the New Components Supplier | ||
| Contract between the Manufacturer and the Recycled-Components Supplier | ||
| Benchmark | Components Reuse ( | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Centralized Decision | Decentralized Decision | Coordination Contracts | Centralized Decision | Decentralized Decision | Coordination Contracts | ||||||
| 0.49500 | 0.48190 | 0.49304 | 0.48190 | 0.48190 | 0.48190 | 0.47650 | 0.49096 | 0.47650 | 0.47650 | 0.47650 | |
| 0.19000 | / | 0.20586 | 0.18995 | 0.19974 | 0.19484 | / | 0.21357 | 0.19291 | 0.20563 | 0.19927 | |
| 0.13100 | / | 0.13296 | 0.12868 | 0.12868 | 0.12868 | / | 0.09904 | 0.09348 | 0.09348 | 0.09348 | |
| 0.01000 | 0.03620 | 0.01392 | 0.03620 | 0.03620 | 0.03620 | 0.04700 | 0.01808 | 0.04700 | 0.04700 | 0.04700 | |
| 0.00005 | / | 0.00013 | 0.00037 | 0.00013 | 0.00025 | / | 0.00021 | 0.00063 | 0.00021 | 0.00042 | |
| 0.00010 | / | 0.00025 | 0.00025 | 0.00050 | 0.00038 | / | 0.00042 | 0.00042 | 0.00084 | 0.00063 | |
| 0.00000 | / | 0.00003 | 0.00003 | 0.00003 | 0.00003 | / | 0.00005 | 0.00005 | 0.00005 | 0.00005 | |
| 0.00015 | 0.00066 | 0.00041 | 0.00066 | 0.00066 | 0.00066 | 0.00110 | 0.00069 | 0.00110 | 0.00110 | 0.00110 | |
| Benchmark | Components Reuse () | Components Reuse () | |||||||||
| Centralized Decision | Decentralized Decision | Coordination Contracts | Centralized Decision | Decentralized Decision | Coordination Contracts | ||||||
| 0.49500 | 0.47380 | 0.49181 | 0.47380 | 0.47380 | 0.47380 | 0.46300 | 0.48844 | 0.46300 | 0.46300 | 0.46300 | |
| 0.19000 | / | 0.24550 | 0.20047 | 0.23143 | 0.21595 | / | 0.27250 | 0.20891 | 0.25263 | 0.23077 | |
| 0.13100 | / | 0.13419 | 0.12856 | 0.12856 | 0.12856 | / | 0.10156 | 0.09361 | 0.09361 | 0.09361 | |
| 0.01000 | 0.05240 | 0.01638 | 0.05240 | 0.05240 | 0.05240 | 0.07400 | 0.02313 | 0.07400 | 0.07400 | 0.07400 | |
| 0.00005 | / | 0.00021 | 0.00086 | 0.00021 | 0.00054 | / | 0.00043 | 0.00172 | 0.00043 | 0.00107 | |
| 0.00010 | / | 0.00043 | 0.00043 | 0.00108 | 0.00075 | / | 0.00086 | 0.00086 | 0.00215 | 0.00150 | |
| 0.00000 | / | 0.00008 | 0.00008 | 0.00008 | 0.00008 | / | 0.00016 | 0.00016 | 0.00016 | 0.00016 | |
| 0.00015 | 0.00137 | 0.00072 | 0.00137 | 0.00137 | 0.00137 | 0.00274 | 0.00144 | 0.00274 | 0.00274 | 0.00274 | |
| Components Reuse ( | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Centralized Decision | Decentralized Decision | Coordination Contracts | Centralized Decision | Decentralized Decision | Coordination Contracts | ||||||
| 1.620 | 1.232 | 1.705 | 1.232 | 1.232 | 1.232 | 1.227 | 1.703 | 1.227 | 1.227 | 1.227 | |
| 0.940 | / | 1.277 | 0.729 | 0.954 | 0.841 | / | 1.285 | 0.733 | 0.960 | 0.846 | |
| 0.506 | / | 0.421 | 0.240 | 0.240 | 0.240 | / | 0.388 | 0.204 | 0.204 | 0.204 | |
| 0.190 | 0.384 | 0.148 | 0.384 | 0.384 | 0.384 | 0.387 | 0.149 | 0.387 | 0.387 | 0.387 | |
| 0.072 | / | 0.057 | 0.134 | 0.074 | 0.104 | / | 0.058 | 0.136 | 0.075 | 0.106 | |
| 0.144 | / | 0.113 | 0.147 | 0.208 | 0.178 | / | 0.115 | 0.150 | 0.211 | 0.180 | |
| 0.000 | / | 0.013 | 0.013 | 0.013 | 0.013 | / | 0.013 | 0.013 | 0.013 | 0.013 | |
| 0.217 | 0.295 | 0.183 | 0.295 | 0.295 | 0.295 | 0.299 | 0.186 | 0.299 | 0.299 | 0.299 | |
| Components Reuse () | Components Reuse () | ||||||||||
| Centralized Decision | Decentralized Decision | Coordination Contracts | Centralized Decision | Decentralized Decision | Coordination Contracts | ||||||
| 1.620 | 1.224 | 1.757 | 1.224 | 1.224 | 1.224 | 1.213 | 1.754 | 1.213 | 1.213 | 1.213 | |
| 0.940 | / | 2.121 | 1.150 | 1.522 | 1.336 | / | 2.148 | 1.164 | 1.540 | 1.352 | |
| 0.506 | / | 0.369 | 0.202 | 0.202 | 0.202 | / | 0.336 | 0.167 | 0.167 | 0.167 | |
| 0.190 | 0.388 | 0.121 | 0.388 | 0.388 | 0.388 | 0.394 | 0.123 | 0.394 | 0.394 | 0.394 | |
| 0.072 | / | 0.047 | 0.133 | 0.075 | 0.104 | / | 0.048 | 0.137 | 0.077 | 0.107 | |
| 0.144 | / | 0.094 | 0.151 | 0.208 | 0.179 | / | 0.097 | 0.155 | 0.214 | 0.184 | |
| 0.000 | / | 0.018 | 0.018 | 0.018 | 0.018 | / | 0.018 | 0.018 | 0.018 | 0.018 | |
| 0.217 | 0.301 | 0.159 | 0.301 | 0.301 | 0.301 | 0.310 | 0.163 | 0.310 | 0.310 | 0.310 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, X.; Bai, X.; Fan, Z.; Liu, T. Game Analysis and Coordination of a Closed-Loop Supply Chain: Perspective of Components Reuse Strategy. Sustainability 2020, 12, 9681. https://doi.org/10.3390/su12229681
Zhao X, Bai X, Fan Z, Liu T. Game Analysis and Coordination of a Closed-Loop Supply Chain: Perspective of Components Reuse Strategy. Sustainability. 2020; 12(22):9681. https://doi.org/10.3390/su12229681
Chicago/Turabian StyleZhao, Xiaomin, Xueli Bai, Zhihui Fan, and Ting Liu. 2020. "Game Analysis and Coordination of a Closed-Loop Supply Chain: Perspective of Components Reuse Strategy" Sustainability 12, no. 22: 9681. https://doi.org/10.3390/su12229681
APA StyleZhao, X., Bai, X., Fan, Z., & Liu, T. (2020). Game Analysis and Coordination of a Closed-Loop Supply Chain: Perspective of Components Reuse Strategy. Sustainability, 12(22), 9681. https://doi.org/10.3390/su12229681





