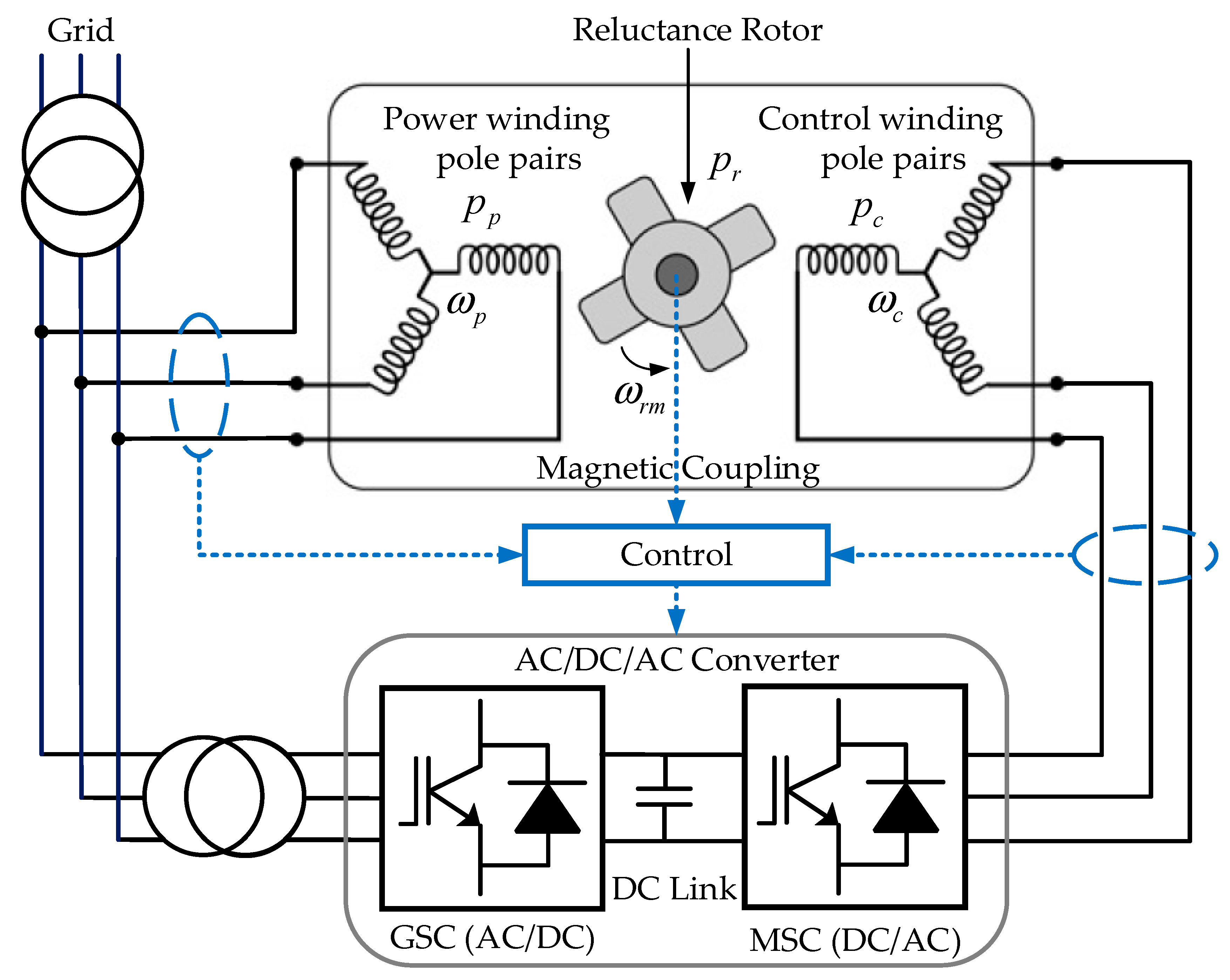
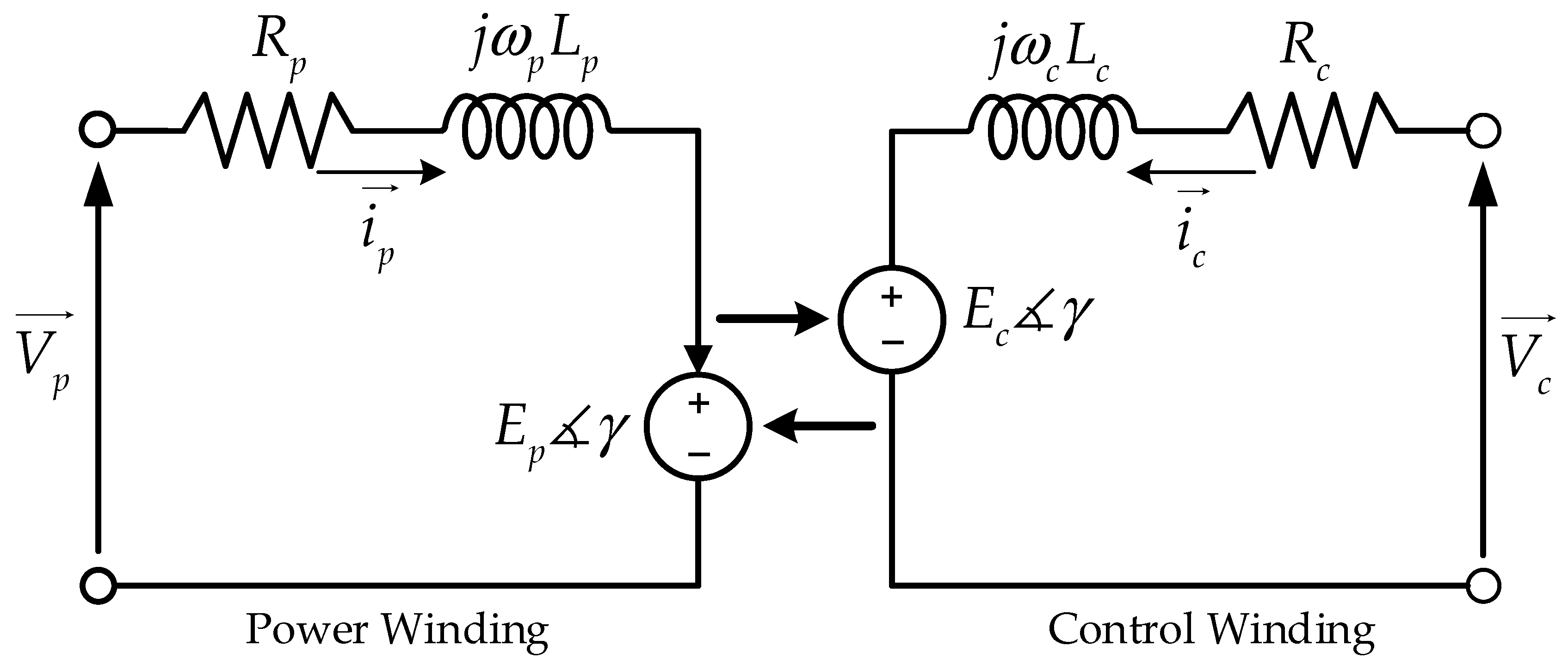
A Comprehensive Review on Brushless Doubly-Fed Reluctance Machine
Abstract
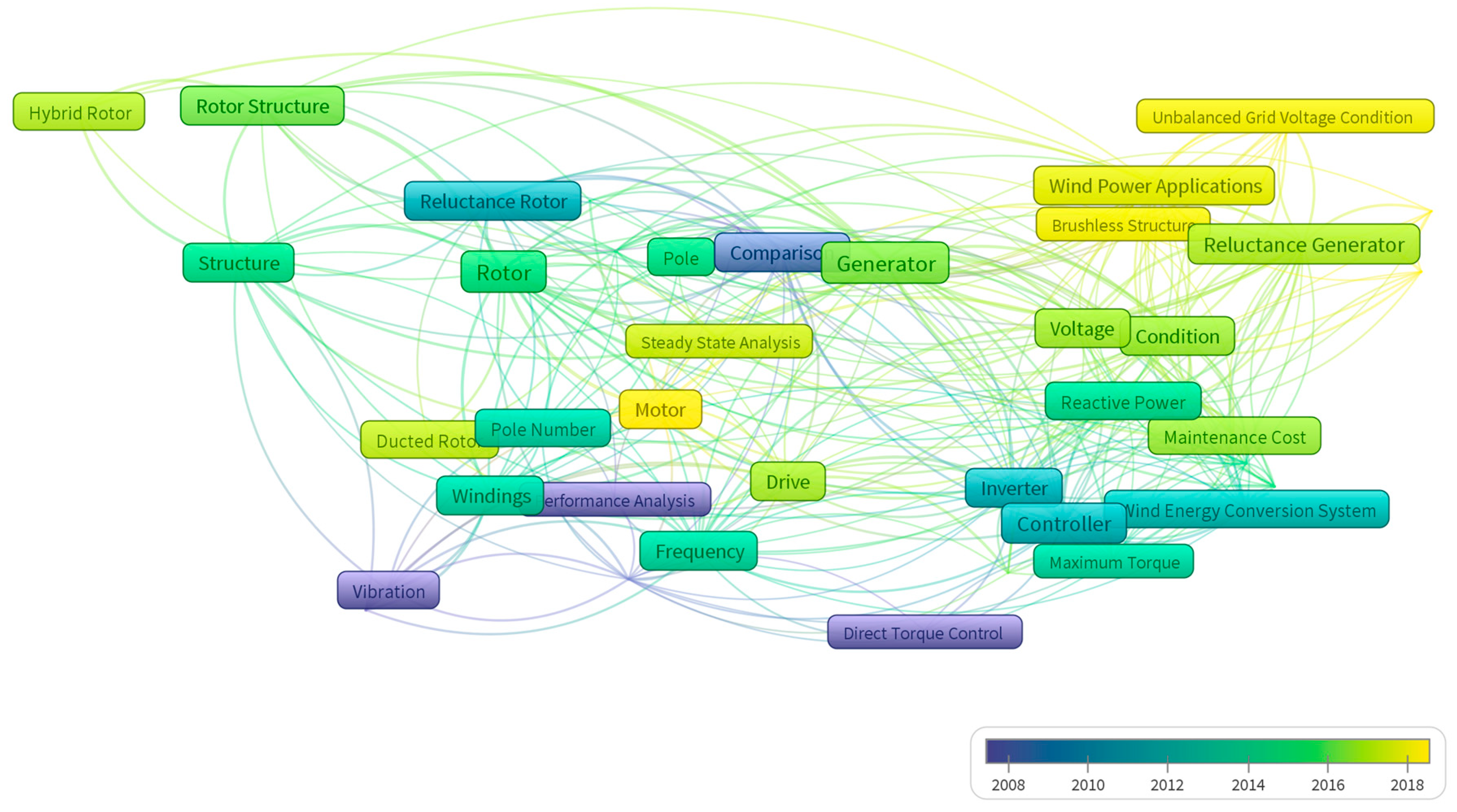
1. Introduction
- A comprehensive review is accomplished on all the previous works about BDFRM.
- Operation principle of BDFRM and its components are discussed.
- Detail characteristics of the previous research studies are presented.
- The research studies are compared in different aspects, such as machine type and machine size, etc.
- The conducted research studies in different fields, such as operation, design, and control of BDFRM, are reviewed.
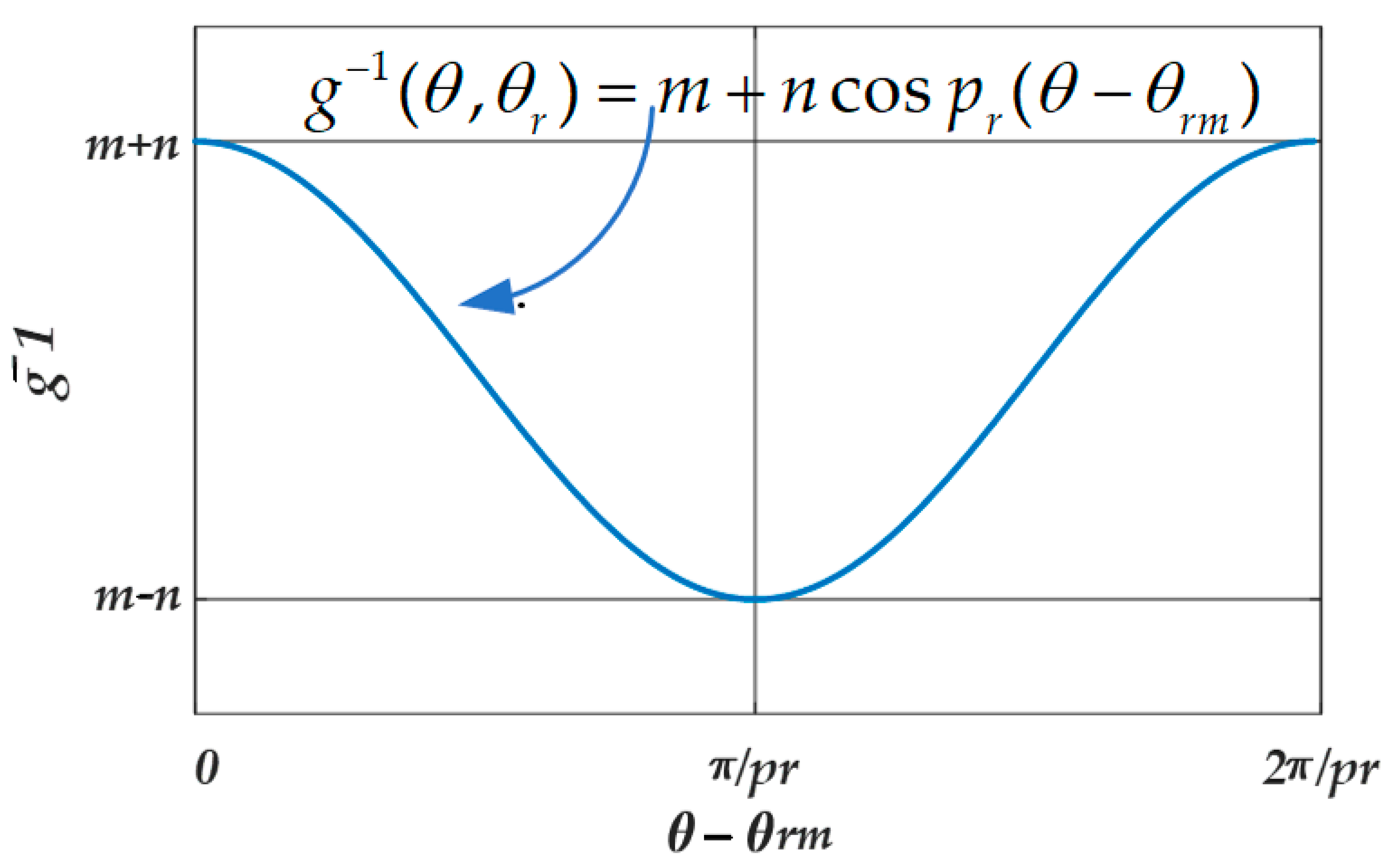
2. BDFRM Principles
- The iron permeability of the machine is infinite.
- The stator windings of the machine are assumed to be adequately modeled as spatially sinusoidally distributed.
- The power and control windings are three phases.
- The three-phase temporal current of the stator windings is ideal.
- The air gap of the machine is modeled using a sinusoidal air gap function.
3. Role of BDFRM in Decarbonization
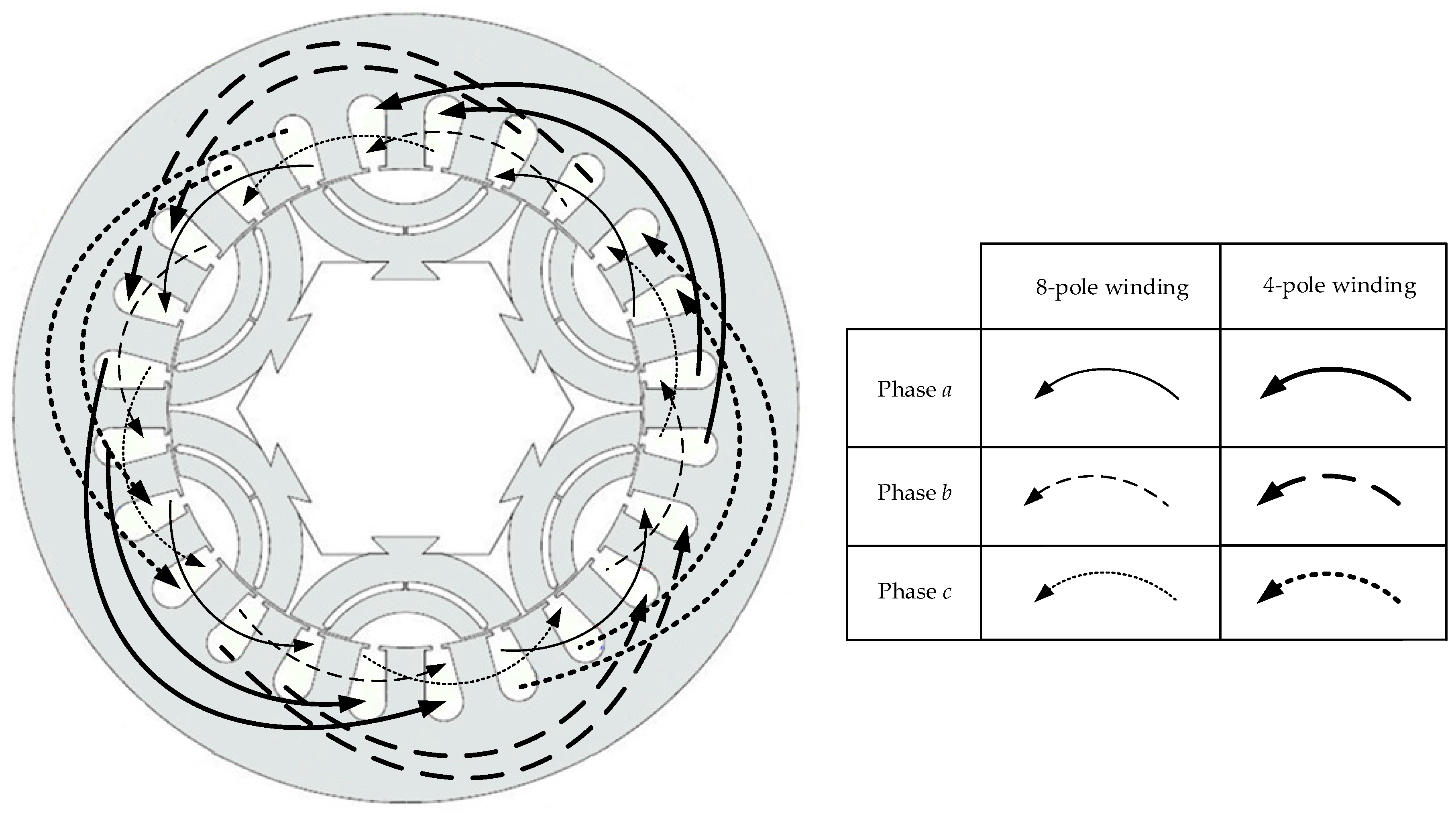
4. BDFRM Operation
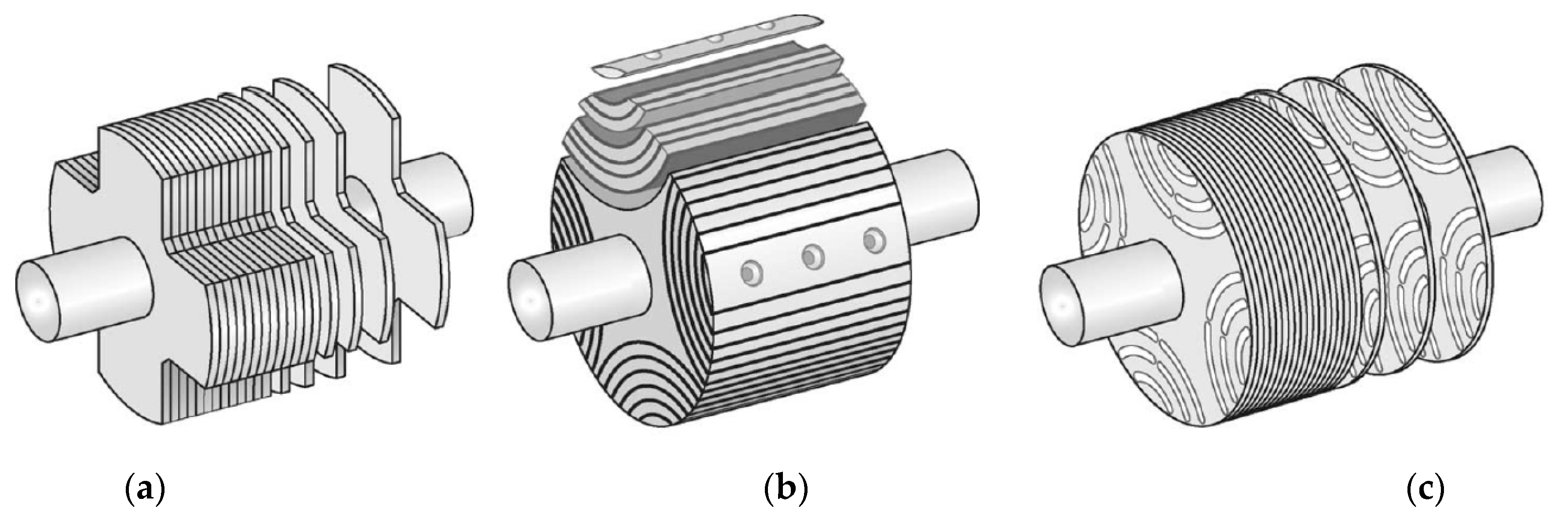
4.1. BDFRM Operation with Different Rotor Types
4.2. Operation in Different Conditions
4.3. Operation of Wind-Driven BDFRMs
4.4. Parameter Determination of BDFRM
4.5. BDFRM Dynamic Model
4.6. BDFRM Transient Model
4.7. Comparison with Other Machines
5. BDFRM Power Factor
6. Maximum Power Point Tracking for Generation Mode of BDFRM
7. BDFRM Losses
8. BDFRM Design
8.1. Special Designs
8.2. Rotor Design
8.3. Optimal Pole Combinations
9. BDFRM Control
9.1. Scalar-Based Approaches
9.2. Vector-Oriented Strategies
9.3. Open-Winding-Based Strategies
9.4. Direct Power Control-Based Strategies
9.5. Sensorless-Based Strategies
9.6. Model Reference Adaptive System-Based Strategies
9.7. Direct Torque Control-Based Approaches
9.8. Reactive Power Control-Based Approaches
9.9. Flux-and Voltage-Vector-Based Strategies
9.10. Multi-Input Controllers
9.11. Field-Oriented Approaches
9.12. Other Approaches
10. Future Directions
- (a)
- The operation principles of BDFRM can be more clarified and different operation modes can be more discussed.
- (b)
- Most of the research studies on BDFRM are accomplished in the field of BDFRM control, while other fields, such as design and operation have been somewhat ignored.
- (c)
- The provided results in the literature clearly show the excellence of BDFRM compared to BDFIM, while due to the design limits, development of BDFRM and its commercialization for practical application need more analyses.
- (d)
- Due to the lower maintenance requirement of BDFRM and other advantages of it, such as higher reliability, lower losses, and capability of attaining to higher speeds due to its robustness feature, the penetration level of such machines can increase in future.
- (e)
- Most of the previous research have been conducted for generating mode of BDFRM, i.e., BDFRG. The motoring mode of BDFRM, i.e., BDFRMT, needs to be thoroughly investigated since the application of BDFRM for pump drives has been widely stated in the literature.
11. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, L.; Cheng, W. Torque and Reactive Power Control of a Doubly Fed Induction Machine by Position Sensorless Scheme. IEEE Trans. Ind. Appl. 1995, 31, 636–642. [Google Scholar] [CrossRef]
- Chaal, H.; Jovanovic, M. Practical implementation of sensorless torque and reactive power control of doubly fed machines. IEEE Trans. Ind. Electron. 2012, 59, 2645–2653. [Google Scholar] [CrossRef]
- Wu, H. Performance Comparisons of Doubly-Fed Machines. Ph.D. Thesis, University of Northumbria, Newcastle, UK, 2013. [Google Scholar]
- Stefani, A.; Yazidi, A.; Rossi, C.; Filippetti, F.; Casadei, D.; Capolino, G.A. Doubly fed induction machines diagnosis based on signature analysis of rotor modulating signals. IEEE Trans. Ind. Appl. 2008, 44, 1711–1721. [Google Scholar] [CrossRef]
- Pena, R.; Clare, J.C.; Asher, G.M. Doubly fed induction generator using back-to-back PWM converters and its application to variablespeed wind-energy generation. IEE Proc. Electr. Power Appl. 1996, 143, 231–241. [Google Scholar] [CrossRef]
- Schulz, E.M.; Betz, R.E. Use of Doubly Fed Reluctance Machines in Wind Power Generation. In Proceedings of the 12th International Power Electronics and Motion Control Conference, Portoroz, Slovenia, 20 August–1 September 2006; pp. 1901–1906. [Google Scholar]
- Kunte, S.; Bhawalkar, M.P.; Gopalakrishnan, N.; Nerkar, Y.P. Design and analysis of brushless doubly fed reluctance machine suitable for wind generation applications. In Proceedings of the 18th International Conference on Electrical Machines and Systems, Pattaya, Thailand, 25–28 October 2015; pp. 2082–2088. [Google Scholar]
- Scian, I.; Dorrell, D.G.; Holik, P.J. Assessment of losses in a brushless doubly-fed reluctance machine. IEEE Trans. Magn. 2006, 42, 3425–3427. [Google Scholar] [CrossRef]
- Ademi, S.; Jovanović, M.G. Vector control methods for brushless doubly fed reluctance machines. IEEE Trans. Ind. Electron. 2015, 62, 96–104. [Google Scholar] [CrossRef]
- Agrawal, S.; Banerjee, A.; Beach, R.F. Brushless Doubly-Fed Reluctance Machine Drive for Turbo- Electric Distributed Propulsion Systems. In Proceedings of the AIAA/IEEE Electric Aircraft Technologies Symposium, Cincinnati, OH, USA, 12–14 July 2018; pp. 1–17. [Google Scholar]
- Tapia, A.; Tapia, G.; Ostolaza, J.X.; Saenz, J.R. Modeling and control of a wind turbine driven doubly fed induction generator. IEEE Trans. Energy Convers. 2003, 18, 194–204. [Google Scholar] [CrossRef]
- Li, H.; Chen, Z. Overview of different wind generator systems and their comparisons. IET Renew. Power Gener. 2008, 2, 123–138. [Google Scholar] [CrossRef]
- Zhang, F.; Yu, S.; Wang, H.; Wang, Y.; Wang, D. Overview of research and development status of brushless doubly-fed machine system. Chin. J. Electr. Eng. 2016, 2, 1–13. [Google Scholar] [CrossRef]
- Li, R.; Wallace, A.; Spee, R. Dynamic simulation of brushless doubly-fed machines. IEEE Trans. Energy Convers. 1991, 6, 445–452. [Google Scholar] [CrossRef]
- Jovanović, M.G. A Comparative Study of Control Strategies for Performance Optimisation of Brushless Doubly-Fed Reluctance Machines. J. Electr. Syst. 2006, 2, 208–255. [Google Scholar]
- Broadway, A.R.W. Cageless induction machine. Proc. Inst. Electr. Eng. 1971, 118, 1593–1600. [Google Scholar] [CrossRef]
- Song, W.K.; Dorrell, D.G. Modeling and simulation study for dynamic model of brushless doubly fed reluctance machine using matlab simulink. In Proceedings of the 3rd International Conference on Artificial Intelligence, Modelling and Simulation, Kota Kinabalu, Malaysia, 2–4 December 2016; pp. 382–386. [Google Scholar]
- Jovanović, M.G.; Betz, R.E. Slip power recovery systems based on brushless doubly fed reluctance machines. In Proceedings of the Power Conversion Conference—Osaka 2002, Osaka, Japan, 2–5 April 2002; Volume 3, pp. 987–992. [Google Scholar]
- Valenciaga, F. Second order sliding power control for a variable speed-constant frequency energy conversion system. Energy Convers. Manag. 2010, 51, 3000–3008. [Google Scholar] [CrossRef]
- Betz, R.E.; Jovanović, M.G. Theoretical analysis of control properties for the brushless doubly fed reluctance machine. IEEE Trans. Energy Convers. 2002, 17, 332–339. [Google Scholar] [CrossRef]
- Jovanovic, M.G. Power factor and inverter rating—A compromise in doubly fed reluctance machine drives. In Proceedings of the 8th International Conference on Power Electronics and Variable Speed Drives, London, UK, 18–19 September 2000; pp. 311–316. [Google Scholar]
- Jovanović, M.; Ademi, S.; Obichere, J.K. Comparisons of vector control algorithms for doubly-fed reluctance wind generators. In Transactions on Engineering Technologies: World Congress on Engineering and Computer Science 2014; Springer: Berlin/Heidelberg, Germany, 2015; pp. 85–99. ISBN 9789401772365. [Google Scholar]
- Sadeghian, O.; Oshnoei, A.; Khezri, R.; Hagh, M.T. Data clustering-based approach for optimal capacitor allocation in distribution systems including wind farms. IET Gener. Transm. Distrib. 2019, 13, 3397–3408. [Google Scholar] [CrossRef]
- Sadeghian, O.; Oshnoei, A.; Kheradmandi, M.; Khezri, R.; Mohammadi-Ivatloo, B. A robust data clustering method for probabilistic load flow in wind integrated radial distribution networks. Int. J. Electr. Power Energy Syst. 2020, 115, 105392. [Google Scholar] [CrossRef]
- Jovanovic, M. Sensored and sensorless speed control methods for brushless doubly fed reluctance motors. IET Electr. Power Appl. 2009, 3, 503–513. [Google Scholar] [CrossRef]
- Knight, A.M.; Betz, R.E.; Dorrell, D.G. Design and analysis of brushless doubly fed reluctance machines. IEEE Trans. Ind. Appl. 2013, 49, 50–58. [Google Scholar] [CrossRef]
- Staudt, T.; Wurtz, F.; Gerbaud, L.; Batistela, N.J.; Kuo-Peng, P. A New Approach on the Design and Optimization of Brushless Doubly-Fed Reluctance Machines. In Proceedings of the SGE 2014—Symposium de Génie Electrique, Cachan, France, 8–10 July 2014. [Google Scholar]
- Schulz, E.M.; Betz, R.E. Optimal torque per amp for brushless doubly fed reluctance machines. In Proceedings of the 14th IAS Annual Meeting, Conference Record of the 2005 Industry Applications Conference, Kowloon, Hong Kong, China, 2–6 October 2005; Volume 3, pp. 1749–1753. [Google Scholar]
- Valenciaga, F.; Puleston, P.F. Variable structure control of a wind energy conversion system based on a brushless doubly fed reluctance generator. IEEE Trans. Energy Convers. 2007, 22, 499–506. [Google Scholar] [CrossRef]
- Jovanović, M.G.; Yu, J.; Levi, E. A doubly-fed reluctance motor drive with sensorless direct torque control. In Proceedings of the IEEE International Electric Machines and Drives Conference, Madison, WI, USA, 1–4 June 2003; Volume 3, pp. 1518–1524. [Google Scholar]
- Chaal, H.; Jovanovic, M. Direct power control of brushless Doubly-Fed Reluctance Machines. In Proceedings of the 5th IET International Conference on Power Electronics, Machines and Drives, Brighton, UK, 19–21 April 2010; pp. 3–8. [Google Scholar]
- A Project Sponsored by The Engineering and Physical Sciences Research Council (EPSRC). Available online: https://www.ncl.ac.uk/engineering/research/eee/projects/performancecomparisonoftraditionalandemergingdoubly-fedgeneratortopologi.html (accessed on 16 November 2021).
- A Project Organized by The University of Newcastle. Available online: https://textarchive.ru/c-2497883-pall.html (accessed on 16 November 2021).
- Betz, R.E.; Jovanovic, M.G. Introduction to Brushless Doubly Fed Reluctance Machines—The Basic Equations; Aalborg University: Aalborg, Denmark, 1998. [Google Scholar]
- Han, P.; Cheng, M.; Ademi, S.; Jovanovic, M.G. Brushless doubly-fed machines: Opportunities and challenges. Chin. J. Electr. Eng. 2018, 4, 1–17. [Google Scholar] [CrossRef]
- Rihan, M.; Nasrallah, M.; Hasanin, B. Performance analysis of grid-integrated brushless doubly fed reluctance generator-based wind turbine: Modelling, control and simulation. SN Appl. Sci. 2020, 2, 1–9. [Google Scholar] [CrossRef]
- Tohidi, S.; Mohammadi Ivatloo, B. A comprehensive review of low voltage ride through of doubly fed induction wind generators. Renew. Sustain. Energy Rev. 2016, 57, 412–419. [Google Scholar] [CrossRef]
- Sadeghian, O.; Oshnoei, A.; Kheradmandi, M.; Mohammadi-Ivatloo, B. Optimal placement of multi-period-based switched capacitor in radial distribution systems. Comput. Electr. Eng. 2020, 82, 106549. [Google Scholar] [CrossRef]
- Valenciaga, F.; Evangelista, C.A. 2-Sliding active and reactive power control of a wind energy conversion system. IET Control Theory Appl. 2010, 4, 2479–2490. [Google Scholar] [CrossRef]
- Betz, R.E.; Jovanovic, M.G. The brushless doubly fed reluctance machine and the synchronous reluctance machine—A comparison. IEEE Trans. Ind. Appl. 2000, 36, 1103–1110. [Google Scholar] [CrossRef]
- Chaal, H.; Jovanovic, M. Toward a generic torque and reactive power controller for doubly fed machines. IEEE Trans. Power Electron. 2012, 27, 113–121. [Google Scholar] [CrossRef]
- Wang, F.; Zhang, F.; Xu, L. Parameter and performance comparison of doubly fed brushless machine with cage and reluctance rotors. IEEE Trans. Ind. Appl. 2002, 38, 1237–1243. [Google Scholar] [CrossRef]
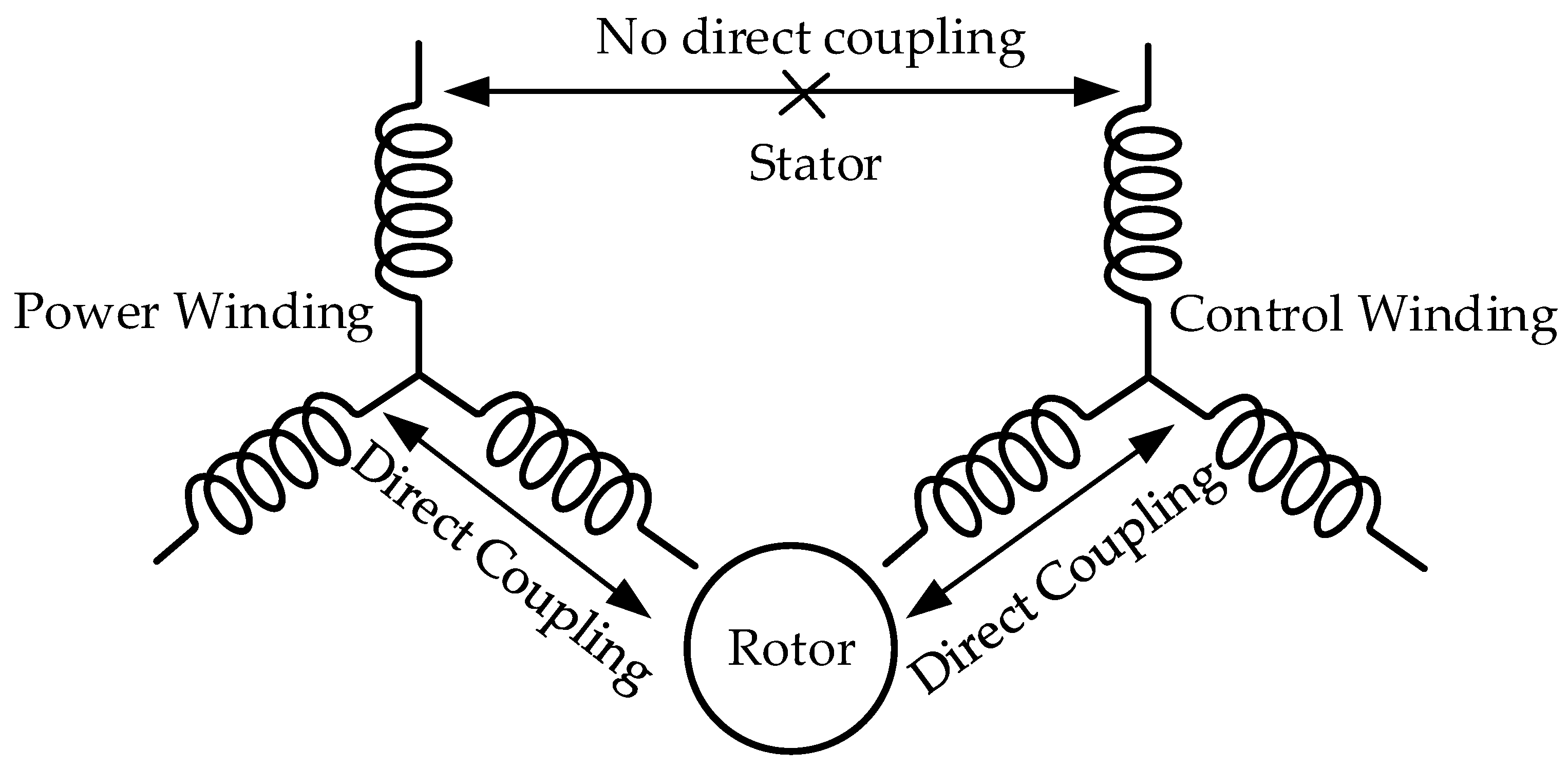
- Han, L.; Ou, X.; Du, J.; Han, X.; Guo, Y. Study of Direct Coupling in Stator Dual Windings of a Brushless Doubly Fed Machine. IEEE Trans. Energy Convers. 2017, 32, 974–982. [Google Scholar] [CrossRef]
- Han, P.; Zhang, J.; Cheng, M. Analytical Analysis and Performance Characterization of Brushless Doubly-Fed Machines with Multi-Barrier Rotors. IEEE Trans. Ind. Appl. 2019, 55, 5758–5767. [Google Scholar] [CrossRef]
- Rebeiro, R.S.; Knight, A.M. Characterization of a ducted rotor brushless doubly fed reluctance machine. IEEE Trans. Energy Convers. 2019, 34, 79–87. [Google Scholar] [CrossRef]
- Betz, R.E.; Jovanović, M.G. Introduction to the space vector modeling of the brushless doubly fed reluctance machine. Electr. Power Compon. Syst. 2003, 31, 729–755. [Google Scholar] [CrossRef]
- Kunte, S.; Bhawalkar, M.; Gopalakrishnan, N.; Nerkar, Y. Optimal design and comparative analysis of different configurations of brushless doubly fed reluctance machine. IEEJ J. Ind. Appl. 2017, 6, 370–380. [Google Scholar] [CrossRef][Green Version]
- Liao, Y.; Xu, L.; Zhen, L. Design of a doubly fed reluctance motor for adjustable-speed drives. IEEE Trans. Ind. Appl. 1996, 32, 1195–1203. [Google Scholar] [CrossRef]
- Khaliq, S.; Modarres, M.; Lipo, T.A.; Kwon, B. Design of Novel Axial-Flux Dual Stator Doubly Fed Reluctance Machine. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Taluo, T.; Ristic, L.; Jovanovic, M. Performance Analysis of Brushless Doubly Fed Reluctance Machines. In Proceedings of the 20th International Symposium on Power Electronics, Novi Sad, Serbia, 23–26 October 2019; pp. 1–6. [Google Scholar]
- Hassan, M.; Jovanovic, M. Improved scalar control using flexible DC-link voltage in Brushless Doubly-Fed Reluctance Machines for wind applications. In Proceedings of the 2nd International Symposium on Environment Friendly Energies and Applications, Newcastle upon Tyne, UK, 25–27 June 2012; pp. 482–487. [Google Scholar]
- Jovanović, M.G.; Betz, R.E.; Yu, J.; Levi, E. Aspects of vector and scalar control of brushless doubly fed reluctance machines. In Proceedings of the International Conference on Power Electronics and Drive Systems, Denpasar, Indonesia, 25 October 2001; Volume 2, pp. 461–467. [Google Scholar]
- Jovanović, M. Control of brushless doubly-fed reluctance motors. In Proceedings of the IEEE International Symposium on Industrial Electronics, Dubrovnik, Croatia, 20–23 June 2005; Volume 4, pp. 1667–1672. [Google Scholar]
- Liao, L.; Sun, C. A Novel Position Sensorless Control Scheme for Dyoubly Fed Reluctance Motor Drives. IEEE Trans. Ind. Appl. 1994, 30, 1210–1218. [Google Scholar] [CrossRef]
- Ojo, O.; Dong, G.; Omoigui, O. Analysis of a synchronous reluctance machine with an auxiliary single-phase winding. IEEE Trans. Ind. Appl. 2003, 39, 1307–1313. [Google Scholar] [CrossRef]
- Xu, L.; Wang, F. Comparative study of magnetic coupling for a doubly fed brushless machine with reluctance and cage rotors. In Proceedings of the Conference Record of the IEEE Industry Applications Conference Thirty-Second IAS Annual Meeting, New Orleans, LA, USA, 5–9 October 1997; Volume 1, pp. 326–332. [Google Scholar]
- Schulz, E.M.; Betz, R.E. Optimal Rotor Design for Brushless Doubly Fed Reluctance Machines. In Proceedings of the 38th IAS Annual Meeting on Conference Record of the Industry Applications Conference, Salt Lake City, UT, USA, 12–16 October 2003; Volume 1, pp. 256–261. [Google Scholar]
- Khaliq, S.; Atiq, S.; Lipo, T.A.; Kwon, B. Rotor Pole Optimization of Novel Axial-Flux Brushless Doubly Fed Reluctance Machine for Torque Enhancement. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
- El-Sayed, H.S.; El-Khouly, F.M.; Osheiba, A.M. Performance analysis and control of a brushless doubly fed reluctance machine. Electr. Power Compon. Syst. 2005, 33, 1105–1122. [Google Scholar] [CrossRef]
- Jovanović, M.; Chaal, H. Wind power applications of doubly-fed reluctance generators with parameter-free hysteresis control. Energy Convers. Manag. 2017, 134, 399–409. [Google Scholar] [CrossRef]
- Moazen, M.; Kazemzadeh, R.; Azizian, M.R. Model-based predictive direct power control of brushless doubly fed reluctance generator for wind power applications. Alex. Eng. J. 2016, 55, 2497–2507. [Google Scholar] [CrossRef]
- Jovanovic, M.; Ademi, S.; Llano, D.X. Control of Doubly-Fed Reluctance Machines without a Shaft Position or Speed Sensor. In Proceedings of the International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Amalfi, Italy, 20–22 June 2018; pp. 1245–1250. [Google Scholar]
- Staudt, T.; Wurtz, F.; Gerbaud, L.; Batistela, N.J.; Kuo-Peng, P. An optimization-oriented sizing model for brushless doubly fed reluctance machines: Development and experimental validation. Electr. Power Syst. Res. 2016, 132, 125–131. [Google Scholar] [CrossRef]
- Moazen, M.; Kazemzadeh, R.; Azizian, M.R. Power control of BDFRG variable-speed wind turbine system covering all wind velocity ranges. Int. J. Renew. Energy Res. 2016, 6, 477–486. [Google Scholar]
- Staudt, T.; Wurtz, F.; Batistela, N.J.; Kuo-Peng, P. Influence of rotor design and geometric parameter variation on global performance of Brushless Doubly-Fed Reluctance Machines. In Proceedings of the International Conference on Electrical Machines, Berlin, Germany, 2–5 September 2014; pp. 537–543. [Google Scholar]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. Maximum power tracking of a grid-connected wind-driven Brushless Doubly-Fed Reluctance Generator using scalar control. In Proceedings of the 8th GCC Conference and Exhibition, Muscat, Oman, 1–4 February 2015; pp. 1–6. [Google Scholar]
- Chaal, H.; Jovanovic, M. Flux observer algorithms for direct torque control of brushless doubly-fed reluctance machines. In Proceedings of the 35th Annual Conference of IEEE Industrial Electronics, Porto, Portugal, 3–5 November 2009; pp. 4440–4445. [Google Scholar]
- Kiran, K.; Das, S.; Singh, D. Model predictive field oriented speed control of brushless doubly-fed reluctance motor drive. In Proceedings of the IEEE International Conference on Power, Instrumentation, Control and Computing, Thrissur, India, 18–20 January 2018; pp. 1–6. [Google Scholar]
- Rebeiro, R.S.; Knight, A.M. Two-converters-based synchronous operation and control of a brushless doubly fed reluctance machine. IEEE Trans. Magn. 2018, 54, 1–5. [Google Scholar] [CrossRef]
- Xu, L.; Tang, Y.; Ye, L. Comparison Study of Rotor Structures of Doubly Excited Brushless Reluctance Machine by Finite Element Analysis. IEEE Trans. Energy Convers. 1994, 9, 165–172. [Google Scholar] [CrossRef]
- Fukami, T.; Momiyama, M.; Shima, K.; Hanaoka, R.; Takata, S. Steady-state analysis of a dual-winding reluctance generator with a multiple-barrier rotor. IEEE Trans. Energy Convers. 2008, 23, 492–498. [Google Scholar] [CrossRef]
- Knight, A.M.; Betz, R.E.; Dorrell, D. Design principles for brushless doubly fed reluctance machines. In Proceedings of the IECON 2011—37th Annual Conference of the IEEE Industrial Electronics Society, Melbourne, VIC, Australia, 7–10 November 2011; pp. 3602–3607. [Google Scholar]
- Dorrell, D.G.; Betz, R.E.; Jovanovic, M. Analysis of design variations in brushless double-fed reluctance generators for wind turbine applications. In Proceedings of the 14th International Power Electronics and Motion Control Conference EPE-PEMC, Ohrid, Macedonia, 6–8 September 2010; pp. 9–14. [Google Scholar]
- Dorrell, D.G.; Jovanovic, M. On the possibilities of using a brushless doubly-fed reluctance generator in a 2 MW wind turbine. In Proceedings of the IEEE Industry Applications Society Annual Meeting, Edmonton, AB, Canada, 5–9 October 2008; pp. 1–8. [Google Scholar]
- Kan, C.; Ren, T.; Hu, Y. Design and experimental analysis of a wound brushless doubly Fed machine based on a rotor with the reluctance effect. IET Electr. Power Appl. 2019, 13, 551–558. [Google Scholar] [CrossRef]
- Gay, D.; Betz, R.E.; Knight, A.; Dorrell, D. An explanation of asymmetric mutual inductances in brushless doubly fed reluctance machines. In Proceedings of the 21st European Conference on Power Electronics and Applications, Genova, Italy, 3–5 September 2019. [Google Scholar]
- Attya, A.B.; Ademi, S.; Jovanović, M.; Anaya-Lara, O. Frequency support using doubly fed induction and reluctance wind turbine generators. Int. J. Electr. Power Energy Syst. 2018, 101, 403–414. [Google Scholar] [CrossRef]
- Ademi, S.; Jovanovic, M.G.; Hasan, M. Control of Brushless Doubly-Fed Reluctance Generators for Wind Energy Conversion Systems. IEEE Trans. Energy Convers. 2015, 30, 596–604. [Google Scholar] [CrossRef]
- Bhawalkar, M.; Gopalakrishnan, N.; Nerkar, Y. Reactive power capability curve of brushless doubly fed reluctance generator. Wind Eng. 2019. [Google Scholar] [CrossRef]
- Ademi, S.; Jovanović, M. A novel sensorless speed controller design for doubly-fed reluctance wind turbine generators. Energy Convers. Manag. 2016, 120, 229–237. [Google Scholar] [CrossRef]
- Liang, F.; Xu, L.; Lipo, T.A. D-q analysis of a variable speed doubly ac excited reluctance motor. Electr. Mach. Power Syst. 1991, 19, 125–138. [Google Scholar] [CrossRef]
- Gay, D.; Betz, R.E.; Dorrell, D.; Knight, A. Brushless doubly fed reluctance machine testing for parameter determination. IEEE Trans. Ind. Appl. 2019, 55, 2611–2619. [Google Scholar] [CrossRef]
- Moazen, M.; Kazemzadeh, R.; Azizian, M.R. Mathematical modeling and analysis of brushless doubly fed reluctance generator under unbalanced grid voltage condition. Int. J. Electr. Power Energy Syst. 2016, 83, 547–559. [Google Scholar] [CrossRef]
- Hsieh, M.F.; Lin, I.H.; Dorrell, D.G. An analytical method combining equivalent circuit and magnetic circuit for BDFRG. IEEE Trans. Magn. 2014, 50, 1–5. [Google Scholar] [CrossRef]
- Xu, L.; Liang, F.; Lipo, T.A. Transient model of a doubly excited reluctance motor. IEEE Trans. Energy Convers. 1991, 6, 126–133. [Google Scholar] [CrossRef]
- Song, W.K.; Dorrell, D.G. Implementation of improved direct torque control method of brushless doubly-fed reluctance machines for wind turbine. In Proceedings of the IEEE International Conference on Industrial Technology, Busan, Korea, 26 February–1 March 2014; pp. 509–513. [Google Scholar]
- Dorrell, D.G.; Knight, A.M.; Song, W.K.; Betz, R.E. Saturation and ducting effects in a brushless doubly-fed reluctance machine. IEEE Trans. Magn. 2013, 49, 3933–3936. [Google Scholar] [CrossRef]
- Kumar, M.; Das, S.; Kiran, K. Sensorless Speed Estimation of Brushless Doubly-Fed Reluctance Generator Using Active Power Based MRAS. IEEE Trans. Power Electron. 2019, 34, 7878–7886. [Google Scholar] [CrossRef]
- Kumar, M.; Das, S. Model reference adaptive system based sensorless speed estimation of brushless doubly-fed reluctance generator for wind power application. IET Power Electron. 2018, 11, 2355–2366. [Google Scholar] [CrossRef]
- Rebeiro, R.S.; Knight, A.M. Operating limits of a Brushless Doubly Fed Reluctance Machine driven by two converters. In Proceedings of the IEEE Energy Conversion Congress and Exposition, Montreal, QC, Canada, 20–24 September 2015; pp. 3872–3877. [Google Scholar]
- Rebeiro, R.S.; Knight, A.M. Comparison of operating modes for a brushless doubly fed reluctance motor drive. In Proceedings of the IEEE Energy Conversion Congress and Exposition, Cincinnati, OH, USA, 1–5 October 2017; pp. 1323–1330. [Google Scholar]
- Guo, X.; Wu, S.; Fu, W.; Liu, Y.; Wang, Y.; Zeng, P. Control of a Dual-Stator Flux-Modulated Motor for Electric Vehicles. Energies 2016, 9, 517. [Google Scholar] [CrossRef]
- Song, W.K.; Dorrell, D.G.; Knight, A.M.; Betz, R.E.; Gay, D. Operating limits and practical operation of a brushless doubly-fed reluctance machine. In Proceedings of the IEEE Energy Conversion Congress and Exposition, Cincinnati, OH, USA, 1–5 October 2017; pp. 5846–5852. [Google Scholar]
- Kiran, K.; Das, S. Variable Speed Operation of Brushless Doubly Fed Reluctance Machine Drive Using Model Predictive Current Control Technique. IEEE Trans. Power Electron. 2020, 35, 8396–8404. [Google Scholar] [CrossRef]
- Zhang, F.; Wang, F.; Grünberger, H.P.; Nolle, E. The structure and adjusting speed characteristics of new type of Doubly-fed brushless machine with ALA rotor. Eur. Trans. Electr. Power 2006, 16, 409–421. [Google Scholar] [CrossRef]
- Dorrell, D.G.; Knight, A.M.; Betz, R.E. Improvements in brushless doubly fed reluctance generators using high-flux-density steels and selection of the correct pole numbers. IEEE Trans. Magn. 2011, 47, 4092–4095. [Google Scholar] [CrossRef]
- Gay, D.; Betz, R.; Dorrell, D.; Knight, A. Brushless Doubly Fed Reluctance Machine Parameter Determination. In Proceedings of the 20th International Conference on Electrical Machines and Systems, Sydney, NSW, Australia, 11–14 August 2017; pp. 1–6. [Google Scholar]
- Schulz, E.M.; Betz, R.E. Impact of winding and gap flux density harmonics on Brushless Doubly Fed Reluctance machines. In Proceedings of the Second International Conference on Power Electronics, Machines and Drives, Edinburgh, UK, 31 March–2 April 2004; Volume 2, pp. 487–491. [Google Scholar]
- Rigatos, G.; Siano, P.; Ademi, S. Flatness-based adaptive fuzzy control of brushless doubly-fed reluctance machines. In Proceedings of the IEEE 26th International Symposium on Industrial Electronics, Edinburgh, UK, 19–21 June 2017; pp. 1913–1920. [Google Scholar]
- Ibrahim, A.K.; Marei, M.I.; El-goharey, H.S. Transient Model of Brushless Doubly Fed Reluctance Machine using ABC Reference Frame. Int. J. Recent Trends Eng. Res. 2018, 4, 26–32. [Google Scholar] [CrossRef]
- Logan, T.; McMahon, R.; Seffen, K. Noise and vibration in brushless doubly fed machine and brushless doubly fed reluctance machine. IET Electr. Power Appl. 2014, 8, 50–59. [Google Scholar] [CrossRef]
- Agrawal, S.; Province, A.; Banerjee, A. An Approach to Maximize Torque Density in a Brushless Doubly-fed Reluctance Machine. In Proceedings of the IEEE Energy Conversion Congress and Exposition, Baltimore, MD, USA, 29 September–3 October 2019; pp. 298–305. [Google Scholar]
- Rebeiro, R.S.; Knight, A.M. Design and torque capability of a ducted rotor brushless doubly fed reluctance machine. IET Electr. Power Appl. 2018, 12, 1058–1064. [Google Scholar] [CrossRef]
- Hsieh, M.F.; Chang, Y.H.; Dorrell, D.G. Design and Analysis of Brushless Doubly Fed Reluctance Machine for Renewable Energy Applications. IEEE Trans. Magn. 2016, 52. [Google Scholar] [CrossRef]
- Rebeiro, R.S.; Knight, A.M. Two-Converter-Based Frequency-Sharing Operation and Control of a Brushless Doubly Fed Reluctance Motor Drive. IEEE Trans. Ind. Appl. 2019, 55, 5873–5880. [Google Scholar] [CrossRef]
- Kunte, S.; Bhawalkar, M.P.; Gopalakrishnan, N.; Nerkar, Y.P.; Vaidya, G.A. Selection of suitable pole configuration of BDFRM for variable speed applications. In Proceedings of the IEEE International WIE Conference on Electrical and Computer Engineering, Pune, India, 19–21 December 2017; pp. 208–211. [Google Scholar]
- Knight, A.M.; Betz, R.E.; Song, W.K.; Dorrell, D.G. Brushless doubly-fed reluctance machine rotor design. In Proceedings of the IEEE Energy Conversion Congress and Exposition, Raleigh, NC, USA, 15–20 September 2012; pp. 2308–2315. [Google Scholar]
- Saeed, M.S.R.; Mohamed, E.E.M. Partitioned stator doubly-fed brushless reluctance machine for wind generating systems. In Proceedings of the 19th International Middle-East Power Systems Conference, Cairo, Egypt, 19–21 December 2018; pp. 864–869. [Google Scholar]
- Jovanovic, M. Brushless doubly fed reluctance machines: An emerging technology. Int. J. Emerg. Electr. Power Syst. 2007, 8. [Google Scholar] [CrossRef]
- Jovanović, M.G.; Betz, R.E.; Yu, J. The use of doubly fed reluctance machines for large pumps and wind turbines. IEEE Trans. Ind. Appl. 2002, 38, 1508–1516. [Google Scholar] [CrossRef]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. A comparative study of vector-control strategies for maximum wind-power extraction of a grid-connected wind-driven brushless doubly-fed reluctance generator. Aust. J. Electr. Electron. Eng. 2017, 14, 1–11. [Google Scholar] [CrossRef]
- Ademi, S.; Jovanovic, M. High-efficiency control of brushless doubly-fed machines for wind turbines and pump drives. Energy Convers. Manag. 2014, 81, 120–132. [Google Scholar] [CrossRef]
- Hargreaves, N.B.; Pantea, S.M.; Taylor, G.A. Control of Emerging Brushless Doubly-Fed Reluctance Wind Turbine Generators. In Large Scale Renewable Power Generation; Springer: Singapore, 2014; pp. 395–411. ISBN 978-981-4585-29-3. [Google Scholar]
- Ademi, S.; Jovanovic, M. Vector control strategies for brushless doubly-fed reluctance wind generators. In Proceedings of the 2nd International Symposium on Environment Friendly Energies and Applications, Newcastle upon Tyne, UK, 25–27 June 2012; pp. 44–49. [Google Scholar]
- Jin, S.; Zhang, F.; Fan, S.; Zhu, L.; Cao, W. Power decoupling control of open-winding brushless doubly-fed reluctance machine for wind power generator application. Int. J. Control. Autom. 2015, 8, 173–182. [Google Scholar] [CrossRef][Green Version]
- Zhang, F.; Zhu, L.; Jin, S.; Cao, W.; Wang, D.; Kirtley, J.L. Developing a new SVPWM control strategy for open-winding brushless doubly-fed reluctance generators. IEEE Trans. Ind. Appl. 2015, 51, 4567–4574. [Google Scholar] [CrossRef]
- Zhang, F.; Zhu, L.; Jin, S.; Su, X.; Ademi, S.; Cao, W. Controller Strategy for Open-Winding Brushless Doubly Fed Wind Power Generator with Common Mode Voltage Elimination. IEEE Trans. Ind. Electron. 2019, 66, 1098–1107. [Google Scholar] [CrossRef]
- Jin, S.; Shi, L.; Zhu, L.; Cao, W.; Dong, T.; Zhang, F. Dual Two-Level Converters Based on Direct Power Control for an Open-Winding Brushless Doubly-Fed Reluctance Generator. IEEE Trans. Ind. Appl. 2017, 53, 3898–3906. [Google Scholar] [CrossRef]
- Chaal, H.; Jovanovic, M. Power control of brushless doubly-fed reluctance drive and generator systems. Renew. Energy 2012, 37, 419–425. [Google Scholar] [CrossRef]
- Kiran, K.; Das, S. Implementation of reactive power-based MRAS for sensorless speed control of brushless doubly fed reluctance motor drive. IET Power Electron. 2018, 11, 192–201. [Google Scholar] [CrossRef]
- Kiran, K.; Das, S.; Kumar, M.; Sahu, A. Sensorless Speed Control of Brushless Doubly-Fed Reluctance Motor Drive using Secondary Flux based MRAS. Electr. Power Compon. Syst. 2018, 46, 701–715. [Google Scholar] [CrossRef]
- Agha Kashkooli, M.R.; Jovanovic, M.G.; Ademi, S. Sensorless power control of doubly-fed reluctance wind turbine generators using a current-based MRAS estimator. In Proceedings of the International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Sorrento, Italy, 24–26 June 2020; pp. 65–70. [Google Scholar]
- Jovanović, M.G.; Yu, J.; Levi, E. Encoderless direct torque controller for limited speed range applications of brushless doubly fed reluctance motors. IEEE Trans. Ind. Appl. 2006, 42, 712–722. [Google Scholar] [CrossRef]
- Jovanovic, M.G.; Yu, J.; Levi, E. Direct torque control of brushless doubly fed reluctance machines. Electr. Power Compon. Syst. 2004, 32, 941–958. [Google Scholar] [CrossRef]
- Jovanović, M.; Yu, J.; Levi, E. A direct torque controller for limited speed range applications of brushless doubly-fed reluctance motors. In Proceedings of the Conference Record of the 2004 IEEE Industry Applications Conference, 39th IAS Annual Meeting, Seattle, WA, USA, 3–7 October 2004; Volume 4, pp. 2403–2410. [Google Scholar]
- Song, W.K.; Dorrell, D.G. Improved direct torque control method of brushless doubly-fed reluctance machines for wind turbine. In Proceedings of the IEEE International Symposium on Industrial Electronics, Taipei, Taiwan, 28–31 May 2013; pp. 1–5. [Google Scholar]
- Jovanović, M.G.; Yu, J.; Levi, E. Real-time implementation of direct torque control scheme for brushless doubly-fed reluctance motors. In Proceedings of the Second International Conference on Power Electronics, Machines and Drives, Edinburgh, UK, 31 March–2 April 2004; Volume 2, pp. 752–757. [Google Scholar]
- Chaal, H.; Jovanovic, M.; Busawon, K. Sliding mode observer based direct torque control of a brushless doubly-fed reluctance machine. In Proceedings of the IEEE Symposium on Industrial Electronics and Applications, Kuala Lumpur, Malaysia, 4–6 October 2009; Volume 2, pp. 866–871. [Google Scholar]
- Jovanović, M.G.; Dorrell, D.G. Sensorless control of brushless doubly-fed reluctance machines using an angular velocity observer. In Proceedings of the International Conference on Power Electronics and Drive Systems, Bangkok, Thailand, 27–30 November 2007; pp. 717–724. [Google Scholar]
- Ademi, S.; Jovanovic, M. Control of doubly-fed reluctance generators for wind power applications. Renew. Energy 2016, 85, 171–180. [Google Scholar] [CrossRef]
- Ademi, S.; Jovanovic, M.G.; Chaal, H.; Cao, W. A New Sensorless Speed Control Scheme for Doubly Fed Reluctance Generators. IEEE Trans. Energy Convers. 2016, 31, 993–1001. [Google Scholar] [CrossRef]
- Sahu, A.; Kiran, K.; Das, S. Particle Swarm Optimization based tuning of brushless doubly-fed reluctance machine drive for speed control applications. In Proceedings of the 1st IEEE International Conference on Power Electronics, Intelligent Control and Energy Systems, Delhi, India, 4–6 July 2017; pp. 1–6. [Google Scholar]
- Jovanović, M.G.; Betz, R.E. Power factor control using brushless doubly fed reluctance machines. In Proceedings of the Thirty-Fifth IAS Annual Meeting and World Conference on Industrial Applications of Electrical Energy, Rome, Italy, 8–12 October 2000; Volume 1, pp. 523–530. [Google Scholar]
- Bhowmik, S.; Spec, R.; Enslin, J.H.R. Performance optimization for doubly fed wind power generation systems. IEEE Trans. Ind. Appl. 1999, 35, 949–958. [Google Scholar] [CrossRef]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. Sensored and sensorless scalar-control strategy of a wind-driven BDFRG for maximum wind-power extraction. J. Control. Decis. 2018, 5, 209–227. [Google Scholar] [CrossRef]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. Maximum power extraction under different vector-control schemes and grid-synchronization strategy of a wind-driven Brushless Doubly-Fed Reluctance Generator. ISA Trans. 2018, 72, 287–297. [Google Scholar] [CrossRef] [PubMed]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. Vector control strategy for maximum wind-power extraction of a grid-connected wind-driven Brushless Doubly-Fed Reluctance Generator. In Proceedings of the 4th International Conference on Electric Power and Energy Conversion Systems, Sharjah, UAE, 24–26 November 2015; pp. 1–6. [Google Scholar]
- Mousa, M.G.; Allam, S.M.; Rashad, E.M. Maximum wind-power extraction of a grid-connected wind-driven brushless Doubly-Fed Reluctance Generator under different vector-control strategies. In Proceedings of the 22nd International Conference on Electrical Machines, Lausanne, Switzerland, 4–7 September 2016; pp. 1105–1111. [Google Scholar]
- Dorrell, D.G.; Knight, A.M.; Betz, R.E. Issues with the design of brushless doubly-fed reluctance machines: Unbalanced magnetic pull, skew and iron losses. In Proceedings of the IEEE International Electric Machines and Drives Conference, Niagara Falls, ON, Canada, 15–18 May 2011; pp. 663–668. [Google Scholar]
- Xu, L.; Zhen, L.; Kim, E.H. Field orientation control of a doubly excited brushless reluctance machine. In Proceedings of the Conference Record of the IEEE Industry Applications Conference Thirty-First IAS Annual Meeting, San Diego, CA, USA, 6–10 October 1996; Volume 1, pp. 319–325. [Google Scholar]
- Sadeghian, O.; Oshnoei, A.; Tarafdar-Hagh, M.; Khezri, R. A clustering-based technoeconomic analysis for wind farm and shunt capacitor allocation in radial distribution systems. Int. Trans. Electr. Energy Syst. 2020, e12708. [Google Scholar] [CrossRef]
- Sadeghian, O.; Nazari-Heris, M.; Abapour, M.; Taheri, S.S.; Zare, K. Improving reliability of distribution networks using plug-in electric vehicles and demand response. J. Mod. Power Syst. Clean Energy 2019, 7, 1189–1199. [Google Scholar] [CrossRef]
- Ahrabi, M.; Abedi, M.; Nafisi, H.; Mirzaei, M.A.; Mohammadi-Ivatloo, B.; Marzband, M. Evaluating the effect of electric vehicle parking lots in transmission-constrained AC unit commitment under a hybrid IGDT-stochastic approach. Int. J. Electr. Power Energy Syst. 2021, 125, 106546. [Google Scholar] [CrossRef]
- Betz, R.E.; Jovanovic, M.G. Brushless Doubly Fed Reluctance Machines—A Tutorial. Available online: http://citeseerx.ist.psu.edu/viewdoc/download;jsessionid=A3A97ADF20F08F33FBE4EC88F8BB5029?doi=10.1.1.565.8777&rep=rep1&type=pdf (accessed on 16 November 2021).
- Hsieh, M.F.; Lin, I.H.; Dorrell, D. Magnetic circuit modeling of brushless doubly-fed machines with induction and reluctance rotors. IEEE Trans. Magn. 2013, 49, 2359–2362. [Google Scholar] [CrossRef]
- Jovanovic, M.; Betz, R.E. Optimal performance of brushless doubly fed reluctance machines. In Proceedings of the 9th International Conference on Electrical Machines and Drives, Canterbury, UK, 1–3 September 1999; pp. 386–390. [Google Scholar]
- Mehdipour, C.; Mohammadi, F.; Mehdipour, I. Analytical Approach to Nonlinear Behavior Study of an Electric Vehicle. In Proceedings of the 6th International Conference on Control, Instrumentation and Automation, Sanandaj, Iran, 30–31 October 2020; pp. 1–7. [Google Scholar]
- Liangzhou, J.; Xiangyu, Y. Optimization design on salient pole rotor of BDFRM using the Taguchi method. In Proceedings of the 4th International Conference on Power Electronics Systems and Applications, Hong Kong, China, 8–10 June 2011; pp. 1–4. [Google Scholar]
- Jovanović, M.G.; Yu, J.; Levi, E. A review of control methods for brushless doubly-fed reluctance machines. In Proceedings of the International Conference on Power Electronics, Machines and Drives, Sante Fe, NM, USA, 4–7 June 2002; pp. 528–533. [Google Scholar]



| Machine Technology | Structure of DFM |
|---|---|
| DFM | Using partially-rated converters |
| BDFM | Using partially-rated converters Brush and slip-ring removal |
| BDFRM | Using partially-rated converters Brush and slip-ring removal Using reluctance-rotor |
| Reference | Operation Mode | Numerical Simulation | Simulation Software | Experimental Test | MachineSize | Field | Analyzing Method | 2, 2, |
|---|---|---|---|---|---|---|---|---|
| [6] | Generator | ✘ | ✘ | ✔ | 7.5 kW | Operation | Finite Element Analysis | Ψ |
| [7] | Generator | ✘ | ✘ | ✔ | 2 kW | Design | Finite Element Analysis | 6, 2, 4 |
| [8] | Motor | ✔ | Ψ | ✔ | 7.5 KW | Losses | Finite Element Analysis | 6, 2, 4 |
| [9] | Both Modes | ✘ | ✘ | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [10] | Motor | ✔ | ANSYS Maxwell | ✔ | 1.5 MW | Design | Finite Element Analysis | 8, 4, 6 |
| [15] | Overall | ✘ | ✘ | ✔ | Ψ | Control/Power Factor/Losses | Space Vector Theory | :4 , : several cases |
| [17] | Motor | ✔ | MATLAB/Simulink | ✘ | Ψ | Operation | Space Vector Theory | 8, 4, 6 |
| [19] | Generator | ✔ | Ψ | ✘ | 50 hp | Control | Reduced Order Model | Ψ |
| [22] | Generator | ✔ | MATLAB/Simulink | ✔ | Sim: 2 MW Test: 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [25] | Motor | ✘ | ✘ | ✔ | 1.0375 W | Control | Space Vector Theory | 6, 2, 4 |
| [26] | Motor | ✔ | JMAG Designer | ✘ | 16 kW | Design | Finite Element Analysis | 8, 6, 4 |
| [27] | Generator | ✔ | Cades/RelucTool | ✘ | Ψ | Design | Finite Element Analysis | 2, 6, 4 |
| [28] | Motor | ✔ | ANSYS Maxwell | ✘ | 7.5 kW | Operation/Control | Finite Element Analysis | 6, 2, 4 |
| [29] | Generator | ✔ | Ψ | ✘ | 5 hp | Control, Losses | Dynamic Analysis | 6, 2, 4 |
| [30] | Motor | ✔ | MATLAB/Simulink | ✘ | 5 hp | Control | Theoretical Analysis | 6, 2, 4 |
| [31] | Generator | ✔ | MATLAB/Simulink | ✘ | 4.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [36] | Generator | ✔ | MATLAB/Simulink | ✘ | 6 kW | Control | Dynamic Analysis | Ψ |
| [39] | Generator | ✔ | Ψ | ✘ | 37 kW | Control, MPPT | Dynamic Analysis | : 4 |
| [40] | ✘ | ✘ | ✘ | ✘ | ✘ | Operation/Losses | Space Vector Theory | 6, 2, 4 |
| [41] | Motor | ✔ | MATLAB/Simulink | ✔ | 4 kW | Control | Space Vector Theory | 6, 2, 4 |
| [42] | Motor | ✘ | ✘ | ✔ | 4 kW | Operation | Steady-State Analysis | 6, 2, 4 |
| [44] | Motor | ✔ | Ψ | ✔ | 1.5 kW | Operation | Finite Element Analysis | 8, 4, 6 |
| [45] | Generator | ✔ | Ψ | ✘ | 4.5 kW | Operation | Space Vector Theory | 6, 2, 4 |
| [47] | Motor | ✔ | MAXWELL | ✔ | 2 kW | Operation | Finite Element Analysis | 8, 4, 6 & 6, 2, 4 |
| [48] | Motor | ✘ | ✘ | ✔ | 2 hp | Design | Finite Element Analysis | 2, 6, 4 |
| [49] | Ψ | ✔ | ANSYS MAXWELL | ✘ | 16 kW | Design | Finite Element Analysis | 4, 8, 6 |
| [50] | Both Modes | ✔ | MATLAB/Simulink | ✘ | 2 MW | Control | Space Vector Theory | 6, 2, 4 |
| [51] | Generator | ✔ | MATLAB/Simulink | ✘ | Ψ | Control | Space Vector Theory | Ψ |
| [52] | Motor | ✔ | MATLAB/Simulink | ✘ | 5 hp | Control | Space Vector Theory | 2, 6, 4 |
| [53] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [54] | Motor | ✔ | ACSL | ✔ | 2 hp | Control | Theoretical Analysis | 2, 6, 4 |
| [55] | Both Modes | ✘ | ✘ | ✔ | 5 KVA | Operation | Dynamic and Steady-State Analysis | 8, 4, 6 |
| [57] | Overall | ✔ | Ψ | ✘ | Ψ | Design/Power Factor | Finite Element Analysis | 6, 2, 4 |
| [58] | Ψ | ✔ | Ψ | ✔ | 1 hp | Operation | Finite Element Analysis | 4, 8, 6 |
| [59] | Motor | ✔ | MATLAB/Simulink | ✘ | 0.75 hp | Operation | Dynamic and Steady-State Analysis | 6, 2, 4 |
| [60] | Generator | ✘ | ✘ | ✔ | 1.5 kW | Operation/Control | Space Vector Theory | 6, 2, 4 |
| [61] | Generator | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Operation | Space Vector Theory | 6, 2, 4 |
| [62] | Generator | ✘ | ✘ | ✔ | 1.6 kW | Control | Space Vector Theory | 6, 2, 4 |
| [63] | Generator | ✔ | CADES | ✔ | 1 kW | Design | Finite Element Analysis | 8, 4, 6 |
| [64] | Generator | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Operation/MPPT | Space Vector Theory | 6, 2, 4 |
| [65] | Overall | ✔ | Ψ | ✘ | 1 kW | Design | Finite Element Analysis | 8, 4, 6 |
| [66] | Generator | ✔ | Ψ | ✘ | 4.5 kW | Control, MPPT | Space Vector Theory | 6, 2, 4 |
| [67] | Ψ | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [68] | Motor | ✔ | MATLAB/Simulink | ✘ | 1.2 kW | Operation/Control | Space Vector Theory | 4, 6, 2 |
| [69] | Motor | ✔ | JMAG Designer | ✔ | 630 W | Operation | Finite Element Analysis | 8, 4, 6 |
| [70] | Generator | ✔ | Ψ | ✘ | 5 hp | Operation | Finite Element Analysis | 2, 4, 3 |
| [72] | Ψ | ✔ | Ψ | ✘ | 50 kW | Design | Steady-State Analysis | 8, 4, 6 |
| [73] | Generator | ✔ | Ψ | ✘ | 2 MW | Design | Finite Element Analysis | 6, 2, 4 |
| [74] | Generator | ✔ | Ψ | ✔ | 2 MW | Design | Finite Element Analysis | 8, 4, 6 |
| [76] | Ψ | ✔ | Ψ | ✘ | Ψ | Operation | Finite Element Analysis | 8, 4, 6 |
| [77] | Generator | ✔ | MATLAB/Simulink | ✘ | 1.5 MW | Operation | Space Vector Theory | 8, 4, 6 |
| [78] | Generator | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control/MPPT | Space Vector Theory | 6, 2, 4 |
| [79] | Generator | ✔ | Ψ | ✔ | 2 kW | Operation | FEA | 8, 4, 6 |
| [80] | Generator | ✘ | MATLAB/Simulink | ✔ | 1.6 kW | Control | Space Vector Theory | 6, 2, 4 |
| [81] | Motor | ✔ | Ψ | ✘ | Ψ | Operation | Electromagnetic Coupling | 6, 2, 4 |
| [82] | Ψ | ✔ | Ψ | ✔ | 16 kW | Operation | Finite Element Analysis | 8, 4, 6 |
| [83] | Generator | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Operation | Space Vector Theory | 6, 2, 4 |
| [84] | Generator | ✔ | ANSYS Maxwell | ✔ | Ψ | Design | MCM, FEA | 4, 8, 6 |
| [85] | Motor | ✔ | Ψ | ✔ | 15 hp | Operation | Space Vector Theory | 8, 4, 6 |
| [86] | Generator | ✔ | Ψ | ✔ | 20 kW | Control | Space Vector Theory | 8, 4, 6 |
| [87] | Generator | ✔ | SPEED PC-FEA | ✘ | 2 MW | Operation | Finite Element Analysis | 8, 4, 6 |
| [88] | Generator | ✔ | MATLAB/Simulink | ✔ | 1.6 kW | Control/Losses | Space-Phasor Model | 6, 2, 4 |
| [89] | Generator | ✔ | MATLAB/Simulink | ✔ | 1.2 kW | Control | Space-Phasor Model | 6, 2, 4 |
| [90] | Motor | ✔ | JMAG Designer | ✔ | 950 W | Operation | Finite Element Analysis | 8, 4, 6 |
| [91] | Motor | ✔ | JMAG Designer | ✔ | 630 W | Operation | Finite Element Analysis | 8, 4, 6 |
| [92] | Motor | ✔ | MATLAB/Simulink | ✔ | Ψ | Electric Vehicles | Theoretical Analysis | 12, 10, 11 |
| [93] | Motor | ✔ | MATLAB/Simulink | ✔ | 42 kW | Operation | Finite Element Analysis | 4, 6, 8 |
| [94] | Both Modes | ✔ | MATLAB/Simulink | ✔ | 1.6 kW | Operation | Dynamic Analysis | 6, 2, 4 |
| [95] | Motor | ✔ | Ψ | ✔ | Ψ | Operation | Field Modulation Theory | 6, 2, 4 |
| [96] | Generator | ✔ | SPEED PC-FEA | ✘ | 200 kW | Operation/Design | Finite Element Analysis | 8, 4, 6 6, 4, 5 |
| [97] | Overall | ✔ | Ψ | ✔ | 16 kW | Operation | Finite Element Analysis | 8, 4, 6 |
| [98] | Ψ | ✔ | MATLAB | ✔ | Ψ | Operation | Finite Element Analysis | 6, 2, 4 & 4, 2, 3 |
| [99] | Generator | ✔ | Ψ | ✘ | 50 hp | Operation/Control | Steady-State Analysis | Ψ |
| [100] | Both Modes | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Operation | Winding Function Theory | 6, 2, 4 |
| [101] | Motor | ✘ | ✘ | ✔ | 250 kW | Operation | Beam Theory | 2, 4, 3 |
| [102] | Motor | ✔ | ANSYS Maxwell | ✘ | 1.5 MW | Design | Finite Element Analysis | 8, 4, 6 |
| [103] | Motor | ✔ | JMAG Designer | ✔ | 630 W | Design | Finite Element Analysis | 8, 4, 6 |
| [104] | Generator | ✔ | Ψ | ✔ | 200 W | Design | Finite Element Analysis | 8, 4, 6 |
| [105] | Motor | ✘ | ✘ | ✔ | 630 W | Design | Finite Element Analysis | 8, 4, 6 |
| [106] | Overall | ✔ | ANSYS Maxwell | ✘ | 1 kW | Design | Finite Element Analysis | 2, 6, 4 & 4, 6, 5 4, 8, 6 |
| [107] | Motor | ✔ | JMAG Designer | ✔ | 16 kW | Design | Finite Element Analysis | 6, 2, 4 |
| [108] | Generator | ✔ | ANSYS Maxwell | ✘ | 1022 kW1059 kW | Design | Finite Element Analysis | 8, 4, 6 |
| [109] | Overall | ✔ | Ψ | ✘ | Ψ | Control/Power Factor | Space Vector Theory | 6, 2, 4 |
| [110] | Both Modes | ✔ | MATLAB/Simulink | ✔ | 10 kW | Control | Finite Element Analysis | 6, 2, 4 |
| [111] | Generator | ✔ | Ψ | ✘ | 4.5 kW | Control/MPPT/Losses | Dynamic Analysis | 6, 2, 4 |
| [112] | Both Modes | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [113] | Generator | ✔ | MATLAB/Simulink | ✘ | 2 MW | Control | Space Vector Theory | 6, 2, 4 |
| [114] | Generator | ✔ | Ψ | ✘ | 4.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [115] | Generator | ✔ | Ψ | ✘ | 1500 W | Control | Space Vector Theory | 6, 2, 4 |
| [116] | Generator | ✔ | MATLAB/Simulink | ✔ | 42 kW | Control | Space Vector Theory | Ψ |
| [117] | Generator | ✔ | MATLAB/Simulink | ✔ | 42 kW | Control/MPPT | Space Vector Theory | 6, 2, 4 |
| [118] | Generator | ✔ | MATLAB/Simulink | ✔ | 42 kW | Control | Theoretical Analysis | 6, 2, 4 |
| [119] | Generator | ✘ | ✘ | ✔ | 1.5 kW | Power Factor | Space Vector Theory | 6, 2, 4 |
| [120] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.6 kW | Control | State-Space Model | 6, 2, 4 |
| [121] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.6 kW | Control | State-Space Model | 6, 2, 4 |
| [122] | Generator | ✔ | MATLAB/Simulink | ✘ | 1.7 kVA | Control | Space Vector Theory | 6, 2, 4 |
| [123] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [124] | Ψ | ✔ | MATLAB/Simulink | ✔ | 5 hp | Control | Space Vector Theory | 6, 2, 4 |
| [125] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [126] | Generator | ✔ | MATLAB/Simulink | ✘ | Ψ | Control | Space Vector Theory | 6, 2, 4 |
| [127] | Motor | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [128] | Motor | ✔ | MATLAB/Simulink | ✘ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [129] | Overall | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [130] | Generator | ✔ | MATLAB/Simulink | ✔ | 1.5 kW | Control | Space Vector Theory | 6, 2, 4 |
| [131] | Generator | ✔ | MATLAB/Simulink | ✔ | 1.6 kW | Control | Space Vector Theory | 6, 2, 4 |
| [132] | Motor | ✔ | MATLAB/Simulink | ✘ | 1.6 kW | Control | Dynamic Theory | 6, 2, 4 |
| [133] | Overall | ✔ | Ψ | ✘ | Ψ | Power Factor | Space Vector Theory | 2, 6, 4 |
| [134] | Generator | ✘ | ✘ | ✔ | 1.5 kW | MPPT | Heuristic-Based Model | 6, 2, 4 |
| [135] | Generator | ✔ | Ψ | ✘ | 4.5 kW | MPPT | Space Vector Theory | 6, 2, 4 |
| [136] | Generator | ✔ | Ψ | ✔ | 4.5 kW | MPPT | Space Vector Theory | 6, 2, 4 |
| [137] | Generator | ✔ | Ψ | ✘ | 4.5 kW | MPPT | Space Vector Theory | 6, 2, 4 |
| [138] | Generator | ✔ | Ψ | ✘ | 4.5 kW | MPPT | Space Vector Theory | 6, 2, 4 |
| [139] | Generator | ✔ | Ψ | ✘ | 2 MW | Losses | FEA | 4, 8, 6 |
| [140] | Both Modes | ✔ | Ψ | ✔ | 5 hp | Control | Space Vector Theory | 6, 2, 4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sadeghian, O.; Tohidi, S.; Mohammadi-Ivatloo, B.; Mohammadi, F. A Comprehensive Review on Brushless Doubly-Fed Reluctance Machine. Sustainability 2021, 13, 842. https://doi.org/10.3390/su13020842
Sadeghian O, Tohidi S, Mohammadi-Ivatloo B, Mohammadi F. A Comprehensive Review on Brushless Doubly-Fed Reluctance Machine. Sustainability. 2021; 13(2):842. https://doi.org/10.3390/su13020842
Chicago/Turabian StyleSadeghian, Omid, Sajjad Tohidi, Behnam Mohammadi-Ivatloo, and Fazel Mohammadi. 2021. "A Comprehensive Review on Brushless Doubly-Fed Reluctance Machine" Sustainability 13, no. 2: 842. https://doi.org/10.3390/su13020842
APA StyleSadeghian, O., Tohidi, S., Mohammadi-Ivatloo, B., & Mohammadi, F. (2021). A Comprehensive Review on Brushless Doubly-Fed Reluctance Machine. Sustainability, 13(2), 842. https://doi.org/10.3390/su13020842