Environmental Impact Modeling for Transportation of Hazardous Liquids
Abstract
1. Introduction
2. Theoretical Background
3. Literary and Information Sources
4. Materials and Methods
4.1. Materials
- Slope of the terrain and the possibility of accelerated drainage;
- Porosity, type, and composition of affected soil and its ability to absorb spills; type of soil microflora;
- Aeration and soil moisture (depending on previous precipitation and current weather);
- Current weather (particularly the temperature, wind direction and speed, humidity, precipitation intensity);
- HL exposure time to soil (time interval between the occurrence of the accident and the arrival of the soil remediation group, and the method and type of remediation intervention).
4.2. Hazard Zone and Risk during HL Transporting
- Environment at the site of the event-necessary to describe the spread of fluid: The description of the medium contains an elevation and topographic model, which was improved several times. It was mainly an automated treatment of data discrepancies adopted from various map materials (e.g., the corner of the building located in the river). At the level of the required accuracy of calculations, this proved to be a significant problem;
- Current physical parameters necessary for research into the dependence of fluid propagation: A total of 10 field experiments were performed. Based on these, it was possible to decide on computational algorithms, but also on the settings and format of input parameters to the model. The sensitivity of the model to the required fineness of spatial and temporal description was tested;
- Uncertainties associated with the solution at a specific location: The objectification of uncertainties was based on stochastic modeling, especially with respect to their variability over time (e.g., soil saturation with water) and in space (e.g., details in uneven terrain and liquid capture on vegetation). The problem was solved by entering the interval values of individual quantities for probabilistic calculations.
4.3. Theoretical Principles of Used Models
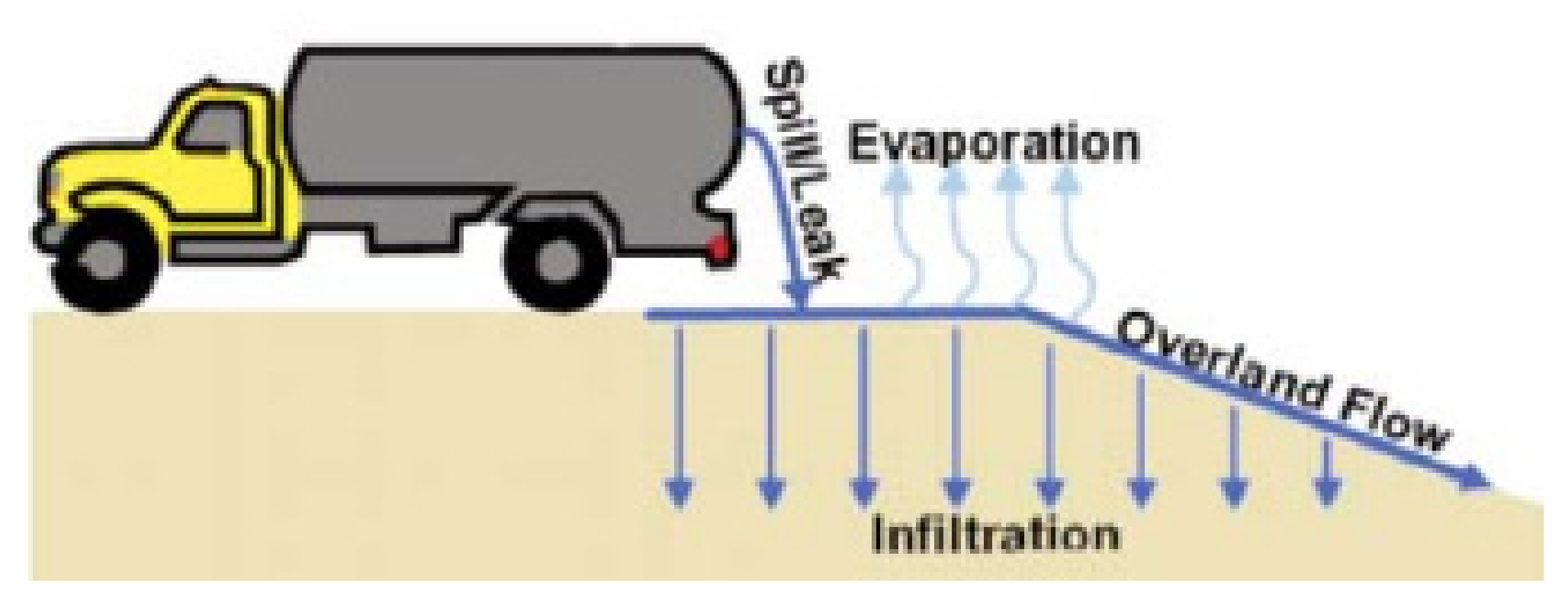
- Liquid overflow from one element to another;
- Trapping of liquid on the earth’s surface;
- Infiltration below the surface;
- Evaporation of the liquid.
- Amount of free liquid (capable of outflow);
- Level height;
- Saturation of capture capacity (or unused capacity);
- Degree of subsoil saturation.
4.4. Probabilistic Approach for Fluid Motion Modeling
- The probability that the liquid will reach the relevant element within a defined time from the moment of leakage. As with one element, this value can be specified for an entire group of elements. Such a group may represent, for example, a line representing the bank of an endangered watercourse, or an area of rare habitat, etc.;
- The probability of exceeding the monitored limit values, for example, a limited infiltrated amount posing a serious threat to the soil or groundwater. By analogy, even in this case, the determined probability can relate not only to one, but to the whole group of elements.
5. Results
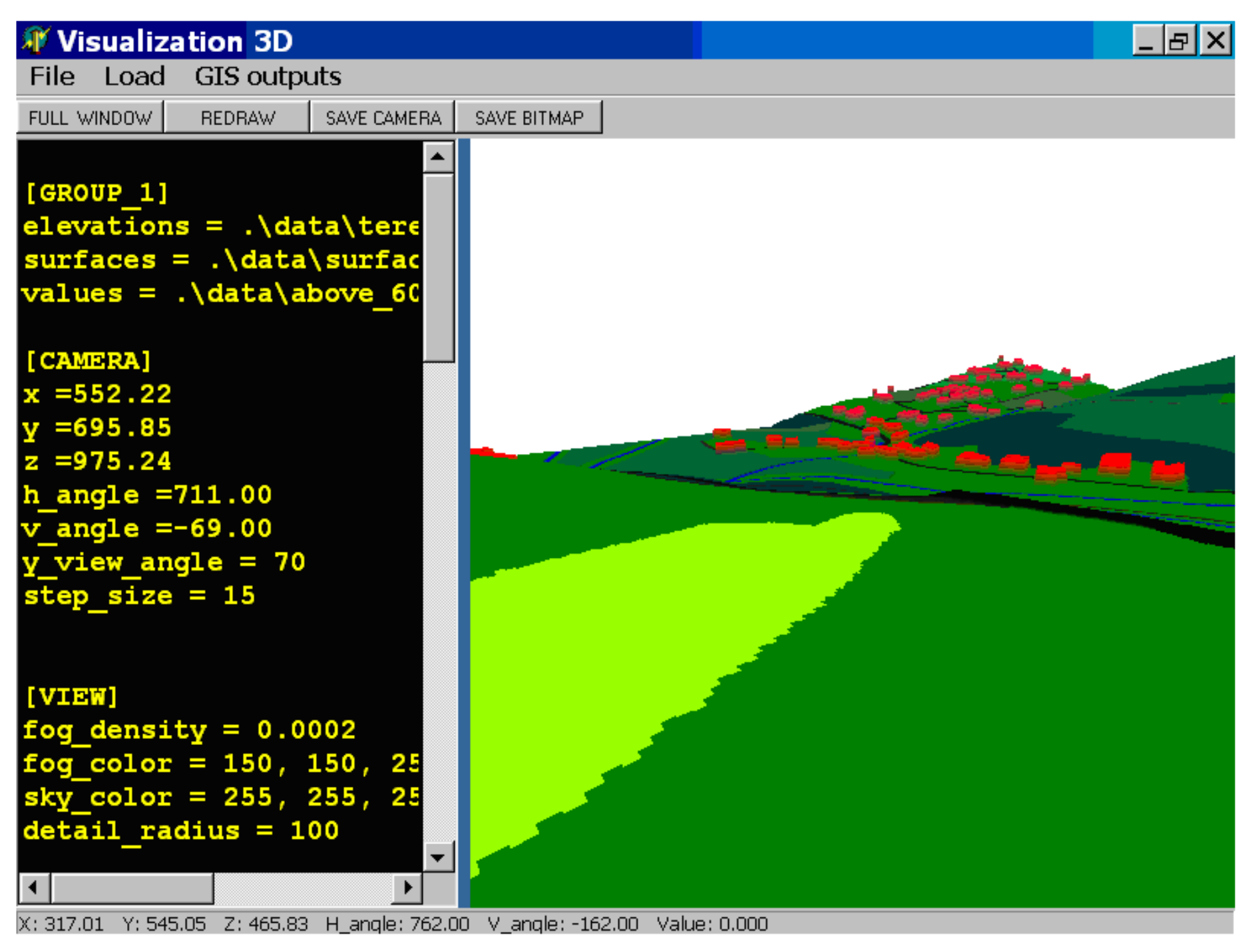
5.1. Software Tool
- Identification of source data files;
- Placement and rendering of objects;
- Requirements for modification of element heights;
- Physical parameters of the element.
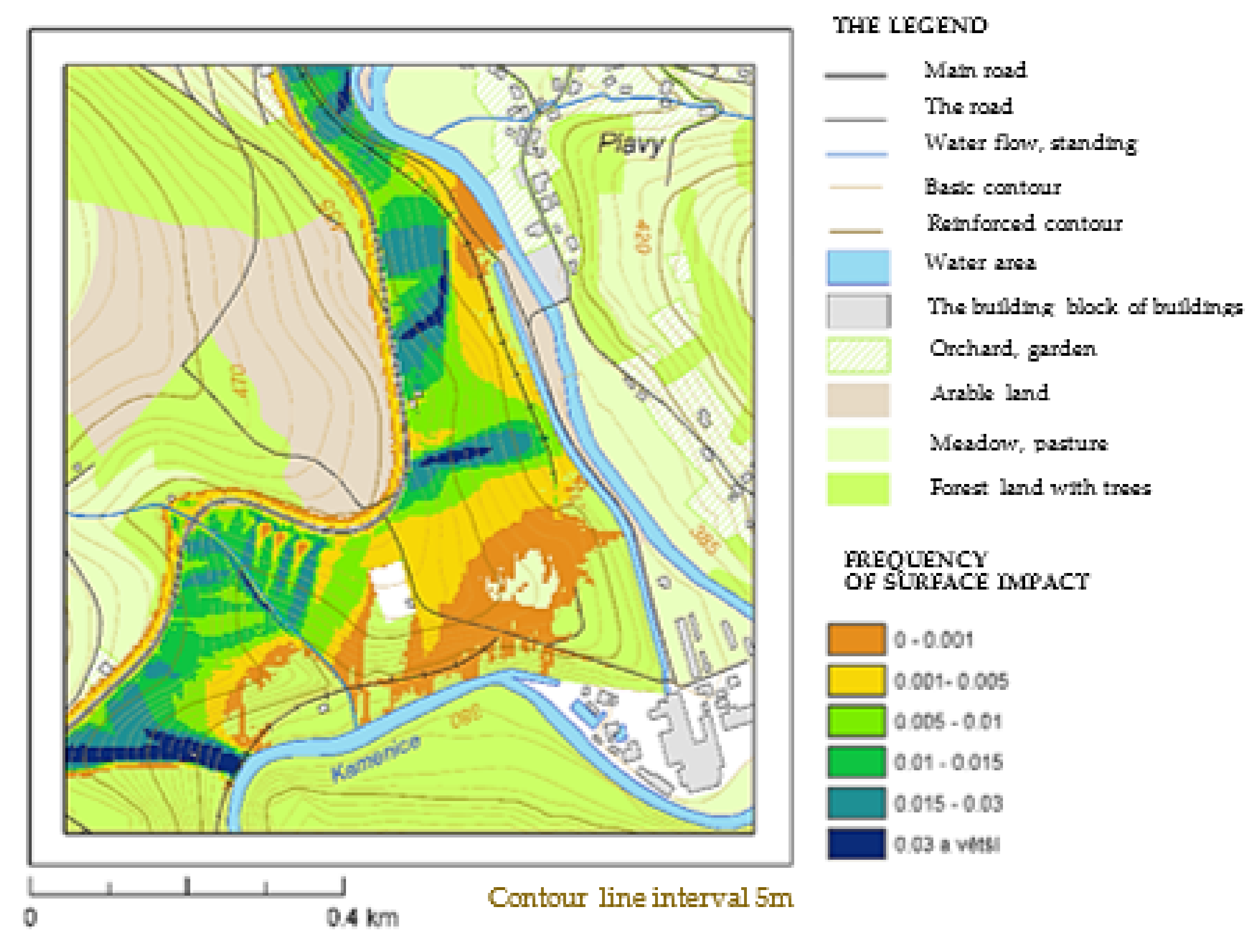
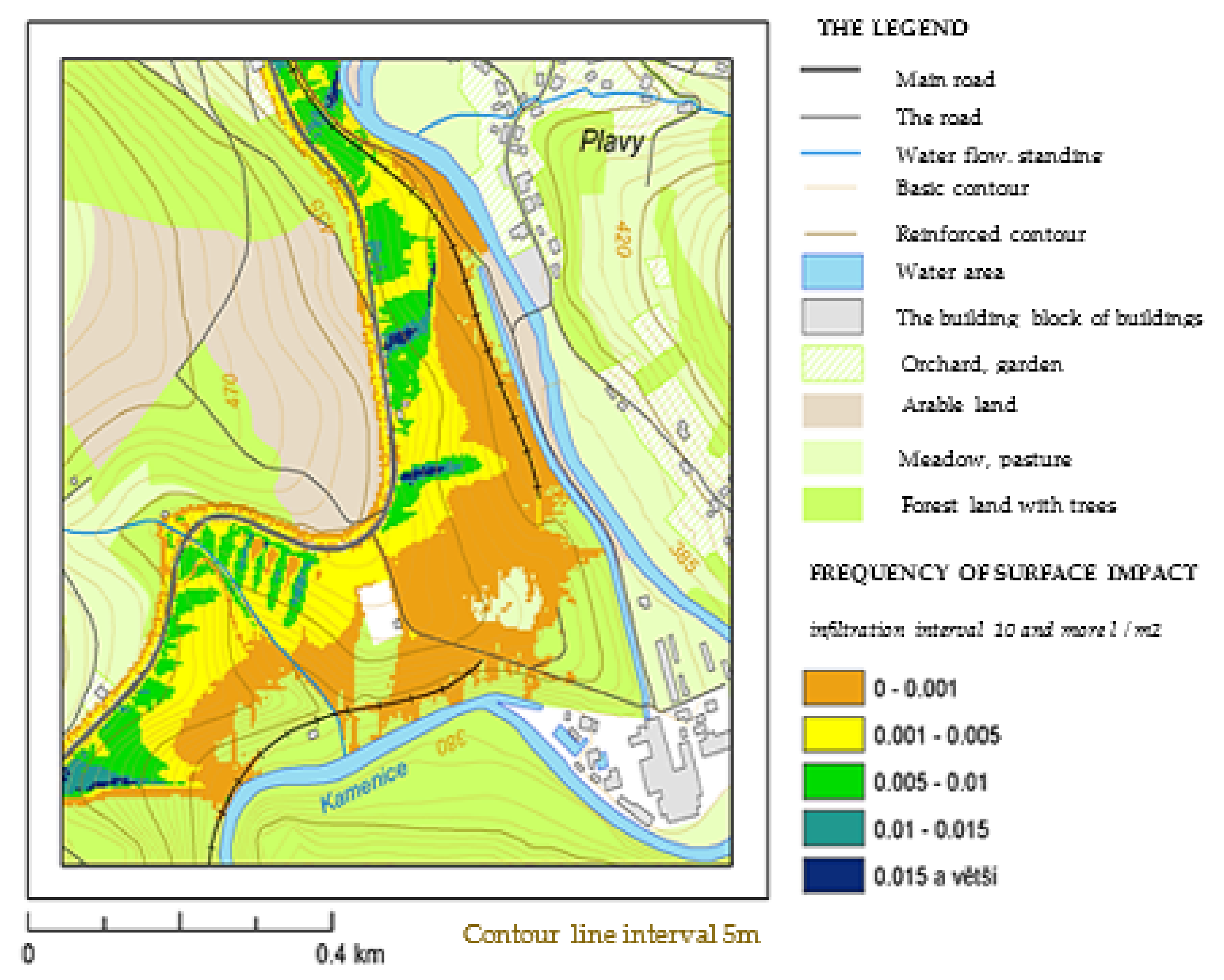
5.2. Dependence of the Pool Area and HL Penetration into Surface Waters on Infiltration Parameters
5.3. Liquid Spreading Time Course
5.4. Rendering of the Dangerous Zone
5.5. Probability of Impacting a Point (Area Element) with Liquid
5.6. Threat Zone and Its Characteristics
- Infiltration of fluid into the soil;
- Pool depth;
- Seepage of liquid into surface waters.
5.7. Procedure for Determining the Frequency of Impacting the Points near the Route
5.8. Frequencies of Impacting A Set of Points near the Route
6. Discussion
- The amount of HL, usually limited by the maximum volume of the transport packaging (typically tanks);
- Leakage time, limited by the time of complete emptying of the transport packaging; the worst-case scenario, the immediate leakage of the entire volume of HL, is usually assumed;
- A place of leakage which is not known in advance and may, with varying probabilities, be located anywhere on the HL transport route;
- After the liquid has been spread onto the earth’s surface, three basic physical processes occur at different intensities: overland flow, infiltration, and evaporation.
- Clarification of the theoretical framework of the proposed method of modeling the spread of HL in the vicinity of the transport route;
- Application of a theoretical apparatus for predicting the magnitude and intensity of action in the event of an HL leak during transport-dangerous zone;
- Presentation of selected functionalities and possibilities of a software tool for stochastically based models of forecasting the spread of liquids in the terrain.
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviation
| ADR | Agreement concerning the international carriage of Dangerous goods by Road |
| ZABAGED | Basic database of geographical data of the Czech Republic |
| DG | Dangerous Goods |
| GIS | Geographical Information System |
| HG | Hazardous Gases |
| HL | Hazardous Liquid |
References
- Ditta, A.; Figueroa, O.; Galindo, G.; Yie-Pinedo, R. A review on research in transportation of hazardous materials. Socio-Econ. Plan. Sci. 2019, 68, 100665. [Google Scholar] [CrossRef]
- Häkkinen, J.; Malk, V.; Posti, A.; Penttinen, O.P.; Mäkelä, R.; Kiiski, A. Environmental risk assessment of the most commonly transported chemicals: Case study of Finnish coastal areas. WMU J. Marit. Aff. 2013, 12, 147–160. [Google Scholar] [CrossRef][Green Version]
- Batarlienė, N. Improving Safety of Transportation of Dangerous Goods by Railway Transport. Infrastructures 2020, 5, 54. [Google Scholar] [CrossRef]
- Zhang, L.; Feng, X.; Yang, Y.; Ding, C. Routing for Hazardous Materials Transportation in Urban Areas. Symmetry 2019, 11, 1091. [Google Scholar] [CrossRef]
- Belvederesi, C.; Thompson, P.; Komers, E. Statistical analysis of environmental consequences of hazardous liquid pipeline accidents. Heliyon 2018, 4, e00901. [Google Scholar] [CrossRef] [PubMed]
- Peng, H.T.; Guo, D.; Duan, M.S. Discussion on environmental risk assessment and management of urban gas pipelines. Environ. Prot. Oil Gas. Fields 2013, 5, 68–71. [Google Scholar] [CrossRef]
- Zhong, Z.L.; Zhang, Q.Y.; Fu, X.S. Exploration on the environmental risk assessment system of natural gas pipelines. Low Carbon World 2017, 45, 1–3. [Google Scholar] [CrossRef]
- Tumanov, A. Risk Assessment of Accidents During the Transportation of Liquid Radioactive Waste in Multimodal Transport. IOP Conf. Ser. Earth Environ. Sci. 2019, 272, 032078. [Google Scholar] [CrossRef]
- Moore, J.E.; Sandquist, G.M.; Slaughter, D.M. A Route-Specific System for Risk Assessment of Radioactive Materials Transportation Accidents. Nucl. Technol. 2017, 112, 63–78. [Google Scholar] [CrossRef]
- EUROSTAT. Available online: https://ec.europa.eu/eurostat/databrowser/view/ttr00005/default/table?lang=en (accessed on 28 July 2021).
- Czech Statistical Office—Public Database. Available online: https://vdb.czso.cz/vdbvo2/faces/en/index.jsf (accessed on 28 July 2021).
- Statistical Office of the Slovak Republic. Available online: http://datacube.statistics.sk/#!/lang/en/?utm_source=susr_portalHP&utm_medium=page_DATAcube&utm_campaign=DATAcube_portalHP (accessed on 28 July 2021).
- Sousek, R.; Dvorak, Z. Risk identification in critical transport infrastructure in case of central Europe with focus on transport of dangerous shipments. In Proceedings of the 3th World Multi-Conference on Systemics, Cybernetics and Informatics (WMSCI 2009), Orlando, FL, USA, 10–13 July 2009; pp. 374–377. [Google Scholar]
- Fuchs, P.; Novak, J.; Saska, T.; Smida, J.; Dvorak, Z.; Kelemen, M.; Sousek, R. Simulation of dangerous substances outflows into the environment because of traffic accidents by dangerous substances transport. In Proceedings of the 14th World Multi-Conference on Systemics, Cybernetics and Informatics (WMSCI 2010), Orlando, FL, USA, 29 June–2 July 2010; pp. 204–207. [Google Scholar]
- Dvorak, Z.; Fuchs, P.; Novak, J.; Sousek, R. Individual and social risk during transportation of dangerous substances. Communications 2011, 13, 92–98. [Google Scholar]
- Fuchs, P.; Saska, T.; Sousek, R.; Valis, D. How to calculate the accident probability of dangerous substance transport? Arch. Transp. 2012, 24, 273–284. [Google Scholar] [CrossRef]
- Dvorak, Z.; Rehak, D.; David, A.; Cekerevac, Z. Qualitative approach to environmental risk assessment in transport. Environ. Res. Public Health 2020, 17, 5494. [Google Scholar] [CrossRef] [PubMed]
- Environmental Modelling & Software. ISSN 1364-8152. Available online: https://www.journals.elsevier.com/environmental-modelling-and-software (accessed on 28 July 2021).
- Jakeman, A.; Voinov, A.; Rizzoli, A.; Chen, S. Environmental Modelling, Software and Decision Support; Johns Hopkins University and Fellow at Gund Institute for Ecological Economics, USA; Elsevier Science & Technology: Amsterdam, The Netherlands, 2008; p. 384. ISBN 9780080568867. [Google Scholar]
- Environmental Protection Agency. Available online: https://www.epa.gov/water-research/infiltration-models (accessed on 15 August 2021).
- Van Raemdonck, K.; Macharis, C.; Mairesse, O. Risk analysis system for transport of hazardous material. J. Saf. Res. 2013, 45, 55–63. [Google Scholar] [CrossRef] [PubMed]
- Torretta, V.; Rada, E.C.; Schiavon, M.; Viotti, P. Decision support systems for assessing risk involved in transporting hazardous material. Saf. Sci. 2017, 92, 1–9. [Google Scholar] [CrossRef]
- ADR European Commission COM (2018) 598 Final. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/HTML/?uri=CELEX:52018PC0598&from=EN (accessed on 1 August 2021).
- Erkut, E.; Verde, V. Modeling of transport risk for hazardous material. Oper. Res. 1998, 46, 625–642. [Google Scholar] [CrossRef]
- Zografos, G.K.; Androutsopoulos, K.N. Decision support system for integrated hazardous materials rounting and emergency response decision. Transp. Res. Part. C 2008, 16, 684–703. [Google Scholar] [CrossRef]
- Smith, K. Environmental Hazards—Assessing Risk and Reducing Disaster; Routlege: New York, NY, USA, 2013; 504p. [Google Scholar]
- Martínez-Alegría, R.; Ordóñez, C.; Taboada, J. A conceptual model for analyzing the risks involved in the transportation of hazardous goods: Implementation in a Geographic information system. Int. J. 2010, 857–873. [Google Scholar] [CrossRef]
- Cordeiro, F.G.; Bezerra, B.S.; Peixoto, A.S.P.; Ramos, R.A.R. Methodological aspects for modeling environmental risk of transporting hazardous materials by road. Transp. Res. 2016, 44, 105–121. [Google Scholar] [CrossRef]
- European Commission CORDIS. Available online: https://cordis.europa.eu/ (accessed on 1 August 2021).
- TRANSrisk Grand Agreement ID: 642260. Available online: https://cordis.europa.eu/project/id/642260 (accessed on 17 August 2021).
- GRACE Grand Agreement ID: 679266. Available online: https://cordis.europa.eu/project/id/679266 (accessed on 17 August 2021).
- Information System of Research, Development of Innovations in the Czech Republic. Available online: https://www.isvavai.cz/ (accessed on 1 August 2021).
- Project 2B08011 BIOTRA—Methodology for Assessing the Impact of Transport Routes on Biodiversity and Environmental Components (2008–2011, MSM/2B). (In Czech). Available online: https://starfos.tacr.cz/en/project/2B08011 (accessed on 18 August 2021).
- Council Directive 96/82/EC of 9 December 1996 on the Control of Major-Accident Hazards Involving Dangerous Substances. Available online: https://eur-lex.europa.eu/legal-content/en/ALL/?uri=CELEX%3A31996L0082 (accessed on 1 August 2021).
- Act 128/2015 Coll. on the Prevention of Serious Industrial Accidents and on the Amendment of Certain Acts. (In Slovak). Available online: https://www.slov-lex.sk/pravne-predpisy/SK/ZZ/2015/128/20160701 (accessed on 1 August 2021).
- Holla, K. Adapting the Directive SEVESO III in the Slovak Enterprises and Identifying Problem Areas during the Years 2013–2017. In MATEC Web Conferences; EDP Sciences: Les Ulis, France, 2018; Volume 210, p. 02020. [Google Scholar] [CrossRef][Green Version]
- Sikorova, K.; Bernatik, A.; Lunghi, E.; Fabiano, B. Lessons learned from environmental risk assessment within the framework of Seveso Directive in Czech Republic and Italy. J. Loss Prev. Process. Ind. 2017, 49, 47–60. [Google Scholar] [CrossRef]
- Yapa, P.D.; Tao Shen, H. Modelling river oil spills: A review. J. Hydraul. Res. 1994, 32, 765–782. [Google Scholar] [CrossRef]
- Adamu, B.; Tansey, K.; Ogutu, B. Remote sensing for detection and monitoring of vegetation affected by oil spills. Int. J. Remote Sens. 2018, 39, 3628–3645. [Google Scholar] [CrossRef]
- Methodological Manual of Geological Survey of the Environment in a Polluted Area. Ministry of the Environment of the Slovak Republic. (In Slovak). 2020. Available online: https://www.minzp.sk/files/sekcia-geologie-prirodnych-zdrojov/538_mppp_fin_2021.pdf (accessed on 1 August 2021).
- Methods for the Calculation of Physical Effects Resulting from Releases of Hazardous Materials (Liquids and Gases), (Yellow Book), 3rd ed.; second print; Committee for the Prevention of Disasters (CPR), Directorate—General of Labour of the Ministry of Social Affairs: The Hague, The Netherlands, 2005; Available online: https://content.publicatiereeksgevaarlijkestoffen.nl/documents/PGS2/PGS2-1997-v0.1-physical-effects.pdf (accessed on 1 August 2021).
- The Fundamental Base of Geographic Data of the Czech Republic (ZABAGED®). Available online: https://geoportal.cuzk.cz/(S(qjsin2lre214w43jrgzzvpd2))/Default.aspx?lng=EN&mode=TextMeta&text=dSady_zabaged&side=zaaged&menu=24 (accessed on 1 August 2021).
- Wallender, W.W. Green-Ampt Infiltration Model. HYD 151. Courses in Hydrologic Science, Fluid Mechanics Fundamentals; University of California: Los Angeles, CA, USA, 2009. [Google Scholar]
- Kallenberg, O. Probabilistic Symmetries and Invariance Principles; Springer: New York, NY, USA, 2005; 510p, ISBN 0-387-25115-4. [Google Scholar]
- Rényi, A. Probability Theory; American Elsevier Publishing Company, Inc.: New York, NY, USA, 1970; 666p. [Google Scholar]
- Balatka, M. The Overland Spill Spreading Simulation Model. Doctoral Thesis, Technical University of Liberec, Liberec, Czech Republic, 2013; 118p. [Google Scholar]
- Balatka, M.; Fuchs, P.; Kamenický, J.; Soušek, R.; Kelemen, M. Exposure of the Environment and Surface Water by Dangerous Liquids—The Slop Outflow Model. In Proceedings of the 8th Symposium Risk Management and Cyber-Informatics (RMCI 2011), Orlando, FL, USA, 19–22 July 2011; ISBN 978-1-936338-42-9. [Google Scholar]
- Bartaliene, N. Essential Safety Factors for the Transport of Dangerous Goods by Road: A Case Study of Lithuania. Sustainability 2020, 12, 4954. [Google Scholar] [CrossRef]
- Rehak, D.; Radimsky, M.; Hromada, M.; Dvorak, Z. Dynamic impact modeling as a road transport crisis management support tool. Adm. Sci. 2019, 9, 29. [Google Scholar] [CrossRef]
- Ziyuan, L.; Yingzhao, W.; Tianle, L.; Xiaoxue, W.; Wenzhuo, L.; Ying, Y.; Xiangfei, X. Double Path Optimization of Transport of Industrial Hazardous Waste Based on Green Supply Chain Management. Sustainability 2021, 13, 5215. [Google Scholar] [CrossRef]
- Transforming Our World: The 2030 Agenda for Sustainable Development, UN. Available online: https://sustainabledevelopment.un.org/post2015/transformingourworld (accessed on 14 August 2021).
















| Group of Substances | Typological Substances | Representative |
|---|---|---|
| Flammable substances | Petroleum products | Diesel, Petrol |
| Corrosive substances | Inorganic acids | Hydrochloric acid, Sulfuric acid |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dvorak, Z.; Leitner, B.; Ballay, M.; Mocova, L.; Fuchs, P. Environmental Impact Modeling for Transportation of Hazardous Liquids. Sustainability 2021, 13, 11367. https://doi.org/10.3390/su132011367
Dvorak Z, Leitner B, Ballay M, Mocova L, Fuchs P. Environmental Impact Modeling for Transportation of Hazardous Liquids. Sustainability. 2021; 13(20):11367. https://doi.org/10.3390/su132011367
Chicago/Turabian StyleDvorak, Zdenek, Bohus Leitner, Michal Ballay, Lenka Mocova, and Pavel Fuchs. 2021. "Environmental Impact Modeling for Transportation of Hazardous Liquids" Sustainability 13, no. 20: 11367. https://doi.org/10.3390/su132011367
APA StyleDvorak, Z., Leitner, B., Ballay, M., Mocova, L., & Fuchs, P. (2021). Environmental Impact Modeling for Transportation of Hazardous Liquids. Sustainability, 13(20), 11367. https://doi.org/10.3390/su132011367







