Teaching Multiplication to Students with Mathematical Learning Disabilities (MLD): Analysis of Preservice Teachers’ Lesson Design

Abstract

This qualitative study investigated 17 preservice teachers’ lesson design for teaching multiplication to an average performing student and a student with mathematical learning disabilities (MLD). Findings reveal how preservice teachers differentiate mathematics instruction to meet the needs of students. They modified mathematical strategies by providing diverse multiplicative concepts and fitting the form of representations. They accommodated lesson design by setting their expectations based on individual needs, managing instructional structure and progress, and adjusting the cognitive demand of tasks. Some formative assessment skills demonstrated how they understood students’ mathematical thinking and responded to it. The needs for further attention and support in lesson differentiation, including content-oriented alternation for equitable responsive teaching and moving away from short-term solutions to sustainable support, were discussed.

1. Introduction

The ultimate goal of teacher education is to support preservice teachers to implement newly acquired knowledge and skills into their practice. This study was designed to provide preservice teachers (PTs) with opportunities to apply their learning to teach all learners in the classroom. Teaching mathematics to all students means each student has access to high quality learning opportunities. Previous research has proven that there is an unequal distribution of high-quality learning opportunities within mathematics classrooms [1,2]. How instructional strategies should be modified to maximize the quality of learning opportunities for each student is an urgent question in the field of mathematics education [3,4]. This connects to the agenda of teacher education—supporting preservice teachers to learn how to provide high quality learning opportunities for every student, and to apply their learning to practice. However, preparing teachers to implement instruction for equity and access remains a challenge, especially for preservice teachers with few experiences in the classroom [5], including inclusive classroom settings.

Modifying and accommodating mathematics instruction for all students with different needs is a highly complex process. Although modification and accommodation of instruction seem similar, there are differences: modification means a change that is being taught or what students are expected to learn, and accommodation means a change that helps a student overcome or work around the disability. Even though helping students with mathematical learning disabilities (MLD) is the common goal for mathematics educators and special educators, it was not common to have systematic intersections between the fields of mathematics education and special education until recently [6]. This is possibly due to the different perspectives on effective instructional approaches or intervention strategies for students with special needs, especially those with MLD. Regardless of the philosophical or practical differences in the two fields, it should be natural for mathematics and special education teachers to collaborate in order to address issues of students with MLD. Our study is aimed at bridging the two fields through a collaborative effort of mathematics educators and a special educator investigating how mathematics teachers should be prepared to teach mathematics to all students, including students with MLD. By doing this, we expect to advance our understanding of current teacher preparation regarding equitable learning opportunities and providing ideas for preparing teachers to be more responsive to learners with special needs.

This study provided a vignette activity involving teaching early multiplication concepts to students with different needs. PTs were asked to develop lesson ideas concerning multiplication with single digit multipliers for a student with average performance and a student with MLD. We investigated the PTs’ approaches for providing access and opportunity for those learners with different needs.

2. Review of the Literature

2.1. Teaching Mathematics for All

The commitment to respond to the need for preparing teachers for a growing diversity in schools and to provide equal opportunity for all students has a long history in the United States: The Elementary and Secondary Education Act [7], No Child Left Behind [8], and The Every Student Succeeds Act [9]. There is similar legislation in the United Kingdom [10]. All these efforts recommend educators identify students’ needs and to build capacity to meet those needs. Similar efforts have been made globally. In order to protect students with disabilities, the Australian Government released Disability Standards for Education [11,12] requiring educators to demonstrate how they meet the needs of students with disabilities and to make changes to reasonably accommodate the needs of a student with a disability.

National Council of Teachers of Mathematics’ position statement [3] states that teaching mathematics for equity means to ensure all students have opportunities to experience high quality mathematics instruction. Teaching mathematics to all students means providing learning opportunities for each and every student, including students with learning disabilities or gifted, behavioral issues, different cultural backgrounds, language barriers, and physical disabilities. Despite this commitment, certain segments of the student population are not well represented among those who succeed in school mathematics [13,14]. While “teaching mathematics for all” is an urgent agenda, the question remains how well preservice teachers are prepared to work with students with special needs [15]. This study focused on how preservice teachers construct different learning opportunities to respond to the hypothetical diverse classroom that includes a typical learner and a student with MLD. The vignette that students were given is a composite of characteristics of a student with MLD.

2.2. Teaching Mathematics to Students with Mathematical Learning Disabilities (MLD)

The operational definition of MLD is multifaceted. We consider students with MLD to be those that create unique patterns or different kinds of errors from their typical or low achieving peers [16]. MLD can also be classified as a learning disorder which hinders the ability to acquire arithmetic skills that are needed to perform mathematics, so can often create hurdles in acquiring mathematics knowledge [17]. Students with MLD have difficulty understanding typical mathematical representations and they often do not benefit from standard instruction [18]. Literature from a Special Education and Psychology perspective tends to focus on a scientific description of a neurological issue and how to solve it. Mathematics education for the general education teacher tends to focus on inquiry, cooperative learning, and discovery [19,20]. It is simplistic to say one approach is deficit minded and one is using a students’ strengths mindset [17,21]. There is value in both approaches for students with identified learning challenges. The researchers presented both viewpoints to PTs and shared literature from multiple perspectives around the term mathematical learning disability (MLD). Students with MLDs can be thought of as having cognitive differences, not cognitive deficits [18]. Our research centers on the idea that some students have MLD, and teachers should differentiate, modify, and accommodate instructional strategies to respond to their special needs.

A student with mathematical learning disability (MLD) has an average to above average IQ (Intelligence quotient) and can acquire information, but the teacher needs to offer an alternative access route to the material. Every student with MLD is unique and may require a differentiated approach to the facilitation of their education, including making links between procedural proficiency and conceptual understanding without emphasizing one over the other. Additionally, mathematics problems and examples should be selected to show the student the critical features of problem [22,23]. Successful teaching approaches for students with MLD can include explicit teaching [24,25,26,27], Antecedent–Behavioral Response–Consequence (ABC) teaching sequences [26] scaffolding [28], and Concrete–Representational–Abstract (CRA) [29,30]. It can also include peer teaching and cooperative learning [19,31,32].

Through the ABC teaching sequence, the student can be prompted and reinforced throughout the process, so there is motivation to continue the practice [26,33]. While ABC requires a great deal of teacher interaction, scaffolding looks to gradually decrease the teacher interaction. Scaffolding starts with the teacher modeling the entire process and moves forward until the student is doing the work independently [28]. CRA is a type of explicit instruction that consists of the following phases: concrete, involving objects; representational, involving pictures or drawings; and abstract, involving numbers only [30]. During CRA phases, the concreteness is faded out and connected to the abstract concepts [29,34]. When followed correctly the student first uses objects to construct meaning through the manipulation of the objects (concrete) [35]. During the representational stage students make their own representations of the operation and internalize the meaning of these representations to other operations [36]. When the student enters the abstract stage, the teacher builds on conceptual understanding and helps the student develop procedural knowledge and fluency. CRA research includes mnemonic strategies; however, it is very important that students have solid conceptual understanding before moving to an emphasis on procedural knowledge and fluency [36]. Research has proven that CRA not only helps students with MLD but also neurotypical students to better develop mathematical concepts [24,25,26,37,38].

Visual representation complements explicit instruction and can be an important tool. Research has reported that visual representations are particularly useful for learners with special needs such as MLD as they allow learners to engage in meaningful mathematics discourse in classroom settings [24,39]. However, students with MLD may struggle with visual representation because they process information differently [40]. The visuals can be broken down into external and internal representation categories. External representation examples include graphic organizers for improving comprehension of text and facts and enhancing the conceptual understanding in mathematics [41,42]. Diagrams such as number lines, schematic, tree, and pictorial are also effective [43]. Internal representation skills need to be taught [44]. Examples of internal representation includes visual chunking and visual schematics. Visual chunking is putting together small parts of information that are related while reducing the overall amount of information to process [45]. Visual schematics are creating diagrams mentally while verbally discussing what they are visualizing [46].

Peer tutoring has a long history and can include many variables such as how pairs are determined, how long they are engaged, the duration and frequency of each session, the topic, and if rewards are implemented [31,47,48]. An example of peer tutoring would be a teacher collecting baseline data, assigning peer tutors, training them to use a tutoring script, and flashcards. Then the students would do short sessions with materials assigned based on prior assessments. Each student has the tutor and tutee role. The teacher monitors the tutoring sessions and rewards students who are following proper procedures by giving them a stamp on their card. Students will end some sessions by doing a short probe to see if the skills are being generalized [31]. This differs from basic peer or collaboration work in that students are trained to help each other. Expectations are clearly stated.

Students with MLD need to be taught overt strategies, but once a teacher connects to the way that is most effective these students can make satisfactory progress. The teacher needs to focus on these strategies as a way to bridge the gap between what usually works for neurotypical students and what helps that student who processes mathematics in a unique manner.

2.3. Teaching Multiplication

Single digit multiplication is introduced in the intermediate grades and covers at least half of the 3rd grade standards in the United States [49]. Multiplication in elementary school is one of the fundamental operations across grades in school mathematics, and it is the basis for early algebra [50,51]. Helping students develop understanding of multiplication from the beginning could help them with issues in understanding the relationship between multiplication and division or overly relying on algorithms [52,53,54]. The use of derived facts including place value, properties of operations, understanding of decomposition and compensation, and using multiplication facts is important for success [55].

2.3.1. Conceptual Understanding of Multiplication

The multiplication symbol × is the result of modeling a computational situation. Until the early 20th century, multiplicative concepts were commonly considered repeated addition. Since the 1960s, the structure of multiplication has highlighted laws of arithmetic such as commutative, associative, and distributive laws so that the Cartesian product has been emphasized as a meaning and structure of multiplication [50]. Research on multiplication has classified situations that can be modeled by multiplication. Greer [56] classified four situations that can be modeled by multiplication, which include (1) equal groups—a number of groups of objects having the same number in each group, (2) multiplicative comparison—verbally expressed by “n times as many as” so that an amount of objects expands as the number of sets, (3) rectangular area and rectangular array—considers an area of an m × n rectangle and an arrangement of mn objects in a rectangular array with m rows and n columns, and (4) the Cartesian product—the formal definition of m × n, where the first component, a set with m elements, and the second component, a set with n elements, are used to produce a set with mn elements [56,57,58]. However, such a variety of multiplicative situations often causes difficulties for children learning multiplication. For example, students easily connect multiplication to the equal group situation by repeated addition, but have difficulties in connecting multiplication to the area of rectangles by the length times width formula [59].

External representation and models including concrete constructs or figures play a significant role not only in identifying psychological processes but also in supporting students for constructing mathematical concepts. Based on previous research [56,58,60], we identified four multiplicative models that externally represent multiplicative situations: (1) grouping model, (2) number-line model, (3) array model, and (4) combination model (detailed in

Table 1. Multiplicative strategies: Multiplicative models representing multiplicative situations.

External Representations of Multiplications		Multiplicative Situations and Structure
		Equal Groups	Multiplicative Comparison	Rectangular Array Rectangular Area	Cartesian Product
Grouping model	A model of grouping of objects having the same number in each group.	Can be represented	Can be represented
Number-line model	A number line model equally divided by constant intervals and drawing regular arcs of each interval.	Can be represented	Can be represented
Array model	A model that uniformly arranges several objects in a rectangular shape of rows and columns.	Can be represented	Can be represented	Can be represented	Can be represented
Combinations	A model that identifies possible ordered pairs made between two or more sets.				Can be represented

). Each model can represent different multiplicative situations externally. Sometimes one model can represent multiple situations. Students can get a better understanding of multiplication when they use various models to represent the multiplicative situation and explain the relationship between them [57]. Therefore, in teaching multiplication, it is necessary to provide an appropriate model for the multiplicative situation. In our study, we used this table to analyze what models PTs use to teach multiplication to students with diverse backgrounds and needs.

2.3.2. Mathematical Representations for Teaching and Learning Multiplication

The term representation refers both to process and to product; in other words, to the act of capturing a mathematical concept or relationship in some form and to the form itself [61]. In contrast, the models representing multiplicative situations include the process of capturing the multiplication concept. The focus of the representation in our study is the product, which refers to the form of representation. The importance of the different forms of mathematical representations in conceptual understanding has been documented in the mathematics standards [3,61,62]. These standards propose the different forms of representations of a mathematical concept, such as physical, visual, contextual, verbal, and symbolic representations, and emphasize connections between and within these different forms of representations. Students struggling with translating a concept among representations usually have difficulty solving problems and understanding computations [63]. Therefore, strengthening students’ ability to work with different representations improves their understanding.

Students’ mathematical understanding deepens, and problem-solving competency is enhanced when students use different types of representations and make connections between them [64,65]. For example, students develop understanding of the meaning of fraction $\frac{7}{4}$ (symbolic form) when they can see it as the quantity formed by “7 parts of size one-fourth” with a tape diagram or on a number line (visual form), or the measure of a string that has a length of 7 one-fourths yards (physical form) [3]. Among various forms of representations, visual representation is an important skill because a visual representation can be a mediator for making connections between different types of representations and ultimately helps students to link to the abstract and symbolic representations. Research has also reported that visual representations are particularly important learners with special needs such as MLD as they allow learners to engage in meaningful mathematics discourse in classroom settings [24,39]. Thus, we consider the use of representation as an important differentiated instructional strategy—particularly as a mathematics strategy. The PTs in this study were presented with these research-based information about multiplication and best practice approaches for teaching multiplication during their university coursework.

2.4. Research Questions

In our study, we investigated elementary preservice teachers’ approaches for providing access and opportunity to a learner with MLD. The following research questions guided this study:

• How do preservice teachers accommodate and modify mathematics strategies to teach multiplication to students with mathematical learning disabilities (MLD)?
• What instructional strategies do preservice teachers use for teaching multiplication in their lesson design for students with mathematical learning disabilities (MLD)?
• Mathematical approaches including multiplicative concepts and its representation are categorized as mathematics strategies; general instructional approaches including lesson goals, structure, flow, and task are categorized as instructional strategies.

3. Method

In this qualitative study, we explored equitable learning opportunities that were used by PTs in their plan for teaching multiplication to a learner with MLD. The approach stems from grounded theory, “in which the researcher derives a general, abstract theory of a process, action, or interaction grounded in the views of participants” [66] (p. 14). The intent is to further understand what mathematical and instructional strategies PTs use to address the needs for specific students through the lesson design activity. The PTs had been exposed to best practice research from multiple perspectives so that their personal toolbox for creating lesson plans should have included strategies that were both learner and teacher focused with the goal of subject mastery.

3.1. Setting and Participants

This study was conducted at a small university in the Midwest United States. Participants (N = 17) were undergraduate students enrolled in the early childhood education (ECE) licensure program that allows them to teach students in grades Pre Kindergarten (age 3)–three (age 9). All participants were female and identified as Caucasian with one identifying as Hispanic. In their junior year, PTs learned general education foundational theories and practice through university coursework and fieldwork. Teaching children with special needs was the main focus of the course, Introduction to Exceptional Children. This course included a component on differentiation of lessons based on student need. Students shared lessons before and after they had learned techniques to assess, modify, and adapt and discussed what they had learned. They were expected to expand on their knowledge base in their final year. In their senior (final) year, PTs continued to learn theories and implementation of strategies that benefit diverse learners. PTs applied their learning in practice through methods courses and fieldwork. The data of the study were collected during a mathematics methods course in their senior year.

3.2. Data Source

Data for this study were collected from the lesson design activity in conjunction with a course assignment that involved realistic classroom scenarios. An activity with realistic classroom scenarios (vignettes) can support PTs in developing a deeper understanding of instructional practices in mathematics teaching for diverse students. Vignettes are a valuable research data collection method, because they are standardized situations for all participants [67]. After achieving IRB approval, the researchers analyzed data from an activity in a mathematics methods course where students were asked to teach a specific task (a standard for 3rd grade level was given). We provided PTs a vignette with a hypothesized classroom consisting of students with different needs (

Table 2. Lesson design for hypothetical context: Teaching multiplication to diverse learners.

Purpose	Description in the Lesson Design Activity
Understand objectives of the lesson (A state standard for 3rd grade math was provided)	You want to teach a math lesson on multiplication 3.OA.1 Interpret products of whole numbers, e.g., interpret 5 × 7 as the total number of objects in five groups of seven objects each. (Note: These standards are written with the convention that a × b means a groups of b objects each; however, because of the commutative property, students may also interpret 5 × 7 as the total number of objects in seven groups of five objects each).
Understand learners and plan a lesson for a typically performing student	Jose is a typically performing 3rd grade student in terms of grade level scores on mathematical assessments.
Understand learners and modify/accommodate a lesson for students with MLD	Liam is a 3rd grade student with MLD. He has difficulty counting backwards, difficulty remembering ‘basic’ facts, is slow to perform calculations, has weak mental arithmetic skills, finds Addition is often the default operation and has high levels of mathematics anxiety. Liam’s strengths include a strong work ethic, the desire to please the teachers, and the ability to work well with classmates.

). In that classroom, PTs were required to teach multiplication based on Common Core State Standards for Mathematics (CCSSM)’s standard 3.OA.1 [62] to students with different learner profiles, an average performing student and a student with MLD. Table 2 describes the detailed hypothetical context for the lesson design activity.

The lesson design activity consisted of two phases. First, PTs were asked to brainstorm and develop a plan to teach a mathematics content standard to typically performing 3rd graders. Once they completed the first phase, PTs were asked to modify their lessons to address the needs of a student with MLD. PTs in the study have learned about MLD in their junior year as well as reading and discussing about teaching mathematics to all learners including students with special needs in the mathematics methods course.

3.3. Data Analysis

The lesson design activities of the 17 PTs were documented on paper. We used open coding [68] to categorize the meaningful strategies that the PTs used for responsive teaching in the mathematical and instructional categories. Open coding analysis is an analytic process through which concepts are identified and their properties and dimensions are discovered in data [69]. It is useful for questioning phenomena by breaking down data into discrete parts, closely examining them, and comparing them for similarities and differences. We employed the open coding method to reveal what the PTs kept for all students and alternated for each student profile within the lesson design so that we could qualitatively explore differentiated multiplication learning opportunities in a mathematics classroom.

For the data analysis, we aimed to derive from the 17 collected lesson plans a consistent category that included the ways in which the PTs responsively differentiated mathematics instruction to students with diverse needs. First, we identified two categories: mathematical strategies and non-mathematical strategies and labeled the lesson design elements. The mathematical strategies involved concepts of multiplication in teachers’ instruction and teachers’ support of students’ better understanding of the multiplicative concept. The mathematical strategies were mainly related to the content of the lesson. The lesson design elements category included task selection, lesson structure and process of instruction.

Next, we reexamined the data according to those two categories and found that we could develop the categories via theoretical and practical frameworks from previous research, as well as create sub-properties for each category. The mathematics strategies category included a multiplicative concept and different forms of representing the multiplicative concept. The concept of multiplication that was revealed in the lesson plan was coded across the modeled situation and the external representation model according to the works of Freudenthal [60], Greer [56] and van de Walle et al. [58]. The type of multiplicative concept used was coded according to the framework of the different representations of the mathematics concept, as suggested by NCTM [3]. The lesson design elements category included setting up expectations with goals and objectives, developing and selecting instructional tasks and activities, deciding instructional structure for students’ action, progression of instructional execution, and planning for assessments. We classified the quality of mathematical tasks according to the four cognitive demand levels of Stein and his colleagues [70], from low level tasks requiring simple memorization and procedures without connections to high level tasks involving procedures with connections and doing mathematics. After repeated examination, we developed two properties for the mathematical strategies’ category—multiplicative concept and types of representing the multiplicative concept. Three properties for the instructional design elements category included goals/objectives, instructional activity, and formative assessment.

Table 3. Categories and codes.

Categories	Properties	Codes and Sub-Codes		Description
Mathematical strategies	Multiplicative concept	Situation modeled	○ Equal groups ○ Multiplicative comparison ○ Rectangular array/area ○ Cartesian product	The distinguishable cases of situations that the multiplication modeled
		External representation	○ Equal group (grouping) ○ Number-line ○ Array ○ Combinations	External representations reflect the diversity of situations
	Types of representing the multiplicative concept	Visual aid		Draw a diagram, pictorial representation
		Physical experience		Hands-on activity, using manipulatives
		Symbolic objectification		Symbol ×, multiplication expressions or equations
		Verbal expression		Focus on the meaning of specific words, such as “groups” for multiplier and “objects” for multiplicand
		Contextual transition		Storytelling using the real-life context: historical, cultural, and artificial examples
Lesson design elements	Articulation of expectations	Mentioned the standard		Revisited standard/key concept
		Stated learning objectives		Stated observable and measurable learning objectives
		None		No discussion of goals/objectives
	Instructional activity	Task selection and development	○ Level of cognitive demand	Low: Immediate/not complicated task, simply using a number, procedural—a product of multiplication is a single-digit number Intermediate: Procedural—a product of multiplication greater than 10; conceptual–easily convertible to multiplication expressions High: Challenging task, open-ended, conceptual—not easily convertible to multiplication expressions
			○ Context of the task	Contextualized: Changing the number or formula Abstract: Changing the story or material of the task
		Instructional structures	○ Structures of people	Whole group instruction; Peer tutor, Group work–centers; Independent work
			○ Structures of time	Time for individualized support
		Instructional progress	○ Initial instruction	Begin with teacher directed modeling/demonstrating/explaining; “I do/You do/We do”; explicit; conventional Begin with student-directed discovery/project-based + group discussion
			○ Instructional flow	Concrete Concreteness fading Abstract
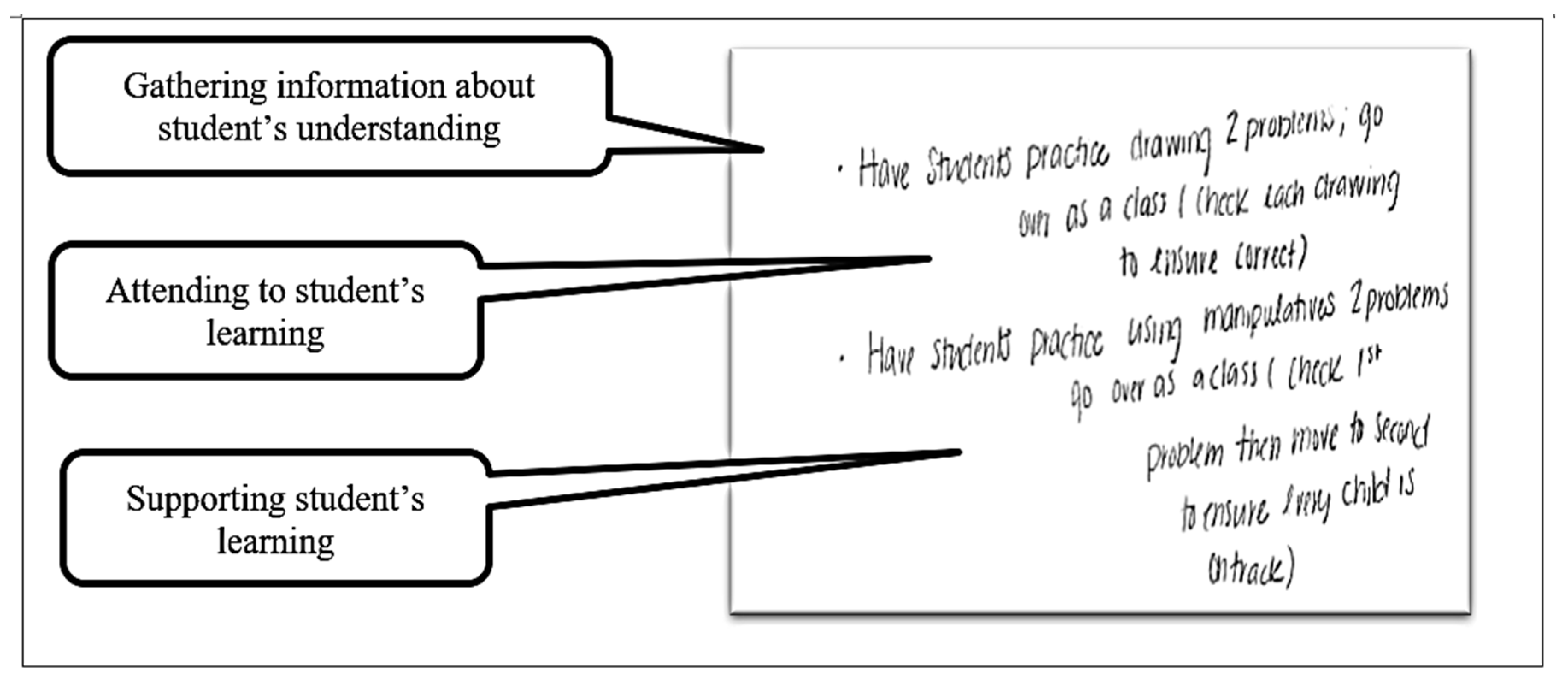
	Formative assessment	Gathering/sharing		Have students share/present their solutions/work
		Attending/interpreting		Looking into patterns or analyzing students’ work
		Supporting/feedback		Discuss ideas to use the interpretation of students’ work for instruction or to support students learning

summarizes our finalized coding scheme with the categories and properties. The coding results were derived through triangular verification between the authors.

4. Results

The results are presented in two sections: (1) mathematical teaching strategies and (2) differentiation (accommodation and modification) in lesson design. The first research question is discussed in the mathematical strategies section, which reports overarching findings regarding the way PTs introduced the multiplicative concept and ways to have students experience the multiplicative concept. The second research question is discussed in the lesson design elements section, which shares findings in relation to expectation setup, choice of instructional structure/outline, and the use of formative assessment.

4.1. Accommodations and Modification in Mathematical Strategies

Mathematical strategies identified in teachers’ lesson designs are approached in two ways: the concept of multiplication and ways of representing the multiplicative concept. This section explores the learning opportunities PTs created in response to two students that present different challenges: Jose, an average-performing student and Liam, who has MLD.

4.1.1. Introduction of the Multiplication Concept

In their plan for teaching multiplication to Jose, all 17 PTs modeled multiplication based on equal group situations. This is possibly due to the assigned standard’s example and description of multiplication—an equal group situation. In teaching multiplication concepts, it is necessary to use an external representation model such as concrete objects or pictures so that students can develop various mathematical strategies on their own and proceed to automation [60,71]. The external representation becomes an opportunity for students to learn multiplication. We were able to identify the use of external representation for multiplication in the lesson designs of the PTs. As mentioned in the theoretical section, three possible external representations for multiplication in equal group situations—grouping, number-line, and array models—appeared in the PTs’ lesson plans (see

Table 4. PTs’ choice of representation models to each student.

External Representations of Equal Group Situation	Jose	Liam
Grouping model	11	2
Number-line model	1	2
Array model	6	2
Combination model	0	0
Sub-total	18	6

Note: Multiple responses were counted in duplicate.

).

The grouping model was used most frequently, in approximately two-thirds of the lesson plans, while the array model was used in approximately one-third. No PT used the number-line model alone, and only one PT used the equal-group and number line models together. The PTs who represented an array model to students provided expanded learning opportunities, resulting in the distributive law, and supporting the students’ progression to the grid method with rectangular array representation, which aligns with the method reported in Izsák [59] shown in Figure 1.

Cross comparison of lesson plans revealed that not all PTs differentiated the concept of multiplication for Liam. PTs’ approach to introducing the multiplication concept to both learners was grounded in the same modeled situation, the equal group situation. Findings revealed that PTs differentiated external representations to accommodate Liam. It was noted that the modification in the instruction of the multiplication concept occurred more prominently for Liam. Among the 17 PTs, four (#11, #13, #16, and #17) modified extremal representations in the lesson plan. Three of the four external representations for Liam were totally different from what was used for Jose. Only one PT (#13) differentiated the use of external representations for both learners. She provided the array model for Jose. For Liam, she provided a number-line model, which is different from the model provided to Jose. PT #13 stated that she presented the number-line to help Liam count numbers. This decision stemmed from the fact that students with MLD may have difficulty in skip counting and must derive the product of multiplication through one-by-one counting. PT #13 reached the didactical decision that the number-line would be effective in preventing the omission of numbers or double counting in counting with manipulatives. This kind of number-line representation is a responsive teaching strategy for students with MLD by enabling them to visualize repeated addition of discrete quantities with directionality. In addition to PT #13, one more PT (#17) provided the number-line model for Liam with other accommodations. PT #16 used the array model for Jose but used the grouping model for Liam.

In summary, the PTs attempted to accommodate learners’ needs by modifying the external representation for the multiplication concept rather than changing the modeling situation of multiplication to teach students with different needs, and such modification was indicated more frequently for the student with MLD.

4.1.2. Experiencing the Multiplicative Concept

The PTs presented the multiplicative concept to students in a variety of ways, and the type of representation used most often provided a different experience for each student (see

Table 5. Types of representations to teach the multiplicative concepts.

Student Profile	Type of Representations					Sub-Total
	Visual Aid	Physical Experience	Symbolic Objectification	Verbal Expression	Contextual Transition
Typical	16 (30.77%)	15 (28.85%)	11 (21.15%)	5 (9.62%)	5 (9.62%)	52
MLD	7 (28%)	15 (60%)	0	0	3 (12%)	25

Note: Multiple responses were counted in duplicate.

). In their plan for Jose, most PTs provided physical experiences with visual aids (e.g., drawings or pictures) and manipulatives (e.g., blocks or cubes). These two types of representation were also frequently used for Liam. Physical experience was the most frequently used for Liam. PTs judged that a student who had difficulty in understanding abstract concepts needs a physical experience such as counting familiar objects or manipulatives.

Discrepancies in the type of representation used for different learners were most clear in the use of symbolic objectification. In total, 11 out of 17 PTs provided a multiplication formula ($a\times b=c$) to Jose, which is an objectified symbol of multiplication learned by visual and physical reification, whereas no PT provided such objectified symbols to Liam. There are two possible reasons. First, PTs may have determined that symbolic expressions do not require any modification to meet Liam’s needs. Another possibility is that PTs assumed that students with MLD are not at the stage of understanding abstract symbols. Since Liam was described as having weak mental arithmetic skills, it is highly likely that the PTs assumed the second case for Liam.

These results show that the PTs make instruction more responsive by encouraging Liam with physical experiences expressed by manipulating concrete materials. This is in line with CRA [29,30], but the PTs did not discuss taking students to the abstract level. They tended not to provide abstract mathematical representations for the student with MLD.

4.2. Accommodations and Modification in Lesson Design

In planning a lesson, teachers go through multi-layered decision-making processes once the math concept or content standard is confirmed. This includes setting up expectations, developing math tasks/activities, managing learning environment, and deciding on assessment means. These elements influence each other and are created based on learners’ needs. The second research question of the study focused on how PTs’ modify lesson design elements for students with different backgrounds and needs. Based on the data analysis, it was revealed that PTs paid more attention to modifying the process than the task selection, instructional structure, or the product of the lesson. Detailed accommodations and modifications in lesson design elements are discussed in the following section. The expectation was that after stating a basic lesson plan PTs would detail accommodations for changing how the student would learn material or discuss what modifications were created to change what the student is expected to learn.

4.2.1. Articulation of Expectations

More than half of the PTs began their lesson design by stating the goal of the lesson or what the teacher would do. For example, PT #2 repeated the math content standard, “Introduce multiplication as repeated addition.” Another PT also started the lesson plan by stating what she (the teacher) would do “Teacher: Introduce multiplication to the students, introduce commutative property, model using cubes and drawings” (PT #4). Important questions to ask in determining the learning goals are “What should my students be able to do when this lesson is over?; What content (conceptual and procedural) is important?; What mathematical practices/processes will be developed?” [58] (p. 62). However, not many PTs used learner-friendly language or stated observable and measurable learning objectives in their lesson plan. Some PTs used the “I can” statement as their cooperating teachers do to meet the state requirement. For example, PT #7 wrote the overall context of the lesson and key math concept in the beginning of her plan:

Students are learning now to multiply. This is the first day of the lesson.

• I can multiply single digit numbers.
• I can recognize multiplication problems.

While there were various statements related to the lesson goal/objectives in the lesson plan for Jose, no discussions or statements regarding learning goals/objectives were found in the lesson plan for students who need additional supports. It is possible that the PTs assumed that they have similar expectations for all learners and only focused on modifying the process of the lesson.

4.2.2. Orchestration of Instructional Activity

In the main instructional activity, we noticed that PTs discussed their plan for how they would execute their lesson in three areas: task/activity selection and development, instructional structure (how they would manage students’ actions), and instructional progress (beginning and flow of the instruction).

• Task Management: Content Modification

We anticipated PTs would select or develop mathematics tasks to meet students’ diverse learning needs so that students can experience important ideas in the classroom [72]. However, out of the 17 PTs, only four PTs mentioned distinct mathematics tasks according to student profiles. Just as the provided standard is exemplified by $5\times 7$, PTs used the exact same number as the standards in their mathematics task for both average performing student and the student with MLD without any modification. Five PTs changed the multipliers to smaller numbers to adjust the level of cognitive demand of the task, e.g., $2\times 3$ instead of $5\times 7$. However, no PTs developed any challenging tasks that required high cognitive demand. It is worth noting that four PTs (#11, #12, #13, and #16) demonstrated ways of responding to each and every student on a task-by-task basis. They provided tasks with the same or a similar level of cognitive demand for Jose, while they provided tasks with a lower level of cognitive demand for Liam. It seems that these four PTs understood the students’ mathematics performance levels. They not only lowered the cognitive demand but also changed the context of the task to reflect real-life situations for Liam. For instance, they changed the task “$2\times 3$” to “two groups of three cubs.” The PTs indicated that this kind of modification was necessary to reduce Liam’s math anxiety and provide emotional support. One PT planned to provide extra repeated addition tasks only for Liam to prepare him to learn multiplication more effectively.

Overall, the PTs tried to be responsive to the student with MLD by modifying tasks. They thought to lower the cognitive demand. They also considered transition to real-life contexts with an intention of providing Liam accommodated opportunities to learn multiplication.

2.

Instructional Structures

Instructional structures considered by PTs were structures of groups or structures of time. The most used modification examples were:

• For Jose: teach multiplication to the whole group and have students (such as Jose) engage in a group activity;
• For Liam: provide individual support separately.

Most PTs implemented a combination of whole group, small group, and/or independent activities for the typically performing student Jose. In their modification plan for Liam, more than half of PTs addressed Liam’s need for additional instructional time. PTs planned to provide individual tutoring during the lesson or additional help outside of the lesson. However, no PTs discussed how class grouping can be arranged differently for Liam, or suggested peer tutoring. There was no discussion of how Liam could join small group activities or contribute to the whole group discussions.

3.

Instructional Flow

Almost all PTs started their lesson with the teacher-direct instruction approach, such as modeling, demonstrating, or explaining to teach typically performing students. No PTs started the lesson with project-based or inquiry-based approaches. The lesson plan had progressed with a combination of concrete examples and representations, concreteness fading, and abstract representation. All PTs used concrete examples and objects at some point in their lesson plan, but the progression of the lesson varied. Approximately 24% of PTs used the concrete approach only, 41% of PTs started their lesson with the concrete approach and progressed to the abstract, and 35% of PTs started with abstract ideas and progressed to the concrete examples/representations (examples provided in Figure 2). Due to the lack of detailed description for teaching Liam, it was not feasible to investigate the progress of the instruction.

4.2.3. Assessment of Students’ Mathematical Thinking

Regarding assessment, we investigated how PTs approached students’ mathematical thinking and responded to individual students based on their mathematical thinking through formative assessment. Effective use of formative assessment would address students’ needs and be truly responsive. PTs used formative assessment for gathering and sharing, attending with interpretation, or supporting with feedback. Approximately half of the PTs used formative assessment geared toward gathering and sharing. For example, a PT #2 asked students to solve problems on the board and explain their thinking (gathering information about students’ understanding and ability to solve the problem) but did not advance her plan for using students’ mathematical thinking (attending to gathered information). Thirty-five percent of PTs gathered information on students’ mathematical thinking and used the information to attend to their learning. Approximately, 15% of PTs gathered information, attended to the information, and supported and provided feedback. An example of the last case can be found in PT #6 (Figure 3).

There was very limited discussion on assessments in PTs’ plans for Liam. Two PTs discussed potential assessment plans in their lesson design for Liam. For example, PT #5 did not discuss any plan for assessment for Jose, but her modified plan for Liam indicated the use of assessment: gathering (give simpler problems), attending (if he still does not understand), and supporting (I will return back to adding). PT #14 had an assessment plan for Jose: gather, attend (make sure you ask students the following questions), and support (redirect individual students when needed); whereas her plan for attending to Liam’s learning was to check his understanding as she walked around the classroom.

5. Discussion

The results in this study highlight the need to reconsider responsive teaching with regard to preparing teachers who can maximize quality of opportunities for all students. While the idea of modifying and accommodating instruction has been discussed in the field of teacher education, the topic of what it means to respond to students with various needs is beginning to attract attention [73]. We describe responsive teaching as accommodations and modifications in mathematics strategies and lesson design that PTs intend to provide to Jose and Liam and interpret the meaning from the perspective of preservice teacher education.

5.1. Content-Oriented Alternation for Equitable Responsive Teaching

PTs who participated in this study mainly altered types of representing the multiplicative concept rather than a multiplicative concept itself within mathematical strategies. PTs also changed the structure type of the class rather than the learning goal within lesson design. When responding to Liam, PTs tended to provide physical experiences with concrete manipulatives which were not provided to other students, rather than modifying the multiplicative concept itself. And the physical experience tended to be implemented outside of the lesson as an individual activity without a specialized goal for Liam. In other words, PTs selected modification of process and environment rather than modification of content in responding to each student profile. Teacher educators need to pay attention to supporting PTs’ modification of content that makes it possible to respond to all students.

The lesson plan approach of the multiplicative concept as described in the lesson design of some PTs suggests how content modification can be supported in preservice teacher education. They altered the external representation according to individual students’ needs while keeping the modeled situation for multiplication. Students can obtain a better understanding when the multiplicative concept is expressed in a variety of models and is explained as the relationship between those expressions [57]. Modification on external representation can serve as a key strategy to enable quality learning opportunities for students with special needs. The good news for teacher educators is that PTs understood that the concept of multiplication in CCSSM standard 3.OA.1 [62] is the equal group situation, and possible external representations for equal group situations are grouping, number-line, and array models. If preservice teacher education provided the experience of modification and accommodations of mathematics concepts itself, PTs would be better prepared for the differentiation of content to respond to all student profiles.

5.2. Modification and Accommodations for Responsive Teaching

The modification and accommodation strategies of PTs responding to each student profile indicated supports with a specific form according to the profile. In the case of the alternation of types of representing the multiplicative concept, most PTs had tended to provide physical experience for Liam. It seems quite responsive and appropriate considering the profile of Liam. The challenges were around diving deeply into understanding individual student needs. PTs indicated more emphasis on deficits than the strengths of the individual student. With Liam there was only discussion of teacher support, when peer support may have lessened his anxiety.

Another point worth noting is that, unlike in the case for Jose, there was no PT who provided symbolic objectification support to Liam. Further, too many of the PTs were focused on low level work and did not seek to challenge Liam with high level thinking requirements. It means that differentiated assistance provided to students with special needs could have the possibility to limit the chances of approaching the abstract mathematical concepts. The PTs were provided with opportunities to read and discuss best practice throughout their university courses, but it is apparent they need more best practice modeled in class and in the field for it to translate into their own lesson planning. If one student does not receive quality opportunities to learn, the instruction cannot be called responsive. Therefore, even though Liam was labeled MLD, PTs need to set high expectations and seek a way to link the student’s personal strengths with those expectations. Given that teachers need to set high expectations for each student and give opportunities to reach these expectations, preservice teacher education needs to focus on learning to support as well as challenge all types of students.

5.3. From Band-Aid Solutions to Sustainable Support

PTs tend to accommodate students’ struggles through one-on-one teacher time during the class or spending additional time with them at an undetermined future period. For students with special needs, PTs did not seek ways to “ensure shared power” through inviting them to engage in whole-class or small group discussion and by encouraging them to share their ideas or respond to one another’s ideas [58] (p. 114). The first step in all good instruction is to look at prior assessments to build upon. The PTs did not mention the use of student data to drive their lesson design process. Preservice teacher educators need to think about PTs’ response strategies more in terms of sustainability rather than as just temporary solutions.

Previous studies have noted that there are several explicit instructional strategies to support students with different needs in a sustainable way. Schema-Based Instruction is a representational technique, such as CRA strategy. It emphasizes understanding of problem schemata and allows the student to solve a group of seemingly different but similarly constructed problems [74,75]. Another alternative instructional strategy from the literature is Class Wide Peer Tutoring (CWPT). CWPT is not unique to multiplication instruction but can be used to help students with MLD learn their multiplication facts. As stated earlier it involves training the teacher and students in peer tutoring procedures. There is a reinforcement system that includes rewards for accurate responses and adhering to procedures [31,76,77]. It could be effective for MLD students in that it goes beyond regular practice, in terms of support and reinforcement.

6. Implications

The implications of this study are many. While the sample size was small, the demographics represent groups similar to those which the researchers have seen for at least 10 years, teaching students from a rather homogenous background about responding to learning differences is a worthwhile challenge for all teacher preparation programs. This study provides insights into defining the most common strategies and knowledge levels demonstrated by the group. We have better understanding of what they are attaining in their university coursework. Deep-diving into what PTs did, subsequently allowed us to identify gaps in the skills needed for applying research-based responsive teaching. The PTs need more instruction in peer tutoring and explicit teaching for MLDs. They all need more understanding of what it means to aim for high levels of cognitive demand for all students. As (pre-) teachers should learn to modify and accommodate instruction for diverse learners with explicit instructional strategies, teacher educators need to support PTs to construct and provide high-quality learning opportunities for improving their knowledge and practice.

Another implication of this study is that the analytical tools for responsive teaching in elementary level multiplication can be useful in capturing equitable learning opportunities in lesson design. By merging the two different perspectives of mathematics and special education, we enhanced the analytical tool to cover both mathematics and instructional strategies. The analytic tool enables teachers to find ways of modifying and accommodating mathematics instruction within the classroom, so that they can provide equitable responsive teaching for all students. We believe that this analytical tool contributed not only to the methodological lens of responsive teaching for all students but also to the theoretical lens. It allows both the mathematics and special education fields to make real progress on persistent, challenging problems in the teaching and learning of mathematics.

7. Limitations of the Study

The limitations of the study include the small sample size and narrow ethnic distribution of the sample. While the demographics were common for most teacher education programs in the United States, the lack of racial and disability diversity among the PTs may pose another barrier to relating to those from backgrounds differing from the majority culture of the teachers. It means that future research needs to be implemented with PTs from more varied demographics.