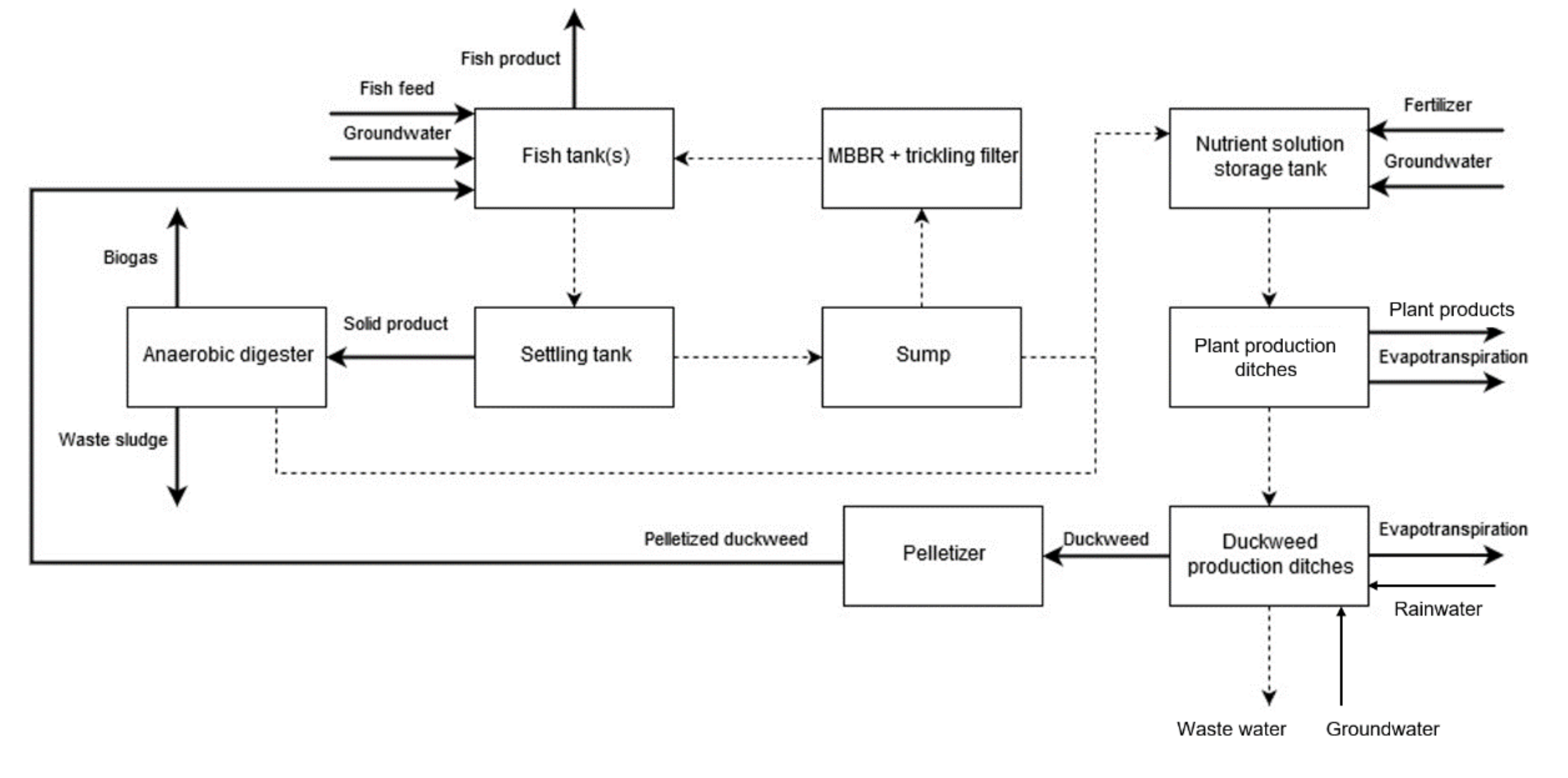
Appendix B. Equations and Symbols
Water balance
A volume balance for water is crucial to give insight on the water usage and nutrients flows within the system. The overall water balance is defined as:
in which
is total volume of water in the system,
is volumetric flow rate of inflowing groundwater in the AP,
is volumetric flow rate of rainwater entering the DCS,
is volumetric flow rate due to evapotranspiration in the HPS,
is volumetric flow rate due to evaporation of the DCS,
is fraction of fish sludge that ends up in the waste sludge, thus leaving the system,
is volumetric flow rate of fish sludge, and
is volumetric flow rate of water removed during dilution of the nutrients solution in the DCS.
Water level in the AP is constant, thus:
The volumetric flow rate of fish sludge is calculated from:
In which
is mass flow rate of commercial feed entering the RAS at time t,
is dry matter content of commercial feed enter the RAS,
is mass flow rate of pelletized duckweed entering the RAS at time t,
is dry matter content of commercial feed enter the RAS,
is total suspended solids, which is mass fraction of feed (dry matter) that ends up as solids in the RAS,
is mass fraction of solids in the fish sludge, and
is density of the fish water.
Volume of water required for dilution of the nutrients solution (NS) in the HPS and DCS is given by:
In which
is volume of dilution in the nutrients solution in the HPS or DCS at time t,
is mass of x in the nutrients solution in the HPS or DCS at time t,
is volume of nutrients solution in the HPS or DCS,
is maximum concentration of x in the nutrients solution in the HPS or DCS,
is concentration of x in groundwater,
is concentration of x in the nutrients solution in the HPS or DCS at time t, x indicates nitrogen (N) or phosphorus (P). The equation holds for both HPS and DCS.
Water level in the RAS and HPS is constant, and the volume balance of water in these units is calculated using the same equation as Dijkgraaf et al. (2019). The volume balance of water in the DCS is also constant and defined as
In which
is total volume of water in the DCS, and
is volumetric flow rate of groundwater into the DCS.
Meanwhile, the volume of water required to replace water in the RAS is defined as
In which
is volume of dilution of the RAS at time t,
is mass of x in the RAS at time t,
is volume of the RAS,
is maximum concentration of x allowed in the RAS,
is concentration of x in groundwater,
is concentration of x in the RAS at time t, and x indicates NH
3-N or NO
3-N.
Nitrogen, Phosphorus, and COD mass balance
The mass balances of N and P for the DCS are shown in this paragraph. In the RAS, HPS, and ADU, the N and P mass balance used for the FW + DW system is similar to the FW − DW system. The COD mass balance in the RAS also holds for both systems. The mass balances follow from Dijkgraaf et al. (2019) [
20]. The only difference is that there are two sources of feed in FW + DW, which are the commercial and duckweed pellet. In the FW − DW, the mass of N and P in the feed is defined as:
while in FW + DW, the mass of N and P from the feed is defined as:
In which
is mass of x in the feed at time t,
mass fraction of x in commercial feed,
is mass fraction of x in duckweed feed, and x indicates nitrogen (N) or phosphorus (P).
Further, the mass balance of N and P in the HPS after dilution is different from what Dijkgraaf et al. (2019) used. In the current study, it is defined as:
in which
is mass of x after dilution,
is mass of x in the HPS at time t (mg), and
is volume of dilution in the nutrient solution in the HPS.
The mass balance of N and P in the DCS is defined as
in which
is total mass of x in the DCS,
is mass concentration of x in the HPS,
is mass concentration of x in the groundwater,
is mass concentration of x in the rainwater,
is mass flow rate of x supplement into the nutrients solution in DCS,
is the volume of water in the DCS, and x indicates nitrogen (N) or phosphorus (P).
For the mass balance of N in the DCS, total N is used, which comes from NO
3-N and TAN. Therefore, the mass concentration of N that enters the DCS from the HPS and groundwater is defined as:
In which
is mass concentration of N in the HPS,
is mass concentration of NO
3-N in the HPS,
is mass concentration of TAN in the HPS,
is mass concentration of N in groundwater,
is mass concentration of NO
3-N in the groundwater, and
is mass concentration of TAN in the groundwater.
The mass flow rate of nutrients supplement N and P in the nutrients solution in HPS and DCS at time t is similar, and is defined as:
In which
is mass flow rate of N supplement into the nutrients solution,
is volume of the nutrients solution,
is mass concentration minimum of nutrients x in the nutrients solution,
is mass of nutrients x in the nutrients solution at time t,
is time period in which supplement is added to the nutrients solution,
is mass concentration of nutrients x in the nutrients solution at time t,
is minimum concentration of x in the nutrients solution in the HPS or DCS, x indicates nitrogen (N) or phosphorus (P), and NS indicates nutrients solution, which is HPS or DCS.
Energy balance
In the energy balance, energy is generated from a generator that converts methane produced from anaerobic digestion into electricity. The electricity is consumed constantly by two identical pumps and a blower, which holds for both systems. When energy shortage occurs, electricity is bought from a national electricity company. Hence, the energy balance can be defined as:
In which
is production energy by the generator,
is external energy bought from a national electricity company,
is power of the pump,
is power of the blower. The production of energy by the anaerobic digestion in both FW + DW and FW − DW system is similar, hence, following Dijkgraaf et al. (2019).
Duckweed growth model
Duckweed growth is relevant to obtain understanding of the dynamics of the duckweed biomass in the system. Duckweed is grown in the DCS and harvested frequently to be used as fish feed in pellet form. Hence, the mass balance of duckweed in the system is defined as:
In which
is total mass of duckweed in the DCS,
is mass of duckweed produced in the DCS, and
is mass of pelletized duckweed from the DCS used as feed fish.
The daily production of duckweed biomass follows a logistic growth curve. The differential equation that describes the duckweed biomass dynamics is given by:
In which
is mass of duckweed produced,
is maximum growth rate of duckweed,
is maximum density of duckweed in the DCS,
is cultivation area of duckweed in the DCS.
The amount of pelletized duckweed available to be used as fish feed is given by:
In which
is mass of duckweed harvested from the DCS at time t,
is dry matter content of fresh duckweed,
is moisture content of pelletized duckweed, and
is dry matter content of pelletized duckweed.
Appendix C. Additional Model Outputs
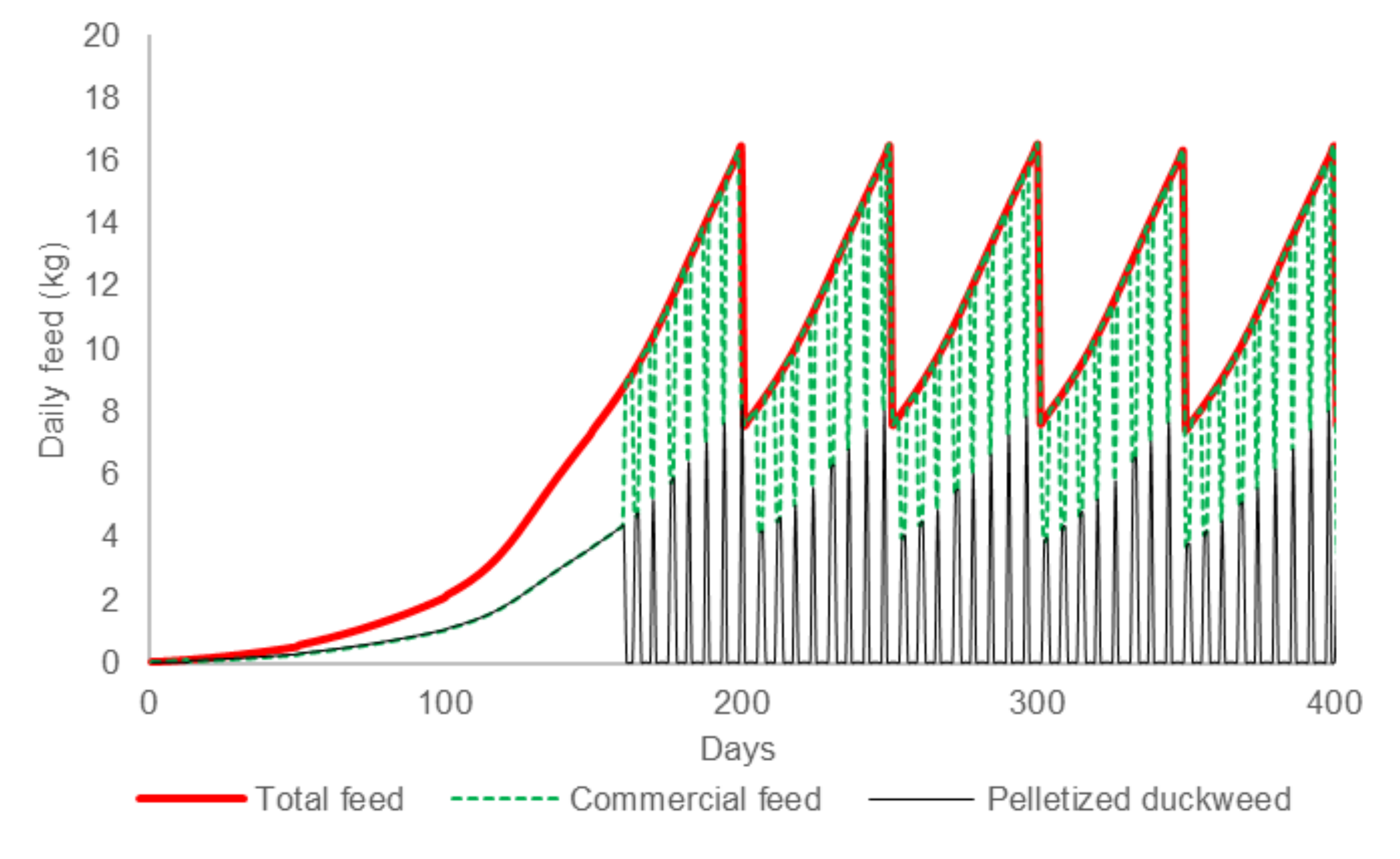
Figure A1.
Total daily feed needed, pelletized duckweed that can be supplied from a 1350 m2 duckweed pond, and commercial feed required to cover all feed requirement in the RAS.
Figure A1.
Total daily feed needed, pelletized duckweed that can be supplied from a 1350 m2 duckweed pond, and commercial feed required to cover all feed requirement in the RAS.
Figure A2.
Cumulative NUE of N of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A2.
Cumulative NUE of N of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A3.
Cumulative NUE of P of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A3.
Cumulative NUE of P of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A4.
Cumulative WUE of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A4.
Cumulative WUE of FW − DW, FW + DW, and BW system in the IndonesiAP system.
Figure A5.
Evaporation of rice in the HPS and duckweed in the DCS in the IndonesiAP system.
Figure A5.
Evaporation of rice in the HPS and duckweed in the DCS in the IndonesiAP system.
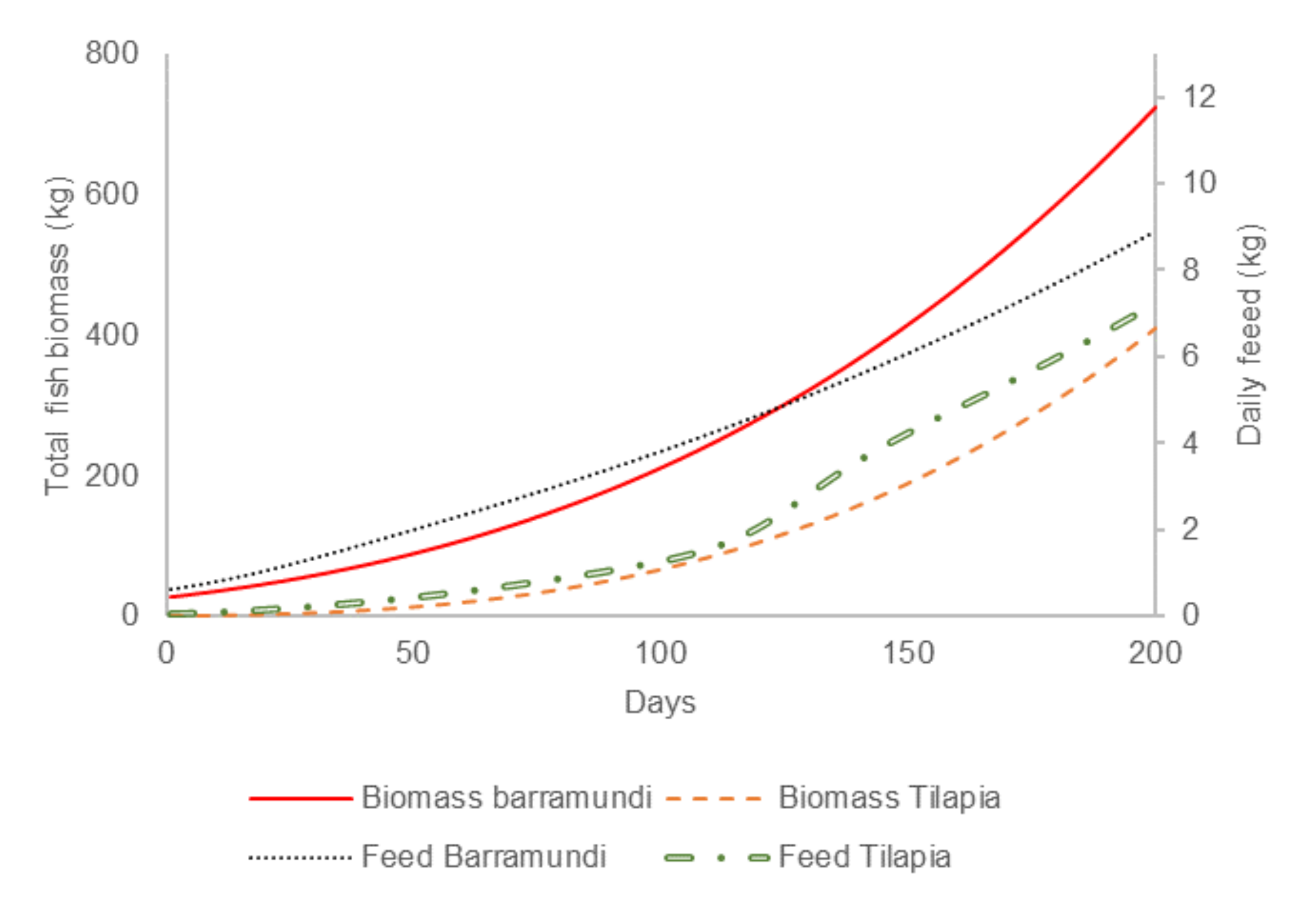
Figure A6.
FCR of Nile tilapia and barramundi during 200 days of cultivation [
20,
38].
Figure A6.
FCR of Nile tilapia and barramundi during 200 days of cultivation [
20,
38].
Figure A7.
NUE and WUE of IndonesiAP system in both FW + DW and FW − DW system at different P concentrations in the feed.
Figure A7.
NUE and WUE of IndonesiAP system in both FW + DW and FW − DW system at different P concentrations in the feed.
Figure A8.
NPV of BW system when tomato selling price, barramundi selling price, and HPS area are increased by 0 to 40% from the nominal value. Intersection between the horizontal line and the lines of the respective parameters shows conditions when NPV of the BW equals NPV of FW tomato.
Figure A8.
NPV of BW system when tomato selling price, barramundi selling price, and HPS area are increased by 0 to 40% from the nominal value. Intersection between the horizontal line and the lines of the respective parameters shows conditions when NPV of the BW equals NPV of FW tomato.
Figure A9.
Normalized sensitivity coefficient of NUE N, NUE P, and WUE after 50% increase and decrease of the values of eight selected parameters: duckweed upper limit density, percentage of duckweed harvested from DCS, DCS area, HPS area, RAS volume, fish density, and feed P and protein content in the IndonesiAP FW − DW and FW + DW systems.
Figure A9.
Normalized sensitivity coefficient of NUE N, NUE P, and WUE after 50% increase and decrease of the values of eight selected parameters: duckweed upper limit density, percentage of duckweed harvested from DCS, DCS area, HPS area, RAS volume, fish density, and feed P and protein content in the IndonesiAP FW − DW and FW + DW systems.
Figure A10.
Normalized sensitivity coefficient of NPV for increasing and decreasing 50% value of 14 selected parameters: fish density, duckweed upper limit density, RAS volume, percentage of duckweed harvested from DCS, feed protein and P content, HPS area, DCS area, labor cost, feed price, land price, investment cost of DCS, HPS, and RAS in the IndonesiAP FW − DW and FW + DW systems.
Figure A10.
Normalized sensitivity coefficient of NPV for increasing and decreasing 50% value of 14 selected parameters: fish density, duckweed upper limit density, RAS volume, percentage of duckweed harvested from DCS, feed protein and P content, HPS area, DCS area, labor cost, feed price, land price, investment cost of DCS, HPS, and RAS in the IndonesiAP FW − DW and FW + DW systems.
Figure A11.
Normalized sensitivity coefficient of NUE N, NUE P, and WUE for increasing and decreasing 50% value of five selected parameters: RAS volume, fish density, feed protein and P content, and HPS area in the IndonesiAP BW system.
Figure A11.
Normalized sensitivity coefficient of NUE N, NUE P, and WUE for increasing and decreasing 50% value of five selected parameters: RAS volume, fish density, feed protein and P content, and HPS area in the IndonesiAP BW system.
Figure A12.
Normalized sensitivity coefficient of NPV for increasing and decreasing 50% value of 12 selected parameters: fish price, tomato price, HPS area, feed price, investment cost HPS and RAS, labor cost, land price, RAS volume, fish density, and feed P and protein content in the IndonesiAP BW system.
Figure A12.
Normalized sensitivity coefficient of NPV for increasing and decreasing 50% value of 12 selected parameters: fish price, tomato price, HPS area, feed price, investment cost HPS and RAS, labor cost, land price, RAS volume, fish density, and feed P and protein content in the IndonesiAP BW system.