Efficiency Evaluation and Selection Strategies for Green Portfolios under Different Risk Appetites
Abstract
:1. Introduction
2. Literature Review
2.1. Research on DEA-Based Efficiency Evaluation
2.2. Research on Cumulative Prospect Theory
2.3. Research on Green Investment
2.4. Review
3. Problem Description and Underlying Assumptions
3.1. Anchor Point Deviation
3.2. Perceived Value Function
3.3. Weighting Function
4. Construction of a Green Portfolio Efficiency Model Based on Cumulative Prospect Theory
4.1. Model Setting
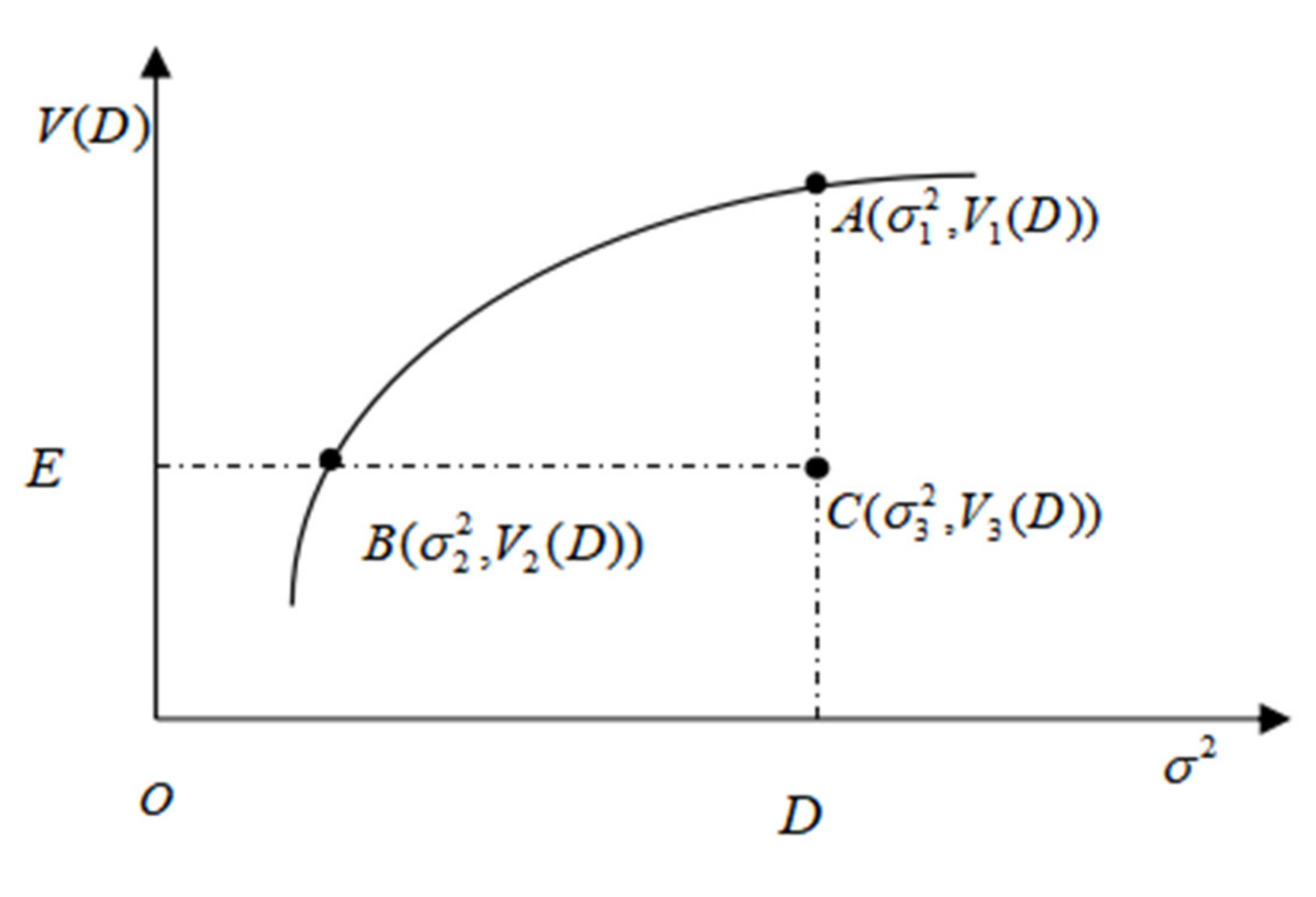
4.2. Definition of Efficiency
4.3. A Green Portfolio Efficiency Evaluation Model Based on Cumulative Prospect Theory
5. Simulation Analysis
5.1. Data Selection and Earnings Distribution
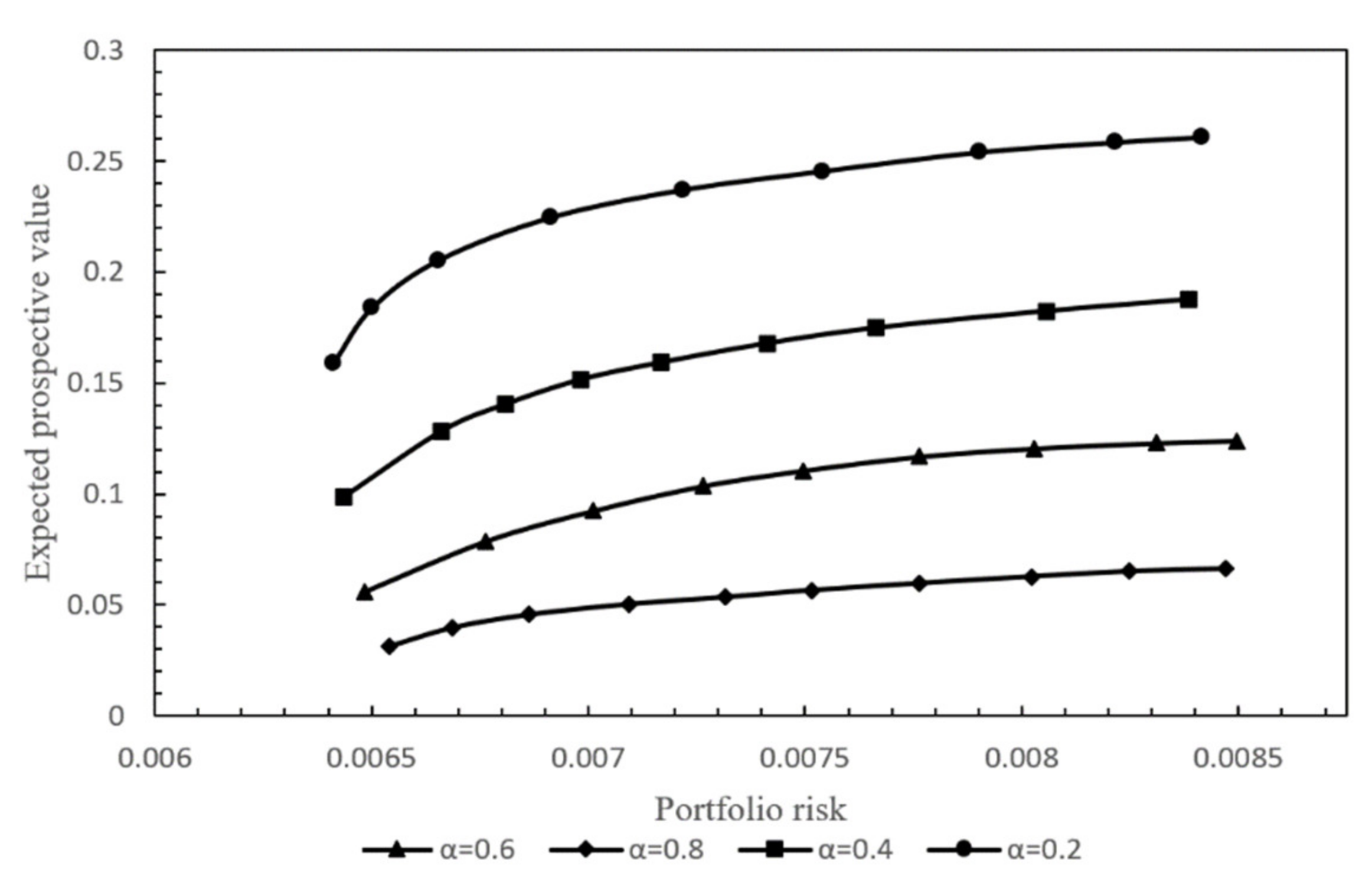
5.2. Comparative Analysis of Efficiency Frontiers under Different Risk Preferences
5.3. Green Portfolio Efficiency Evaluation Analysis Based on the Cumulative Prospect Theory Framework
6. Conclusions and Insights
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

| DMU | α = 0.2 | α = 0.4 | α = 0.6 | α = 0.8 | ||||
|---|---|---|---|---|---|---|---|---|
| Score | Rank | Score | Rank | Score | Rank | Score | Rank | |
| 1 | 0.3563 | 14 | 0.6214 | 14 | 0.7703 | 11 | 1.0000 | 1 |
| 2 | 0.9676 | 6 | 0.8501 | 6 | 0.1231 | 14 | 0.9995 | 4 |
| 3 | 0.9895 | 5 | 0.8514 | 5 | 1.0000 | 1 | 0.9621 | 5 |
| 4 | 0.7124 | 12 | 1.0000 | 1 | 0.7855 | 9 | 0.8016 | 12 |
| 5 | 0.9490 | 7 | 0.8438 | 7 | 1.0000 | 1 | 0.7653 | 13 |
| 6 | 1.0000 | 1 | 0.7569 | 11 | 0.8791 | 7 | 0.9329 | 7 |
| 7 | 0.7968 | 10 | 1.0000 | 1 | 0.8648 | 8 | 1.0000 | 1 |
| 8 | 0.7013 | 13 | 0.6436 | 13 | 0.8851 | 6 | 0.8427 | 11 |
| 9 | 1.0000 | 1 | 0.7512 | 12 | 0.9835 | 3 | 0.8427 | 10 |
| 10 | 0.8392 | 9 | 0.7753 | 9 | 0.7113 | 12 | 1.0000 | 1 |
| 11 | 1.0000 | 1 | 1.0000 | 1 | 0.9124 | 5 | 0.7123 | 14 |
| 12 | 0.8608 | 8 | 0.8124 | 8 | 0.7720 | 10 | 0.9590 | 6 |
| 13 | 0.7427 | 11 | 0.8887 | 4 | 0.9785 | 4 | 0.8759 | 8 |
| 14 | 1.0000 | 1 | 0.7627 | 10 | 0.1242 | 13 | 0.8524 | 9 |
| DMU | β = 0.3 | β = 0.5 | β = 0.7 | β = 0.9 | ||||
|---|---|---|---|---|---|---|---|---|
| Score | Rank | Score | Rank | Score | Rank | Score | Rank | |
| 1 | 0.9827 | 6 | 0.5593 | 8 | 1.0000 | 1 | 0.3463 | 14 |
| 2 | 0.9282 | 11 | 0.4366 | 13 | 0.4153 | 12 | 0.9013 | 7 |
| 3 | 1.0000 | 1 | 1.0000 | 1 | 0.6714 | 10 | 0.4325 | 13 |
| 4 | 0.9879 | 5 | 0.3253 | 14 | 0.5973 | 11 | 0.5468 | 12 |
| 5 | 0.9984 | 4 | 0.7621 | 5 | 1.0000 | 1 | 0.9812 | 3 |
| 6 | 0.6565 | 12 | 1.0000 | 1 | 0.7488 | 8 | 1.0000 | 1 |
| 7 | 0.5644 | 13 | 1.0000 | 1 | 1.0000 | 1 | 0.6751 | 11 |
| 8 | 0.1167 | 14 | 0.6160 | 7 | 0.7355 | 9 | 0.9022 | 6 |
| 9 | 1.0000 | 1 | 0.5572 | 9 | 0.0667 | 14 | 0.9169 | 5 |
| 10 | 0.9436 | 10 | 0.5031 | 11 | 0.7590 | 7 | 0.9211 | 4 |
| 11 | 0.9533 | 8 | 1.0000 | 1 | 1.0000 | 1 | 0.8890 | 9 |
| 12 | 1.0000 | 1 | 0.6312 | 6 | 0.2365 | 13 | 1.0000 | 1 |
| 13 | 0.9765 | 7 | 0.4938 | 12 | 1.0000 | 1 | 0.8891 | 8 |
| 14 | 0.9524 | 9 | 0.5556 | 10 | 0.7732 | 6 | 0.8875 | 10 |
References
- Markowitz, H. Portfolio selection. J. Financ. 1952, 7, 77–91. [Google Scholar]
- Charnes, A.; Cooper, W.W.; Rhodes, E. Measuring the efficiency of decision making units. Eur. J. Oper. Res. 1978, 2, 429–444. [Google Scholar] [CrossRef]
- Daraioa, C.; Simarb, L. A robust nonparametric approach to evaluate and explain the performance of mutual funds. Eur. J. Oper. Res. 2006, 175, 516–542. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, S.; Lai, K.K. Mutual funds performance evaluation based on endogenous benchmarks. Expert Syst. Appl. 2011, 38, 3663–3670. [Google Scholar] [CrossRef]
- Galagedera, D.U.A.; Silvapulle, P. Australian mutual fund performance appraisal using data envelopment analysis. Manag. Financ. 2002, 28, 60–73. [Google Scholar] [CrossRef]
- Liu, W.B.; Zhou, Z.B.; Liu, D.B.; Xiao, H.L. Estimation of portfolio efficiency via DEA. Omega 2015, 52, 107–118. [Google Scholar] [CrossRef]
- Lim, S.; Oh, K.W.; Zhu, J. Use of DEA cross-efficiency evaluation in portfolio selection: An application to Korean stock market. Eur. J. Oper. Res. 2014, 236, 361–368. [Google Scholar] [CrossRef]
- Choi, H.S.; Min, D. Efficiency of well-diversified portfolios: Evidence from data envelopment analysis. Omega 2017, 73, 104–113. [Google Scholar] [CrossRef]
- Banihashemi, S.; Navid, A.S. Portfolio performance evaluation in mean-CVaR framework: A comparison with non-parametric methods value at risk in mean-VaR Analysis. Oper. Res. Perspect. 2017, 4, 21–28. [Google Scholar] [CrossRef]
- Zhou, Z.B.; Xiao, H.L.; Jin, Q.Y.; Liu, W.B. DEA frontier improvement and portfolio rebalancing: An application of China mutual funds on considering sustainability information disclosure. Eur. J. Oper. Res. 2017, 269, 1–21. [Google Scholar] [CrossRef]
- Zhou, Z.B.; Ding, H.; Ma, C.Q.; Wang, M.; Liu, W.B. Technical efficiency evaluation approach for portfolios with transaction costs. Chin. J. Manag. Sci. 2015, 23, 25–33. [Google Scholar]
- Zhou, Z.B.; Lu, M.; Xiao, H.L.; Jin, Q.Y. Performance evaluation of multi-period portfolios based on the network index DEA. Commer. Sci. Res. 2016, 23, 98–103. [Google Scholar]
- Zhou, Z.B.; Liu, P.; Yu, H.N.; Ma, C.Q.; Liu, W.B. Performance evaluation of multi-period portfolios on considering transaction costs. Chin. J. Manag. Sci. 2015, 23, 1–6. [Google Scholar]
- Zhou, Z.B.; Jin, Q.Y.; Zeng, X.M.; Wu, Q.; Liu, W.B. Performance evaluation of portfolios with cardinality constraints. Chin. J. Manag. Sci. 2017, 25, 174–179. [Google Scholar]
- Lin, R.Y.; Li, Z.X. Directional distance based diversification super-efficiency DEA models for mutual funds. Omega 2020, 97. [Google Scholar] [CrossRef]
- Xiao, H.L.; Ren, T.T.; Zhou, Z.B.; Liu, W.B. Parameter uncertainty in estimation of portfolio efficiency: Evidence from an interval diversification-consistent DEA approach. Omega 2020. [Google Scholar] [CrossRef]
- Allais, M. Le Comportement de L’homme Rationnel Devant Le Risque: Critique des postulats et axiomes de l’ecole Americaine. Econometrica 1953, 21, 503–546. [Google Scholar] [CrossRef]
- Ellsberg, D. Risk, Ambiguity, and the Savage axioms. Q. J. Econ. 1961, 75, 643–669. [Google Scholar] [CrossRef] [Green Version]
- Tversky, K.A. Prospect theory: An analysis of decision under risk. Econometrica 1979, 47, 263–292. [Google Scholar]
- Kahneman, A.T. Advances in prospect theory: Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar]
- Pirvu, T.A.; Schulze, K. Multi-stock portfolio optimization under prospect theory. Math. Financ. Econ. 2012, 6, 337–362. [Google Scholar] [CrossRef]
- Gong, C.; Xu, C.; Wang, J. An efficient adaptive real coded genetic algorithm to solve the portfolio choice problem under cumulative prospect theory. Comput. Econ. 2018, 52, 227–252. [Google Scholar] [CrossRef]
- Gong, C.; Xu, C.; Ando, M.; Xi, X.M. A new method of portfolio optimization under cumulative prospect theory. Tsinghua Sci. Technol. 2018, 23, 75–86. [Google Scholar] [CrossRef]
- Consigli, G.; Hitaj, A.; Mastrogiacomo, E. Portfolio choice under cumulative prospect theory: Sensitivity analysis and an empirical study. Comput. Manag. Sci. 2019, 16, 129–154. [Google Scholar] [CrossRef]
- Bernheim, B.D.; Sprenger, C. On the empirical validity of cumulative prospect theory: Experimental evidence of rank-independent probability weighting. Econometrica 2020, 88, 1363–1409. [Google Scholar] [CrossRef]
- Hens, T.; Mayer, J. Cumulative prospect theory and mean variance analysis: A rigorous comparison. Swiss Financ. Inst. Res. Pap. Ser. 2014, 21, 14–23. [Google Scholar] [CrossRef] [Green Version]
- Ingersoll, J. Non-monotonicity of the Tversky-Kahneman probability-weighting function: A cautionary note. Eur. Financ. Manag. 2016, 14, 385–390. [Google Scholar] [CrossRef]
- Grishina, N.; Lucas, C.A.; Date, P. Prospect theory-based portfolio optimization: An empirical study and analysis using intelligent algorithms. Quant. Financ. 2017, 17, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Ino, H.; Matsumura, T. Promoting green or restricting gray? An analysis of green portfolio standards. Econ. Lett. 2021, 198. [Google Scholar] [CrossRef]
- Bento, A.M.; Garg, T.; Kaffine, D. Emissions reductions or green booms? General equilibrium effects of a renewable portfolio standard. J. Environ. Econ. Manag. 2018, 90, 78–100. [Google Scholar] [CrossRef] [Green Version]
- Chakrabarti, G.; Sen, C. Time series momentum trading in green stocks. Stud. Econ. Financ. 2020, 37, 361–389. [Google Scholar] [CrossRef]
- Allevi, E.; Basso, A.; Bonenti, F.; Oggioni, G.; Riccardi, R. Measuring the environmental performance of green SRI funds: A DEA approach. Energy Econ. 2019, 79, 32–44. [Google Scholar] [CrossRef]
- Zhai, J.; Bai, M.Y. Mean-risk model for uncertain portfolio selection with background risk. J. Comput. Appl. Math. 2018, 330, 59–69. [Google Scholar] [CrossRef]
- Liu, Y.J.; Zhou, M.N.; Zhang, W.G. Mean-semivariance portfolio optimization model with background risk. Syst. Eng. Theory Pract. 2020, 40, 2282–2291. [Google Scholar]
- Zhang, Y.J.; Chen, M.Y. Evaluating the dynamic performance of energy portfolios: Empirical evidence from the DEA directional distance function. Eur. J. Oper. Res. 2018, 269, 64–78. [Google Scholar] [CrossRef]
- Jin, J.Y.; Han, L.Y. Assessment of Chinese green funds: Performance and industry allocation. J. Clean. Prod. 2018, 171, 1084–1093. [Google Scholar] [CrossRef]


| DMU | α = 0.2 | α = 0.4 | α = 0.6 | α = 0.8 | ||||
|---|---|---|---|---|---|---|---|---|
| Score | Rank | Score | Rank | Score | Rank | Score | Rank | |
| 1 | 0.8429 | 9 | 0.9649 | 5 | 1 | 1 | 0.7448 | 13 |
| 2 | 0.8374 | 11 | 0.9429 | 7 | 0.9983 | 5 | 0.9052 | 6 |
| 3 | 0.8435 | 8 | 0.9735 | 4 | 0.9565 | 9 | 0.9597 | 4 |
| 4 | 1 | 1 | 0.9121 | 8 | 1 | 1 | 0.7089 | 14 |
| 5 | 0.9423 | 4 | 1 | 1 | 0.8920 | 11 | 0.8748 | 8 |
| 6 | 1 | 1 | 0.9012 | 9 | 1 | 1 | 0.9776 | 3 |
| 7 | 1 | 1 | 1 | 1 | 0.9654 | 8 | 1 | 1 |
| 8 | 0.9590 | 5 | 0.7561 | 11 | 1 | 1 | 0.9591 | 5 |
| 9 | 0.8481 | 7 | 0.7401 | 12 | 0.7784 | 14 | 0.8429 | 10 |
| 10 | 1 | 1 | 0.7392 | 13 | 0.9273 | 10 | 0.8526 | 9 |
| 11 | 0.8989 | 6 | 1 | 1 | 0.9926 | 6 | 0.8761 | 7 |
| 12 | 0.8420 | 10 | 0.7371 | 14 | 0.8603 | 12 | 0.8018 | 12 |
| 13 | 0.7765 | 13 | 0.9619 | 6 | 0.8177 | 13 | 1 | 1 |
| 14 | 0.8190 | 12 | 0.8159 | 10 | 0.9819 | 7 | 0.8411 | 11 |
| DMU | β = 0.3 | β = 0.5 | β = 0.7 | β = 0.9 | ||||
|---|---|---|---|---|---|---|---|---|
| Score | Rank | Score | Rank | Score | Rank | Score | Rank | |
| 1 | 0.8385 | 11 | 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 0.8828 | 10 | 0.8488 | 11 | 0.9534 | 11 | 0.9164 | 10 |
| 3 | 1 | 1 | 0.7079 | 14 | 0.9748 | 5 | 0.9176 | 9 |
| 4 | 0.8048 | 12 | 0.7842 | 12 | 0.9667 | 7 | 0.9639 | 5 |
| 5 | 0.8985 | 8 | 1 | 1 | 1 | 1 | 1 | 1 |
| 6 | 1 | 1 | 0.7719 | 13 | 0.8031 | 12 | 0.8972 | 13 |
| 7 | 0.8879 | 9 | 0.9223 | 8 | 0.8019 | 13 | 1 | 1 |
| 8 | 1 | 1 | 0.9429 | 7 | 0.9650 | 8 | 0.9264 | 8 |
| 9 | 1 | 1 | 1 | 1 | 0.9627 | 9 | 0.9888 | 4 |
| 10 | 0.9603 | 5 | 0.9847 | 4 | 0.9739 | 6 | 0.9308 | 7 |
| 11 | 0.6977 | 14 | 0.9669 | 5 | 0.9559 | 10 | 0.8924 | 14 |
| 12 | 0.9240 | 7 | 0.9040 | 9 | 1 | 1 | 0.9091 | 12 |
| 13 | 0.7855 | 13 | 0.8721 | 10 | 0.9898 | 4 | 0.9392 | 6 |
| 14 | 0.6814 | 15 | 0.9513 | 6 | 0.7739 | 14 | 0.9103 | 11 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, W.; Liu, S.; Ding, L. Efficiency Evaluation and Selection Strategies for Green Portfolios under Different Risk Appetites. Sustainability 2021, 13, 1933. https://doi.org/10.3390/su13041933
Yu W, Liu S, Ding L. Efficiency Evaluation and Selection Strategies for Green Portfolios under Different Risk Appetites. Sustainability. 2021; 13(4):1933. https://doi.org/10.3390/su13041933
Chicago/Turabian StyleYu, Wencheng, Shaobo Liu, and Lili Ding. 2021. "Efficiency Evaluation and Selection Strategies for Green Portfolios under Different Risk Appetites" Sustainability 13, no. 4: 1933. https://doi.org/10.3390/su13041933
APA StyleYu, W., Liu, S., & Ding, L. (2021). Efficiency Evaluation and Selection Strategies for Green Portfolios under Different Risk Appetites. Sustainability, 13(4), 1933. https://doi.org/10.3390/su13041933





