Optimal Operation Strategy for Wind–Hydrogen–Water Power Grids Facing Offshore Wind Power Accommodation
Abstract
:1. Introduction
- (1)
- Considering both the energy accommodation and freshwater input for electrolyzation, which are regarded as the energy input and raw material input of the system, this paper formulates a joint-operation power control strategy, establishing a wind–hydrogen–water power grid system to improve offshore wind power accommodation rate, freshwater production, and energy utilization rate;
- (2)
- The electrolyzer variable efficiency model is introduced to make full use of the flexible adjustment characteristics of the electrolyzer as a kind of detailed controllable load to match wind power fluctuations and to improve the system economy and reality;
- (3)
- In view of the problem of operation restriction arising from the direct connection between desalination and electrolysis, reservoir regulation is considered to reveal the uncertainty impacts of the reservoir capacity.
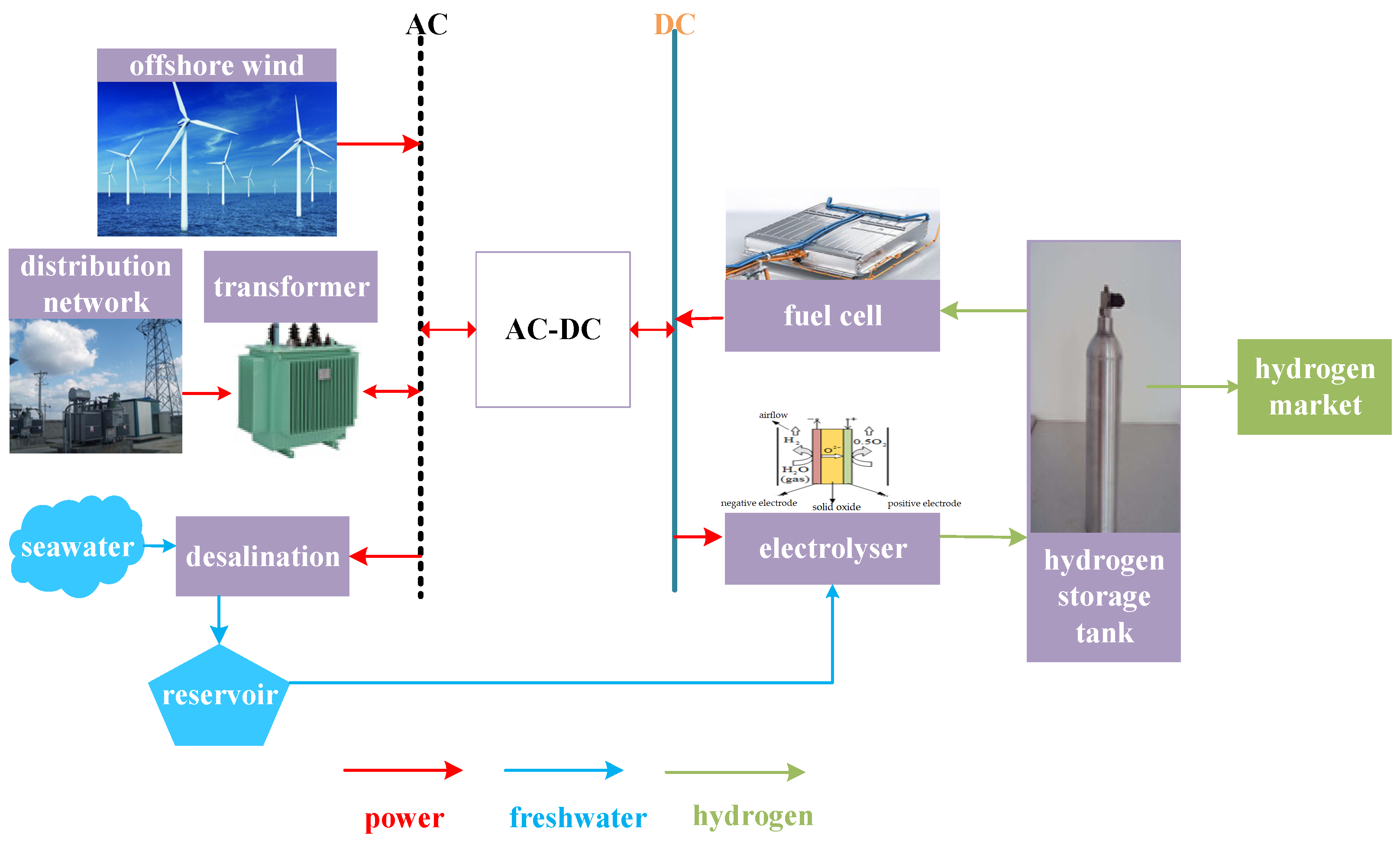
2. WHW-PGS Architecture and Mathematical Model
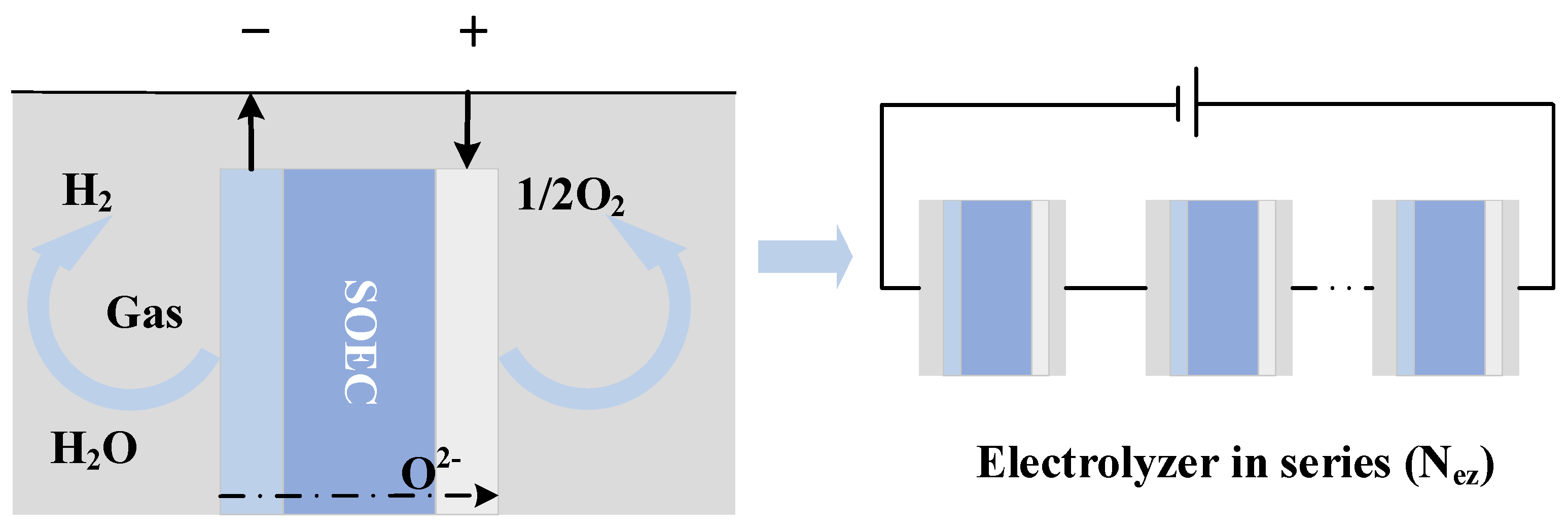
2.1. HES Mathematical Model
2.2. Desalination Mathematical Model
3. Optimal Operation Strategy of HES and Desalination
3.1. Operation Rules
3.2. Operation Strategy
4. Optimal Operation Model of WHW-HS
4.1. Objective Function
4.2. Constraints
5. Case Studies
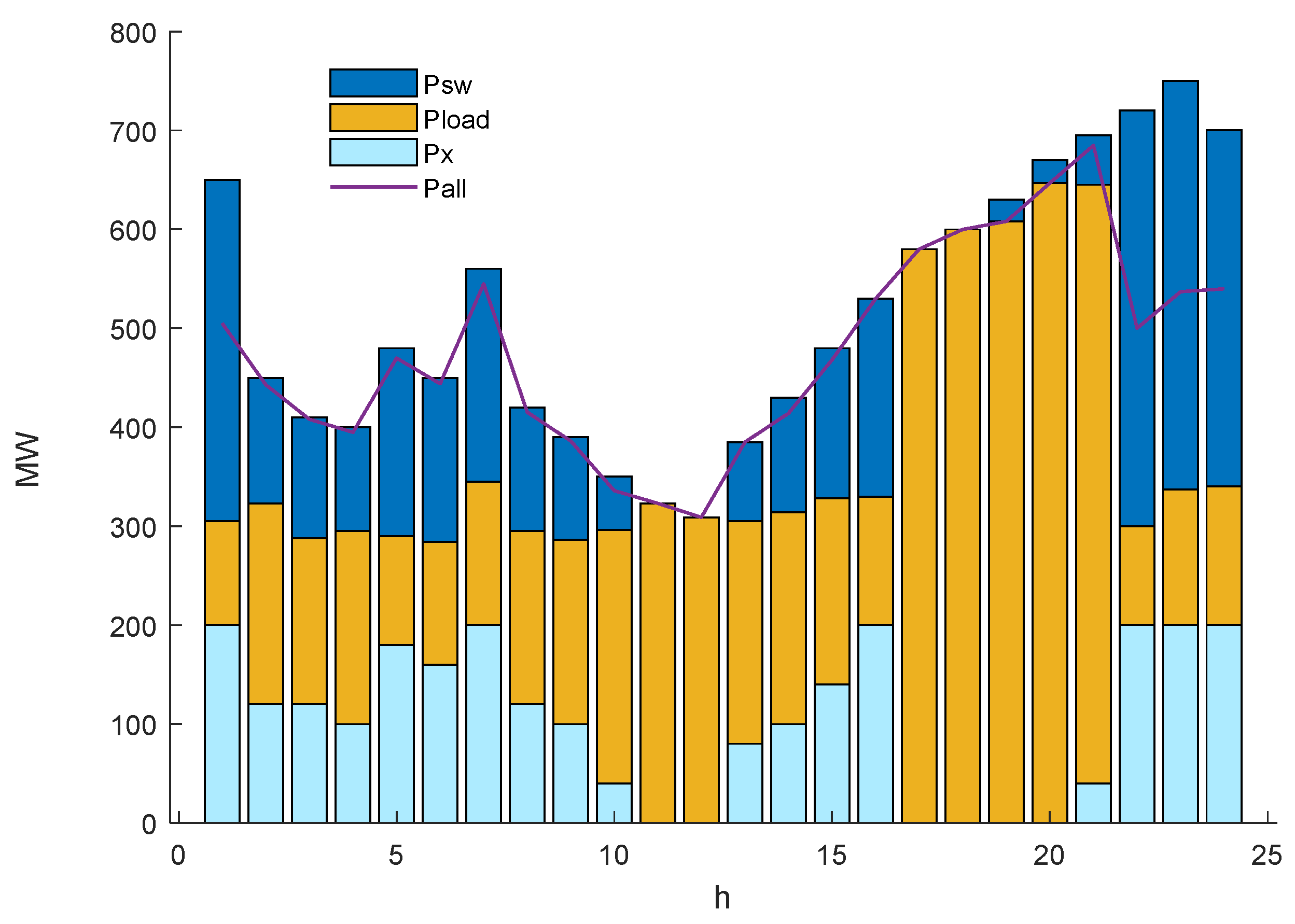
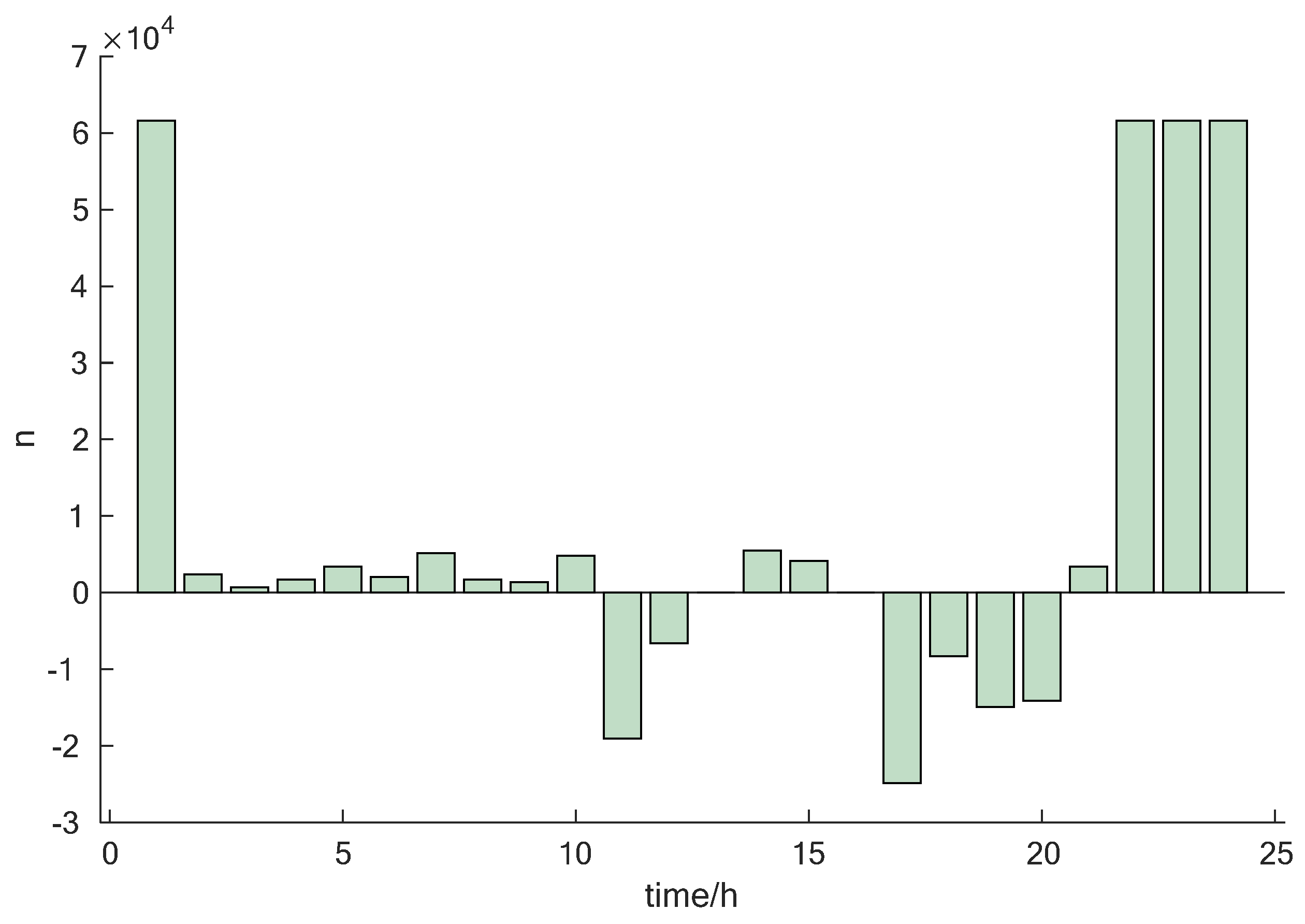
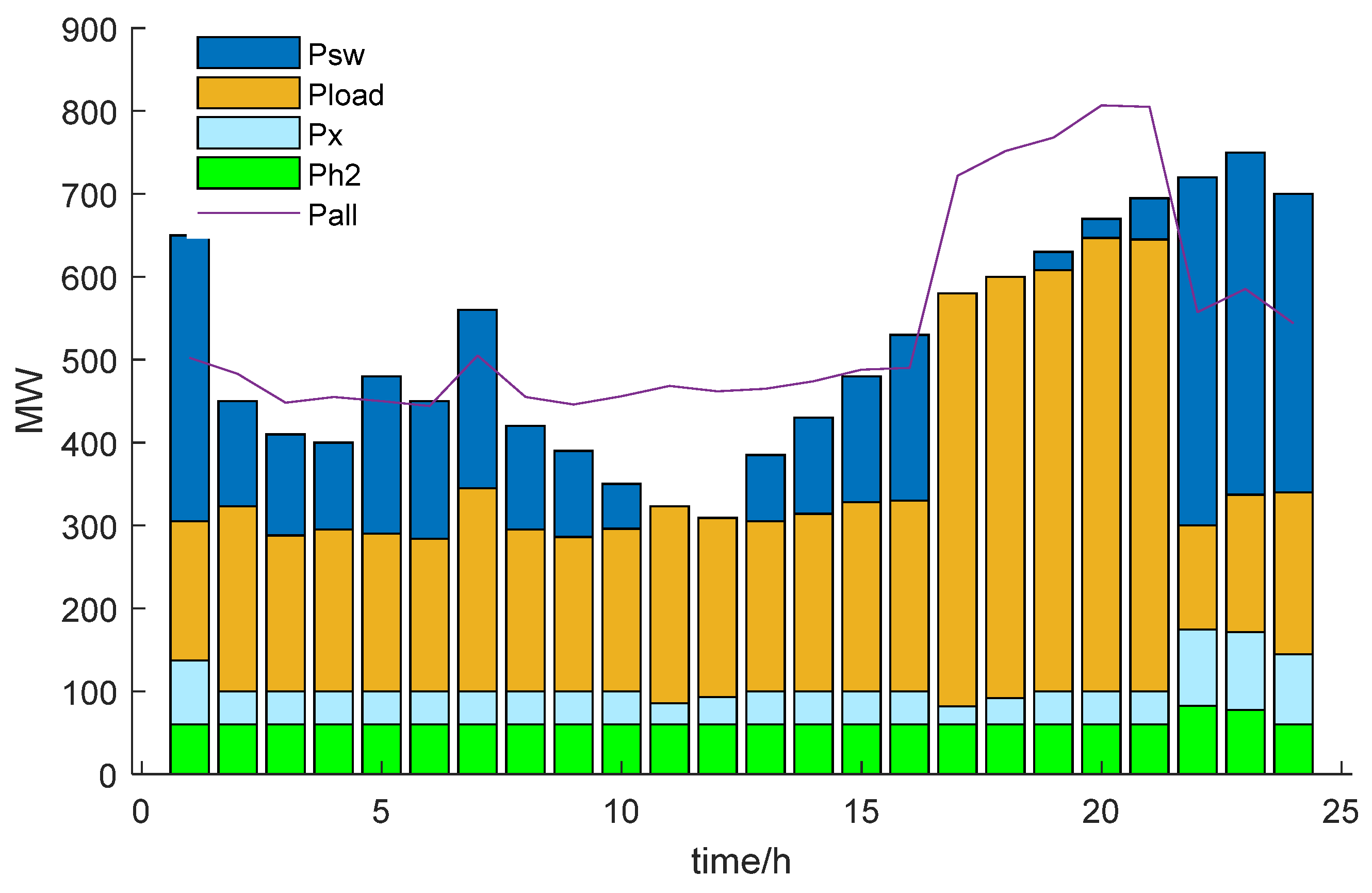
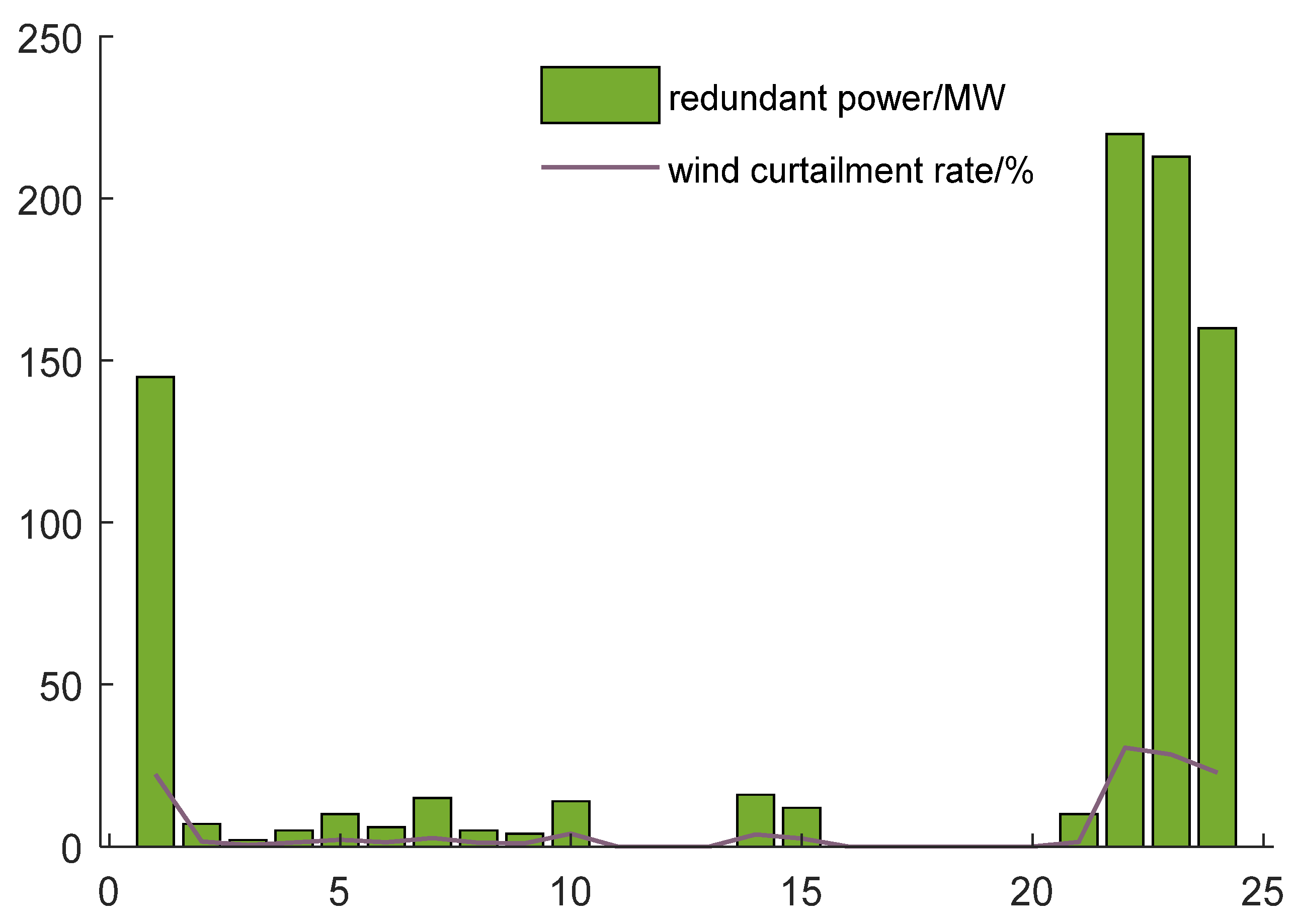
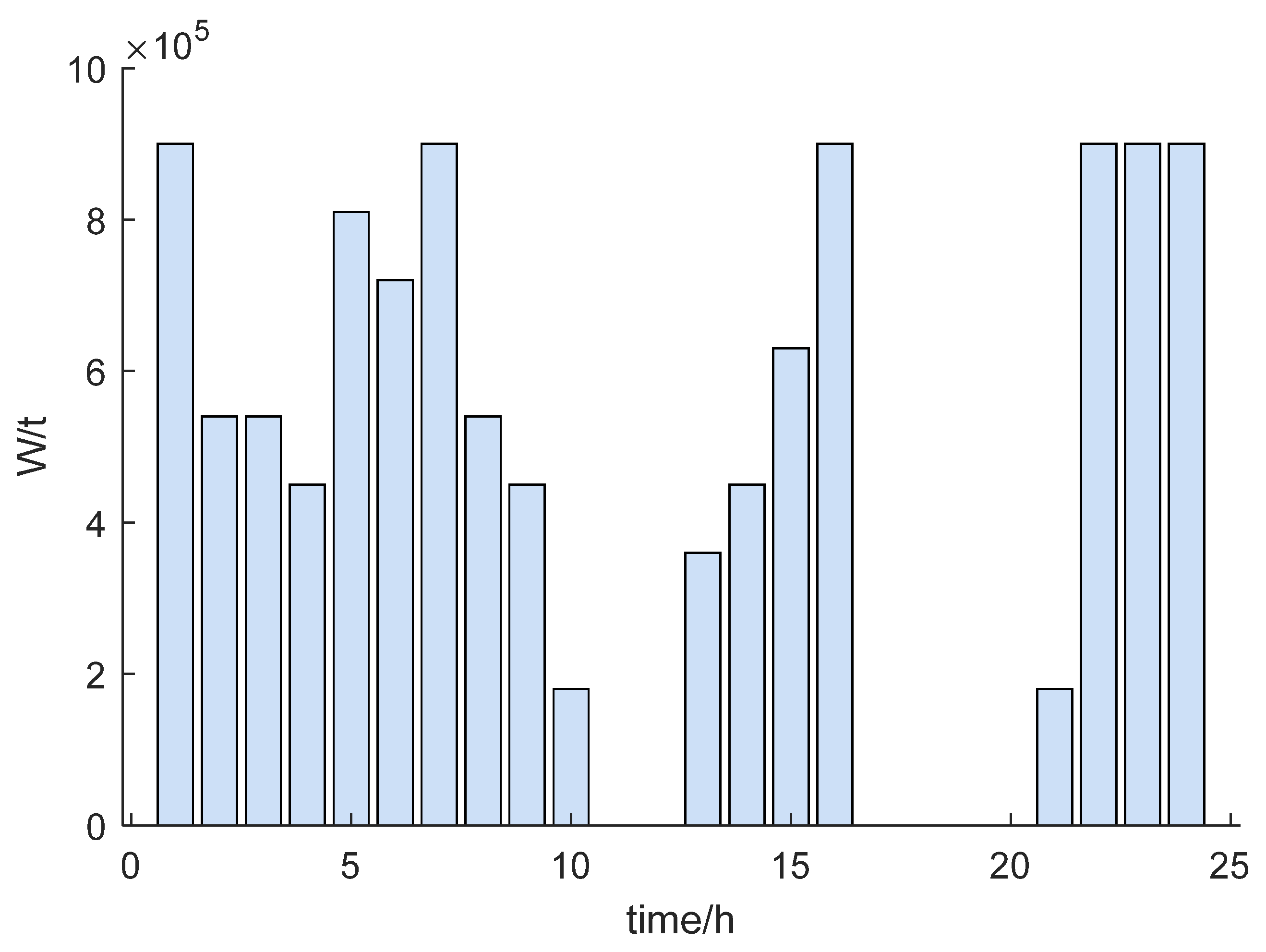
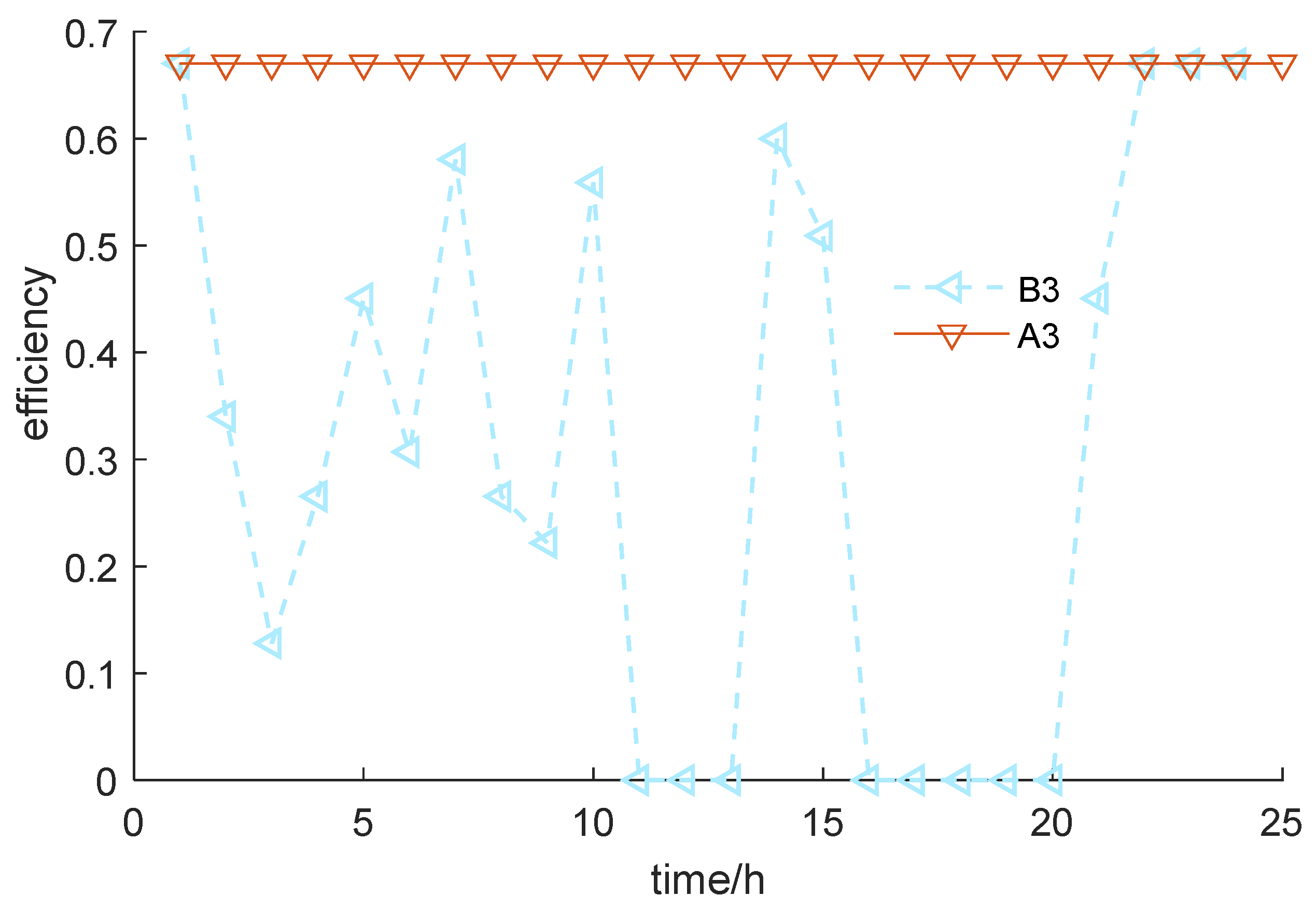
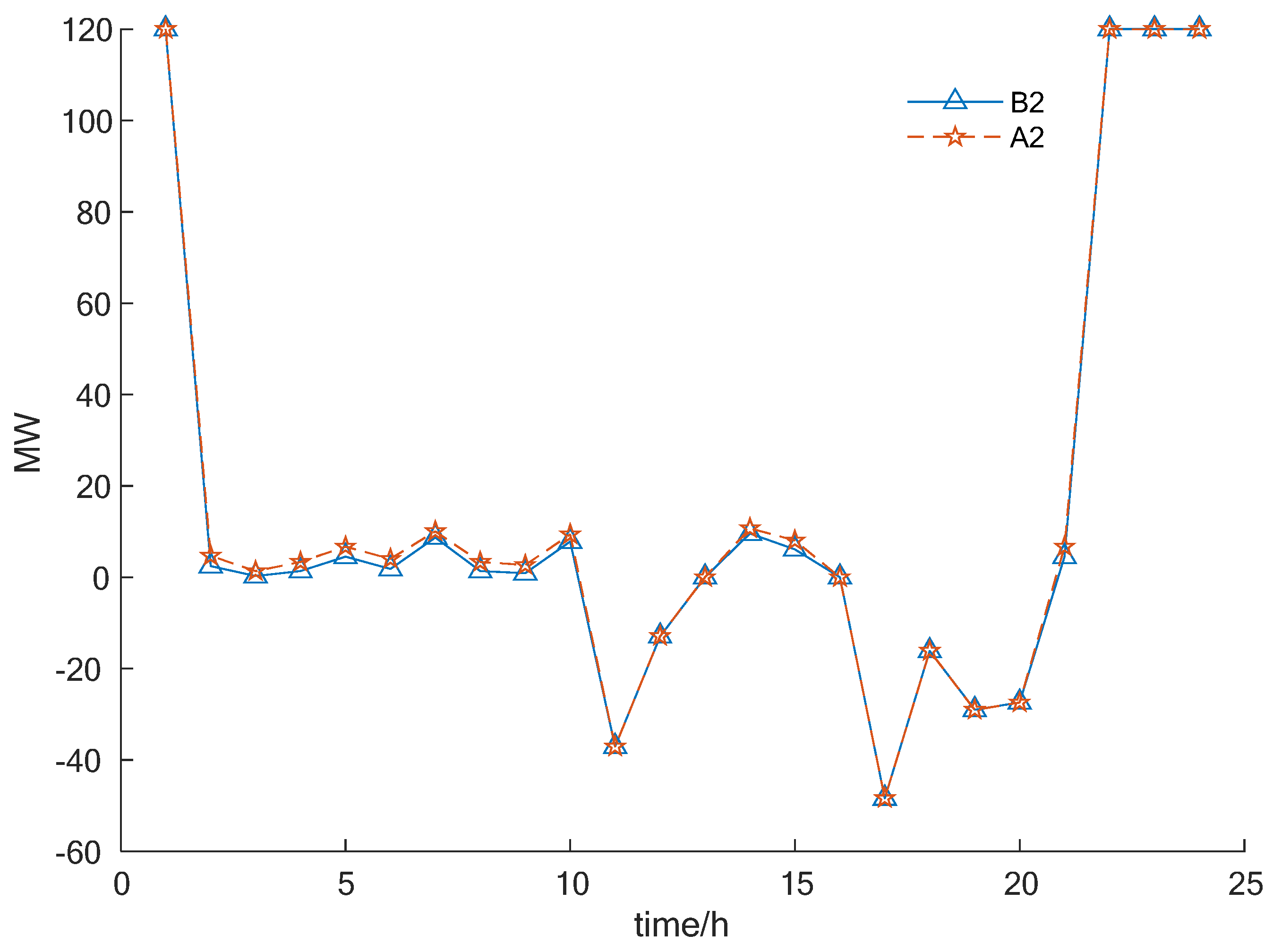
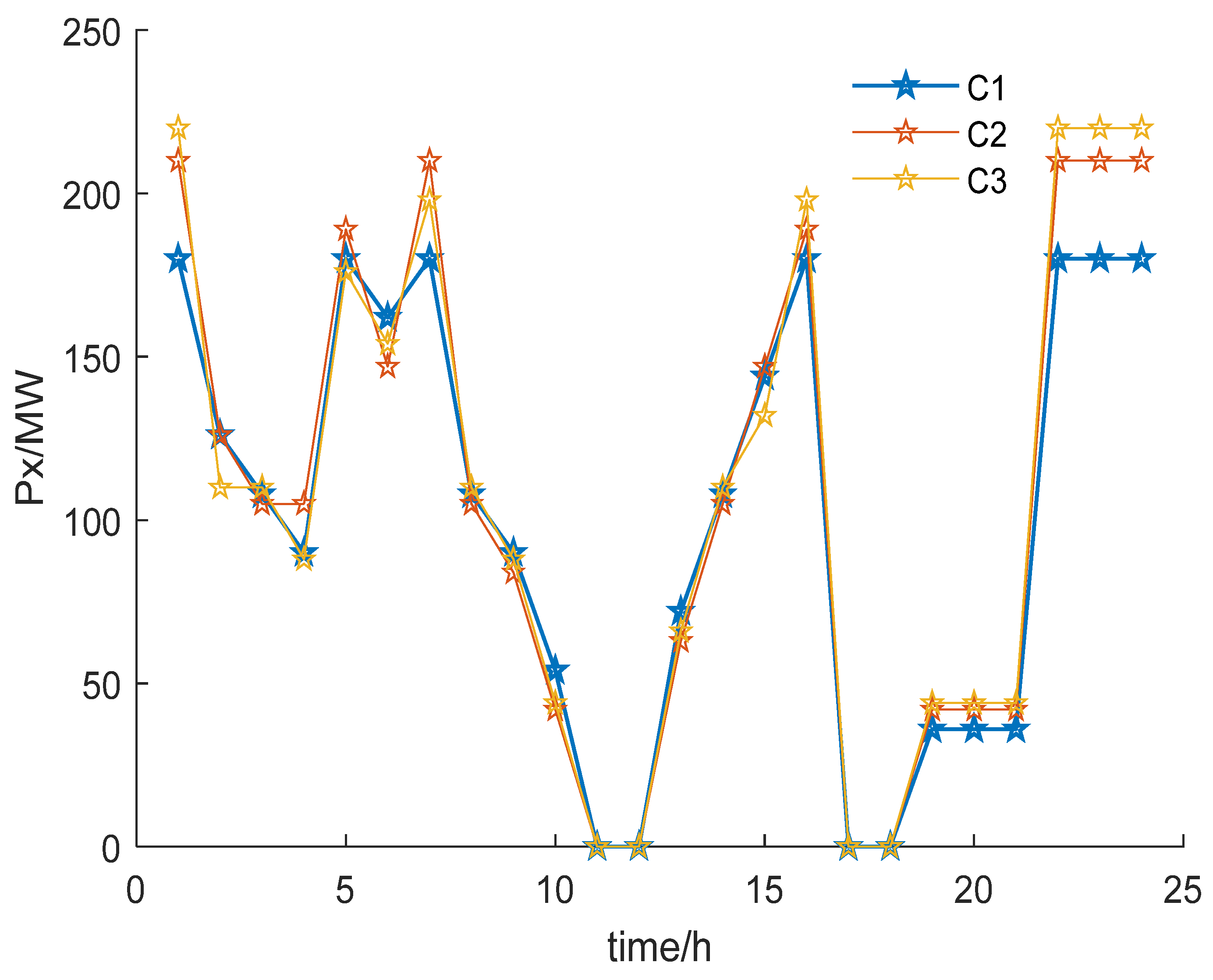
5.1. Analysis of the Impact of Different Operation Modes on the Plan
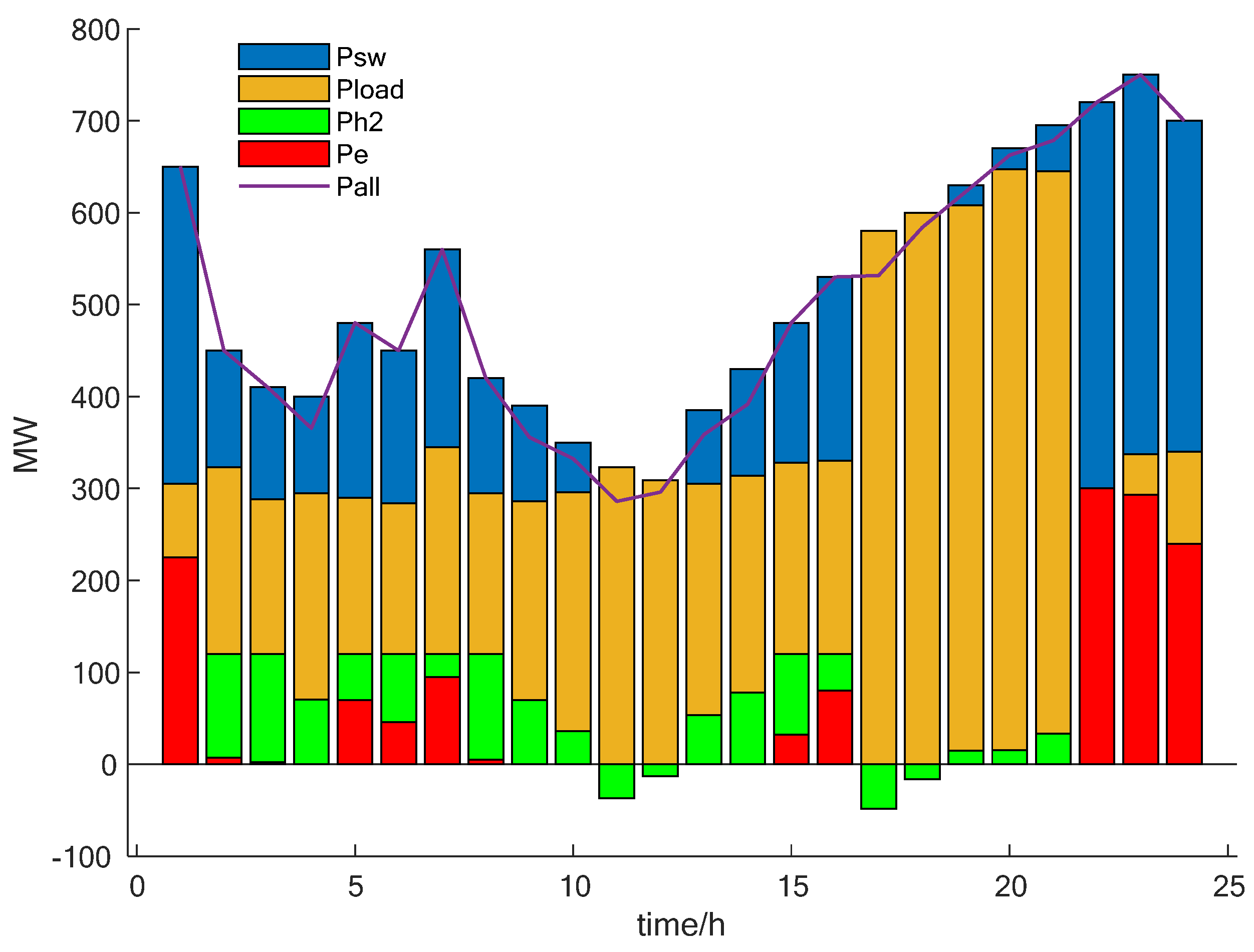
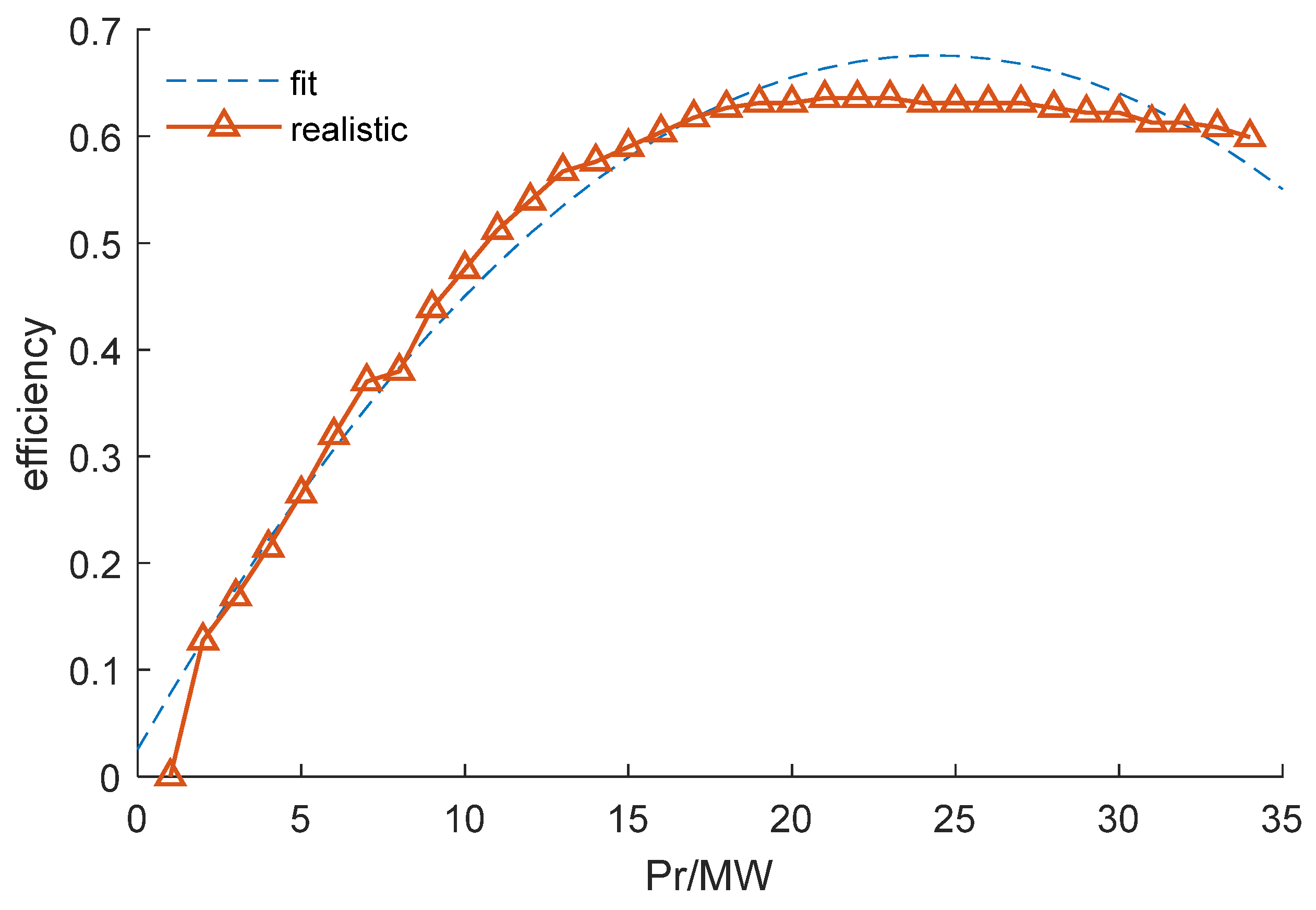
5.2. Analysis of the Influence of Changes in the Efficiency of Electrolyzer
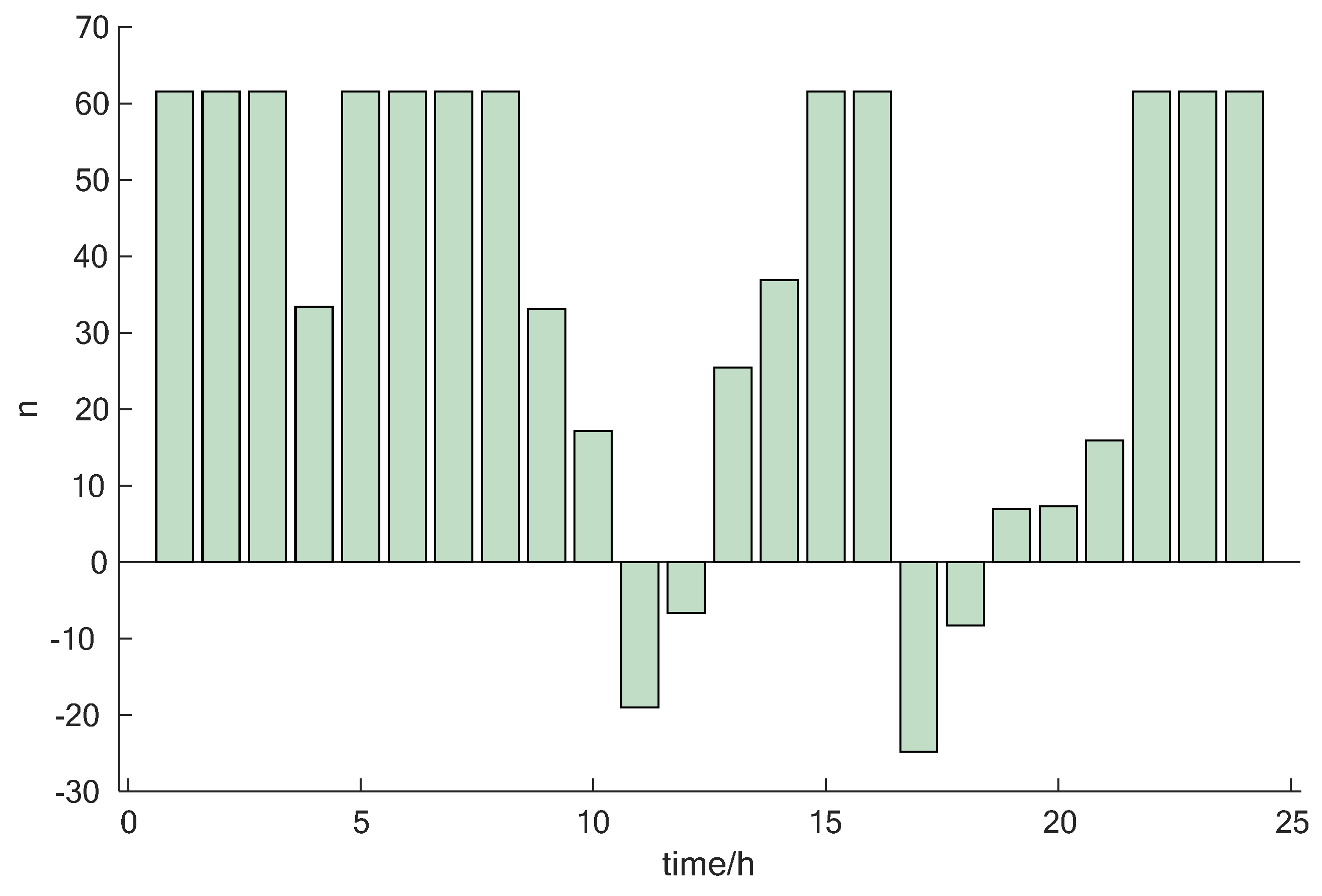
5.3. Analysis of Uncertainty Influence of Reservoir Capacity
6. Conclusions
- (1)
- Introducing the “Coastal multi-energy complementation” optimal operation strategy (JO-PCS), which can flexibly adjust and match wind power fluctuations, achieving peak shaving and valley filling, greatly reduces interactions with the public grid and reduces the average daily operating cost of the system.
- (2)
- Aiming at the efficiency-power characteristics of the electrolyzer, the variable efficiency model of the electrolytic cell is introduced to improve the operation strategy, which can make the system more economic and practical and avoid resource loss caused by the improper use of system equipment.
- (3)
- When the reservoir drops, the emergency freshwater supply of the system is insufficient, resulting in an increase in the number of desalination devices. Although energy consumption increases, the cost also increases.
- (1)
- With the increase in OWP offshore deep-sea HVDC transmission projects and the reduction in the investment cost of HES, it can effectively increase the utilization rate of renewable energy and promote the prosperity and development of coastal microgrids.
- (2)
- The double objective optimization operation, which considers the relationship between operation cost and the overall system’s energy efficiency as well as the impact of hydrogen storage on the system, will be a part of future studies.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| OWP | Offshore wind power |
| WHW-PGS | Wind–hydrogen–water power grid system |
| JO-PCS | Joint operation power control strategy |
| EVEM | Electrolyzer variable efficiency model |
| EHP | Electrolytic hydrogen production |
| HES | Hydrogen energy system |
| HST | Hydrogen storage tank |
| USE-WU | User satisfaction evaluation of water use |
| Performance parameter | |
| k | Impurities |
| SOC | State of charge |
| O | Rated capacity |
| Load | Conventional power consumption |
| a | Net |
| ov | Redundancy |
| sh | Defect |
| Cf | The average daily operating cost |
| Cu | Daily energy accommodation revenue |
| Cm | Daily average operation and maintenance cost |
| u | Unit price (accommodation) |
| m | Unit price (benefit) |
| ∆P | The climbing power |
| E | Battery capacity |
| Self-discharge coefficient | |
| S | User water satisfaction |
| Curtailment rate | |
| HHV | Calorific value of hydrogen |
| Q1 | External heat required for the electrolysis reaction |
| Q2 | Heat water to meet reaction heat |
| P | Electric power (MW) |
| W | Fresh water load in the reservoir (t) |
| N | Desalination’s Number |
| G | Freshwater production(t) |
| Y | Pressure |
| (t) | At time t |
| ez | Electrolyzer |
| fc | Full cell |
| x/w | Desalination |
| sw | Offshore wind power |
| e | Grid (1—forward, 2—opposite) |
| max | Upper limit |
| min | Lower limit |
| H2/H | Hydrogen |
| V | Certain voltage (Volt) |
| A | Whether the electrolytic cell is opened, run to take 1; otherwise take 0. |
| i | Electrolyzer’s number |
| F | Faraday constant |
| Faraday efficiency | |
| Efficiency | |
| T | Adiabatic temperature (K) |
| M | Quality of hydrogen (kg) |
| V | Volume (m3) |
| n | Moles of hydrogen |
| H2in | Input of hydrogen load |
| H2out | Output of hydrogen load |
| uw | Electrolytic water accommodation related to power accommodation |
| T1 | Temperature of the heated substance |
| T0 | Ambient temperature |
| user | Use |
References
- British Petroleum. Energy Outlook 2020 Edition; International Energy Agency: Paris, France, 2020. [Google Scholar]
- Anton, M.Z.G.; Chung, H.H.; Jui, C.L. Review of recent offshore wind power strategy in Taiwan: Onshore wind power comparison. Energy Strategy Rev. 2021, 38, 100747. [Google Scholar]
- Zheng, X.H.; Shi, C.X.; Wen, X.S. Review of factors affecting China’s offshore wind power industry. Renew. Sustain. Energy Rev. 2016, 56, 1372–1386. [Google Scholar]
- Qian, L.; Yan, S.; Mengcheng, W. Decision-making methodologies in offshore wind power investments: A review. J. Clean. Prod. 2021, 295, 126459. [Google Scholar]
- Kamal, J.R.G.L.; Fred, F.A. Structural dynamics of offshore Wind Turbines: A review. Ocean Eng. 2022, 251, 111136. [Google Scholar]
- Lin, S.; Zhao, H.; Zhu, L. Seawater desalination technology and engineering in China: A review. Desalination 2021, 498, 46–59. [Google Scholar] [CrossRef]
- National Development and Reform Commission and the State Oceanic Administration. The 13th Five-Year Plan for Nation; Seawater Utilization; National Development and Reform Commission: Beijing, China, 2016.
- Zheng, X.; Chen, D.; Wang, Q.; Zhang, Z. Seawater desalination in China: Retrospect and prospect. Chem. Eng. J. 2014, 242, 404–413. [Google Scholar] [CrossRef]
- Gong, S.; Wang, H.; Zhu, Z.; Bai, Q.; Wang, C. Comprehensive utilization of seawater in China: A description of the present situation, restrictive factors and potential countermeasures. Water 2019, 11, 397. [Google Scholar] [CrossRef] [Green Version]
- Prathapaneni, D.R.; Detroja, K. Optimal design of energy sources and reverse osmosis desalination plant with demand side management for cost-effective freshwater production. Desalination 2020, 496, 114741. [Google Scholar] [CrossRef]
- Karavas, C.; Arvanitis, K.G.; Papadakis, G. Optimal technical and economic configuration of photovoltaic powered reverse osmosis desalination systems operating in autonomous mode. Desalination 2019, 466, 97–106. [Google Scholar] [CrossRef]
- Abdelshafy, A.M.; Hassan, H.; Jurasz, J. Optimal design of a grid-connected desalination plant powered by renewable energy resources using a hybrid PSO–GWO approach. Energy Convers. Manag. 2018, 173, 331–347. [Google Scholar] [CrossRef]
- Liu, B.; Zhou, B.; Yang, D.; Li, G.; Cao, J.; Bu, S.; Littler, T. Optimal planning of coastal hybrid renewable energy system considering virtual energy storage of desalination plant based on mixed-integer NSGA-III. Desalination 2022, 521, 115382. [Google Scholar] [CrossRef]
- Zhou, B.; Liu, B.; Yang, D.; Cao, J.; Littler, T. Multi-objective optimal operation of coastal hydro-electrical energy system with seawater reverse osmosis desalination based on constrained NSGA-III. Energy Convers. Manag. 2020, 207, 112533. [Google Scholar] [CrossRef]
- Liu, X.; Li, N.; Mu, H.; Li, M.; Liu, X. Techno-energy-economic assessment of a high capacity offshore wind-pumped-storage hybrid power system for regional power system. J. Energy Storage 2021, 41, 102892. [Google Scholar] [CrossRef]
- Dakic, J.; Mane, M.C.; Bellmunt, O.G. Low frequency AC transmission systems for offshore wind power plants: Design, optimization and comparison to high voltage AC and high voltage DC. Int. J. Electr. Power Energy Syst. 2021, 133, 107273. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, T.; Xu, C.; Zhang, B.; Li, L.; Ke, Y.; Yan, Y.; Xu, R. Optimal location selection for offshore wind-PV-seawater pumped storage power plant using a hybrid MCDM approach: A two-stage framework. Energy Convers. Manag. 2019, 199, 112066. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhou, J.; Zong, Z.; Chen, Q.; Zhang, P.; Wu, K. Development and modelling of a novel electricity-hydrogen energy system based on reversible solid oxide cells and power to gas technology. Int. J. Hydrogen Energy 2019, 44, 28305–28315. [Google Scholar] [CrossRef]
- Xiao, P.; Hu, W.; Xu, X.; Liu, W.; Huang, Q.; Chen, Z. Optimal operation of a wind-electrolytic hydrogen storage system in the electricity/hydrogen markets. Int. J. Hydrogen Energy 2020, 45, 24412–24423. [Google Scholar] [CrossRef]
- Beyrami, J.; Chitsaz, A.; Parham, K. Optimum performance of a single effect desalination unit integrated with a SOFC system by multi-objective thermos-economic optimization based on genetic algorithm. Energy 2019, 186, 115811. [Google Scholar] [CrossRef]
- Wu, X.; Li, H.; Wang, X.; Zhao, W. Cooperative Operation for Wind Turbines and Hydrogen Fueling Stations with On-Site Hydrogen Production. IEEE Trans. Sustain. 2020, 11, 2775–2789. [Google Scholar] [CrossRef]
- Wei, F.; Sui, Q.; Lin, X.; Li, Z.; Chen, L.; Zhao, B.; Xu, C.; Owolabi, S.A. Energy Control Scheduling Optimization Strategy for Coal-Wind-Hydrogen Energy Grid under Consideration of the Efficiency Features of Hydrogen Production Equipment. Proc. CSEE 2018, 38, 1428–1438. [Google Scholar]
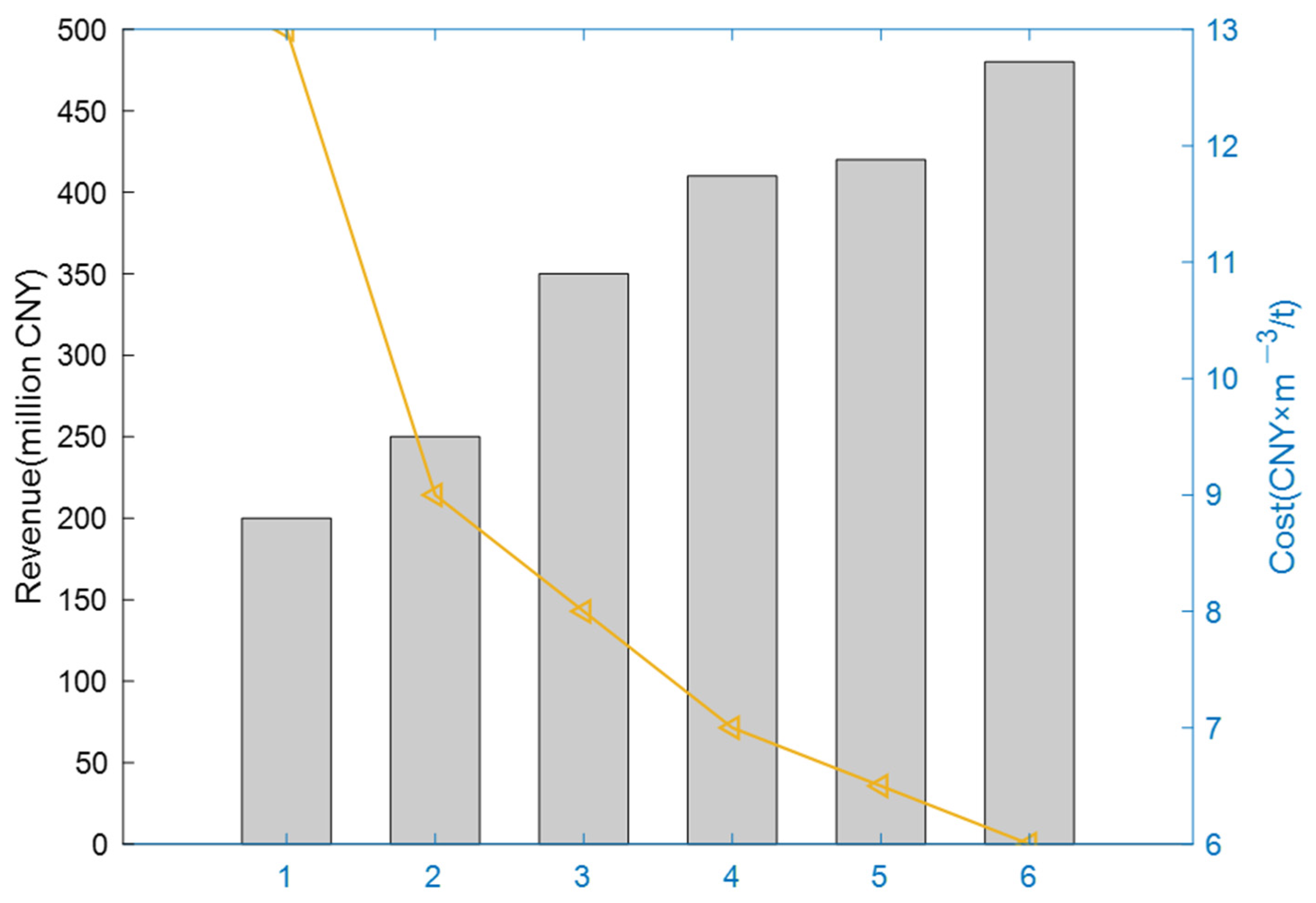

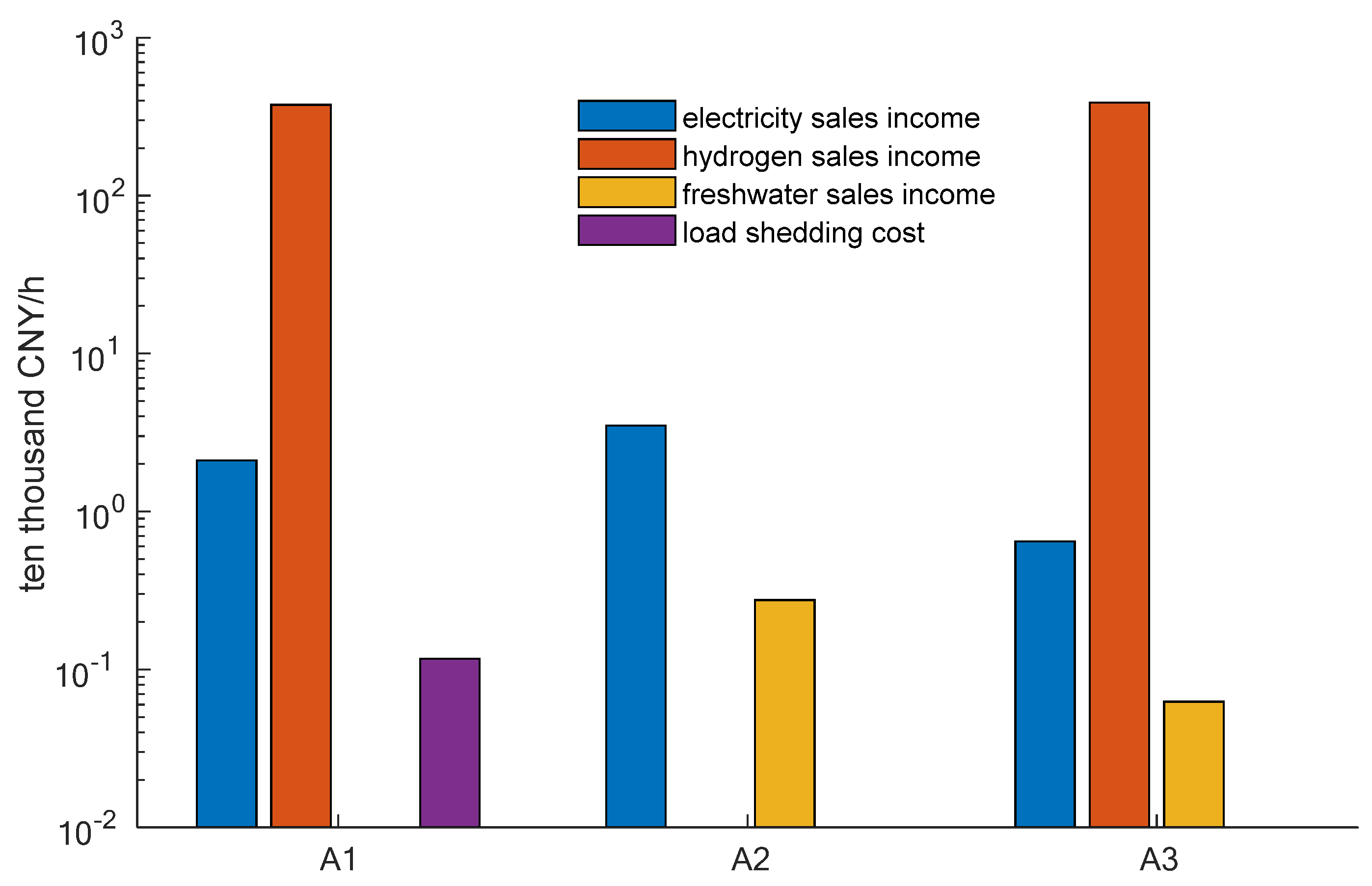



| Class | P (MPa) | T (°C) | Cost (CNY/kW) | Efficiency (%) |
|---|---|---|---|---|
| ALK | 0.1~3 | 60~80 | 410~1030 | 63~70 |
| PEM | 3~8 | 50~80 | 280 | 56~67 |
| SOEC | 0 | 650~1000 | 560 | 74~81 |
| Layer 1 Control Strategy | Layer 2 Control Strategy | ||||
|---|---|---|---|---|---|
| No. | Condition | Strategy | No. | Condition | Strategy |
| Strategy1 | Desalination start Electrolyzer start | Strategy1 | No interaction with power grid | ||
| Strategy2 | Desalination start Fuel cell start | Strategy2 | Sell power | ||
| Strategy3 | Desalination close Fuel cell start | Strategy3 | √ | Power purchase | |
| Strategy4 | Desalination start Electrolyzer start | Strategy4 | × | Load shedding | |
| Class | Desalination | HES | JO-PCS |
|---|---|---|---|
| A1 | √ | ||
| A2 | √ | ||
| A3 | √ | √ | √ |
| A4 | √ | √ |
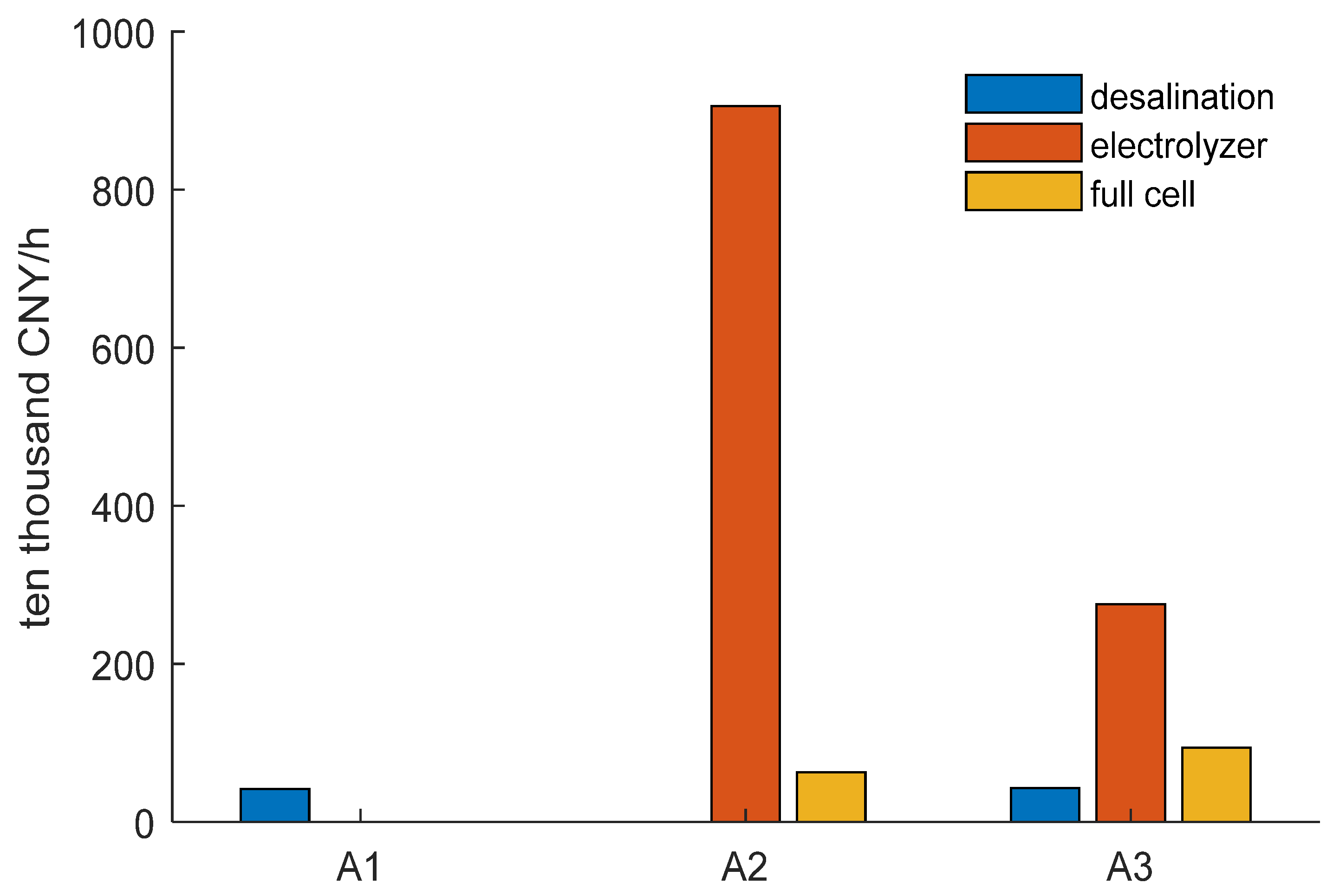
| Class (104 × USD/h) | A1 | A2 | A3 |
|---|---|---|---|
| 59.2 | 0.6 | 60.4 | |
| 6.5 | 150.6 | 64.1 | |
| 52.1 | 150.1 | 3.9 |
| Class | A1 | A2 | A3 | A4 |
|---|---|---|---|---|
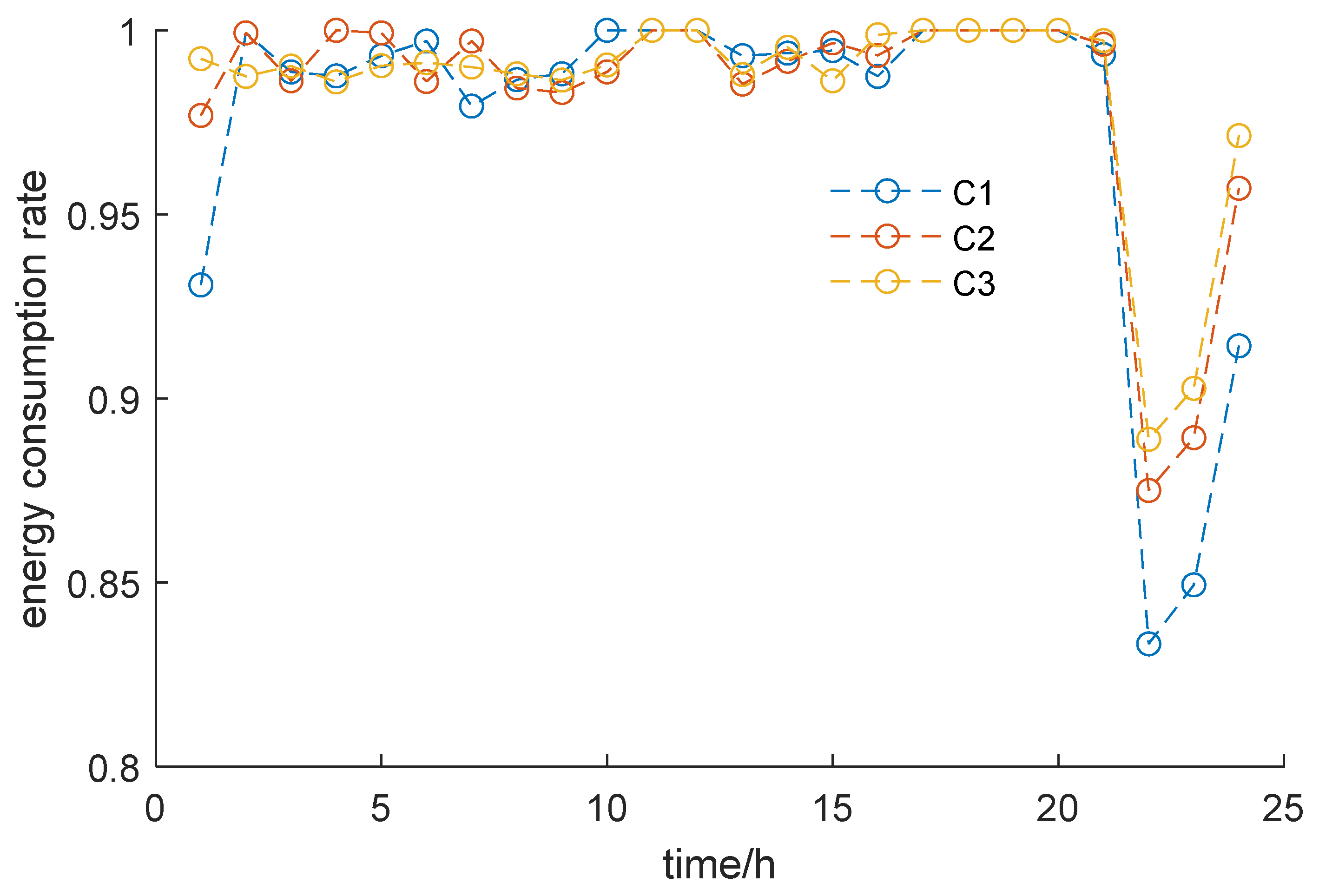
| Accommodation | 94.4% | 89.2% | 98.2% | 95.2% |
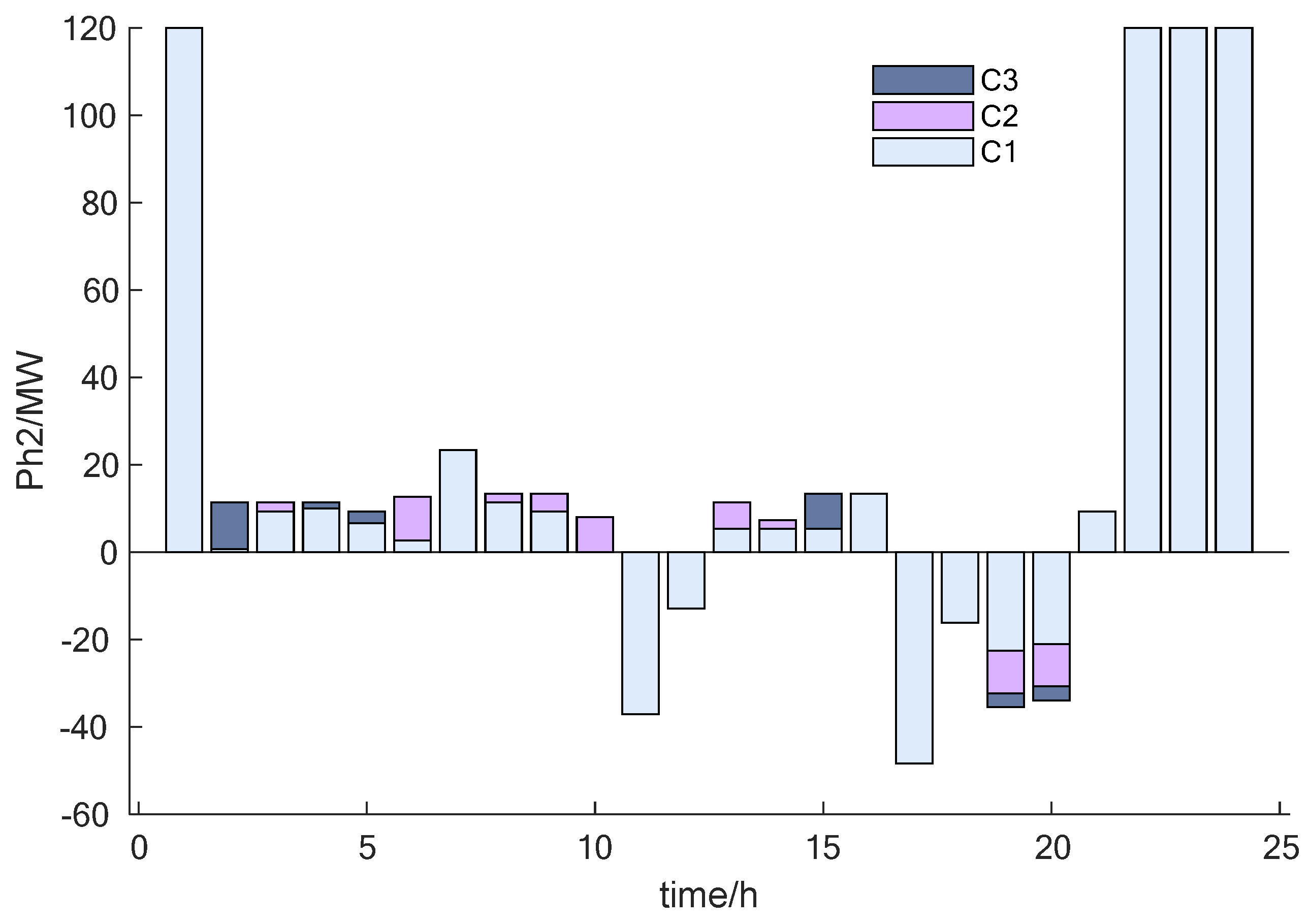
| Class (104 × USD/h) | B2 | B3 | C1 | C2 | C3 |
|---|---|---|---|---|---|
| 0.58 | 60.7 | 63.5 | 57.9 | 55.5 | |
| 138.5 | 62.9 | 66.3 | 67.3 | 69.5 | |
| 137.9 | 2.17 | 2.8 | 9.4 | 14.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Wang, H.; Zhou, B.; Yang, D.; Li, G.; Yang, B.; Xi, C.; Hu, B. Optimal Operation Strategy for Wind–Hydrogen–Water Power Grids Facing Offshore Wind Power Accommodation. Sustainability 2022, 14, 6871. https://doi.org/10.3390/su14116871
Liu Z, Wang H, Zhou B, Yang D, Li G, Yang B, Xi C, Hu B. Optimal Operation Strategy for Wind–Hydrogen–Water Power Grids Facing Offshore Wind Power Accommodation. Sustainability. 2022; 14(11):6871. https://doi.org/10.3390/su14116871
Chicago/Turabian StyleLiu, Zhen, He Wang, Bowen Zhou, Dongsheng Yang, Guangdi Li, Bo Yang, Chao Xi, and Bo Hu. 2022. "Optimal Operation Strategy for Wind–Hydrogen–Water Power Grids Facing Offshore Wind Power Accommodation" Sustainability 14, no. 11: 6871. https://doi.org/10.3390/su14116871
APA StyleLiu, Z., Wang, H., Zhou, B., Yang, D., Li, G., Yang, B., Xi, C., & Hu, B. (2022). Optimal Operation Strategy for Wind–Hydrogen–Water Power Grids Facing Offshore Wind Power Accommodation. Sustainability, 14(11), 6871. https://doi.org/10.3390/su14116871









