An Experimental Method to Determine Rock Joint Stiffness under Constant Normal Load Conditions
Abstract
:1. Introduction
- dσn: the normal stress increment,
- dτ: the shear stress increment,
- dun: the joint normal displacement increment,
- dus: the shear displacement increment.
2. Lab Test Set-Up
2.1. Sample
2.2. Joint Closure and Direct Shear Tests Set-Up
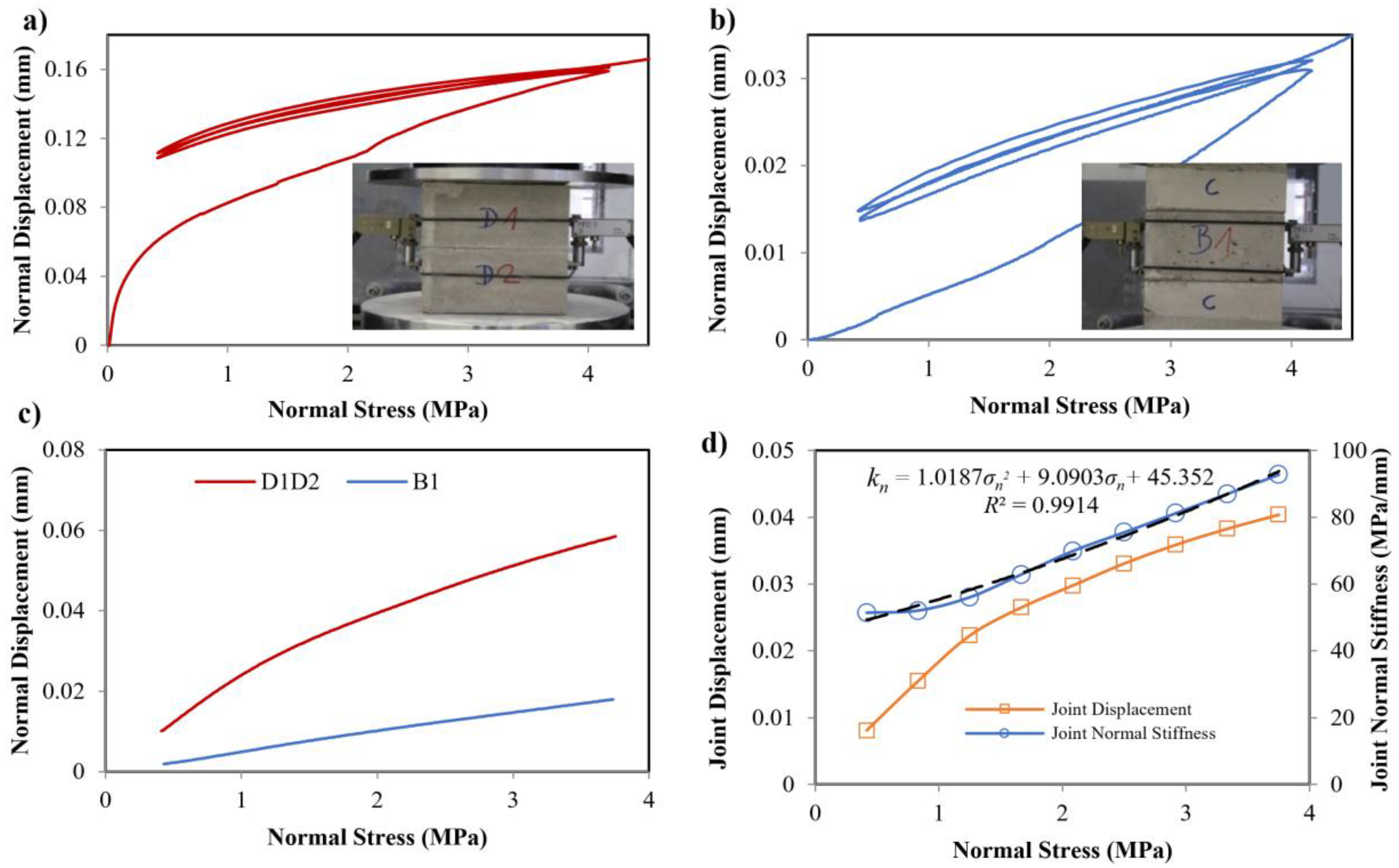
2.2.1. Joint Closure Tests
- lJoint is the actual displacement of the joint,
- lD1D2 is the displacement of the jointed specimen (sensors measured),
- lB1 is the displacement of the intact specimen (sensors measured).kn= σn/lJoint
- σn is the applied normal stress.
2.2.2. Direct Shear Tests
3. Test Results
3.1. Joint Closure Test Results
- kn is the joint normal stiffness,
- σn is the normal stress.
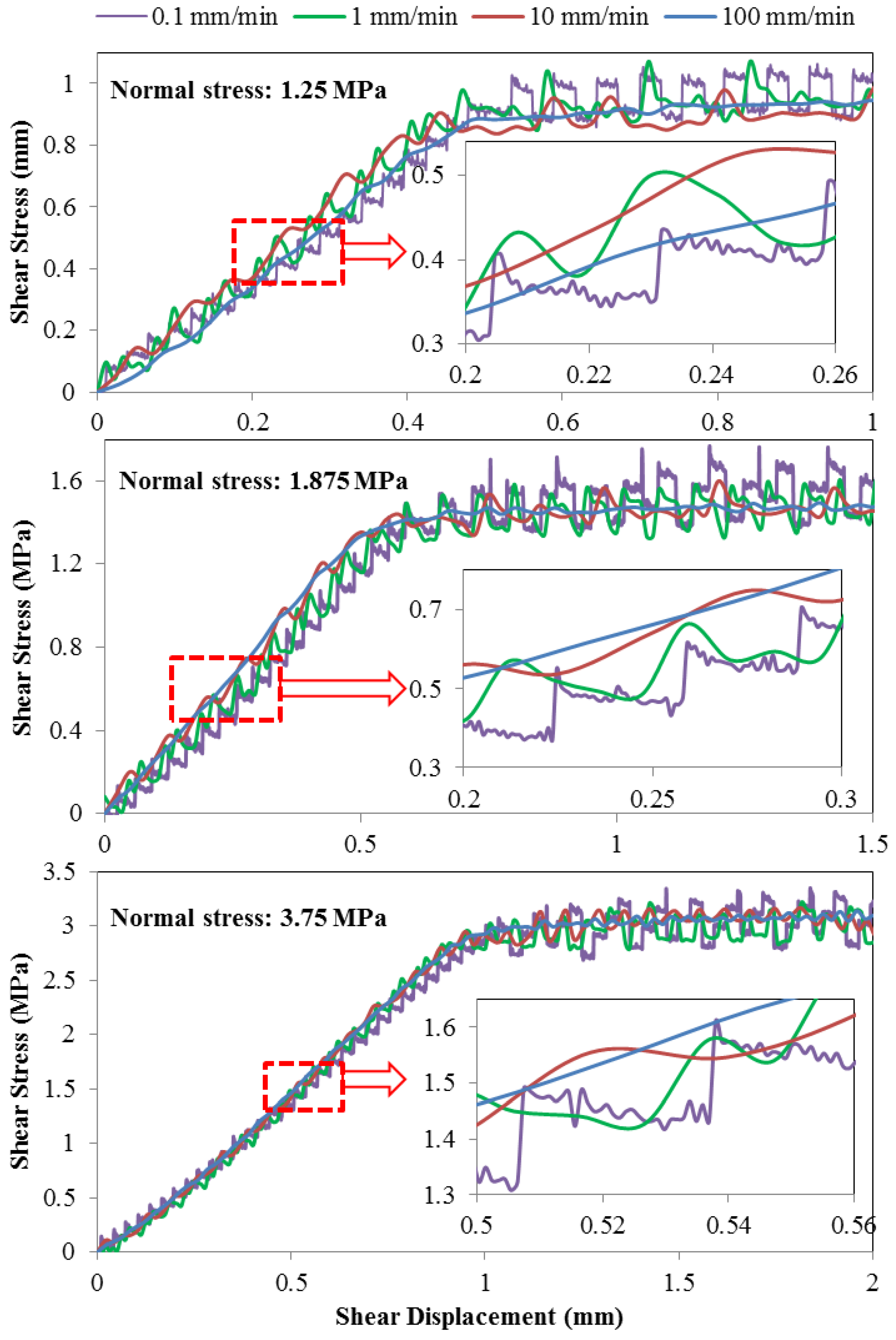
3.2. Direct Shear Test Results
- Stage 1 (A–B): At this stage, the shear distance was not big enough and the interface was mainly compacted. There was an irregular relationship between initial shear stress and shear displacement. At this stage, the specimen and the shear box spontaneously adjusted to each other to form a stable contact.
- Stage 2 (B–C): The second stage comprised linear elastic deformations with increasing shear stress until peak value was reached. Unloading of shear stress may have recovered the shear displacement elastically.
- Stage 3 (After point C): The third stage was characterized by friction sliding. In this stage, the shear stress remained stable with a little fluctuation owing to the surface roughness and damage.
4. Discussion
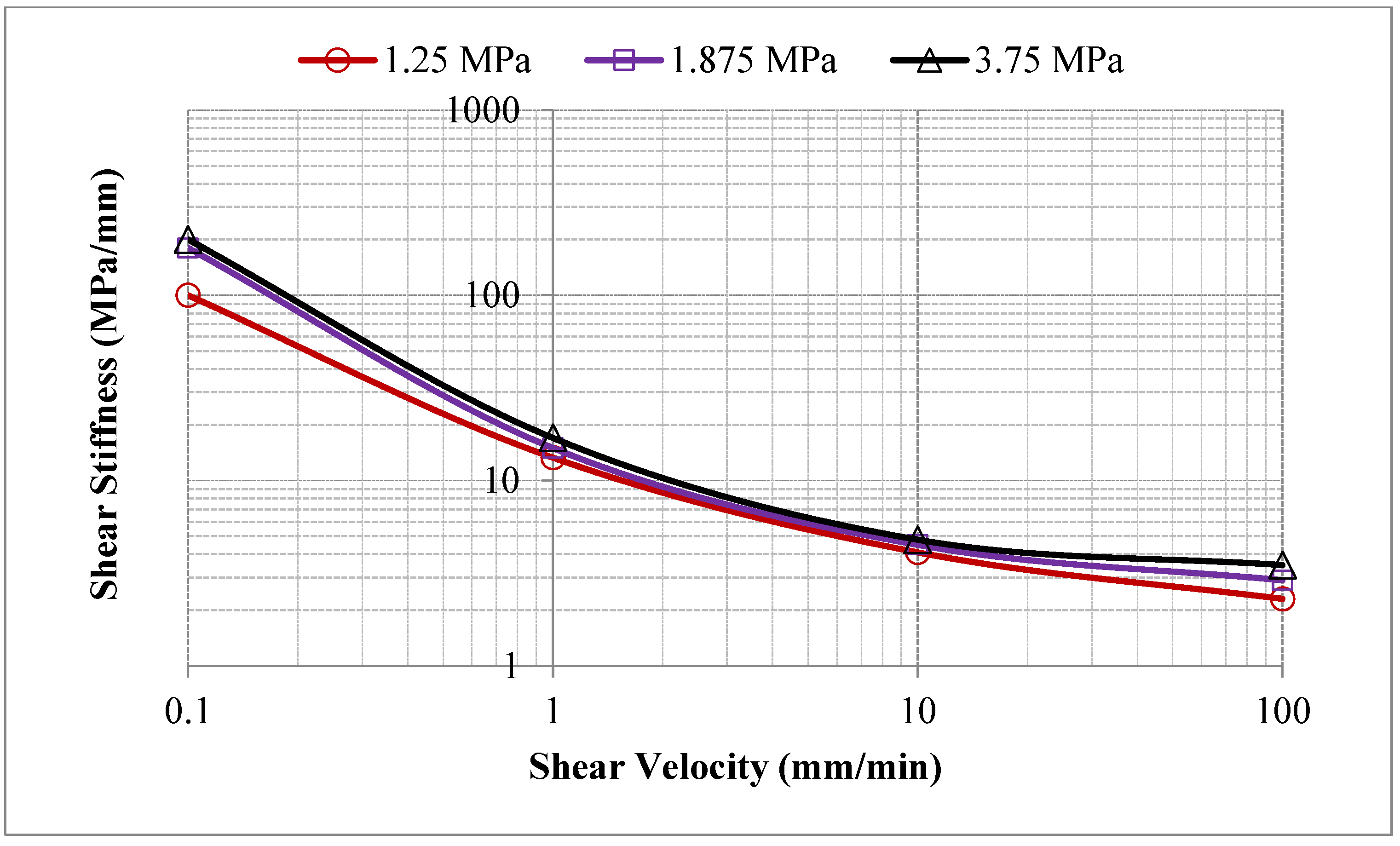
4.1. Calculation of Shear Stiffness
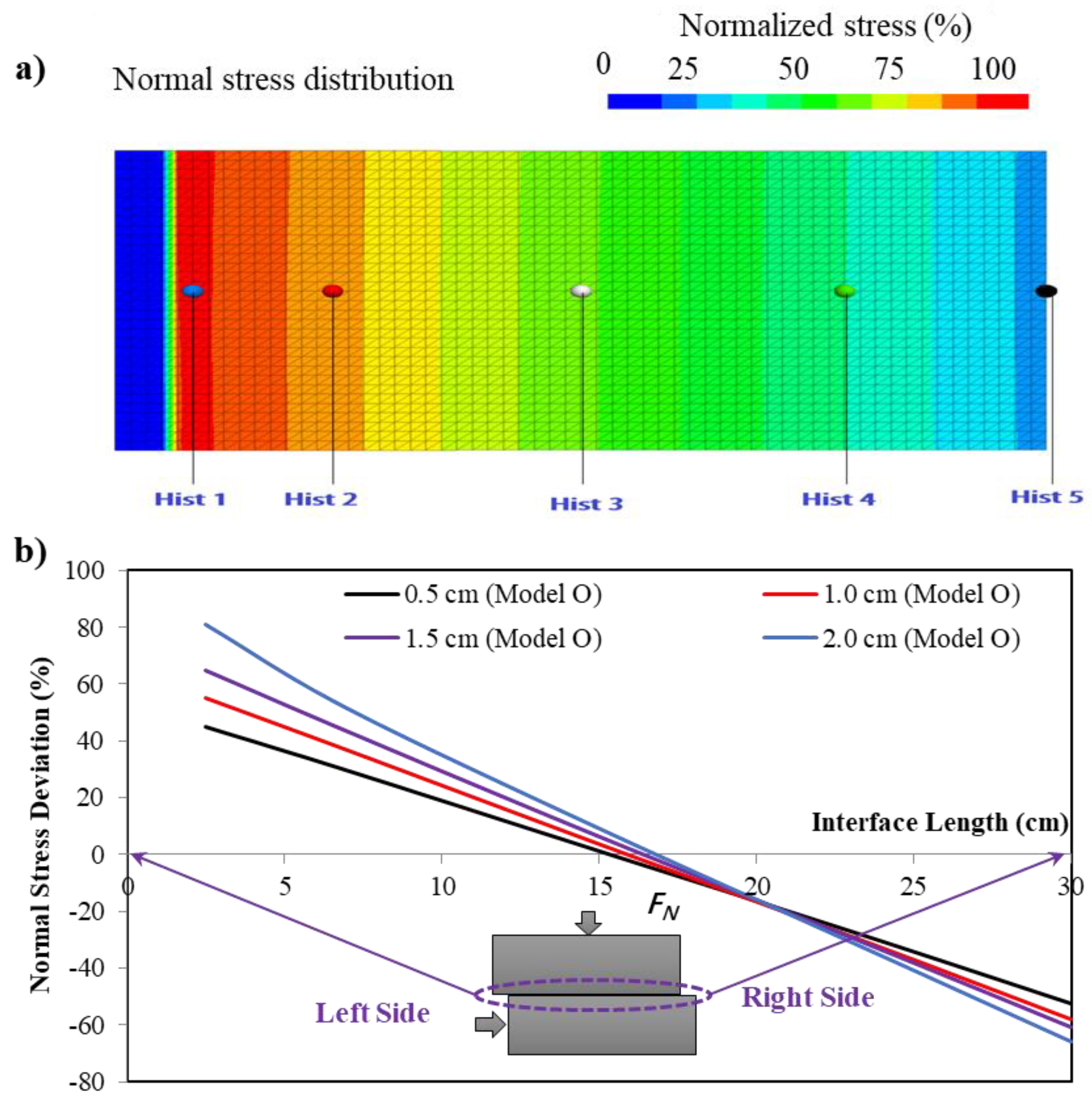
4.2. Stiffness Non-Uniform Distribution
- kn is the joint normal stiffness,
- ks is the joint shear stress,
- K is the Young modulus,
- G is the bulk modulus,
- Δzmin is the minimum size of the mesh of contact surfaces.
5. Conclusions
- Joint normal and shear stiffnesses are time- and spatially varying parameters during direct shearing.
- With increasing normal stress, average shear stiffness, instantaneous shear stiffness, and normal stiffness increase. Normal stiffness is about 26–28 times higher than the average shear stiffness.
- Instantaneous shear stiffness of joints decreases rapidly when the shear velocity is increased.
- At lower shear velocities, stick–slip behavior is remarkable, instantaneous shear stiffness can be higher than the normal stiffness, minimum instantaneous shear stiffness can be negative, and the absolute value decreases with increase in shear velocity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hoek, E.; Brown, E.T. Underground Excavations in Rock; The Institution of Mining and Metallurgy: London, UK, 1980. [Google Scholar]
- Hoek, E.; Bray, J. Rock Slope Engineering; Taylor & Francis: London, UK, 1981. [Google Scholar]
- Liu, Z.; Dang, W.; He, X. Undersea safety mining of the large gold deposit in Xinli District of Sanshandao Gold Mine. Int. J. Miner. Metall. Mater. 2012, 19, 574–583. [Google Scholar] [CrossRef]
- Liu, Z.-X.; Dang, W.-G.; He, X.-Q.; Li, D.-Y. Cancelling ore pillars in large-scale coastal gold deposit: A case study in Sanshandao gold mine, China. Trans. Nonferrous Met. Soc. China 2013, 23, 3046–3056. [Google Scholar] [CrossRef]
- Liu, Z.; Dang, W. Rock quality classification and stability evaluation of undersea deposit based on M-IRMR. Tunn. Undergr. Space Technol. 2014, 40, 95–101. [Google Scholar] [CrossRef]
- Dang, W.; Konietzky, H.; Frühwirt, T. Shear behaviour of a plane joint under dynamic normal load (DNL) conditions. Eng. Geol. 2016, 213, 133–141. [Google Scholar] [CrossRef]
- Nguyen, V.H.; Konietzky, H.; Frühwirt, T. New methodology to characterize shear behavior of joints by combination of direct shear box testing and numerical simulations. Geo. Geol. Eng. 2014, 32, 829–846. [Google Scholar] [CrossRef]
- Dang, W.; Konietzky, H.; Frühwirt, T. Rotation and stress changes on a plane joint during direct shear tests. Int. J. Rock Mech. Min. Sci. 2016, 89, 129–135. [Google Scholar] [CrossRef]
- Krolikowski, J.; Szczepek, J.; Witczak, Z. Ultrasonic investigation of contact between solids under high hydrostatic-pressure. Ultrasonics 1989, 27, 45–49. [Google Scholar] [CrossRef]
- Sherif, H.A.; Kossa, S.S. Relationship between normal and tangential contact stiffness of nominally flat surfaces. Wear 1991, 151, 49–62. [Google Scholar] [CrossRef]
- Krolikowski, J.; Szczepek, J. Assessment of tangential and normal stiffness of contact between rough surfaces using ultrasonic method. Wear 1993, 160, 253–258. [Google Scholar] [CrossRef]
- Baltazar, A.; Rokhlin, S.I.; Pecorari, C. On the relationship between ultrasonic and micromechanical properties of contacting rough surfaces. J. Mech. Phys. Solids 2002, 50, 1397–1416. [Google Scholar] [CrossRef]
- Dwyer, J.R.S.; Gonzalez, V.M. Ultrasonic determination of normal and shear interface stiffness and the effect of Poisson’s ratio. Tribol. Ser. 2003, 43, 143–149. [Google Scholar]
- Wang, J.G.; Ichikawa, Y.; Leung, C.F. A constitutive model for rock interfaces and joints. Int. J. Rock Mech. Min. Sci. 2003, 40, 41–53. [Google Scholar] [CrossRef]
- Gonzalez, V.M.; Baltazar, A.; Dwyer, J.R.S. Study of interfacial stiffness ratio of a rough surface in contact using a spring model. Wear 2010, 268, 373–379. [Google Scholar] [CrossRef]
- Kumar, S.; Scanlan, J.P. On axisymmetric adhesive joints with graded interface stiffness. Int. J. Adhes. Adhes. 2013, 41, 57–72. [Google Scholar] [CrossRef]
- Atapour, H.; Moosavi, M. The influence of shearing velocity on shear behavior of artificial joints. Rock Mech. Rock Eng. 2014, 47, 1745–1761. [Google Scholar] [CrossRef]
- Kumar, R.; Verma, A.K. Anisotropic shear behavior of rock joint replicas. Int. J. Rock Mech. Min. Sci. 2016, 90, 62–73. [Google Scholar] [CrossRef]
- Zhang, C.; Curiel-Sosa, J.L.; Bui, T.Q. A novel interface constitutive model for prediction of stiffness and strength in 3D braided composites. Compos. Struct. 2017, 163, 32–43. [Google Scholar] [CrossRef]
- Desai, C.S.; Zaman, M.M.; Lightner, J.G.; Siriwardane, H.J. Thin-layer element for interfaces and joints. Int. J. Numer. Anal. Meth. Geomech. 1984, 8, 19–43. [Google Scholar] [CrossRef]
- Goodman, R.E. Methods of Geological Engineering in Discontinuous Rocks; West Publishing Company: San Francisco, CA, USA, 1976. [Google Scholar]
- Sharma, K.G.; Desai, C.S. Analysis and implementation of thin-layer element for interfaces and joints. J. Eng. Mech. 1992, 118, 2442–2462. [Google Scholar] [CrossRef]
- Paggi, M.; Wriggers, P. Stiffness and strength of hierarchical polycrystalline materials with imperfect interfaces. J. Mech. Phys. Solids 2012, 60, 557–572. [Google Scholar] [CrossRef]
- Xiao, H.; Shao, Y.; Brennan, M.J. On the contact stiffness and nonlinear vibration of an elastic body with a rough surface in contact with a rigid flat surface. Eur. J. Mech. A Solids 2015, 49, 321–328. [Google Scholar] [CrossRef]
- Raffa, M.L.; Lebon, F.; Vairo, G. Normal and tangential stiffnesses of rough surfaces in contact via an imperfect interface model. Int. J. Solids Struct. 2016, 87, 245–253. [Google Scholar] [CrossRef]
- Itasca. FLAC3D—Fast Lagrangian Analysis of Continua in 3 Dimensions—Theory and Background; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2012. [Google Scholar]
- Konietzky, H.; Frühwirt, T.; Luge, H. A new large dynamic rock mechanical direct shear box device. Rock Mech. Rock Eng. 2012, 45, 427–432. [Google Scholar] [CrossRef]
- Muralha, J.; Grasselli, G.; Tatone, B.; Blümel, M.; Chryssanthakis, P.; Yujing, J. ISRM Suggested Method for Laboratory Determination of the Shear Strength of Rock Joints: Revised Version. Rock Mech. Rock Eng. 2014, 47, 291–302. [Google Scholar] [CrossRef]
- Dang, W.; Konietzky, H.; Herbst, M.; Frühwirt, T. Complex analysis of shear box tests with explicit consideration of interaction between test device and sample. Measurement 2017, 102, 1–9. [Google Scholar] [CrossRef]
- Li, W.S.; Jiang, B.Y.; Gu, S.T.; Yang, X.X.; Shaikh, F.U.A. Experimental study on the shear behavior of grout-infilled specimens and micromechanical properties of grout-rock interface. J. Cent. South Univ. 2022, 29, 1686–1700. [Google Scholar] [CrossRef]
- Du, K.; Yang, C.Z.; Su, R.; Tao, M.; Wang, S.F. Failure properties of cubic granite, marble, and sandstone specimens under true triaxial stress. Int. J. Rock Mech. Min. Sci. 2020, 130, 104309. [Google Scholar] [CrossRef]
- Du, K.; Li, X.F.; Tao, M.; Wang, S.F. Experimental study on acoustic emission (AE) characteristics and crack classification during rock fracture in several basic lab tests. Int. J. Rock Mech. Min. Sci. 2020, 133, 104411. [Google Scholar] [CrossRef]
- Du, K.; Li, X.F.; Yang, C.Z.; Zhou, J.; Chen, S.J.; Manoj, K. Experimental investigations on mechanical performance of rocks under fatigue loads and biaxial confinements. J. Cent. South Univ. 2020, 27, 2985–2998. [Google Scholar] [CrossRef]
- Dou, L.; Yang, K.; Chi, X. Fracture behavior and acoustic emission characteristics of sandstone samples with inclined precracks. Int. J. Coal Sci. Technol. 2021, 8, 77–87. [Google Scholar] [CrossRef]
- Petr, V.N.; Svetlana, A.E.; Vladimir, L.S.; Polina, S.A. Experimental study of coal fracture dynamics under the influence of cyclic freezing–thawing using shear elastic waves. Int. J. Coal Sci. Technol. 2021, 8, 562–574. [Google Scholar]




| Items | Values |
|---|---|
| Tensile strength (MPa) | 2.55 |
| Compression strength (MPa) | 20.1 |
| Cohesion (MPa) | 7.4 |
| Internal friction angle | 39° |
| Poisson’s ratio | 0.21 |
| Density (g/cm3) | 2.52 |
| Young’s modulus (GPa) | 29.5 |
| Sample | Stage | Input Parameters | ||
|---|---|---|---|---|
| σn | v | us_max | ||
| [MPa] | [mm/min] | [mm] | ||
| CNL_1 | 1 | 1.25 | 100 | 25 |
| 2 | 1.25 | −10 | 25 | |
| 3 | 1.25 | 10 | 20 | |
| 4 | 1.25 | −1 | 20 | |
| 5 | 1.25 | 1 | 20 | |
| 6 | 1.25 | −0.1 | 20 | |
| CNL_2 | 1 | 1.875 | 100 | 25 |
| 2 | 1.875 | −10 | 25 | |
| 3 | 1.875 | 10 | 20 | |
| 4 | 1.875 | −1 | 20 | |
| 5 | 1.875 | 1 | 20 | |
| 6 | 1.875 | −0.1 | 20 | |
| CNL_3 | 1 | 3.75 | 100 | 25 |
| 2 | 3.75 | −10 | 25 | |
| 3 | 3.75 | 10 | 20 | |
| 4 | 3.75 | −1 | 20 | |
| 5 | 3.75 | 1 | 20 | |
| 6 | 3.75 | −0.1 | 20 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; An, G.; Chen, X.; Dang, W.; Li, D. An Experimental Method to Determine Rock Joint Stiffness under Constant Normal Load Conditions. Sustainability 2022, 14, 11734. https://doi.org/10.3390/su141811734
Li D, An G, Chen X, Dang W, Li D. An Experimental Method to Determine Rock Joint Stiffness under Constant Normal Load Conditions. Sustainability. 2022; 14(18):11734. https://doi.org/10.3390/su141811734
Chicago/Turabian StyleLi, Dong, Guanfeng An, Xiangsheng Chen, Wengang Dang, and Dongyang Li. 2022. "An Experimental Method to Determine Rock Joint Stiffness under Constant Normal Load Conditions" Sustainability 14, no. 18: 11734. https://doi.org/10.3390/su141811734
APA StyleLi, D., An, G., Chen, X., Dang, W., & Li, D. (2022). An Experimental Method to Determine Rock Joint Stiffness under Constant Normal Load Conditions. Sustainability, 14(18), 11734. https://doi.org/10.3390/su141811734







