Effects of Influence Parameters on Freezing Wall Temperature Field in Subway Tunnel
Abstract
:1. Introduction
2. Freezing Wall Temperature Field
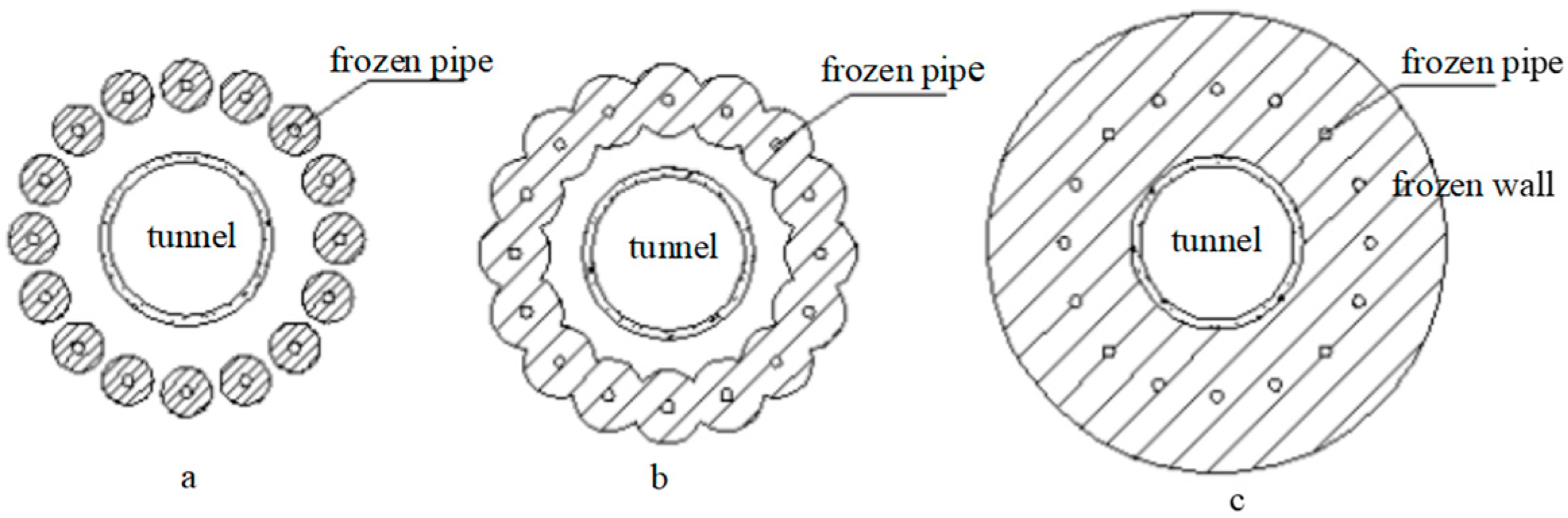
2.1. Formation Process of Frozen Wall
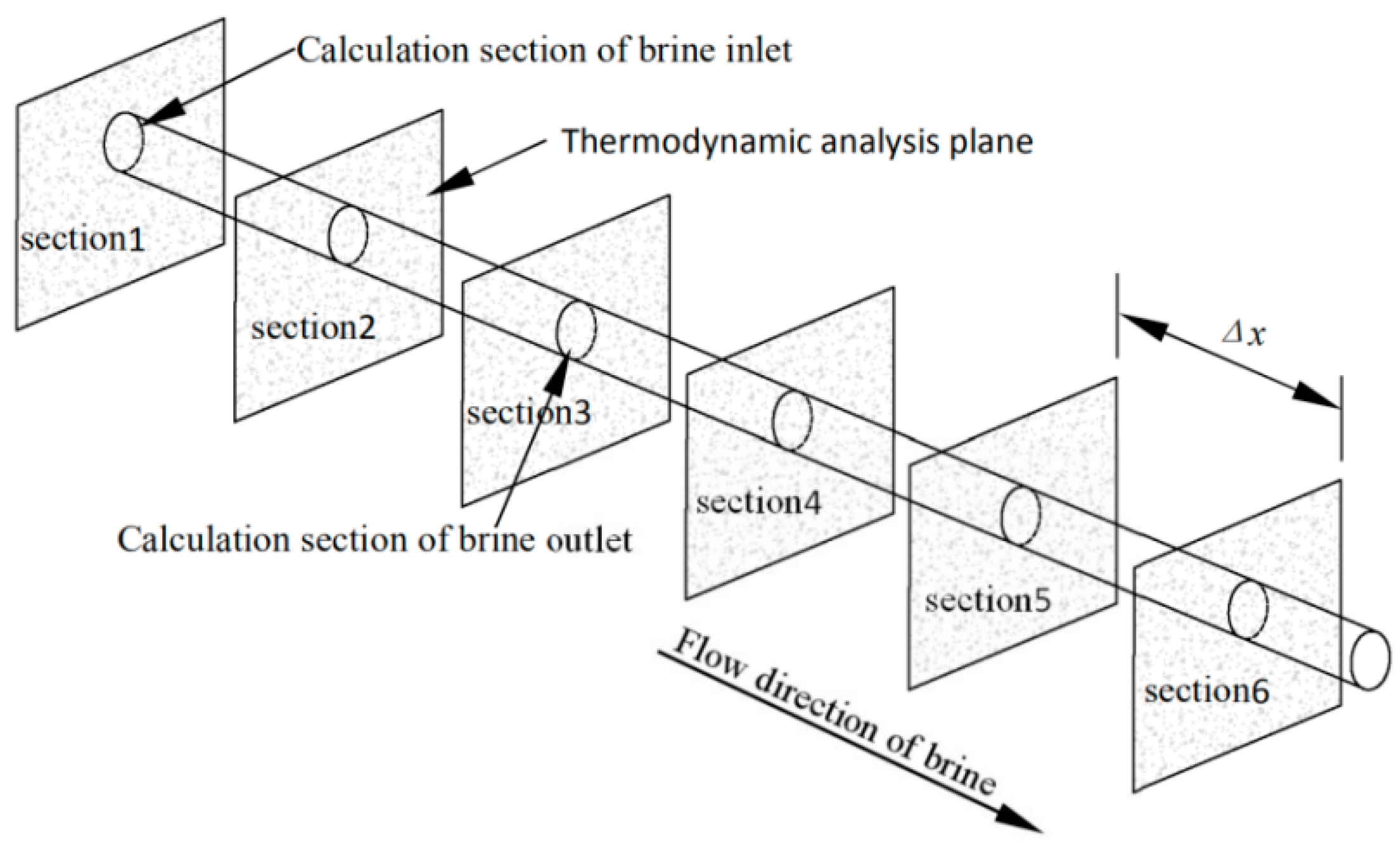
2.2. Freezing Wall Temperature Field
2.3. Horizontal Analysis of Temperature Field
3. Computational Model
3.1. Basic Assumptions
- (1)
- The stratum is an isotropic elastoplastic body with independent thermal parameters in the freeze-thaw state;
- (2)
- The loss of cooling capacity is perpendicular to the direction of the freezing tube, regardless of the loss along the direction of the freezing tube;
- (3)
- The temperature around the freezing tube is evenly distributed. Considering the temperature loss of low-temperature circulating brine, the temperature of the cold source applied at the location of the freezing tube is 2 °C higher than the actual low-temperature brine temperature;
- (4)
- Considering the heat conduction and ice-water phase transition effect of the cold in the stratum, the convection and heat radiation caused by temperature is ignored, the unfrozen water content is taken as a function of temperature, the latent heat calculated from the unfrozen water content is only in the phase transition temperature range internally generated, and the temperature range of the stratum phase transition is taken from [−1~0] °C;
- (5)
- Regardless of the effect of construction on the stratum freezing, it is assumed that the outer boundary away from the frozen wall area of the geometric model is an adiabatic boundary;
- (6)
- Assuming that the density ρ, the specific heat capacity c, and the thermal conductivity λ are all constant, only the freezing and thawing state of the formation is considered.
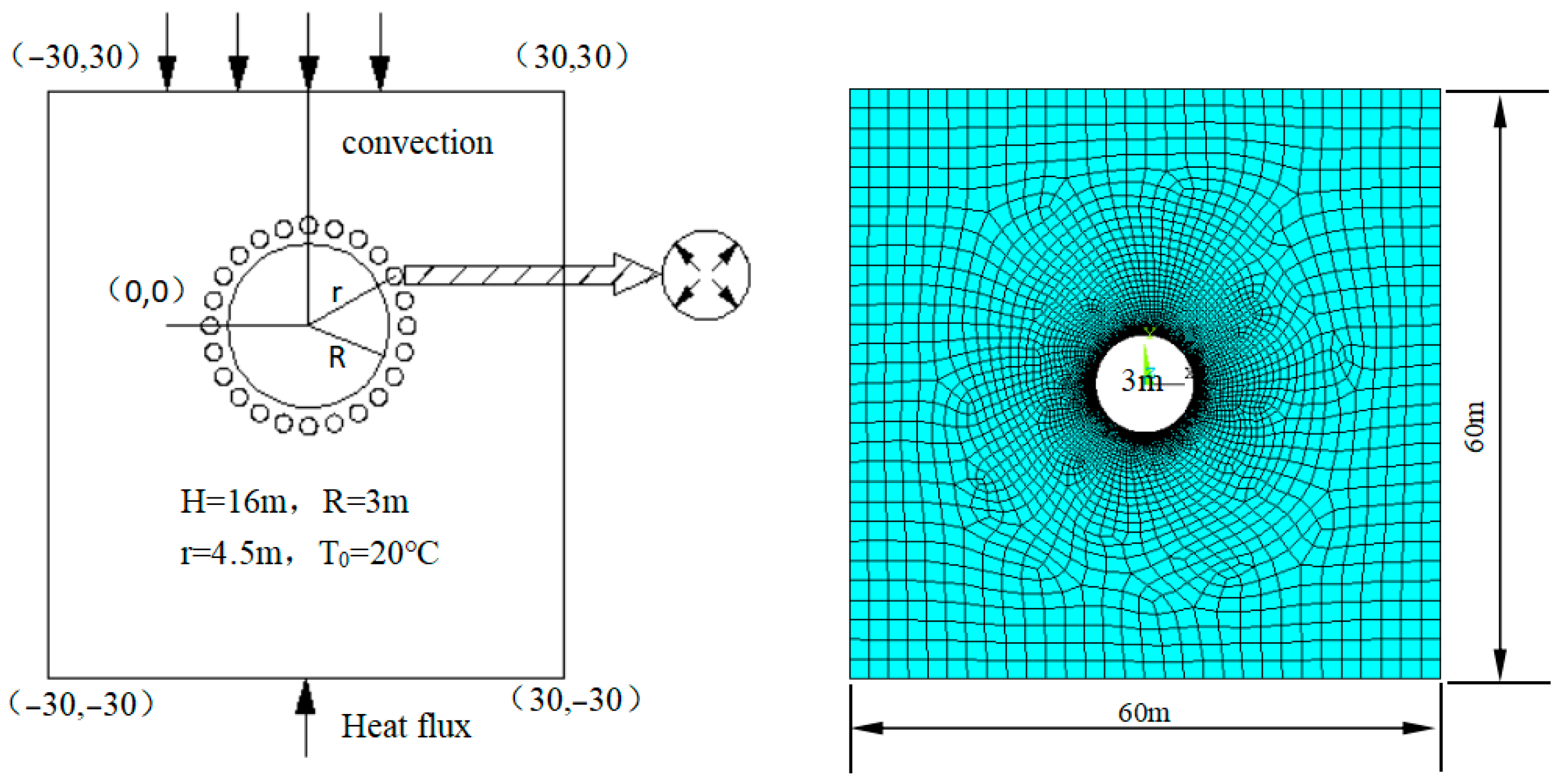
3.2. Calculation Model and Parameters
4. Discussion
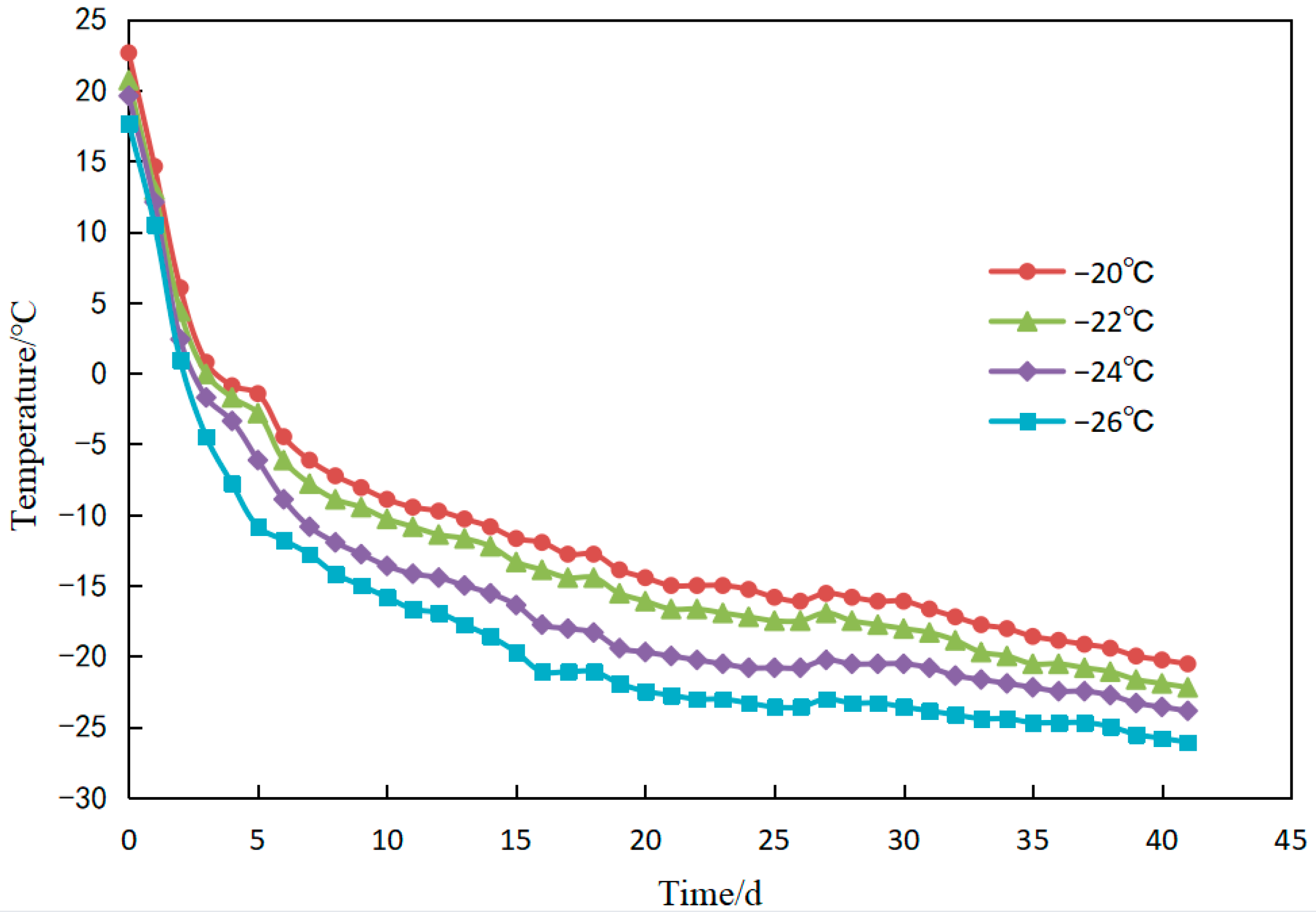
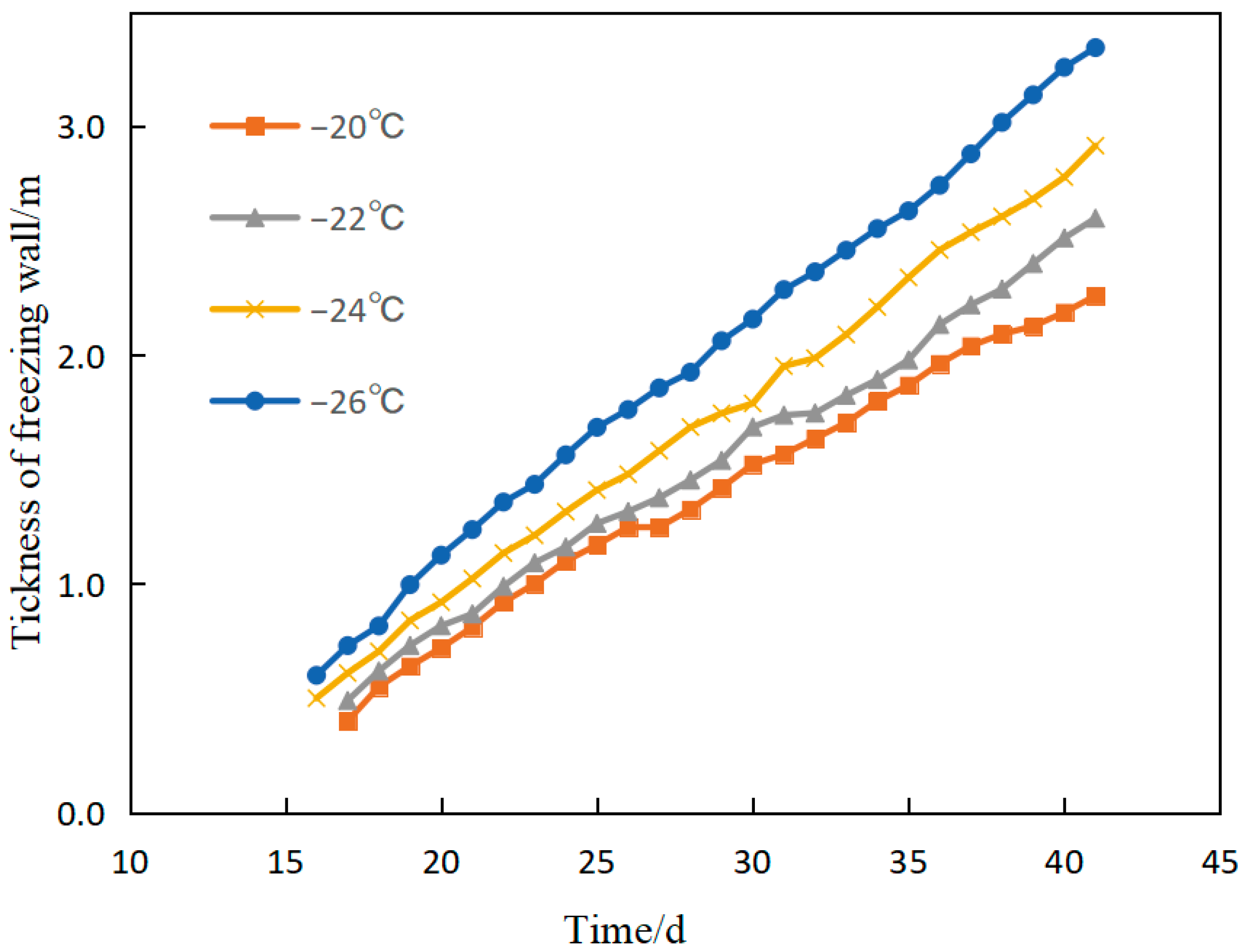
4.1. Influence of Brine Temperature
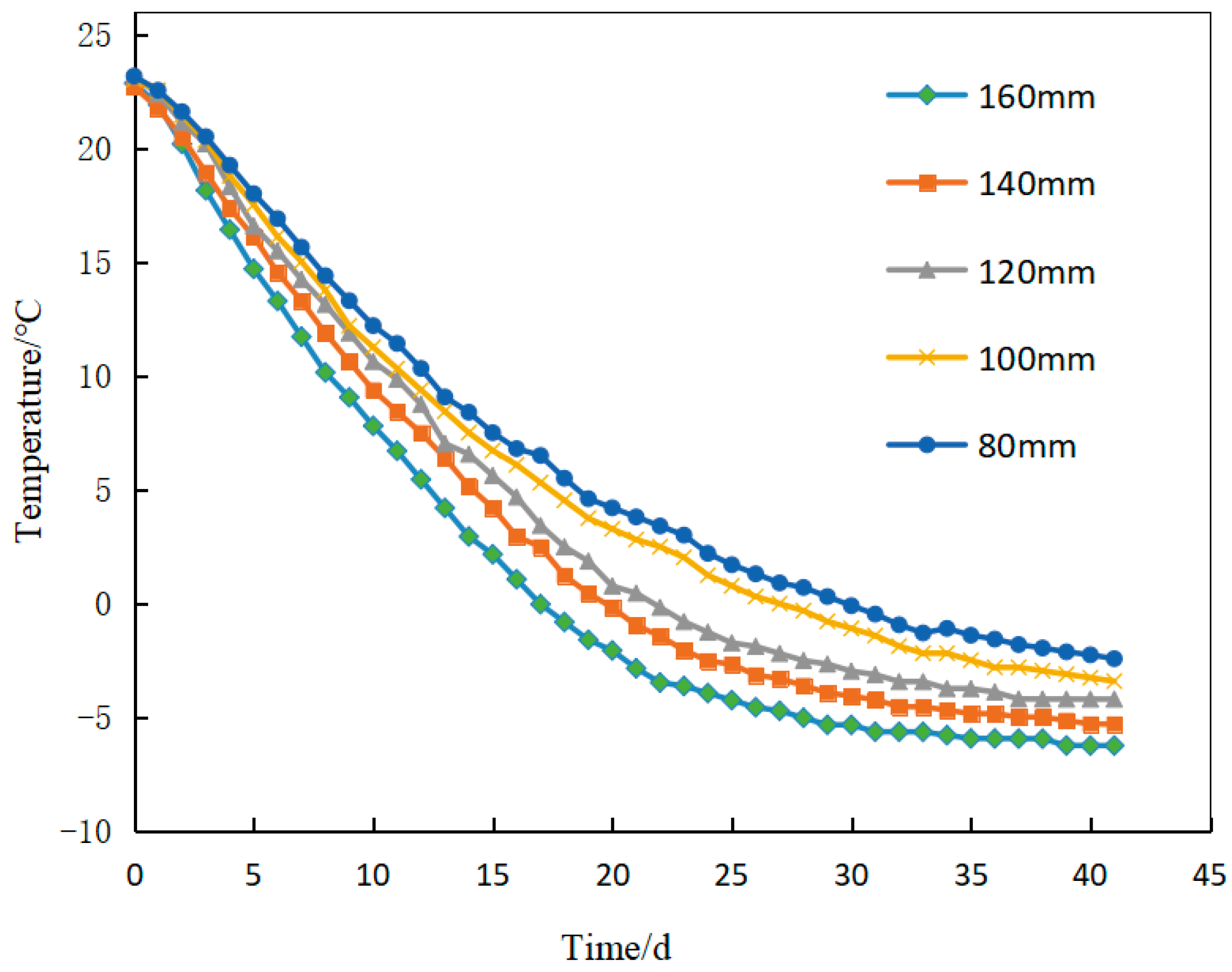
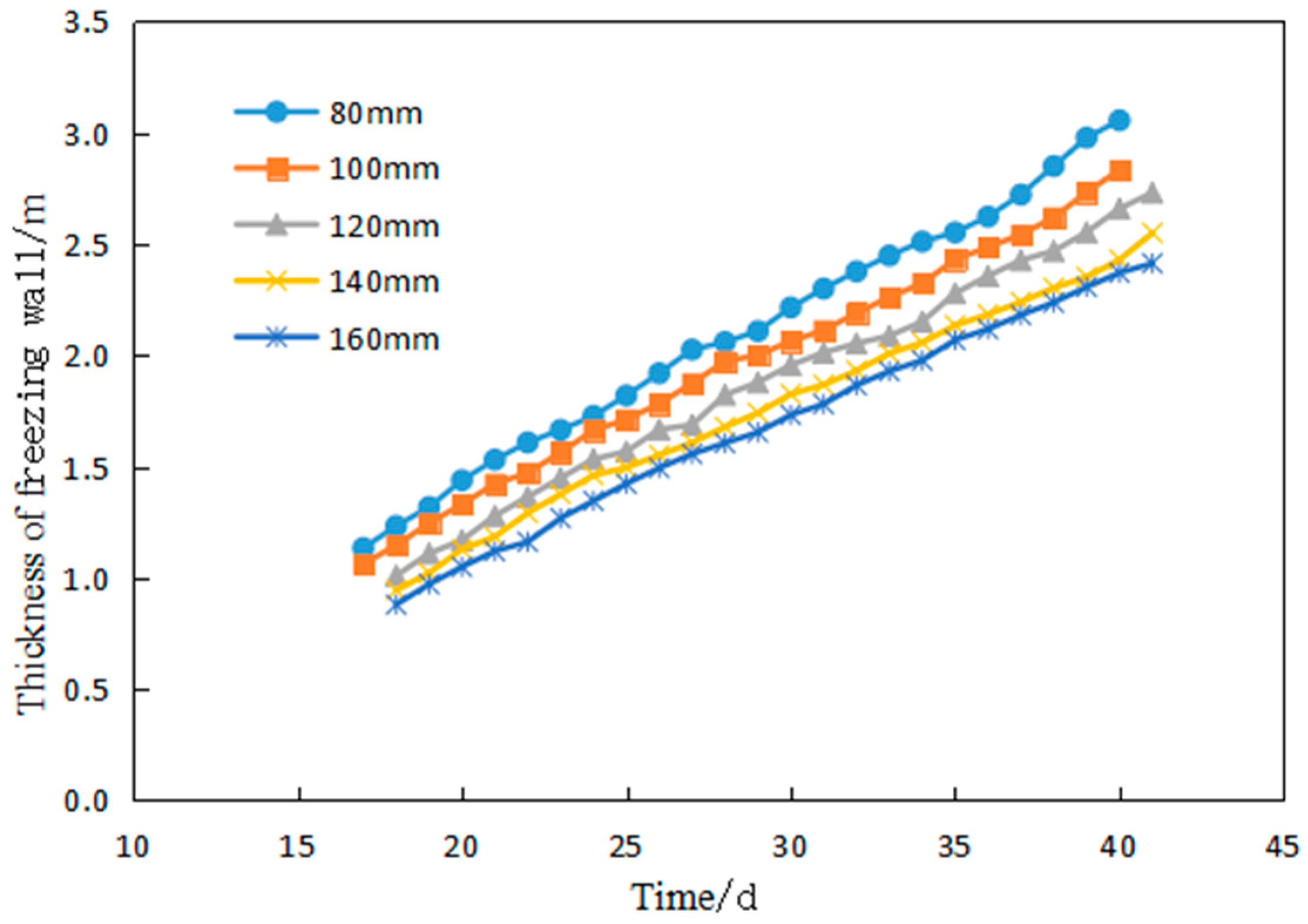
4.2. Influence of Freezing Pipe Diameter
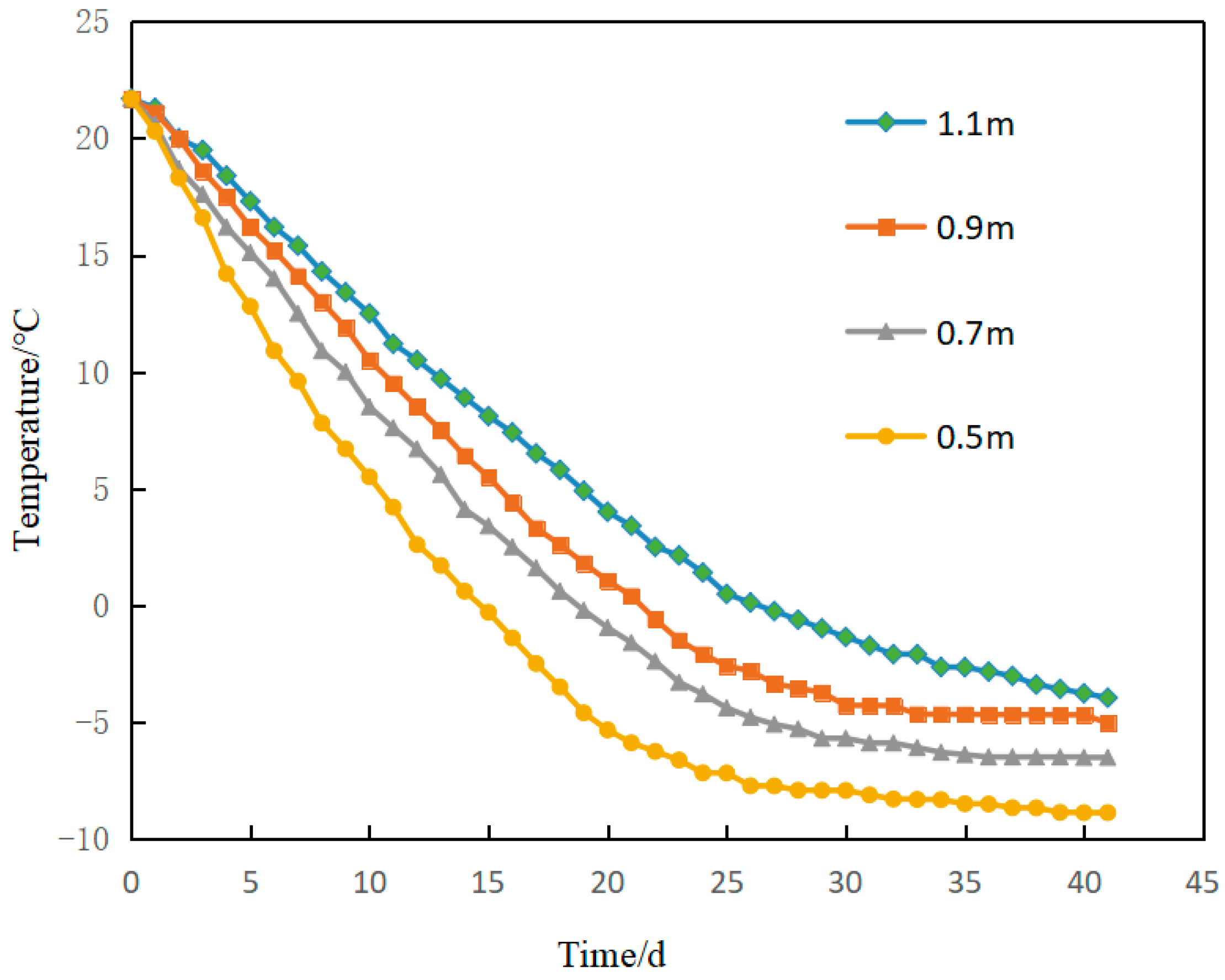
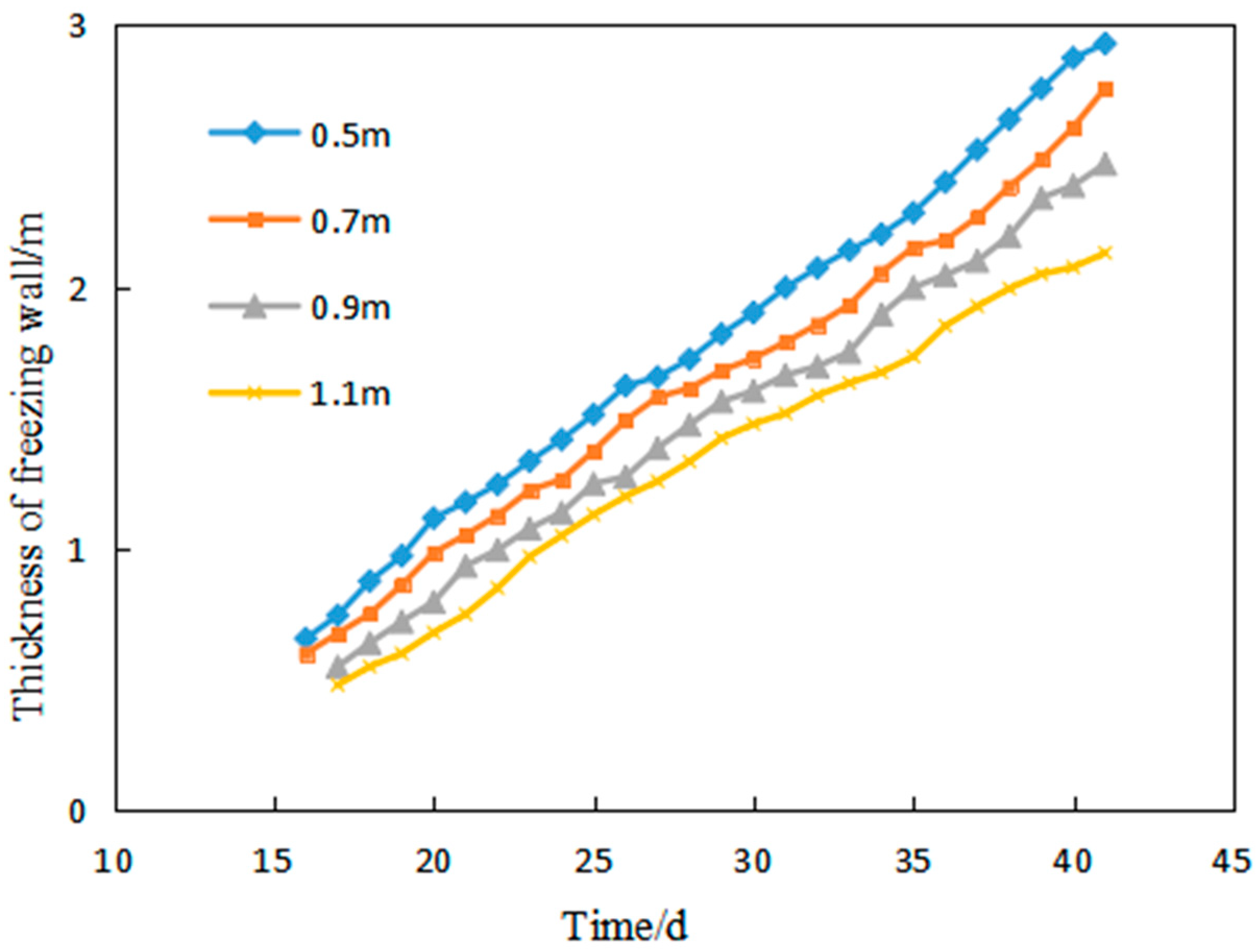
4.3. Influence of Freezing Pipe Spacing
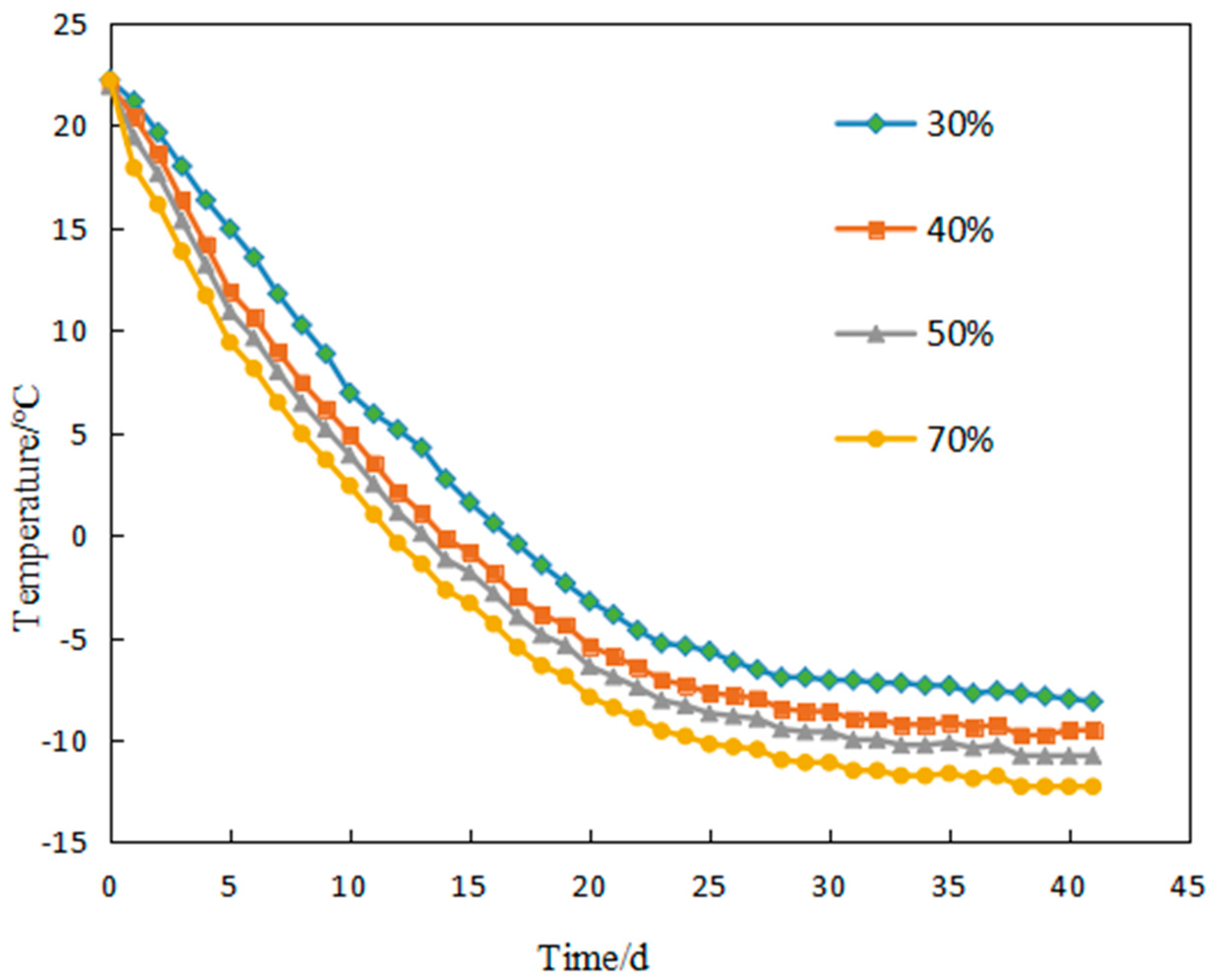
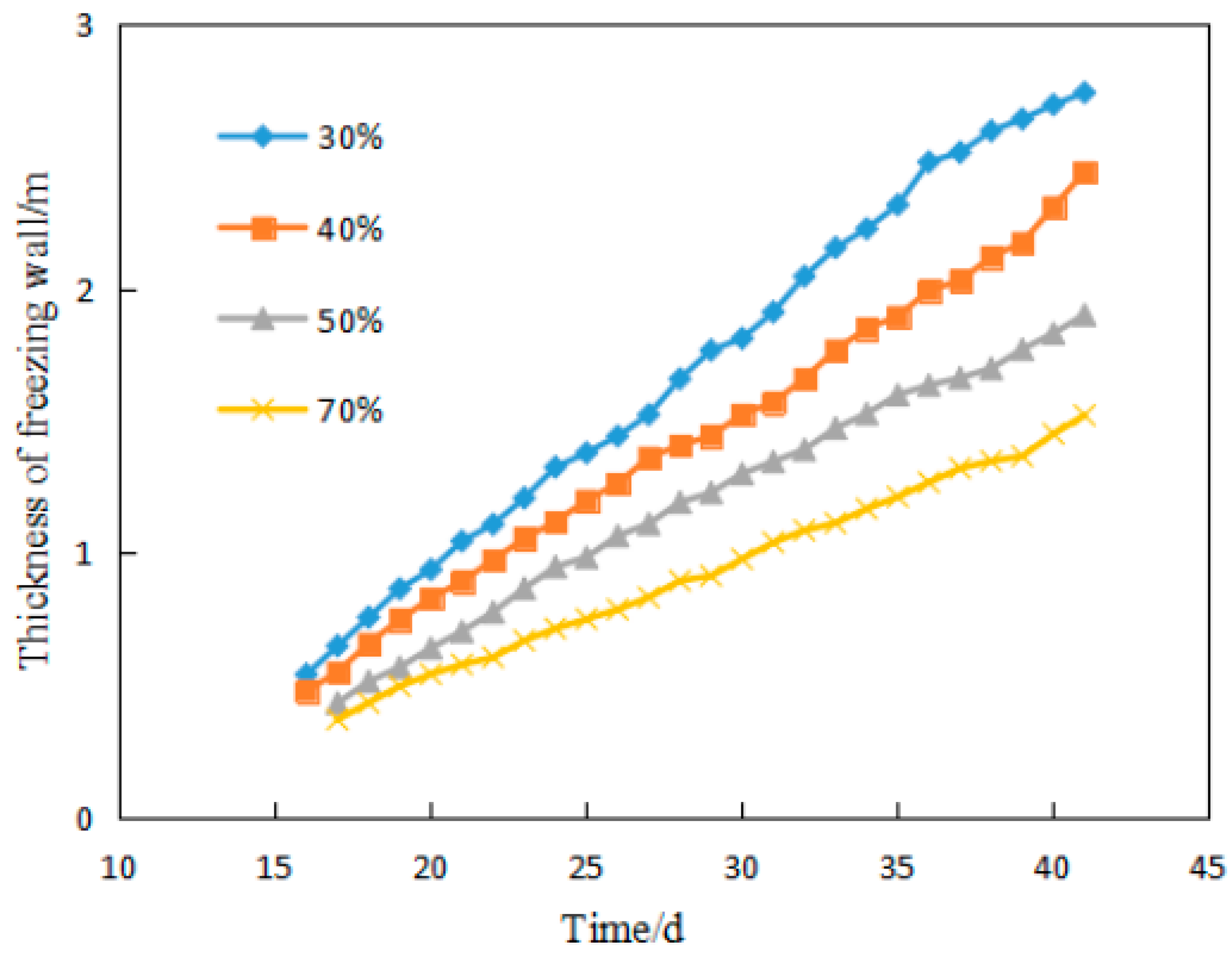
4.4. Influence of Soil Water Content
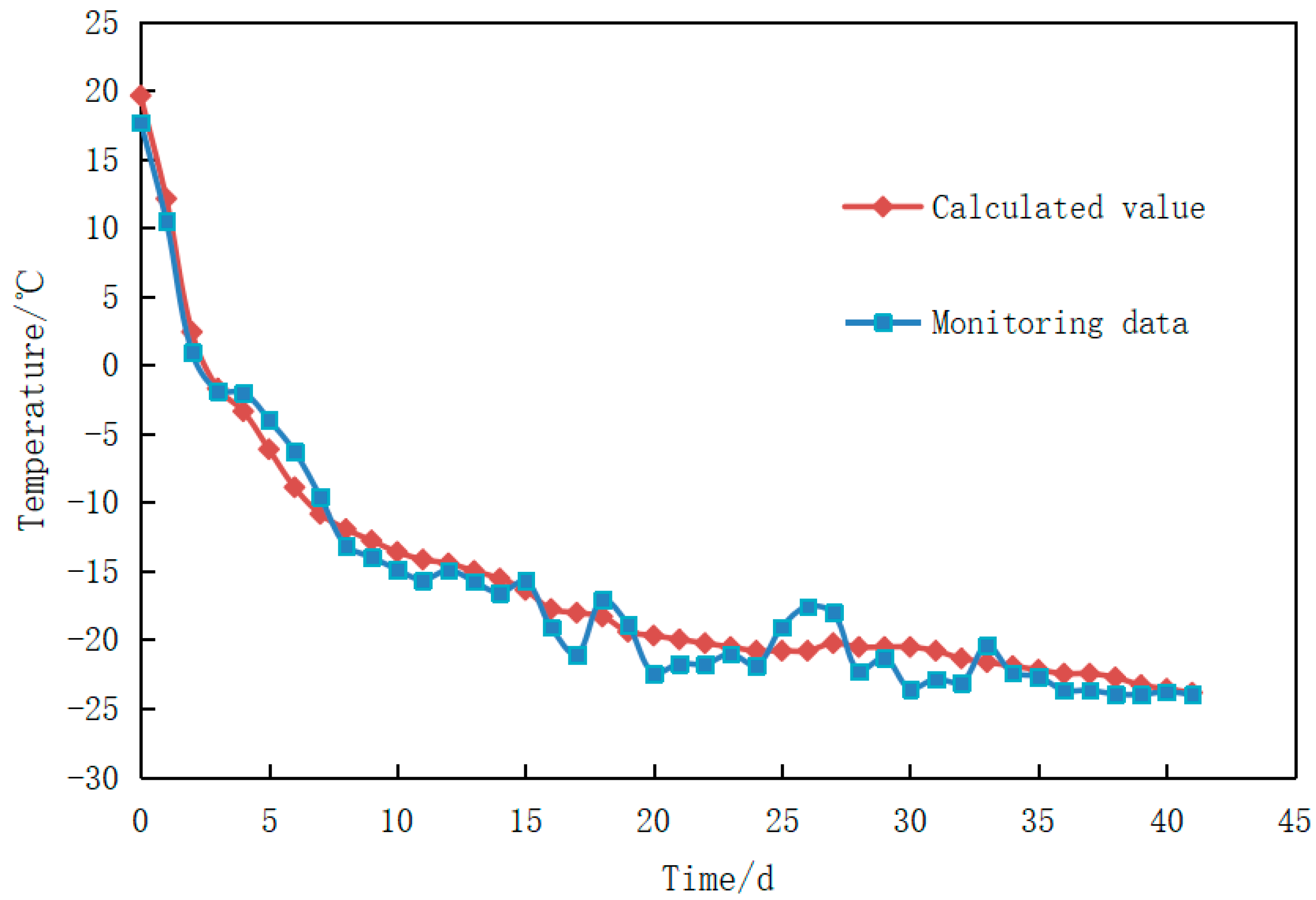
4.5. Comparison of Numerical Simulation and Field Monitoring Data
4.6. Engineering Suggestions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- An, W. Temperature, Moisture, Stress and Interaction of Frozen Soil; Lanzhou University Press: Lanzhou, China, 1990. [Google Scholar]
- Li, S.; Wu, T. Research method and application analysis of frozen soil temperature status. Glacier Frozen Soil 2004, 26, 377–383. [Google Scholar]
- Cheng, H. Status and prospect of artificial ground freezing technology for urban underground engineering. J. Huainan Inst. Technol. 2000, 20, 17–22. [Google Scholar]
- Tritovic, H.A. Permafrost Mechanics; Science Press: Beijing, China, 1985. [Google Scholar]
- Bonacina, C.; Comini, G.; Fasano, A.; Primicerio, M. Numerical solution of phase-change problems. Int. J. Heat Mass Transf. 1973, 16, 1825–1832. [Google Scholar] [CrossRef]
- Comini, G.; Guidice, S.D.I.; Ewis, R.W.; Zienkiewicz, O. Finite element solution of nonlinear heat conduction problems with special referenceto phase change. Int. J. Numer. Methods Eng. 1974, 8, 613–624. [Google Scholar] [CrossRef]
- Ershov, E.D. Phase Composition in the Frozen Rocks; Intelligent Case Study System for Scientometric Data: Moscow, Russia, 1979; pp. 36–37. [Google Scholar]
- Mottagy, D.; Rath, V. Latent heat effects in subsurface heat transport modeling and their impact on palaeo temperature reconstruction. Geophys. J. Int. 2006, 164, 236–245. [Google Scholar] [CrossRef]
- Jia, C.; Xu, W.; Wang, R.; Wang, W.; Zhang, J.; Yu, J. Characterization of the deformation behavior of fine-grained sandstone by triaxial cyclic loading. Constr. Build. Mater. 2018, 162, 113–123. [Google Scholar] [CrossRef]
- Tan, X.; Yu, X.; Chen, W.; Wei-Zhong, C. Temperature field research and engineering application of geotechnical media during freezing and thawing process. J. Rock Mech. Eng. 2012, 31, 2867–2874. [Google Scholar]
- Yang, F.; Ni, L. Numerical simulation of temperature field by horizontal freezing method. Transp. Sci. Technol. 2014, 5, 100–106. [Google Scholar]
- Zhang, T.; Yang, P. Effect of different factors on the short pipe end’ s thickness of frozen wall and horizontal freezing temperature field. J. Wuhan Univ. Technol. 2012, 34, 111–116. [Google Scholar]
- Hou, S.; Sha, A. Temperature-displacement field coupling analysis in freeze-thaw process of soil. J. Chang’an Univ. 2009, 29, 26–29. [Google Scholar]
- Xia, J.; Cai, R.; Yang, P. Analysis of influence parameters of temperature field of cup-shaped frozen soil wall constructed by horizontal freezing method. Railw. Constr. 2010, 12, 38–41. [Google Scholar]
- Jia, C.; Zhang, S.; Xu, W. Experimental Investigation and Numerical Modeling of Coupled Elastoplastic Damage and Permeability of Saturated Hard Rock. Rock Mech. Rock Eng. 2021, 54, 1151–1169. [Google Scholar] [CrossRef]
- Jia, C.J.J.; Xu, W.Y.; Wang, R.B.; Wang, S.S.; Lin, Z.N. Experimental investigation on shear creep properties of undisturbed rock discontinuity in Baihetan Hydropower Station. Int. J. Rock Mech. Min. Sci. 2018, 104, 27–33. [Google Scholar] [CrossRef]
- Li, B.; Zhang, S.Z.; Guo, C.; Zhao, W.; Liu, Y. Analysis of temperature field of Lanzhou Metro connecting passage by artificial freezing method. Spec. Struct. 2021, 38, 79–83. [Google Scholar]
- Lu, X.; Chen, X.; Chen, X. Risk prevention and control of artificial ground freezing (AGF). Chin. J. Geotech. Eng. 2021, 43, 2308–2314. [Google Scholar]
- Jia, C.J.; Zhang, Q.; Lei, M.; Zheng, Y.; Huang, J.; Wang, L. Anisotropic properties of shale and its impact on underground structures: An experimental and numerical simulation. Bull. Eng. Geol. Environ. 2021, 80, 7731–7745. [Google Scholar] [CrossRef]
- Mei, Y.; Zhao, L.; Zhou, D.; Liu, J.; Zhu, J. Application of AGF in Underground Excavation Construction of Water-Rich Sand Layer. China Railw. Sci. 2020, 41, 1–10. [Google Scholar]
- Cai, H.; Peng, L.; Zheng, T. A model for predicting the duration of surface thawing during horizontal freezing construction of tunnels. Rock Soil Mech. 2014, 35, 504–510. [Google Scholar]
- Peng, L. Study on Frost Heave Behavior of Subway Connecting Passage Surrounded by Frozen Coastal Strata; Fujian Institute of Engineering: Fuzhou, China, 2017. [Google Scholar]
- Nixon, J.F.; Maclnnes, K.L. Application of pipe temperature simulator for Norman Walls oil pipeline. Can. Geotech. J. 1996, 30, 140–149. [Google Scholar] [CrossRef]
- Mu, Y.; Li, N.; Li, G. Study of the characteristics of frozen wall formation in the ultra-long horizontal freezing process of a subway construction. J. Glaciol. Geocryol. 2009, 31, 377–382. [Google Scholar]
- Bi, G.; Cheng, X.; Shi, L. Analysis of influencing parameters of temperature field within freezing wall of subway tunnel construction with horizontal ground freezing. J. Lanzhou Univ. Technol. 2009, 35, 121–126. [Google Scholar]
- Xia, J. Study on Temperature Field of Cup Type Frozen Soil Wall Strengthened by Horizontal Freezing of Shield Tunnel; Nanjing Forestry University: Nanjing, China, 2009. [Google Scholar]
- Lin, Y.X.; Yin, Z.; Wang, X.; Huang, L. A systematic 3D simulation method for geomaterials with block inclusions from image recognition to fracturing modelling. Theor. Appl. Fract. Mech. 2021, 117, 103194. [Google Scholar] [CrossRef]
- Lin, Y.X.; Wang, X.; Ma, J.; Huang, L. A systematic framework for the 3D finite-discrete modelling of binary mixtures considering irregular block shapes and cohesive block-matrix interfaces. Powder Technol. 2021, 398, 117070. [Google Scholar] [CrossRef]
- Lin, Y.X.; Wang, X.; Ma, J.; Huang, L. A finite-discrete element based approach for modelling the hydraulic fracturing of rocks with irregular inclusions. Eng. Fract. Mech. 2022, 261, 108209. [Google Scholar] [CrossRef]





| Parameters | Freezing Soil | Unfreezing Soil | ||
|---|---|---|---|---|
| Temperature/°C | −28 | −1 | 0 | 28 |
| Thermal conductivity/(W·m−1·K−1) | 1.17 | 1.17 | 1.07 | 1.07 |
| Specific heat/(kJ·kg·°C−1) | 1.45 | 1.45 | 1.69 | 1.69 |
| Density/(kg·m3) | 1800 | 1800 | 1850 | 1850 |
| Serial Number | Category (Name) | Thickness h (m) | Weight (kN/m3) | Water Content w (%) | Plastic Limit wL | Liquid Limit wP | Permeability Coefficient k (m/d) | Internal Friction Angle | Cohesion | Compression Modulus |
| ① | Miscellaneous fill | 1.30 | 17.8 | 13.6 | 1.78 | 7.08 | 2.3 | 16.5 | 10.5 | 14.3 |
| ② | clay | 1.70 | 18.0 | 31.5 | 3.32 | 26.42 | 0.004 | 21.8 | 8 | 14.4 |
| ③ | Silty clay | 4.13 | 19.2 | 36.7 | 16.96 | 29.16 | 0.08 | 22.2 | 12 | 4.09 |
| ④ | Silt | 4.87 | 19.0 | 34.2 | 4.15 | 9.75 | 0.3 | 24.6 | 23 | 10.5 |
| ⑤ | Silty clay | 15.2 | 18.6 | 29.7 | 4.75 | 22.55 | 0.06 | 23.1 | 34 | 11.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Wei, Y.; Jiang, J.; Jin, H. Effects of Influence Parameters on Freezing Wall Temperature Field in Subway Tunnel. Sustainability 2022, 14, 12245. https://doi.org/10.3390/su141912245
Zhao Y, Wei Y, Jiang J, Jin H. Effects of Influence Parameters on Freezing Wall Temperature Field in Subway Tunnel. Sustainability. 2022; 14(19):12245. https://doi.org/10.3390/su141912245
Chicago/Turabian StyleZhao, Yanxi, Youxin Wei, Jingshan Jiang, and Hua Jin. 2022. "Effects of Influence Parameters on Freezing Wall Temperature Field in Subway Tunnel" Sustainability 14, no. 19: 12245. https://doi.org/10.3390/su141912245
APA StyleZhao, Y., Wei, Y., Jiang, J., & Jin, H. (2022). Effects of Influence Parameters on Freezing Wall Temperature Field in Subway Tunnel. Sustainability, 14(19), 12245. https://doi.org/10.3390/su141912245








