Glare-Free Airport-Based Photovoltaic System via Optimization of Its Azimuth Angle
Abstract
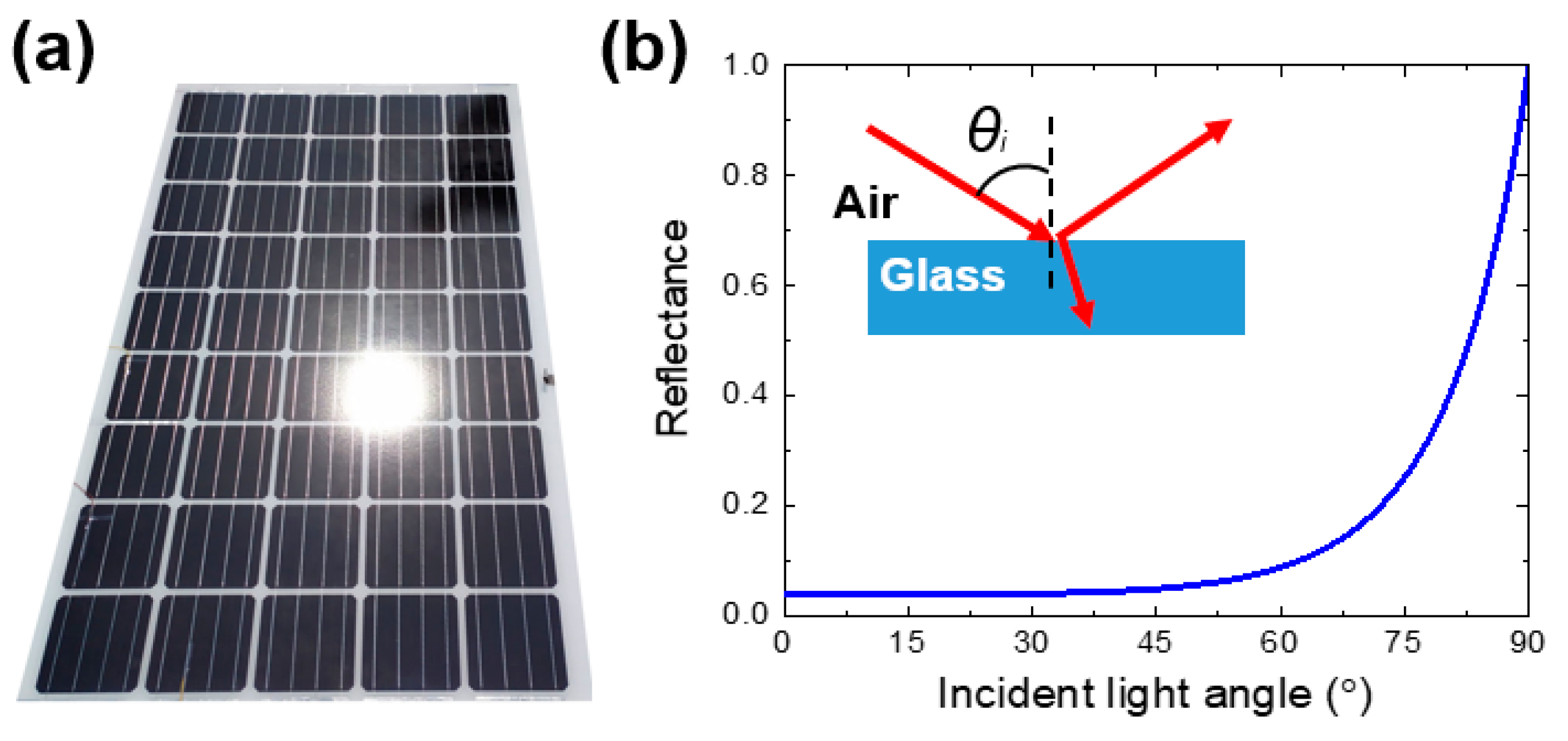
:1. Introduction
2. Simulation Methods
3. Results
3.1. Glare of A-PVs According to Their Installation Angle at a Single Site
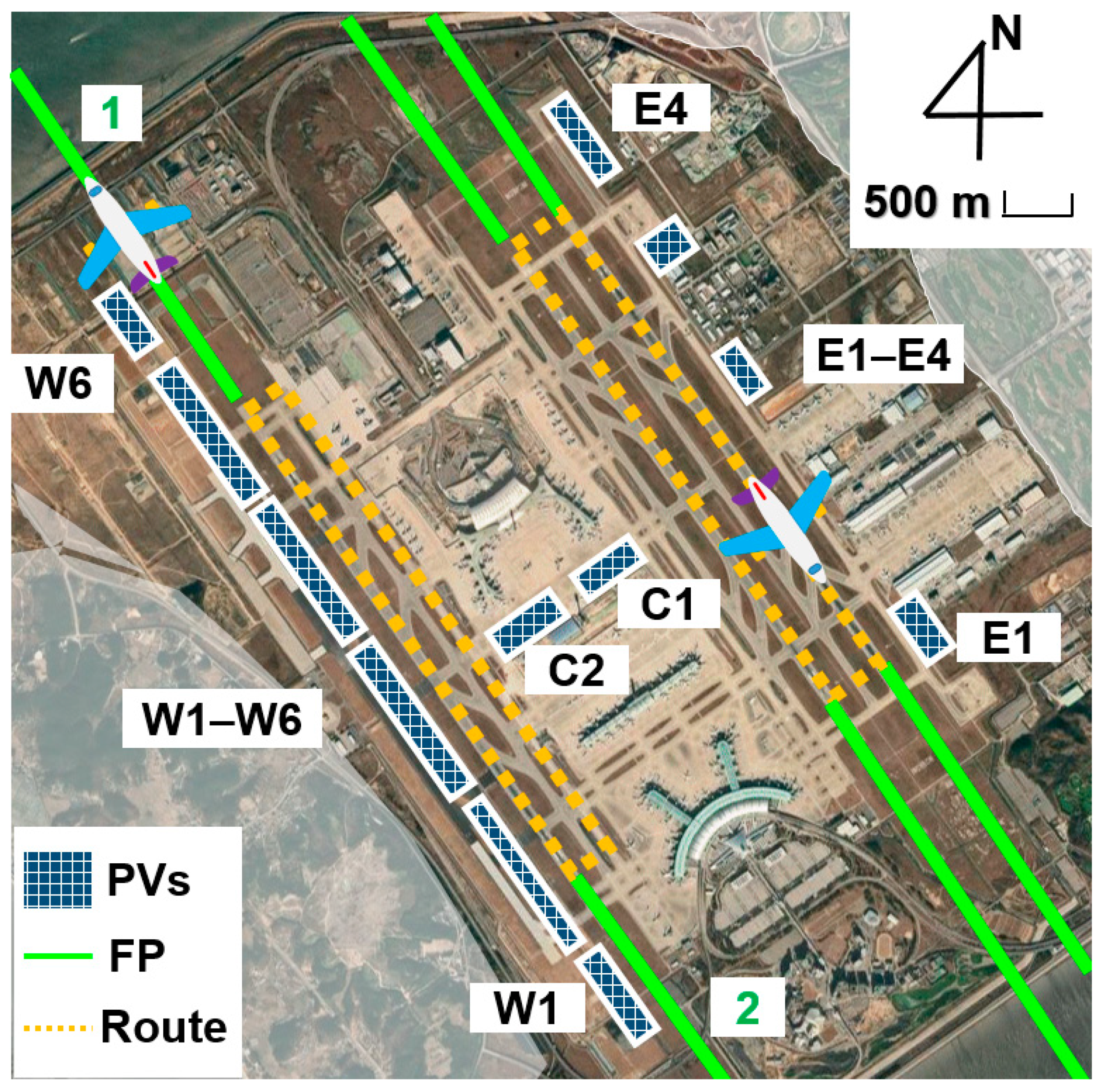
3.2. Glare and AER of A-PVs Located at Various Positions
3.3. Guideline for Designing Glare-Free A-PVs
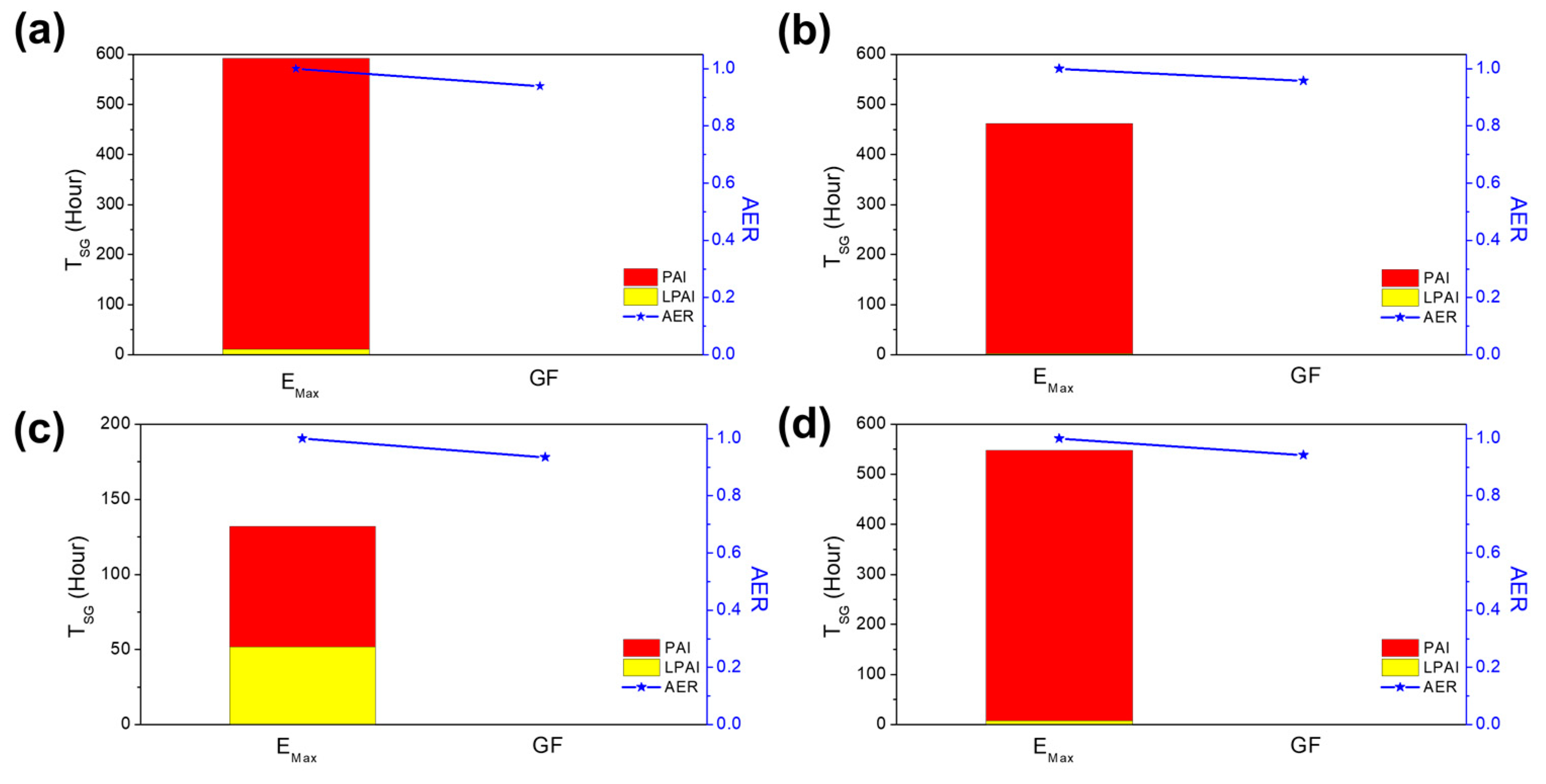
3.4. Glare and AER of A-PVs with Optimized θPV in the ICN
3.5. Verification of Proposed A-PV Azimuth Angle Optimization Method
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xue, J. Photovoltaic agriculture—New opportunity for photovoltaic applications in China. Renew. Sust. Energ. Rev. 2017, 73, 1–9. [Google Scholar] [CrossRef]
- Mitrašinović, A.M. Photovoltaics advancements for transition from renewable to clean energy. Energy 2021, 237, 121510. [Google Scholar] [CrossRef]
- Li, Q.; Zhu, L.; Sun, Y.; Lu, L.; Yang, Y. Performance prediction of Building Integrated Photovoltaics under no-shading, shading and masking conditions using a multi-physics model. Energy 2020, 213, 118795. [Google Scholar] [CrossRef]
- Prasad, R.M.; Krishnamoorthy, A. Design, construction, testing and performance of split power solar source using mirror photovoltaic glass for electric vehicles. Energy 2018, 145, 374–387. [Google Scholar] [CrossRef]
- Ruther, R.; Braun, P. Solar airports. Refocus 2005, 4, 30–34. [Google Scholar] [CrossRef]
- Fukui, H.; Miyoshi, C. The impact of aviation fuel tax on fuel consumption and carbon emissions: The case of the US airline industry. Transp. Res. D Transp. Environ. 2017, 50, 234–253. [Google Scholar] [CrossRef] [Green Version]
- Sreenath, S.; Sudhakar, K.; Yusop, A.F. Airport-based photovoltaic applications. Prog. Photovolt. Res. Appl. 2020, 28, 833–853. [Google Scholar] [CrossRef]
- Wang, J.; Xiao, F.; Zhao, H. Thermoelectric, piezoelectric and photovoltaic harvesting technologies for pavement engineering. Renew. Sust. Energ. Rev. 2021, 151, 111522. [Google Scholar] [CrossRef]
- Ho, C.K.; Ghanbari, C.M.; Diver, R.B. Methodology to assess potential glint and glare hazards from concentrating solar power plants: Analytical models and experimental validation. J. Sol. Energy Eng. 2011, 133, 031021. [Google Scholar] [CrossRef]
- Speroni, A.; Mainini, A.G.; Zani, A.; Paolini, R.; Pagnacco, T.; Poli, T. Experimental Assessment of the Reflection of Solar Radiation from Façades of Tall Buildings to the Pedestrian Level. Sustainability 2022, 14, 5781. [Google Scholar] [CrossRef]
- Yoo, S.; Kang, S. A Study on the Risk of Traffic Accidents using Smart Devices while Walking. J. Korean Soc. Saf. 2017, 32, 74–82. [Google Scholar]
- Sliney, D.H.; Freasier, B.C. Evaluation of optical radiation hazards. Appl. Opt. 1973, 12, 1–24. [Google Scholar] [CrossRef]
- Federal Aviation Administration, US Department of transportation, Interim Policy, FAA Review of Solar Energy System Projects on Federally Obligated Airports. 2013. Available online: https://www.federalregister.gov/documents/2013/10/23/2013-24729/interim-policy-faa-review-of-solar-energy-system-projects-on-federally-obligated-airports (accessed on 20 August 2022).
- Riley, E.; Olson, S. A Study of the Hazardous Glare Potential to Aviators from Utility-Scale Flat-Plate Photovoltaic Systems. Int. Sch. Res. Not. 2011, 2011, 651857. [Google Scholar] [CrossRef] [Green Version]
- Zahid, M.A.; Agrawal, K.S.; Khokhar, M.Q.; Cho, Y.H.; Yi, J. Improved optical performance of hydrophobic silica nanoparticles as antireflection coating on glass and its electrical performance for photovoltaic module applications. Opt. Eng. 2021, 60, 057101. [Google Scholar] [CrossRef]
- Sarkın, A.S.; Ekren, N.; Sağlam, Ş. A review of antireflection and self-cleaning coatings on photovoltaic panels. Sol. Energy 2020, 199, 63–73. [Google Scholar] [CrossRef]
- Dhoke, A.; Mengede, A. UV radiation effect on antireflection coating module degradation in Australia. In Proceedings of the 2016 Australasian Universities Power Engineering Conference (AUPEC), Brisbane, QLD, Australia, 25–28 September 2016; pp. 1–5. [Google Scholar]
- Womack, G.; Isbilir, K.; Lisco, F.; Durand, G.; Taylor, A.; Walls, J.M. The performance and durability of single-layer sol-gel antireflection coatings applied to solar module cover glass. Surf. Coat. Technol. 2019, 358, 76–83. [Google Scholar] [CrossRef] [Green Version]
- Sreenath, S.; Sudhakar, K.; Yusop, A.; Solomin, E.; Kirpichnikova, I. Solar PV energy system in Malaysian airport: Glare analysis, general design and performance assessment. Energy Rep. 2020, 6, 698–712. [Google Scholar] [CrossRef]
- Sreenath, S.; Sudhakar, K.; Yusop, A. Solar PV in the airport environment: A review of glare assessment approaches & metrics. Sol. Energy 2021, 216, 439–451. [Google Scholar]
- Sreenath, S.; Sudhakar, K.; Yusop, A. Glare prediction of solar PV system in airport environment: A scenario analysis of material, tilt and orientation. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1068, 012014. [Google Scholar] [CrossRef]
- Sreenath, S.; Sudhakar, K.; Yusop, A. Technical assessment of captive solar power plant: A case study of Senai airport, Malaysia. Renew. Energy 2020, 152, 849–866. [Google Scholar] [CrossRef]
- Sreenath, S.; Sudhakar, K.; Yusop, A. Effect of PV material, texturing, orientation and tracking on glare impact: A simulation study from an Indian airport. Clean. Eng. Technol. 2021, 4, 100236. [Google Scholar] [CrossRef]
- Sreenath, S.; Sudhakar, K.; Yusop, A. Solar photovoltaics in airport: Risk assessment and mitigation strategies. Environ. Impact Assess. Rev. 2020, 84, 106418. [Google Scholar] [CrossRef]
- Rose, T.; Wollert, A. The dark side of photovoltaic—3D simulation of glare assessing risk and discomfort. Environ. Impact Assess. Rev. 2015, 52, 24–30. [Google Scholar] [CrossRef]
- Sher, F.; Hazafa, A.; Marintseva, K.; Rasheed, T.; Ali, U.; Rashid, T.; Babu, A.; Khzouz, M. Fully solar powered Doncaster Sheffield Airport: Energy evaluation, glare analysis and CO2 mitigation. Sustain. Energy Technol. Assess. 2021, 45, 101122. [Google Scholar] [CrossRef]
- Hwang, T.; Kang, S.; Kim, J.T. Optimization of the building integrated photovoltaic system in office buildings—Focus on the orientation, inclined angle and installed area. Energy Build. 2012, 46, 92–104. [Google Scholar] [CrossRef]
- Al Garni, H.Z.; Awasthi, A.; Wright, D. Optimal orientation angles for maximizing energy yield for solar PV in Saudi Arabia. Renew. Energy 2019, 133, 538–550. [Google Scholar] [CrossRef]
- Hartner, M.; Ortner, A.; Hiesl, A.; Haas, R. East to west—The optimal tilt angle and orientation of photovoltaic panels from and electricity system perspective. Appl. Energy 2015, 160, 94–107. [Google Scholar] [CrossRef]
- National Renewable Energy Laboratory. U.S Analyzing Glare Potential of Solar Photovoltaic Arrays; National Renewable Energy Laboratory: Golden, CO, USA, 2016.
- Sreenath, S.; Sudhakar, K.; Yusop, A.; Cuce, E.; Solomin, E. Analysis of solar PV glare in airport environment: Potential solutions. Results Eng. 2020, 5, 100079. [Google Scholar] [CrossRef]
- Jakubiec, J.A.; Reinhart, C.F. Assessing disability glare potential of reflections from new construction: Case study analysis and recommendations for the future. Transp. Res. Rec. 2014, 2449, 114–122. [Google Scholar] [CrossRef]
- Mitra, S. Sun glare and road safety: An empirical investigation of intersection crashes. Saf. Sci. 2014, 70, 246–254. [Google Scholar] [CrossRef]
- Anurag, A.; Zhang, J.; Gwamuri, J. General design procedures for airport-based solar photovoltaic systems. Energies 2017, 10, 1194. [Google Scholar] [CrossRef] [Green Version]
- Sukumaran, S.; Sudhakar, K. Fully solar powered airport: A case study of Cochin International Airport. J. Air Transp. Manag. 2017, 62, 176–188. [Google Scholar] [CrossRef]
- Barrett, S.; Devita, P.; Ho, C.; Miller, B. Energy technologies compatibility with airports and airspace: Guidance for aviation and energy planners. J. Airpt. Manag. 2014, 8, 318–326. [Google Scholar]
- Rogers, J.A.; Ho , C.K.; Millan, A.; Beben, M.; Drechsler, G. Evaluation of Glare as a Hazard for General Aviation Pilots on Final Approach; Civil Aerospace Medical Institute: Oklahoma City, OK, USA, 2015. [Google Scholar]



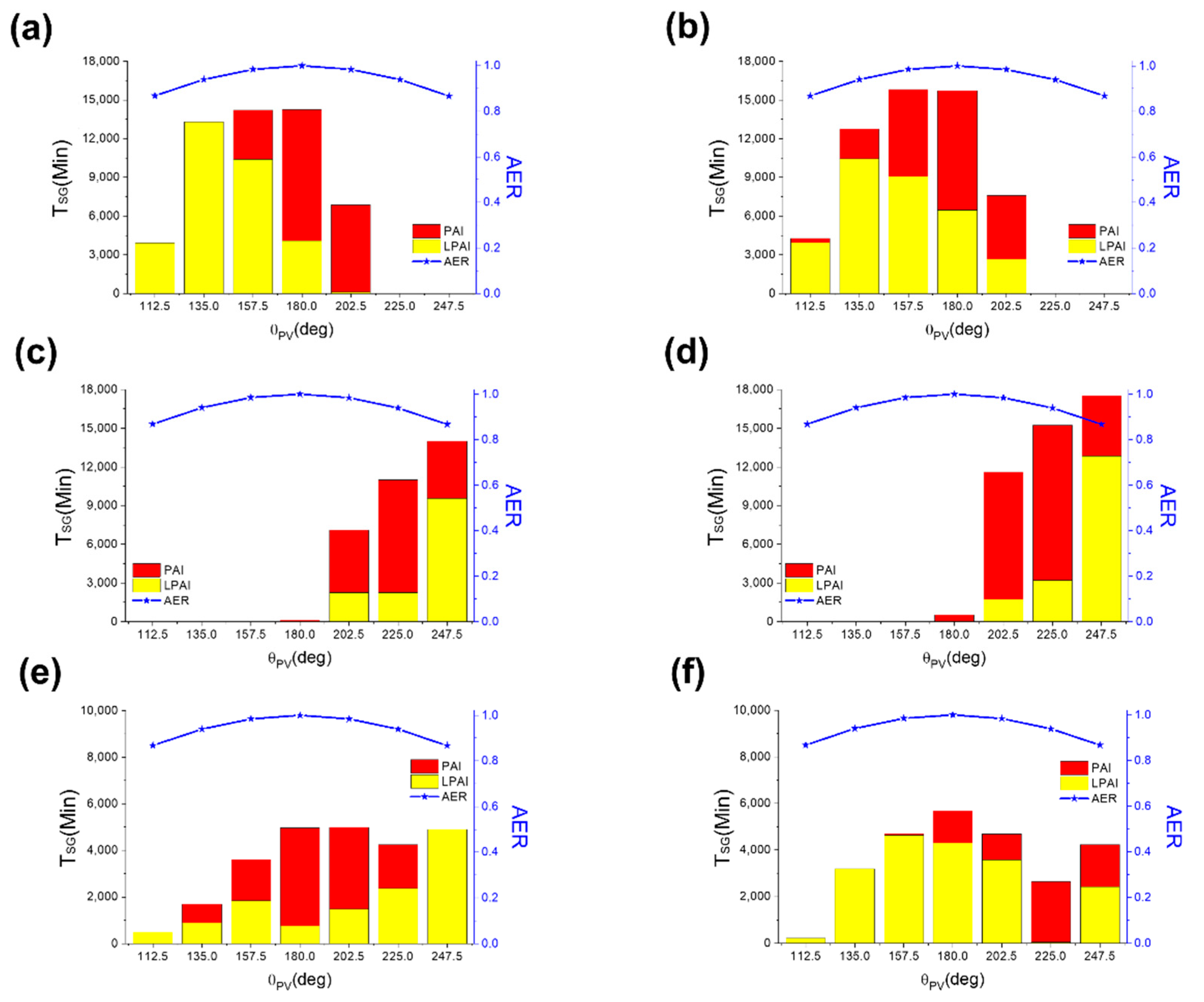





| θPV | 112.5° | 135° | 157.5° | 180° | 202.5° | 225° | 247.5° | |
|---|---|---|---|---|---|---|---|---|
| A-PV | ||||||||
| W1 | TSG | 3899 | 13,310 | 14,223 | 14,232 | 6860 | 0 | 0 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 | |
| W2 | TSG | 4272 | 12,767 | 15,816 | 15,742 | 7591 | 0 | 0 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 | |
| E3 | TSG | 0 | 0 | 0 | 123 | 7098 | 10,980 | 13,990 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 | |
| E4 | TSG | 0 | 0 | 0 | 531 | 11,611 | 15,244 | 17,547 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 | |
| C1 | TSG | 479 | 1686 | 3610 | 4965 | 4993 | 4241 | 4906 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 | |
| C2 | TSG | 227 | 3199 | 4696 | 5682 | 4687 | 2634 | 4236 |
| AER | 0.87 | 0.94 | 0.98 | 1 | 0.98 | 0.94 | 0.87 |
| Sites | W1 | W2 | W3 | W4 | W5 | W6 | C1 | C2 | E1 | E2 | E3 | E4 | Sum | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Model | ||||||||||||||
| Proposed | 2 | 2 | 2 | 2 | 2 | 2 | 0 | 0 | 2 | 3 | 3 | 3 | 23 | |
| Start from south facing A-PV | 3 | 3 | 3 | 3 | 3 | 3 | 7 | 7 | 3 | 2 | 2 | 2 | 41 | |
| Site | GMP | KWJ | PEK | NRT | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| LPAI | PAI | AER | LPAI | PAI | AER | LPAI | PAI | AER | LPAI | PAI | AER | |
| EMax | 651 | 34,837 | 1 | 158 | 27,542 | 1 | 3106 | 4803 | 1 | 489 | 32,398 | 1 |
| GF. | 0 | 0 | 0.94 | 0 | 0 | 0.96 | 0 | 0 | 0.94 | 0 | 0 | 0.94 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, C.; Song, H.-J. Glare-Free Airport-Based Photovoltaic System via Optimization of Its Azimuth Angle. Sustainability 2022, 14, 12781. https://doi.org/10.3390/su141912781
Kim C, Song H-J. Glare-Free Airport-Based Photovoltaic System via Optimization of Its Azimuth Angle. Sustainability. 2022; 14(19):12781. https://doi.org/10.3390/su141912781
Chicago/Turabian StyleKim, Chungil, and Hyung-Jun Song. 2022. "Glare-Free Airport-Based Photovoltaic System via Optimization of Its Azimuth Angle" Sustainability 14, no. 19: 12781. https://doi.org/10.3390/su141912781
APA StyleKim, C., & Song, H.-J. (2022). Glare-Free Airport-Based Photovoltaic System via Optimization of Its Azimuth Angle. Sustainability, 14(19), 12781. https://doi.org/10.3390/su141912781






