Brake Instability Dynamic Model and Active Control Strategy for a Multiunit Articulated Rubber-Wheel Autonomous Rail Rapid Transit System
Abstract
:1. Introduction
2. Dynamic Analysis Model of the Emergency Braking of Multiunit Vehicle
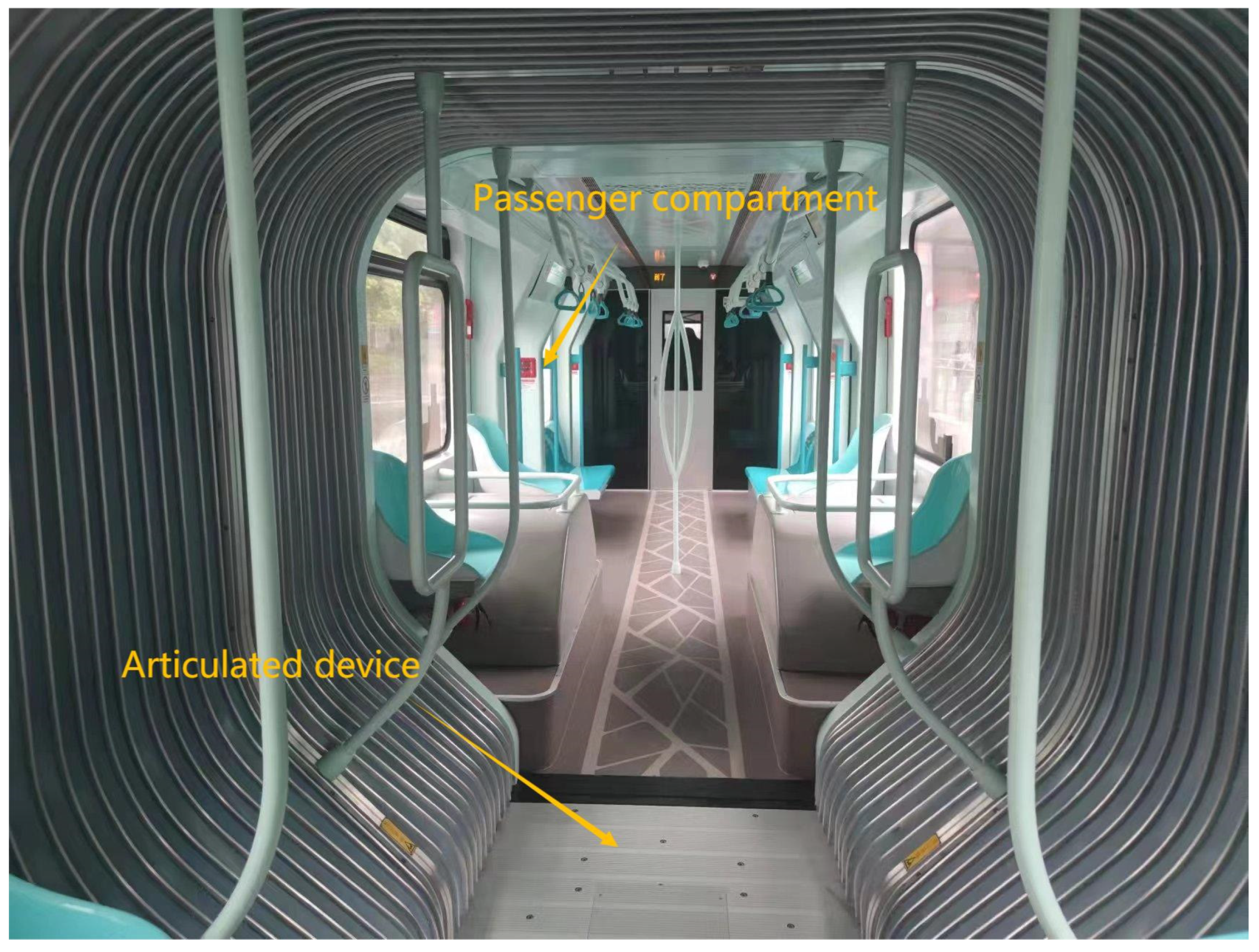
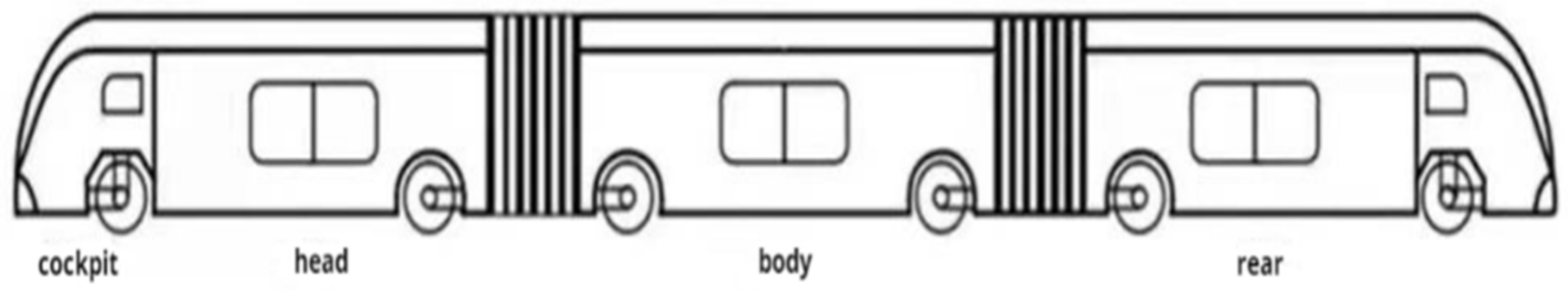
2.1. Parametric Model Construction of Multiunit Vehicle
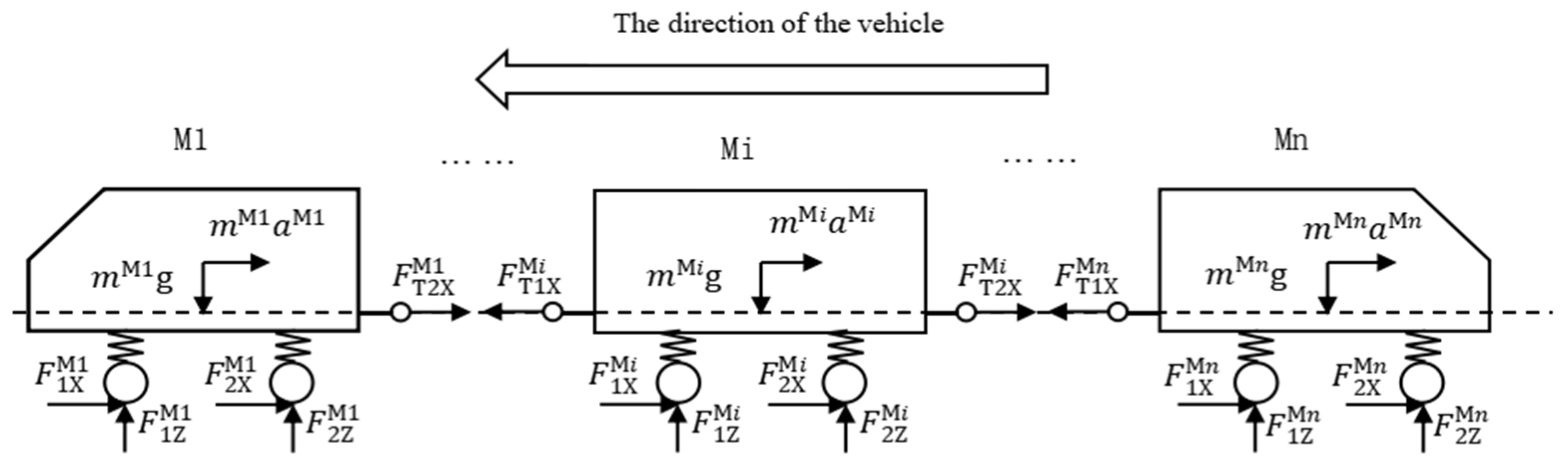
2.2. Model of Braking Dynamics of the Multiunit Vehicle
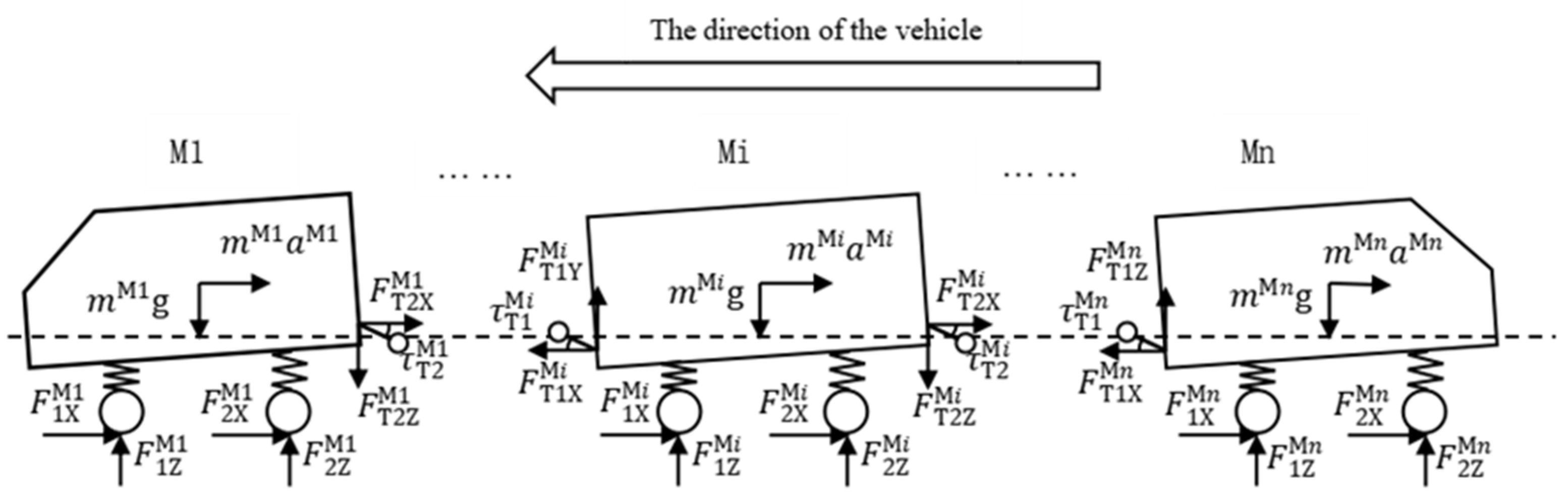
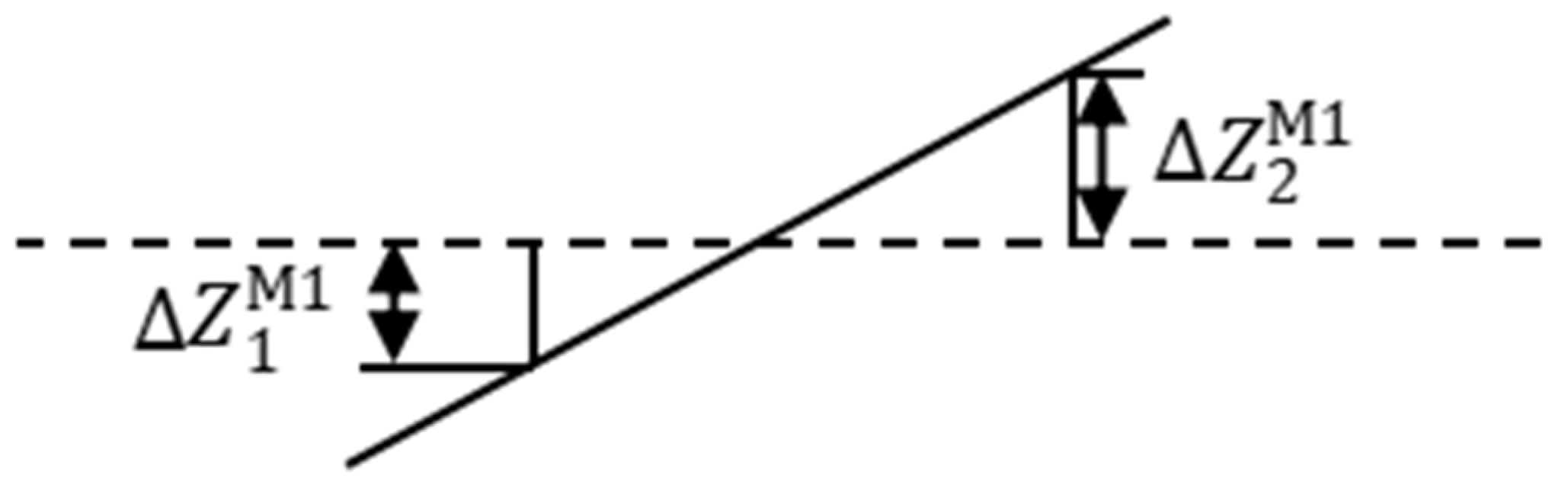
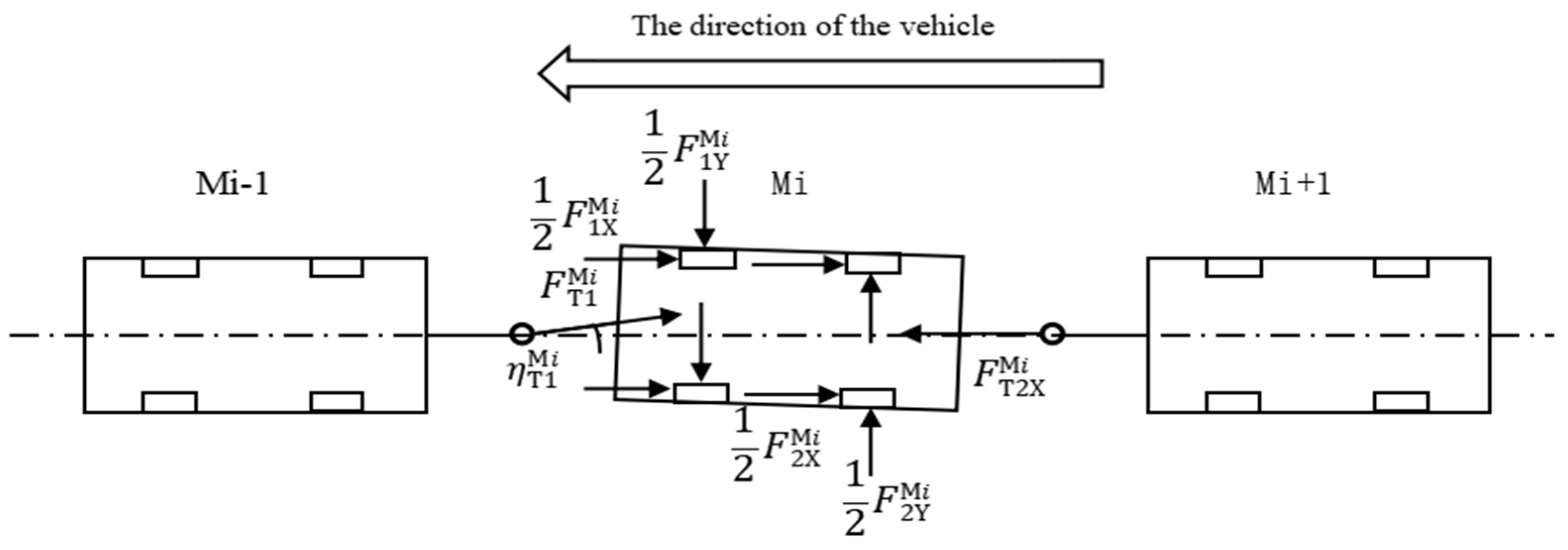
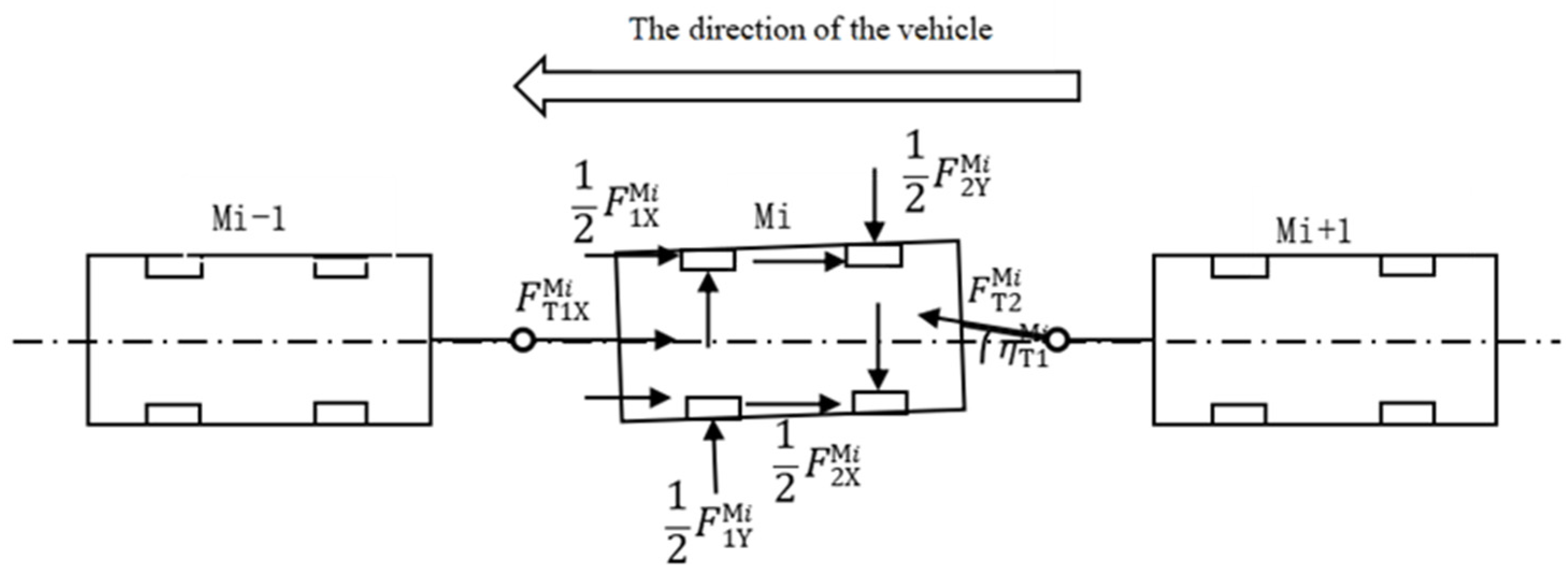
2.3. Articulation Force Analysis Model for the Multiunit Vehicle
3. Analysis of Linear Emergency Brake Instability of the Multiunit Vehicle
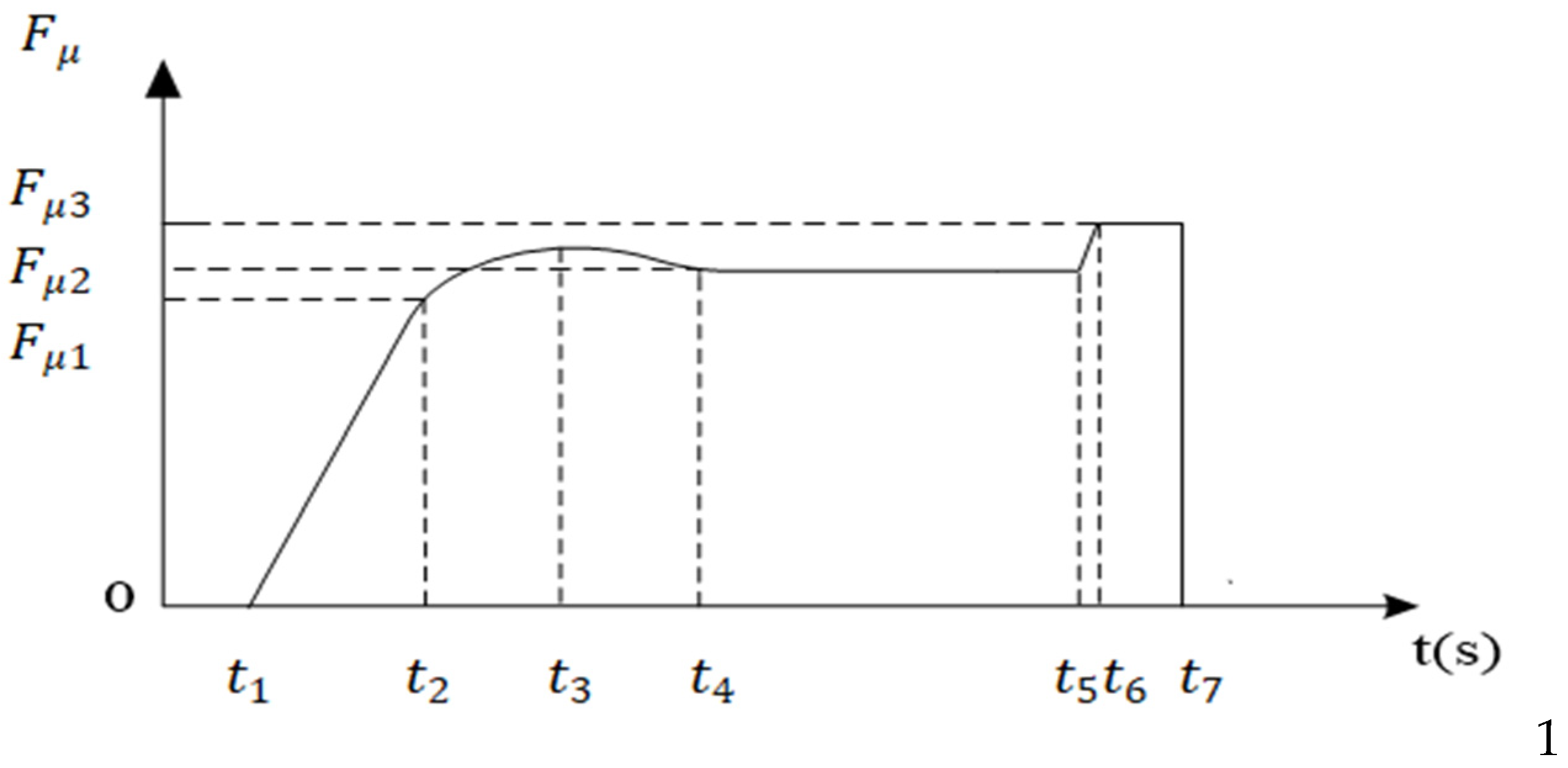
3.1. Analysis of the Emergency Braking Instability Characteristics of the Multiunit Vehicle
3.2. Modeling of Emergency Braking Instability Conditions of the Multiunit Vehicle
3.3. Critical Conditions for the Emergency Braking Instability of the Multiunit Vehicle
4. Active Control Strategies for the Linear Emergency Braking of the Multiunit Vehicle
4.1. Solution of the Ground Braking Force of the Multiunit Vehicle
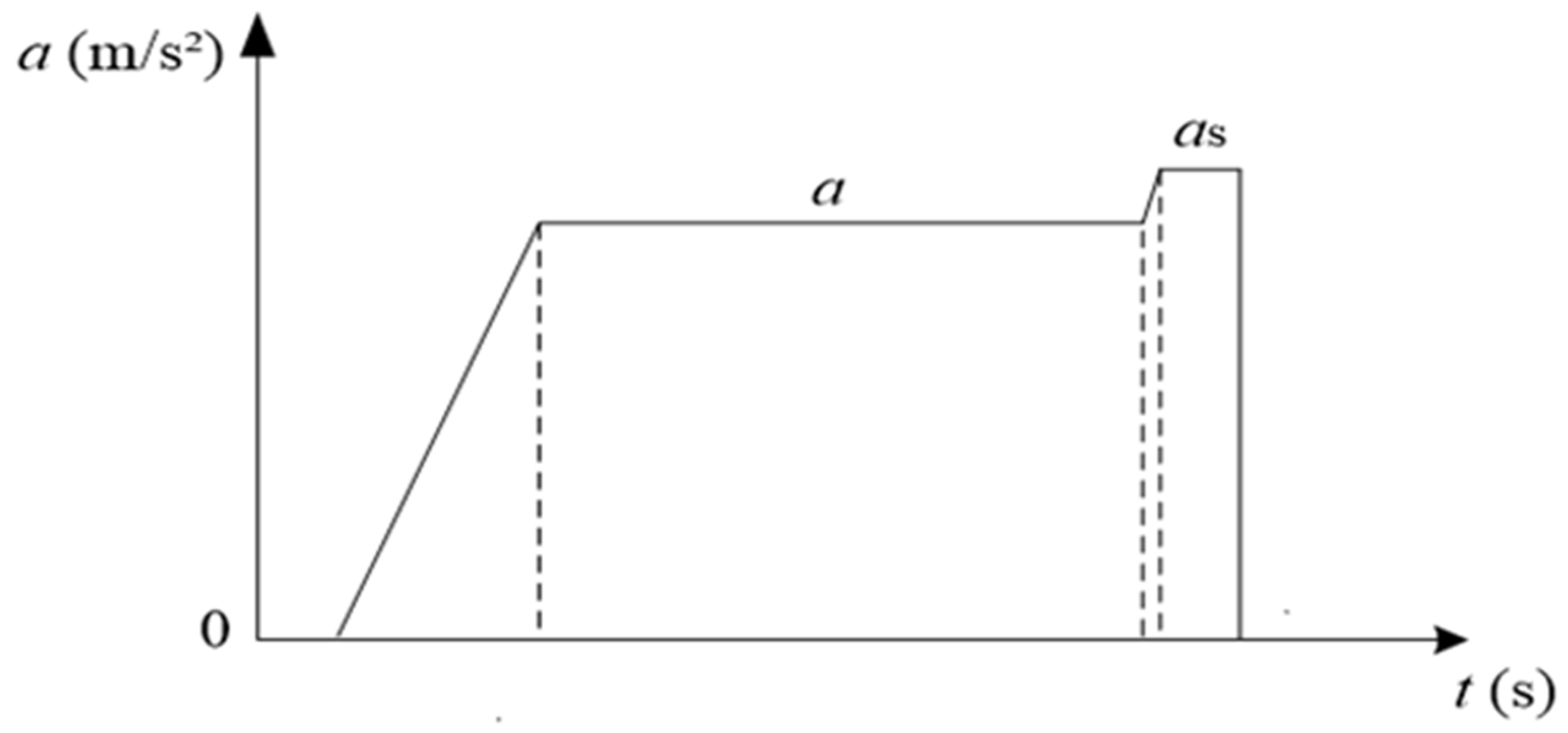
4.2. Construction of a Multiunit Vehicle Braking Distance Model
4.3. Emergency Braking Control Strategy for the Multiunit Vehicle
5. Simulation of the Emergency Braking Control Process of Three-Unit Vehicle
5.1. Simulation Model Establishment
- (1)
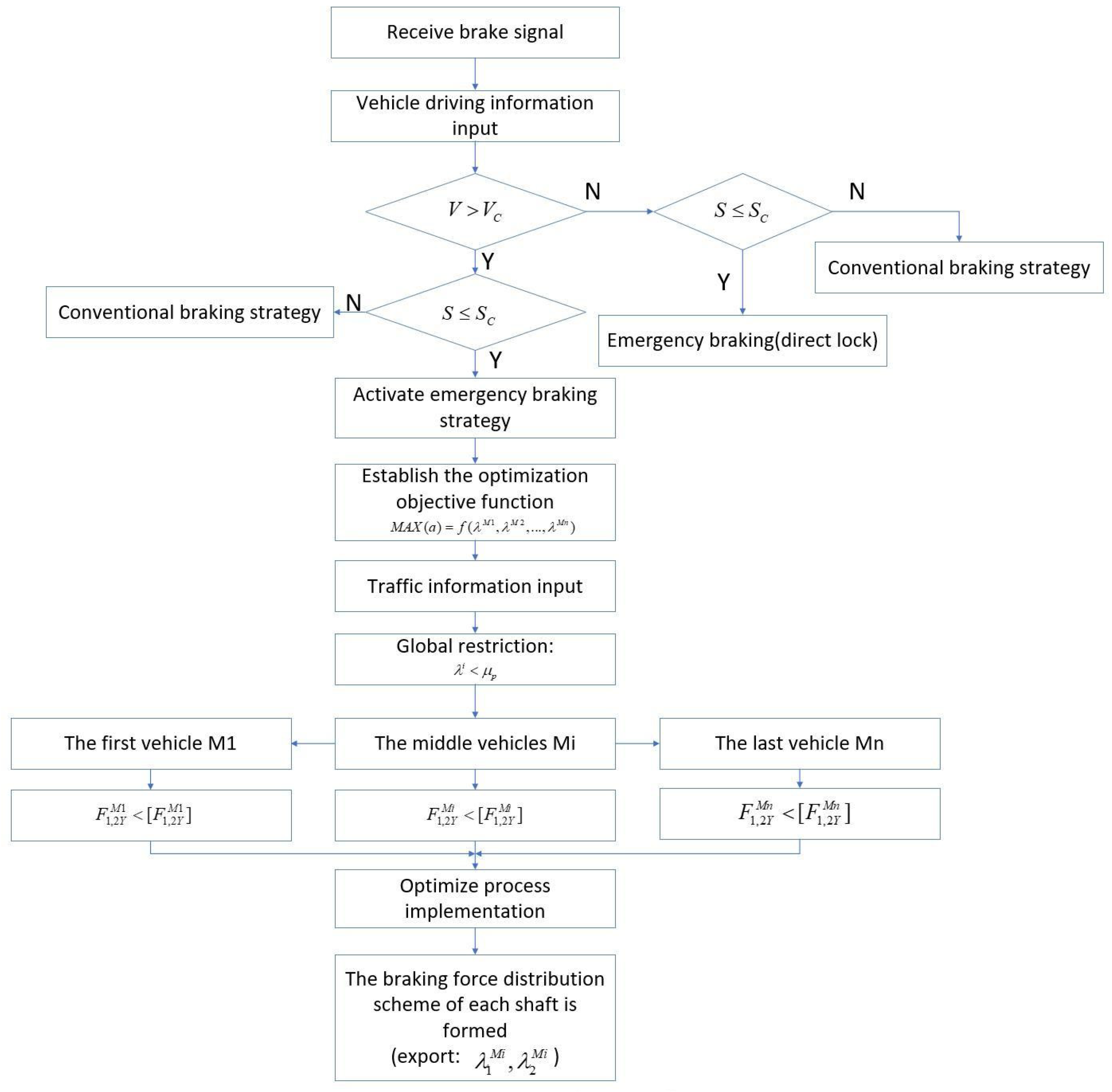
- Emergency vehicle condition determination module. This module includes a longitudinal safety distance determination module and a braking speed determination module. When a vehicle is located directly in front of the self-driving vehicle, the latter mainly determines whether there is a risk of collision by using the longitudinal relative distance and longitudinal relative speed of the two combined with the driving speed of the self-driving vehicle. When the vehicle receives a braking signal, the emergency vehicle condition determination module receives real-time information on the vehicle’s operating conditions and the surrounding obstacle vehicles obtained by the on-board sensor. It then obtains a certain amount of information that can indicate the degree of danger according to the calculation strategy designed in advance. The degree of danger is determined after comparing the obtained information with the set threshold, which then determines whether the emergency braking program should be activated. The entire module eventually outputs zero or one depending on the value of the input signal. When the module outputs one, the emergency braking system can be triggered. Otherwise, it makes the vehicle brake according to the conventional braking strategy.
- (2)
- Vehicle dynamics module. This module analyzes the ground braking force, ground lateral reaction force, ground normal reaction force, and the X-, Y-, and Z-direction forces at the articulation of the multiunit vehicles during emergency braking. This is done by introducing the suspension deformation compatibility equation, the articulation force relationship equation, the tire force balance equation, and so on. Thus, the vehicle braking force, braking deceleration, and braking distance corresponding to the different braking force distribution coefficients of the vehicle are obtained. This paper examined the braking process of the multiunit vehicles in an ideal state so that the sum of the braking force distribution coefficients of each vehicle’s front and rear axles is one.
- (3)
- Stability discriminant module. By analyzing the emergency braking instability characteristics of the multiunit vehicles, this module mainly examines the tire lateral forces of the front and rear axles in the case of left and right declination angles at the front and rear articulations, as well as the stability of the vehicle. With the maximum tire lateral force, the vehicle remains stable when the tire lateral force is less than the maximum tire lateral force; otherwise, the vehicle is unstable. The ultimate goal of this paper is to obtain an emergency brake control model that enables the multiunit vehicles to achieve braking at the shortest braking distance while maintaining stability and to output the optimal braking force distribution coefficient at this time. Therefore, stability discrimination for the multiunit vehicles is particularly important.
- (4)
- Gradient descent control module. This paper mainly studies the emergency braking control process of three-unit vehicles. Thus, it features the multiple parameters of the braking force distribution coefficient of three-unit vehicles. Considering the optimization problem of multiple parameters is necessary to achieve optimal braking for the multiunit vehicles, that is, to achieve the braking force distribution coefficient with the shortest braking distance while ensuring stability. Gradient descent is the simplest and most effective algorithm to solve convex optimization problems and has important applications in engineering practice, such as parameter identification, machine learning, and optimization control. By using the gradient descent strategy, the module obtains a braking force distribution coefficient that can achieve optimal braking and is sent to the brake controller so that each shaft distributes the braking force according to the partition coefficient, thereby completing the braking.
5.2. Simulation Parameter Settings
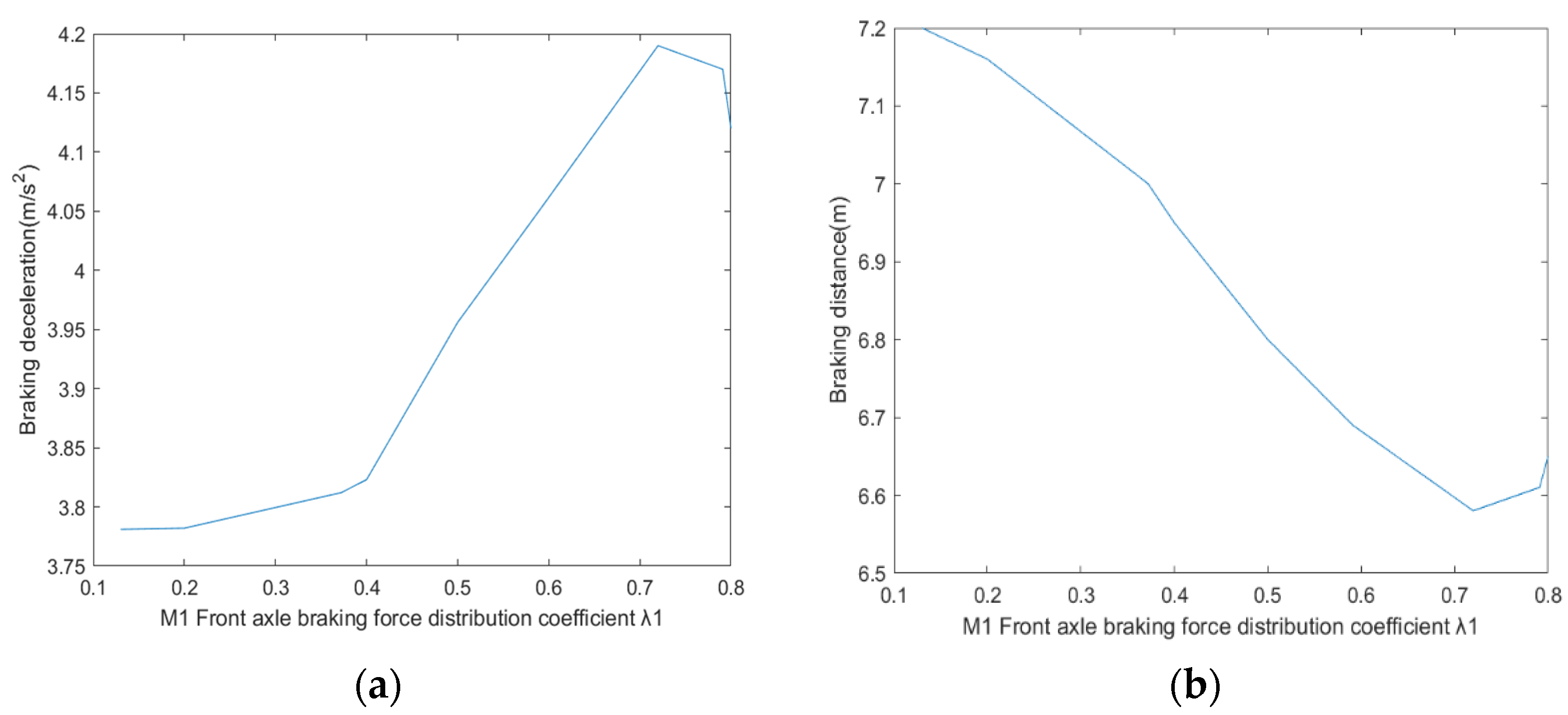
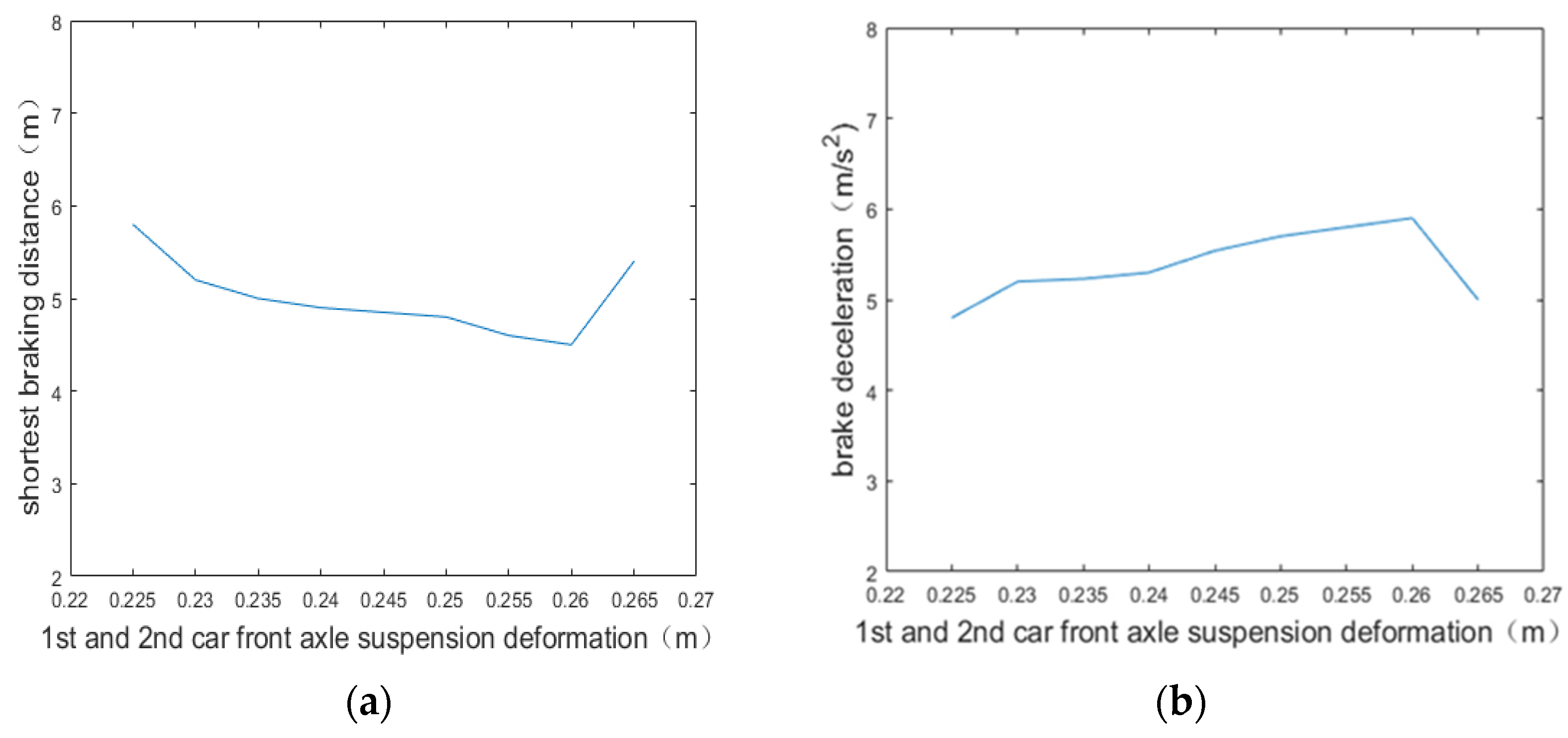
5.3. Simulation of the Emergency Braking Control Process of Three-Unit Vehicle
6. Experiments
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Carriage Mi mass (kg); | |
| Acceleration due to gravity (m/); | |
| Carriage Mi braking deceleration (m/); | |
| Average braking deceleration (m/) of the carriages from M1 to Mn; | |
| Average braking deceleration (m/) of the carriages from M1 to Mm; | |
| Average braking deceleration (m/) of the carriages from Mm+1 to Mn; | |
| Ground braking force (N) on the front and rear axles of the carriage Mi; | |
| Ground lateral reaction force (N) on the front and rear axles of the carriage Mi; | |
| Ground normal reaction force (N) on the front and rear axles of the carriage Mi; | |
| X-directional force (N) at the front and rear articulations of the carriage Mi; | |
| Y-directional force (N) at the front and rear articulations of the carriage Mi; | |
| Z-directional force (N) at the front and rear articulations of the carriage Mi; | |
| Total ground braking force (N) of the carriages from M1 to Mn; | |
| Total braking force (N) of the carriages from M1 to Mm and the carriages from Mm+1 to Mn; | |
| , | Front and rear hinges of the carriage Mi with a horizontal angle (°); |
| Front and rear hinges of the carriage Mi with left and right angles to a horizontal plane (°); | |
| Braking force distribution coefficient for the front and rear axles of the carriage Mi; | |
| Rolling attachment coefficient; | |
| Maximum ground adhesion coefficient; | |
| Equivalent stiffness of the axle suspension (N/m); | |
| Horizontal distance between the two axles of the carriage Mi (m); | |
| Horizontal distance from the center of mass to the front and rear axle centerlines of the carriage Mi (m); | |
| Length of hinges between vehicle carriages (m); | |
| Articulation to the nearest adjacent axis (m); | |
| Distance from the center of mass of the carriage Mi to the ground (m); | |
| Distance from the front articulation to the ground and from the rear articulation to the ground (m); | |
| Amount of deformation of the front and rear axle suspensions of the carriage Mi (m). |
References
- Lei, T.; Wang, J.; Yao, Z. Modelling and stability analysis of articulated vehicles. Appl. Sci. 2021, 11, 3663. [Google Scholar] [CrossRef]
- Wei, L.; Zeng, J.; Qu, S.; Huang, C.; Wang, Q. Longitudinal-vertical dynamics of a high-speed train rescued by locomotives during braking on grades. Veh. Syst. Dyn. 2022, 1–24. [Google Scholar] [CrossRef]
- Garcia-Bedoya, O.; Hirota, S.; Ferreira, J.V. Control system design for an automatic emergency braking system in a sedan vehicle. In Proceedings of the 2nd Latin American Conference on Intelligent Transportation Systems (ITS LATAM), Bogota, Colombia, 19–20 March 2019; pp. 1–6. [Google Scholar]
- Liu, Y.; Zhou, Y.; Su, S.; Xun, J.; Tang, T. Control strategy for stable formation of high-speed virtually coupled trains with disturbances and delays. Comput.-Aided Civ. Infrastruct. Eng. 2022. [Google Scholar] [CrossRef]
- Wu, Q.; Cole, C.; Spiryagin, M.; Chang, C.; Wei, W.; Ursulyak, L.; Shvets, A.; Murtaza, M.A.; Mirza, I.M.; Zhelieznov, K.; et al. Freight train air brake models. Int. J. Rail Transp. 2021, 1–49. [Google Scholar] [CrossRef]
- Zong, C.; He, J.; Yang, J.; Li, X. Research on correlation between static and dynamic braking performance characteristics of articulated vehicle. In Proceedings of the 2021 6th International Conference on Transportation Information and Safety (ICTIS), Wuhan, China, 22–24 October 2021; pp. 1532–1539. [Google Scholar]
- Ataei, M.; Khajepour, A.; Jeon, S. Model predictive control for integrated lateral stability, traction/braking control, and rollover prevention of electric vehicles. Veh. Syst. Dyn. 2020, 58, 49–73. [Google Scholar] [CrossRef]
- Termous, H.; Shraïm, H.; Talj, R.; Francis, C.; Charara, A. Coordinated control strategies for active steering, differential braking and active suspension for vehicle stability, handling and safety improvement. Veh. Syst. Dyn. 2019, 57, 1494–1529. [Google Scholar] [CrossRef]
- Skotnikov, G.I.; Jileykin, M.M.; Komissarov, A.I. Increasing the stability of the articulated lorry at braking by locking the fifth wheel coupling. In IOP Conference Series: Materials Science and Engineering, Proceedings of the International Automobile Scientific Forum (IASF-2017) Intelligent Transport Systems, Moscow, Russia, 18–19 October 2017; IOP Publishing: Bristol, England, 2018; Volume 315, p. 012027. [Google Scholar]
- Gao, Z.; Li, D.; Xie, L. Study on the differential braking of an eddy current retarder axle used for articulated vehicles. In Proceedings of the IEEE 5th International Conference on Intelligent Transportation Engineering (ICITE), Beijing, China, 11–13 September 2020; pp. 596–600. [Google Scholar]
- Dudziński, P.; Skurjat, A. System for improving directional stability for articulated vehicles. In AIP Conference Proceedings, Proceedings of the 15th Conference on Computational Technologies in Engineering, Jora Wielka, Poland, 16–19 October 2018; AIP Publishing LLC: New York, NY, USA, 2019; Volume 2078, p. 020084. [Google Scholar]
- Zhang, Y.; Khajepour, A.; Ataei, M. A universal and reconfigurable stability control methodology for articulated vehicles with any configurations. IEEE Trans. Veh. Technol. 2020, 69, 3748–3759. [Google Scholar] [CrossRef]
- Sharma, S.K.; Kumar, A. Impact of longitudinal vehicle dynamics on vehicle operations: A simulation-based study. J. Vib. Eng. Technol. 2018, 6, 197–203. [Google Scholar] [CrossRef]
- Saeedi, M.A. A new robust combined control system for improving manoeuvrability, lateral stability and rollover prevention of a vehicle. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2020, 234, 198–213. [Google Scholar] [CrossRef]
- Su, S.; Liu, W.; Zhu, Q.; Li, R.; Tang, T.; Lv, J. A cooperative collision-avoidance control methodology for virtual coupling trains. Accid. Anal. Prev. 2022, 173, 106703. [Google Scholar]





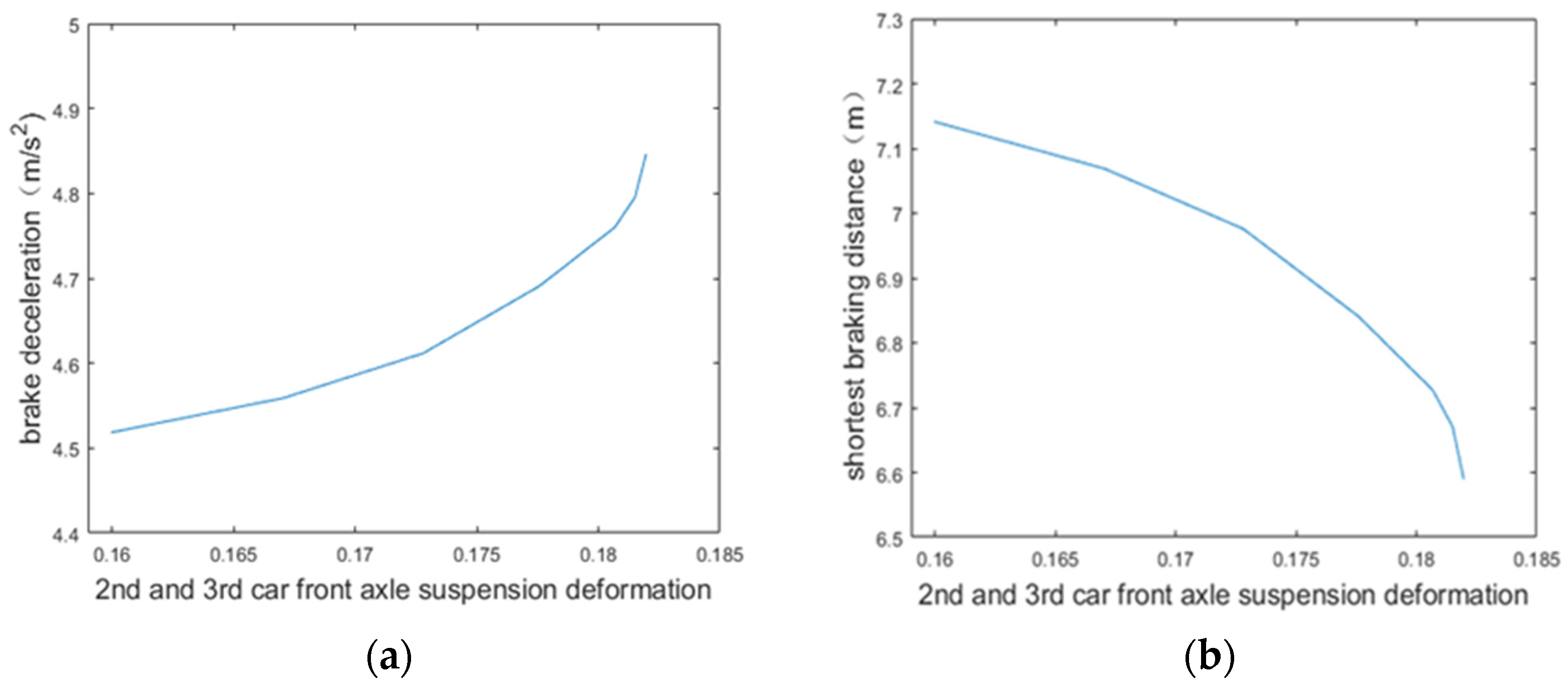

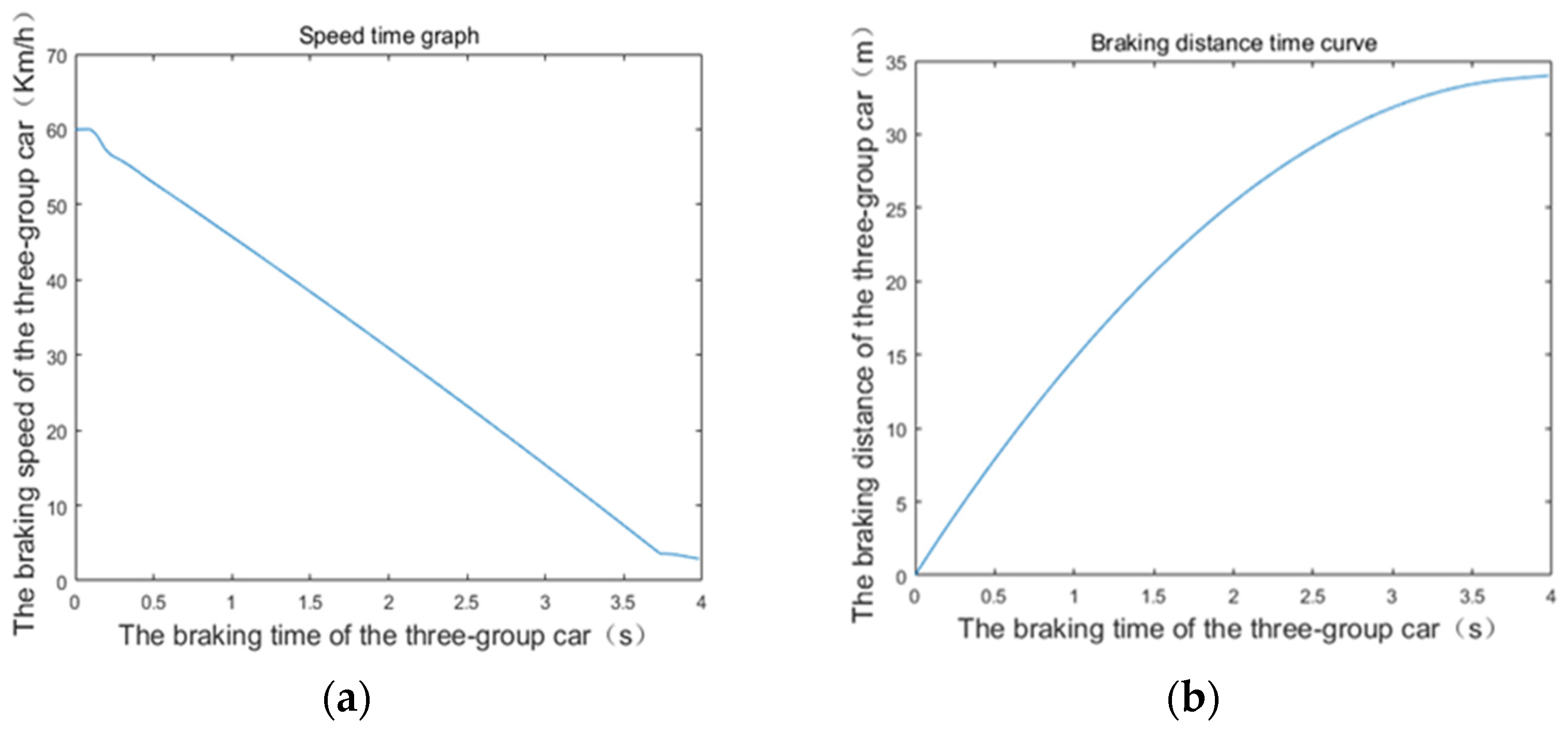
| Relevant Simulation Parameters | Numeric Value |
|---|---|
| Mass of each carriage (kg) | 15,000 |
| Equivalent stiffness of the axle suspension (N/m) | 124,000 |
| Maximum ground adhesion coefficient | 0.75 |
| Rolling attachment coefficients | 0.5 |
| Horizontal distance between the two axles of each vehicle (m) | 6 |
| Horizontal distance from the center of mass of carriage M1 to the front axle (m) | 2.7 |
| Horizontal distance from the center of mass of carriage M1 to the rear axle (m) | 3.3 |
| Horizontal distance from the center of mass of carriage M2 to the front axle (m) | 3 |
| Horizontal distance from the center of mass of carriage M2 to the rear axle (m) | 3 |
| Horizontal distance from the center of mass of carriage M3 to the front axle (m) | 3.3 |
| Horizontal distance from the center of mass of carriage M3 to the rear axle (m) | 2.7 |
| Changes in the Centroid Position (m) | Braking Distance (m) | Braking Deceleration (m/s2) |
|---|---|---|
| Move forward 0.05 | 4.77 | 5.62 |
| Move forward 0.08 | 4.91 | 5.51 |
| Unchanged | 4.58 | 5.78 |
| Move back 0.08 | 4.37 | 5.97 |
| Move back 0.10 | 4.31 | 6.03 |
| Move back 0.15 | 4.34 | 6.01 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, T.; Zhang, S.; Xiao, G.; Wang, M.; Zhong, H.; Feng, J. Brake Instability Dynamic Model and Active Control Strategy for a Multiunit Articulated Rubber-Wheel Autonomous Rail Rapid Transit System. Sustainability 2022, 14, 14531. https://doi.org/10.3390/su142114531
Li T, Zhang S, Xiao G, Wang M, Zhong H, Feng J. Brake Instability Dynamic Model and Active Control Strategy for a Multiunit Articulated Rubber-Wheel Autonomous Rail Rapid Transit System. Sustainability. 2022; 14(21):14531. https://doi.org/10.3390/su142114531
Chicago/Turabian StyleLi, Tao, Shuo Zhang, Gang Xiao, Minqi Wang, Hanwen Zhong, and Jianghua Feng. 2022. "Brake Instability Dynamic Model and Active Control Strategy for a Multiunit Articulated Rubber-Wheel Autonomous Rail Rapid Transit System" Sustainability 14, no. 21: 14531. https://doi.org/10.3390/su142114531
APA StyleLi, T., Zhang, S., Xiao, G., Wang, M., Zhong, H., & Feng, J. (2022). Brake Instability Dynamic Model and Active Control Strategy for a Multiunit Articulated Rubber-Wheel Autonomous Rail Rapid Transit System. Sustainability, 14(21), 14531. https://doi.org/10.3390/su142114531







